Microstructural Evolution and Failure in Fibrous Network Materials: Failure Mode Transition from the Competition between Bond and Fiber
Abstract
1. Introduction
2. Material and Method
2.1. Experimental Section
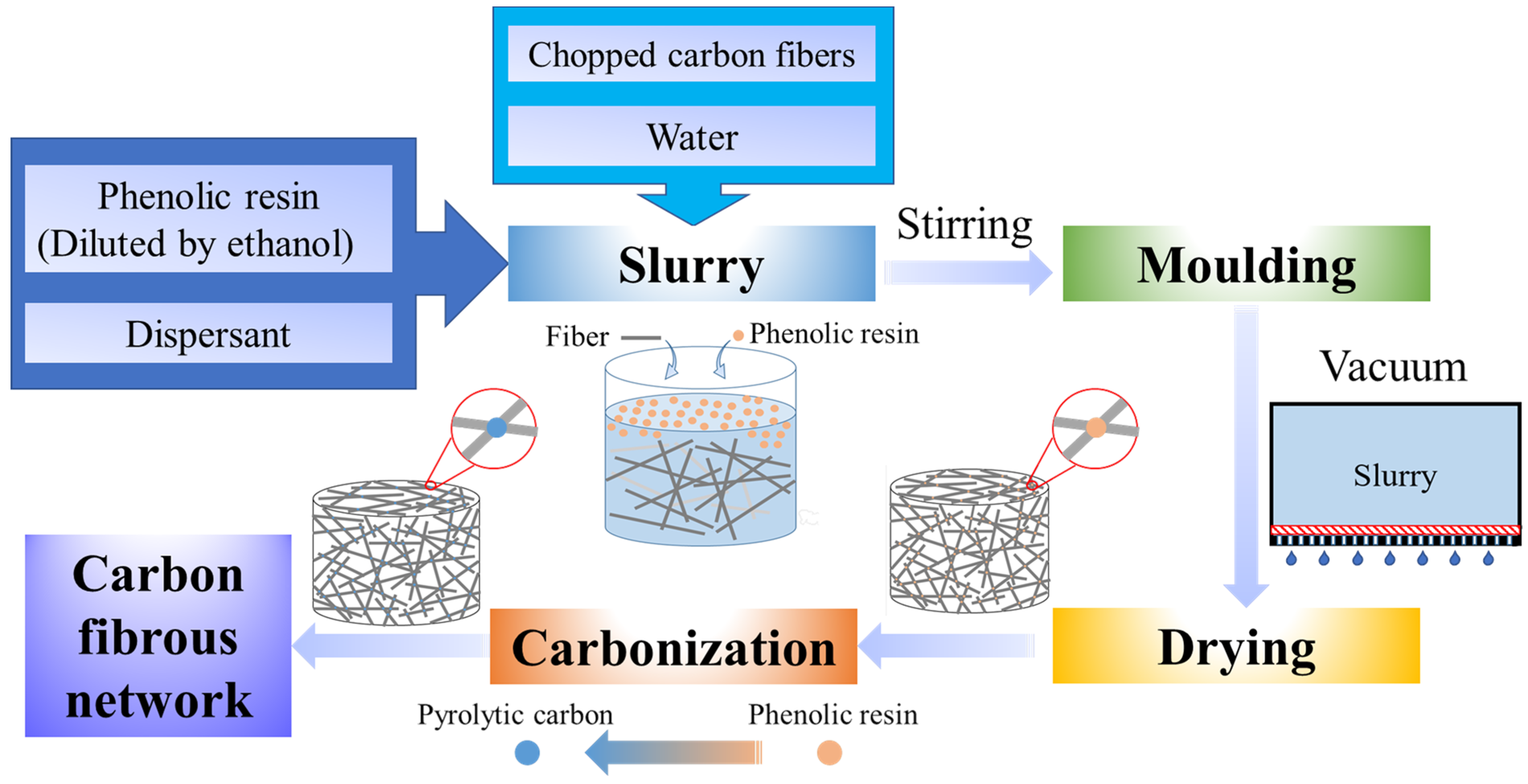
2.1.1. Materials
2.1.2. Quasi-Static Tensile Tests
2.2. Numerical Simulations
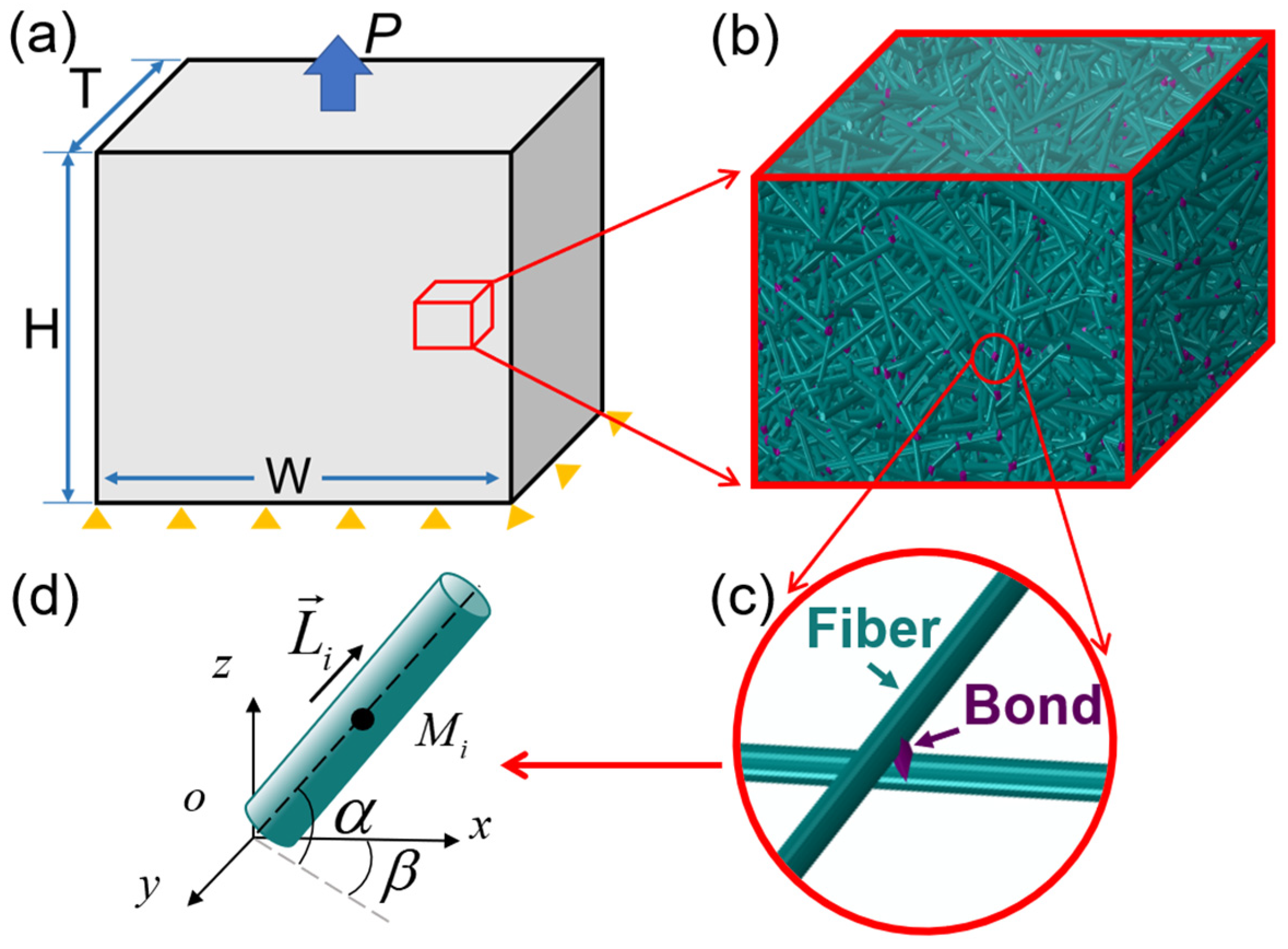
2.2.1. Fibrous Network Model
2.2.2. Finite Element Analysis
3. Results
3.1. Experimental Results
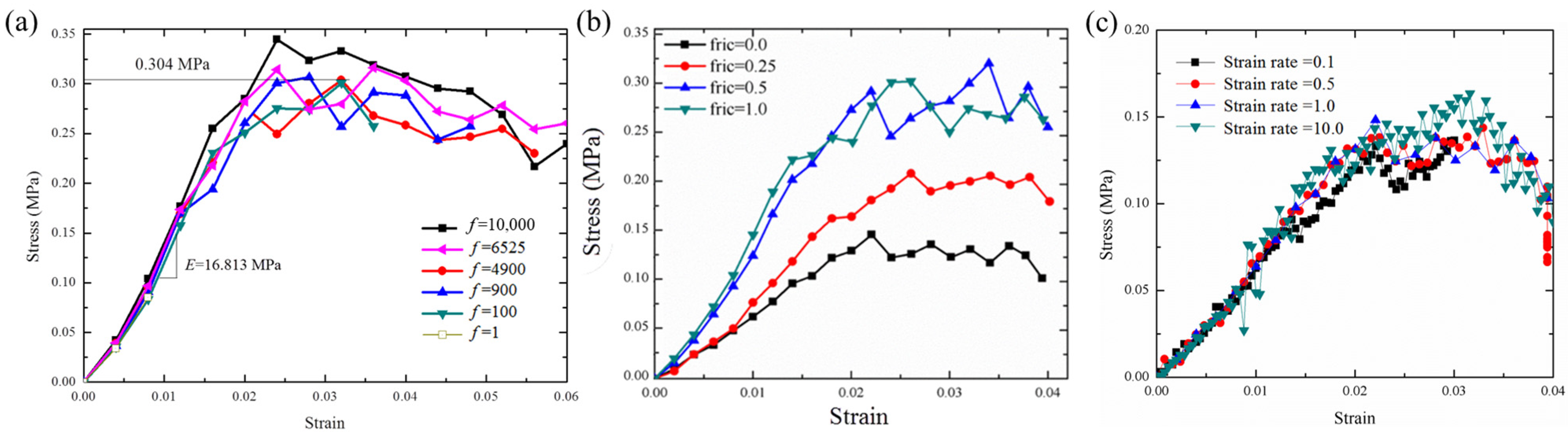
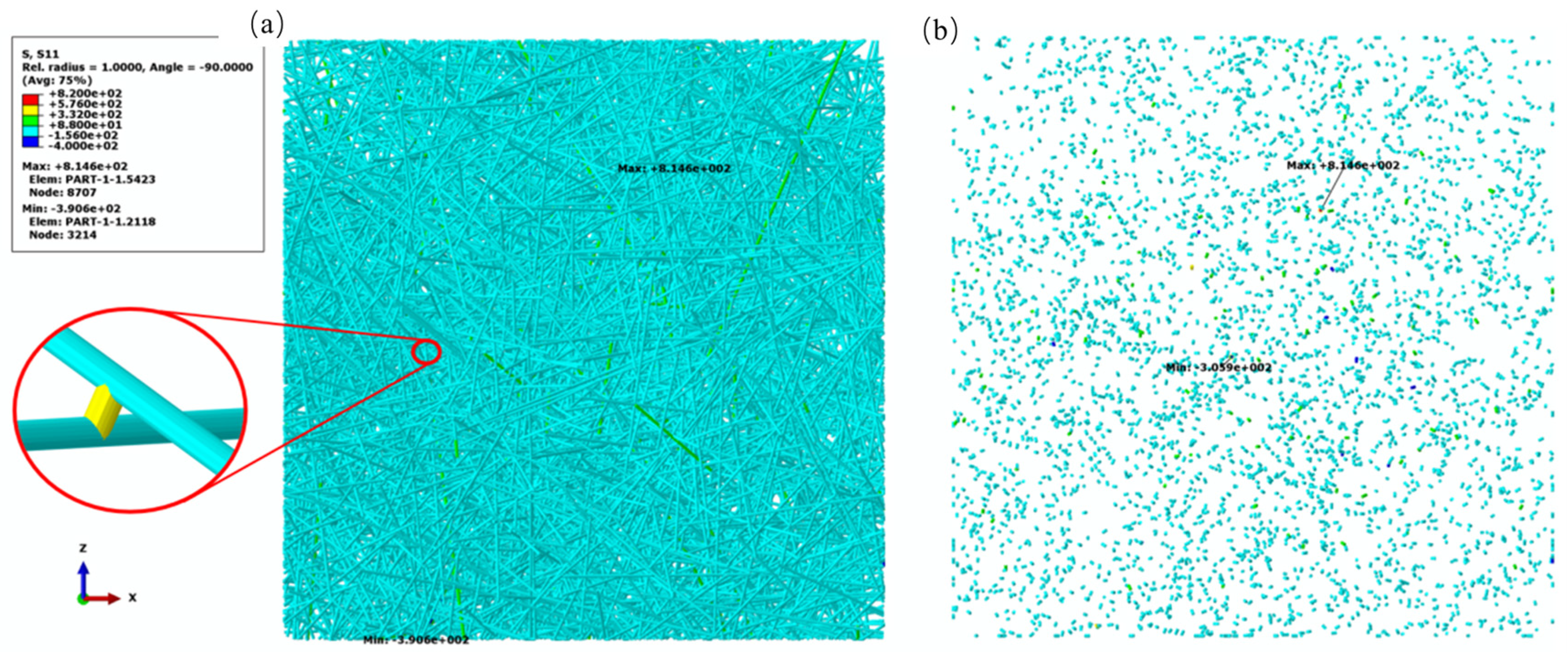
3.2. Numerical Results
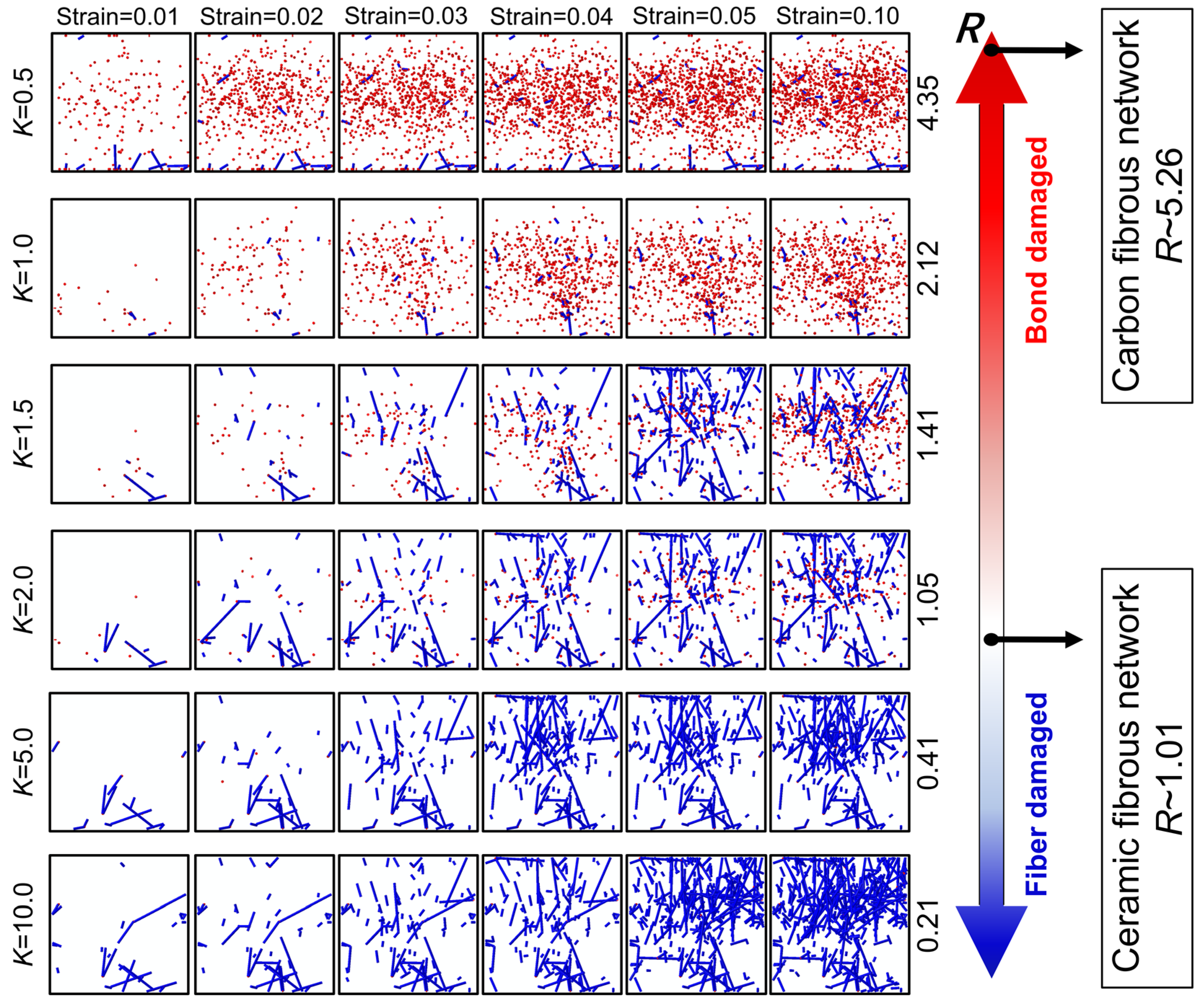
4. Discussion
5. Conclusions
- Based on the numerical results of 3D network models, it was found that the failure mode transition of network is from the bond damage domain to the fiber damage domain.
- For the variable R in the failure criterion, R > 1 means the failure mode of network tends to be bond damage; R < 1 indicates the failure mode of network tends to be fiber damage. This formula is verified by our experimental results, and is to be further confirmed by other network materials, such as paper and non-woven network.
- The failure mode of network depends on the network structure and mechanical properties of fibers and bonds. The value of variable R decreases with the increase in relative density, and increases with the increase of the ratio of bond strength to fiber strength.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Pritchard, R.H.; Huang, Y.Y.S.; Terentjev, E.M. Mechanics of biological networks: From the cell cytoskeleton to connective tissue. Soft Matter 2014, 10, 1864–1884. [Google Scholar] [CrossRef] [PubMed]
- Xie, B.; Liu, Y.; Ding, Y.; Zheng, Q.; Xu, Z. Mechanics of carbon nanotube networks: Microstructural evolution and optimal design. Soft Matter 2011, 7, 10039–10047. [Google Scholar] [CrossRef]
- Chen, Y.; Ridruejo, A.; González, C.; Llorca, J.; Siegmund, T. Notch effect in failure of fiberglass non-woven materials. Int. J. Solids Struct. 2016, 96, 254–264. [Google Scholar] [CrossRef]
- Ridruejo, A.; Jubera, R.; González, C.; Llorca, J. Inverse notch sensitivity: Cracks can make nonwoven fabrics stronger. J. Mech. Phys. Solids 2015, 77, 61–69. [Google Scholar] [CrossRef]
- Mao, R.; Goutianos, S.; Tu, W.; Meng, N.; Yang, G.; Berglund, L.A.; Peijs, T. Comparison of fracture properties of cellulose nanopaper, printing paper and buckypaper. J. Mater. Sci. 2017, 52, 9508–9519. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, Z.; Yang, Z.; Zhang, D.; Li, J.; Shi, J.; Chen, H. Resilient carbon fiber network materials under cyclic compression. Carbon 2019, 155, 344–352. [Google Scholar] [CrossRef]
- Zhang, Y.; Lu, Z.; Yang, Z.; Zhang, D.; Shi, J.; Yuan, Z.; Liu, Q. Compression behaviors of carbon-bonded carbon fiber composites: Experimental and numerical investigations. Carbon 2017, 116, 398–408. [Google Scholar] [CrossRef]
- Liu, Q.; Lu, Z.; Zhu, M.; Yang, Z.; Hu, Z.; Li, J. Experimental and FEM analysis of the compressive behavior of 3D random fibrous materials with bonded networks. J. Mater. Sci. 2014, 49, 1386–1398. [Google Scholar] [CrossRef]
- Lu, Z.; Liu, Q.; Han, H.; Zhang, D. Experiment and modeling on the compressive behaviors for porous silicon nitride ceramics. Mater. Sci. Eng. A-Struct. 2013, 559, 201–209. [Google Scholar] [CrossRef]
- Huang, X.; Wang, Q.; Zhou, W.; Li, J. A simple fracture energy prediction method for fiber network based on its morphological features extracted by X-ray tomography. Mater. Sci. Eng. A-Struct. 2013, 585, 297–303. [Google Scholar] [CrossRef]
- Jin, M.Z.; Chen, C.Q.; Lu, T.J. The mechanical behavior of porous metal fiber sintered sheets. J. Mech. Phys. Solids 2013, 61, 161–174. [Google Scholar] [CrossRef]
- Hasan, A.; Ragaert, K.; Swieszkowski, W.; Selimović, Š.; Paul, A.; Camci-Unal, G.; Khademhosseini, A. Biomechanical properties of native and tissue engineered heart valve constructs. J. Biomech. 2014, 47, 1949–1963. [Google Scholar] [CrossRef] [PubMed]
- Liu, G.; Song, W.; Li, P. Compressive mechanical properties of metal fiber sintered sheets at different strain rates. Compos. Struct. 2020, 233, 111703. [Google Scholar] [CrossRef]
- Karakoҫ, A.; Paltakari, J.; Taciroglu, E. On the computational homogenization of three-dimensional fibrous materials. Compos. Struct. 2020, 242, 112151. [Google Scholar] [CrossRef]
- Bergström, P.; Hossain, S.; Uesaka, T. Scaling behaviour of strength of 3D-, semi-flexible-, cross-linked fibre network. Int. J. Solids Struct. 2019, 166, 68–74. [Google Scholar] [CrossRef]
- Barbier, C.; Dendievel, R.; Rodney, D. Role of friction in the mechanics of nonbonded fibrous materials. Phys. Rev. E 2009, 80, 016115. [Google Scholar] [CrossRef] [PubMed]
- Heyden, S.; Gustafsson, P.J. Simulation of fracture in a cellulose fibre network. J. Pulp Pap. Sci. 1998, 24, 160–165. [Google Scholar]
- Mao, R.; Goutianos, S.; Tu, W.; Meng, N.; Chen, S.; Peijs, T. Modelling the elastic properties of cellulose nanopaper. Mater. Design 2017, 126, 183–189. [Google Scholar] [CrossRef]
- Koh, C.T.; Oyen, M.L. Branching toughens fibrous networks. J. Mech. Behav. Biomed. Mater. 2012, 12, 74–82. [Google Scholar] [CrossRef]
- Goutianos, S.; Mao, R.; Peijs, T. Effect of inter-fibre bonding on the fracture of fibrous networks with strong interactions. Int. J. Solids Struct. 2018, 136, 271–278. [Google Scholar] [CrossRef]
- Long, K.; Shi, L.; Zhong, Y.; Luo, G.; Ma, X.; Li, M.; Guan, C. Effects of equivalent beam element on the in-plane shear performance of 3D stochastic fibrous networks. Ceram. Int. 2019, 45, 12734–12741. [Google Scholar] [CrossRef]
- Luo, G.; Shi, L.; Zhong, Y.; Yang, L.; Ma, X.; Li, M.; He, X. Compressive damage of three-dimensional random fibrous ceramic materials: A meso-mechanics modeling and experimental study. Ceram. Int. 2018, 44, 15690–15699. [Google Scholar] [CrossRef]
- Shi, L.; Long, K.; Zhong, Y.; Luo, G.; Ma, X.; Li, M.; He, X.; Yu, J. Compressive and shear performance of three-dimensional rigid stochastic fibrous networks: Experiment, finite element simulation, and factor analysis. J. Eur. Ceram. Soc. 2020, 40, 115–126. [Google Scholar] [CrossRef]
- Yang, M.; Ji, M.; Taghipour, E.; Soghrati, S. Cross-linked fiberglass packs: Microstructure reconstruction and finite element analysis of the micromechanical behavior. Comput. Struct. 2018, 209, 182–196. [Google Scholar] [CrossRef]
- Chen, Y.; Pan, F.; Guo, Z.; Liu, B.; Zhang, J. Stiffness threshold of randomly distributed carbon nanotube networks. J. Mech. Phys. Solids 2015, 84, 395–423. [Google Scholar] [CrossRef]
- Bronkhorst, C.A. Modelling paper as a two-dimensional elastic–plastic stochastic network. Int. J. Solids Struct. 2003, 40, 5441–5454. [Google Scholar] [CrossRef]
- Huang, Y.; Qiu, Y.; Wei, Y. Composite interlaminar fracture toughness imparted by electrospun PPO veils and interleaf particles: A mechanistical comparison. Compos. Struct. 2023, 312, 116865. [Google Scholar] [CrossRef]
- Zhang, H.; Huang, Y.J.; Xu, S.L.; Natarajan, S.; Yao, F. An explicit methodology of random fibre modelling for FRC fracture using non-conforming meshes and cohesive interface elements. Compos. Struct. 2023, 310, 116762. [Google Scholar] [CrossRef]
- Borodulina, S.; Motamedian, H.R.; Kulachenko, A. Effect of fiber and bond strength variations on the tensile stiffness and strength of fiber networks. Int. J. Solids Struct. 2018, 154, 19–32. [Google Scholar] [CrossRef]
- Negi, V.; Picu, R.C. Mechanical behavior of cross-linked random fiber networks with inter-fiber adhesion. J. Mech. Phys. Solids 2019, 122, 418–434. [Google Scholar] [CrossRef]
- Deogekar, S.; Islam, M.R.; Picu, R.C. Parameters controlling the strength of stochastic fibrous materials. Int. J. Solids Struct. 2019, 168, 194–202. [Google Scholar] [CrossRef] [PubMed]
- Deogekar, S.; Picu, R.C. On the strength of random fiber networks. J. Mech. Phys. Solids 2018, 116, 1–16. [Google Scholar] [CrossRef]
- Malakhovsky, I.; Michels, M.A.J. Effect of disorder strength on the fracture pattern in heterogeneous networks. Phys. Rev. B 2007, 76, 144201. [Google Scholar] [CrossRef]
- Berthier, E.; Kollmer, J.E.; Henkes, S.E.; Liu, K.; Schwarz, J.M.; Daniels, K.E. Rigidity percolation control of the brittle-ductile transition in disordered networks. Phys. Rev. Mater. 2019, 3, 075602. [Google Scholar] [CrossRef]
- Luo, G.; Shi, L.; Li, M.; Zhong, Y.; He, X.; Wang, J. Ductile–brittle transition in transverse isotropic fibrous networks. Appl. Phys. Lett. 2018, 112, 051904. [Google Scholar] [CrossRef]
- Liu, Q.; Lu, Z.; Hu, Z.; Li, J. Finite element analysis on tensile behaviour of 3D random fibrous materials: Model description and meso-level approach. Mater. Sci. Eng. A-Struct. 2013, 587, 36–45. [Google Scholar] [CrossRef]
- Davies, I.J.; Rawlings, R.D. Mechanical properties in flexure and tension of low density carbon-carbon composites. Carbon 1994, 32, 1449–1456. [Google Scholar] [CrossRef]
- Hatta, H.; Suzuki, K.; Shigei, T.; Somiya, S.; Sawada, Y. Strength improvement by densification of C/C composites. Carbon 2001, 39, 83–90. [Google Scholar] [CrossRef]
- Peters, P.W.; Daniels, B.; Clemens, F.; Vogel, W.D. Mechanical characterisation of mullite-based ceramic matrix composites at test temperatures up to 1200 °C. J. Eur. Ceram. Soc. 2000, 20, 531–535. [Google Scholar] [CrossRef]
- Allaoui, A.; Bai, S.; Cheng, H.M.; Bai, J.B. Mechanical and electrical properties of a MWNT/epoxy composite. Compos. Sci. Technol. 2002, 62, 1993–1998. [Google Scholar] [CrossRef]
- Camus, G.; Guillaumat, L.; Baste, S. Development of damage in a 2D woven C/SiC composite under mechanical loading: I. Mechanical characterization. Compos. Sci. Technol. 1996, 56, 1363–1372. [Google Scholar] [CrossRef]
- Morscher, G.N.; Ojard, G.; Miller, R.; Gowayed, Y.; Santhosh, U.; Ahmad, J.; John, R. Tensile creep and fatigue of Sylramic-iBN melt-infiltrated SiC matrix composites: Retained properties, damage development, and failure mechanisms. Compos. Sci. Technol. 2008, 68, 3305–3313. [Google Scholar] [CrossRef]
- Olurin, O.B.; Fleck, N.A.; Ashby, M.F. Deformation and fracture of aluminium foams. Mater. Sci. Eng. A-Struct. 2000, 291, 136–146. [Google Scholar] [CrossRef]
- Kabir, M.E.; Saha, M.C.; Jeelani, S. Tensile and fracture behavior of polymer foams. Mater. Sci. Eng. A-Struct. 2006, 429, 225–235. [Google Scholar] [CrossRef]
- Viana, G.M.; Carlsson, L.A. Mechanical properties and fracture characterization of cross-linked PVC foams. J. Sandw. Struct. Mater. 2002, 4, 99–113. [Google Scholar] [CrossRef]
- Matuana, L.M.; Park, C.B.; Balatinecz, J.J. Cell morphology and property relationships of microcellular foamed pvc/wood-fiber composites. Polym. Eng. Sci. 1998, 38, 1862–1872. [Google Scholar] [CrossRef]
- Mae, H.; Omiya, M.; Kishimoto, K. Effects of strain rate and density on tensile behavior of polypropylene syntactic foam with polymer microballoons. Mater. Sci. Eng. A-Struct. 2008, 477, 168–178. [Google Scholar] [CrossRef]
- Zhao, T.F.; Chen, C.Q.; Deng, Z.C. Elastoplastic properties of transversely isotropic sintered metal fiber sheets. Mater. Sci. Eng. A-Struct. 2016, 662, 308–319. [Google Scholar] [CrossRef]
- Ridruejo, A.; González, C.; Llorca, J. Micromechanisms of deformation and fracture of polypropylene nonwoven fabrics. Int. J. Solids Struct. 2011, 48, 153–162. [Google Scholar] [CrossRef]
- Gao, H.L.; Zhu, Y.B.; Mao, L.B.; Wang, F.C.; Luo, X.S.; Liu, Y.Y.; Lu, Y.; Pan, Z.; Ge, J.; Shen, W.; et al. Super-elastic and fatigue resistant carbon material with lamellar multi-arch microstructure. Nat. Commun. 2016, 7, 12920. [Google Scholar] [CrossRef]
- Isaksson, P.; Gradin, P.A.; Kulachenko, A. The onset and progression of damage in isotropic paper sheets. Int. J. Solids Struct. 2006, 43, 713–726. [Google Scholar] [CrossRef]
- Chen, N.; Koker, M.K.A.; Uzun, S.; Silberstein, M.N. In-situ X-ray study of the deformation mechanisms of non-woven polypropylene. Int. J. Solids Struct. 2016, 97, 200–208. [Google Scholar] [CrossRef]
- Chen, N.; Silberstein, M.N. Determination of bond strengths in non-woven fabrics: A combined experimental and computational approach. Exp. Mech. 2018, 58, 343–355. [Google Scholar] [CrossRef]




| Silica Fiber | Mullite Fiber | Carbon Fiber | B2O3 Bond | Pyrolytic Carbon Bond | |
|---|---|---|---|---|---|
| Elastic modulus | 78 GPa | 193 GPa | 230 GPa | 27 GPa | 4.65 GPa |
| Strength | 1.7 GPa | 2.0 GPa | 3.5 GPa | 2.0 GPa | 0.2 GPa |
| Geometrical Parameters | Mechanical Properties | ||
|---|---|---|---|
| Relative density (ρ) | 0.08~0.45 | The elastic modulus of the fibers (Ef) | 100 GPa |
| Fiber diameter (D) | 9.0 μm | The elastic modulus of the bonds (Eb) | 100 GPa |
| Bond diameter | 9.0 μm | The strength of the fibers (σf) | 1.0 GPa |
| Fiber length (L) | 800 μm | The strength of the bonds (σb) | 0.1~10 GPa |
| Bond length | 0~9.0 μm | Poisson ratio of the fibers and the bonds | 0.26 |
| W × T × H | 1.5 L × 1.0 L × 1.5 L | The density of the fibers and the bonds | 100 g/cm3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Wang, W.; Wang, P.; Lu, Z.; Yang, Z. Microstructural Evolution and Failure in Fibrous Network Materials: Failure Mode Transition from the Competition between Bond and Fiber. Materials 2024, 17, 2110. https://doi.org/10.3390/ma17092110
Zhang Y, Wang W, Wang P, Lu Z, Yang Z. Microstructural Evolution and Failure in Fibrous Network Materials: Failure Mode Transition from the Competition between Bond and Fiber. Materials. 2024; 17(9):2110. https://doi.org/10.3390/ma17092110
Chicago/Turabian StyleZhang, Yao, Weihua Wang, Pengfei Wang, Zixing Lu, and Zhenyu Yang. 2024. "Microstructural Evolution and Failure in Fibrous Network Materials: Failure Mode Transition from the Competition between Bond and Fiber" Materials 17, no. 9: 2110. https://doi.org/10.3390/ma17092110
APA StyleZhang, Y., Wang, W., Wang, P., Lu, Z., & Yang, Z. (2024). Microstructural Evolution and Failure in Fibrous Network Materials: Failure Mode Transition from the Competition between Bond and Fiber. Materials, 17(9), 2110. https://doi.org/10.3390/ma17092110





