Process Maps for Predicting Austenite Fraction (vol.%) in Medium-Mn Third-Generation Advanced High-Strength Steels
Abstract
1. Introduction
2. Overview of Models
2.1. DICTRA-Based Model
2.2. Koistinen–Marburger Model
3. Results
3.1. Tempered Martensite Starting Microstructure
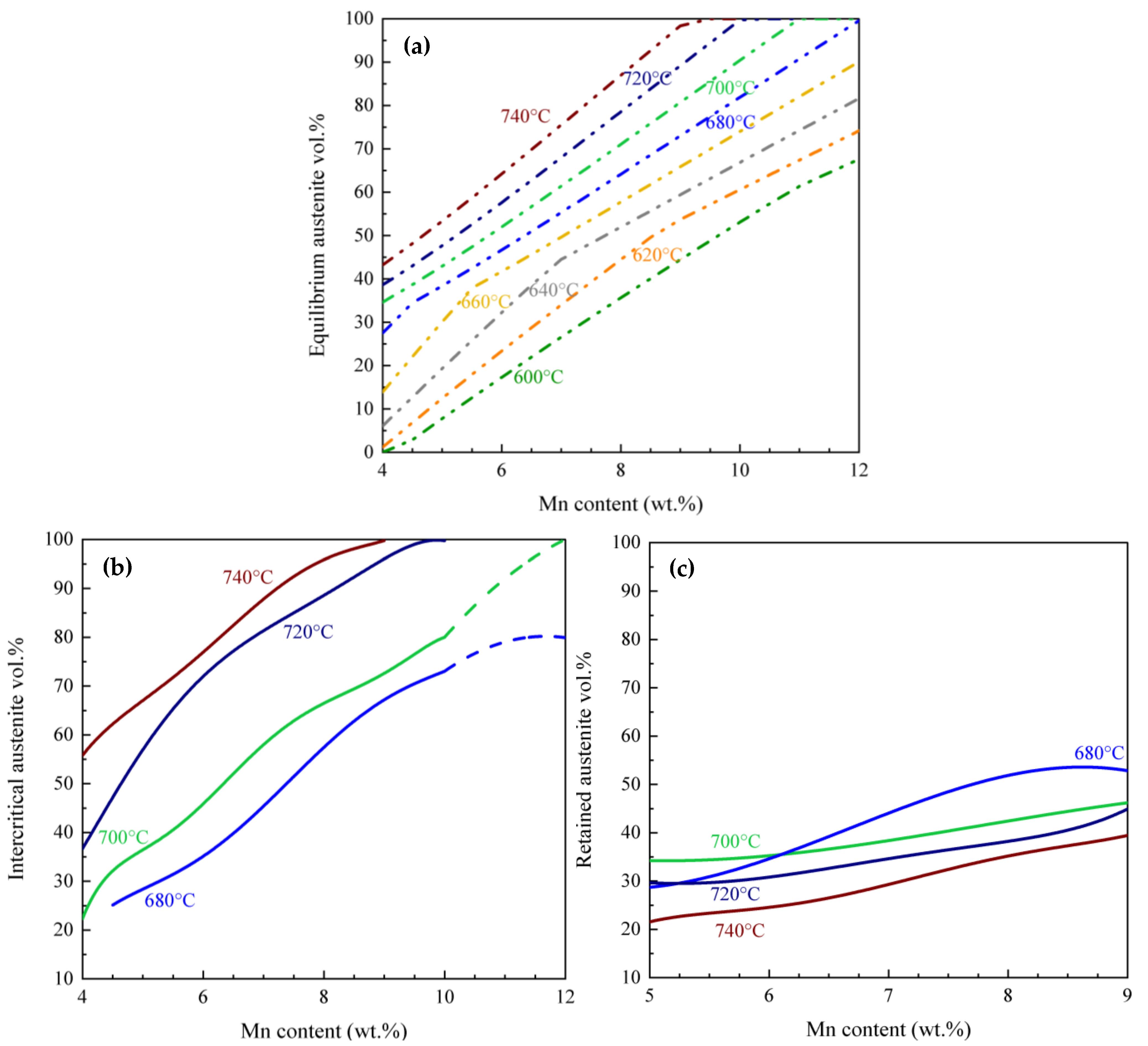
3.1.1. Intercritical Austenite vol.% Calculation Using DICTRA
3.1.2. Retained Austenite Calculation
3.2. Martensite Starting Microstructure
3.2.1. Intercritical Austenite vol.% Calculation Using DICTRA
3.2.2. Retained Austenite Calculation
3.3. Comparing the Model with the Literature
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bouaziz, O.; Zurob, H.; Huang, M. Driving force and logic of development of advanced high strength steels for automotive applications. Steel Res. Int. 2013, 84, 937–947. [Google Scholar] [CrossRef]
- Lesch, C.; Kwiaton, N.; Klose, F.B. Advanced High Strength Steels (AHSS) for Automotive Applications—Tailored Properties by Smart Microstructural Adjustments. Steel Res. Int. 2017, 88, 1700210. [Google Scholar] [CrossRef]
- Kuziak, R.; Kawalla, R.; Waengler, S. Advanced high strength steels for automotive industry: A review. Arch. Civ. Mech. Eng. 2008, 8, 103–117. [Google Scholar] [CrossRef]
- De Cooman, B.C.; Estrin, Y.; Kim, S.K. Twinning-induced plasticity (TWIP) steels. Acta Mater. 2018, 142, 283–362. [Google Scholar] [CrossRef]
- Liu, L.; He, B.; Huang, M. The Role of Transformation-Induced Plasticity in the Development of Advanced High Strength Steels. Adv. Eng. Mater. 2018, 20, 1701083. [Google Scholar] [CrossRef]
- Liu, C.; Peng, Q.; Xue, Z.; Wang, S.; Yang, C. Microstructure and mechanical properties of hot- rolled and cold-rolled medium-Mn TRIP steels. Materials 2018, 11, 2242. [Google Scholar] [CrossRef] [PubMed]
- Jang, J.-M.; Kim, S.-J.; Kang, N.H.; Cho, K.-M.; Suh, D.-W. Effects of annealing conditions on microstructure and mechanical properties of low carbon, manganese transformation-induced plasticity steel. Met. Mater. Int. 2009, 15, 909–916. [Google Scholar] [CrossRef]
- Lee, Y.-K.; Han, J. Current opinion in medium manganese steel. Mater. Sci. Technol. 2015, 31, 843–856. [Google Scholar] [CrossRef]
- Hu, B.; Luo, H.; Yang, F.; Dong, H. Recent progress in medium-Mn steels made with new designing strategies, a review. J. Mater. Sci. Technol. 2017, 33, 1457–1464. [Google Scholar] [CrossRef]
- Ma, Y. Medium-manganese steels processed by austenite-reverted-transformation annealing for automotive applications. Mater. Sci. Technol. 2017, 33, 1713–1727. [Google Scholar] [CrossRef]
- Suh, D.-W.; Kim, S.-J. Medium Mn transformation-induced plasticity steels: Recent progress and challenges. Scr. Mater. 2017, 126, 63–67. [Google Scholar] [CrossRef]
- Soleimani, M.; Kalhor, A.; Mirzadeh, H. Transformation-induced plasticity (TRIP) in advanced steels: A review. Mater. Sci. Eng. A 2020, 795, 140023. [Google Scholar] [CrossRef]
- Dai, Z.; Chen, H.; Ding, R.; Lu, Q.; Zhang, C.; Yang, Z.; van der Zwaag, S. Fundamentals and application of solid-state phase transformations for advanced high strength steels containing metastable retained austenite. Mater. Sci. Eng. R Rep. 2021, 143, 100590. [Google Scholar] [CrossRef]
- Chang, Y.; Wang, C.; Zhao, K.; Dong, H.; Yan, J. An introduction to medium-Mn steel: Metallurgy, mechanical properties and warm stamping process. Mater. Des. 2016, 94, 424–432. [Google Scholar] [CrossRef]
- Miller, R.L. Ultrafine-grained microstructures and mechanical properties of alloy steels. Met. Trans. 1972, 3, 905–912. [Google Scholar] [CrossRef]
- Suh, D.W.; Ryu, J.H.; Joo, M.S.; Yang, H.S.; Lee, K.Y. Medium—Alloy Manganese—Rich Transformation—Induced Plasticity Steels. Metall. Mater. Trans. A 2013, 46A, 286–293. [Google Scholar] [CrossRef]
- Luo, H.; Dong, H.; Huang, M. Effect of intercritical annealing on the Lüders strains of medium Mn transformation-induced plasticity steels. Mater. Des. 2015, 83, 42–48. [Google Scholar] [CrossRef]
- Schneider, R.; Steineder, K.; Krizan, D.; Sommitsch, C. Effect of the heat treatment on the microstructure and mechanical properties of medium-Mn-steels. Mater. Sci. Technol. 2019, 35, 2045–2053. [Google Scholar] [CrossRef]
- De Moor, E.; Matlock, D.K.; Speer, J.G.; Merwin, M.J. Austenite stabilization through manganese enrichment. Scr. Mater. 2011, 64, 185–188. [Google Scholar] [CrossRef]
- Marder, A.R. The metallurgy of zinc-coated steel. Prog. Mater. Sci. 2000, 45, 191–271. [Google Scholar] [CrossRef]
- Luo, H.; Dong, H. New ultrahigh-strength Mn-alloyed TRIP steels with improved formability manufactured by intercritical annealing. Mater. Sci. Eng. A 2015, 46A, 207–212. [Google Scholar] [CrossRef]
- Cao, W.; Wang, C.; Shi, J.; Wang, M.; Hui, W.; Dong, H. Microstructure and mechanical properties of Fe-0.2C-5Mn steel processed by ART-annealing. Mater. Sci. Eng. A 2011, 528, 6661–6666. [Google Scholar] [CrossRef]
- Arlazarov, A.; Gouné, M.; Bouaziz, O.; Hazotte, A.; Petitgand, G.; Barges, P. Evolution of microstructure and mechanical properties of medium Mn steels during double annealing. Mater. Sci. Eng. A 2012, 542, 31–39. [Google Scholar] [CrossRef]
- Gibbs, P.J.; De Moor, E.; Merwin, M.J.; Clausen, B.; Speer, J.G.; Matlock, D.K. Austenite stability effects on tensile behavior of manganese-enriched- austenite transformation-induced plasticity steel. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2011, 42, 3691–3702. [Google Scholar] [CrossRef]
- Shi, J.; Sun, X.; Wang, M.; Hui, W.; Dong, H.; Cao, W. Enhanced work-hardening behavior and mechanical properties in ultrafine-grained steels with large-fractioned metastable austenite. Scr. Mater. 2010, 63, 815–818. [Google Scholar] [CrossRef]
- Pallisco, D.M.; McDermid, J.R. Mechanical property development of a 0.15C–6Mn–2Al–1Si third-generation advanced high strength steel using continuous galvanizing heat treatments. Mater. Sci. Eng. A 2020, 778, 139111. [Google Scholar] [CrossRef]
- Lee, S.; De Cooman, B.C. On the selection of the optimal intercritical annealing temperature for medium Mn TRIP steel. Metall. Mater. Trans. A Phys. Metall. Mater. Sci. 2013, 44, 5018–5024. [Google Scholar] [CrossRef]
- Bhadhon, K.; Wang, X.; McDermid, J. Effects of CGL-compatible thermal processing, starting microstructure, and Sn micro-alloying on the mechanical properties of a medium-Mn third generation advanced high strength steel. Mater. Sci. Eng. A 2022, 833, 142563. [Google Scholar] [CrossRef]
- Andersson, J.-O.; Helander, T.; Höglund, L.; Shi, P.; Sundman, B. THERMO-CALC & DICTRA, Computational Tools for Materials Science. Calphad 2002, 26, 273–312. [Google Scholar]
- Borgenstam, A.; Höglund, L.; Ågren, J.; Engström, A. DICTRA, a tool for simulation of diffusional transformations in alloys. J. Phase Equilibria 2000, 21, 269–280. [Google Scholar] [CrossRef]
- Mehrabi, A.; McDermid, J.R.; Wang, X.; Zurob, H. Austenite Nucleation and Growth as a Function of Starting Microstructure for a Fe–0.15C–5.56Mn–1.1Si–1.89Al Medium-Mn Steel. Steel Res. Int. 2023, 94, 2200952. [Google Scholar] [CrossRef]
- Mehrabi, A.; Zurob, H.S.; Benrabah, I.E.; McDermid, J.R. Austenite formation in a medium-Mn steel during intercritical annealing via in situ high-energy X-ray diffraction. J. Mater. Res. Technol. 2024. [Google Scholar] [CrossRef]
- Dmitrieva, O.; Ponge, D.; Inden, G.; Millán, J.; Choi, P.; Sietsma, J.; Raabe, D. Chemical gradients across phase boundaries between martensite and austenite in steel studied by atom probe tomography and simulation. Acta Mater. 2011, 59, 364–374. [Google Scholar] [CrossRef]
- Luo, H.; Shi, J.; Wang, C.; Cao, W.; Sun, X.; Dong, H. Experimental and numerical analysis on formation of stable austenite during the intercritical annealing of 5Mn steel. Acta Mater. 2011, 59, 4002–4014. [Google Scholar] [CrossRef]
- Huyan, F.; Yan, J.-Y.; Höglund, L.; Ågren, J.; Borgenstam, A. Simulation of the Growth of Austenite from As-Quenched Martensite in Medium Mn Steels. Metall. Mater. Trans. A 2018, 49, 1053–1060. [Google Scholar] [CrossRef]
- Zhang, X.; Miyamoto, G.; Kaneshita, T.; Yoshida, Y.; Toji, Y.; Furuhara, T. Growth mode of austenite during reversion from martensite in Fe-2Mn-1.5Si-0.3C alloy: A transition in kinetics and morphology. Acta Mater. 2018, 154, 1–13. [Google Scholar] [CrossRef]
- Luo, H.; Liu, J.; Dong, H. A Novel Observation on Cementite Formed during Intercritical Annealing of Medium Mn Steel. Metall. Mater. Trans. A 2016, 47, 3119–3124. [Google Scholar] [CrossRef]
- Koistinen, D.P.; Marburger, R.E. A general equation prescribing the extent of the austenite-martensite transformation in pure iron-carbon alloys and plain carbon steels. Acta Metall. 1959, 7, 59–60. [Google Scholar] [CrossRef]
- Lee, S.J.; Lee, S.; De Cooman, B.C. Martensite transformation of sub-micron retained austenite in ultra-fine grained manganese transformation-induced plasticity steel. Int. J. Mater. Res. 2013, 104, 423–429. [Google Scholar] [CrossRef]
- Jimenez-Melero, E.; van Dijk, N.; Zhao, L.; Sietsma, J.; Offerman, S.; Wright, J.; van der Zwaag, S. Martensitic transformation of individual grains in low-alloyed TRIP steels. Scr. Mater. 2007, 56, 421–424. [Google Scholar] [CrossRef]
- Bhadhon, K.M.H.; Wang, X.; McNally, E.A.; McDermid, J.R. Effect of Intercritical Annealing Parameters and Starting Microstructure on the Microstructural Evolution and Mechanical Properties of a Medium-Mn Third Generation Advanced High Strength Steel. Metals 2022, 12, 356. [Google Scholar] [CrossRef]
- Mehrabi, A.; McDermid, J.R.; Zurob, H.S.; Goodwin, F.E. Austenite Nucleation and Growth. In Proceedings of the 5th International High Manganese Steel Conference, Linz, Austria, 23–25 May 2022. [Google Scholar]
- Nakada, N.; Mizutani, K.; Tsuchiyama, T.; Takaki, S. Difference in transformation behavior between ferrite and austenite formations in medium manganese steel. Acta Mater. 2014, 65, 251–258. [Google Scholar] [CrossRef]
- Caballero, F.G.; Garcia-Mateo, C.; Chao, J.; Santofimia, M.J.; Capdevila, C.; García de Andres, C. Effects of Morphology and Stability of Retained Austenite on the Ductility of TRIP-aided Bainitic Steels. ISIJ Int. 2008, 48, 1256–1262. [Google Scholar] [CrossRef]
- Lee, S.; Estrin, Y.; De Cooman, B.C. Constitutive modeling of the mechanical properties of V-added medium manganese TRIP steel. Metall. Mater. Trans. A 2013, 44, 3136–3146. [Google Scholar] [CrossRef]
- Lee, S.; Lee, S.-J.; Kumar, S.S.; Lee, K.; De Cooman, B.C. Localized deformation in multiphase, ultra-fine-grained 6 Pct Mn transformation-induced plasticity steel. Metall. Mater. Trans. A 2011, 42, 3638–3651. [Google Scholar] [CrossRef]
- Lee, D.; Kim, J.-K.; Lee, S.; Lee, K.; De Cooman, B.C. Microstructures and mechanical properties of Ti and Mo micro-alloyed medium Mn steel. Mater. Sci. Eng. A 2017, 706, 1–14. [Google Scholar] [CrossRef]
- Kim, J.I.; Ryu, J.H.; Lee, S.W.; Lee, K.; Heo, Y.-U.; Suh, D.-W. Influence of the Initial Microstructure on the Reverse Transformation Kinetics and Microstructural Evolution in Transformation-Induced Plasticity–Assisted Steel. Metall. Mater. Trans. A 2016, 47, 5352–5361. [Google Scholar] [CrossRef]
- Nakada, N.; Tsuchiyama, T.; Takaki, S.; Miyano, N. Temperature dependence of austenite nucleation behavior from lath martensite. ISIJ Int. 2011, 51, 299–304. [Google Scholar] [CrossRef]
- Wei, R.; Enomoto, M.; Hadian, R.; Zurob, H.; Purdy, G. Growth of austenite from as-quenched martensite during intercritical annealing in an Fe-0.1C-3Mn-1.5Si alloy. Acta Mater. 2013, 61, 697–707. [Google Scholar] [CrossRef]
- Ågren, J. Computer simulations of the austenite/ferrite diffusional transformations in low alloyed steels. Acta Metall. 1982, 30, 841–851. [Google Scholar] [CrossRef]
- Garcia, C.I.; Deardo, A.J. Formation of Austenite in 1.5 Pct Mn Steels. Met. Trans. A 1981, 12, 521–530. [Google Scholar] [CrossRef]
- Wycliffe, P.A.; Purdy, G.R.; Embury, J.D. Growth of austenite in the intercritical annealing of Fe-C-Mn dual phase steels. Can. Metall. Q. 1981, 20, 339–350. [Google Scholar] [CrossRef]
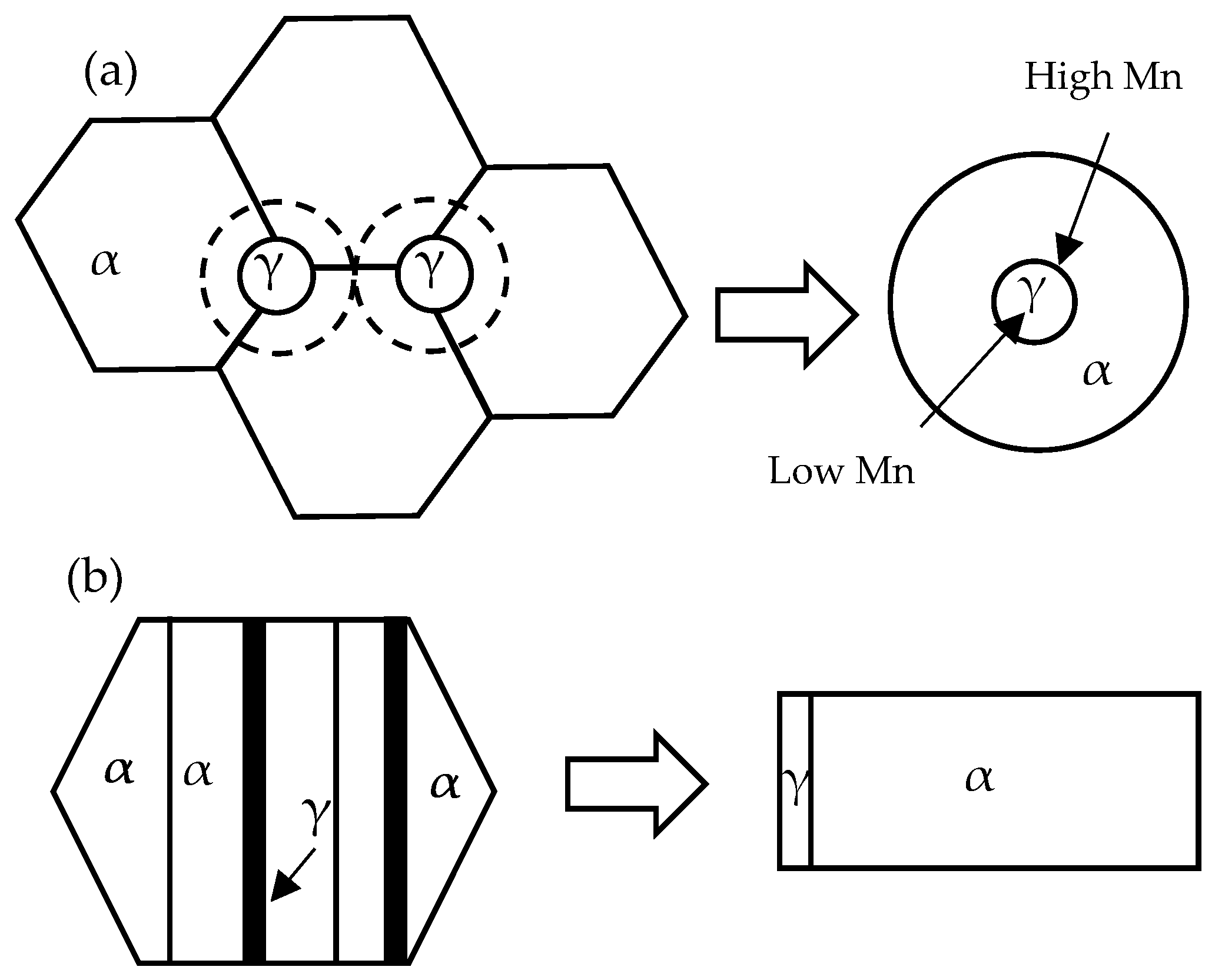


| Composition | Starting Microstructure | IAT (°C) | Measured γ (vol.%) | Calculated γ (vol.%) | Measured RA (vol.%) | Calculated RA (vol.%) |
|---|---|---|---|---|---|---|
| 0.18 C–5.91 Mn–1.5 Si–0.4 Al-0.6Cr [28] | TM | 675 | 15.0 | 19.6 | ||
| 0.15C–5.56Mn–1.89Al–1.1Si [31] | TM | 710 | 40.0 | 41.8 | 27.0 | 30.5 |
| 0.18 C–5.91 Mn–1.5 Si–0.4 Al-0.6Cr [28] | TM | 710 | 25.0 | 23.3 | ||
| 0.15C–5.56Mn–1.89Al–1.1Si [26,32] | M | 665 | 30.8 | 25.0 | 21.0 | 22.3 |
| 0.15C–5.56Mn–1.89Al–1.1Si [26,32] | M | 710 | 45.0 | 42.0 | 31.0 | 32.5 |
| 0.18 C–5.91 Mn–1.5 Si–0.4 Al-0.6Cr [28] | M | 675 | 25.0 | 28.4 | ||
| 0.18 C–5.91 Mn–1.5 Si–0.4 Al-0.6Cr [28] | M | 690 | 33.0 | 32.6 | ||
| 0.18 C–5.91 Mn–1.5 Si–0.4 Al-0.6Cr [28] | M | 710 | 37.0 | 35.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mehrabi, A.; Zurob, H.S.; McDermid, J.R. Process Maps for Predicting Austenite Fraction (vol.%) in Medium-Mn Third-Generation Advanced High-Strength Steels. Materials 2024, 17, 993. https://doi.org/10.3390/ma17050993
Mehrabi A, Zurob HS, McDermid JR. Process Maps for Predicting Austenite Fraction (vol.%) in Medium-Mn Third-Generation Advanced High-Strength Steels. Materials. 2024; 17(5):993. https://doi.org/10.3390/ma17050993
Chicago/Turabian StyleMehrabi, Azin, Hatem S. Zurob, and Joseph R. McDermid. 2024. "Process Maps for Predicting Austenite Fraction (vol.%) in Medium-Mn Third-Generation Advanced High-Strength Steels" Materials 17, no. 5: 993. https://doi.org/10.3390/ma17050993
APA StyleMehrabi, A., Zurob, H. S., & McDermid, J. R. (2024). Process Maps for Predicting Austenite Fraction (vol.%) in Medium-Mn Third-Generation Advanced High-Strength Steels. Materials, 17(5), 993. https://doi.org/10.3390/ma17050993







