Influence of Structural Optimization on the Physical Properties of an Innovative FDM 3D Printed Thermal Barrier
Abstract
1. Introduction
2. Materials and Methods
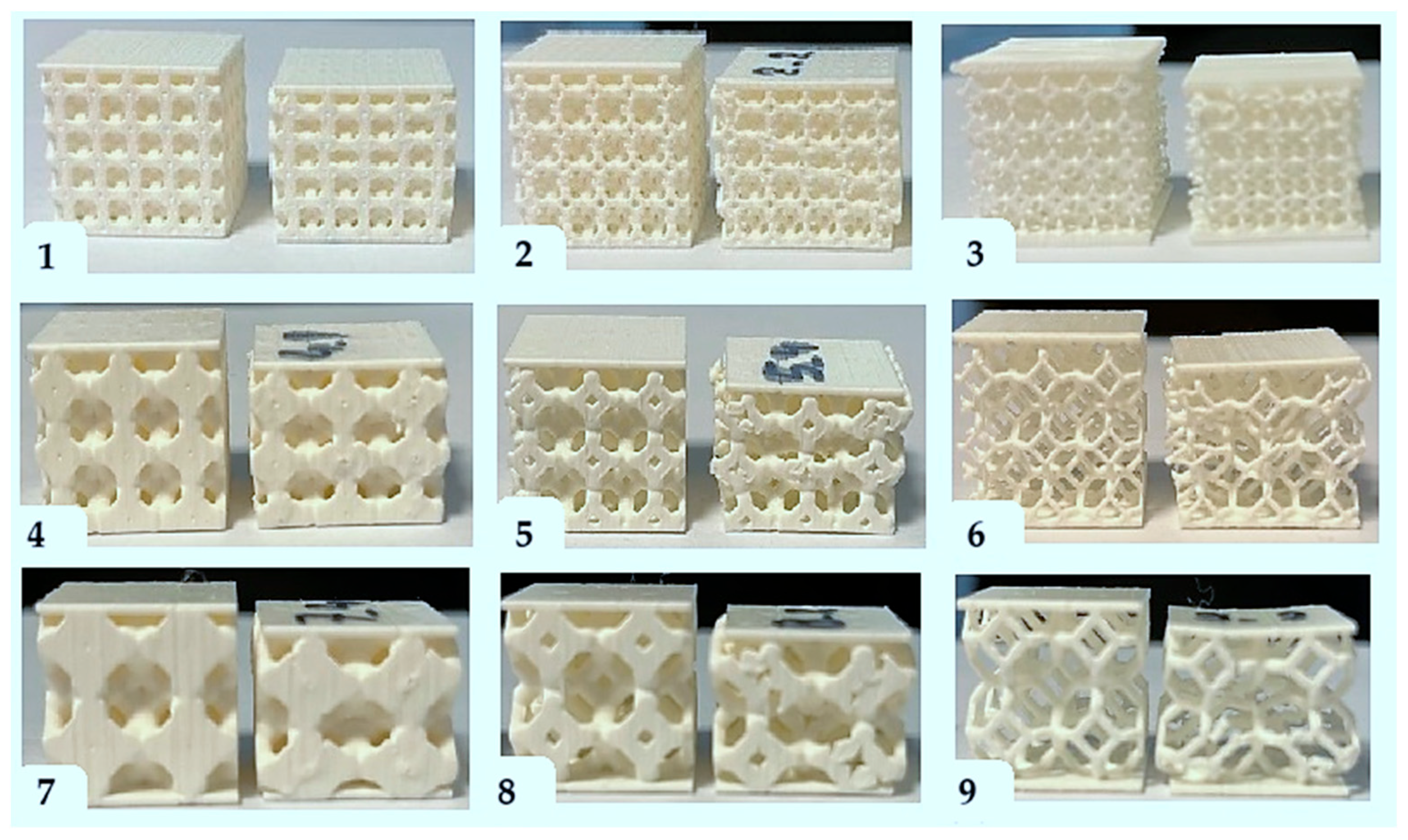
2.1. Research Object
2.2. Research Design
2.3. Sample Manufacturing Process
2.4. Determination of Properties
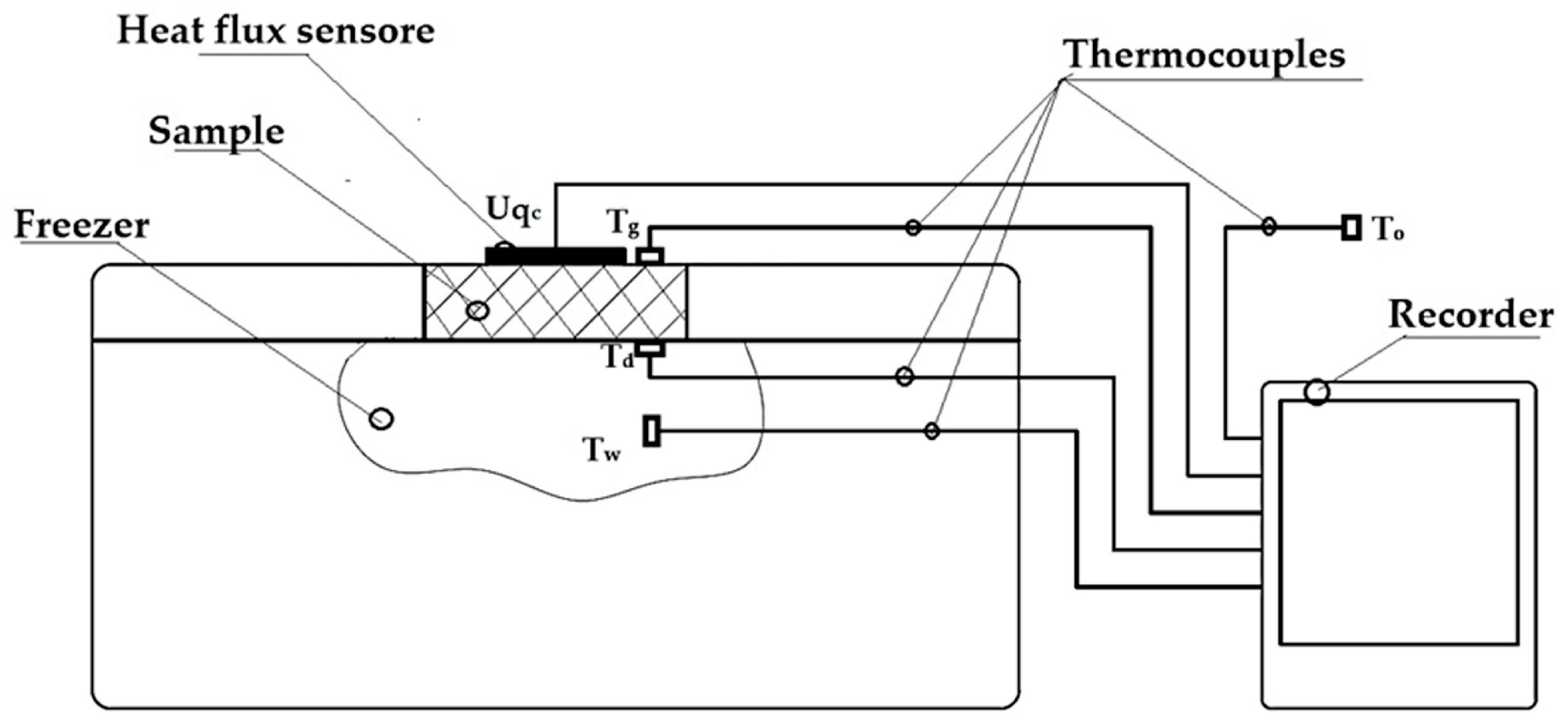
2.4.1. Thermal Conductivity and Thermal Resistance
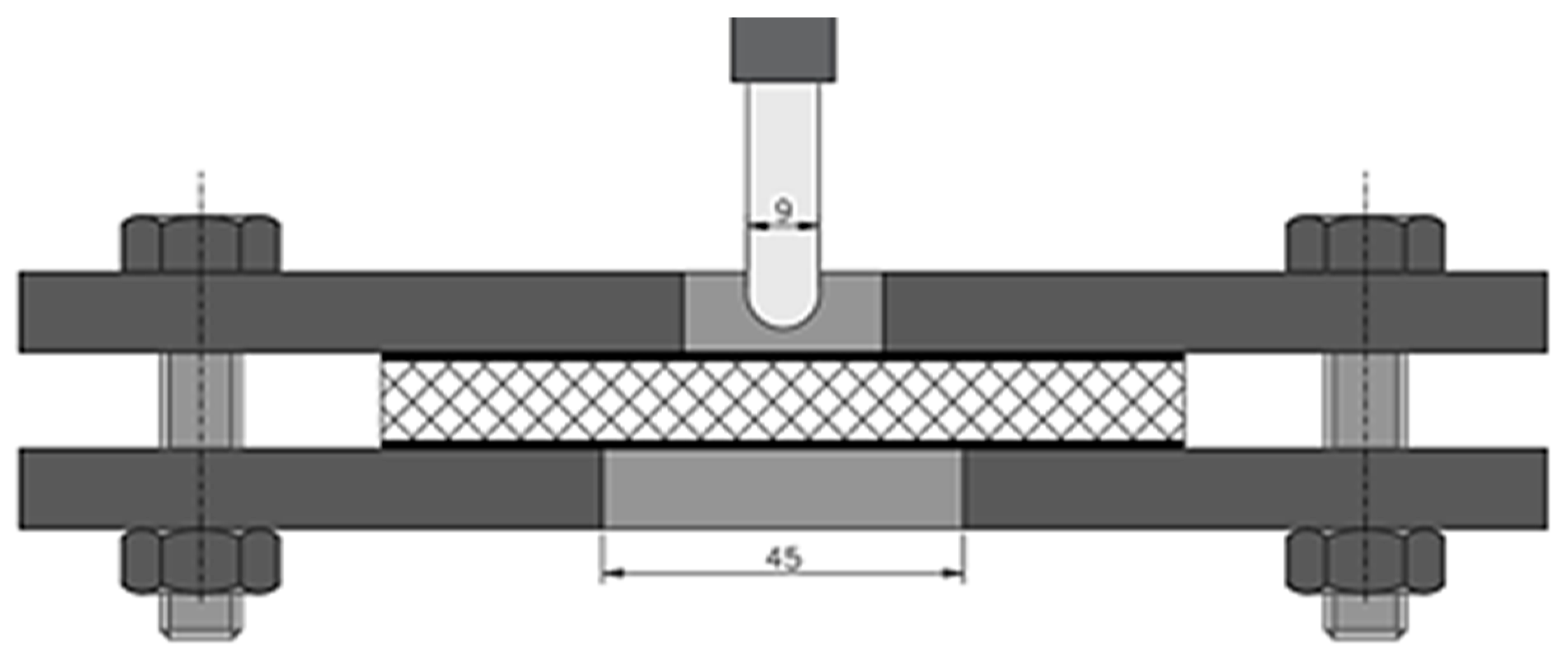
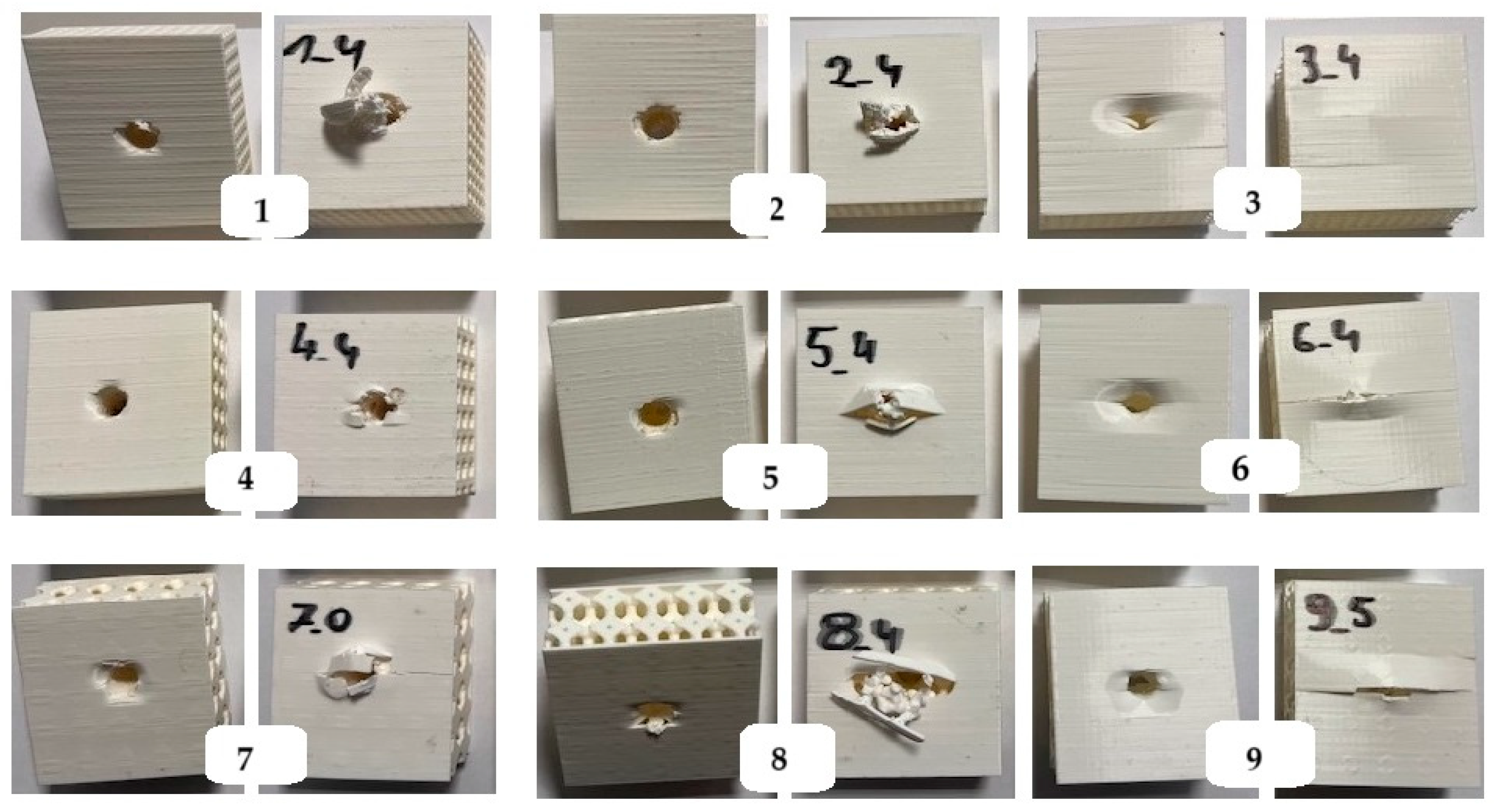
2.4.2. Compressive Strength and Quasi-Static Indentation Strength
- Energy absorbed by the material (Ea) refers to the total amount of energy that the material was able to absorb during the puncture process. It is the work performed by the penetrating force to cause damage to the material. The value of the absorbed energy was determined by integrating the area under the force-displacement curve:
- Punch Shear Strength (PSS) is an index used to assess the ability of a material to resist punching forces:
- Specific Energy Absorption index (SEA) is a measure that determines the amount of energy absorbed by a unit of mass of a material:
3. Results and Discussion
3.1. Thermal Conductivity and Thermal Resistance
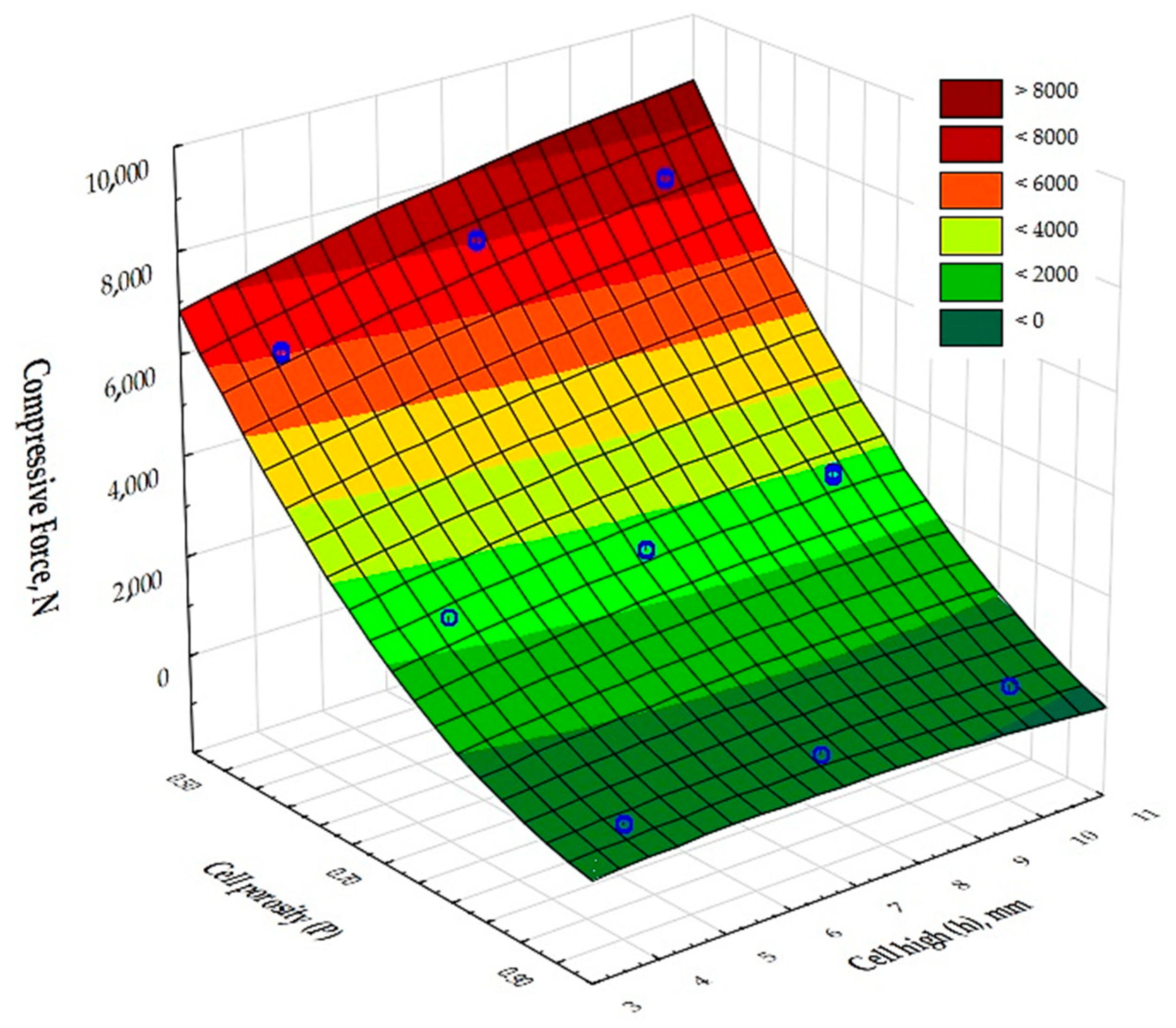
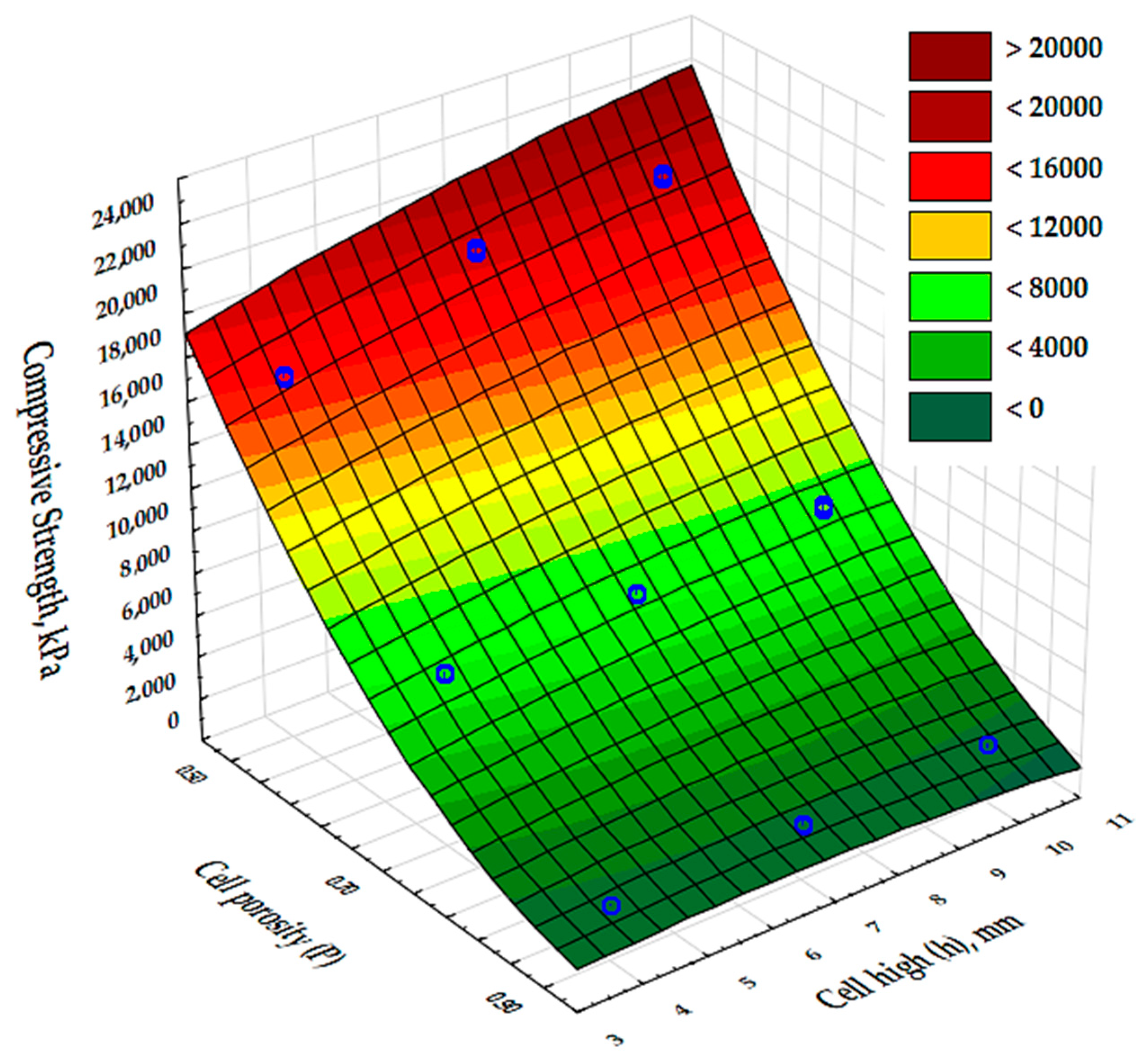
3.2. Compressive Strength
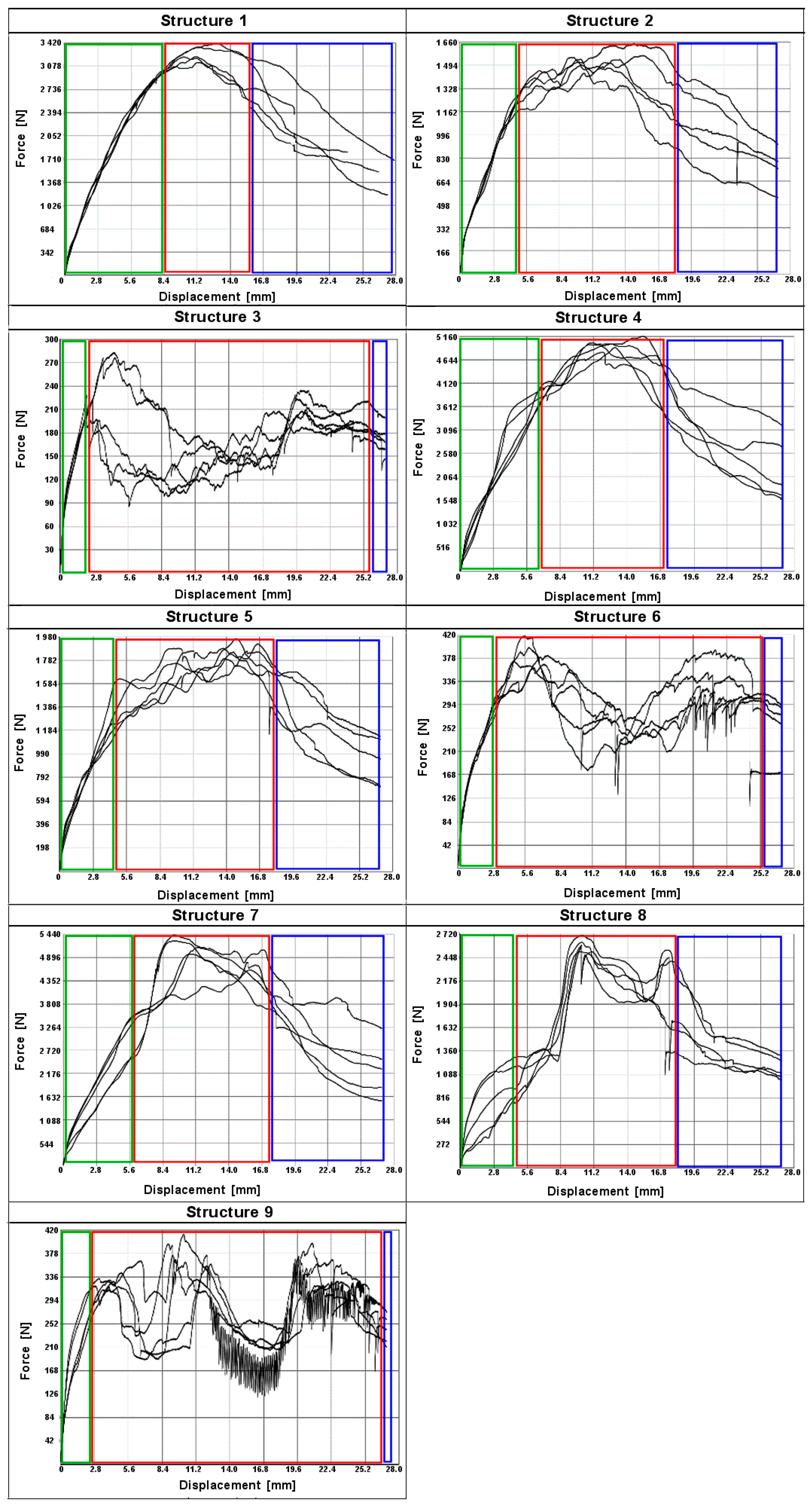
3.3. Quasi-Static Indentation Strength
4. Conclusions
- -
- The two-factor ANOVA analysis of variance showed a significant effect of porosity (P) and cell height (h) in the inner core of the composite on the thermal conductivity coefficient (λ), thermal resistance (R), compressive strength (CS) and punch shear strength (PSS);
- -
- The input variable composite porosity (P) was shown to be highly dominant over the other input factors;
- -
- The values of the thermal conductivity coefficient (λ) of all the tested samples were between 0.037 and 0.052 W/(m·K), which is lower than the maximum value (0.065 W/(m·K)) required for insulating materials according to PN-EN 2020-12 [46]. This means that the obtained composites meet the thermal efficiency standards;
- -
- The best insulation results were obtained for single-layer composites with a cell height of 4 mm and a porosity of 90%, where the coefficient of thermal conductivity was 0.038 W/(m-K) and the thermal resistance was 0.537 (m2·K)/W;
- -
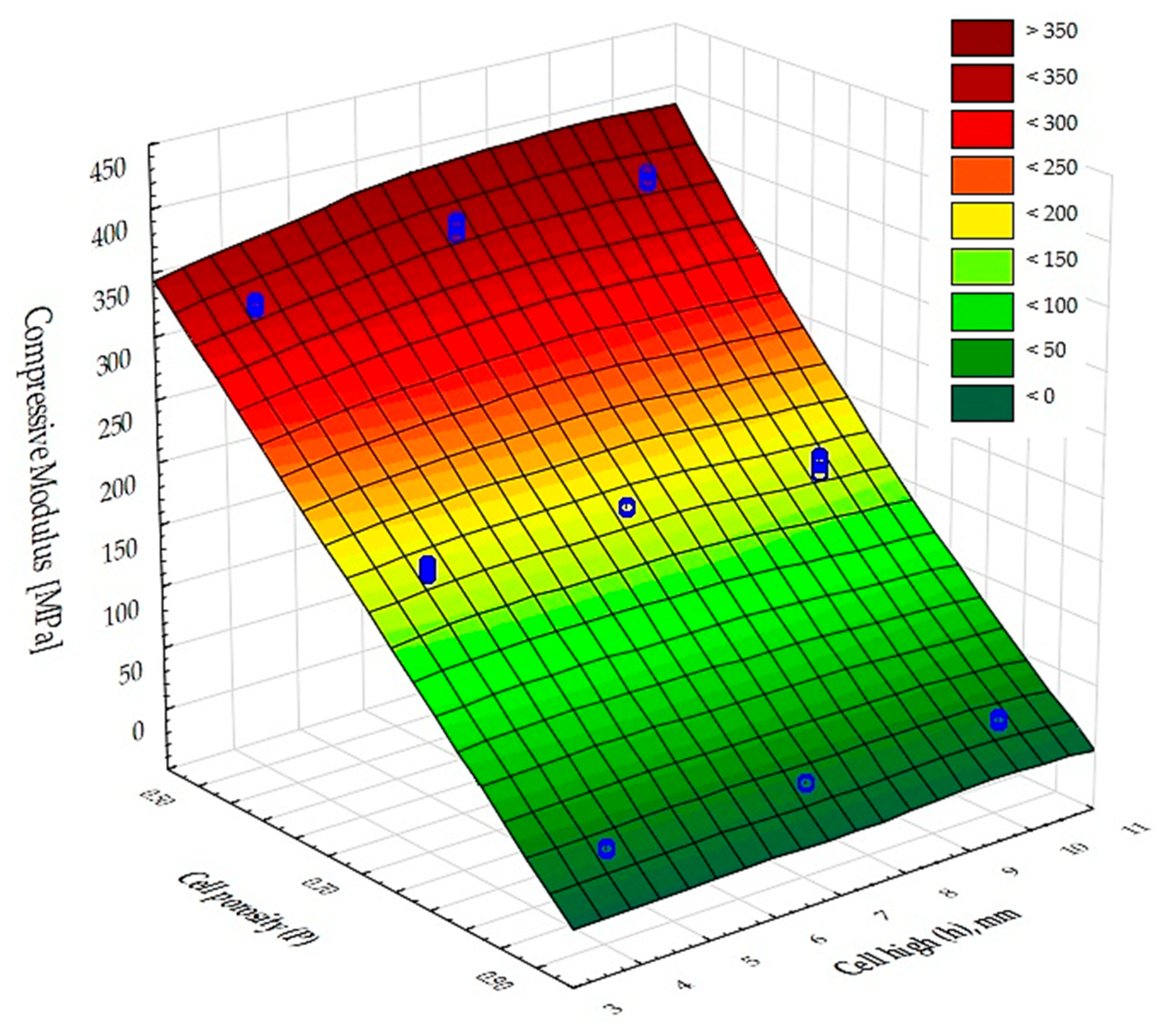
- The density-dependent compressive moduli for samples 1, 4, and 7 (P = 50%) and 2, 5, and 8 (P = 70%) are comparable and differ by only about 25%;
- -
- The compressive moduli for samples 1, 4, and 7 are almost the same at about 350 MPa, while the moduli for samples 2, 5, and 8 are twice as low at 175 MPa, and samples 3, 6, and 9 (P = 90%) have a modulus of about 26 MPa;
- -
- The size of the cells in the fabricated structure has a significant effect on the puncture resistance value. The highest puncture resistance value of 9 MPa was recorded for sample 7, a slightly lower PSS value of 8.8 MPa was recorded for sample 4, while sample 1 had a PSS value of approximately 5.7 MPa;
- -
- The porosity (P) of the composite structure has a significant effect on the puncture resistance. The lowest values were obtained for samples with 90% porosity; the value for all three samples, 3, 6, 9, did not exceed 1 MPa.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- International Energy Agency (IEA). Renewables 2023: Analysis and Forecast to 2028; IEA: Paris, France, 2024.
- Androniceanu, A.; Georgescu, I. The Impact of CO2 Emissions and Energy Consumption on Economic Growth: A Panel Data Analysis. Energies 2023, 16, 1342. [Google Scholar] [CrossRef]
- Malik, A.; Ul Haq, M.I.; Raina, A.; Gupta, K. 3D Printing towards Implementing Industry 4.0: Sustainability Aspects, Barriers and Challenges. Ind. Robot 2022, 49, 491–511. [Google Scholar] [CrossRef]
- Hu, F.; Wu, S.; Sun, Y. Hollow-Structured Materials for Thermal Insulation. Adv. Mater. 2019, 31, 1801001. [Google Scholar] [CrossRef] [PubMed]
- Zakari, A.; Khan, I.; Tan, D.; Alvarado, R.; Dagar, V. Energy Efficiency and Sustainable Development Goals (SDGs). Energy 2022, 239, 122365. [Google Scholar] [CrossRef]
- Santoni, A.; Bonfiglio, P.; Fausti, P.; Marescotti, C.; Mazzanti, V.; Mollica, F.; Pompoli, F. Improving the Sound Absorption Performance of Sustainable Thermal Insulation Materials: Natural Hemp Fibres. Appl. Acoust. 2019, 150, 279–289. [Google Scholar] [CrossRef]
- Javaid, M.; Haleem, A.; Singh, R.P.; Suman, R.; Rab, S. Role of Additive Manufacturing Applications towards Environmental Sustainability. Adv. Ind. Eng. Polym. Res. 2021, 4, 312–322. [Google Scholar] [CrossRef]
- Dodziuk, H. Druk 3D/AM. Zastosowania Oraz Skutki Społeczne i Gospodarcze; Wydawnictwo Naukowe PWN: Warszawa, Poland, 2019. [Google Scholar]
- Delgado Camacho, D.; Clayton, P.; O’Brien, W.J.; Seepersad, C.; Juenger, M.; Ferron, R.; Salamone, S. Applications of Additive Manufacturing in the Construction Industry—A Forward-Looking Review. Autom. Constr. 2018, 89, 110–119. [Google Scholar] [CrossRef]
- Sola, A.; Rosa, R.; Ferrari, A.M. Environmental Impact of Fused Filament Fabrication: What Is Known from Life Cycle Assessment? Polymers 2024, 16, 1986. [Google Scholar] [CrossRef]
- Babagowda; Kadadevara Math, R.S.; Goutham, R.; Srinivas Prasad, K.R. Study of Effects on Mechanical Properties of PLA Filament Which Is Blended with Recycled PLA Materials. IOP Conf. Ser. Mater. Sci. Eng. 2018, 310, 012103. [Google Scholar] [CrossRef]
- Patti, A.; Acierno, S.; Cicala, G.; Zarrelli, M.; Acierno, D. Assessment of Recycled PLA-Based Filament for 3D Printing. Mater. Proc. 2021, 7, 16. [Google Scholar] [CrossRef]
- Shi, B.; Xie, L.; Ma, B.; Zhou, Z.; Xu, B.; Qu, L. Preparation and Properties of Highly Transparent SiO2 Aerogels for Thermal Insulation. Gels 2022, 8, 744. [Google Scholar] [CrossRef] [PubMed]
- Ghica, M.E.; Mandinga, J.G.S.; Linhares, T.; Almeida, C.M.R.; Durães, L. Improvement of the Mechanical Properties of Silica Aerogels for Thermal Insulation Applications through a Combination of Aramid Nanofibres and Microfibres. Gels 2023, 9, 535. [Google Scholar] [CrossRef]
- Ali, M.; Almuzaiqer, R.; Al-Salem, K.; Alshehri, H.; Nuhait, A.; Alabdullatif, A.; Almubayrik, A. New Eco-Friendly Thermal Insulation and Sound Absorption Composite Materials Derived from Waste Black Tea Bags and Date Palm Tree Surface Fibers. Polymers 2024, 16, 2989. [Google Scholar] [CrossRef] [PubMed]
- Malet-Damour, B.; Habas, J.-P.; Bigot, D. Is Loose-Fill Plastic Waste an Opportunity for Thermal Insulation in Cold and Humid Tropical Climates? Sustainability 2023, 15, 9483. [Google Scholar] [CrossRef]
- Marín-Calvo, N.; González-Serrud, S.; James-Rivas, A. Thermal Insulation Material Produced from Recycled Materials for Building Applications: Cellulose and Rice Husk-Based Material. Front. Built Environ. 2023, 9, 1271317. [Google Scholar] [CrossRef]
- Rojas, C.; Cea, M.; Iriarte, A.; Valdés, G.; Navia, R.; Cárdenas-R, J.P. Thermal Insulation Materials Based on Agricultural Residual Wheat Straw and Corn Husk Biomass, for Application in Sustainable Buildings. Sustain. Mater. Technol. 2019, 20, e00102. [Google Scholar] [CrossRef]
- Zhang, Y.; Sun, X.; Medina, M.A. Thermal Performance of Concrete Masonry Units Containing Insulation and Phase Change Material. J. Build. Eng. 2023, 76, 107184. [Google Scholar] [CrossRef]
- Geng, L.; Wang, J.; Yang, X.; Jiang, J.; Li, R.; Yan, Y.; Zhao, J.; Liu, C. Synergistic Enhancement of Phase Change Materials through Three-Dimensional Porous Layered Covalent Triazine Framework/Expanded Graphite Composites for Solar Energy Storage and Beyond. Chem. Eng. J. 2024, 487, 150749. [Google Scholar] [CrossRef]
- Geng, L.; Xiao, T.; Jiang, J.; Luo, K.; Yan, Y.; Liu, C. Wide Temperature Range Phase Change Cold Energy Storage by Using Esterification between Polyethylene Glycol and Lauric Acid. Chem. Eng. J. 2024, 496, 154005. [Google Scholar] [CrossRef]
- Rathore, P.K.S.; Patel, B.; Gupta, M.K.; Sikarwar, B.S.; Sharma, R.K. Experimental Analysis of Thermal Energy Efficient Clay Brick Incorporated with Phase Change Material and Insulation. Process Saf. Environ. Prot. 2024, 190, 529–541. [Google Scholar] [CrossRef]
- Gama, N.; Ferreira, A.; Barros-Timmons, A. 3D Printed Cork/Polyurethane Composite Foams. Mater. Des. 2019, 179, 107905. [Google Scholar] [CrossRef]
- Islam, S.; Bhat, G.; Sikdar, P. Thermal and Acoustic Performance Evaluation of 3D-Printable PLA Materials. J. Build. Eng. 2023, 67, 105979. [Google Scholar] [CrossRef]
- de Rubeis, T. 3D-Printed Blocks: Thermal Performance Analysis and Opportunities for Insulating Materials. Sustainability 2022, 14, 1077. [Google Scholar] [CrossRef]
- de Rubeis, T.; Ciccozzi, A.; Giusti, L.; Ambrosini, D. The 3D Printing Potential for Heat Flow Optimization: Influence of Block Geometries on Heat Transfer Processes. Sustainability 2022, 14, 15830. [Google Scholar] [CrossRef]
- de Rubeis, T.; Ciccozzi, A.; Pasqualoni, G.; Paoletti, D.; Ambrosini, D. On the Use of Waste Materials for Thermal Improvement of 3D-Printed Block—An Experimental Comparison. Buildings 2023, 13, 1136. [Google Scholar] [CrossRef]
- Gladysz, G.M.; Chawla, K.K. Voids in Materials: From Unavoidable Defects to Designed Cellular Materials. MRS Bull. 2015, 40, 371. [Google Scholar] [CrossRef][Green Version]
- Șova, D.; Stanciu, M.D.; Georgescu, S.V. Design of Thermal Insulation Materials with Different Geometries of Channels. Polymers 2021, 13, 2217. [Google Scholar] [CrossRef] [PubMed]
- Calati, M.; De Monte, E.; Mancin, S. Numerical Analysis of the Effects of the Structure Shape and Orientation of Kelvin Cell Porous Structures during Air Forced Convection. Appl. Sci. 2021, 11, 6189. [Google Scholar] [CrossRef]
- Pavon, C.; Aldas, M.; Samper, M.D.; Motoc, D.L.; Ferrandiz, S.; López-Martínez, J. Mechanical, Dynamic-Mechanical, Thermal and Decomposition Behavior of 3D-Printed PLA Reinforced with CaCO3 Fillers from Natural Resources. Polymers 2022, 14, 2646. [Google Scholar] [CrossRef]
- Gnanamani Sankaravel, S.; Syed, R.B.; Manivachakan, V. In Vitro and Mechanical Characterization of PLA/Egg Shell Biocomposite Scaffold Manufactured Using Fused Deposition Modeling Technology for Tissue Engineering Applications. Polym. Compos. 2022, 43, 173–186. [Google Scholar] [CrossRef]
- Joseph, T.M.; Kallingal, A.; Suresh, A.M.; Mahapatra, D.K.; Hasanin, M.S.; Haponiuk, J.; Thomas, S. 3D Printing of Polylactic Acid: Recent Advances and Opportunities. Int. J. Adv. Manuf. Technol. 2023, 125, 1015–1035. [Google Scholar] [CrossRef] [PubMed]
- Rodríguez-Panes, A.; Claver, J.; Camacho, A.M. The Influence of Manufacturing Parameters on the Mechanical Behaviour of PLA and ABS Pieces Manufactured by FDM: A Comparative Analysis. Materials 2018, 11, 1333. [Google Scholar] [CrossRef] [PubMed]
- EN ISO 9869-1:2014; Thermal Insulation—Building Elements—In Situ Measurement of Thermal Resistance and Thermal Transmittance. Part 1: Heat Flow Meter Method. International Organization for Standardization: Geneva, Switzerland, 2014.
- Anwajler, B.; Szulc, P. The Impact of 3D Printing Technology on the Improvement of External Wall Thermal Efficiency—An Experimental Study. J. Compos. Sci. 2024, 8, 389. [Google Scholar] [CrossRef]
- Anwajler, B.; Szołomicki, J.; Noszczyk, P.; Baryś, M. The Potential of 3D Printing in Thermal Insulating Composite Materials—Experimental Determination of the Impact of the Geometry on Thermal Resistance. Materials 2024, 17, 1202. [Google Scholar] [CrossRef] [PubMed]
- Anwajler, B. Modern Insulation Materials for Sustainability Based on Natural Fibers: Experimental Characterization of Thermal Properties. Fibers 2024, 12, 76. [Google Scholar] [CrossRef]
- PN-EN ISO 604:2006; Tworzywa Sztuczne—Oznaczanie Właściwości Przy Ściskaniu. Polski Komitet Normalizacyjny: Warszawa, Poland, 2006.
- ASTM D732-17; Test Method for Shear Strength of Plastics by Punch Tool. ASTM: West Conshohocken, PA, USA, 2017.
- Piwowar, A.; Anwajler, B.; Szulc, P. Właściwości Cieplne Materiałów Izolacyjnych Wykonanych w Technologii Druku 3D—Wpływ Optymalizacji Struktury Opartej Na Modelu Piany Kelvina. Rynek Energii 2024, 1, 60–68. [Google Scholar]
- Anwajler, B.; Piwowar, A. Bioniczny Kompozyt Komórkowy o Właściwościach Izolacyjnych Wykonany w Technologii Addytywnej SLS. Izolacje 2023, 28, 116–123. [Google Scholar]
- Barzgar Torghabeh, A.; Barzgar Torghabeh, I.; Kafaee Razavi, M. 3D Printed PLA Porous Scaffolds with Engineered Cell Size and Porosity Promote the Effectiveness of the Kelvin Model for Bone Tissue Engineering. Macromol. Mater. Eng. 2024, 12, 2400212. [Google Scholar] [CrossRef]
- Park, K.-M.; Kim, G.-O.; Kim, J.-G.; Roh, Y. Mechanical Properties of Additive Manufactured Variable-Density Kelvin Lattice Structures: A Novel Design Method for Kelvin Unit Cells. J. Struct. Integr. Maint. 2022, 7, 34–45. [Google Scholar] [CrossRef]
- Daya Karthic, R.; Joy, J.; Sakthivel, G.; Nadimpalli, R. Mechanical Characterization of 3D-Printed Kelvin Cell with Varying Infill Densities. Mater. Today Proc. 2023, 84, 41–46. [Google Scholar] [CrossRef]
- PN-EN ISO 9229:2020-12; Izolacja Cieplna—Materiały, Wyroby i Systemy—Terminologia. SBD, Sektor Budownictwa i Konstrukcji Budowlanych, KT 211, Wyrobów do Izolacji Cieplnej w Budownictwie. Polski Komitet Normalizacyjny: Warszawa, Poland, 2020.
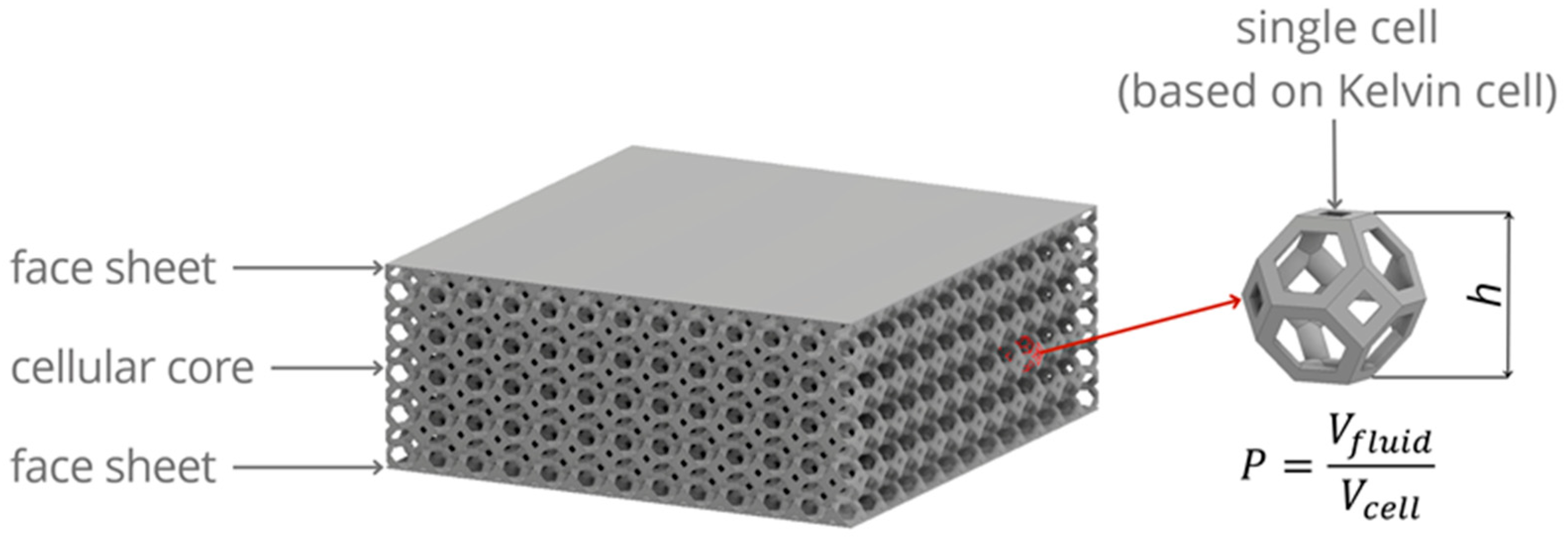








| Coded Levels | Cell Porosity P | Cell Height h, mm |
|---|---|---|
| −1 | 0.50 | 4 |
| 0 | 0.70 | 7 |
| 1 | 0.90 | 10 |
| Experiment Code | Cell Porosity P | Cell Height h, mm |
|---|---|---|
| 1 | 0.50 | 4 |
| 2 | 0.70 | 4 |
| 3 | 0.90 | 4 |
| 4 | 0.50 | 7 |
| 5 | 0.70 | 7 |
| 6 | 0.90 | 7 |
| 7 | 0.50 | 10 |
| 8 | 0.70 | 10 |
| 9 | 0.90 | 10 |
| Parameter | Value |
|---|---|
| Nozzle diameter | 0.4 mm |
| Layer thickness | 0.16 mm |
| Extrusion temperature | 230 °C |
| Print speed | Up to 40 mm/s |
| Infill density | 100% |
| Nozzle diameter | 0.4 mm |
| Variability | Valid N | M | Me | Min | Max | SD |
|---|---|---|---|---|---|---|
| λ, W/m·K | 27 | 0.044 | 0.043 | 0.037 | 0.052 | 0.005 |
| R, K·m2/W | 27 | 0.463 | 0.459 | 0.399 | 0.537 | 0.048 |
| Variability | Valid N | M | Me | Min | Max | SD |
|---|---|---|---|---|---|---|
| CS, kPa | 45 | 8075 | 6380 | 577 | 18,400 | 6914 |
| PSS, MPa | 45 | 3.9 | 3.3 | 0.4 | 9.6 | 3.2 |
| Symbol That Identifies the Input Factors | df | SS | MS | F | p |
|---|---|---|---|---|---|
| λ, W/(m·K) | |||||
| absolute term | 1 | 0.052 | 0.052 | 53,581.2 | 0.000 |
| P | 2 | 0.0005 | 0.00024 | 247.9 | 0.000 |
| h | 2 | 0.0001 | 0.00005 | 48.95 | 0.000 |
| error | 22 | 0.00002 | 0.000001 | ||
| general | 26 | 0.0006 | |||
| R, (m2·K)/W | |||||
| absolute term | 1 | 5.7912 | 5.79116 | 69,336.75 | 0.000 |
| P | 2 | 0.05153 | 0.0258 | 308.49 | 0.000 |
| h | 2 | 0.00768 | 0.0038 | 45.99 | 0.000 |
| error | 22 | 0.00184 | 0.0001 | ||
| general | 26 | 0.06105 | |||
| CS, GPa | |||||
| absolute term | 1 | 2934.06 | 2934.06 | 0.007 | 0.000 |
| P | 2 | 2068.103 | 1034.05 | 0.0023 | 0.000 |
| h | 2 | 17.865 | 8.93 | 2.064 × 10−5 | 0.000 |
| error | 40 | 17.307 | 0.432 | ||
| general | 44 | 2103.276 | |||
| PSS, MPa | |||||
| absolute term | 1 | 717.86 | 717.86 | 1469.1 | 0.000 |
| P | 2 | 395.21 | 197.60 | 404.4 | 0.000 |
| h | 2 | 26.05 | 13.02 | 26.6 | 0.000 |
| error | 40 | 19.55 | 0.49 | ||
| general | 44 | 440.80 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anwajler, B.; Iwko, J.; Piwowar, A.; Wróblewski, R.; Szulc, P. Influence of Structural Optimization on the Physical Properties of an Innovative FDM 3D Printed Thermal Barrier. Materials 2024, 17, 6293. https://doi.org/10.3390/ma17246293
Anwajler B, Iwko J, Piwowar A, Wróblewski R, Szulc P. Influence of Structural Optimization on the Physical Properties of an Innovative FDM 3D Printed Thermal Barrier. Materials. 2024; 17(24):6293. https://doi.org/10.3390/ma17246293
Chicago/Turabian StyleAnwajler, Beata, Jacek Iwko, Anna Piwowar, Roman Wróblewski, and Piotr Szulc. 2024. "Influence of Structural Optimization on the Physical Properties of an Innovative FDM 3D Printed Thermal Barrier" Materials 17, no. 24: 6293. https://doi.org/10.3390/ma17246293
APA StyleAnwajler, B., Iwko, J., Piwowar, A., Wróblewski, R., & Szulc, P. (2024). Influence of Structural Optimization on the Physical Properties of an Innovative FDM 3D Printed Thermal Barrier. Materials, 17(24), 6293. https://doi.org/10.3390/ma17246293