Effect of Grain Size and Porosity/Binder Index on the Unconfined Compressive Strength, Stiffness and Microstructure of Cemented Colombian Sands
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
| Property | Standard/Reference | Unit | Value | |||
|---|---|---|---|---|---|---|
| LOR | LUR | MED | BOG | |||
| Maximum γd | [29] | kN/m3 | 1.56 | 1.78 | 2.02 | 1.70 |
| Minimum γd | [30] | kN/m3 | 1.37 | 1.52 | 1.63 | 1.55 |
| Specific Gravity, Gs | [31] | - | 2.73 | 2.73 | 2.83 | 2.69 |
| Gravel (4.75–76.2 mm) | [32] | % | 0 | 10.8 | 13.6 | 0 |
| Coarse Sand (2.00–4.75 mm) | [32] | % | 0 | 20.1 | 26.3 | 29.5 |
| Medium Sand (0.425–2.0 mm) | [32] | % | 5.1 | 42.3 | 35.5 | 69.4 |
| Fine Sand (0.075–0.425 mm) | [32] | % | 94.1 | 26.6 | 22.1 | 1.0 |
| Silt (0.002–0.075 mm) | [32] | % | 0.8 | 0.2 | 2.5 | 0.1 |
| Mean Diameter (d50) | [32] | mm | 0.24 | 0.85 | 1.38 | 1.75 |
| Effective Diameter (d10) | [32] | mm | 0.16 | 0.20 | 0.13 | 1.23 |
| Uniformity Coefficient Cu | [32] | - | 1.6 | 5.9 | 14.6 | 1.4 |
| Coefficient of Curvature Cc | [32] | - | 1.0 | 0.7 | 1.0 | 0.9 |
| USCS Classification | [32] | - | SP | SP | SW | SP |
| Color | Munsell Chart | - | Dark greenish gray | Olive | Greenish gray | Pale yellow |
2.2. Methods
2.2.1. Specimen Molding and Preparation
2.2.2. USC and Stiffness (Non-Destructive) Program: Effects of Porosity/Cement Ratio
2.2.3. Microstructure and Microanalysis of Artificially Cemented Soil
3. Results and Discussions
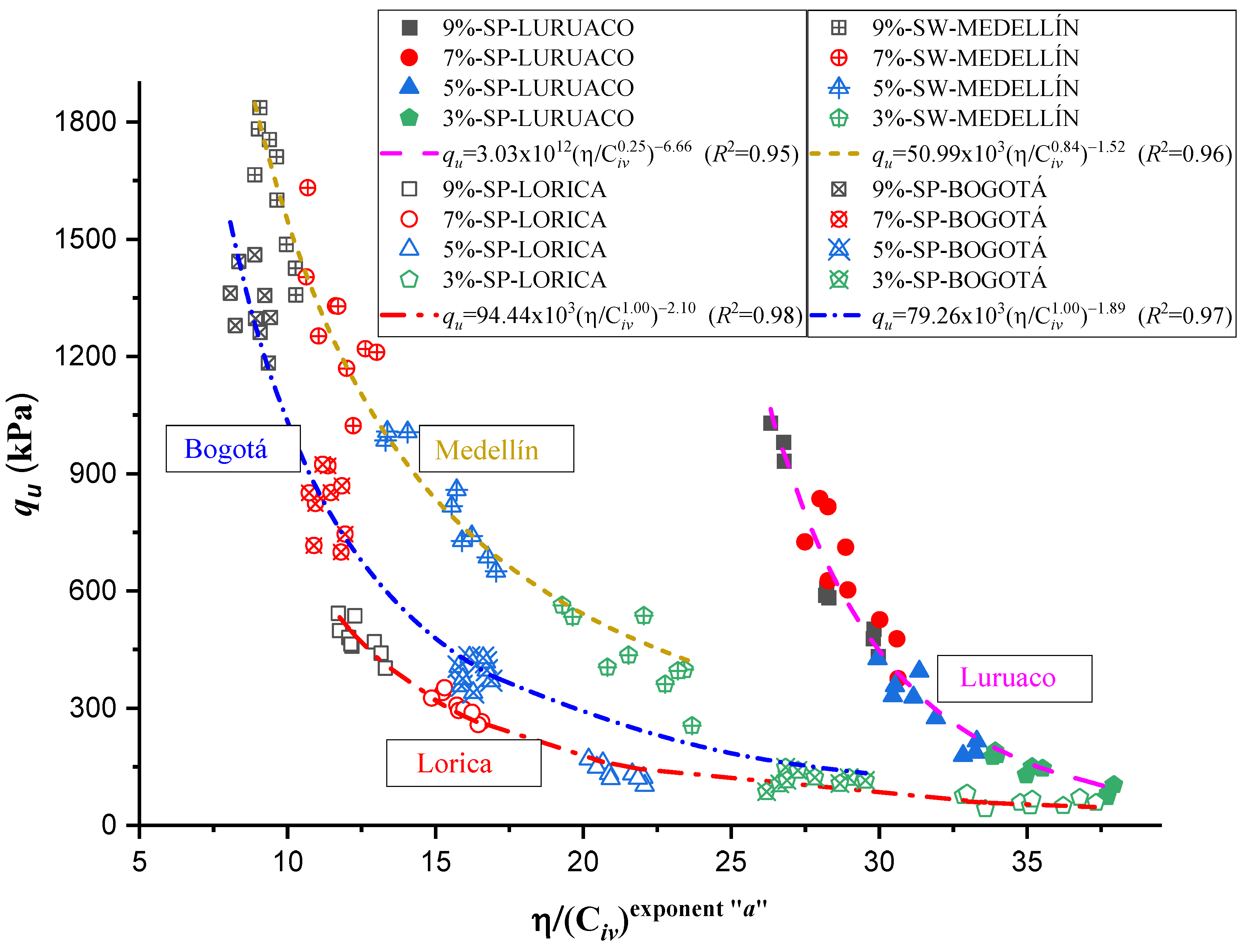
3.1. Effects of Porosity/Cement Content Ratio on the Strength of Soil-Cement Mixtures
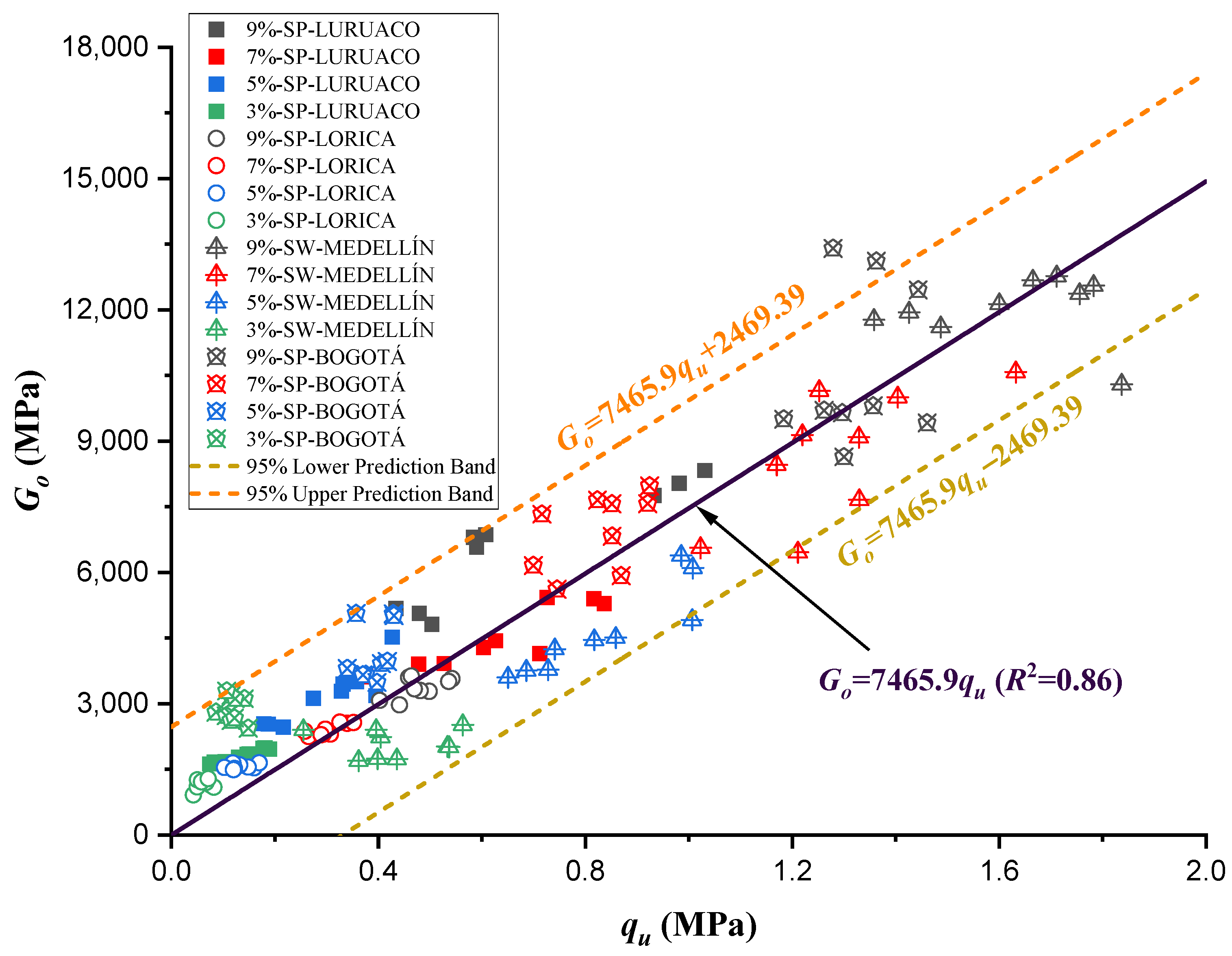
3.2. Effects of Porosity/Cement Content Ratio on the Stiffness of SoilCement Mixtures
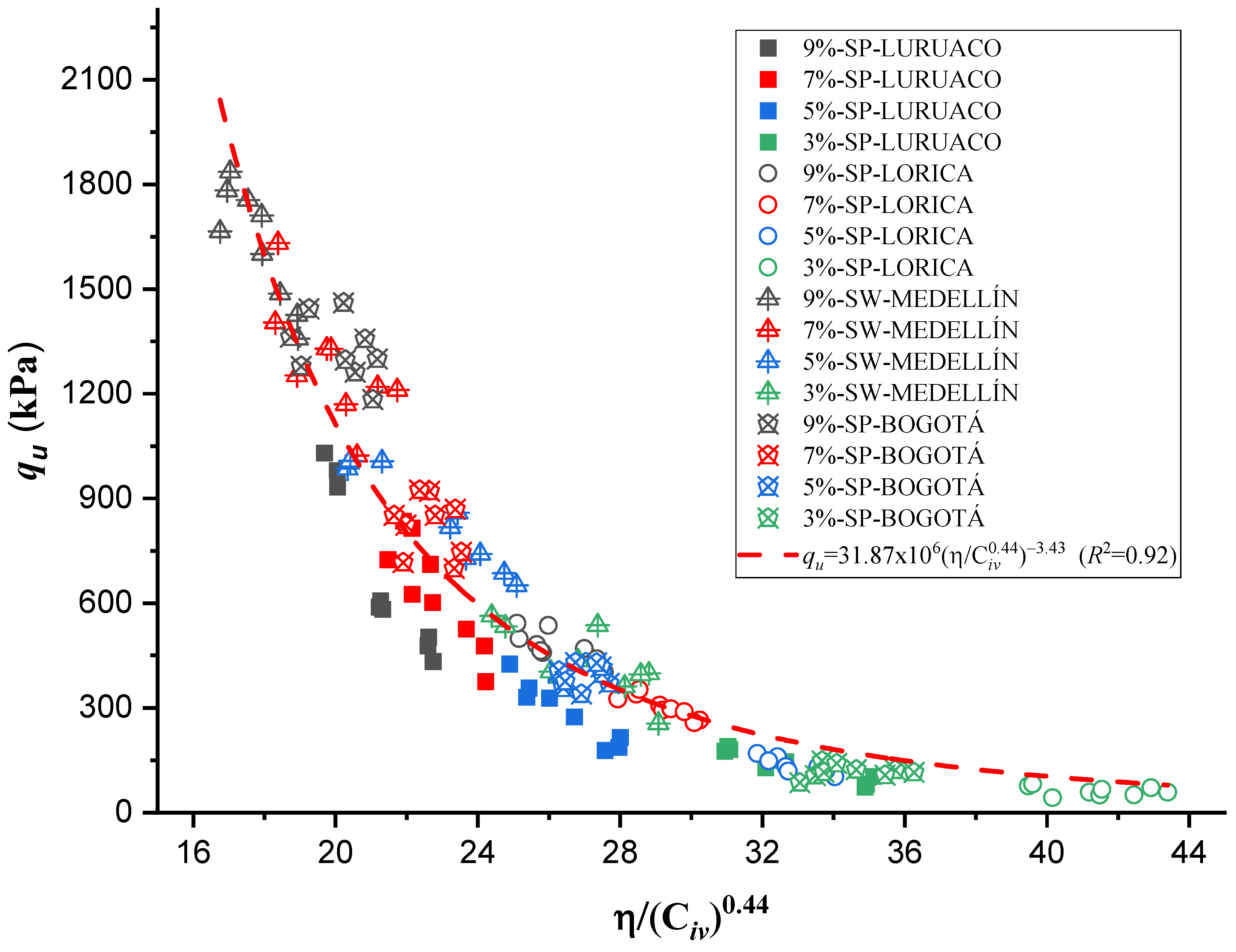
3.3. Normalization of Unconfined Compressive Strength and Initial Stiffness at Small Deformations
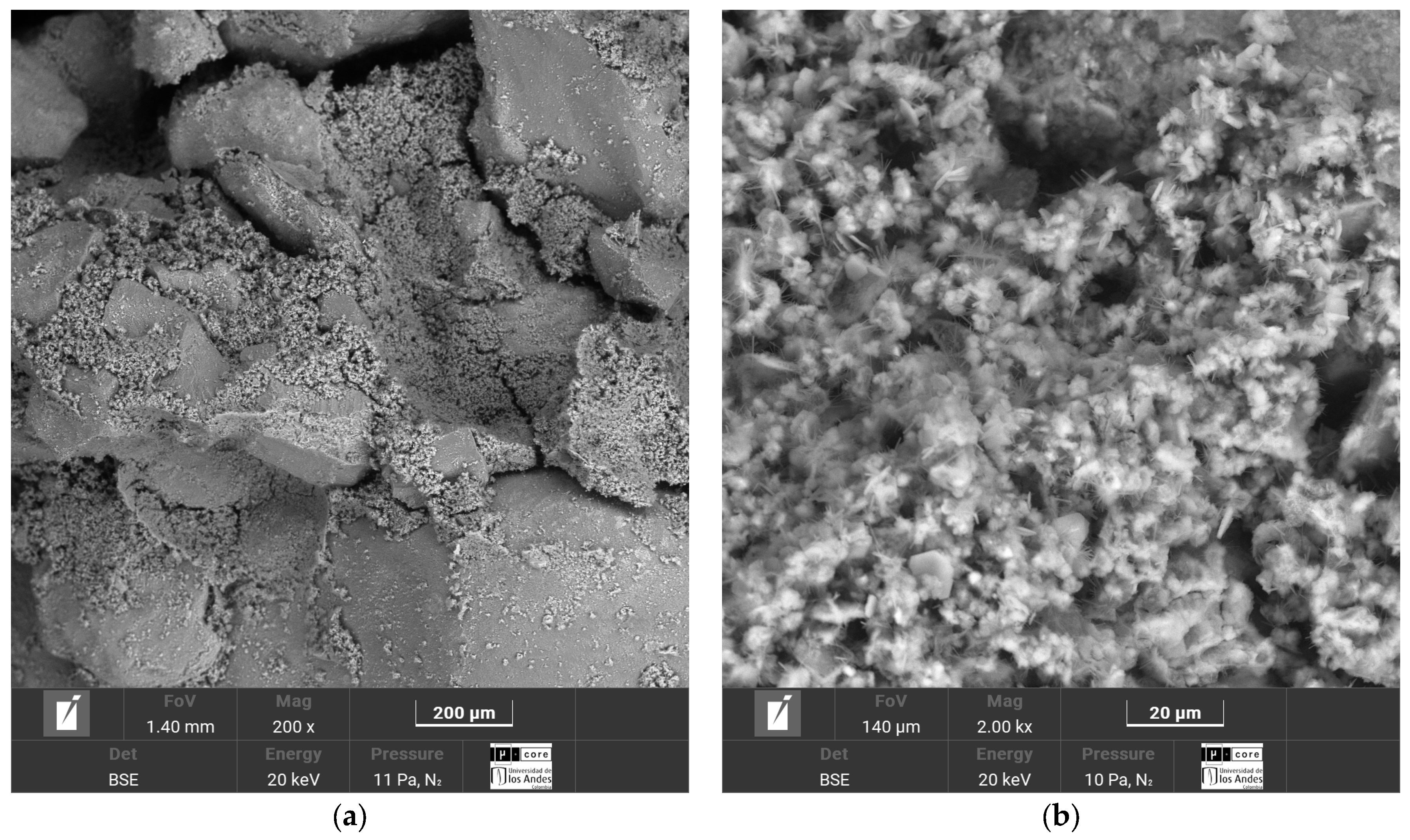
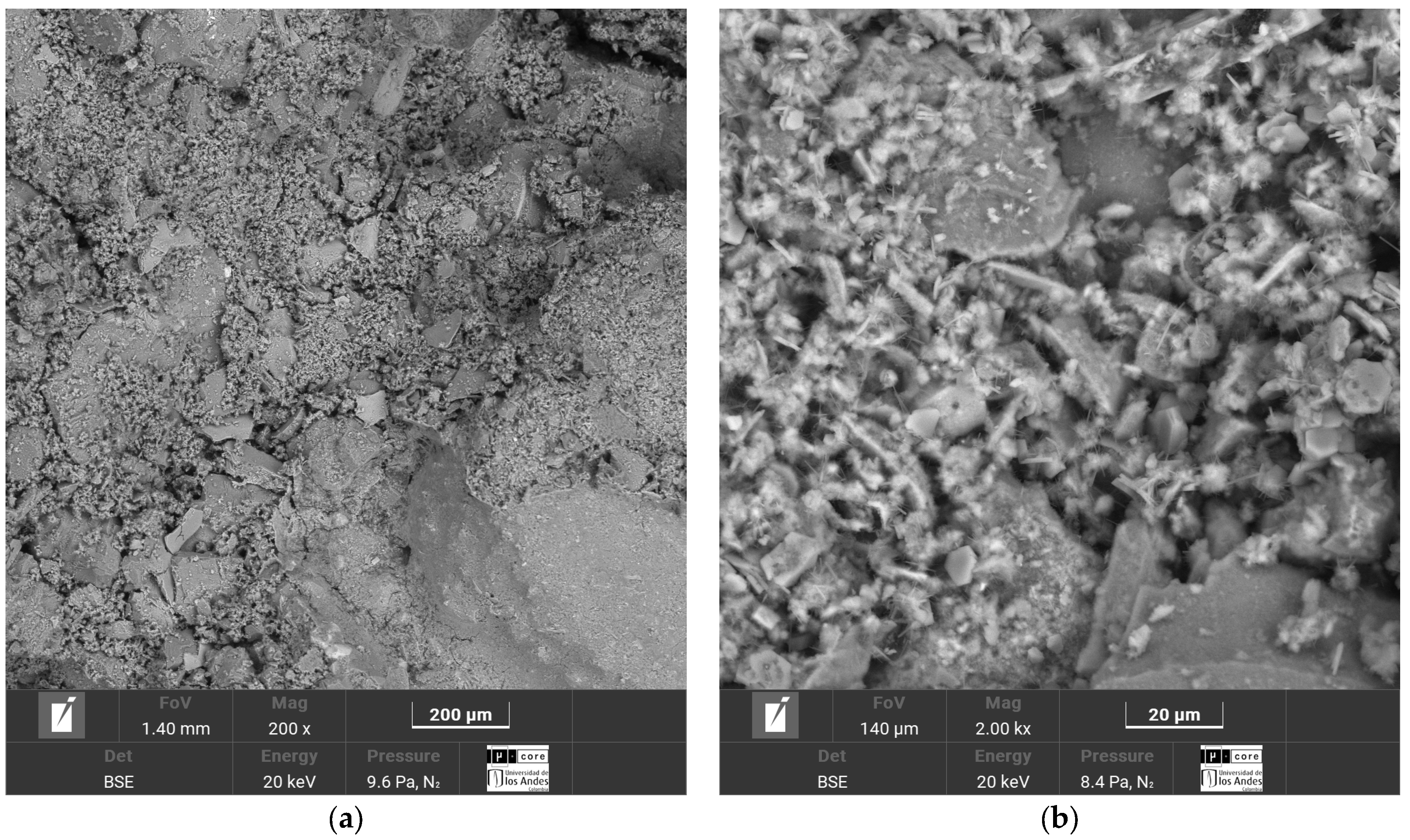
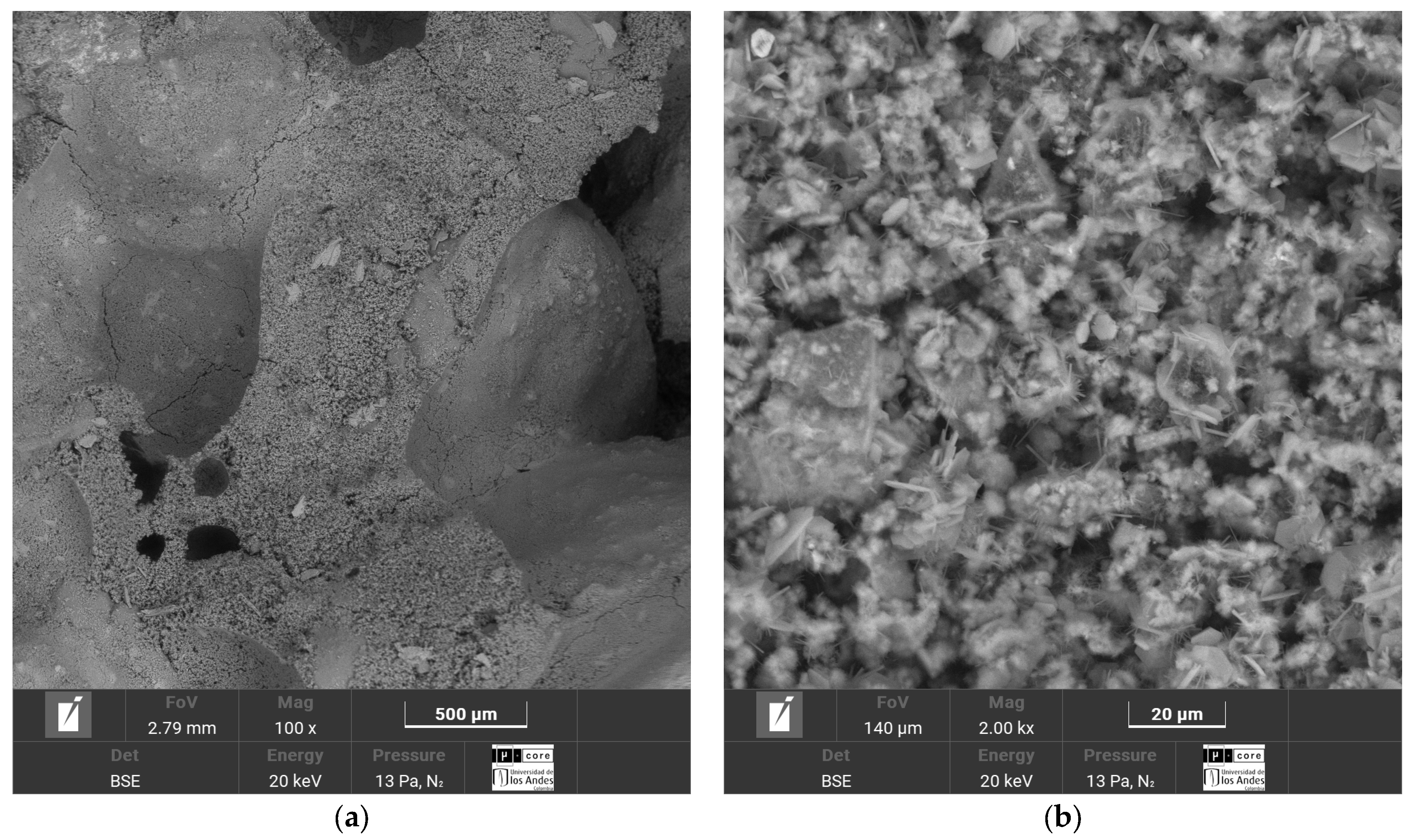
3.4. Microstructure and Microanalysis of Artificially Cemented Mixtures
3.5. Influence of Grain Size and Mineralogy on the Porosity/Cement Ratio
4. Conclusions
- The parameter “a” was the determinant in obtaining a good correlation coefficient (R2) in the four sands studied. The fits were satisfactory for the unconfined compressive strength () and the initial stiffness at small deformations (). The equations obtained allow engineers to calculate the cement content and porosity required to achieve the desired mechanical properties in geotechnical engineering projects. Remarkably, a standard equation was obtained for all sands, using an “a” value of 0.44, showing a better fit than the normalization of individual equations (Figure 7, Figure 9, Figure 11, and Figure 12). This result facilitates the unification of criteria for similar soils in future projects, although its applicability should be verified in a broader range of sands.
- The sands’ grain size distribution impacted the parameter “a” adjustment. In Bogotá and Lorica, the “a” value was 1.00, which coincides with the behavior described in the literature for sands with a low coefficient of uniformity () (1.4 and 1.6, respectively). However, mineralogy and particle shape are predominant factors in the Medellín and Luruaco sands, where Cu is higher. The Medellín sand, with more angular particles (Figure 4), presented a higher “a” value, while the Luruaco sand, with more rounded particles (Figure 3), showed a lower “a” value. This suggests an inverse relationship between particle angularity and “a” value, but further studies on other types of sands are needed to corroborate this hypothesis.
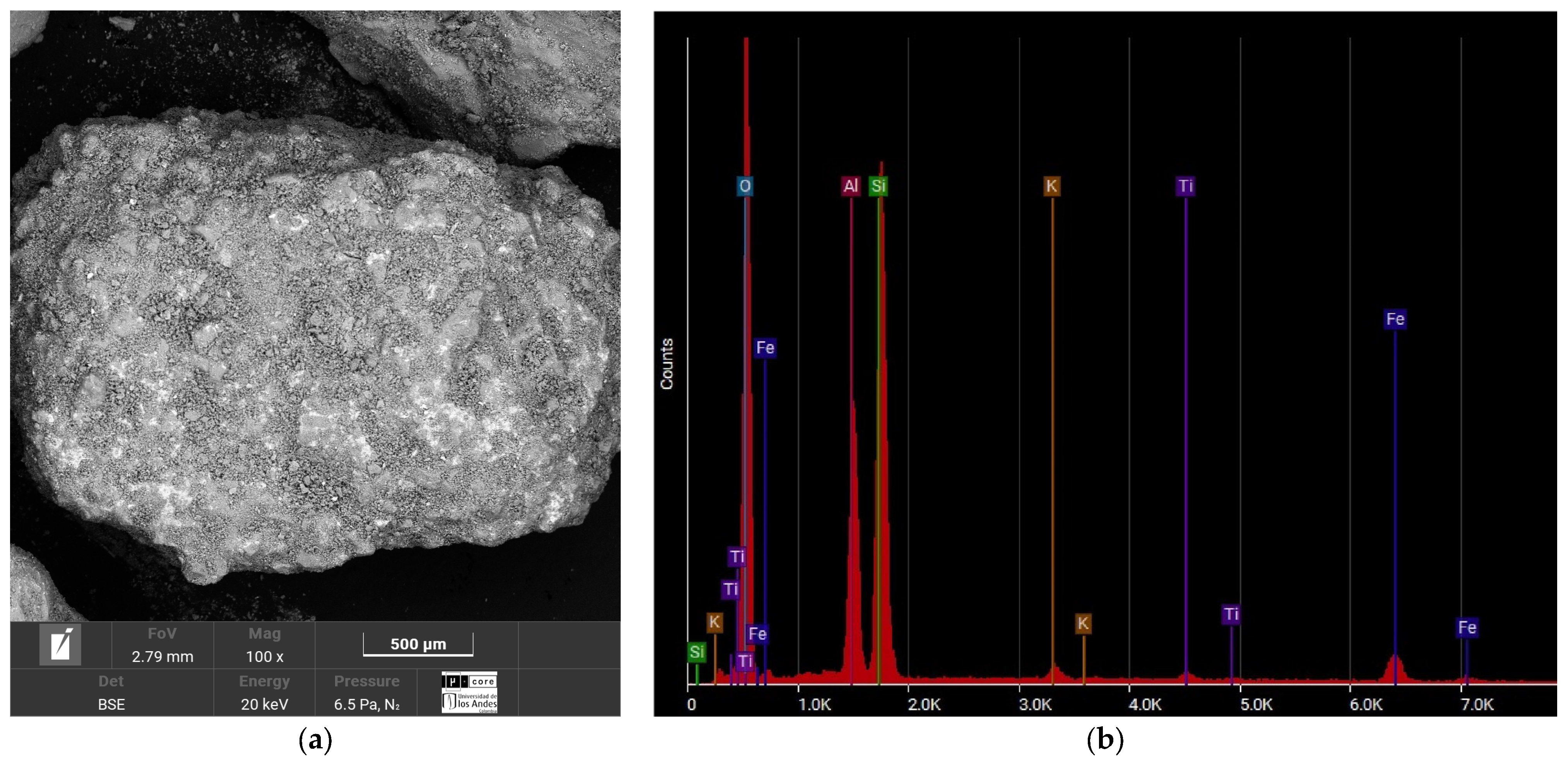
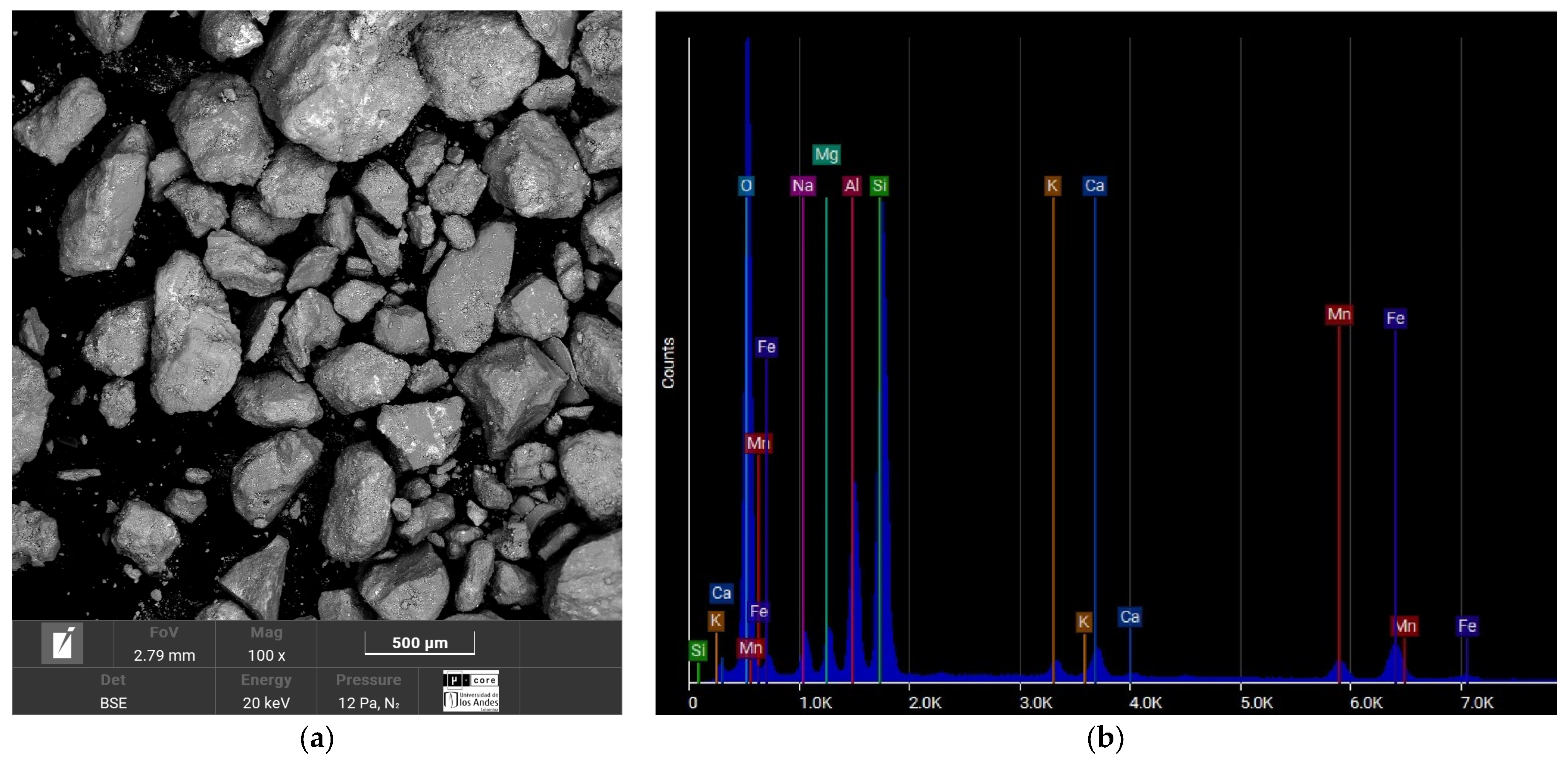
- In all the sands, the cementitious matrix, with a cement content of 5%, maximum density, and 7 days of curing, presented minerals such as Ettringite and hydrated calcium silicate (C-S-H), responsible for improved mechanical strength. However, the Medellín sand showed a better distribution of the soil–cement matrix and a more significant reduction of voids (Figure 15), which explains its higher strength values. On the other hand, the Lorica sand presented a matrix with a greater presence of voids (Figure 16), which resulted in lower strength values. This behavior suggests that, in addition to the mineral composition, the distribution of voids is crucial for the performance of the soil–cement mixture. The differences observed in the microstructure of the sands point to the need to carefully evaluate the interaction between soil and cement for each specific case.
- In technical aspects, the equations generated from the research results will serve as a tool for professionals responsible for soil stabilization. With these equations, it will be possible to determine a value for the density and cement content of soils from the open-pit mines involved in the study, allowing the required strength to be achieved depending on the structure to be built on them. Additionally, applying the optimal cement content will reduce costs in soil–cement design and, furthermore, help decrease the carbon footprint by using the minimum amount necessary to meet the specifications of any civil engineering project.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Diambra, A.; Ibraim, E.; Peccin, A.; Consoli, N.C.; Festugato, L. Theoretical Derivation of Artificially Cemented Granular Soil Strength. J. Geotech. Geoenviron. Eng. 2017, 143, 04017003. [Google Scholar] [CrossRef]
- Haeri, S.M.; Hamidi, A.; Hosseini, S.M.; Asghari, E.; Toll, D.G. Effect of Cement Type on the Mechanical Behavior of a Gravely Sand. Geotech. Geol. Eng. 2006, 24, 335–360. [Google Scholar] [CrossRef]
- Diambra, A.; Festugato, L.; Ibraim, E.; Peccin da Silva, A.; Consoli, N.C. Modelling Tensile/Compressive Strength Ratio of Artificially Cemented Clean Sand. Soils Found. 2018, 58, 199–211. [Google Scholar] [CrossRef]
- Bastos, Í.J.F.S.; Filho, F.C.S.; Monteiro, F.F.; Soares, A.B. Proposal of a Failure Envelope for Artificially Cemented Sand. REM-Int. Eng. J. 2022, 75, 205–214. [Google Scholar] [CrossRef]
- Moreira, E.B.; Baldovino, J.A.; Rose, J.L.; Luis dos Santos Izzo, R. Effects of Porosity, Dry Unit Weight, Cement Content and Void/Cement Ratio on Unconfined Compressive Strength of Roof Tile Waste-Silty Soil Mixtures. J. Rock Mech. Geotech. Eng. 2019, 11, 369–378. [Google Scholar] [CrossRef]
- Mosavat, N.A. Review of Electrokinetic Treatment Technique for Improving the Engineering Characteristics of Low Permeable Problematic Soils. Int. J. Geomate 2012, 28, 266–272. [Google Scholar] [CrossRef]
- MolaAbasi, H.; Saberian, M.; Li, J. Prediction of Compressive and Tensile Strengths of Zeolite-Cemented Sand Using Porosity and Composition. Constr. Build. Mater. 2019, 202, 784–795. [Google Scholar] [CrossRef]
- Consoli, N.C.; da Silva, A.; Barcelos, A.M.; Festugato, L.; Favretto, F. Porosity/Cement Index Controlling Flexural Tensile Strength of Artificially Cemented Soils in Brazil. Geotech. Geol. Eng. 2020, 38, 713–722. [Google Scholar] [CrossRef]
- Consoli, N.C.; Cruz, R.C.; Floss, M.F.; Festugato, L. Parameters Controlling Tensile and Compressive Strength of Artificially Cemented Sand. J. Geotech. Geoenviron. Eng. 2010, 136, 759–763. [Google Scholar] [CrossRef]
- Corte, M.B.; Festugato, L.; Consoli, N.C.; Ibraim, E.; Diambra, A. Assessing the Mechanical Properties of a Cemented Sand Focusing on Experimental and Theoretical Studies. E3S Web Conf. 2024, 544, 11001. [Google Scholar] [CrossRef]
- Amini, Y.; Hamidi, A. Triaxial Shear Behavior of a Cement-Treated Sand–Gravel Mixture. J. Rock Mech. Geotech. Eng. 2014, 6, 455–465. [Google Scholar] [CrossRef]
- Horpibulsuk, S.; Rachan, R.; Chinkulkijniwat, A.; Raksachon, Y.; Suddeepong, A. Analysis of Strength Development in Cement-Stabilized Silty Clay from Microstructural Considerations. Constr. Build. Mater. 2010, 24, 2011–2021. [Google Scholar] [CrossRef]
- Li, D.; Liu, X.; Liu, X. Experimental Study on Artificial Cemented Sand Prepared with Ordinary Portland Cement with Different Contents. Materials 2015, 8, 3960–3974. [Google Scholar] [CrossRef] [PubMed]
- Consoli, N.C.; Festugato, L.; da Rocha, C.G.; Cruz, R.C. Key Parameters for Strength Control of Rammed Sand–Cement Mixtures: Influence of Types of Portland Cement. Constr. Build. Mater. 2013, 49, 591–597. [Google Scholar] [CrossRef]
- Consoli, N.C.; Viana da Fonseca, A.; Cruz, R.C.; Heineck, K.S. Fundamental Parameters for the Stiffness and Strength Control of Artificially Cemented Sand. J. Geotech. Geoenviron. Eng. 2009, 135, 1347–1353. [Google Scholar] [CrossRef]
- Consoli, N.C.; Foppa, D.; Festugato, L.; Heineck, K.S. Key Parameters for Strength Control of Artificially Cemented Soils. J. Geotech. Geoenviron. Eng. 2007, 133, 197–205. [Google Scholar] [CrossRef]
- Diambra, A.; Ibraim, E.; Festugato, L.; Corte, M.B. Stiffness of Artificially Cemented Sands: Insight on Characterisation through Empirical Power Relationships. Road Mater. Pavement Des. 2019, 22, 1469–1479. [Google Scholar] [CrossRef]
- Scheuermann Filho, H.C.; Dias Miguel, G.; Cesar Consoli, N. Porosity/Cement Index over a Wide Range of Porosities and Cement Contents. J. Mater. Civ. Eng. 2022, 34, 06021011. [Google Scholar] [CrossRef]
- Rios Silva, S.; Viana Da Fonseca, A.; Consoli, N.C. Strength and Stiffness Properties of Mixtures of Granitic Soil-Cement. In Proceedings of the 17th International Conference on Soil Mechanics and Geotechnical Engineering: The Academia and Practice of Geotechnical Engineering, Alexandria, Egypt, 5–9 October 2009; Volume 1. [Google Scholar]
- Consoli, N.C.; da Fonseca, A.V.; Silva, S.R.; Cruz, R.C.; Fonini, A. Parameters Controlling Stiffness and Strength of Artificially Cemented Soils. Geotechnique 2012, 62, 177–183. [Google Scholar] [CrossRef]
- Baldovino, J.d.J.A.; Moreira, E.B.; Carazzai, É.; Rocha, E.V.d.G.; dos Santos Izzo, R.; Mazer, W.; Rose, J.L. Equations Controlling the Strength of Sedimentary Silty Soil–Cement Blends: Influence of Voids/Cement Ratio and Types of Cement. Int. J. Geotech. Eng. 2021, 15, 359–372. [Google Scholar] [CrossRef]
- Scheuermann Filho, H.C.; Consoli, N.C. Effect of Porosity/Cement Index on Behavior of a Cemented Soil: The Role of Dosage Change. Geotech. Geol. Eng. 2024, 42, 351–368. [Google Scholar] [CrossRef]
- Zhang, X.; Hu, M.; Zuo, J.; Wang, Z.; Baudet, B.A.; Coop, M.R. Effect of grain size on ductility and failure mechanism of fiber-reinforced coral sand cement-based composites. J. Build. Eng. 2024, 92, 109733. [Google Scholar] [CrossRef]
- Zhang, X.; Baudet, B.A.; Yao, T. The influence of particle shape and mineralogy on the particle strength, breakage and compressibility. Int. J. Geo-Eng. 2020, 11, 1. [Google Scholar] [CrossRef]
- Consoli, N.C.; Scheuermann Filho, H.C.; Leon, H.B.; da Silva Carretta, M.; Corte, M.B.; Cordeiro, R.E.; Caballero, R.D.; Lourenço, D.E. General Relationships Controlling Loss of Mass, Stiffness and Strength of Sustainable Binders Amended Sand. Transp. Geotech. 2021, 27, 100473. [Google Scholar] [CrossRef]
- Ferrazzo, S.T.; de Araújo, M.T.; Consoli, N.C. Which solution is more sustainable: Waste foundry sand stabilized with alkali-activated binder or Portland cement? J. Build. Eng. 2024, 84, 108448. [Google Scholar] [CrossRef]
- ASTM C150; Standard Specification for Portland Cement. ASTM International: West Conshohocken, PA, USA, 2019.
- Consoli, N.C.; Arcari Bassani, M.A.; Festugato, L. Effect of Fiber-Reinforcement on the Strength of Cemented Soils. Geotext. Geomembr. 2010, 28, 344–351. [Google Scholar] [CrossRef]
- ASTM 4253-16; Standard Test Methods for Maximum Index Density and Unit Weight of Soils Using a Vibratory Table. ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM D4254; Standard Test Methods for Minimum Index Density and Unit Weight of Soils and Calculation of Relative Density. ASTM Standards: West Conshohocken, PA, USA, 2006.
- ASTM D854; Standard Test Methods for Specific Gravity of Soil Solids by Water Pycnometer. ASTM International: West Conshohocken, PA, USA, 2014.
- ASTM D2487; Standard Practice for Classification of Soils for Engineering Purposes (Unified Soil Classification System). ASTM International: West Conshohocken, PA, USA, 2011; pp. 1–16. [CrossRef]
- ACI (American Concrete Institute). ACI PRC-230.1-09; Report on Soil Cement (ACI 230-09) and Commentary (ACI 230.1R-09). ACI (American Concrete Institute): Farmington Hills, MI, USA, 2009.
- Baldovino, J.d.J.A.; Nuñez de la Rosa, Y.E.; Calabokis, O.P. Effect of Porosity/Binder Index on Strength, Stiffness and Microstructure of Cemented Clay: The Impact of Sustainable Development Geomaterials. Materials 2024, 17, 921. [Google Scholar] [CrossRef]
- ASTM D1631; Standard Practice for Making and Curing Soil-Cement Compression and Flexure Test Specimens in the Laboratory. ASTM International: West Conshohocken, PA, USA, 2007.
- Consoli, N.C.; Quiñonez, R.A.; González, L.E.; López, R.A.; Quiñónez, R.A.; González, L.E.; López, R.A.; Quiñonez, R.A.; González, L.E.; López, R.A.; et al. Influence of Molding Moisture Content and Porosity/Cement Index on Stiffness, Strength, and Failure Envelopes of Artificially Cemented Fine-Grained Soils. J. Mater. Civ. Eng. 2016, 29, 04016277. [Google Scholar] [CrossRef]
- Consoli, N.C.; Vaz Ferreira, P.M.; Tang, C.S.; Veloso Marques, S.F.; Festugato, L.; Corte, M.B. A Unique Relationship Determining Strength of Silty/Clayey Soils-Portland Cement Mixes. Soils Found. 2016, 56, 1082–1088. [Google Scholar] [CrossRef]
- ASTM C597-22; Standard Test Method for Ultrasonic Pulse Velocity Through Concrete. ASTM International: West Conshohocken, PA, USA, 2022; pp. 1–4.
- Baldovino, J.A.; Izzo, R.; Ekinci, A. Strength Relationship Equation for Artificially Stabilized Rammed Sedimentary Soils. Buildings 2022, 12, 1433. [Google Scholar] [CrossRef]
- Consoli, N.C.; Tomasi, L.F.; Veloso Marques, S.F. Cement-Enhancing Mechanical Behavior of Tailings Behind Upstream Tailings Dam for Safe Decommissioning. J. Mater. Civ. Eng. 2023, 35, 06023001. [Google Scholar] [CrossRef]
- Quiñónez Samaniego, R.A.; Scheuermann Filho, H.C.; de Araújo, M.T.; Bruschi, G.J.; Festugato, L.; Consoli, N.C. Key Parameters Controlling Strength and Resilient Modulus of a Stabilised Dispersive Soil. Road Mater. Pavement Des. 2023, 24, 279–294. [Google Scholar] [CrossRef]
- Henzinger, C.; Schuhmacher, S.A.; Festugato, L. Applicability of the Porosity/Binder Index to Nonhomogeneous Mixtures of Fine-Grained Soil with Lignite Fly Ash. J. Mater. Civ. Eng. 2018, 30, 06018013. [Google Scholar] [CrossRef]
- Román Martínez, C.; Nuñez de la Rosa, Y.E.; Estrada Luna, D.; Baldovino, J.A.; Jordi Bruschi, G. Strength, Stiffness, and Microstructure of Stabilized Marine Clay-Crushed Limestone Waste Blends: Insight on Characterization through Porosity-to-Cement Index. Materials 2023, 16, 4983. [Google Scholar] [CrossRef]
- Baldovino, J.d.J.A.; Izzo, R.L.d.S.; Pereira, M.D.; Rocha, E.V.D.G.; Rose, J.L.; Bordignon, V.R. Equations Controlling Tensile and Compressive Strength Ratio of Sedimentary Soil–Cement Mixtures under Optimal Compaction Conditions. J. Mater. Civ. Eng. 2020, 32, 04019320. [Google Scholar] [CrossRef]
- Pham, T.A.; Kyokawa, H.; Koseki, J.; Dias, D. A New Index for the Strength Analysis and Prediction of Cement-Mixed Soils. Eur. J. Environ. Civ. Eng. 2023, 27, 1512–1534. [Google Scholar] [CrossRef]
- Liu, L.; Zhou, A.; Deng, Y.; Cui, Y.; Yu, Z.; Yu, C. Strength Performance of Cement/Slag-Based Stabilized Soft Clays. Constr. Build. Mater. 2019, 211, 909–918. [Google Scholar] [CrossRef]
- Baldovino, J.A.; Nuñez de la Rosa, Y.E.; Palma Calabokis, O. Analysis of Novel Porosity–Water-to-Binder Index for Prediction of Strength, Stiffness and Durability for Cemented Soils. Materials 2023, 16, 6354. [Google Scholar] [CrossRef]
- Baldovino, J.J.; Izzo, R.L.; Rose, J.L.; Domingos, M.D. Strength, durability, and microstructure of geopolymers based on recycled-glass powder waste and dolomitic lime for soil stabilization. Constr. Build. Mater. 2021, 271, 121874. [Google Scholar] [CrossRef]
- Rios, S.; Viana da Fonseca, A.; Consoli, N.C.; Floss, M.; Cristelo, N. Influence of Grain Size and Mineralogy on the Porosity/Cement Ratio. Géotechnique Lett. 2013, 3, 130–136. [Google Scholar] [CrossRef]
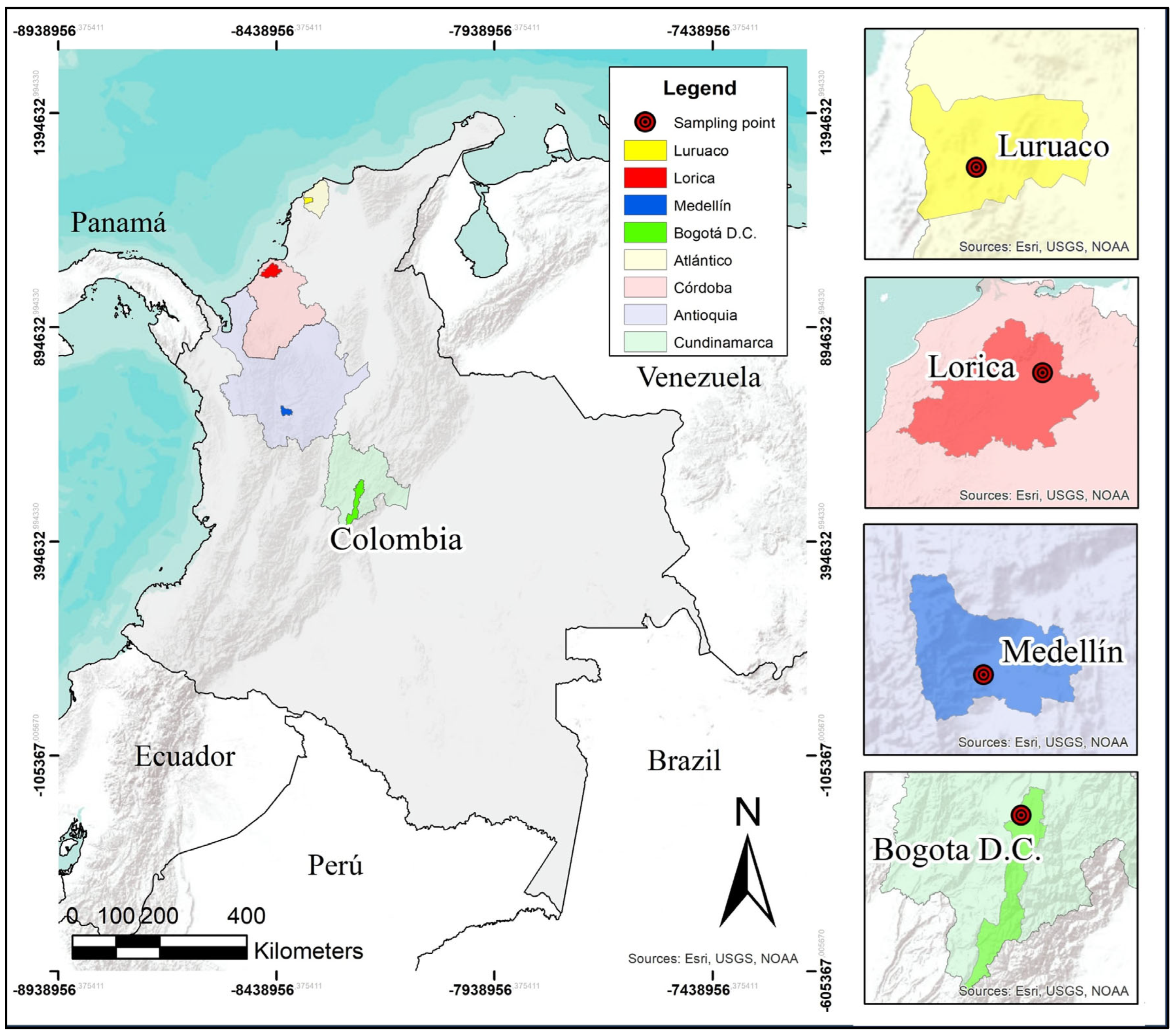
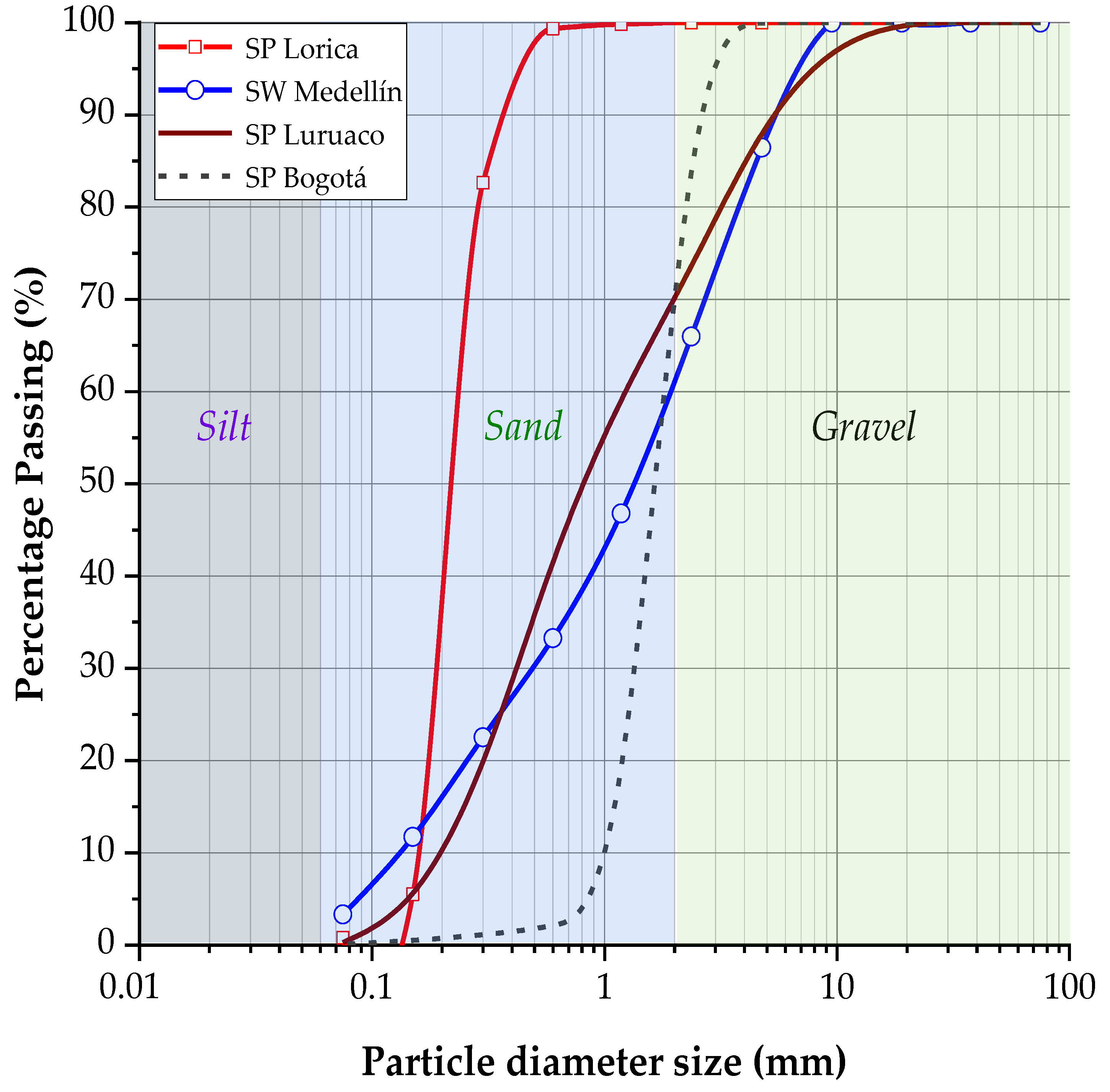







| Mix | Weight (%) | Curing Times (d) | Molding γd (kN/m3) | Number of Specimens | |
|---|---|---|---|---|---|
| Soil | Cement | ||||
| Luruaco soil–cement | 100 | 3, 5, 7, 9 | 7 | 17.1, 16.5, 15.8 | 36 |
| Medellin soil–cement | 100 | 3, 5, 7, 9 | 7 | 19.5, 18.5, 17.5 | 36 |
| Lorica soil–cement | 100 | 3, 5, 7, 9 | 7 | 15.3, 14.8, 14.3 | 36 |
| Bogotá soil–cement | 100 | 3, 5, 7, 9 | 7 | 16.6, 16.2, 15.8 | 36 |
| Soil | Compressive Strength Equation | Adjustment of Volumetric Index “a” | Coefficient of Determination |
|---|---|---|---|
| Luruaco sand | 0.25 | ||
| Lorica sand | 1.00 | ||
| Medellin sand | 0.84 | ||
| Bogotá sand | 1.00 |
| Soil | Stiffness Equation | Adjustment of Volumetric Index “a” | Coefficient of Determination |
|---|---|---|---|
| Luruaco sand | 0.25 | ||
| Lorica sand | 1.00 | ||
| Medellin sand | 0.84 | ||
| Bogotá sand | 1.00 |
| Soil Type | For Normalization | ||||
|---|---|---|---|---|---|
| Luruaco sand | |||||
| Lorica sand | |||||
| Medellin sand | |||||
| Bogotá sand | |||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
López, L.C.S.; Vergara, J.A.A.; de la Rosa, Y.E.N.; Arrieta, A.; Baldovino, J.d.J.A. Effect of Grain Size and Porosity/Binder Index on the Unconfined Compressive Strength, Stiffness and Microstructure of Cemented Colombian Sands. Materials 2024, 17, 5193. https://doi.org/10.3390/ma17215193
López LCS, Vergara JAA, de la Rosa YEN, Arrieta A, Baldovino JdJA. Effect of Grain Size and Porosity/Binder Index on the Unconfined Compressive Strength, Stiffness and Microstructure of Cemented Colombian Sands. Materials. 2024; 17(21):5193. https://doi.org/10.3390/ma17215193
Chicago/Turabian StyleLópez, Luis Carlos Suárez, Jesús Alberto Alcalá Vergara, Yamid E. Nuñez de la Rosa, Alvaro Arrieta, and Jair de Jesús Arrieta Baldovino. 2024. "Effect of Grain Size and Porosity/Binder Index on the Unconfined Compressive Strength, Stiffness and Microstructure of Cemented Colombian Sands" Materials 17, no. 21: 5193. https://doi.org/10.3390/ma17215193
APA StyleLópez, L. C. S., Vergara, J. A. A., de la Rosa, Y. E. N., Arrieta, A., & Baldovino, J. d. J. A. (2024). Effect of Grain Size and Porosity/Binder Index on the Unconfined Compressive Strength, Stiffness and Microstructure of Cemented Colombian Sands. Materials, 17(21), 5193. https://doi.org/10.3390/ma17215193







