On the Susceptibility of Reinforced Concrete Beam and Rigid-Frame Bridges Subjected to Spatially Varying Mining-Induced Seismic Excitation
Abstract
1. Introduction
2. Materials and Methods
2.1. Characteristics of Mining-Induced Tremors and the Shock Used for Dynamic Analysis
2.2. Theoretical Framework for the Dynamic Response of Multiple-Support Structures to Spatially Varying Ground Motion
- Mss, Css, and Kss—mass, damping, and stiffness matrix corresponding to non-support DOFs;
- Mgg, Cgg, and Kgg—mass, damping, and stiffness matrix corresponding to support DOFs;
- Msg, Csg, and Ksg—mass, damping, and stiffness coupling matrix;
- ,, and —accelerations, velocities, and displacements vector for each of the DOFs of the structure;
- , , and —accelerations, velocities, and displacements vector for each of the DOFs of the ground;
- —forces generated at the supports.
2.3. Theorethical Basis of the Large Mass Method
- M0—large mass added to supported DOFs;
- —inertia forces;
- —vector of large mass acceleration.
- n—number of modes;
- mn, kn, and cn—vector of modal mass, modal stiffness, and modal damping for n-th mode;
- qn—generalized coordinate;
- ϕn—n-th modal vector.
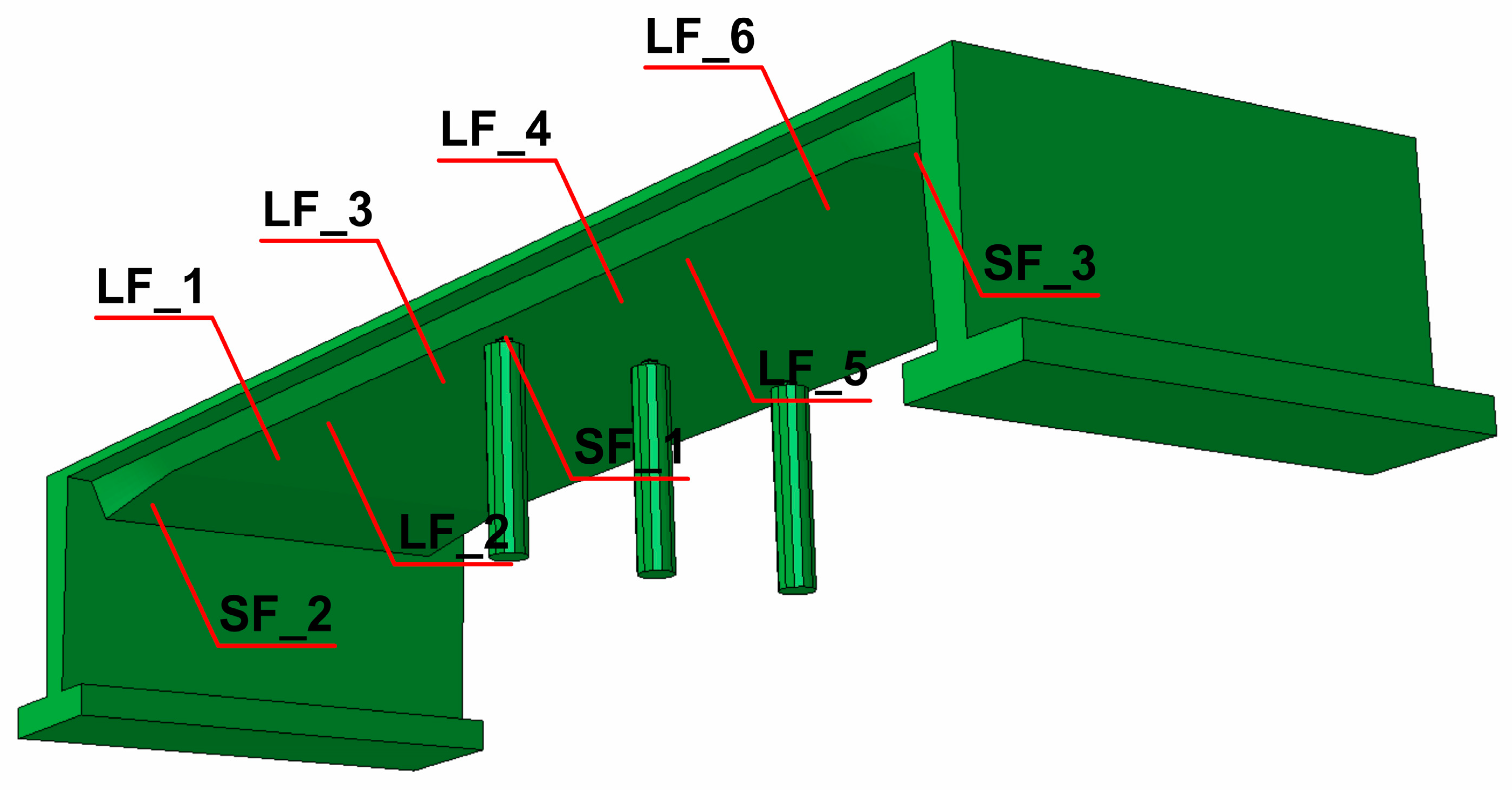
2.4. Structural Solutions for Bridges Subjected to Dynamic Analysis
2.4.1. Structural Design and Numerical Model of a Beam Bridge
2.4.2. Structural Design and Numerical Model of a Rigid-Frame Bridge
3. Results
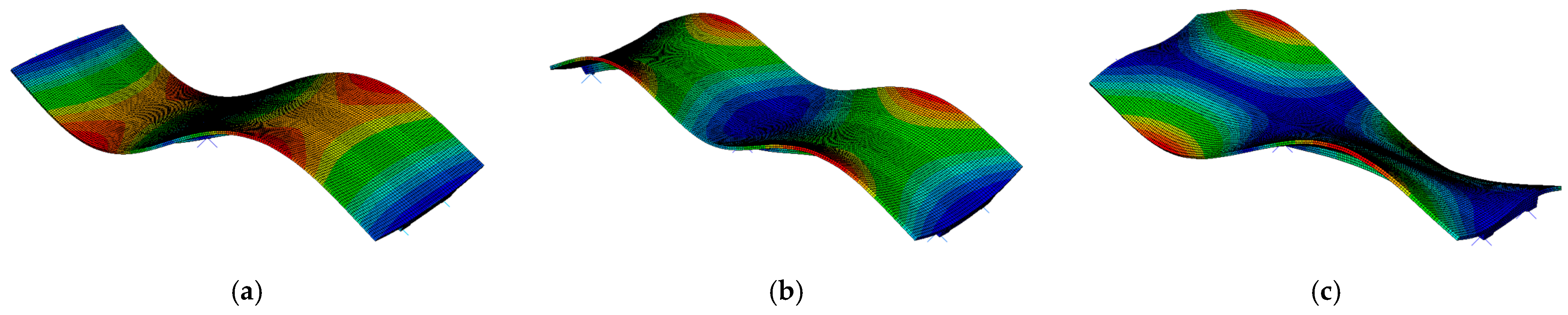
3.1. Numerical Evaluation of Natural Frequencies and Modes of Vibration of the Bridges
3.2. Time History Analysis of Principal Stresses in the Bridges Subjected to the Mining Shock
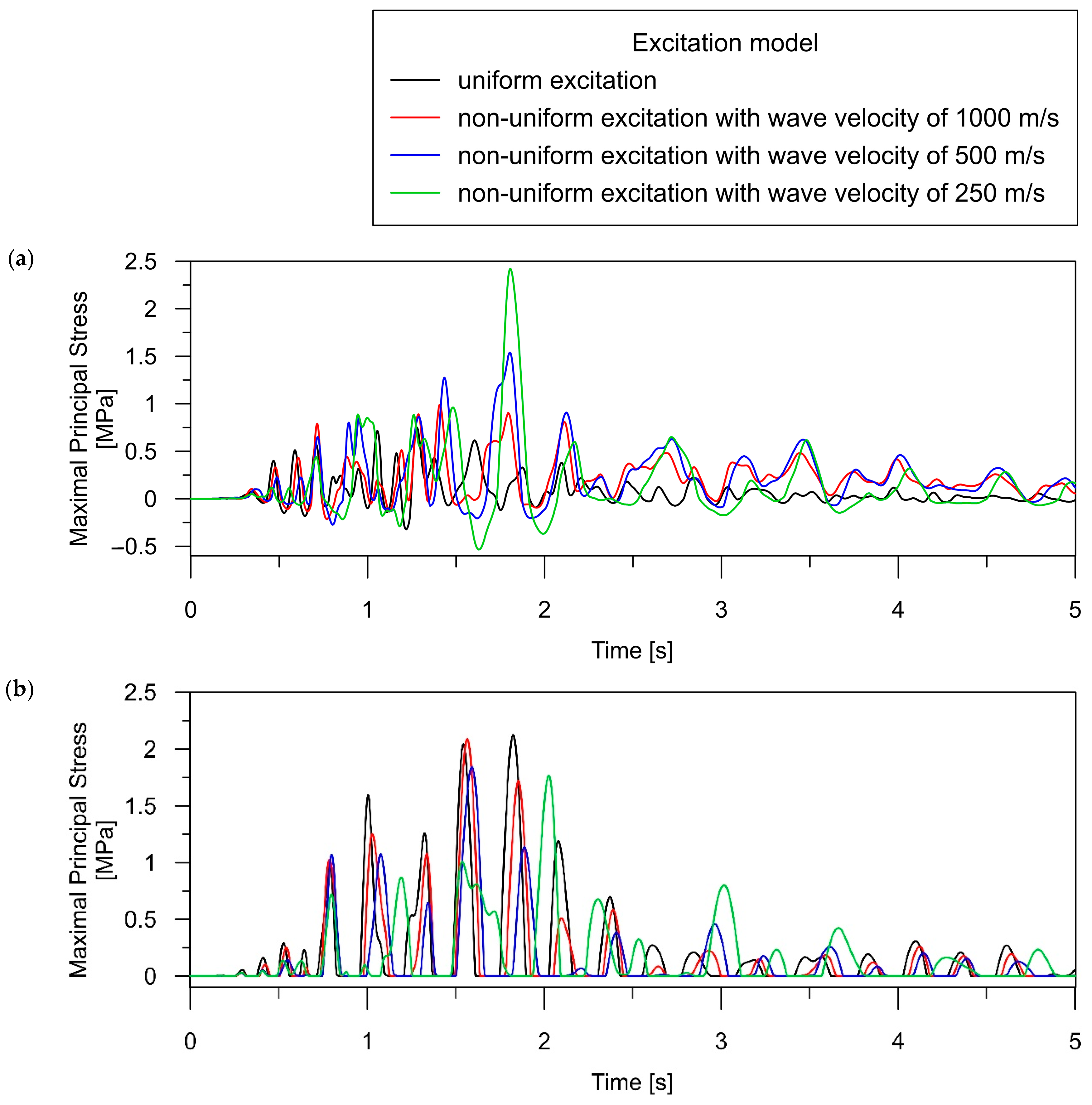
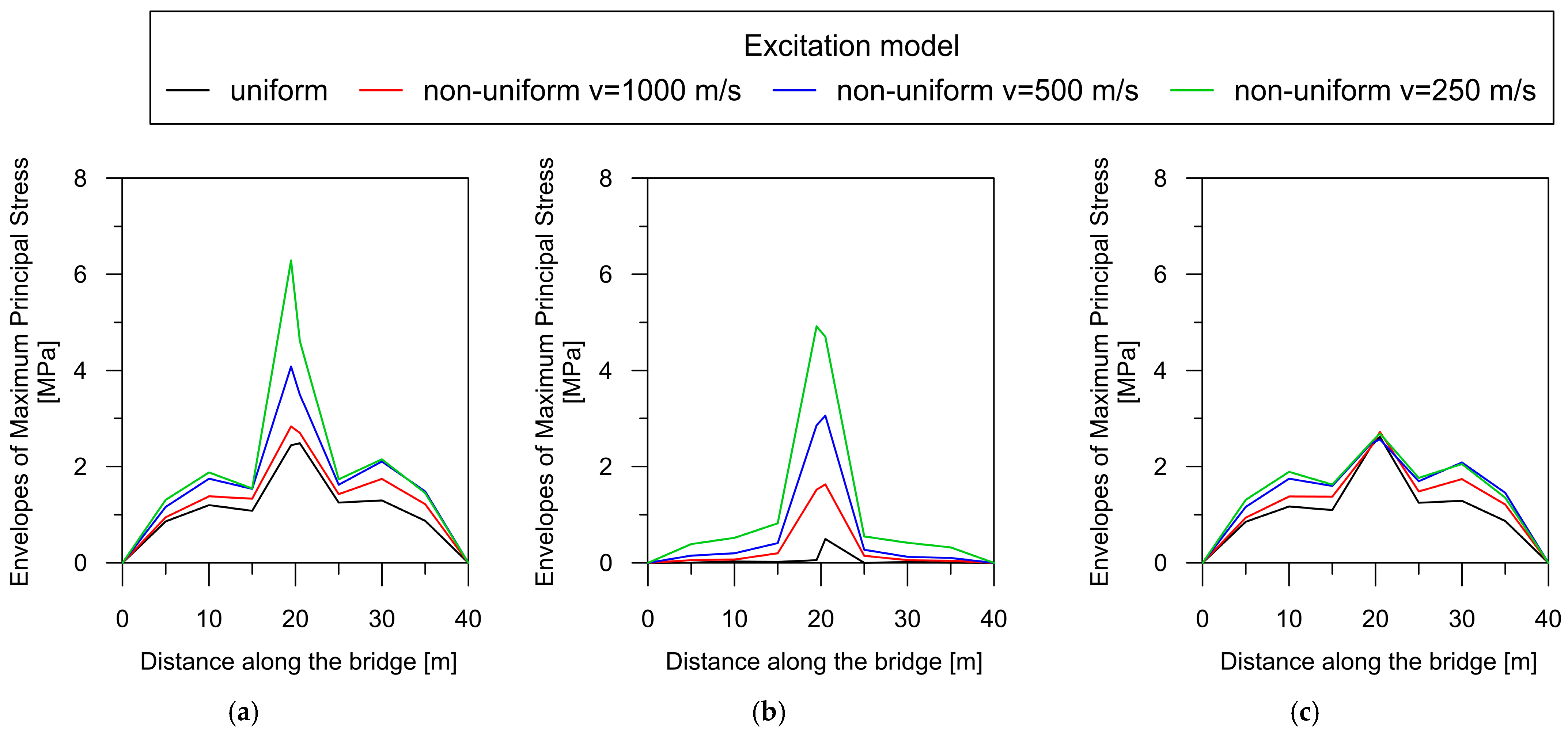
- For point SB_1, located above the support, the maximum stress caused by the tremor is higher for the non-uniform model application (see Figure 10a) compared to the uniform model. We can observe that reducing the velocity of the shock wave results in an increase in dynamic response. It is worth emphasizing that the differences in the dynamic performance of the bridge under various excitation models are significant. The stresses obtained for two extreme excitation scenarios (uniform and non-uniform with a velocity of 250 m/s) differ 2.5-fold.
- Applying the non-uniform excitation model results in elevated stress levels compared to the uniform excitation model, as seen in element LB_2 (refer to Figure 10b). The maximum stress for non-uniform excitation with a velocity of 250 m/s is approximately 1.5 times higher than the stresses obtained for uniform excitation.
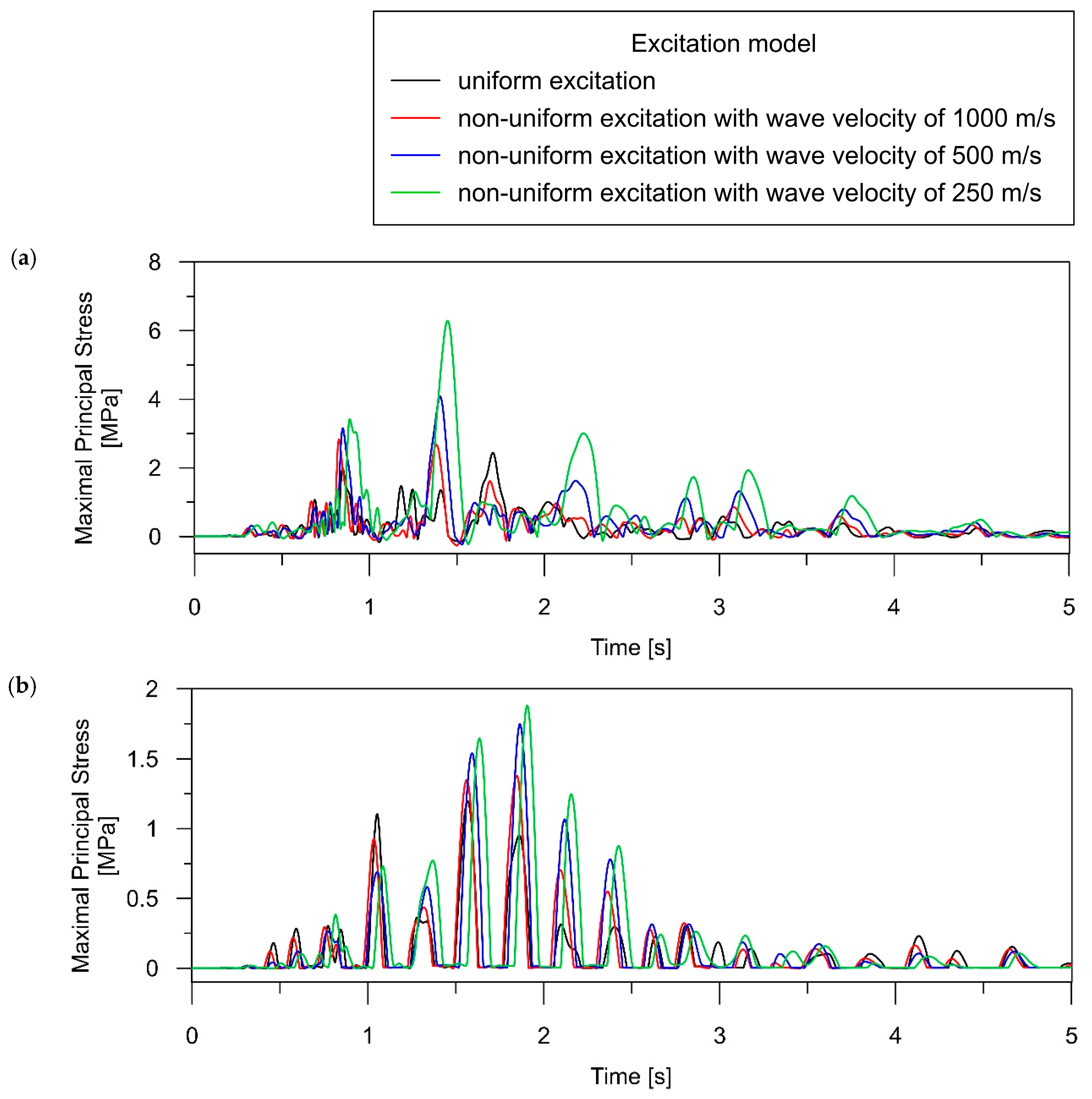
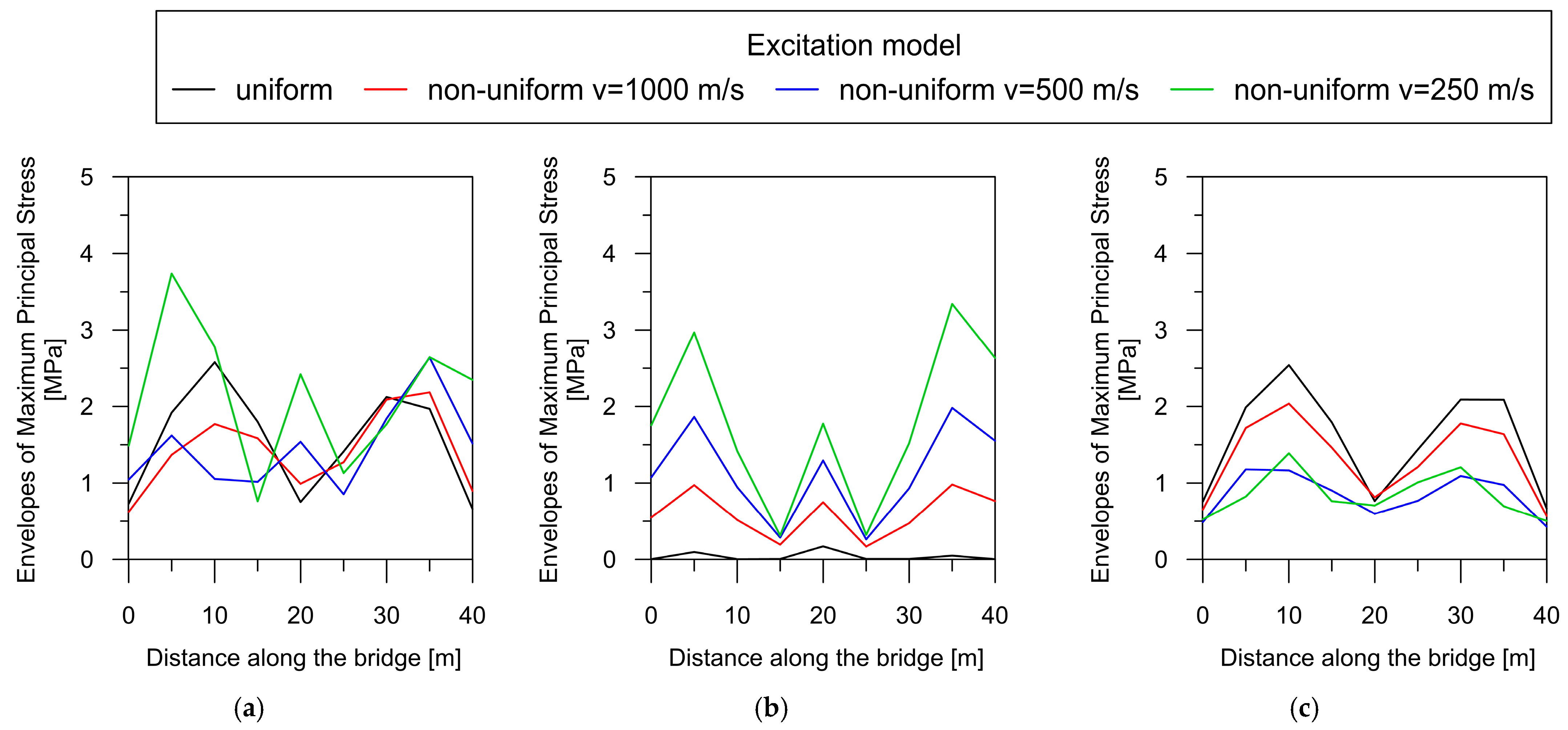
- The stress analysis of the rigid-frame bridge in the support zone leads to both qualitative and quantitative conclusions analogous to those determined in the case of the beam bridge. For point SF_1, the maximum stress is higher with the application of the non-uniform model (see Figure 11a) compared to the uniform model, and a decrease in the wave velocity corresponds to an increase in the dynamic response. The stresses obtained for the uniform and non-uniform model with a velocity of 250 m/s exhibit 3-fold differences.
- The non-uniform excitation model produces distinct performances in the span zones compared to the supports. The maximum stress at the midspan point LF_2 is higher for the uniform model than for the non-uniform model of excitation (see Figure 11b). The maximum stress values decrease as the wave velocity decreases, reaching the smallest value for the slowest wave at 250 m/s. The maximum stress for non-uniform excitation with a velocity of 250 m/s is approximately 20% lower than for the stresses obtained for uniform excitation.
3.3. Dependence of the Dynamic Behavior of the Bridges on Wave Velocity
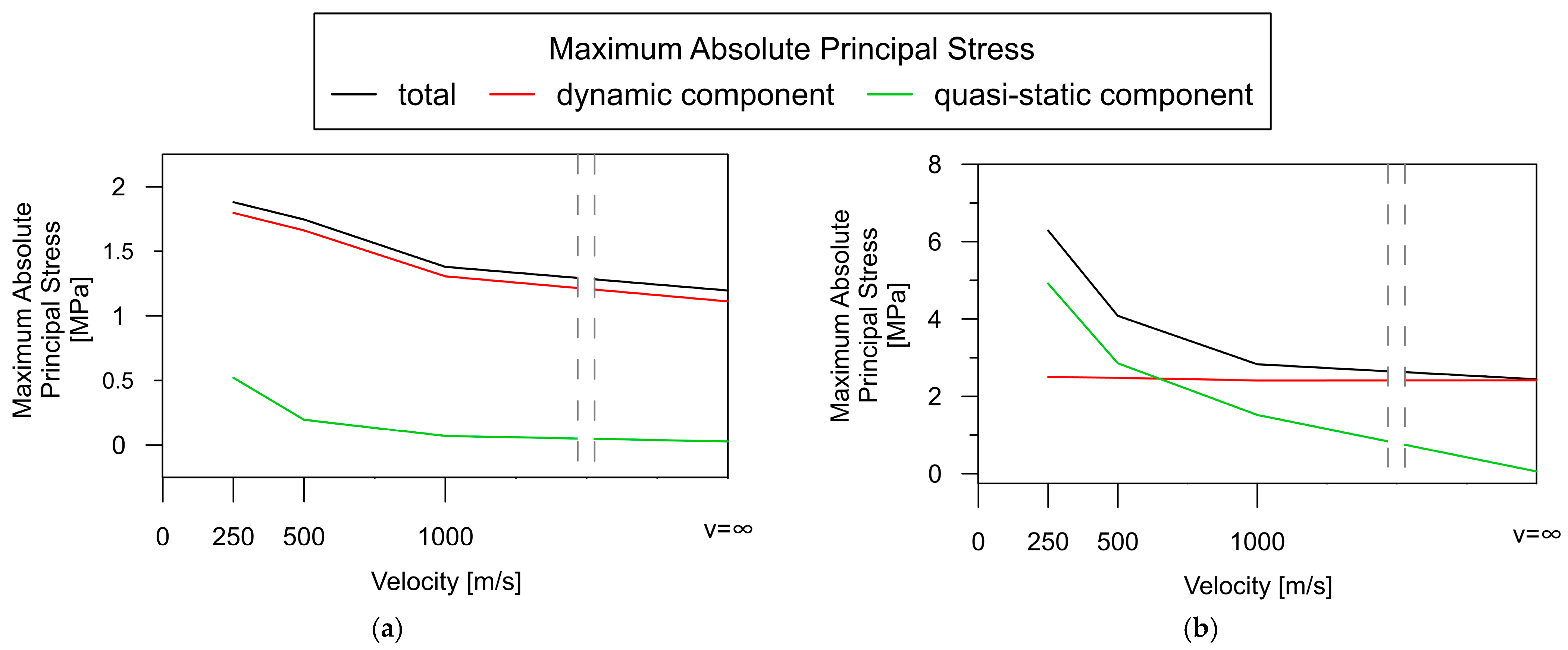
3.3.1. Stress–Velocity Dependence for the Beam Bridge
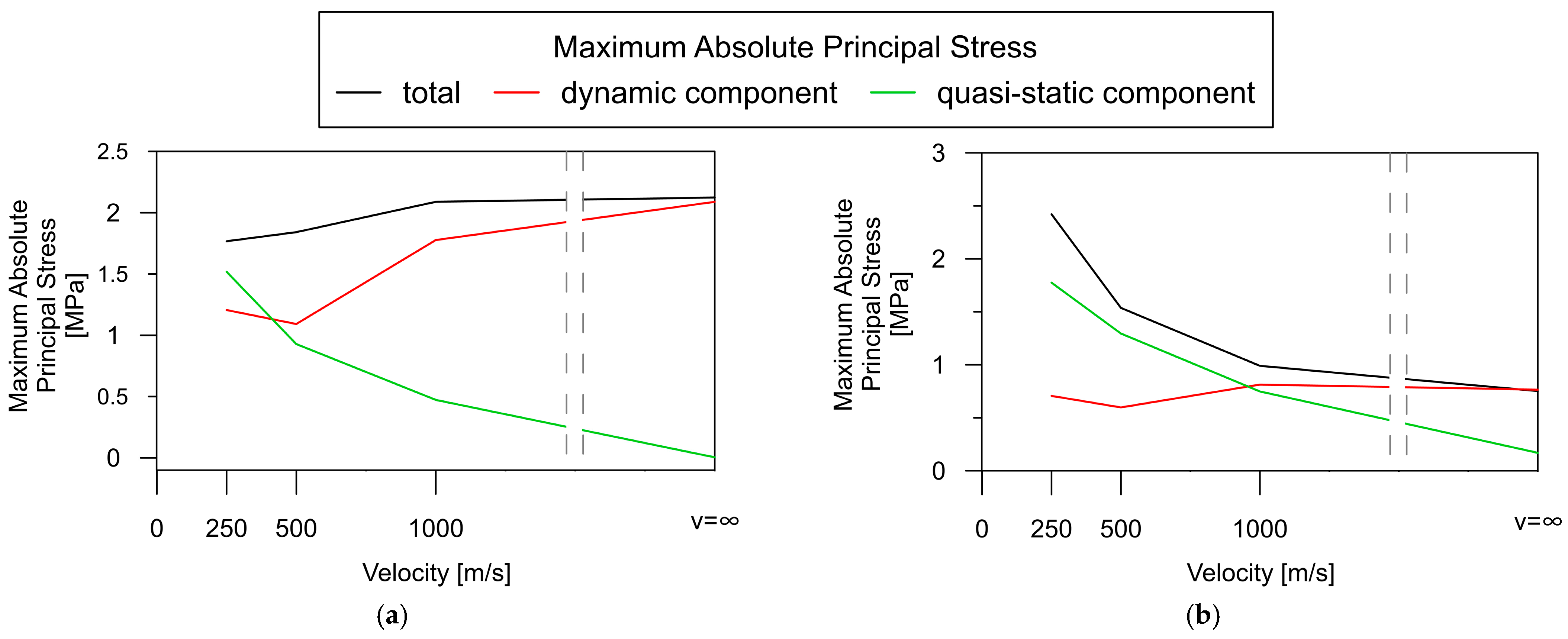
3.3.2. Stress–Velocity Dependence for the Rigid-Frame Bridge
3.3.3. The Obtained Results in the Context of the Literature Research
4. Discussion on the Susceptibility of the Bridges to Quasi-Static and Dynamic Effects
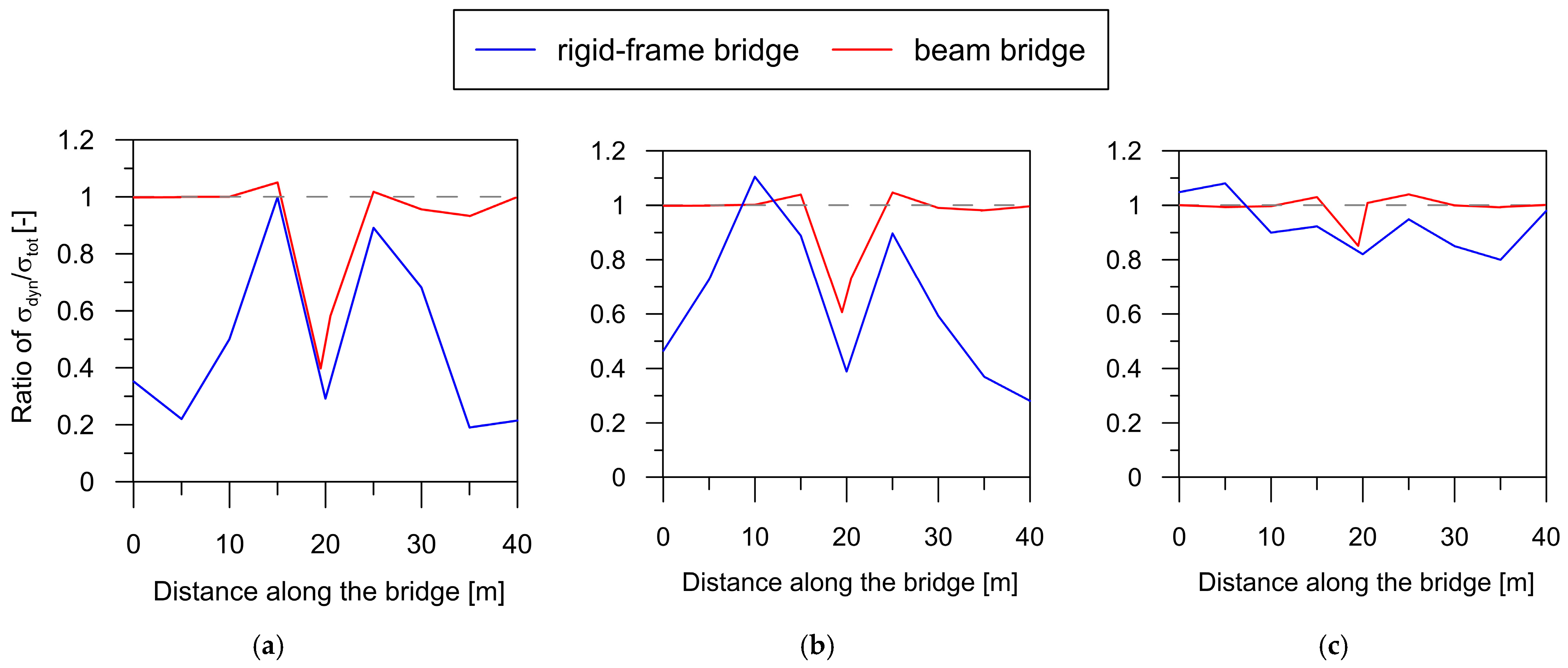
4.1. Rigid-Frame Bridge vs. Beam Bridge: A Comparison of Susceptibility to Quasi-Static Effect
4.2. Rigid-Frame Bridge vs. Beam Bridge: A Comparison of Susceptibility to Dynamic Effects
5. Conclusions
- The dynamic responses of both types of bridges undergo significant changes when subjected to the model of spatially varying ground mining-induced excitation, compared to the model of uniform excitation.
- In the case of the beam bridge, the impact of non-uniform excitation is evident across the entire structure, with the most notable effects observed at the central support, where the dynamic response evidently increases with decreasing wave velocity.
- The relationship between the total dynamic response and the wave velocity is less straightforward for the rigid-frame bridge. Similarly, as observed in the case of the beam bridge, applying the spatially varying excitation model results in increased stress in the support zones. However, in the spans, the highest stresses are obtained under uniform excitation.
- The quasi-static component plays a crucial role in the overall dynamic responses for both beam and rigid-frame bridges. However, rigid-frame bridges exhibit much greater susceptibility to quasi-static effects compared to beam bridges. This is related to the higher stiffness of the rigid-frame bridge. On the other hand, beam bridges are more susceptible to the dynamic components of stresses.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Siwowski, T.; Kaleta, D.; Reizer, E.; Macheta, D. Catalog of Typical Structures of Road Bridges and Culverts. In Part I: Structures Design; Promost Consulting: Rzeszów, Poland, 2018. (In Polish) [Google Scholar]
- Gueha, S.K. Mining Induced Seismicity. In Induced Earthquakes; Springer: Dordrecht, The Netherlands, 2020. [Google Scholar] [CrossRef]
- Gibowicz, S. Chapter 1—Seismicity Induced by Mining: Recent Research. Adv. Geophys. 2009, 51, 1–53. [Google Scholar] [CrossRef]
- Bischoff, M.; Cete, A.; Fritschen, R.; Meier, T. Coal mining induced seismicity in the Ruhr Area, Germany. Pure Appl. Geophys. 2010, 167, 63–75. [Google Scholar] [CrossRef]
- Mutke, G.; Lurka, A.; Zembaty, Z. Prediction of rotational ground motion for mining-induced seismicity—Case study from Upper Silesian Coal Basin, Poland. Eng. Geol. 2020, 276, 105767. [Google Scholar] [CrossRef]
- Dubiński, J.; Stec, K.; Bukowska, M. A geomechanical and tectonophysical conditions of mining-induced seismicity in the Upper Silesian Coal Basin in Poland: A Case study. Arch Min. Sci. 2019, 64, 163–180. [Google Scholar] [CrossRef]
- Tatara, T. Mining activity in Poland: Challenges. Brick and Block Masonry-From Historical to Sustainable Masonry. In Proceedings of the 17th International Brick/Block Masonry Conference (17thIB2MaC 2020), Krakow, Poland, 5–8 July 2020. [Google Scholar]
- Zerva, A. Spatial Variation of Seismic Ground Motions: Modeling and Engineering Applications; CRC Press/Balkema–Taylor & Francis Group: Boca Raton, FL, USA, 2009. [Google Scholar]
- Schiappapietra, E.; Douglas, J. Modelling the spatial correlation of earthquake ground motion: Insights from the literature, data from the 2016-2017 Central Italy earthquake sequence and ground-motion simulations. Earth-Sci. Rev. 2020, 203, 103139. [Google Scholar] [CrossRef]
- Dalguer, L. Review on Studies Related to Spatial Variability of Ground Motion in the Near Field. Report of Network of European Research Infrastructures for Earthquake Risk Assessment and Mitigation. Seventh Framework Programme EC Project Number: 262330. Available online: https://cordis.europa.eu/docs/results/262/262330/final1-nerafinalreport.pdf (accessed on 15 January 2024).
- EN 1998-1; Eurocode 8: Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings. CEN: Brussels, Belgium, 2004.
- Trifunac, M.D. Recording Strong Earthquake Motion—Instruments, Recording Strategies and Data Processing; Report No. CE 07-03; University of Southern California: Los Angeles, CA, USA, 2007. [Google Scholar]
- Erdik, M.; Fahjan, Y.; Ozel, O.; Alcik, H.; Mert, A.; Gul, M. Istanbul Earthquake Rapid Response and the Early Warning System. Bull. Earthq. Eng. 2003, 1, 157–163. [Google Scholar] [CrossRef]
- Stephenson, W.R. Visualisation of resonant basin response at the Parkway array, New Zealand. Soil Dyn. Earthq. Eng. 2007, 27, 487–496. [Google Scholar] [CrossRef]
- Fuławka, K.; Pytel, W.; Pałac-Walko, B. Near-Field Measurement of Six Degrees of Freedom Mining-Induced Tremors in Lower Silesian Copper Basin. Sensors 2020, 20, 6801. [Google Scholar] [CrossRef]
- Fuławka, K.; Mertuszka, P.; Szumny, M.; Stolecki, L.; Szczerbiński, K. Application of MEMS-Based Accelerometers for Near-Field Monitoring of Blasting-Induced Seismicity. Minerals 2022, 12, 533. [Google Scholar] [CrossRef]
- Boroń, P.; Dulińska, J.M.; Jasińska, D. Advanced model of spatiotemporal mining-induced kinematic excitation for multiple-support bridges based on the regional seismicity characteristics. Appl. Sci. 2022, 12, 7036. [Google Scholar] [CrossRef]
- Parvanehro, P.; Shiravand, M.R.; Safi, M. A method for linear response analysis of long bridges under multi-support seismic excitation. Bull. Earthq. Eng. 2022, 20, 8381–8410. [Google Scholar] [CrossRef]
- Papadopoulos, S.; Sextos, A. Simplified design of bridges for multiple-support earthquake excitation. Soil Dyn. Earthq. Eng. 2020, 131, 106013. [Google Scholar] [CrossRef]
- Kumar, A.; Debnath, N. Seismic behaviour of a typical rail bridge using north-east India specific synthetic ground motions under multi-support excitation. In Recent Developments in Sustainable Infrastructure. Lecture Notes in Civil Engineering; Das, B., Barbhuiya, S., Gupta, R., Saha, P., Eds.; Springer: Singapore, 2021. [Google Scholar] [CrossRef]
- Bakhshizadeh, A.; Sadeghi, K.; Ahmadi, S.; Royaei, J. Damage identification in long-span cable-stayed bridges under multiple support excitations. Int. J. Civ. Eng. 2023, 21, 1275–1290. [Google Scholar] [CrossRef]
- Lupoi, A.; Franchin, P.; Pinto, P.E.; Monti, G. Seismic design of bridges accounting for spatial variability of ground motion. Earthq. Eng. Struct. Dyn. 2005, 34, 327–348. [Google Scholar] [CrossRef]
- Tzanetos, N.; Elnashai, A.S.; Hamdan, F.H.; Antoniou, S. Inelastic dynamic response of RC bridges subjected to spatially non synchronous earthquake motion. Adv. Struct. Eng. 2000, 3, 191–214. [Google Scholar] [CrossRef]
- Monti, G.; Nuti, C.; Pinto, P.E. Nonlinear response of bridges under multi support excitation. J. Struct. Eng. 1996, 122, 1147–1159. [Google Scholar] [CrossRef]
- Burdette, N.J.; Elnashai, A.S.; Lupoi, A.; Sextos, A.G. Effect of asynchronous earthquake motion on complex bridges. I: Methodology and input motion. J. Bridge Eng. 2008, 13, 158–165. [Google Scholar] [CrossRef]
- Sextos, A.G.; Kappos, A.J. Evaluation of seismic response of bridges under asynchronous excitation and comparisons with Eurocode 8-2 provisions. Bull. Earthq. Eng. 2009, 7, 519–545. [Google Scholar] [CrossRef]
- Zhang, C.; Fu, G.; Lai, Z.; Du, X.; Wang, P.; Dong, H.; Jia, H. Shake table test of long span cable-stayed bridge subjected to near-fault ground motions considering velocity pulse effect and non-uniform excitation. Appl. Sci. 2020, 10, 6969. [Google Scholar] [CrossRef]
- Xie, W.; Sun, L.; Lou, M. Wave-passage effects on seismic responses of pile–soil–cable-stayed bridge model under longitudinal non-uniform excitation: Shaking table tests and numerical simulations. Bull. Earthq. Eng. 2020, 18, 5221–5246. [Google Scholar] [CrossRef]
- Feng, R.; Papadopoulos, S.; Yuan, W.; Sextos, A. Loss estimation of curved bridges considering the incidence angle and spatial variability of earthquake ground motion. Soil Dyn. Earthq. Eng. 2022, 163, 107523. [Google Scholar] [CrossRef]
- Li, N.; Li, B.; Chen, D.; Wang, E.; Tan, Y.; Qian, Y.; Jia, H. Waveform characteristics of earthquakes induced by hydraulic fracturing and mining activities: Comparison with those of natural earthquakes. Nat. Resour. Res. 2020, 29, 3653–3674. [Google Scholar] [CrossRef]
- Meng, S.; Mu, H.; Wang, M.; Yang, W.; Liu, Y.; Sun, Y.; Yuan, X. Characteristics and identification method of natural and mine earthquakes: A Case study on the Hegang Mining Area. Minerals 2022, 12, 1256. [Google Scholar] [CrossRef]
- Tatara, T. An Influence of Surface Mining-Related Vibration on Low-Rise Buildings; Scientific Notebooks of Cracow University of Technology: Cracow, Poland, 2002. (In Polish) [Google Scholar]
- Bal, I.; Dais, D.; Smyrou, E. “Differences” between induced and natural seismic events. In Proceedings of the 16th European Conference on Earthquake Engineering, (16ECEE), Thessaloniki, Greece, 18–21 June 2018. [Google Scholar]
- Siwek, S. Earth tides and seismic activity in deep coal mining. Int. J. Rock Mech. Min. 2021, 148, 104972. [Google Scholar] [CrossRef]
- Pachla, F.; Tatara, T. Resistance of residential and rural buildings due to mining exploitation in the coal mining area. Zesz. Nauk. Inst. Gospod. Surowcami Miner. Energ. PAN 2017, 101, 45–60. (In Polish) [Google Scholar]
- Tatara, T. Dynamic Resistance of Building under Mining Tremors; Cracow University of Technology Press: Krakow, Poland, 2012. (In Polish) [Google Scholar]
- Pachla, F.; Tatara, T. Nonlinear analysis of a hoist tower for seismic loads. Arch. Civ. Eng. 2022, 68, 177–198. [Google Scholar] [CrossRef]
- Clough, R.W.; Penzien, J. Dynamics of Structures; McGraw Hill Kogakusha Ltd.: New York, NY, USA, 1975. [Google Scholar]
- Datta, T.K. Seismic Analysis of Structure; John Wiley & Sons (Asia) LTD: Singapore, 2010. [Google Scholar]
- Chopra, A.K. Dynamic of Structures. Theory and Applications to Earthquake Engineering; Pearson Ltd.: Singapore, 2011. [Google Scholar]
- EN 1998-2; Eurocode 8: Design of Structures for Earthquake Resistance—Part 2: Bridges. CEN: Brussels, Belgium, 2005.
- Leger, P.; Ide, I.; Paultre, P. Multiple-support seismic analysis of large structures. Comput. Struct. 1990, 36, 1153–1158. [Google Scholar] [CrossRef]
- Kim, Y.-W.; Jhung, M. Mathematical analysis using two modeling techniques for dynamic responses of a structure subjected to a ground acceleration time history. Nucl. Eng. Technol. 2011, 43, 361–374. [Google Scholar] [CrossRef]
- Bai, F.; Liu, J.; Li, L. Elasto-plastic analysis of large span reticulated shell structure under multi-support excitations. Adv. Mat. Res. 2012, 446–449, 54–58. [Google Scholar] [CrossRef]
- Kim, Y.-W.; Jhoung, M. A study on large mass method for dynamic problem of multiple degree-of-freedom system excited by ground acceleration time history. J. Mech. Sci. Technol. 2014, 28, 25–41. [Google Scholar] [CrossRef]
- Zhang, J.; Mu, Z.; Gan, M. Study of the analysis methods of wave-passage effect based on ABAQUS. Adv. Mat. Res. 2011, 163–167, 4316–4319. [Google Scholar] [CrossRef]
- Siwowski, T.; Kaleta, D.; Reizer, E.; Macheta, D. Catalog of Typical Structures of Road Bridges and Culverts. In Assessment of Bridges Built in Poland after 2008; Promost Consulting: Rzeszów, Poland, 2018. (In Polish) [Google Scholar]
- Abaqus/Standard User’s Manual; Dassault Systèmes Simulia Corp.: Johnston, RI, USA, 2020.
- Sextos, A.; Karakostas, C.; Lekidis, V.; Papadopoulos, S. Multiple supportseismic excitation of the Evripos bridge based on free-field and on-structure recordings. Struct Infrastruct. Eng. 2015, 11, 1510–1523. [Google Scholar] [CrossRef]
- Harichandran, R. Spatial Variation of Earthquake Ground Motion; Department of Civil and Environmental Engineering Michigan State University: East Lansing, MI, USA, 1999. [Google Scholar]
- Drygała, I.J.; Dulińska, J.M.; Polak, M.A. Seismic Assessment of Footbridges under Spatial Variation of Earthquake Ground Motion (SVEGM): Experimental Testing and Finite Element Analyses. Sensors 2020, 20, 1227. [Google Scholar] [CrossRef] [PubMed]
- Boroń, P. Dynamic Response Analysis of the Multi-Support Structure to Mining Shocks Using Multiple Support Response Spectrum. Ph.D. Thesis, Cracow University of Technology, Cracow, Poland, 2019. (In Polish). [Google Scholar]
- Boroń, P.; Dulińska, J.M.; Jasińska, D. Impact of High Energy Mining-Induced Seismic Shocks from Different Mining Activity Regions on a Multiple-Support Road Viaduct. Energies 2020, 13, 4045. [Google Scholar] [CrossRef]
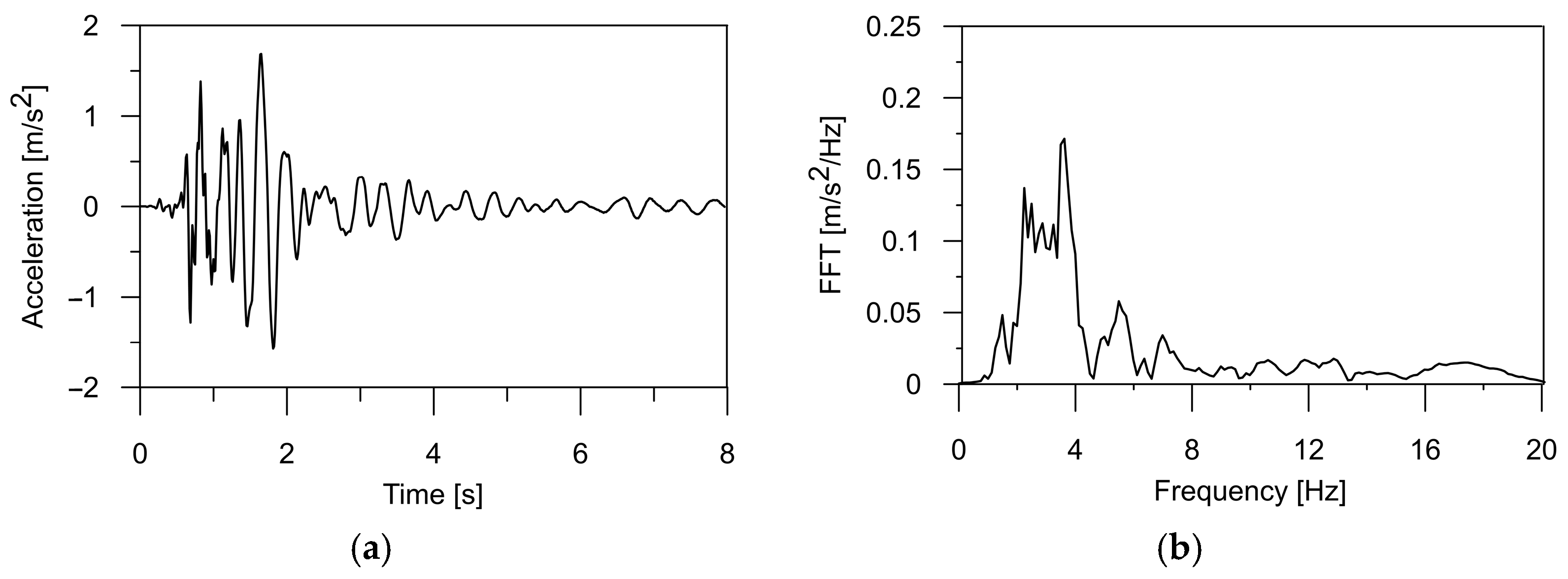
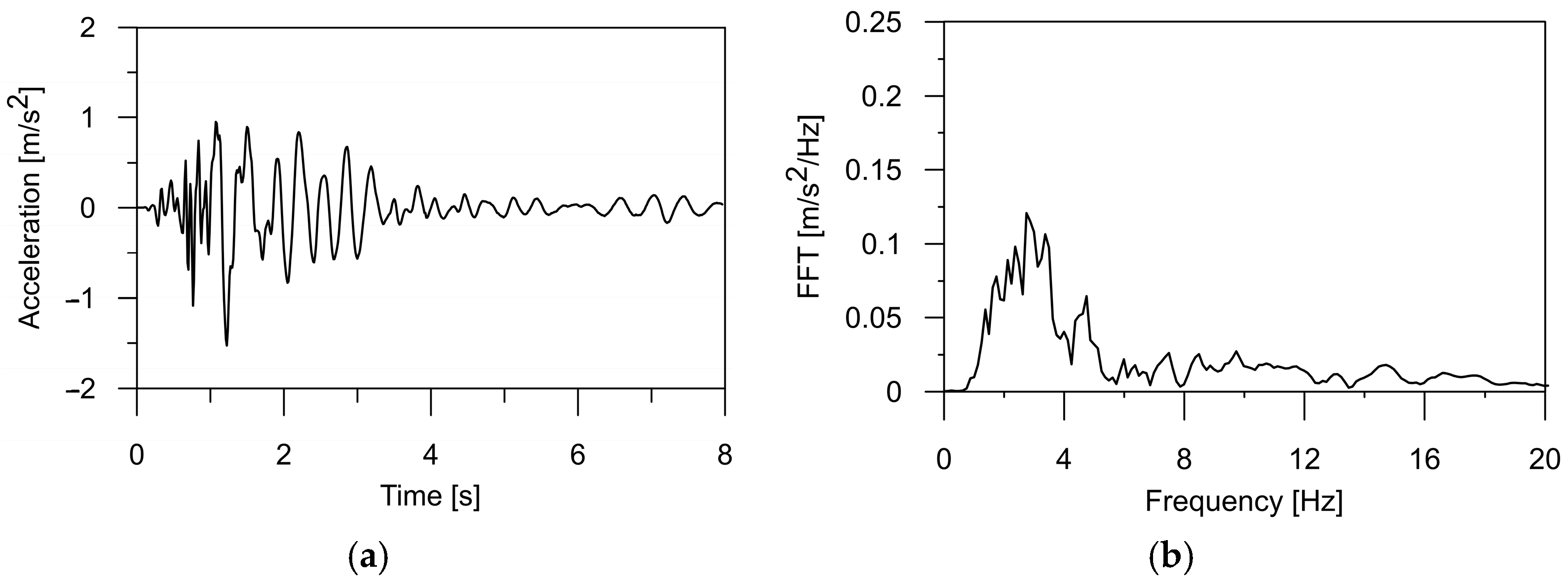

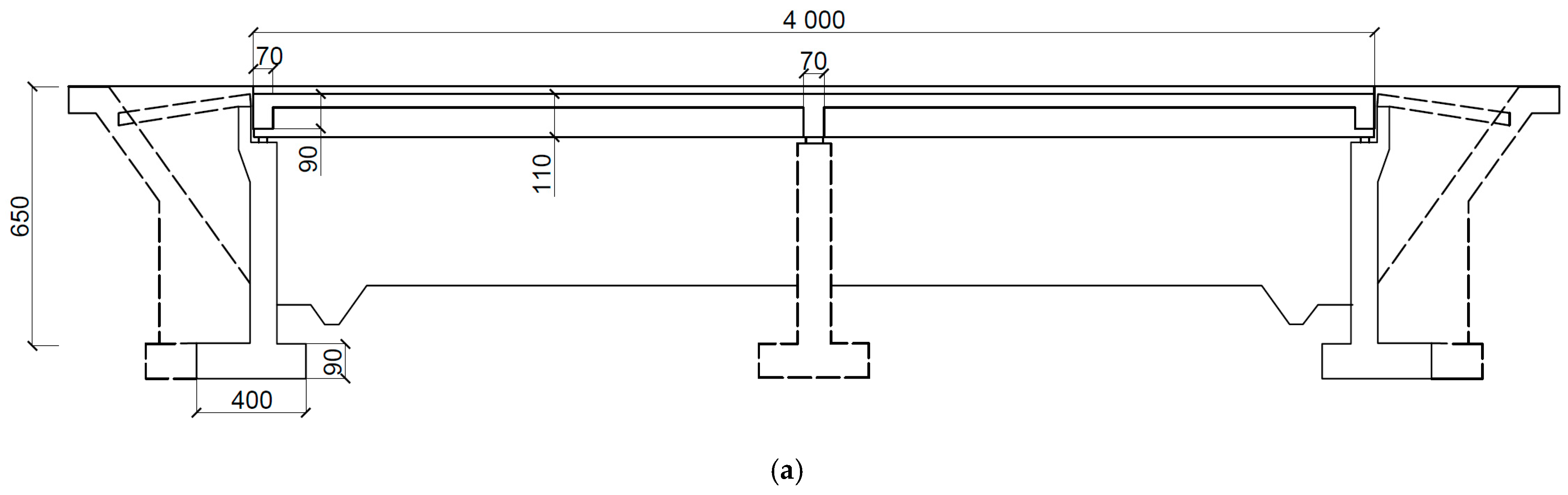


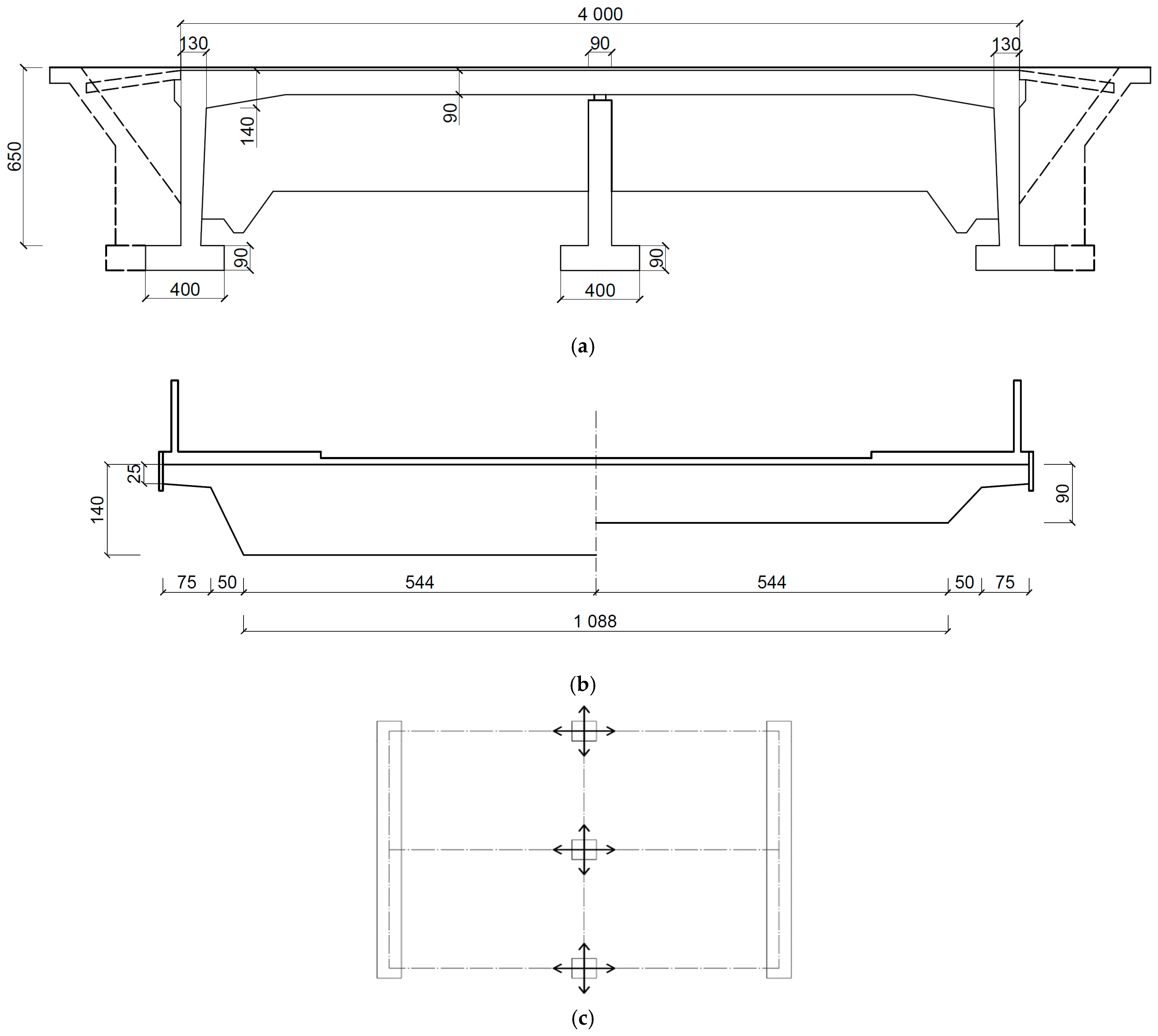


| Basic Characteristics | Natural Seismic Shocks | Minig-Induced Seismic Shocks |
|---|---|---|
| Source of the shock | Natural tectonic processes | Human activities related to mining, such as the extraction of minerals |
| Intensity and occurrence frequency | High magnitudes, frequency varying due to geological factors | Lower magnitudes but frequent in areas with extensive mining operations |
| Influence range | Widespread; significant environmental impacts, including infrastructure damage and life loss | Up to 10 km; primarily affect the mining area, potentially causing ground instability and damage to mine infrastructure |
| Shock duration | Lasts in minutes | Lasts up to 15 s |
| Intense phase time | Minutes | 0.5–5 s |
| Dominant frequency | Low range: 0.5–2 Hz | Higher range: 2–7 Hz |
| Seismic wave arrival sequence | Different types of seismic waves (primary, shear, and Raleigh) reach the receiver sequentially | Due to the proximity of the source, all types of body waves, along with surface waves, arrive at the receiver almost simultaneously |
| Magnitude of shock spatial components | The greatest amplitudes occur in the horizontal direction, parallel to the Raileigh wave propagation | Amplitudes in three directions are comparable; vertical amplitudes may even exceed those of horizontal vibrations |
| Decay rate of the shock | Decrease in vibration amplitudes depends on the geological site conditions | Impulse-like nature of amplitudes; the decay in amplitudes with increasing distance from the source occurs much more rapidly |
| Acceleration and frequency content | Acceleration and frequency range predictable, typical for given energies and epicentral distances | Unpredictable, wide acceleration and frequency range for given energies and epicentral distances |
| Mode | Natural Frequency [Hz] | |
|---|---|---|
| Beam Bridge | Rigid-Frame Bridge | |
| I | 4.12 | 4.04 |
| II | 6.18 | 7.97 |
| III | 6.97 | 8.48 |
| IV | 7.38 | 10.96 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boroń, P.; Drygała, I.; Dulińska, J.M.; Burdak, S. On the Susceptibility of Reinforced Concrete Beam and Rigid-Frame Bridges Subjected to Spatially Varying Mining-Induced Seismic Excitation. Materials 2024, 17, 512. https://doi.org/10.3390/ma17020512
Boroń P, Drygała I, Dulińska JM, Burdak S. On the Susceptibility of Reinforced Concrete Beam and Rigid-Frame Bridges Subjected to Spatially Varying Mining-Induced Seismic Excitation. Materials. 2024; 17(2):512. https://doi.org/10.3390/ma17020512
Chicago/Turabian StyleBoroń, Paweł, Izabela Drygała, Joanna Maria Dulińska, and Szymon Burdak. 2024. "On the Susceptibility of Reinforced Concrete Beam and Rigid-Frame Bridges Subjected to Spatially Varying Mining-Induced Seismic Excitation" Materials 17, no. 2: 512. https://doi.org/10.3390/ma17020512
APA StyleBoroń, P., Drygała, I., Dulińska, J. M., & Burdak, S. (2024). On the Susceptibility of Reinforced Concrete Beam and Rigid-Frame Bridges Subjected to Spatially Varying Mining-Induced Seismic Excitation. Materials, 17(2), 512. https://doi.org/10.3390/ma17020512







