Structural Phenomena Introduced by Rotary Swaging: A Review
Abstract
1. Introduction
1.1. Grain Refinement Mechanisms
1.2. Methods of Grain Refinement
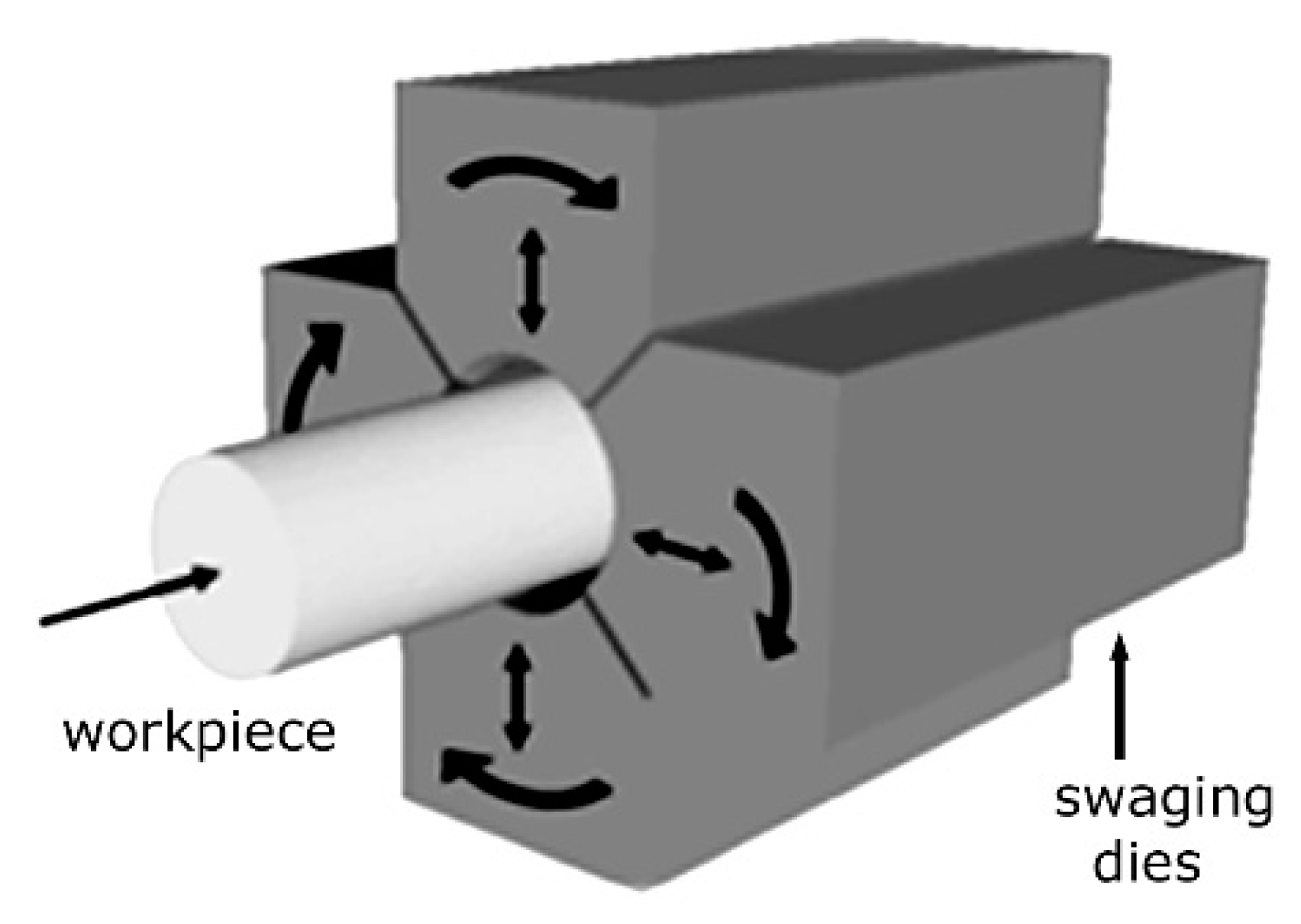
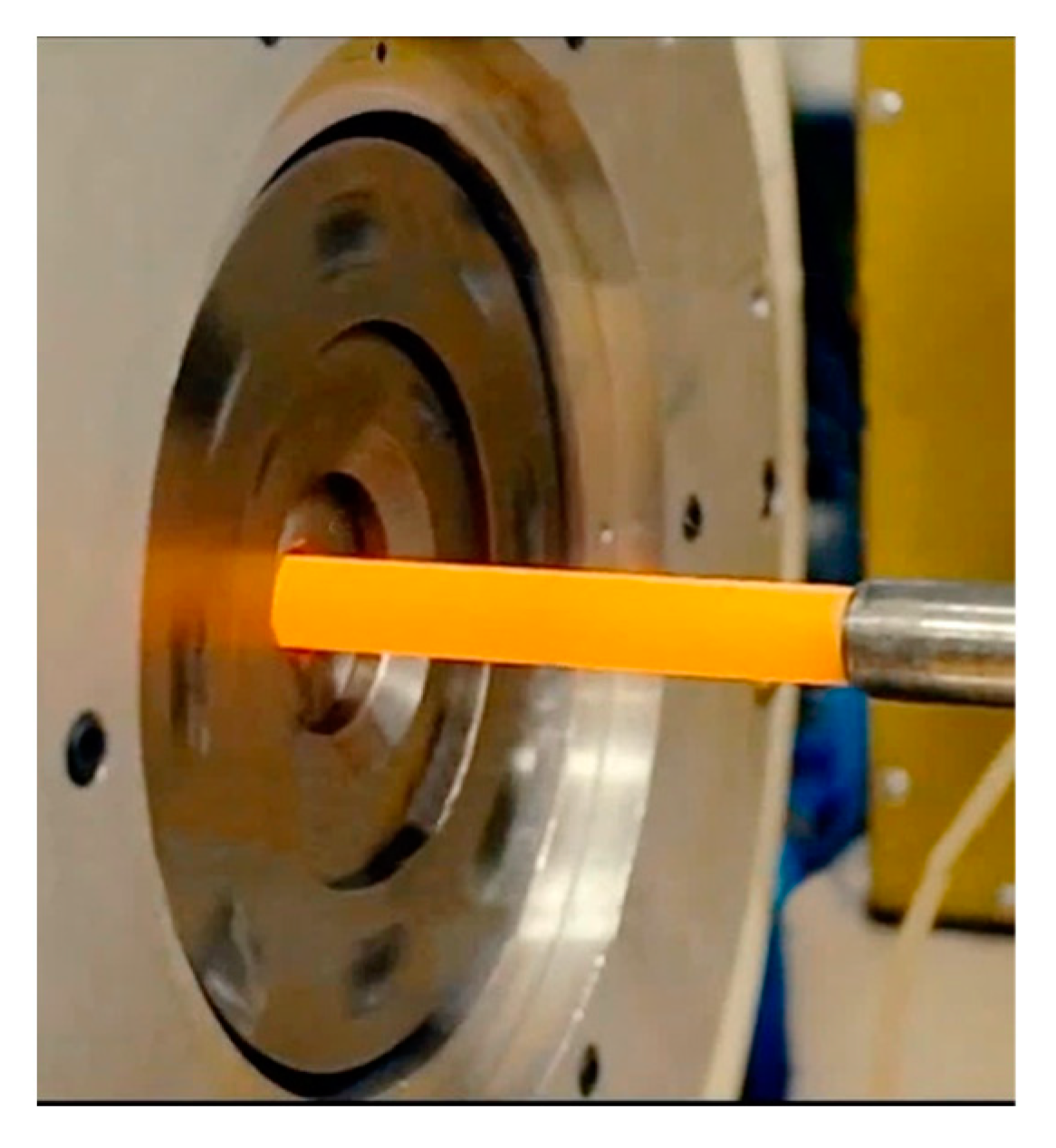
2. Rotary Swaging Method
3. Microstructure Development
3.1. FCC Lattice
3.1.1. Single-Phase Metallic Materials
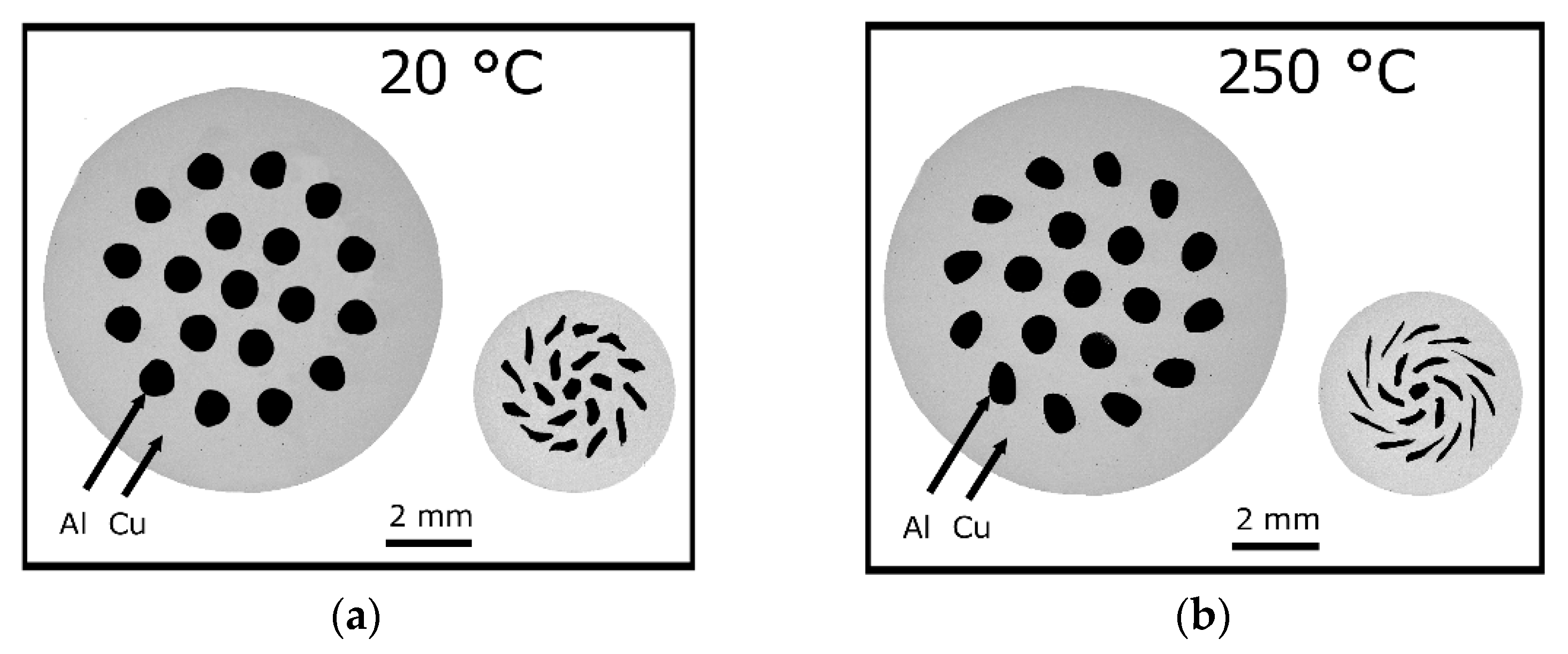
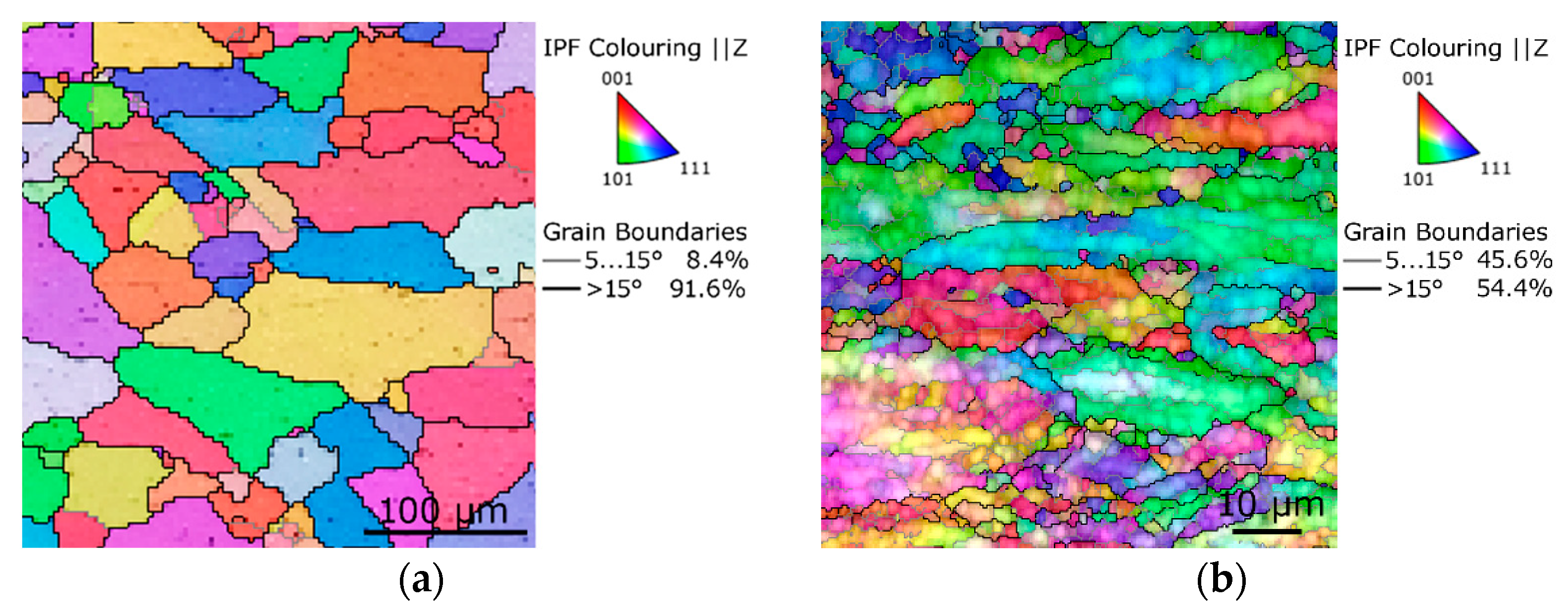
3.1.2. FCC Composites
3.2. BCC Lattice
3.2.1. Single-Phase Metallic Materials
3.2.2. BCC Composites and Multi-Phase Materials
3.3. Metals with HCP Lattice
4. Conclusions and Outlook
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Park, G.D.; Tran, V.L.; Hong, S.-T.; Jeong, Y.-H.; Yeo, T.S.; Nam, M.J.; Kim, M.-J.; Jin, S.-W.; Han, H.N. Electrically Assisted Stress Relief Annealing of Automotive Springs. J. Mech. Sci. Technol. 2017, 31, 3943–3948. [Google Scholar] [CrossRef]
- Javier, C.; LeBlanc, J.; Shukla, A. Shock Response of Composite Materials Subjected to Aggressive Marine Environments. In Proceedings of the International Digital Imaging Correlation Society, Proceedings of the First Annual Conference, Philadelphia, PA, USA, 7–10 November 2016; Sutton, M., Reu, P.L., Eds.; Springer: Cham, Swizterland, 2017; pp. 169–171. [Google Scholar]
- Macháčková, A.; Kocich, R.; Bojko, M.; Klečková, Z. Numerical Analysis of Secondary Heat Exchanger Designed for CHP Units with Microturbine. Int. J. Heat Mass Transf. 2015, 83, 487–498. [Google Scholar] [CrossRef]
- Kocich, R.; Bojko, M.; Macháčková, A.; Klečková, Z. Numerical Analysis of the Tubular Heat Exchanger Designed for Co-Generating Units on the Basis of Microturbines. Int. J. Heat Mass Transf. 2012, 55, 5336–5342. [Google Scholar] [CrossRef]
- Macháčková, A.; Kocich, R.; Bojko, M.; Kunčická, L.; Polko, K. Numerical and Experimental Investigation of Flue Gases Heat Recovery via Condensing Heat Exchanger. Int. J. Heat Mass Transf. 2018, 124, 1321–1333. [Google Scholar] [CrossRef]
- Greger, M.; Kocich, R.; Kander, L.; Jonsta, P. Nanostructured Titanium for Dental Applications. In Proceedings of the Metal 2010: 19th International Metallurgical and Materials Conference, Roznov pod Radhostem, Czech Republic, 18–20 May 2020; Tanger Ltd.: Ostrava, Czech Republic, 2010; pp. 182–186. [Google Scholar]
- Zach, L.; Kunčická, L.; Růžička, P.; Kocich, R. Design, Analysis and Verification of a Knee Joint Oncological Prosthesis Finite Element Model. Comput. Biol. Med. 2014, 54, 53–60. [Google Scholar] [CrossRef]
- Malygin, G.A. Strength and Plasticity of Nanocrystalline Metals with a Bimodal Grain Structure. Phys. Solid State 2008, 50, 1032–1038. [Google Scholar] [CrossRef]
- Kudrya, A.V.; Sokolovskaya, E.A.; Ngo, K.N.; Kaikibaeva, A.S. Relation between the Nonuniformity of the Properties and the Structure of Large Forgings. Russ. Metall. 2018, 2018, 589–592. [Google Scholar] [CrossRef]
- Panin, V.E.; Moiseenko, D.D.; Elsukova, T.F. Multiscale Model of Deformed Polycrystals. Hall-Petch Problem. Phys. Mesomech. 2014, 17, 1–14. [Google Scholar] [CrossRef]
- Trusov, P.V.; Gribov, D.S. The Three-Level Elastoviscoplastic Model and Its Application to Describing Complex Cyclic Loading of Materials with Different Stacking Fault Energies. Materials 2022, 15, 760. [Google Scholar] [CrossRef] [PubMed]
- Haddou, H.; Gaudin, C.; Feaugas, X. Stacking Fault Energy (s.f.e.) and Grain Size Effects (d) on the Tensile Behaviour of f.c.c. Polycrystalline Alloys at 300 K: Back Stress and Effective Stress Evolutions. J. Phys. IV 2001, 11, Pr4–Pr283. [Google Scholar] [CrossRef]
- Wang, H.; Lu, C.; Tieu, K. Crystal Plasticity Modelling of Microbands in a Rolled Aluminium Single Crystal. Materialia 2019, 8, 100488. [Google Scholar] [CrossRef]
- Jagatramka, R.; Daly, M. The Competition Between Deformation Twinning and Dislocation Slip in Deformed Face-Centered Cubic Metals. JOM 2022, 74, 3799–3810. [Google Scholar] [CrossRef]
- Chen, B.; Zhu, L.; Xin, Y.; Lei, J. Grain Rotation in Plastic Deformation. Quantum Beam Sci. 2019, 3, 17. [Google Scholar] [CrossRef]
- Panin, V.E.; Egorushkin, V.E.; Elsukova, T.F. Physical Mesomechanics of Grain Boundary Sliding in a Deformable Polycrystal. Phys. Mesomech. 2013, 16, 1–8. [Google Scholar] [CrossRef]
- Cao, Y.; Ni, S.; Liao, X.; Song, M.; Zhu, Y. Structural Evolutions of Metallic Materials Processed by Severe Plastic Deformation. Mater. Sci. Eng. R Rep. 2018, 133, 1–59. [Google Scholar] [CrossRef]
- Verlinden, B.; Driver, J.; Samajdar, I.; Doherty, R.D. Thermo-Mechanical Processing of Metallic Materials; Elsevier: Amsterdam, The Netherlands, 2007; ISBN 9780080444970. [Google Scholar]
- Liao, B.; Cao, L.; Wu, X.; Zou, Y.; Huang, G.; Rometsch, P.; Couper, M.; Liu, Q. Effect of Heat Treatment Condition on the Flow Behavior and Recrystallization Mechanisms of Aluminum Alloy 7055. Materials 2019, 12, 311. [Google Scholar] [CrossRef]
- Borodin, E.N.; Mayer, A.E. A Simple Mechanical Model for Grain Boundary Sliding in Nanocrystalline Metals. Mater. Sci. Eng. A 2012, 532, 245–248. [Google Scholar] [CrossRef]
- Trusov, P.V.; Shveykin, A.I.; Sharifullina, E.R.; Kondratev, N.S. Model of Polycrystalline Inelastic Deformation with Grain Boundary Sliding Description. Adv. Mater. Res. 2014, 1040, 86–91. [Google Scholar]
- Mohammadi, A.; Enikeev, N.A.; Murashkin, M.Y.; Arita, M.; Edalati, K. Examination of Inverse Hall-Petch Relation in Nanostructured Aluminum Alloys by Ultra-Severe Plastic Deformation. J. Mater. Sci. Technol. 2021, 91, 78–89. [Google Scholar] [CrossRef]
- Kocich, R.; Lukáč, P. SPD Processes–Methods for Mechanical Nanostructuring. In Handbook of Mechanical Nanostructuring; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2015; pp. 235–262. [Google Scholar]
- Chuvil’deev, V.N.; Myshlyaev, M.M.; Nokhrin, A.V.; Kopylov, V.I.; Lopatin, Y.G.; Pirozhnikova, O.E.; Piskunov, A.V.; Semenycheva, A.V.; Bobrov, A.A. Effect of the Severe Plastic Deformation Temperature on the Diffusion Properties of the Grain Boundaries in Ultrafine-Grained Metals. Russ. Metall. 2017, 2017, 413–425. [Google Scholar] [CrossRef]
- Purcek, G.; Saray, O.; Nagimov, M.I.; Nazarov, A.A.; Safarov, I.M.; Danilenko, V.N.; Valiakhmetov, O.R.; Mulyukov, R.R. Microstructure and Mechanical Behavior of UFG Copper Processed by ECAP Following Different Processing Regimes. Philos. Mag. 2012, 92, 690–704. [Google Scholar] [CrossRef]
- Stolyarov, V.V.; Ermolenko, A.S.; Gunderov, D.; Popov, A.G.; Raab, G.I.; Puzanova, T.Z.; Valiev, R.; Gaviko, V.S.; Belozerov, E.V. Equal-Channel Angular Pressing, Microstructure and Hysteresis Properties of Ultrafine–Grained Pr20Fe73.5B5Cu1.5–Alloy. Mater. Sci. Forum 2001, 373–376, 265–268. [Google Scholar] [CrossRef]
- Hlaváč, L.M.; Kocich, R.; Gembalová, L.; Jonšta, P.; Hlaváčová, I.M. AWJ Cutting of Copper Processed by ECAP. Int. J. Adv. Manuf. Technol. 2016, 86, 885–894. [Google Scholar] [CrossRef]
- Kocich, R.; Szurman, I.; Kursa, M.; Fiala, J. Investigation of Influence of Preparation and Heat Treatment on Deformation Behaviour of the Alloy NiTi after ECAE. Mater. Sci. Eng. A 2009, 512, 100–104. [Google Scholar] [CrossRef]
- Kocich, R.; Greger, M.; Macháčková, A. Finite Element Investigation of Influence of Selected Factors on ECAP Process. In Proceedings of the Metal 2010: 19th International Metallurgical and Materials Conference, Roznov pod Radhostem, Czech Republic, 18–20 May 2020; Tanger Ltd.: Ostrava, Czech Republic, 2010; pp. 166–171. [Google Scholar]
- Kocich, R.; Kursa, M.; Szurman, I.; Dlouhý, A. The Influence of Imposed Strain on the Development of Microstructure and Transformation Characteristics of Ni–Ti Shape Memory Alloys. J. Alloys Compd. 2011, 509, 2716–2722. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Drápala, J.; Andreyachshenko, V.A. FEM Simulations and Comparison of the Ecap and ECAP-PBP Influence on Ti6Al4V Alloy’s Deformation Behaviour. In Proceedings of the Metal 2013: 22nd International Metallurgical and Materials Conference, Brno, Czech Republic, 15–17 March 2013; Tanger Ltd.: Ostrava, Czech Republic, 2013; pp. 391–396. [Google Scholar]
- Kocich, R.; Kunčická, L.; Král, P.; Macháčková, A. Sub-Structure and Mechanical Properties of Twist Channel Angular Pressed Aluminium. Mater. Charact. 2016, 119, 75–83. [Google Scholar] [CrossRef]
- Kocich, R.; Macháčková, A.; Kunčická, L. Twist Channel Multi-Angular Pressing (TCMAP) as a New SPD Process: Numerical and Experimental Study. Mater. Sci. Eng. A 2014, 612, 445–455. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Král, P.; Pohludka, M.; Marek, M. Effect of Strain Path on Severely Deformed Aluminium. Mater. Lett. 2016, 180, 280–283. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L.; Macháčková, A. Twist Channel Multi-Angular Pressing ( TCMAP ) as a Method for Increasing the Efficiency of SPD. IOP Conf. Ser. Mater. Sci. Eng. 2014, 63, 012006. [Google Scholar] [CrossRef]
- Naizabekov, A.B.; Andreyachshenko, V.A.; Kocich, R. Study of Deformation Behavior, Structure and Mechanical Properties of the AlSiMnFe Alloy during ECAP-PBP. Micron 2013, 44, 210–217. [Google Scholar] [CrossRef]
- Kocich, R. Deformation Behavior of Al/Cu Clad Composite During Twist Channel Angular Pressing. Materials 2020, 13, 4047. [Google Scholar] [CrossRef]
- Kocich, R.; Macháčková, A.; Andreyachshenko, V.A. A Study of Plastic Deformation Behaviour of Ti Alloy during Equal Channel Angular Pressing with Partial Back Pressure. Comput. Mater. Sci. 2015, 101, 233–241. [Google Scholar] [CrossRef]
- Naizabekov, A.B.; Andreyachshenko, V.A.; Kliber, J.; Kocich, R. Tool for Realization Severe Plastic Deformation. In Proceedings of the Metals 2013: 22nd International Metallurgical and Materials Conference, Brno, Czech Republic, 15–17 May 2013; Tanger Ltd.: Ostrava, Czech Republic, 2013; pp. 317–321. [Google Scholar]
- Straumal, B.; Korneva, a.; Zięba, P. Phase Transitions in Metallic Alloys Driven by the High Pressure Torsion. Arch. Civ. Mech. Eng. 2014, 14, 242–249. [Google Scholar] [CrossRef]
- Straumal, B.B.; Pontikis, V.; Kilmametov, A.R.; Mazilkin, A.A.; Dobatkin, S.V.; Baretzky, B. Competition between Precipitation and Dissolution in Cu–Ag Alloys under High Pressure Torsion. Acta Mater. 2017, 122, 60–71. [Google Scholar] [CrossRef]
- You, G.L.; Ho, N.J.; Kao, P.W. Aluminum Based in Situ Nanocomposite Produced from Al-Mg-CuO Powder Mixture by Using Friction Stir Processing. Mater. Lett. 2013, 100, 219–222. [Google Scholar] [CrossRef]
- Kocich, R.; Macháčková, A.; Fojtík, F. Comparison of Strain and Stress Conditions in Conventional and ARB Rolling Processes. Int. J. Mech. Sci. 2012, 64, 54–61. [Google Scholar] [CrossRef]
- Sun, Y.F.; Tsuji, N.; Fujii, H.; Li, F.S. Cu/Zr Nanoscaled Multi-Stacks Fabricated by Accumulative Roll Bonding. J. Alloys Compd. 2010, 504, S443–S447. [Google Scholar] [CrossRef]
- Kozhevnikov, A.V.; Skripalenko, M.M.; Kozhevnikova, I.A.; Skripalenko, M.N. Comparative Evaluation of the Kinematic Parameters at Symmetric and Asymmetric Cold Rolling of Strip Using Computer Simulation. CIS Iron Steel Rev. 2023, 25, 51–57. [Google Scholar] [CrossRef]
- Pustovoytov, D.; Pesin, A.; Tandon, P. Asymmetric (Hot, Warm, Cold, Cryo) Rolling of Light Alloys: A Review. Metals 2021, 11, 956. [Google Scholar] [CrossRef]
- Pesin, A.; Pustovoytov, D.; Lokotunina, N. Modeling of the Roll Wear and Material Damage during High-Ratio Differential Speed Rolling of Aluminium Alloy 7075. MATEC Web Conf. 2016, 80, 04006. [Google Scholar] [CrossRef]
- Yang, Q.; Zhang, D.; Peng, P.; Wei, G.; Zhang, J.; Jiang, B.; Pan, F. Asymmetric Extrusion Technology of Mg Alloy: A Review. Materials 2023, 16, 5255. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Ryukhtin, V.; Cullen, J.C.T.; Lavery, N.P. Study of Structure of Naturally Aged Aluminium after Twist Channel Angular Pressing. Mater. Charact. 2019, 152, 94–100. [Google Scholar] [CrossRef]
- Canelo-Yubero, D.; Kocich, R.; Hervoches, C.; Strunz, P.; Kunčická, L.; Krátká, L. Neutron Diffraction Study of Residual Stresses in a W–Ni–Co Heavy Alloy Processed by Rotary Swaging at Room and High Temperatures. Met. Mater. Int. 2022, 28, 919–930. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Németh, G.; Dvořák, K.; Pagáč, M. Effect of Post Process Shear Straining on Structure and Mechanical Properties of 316 L Stainless Steel Manufactured via Powder Bed Fusion. Addit. Manuf. 2022, 59, 103128. [Google Scholar] [CrossRef]
- Kocich, R.; Kursa, M.; Macháčková, A. FEA of Plastic Flow in AZ63 Alloy during ECAP Process. Acta Phys. Pol. A 2012, 122, 581–587. [Google Scholar] [CrossRef]
- Vinogradov, A.; Serebryany, V.N.; Dobatkin, S.V. Tailoring Microstructure and Properties of Fine Grained Magnesium Alloys by Severe Plastic Deformation. Adv. Eng. Mater. 2018, 20. [Google Scholar] [CrossRef]
- Mazilkin, A.A.; Abrosimova, G.E.; Protasova, S.G.; Straumal, B.B.; Schütz, G.; Dobatkin, S.V.; Bakai, A.S. Transmission Electron Microscopy Investigation of Boundaries between Amorphous “Grains” in Ni50Nb20Y30 Alloy. J. Mater. Sci. 2011, 46, 4336–4342. [Google Scholar] [CrossRef]
- Straumal, B.B.; Mazilkin, A.A.; Protasova, S.G.; Goll, D.; Baretzky, B.; Bakai, B.; Dobatkin, S.V. Formation of Two Amorphous Phases in the Ni60Nb18Y22 Alloy after High Pressure Torsion. Met. Mater. 2021, 49, 17–22. [Google Scholar] [CrossRef]
- Korznikova, G. Modifications de Structure et de Propriétés de Composés Intermétalliques Par Déformation Plastique Intense. Ann. Chim. Sci. Matériaux 2002, 27, 35–44. [Google Scholar] [CrossRef]
- Suru, M.G.; Lohan, N.M.; Pricop, B.; Spiridon, I.P.; Mihalache, E.; Comaneci, R.I.; Bujoreanu, L.G. Structural Effects of High-Temperature Plastic Deformation Process on Martensite Plate Morphology in a Fe-Mn-Si-Cr SMA. Int. J. Mater. Prod. Technol. 2015, 50, 276. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, J.; Besnard, C.; Kunčická, L.; Kocich, R.; Korsunsky, A.M. In Situ Neutron Diffraction Investigation of Texture-Dependent Shape Memory Effect in a near Equiatomic NiTi Alloy. Acta Mater. 2021, 202, 135–148. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R. Effect of Stacking Sequence on Mechanical Properties and Microstructural Features within Al/Cu Laminates. Materials 2023, 16, 6555. [Google Scholar] [CrossRef] [PubMed]
- Kunčická, L.; Lowe, T.C.; Davis, C.F.; Kocich, R.; Pohludka, M. Synthesis of an Al/Al2O3 Composite by Severe Plastic Deformation. Mater. Sci. Eng. A 2015, 646, 234–241. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L.; Benč, M. Development of Microstructure and Properties within Oxide Dispersion Strengthened Steel Directly Consolidated by Hot Rotary Swaging. Mater. Lett. 2023, 353, 135276. [Google Scholar] [CrossRef]
- Kocich, R.; Opěla, P.; Marek, M. Influence of Structure Development on Performance of Copper Composites Processed via Intensive Plastic Deformation. Materials 2023, 16, 4780. [Google Scholar] [CrossRef] [PubMed]
- Böhmermann, F.; Hasselbruch, H.; Herrmann, M.; Riemer, O.; Mehner, A.; Zoch, H.-W.; Kuhfuss, B. Dry Rotary Swaging–Approaches for Lubricant Free Process Design. Int. J. Precis. Eng. Manuf. Technol. 2015, 2, 325–331. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R. Effect of Activated Slip Systems on Dynamic Recrystallization during Rotary Swaging of Electro-Conductive Al-Cu Composites. Mater. Lett. 2022, 321, 10–13. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L. Development of Structure and Properties in Bimetallic Al/Cu Sandwich Composite during Cumulative Severe Plastic Deformation. J. Sandw. Struct. Mater. 2021, 23, 4252–4275. [Google Scholar] [CrossRef]
- Lukáč, P.; Kocich, R.; Greger, M.; Padalka, O.; Szaraz, Z. Microstructure of AZ31 and AZ61 Mg Alloys Prepared by Rolling and ECAP. Kov. Mater. Mater. 2007, 45, 115–120. [Google Scholar]
- Kuhfuss, B.; Moumi, E.; Piwek, V. Micro Rotary Swaging: Process Limitations and Attempts to Their Extension. Microsyst. Technol. 2008, 14, 1995–2000. [Google Scholar] [CrossRef]
- Şchiopu, V.; Luca, D. A New Net-Shape Plating Technology for Axisymmetric Metallic Parts Using Rotary Swaging. Int. J. Adv. Manuf. Technol. 2016, 85, 2471–2482. [Google Scholar] [CrossRef]
- Hao, Q.L.; Han, J.T.; Song, J.J.; Ji, S. Causes and Remedies for Some Physical Defects in Rotary Swaged Products. Adv. Mater. Res. 2014, 941–944, 1797–1801. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R. Optimizing Electric Conductivity of Innovative Al-Cu Laminated Composites via Thermomechanical Treatment. Mater. Des. 2022, 215, 110441. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R. Deformation Behaviour of Cu-Al Clad Composites Produced by Rotary Swaging. IOP Conf. Ser. Mater. Sci. Eng. 2018, 369, 012029. [Google Scholar] [CrossRef]
- Kunčická, L.; Kocich, R.; Strunz, P.; Macháčková, A. Texture and Residual Stress within Rotary Swaged Cu/Al Clad Composites. Mater. Lett. 2018, 230, 88–91. [Google Scholar] [CrossRef]
- Macháčková, A.; Krátká, L.; Petrmichl, R.; Kunčická, L.; Kocich, R. Affecting Structure Characteristics of Rotary Swaged Tungsten Heavy Alloy Via Variable Deformation Temperature. Materials 2019, 12, 4200. [Google Scholar] [CrossRef] [PubMed]
- Moumi, E.; Ishkina, S.; Kuhfuss, B.; Hochrainer, T.; Struss, A.; Hunkel, M. 2D-Simulation of Material Flow during Infeed Rotary Swaging Using Finite Element Method. Procedia Eng. 2014, 81, 2342–2347. [Google Scholar] [CrossRef]
- Panov, D.O.; Chernichenko, R.S.; Naumov, S.V.; Pertcev, A.S.; Stepanov, N.D.; Zherebtsov, S.V.; Salishchev, G.A. Excellent Strength-Toughness Synergy in Metastable Austenitic Stainless Steel Due to Gradient Structure Formation. Mater. Lett. 2021, 303, 130585. [Google Scholar] [CrossRef]
- Panov, D.; Chernichenko, R.; Kudryavtsev, E.; Klimenko, D.; Naumov, S.; Pertcev, A. Effect of Cold Swaging on the Bulk Gradient Structure Formation and Mechanical Properties of a 316-Type Austenitic Stainless Steel. Materials 2022, 15, 2468. [Google Scholar] [CrossRef]
- Kocich, R.; Fiala, J.; Szurman, I.; Macháčková, A.; Mihola, M. Twist-Channel Angular Pressing: Effect of the Strain Path on Grain Refinement and Mechanical Properties of Copper. J. Mater. Sci. 2011, 46, 7865–7876. [Google Scholar] [CrossRef]
- Kocich, R.; Greger, M.; Kursa, M.; Szurman, I.; Macháčková, A. Twist Channel Angular Pressing (TCAP) as a Method for Increasing the Efficiency of SPD. Mater. Sci. Eng. A 2010, 527, 6386–6392. [Google Scholar] [CrossRef]
- Kopeček, J.; Bajtošová, L.; Veřtát, P.; Šimek, D. (Sub)Structure Development in Gradually Swaged Electroconductive Bars. Materials 2023, 16, 5324. [Google Scholar] [CrossRef] [PubMed]
- Huang, A.H.; Wang, Y.F.; Wang, M.S.; Song, L.Y.; Li, Y.S.; Gao, L.; Huang, C.X.; Zhu, Y.T. Optimizing the Strength, Ductility and Electrical Conductivity of a Cu-Cr-Zr Alloy by Rotary Swaging and Aging Treatment. Mater. Sci. Eng. A 2019, 746, 211–216. [Google Scholar] [CrossRef]
- Martynenko, N.; Rybalchenko, O.; Bodyakova, A.; Prosvirnin, D.; Rybalchenko, G.; Morozov, M.; Yusupov, V.; Dobatkin, S. Effect of Rotary Swaging on the Structure, Mechanical Characteristics and Aging Behavior of Cu-0.5%Cr-0.08%Zr Alloy. Materials 2022, 16, 105. [Google Scholar] [CrossRef]
- Martynenko, N.S.; Bochvar, N.R.; Rybalchenko, O.V.; Prosvirnin, D.V.; Rybalchenko, G.V.; Kolmakov, A.G.; Morozov, M.M.; Yusupov, V.S.; Dobatkin, S.V. Increase in the Strength and Electrical Conductivity of a Cu–0.8Hf Alloy after Rotary Swaging and Subsequent Aging. Russ. Metall. 2023, 2023, 466–474. [Google Scholar] [CrossRef]
- Ouyang, Y.; Gan, X.; Li, Z.; Zhou, K.; Zhang, S.; Jiang, Y.; Zhang, X. Microstructure Evolution of a Cu-15Ni-8Sn-0.8Nb Alloy during Prior Deformation and Aging Treatment. Mater. Sci. Eng. A 2017, 704, 128–137. [Google Scholar] [CrossRef]
- Zhao, L.; Chen, L.; Luo, B.; Liang, Y.; Shi, J.; Zhang, S.; Lin, Z.; Shi, P.; Zheng, T.; Zhou, B.; et al. Low-Dislocation-Density Ultrafine Lamellar Structure Buffering Triples Ductility in Cu-8wt.%Sn Alloy Treated by Rotary Swaging and Appropriate Annealing. Mater. Sci. Eng. A 2024, 889, 145847. [Google Scholar] [CrossRef]
- Cao, M.; Wang, J.; Zhang, Q.; Huang, K. In Situ Observation of Deformation-Induced Spherical Grains in Semi-Solid State of C5191 Copper Alloy. Materials 2020, 13, 5496. [Google Scholar] [CrossRef]
- Abdulstaar, M.A.; El-Danaf, E.A.; Waluyo, N.S.; Wagner, L. Severe Plastic Deformation of Commercial Purity Aluminum by Rotary Swaging: Microstructure Evolution and Mechanical Properties. Mater. Sci. Eng. A 2013, 565, 351–358. [Google Scholar] [CrossRef]
- Yang, Y.; Nie, J.; Mao, Q.; Zhao, Y. Improving the Combination of Electrical Conductivity and Tensile Strength of Al 1070 by Rotary Swaging Deformation. Results Phys. 2019, 13, 102236. [Google Scholar] [CrossRef]
- Nokhrin, A.V.; Nagicheva, G.S.; Chuvil’deev, V.N.; Kopylov, V.I.; Bobrov, A.A.; Tabachkova, N.Y. Effect of Er, Si, Hf and Nb Additives on the Thermal Stability of Microstructure, Electrical Resistivity and Microhardness of Fine-Grained Aluminum Alloys of Al-0.25%Zr. Materials 2023, 16, 2114. [Google Scholar] [CrossRef]
- Bochvar, N.R.; Rybalchenko, O.V.; Leonova, N.P.; Tabachkova, N.Y.; Rybalchenko, G.V.; Rokhlin, L.L. Effect of Cold Plastic Deformation and Subsequent Aging on the Strength Properties of Al-Mg2Si Alloys with Combined (Sc + Zr) and (Sc + Hf) Additions. J. Alloys Compd. 2020, 821, 153426. [Google Scholar] [CrossRef]
- Jin, H.; Guan, R.; Tie, D. Mechanical and Conductive Performance of Aged 6xxx Aluminum Alloy during Rotary Swaging. Crystals 2022, 12, 530. [Google Scholar] [CrossRef]
- Lourenço, J.C.; Souza, L.P.; Silva, G.; Suzuki, P.A.; Robin, A.L.M.; Nunes, C.A.; Tomachuk, C.R.; Lourenço, J.C.; Souza, L.P.; Silva, G.; et al. Effects of Solidification, Rotary Swaging and Recrystallization on the Microstructure, Crystallographic Orientation and Electrochemical Behavior of an Al-4.5 Wt.% Cu Alloy. Int. J. Electrochem. Sci. 2021, 16, 211054. [Google Scholar] [CrossRef]
- Lin, C.-W.; Chen, K.-J.; Hung, F.-Y.; Lui, T.-S.; Chen, H.-P. Impact of Solid-Solution Treatment on Microstructural Characteristics and Formability of Rotary-Swaged 2024 Alloy Tubes. J. Mater. Res. Technol. 2019, 8, 3137–3148. [Google Scholar] [CrossRef]
- Rogachev, S.O.; Sundeev, R.V.; Andreev, V.A.; Andreev, N.V.; Tabachkova, N.Y.; Korotkova, N.O. The Microstructure and Conductivity of Copper–Aluminum Composites Prepared by Rotary Swaging. Phys. Met. Metallogr. 2022, 123, 1193–1200. [Google Scholar] [CrossRef]
- Wang, H.; Han, J.; Hao, Q. Fabrication of Laminated-Metal Composite Tubes by Multi-Billet Rotary Swaging Technique. Int. J. Adv. Manuf. Technol. 2015, 76, 713–719. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L.; Davis, C.F.; Lowe, T.C.; Szurman, I.; Macháčková, A. Deformation Behavior of Multilayered Al-Cu Clad Composite during Cold-Swaging. Mater. Des. 2016, 90, 379–388. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L.; Macháčková, A.; Šofer, M. Improvement of Mechanical and Electrical Properties of Rotary Swaged Al-Cu Clad Composites. Mater. Des. 2017, 123, 137–146. [Google Scholar] [CrossRef]
- Canelo-Yubero, D.; Kocich, R.; Šaroun, J.; Strunz, P. Residual Stress Distribution in a Copper-Aluminum Multifilament Composite Fabricated by Rotary Swaging. Materials 2023, 16, 2102. [Google Scholar] [CrossRef]
- Kocich, R.; Kunčická, L. Optimizing Structure and Properties of Al/Cu Laminated Conductors via Severe Shear Strain. J. Alloys Compd. 2023, 953, 170124. [Google Scholar] [CrossRef]
- Kocich, R. Optimizing Thermomechanical Processing of Bimetallic Laminates. Materials 2023, 16, 3480. [Google Scholar] [CrossRef]
- Kunčická, L.; Macháčková, A.; Krátká, L.; Kocich, R. Analysis of Deformation Behaviour and Residual Stress in Rotary Swaged Cu/Al Clad Composite Wires. Materials 2019, 12, 3462. [Google Scholar] [CrossRef]
- Kocich, R. Effects of Twist Channel Angular Pressing on Structure and Properties of Bimetallic Al/Cu Clad Composites. Mater. Des. 2020, 196, 109255. [Google Scholar] [CrossRef]
- Tian, W.; Zhang, F.; Han, S.; Chen, X.; Gao, P.; Zheng, K. Analysis of Microstructure and Properties in Cold Rotary Swaged Copper-Clad Magnesium Wires. Metals 2023, 13, 467. [Google Scholar] [CrossRef]
- Yu, Y.; Zhang, W. Tungsten Copper Composite Fabricated by Compound Plastic Deformation Technologies. Mater. Res. Express 2018, 5, 066551. [Google Scholar] [CrossRef]
- Lu, F.; Nie, J.; Ma, X.; Li, Y.; Jiang, Z.; Zhang, Y.; Zhao, Y.; Liu, X. Simultaneously Improving the Tensile Strength and Ductility of the AlNp/ Al Composites by the Particle’s Hierarchical Structure with Bimodal Distribution and Nano-Network. Mater. Sci. Eng. A 2020, 770, 138519. [Google Scholar] [CrossRef]
- Nie, J.; Lu, F.; Huang, Z.; Ma, X.; Zhou, H.; Chen, C.; Chen, X.; Yang, H.; Cao, Y.; Liu, X.; et al. Improving the High-Temperature Ductility of Al Composites by Tailoring the Nanoparticle Network. Materialia 2020, 9, 100523. [Google Scholar] [CrossRef]
- Barkov, L.A.; Mymrin, S.A.; Samodurova, M.N.; Dzhigun, N.S.; Latfulina, Y.S. Compressibility of Tungsten and Molybdenum Bars during Rotary Swaging and Rolling. Russ. Metall. 2015, 2015, 360–366. [Google Scholar] [CrossRef]
- Wang, F.-Z.; Zhang, H.; Ding, B.-J.; Zhu, R.-H. A Thermionic Tungsten Cathode Activated with Nanothoria and Prepared by Swaging Method. Mater. Sci. Eng. A 2002, 336, 59–63. [Google Scholar] [CrossRef]
- Rieth, M.; Hoffmann, A. Influence of Microstructure and Notch Fabrication on Impact Bending Properties of Tungsten Materials. Int. J. Refract. Met. Hard Mater. 2010, 28, 679–686. [Google Scholar] [CrossRef]
- Liu, R.; Xie, Z.M.; Fang, Q.F.; Zhang, T.; Wang, X.P.; Hao, T.; Liu, C.S.; Dai, Y. Nanostructured Yttria Dispersion-Strengthened Tungsten Synthesized by Sol–Gel Method. J. Alloys Compd. 2016, 657, 73–80. [Google Scholar] [CrossRef]
- Xing, H.; Sun, J. Electron Microscopy Study of Deformation Microstructure in Metastable β Titanium Alloy. Mater. Sci. Forum 2010, 654–656, 867–870. [Google Scholar] [CrossRef]
- Naydenkin, E.V.; Mishin, I.P.; Zabudchenko, O.V.; Lykova, O.N.; Manisheva, A.I. Structural-Phase State and Mechanical Properties of β Titanium Alloy Produced by Rotary Swaging with Subsequent Aging. J. Alloys Compd. 2023, 935, 167973. [Google Scholar] [CrossRef]
- Thirathipviwat, P.; Song, G.; Bednarcik, J.; Kühn, U.; Gemming, T.; Nielsch, K.; Han, J. Compositional Complexity Dependence of Dislocation Density and Mechanical Properties in High Entropy Alloy Systems. Prog. Nat. Sci. Mater. Int. 2020, 30, 545–551. [Google Scholar] [CrossRef]
- Silva, H.M.; Schneider, S.G.; Neto, C.M. Study of Nontoxic Aluminum and Vanadium-Free Titanium Alloys for Biomedical Applications. Mater. Sci. Eng. C 2004, 24, 679–682. [Google Scholar] [CrossRef]
- Hanada, S.; Masahashi, N.; Jung, T.K. Effect of Stress-Induced A″ Martensite on Young’s Modulus of β Ti-33.6Nb-4Sn Alloy. Mater. Sci. Eng. A 2013, 588, 403–410. [Google Scholar] [CrossRef]
- Jung, T.-K.; Semboshi, S.; Masahashi, N.; Hanada, S. Mechanical Properties and Microstructures of β Ti–25Nb–11Sn Ternary Alloy for Biomedical Applications. Mater. Sci. Eng. C 2013, 33, 1629–1635. [Google Scholar] [CrossRef]
- Baptista, C.A.R.; Schneider, S.; Taddei, E.; da Silva, H. Fatigue Behavior of Arc Melted Ti–13Nb–13Zr Alloy. Int. J. Fatigue 2004, 26, 967–973. [Google Scholar] [CrossRef]
- Thomasová, M.; Seiner, H.; Sedlák, P.; Frost, M.; Ševčík, M.; Szurman, I.; Kocich, R.; Drahokoupil, J.; Šittner, P.; Landa, M. Evolution of Macroscopic Elastic Moduli of Martensitic Polycrystalline NiTi and NiTiCu Shape Memory Alloys with Pseudoplastic Straining. Acta Mater. 2017, 123, 146–156. [Google Scholar] [CrossRef]
- Wang, Z.; Chen, J.; Kocich, R.; Tardif, S.; Dolbnya, I.P.; Kunčická, L.; Micha, J.-S.; Liogas, K.; Magdysyuk, O.V.; Szurman, I.; et al. Grain Structure Engineering of NiTi Shape Memory Alloys by Intensive Plastic Deformation. ACS Appl. Mater. Interfaces 2022, 14, 31396–31410. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, Y.; Liogas, K.; Chen, J.; Vaughan, G.B.M.; Kocich, R.; Kunčická, L.; Uzun, F.; You, Z.; Korsunsky, A.M. In Situ Synchrotron X-Ray Diffraction Analysis of Two-Way Shape Memory Effect in Nitinol. Mater. Sci. Eng. A 2023, 878, 145226. [Google Scholar] [CrossRef]
- Kocich, R. Design and Optimization of Induction Heating for Tungsten Heavy Alloy Prior to Rotary Swaging. Int. J. Refract. Met. Hard Mater. 2020, 93, 105353. [Google Scholar] [CrossRef]
- Strunz, P.; Kocich, R.; Canelo-Yubero, D.; Macháčková, A.; Beran, P.; Krátká, L. Texture and Differential Stress Development in W/Ni-Co Composite after Rotary Swaging. Materials 2020, 13, 2869. [Google Scholar] [CrossRef]
- Strunz, P.; Kunčická, L.; Beran, P.; Kocich, R.; Hervoches, C. Correlating Microstrain and Activated Slip Systems with Mechanical Properties within Rotary Swaged WNiCo Pseudoalloy. Materials 2020, 13, 208. [Google Scholar] [CrossRef] [PubMed]
- Panchal, A.; Reddy, K.V.; Azeem, P.A.; Nandy, T.K.; Singh, A.K. Microstructure, Texture, Tensile Flow and Work Hardening Behavior of Tungsten Heavy Alloys in Swaged and Swaged + Aged Conditions. Metallogr. Microstruct. Anal. 2020, 9, 438–456. [Google Scholar] [CrossRef]
- Durlu, N.; Caliskan, N.K.; Sakir, B. Effect of Swaging on Microstructure and Tensile Properties of W-Ni-Fe Alloys. Int. J. Refract. Met. Hard Mater. 2014, 42, 126–131. [Google Scholar] [CrossRef]
- Ravi Kiran, U.; Sambasiva Rao, A.; Sankaranarayana, M.; Nandy, T.K. Swaging and Heat Treatment Studies on Sintered 90W-6Ni-2Fe-2Co Tungsten Heavy Alloy. Int. J. Refract. Met. Hard Mater. 2012, 33, 113–121. [Google Scholar] [CrossRef]
- Ravi Kiran, U.; Rajavardhan, B.; Kumari, A.; Panchal, A.; Nageswara Rao, G.V.S.; Nandy, T.K. Effect of Swaging on Microstructure and Mechanical Properties of W-Ni-Co Alloys. Int. J. Refract. Met. Hard Mater. 2023, 116, 106333. [Google Scholar] [CrossRef]
- Sun, J.; Zhang, L.; Huang, Y.; Chen, B.; Fan, P.; Liu, W.; Ma, Y. Effect of Rotary Swaging on Microstructure Evolution and Adiabatic Shear Sensitivity of 90W–7Ni–3Fe Alloy under Dynamic Loading. Mater. Sci. Eng. A 2022, 860, 144333. [Google Scholar] [CrossRef]
- Ravi Kiran, U.; Panchal, A.; Sankaranarayana, M.; Nageswara Rao, G.V.S.; Nandy, T.K. Effect of Alloying Addition and Microstructural Parameters on Mechanical Properties of 93{%} Tungsten Heavy Alloys. Mater. Sci. Eng. A 2015, 640, 82–90. [Google Scholar] [CrossRef]
- Lin, Q.; Peng, B.; Liu, Z.Y. Four Dimensional Description of the Stress-Strain Relationship of Sintered and Swaged Tungsten Powders. Mater. Sci. Forum 2013, 762, 757–762. [Google Scholar] [CrossRef]
- Huang, Z.W.; Jin, S.B.; Zhou, H.; Li, Y.S.; Cao, Y.; Zhu, Y.T. Evolution of Twinning Systems and Variants during Sequential Twinning in Cryo-Rolled Titanium. Int. J. Plast. 2019, 112, 52–67. [Google Scholar] [CrossRef]
- Sun, J.L.; Trimby, P.W.; Yan, F.K.; Liao, X.Z.; Tao, N.R.; Wang, J.T. Grain Size Effect on Deformation Twinning Propensity in Ultrafine-Grained Hexagonal Close-Packed Titanium. Scr. Mater. 2013, 69, 428–431. [Google Scholar] [CrossRef]
- Wang, M.; Wang, Y.; Huang, A.; Gao, L.; Li, Y.; Huang, C. Promising Tensile and Fatigue Properties of Commercially Pure Titanium Processed by Rotary Swaging and Annealing Treatment. Materials 2018, 11, 2261. [Google Scholar] [CrossRef]
- Molina-Aldareguia, J.M.; Perez-Prado, M.T.; Valiev, R.Z.; Semenova, I.P.; Sabirov, I. High Strength Ultra-Fine Grained Titanium Produced via a Novel SPD Processing Route. Int. J. Mater. Form. 2010, 3, 407–410. [Google Scholar] [CrossRef]
- Sabirov, I.; Perez-Prado, M.T.; Molina-Aldareguia, J.M.; Semenova, I.P.; Salimgareeva, G.K.; Valiev, R.Z. Anisotropy of Mechanical Properties in High-Strength Ultra-Fine-Grained Pure Ti Processed via a Complex Severe Plastic Deformation Route. Scr. Mater. 2011, 64, 69–72. [Google Scholar] [CrossRef]
- Chuvil’deev, V.N.; Kopylov, V.I.; Nokhrin, A.V.; Tryaev, P.V.; Tabachkova, N.Y.; Chegurov, M.K.; Kozlova, N.A.; Mikhaylov, A.S.; Ershova, A.V.; Grayznov, M.Y.; et al. Effect of Severe Plastic Deformation Realized by Rotary Swaging on the Mechanical Properties and Corrosion Resistance of Near-α-Titanium Alloy Ti-2.5Al-2.6Zr. J. Alloys Compd. 2019, 785, 1233–1244. [Google Scholar] [CrossRef]
- Dyakonov, G.S.; Yakovleva, T.V.; Mironov, S.Y.; Stotskiy, A.G.; Modina, I.M.; Semenova, I.P. Microstructure of the Advanced Titanium Alloy VT8M-1 Subjected to Rotary Swaging. Materials 2023, 16, 6851. [Google Scholar] [CrossRef]
- Wei, K.; Hu, R.; Yin, D.; Xiao, L.; Pang, S.; Cao, Y.; Zhou, H.; Zhao, Y.; Zhu, Y. Grain Size Effect on Tensile Properties and Slip Systems of Pure Magnesium. Acta Mater. 2021, 206, 116604. [Google Scholar] [CrossRef]
- Greger, M.; Kocich, R. Superplasticity Properties of Magnesium Alloys. In Recent Advances in Mechatronics; Jablonski, R., Turkowski, M., Szewczyk, R., Eds.; Springer: Berlin/Heidelberg, Germany, 2007; pp. 421–425. [Google Scholar]
- Li, B.; Chen, H.; Ke, X.; Wei, G.; Yang, Q. Improving the Mechanical Properties of Mg-5Al-2Ca-1Mn-0.5Zn Alloy through Rotary Swaging. Materials 2023, 16, 4489. [Google Scholar] [CrossRef]
- Yang, Y.; Chen, X.; Nie, J.; Wei, K.; Mao, Q.; Lu, F.; Zhao, Y. Achieving Ultra-Strong Magnesium–Lithium Alloys by Low-Strain Rotary Swaging. Mater. Res. Lett. 2021, 9, 255–262. [Google Scholar] [CrossRef]
- Zhou, G.; Yang, Y.; Luo, Y.; Li, Q.; Luo, Q.; Zhang, Y.; Jiang, B.; Peng, X.; Pan, F. Synergistic Improvement of Strength and Plasticity of Mg-6Li-3Al-1Sn Alloy by Microstructure Regulation via Rotary Swaging. Mater. Res. Lett. 2023, 11, 1031–1039. [Google Scholar] [CrossRef]
- Wan, Y.; Tang, B.; Gao, Y.; Tang, L.; Sha, G.; Zhang, B.; Liang, N.; Liu, C.; Jiang, S.; Chen, Z.; et al. Bulk Nanocrystalline High-Strength Magnesium Alloys Prepared via Rotary Swaging. Acta Mater. 2020, 200, 274–286. [Google Scholar] [CrossRef]
- Chen, X.; Liu, C.; Jiang, S.; Chen, Z.; Wan, Y. Effect of Yttrium on Nanocrystallization of Magnesium Alloys during Cold Rotary Swaging. Mater. Charact. 2022, 184, 111696. [Google Scholar] [CrossRef]
- Wang, C.; Yu, Z.; Cui, Y.; Yu, S.; Ma, X.; Liu, H. Effect of Hot Rotary Swaging and Subsequent Annealing on Microstructure and Mechanical Properties of Magnesium Alloy WE43. Met. Sci. Heat Treat. 2019, 60, 777–782. [Google Scholar] [CrossRef]
- Wang, J.; Bao, Z.; Wu, C.; Zhang, S.; Wang, N.; Wang, Q.; Yi, Z. Progress in Partially Degradable Titanium-Magnesium Composites Used as Biomedical Implants. Front. Bioeng. Biotechnol. 2022, 10, 996195. [Google Scholar] [CrossRef]
- Chen, X.; Liu, C.; Wan, Y.; Jiang, S.; Han, X.; Chen, Z. Formation of Nanocrystalline AZ31B Mg Alloys via Cryogenic Rotary Swaging. J. Magnes. Alloy. 2023, 11, 1580–1591. [Google Scholar] [CrossRef]
- Martynenko, N.; Anisimova, N.; Rybalchenko, G.; Rybalchenko, O.; Serebryany, V.; Zheleznyi, M.; Shinkareva, M.; Gorbenko, A.; Temralieva, D.; Lukyanova, E.; et al. Effect of Rotary Swaging on Mechanical and Operational Properties of Zn–1%Mg and Zn–1%Mg–0.1%Ca Alloys. Metals 2023, 13, 1386. [Google Scholar] [CrossRef]
- Rogachev, S.O.; Andreev, V.A.; Gorshenkov, M.V.; Ten, D.V.; Kuznetsova, A.S.; Shcherbakov, A.B. Rotary Forging to Improve the Strength Properties of the Zr–2.5% Nb Alloy. Phys. Met. Metallogr. 2022, 123, 939–944. [Google Scholar] [CrossRef]
- Ashida, M.; Morita, M.; Tsutsumi, Y.; Nomura, N.; Doi, H.; Chen, P.; Hanawa, T. Effects of Cold Swaging on Mechanical Properties and Magnetic Susceptibility of the Zr–1Mo Alloy. Metals 2018, 8, 454. [Google Scholar] [CrossRef]








Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kunčická, L. Structural Phenomena Introduced by Rotary Swaging: A Review. Materials 2024, 17, 466. https://doi.org/10.3390/ma17020466
Kunčická L. Structural Phenomena Introduced by Rotary Swaging: A Review. Materials. 2024; 17(2):466. https://doi.org/10.3390/ma17020466
Chicago/Turabian StyleKunčická, Lenka. 2024. "Structural Phenomena Introduced by Rotary Swaging: A Review" Materials 17, no. 2: 466. https://doi.org/10.3390/ma17020466
APA StyleKunčická, L. (2024). Structural Phenomena Introduced by Rotary Swaging: A Review. Materials, 17(2), 466. https://doi.org/10.3390/ma17020466





