Optimization and Modelling the Mechanical Performance of Date Palm Fiber-Reinforced Concrete Incorporating Powdered Activation Carbon Using Response Surface Methodology
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Mix Proportioning with RSM
2.3. Sample Preparations and Experimental Methods
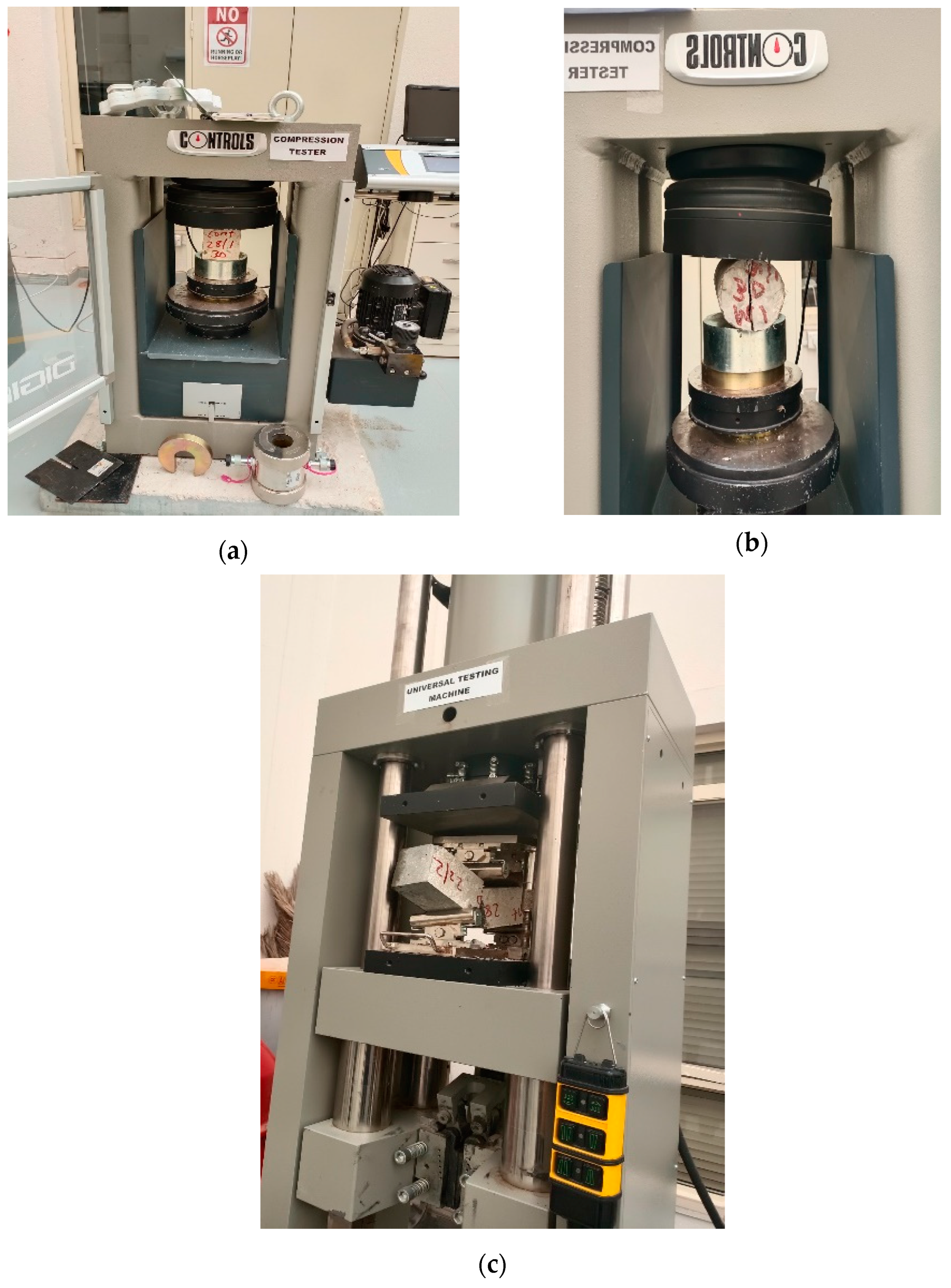
2.4. Experimental Methods
3. Results and Discussion
3.1. Analysis of Variance for RSM Models
3.1.1. Analysis of Variance for Slump and Density
3.1.2. Analysis of Variance for Compressive Strength Models
3.1.3. Analysis of Variance for Split Tensile Strength Models
3.1.4. Analysis of Variance for Flexural Strengths and Water Absorption
3.2. Diagnostic Plots for All the Models
3.3. Influence of DPF and PAC on the Fresh Properties of DPFRC
3.4. Influence of DPF and PAC on the Hardened Properties of DPFRC
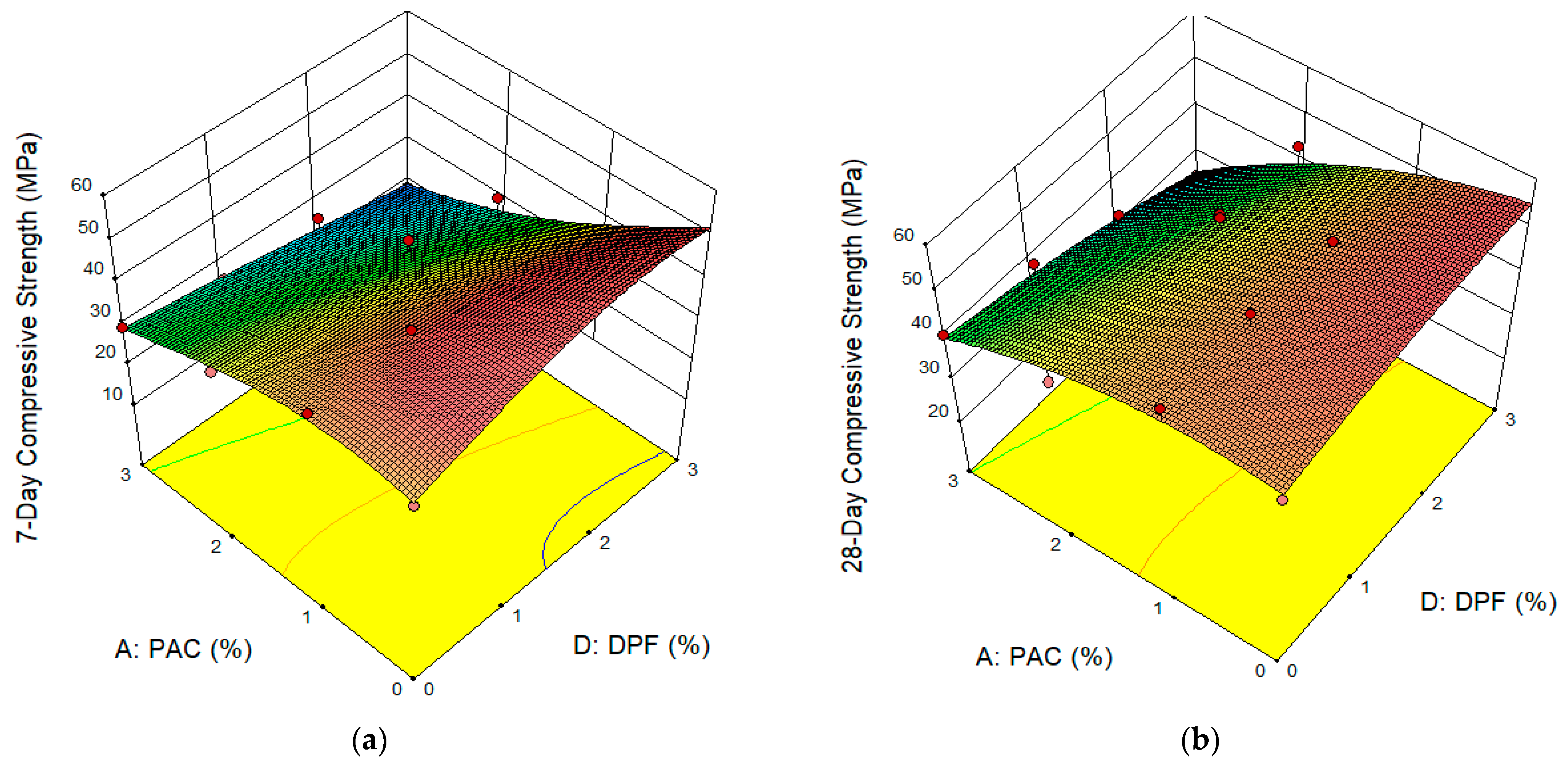
3.4.1. Compressive Strength of DPFRC
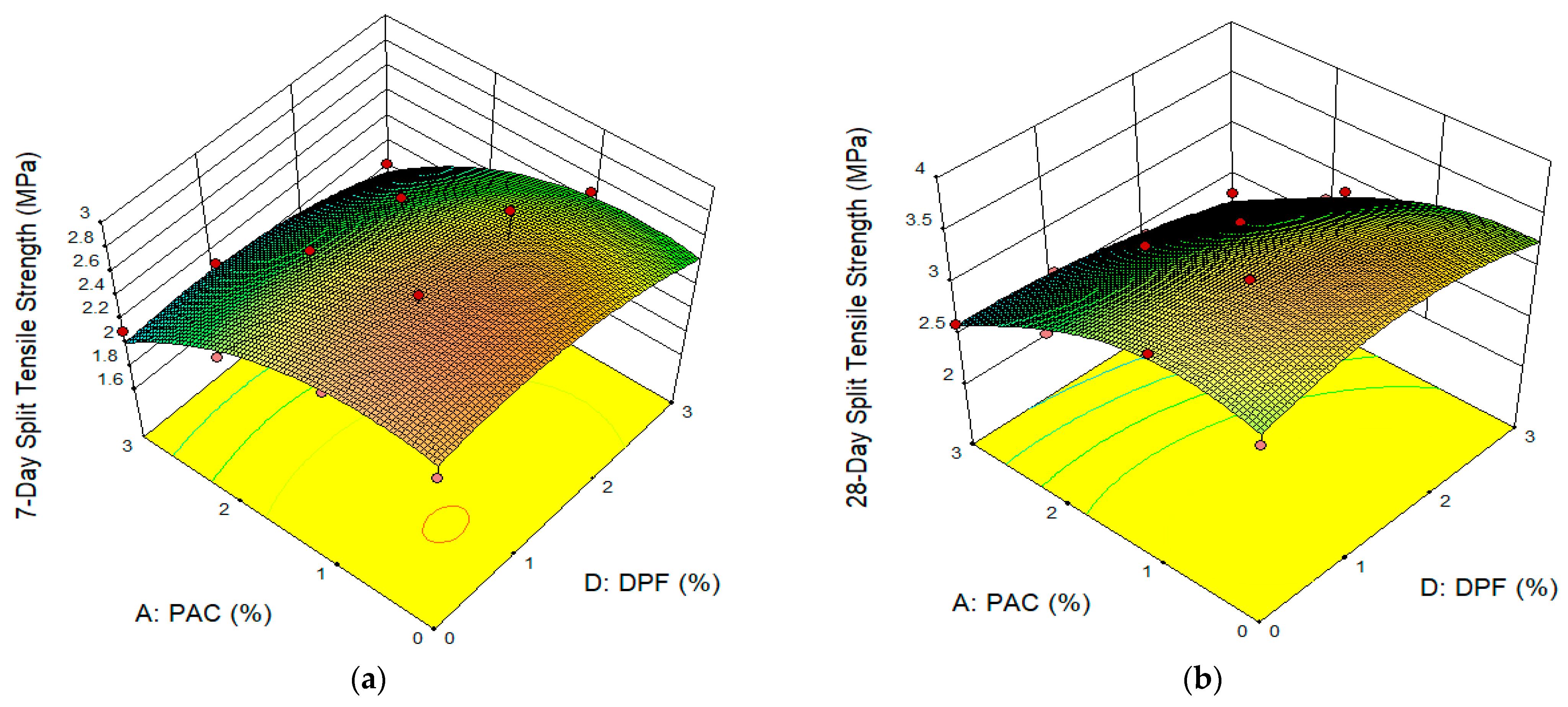
3.4.2. Split Tensile Strength of DPFRC
3.4.3. Flexural Strength of DPFRC
3.4.4. Water Absorption of DPFRC
3.5. Multi-Objective Optimization
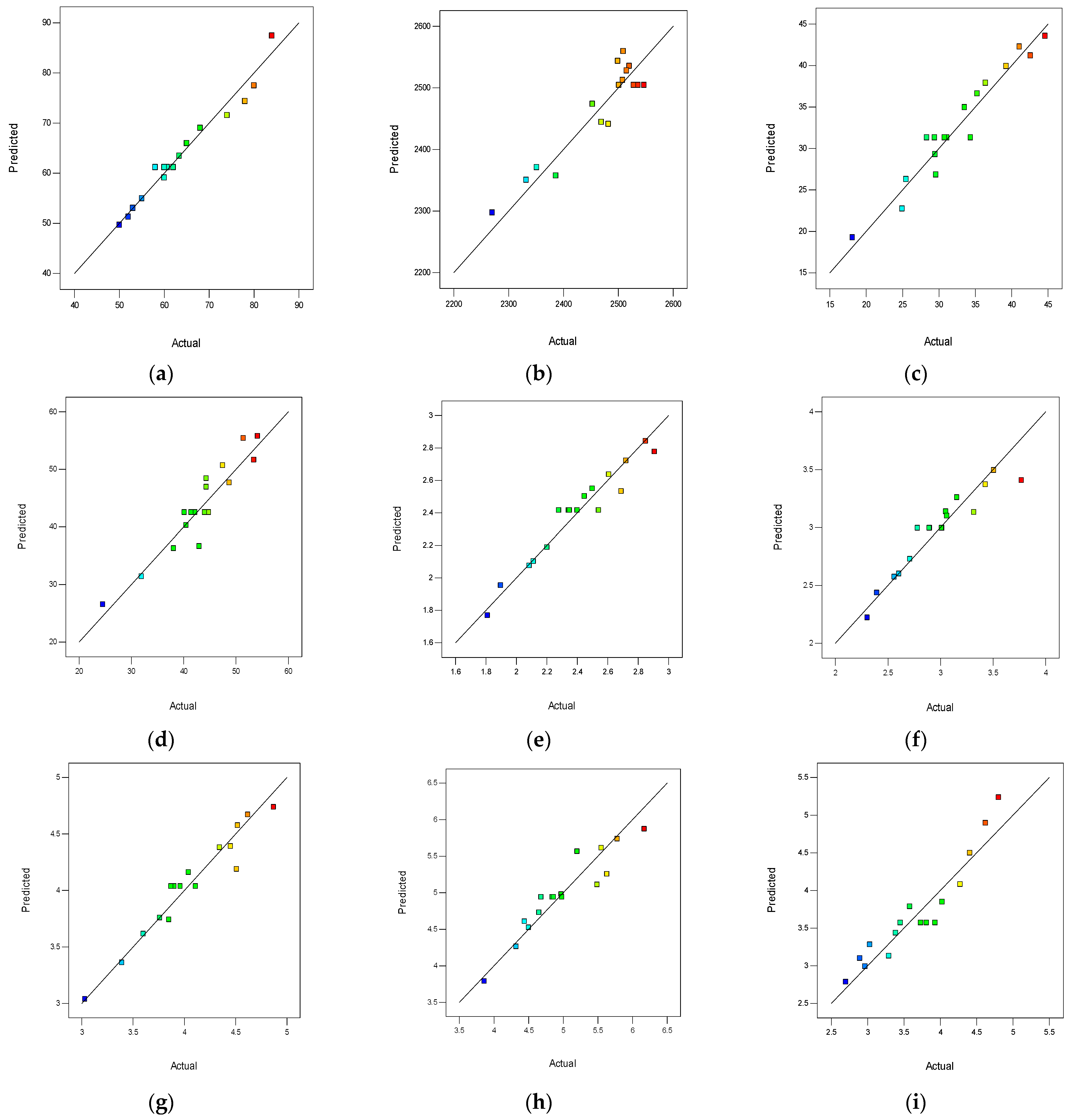
3.6. Model Validations
4. Conclusions
- (1)
- Adding both PAC and DPF led to a reduction in workability (slump) of the DPFRC. Furthermore, DPF addition reduced the density of the concrete, whereas up to 2% PAC addition enhanced the concrete’s density.
- (2)
- The combinations of 1 to 3% DPF with up to 2% PAC resulted in improvement in the compressive, split tensile and flexural strengths of the DPFRC. The combination of 1 to 3% DPF with 3% PAC yielded the lowest mechanical strengths.
- (3)
- The DPFRC’s strengths were increased, and the amount of water absorption was minimized by adding 2 wt% of PAC.
- (4)
- The models developed to estimate the slump, density, strength, and water absorption of DPFRC were highly significant with excellent correlations and predictive power. When experimentally validated, all the models exhibited average errors that were lower than 5.5%.
- (5)
- From the multi-objective optimization results, the highest slump, compressive strength, flexural strength and split tensile strength and lowest water absorption rate were achieved using a combination of 0.93 wt% of DPF and 0.37 wt% of PAC as an additive. According to the results of the multi-objective optimization, the optimization’s outcome had a 91% desirability.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Al-Oqla, F.M.; Sapuan, S. Natural fiber reinforced polymer composites in industrial applications: Feasibility of date palm fibers for sustainable automotive industry. J. Clean. Prod. 2014, 66, 347–354. [Google Scholar] [CrossRef]
- Makul, N. Advanced smart concrete-A review of current progress, benefits and challenges. J. Clean. Prod. 2020, 274, 122899. [Google Scholar] [CrossRef]
- Gagg, C.R. Cement and concrete as an engineering material: An historic appraisal and case study analysis. Eng. Fail. Anal. 2014, 40, 114–140. [Google Scholar] [CrossRef]
- Ibrahim, Y.E.; Adamu, M.; Marouf, M.L.; Ahmed, O.S.; Drmosh, Q.; Malik, M.A. Mechanical Performance of Date-Palm-Fiber-Reinforced Concrete Containing Silica Fume. Buildings 2022, 12, 1642. [Google Scholar] [CrossRef]
- Ozerkan, N.G.; Ahsan, B.; Mansour, S.; Iyengar, S.R. Mechanical performance and durability of treated palm fiber reinforced mortars. Int. J. Sustain. Built Environ. 2013, 2, 131–142. [Google Scholar] [CrossRef]
- Bentur, A.; Mindess, S. Fibre Reinforced Cementitious Composites; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Shen, L.; Yao, X.; Di Luzio, G.; Jiang, M.; Han, Y. Mix optimization of hybrid steel and polypropylene fiber-reinforced concrete for anti-thermal spalling. J. Build. Eng. 2023, 63, 105409. [Google Scholar] [CrossRef]
- Li, Z.; Wang, X.; Wang, L. Properties of hemp fibre reinforced concrete composites. Compos. Part A Appl. Sci. Manuf. 2006, 37, 497–505. [Google Scholar] [CrossRef]
- Kalia, S.; Avérous, L.; Njuguna, J.; Dufresne, A.; Cherian, B.M. Natural fibers, bio-and nanocomposites. Int. J. Polym. Sci. 2011, 2011, 735932. [Google Scholar] [CrossRef]
- Faruk, O.; Bledzki, A.K.; Fink, H.-P.; Sain, M. Biocomposites reinforced with natural fibers: 2000–2010. Prog. Polym. Sci. 2012, 37, 1552–1596. [Google Scholar] [CrossRef]
- Nordin, N.I.A.A.; Ariffin, H.; Andou, Y.; Hassan, M.A.; Shirai, Y.; Nishida, H.; Yunus, W.M.Z.W.; Karuppuchamy, S.; Ibrahim, N.A. Modification of oil palm mesocarp fiber characteristics using superheated steam treatment. Molecules 2013, 18, 9132–9146. [Google Scholar] [CrossRef]
- Bachtiar, D.; Sapuan, S.; Khalina, A.; Zainudin, E.; Dahlan, K. Flexural and impact properties of chemically treated sugar palm fiber reinforced high impact polystyrene composites. Fibers Polym. 2012, 13, 894–898. [Google Scholar] [CrossRef]
- Aziz, S.H.; Ansell, M.P. The effect of alkalization and fibre alignment on the mechanical and thermal properties of kenaf and hemp bast fibre composites: Part 1—Polyester resin matrix. Compos. Sci. Technol. 2004, 64, 1219–1230. [Google Scholar] [CrossRef]
- Adamu, M.; Alanazi, F.; Ibrahim, Y.E.; Alanazi, H.; Khed, V.C. A Comprehensive Review on Sustainable Natural Fiber in Cementitious Composites: The Date Palm Fiber Case. Sustainability 2022, 14, 6691. [Google Scholar] [CrossRef]
- Bamaga, S. A Review on the Utilization of Date Palm Fibers as Inclusion in Concrete and Mortar. Fibers 2022, 10, 35. [Google Scholar] [CrossRef]
- Lahouioui, M.; Ben Arfi, R.; Fois, M.; Ibos, L.; Ghorbal, A. Investigation of fiber surface treatment effect on thermal, mechanical and acoustical properties of date palm fiber-reinforced cementitious composites. Waste Biomass Valorization 2020, 11, 4441–4455. [Google Scholar] [CrossRef]
- Adamu, M.; Marouf, M.L.; Ibrahim, Y.E.; Ahmed, O.S.; Alanazi, H.; Marouf, A.L. Modelling and Optimization of the Mechanical Properties of Date Fiber Reinforced Concrete Containing Silica Fume using Response Surface Methodology. Case Stud. Constr. Mater. 2022, 17, e01633. [Google Scholar]
- Kriker, A.; Debicki, G.; Bali, A.; Khenfer, M.; Chabannet, M. Mechanical properties of date palm fibres and concrete reinforced with date palm fibres in hot-dry climate. Cem. Concr. Compos. 2005, 27, 554–564. [Google Scholar] [CrossRef]
- Khelifa, H.; Bezazi, A.; Boumediri, H.; del Pino, G.G.; Reis, P.N.; Scarpa, F.; Dufresne, A. Mechanical characterization of mortar reinforced by date palm mesh fibers: Experimental and statistical analysis. Constr. Build. Mater. 2021, 300, 124067. [Google Scholar] [CrossRef]
- Mahoutian, M.; Lubell, A.S.; Bindiganavile, V.S. Effect of powdered activated carbon on the air void characteristics of concrete containing fly ash. Constr. Build. Mater. 2015, 80, 84–91. [Google Scholar] [CrossRef]
- Moses, M.T.; Thomas, L.B.; Scaria, J.; Dev, G.V. Prospective benefits of using activated carbon in cement composites-An overview. Technology 2019, 10, 946–953. [Google Scholar]
- Morgan, B.; Dumbauld, G. Use of activated charcoal in cement to combat effects of contamination by drilling muds. J. Pet. Technol. 1952, 4, 225–232. [Google Scholar] [CrossRef]
- Justo-Reinoso, I.; Srubar, W.V., III; Caicedo-Ramirez, A.; Hernandez, M.T. Fine aggregate substitution by granular activated carbon can improve physical and mechanical properties of cement mortars. Constr. Build. Mater. 2018, 164, 750–759. [Google Scholar] [CrossRef]
- Horgnies, M.; Dubois-Brugger, I.; Gartner, E. NOx de-pollution by hardened concrete and the influence of activated charcoal additions. Cem. Concr. Res. 2012, 42, 1348–1355. [Google Scholar] [CrossRef]
- Krou, N.; Batonneau-Gener, I.; Belin, T.; Mignard, S.; Horgnies, M.; Dubois-Brugger, I. Mechanisms of NOx entrapment into hydrated cement paste containing activated carbon—Influences of the temperature and carbonation. Cem. Concr. Res. 2013, 53, 51–58. [Google Scholar] [CrossRef]
- Erşan, Y.Ç.; Da Silva, F.B.; Boon, N.; Verstraete, W.; De Belie, N. Screening of bacteria and concrete compatible protection materials. Constr. Build. Mater. 2015, 88, 196–203. [Google Scholar] [CrossRef]
- Resheidat, M.; Al-Araji, N.; Ghanma, M. Effect of charcoal on the porosity and the properties of concrete. In Proceedings of the Innovations and Developments in Concrete Materials and Construction: Proceedings of the International Conference, Scotland, UK, 9–11 September 2002; pp. 615–624. [Google Scholar]
- Krou, N.; Batonneau-Gener, I.; Belin, T.; Mignard, S.; Javierre, I.; Dubois-Brugger, I.; Horgnies, M. Reactivity of volatile organic compounds with hydrated cement paste containing activated carbon. Build. Environ. 2015, 87, 102–107. [Google Scholar] [CrossRef]
- Elmouwahidi, A.; Zapata-Benabithe, Z.; Carrasco-Marín, F.; Moreno-Castilla, C. Activated carbons from KOH-activation of argan (Argania spinosa) seed shells as supercapacitor electrodes. Bioresour. Technol. 2012, 111, 185–190. [Google Scholar] [CrossRef]
- Na, S.; Lee, S.; Youn, S. Experiment on activated carbon manufactured from waste coffee grounds on the compressive strength of cement mortars. Symmetry 2021, 13, 619. [Google Scholar] [CrossRef]
- Zheng, C.; Liu, Z.; Xu, J.; Li, X.; Yao, Y. Compressive strength and microstructure of activated carbon-fly ash cement composites. Chem. Eng. Trans. 2017, 59, 475–480. [Google Scholar]
- Wang, Y.; Ge, Y.; Wang, X.; Chen, X.; Li, Q. The effect of powder activated carbon on mechanical properties and pore structures of cement-based mortars. Constr. Build. Mater. 2022, 316, 125798. [Google Scholar] [CrossRef]
- ASTM C150/150M; Standard Specification for Portland Cement. ASTM International: West Conshohocken, PA, USA, 2015.
- ACI 211.1R; Standard Practice for Selecting Proportions for Normal, Heavyweight, and Mass Concrete. American Concrete Institute: Farmington Hills, MI, USA, 2002.
- Adamu, M.; Trabanpruek, P.; Jongvivatsakul, P.; Likitlersuang, S.; Iwanami, M. Mechanical performance and optimization of high-volume fly ash concrete containing plastic wastes and graphene nanoplatelets using response surface methodology. Constr. Build. Mater. 2021, 308, 125085. [Google Scholar] [CrossRef]
- Montgomery, D.C. Design and Analysis of Experiments; John Wiley & Sons: New York, NY, USA, 2017. [Google Scholar]
- Adamu, M.; Haruna, S.I.; Ibrahim, Y.E.; Alanazi, H. Evaluation of the mechanical performance of concrete containing calcium carbide residue and nano silica using response surface methodology. Environ. Sci. Pollut. Res. 2022, 29, 67076–67102. [Google Scholar] [CrossRef] [PubMed]
- Mohammed, B.S.; Adamu, M. Mechanical performance of roller compacted concrete pavement containing crumb rubber and nano silica. Constr. Build. Mater. 2018, 159, 234–251. [Google Scholar] [CrossRef]
- ASTM C192/C192M; Standard Practice for Making and Curing Test Specimens in the Laboratory. ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM C143/C143M; Standard Test Method for Slump of Hydraulic-Cement Concrete. ASTM International: West Conshohocken, PA, USA, 2012.
- ASTM C138/C138M; Standard Test Method for Density (Unit Weight), Yield, and Air Content (Gravimetric) of Concrete. ASTM International: West Conshohocken, PA, USA, 2017.
- BS EN 12390-3; Testing Hardened Concrete. Compressive Strength of Test Specimens. British Standards Institution: London, UK, 2009.
- BS EN 12390-6; Testing Hardened Concrete. Tensile Splitting Strength of Test Specimens. British Standards Institution: London, UK, 2009.
- ASTM C78/C78M; Standard Test Method for Flexural Strength of Concrete (Using Simple Beam with Third-Point Loading). ASTM International: West Conshohocken, PA, USA, 2015.
- ASTM C642; Standard Test Method for Density, Absorption, and Voids in Hardened Concrete. ASTM International: West Conshohocken, PA, USA, 2001.
- Adamu, M.; Ibrahim, Y.E.; Alanazi, H. Evaluating the Influence of Elevated Temperature on Compressive Strength of Date-Palm-Fiber-Reinforced Concrete Using Response Surface Methodology. Materials 2022, 15, 8129. [Google Scholar] [CrossRef]
- Stat-Ease hwsc. Design-Expert 11 User’s Guide-Response Surface Methods (RSM) Tutorials-Section 6: Stat-Ease. Available online: https://www.statease.com/docs/v11/tutorials/multifactor-rsm/ (accessed on 28 January 2023).
- Ali-Boucetta, T.; Ayat, A.; Laifa, W.; Behim, M. Treatment of date palm fibres mesh: Influence on the rheological and mechanical properties of fibre-cement composites. Constr. Build. Mater. 2021, 273, 121056. [Google Scholar] [CrossRef]
- Rashad, A.M.; Said, N.; Abdel-Gawwad, H.A. An initial study about the effect of activated carbon nano-sheets from residual biomass of olive trees pellets on the properties of alkali-activated slag pastes. J. Build. Eng. 2021, 44, 102661. [Google Scholar]
- Lekkam, M.; Benmounah, A.; Kadri, E.-H.; Soualhi, H.; Kaci, A. Influence of saturated activated carbon on the rheological and mechanical properties of cementitious materials. Constr. Build. Mater. 2019, 198, 411–422. [Google Scholar] [CrossRef]
- Abbass, W.; Khan, M.I.; Mourad, S. Evaluation of mechanical properties of steel fiber reinforced concrete with different strengths of concrete. Constr. Build. Mater. 2018, 168, 556–569. [Google Scholar] [CrossRef]
- Benmansour, N.; Agoudjil, B.; Gherabli, A.; Kareche, A.; Boudenne, A. Thermal and mechanical performance of natural mortar reinforced with date palm fibers for use as insulating materials in building. Energy Build. 2014, 81, 98–104. [Google Scholar] [CrossRef]
- Adamu, M. Development of Nanosilica Modified High Volume Fly Ash Roller Compacted Rubbercrete for Pavement Application. Ph.D. Thesis, Universiti Teknologi Petronas, Seri Iskandar, Malaysia, 2018. [Google Scholar]
- Benaniba, S.; Driss, Z.; Djendel, M.; Raouache, E.; Boubaaya, R. Thermo-mechanical characterization of a bio-composite mortar reinforced with date palm fiber. J. Eng. Fibers Fabr. 2020, 15, 1558925020948234. [Google Scholar] [CrossRef]




| Oxides | Compositions (%) | |
|---|---|---|
| OPC | PAC | |
| C | - | 91 |
| CaO | 65.18 | 0.53 |
| Al2O3 | 5.39 | 0.64 |
| Fe2O3 | 3.4 | 0.60 |
| SiO2 | 19.17 | 1.57 |
| MgO | 0.91 | 0.22 |
| TiO2 | 0.24 | 0.06 |
| Na2O | 0.17 | - |
| K2O | 1.22 | 0.06 |
| P2O5 | 0.09 | 0.15 |
| SO3 | 3.51 | 1.31 |
| MnO | 0.18 | - |
| Properties | Fine Aggregate | Coarse Aggregate |
|---|---|---|
| Maximum Size (mm) | 4.75 | 19 |
| Specific gravity | 2.63 | 2.67 |
| Bulk density (kg/m3) | 1565 | 1455 |
| Water absorption (%) | 1.87 | 0.65 |
| Fineness modulus | 2.26 | − |
| Mud content (%) | 1.1 | − |
| Mix No. | Variables | Quantities for 1 kg/m3 (kg/m3) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| DPF (%) | PAC (%) | Cement | DPF | PAC | Fine Aggregate | Coarse Aggregate | Water | S. P | |
| M1 | 0 | 0 | 480 | 0.0 | 0.0 | 730 | 890 | 180 | 4.8 |
| M2 | 0 | 1 | 480 | 0.0 | 4.8 | 730 | 890 | 180 | 4.8 |
| M3 | 1 | 1 | 480 | 4.8 | 4.8 | 730 | 890 | 180 | 4.8 |
| M4 | 2 | 1 | 480 | 9.7 | 4.8 | 730 | 890 | 180 | 4.8 |
| M5 | 3 | 1 | 480 | 14.5 | 4.8 | 730 | 890 | 180 | 4.8 |
| M6 | 0 | 2 | 480 | 0.0 | 9.6 | 730 | 890 | 180 | 4.9 |
| M7 | 1 | 2 | 480 | 4.9 | 9.6 | 730 | 890 | 180 | 4.9 |
| M8 * | 2 | 2 | 480 | 9.8 | 9.6 | 730 | 890 | 180 | 4.9 |
| M9 | 3 | 2 | 480 | 14.7 | 9.6 | 730 | 890 | 180 | 4.9 |
| M10 | 0 | 3 | 480 | 0.0 | 14.4 | 730 | 890 | 180 | 4.9 |
| M11 | 1 | 3 | 480 | 4.9 | 14.4 | 730 | 890 | 180 | 4.9 |
| M12 | 2 | 3 | 480 | 9.9 | 14.4 | 730 | 890 | 180 | 4.9 |
| M13 | 3 | 3 | 480 | 14.8 | 14.4 | 730 | 890 | 180 | 4.9 |
| Mix No. | Variables | Slump (mm) | Fresh Density (kg/m3) | Compressive Strength (MPa) | Splitting Tensile Strength (MPa) | Flexural Strength (MPa) | Water Absorption (%) | ||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| DPF (%) | PAC (%) | 7-Day | 28-Day | 7-Day | 28-Day | 7-Day | 28-Day | ||||
| M1 | 0 | 0 | 84 | 2469 | 39.24 | 48.71 | 2.61 | 3.16 | 4.34 | 5.2 | 3.29 |
| M2 | 0 | 1 | 80 | 2506 | 42.58 | 53.42 | 2.72 | 3.43 | 4.45 | 5.63 | 2.70 |
| M3 | 1 | 1 | 78 | 2515 | 44.61 | 54.13 | 2.85 | 3.51 | 4.62 | 5.78 | 2.89 |
| M4 | 2 | 1 | 74 | 2487 | 41.08 | 51.39 | 2.91 | 3.77 | 4.87 | 6.17 | 3.39 |
| M5 | 3 | 1 | 68 | 2420 | 36.42 | 47.48 | 2.50 | 3.05 | 4.52 | 5.55 | 3.58 |
| M6 | 0 | 2 | 65 | 2528 | 35.25 | 44.32 | 2.45 | 3.06 | 4.04 | 4.97 | 2.97 |
| M7 | 1 | 2 | 63 | 2539 | 33.52 | 44.32 | 2.69 | 3.32 | 4.51 | 5.49 | 3.03 |
| M8 | 2 | 2 | 61 | 2448 | 31.11 | 42.15 | 2.34 | 2.90 | 3.96 | 4.84 | 3.73 |
| M9 | 3 | 2 | 60 | 2404 | 29.59 | 42.96 | 2.20 | 2.71 | 3.85 | 4.5 | 4.03 |
| M10 | 0 | 3 | 55 | 2373 | 29.47 | 40.48 | 2.11 | 2.60 | 3.76 | 4.65 | 4.27 |
| M11 | 1 | 3 | 53 | 2348 | 25.48 | 38.09 | 2.08 | 2.56 | 3.60 | 4.44 | 4.41 |
| M12 | 2 | 3 | 52 | 2312 | 24.95 | 31.95 | 1.90 | 2.39 | 3.39 | 4.32 | 4.62 |
| M13 | 3 | 3 | 50 | 2270 | 18.10 | 24.53 | 1.81 | 2.30 | 3.03 | 3.86 | 4.80 |
| M8 * | 2 | 3 | 60 | 2446 | 29.42 | 40.13 | 2.28 | 3.01 | 4.11 | 4.68 | 3.93 |
| M8 * | 2 | 3 | 60 | 2467 | 34.35 | 44.76 | 2.40 | 2.89 | 3.90 | 4.98 | 3.45 |
| M8 * | 2 | 3 | 62 | 2431 | 28.34 | 41.43 | 2.35 | 2.78 | 3.87 | 4.86 | 3.81 |
| M8 * | 2 | 3 | 58 | 2448 | 30.76 | 44.00 | 2.54 | 3.01 | 3.96 | 4.84 | 3.73 |
| Response | Source | F Value | p-Value Prob > F | Significance | R2 | Adjusted R2 | Predicted R2 | A.P |
|---|---|---|---|---|---|---|---|---|
| Slump (mm) | Model | 54.61 | <0.0001 | Yes | 0.961 | 0.944 | 0.777 | 26.88 |
| D-DPF | 14.04 | 0.0032 | Yes | |||||
| A-PAC | 108.39 | <0.0001 | Yes | |||||
| D × A | 0.27 | 0.6131 | No | |||||
| D2 | 0.00339 | 0.9546 | No | |||||
| A2 | 0.26 | 0.6178 | No | |||||
| Lack of Fit | 3.69 | 0.1123 | No | |||||
| Fresh Density (kg/m3) | Model | 39.54 | <0.0001 | Yes | 0.95 | 0.923 | 0.852 | 22.35 |
| D-DPF | 41.64 | <0.0001 | Yes | |||||
| A-PAC | 9.43 | 0.0106 | Yes | |||||
| D × A | 0.00792 | 0.9307 | No | |||||
| D2 | 2.71 | 0.1280 | No | |||||
| A2 | 52.35 | <0.0001 | Yes | |||||
| Lack of Fit | 3.63 | 0.1152 | No |
| Response | Source | Before Model Reduction | After Model Reduction | ||||
|---|---|---|---|---|---|---|---|
| F Value | p-Value Prob > F | Significant | F Value | p-Value Prob > F | Significant | ||
| 7-Day Compressive Strength (MPa) | Model | 20.00 | <0.0001 | Yes | 29.84 | <0.0001 | Yes |
| D-DPF | 5.51 | 0.0387 | Yes | 7.81 | 0.0174 | Yes | |
| A-PAC | 35.45 | <0.0001 | Yes | 46.41 | <0.0001 | Yes | |
| DA | 3.48 | 0.0892 | No | 13.05 | 0.0041 | Yes | |
| D2 | 0.54 | 0.4774 | No | 2.27 | 0.1602 | No | |
| A2 | 2.01 | 0.1843 | No | 4.38 | 0.0604 | Yes | |
| Lack of Fit | 1.51 | 0.3634 | No | 1.24 | 0.4427 | No | |
| 28-Day Compressive Strength (MPa) | Model | 26.92 | <0.0001 | Yes | |||
| D-DPF | 5.04 | 0.0462 | Yes | ||||
| A-PAC | 44.03 | <0.0001 | Yes | ||||
| DA | 8.93 | 0.0124 | Yes | ||||
| D2 | 0.97 | 0.3456 | No | ||||
| A2 | 4.84 | 0.0500 | Yes | ||||
| Lack of Fit | 2.06 | 0.2532 | No | ||||
| Factor | 7-Day Compressive Strength (MPa) | 28-Day Compressive Strength (Mpa) | |
|---|---|---|---|
| No Transformation | After Transformation | No Transformation | |
| R2 | 0.901 | 0.931 | 0.925 |
| Adjusted R2 | 0.856 | 0.900 | 0.890 |
| Predicted R2 | 0.562 | 0.797 | 0.730 |
| Adequate Precision | 15.67 | 19.49 | 17.77 |
| Standard Deviation | 2.61 | 0.00249 | 2.44 |
| Mean | 32.60 | 0.032 | 43.19 |
| C.V.% | 8.01 | 7.74 | 5.65 |
| PRESS | 331.92 | 0.0002 | 234.47 |
| Responses | Sources | No Model Transformation | After Model Transformation | ||||
|---|---|---|---|---|---|---|---|
| F Values | p-Values Prob > F | Significant | F Values | p-Values Prob > F | Significant | ||
| 7-Day Splitting Tensile Strength (MPa) | Model | 20.91 | <0.0001 | Yes | 42.30 | <0.0001 | Yes |
| D-DPF | 4.26 | 0.0636 | No | 9.17 | 0.0115 | Yes | |
| A-PAC | 31.37 | 0.0002 | Yes | 57.02 | <0.0001 | Yes | |
| D × A | 1.95 | 0.1905 | No | 5.68 | 0.0363 | Yes | |
| D2 | 5.07 | 0.0457 | Yes | 8.06 | 0.0161 | Yes | |
| A2 | 5.58 | 0.0377 | Yes | 14.86 | 0.0027 | Yes | |
| Lack of Fit | 1.63 | 0.3325 | No | 0.79 | 0.6303 | No | |
| 28-Day Splitting Tensile Strength (MPa) | Model | 12.22 | 0.0003 | Yes | 26.71 | <0.0001 | Yes |
| D-DPF | 2.51 | 0.1417 | No | 6.23 | 0.0297 | Yes | |
| A-PAC | 17.86 | 0.0014 | Yes | 34.54 | 0.0001 | Yes | |
| D × A | 1.07 | 0.3233 | No | 2.69 | 0.1292 | No | |
| D2 | 2.89 | 0.1174 | No | 4.74 | 0.0520 | No | |
| A2 | 3.55 | 0.0861 | No | 10.46 | 0.0079 | Yes | |
| Lack of Fit | 5.27 | 0.0636 | No | 2.24 | 0.2277 | No | |
| Factors | 7-Day Splitting Tensile Strength (MPa) | 28-Day Splitting Tensile Strength (MPa) | ||
|---|---|---|---|---|
| No Transform | Model Transform | No Transform | Model Transform | |
| R2 | 0.905 | 0.951 | 0.847 | 0.924 |
| Adjusted R2 | 0.862 | 0.928 | 0.778 | 0.889 |
| Predicted R2 | 0.657 | 0.884 | 0.309 | 0.728 |
| Adequate Precision | 15.96 | 22.88 | 12.17 | 17.99 |
| Standard Deviation | 0.12 | 0.02 | 0.19 | 0.015 |
| Mean | 2.40 | 0.42 | 2.97 | 0.34 |
| C.V.% | 4.85 | 3.70 | 6.27 | 4.47 |
| PRESS | 0.54 | 0.0064 | 1.73 | 0.0092 |
| Responses | Sources | Before Model Transformation | After Model Transformation | ||||
|---|---|---|---|---|---|---|---|
| F Values | p-Values Prob > F | Significant | F Values | p-Values Prob > F | Significant | ||
| 7-Day Flexural Strength (MPa) | Model | 23.67 | <0.0001 | Yes | - | - | - |
| D-DPF | 0.18 | 0.6754 | No | - | - | - | |
| A-PAC | 52.12 | <0.0001 | Yes | - | - | - | |
| D × A | 13.06 | 0.0041 | Yes | - | - | - | |
| D2 | 3.12 | 0.1049 | No | - | - | - | |
| A2 | 1.42 | 0.2587 | No | - | - | - | |
| Lack of Fit | 4.48 | 0.0830 | No | - | - | - | |
| 28-Day Flexural Strength (MPa) | Model | 12.67 | 0.0003 | Yes | 29.64 | <0.0001 | Yes |
| D-DPF | 0.20 | 0.6651 | No | 0.38 | 0.5506 | No | |
| A-PAC | 28.41 | 0.0002 | Yes | 96.95 | <0.0001 | No | |
| D × A | 7.43 | 0.0197 | Yes | 24.01 | 0.0004 | No | |
| D2 | 2.63 | 0.1331 | No | 7.49 | 0.0181 | No | |
| A2 | 0.45 | 0.5140 | No | - | - | ||
| Lack of Fit | 9.77 | 0.0219 | Yes | 4.63 | 0.0779 | No | |
| Water Absorption (%) | Model | 33.93 | <0.0001 | Yes | - | - | - |
| D-DPF | 36.56 | <0.0001 | Yes | - | - | - | |
| A-PAC | 12.35 | 0.0049 | Yes | - | - | - | |
| D × A | 0.52 | 0.4871 | No | - | - | - | |
| D2 | 0.00508 | 0.9444 | No | - | - | - | |
| A2 | 37.71 | <0.0001 | Yes | - | - | - | |
| Lack of Fit | 1.11 | 0.4865 | No | - | - | - | |
| Factors | 7-Day Flexural Strength (MPa) | 28-Day Flexural Strength (MPa) | Water Absorption (%) | |
|---|---|---|---|---|
| No Transform | Model Transform | |||
| R2 | 0.915 | 0.852 | 0.908 | 0.939 |
| Adjusted R2 | 0.876 | 0.785 | 0.878 | 0.911 |
| Predicted R2 | 0.753 | 0.235 | 0.740 | 0.804 |
| Adequate Precision | 17.57 | 13.14 | 20.30 | 20.57 |
| Standard Deviation | 0.17 | 0.27 | 0.0085 | 0.18 |
| Mean | 4.05 | 4.99 | 0.20 | 3.68 |
| C.V.% | 4.10 | 5.49 | 4.18 | 4.97 |
| PRESS | 0.88 | 4.27 | 0.0025 | 1.19 |
| Names | Units | Goals | Lower Limit | Upper Limit | Solution |
|---|---|---|---|---|---|
| A: DPF | % | In range | 0 | 3 | 0.93 |
| A: PAC | % | In range | 0 | 3 | 0.37 |
| Slump | mm | Maximize | 50 | 84 | 80 |
| Fresh Density | Kg/m3 | In range | 2270 | 2539 | 2482 |
| 7-Day Compressive Strength | MPa | Maximize | 18.10 | 44.61 | 46.55 |
| 28-Day Compressive Strength | MPa | Maximize | 24.53 | 54.13 | 52.80 |
| 7-Day Split Tensile Strength | MPa | Maximize | 1.81 | 2.91 | 2.80 |
| 28-Day Split Tensile Strength | MPa | Maximize | 2.30 | 3.77 | 3.52 |
| 7-Day Flexural Strength | MPa | Maximize | 3.03 | 4.87 | 4.75 |
| 28-Day Flexural Strength | MPa | Maximize | 3.86 | 6.17 | 6.17 |
| Water Absorption | % | Minimize | 2.70 | 4.80 | 3.26 |
| Desirability | % | - | - | - | 0.91 |
| Responses | Variables (%) | Predicted | Experimental | Errors (%) | Average Error (%) | |||||
|---|---|---|---|---|---|---|---|---|---|---|
| DPF | PAC | |||||||||
| Slump (mm) | 0.93 | 0.37 | 80.5 | 84 | 4.21 | 4.97 | ||||
| 2 | 2 | 61.6 | 65 | 5.29 | ||||||
| 1.5 | 0.5 | 77.6 | 75 | 3.42 | ||||||
| Fresh Density (kg/m3) | 0.93 | 0.37 | 2482 | 2411 | 2.94 | 3.41 | ||||
| 2 | 2 | 2454 | 2336 | 5.07 | ||||||
| 1.5 | 0.5 | 2474 | 2376 | 4.13 | ||||||
| Water absorption (%) | 0.93 | 0.37 | 3.26 | 3.11 | 4.87 | 5.34 | ||||
| 2 | 2 | 3.62 | 3.85 | 5.85 | ||||||
| 1.5 | 0.5 | 3.39 | 3.22 | 5.27 | ||||||
| Compressive Strength (MPa) | DPF | PAC | 7D | 28D | 7D | 28D | 7D | 28D | 7D | 28D |
| 0.93 | 0.37 | 45.95 | 52.80 | 43.76 | 50.41 | 6.71 | 5.00 | 5.17 | 3.94 | |
| 2 | 2 | 31.15 | 43.23 | 32.16 | 45.17 | 3.74 | 3.13 | |||
| 1.5 | 0.5 | 47.00 | 53.15 | 44.74 | 51.26 | 5.06 | 3.68 | |||
| Split Tensile Strength (MPa) | 0.93 | 0.37 | 2.86 | 3.51 | 2.98 | 3.70 | 3.92 | 5.24 | 3.93 | 4.56 |
| 2 | 2 | 2.40 | 2.99 | 2.29 | 2.87 | 4.97 | 4.07 | |||
| 1.5 | 0.5 | 2.88 | 3.53 | 2.97 | 3.38 | 2.90 | 4.36 | |||
| Flexural Strength (MPa) | 0.93 | 0.37 | 4.83 | 6.35 | 5.15 | 6.63 | 4.30 | 4.87 | 4.56 | 5.33 |
| 2 | 2 | 4.03 | 4.96 | 4.31 | 5.29 | 6.23 | 5.85 | |||
| 1.5 | 0.5 | 4.96 | 6.89 | 5.27 | 7.11 | 3.15 | 5.27 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Adamu, M.; Ibrahim, Y.E.; Abdel daiem, M.M.; Alanazi, H.; Elalaoui, O.; Ali, N.M. Optimization and Modelling the Mechanical Performance of Date Palm Fiber-Reinforced Concrete Incorporating Powdered Activation Carbon Using Response Surface Methodology. Materials 2023, 16, 2977. https://doi.org/10.3390/ma16082977
Adamu M, Ibrahim YE, Abdel daiem MM, Alanazi H, Elalaoui O, Ali NM. Optimization and Modelling the Mechanical Performance of Date Palm Fiber-Reinforced Concrete Incorporating Powdered Activation Carbon Using Response Surface Methodology. Materials. 2023; 16(8):2977. https://doi.org/10.3390/ma16082977
Chicago/Turabian StyleAdamu, Musa, Yasser E. Ibrahim, Mahmoud M. Abdel daiem, Hani Alanazi, Oussama Elalaoui, and Nageh M. Ali. 2023. "Optimization and Modelling the Mechanical Performance of Date Palm Fiber-Reinforced Concrete Incorporating Powdered Activation Carbon Using Response Surface Methodology" Materials 16, no. 8: 2977. https://doi.org/10.3390/ma16082977
APA StyleAdamu, M., Ibrahim, Y. E., Abdel daiem, M. M., Alanazi, H., Elalaoui, O., & Ali, N. M. (2023). Optimization and Modelling the Mechanical Performance of Date Palm Fiber-Reinforced Concrete Incorporating Powdered Activation Carbon Using Response Surface Methodology. Materials, 16(8), 2977. https://doi.org/10.3390/ma16082977









