Inorganic Particles Contribute to the Compatibility of Polycarbonate/Polystyrene Polymer Blends
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Melt-Blending
2.3. Viscosity Measurements
2.4. Injection Molding
2.5. Tensile Tests
2.6. Determination of Elastic Properties
2.7. Transmittance
2.8. Charpy Impact Tests
2.9. Morphology Observation
2.10. Differential Scanning Calorimetry (DSC)
2.11. Fourier Transform Infrared Spectroscopy
3. Results and Discussion
3.1. Morphology of PC/PS Polymer Blends
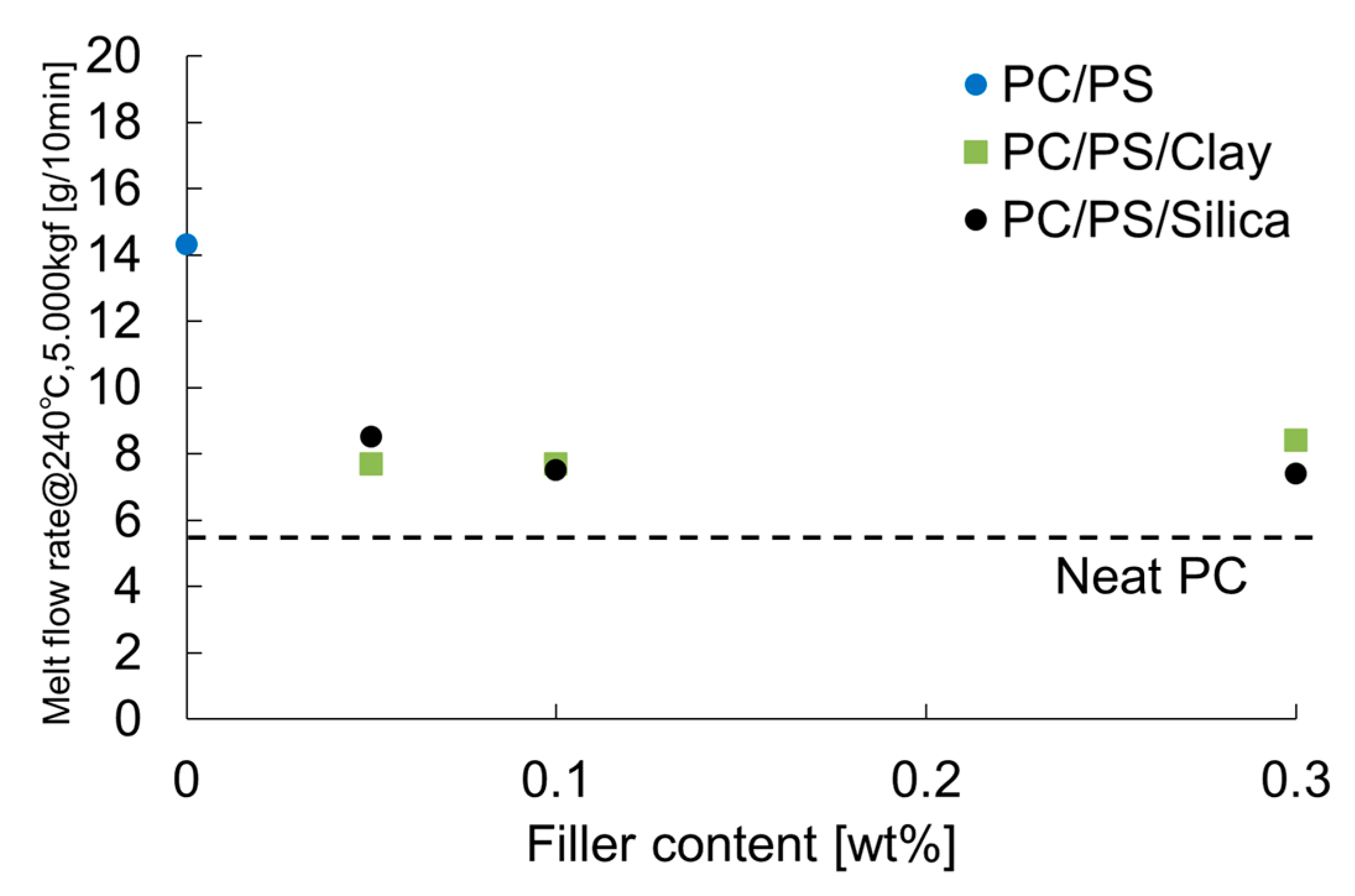
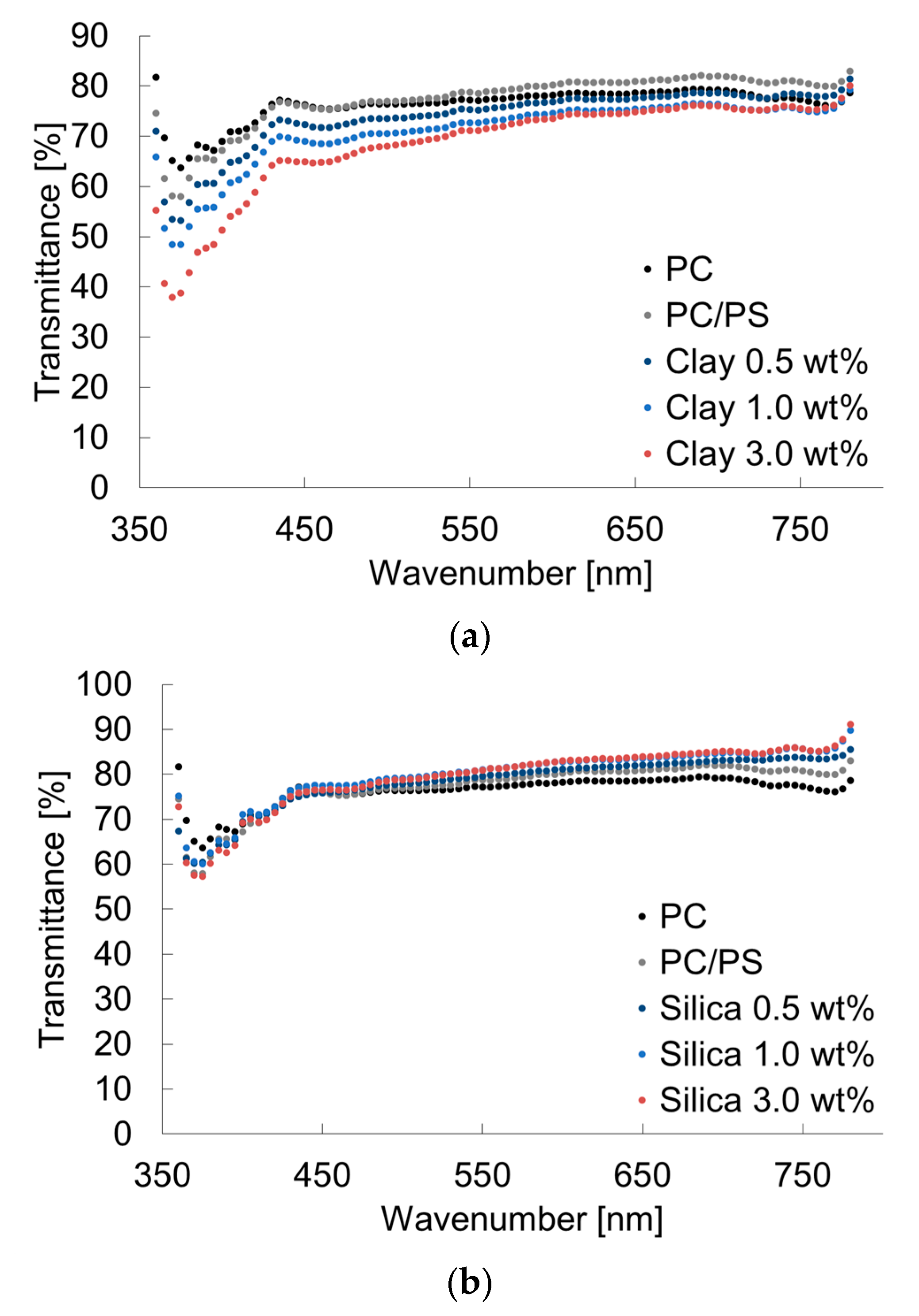
3.2. PS Blending Effects on Viscosity and Transmittance
3.3. Glass Transition Temperatures of PC/PS Polymer Blends
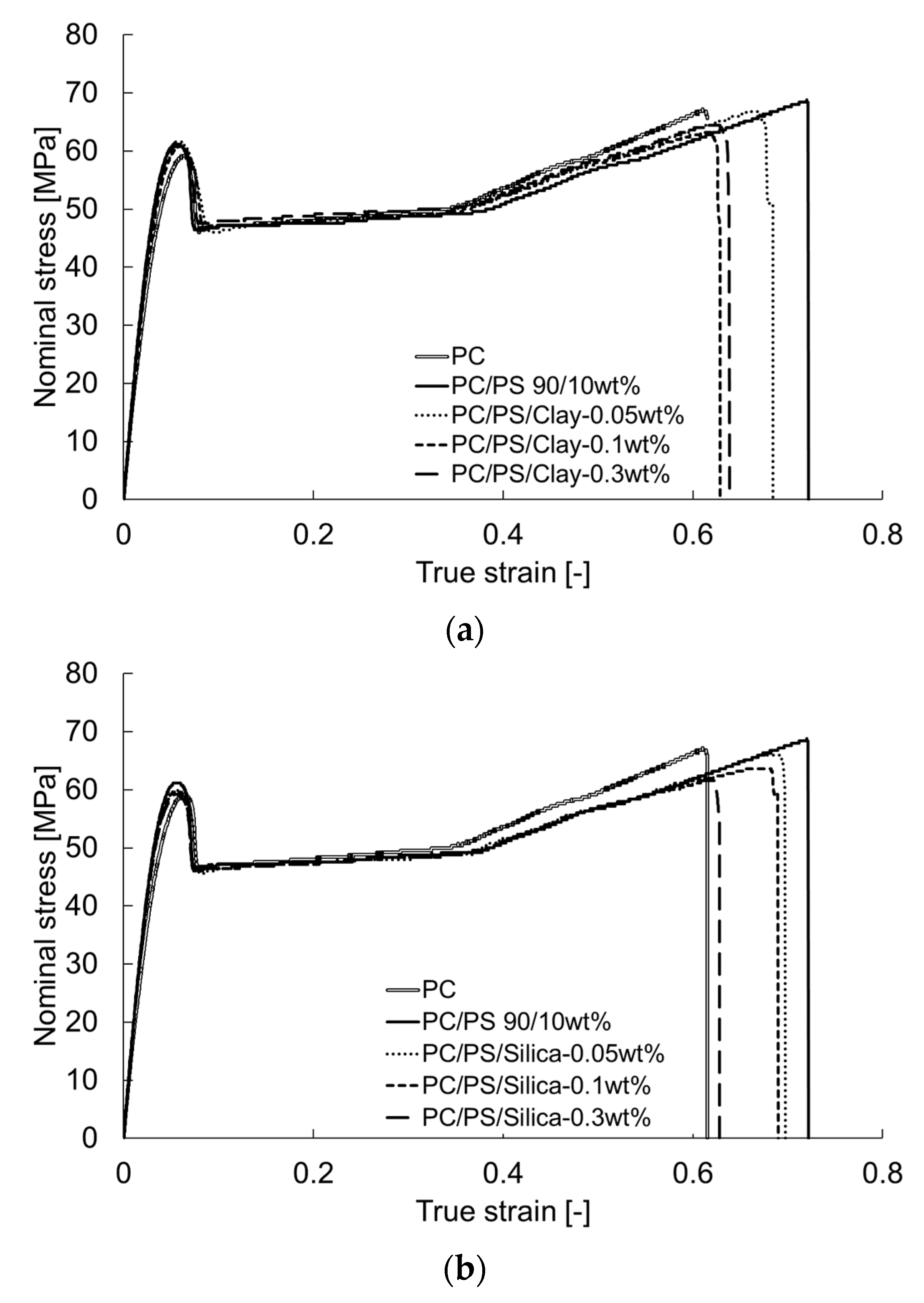
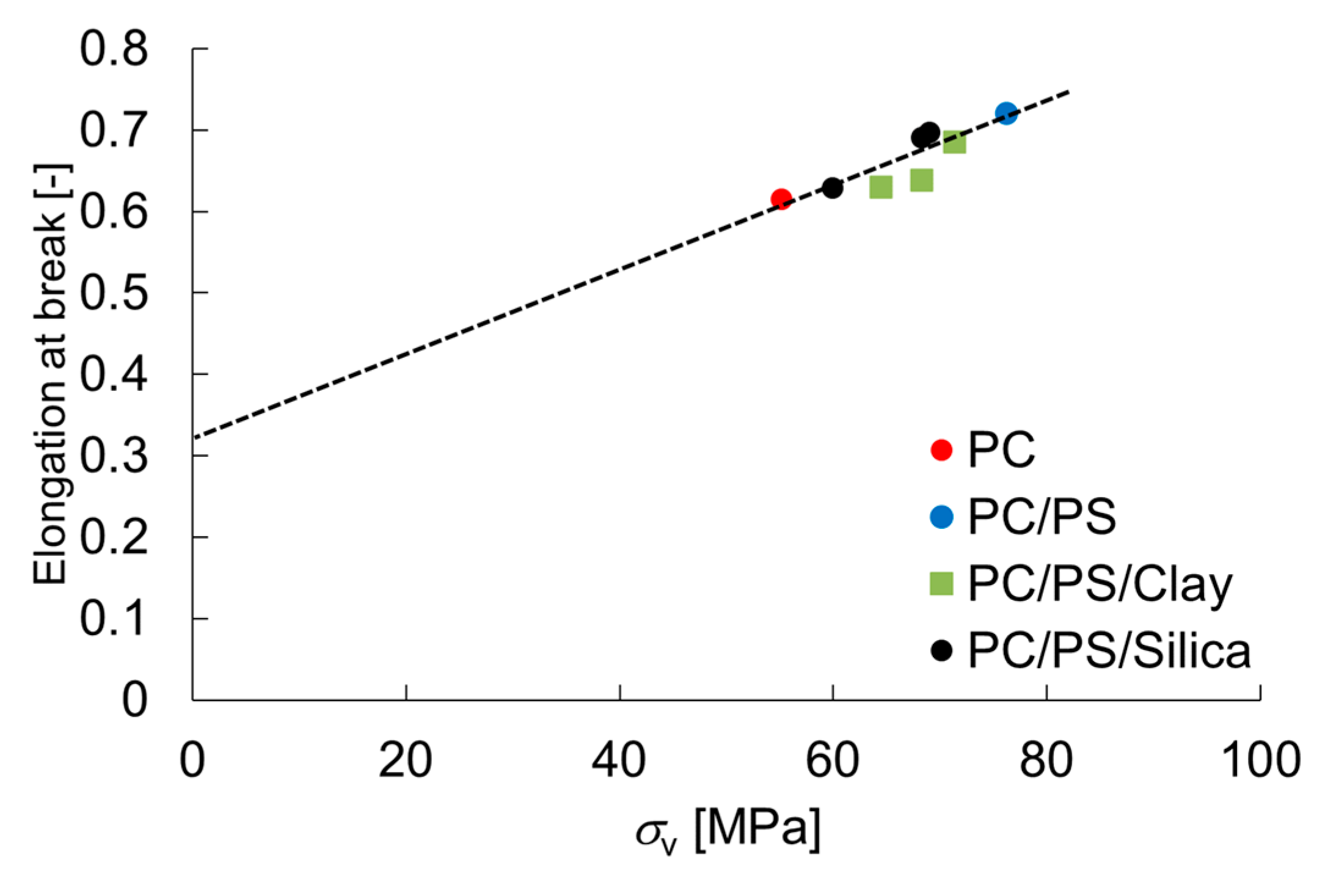
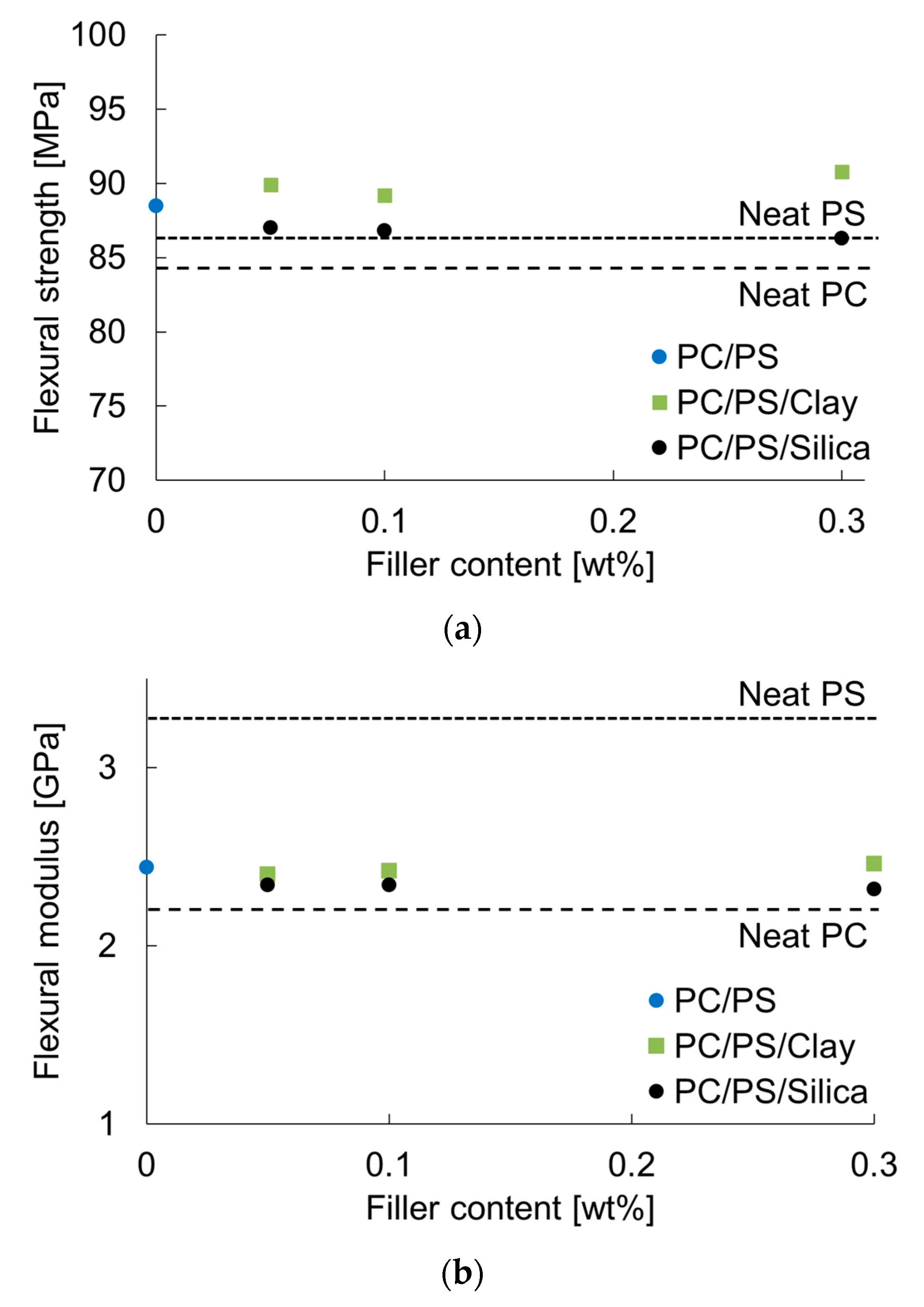
3.4. Mechanical Properties of PC/PS Polymer Blends
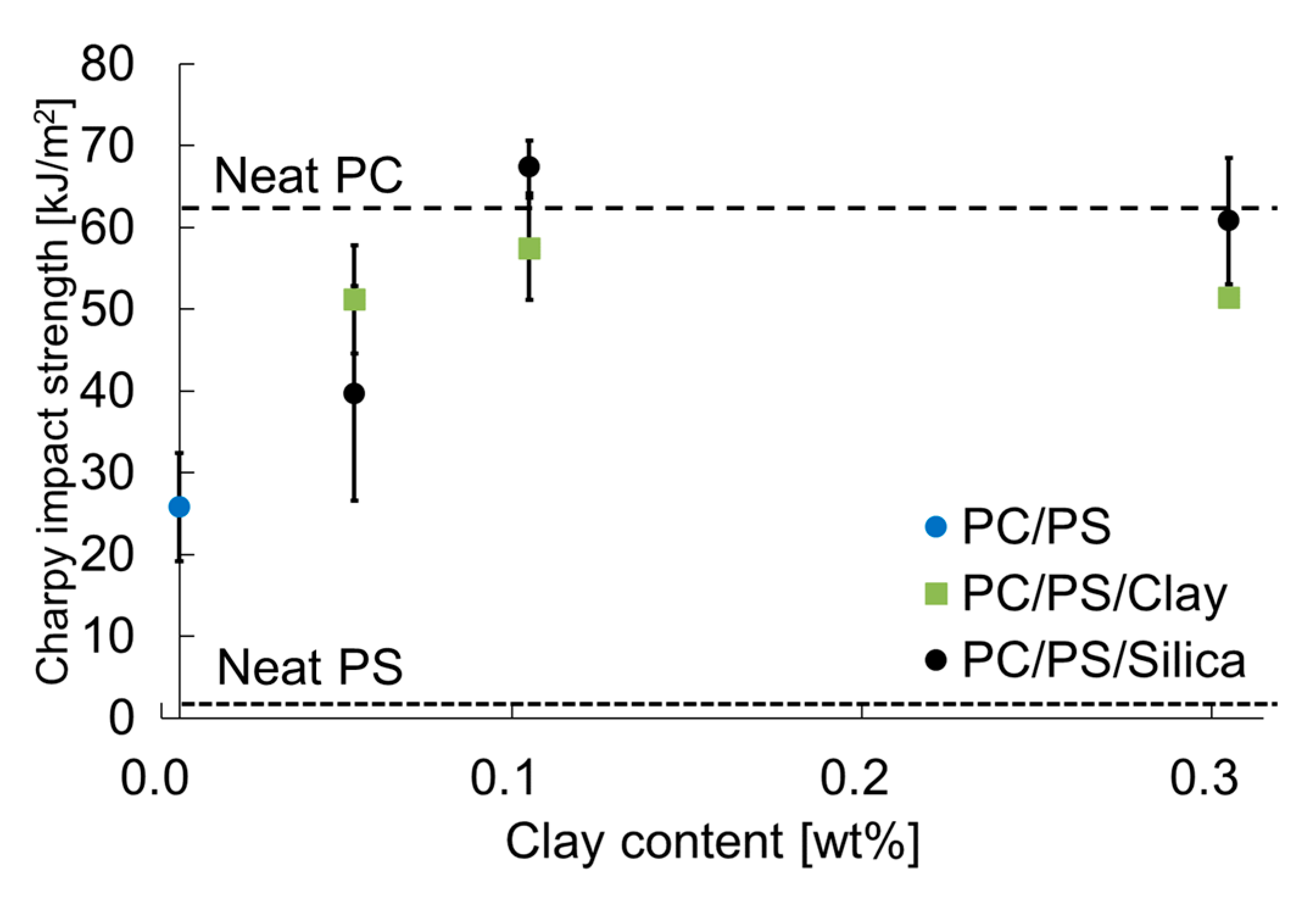
3.5. Notched Impact Strength of PC/PS Polymer Blends
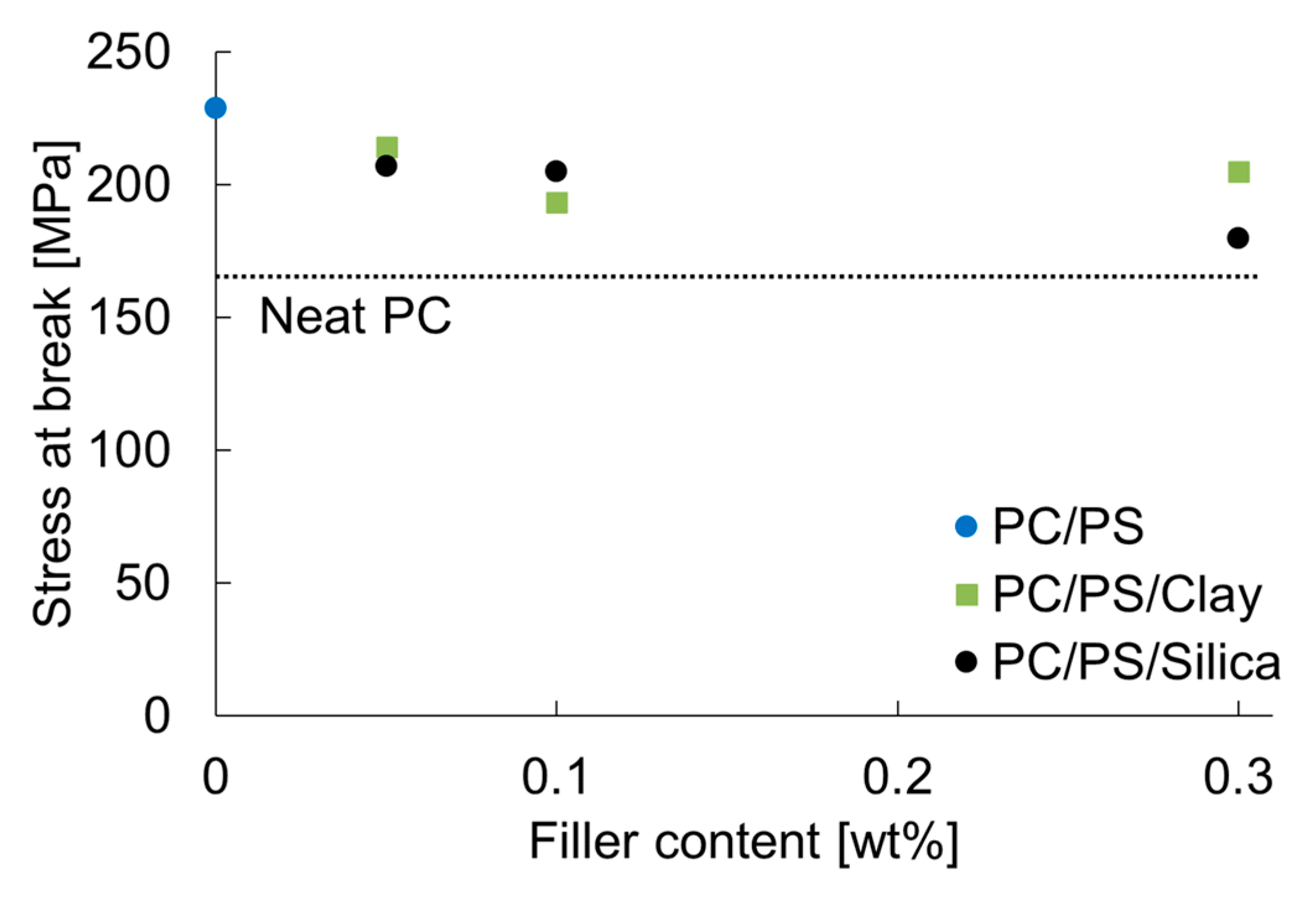
3.6. Morphology and Compatibility Effects of Inorganic Particles
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Sako, T.; Date, J.; Hagi, M.; Hiraoka, T.; Matsuoka, S.; Yamaguchi, M. Anomalous Viscosity Decrease of Polycarbonate by Addition of Polystyrene. Polymer 2019, 170, 135–141. [Google Scholar] [CrossRef]
- Ramakrishnan, V.; Goossens, J.G.P.; Hoeks, T.L.; Peters, G.W.M. Anomalous Terminal Shear Viscosity Behavior of Polycarbonate Nanocomposites Containing Grafted Nanosilica Particles. Nanomaterials 2021, 11, 1839. [Google Scholar] [CrossRef] [PubMed]
- Nishitsuji, S.; Sato, T.; Ishikawa, M.; Inoue, T.; Ito, H. Effects of molecular weight and annealing conditions on the essential work of fracture of polycarbonate. Polym. Eng. Sci. 2022, 62, 3441–3449. [Google Scholar] [CrossRef]
- Zolali, A.M.; Heshmati, V.; Favis, B.D. Ultratough Co-Continuous PLA/PA11 by Interfacially Percolated Poly(ether-b-amide). Macromolecules 2017, 50, 264–274. [Google Scholar] [CrossRef]
- Nishino, K.; Shindo, Y.; Takayama, T.; Ito, H. Improvement of impact strength and hydrolytic stability of PC/ABS blend using reactive polymer. J. Appl. Polym. Sci. 2017, 134, 44550. [Google Scholar] [CrossRef]
- Kim, D.Y.; Lee, J.B.; Lee, D.Y. Selective Localization of Nanofiller on Mechanical Properties of Poly(lactic acid)/Poly(butylene adipate-co-terephthalate) Nanocomposites via the Surface Energy and Melt Blending Technique. Macromolecules 2022, 55, 3287–3300. [Google Scholar] [CrossRef]
- Taguet, A.; Cassagnau, P.; Lopez-Cuesta, J.M. Structuration, selective dispersion and compatibilizing effect of (nano)fillers in polymer blends. Prog. Polym. Sci. 2014, 39, 1526–1563. [Google Scholar] [CrossRef]
- Filippone, G.; Salzano de Luna, M. Effects of Nanoparticles on the Morphology of Immiscible Polymer Blends—Challenges and Opportunities. Eur. Polym. J. 2016, 79, 198–218. [Google Scholar]
- Fang, Z.; Harrats, C.; Moussaif, N.; Groeninckx, G. Location of a nanoclay at the interface in an immiscible poly (ε-caprolactone)/poly (ethylene oxide) blend and its effect on the compatibility of the components. J. Appl. Polym. Sci. 2007, 106, 3125–3135. [Google Scholar] [CrossRef]
- Mederic, P.; Ville, J.; Huitric, J.; Moan, M.; Aubry, T. Effect of processing procedures and conditions on structural, morphological, and rheological properties of polyethylene/polyamide/nanoclay blends. Polym. Eng. Sci. 2011, 51, 969–978. [Google Scholar] [CrossRef]
- Moonprasith, N.; Date, J.; Sako, T.; Kida, T.; Hiraoka, T.; Yamaguchi, M. Segregation behavior of miscible PC/PMMA blends during injection molding. Materials 2022, 15, 2994. [Google Scholar] [CrossRef] [PubMed]
- Yamaguchi, M.; Nakamura, K.; Kimura, T.; Moonprasith, N.; Kida, T.; Tsubouchi, K.; Narita, T.; Hiraoka, T. Complicated structure change during capillary extrusion of binary blends of polycarbonate and poly (methyl methacrylate). Materials 2022, 15, 2783. [Google Scholar] [CrossRef] [PubMed]
- Bubmann, T.; Seidel, A.; Ruckdäschel, H.; Altstädt, V. Transparent PC/PMMA Blends with Enhanced Mechanical Properties via Reactive Compounding of Functionalized Polymers. Polymers 2022, 14, 73. [Google Scholar] [CrossRef]
- Kunori, T.; Geil, P.H. Morphology-property relationships in polycarbonate-based blends. II. Tensile and impact strength. J. Macromol. Sci. Part B Phys. 1980, 18, 135–175. [Google Scholar] [CrossRef]
- Takayama, T.; Motoyama, Y. Injection molding temperature dependence of elastic coefficients obtained using three-point bending tests to ascertain thermoplastic polymer coefficients. Mech. Eng. J. 2021, 8, 20-00414. [Google Scholar] [CrossRef]
- Unger, D.J. Generalized Tresca yield condition as a family of elliptic curves with application to mode I crack problems. Z. Für Angew. Math. Und Phys. 2022, 73, 184. [Google Scholar] [CrossRef]
- Hashima, K.; Nishitsuji, S.; Inoue, T. Structure-properties of super-tough PLA alloy with excellent heat resistance. Polymer 2010, 51, 3934–3939. [Google Scholar] [CrossRef]
- Drass, M.; Schneider, J.; Kolling, S. Novel volumetric helmholtz free energy function accounting for isotropic cavitation at finite strains. Mater. Des. 2018, 138, 71–89. [Google Scholar] [CrossRef]
- Lee, R.E.; Ghazi, A.A.; Azdast, T.; Hasanzadeh, R.; Shishavan, S.M. Tensile and hardness properties of polycarbonate nanocomposites in the presence of styrene maleic anhydride as compatibilizer. Adv. Polym. Technol. 2018, 37, 1737–1743. [Google Scholar]




| PC [wt%] | PS [wt%] | Clay [wt%] | Silica [wt%] |
|---|---|---|---|
| 100 | - | - | - |
| 90 | 10 | - | - |
| 90 | 9.95 | 0.05 | - |
| 90 | 9.9 | 0.1 | - |
| 90 | 9.7 | 0.3 | - |
| 90 | 9.95 | - | 0.05 |
| 90 | 9.9 | - | 0.1 |
| 90 | 9.7 | - | 0.3 |
| Parameter | Dumbbell | Beam |
|---|---|---|
| Injection temp. [°C] | 310 | 310 |
| Mold temp. [°C] | 90 | 110 |
| Injection speed [mm/s] | 10 | 30 |
| Holding pressure [MPa] | 105 | 105 |
| Injection time [s] | 15 | 15 |
| Cooling time [s] | 10 | 10 |
| Tg,PS [°C] | Tg,PC [°C] | |
|---|---|---|
| PC | - | 152.4 |
| PC/PS | 106.5 | 149.5 |
| PC/PS/Clay 0.1 wt% | 102.2 | 150.6 |
| PC/PS/Silica 0.1 wt% | 104.1 | 150.7 |
| PS | 98.3 | - |
| Materials | Tinj [°C] | FS※ [MPa] | FM※ [MPa] | E [MPa] | υ [-] |
|---|---|---|---|---|---|
| PC | 310 | 84.4 | 2250 | 1260 | 0.373 |
| PS | 310 | 86.7 | 3330 | 1570 | 0.399 |
| PC [wt%] | PS [wt%] | Clay [wt%] | Silica [wt%] | Tinj [°C] | FS [MPa] | FM [MPa] | E [MPa] | υ [-] |
|---|---|---|---|---|---|---|---|---|
| 90 | 10 | - | - | 310 | 88.5 | 2440 | 1380 | 0.371 |
| 90 | 9.95 | 0.05 | - | 310 | 89.9 | 2400 | 1380 | 0.367 |
| 90 | 9.9 | 0.1 | - | 310 | 89.2 | 2420 | 1380 | 0.369 |
| 90 | 9.7 | 0.3 | - | 310 | 90.8 | 2460 | 1420 | 0.368 |
| 90 | 9.95 | - | 0.05 | 310 | 87 | 2340 | 1320 | 0.371 |
| 90 | 9.9 | - | 0.1 | 310 | 86.8 | 2340 | 1320 | 0.371 |
| 90 | 9.7 | - | 0.3 | 310 | 86.3 | 2320 | 1310 | 0.371 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Takayama, T. Inorganic Particles Contribute to the Compatibility of Polycarbonate/Polystyrene Polymer Blends. Materials 2023, 16, 1536. https://doi.org/10.3390/ma16041536
Takayama T. Inorganic Particles Contribute to the Compatibility of Polycarbonate/Polystyrene Polymer Blends. Materials. 2023; 16(4):1536. https://doi.org/10.3390/ma16041536
Chicago/Turabian StyleTakayama, Tetsuo. 2023. "Inorganic Particles Contribute to the Compatibility of Polycarbonate/Polystyrene Polymer Blends" Materials 16, no. 4: 1536. https://doi.org/10.3390/ma16041536
APA StyleTakayama, T. (2023). Inorganic Particles Contribute to the Compatibility of Polycarbonate/Polystyrene Polymer Blends. Materials, 16(4), 1536. https://doi.org/10.3390/ma16041536






