The Effect of Viscous Drag on the Maximum Residual Stresses Achievable in High-Yield-Strength Materials in Laser Shock Processing
Abstract
:1. Introduction
2. Theoretical Basis: On the Consideration of the Viscous Drag Mechanism in Metal Material Alloys Subject to LSP
2.1. Generalization of the Conventional Analytical Methods to Consider the Abrupt Yield Stress Increase in Materials Subject to LSP
2.2. Expected Response in Low/High-Yield-Stress Alloys Subject to LSP
3. Materials and Methods
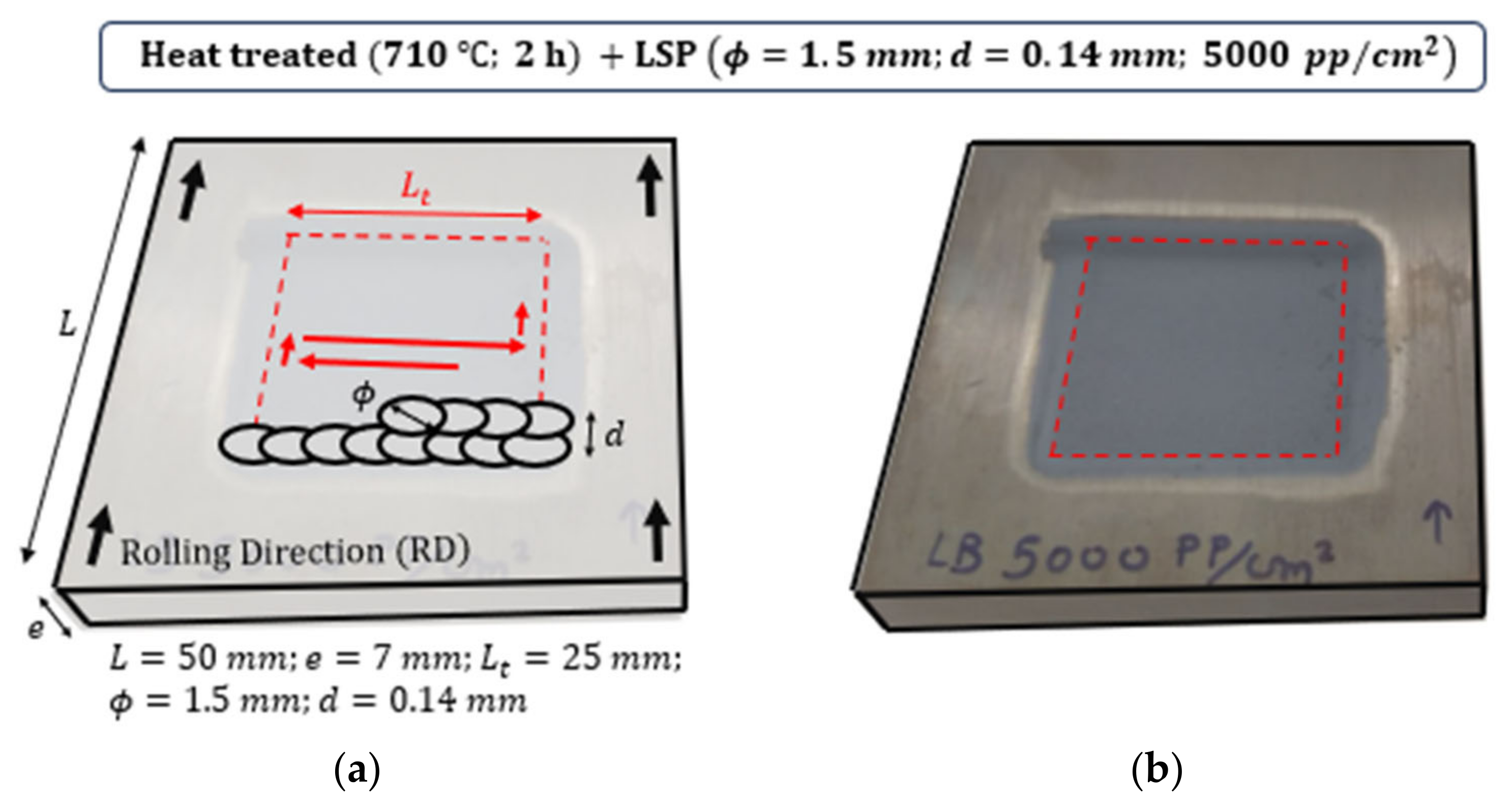
3.1. Experimental Set-Up for LSP Irradiation and Measurement of In-Depth Residual Stresses
3.2. FEM Model
3.2.1. Model Definition for LSP Simulation
3.2.2. Near-Surface Thermal Effect Simulation (Implicit Analysis)
4. Results
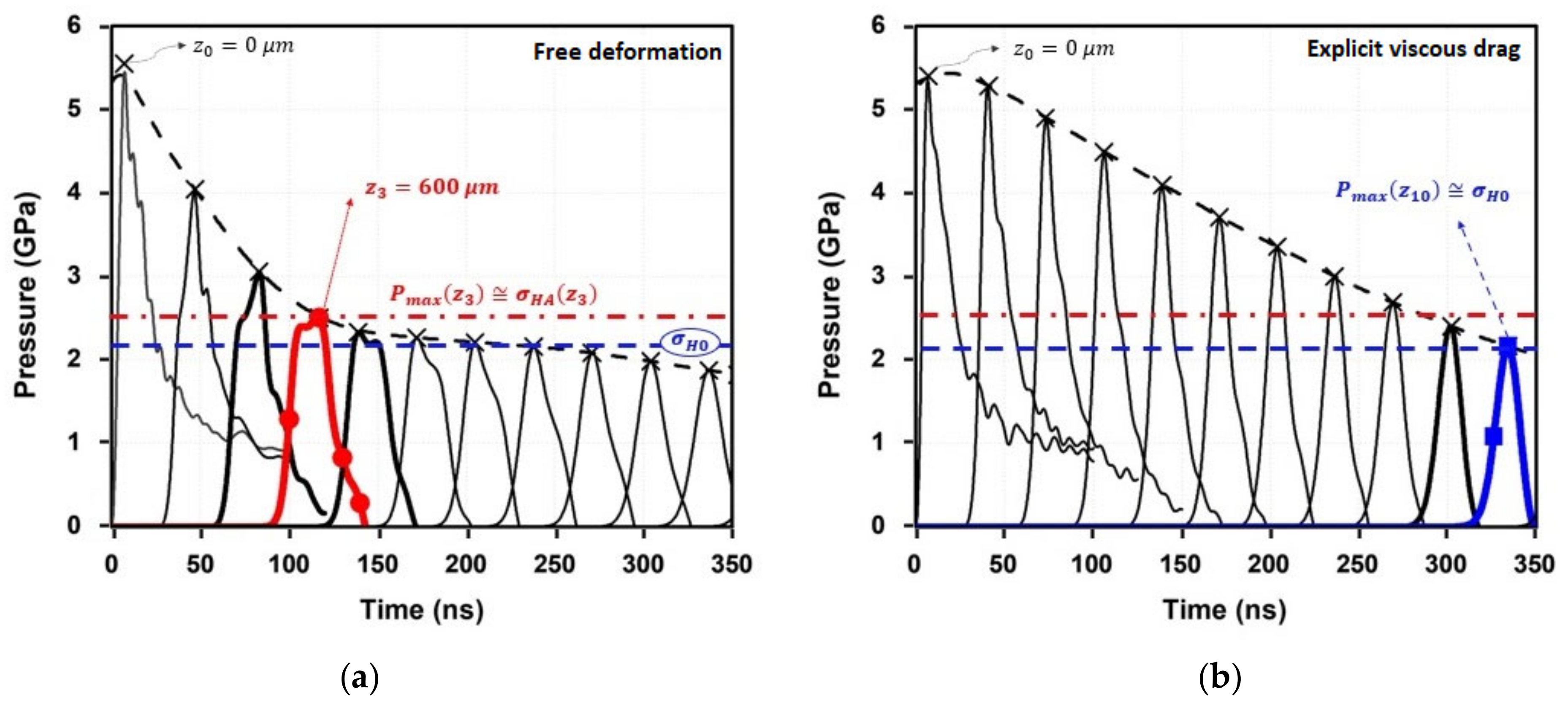
4.1. Analytical–Numerical Results for LSP Single Shots in Ti6Al4V
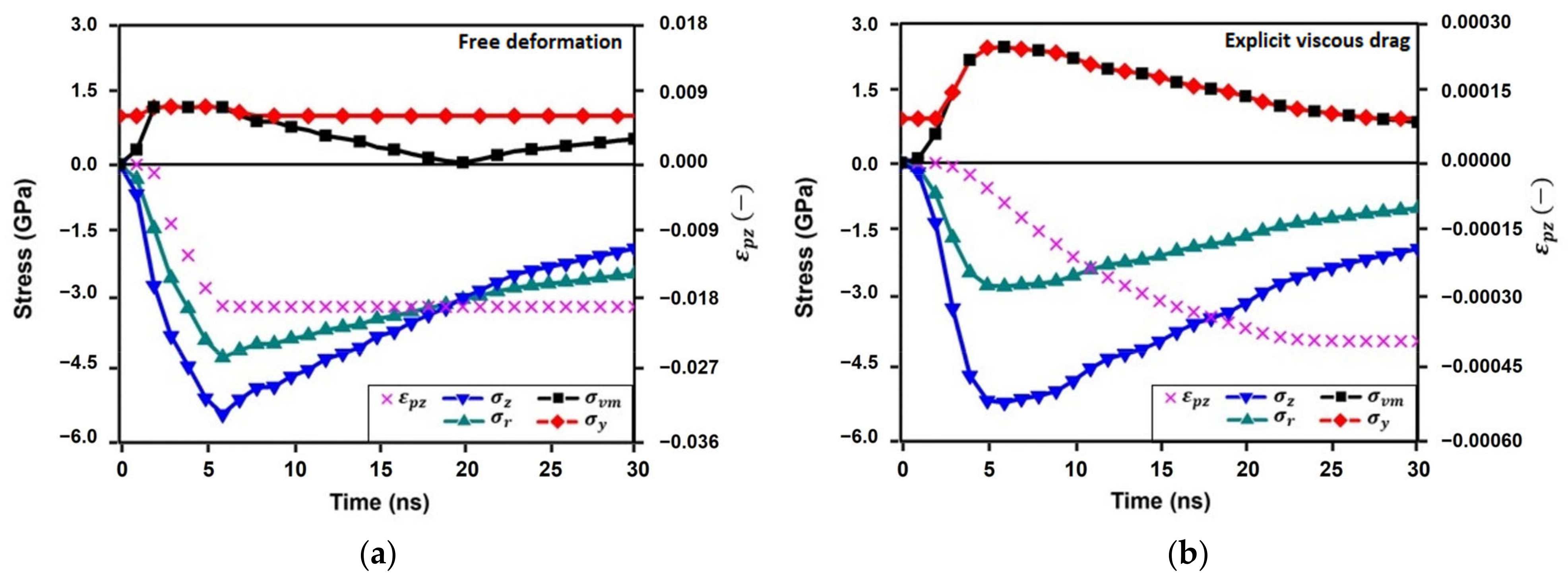
4.2. Realistic Thermomechanical Modeling of Extended Surface High-Coverage LSP Treatments with Explicit Consideration of the Viscous Drag Mechanism
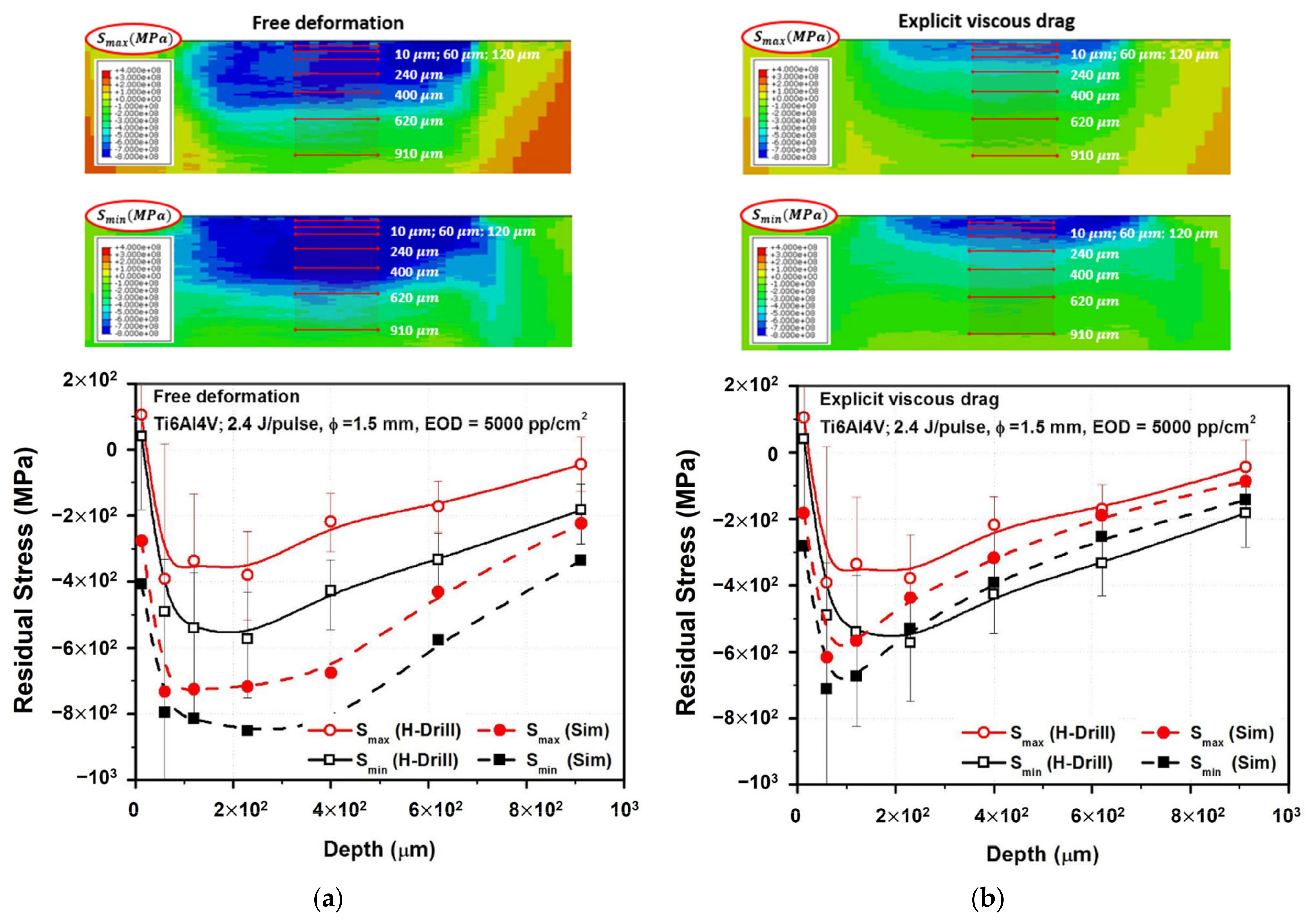
4.2.1. Near-Surface Residual Stress Calculation
4.2.2. In-Depth Numerical–Experimental Residual Stress Determination
5. Conclusions
- a.
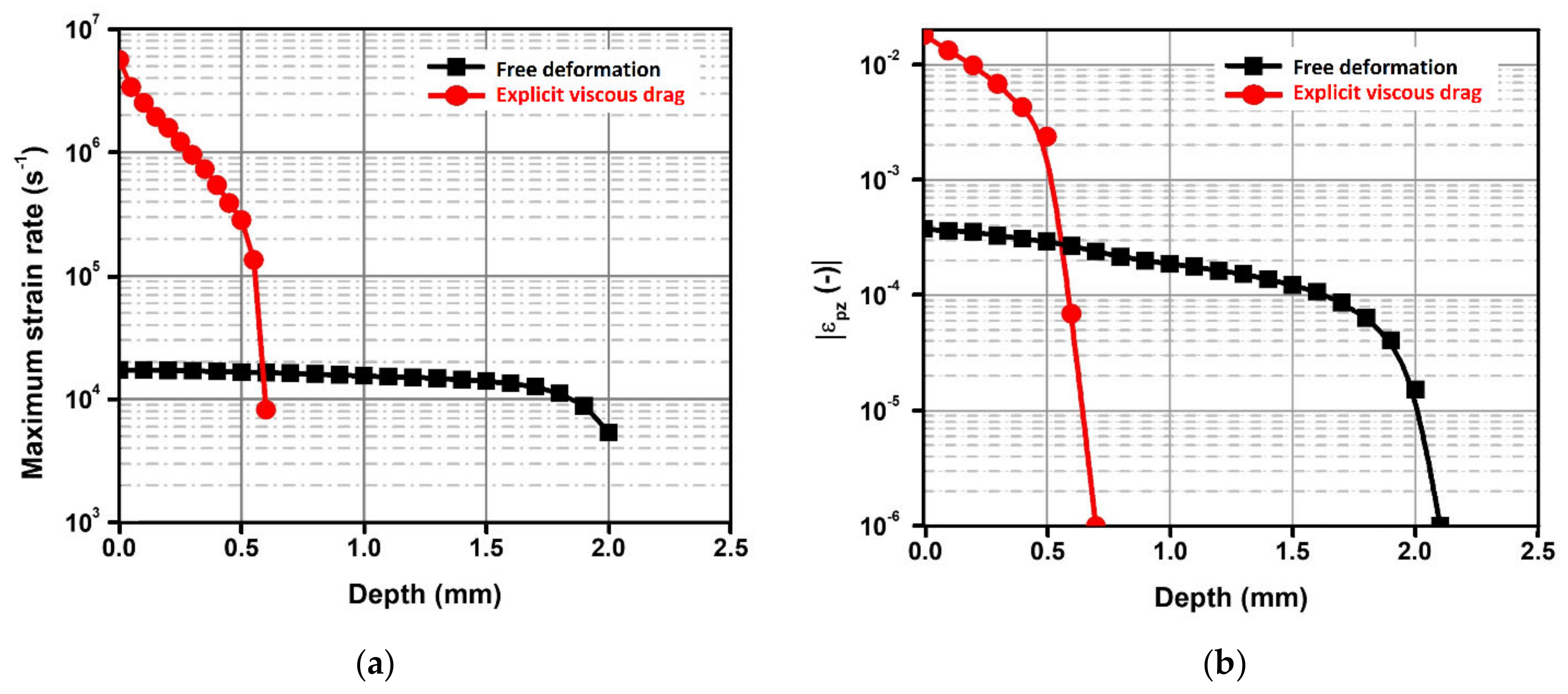
- The conventional models used in LSP modeling (i.e., the Johnson–Cook model) predict plastic strain rates of about 107 s−1. However, the solid theoretical argument supports the idea of a notable reduction in the plastic strain rate to 104 s−1 in alloys characterized by high yield stresses, for instance, Ti6Al4V alloy. The numerical–experimental results presented in this paper are consistent with this hypothesis.
- b.
- The time pressure decrease plays an extremely important role in the deformation mechanism. In fact, the significant increase in the yield stress at the viscous regime (104 s−1) imposes a threshold on the maximum plastic strain rate, and hence most of the deformation takes place during pressure decay, where two simultaneous conditions are presented: relatively high pressures with a smooth time evolution, which is responsible for extending the time during which deformation rates of about 104 s−1 take place. This fact justifies that reasonable deformations are obtained (with their corresponding conventional residual stresses), although much lower deformation rates are involved. The numerical–experimental results confirm that notable overestimations in the in-depth compressions are predicted by means of conventional modeling in very high-density treatments, whereas good agreement is obtained when viscous drag is explicitly considered.
- c.
- The calculated thermal effects in LSP induce significant reverse plastic straining through a very thin layer of material (about 10 μm is estimated for Ti6Al4V). This justifies the abrupt near-surface stress gradients: from very high tensile stresses (about the yield stress) at the surface to relatively high compressions (about −600 MPa) at 10 μm. Therefore, near-surface numerical–experimental validation can hardly be approached using the usual semi-destructive experimental residual stress measurements (i.e., the hole drilling method). X-ray diffraction methods may be suitable for this purpose.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Li, Y.; Gan, W.; Zhou, W.; Li, D. Review on residual stress and its effects on manufacturing of aluminium alloy structural panels with typical multi-processes. Chin. J. Aeronaut. 2023, 36, 96–124. [Google Scholar] [CrossRef]
- Li, C.; Liu, Z.Y.; Fang, X.Y.; Guo, Y.B. Residual stress in metal additive manufacturing. Procedia CIRP 2018, 71, 348–353. [Google Scholar] [CrossRef]
- Zhu, Q.; Chen, J.; Gou, G.; Chen, H.; Li, P. Ameliorated longitudinal critically refracted—Attenuation velocity method for welding residual stress measurement. J. Mater. Process. Technol. 2017, 246, 267–275. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, S.; Zhang, T.; Zhou, X.; Li, S. Effects of post-weld heat treatment on the microstructure and mechanical properties of laser-welded NiTi/304SS joint with Ni filler. Mater. Sci. Eng. A 2020, 771, 138545. [Google Scholar] [CrossRef]
- Morales, M.; Ocaña, J.; Molpeceres, C.; Porro, J.; García-Beltrán, A. Model based optimization criteria for the generation of deep compressive residual stress fields in high elastic limit metallic alloys by ns-laser shock processing. Surf. Coat. Technol. 2008, 202, 2257–2262. [Google Scholar] [CrossRef]
- Morales, M.; Porro, J.A.; Blasco, M.; Molpeceres, C.; Ocaña, J.L. Numerical simulation of plasma dynamics in laser shock processing experiments. Appl. Surf. Sci. 2009, 255, 5181–5185. [Google Scholar] [CrossRef]
- Angulo, I.; Cordovilla, F.; García-Beltrán, A.; Smyth, N.S.; Langer, K.; Fitzpatrick, M.E.; Ocaña, J.L. The effect of material cyclic deformation properties on residual stress generation by laser shock processing. Int. J. Mech. Sci. 2019, 156, 370–381. [Google Scholar] [CrossRef]
- Correa, C.; de Lara, L.R.; Díaz, M.; Porro, J.A.; García-Beltrán, A.; Ocaña, J.L. Influence of pulse sequence and edge material effect on fatigue life of Al2024-T351 specimens treated by laser shock processing. Int. J. Fatigue 2015, 70, 196–204. [Google Scholar] [CrossRef]
- Zhang, L.; Lu, J.Z.; Zhang, Y.K.; Luo, K.Y.; Zhong, J.W.; Cui, C.Y.; Kong, D.J.; Guan, H.B.; Qian, X.M. Effects of different shocked paths on fatigue property of 7050-T7451 aluminum alloy during two-sided laser shock processing. Mater. Des. 2011, 32, 480–486. [Google Scholar] [CrossRef]
- Zhang, X.Q.; Li, H.; Yu, X.L.; Zhou, Y.; Duan, S.W.; Li, S.Z.; Huang, Z.L.; Zuo, L.S. Investigation on effect of laser shock processing on fatigue crack initiation and its growth in aluminum alloy plate. Mater. Des. 2015, 65, 425–431. [Google Scholar] [CrossRef]
- Tang, Z.; Gao, J.; Xu, Z.; Guo, B.; Jiang, Q.; Chen, X.; Weng, J.; Li, B.; Chen, J.; Zhao, Z. Effect of Laser Shock Peening on the Fatigue Life of 1Cr12Ni3Mo2VN Steel for Steam Turbine Blades. Coatings 2023, 13, 1524. [Google Scholar] [CrossRef]
- Ganesh, P.; Sundar, R.; Kumar, H.; Kaul, R.; Ranganathan, K.; Hedaoo, P.; Raghavendra, G.; Kumar, S.A.; Tiwari, P.; Nagpure, D.; et al. Studies on fatigue life enhancement of pre-fatigued spring steel specimens using laser shock peening. Mater. Des. 2014, 54, 734–741. [Google Scholar] [CrossRef]
- Liu, Q.C.; Baburamani, P.; Zhuang, W.; Gerrard, D.; Hinton, B.; Janardhana, M.; Sharp, K. Surface modification and repair for aircraft life enhancement and structural restoration. In Materials Science Forum. Mater. Sci. Forum 2010, 654–656, 763–766. [Google Scholar] [CrossRef]
- Sharp, P.K.; Liu, Q.; Barter, S.A.; Baburamani, P.; Clark, G. Fatigue life recovery in aluminium alloy aircraft structure. Fatigue Fract. Eng. Mater. Struct. 2002, 25, 99–110. [Google Scholar] [CrossRef]
- Correa, C.; de Lara, L.R.; Díaz, M.; Gil-Santos, A.; Porro, J.A.; Ocaña, J.L. Effect of advancing direction on fatigue life of 316L stainless steel specimens treated by double-sided laser shock peening. Int. J. Fatigue 2015, 79, 1–9. [Google Scholar] [CrossRef]
- Nikitin, I.; Scholtes, B.; Maier, H.J.; Altenberger, I. High temperature fatigue behavior and residual stress stability of laser-shock peened and deep rolled austenitic steel AISI 304. Scr. Mater. 2004, 50, 1345–1350. [Google Scholar] [CrossRef]
- Prime, M.B.; Dewald, A.T. The contour method. In Practical Residual Stress Measurement Methods; Wiley: Hoboken, NJ, USA, 2013; pp. 109–138. [Google Scholar] [CrossRef]
- ASTM E837-13a; Standard Test Method for Determining Residual Stresses by the Hole-Drilling Strain-Gage Method. ASTM International: West Conshohocken, PA, USA, 2013.
- Shao, Z.; Zhang, C.; Li, Y.; Shen, H.; Zhang, D.; Yu, X.; Zhang, Y. A Review of Non-Destructive Evaluation (NDE) Techniques for Residual Stress Profiling of Metallic Components in Aircraft Engines. Aerospace 2022, 9, 534. [Google Scholar] [CrossRef]
- Couque, H. The use of the direct impact Hopkinson pressure bar technique to describe thermally activated and viscous regimes of metallic materials. Philos. Trans. R. Soc. A 2014, 372, 20130218. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures. Eng. Fract. Mech. 1985, 21, 31–48. [Google Scholar] [CrossRef]
- Khan, A.S.; Huang, S. Experimental and theoretical study of mechanical behavior of 1100 aluminum in the strain rate range 10−5–104 s−1. Int. J. Plast. 1992, 8, 397–424. [Google Scholar] [CrossRef]
- Khan, A.S.; Liang, R. Behaviors of three BCC metal over a wide range of strain rates and temperatures: Experiments and modeling. Int. J. Plast. 1999, 15, 1089–1109. [Google Scholar] [CrossRef]
- Khan, A.S.; Zhang, H. Mechanically alloyed nanocrystalline iron and copper mixture: Behavior and constitutive modeling over a wide range of strain rates. Int. J. Plast. 2000, 16, 1477–1492. [Google Scholar] [CrossRef]
- Khan, A.S.; Zhang, H. Finite deformation of a polymer: Experiments and modeling. Int. J. Plast. 2001, 17, 1167–1188. [Google Scholar] [CrossRef]
- Kim, H.; Yoon, J.W.; Chung, K.; Lee, M.-G. A multiplicative plastic hardening model in consideration of strain softening and strain rate: Theoretical derivation and characterization of model parameters with simple tension and creep test. Int. J. Mech. Sci. 2020, 187, 105913. [Google Scholar] [CrossRef]
- Gao, C.Y.; Zhang, L.C.; Yan, H.X. A new constitutive model for HCP metals. Mater. Sci. Eng. A 2011, 528, 4445–4452. [Google Scholar] [CrossRef]
- Gao, C.Y.; Zhang, L.C. Constitutive modelling of plasticity of fcc metals under extremely high strain rates. Int. J. Plast. 2012, 32–33, 121–133. [Google Scholar] [CrossRef]
- Sedaghat, H.; Xu, W.; Zhang, L.; Liu, W. On the constitutive models for ultra-high strain rate deformation of metals. Int. J. Automot. Technol. 2019, 20, 31–37. [Google Scholar] [CrossRef]
- Zerilli, F.J.; Armstrong, R.W. Dislocation-mechanics-based constitutive relations for material dynamics calculations. J. Appl. Phys. 1987, 61, 1816–1825. [Google Scholar] [CrossRef]
- Dharan, C.K.H.; Hauser, F.E. Determination of stress-strain characteristics at very high strain rates. Exp. Mech. 1970, 10, 370–376. [Google Scholar] [CrossRef]
- Couque, H.; Boulanger, R.; Bornet, F. A modified Johnson-Cook model for strain rates ranging from 10−3 to 105 s−1. J. Phys. IV Fr. 2006, 134, 87–93. [Google Scholar] [CrossRef]
- Nabarro, F.R.N. Dislocations in a simple cubic lattice. Proc. Phys. Soc. 1947, 59, 256. [Google Scholar] [CrossRef]
- Wickham, L.K.; Schwarz, K.W.; Stölken, J.S. Rules for Forest Interactions between Dislocations. Phys. Rev. Lett. 1999, 83, 4574. [Google Scholar] [CrossRef]
- Swegle, J.W.; Grady, D.E. Shock viscosity and the prediction of shock wave rise times. J. Appl. Phys. 1985, 58, 692–701. [Google Scholar] [CrossRef]
- Kattoura, M.; Shehadeh, M.A. On the ultra-high-strain rate shock deformation in copper single crystals: Multiscale dislocation dynamics simulations. Philos. Mag. Lett. 2014, 94, 415–423. [Google Scholar] [CrossRef]
- Adu-Gyamfi, S.; Ren, X.D.; Larson, E.A.; Ren, Y.; Tong, Z. The effects of laser shock peening scanning patterns on residual stress distribution and fatigue life of AA2024 aluminium alloy. Opt. Laser Technol. 2018, 108, 177–185. [Google Scholar] [CrossRef]
- Bikdeloo, R.; Farrahi, G.H.; Mehmanparast, A.; Mahdavi, S.M. Multiple laser shock peening effects on residual stress distribution and fatigue crack growth behaviour of 316L stainless steel. Theor. Appl. Fract. Mech. 2020, 105, 102429. [Google Scholar] [CrossRef]
- Zhang, X.; Huang, Z.; Chen, B.; Zhang, Y.; Tong, J.; Fang, G.; Duan, S. Investigation on residual stress distribution in thin plate subjected to two sided laser shock processing. Opt. Laser Technol. 2019, 111, 146–155. [Google Scholar] [CrossRef]
- Zhou, W.; Ren, X.; Yang, Y.; Tong, Z.; Larson, E.A. Finite element analysis of laser shock peening induced near-surface deformation in engineering metals. Opt. Laser Technol. 2019, 119, 105608. [Google Scholar] [CrossRef]
- Simon, P.; Demarty, Y.; Rusinek, A.; Voyiadjis, G.Z. Material behavior description for a large range of strain rates from low to high temperatures: Application to high strength steel. Metals 2018, 8, 795. [Google Scholar] [CrossRef]
- Wang, C.; Wang, X.; Xu, Y.; Gao, Z. Numerical modeling of the confined laser shock peening of the OFHC copper. Int. J. Mech. Sci. 2016, 108–109, 104–114. [Google Scholar] [CrossRef]
- Wang, C.; Li, K.; Hu, X.; Yang, H.; Zhou, Y. Numerical study on laser shock peening of TC4 titanium alloy based on the plate and blade model. Opt. Laser Technol. 2021, 142, 107163. [Google Scholar] [CrossRef]
- Zhou, Z.; Bhamare, S.; Ramakrishnan, G.; Mannava, S.R.; Langer, K.; Wen, Y.; Qian, D.; Vasudevan, V.K. Thermal relaxation of residual stress in laser shock peened Ti–6Al–4V alloy. Surf. Coat. Technol. 2012, 206, 4619–4627. [Google Scholar] [CrossRef]
- Ballard, P.; Fournier, J.; Fabbro, R.; Frelat, J. Residual stresses induced by laser-shocks. J. Phys. IV Fr. 1991, 1, C3-487–C3-494. [Google Scholar] [CrossRef]
- Yao, S.; Yu, J.; Cui, Y.; Pei, X.; Yu, Y.; Wu, Q. Revisiting the power law characteristics of the plastic shock front under shock loading. Phys. Rev. Lett. 2021, 126, 085503. [Google Scholar] [CrossRef] [PubMed]
- Angulo, I.; Cordovilla, F.; García-Beltrán, A.; Porro, J.A.; Díaz, M.; Ocaña, J.L. Integrated Numerical-Experimental Assessment of the Effect of the AZ31B Anisotropic Behaviour in Extended-Surface Treatments by Laser Shock Processing. Metals 2020, 10, 195. [Google Scholar] [CrossRef]
- Ocaña, J.; Morales, M.; Porro, J.; Blasco, M.; Molpeceres, C.; Iordachescu, D.; Gómez-Rosas, G.; Rubio-González, C. Induction of engineered residual stresses fields and associate surface properties modification by short pulse laser shock processing. Mater. Sci. Forum 2010, 638–642, 2446–2451. [Google Scholar] [CrossRef]
- Peral, D.; de Vicente, J.; Porro, J.A.; Ocaña, J.L. Uncertainty analysis for non-uniform residual stresses determined by the hole drilling strain gauge method. Measurement 2017, 97, 51–63. [Google Scholar] [CrossRef]
- Morales, M.; Correa, C.; Porro, J.A.; Molpeceres, C.; Ocaña, J.L. Thermomechanical modelling of stress fields in metallic targets subject to laser shock processing. Int. J. Struct. Integr. 2011, 2, 51–61. [Google Scholar] [CrossRef]
- Peyre, P.; Carboni, C.; Forget, P.; Beranger, G.; Lemaitre, C.; Stuart, D. Influence of thermal and mechanical surface modifications induced by laser shock processing on the initiation of corrosion pits in 316L stainless steel. J. Mater. Sci. 2007, 42, 6866–6877. [Google Scholar] [CrossRef]
- Lesuer, D.R. Experimental Investigations of Material Models for Ti-6A1-4V Titanium and 2024-T3 Aluminum; No. DOT/FAA/AR-00/25; U.S. Department of Transportation: Washington, DC, USA, 2000. [Google Scholar]

| Element | Al | V | C | O | N | Ti |
|---|---|---|---|---|---|---|
| Weight % | 6.1 | 4.2 | 0.01 | 0.12 | 0.006 | Bal. |
| Time (ns) | Irradiation, (GW/cm2) | Heat Flux, (MW/cm2) | Pressure, (MPa) |
|---|---|---|---|
| 0 | 0 | 0 | 0 |
| 2 | 8 | 20 | 3370 |
| 5 | 20 | 50 | 5350 |
| 6 | 18 | 60 | 5183 |
| 10 | 11 | 110 | 4514 |
| 16 | 3 | 63 | 3511 |
| 23 | 0 | 42 | 2340 |
| 25 | 0 | 36 | 2177 |
| 30 | 0 | 32 | 1770 |
| 50 | 0 | 18 | 1160 |
| 100 | 0 | 9 | 840 |
| 300 | 0 | 3 | 617 |
| 400 | 0 | 2 | 506 |
| 770 | 0 | 0 | 0 |
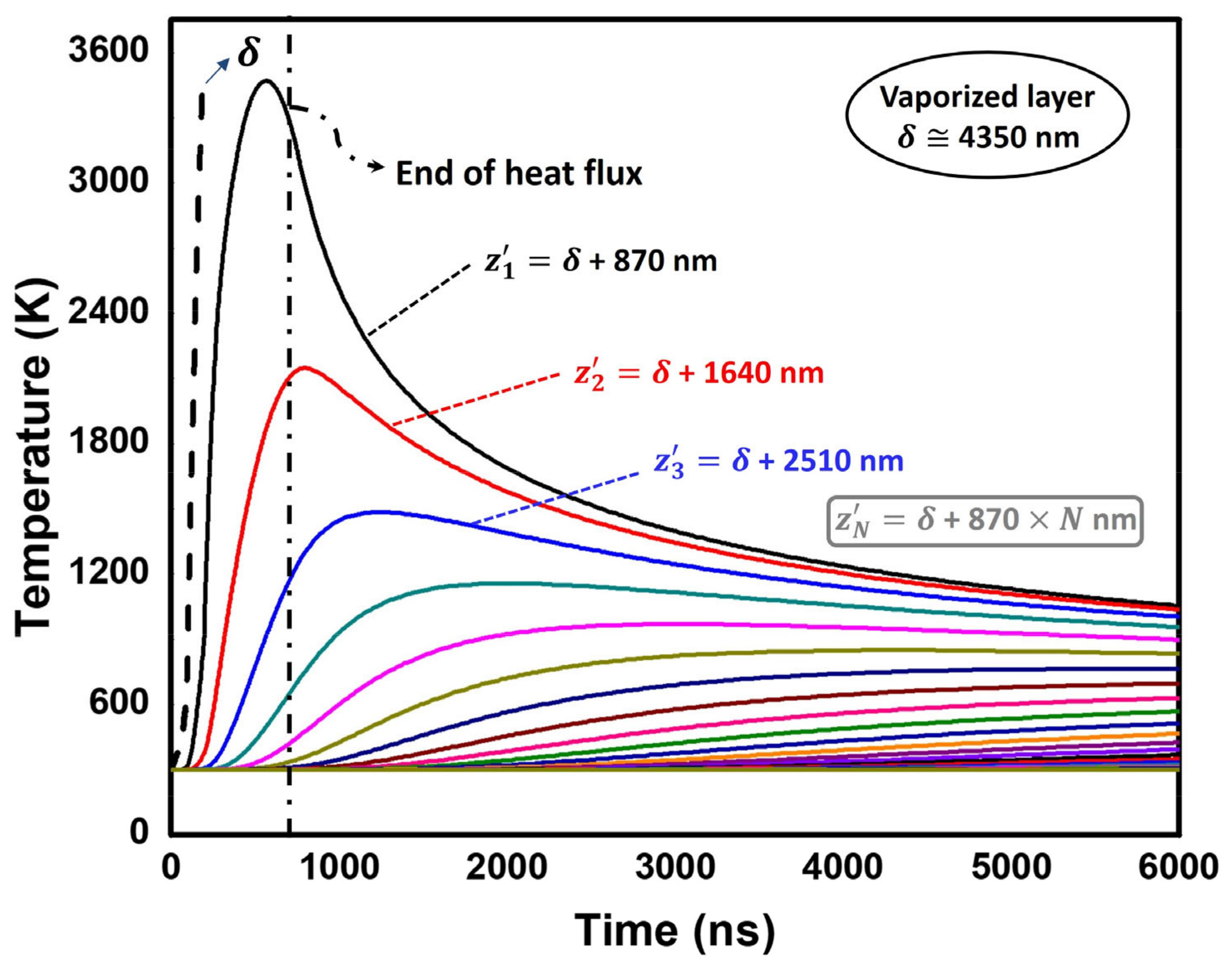
| Depth (nm) | (MPa) | (MPa) |
|---|---|---|
| 0 | 1254 | 1398 |
| 870 | 849 | 1071 |
| 1740 | 473 | 657 |
| 2610 | 153 | 328 |
| 3480 | −48 | 102 |
| 4350 | −190 | −44 |
| 5220 | −308 | −178 |
| 6090 | −408 | −296 |
| 6960 | −504 | −410 |
| 7830 | −576 | −499 |
| 8700 | −609 | −541 |
| 9570 | −613 | −544 |
| Experimental Results | Free Deformation | Explicit Viscous Drag | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Depth (µm) | (MPa) | (MPa) | (MPa) | (MPa) | (MPa) | (MPa) | ||||
| 10 | 41 | 104 | −405 | −276 | 1088 | 365 | −283 | −181 | 790 | 274 |
| 60 | −488 | −392 | −795 | −732 | 63 | 87 | −711 | −619 | 46 | 58 |
| 120 | −541 | −336 | −812 | −723 | 50 | 115 | −675 | −566 | 25 | 68 |
| 240 | −571 | −379 | −852 | −717 | 49 | 89 | −533 | −438 | 7 | 16 |
| 400 | −426 | −218 | −803 | −678 | 88 | 211 | −388 | −316 | 9 | 45 |
| 620 | −335 | −176 | −579 | −434 | 73 | 147 | −254 | −188 | 24 | 7 |
| 910 | −184 | −48 | −336 | −227 | 83 | 373 | −142 | −86 | 23 | 79 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Angulo, I.; Warzanskyj, W.; Cordovilla, F.; Díaz, M.; Porro, J.A.; García-Beltrán, Á.; Ocaña, J.L. The Effect of Viscous Drag on the Maximum Residual Stresses Achievable in High-Yield-Strength Materials in Laser Shock Processing. Materials 2023, 16, 6858. https://doi.org/10.3390/ma16216858
Angulo I, Warzanskyj W, Cordovilla F, Díaz M, Porro JA, García-Beltrán Á, Ocaña JL. The Effect of Viscous Drag on the Maximum Residual Stresses Achievable in High-Yield-Strength Materials in Laser Shock Processing. Materials. 2023; 16(21):6858. https://doi.org/10.3390/ma16216858
Chicago/Turabian StyleAngulo, Ignacio, Wsewolod Warzanskyj, Francisco Cordovilla, Marcos Díaz, Juan Antonio Porro, Ángel García-Beltrán, and José Luis Ocaña. 2023. "The Effect of Viscous Drag on the Maximum Residual Stresses Achievable in High-Yield-Strength Materials in Laser Shock Processing" Materials 16, no. 21: 6858. https://doi.org/10.3390/ma16216858
APA StyleAngulo, I., Warzanskyj, W., Cordovilla, F., Díaz, M., Porro, J. A., García-Beltrán, Á., & Ocaña, J. L. (2023). The Effect of Viscous Drag on the Maximum Residual Stresses Achievable in High-Yield-Strength Materials in Laser Shock Processing. Materials, 16(21), 6858. https://doi.org/10.3390/ma16216858







