Benchmarking Standard and Micromechanical Models for Creep and Shrinkage of Concrete Relevant for Nuclear Power Plants
Abstract
:1. Introduction
- Micromechanical-based models, particularly
2. Materials
2.1. NPP Concretes and Conditions
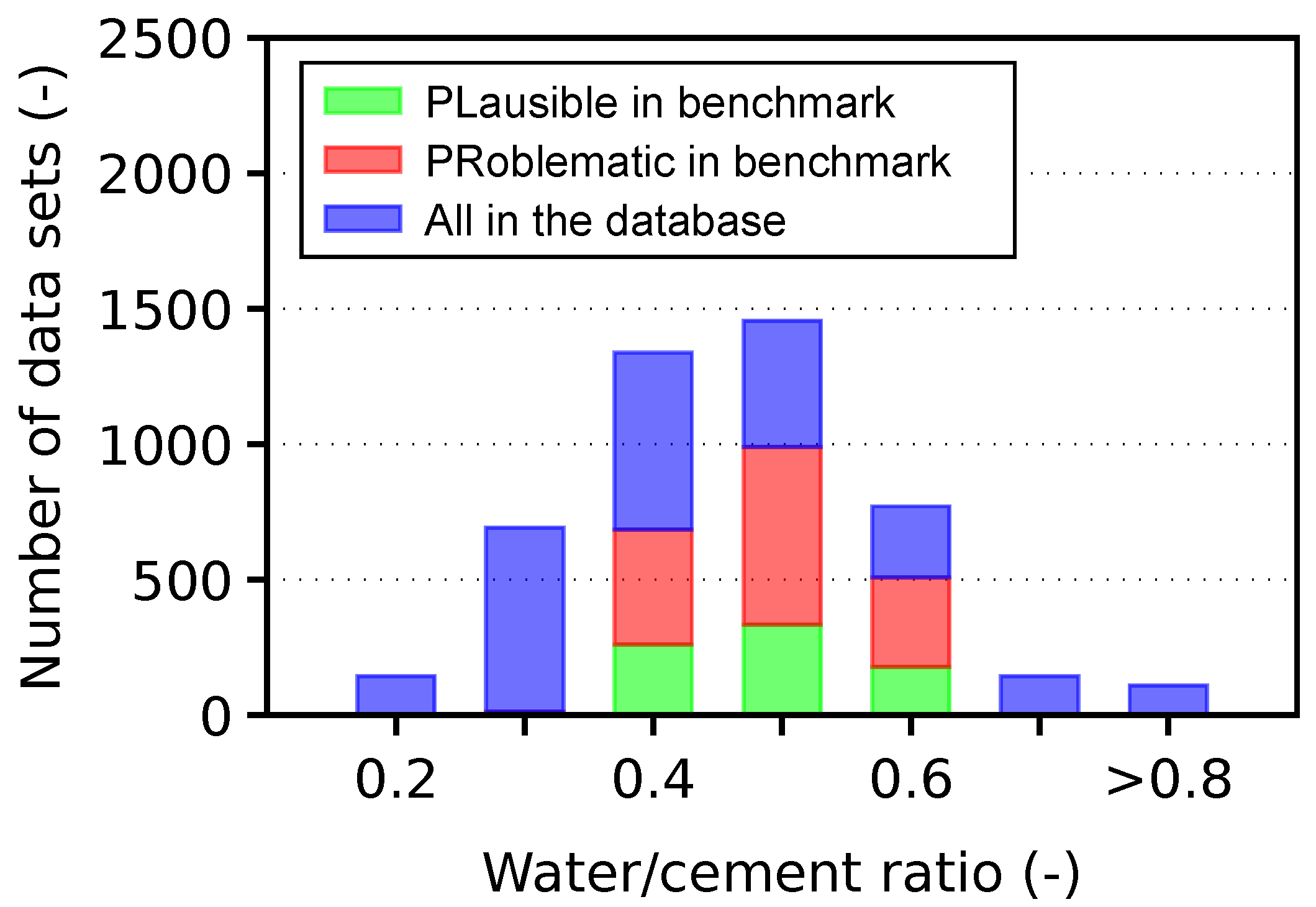
- Water/cement ratio 0.30 0.65;
- Cement mass 250 450 kg/m;
- Cylinder mean compressive strength at 28 days 20 MPa ≤≤ 80 MPa;
- Relative humidity 0.30 ≤ RH ≤ 1.0;
- Constant temperature 0 °C < T ≤ 65 °C.
2.2. VeRCoRs Concrete
3. Creep and Shrinkage Models
3.1. Strain Decomposition in the Standard B3, B4, and EC2 Models
3.2. Micromechanical Vi(CA)T Model
- Cement hydration models to estimate the evolutions of the amounts of each cement paste phase based on the initial mix design information and the aging conditions, combining the chemistry and kinetics of the hydration reactions;
- Homogenization models to estimate the effective properties and behaviors based on the volume fractions and elementary behaviours of phases and morphological models using upscaling techniques.
3.3. Micromechanical SCK CEN Model for Drying Shrinkage
3.4. Coefficient of Variation of Error
4. Results and Discussion for Creep and Shrinkage Models
4.1. Revamping of the NU Database and Data Credibility
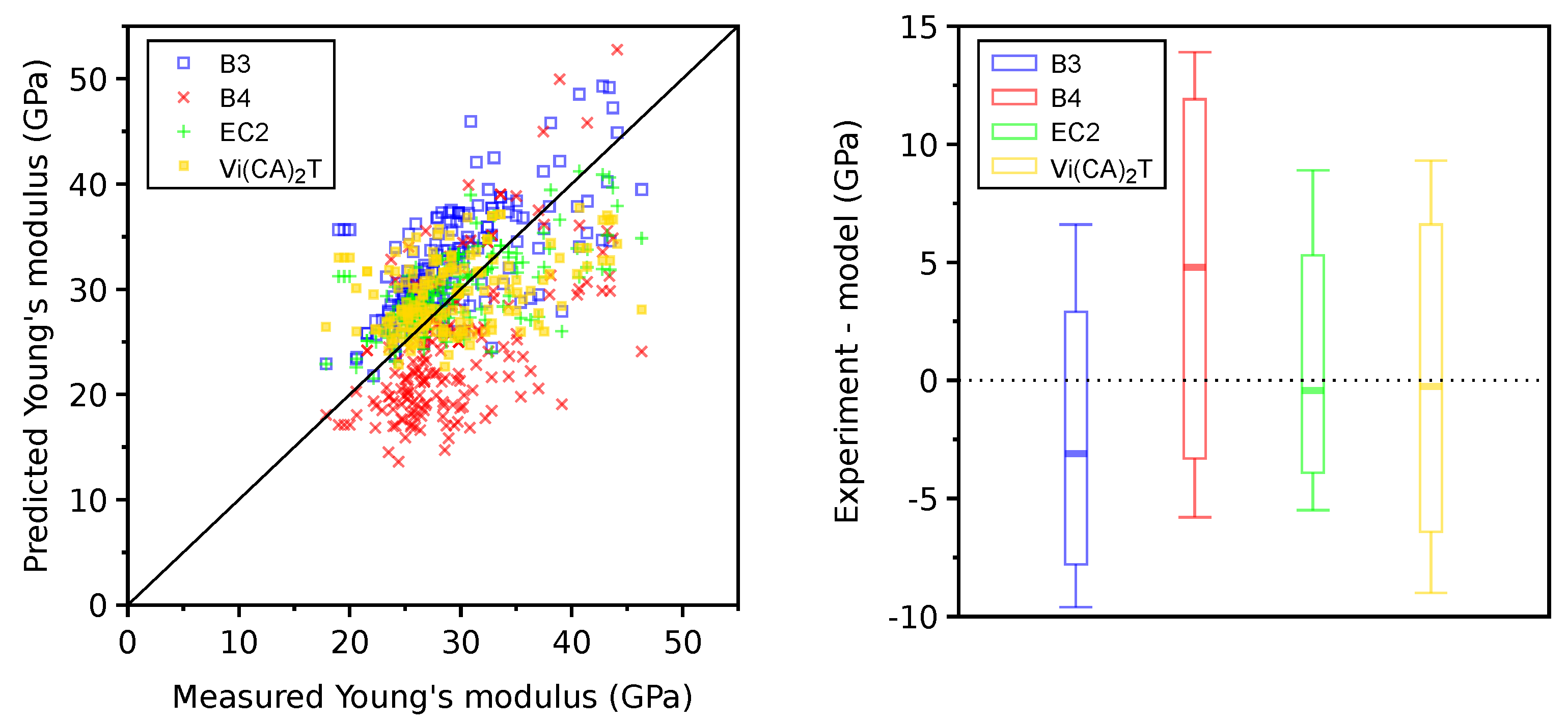
4.2. Young’s Modulus at 28 Days
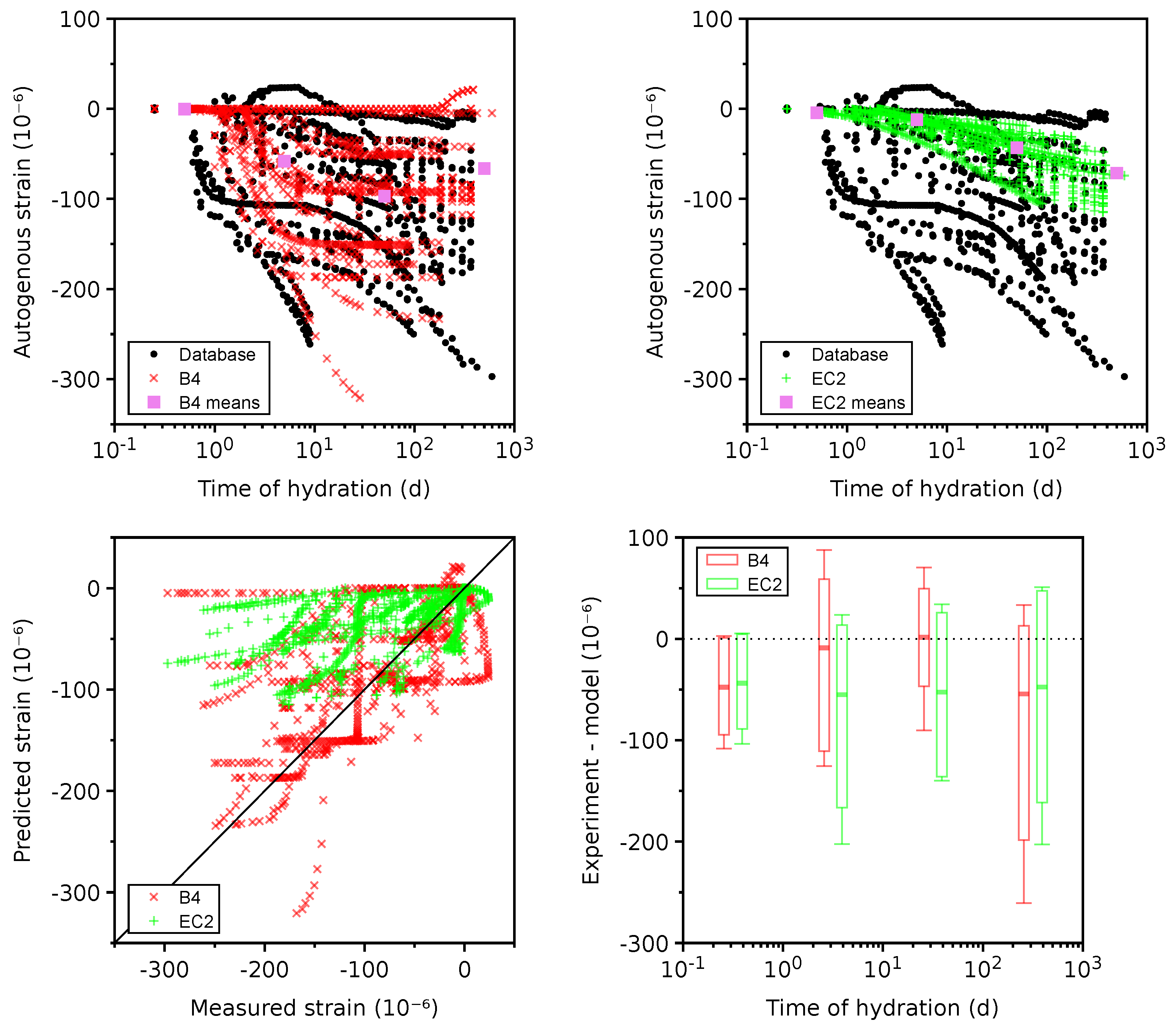
4.3. Autogenous Shrinkage
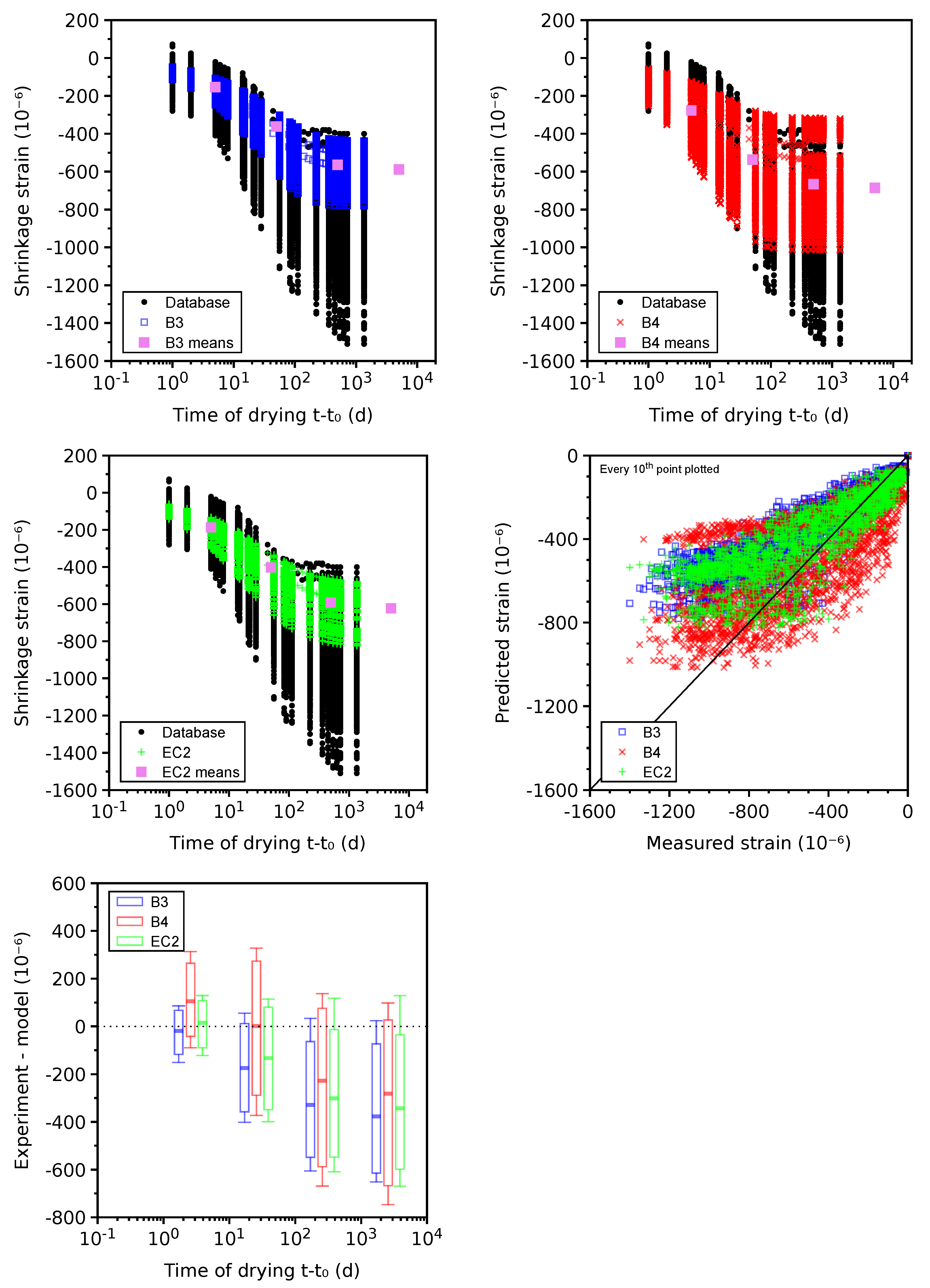
4.4. Drying Shrinkage
4.5. Total Shrinkage
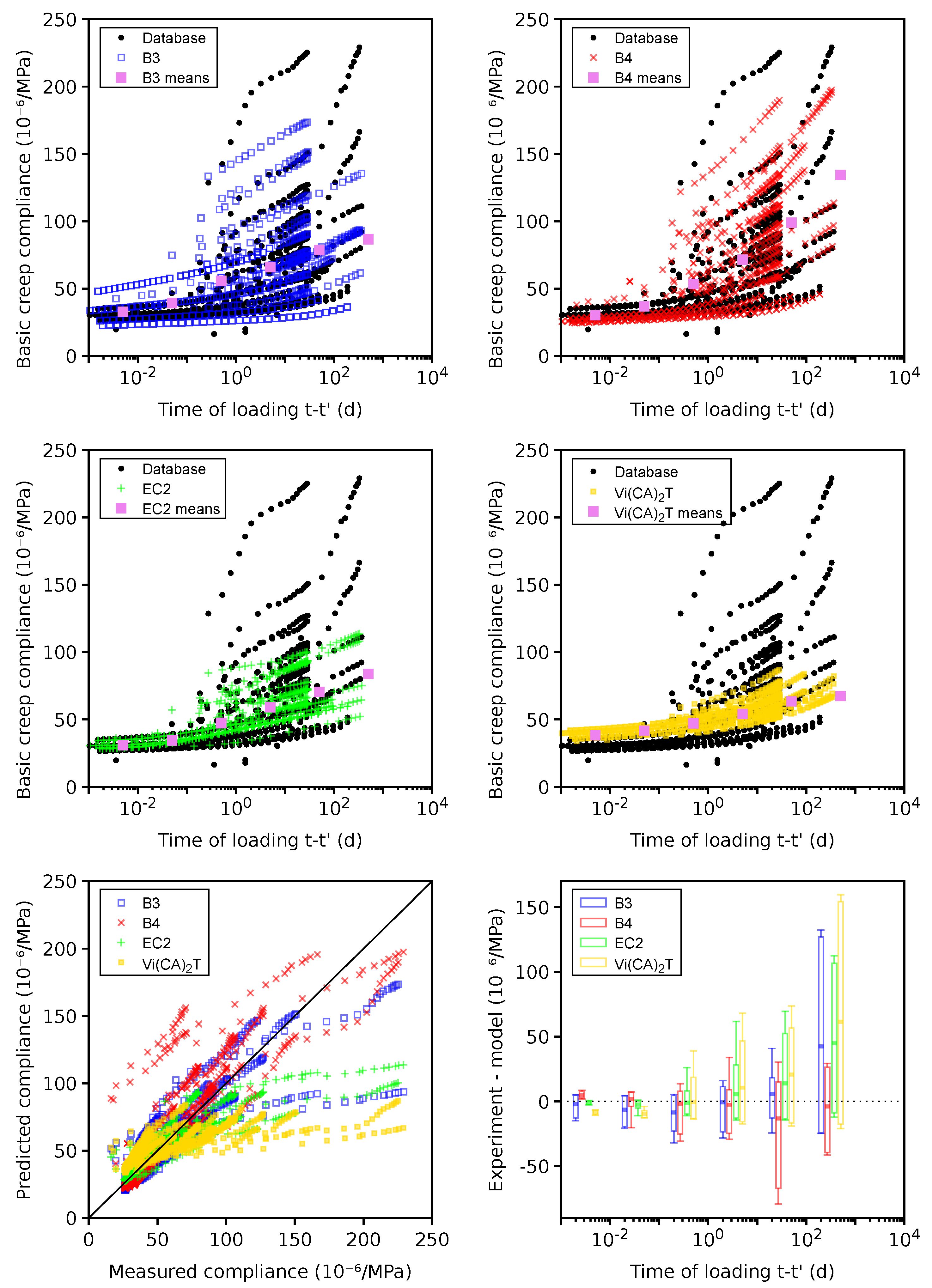
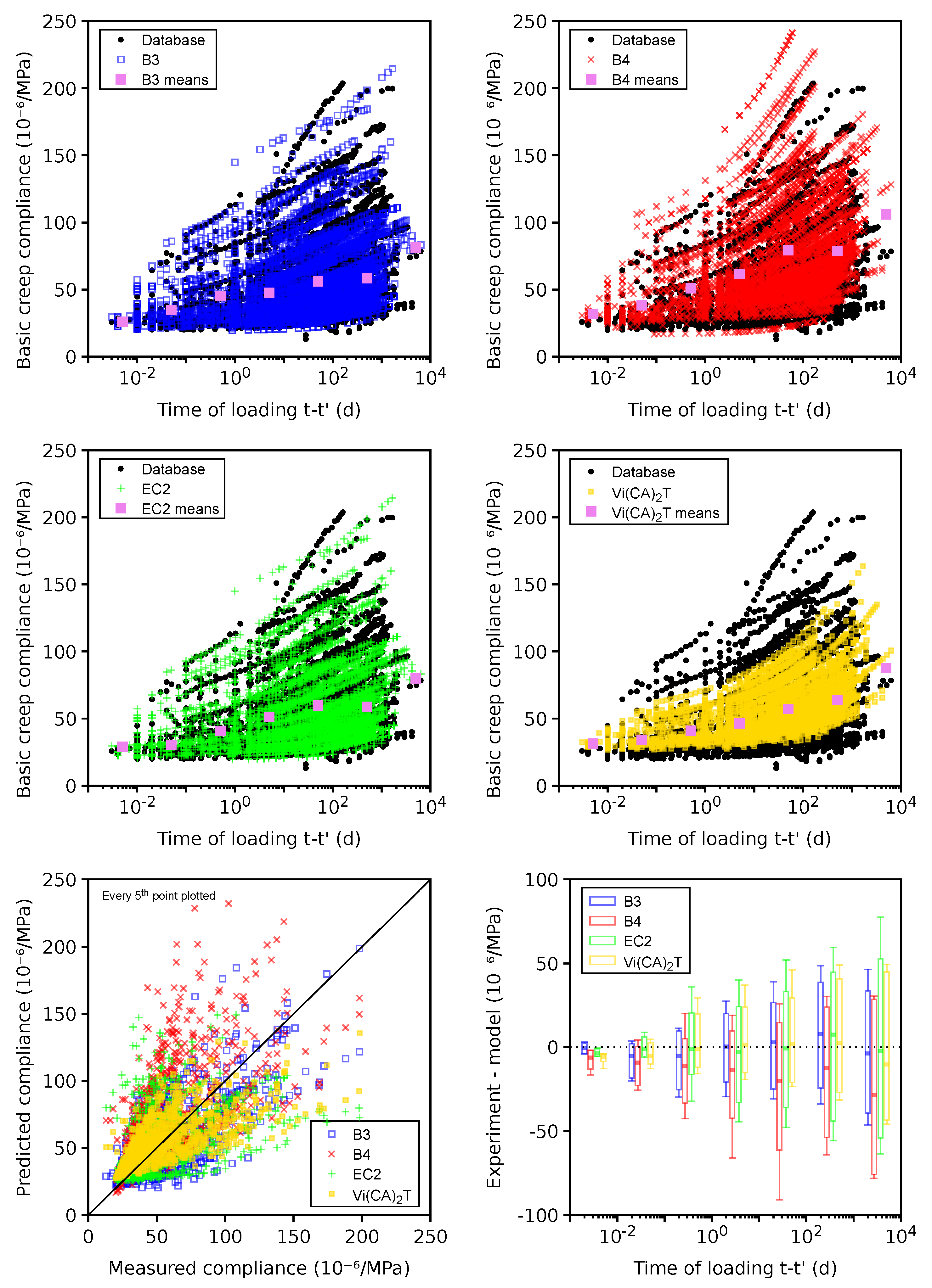
4.6. Basic Creep
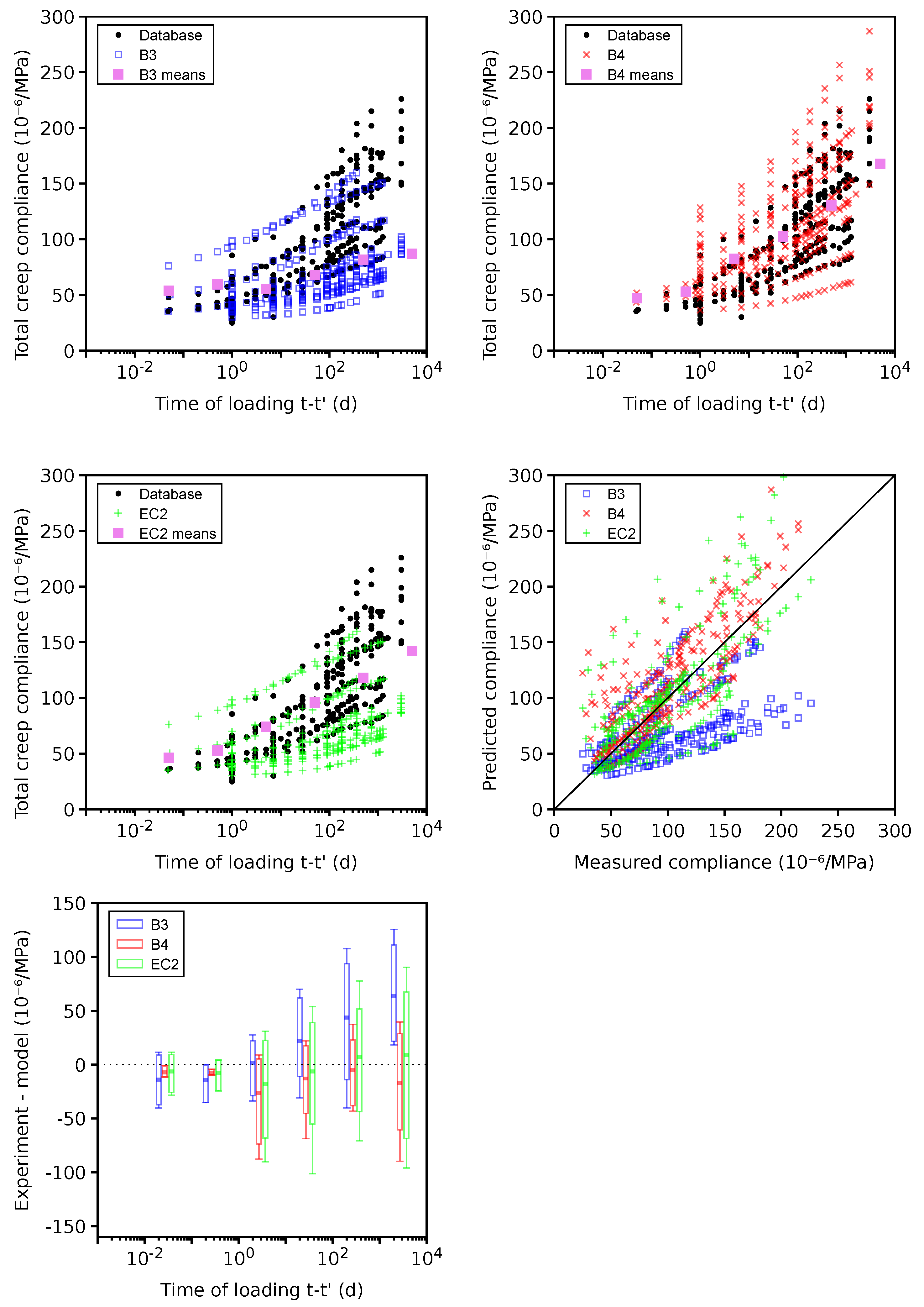
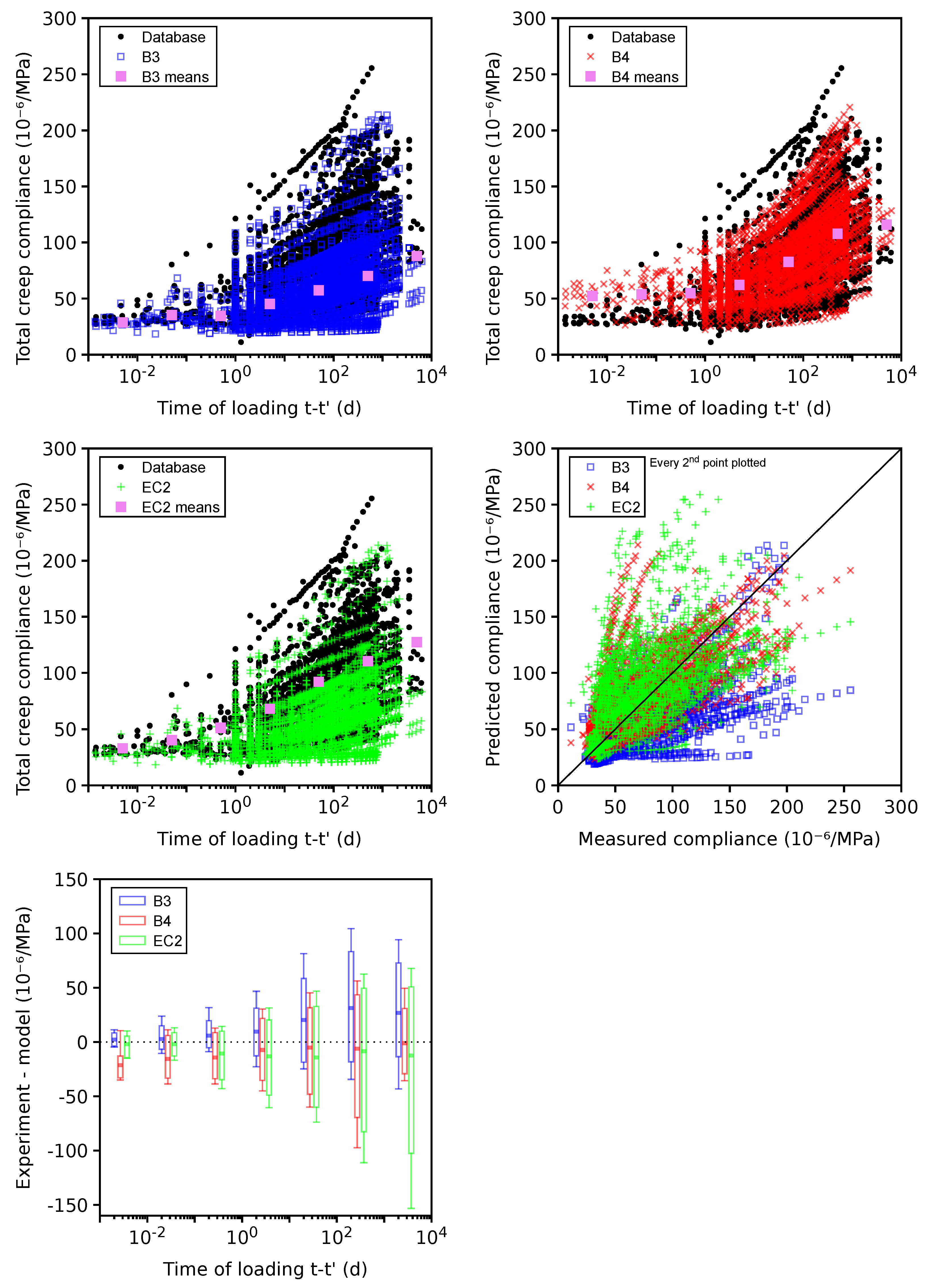
4.7. Total Creep
4.8. VeRCoRs Concrete
4.9. Differences in Total Shrinkage between Portland and Blended Cements
4.10. SCK CEN Model for Drying Shrinkage
5. Conclusions
- The EC2 model shows the best prediction for the autogenous, drying, and total shrinkage, both for the plausible and problematic data sets in terms of CoV of error. The EC2 model provides the best prediction of the Young’s modulus at 28 days;
- The B3 and B4 models exhibit the best performance for the basic and total creep, both for the plausible and problematic data sets;
- The SCK CEN model for water desorption isotherm yields a reasonably good estimation compared to the experimental results. This is also reflected in the reasonably good estimation of ultimate shrinkage strain, with the model overestimating the shrinkage strain of the cement paste and concrete by ≈20% and ≈7%, respectively.
- The micromechanical model Vi(CA)T shows the second best prediction for the 28-day Young’s modulus and yields comparable CoV values for the basic creep. It was the first time that a micromechanical model was benchmarked against such large data sets. The model used the constant intrinsic properties of aggregates and the same generic model for cement, which can be improved;
- Autogenous shrinkage shows in general a high coefficient of variation, which is likely caused by various chemical admixtures. When is approximately below 0.50, the autogenous shrinkage becomes non-negligible and direct measurements are preferred due to low time demand. The question of the final asymptotic value has not been resolved yet [68];
- Concretes made from blended cement increase the final total shrinkage by a factor of 1.27 on average when matched with comparable Portland cement concretes. Future development needs to take into account the dominance of blended cement and the impact on creep and shrinkage;
- Although the focus of this paper deals with NPP concretes, the findings are transferable to similar concretes in buildings, bridges, dams, and near surface and deep geological nuclear waste disposals.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Moore, J.; Tcherner, J.; Naus, D.; Bakirov, M.; Puttonen, J.; Móga, I. Ageing Management of Concrete Structures in Nuclear Power Plants; Number NP-T-3.5 in Nuclear Energy Series; International Atomic Energy Agency: Vienna, Austria, 2016. [Google Scholar]
- Do, M.J.; Chockie, A.D. Aging Degradation of Concrete Structures in Nuclear Power Plants; Report; Battelle Seattle Research Center: Seattle, WA, USA, 1994. [Google Scholar]
- Basu, P.C.; Labbe, P.; Naus, D. Nuclear power plant concete structures. In Proceedings of the 22nd International Conference on Structural Mechanics in Reactor Technology (SMiRT-22), San Francisco, CA, USA, 18–23 August 2013; pp. 1–30. [Google Scholar]
- Wang, Z.; Yan, J.; Lin, Y.; Fang, T.; Ma, J. Study on failure mechanism of prestressed concrete containments following a loss of coolant accident. Eng. Struct. 2020, 202, 109860. [Google Scholar] [CrossRef]
- Charpin, L.; Niepceron, J.; Corbin, M.; Masson, B.; Mathieu, J.P.; Haelewyn, J.; Hamon, F.; Åhs, M.; Aparicio, S.; Asali, M.; et al. Ageing and air leakage assessment of a nuclear reactor containment mock-up: VERCORS 2nd benchmark. Nucl. Eng. Des. 2021, 377, 111136. [Google Scholar] [CrossRef]
- Meng, T.; Jason, L.; Heitz, T.; Richard, B. Numerical methodology on prestressed reinforced concrete containment building: Creep, aging and leakage. Application to VERCORS mock-up. Eng. Struct. 2023, 280, 115625. [Google Scholar] [CrossRef]
- Özen, S.; Şengül, C.; Erenoğlu, T.; Çolak, U.; Reyhancan, I.A.; TaŞdemir, M.A. Properties of Heavyweight Concrete for Structural and Radiation Shielding Purposes. Arab. J. Sci. Eng. 2016, 41, 1573–1584. [Google Scholar] [CrossRef]
- Kanagaraj, B.; Anand, N.; Diana Andrushia, A.; Naser, M. Recent developments of radiation shielding concrete in nuclear and radioactive waste storage facilities—A state of the art review. Constr. Build. Mater. 2023, 404, 133260. [Google Scholar] [CrossRef]
- Giorla, A.; Vaitová, M.; Le Pape, Y.; Štemberk, P. Meso-scale modeling of irradiated concrete in test reactor. Nucl. Eng. Des. 2015, 295, 59–73. [Google Scholar] [CrossRef]
- Giorla, A.B.; Pape, Y.L.; Dunant, C.F. Computing Creep-Damage Interactions inIrradiated Concrete. J. Nanomech. Micromech. 2017, 17, 04017001. [Google Scholar] [CrossRef]
- Bažant, Z.; Baweja, S. Creep and shrinkage prediction model for analysis and design of concrete structures: Model B3. ACI Spec. Publ. 2000, 194, 1–77. [Google Scholar]
- Baweja, S.; Bažant, Z.P. Creep and shrinkage prediction model for analysis and design of concrete structures—Model B3. Mater. Struct. 1995, 28, 357–365. [Google Scholar]
- RILEM Technical Committee TC-242-MDC. Model B4 for creep, drying shrinkage and autogenous shrinkage of normal and high-strength concretes with multi-decade applicability (RILEM Technical Committee TC-242-MDC multi-decade creep and shrinkage of concrete: Material model and structural analysis). Mater. Struct. 2015, 48, 753–770. [Google Scholar] [CrossRef]
- CEN/TC 250; Eurocode 2: Design of Concrete Structures—Part 1–1: General Rules—Rules for Buildings, Bridges and Civil Engineering Structures; Technical Report. The European Committee for Standardization (CEN): Brussels, Belgium, 2022.
- Sanahuja, J.; Soleilhet, F.; Adia, J.L. A multiscale multiphysics platform to investigate cement based materials. In Proceedings of the SynerCrete23—The International Rilem Conference on Synergising Expertise towards Sustainability and Robustness of Cement-Based Materials and Concrete Structures, Milos Island, Greece, 14–16 June 2023. [Google Scholar]
- Babaei, S.; Seetharam, S.; Dizier, A.; Steenackers, G.; Craeye, B. An analytical framework for estimating drying shrinkage strain of OPC based hardened cement paste. Cem. Concr. Compos. 2021, 115, 103833. [Google Scholar] [CrossRef]
- Zhang, J.; Binder, E.; Wang, H.; Aminbaghai, M.; La Pichler, B.; Yuan, Y.; Mang, H.A. On the added value of multi-scale modeling of concrete. Front. Struct. Civ. Eng. 2022, 16, 1–23. [Google Scholar] [CrossRef]
- Bažant, Z. Prediction of concrete creep and shrinkage: Past, present and future. Nucl. Eng. Des. 2001, 203, 27–38. [Google Scholar] [CrossRef]
- Wittmann, F.H. Creep and shrinkage mechanisms. In Creep and Shrinkage in Concrete Structures; Bažant, Z.P., Wittmann, F.H., Eds.; Wiley: Chichester, UK, 1982; pp. 129–161. [Google Scholar]
- Rossi, P.; Tailhan, J.L.; Le Maou, F.; Gaillet, L.; Martin, E. Basic creep behavior of concretes investigation of the physical mechanisms by using acoustic emission. Cem. Concr. Res. 2012, 42, 61–73. [Google Scholar] [CrossRef]
- Tamtsia, B.T.; Beaudoin, J.J. Basic creep of hardened cement paste, a re-examination of the role of water. Cem. Concr. Res. 2000, 30, 1465–1475. [Google Scholar] [CrossRef]
- Sanahuja, J.; Dormieux, L. Creep of a C-S-H gel: Micromechanical approach. Int. J. Multiscale Comput. Eng. 2010, 8, 357–368. [Google Scholar] [CrossRef]
- Sanahuja, J.; Dormieux, L.; Le Pape, Y.; Toulemonde, C. Modélisation micro-macro du fluage propre du béton. In Proceedings of the 19e Congrès FranÇais de Mécanique, Marseille, France, 24–28 August 2009. [Google Scholar]
- Powers, T.C. Mechanisms of Shrinkage and Reversible Creep of Hardened Cement Paste, The Structure of Concrete. In The Structure of Concrete and Its Behaviour Under Load, Proceedings of an International Conference, London, September 1965; Cement and Concrete Association: London, UK, 1965; pp. 319–344. [Google Scholar]
- Brochard, L.; Vandamme, M.; Pellenq, R. Poromechanics of microporous media. J. Mech. Phys. Solids 2012, 60, 606–622. [Google Scholar] [CrossRef]
- Vandamme, M.; Dangla, P.; Nikoosokhan, S.; Brochard, L. Modeling the Poromechanical Behavior of Microporous and Mesoporous Solids. J. Mech. Phys. Solids 2012, 60, 606–622. [Google Scholar]
- Pinson, M.B.; Masoero, E.; Jennings, H.M. Hysteresis from Multiscale Porosity: Modeling Water Sorption and Shrinkage in Cement Paste. Am. Phys. Soc. 2015, 3, 064009. [Google Scholar] [CrossRef]
- Luan, Y.; Ishida, T. Enhanced Shrinkage Model Based on Early Age Hydration and Moisture Status in Pore Structure. J. Adv. Concr. Technol. 2013, 11, 360–373. [Google Scholar] [CrossRef]
- Nguyen, H.; Rahimi-Aghdam, S.; Bažant, Z. Unsaturated nanoporomechanics. Proc. Natl. Acad. Sci. USA 2020, 117, 3440–3445. [Google Scholar] [CrossRef]
- Hubler, M.H.; Wendner, R.; Bažant, Z.P. Statistical justification of Model B4 for drying and autogenous shrinkage of concrete and comparisons to other models. Mater. Struct. 2015, 48, 797–814. [Google Scholar] [CrossRef]
- Hubler, M.; Wendner, R.; Bažant, Z. Comprehensive database for concrete creep and shrinkage: Analysis and recommendations for testing and recording. ACI 2015, 112, 547–558. [Google Scholar] [CrossRef]
- Wendner, R.; Hubler, M.H.; Bažant, Z.P. Optimization method, choice of form and uncertainty quantification of Model B4 using laboratory and multi-decade bridge databases. Mater. Struct. 2015, 48, 771–796. [Google Scholar] [CrossRef]
- Šmilauer, V.; Havlásek, P.; Dohnalová, L.; Wan-Wendner, R.; Bažant, Z. Revamp of Creep and Shrinkage NU Database. In Proceedings of the Biot-Bažant Conference, Evanston, IL, USA, 1–3 June 2021. [Google Scholar] [CrossRef]
- Liao, W.C.; Chern, J.C.; Huang, H.C.; Liu, T.K.; Chin, W.Y. Establishment of analysis system and fast-access cloud-based database of concrete deformation. Comput. Concr. 2021, 28, 441–450. [Google Scholar] [CrossRef]
- Sakata, K.; Ayano, T.; Imamoto, K.; Sato, Y. Database of creep and shrinkage based on Japanese researches. In Proceedings of the Creep, Shrinkage and Durability Mechanics of Concrete and Concrete Structures, CONCREEP-8, Ise-Shima, Japan, 30 September–2 October 2008; pp. 1253–1274. [Google Scholar]
- De Larrard, F.; Ithurralde, G.; Acker, P.; Chauvel, D. High-Performance Concrete for a Nuclear Containment. Spec. Publ. 1990, 121, 549–576. [Google Scholar]
- Bažant, Z.P.; Baweja, S. Creep and Shrinkage Prediction Model for Analysis and Design of Concrete Structures: Model B3; Technical Report; American Concrete Institute: Farmington Hills, MI, USA, 2000. [Google Scholar]
- Avrami, M. Kinetics of phase change. I. General theory. J. Chem. Phys. 1939, 7, 1103–1112. [Google Scholar] [CrossRef]
- Fuji, K.; Kondo, W. Kinetics of the hydration of tricalcium silicate. J. Am. Ceram. Soc. 1974, 57, 492–502. [Google Scholar] [CrossRef]
- Yajun, J.; Cahyadi, J. Simulation of silica fume blended cement hydration. Mater. Struct. 2004, 37, 397–404. [Google Scholar] [CrossRef]
- Kolani, B.; Buffo-Lacarriere, L.; Sellier, A.; Escadeillas, L.; Boutillon, L.; Linger, L. Hydration of slag blended cements. Cem. Concr. Compos. 2012, 34, 1009–1018. [Google Scholar] [CrossRef]
- Bentz, D.; Jensen, O.; Coats, A.; Glasser, F. Influence of silica fume on diffusivity in cement-based materials: I. Experimental and computer modeling studies on cement pastes. Cem. Concr. Res. 2000, 30, 953–962. [Google Scholar] [CrossRef]
- Chen, W. Hydration of Slag Cement: Theory, Modeling and Application. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2006. [Google Scholar]
- Brown, P.; Byrne, G.; Hindmarsh, A. VODE: A variable coefficient ODE solver. SIAM J. Sci. Stat. Comput. 1989, 10, 1038–1051. [Google Scholar] [CrossRef]
- Sanahuja, J.; Dormieux, L.; Chanvillard, G. Modelling elasticity of a hydrating cement paste. Cem. Concr. Res. 2007, 37, 1427–1439. [Google Scholar] [CrossRef]
- Charpin, L.; Sanahuja, J.; Tran, N.C.; Petit, L.; Bremond, O.; Montalvo, J.; Azenha, M.; Granja, J. Multiscale modeling of hydration, elasticity and creep of VeRCrcors concrete. Focus on creep characteristic times. In Proceedings of the 3rd Conference on Technological Innovations in Nuclear Civil Engineering, Paris, France, 5–9 September 2016. [Google Scholar]
- Lee, E.H. Stress analysis in visco-elastic bodies. Q. Appl. Math. 1955, 13, 183–190. [Google Scholar] [CrossRef]
- Mandel, J. Cours de Mécanique des Milieux Continus; Gauthier-Villars: Paris, France, 1966. [Google Scholar]
- Abate, J.; Whitt, W. A unified framework for numerically inverting Laplace transforms. INFORMS J. Comput. 2006, 18, 408–421. [Google Scholar] [CrossRef]
- Gaver, D.P. Observing stochastic processes and approximate transform inversion. Oper. Res. 1966, 14, 444–459. [Google Scholar] [CrossRef]
- Stehfest, H. Algorithm 368: Numerical inversion of Laplace transforms. Commun. Assoc. Comput. Mach. 1970, 13, 47–49. [Google Scholar] [CrossRef]
- Azenha, M.; Staquet, S.; Serdar, M.; Wyrzykowski, M.; Toutlemonde, F.; Bokan Bosiljkov, V. Cost Action Final Achievement Report—Tu1404: Towards the Next Generation of Standards for Service Life of Cement-Based Materials and Structures; Technical Report; Cost Association: Brussels, Belgium, 2018. [Google Scholar]
- Wyrzykowski, M.; Sanahuja, J.; Charpin, L.; KÖnigsberger, M.; Hellmich, C.; Pichler, B.; Valentini, L.; Honorio, T.; Smilauer, V.; Hajkova, K.; et al. Numerical benchmark campaign of cost action tu1404—Microstructural modelling. RILEM Tech. Lett. 2017, 2, 99–107. [Google Scholar] [CrossRef]
- Guang, Y.; Gao, P.; Smilauer, V.; Valentini, L.; Sherzer, G.; Charpin, L.; Bishnoi, S.; Patel, R. GP2a—Benchmark numerical simulation in Micro-level RRT+. In Proceedings of the Synercrete18—Interdisciplinary Approaches for Cement-Based Materials and Structural Concrete—Synergizing Expertise and Bridging Scales of Space and Time, Funchal, Portugal, 24–26 October 2018. [Google Scholar]
- Königsberger, M.; Honório, T.; Sanahuja, J.; Delsaute, B.; Pichler, B. Homogenization of nonaging basic creep of cementitious materials: A multiscale modeling benchmark. Constr. Build. Mater. 2021, 290, 123144. [Google Scholar] [CrossRef]
- Irfan-ul-Hassan, M.; Pichler, B.; Reihsner, R.; Hellmich, C. Elastic and creep properties of young cement paste, as determined from hourly repeated minute-long quasi-static tests. Cem. Concr. Res. 2016, 82, 36–49. [Google Scholar] [CrossRef]
- Sanahuja, J.; Tran, N.C.; Charpin, L.; Petit, L. Material properties prediction for long term operation of nuclear power plants civil engineering structures: Challenges at edf. In Proceedings of the 1st International Conference on Grand Challenges in Construction Materials, UCLA, Los Angeles, CA, USA, 17 March 2016. [Google Scholar]
- Sanahuja, J.; Tran, N.C.; Charpin, L.; Petit, L. Vi(CA)2T v2: Can a concrete material properties simulation code be both physics-based and engineer-friendly? In Proceedings of the Technological Innovations in Nuclear Civil Engineering 2016, Paris, France, 5–9 September 2016.
- Sanahuja, J.; Le Pape, Y.; Tran, N.C.; Charpin, L.; Adia, J.L.; Huang, S.; Guihard, V. Cementitious materials properties prediction: Challenges and contributions from edf r&d. In Proceedings of the Conference to Celebrate the Centennial of lmc and Karen Scrivener’s 60th Birthday, Lausanne, France, 19–22 August 2018. [Google Scholar]
- Powers, T. The thermodynamics of volume change and creep. Matériaux Constr. 2021, 1, 487–507. [Google Scholar] [CrossRef]
- Babaei, S.; Seetharam, S.; Muehlich, U.; Dizier, A.; Steenackers, G.; Craeye, B. A multiscale framework to estimate water sorption isotherms for OPC-based materials. Cem. Concr. Compos. 2020, 105, 103415. [Google Scholar] [CrossRef]
- Bishop, A. The effective stress principle. Tek. Ukebl. 1959, 39, 859–863. [Google Scholar]
- Bangham, D.; Razouk, R. The wetting of charcoal and the nature of the adsorbed phase formed from saturated vapours. Trans. Faraday Soc. 1937, 33, 1463–1472. [Google Scholar] [CrossRef]
- Xi, Y.; Jennings, H. Shrinkage of cement paste and concrete modelled by a multiscale effective homogeneous theory. Mater. Struct. 1997, 30, 329. [Google Scholar] [CrossRef]
- Bažant, Z.; Jirásek, M. Creep and Hygrothermal Effects in Concrete Structures; Springer: Dordrecht, The Netherlands, 2018. [Google Scholar]
- FAIR-DI e.V. Conference on a FAIR Data Infrastructure for Materials Genomics, Virtual Conference, 3–5 June 2020. Available online: https://www.fhi.mpg.de/events/24615/2552 (accessed on 7 October 2023).
- The European Cement Association. Cements for a Low-Carbon Europe; Report; CEMBUREAU: Brussels, Belgium, 2012. [Google Scholar]
- Aili, A.; Vandamme, M.; Torrenti, J.M.; Masson, B. Is long-term autogenous shrinkage a creep phenomenon induced by capillary effects due to self-desiccation? Cem. Concr. Res. 2018, 108, 186–200. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Donmez, A. Extrapolation of short-time drying shrinkage tests based on measured diffusion size effect: Concept and reality. Mater. Struct. 2016, 49, 411–420. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Xi, Y. Drying creep of concrete: Constitutive model and new experiments separating its mechanisms. Mater. Struct. 1994, 27, 3–14. [Google Scholar] [CrossRef]
- Sanahuja, J. Effective behaviour of ageing linear viscoelastic composites: Homogenization approach. Int. J. Solids Struct. 2013, 50, 2846–2856. [Google Scholar] [CrossRef]
- Huang, S. Ageing Behaviour of Concrete Creep: Application to the VeRCrcors Concrete. Ph.D. Thesis, Université Paris-Est, Paris, France, 2018. [Google Scholar]
- Liu, Y.; Lei, S.; Lin, M.; Xia, Z.; Pei, Z.; Li, B. Influence of calcined coal-series kaolin fineness on properties of cement paste and mortar. Constr. Build. Mater. 2018, 171, 558–565. [Google Scholar] [CrossRef]
- Troxel, G.; Raphael, J.; Davis, R. Long-time creep and shrinkage tests of plain and reinforced concrete. Proc. ASTM 1958, 58, 1101–1120. [Google Scholar]
- Sakthivel, T.; R, G.; Pillai, R.G. Adjustment of RILEM B4 model parameters for better prediction of the shrinkage response of blended cement concrete. Indian Concr. J. 2021, 95, 51–57. [Google Scholar]
- Kinda, J. Impact of Drying Rate on Delayed Strain Behavior of Cement-Based Materials—Experimental and Numerical Study. Ph.D. Thesis, Université Paris-Saclay, Paris, France, 2021. [Google Scholar]
- Bullard, J. VCCTL User Guide, Version 9.5; Special Publication 1173; National Institute of Standards and Technology: Gaithersburg, MD, USA, 2014. [Google Scholar]




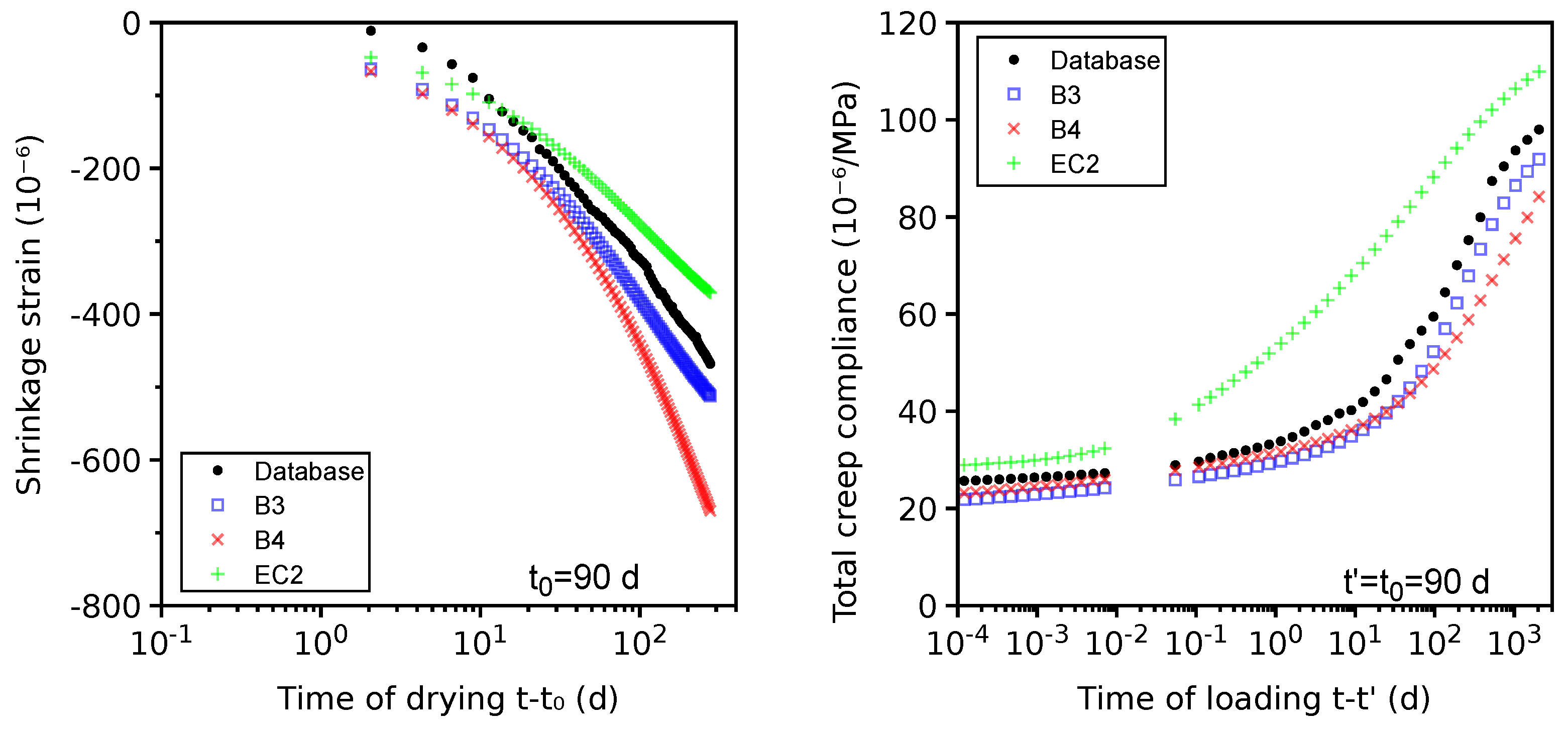

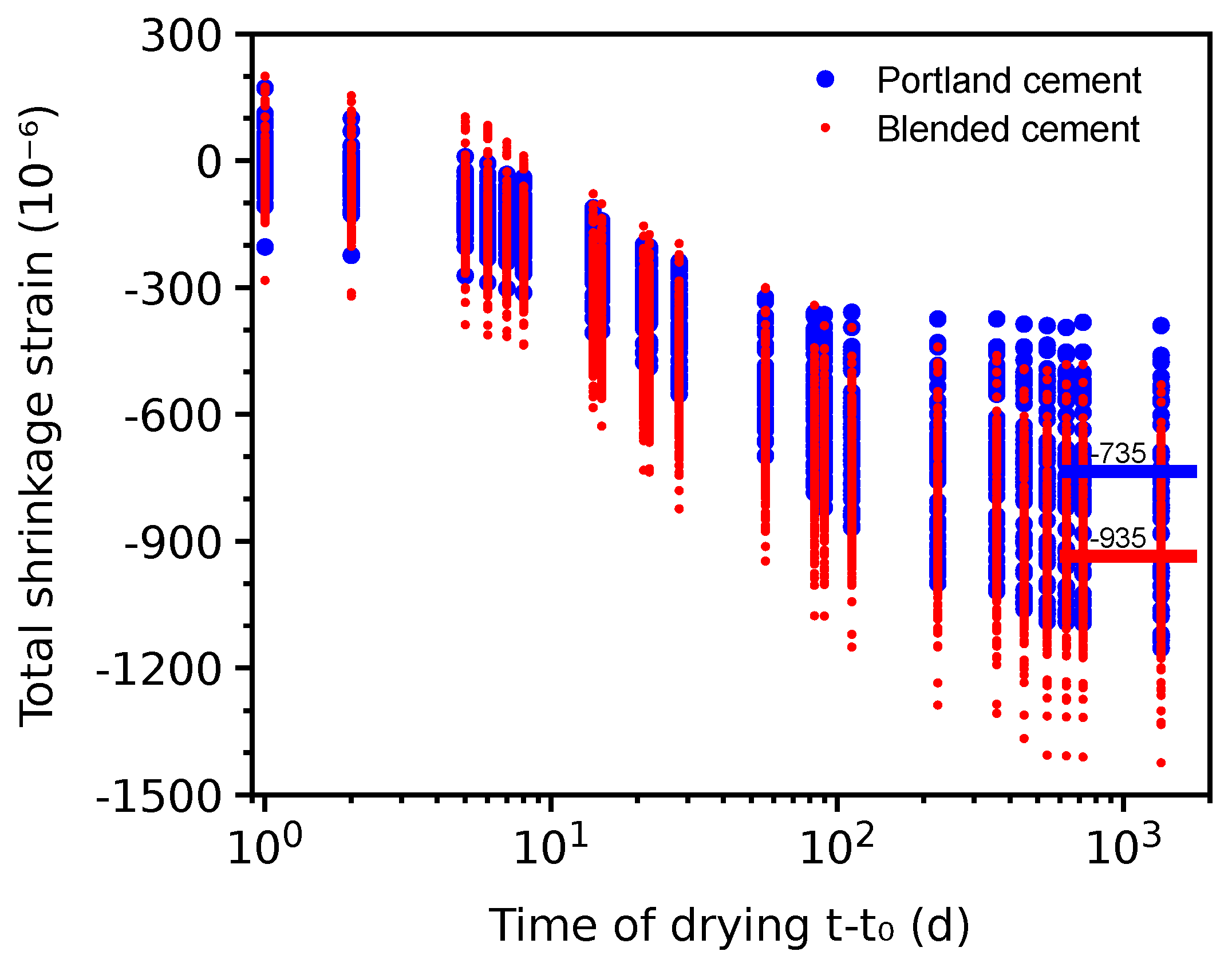
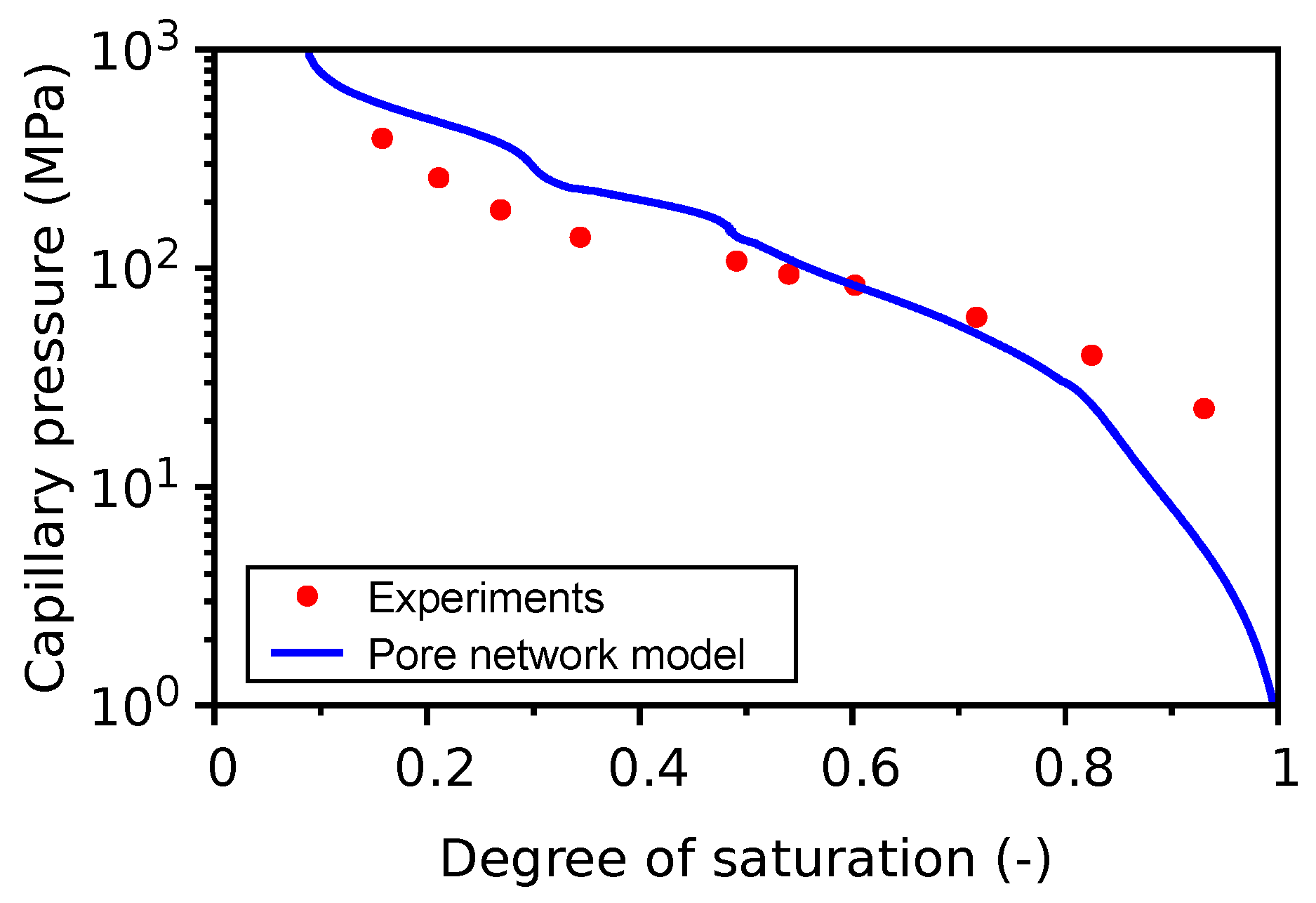

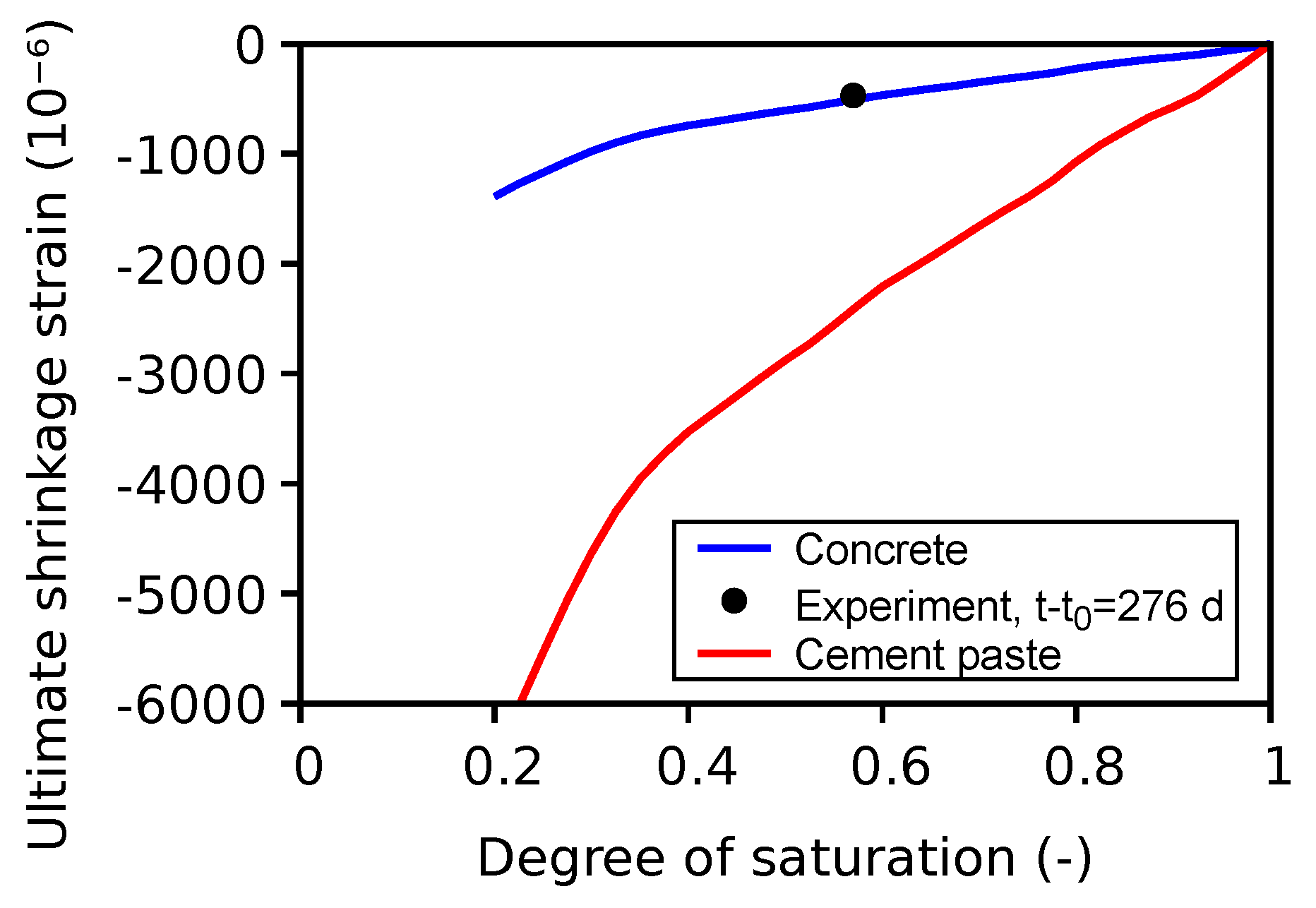
| Component | kg/m |
|---|---|
| CEM I 52.5 N | 314 |
| Effective water | 165 |
| Fine aggregate | 814 |
| Coarse aggregate | 976 |
| Test Type | PLausible/ PRoblematic | Data Sets | Points | Weighted Mean | B3 CoV | B4 CoV | EC2 CoV | CoV |
|---|---|---|---|---|---|---|---|---|
| Young’s modulus at 28 d | - | 173 | 173 | 29.29 GPa | 0.19 | 0.26 | 0.14 | 0.18 |
| Autogenous shrinkage | PL PR | 42 3 | 870 37 | −82 −205 | - - | 0.82 1.05 | 0.97 1.37 | - - |
| Drying shrinkage | PL PR | 32 899 | 1344 19,647 | −327 −642 | 0.56 0.48 | 0.56 0.44 | 0.43 0.47 | - - |
| Total shrinkage | PL PR | 655 165 | 15,117 1281 | −376 −340 | 0.58 0.55 | 0.54 0.62 | 0.53 0.46 | - - |
| Basic creep | PL | 32 | 749 | 64 /MPa | 0.46 | 0.30 | 0.46 | 0.65 |
| PR | 181 | 4241 | 49 /MPa | 0.37 | 0.61 | 0.54 | 0.42 | |
| Total creep | PL | 20 | 261 | 85 /MPa | 0.50 | 0.33 | 0.40 | - |
| PR | 169 | 3262 | 65 /MPa | 0.47 | 0.43 | 0.56 | - |
| Parameter | Value |
|---|---|
| Degree of hydration | 0.85 |
| Volume fraction low-density (LD) C-S-H | 0.27 |
| Volume fraction high-density (HD) C-S-H | 0.28 |
| Total C-S-H | 0.55 |
| Capillary porosity | 0.21 |
| Total porosity | 0.37 |
| Portlandite | 0.14 |
| Unhydrated clinker | 0.06 |
| Other products | 0.04 |
| Bulk Modulus | GPa |
|---|---|
| Experimental () | 10.5 |
| Calculated () | 9.6 |
| Calculated solid () | 17.5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Šmilauer, V.; Dohnalová, L.; Jirásek, M.; Sanahuja, J.; Seetharam, S.; Babaei, S. Benchmarking Standard and Micromechanical Models for Creep and Shrinkage of Concrete Relevant for Nuclear Power Plants. Materials 2023, 16, 6751. https://doi.org/10.3390/ma16206751
Šmilauer V, Dohnalová L, Jirásek M, Sanahuja J, Seetharam S, Babaei S. Benchmarking Standard and Micromechanical Models for Creep and Shrinkage of Concrete Relevant for Nuclear Power Plants. Materials. 2023; 16(20):6751. https://doi.org/10.3390/ma16206751
Chicago/Turabian StyleŠmilauer, Vít, Lenka Dohnalová, Milan Jirásek, Julien Sanahuja, Suresh Seetharam, and Saeid Babaei. 2023. "Benchmarking Standard and Micromechanical Models for Creep and Shrinkage of Concrete Relevant for Nuclear Power Plants" Materials 16, no. 20: 6751. https://doi.org/10.3390/ma16206751
APA StyleŠmilauer, V., Dohnalová, L., Jirásek, M., Sanahuja, J., Seetharam, S., & Babaei, S. (2023). Benchmarking Standard and Micromechanical Models for Creep and Shrinkage of Concrete Relevant for Nuclear Power Plants. Materials, 16(20), 6751. https://doi.org/10.3390/ma16206751






