Numerical Simulation Study of the Mechanical Behaviour of 1D and 2D Germanium Carbide and Tin Carbide Nanostructures
Abstract
1. Introduction
2. Materials and Methods
2.1. Atomic Structure of Germanium Carbide and Tin Carbide Nanosheets and Nanotubes
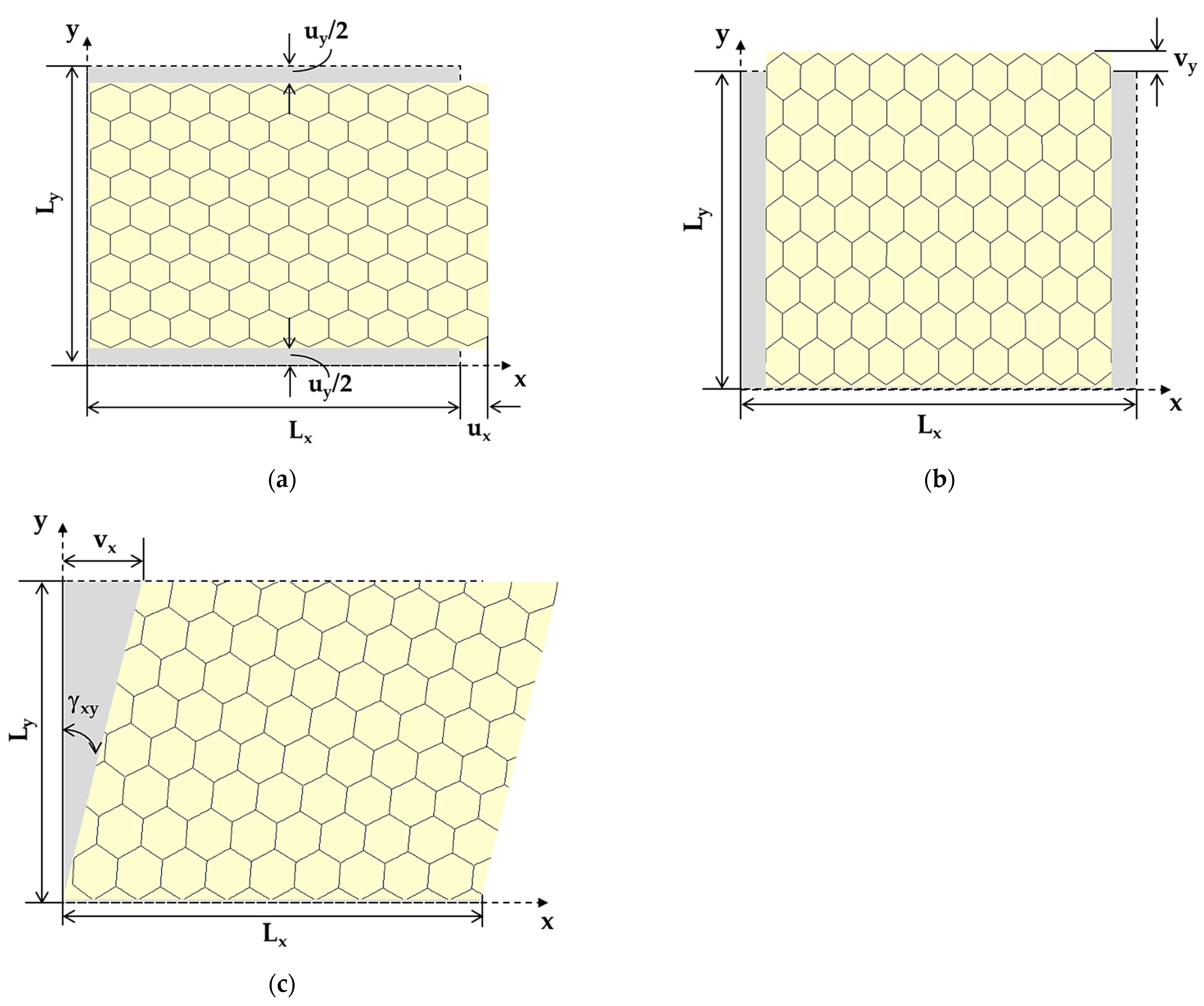
2.2. Numerical Modeling of Elastic Properties of GeC and SnC Nanosheets and Nanotubes
2.2.1. Input for FE Model of 1D and 2D GeC and SnC Nanostructures
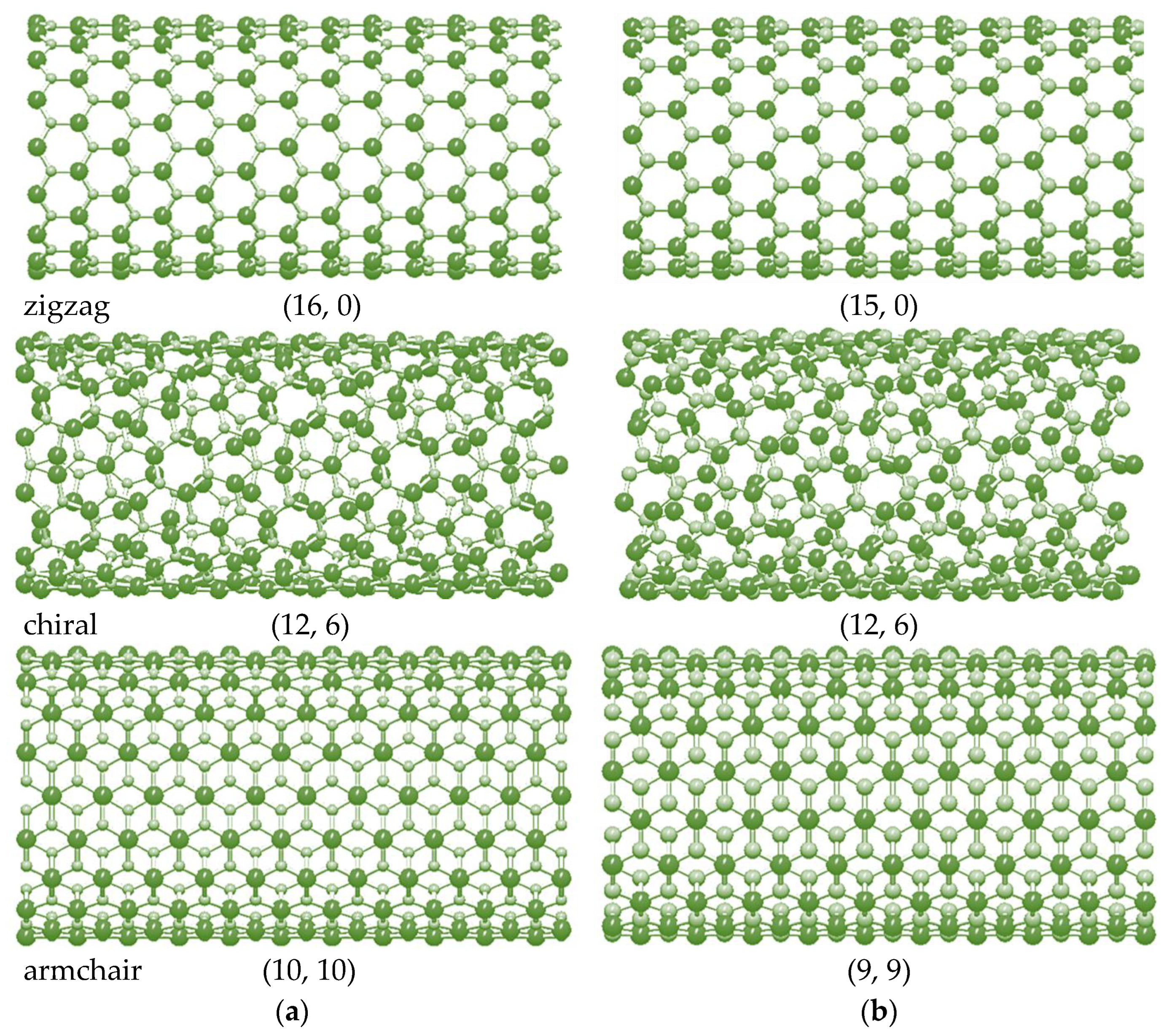
2.2.2. Geometrical Characteristics of GeC and SnC Nanosheets and Single-Walled GeC and SnC Nanotubes
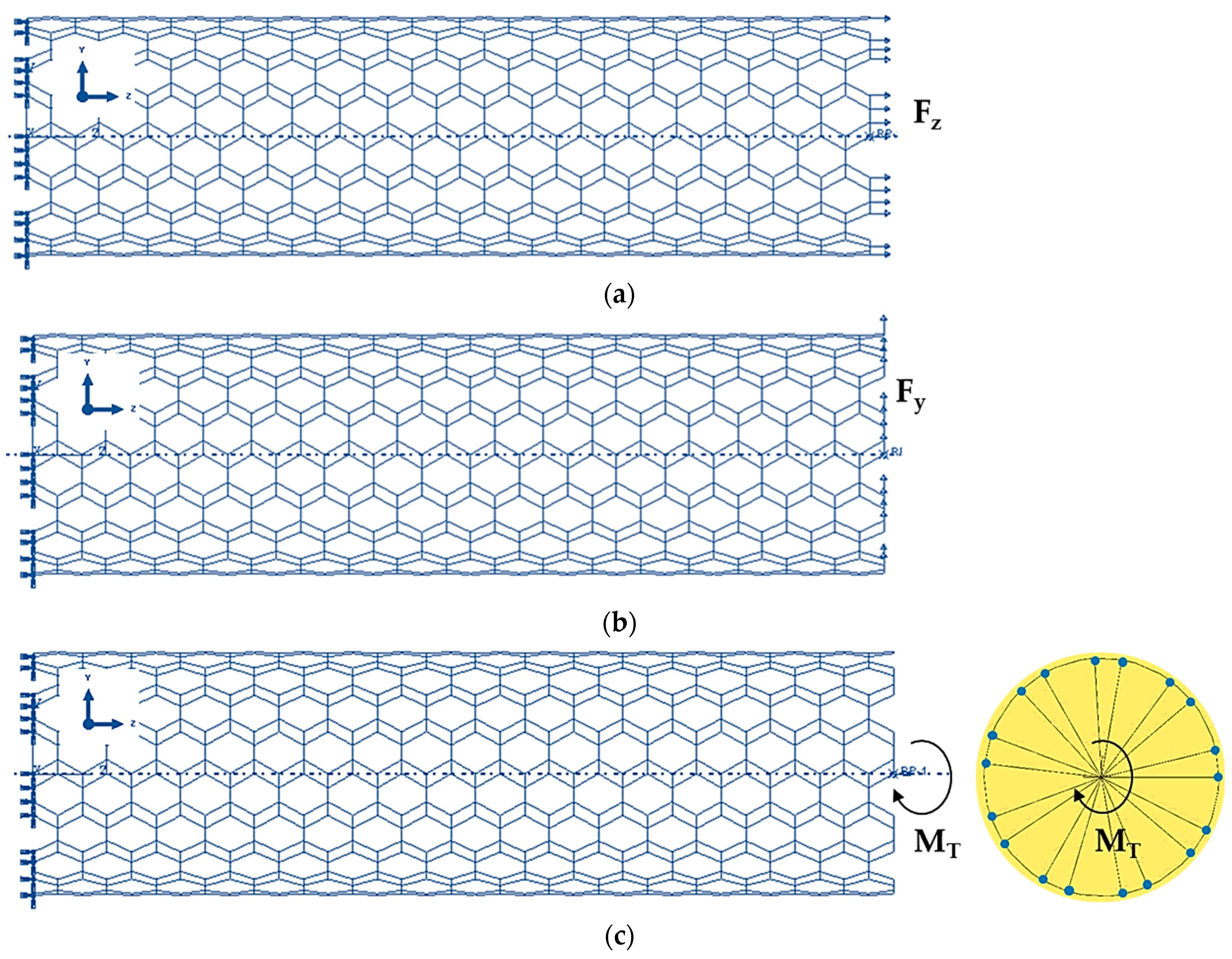
2.2.3. FEA and Determination Elastic Properties of GeC and SnC Nanosheets and Nanotubes
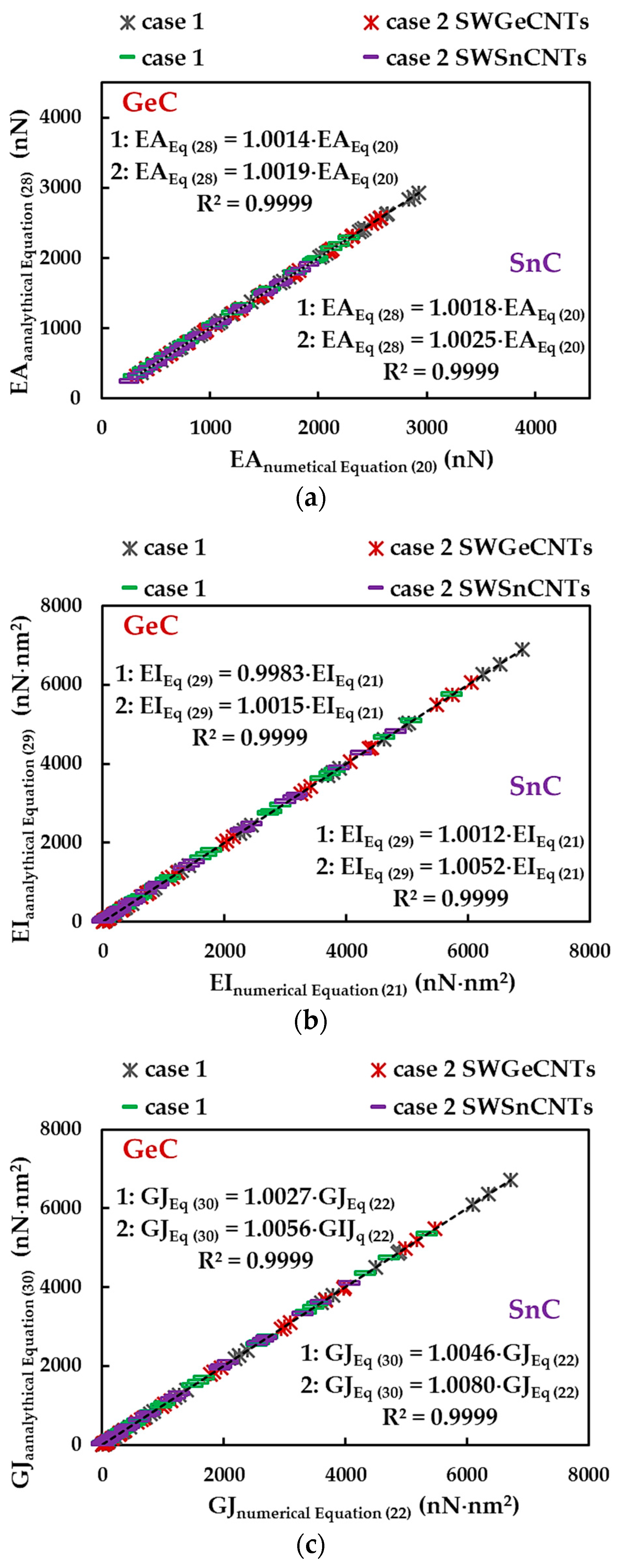
3. Results and Discussion
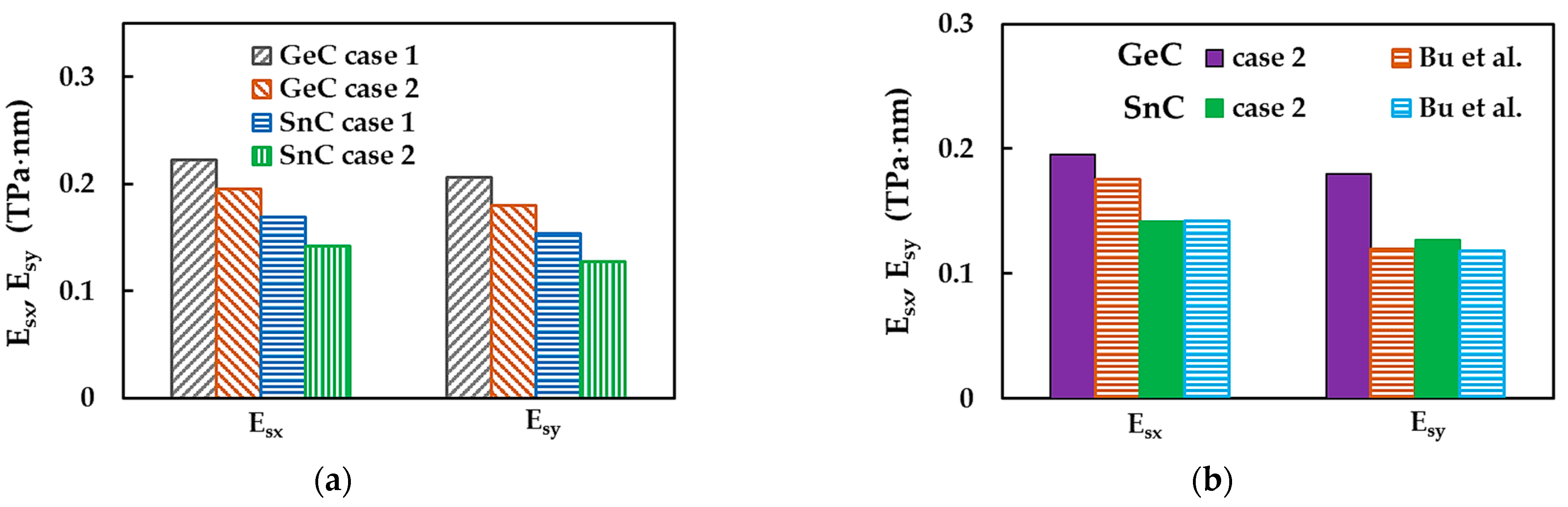
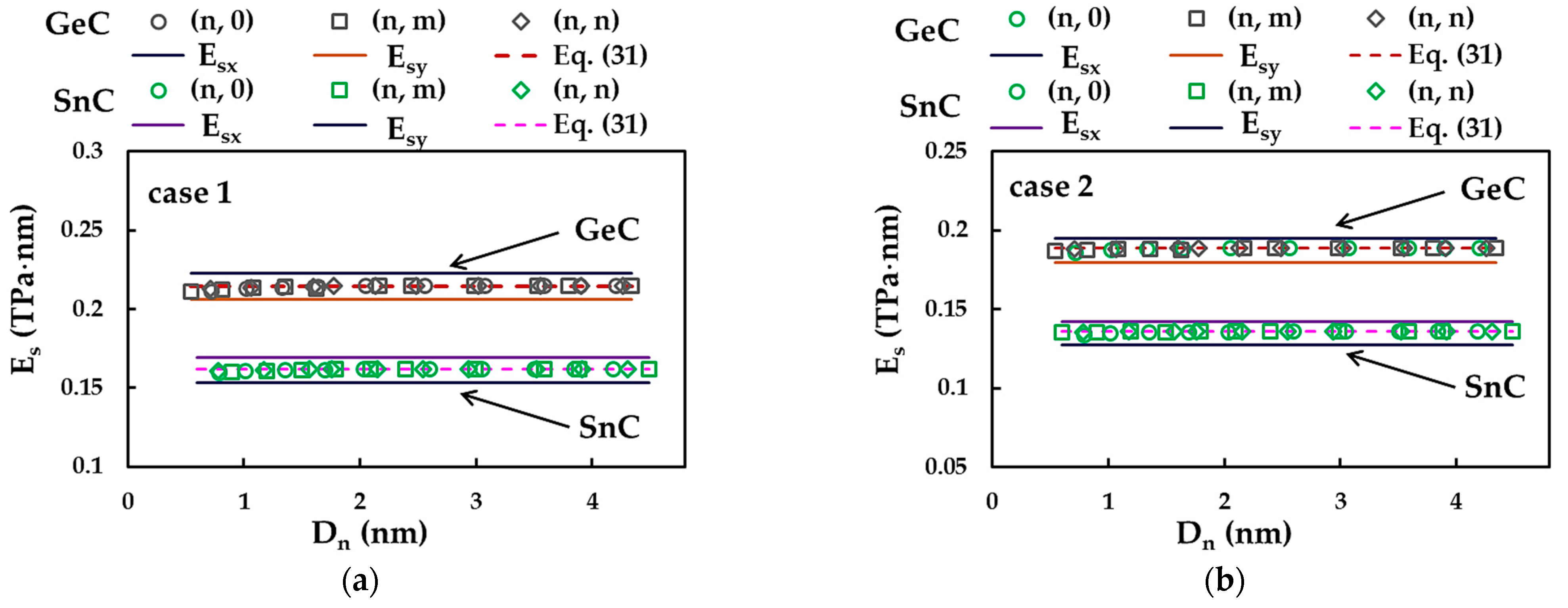
3.1. Elastic Properties of GeC and SnC Nanosheets
3.2. Elastic Properties of Single-Walled Germanuim Carbide and Tin Carbide NTs
3.2.1. Rigidities of the SWGeCNTs and SWSnCNTs
3.2.2. Young’s Modulus of Single-Walled GeCNTs and SnCNTs
3.2.3. Shear Modulus and Poisson’s Ration of SWGeCNTs and SWSnCNTs
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Şahin, H.; Cahangirov, S.; Topsakal, M.; Bekaroglu, E.; Akturk, E.; Senger, R.T.; Ciraci, S. Monolayer honeycomb structures of group-IV elements and III-V binary compounds: First-principles calculations. Phys. Rev. B 2009, 80, 155453. [Google Scholar] [CrossRef]
- Lu, T.-Y.; Liao, X.-X.; Wang, H.-Q.; Zheng, J.-C. Tuning the indirect–direct band gap transition of SiC, GeC and SnC monolayer in a graphene-like honeycomb structure by strain engineering: A quasiparticle GW study. J. Mater. Chem. 2012, 22, 10062–10068. [Google Scholar] [CrossRef]
- Peng, Q.; Liang, C.; Ji, W.; De, S. A first-principles study of the mechanical properties of g-GeC. Mech. Mater. 2013, 64, 135–141. [Google Scholar] [CrossRef]
- Behzad, S.; Chegel, R. First-principles study of the band structure and optical spectra of germanium carbide under mechanical strain. J. Electron Spectrosc. Relat. Phenom. 2020, 242, 146969. [Google Scholar]
- Majidi, S.; Elahi, S.M.; Esmailian, A.; Kanjouri, F. First principle study of electronic and optical properties of planar GeC, SnC and SiC nanosheets. Protect. Met. Phys. Chem. Surf. 2017, 53, 773–779. [Google Scholar]
- Li, R.-X.; Tian, X.-L.; Zhu, S.-C.; Ding, J.; Li, H.-D. Exploring the structural and electronic properties of GeC/BP van der Waals heterostructures. Physica E 2021, 134, 114804. [Google Scholar] [CrossRef]
- Peng, Q.; Rehman, J.; Eid, K.; Alofi, A.S.; Laref, A.; Albaqami, M.D.; Alotabi, R.G.; Shibl, M.F. Vanadium Carbide (V4C3) MXene as an Efficient Anode for Li-Ion and Na-Ion Batteries. Nanomaterials 2022, 12, 2825. [Google Scholar] [CrossRef]
- Rehman, J.; Lin, S.; Butt, M.K.; Fan, X.; Khattab, T.; Elsayed, K.A.; Shibl, M.F. An overview of 2D metal sulfides and carbides as Na host materials for Na-ion batteries. J. Chem. Eng. 2023, 461, 141924. [Google Scholar] [CrossRef]
- Ji, Y.; Dong, H.; Hou, T.; Li, Y. Monolayer graphitic germanium carbide (g-GeC): The promising cathode catalyst for fuel cell and lithium–oxygen battery applications. J. Mater. Chem. A 2018, 6, 2212–2218. [Google Scholar] [CrossRef]
- Khossossi, N.; Banerjee, A.; Essaoudi, I.; Ainane, A.; Jena, P.; Ahuja, R. Thermodynamics and kinetics of 2D g-GeC monolayer as an anode material for Li/Na-ion batteries. J. Power Sources 2021, 485, 229318. [Google Scholar] [CrossRef]
- Fadaie, M.; Shahtahmassebi, N.; Roknabad, M.R.; Gulseren, O. Investigation of new two-dimensional materials derived from stanine. Comput. Mater. Sci. 2017, 137, 208–214. [Google Scholar] [CrossRef]
- Mogulkoc, Y.; Modarresi, M.; Mogulkoc, A.; Ciftci, Y.O.; Alkan, B. First principle and tight-binding study of strained SnC. J. Phys. Chem. Solids 2017, 111, 458–463. [Google Scholar]
- Rehman, J.; Fan, X.; Zheng, W.T. 2D SnC sheet with a small strain is a promising Li host material for Li-ion batteries. Mater. Today Commun. 2021, 26, 101768. [Google Scholar] [CrossRef]
- Marcos-Viquez, A.L.; Miranda, Á.; Cruz-Irisson, M.; Pérez, L.A. Tin carbide monolayers as potential gas sensors. Mater. Lett. 2021, 294, 129751. [Google Scholar] [CrossRef]
- Sohbatzadeh, Z.; Eivarib, H.A.; Fakhrabad, D.V. Formation energy and some mechanical properties of hydrogenated hexagonal monolayer of GeC. Physica B 2018, 547, 88–91. [Google Scholar] [CrossRef]
- Hoat, D.M.; Naseri, M.; Ponce-Péreze, R.; Hieu, N.N.; Rivas-Silva, J.F.; Vu, T.V.; Tong, H.D.; Cocoletzi, G.H. Structural and electronic properties of chemically functionalized SnC monolayer: A first principles study. Mater. Res. Express 2020, 7, 015013. [Google Scholar] [CrossRef]
- Kumar, A.; Devi, A.; Kumar, R.; Singh, A.; Ahluwalia, P.K. First Principle Study of Structural, Electronic and Magnetic Properties of GeC nanoribbon. AIP Conf. Proc. 2019, 2115, 030376. [Google Scholar]
- Yu, W.-Z.; Yan, J.-A.; Gao, S.-P. Band gap characters and ferromagnetic/antiferromagnetic coupling in group-IV monolayers tuned by chemical species and hydrogen adsorption configurations. Nanoscale Res. Lett. 2015, 10, 351. [Google Scholar] [CrossRef]
- Majidi, S.; Achour, A.; Rai, D.P.; Nayebi, P.; Solaymani, S.; Nezafat, N.B.; Elahi, S.M. Effect of point defects on the electronic density states of SnC nanosheets: First-principles calculations. Results Phys. 2017, 7, 3209–3215. [Google Scholar]
- Jin, H.; Dai, Y.; Huang, B.B. Design of advanced photocatalysis system by adatom decoration in 2D nanosheets of group-IV and III-V binary compounds. Sci. Rep. 2016, 6, 23104. [Google Scholar]
- Saha, M.S.; Li, R.; Cai, M.; Sun, X. Nanowire-based three-dimensional hierarchical core/shell heterostructured electrodes for high performance proton exchange membrane fuel cells. J. Power Sources 2008, 185, 1079–1085. [Google Scholar]
- Zhu, H.; Zhang, L.; Zhang, L.; Song, Y.; Huang, Y.; Zhang, Y. Electromagnetic absorption properties of Sn-filled multi-walled carbon nanotubes synthesized by pyrolyzing. Mater. Lett. 2010, 64, 227–230. [Google Scholar]
- Baei, M.T.; Peyghan, A.A.; Moghimi, M.; Hashemian, S. First-principles calculations of structural stability, electronic, and electrical responses of GeC nanotube under electric field effect for use in nanoelectronic devices. Superlattices Microstruct. 2012, 52, 1119–1130. [Google Scholar]
- Wang, S.F.; Chen, L.Y.; Zhang, J.M.; Xu, K.W. Electronic and magnetic properties of single-wall GeC nanotubes filled with iron nanowires. Superlattices Microstruct. 2012, 51, 754–764. [Google Scholar]
- Rathi, S.J.; Ray, A.K. On the electronic and geometric structures of armchair GeC nanotubes: A hybrid density functional study. Nanotechnology 2008, 19, 335706–335716. [Google Scholar]
- Samanta, P.N.; Das, K.K. Electron transport properties of zigzag single walled tin carbide nanotubes. Comput. Mater. Sci. 2014, 81, 326–331. [Google Scholar]
- Song, J.; Henry, D.J. Stability and electronic structures of double-walled armchair germanium carbide nanotubes. Comput. Mater. Sci. 2016, 111, 86–90. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.W. A structural mechanics approach for the analysis of carbon nanotubes. Int. J. Solids Struct. 2003, 40, 2487–2499. [Google Scholar]
- Rappé, A.K.; Casewit, C.J.; Colwell, K.S.; Goddard, W.A.; Skid, W.M. UFF, a full periodic table force field for molecular mechanics and molecular dynamics simulations. J. Am. Chem. Soc. 1992, 114, 10024–10039. [Google Scholar] [CrossRef]
- Badger, R.M. A Relation between internuclear distances and bond force constants. J. Chem. Phys. 1934, 2, 128–131. [Google Scholar]
- Genoese, A.; Genoese, A.; Rizzi, N.L.; Salerno, G. Force constants of BN, SiC, AlN and GaN sheets through discrete homogenization. Meccanica 2018, 53, 593–611. [Google Scholar]
- Mayo, S.L.; Barry, D.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar]
- Chang, T.; Gao, H. Size-dependent elastic properties of a single-walled carbon nanotube via a molecular mechanics model. J. Mech. Phys. Solids 2003, 51, 1059–1074. [Google Scholar]
- Sakharova, N.A.; Pereira, A.F.G.; Antunes, J.M.; Chaparro, B.M.; Fernandes, J.V. On the determination of elastic properties of indium nitride nanosheets and nanotubes by numerical simulation. Metals 2023, 13, 73. [Google Scholar]
- Tapia, A.; Cab, C.; Hernández-Pérez, A.; Villanueva, C.; Peñuñuri, F.; Avilés, F. The bond force constants and elastic properties of boron nitride nanosheets and nanoribbons using a hierarchical modeling approach. Physica E 2017, 89, 183–193. [Google Scholar]
- Sakharova, N.A.; Antunes, J.M.; Pereira, A.F.G.; Chaparro, B.M.; Fernandes, J.V. On the determination of elastic properties of single-walled boron nitride nanotubes by numerical simulation. Materials 2021, 14, 3183. [Google Scholar]
- Sakharova, N.A.; Pereira, A.F.G.; Antunes, J.M.; Brett, C.M.A.; Fernandes, J.V. Mechanical characterization of single-walled carbon nanotubes. Numerical simulation study. Compos. B-Eng. 2015, 75, 73–85. [Google Scholar]
- Pereira, A.F.G.; Antunes, J.M.; Fernandes, J.V.; Sakharova, N.A. Shear modulus and Poisson’s ratio of single-walled carbon nanotubes: Numerical evaluation. Phys. Status Solidi B 2016, 253, 366–376. [Google Scholar]
- Bu, H.; Liu, X.; Yuan, H.; Yuana, X.; Zhao, M. Two-dimensional XC6-enes (X = Ge, Sn, Pb) with moderate band gaps, biaxial negative Poisson’s ratios, and high carrier mobility. Phys. Chem. Chem. Phys. 2021, 23, 26468. [Google Scholar]
- Sadki, K.; Kourra, M.H.; Drissi, L.B. Non linear and thermoelastic behaviors of group-IV hybrid 2D nanosheets. Superlattices Microstruct. 2013, 132, 106172. [Google Scholar]
- Hao, A.; Yang, X.; Wang, X.; Zhu, Y.; Liu, X.; Liu, R. First-principles investigations on electronic, elastic andoptical properties of XC (X = Si, Ge, and Sn) under high pressure. J. Appl. Phys. 2010, 108, 063531. [Google Scholar]
- Souadkia, M.; Bennecer, B.; Kalarasse, F. Elastic, vibrational and thermodynamic properties of α-Sn based group IV semiconductors and GeC under pressure. J. Phys. Chem. Solids 2013, 74, 615–1625. [Google Scholar]
- Muthaiah, R.; Garg, J. First principles investigation of high thermal conductivity in hexagonal germanium carbide(2H-GeC). Carbon Trends 2021, 5, 100113. [Google Scholar]
- Hess, P. Thickness of elemental and binary single atomic monolayers. Nanoscale Horiz. 2020, 5, 385–399. [Google Scholar] [PubMed]
- Sakharova, N.A.; Antunes, J.M.; Pereira, A.F.G.; Chaparro, B.M.; Fernandes, J.V. Elastic properties of single-walled phosphide nanotubes: Numerical Simulation Study. Nanomaterials 2022, 12, 2360. [Google Scholar] [PubMed]
- Sakharova, N.A.; Pereira, A.F.G.; Antunes, J.M. Elastic moduli of non-chiral singe-walled silicon carbide nanotubes: Numerical simulation study. Materials 2022, 15, 8153. [Google Scholar]
- Jiang, L.; Guo, W. Analytical solutions for elastic binary nanotubes of arbitrary chirality. Acta Mech. Sin. 2016, 32, 1046–1057. [Google Scholar]
- Salazar, F.; Pérez, L.A. Theoretical study of electronic and mechanical properties of GeC nanowires. Comput. Mater. Sci. 2012, 63, 47–51. [Google Scholar]
- Marcos-Viquez, A.L.; Miranda, Á.; Cruz-Irisson, M.; Pérez, L.A. Mechanical and electronic properties of tin carbide nanowires. Phys. Status Solidi A 2020, 217, 1900590. [Google Scholar]






| Compound | Atom 1 | Atom 2 | Es, nN/nm [1] | [1] | |||
|---|---|---|---|---|---|---|---|
| Charge [29] | Charge [29] | ||||||
| GeC | Ge | C | 2.789 | 1.912 | 0.186 | 142 | 0.33 |
| SnC | Sn | 2.961 | 0.205 | 98 | 0.41 |
| Compound | Case 1 | , nN/nm | , nN nm/rad2 | , nN nm/rad2 | , nN nm/rad2 |
|---|---|---|---|---|---|
| GeC | 1 | 383 | 0.631 | 1.343 | 0.625 |
| 2 | 367 | 0.456 | 0.970 | ||
| SnC | 1 | 303 | 0.471 | 1.130 | |
| 2 | 288 | 0.314 | 0.753 |
| Compound | Case 1 | l, nm [1] | d, nm Equation (10) | Eb, GPa Equation (11) | Gb, GPa Equation (12) | νb Equation (13) |
|---|---|---|---|---|---|---|
| GeC | 1 | 0.186 | 0.2031 | 2196 | 695 | 0.24 |
| 2 | 0.1762 | 2799 | 1227 | 0.33 | ||
| SnC | 1 | 0.205 | 0.2055 | 1875 | 732 | 0.29 |
| 2 | 0.1722 | 2533 | 1485 | 0.41 |
| NT Type | SWGeCNTs | SWSnCNTs | ||||
|---|---|---|---|---|---|---|
| (n, m) | Diameter, , nm | Length, , nm | (n, m) | Diameter, , nm | Length, , nm | |
| zigzag (n, 0), θ = 0 | (7, 0) | 0.718 | 22.13 | (7, 0) | 0.791 | 24.09 |
| (10, 0) | 1.025 | 31.34 | (9, 0) | 1.017 | 30.55 | |
| (13, 0) | 1.333 | 39.13 | (12, 0) | 1.356 | 41.00 | |
| (16, 0) | 1.641 | 49.17 | (15, 0) | 1.695 | 50.23 | |
| (20, 0) | 2.051 | 60.88 | (18, 0) | 2.034 | 61.30 | |
| (25, 0) | 2.564 | 77.61 | (23, 0) | 2.600 | 77.90 | |
| (30, 0) | 3.076 | 92.67 | (27, 0) | 3.052 | 92.66 | |
| (35, 0) | 3.589 | 107.72 | (31, 0) | 3.504 | 105.57 | |
| (38, 0) | 3.897 | 117.76 | (34, 0) | 3.843 | 116.64 | |
| (41, 0) | 4.204 | 126.13 | (37, 0) | 4.182 | 125.87 | |
| chiral (n, m), θ = 19.1° | (4, 2) | 0.543 | 16.91 | (4, 2) | 0.598 | 17.90 |
| (6, 3) | 0.814 | 25.41 | (6, 3) | 0.897 | 26.81 | |
| (8, 4) | 1.085 | 33.25 | (8, 4) | 1.196 | 35.80 | |
| (10, 5) | 1.357 | 42.08 | (10, 5) | 1.495 | 46.25 | |
| (12, 6) | 1.628 | 49.52 | (12, 6) | 1.794 | 54.43 | |
| (16, 8) | 2.171 | 65.68 | (14, 7) | 2.093 | 64.15 | |
| (18, 9) | 2.442 | 74.52 | (16, 8) | 2.392 | 72.09 | |
| (22, 11) | 2.984 | 90.82 | (20, 10) | 2.990 | 90.22 | |
| (26, 13) | 3.527 | 106.97 | (24, 12) | 3.588 | 107.89 | |
| (28, 14) | 3.798 | 114.42 | (26, 13) | 3.887 | 117.88 | |
| (32, 16) | 4.341 | 130.57 | (30, 15) | 4.485 | 135.74 | |
| armchair (n, n), θ = 30° | (4, 4) | 0.710 | 21.91 | (4, 4) | 0.783 | 23.97 |
| (6, 6) | 1.066 | 33.02 | (6, 6) | 1.175 | 35.33 | |
| (9, 9) | 1.599 | 48.81 | (8, 8) | 1.566 | 48.61 | |
| (10, 10) | 1.776 | 54.61 | (9, 9) | 1.762 | 53.93 | |
| (12, 12) | 2.131 | 64.27 | (11, 11) | 2.153 | 64.58 | |
| (14, 14) | 2.487 | 75.87 | (13, 13) | 2.545 | 76.99 | |
| (17, 17) | 3.019 | 91.33 | (15, 15) | 2.936 | 89.41 | |
| (20, 20) | 3.552 | 106.80 | (18, 18) | 3.524 | 107.15 | |
| (22, 22) | 3.908 | 118.40 | (20, 20) | 3.915 | 117.80 | |
| (24, 24) | 4.263 | 128.06 | (22, 22) | 4.307 | 128.44 | |
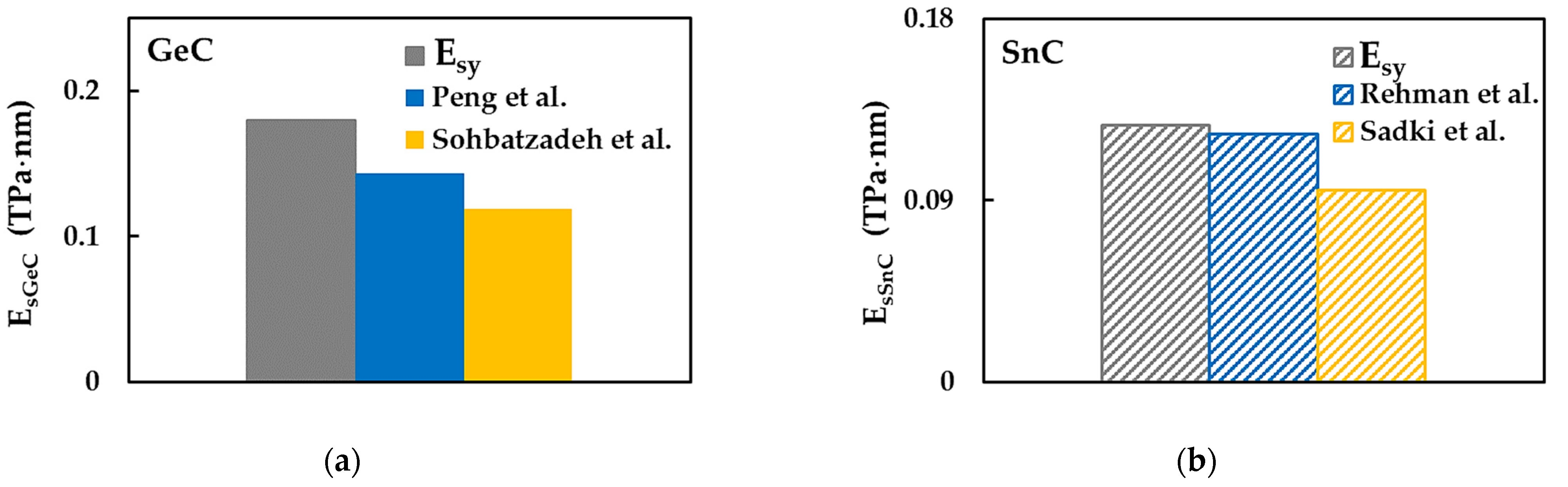
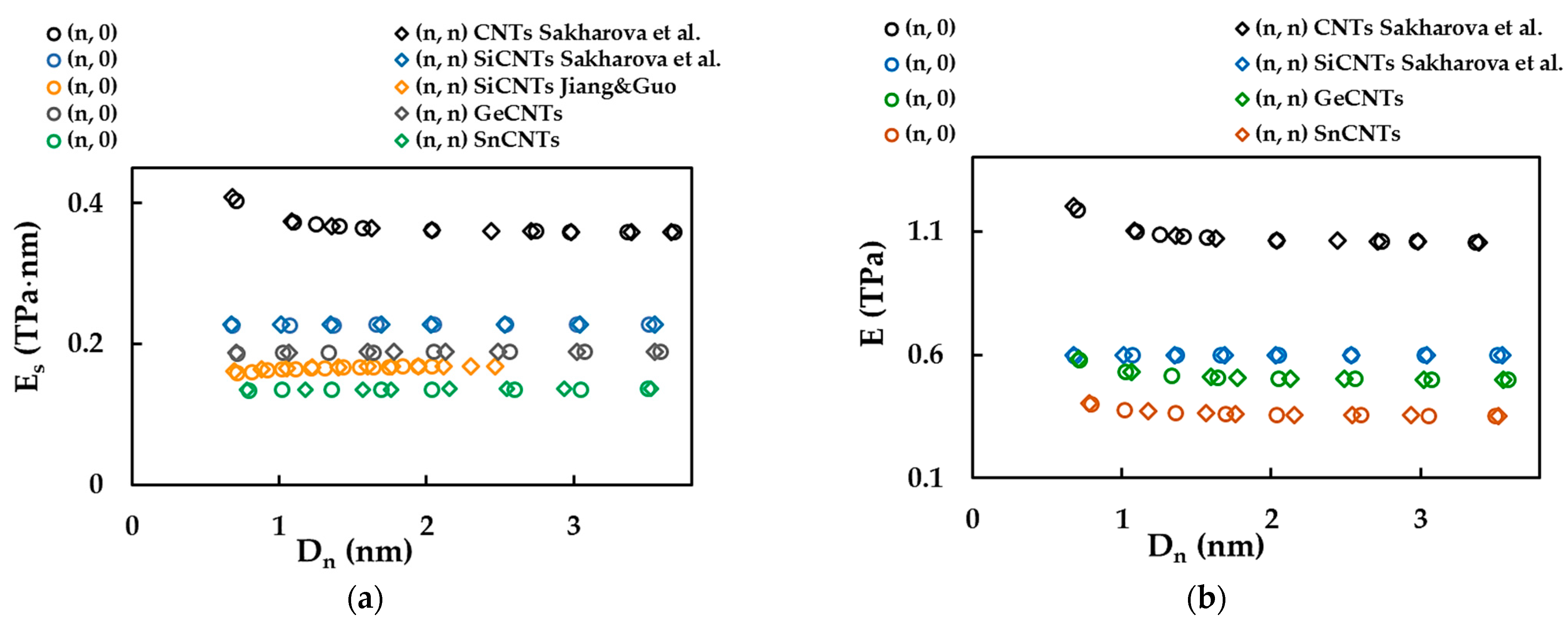
| Reference | Compound | , TPa nm | , TPa nm | |||
|---|---|---|---|---|---|---|
| Current study | GeC | 0.223 1 | 0.206 1 | 1.080 1 | 1.14 | 1.14 |
| 0.195 2 | 0.180 2 | 1.086 2 | ||||
| SnC | 0.170 1 | 0.154 1 | 1.104 1 | 1.19 | 1.19 | |
| 0.142 2 | 0.127 2 | 1.116 2 | ||||
| Bu et al. [39] | ph-GeC | 0.176 | 0.120 | 1.470 | – | – |
| ph-SnC | 0.142 | 0.118 | 1.200 | |||
| Peng et al. [3] | GeC | 0.143 | – | – | – | |
| Sohbatzadeh et al. [15] | 0.119 | |||||
| Rehnam et al. [13] | SnC | 0.123 | – | – | – | |
| Sadki et al. [40] | 0.095 | – | – | – | ||
| Compound | Case | , TPa nm | |||
|---|---|---|---|---|---|
| GeC | 1 | 0.047 | 0.014 | 1.18 | 4.00 |
| 2 | 0.040 | 0.055 | |||
| SnC | 1 | 0.040 | 0.039 | 1.25 | 2.50 |
| 2 | 0.032 | 0.095 |
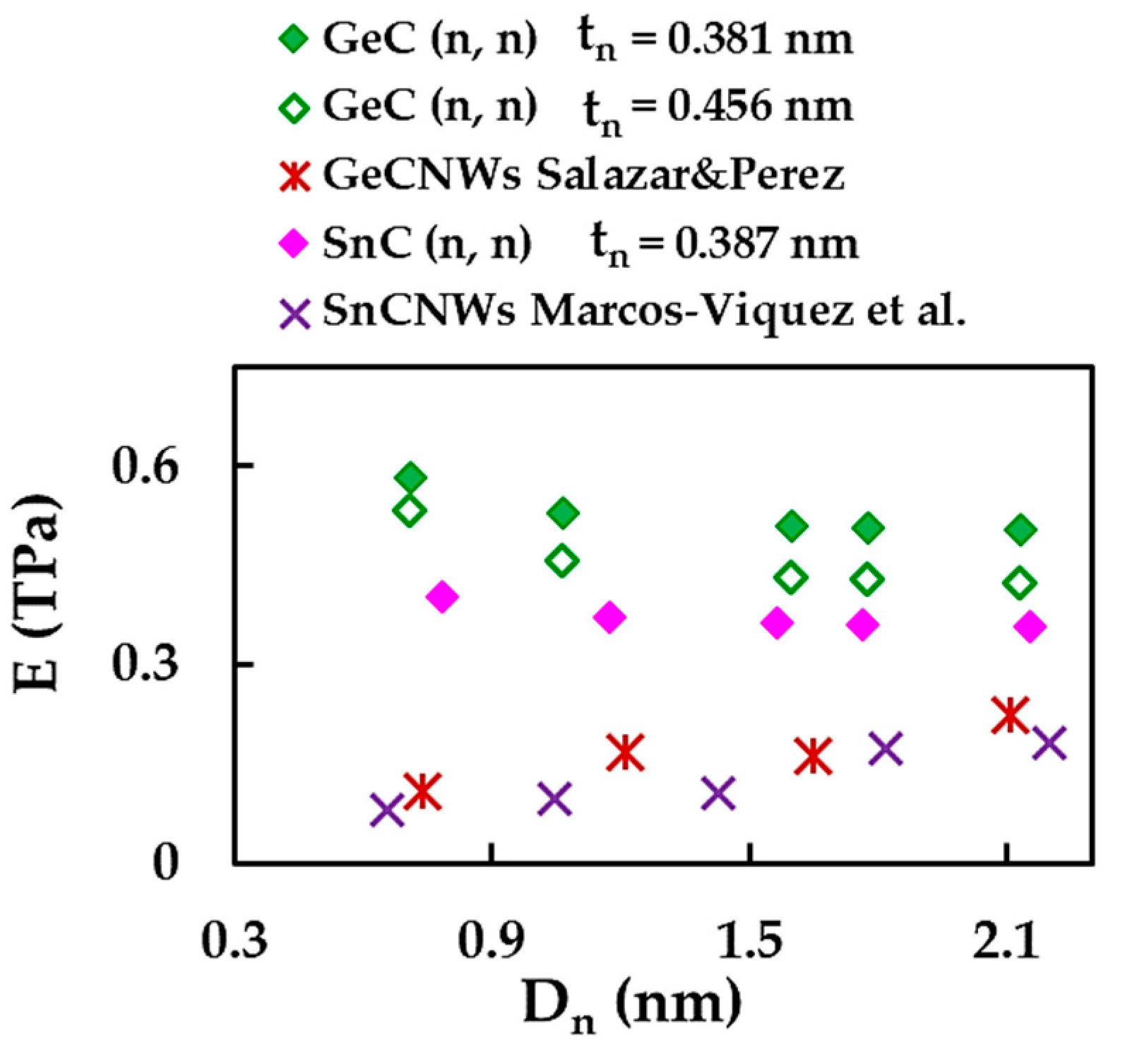
| Reference | Compound | , TPa | , TPa |
|---|---|---|---|
| current work | GeC (2D) | 0.563 1 | 0.123 1 |
| 0.492 2 | 0.104 2 | ||
| SnC (2D) | 0.418 1 | 0.103 1 | |
| 0.348 2 | 0.082 2 | ||
| Hao et al. [41] | GeC (3D) | 0.354 | 0.152 |
| SnC (3D) | 0.211 | 0.086 | |
| Souadkia et al. [42] | GeC (3D) | 0.395 | 0.168 |
| SnC (3D) | 0.257 | 0.104 | |
| Muthaiah and Garg [43] | GeC (3D) | 0.389 | 0.169 |
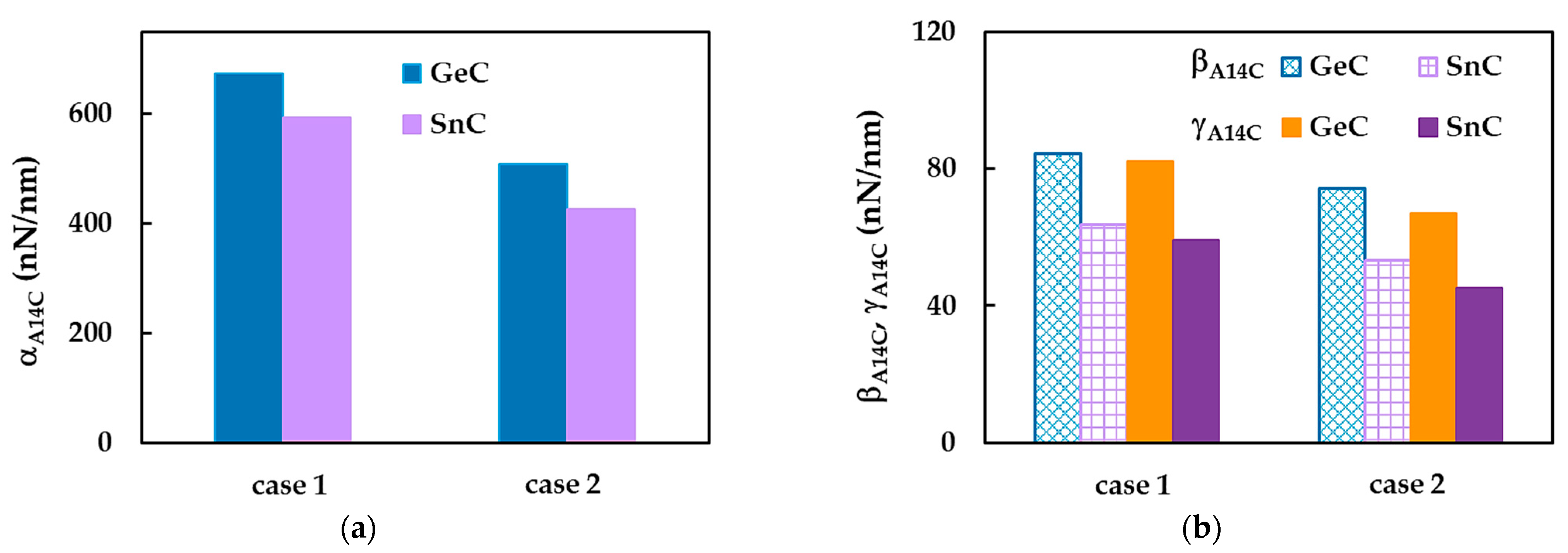
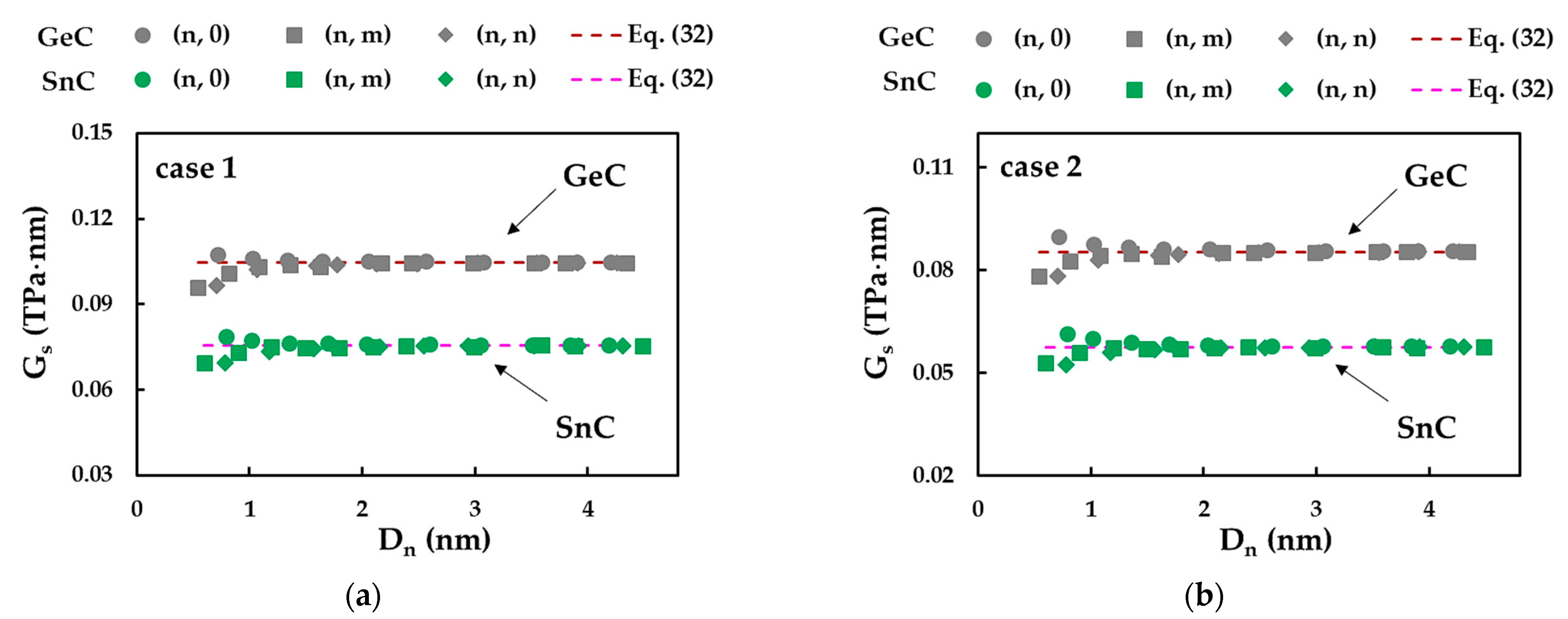
| Compound | Case | , nN/nm | , nN/nm | , nN/nm | |||
|---|---|---|---|---|---|---|---|
| GeC | 1 | 674.39 | 84.25 | 82.08 | 1.14 | 1.14 | 1.23 |
| 2 | 593.09 | 74.06 | 66.98 | ||||
| SnC | 1 | 509.02 | 63.60 | 59.24 | 1.19 | 1.19 | 1.31 |
| 2 | 426.65 | 53.28 | 45.16 |
| Compound | Case | , TPa nm | Mean Difference, % | |
|---|---|---|---|---|
| GeC | 1 | 0.214 | 1.14 | 0.33 |
| 2 | 0.189 | 0.25 | ||
| SnC | 1 | 0.162 | 1.19 | 0.27 |
| 2 | 0.136 | 0.20 |
| Compound | Case | 1 , TPa nm | G, TPa | Mean Difference, % | |
|---|---|---|---|---|---|
| GeC | 1 | 0.104 | 0.273 | 1.22 | 0.01 |
| 2 | 0.085 | 0.223 | 0.03 | ||
| SnC | 1 | 0.076 | 0.196 | 1.31 | 0.05 |
| 2 | 0.058 | 0.150 | 0.09 |
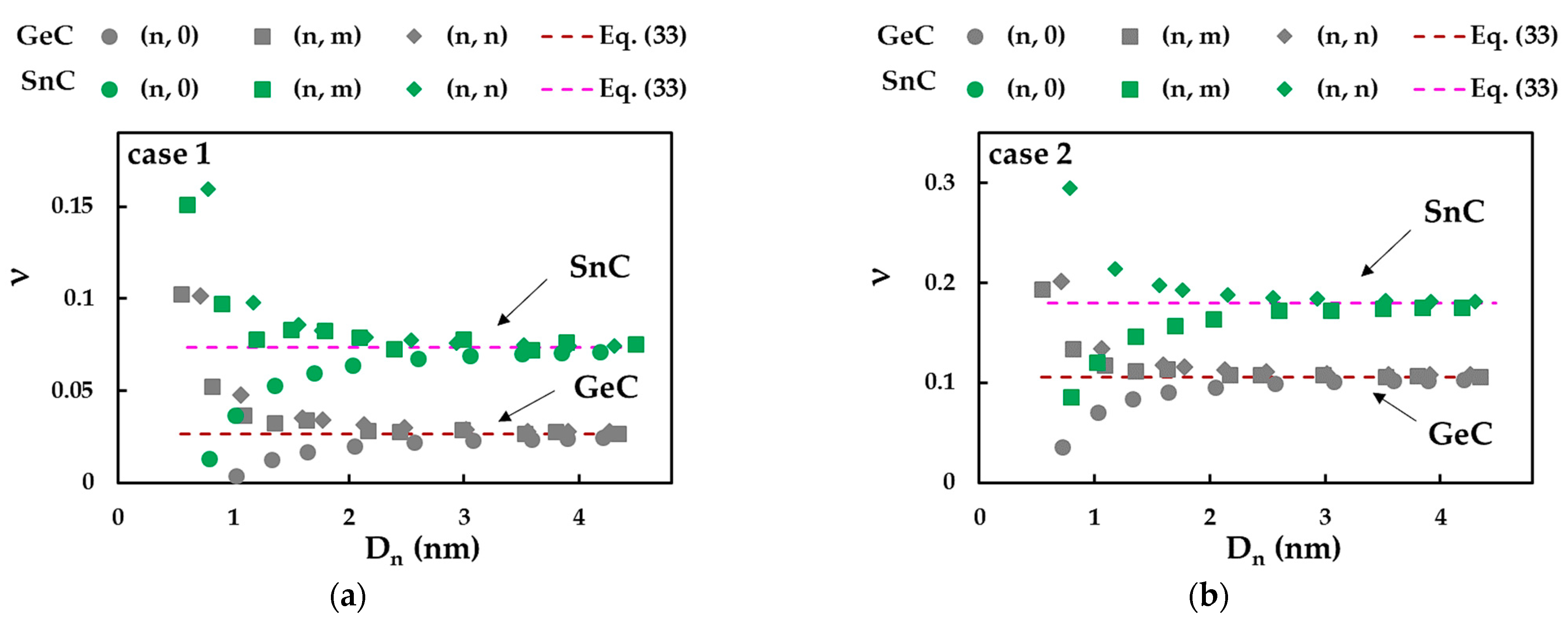
| Compound | Case | 1 ν | Mean Difference, % | |
|---|---|---|---|---|
| GeC | 1 | 0.026 | 4.0 | 0.20 |
| 2 | 0.106 | 0.10 | ||
| SnC | 1 | 0.074 | 2.4 | 0.11 |
| 2 | 0.180 | 0.04 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fernandes, J.V.; Pereira, A.F.G.; Antunes, J.M.; Chaparro, B.M.; Sakharova, N.A. Numerical Simulation Study of the Mechanical Behaviour of 1D and 2D Germanium Carbide and Tin Carbide Nanostructures. Materials 2023, 16, 5484. https://doi.org/10.3390/ma16155484
Fernandes JV, Pereira AFG, Antunes JM, Chaparro BM, Sakharova NA. Numerical Simulation Study of the Mechanical Behaviour of 1D and 2D Germanium Carbide and Tin Carbide Nanostructures. Materials. 2023; 16(15):5484. https://doi.org/10.3390/ma16155484
Chicago/Turabian StyleFernandes, José V., André F. G. Pereira, Jorge M. Antunes, Bruno M. Chaparro, and Nataliya A. Sakharova. 2023. "Numerical Simulation Study of the Mechanical Behaviour of 1D and 2D Germanium Carbide and Tin Carbide Nanostructures" Materials 16, no. 15: 5484. https://doi.org/10.3390/ma16155484
APA StyleFernandes, J. V., Pereira, A. F. G., Antunes, J. M., Chaparro, B. M., & Sakharova, N. A. (2023). Numerical Simulation Study of the Mechanical Behaviour of 1D and 2D Germanium Carbide and Tin Carbide Nanostructures. Materials, 16(15), 5484. https://doi.org/10.3390/ma16155484






