Calculation Method for the Cracking Resistance and Bearing Performance of SFRAC Beams
Abstract
1. Introduction
2. Experimental Program
- (i)
- Water–cement ratio: Recycled concrete with three water binder ratios of 0.55, 0.4, and 0.3 was studied.
- (ii)
- Recycled aggregate replacement rate (by a mass fraction): Four recycled aggregate replacement rates of 0, 30%, 50%, and 100% were studied.
- (iii)
- Steel fiber volume fraction: Five different steel fiber contents of 0, 0.5%, 1.0%, 1.5%, and 2.0% were adopted.
2.1. Materials and Mixture Proportions
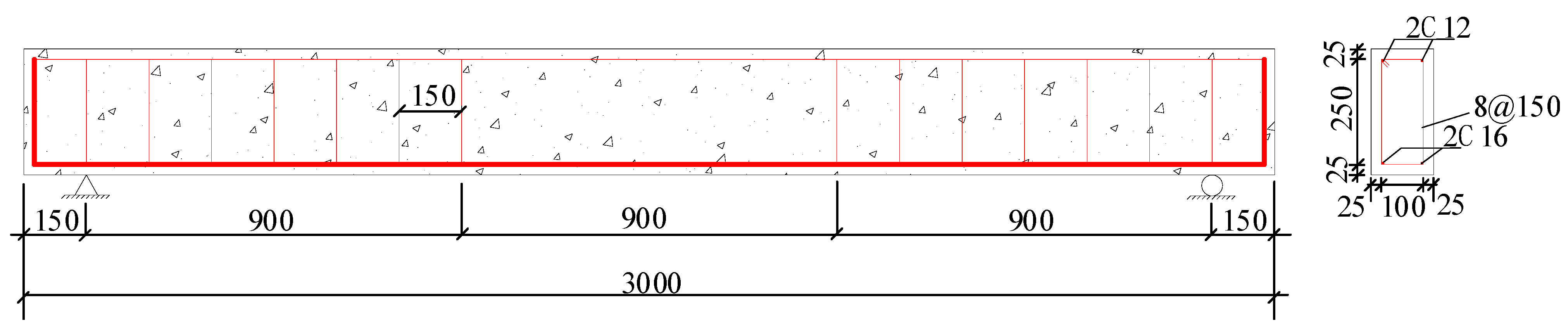
2.2. Dimensional and Geometrical Properties of the Specimens
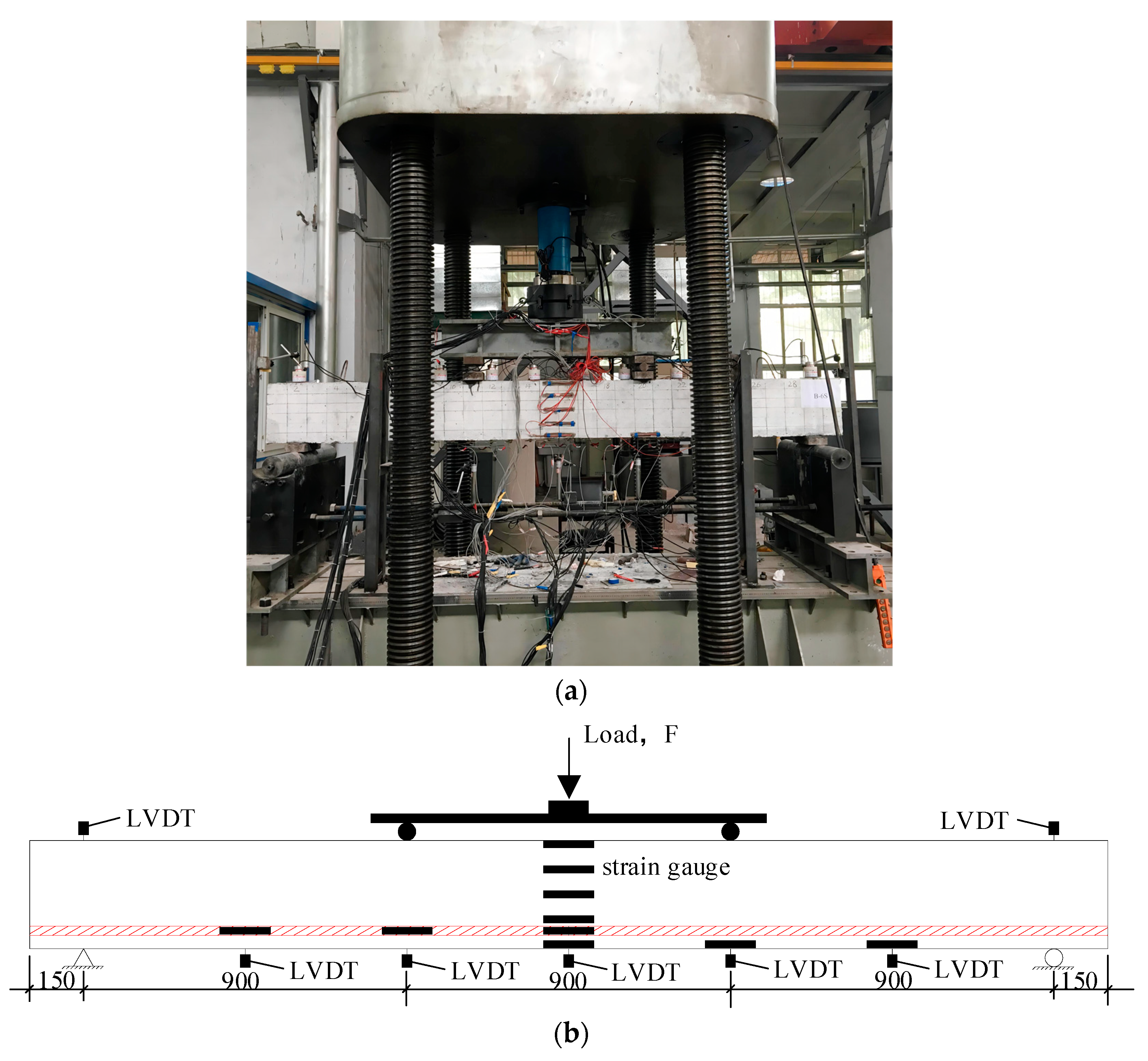
2.3. Test Equipment
3. Test Results and Analysis
3.1. Test Phenomenon and Failure Mode
3.2. Effects of Water–Cement Ratio
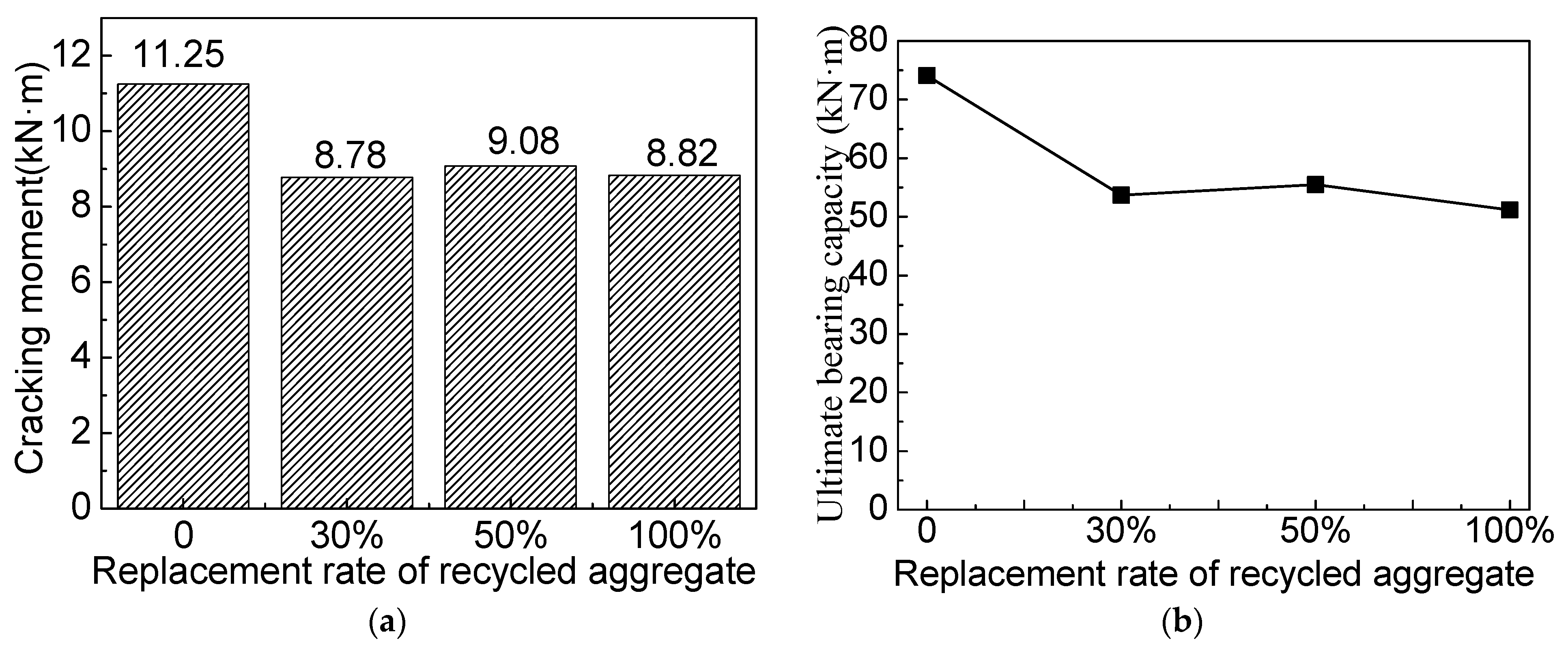
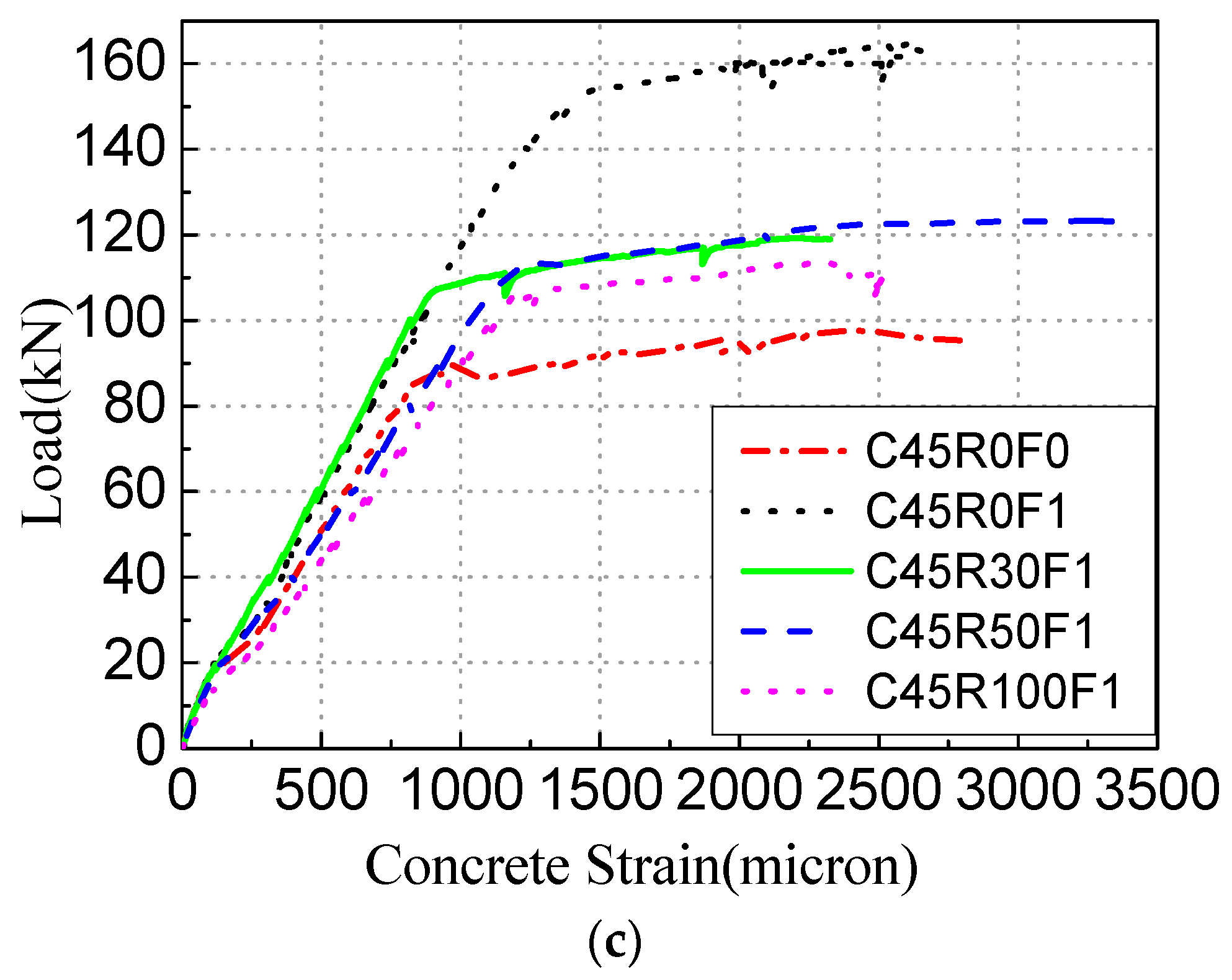
3.3. Effects of the Recycled Aggregate Replacement Rate
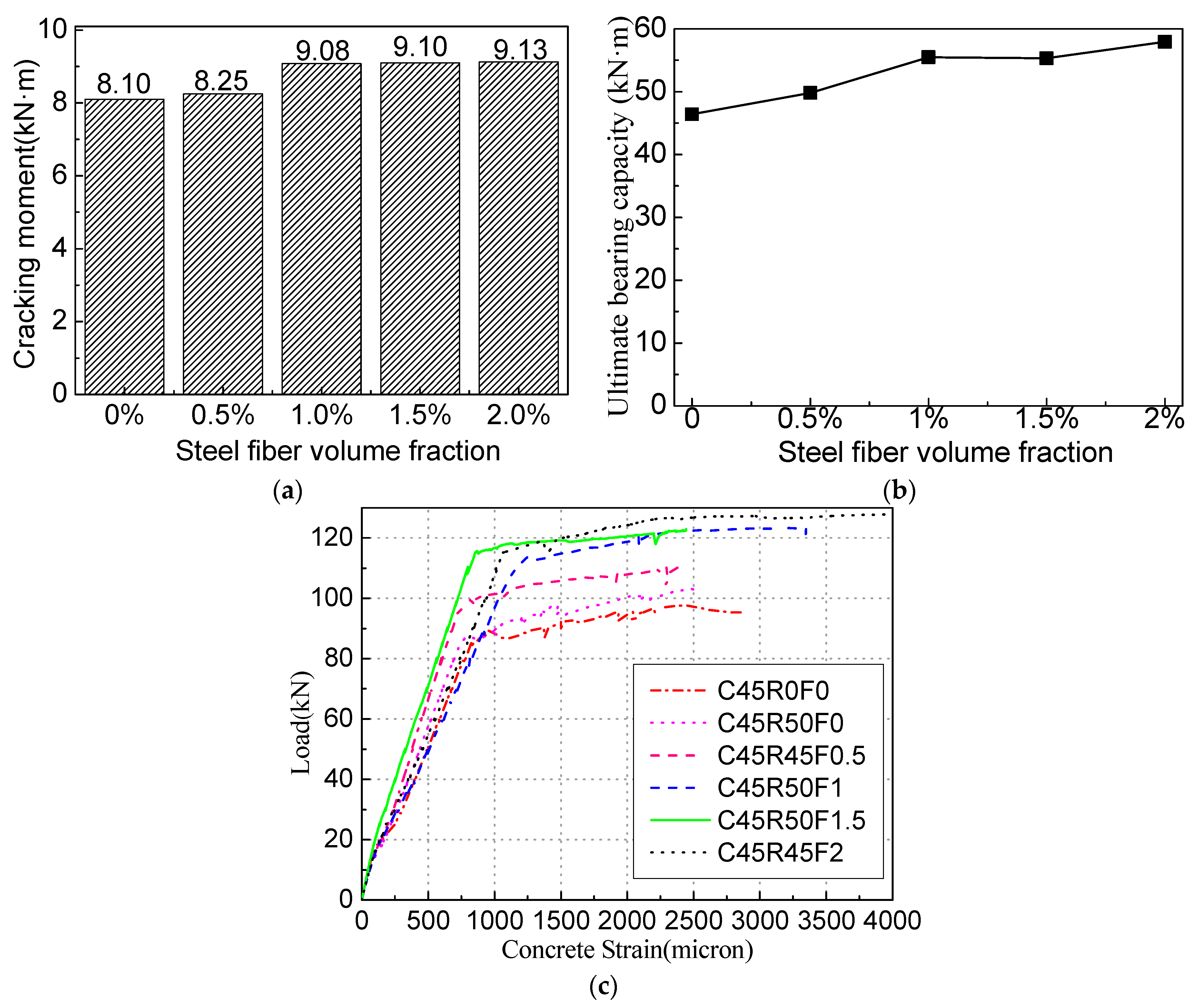
3.4. Effects of the Steel Fiber Volume Fraction
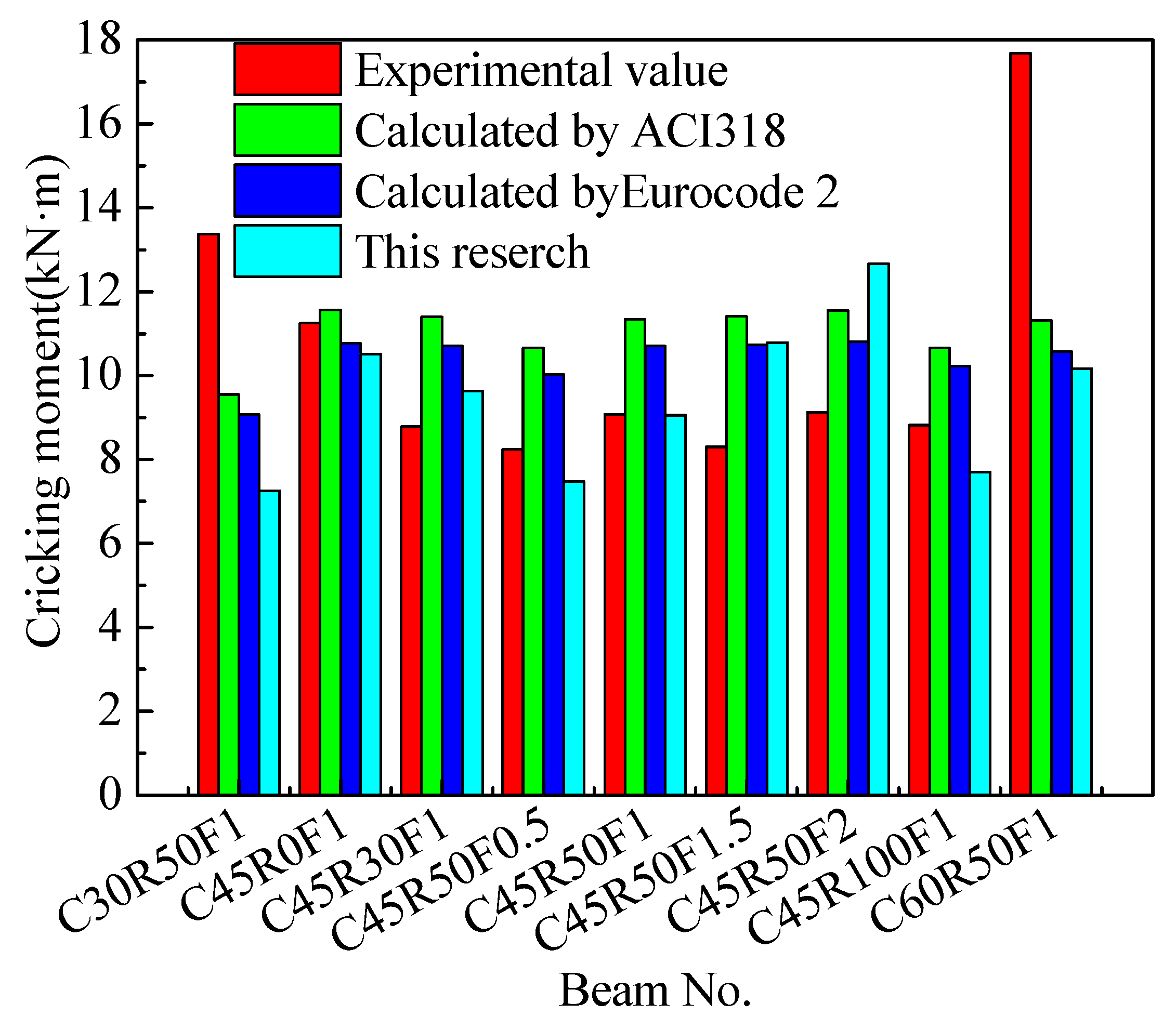
4. Calculation of Cracking Moment and Ultimate Bending Moment
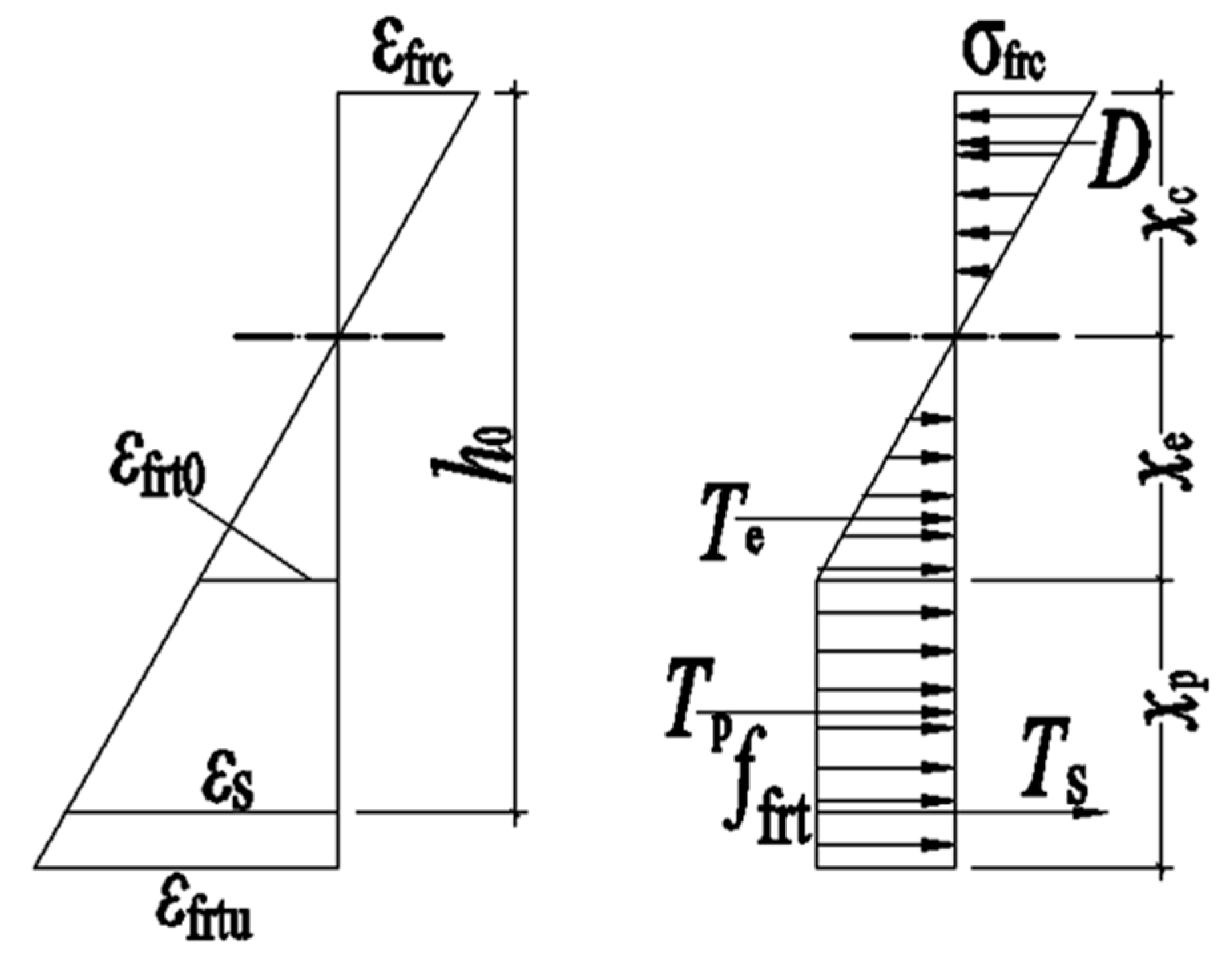
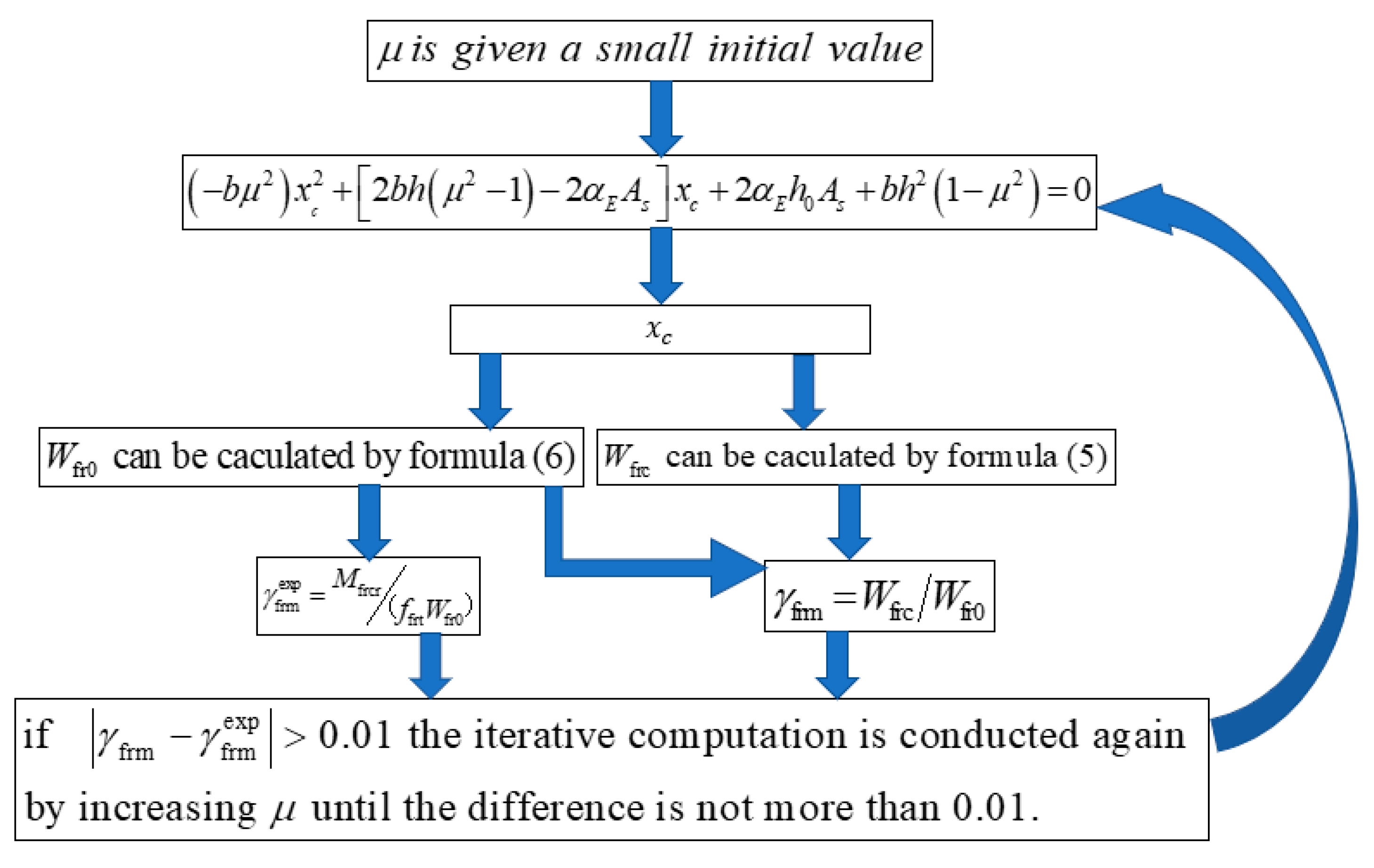
4.1. Calculation Method of the SFRAC Beam’s Cracking Moment
4.2. Calculation Method for the Flexural Bearing Capacity of the SFRAC Beam
5. Conclusions
- (1)
- The cracking and ultimate moment of SFRAC beams are higher than those of ordinary concrete reference beams.
- (2)
- The cracking and ultimate moment of SFRAC beams increase with the decrease in the water–cement ratio. The water–cement ratio decreases from 0.55 to 0.3, and the cracking moment and ultimate moment of SFRAC beams increase by 32.2% and 7.6%, respectively.
- (3)
- The cracking and ultimate bending moment of SFRAC beams are significantly higher than those of reference beams, and the cracking and ultimate moment of SFRAC beams increase with the increase in the steel fiber volume ratio. Compared with recycled concrete beams without steel fiber, the cracking moment and ultimate moment of SFRAC beams are increased by 12.6% and 24.8%, respectively, when the volume fraction of the steel fiber is 2.0%.
- (4)
- After adding recycled aggregate, the cracking moment and ultimate load of steel-fiber-reinforced concrete beams decrease, but the replacement rate of recycled aggregate has little effect on the cracking moment and ultimate load of SFRAC beams. When the replacement rate of recycled aggregate is increased from 30% to 100%, the cracking moment of the SFRAC beam is increased by 0.6%, and the ultimate moment is decreased by 4.7%.
- (5)
- Considering the influence of the recycled aggregate replacement rate and fiber volume ratio, the calculation formula of the cracking moment of SFRAC beams is established, and the prediction accuracy is better than ACI318 and Eurocode 2.
- (6)
- The calculated values of the ultimate bending moment bearing capacity based on ACI 318 and ACI 544 agree with the experimental values, which can reasonably predict the bending bearing capacity of SFRAC beams.
- (7)
- Steel fiber recycled concrete is a material with good performance, and the performance of SFRAC beams with 100% recycled aggregate replacement rate is still higher than that of ordinary concrete benchmark beams, so SFRAC can be used for general load-bearing beam structural members. Applying SFRAC to structural members increases the resource utilization of construction waste and meets the requirements of sustainable development and the transition to green building materials and technologies. In addition, the fatigue performance of SFRAC beams is also a point worthy of further study to expand its engineering application scope.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Reinforcement stress (MPa) | |
| Elastic modulus of steel bar (MPa) | |
| xc | Height of the compression zone (mm) |
| xe | Height of the elastic part of the tension zone (mm) |
| xp | Height of the elastic part of the plastic part (mm) |
| μ | Ratio of the height of the plastic zone to the height of the tension zone, μ = xp/(h − xc); |
| Ultimate compressive strain of SFRAC (με) | |
| Ultimate compressive stress of SFRAC (με) | |
| Peak tensile strain (με) | |
| Ultimate tensile strain of SFRAC (με) | |
| D | Resultant force of the compression zone (N) |
| Te | Resultant force of the elastic zone in the tension zone. (N) |
| Tp | Resultant force of the plastic region zone in the tensile region (N) |
| Ts | Resultant force of the longitudinal tensile bar (N) |
| Recycled Aggregate Replacement Rate | |
| Steel Fiber Volume Fraction | |
| ffrt | Tensile strength of SFRAC (N) |
| fy | Designed strength of the steel bar (MPa) |
| ft | Tensile strength of ordinary concrete with the same SFRAC strength grade (MPa) |
| Wfr0 | Resistance moment of the converted section after the longitudinal tensile reinforcement is converted into the SFRAC area. |
| b | Width of the beam section (mm) |
| h | Height of the beam section (mm) |
| h0 | Effective height of the beam section (mm) |
| Ratio of the elastic modulus of the longitudinal tensile steel bar to the SFRAC elastic modulus | |
| Measured value of the resistance moment plastic influence coefficient of the SFRAC section | |
| Measured cracking moment (kN·m) | |
| Calculation value of cracking moment (kN·m) | |
| Mfrcr | Cracking moment of the SFRAC beam (kN·m) |
| Resistance moment plastic influence coefficient of the SFRAC section | |
| Wfr0 | Resistance moment of the converted section after the longitudinal tensile reinforcement is converted into the SFRAC area (mm3) |
| Wfrc | Elastoplastic resistance moment of the section of the SFRAC beam to the tension zone edge of the section considering the plastic deformation (mm3) |
| Ultimate bending moment of SFRAC beam is calculated according to ACI 544.4 R-88 | |
| Ultimate bending moment of SFRAC beam is calculated according to ACI 318-2014 | |
| d | Distance from extreme compression fiber to centroid oftension reinforcement (mm) |
| a | Depth of rectangular stress block b =width of beam (mm) |
| c | Distance from extreme compression fiber to neutral axis found byequating the internal tension (mm) |
| e | Distance from extreme compression fiber to top of tensile stress block of fibrous concrete (mm) |
| Compressive strength of concrete (MPa) | |
| Tensile stress in fibrous concrete (MPa) | |
| Bond efficiency of the fiber | |
| Tensile strain in steel fiber at theoretical moment strength of beam | |
| Theoretically calculated value of the ultimate bending moment (kN·m) | |
| Measured value of the ultimate bearing capacity (kN·m) | |
| As | Section area of the longitudinal tensile bar (mm2) |
| Percent by volume of steel fibers |
References
- U.S. Geological Survey. Mineral Commodity Summaries 2020, U.S. Department of Interior. 2020. Available online: https://pubs.usgs.gov/periodicals/mcs2020/mcs2020.pdf (accessed on 18 April 2023).
- Deng, Q.; Zhang, R.; Liu, C.; Duan, Z.; Xiao, J. Influence of fiber properties on abrasion resistance of recycled aggregate concrete: Length, volume fraction, and types of fibers. Constr. Build. Mater. 2023, 362, 129750. [Google Scholar] [CrossRef]
- Ding, T.; Xiao, J. Estimation of building-related construction and demolition waste in Shanghai. Waste Manag. 2014, 34, 2327–2334. [Google Scholar] [CrossRef] [PubMed]
- Ketov, A.; Rudakova, L.; Vaisman, I.; Ketov, I.; Haritonovs, V.; Sahmenko, G. Recycling of rice husks ash for the preparation of resistant, lightweight and environment-friendly fired bricks. Constr. Build. Mater. 2021, 302, 124385. [Google Scholar] [CrossRef]
- Zhu, Q.; Chen, J.; He, Y.; Sun, X. Bond Stress Distribution and Bond-Slip Model of Deformed Steel Bars in Iron Tailing Sand Recycled Aggregate Concrete. Buildings 2023, 13, 1176. [Google Scholar] [CrossRef]
- Cakiroglu, C.; Bekdaş, G. Predictive Modeling of Recycled Aggregate Concrete Beam Shear Strength Using Explainable Ensemble Learning Methods. Sustainability 2023, 15, 4957. [Google Scholar] [CrossRef]
- Li, T.; Wang, C.; Wang, C. Calculation on flexural capacity of recycled aggregate concrete beams. Adv. Struct. Eng. 2023, 26, 1078–1092. [Google Scholar] [CrossRef]
- Seara-Paz, S.; González-Fonteboa, B.; Martínez-Abella, F.; Eiras-López, J. Flexural performance of reinforced concrete beams made with recycled concrete coarse aggregate. Eng. Struct. 2018, 156, 32–45. [Google Scholar] [CrossRef]
- Wu, J.; Geng, J.; Yang, X. Experimental research on crack widths of normal sections of recycled aggregate concrete beams under short term loading. J. Build. Struct. 2011, 32, 107–114. [Google Scholar] [CrossRef]
- Yang, G.; Wu, J.; Ye, Q. Study on Deflection of Recycled Concrete Beams. Eng. Mech. 2011, 147–151. Available online: http://www.engineeringmechanics.cn/article/id/1497 (accessed on 18 April 2023).
- Sadowska-Buraczewska, B.; Barnat-Hunek, D.; Szafraniec, M. Influence of Recycled High-Performance Aggregate on Deformation and Load-Carrying Capacity of Reinforced Concrete Beams. Materials 2020, 13, 186. [Google Scholar] [CrossRef]
- Cao, W.; Peng, S.; Qiao, Q.; Lin, D.; Zhu, K. Experimental study on deformation performance of full-sized high strength recycled reinforced concrete beams under long-term loading. J. Build. Struct. 2017, 38, 142–148. [Google Scholar] [CrossRef]
- Bai, G.; Qin, C.; Zhang, Y.; Lin, C.; Han, Y. Time-dependent calculation method for the long-term deformation of recycled aggregate concrete beams. China Civ. Eng. J. 2016, 49, 1–8. [Google Scholar] [CrossRef]
- Liu, C.; Lv, Z.; Zhu, C.; Bai, G.; Zhang, Y. Study on Calculation Method of Long Term Deformation of RAC Beam based on Creep Adjustment Coefficient. KSCE J. Civ. Eng. 2019, 23, 260–267. [Google Scholar] [CrossRef]
- VTam, W.Y.; Kotrayothar, D.; Xiao, J. Long-term deformation behaviour of recycled aggregate concrete. Constr. Build. Mater. 2015, 100, 262–272. [Google Scholar] [CrossRef]
- Cao, W.; Liu, Y.; Qiao, Q.; Feng, Y.; Peng, S. Time-Dependent Behavior of Full-Scale Recycled Aggregate Concrete Beams under Long-Term Loading. Materials 2020, 13, 4862. [Google Scholar] [CrossRef] [PubMed]
- Arora, S.; Singh, S.P. Fatigue strength and failure probability of concrete made with RCA. Mag. Concr. Res. 2017, 69, 55–67. [Google Scholar] [CrossRef]
- Rao, A.; Jha, K.N.; Misra, S. Use of aggregates from recycled construction and demolition waste in concrete. Resour. Conserv. Recycl. 2007, 50, 71–81. [Google Scholar] [CrossRef]
- Heeralal, M.; Kumar, R.; Rao, Y.V. Flexural fatigue characteristics of steel fiber reinforced recycled aggregate concrete (SFRRAC). Facta Univ.-Ser. Archit. Civ. Eng. 2009, 7, 19–33. [Google Scholar] [CrossRef]
- Senaratne, S.; Gerace, D.; Mirza, O.; Tam, V.W.Y.; Kang, W. The costs and benefits of combining recycled aggregate with steel fibres as a sustainable, structural material. J. Clean. Prod. 2016, 112, 2318–2327. [Google Scholar] [CrossRef]
- Ali, B.; Qureshi, L.A. Influence of glass fibers on mechanical and durability performance of concrete with recycled aggregates. Constr. Build. Mater. 2019, 228, 396–406. [Google Scholar] [CrossRef]
- Kachouh, N.; El-Hassan, H.; El-Maaddawy, T. Influence of steel fibers on the flexural performance of concrete incorporating recycled concrete aggregates and dune sand. J. Sustain. Cem. Based Mater. 2021, 10, 165–192. [Google Scholar] [CrossRef]
- Gao, D.; Zhang, L. Flexural performance and evaluation method of steel fiber reinforced recycled coarse aggregate concrete. Constr. Build. Mater. 2018, 159, 126–136. [Google Scholar] [CrossRef]
- Ghalehnovi, M.; Karimipour, A.; de Brito, J.; Chaboki, H.R. Crack Width and Propagation in Recycled Coarse Aggregate Concrete Beams Reinforced with Steel Fibres. Appl. Sci. 2020, 10, 7587. [Google Scholar] [CrossRef]
- Kang, W.; Ramesh, R.B.; Mirza, O.; Senaratne, S.; Tam, V.; Wigg, D. Reliability Based Design of RC Beams with Recycled Aggregate and Steel Fibres. Structures 2017, 11, 135–145. [Google Scholar] [CrossRef]
- Chaboki, H.R.; Ghalehnovi, M.; Karimipour, A.; de Brito, J. Experimental study on the flexural behaviour and ductility ratio of steel fibres coarse recycled aggregate concrete beams. Constr. Build. Mater. 2018, 186, 400–422. [Google Scholar] [CrossRef]
- Sadowska-Buraczewska, B.; Skrzypczak, I. Reinforced Concrete Beams Made of High-Performance Recycled Aggregate with Use Steel Fibre. Iop Conf. Ser. Mater. Sci. Eng. 2019, 471, 52021. [Google Scholar] [CrossRef]
- GB 175-2020; Common Portland Cement. China Standard Press: Beijing, China, 2007.
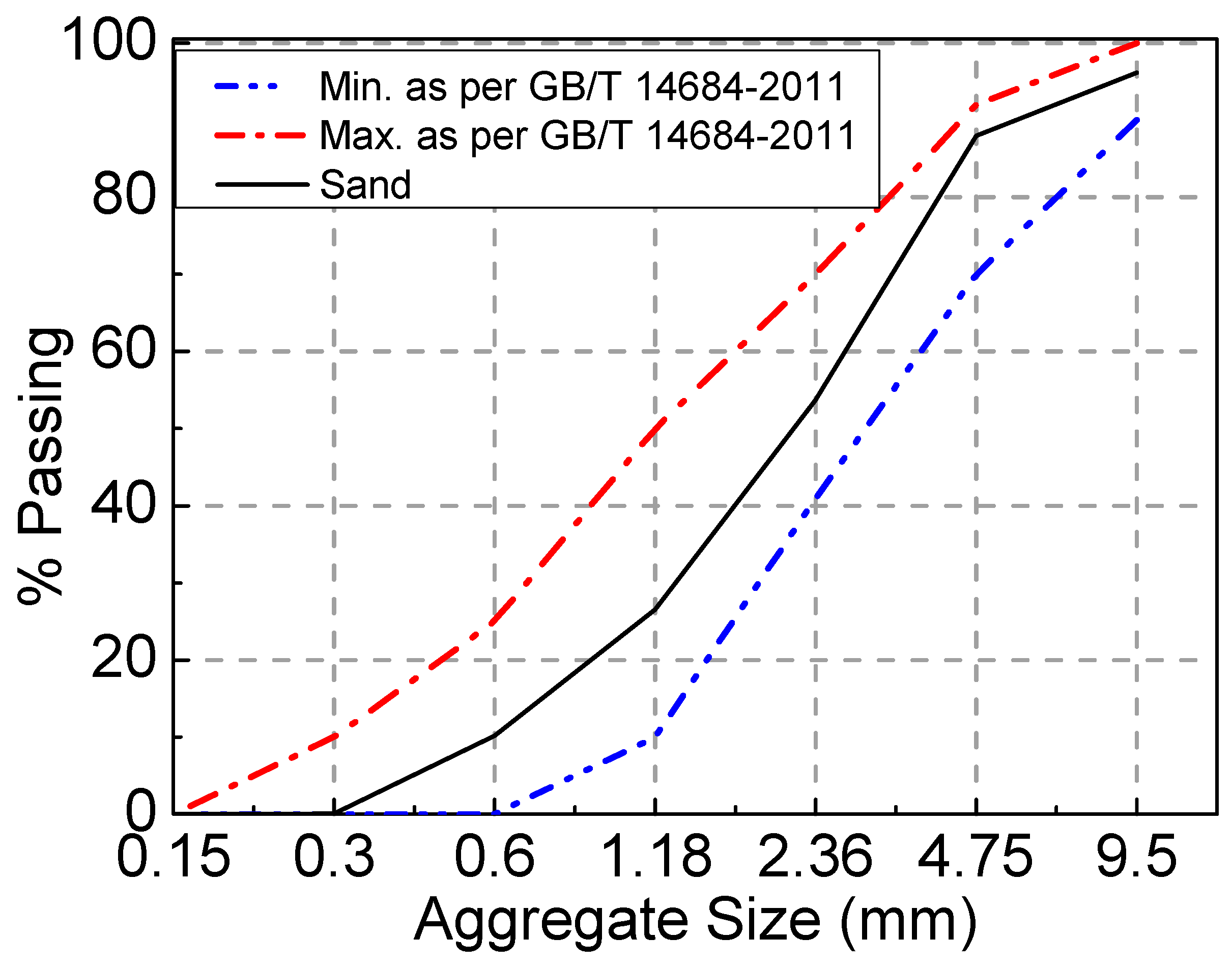
- GB/T 14685-2011; Pebble and Crushed Stone for Construction. China Standard Press: Beijing, China, 2011.
- Gao, D.; Zhu, Q. Constitutive Model and Bond Stress-slip Behavior Between Rebar and Steel Fiber Reinforced Recycled Concrete. China J. Highw. Transp. 2018, 31, 172–180. Available online: http://zgglxb.chd.edu.cn/CN/Y2018/V31/I6/172 (accessed on 18 April 2023).
- GB/T50152-2012; Concrete Structure Test Method Standard. China Standard Press: Beijing, China, 2012.
- Zhu, Q. Performance and Calculation Method of Steel Fiber Reinforced Recycled Aggregate Concrete; Zhengzhou University: Zhengzhou, China, 2018; pp. 54–96. [Google Scholar]
- Alnahhal, W.; Aljidda, O. Flexural behavior of basalt fiber reinforced concrete beams with recycled concrete coarse aggregates. Constr. Build. Mater. 2018, 169, 165–178. [Google Scholar] [CrossRef]
- Oh, B.H. Flexural Analysis of Reinforced Concrete Beams Containing Steel Fibers. J. Struct. Eng. 1992, 120, 2821–2835. [Google Scholar] [CrossRef]
- ACI Committee 544. Guide to Design with Fiber-Reinforced Concrete (ACI 544.4R-88); American Concrete Institute: Farmington Hills, MI, USA, 1988. [Google Scholar]
- ACI Committee 318. Building Code Requirements for Structural Concrete (ACI 318-14) and Commentary (ACI 318R-14); American Concrete Institute: Farmington Hills, MI, USA, 2014. [Google Scholar]
- Gao, D.; Zhu, Q.; Liu, J. Constitutive Model of SFRCAC under uniaxial compression. J. Basic Sci. Eng. 2020, 28, 396–406. [Google Scholar] [CrossRef]
- GB 50010-2010; Code for Design of Concrete Structures. China Architecture & Building Press: Beijing, China, 2015.
- EN-1992-1-1; Eurocode 2—Design of Concrete Structures. Part 1–1: GeneralRules and Rules for Buildings. Comité Européen de Normalisation (CEN): Brussels, Belgium, 2008; p. 259.




| Standard Consistency (%) | Fineness (%) | Specific Surface Area (m2/kg) | Density (kg/m3) | Loss on Ignition of SO3 (%) | Stability |
|---|---|---|---|---|---|
| 28.5 | 4.7 | 3460 | 3043 | 2.3 | Qualified |
| Setting time (min) | Compressive strength (MPa) | Flexural strength (MPa) | |||
| Initial setting | Final setting | 3 d | 28 d | 3 d | 28 d |
| 142 | 229 | 26.1 | 49.4 | 4.97 | 8.64 |
| Aggregate | Apparent Density (kg/m3) | Bulk Density (kg/m3) | Water Absorption (%) | Acicular Content (%) | Mud Content (%) | Porosity (%) | Crush Index (%) |
|---|---|---|---|---|---|---|---|
| RCA | 2660 | 1410 | 3.74 | 1.4 | 0.423 | 47 | 13.5 |
| NCA | 2730 | 1360 | 0.6 | 3.2 | 0.925 | 40 | 12.0 |
| Beam ID | Water/Cement | RCA Replacement (%) | Steel Fiber Content (%) | Water (kg/m3) | Cement (kg/m3) | River Sand (kg/m3) | RCA (kg/m3) | NCA (kg/m3) |
|---|---|---|---|---|---|---|---|---|
| C30R0F0 | 0.55 | 0 | 0 | 166 | 302 | 884 | 0 | 1080 |
| C45R0F0 | 0.4 | 0 | 0 | 166 | 415 | 839 | 0 | 1024 |
| C60R0F0 | 0.3 | 0 | 0 | 166 | 553 | 783 | 0 | 958 |
| C45R0F1 | 0.4 | 0 | 1 | 166 | 415 | 839 | 0 | 1024 |
| C45R30F1 | 0.4 | 30 | 1 | 166 | 415 | 839 | 307 | 717 |
| C45R50F1 | 0.4 | 50 | 1 | 166 | 415 | 839 | 512 | 512 |
| C45R100F1 | 0.4 | 100 | 1 | 166 | 415 | 839 | 1024 | 0 |
| C45R50F0 | 0.4 | 50 | 0 | 166 | 415 | 839 | 512 | 512 |
| C45R50F0.5 | 0.4 | 50 | 0.5 | 166 | 415 | 839 | 512 | 512 |
| C45R50F1.5 | 0.4 | 50 | 1.5 | 166 | 415 | 839 | 512 | 512 |
| C45R50F2 | 0.4 | 50 | 2 | 166 | 415 | 839 | 512 | 512 |
| C30R50F1 | 0.55 | 50 | 1 | 166 | 302 | 884 | 540 | 540 |
| C60R50F1 | 0.3 | 50 | 1 | 166 | 553 | 783 | 479 | 479 |
| Beam ID | Cracking Moment (kN) | Ultimate Load (kN) | |||
|---|---|---|---|---|---|
| MPa | MPa | ×104 MPa | |||
| C30R0F0 | 36.18 | 1.92 | 3 | 12.5 | 104.3 |
| C45R0F0 | 45.68 | 2.49 | 3.59 | 18.2 | 97.6 |
| C60R0F0 | 58.43 | 2.88 | 3.6 | 16.2 | 106.5 |
| C45R0F1 | 47.69 | 5.34 | 3.64 | 17.6 | 164.7 |
| C45R30F1 | 46.39 | 5.75 | 3.45 | 19.5 | 119.3 |
| C45R50F1 | 45.88 | 4.81 | 3.3 | 20.2 | 123.3 |
| C45R100F1 | 40.5 | 4.68 | 2.97 | 19.6 | 113.7 |
| C45R50F0 | 37.26 | 1.99 | 3.13 | 18 | 103.2 |
| C45R50F0.5 | 40.58 | 3.45 | 3.42 | 18.3 | 110.7 |
| C45R50F1.5 | 46.44 | 6.14 | 3.38 | 18.5 | 122.9 |
| C45R50F2 | 47.62 | 7.18 | 3.55 | 20.3 | 128.7 |
| C30R50F1 | 32.58 | 4.39 | 3.16 | 13.4 | 114.8 |
| C60R50F1 | 45.74 | 4.22 | 3.38 | 17.7 | 123.5 |
| Beam No. | (kN·m) | μ | (kN·m) | ||
|---|---|---|---|---|---|
| C30R0F0 | 5.63 | 0.43 | 1.54 | 1.55 | 5.67 |
| C45R0F0 | 8.20 | 0.58 | 1.80 | 1.55 | 7.08 |
| C60R0F0 | 7.29 | 0.35 | 1.42 | 1.55 | 7.93 |
| C45R0F1 | 11.25 | 0.66 | 1.98 | 1.83 | 10.51 |
| C45R30F1 | 8.78 | 0.48 | 1.63 | 1.78 | 9.63 |
| C45R50F1 | 9.08 | 0.55 | 1.74 | 1.74 | 9.06 |
| C45R100F1 | 8.82 | 0.59 | 1.86 | 1.65 | 7.70 |
| C45R50F0 | 8.10 | 0.63 | 1.93 | 1.46 | 6.04 |
| C45R50F0.5 | 8.25 | 0.56 | 1.77 | 1.60 | 7.48 |
| C45R50F1.5 | 8.30 | 0.37 | 1.45 | 1.88 | 10.79 |
| C45R50F2 | 9.13 | 0.38 | 1.47 | 2.02 | 12.67 |
| C30R50F1 | 6.02 | 0.36 | 1.44 | 1.74 | 7.25 |
| C60R50F1 | 7.96 | 0.31 | 1.37 | 1.74 | 10.17 |
| Beam No. | |||
|---|---|---|---|
| C30R0F0 | 47.3 | 46.9 | 1.0 |
| C45R0F0 | 48.2 | 51.7 | 0.9 |
| C45R50F0 | 47.4 | 43.9 | 1.1 |
| C60R0F0 | 49.0 | 74.1 | 0.7 |
| C45R50F0.5 | 47.8 | 53.7 | 0.9 |
| C30R50F1 | 46.8 | 46.4 | 1.0 |
| C45R0F1 | 48.4 | 49.8 | 1.0 |
| C45R100F1 | 47.8 | 55.5 | 0.9 |
| C45R30F1 | 48.3 | 55.3 | 0.9 |
| C45R50F1 | 48.3 | 57.9 | 0.8 |
| C60R50F1 | 48.3 | 51.2 | 0.9 |
| C45R50F1.5 | 48.3 | 47.9 | 1.0 |
| C45R50F2 | 48.4 | 55.6 | 0.9 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhu, Q.; Liu, J. Calculation Method for the Cracking Resistance and Bearing Performance of SFRAC Beams. Materials 2023, 16, 4769. https://doi.org/10.3390/ma16134769
Zhu Q, Liu J. Calculation Method for the Cracking Resistance and Bearing Performance of SFRAC Beams. Materials. 2023; 16(13):4769. https://doi.org/10.3390/ma16134769
Chicago/Turabian StyleZhu, Qian, and Jie Liu. 2023. "Calculation Method for the Cracking Resistance and Bearing Performance of SFRAC Beams" Materials 16, no. 13: 4769. https://doi.org/10.3390/ma16134769
APA StyleZhu, Q., & Liu, J. (2023). Calculation Method for the Cracking Resistance and Bearing Performance of SFRAC Beams. Materials, 16(13), 4769. https://doi.org/10.3390/ma16134769