Co-Pyrolysis for Pine Sawdust with Potassium Chloride: Insight into Interactions and Assisting Biochar Graphitization
Abstract
1. Introduction
2. Experimental Section
2.1. Material Preparation
2.2. Thermogravimetric Analysis
3. Results and Discussion
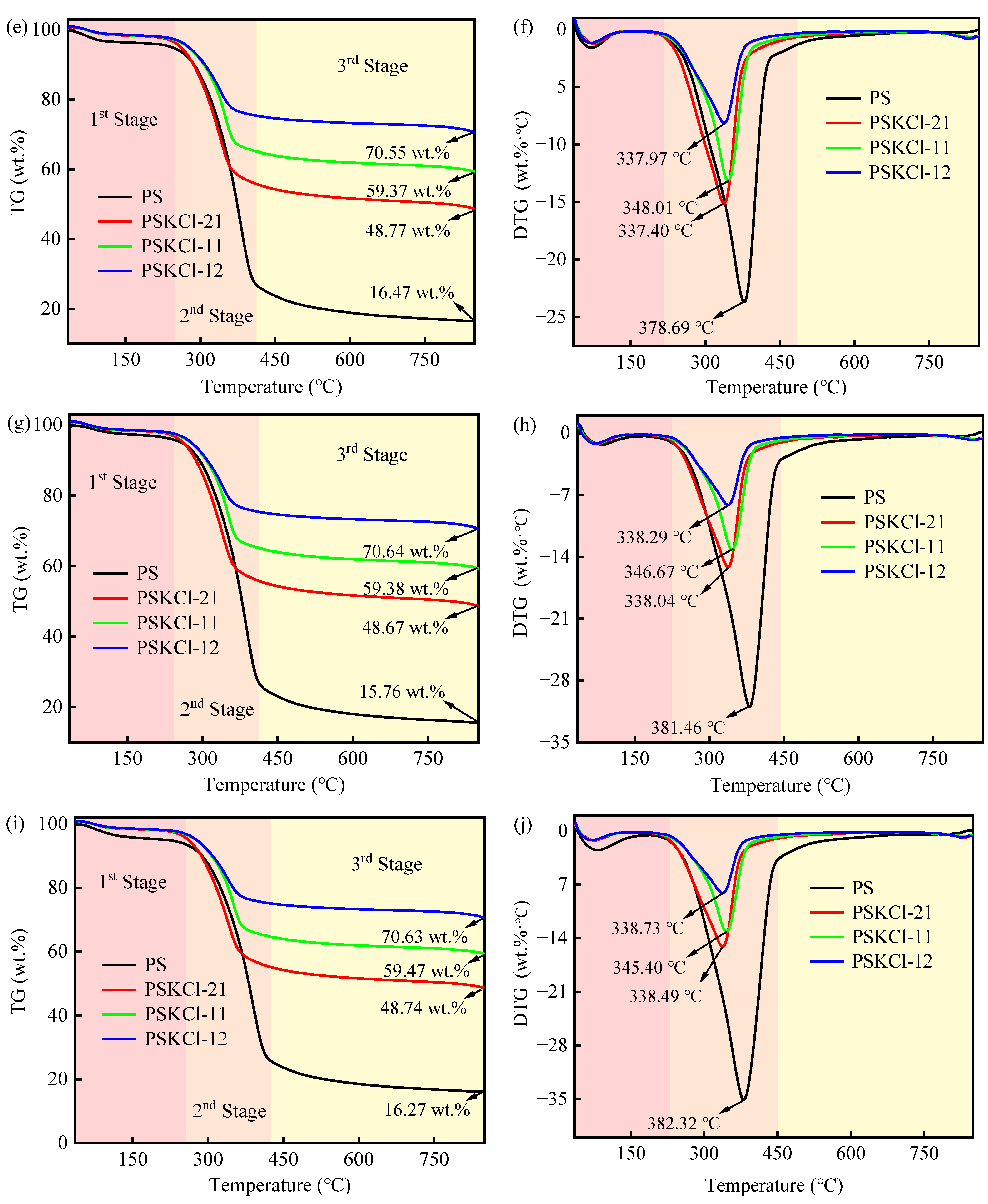
3.1. Weightless Behavior
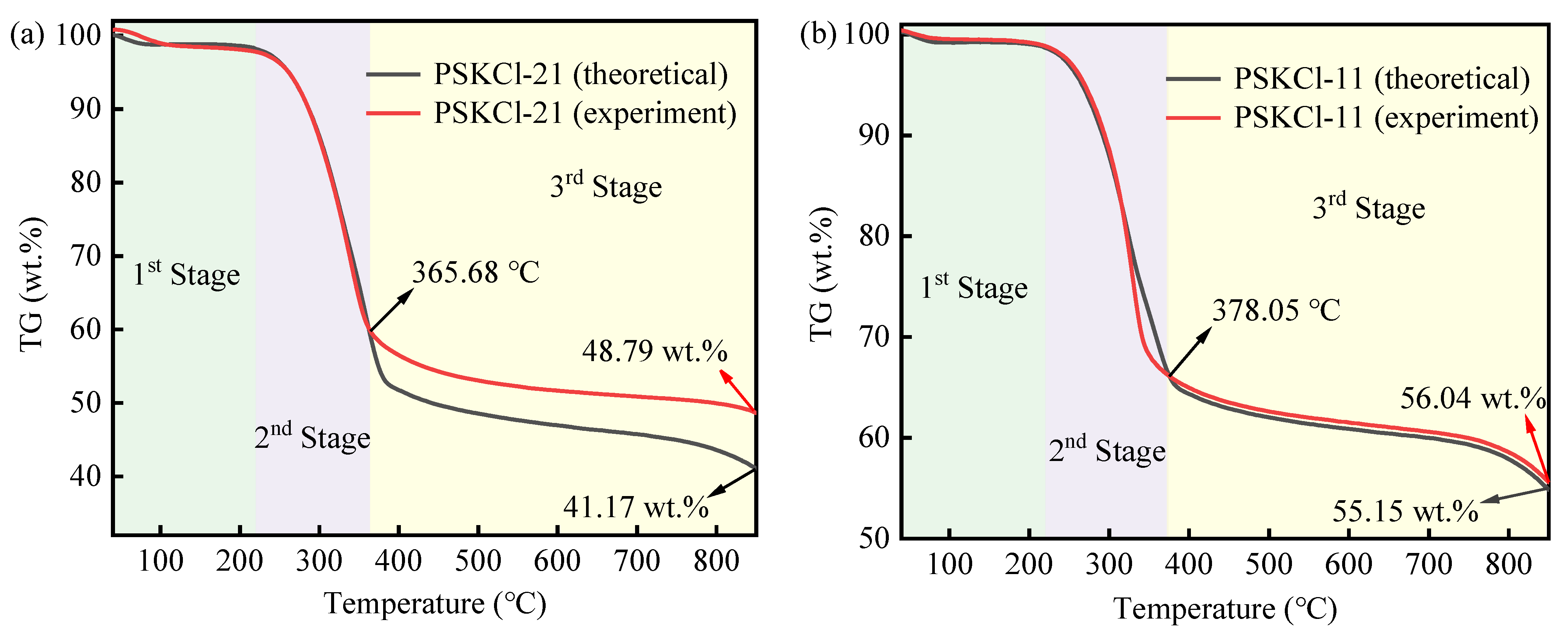
3.2. Theoretical and Experimental TG Curves
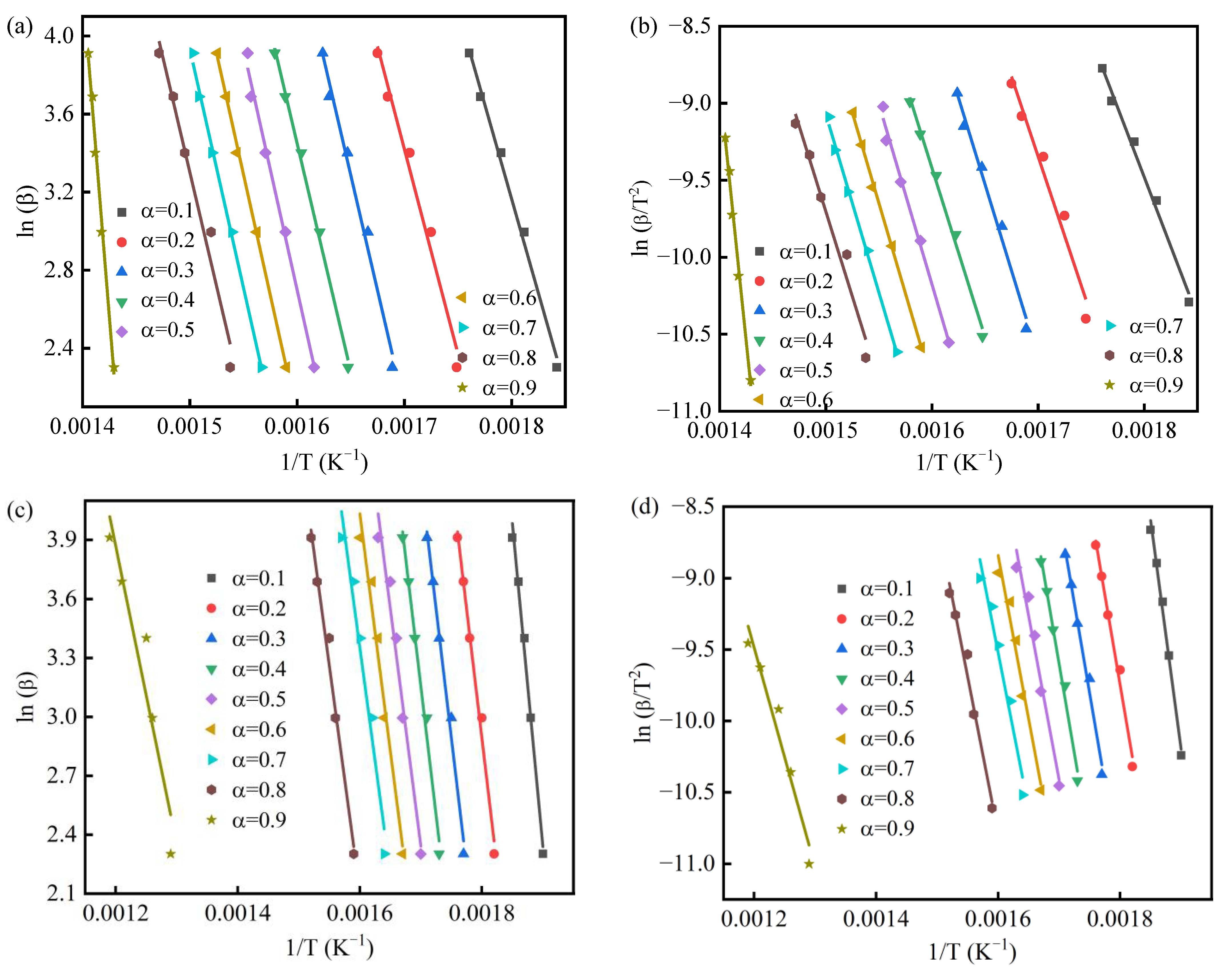
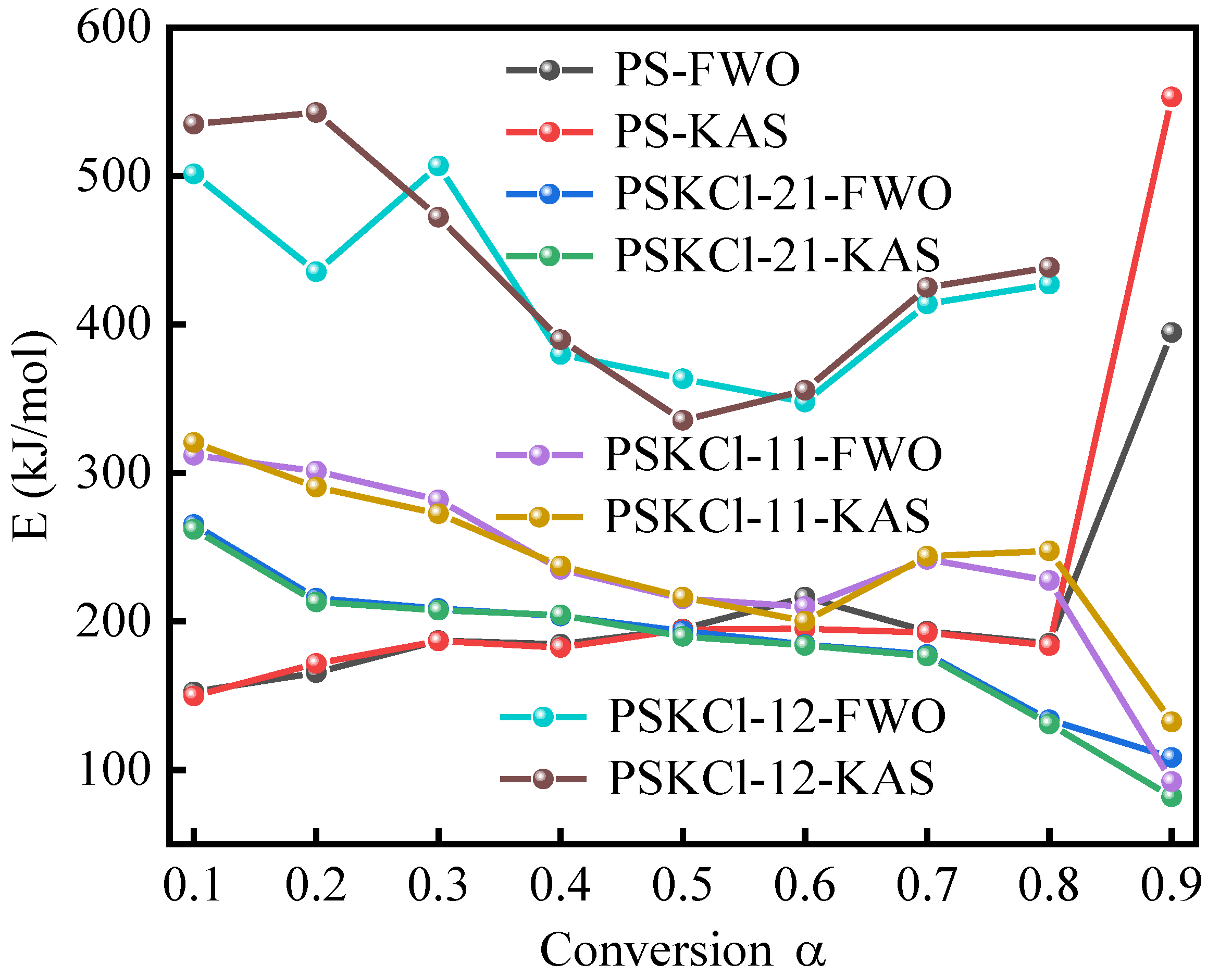
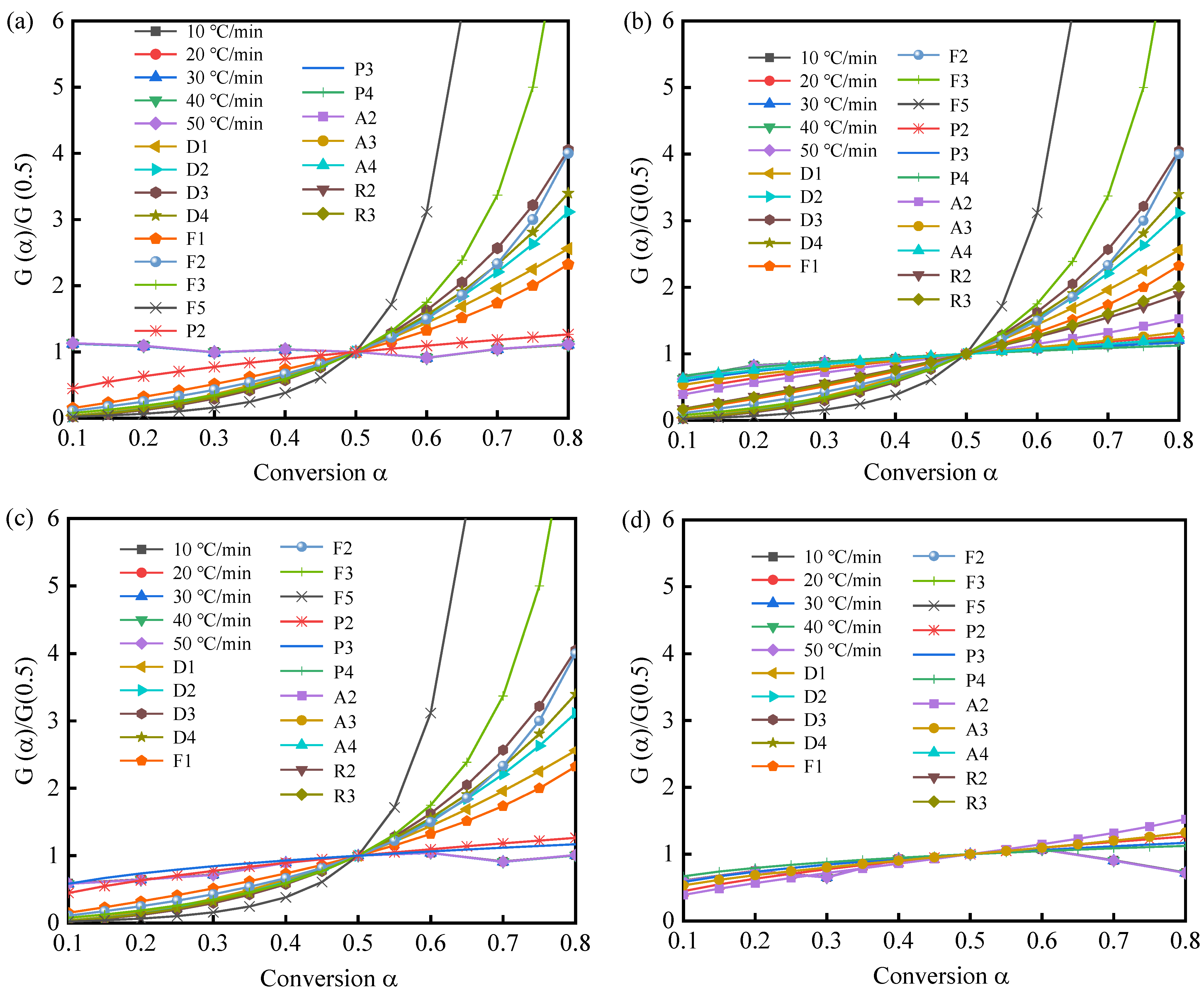
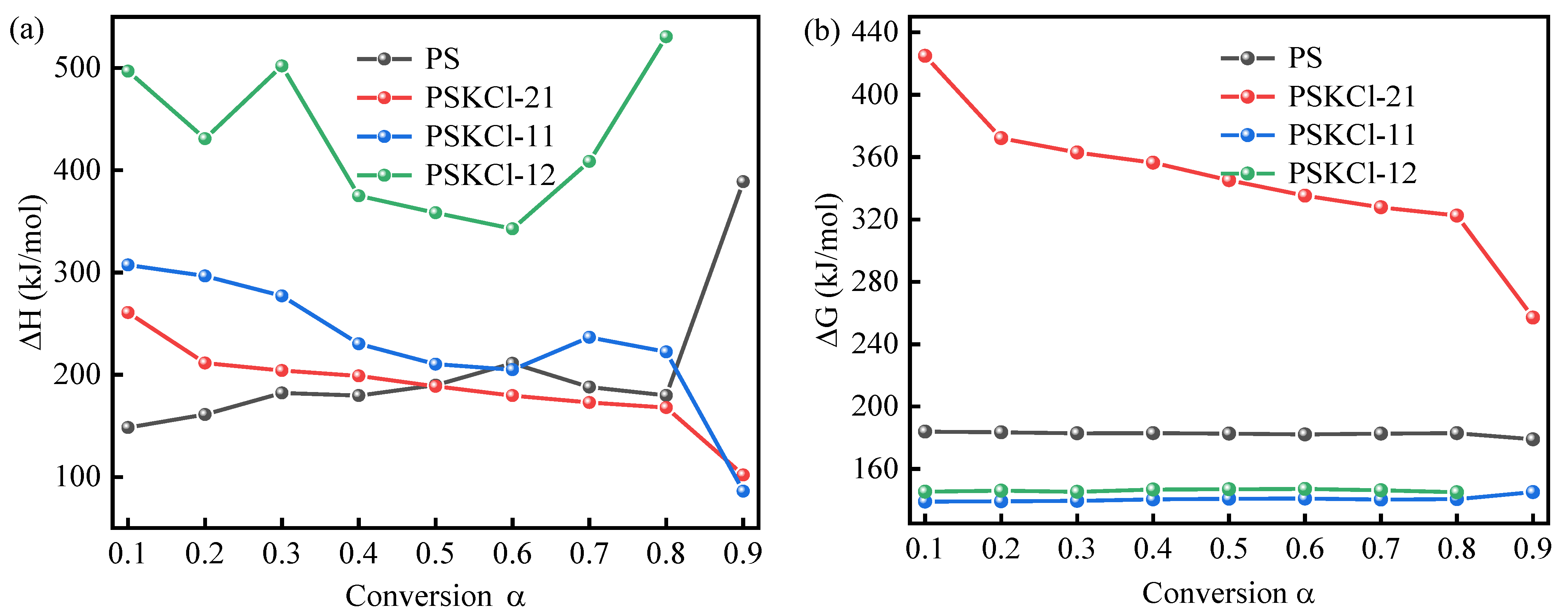
3.3. Pyrolysis Mechanism
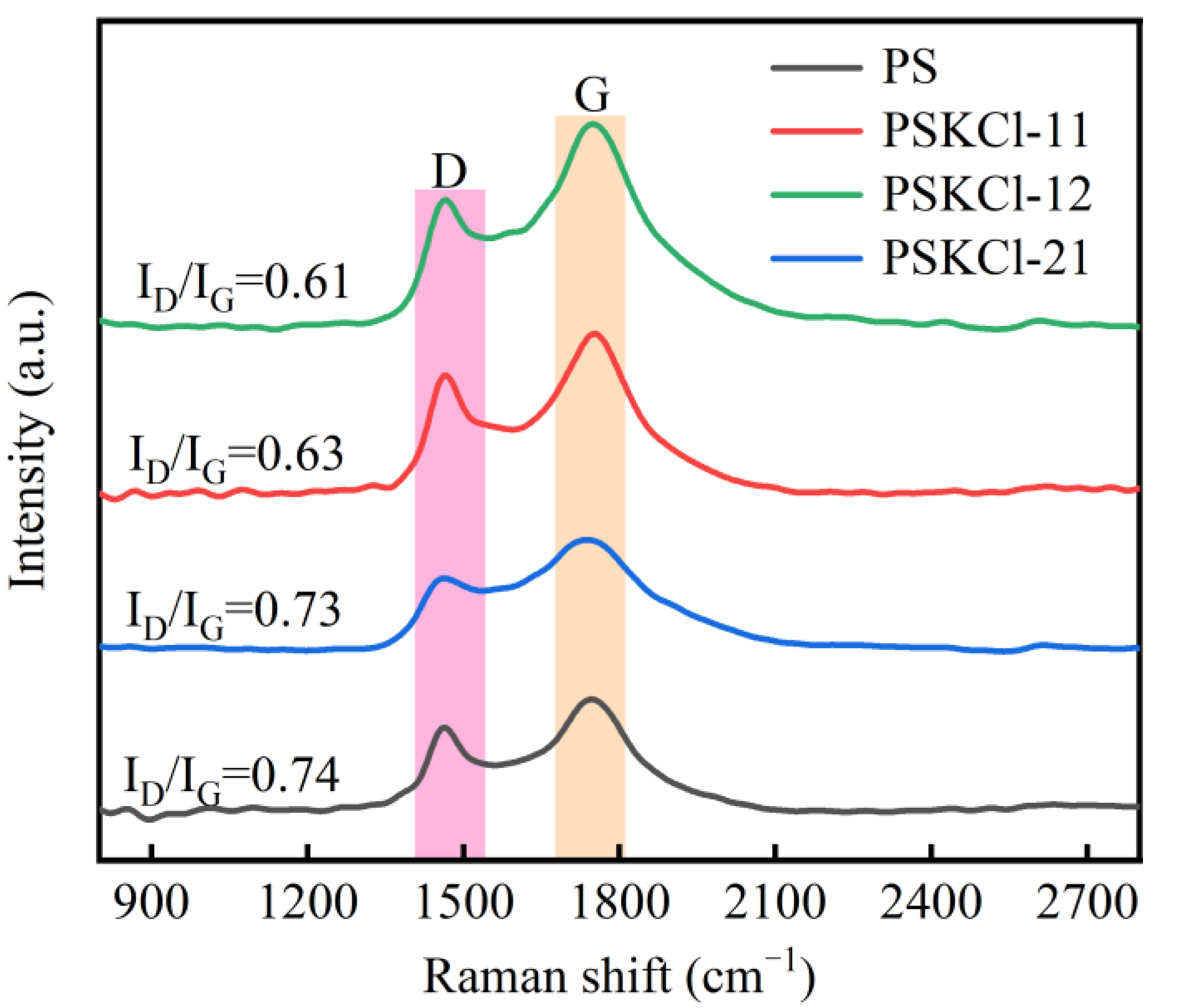
3.4. Disorder Analysis
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| Acronym | Meaning |
| PS | Pine sawdust |
| PSKCl-21 | The sample was obtained by mixing PS and KCl with a weight ratio of 2:1 |
| PSKCl-11 | The sample was obtained by mixing PS and KCl with a weight ratio of 1:1 |
| PSKCl-12 | The sample was obtained by mixing PS and KCl with a weight ratio of 1:2 |
| TGA | Thermogravimetric analysis |
| A | Pre-exponential factor |
| ΔH | Enthalpy |
| ΔG | Gibbs free energy |
| ΔS | Entropy |
| FWO | Flynn-Wall-Ozawa |
| KAS | Kissinger-Akahira-Sunose |
| E | Activation energy |
| T | Absolute temperature |
| R2 | Correlation coefficient |
| K | Boltzmann constant |
| Tm | The peak temperature for the DTG curve |
| h | Plank constant |
References
- Chen, D.Y.; Zhuang, X.Z.; Gan, Z.Y.; Cen, K.H.; Ba, Y.P.; Jia, D.X. Co-pyrolysis of light bio-oil leached bamboo and heavy bio-oil: Effects of mass ratio, pyrolysis temperature, and residence time on the biochar. Chem. Eng. J. 2022, 437, 135253. [Google Scholar] [CrossRef]
- Chen, D.Y.; Chen, F.; Cen, K.H.; Cao, X.B.; Zhang, J.; Zhou, J.B. Upgrading rice husk via oxidative torrefaction: Characterization of solid, liquid, gaseous products and a comparison with non-oxidative torrefaction. Fuel 2020, 275, 117936. [Google Scholar] [CrossRef]
- Wang, L.C.; Li, X.; Zhou, J.B.; Ma, H.H.; Wang, H.L. Tailoring texture properties in porous carbon for enhanced capacitive performance: Towards investigating the CO2 activation behaviors of carbonaceous matter. Colloid. Surf. A 2022, 652, 129810. [Google Scholar] [CrossRef]
- Chen, D.Y.; Cen, K.H.; Cao, X.B.; Chen, F.; Zhang, J.; Zhou, J.B. Insight into a new phenolic-leaching pretreatment on bamboo pyrolysis: Release characteristics of pyrolytic volatiles, upgradation of three phase products, migration of elements, and energy yield. Renew. Sust. Energy Rev. 2021, 136, 110444. [Google Scholar] [CrossRef]
- Singh, S.V.; Chaturvedi, S.; Dhyani, V.C.; Kasivelu, G. Pyrolysis temperature influences the characteristics of rice straw and husk biochar and sorption/desorption behaviour of their biourea composite. Bioresour. Technol. 2020, 314, 123674. [Google Scholar] [CrossRef]
- Almeida, L.S.; Oreste, E.Q.; Maciel, J.V.; Heinemann, M.G.; Dias, D. Electrochemical devices obtained from biochar: Advances in renewable and environmentally-friendly technologies applied to analytical chemistry. Trends Environ. Anal. 2020, 26, e00089. [Google Scholar] [CrossRef]
- Cen, K.H.; Zhang, J.; Ma, Z.Q.; Chen, D.Y.; Zhou, J.B.; Ma, H.H. Investigation of the relevance between biomass pyrolysis polygeneration and washing pretreatment under different severities: Water, dilute acid solution and aqueous phase bio-oil. Bioresour. Technol. 2019, 278, 26–33. [Google Scholar] [CrossRef]
- Wang, L.C.; Xie, L.E.; Feng, X.; Li, X.; Ma, H.H.; Han, G.L.; Yang, D.R.; Zhou, J.B. Optimization process of porous carbon derived from coconut shell: A novel preparation condition and investigation on pore surface roughness. Diam. Relat. Mater. 2022, 123, 108854. [Google Scholar] [CrossRef]
- Zhang, H.F.; He, X.J.; Wei, F.; Dong, S.A.; Xiao, N.; Qiu, J.S. Moss-covered rock-like hybrid porous carbons with enhanced electrochemical properties. ACS Sustain. Chem. 2020, 8, 3065–3071. [Google Scholar] [CrossRef]
- Wang, Q.; Yan, J.; Fan, Z.J. Carbon materials for high volumetric performance supercapacitors: Design, progress, challenges and opportunities. Energy Environ. Sci. 2016, 9, 729–762. [Google Scholar] [CrossRef]
- Wang, L.C.; Wu, J.L.; Gao, Y.; Sha, L.K.; Ma, H.H.; Li, X.; Zhou, J.B. H3PO4-assisted preparation of precursor-derived porous carbon: Construction of precursor/precursor-derived porous carbon texture properties relationship. Diam. Relat. Mater. 2021, 119, 108596. [Google Scholar] [CrossRef]
- Wang, L.C.; Feng, X.; Li, X.; Wang, H.L.; Wu, J.L.; Ma, H.H.; Zhou, J.B. Hydrothermal, KOH-assisted synthesis of lignin-derived porous carbon for supercapacitors: Value-added of lignin and constructing texture properties/specific capacitance relationships. J. Mater. Res. Technol. 2022, 16, 570–580. [Google Scholar] [CrossRef]
- Li, B.W.; Hu, J.C.; Xiong, H.; Xiao, Y. Application and properties of microporous carbons activated by ZnCl2: Adsorption behavior and activation mechanism. ACS Omega 2020, 5, 9398–9407. [Google Scholar] [CrossRef]
- Guo, S.S.; Chen, Y.X.; Shi, L.L.; Dong, Y.; Ma, J.; Chen, X.H.; Song, H.H. Nitrogen-doped biomass-based ultra-thin carbon nanosheets with interconnected framework for high-performance lithium-ion batteries. Appl. Surf. Sci. 2018, 437, 136–143. [Google Scholar] [CrossRef]
- Zhang, M.D.; Yu, C.; Li, Z.; Yu, J.H.; Li, S.F.; Zhao, C.T.; Huang, H.L.; Qiu, J.S. A recyclable route to produce biochar with a tailored structure and surface chemistry for enhanced charge storage. Green Chem. 2019, 21, 2095–2103. [Google Scholar] [CrossRef]
- Li, S.S.; Wang, C.; Luo, Z.J.; Zhu, X.F. Investigation on the catalytic behavior of alkali metals and alkaline earth metals on the biomass pyrolysis assisted with real-time monitoring. Energy Fuels 2020, 34, 12654–12664. [Google Scholar] [CrossRef]
- Mahadevan, R.; Adhikari, S.; Shakya, R.; Wang, K.; Dayton, D.; Lehrich, M.; Taylor, S.E. Effect of alkali and alkaline earth metals on in-situ catalytic fast pyrolysis of lignocellulosic biomass: A microreactor study. Energy Fuels 2016, 30, 3045–3056. [Google Scholar] [CrossRef]
- Zhang, X.H.; Li, H.X.; Zhang, K.; Wang, Q.; Qin, B.; Cao, Q.; Jin, L. Strategy for preparing porous graphitic carbon for supercapacitor: Balance on porous structure and graphitization degree. J. Electrochem. Soc. 2018, 165, A2084–A2092. [Google Scholar] [CrossRef]
- Zhang, X.H.; Li, H.X.; Qin, B.; Wang, Q.; Xing, X.H.; Yang, D.H.; Jin, L.; Cao, Q. Direct synthesis of porous graphitic carbon sheets grafted on carbon fibers for high-performance supercapacitors. J. Mater. Chem. A 2019, 7, 3298. [Google Scholar] [CrossRef]
- Xie, J.; Tong, L.; Su, L.W.; Xu, Y.W.; Wang, L.B.; Wang, Y.H. Good electronic conductivity provides a low resistance channel for electron transport. J. Power. Sources 2017, 342, 529–536. [Google Scholar] [CrossRef]
- Li, M.; He, J.J. Effect of stress state on graphitization behavior of diamond under high pressure and high temperature. Diam. Relat. Mater. 2021, 116, 108368. [Google Scholar] [CrossRef]
- Yoon, S.B.; Chai, G.S.; Kang, S.K.; Yu, J.S.; Gierszal, K.P.; Jaroniec, M. Graphitized pitch-based carbons with ordered nanopores synthesized by using colloidal crystals as templates. J. Am. Chem. Soc. 2005, 127, 4188–4189. [Google Scholar] [CrossRef]
- Krivoruchko, O.P.; Maksimova, N.I.; Zaikovskii, V.I.; Salanov, A.N. Study of multiwalled graphite nanotubes and filaments formation from carbonized products of polyvinyl alcohol via catalytic graphitization at 600–800 °C in nitrogen atmosphere. Carbon 2000, 38, 1075–1082. [Google Scholar] [CrossRef]
- Sevilla, M.; Sanchis, C.; Valdés-Solís, T.; Morallón, E.; Fuertes, A.B. Synthesis of graphitic carbon nanostructures from sawdust and their application as electrocatalyst supports. J. Phys. Chem. C 2007, 111, 9749–9756. [Google Scholar] [CrossRef]
- Oya, A.; Yoshida, S.; Alcaniz-Monge, J.; Linares-Solano, A. Formation of mesopores in phenolic resin-derived carbon fiber by catalytic activation using cobalt. Carbon 1995, 33, 1085–1090. [Google Scholar] [CrossRef]
- Uzoejinwa, B.B.; He, X.H.; Wang, S.; Abomohra, A.E.; Hu, Y.M.; He, Z.X.; Wang, Q. Co-pyrolysis of macroalgae and lignocellulosic biomass. J. Therm. Anal. Calorim. 2019, 136, 2001–2016. [Google Scholar] [CrossRef]
- Singh, S.; Prasad, C.J.; Kumar, M.M. Intrinsic kinetics, thermodynamic parameters and reaction mechanism of non-isothermal degradation of torrefied Acacia nilotica using isoconversional methods. Fuel 2020, 259, 116263. [Google Scholar] [CrossRef]
- Zhang, Y.M.; Wang, L.C.; Wu, Y.S.; Chen, Y.; Ma, H.H.; Zhou, H.; Zhu, Z.X.; Liu, S.H.; Zhou, J.B. Pyrolysis characteristics, kinetics, and its product characteristics of grape stem. Bioresources 2019, 14, 7901–7919. [Google Scholar] [CrossRef]
- Tabal, A.; Barakat, A.; Aboulkas, A.; Harfi, K.E. Pyrolysis of ficus nitida wood: Determination of kinetic and thermodynamic parameters. Fuel 2021, 283, 119253. [Google Scholar] [CrossRef]
- Wang, L.C.; Wu, J.L.; Ma, H.H.; Han, G.L.; Yang, D.R.; Chen, Y.; Zhou, J.B. H3PO4-assisted synthesis of apricot shell lignin-based activated carbon for capacitors: Understanding the pore structure/electrochemical performance relationship. Energy Fuel 2021, 35, 8303–8312. [Google Scholar] [CrossRef]
- Chen, D.Y.; Zhou, J.B.; Zhang, Q.S. Effects of torrefaction on the pyrolysis behavior and Bio-oil properties of rice husk by using TG-FTIR and Py-GC/MS. Energy Fuel 2014, 28, 5857–5863. [Google Scholar] [CrossRef]
- Chen, D.Y.; Wang, Y.; Liu, Y.X.; Cen, K.H.; Cao, X.B.; Ma, Z.Q.; Li, Y.J. Comparative study on the pyrolysis behaviors of rice straw under different washing pretreatments of water, acid solution, and aqueous phase bio-oil by using TG-FTIR and PY-GC/MS. Fuel 2019, 252, 1–9. [Google Scholar] [CrossRef]
- Kumar, A.; Wang, L.; Dzenis, Y.A.; Jones, D.D.; Hanna, M.A. Thermogravimetric characterization of corn stover as gasification and pyrolysis feedstock. Biomass Bioenerg. 2008, 32, 460–467. [Google Scholar] [CrossRef]
- Azargohar, R.; Nanda, S.; Kozinski, J.A.; Dalai, K.A. Effects of temperature on the physicochemical characteristics of fast pyrolysis bio-chars derived from Canadian waste biomass. Fuel 2014, 125, 90–100. [Google Scholar] [CrossRef]
- Xue, B.C.; Wang, Z.C.; Zhu, Y.C.; Wang, X.F.; Xiao, R. Sustainable and recyclable synthesis of porous carbon sheets from rice husks for energy storage: A strategy of comprehensive utilization. Ind. Crop. Prod. 2021, 170, 113724. [Google Scholar] [CrossRef]
- Jones, F.; Tran, H.; Lindberg, D.; Zhao, L.M.; Hupa, M. Thermal stability of Zinc compounds. Energy Fuels 2013, 27, 5663–5669. [Google Scholar] [CrossRef]
- Vyazovkin, S.; Burnham, A.K.; Favergeon, L.; Koga, N.; Moukhina, E.; Pérez-Maqueda, L.A.; Sbirrazzuoli, N. ICTAC kinetics committee recommendations for analysis of multi-step kinetics. Thermochim. Acta 2020, 689, 178597. [Google Scholar] [CrossRef]
- López-González, D.; Fernandez-Lopez, M.; Valverde, J.L.; Sanchez-Silva, L. Thermogravimetric-mass spectrometric analysis on combustion of lignocellulosic biomass. Bioresour. Technol. 2013, 143, 562–574. [Google Scholar] [CrossRef]
- Lah, B.; Klinar, D.; Likozar, B. Pyrolysis of natural, butadiene, styrene-butadiene rubber and tyre components: Modelling kinetics and transport phenomena at different heating rates and formulations. Chem. Eng. Sci. 2013, 87, 1–13. [Google Scholar] [CrossRef]
- Mallick, D.; Kumar, P.M.; Pinakeswar, M.; Vijayanand, S.M. Discernment of synergism in pyrolysis of biomass blends using thermogravimetric analysis. Bioresour. Technol. 2018, 261, 294–305. [Google Scholar] [CrossRef]
- Guo, D.B.; Hu, M.; Chen, Z.H.; Cui, B.H.; Zhang, Q.; Liu, Y.H.; Luo, S.Y.; Ruan, R.; Liu, Y. Catalytic pyrolysis of rain tree biomass with nano nickel oxide synthetized from nickel plating slag: A green path for treating waste by waste. Bioresour. Technol. 2020, 315, 123831. [Google Scholar] [CrossRef] [PubMed]
- Nisar, J.; Rahman, A.; Ali, G.; Shah, A.; Farooqi, Z.; Ahmad, I.; Iqbal, M.; Rehman, N.U. Pyrolysis of almond shells waste: Effect of zinc oxide on kinetics and product distribution. Biomass Convers. Bior. 2022, 12, 2583–2595. [Google Scholar] [CrossRef]
- Nisar, J.; Ali, G.; Shah, A.; Iqbal, M.; Khan, R.; Uddin, S.; Anwar, F.; Ullah, R.; Akhter, M.S. Fuel production from waste polystyrene via pyrolysis: Kinetics and products distribution. Waste Manag. 2019, 88, 236–247. [Google Scholar] [CrossRef]
- Nisar, J.; Ali, F.; Malana, M.A.; Ali, G.; Iqbal, M.; Shah, A.; Ahmad, I.; Khan, T.A.; Rashid, U. Kinetics of the pyrolysis of cobalt-impregnated sesame stalk biomass. Biomass Convers. Bior. 2020, 10, 1179–18710. [Google Scholar] [CrossRef]
- Ma, H.H.; Zhang, Y.M.; Wang, L.C.; Zhu, Z.X.; Chen, Y.; Wang, H.L.; Deng, C.J.; Zhou, J.B. Kinetic analysis of the pyrolysis of apricot stone and its main components via distributed activation energy mode. Bioresources 2020, 15, 1187–1204. [Google Scholar] [CrossRef]
- Yang, H.P.; Yan, R.; Chin, T.; Liang, D.T.; Chen, H.P.; Zheng, C.G. Thermogravimetric analysis-fourier transform infrared analysis of palm oil waste pyrolysis. Energy Fuel 2004, 18, 1814–1821. [Google Scholar] [CrossRef]
- Yao, F.; Wu, Q.L.; Lei, Y.; Guo, W.H.; Xu, Y.J. Thermal decomposition kinetics of natural fibers: Activation energy with dynamic thermogravimetric analysis. Polym. Degrad. Stabll. 2008, 93, 90–98. [Google Scholar] [CrossRef]
- Wang, L.C.; Xie, L.E.; Ma, H.H.; Zhou, J.B. Co-pyrolysis of pine sawdust with nickel formate for understanding interaction mechanisms and enhancing resistance toward biochar deposition. J. Mater. Res. Technol. 2022, 18, 3751–3763. [Google Scholar] [CrossRef]
- Min, F.F.; Zhang, M.X.; Chen, Q.R. Non-isothermal kinetics of pyrolysis of three kinds of fresh biomass. J. China Univ. Min. Technol. 2007, 17, 105–111. [Google Scholar] [CrossRef]
- Dhyani, V.; Kumar, J.; Bhaskar, T. Thermal decomposition kinetics of sorghum straw via thermogravimetric analysis. Bioresour. Technol. 2017, 245, 1122–1129. [Google Scholar] [CrossRef] [PubMed]
- Mishra, G.; Kumar, J.; Bhaskar, T. Kinetic studies on the pyrolysis of pinewood. Bioresour. Technol. 2015, 182, 282–288. [Google Scholar] [CrossRef] [PubMed]
- Khawam, A.; Flanagan, D.R. Solid-state kinetic models: Basics and mathematical fundamentals. J. Phys. Chem. B 2006, 110, 17315–17328. [Google Scholar] [CrossRef]
- Vlaev, L.; Georgieva, V.; Genieva, S. Products and kinetics of non-isothermal decomposition of vanadium oxide compounds. J. Therm. Anal. Calorim. 2007, 88, 805–812. [Google Scholar] [CrossRef]
- Xu, Y.L.; Chen, B.L. Investigation of thermodynamic parameters in the pyrolysis conversion of biomass and manure to biochars using thermogravimetric analysis. Bioresour. Technol. 2013, 146, 485–493. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, M.S.; Mehmood, M.A.; Al-Ayed, O.S.; Ye, G.B.; Imededdine, N.; Qadir, G. Kinetic analyses and pyrolytic behavior of para grass for its bioenergy potential. Bioresour. Technol. 2017, 224, 708–713. [Google Scholar] [CrossRef]
- Huang, L.M.; Liu, J.Y.; He, Y.; Sun, S.Y.; Chen, J.C.; Sun, J.; Chang, K.L.; Kuo, J.H.; Ning, X.A. Thermodynamics and kinetics parameters of co-combustion between sewage sludge and water hyacinth in CO2/O2 atmosphere as biomass to solid biofuel. Bioresour. Technol. 2016, 218, 631–642. [Google Scholar] [CrossRef]
- Chen, L.; Wang, F.; Tian, Z.W.; Guo, H.T.; Cai, C.Y.; Wu, Q.J.; Du, H.J.; Liu, K.M.; Hao, Z.F.; He, S.J.; et al. Wood-derived high-mass-loading MnO2 composite carbon electrode enabling high energy density and high-rate supercapacitor. Small 2022, 18, 2201307. [Google Scholar] [CrossRef] [PubMed]
- Du, Y.F.; Sun, G.H.; Li, Y.; Cheng, J.Y.; Chen, J.P.; Song, G.; Kong, Q.Q.; Xie, L.J.; Chen, C.M. Pre-oxidation of lignin precursors for hard carbon anode with boosted lithium-ion storage capacity. Carbon 2021, 178, 243–255. [Google Scholar] [CrossRef]
- Lin, X.Y.; Liu, Y.Z.; Tan, H.; Zhang, B. Advanced lignin-derived hard carbon for Na-ion batteries and a comparison with Li and K ion storage. Carbon 2020, 157, 316–323. [Google Scholar] [CrossRef]
- Zou, L.Y.; Zhong, G.Y.; Nie, Y.J.; Tan, Z.X.; Liao, W.B.; Fu, X.B.; Pan, Z.C. Porous carbon nanosheets derived from ZIF-8 treated with KCl as highly efficient electrocatalysts for the oxygen reduction reaction. Energy Technol. 2021, 9, 210003. [Google Scholar] [CrossRef]
- Zhao, J.H.; He, X.X.; Lai, W.H.; Yang, Z.; Liu, X.H.; Li, L.; Qiao, Y.; Xiao, Y.; Li, L.; Wu, X.Q.; et al. Catalytic defect-repairing using manganese ions for hard carbon anode with high-capacity and high-initial-coulombic-efficiency in sodium-ion batteries. Adv. Energy Mater. 2023, 2300444. [Google Scholar] [CrossRef]
- Wang, M.L.; Zhang, T.Y.; Cui, M.Z.; Liu, W.F.; Liu, X.G.; Zhao, J.W.; Zhou, J.D. Sub-nanopores-containing N,O-codoped porous carbon from molecular-scale networked polymer hydrogel for solid-state supercapacitor. Chin. Chem. Lett. 2020, 32, 1111–1116. [Google Scholar] [CrossRef]
- Zhou, Y.; Jia, Z.X.; Shi, L.L.; Wu, Z.; Jie, B.Y.; Zhao, S.Y.; Wei, L.Y.; Zhou, A.G.; Zhu, J.W.; Wang, X.; et al. Pressure difference-induced synthesis of P-doped carbon nanobowls for high-performance supercapacitors. Chem. Eng. J. 2020, 385, 123858. [Google Scholar] [CrossRef]




| Conversion α | PS | PSKCl-21 | PSKCl-11 | PSKCl-12 | ||||
|---|---|---|---|---|---|---|---|---|
| FWO E (kJ/mol) | KAS E (kJ/mol) | FWO E (kJ/mol) | KAS E (kJ/mol) | FWO E (kJ/mol) | KAS E (kJ/mol) | FWO E (kJ/mol) | KAS E (kJ/mol) | |
| 0.1 | 152.52 | 149.81 | 265.27 | 262.03 | 311.86 | 320.55 | 501.38 | 534.96 |
| 0.2 | 165.55 | 171.68 | 215.58 | 213.06 | 301.23 | 290.39 | 435.48 | 542.65 |
| 0.3 | 187.10 | 186.79 | 208.74 | 207.48 | 281.86 | 272.59 | 506.78 | 472.18 |
| 0.4 | 184.58 | 182.44 | 203.65 | 204.36 | 235.12 | 237.38 | 379.85 | 389.82 |
| 0.5 | 194.97 | 194.61 | 193.57 | 189.97 | 215.34 | 216.39 | 363.42 | 335.48 |
| 0.6 | 216.37 | 195.00 | 184.51 | 183.87 | 210.02 | 200.25 | 347.79 | 355.70 |
| 0.7 | 193.28 | 192.50 | 177.82 | 176.66 | 241.73 | 243.86 | 413.86 | 424.96 |
| 0.8 | 185.18 | 183.76 | 133.73 | 130.97 | 227.65 | 247.45 | 427.12 | 438.52 |
| 0.9 | 394.61 | 553.18 | 108.32 | 81.94 | 92.16 | 132.35 | n.d | n.d |
| Model | Integral form G (α) | ||
|---|---|---|---|
| Nucleation models | |||
| Power law | P2 | 2α1/2 | α1/2 |
| Power law | P3 | 3α2/3 | α1/3 |
| Power law | P4 | 4α3/4 | α1/4 |
| Avarami-Erofeyev | A2 | 2(1 − α)[−ln(1 − α)]1/2 | [−ln(1 − α)]1/2 |
| Avarami-Erofeyev | A3 | 3(1 − α)[−ln(1 − α)]2/3 | [−ln(1 − α)]1/3 |
| Avarami-Erofeyev | A4 | 4(1 − α)[−ln(1 − α)]3/4 | [−ln(1 − α)]1/4 |
| Geometrical contraction models | |||
| Contracting area | R2 | 2(1 − α)1/2 | [1 − (1 − α)1/2] |
| Contracting volume | R3 | 3(1 − α)2/3 | [1 − (1 − α)1/3] |
| Diffusion models | |||
| 1-D diffusion | D1 | 1/2α | α2 |
| 2-D diffusion | D2 | [−ln(1 − α)]−1 | [(1 − α)ln(1 − α)] + α |
| 3-D diffusion, Jander | D3 | 3(1 − α)2/3/[2(1 − (1 − α)1/3)] | [1 − (1 − α)1/3]2 |
| Ginstling-Brounshtein | D4 | 3/[2((1 − α)−1/3 − 1)] | 1 − (2α/3) − (1 − α)2/3 |
| Reaction-order models | |||
| First-order | F1 | (1 − α) | −ln(1 − α) |
| Second-order | F2 | (1 − α)2 | (1 − α)−1 − 1 |
| Third-order | F3 | (1 − α)3 | [(1 − α)−2 − 1]/2 |
| Fifth-order | F5 | (1 − α)5 | [(1 − α)−4 − 1]/4 |
| Conversion α | A (s−1) | |||
|---|---|---|---|---|
| PS | PSKCl-21 | PSKCl-11 | PSKCl-12 | |
| 0.1 | 3.49 × 1010 | 2.90 × 1021 | 2.14 × 1025 | 3.65 × 1044 |
| 0.2 | 4.58 × 1011 | 1.11 × 1017 | 2.46 ×1024 | 4.74 × 1038 |
| 0.3 | 2.96 × 1013 | 2.49 × 1016 | 4.79 × 1022 | 1.11 × 1045 |
| 0.4 | 1.80 × 1013 | 8.69 × 1015 | 3.48 × 1018 | 4.99 × 1033 |
| 0.5 | 1.38 × 1014 | 1.07 × 1015 | 6.08 × 1016 | 1.68 × 1032 |
| 0.6 | 9.08 × 1015 | 1.64 × 1014 | 2.05 × 1016 | 6.68 × 1030 |
| 0.7 | 9.93 × 1013 | 4.10 × 1013 | 1.34 × 1019 | 5.52 × 1036 |
| 0.8 | 2.03 × 1013 | 1.54 × 1013 | 7.54 × 1017 | 4.58 × 1047 |
| 0.9 | 9.54 × 1030 | 1.98 × 107 | 5.18 × 105 | n.d |
| Conversion α | PS | PSKCl-21 | PSKCl-11 | PSKCl-12 | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| ΔH | ΔG | ΔS | ΔH | ΔG | ΔS | ΔH | ΔG | ΔS | ΔH | ΔG | ΔS | |
| 0.1 | 148.30 | 183.91 | −5.65 × 10−2 | 260.87 | 424.86 | 15.44 × 10−2 | 307.42 | 139.07 | 26.11 × 10−2 | 496.99 | 145.39 | 59.51 × 10−2 |
| 0.2 | 161.12 | 183.48 | −3.55 × 10−2 | 211.40 | 372.14 | 6.96 × 10−2 | 296.56 | 139.24 | 24.33 × 10−2 | 430.89 | 146.08 | 48.20 × 10−2 |
| 0.3 | 182.18 | 182.85 | −0.11 × 10−2 | 204.03 | 362.90 | 5.69 × 10−2 | 277.05 | 139.57 | 21.15 × 10−2 | 502.03 | 145.33 | 60.37 × 10−2 |
| 0.4 | 179.53 | 182.92 | −0.54 × 10−2 | 198.84 | 356.38 | 4.80 × 10−2 | 230.21 | 140.48 | 13.48 × 10−2 | 375.00 | 146.75 | 38.63 × 10−2 |
| 0.5 | 189.82 | 182.64 | 1.14 × 10−2 | 188.68 | 345.20 | 3.05 × 10−2 | 210.36 | 140.92 | 10.22 × 10−2 | 358.48 | 146.97 | 35.80 × 10−2 |
| 0.6 | 211.14 | 182.09 | 4.61 × 10−2 | 179.54 | 335.24 | 1.47 × 10−2 | 204.98 | 141.04 | 9.33 × 10−2 | 342.76 | 147.18 | 33.10 × 10−2 |
| 0.7 | 187.98 | 182.68 | 0.84 × 10−2 | 172.77 | 327.79 | 0.31 × 10−2 | 236.59 | 140.34 | 14.52 × 10−2 | 408.70 | 146.33 | 44.40 × 10−2 |
| 0.8 | 179.77 | 182.91 | −0.50 × 10−2 | 167.81 | 322.41 | −0.55 × 10−2 | 222.35 | 140.64 | 12.18 × 10−2 | 530.62 | 145.06 | 65.25 × 10−2 |
| 0.9 | 388.79 | 178.94 | 33.27 × 10−2 | 101.88 | 257.04 | −12.02 × 10−2 | 86.14 | 145.16 | −10.48 × 10−2 | n.d | n.d | n.d |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, L.; Wang, L.; Zhou, J.; Ma, H. Co-Pyrolysis for Pine Sawdust with Potassium Chloride: Insight into Interactions and Assisting Biochar Graphitization. Materials 2023, 16, 3667. https://doi.org/10.3390/ma16103667
Xie L, Wang L, Zhou J, Ma H. Co-Pyrolysis for Pine Sawdust with Potassium Chloride: Insight into Interactions and Assisting Biochar Graphitization. Materials. 2023; 16(10):3667. https://doi.org/10.3390/ma16103667
Chicago/Turabian StyleXie, Linen, Liangcai Wang, Jianbin Zhou, and Huanhuan Ma. 2023. "Co-Pyrolysis for Pine Sawdust with Potassium Chloride: Insight into Interactions and Assisting Biochar Graphitization" Materials 16, no. 10: 3667. https://doi.org/10.3390/ma16103667
APA StyleXie, L., Wang, L., Zhou, J., & Ma, H. (2023). Co-Pyrolysis for Pine Sawdust with Potassium Chloride: Insight into Interactions and Assisting Biochar Graphitization. Materials, 16(10), 3667. https://doi.org/10.3390/ma16103667





