Wear Estimation of DLC Films Based on Energy-Dissipation Analysis: A Molecular Dynamics Study
(This article belongs to the Section Manufacturing Processes and Systems)
Abstract
:1. Introduction
2. Modeling
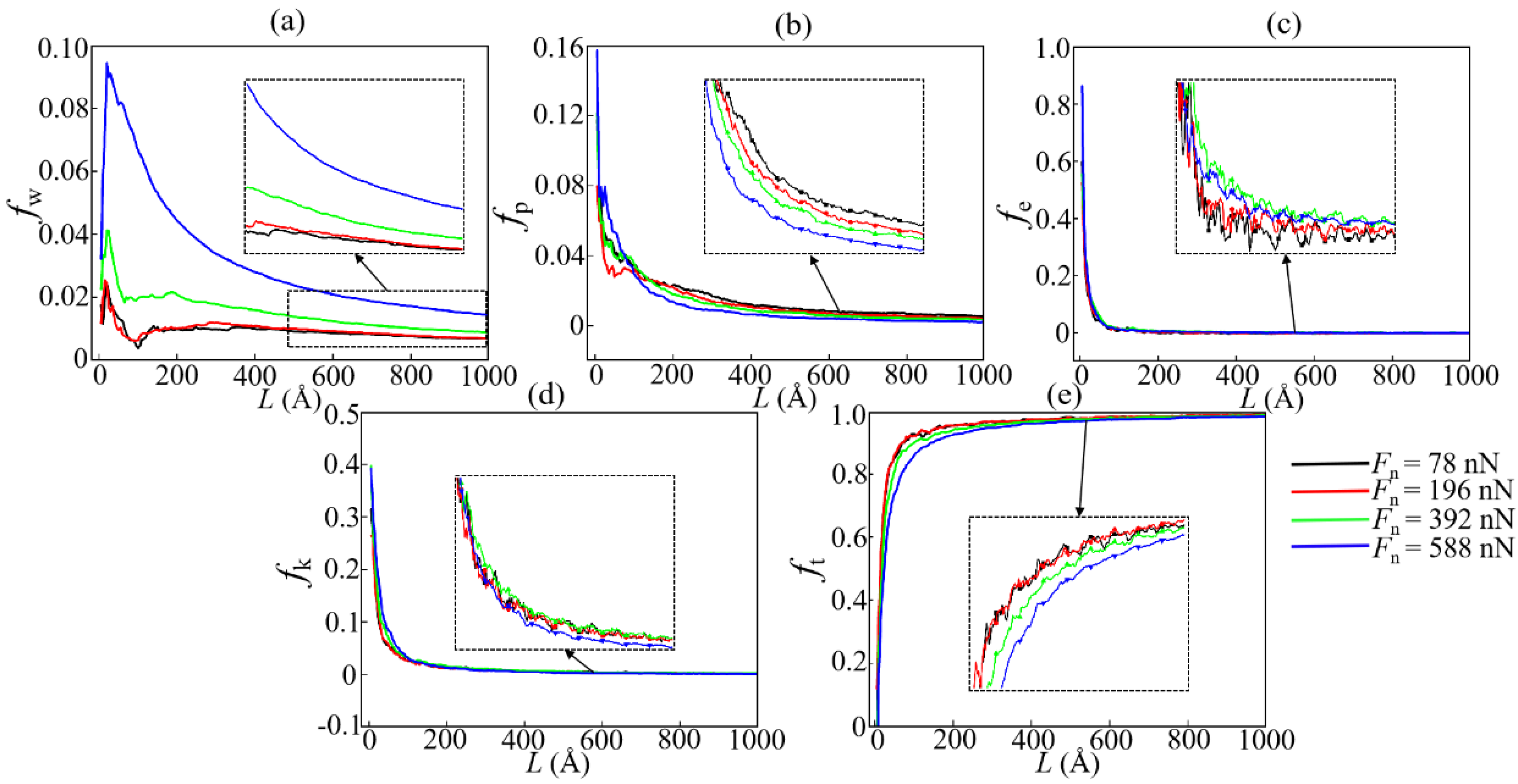
3. Results
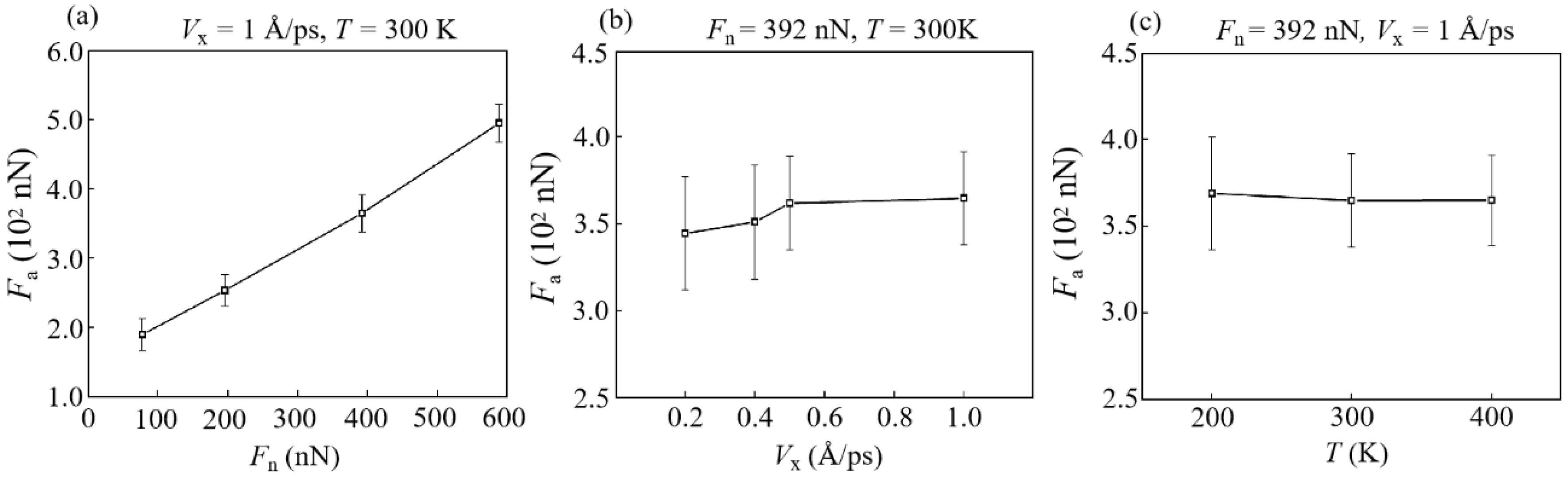
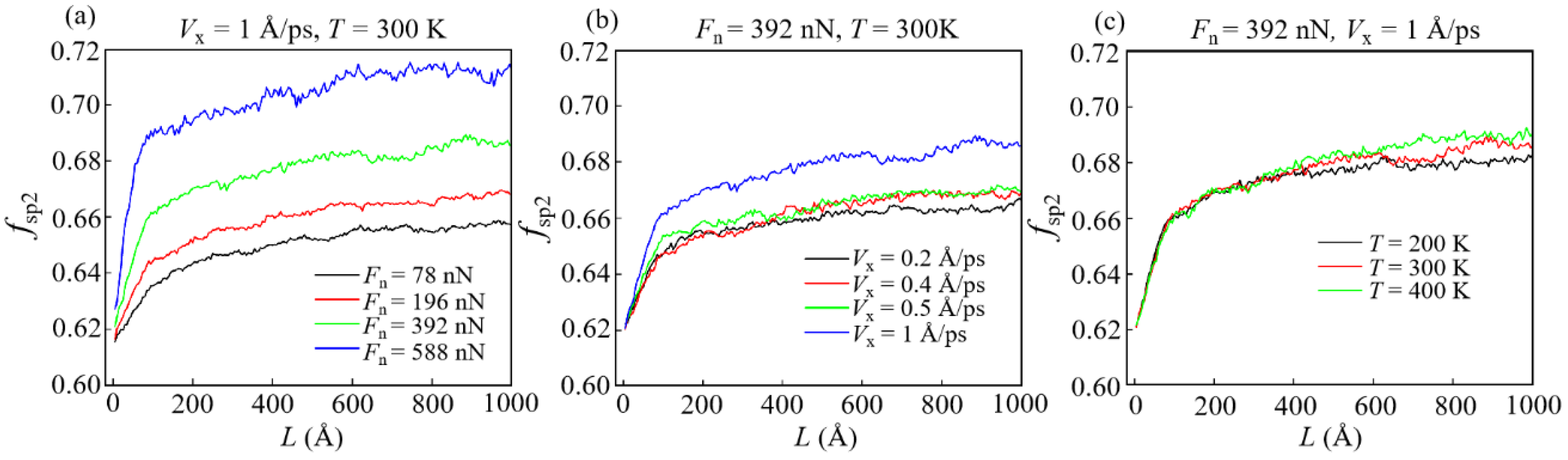
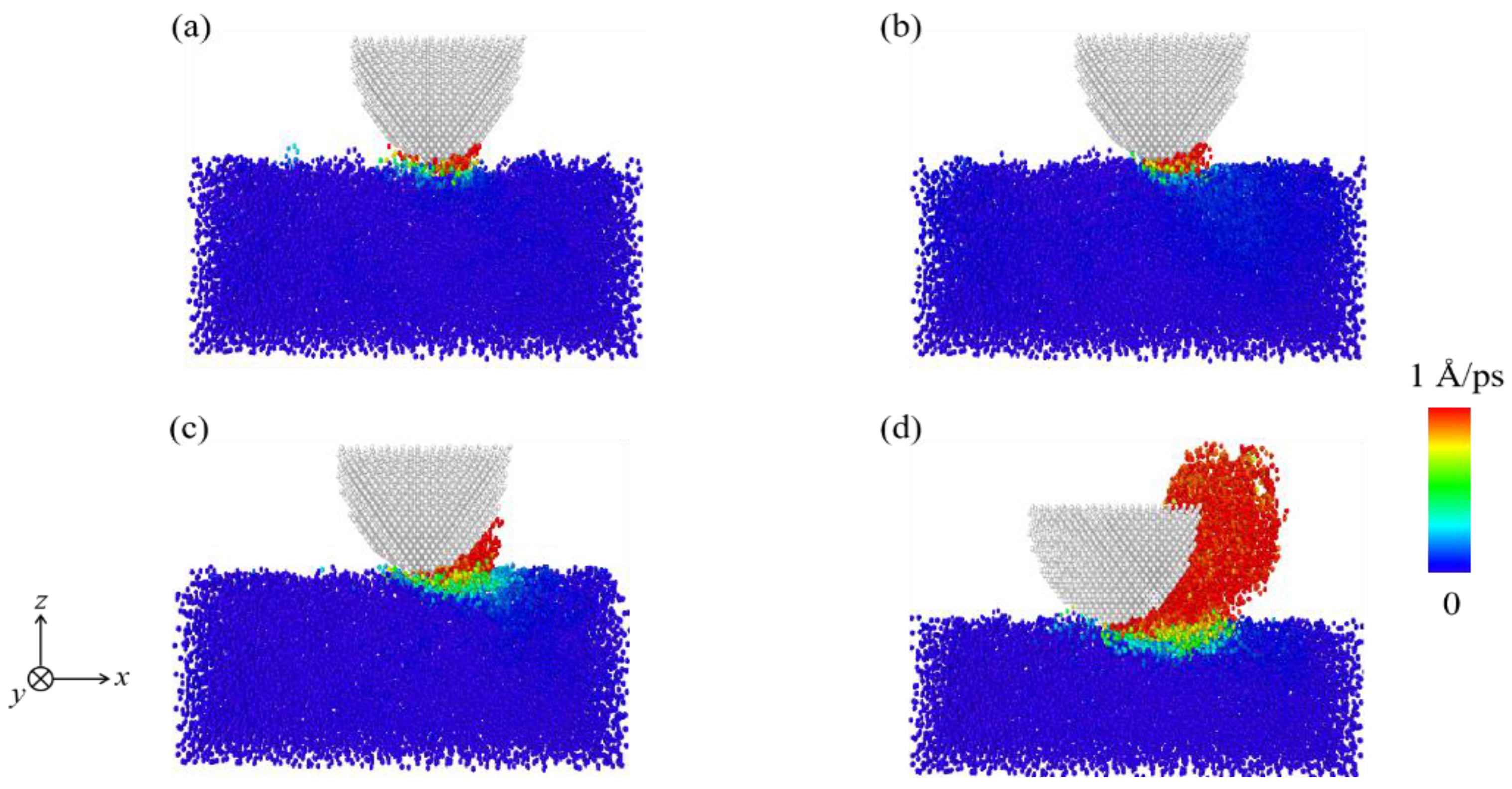
3.1. Friction Interface and Friction Behavior
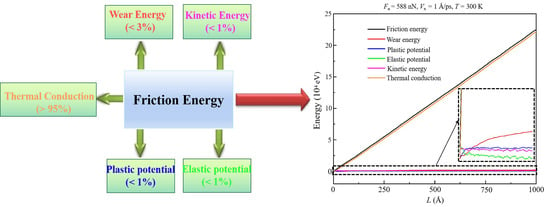
3.2. Analysis of Energy Forms
3.3. Analysis of Energy Dissipation
4. Discussions
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fouvry, S.; Kapsa, P. An energy description of hard coating wear mechanisms. Surf. Coat. Technol. 2001, 138, 141–148. [Google Scholar] [CrossRef]
- Fouvry, S.; Kapsa, P.; Zahouani, H.; Vincent, L. Wear analysis in fretting of hard coatings through a dissipated energy concept. Wear 1997, 203–204, 393–403. [Google Scholar] [CrossRef]
- Ramalho, A.; Miranda, J.C. The relationship between wear and dissipated energy in sliding systems. Wear 2006, 260, 361–367. [Google Scholar] [CrossRef] [Green Version]
- Fouvry, S.; Paulin, C.; Liskiewicz, T. Application of an energy wear approach to quantify fretting contact durability: Introduction of a wear energy capacity concept. Tribol. Int. 2007, 40, 1428–1440. [Google Scholar] [CrossRef]
- Fouvry, S.; Liskiewicz, T.; Kapsa, P.; Hannel, S.; Sauger, E. An energy description of wear mechanisms and its applications to oscillating sliding contacts. Wear 2003, 255, 287–298. [Google Scholar] [CrossRef] [Green Version]
- Lu, Z.; Wang, L.; Zhang, G.; Xue, Q. The Dependence of Energy Dissipation on the Elastic Energy Density of Friction Pairs in Hard Coating Films. Tribol. Lett. 2011, 41, 435–438. [Google Scholar] [CrossRef]
- Wang, L.; Bai, L.; Lu, Z.; Zhang, G.; Wu, Z. Influence of Load on the Tribological Behavior of a-C Films: Experiment and Calculation Coupling. Tribol. Lett. 2013, 52, 469–475. [Google Scholar] [CrossRef]
- Bai, L.; Zhang, G.; Lu, Z.; Wu, Z.G.; Wang, Y.; Wang, L.; Yan, P. Tribological mechanism of hydrogenated amorphous carbon film against pairs: A physical description. J. Appl. Phys. 2011, 110, 033521. [Google Scholar] [CrossRef]
- Mishra, T.; Nordin, B.; Svanbäck, D.; Tervakangas, S.; Prakash, B. The effects of contact configuration and coating morphology on the tribological behaviour of tetrahedral amorphous diamond-like carbon (ta-C DLC) coatings under boundary lubrication. Tribol. Mater. Surf. Interfaces 2019, 13, 120–129. [Google Scholar] [CrossRef]
- Jarry, O.; Jaoul, C.; Tristant, P.; Merle-Méjean, T.; Colas, M.; Dublanche-Tixier, C.; Ageorges, H.; Lory, C.; Jacquet, J.-M. Tribological Behaviour of Diamond-Like Carbon Films used in Automotive Application: A Comparison. Plasma Processes Polym. 2009, 6, S478–S482. [Google Scholar] [CrossRef]
- Weiss, M.; Elmer, F.-J. Dry friction in the Frenkel-Kontorova-Tomlinson model: Dynamical properties. Z. Phys. B Condens. Matter 1997, 104, 55–69. [Google Scholar] [CrossRef] [Green Version]
- Kuhlmann, D. On the Theory of Plastic Deformation. Proc. Phys. Soc. Sect. A 1951, 64, 140. [Google Scholar] [CrossRef]
- Matsuzawa, N.N.; Kishii, N. Theoretical Calculations of Coefficients of Friction between Weakly Interacting Surfaces. J. Phys. Chem. A 1997, 101, 10045–10052. [Google Scholar] [CrossRef]
- Peyrard, M.; Aubry, S. Critical behaviour at the transition by breaking of analyticity in the discrete Frenkel-Kontorova model. J. Phys. C Solid State Phys. 1983, 16, 1593–1608. [Google Scholar] [CrossRef]
- Tabor, D. The Role of Surface and Intermolecular Forces in Thin Film Lubrication. In Tribology Series; Georges, J.M., Ed.; Elsevier: Amsterdam, The Netherlands, 1981; Volume 7, pp. 651–682. [Google Scholar]
- Homola, A.M.; Israelachvili, J.N.; Gee, M.L.; McGuiggan, P.M. Measurements of and Relation Between the Adhesion and Friction of Two Surfaces Separated by Molecularly Thin Liquid Films. J. Tribol. 1989, 111, 675–682. [Google Scholar] [CrossRef]
- Al Mahmud, K.A.H.; Kalam, M.A.; Masjuki, H.H.; Mobarak, H.M.; Zulkifli, N.W.M. An updated overview of diamond-like carbon coating in tribology. Crit. Rev. Solid State Mater. Sci. 2015, 40, 90–118. [Google Scholar] [CrossRef] [Green Version]
- Bai, L.; Narasimalu, S.; Wu, H.; Liu, F.; Liu, B.; Zhou, K. Effect of Environmental Hydrogen Atoms on the Tribological Behaviors of Diamond-like Carbon Films. Tribol. Int. 2016, 99, 258–266. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, J.; Zhang, J.; Chen, Q.; Ootani, Y.; Higuchi, Y.; Ozawa, N.; Martin, J.M.; Adachi, K.; Kubo, M. Tribochemical reactions and graphitization of diamond-like carbon against alumina give volcano-type temperature dependence of friction coefficients: A tight-binding quantum chemical molecular dynamics simulation. Carbon 2018, 133, 350–357. [Google Scholar] [CrossRef]
- Liu, Y.A.N.; Meletis, E.I. Evidence of graphitization of diamond-like carbon films during sliding wear. J. Mater. Sci. 1997, 32, 3491–3495. [Google Scholar] [CrossRef]
- Jacobs, T.D.B.; Carpick, R.W. Nanoscale wear as a stress-assisted chemical reaction. Nat. Nanotechnol. 2013, 8, 108–112. [Google Scholar] [CrossRef] [PubMed]
- Vahdat, V.; Grierson, D.S.; Turner, K.T.; Carpick, R.W. Mechanics of Interaction and Atomic-Scale Wear of Amplitude Modulation Atomic Force Microscopy Probes. ACS Nano 2013, 7, 3221–3235. [Google Scholar] [CrossRef] [PubMed]
- Sha, Z.-D.; Sorkin, V.; Branicio, P.S.; Pei, Q.-X.; Zhang, Y.-W.; Srolovitz, D.J. Large-scale molecular dynamics simulations of wear in diamond-like carbon at the nanoscale. Appl. Phys. Lett. 2013, 103, 073118. [Google Scholar] [CrossRef]
- Yin, Z.; Yu, Y.; Chen, H.; Li, J.; Bai, L. Nanofriction behaviors between silicon-doped diamond-like carbon films under different testing conditions. Comput. Mater. Sci. 2021, 188, 110182. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Sha, Z.D.; Branicio, P.S.; Pei, Q.X.; Sorkin, V.; Zhang, Y.W. A modified Tersoff potential for pure and hydrogenated diamond-like carbon. Comput. Mater. Sci. 2013, 67, 146–150. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2009, 18, 015012. [Google Scholar] [CrossRef]
- Chen, Y.-N.; Ma, T.-B.; Chen, Z.; Hu, Y.-Z.; Wang, H. Combined Effects of Structural Transformation and Hydrogen Passivation on the Frictional Behaviors of Hydrogenated Amorphous Carbon Films. J. Phys. Chem. C 2015, 119, 16148–16155. [Google Scholar] [CrossRef]
- Zhong, J.; Shakiba, R.; Adams, J.B. Molecular dynamics simulation of severe adhesive wear on a rough aluminum substrate. J. Phys. D Appl. Phys. 2013, 46, 055307. [Google Scholar] [CrossRef]
- Hu, X.; Martini, A. Atomistic simulation of the effect of roughness on nanoscale wear. Comput. Mater. Sci. 2015, 102, 208–212. [Google Scholar] [CrossRef]
- Erhart, P.; Albe, K. Analytical potential for atomistic simulations of silicon, carbon, and silicon carbide. Phys. Rev. B 2005, 71, 035211. [Google Scholar] [CrossRef] [Green Version]
- Rymuza, Z. Energy concept of the coefficient of friction. Wear 1996, 199, 187–196. [Google Scholar] [CrossRef]
- Hu, Y.-z.; Ma, T.-b.; Wang, H. Energy dissipation in atomic-scale friction. Friction 2013, 1, 24–40. [Google Scholar] [CrossRef] [Green Version]
- Archard, J.F. Microscopic aspects of adhesion and lubrication. Tribol. Int. 1982, 15, 242. [Google Scholar] [CrossRef]
- Bai, L.; Srikanth, N.; Korznikova, E.A.; Baimova, J.A.; Dmitriev, S.V.; Zhou, K. Wear and friction between smooth or rough diamond-like carbon films and diamond tips. Wear 2017, 372–373, 12–20. [Google Scholar] [CrossRef]
- Mo, Y.; Turner, K.T.; Szlufarska, I. Friction laws at the nanoscale. Nature 2009, 457, 1116–1119. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.-J.; Kim, D.-E. Nano-scale friction: A review. Int. J. Precis. Eng. Manuf. 2009, 10, 141–151. [Google Scholar] [CrossRef]
- Xu, S.; Wan, Q.; Sha, Z.; Liu, Z. Molecular dynamics simulations of nano-indentation and wear of the γTi-Al alloy. Comput. Mater. Sci. 2015, 110, 247–253. [Google Scholar] [CrossRef]
- Chattoraj, J.; Caroli, C.; Lemaître, A. Universal Additive Effect of Temperature on the Rheology of Amorphous Solids. Phys. Rev. Lett. 2010, 105, 266001. [Google Scholar] [CrossRef] [Green Version]
- Erdemir, A.; Donnet, C. Tribology of diamond-like carbon films: Recent progress and future prospects. J. Phys. D Appl. Phys. 2006, 39, R311–R327. [Google Scholar] [CrossRef]
- Liu, Y.; Erdemir, A.; Meletis, E.I. A study of the wear mechanism of diamond-like carbon films. Surf. Coat. Technol. 1996, 82, 48–56. [Google Scholar] [CrossRef]
- Ma, T.-B.; Hu, Y.-Z.; Xu, L.; Wang, L.-F.; Wang, H. Shear-induced lamellar ordering and interfacial sliding in amorphous carbon films: A superlow friction regime. Chem. Phys. Lett. 2011, 514, 325–329. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Kleinsorge, B.; Morrison, N.; Hart, A.; Stolojan, V.; Robertson, J. Stress Reduction and Bond Stability During Thermal Annealing of Tetrahedral Amorphous Carbon. J. Appl. Phys. 1999, 85, 7191–7197. [Google Scholar] [CrossRef]
- Smerdova, O.; Mazuyer, D.; Cayer-Barrioz, J. Links between energy dissipation and wear mechanisms in solid epoxy/epoxy sliding contact. Tribol. Int. 2014, 77, 148–159. [Google Scholar] [CrossRef]
- Ma, X.G.; Komvopoulos, K.; Wan, D.; Bogy, D.B.; Kim, Y.S. Effects of film thickness and contact load on nanotribological properties of sputtered amorphous carbon thin films. Wear 2003, 254, 1010–1018. [Google Scholar] [CrossRef]
- Zhang, H.S.; Endrino, J.L.; Anders, A. Comparative surface and nano-tribological characteristics of nanocomposite diamond-like carbon thin films doped by silver. Appl. Surf. Sci. 2008, 255, 2551–2556. [Google Scholar] [CrossRef] [Green Version]
- Yoon, E.-S.; Singh, R.A.; Oh, H.-J.; Kong, H. The effect of contact area on nano/micro-scale friction. Wear 2005, 259, 1424–1431. [Google Scholar] [CrossRef]
- Yang, Y.; Huang, L.; Shi, Y. Adhesion suppresses atomic wear in single-asperity sliding. Wear 2016, 352–353, 31–41. [Google Scholar] [CrossRef] [Green Version]
- Bowden, F.P.; Tabor, D. The friction and lubrication of solids. J. Chem. Educ. 1951, 28, 230. [Google Scholar] [CrossRef]
- Collins, I.F. A systematic procedure for constructing critical state models in three dimensions. Int. J. Solids Struct. 2003, 40, 4379–4397. [Google Scholar] [CrossRef]
- Collins, I.; Kelly, P. A thermomechanical analysis of a family of soil models. Geotechnique 2002, 52, 507–518. [Google Scholar] [CrossRef]
- Yang, H.; Sinha, S.K.; Feng, Y.; McCallen, D.B.; Jeremić, B. Energy dissipation analysis of elastic–plastic materials. Comput. Methods Appl. Mech. Eng. 2018, 331, 309–326. [Google Scholar] [CrossRef] [Green Version]
- Jahangiri, M.; Hashempour, M.; Razavizadeh, H.; Rezaie, H.R. A new method to investigate the sliding wear behaviour of materials based on energy dissipation: W–25wt%Cu composite. Wear 2012, 274–275, 175–182. [Google Scholar] [CrossRef]
- Singer, I.L. Friction and Energy Dissipation at the Atomic Scale—A Review. In Tribology Series; Dowson, D., Taylor, C.M., Childs, T.H.C., Godett, M., Dalmaz, G., Eds.; Elsevier: Amsterdam, The Netherlands, 1994; Volume 27, pp. 3–20. [Google Scholar]
- Uetz, H.; Föhl, J. Wear as an energy transformation process. Wear 1978, 49, 253–264. [Google Scholar] [CrossRef]
- Konca, E.; Cheng, Y.T.; Weiner, A.M.; Dasch, J.M.; Alpas, A.T. Vacuum tribological behavior of the non-hydrogenated diamond-like carbon coatings against aluminum: Effect of running-in in ambient air. Wear 2005, 259, 795–799. [Google Scholar] [CrossRef]
- Andersson, J.; Erck, R.; Erdemir, A. Frictional behavior of diamondlike carbon films in vacuum and under varying water vapor pressure. Surf. Coat. Technol. 2003, 163, 535–540. [Google Scholar] [CrossRef] [Green Version]
- Gongyang, Y.; Ouyang, W.; Qu, C.; Urbakh, M.; Quan, B.; Ma, M.; Zheng, Q. Temperature and velocity dependent friction of a microscale graphite-DLC heterostructure. Friction 2020, 8, 462–470. [Google Scholar] [CrossRef] [Green Version]
- Tambe, N.S.; Bhushan, B. Nanoscale friction-induced phase transformation of diamond-like carbon. Scr. Mater. 2005, 52, 751–755. [Google Scholar] [CrossRef]
- Hee, A.C.; Zhao, Y.; Choudhury, D.; Ghosh, S.; Zhu, Q.; Zhu, H. Tribological behavior of hydrogenated diamond-like carbon on polished alumina substrate with chromium interlayer for biomedical application. Biotribology 2016, 7, 38–46. [Google Scholar] [CrossRef]
- Miyake, S.; Kawasaki, S.; Yamazaki, S. Nanotribology properties of extremely thin diamond-like carbon films at high temperatures with and without vibration. Wear 2013, 300, 189–199. [Google Scholar] [CrossRef]



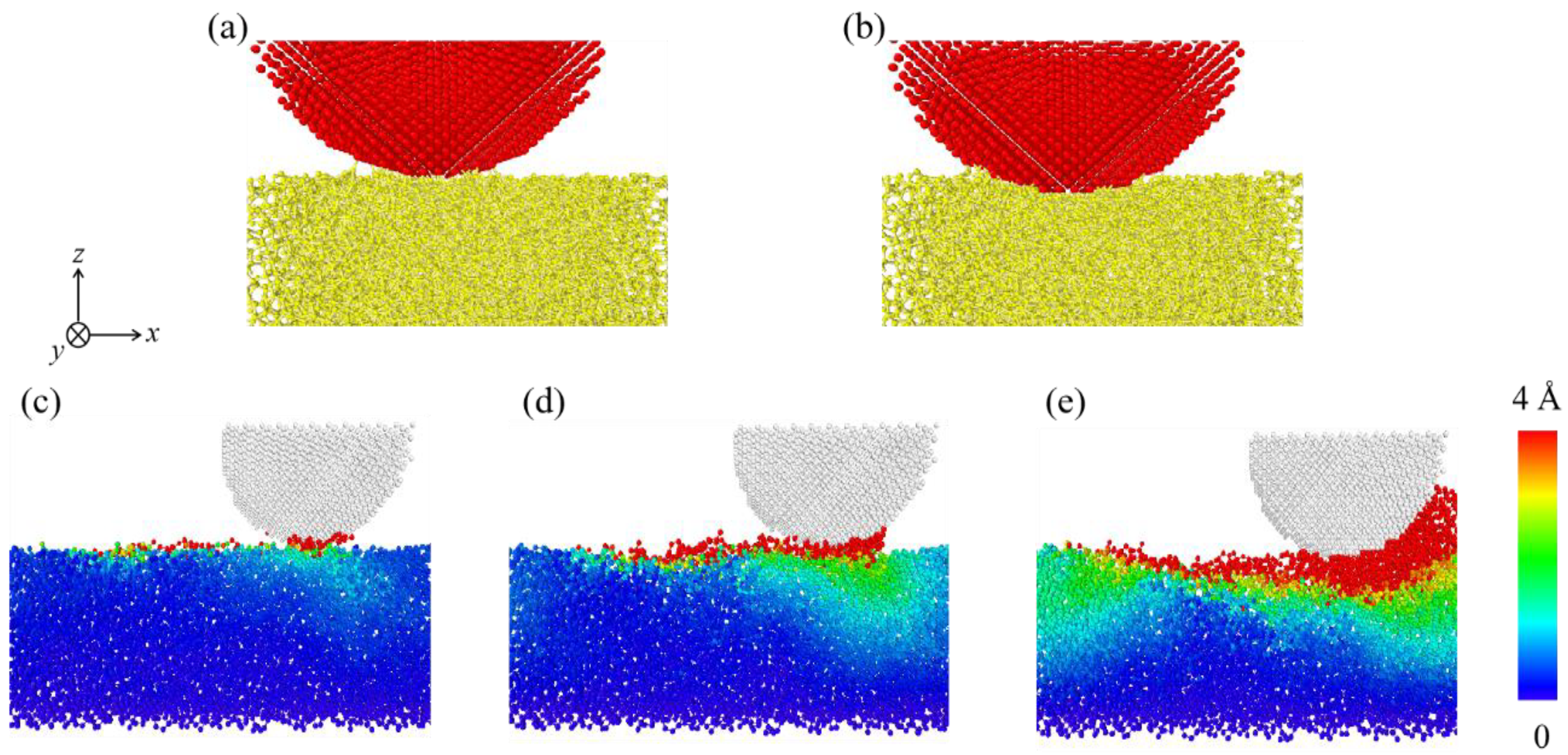
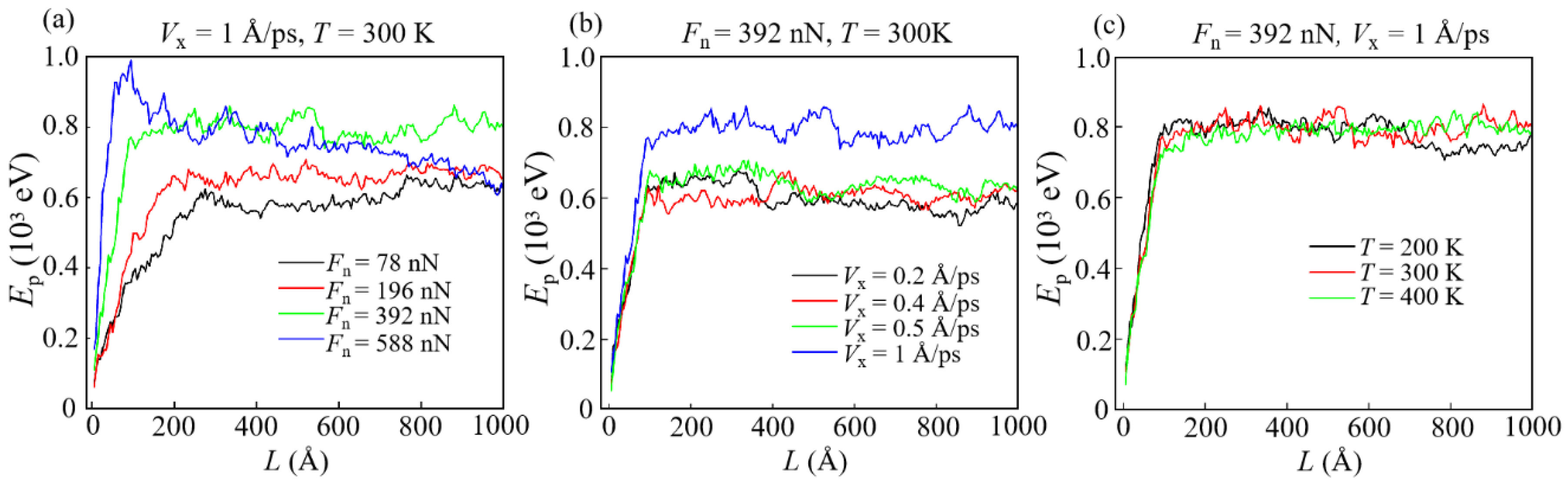
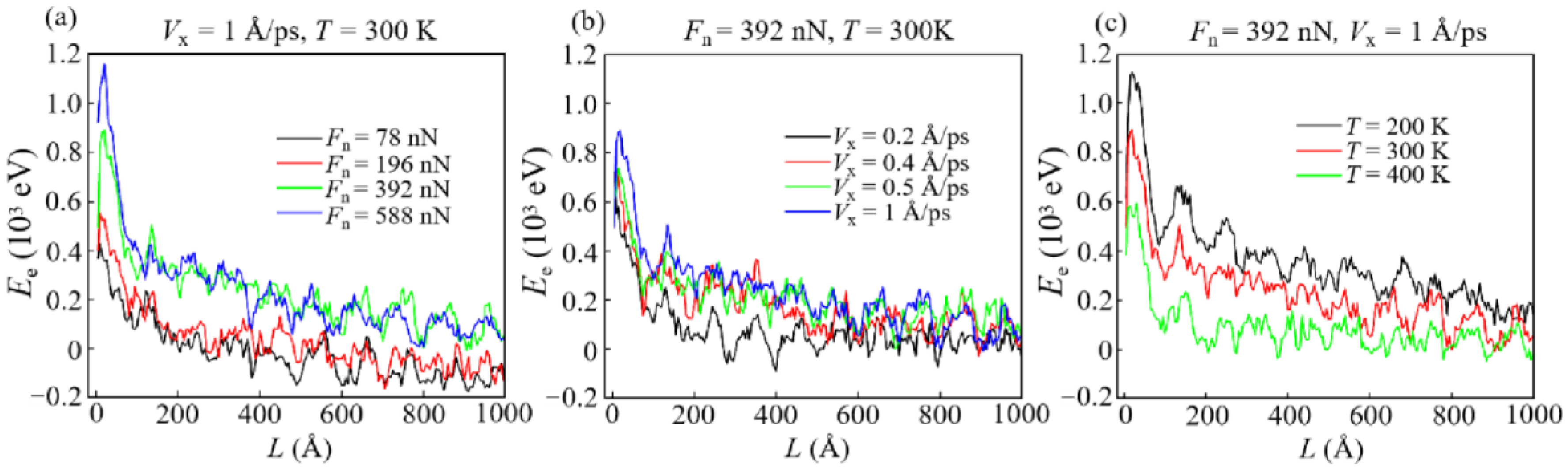
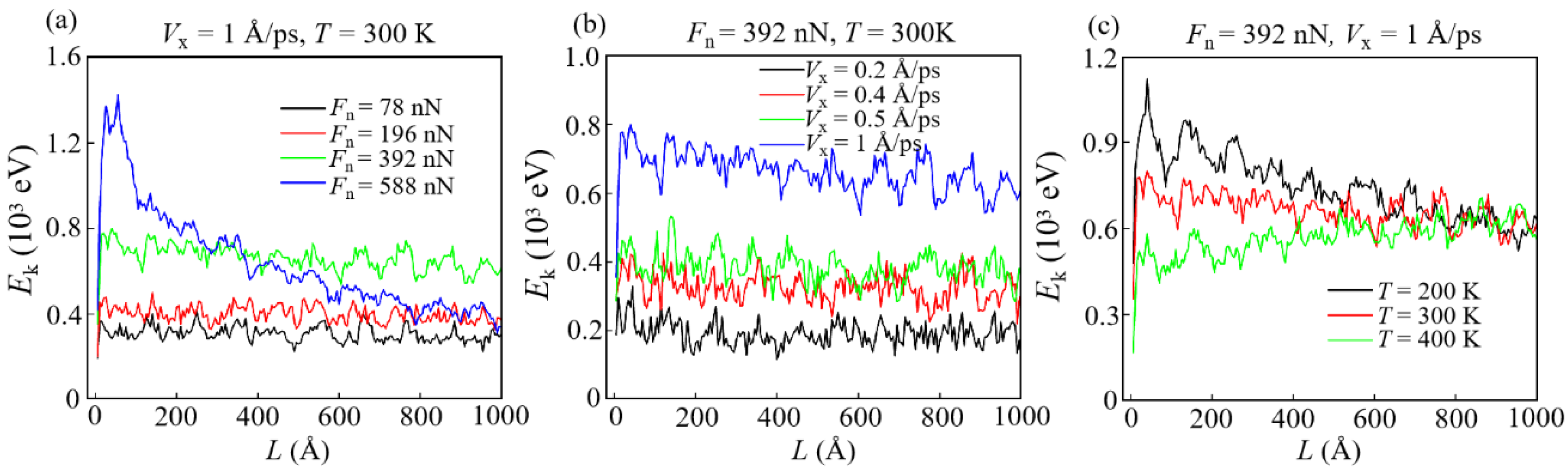

| Fn (nN) | kw (10−4 mm3/Nm) |
|---|---|
| 78 | 9.75 |
| 196 | 6.13 |
| 392 | 5.58 |
| 588 | 8.47 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yin, Z.; Wu, H.; Zhang, G.; Mu, C.; Bai, L. Wear Estimation of DLC Films Based on Energy-Dissipation Analysis: A Molecular Dynamics Study. Materials 2022, 15, 893. https://doi.org/10.3390/ma15030893
Yin Z, Wu H, Zhang G, Mu C, Bai L. Wear Estimation of DLC Films Based on Energy-Dissipation Analysis: A Molecular Dynamics Study. Materials. 2022; 15(3):893. https://doi.org/10.3390/ma15030893
Chicago/Turabian StyleYin, Zhiyuan, Hong Wu, Guangan Zhang, Chenzhong Mu, and Lichun Bai. 2022. "Wear Estimation of DLC Films Based on Energy-Dissipation Analysis: A Molecular Dynamics Study" Materials 15, no. 3: 893. https://doi.org/10.3390/ma15030893
APA StyleYin, Z., Wu, H., Zhang, G., Mu, C., & Bai, L. (2022). Wear Estimation of DLC Films Based on Energy-Dissipation Analysis: A Molecular Dynamics Study. Materials, 15(3), 893. https://doi.org/10.3390/ma15030893