Mathematical Simulation of Casson MHD Flow through a Permeable Moving Wedge with Nonlinear Chemical Reaction and Nonlinear Thermal Radiation
Abstract
:1. Introduction
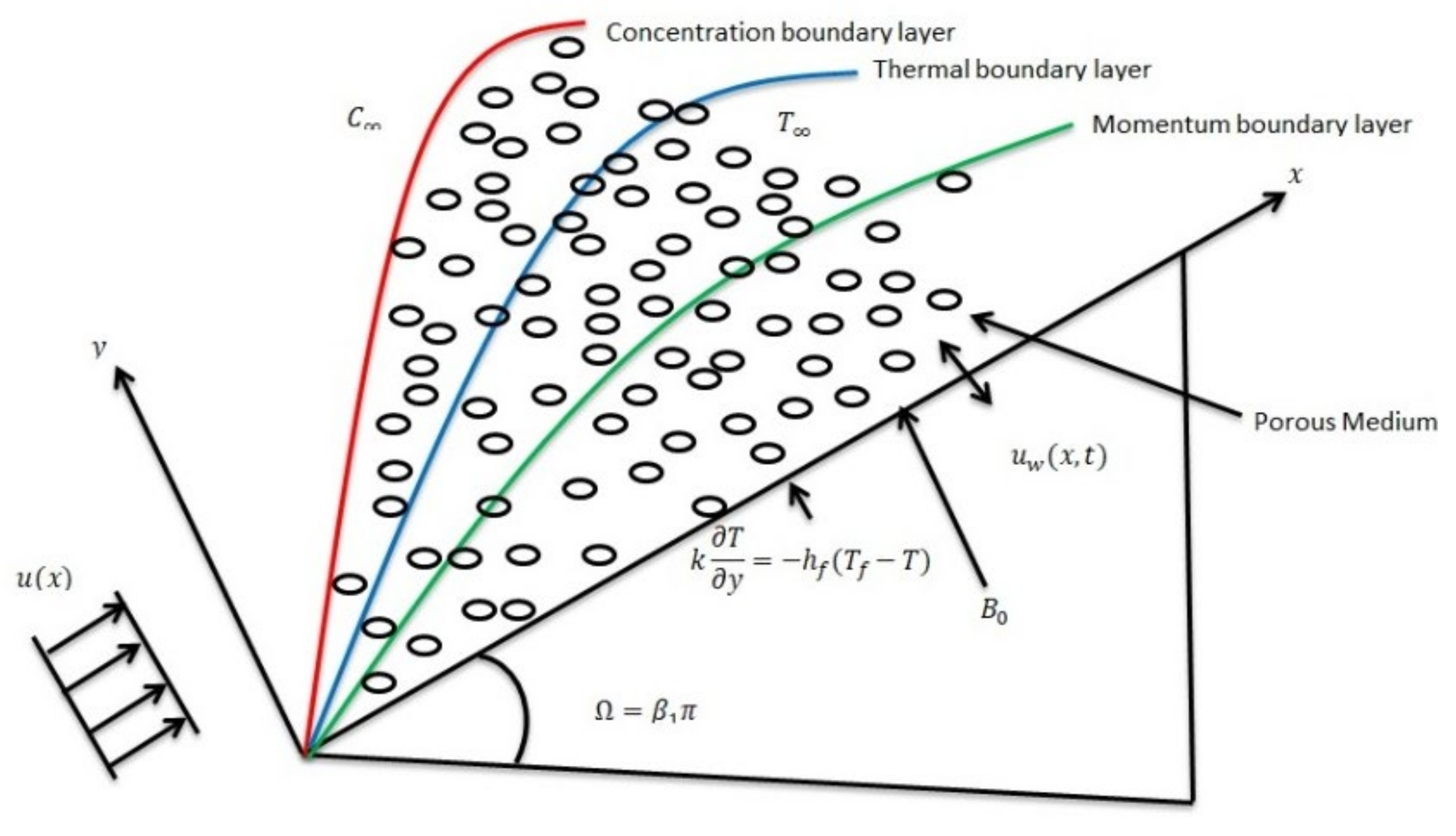
2. Mathematical Modeling
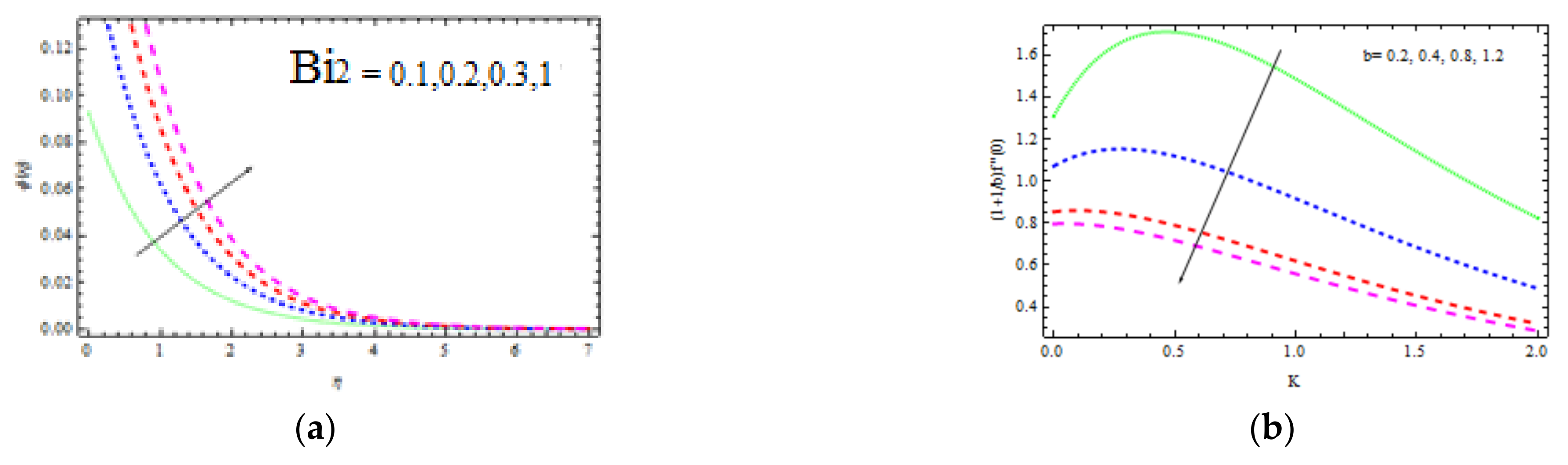
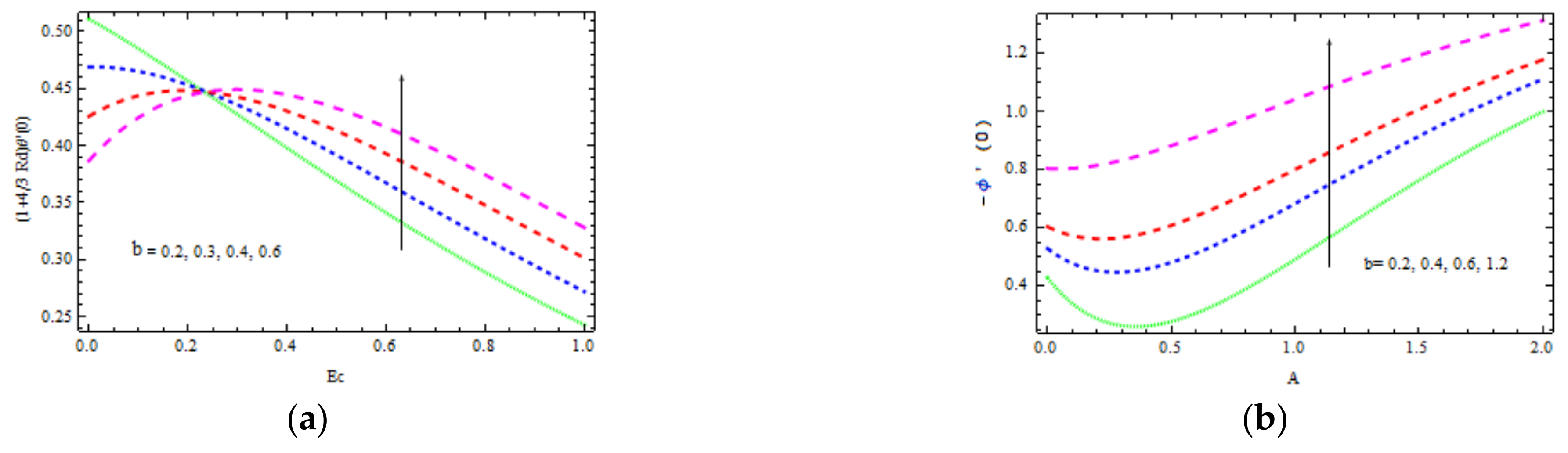
3. Result and Discussion
4. Conclusions
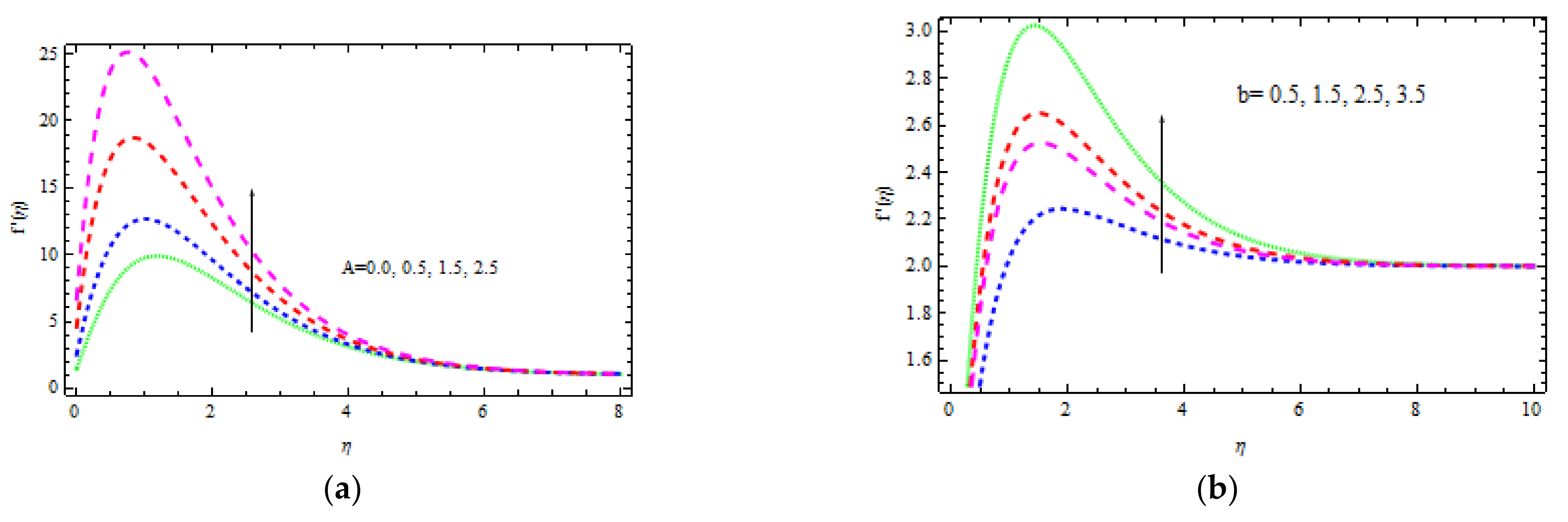
- Increases in A, b, M, and δ decreased the moment boundary layer thickness.
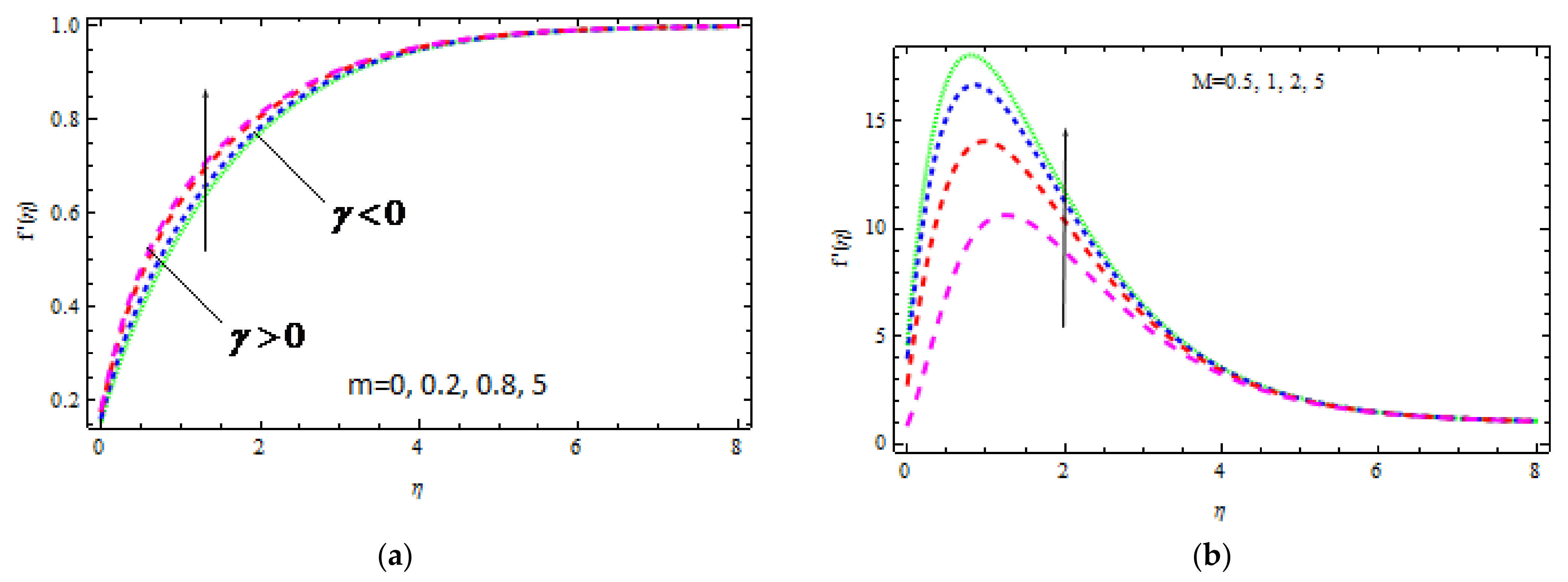
- The influence of k on the flow field was more prominent in γ = 0 and γ < 0 cases.
- The impact of δ on the flow field was less noticeable in the presence of a magnetic field.
- The nonlinear thermal radiation along with concentration thickness boosted with A.
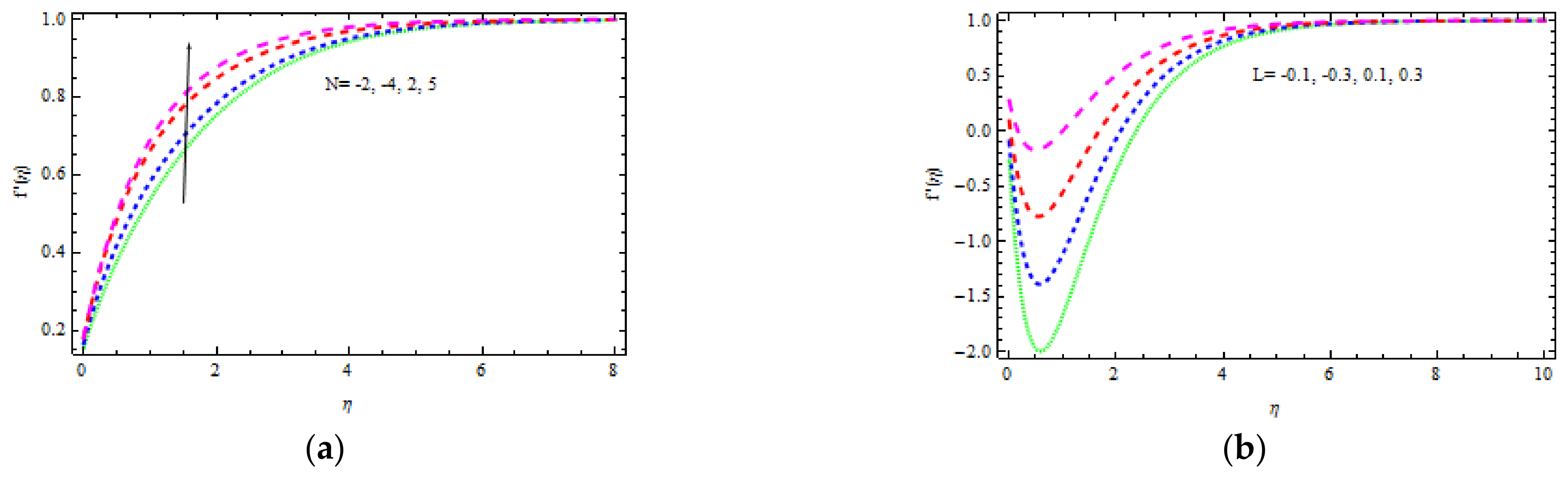
- Rises in λ and N intensely influenced the fluid flow; however, the influence of these factors on heat and concentration field was found slighter.
- The barrier yield stress was detected to decrease with but improve with increases in A and M.
- The temperature and rate of heat transfer were perceived higher with the increase in Rd.
- The mass transfer rate was observed to be greater with the variation in chemical reaction.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| . | Moving wedge parameter |
| , | Biot numbers |
| Unsteadiness parameter | |
| Schmidt number | |
| Eckert number | |
| Chemical reaction parameter | |
| Slip parameter | |
| Prandtl number | |
| Edge angle parameter | |
| Buoyancy ratio parameter | |
| Radiation parameter | |
| b | Casson fluid parameter |
| Thermal buoyancy parameter | |
| Reynold number | |
| Buoyancy ratio parameter | |
| M | Magnetic parameter |
| Sherwood number | |
| Porosity parameter | |
| Edge moving velocity | |
| Hartee pressure gradient parameter | |
| Convective heat | |
| Stream velocity | |
| Injected orthogonal toward edge | |
| Heat transfer | |
| Total angle of wedge | |
| Wall temperature edge | |
| Heat flux | |
| Constants | |
| Concentration | |
| Negative flow | |
| Time | |
| Slip velocity | |
| Assisting flow | |
| Nonisothermal flow | |
| Local skin friction | |
| Nusselt number |
References
- Casson, N. A flow equation for pigment-oil suspensions of the printing ink type. In Rheology of Disperse Systems; Mill, C.C., Ed.; Pergamon Press: Oxford, UK, 1959; pp. 84–104. [Google Scholar]
- Rao, A.S.; Prasad, V.R.; Reddy, N.B.; Bég, O.A. Heat Transfer in a Casson Rheological Fluid from a Semi-infinite Vertical Plate with Partial Slip. Heat Transf. Asian Res. 2015, 44, 272–291. [Google Scholar] [CrossRef]
- Mitsoulis, E. Flows of viscoplastic materials: Models and computations. Br. Soc. Rheol. 2007, 64, 135–178. [Google Scholar]
- Mukhopadhyay, S. Casson fluid flow and heat transfer over a nonlinearly stretching surface. Chin. Phys. B 2013, 22, 074701. [Google Scholar] [CrossRef]
- Hayat, T.; Shehzad, S.A.; Alsaedi, A. Soret and Dufour effects on magnetohydrodynamic (MHD) flow of Casson fluid. Appl. Math. Mech. 2012, 33, 1301–1312. [Google Scholar] [CrossRef]
- Mustafa, M.; Hayat, T.; Pop, I.; Aziz, A. Unsteady boundary layer flow of a Casson fluid due to an impulsively started moving flat plate. Heat Transf. Asian Res. 2011, 40, 563–576. [Google Scholar] [CrossRef]
- Chinyoka, T.; Makinde, O.D. Analysis of Entropy Generation Rate in an Unsteady Porous Channel Flow with Navier Slip and Convective Cooling. Entropy 2013, 15, 2081–2099. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.-T.; Wang, Y.-H.; Huang, B.-Y. Numerical Optimization for Nanofluid Flow in Microchannels Using Entropy Generation Minimization. Numer. Heat Transf. Part A Appl. 2014, 67, 571–588. [Google Scholar] [CrossRef]
- Slimi, K.; Saati, A.A. Entropy Generation Rate Due to Radiative Transfer Within a Vertical Channel Filled with a Semi-Transparent Porous Medium. Arab. J. Sci. Eng. 2012, 37, 803–820. [Google Scholar] [CrossRef]
- Das, S.; Jana, R.N. Entropy generation in MHD porous channel flow under constant pressure gradient. Appl. Math. 2013, 1, 78–89. [Google Scholar]
- Abbaszadeh, M.; Ababei, A.; Arani, A.A.A.; Sharifabadi, A.A. MHD forced convection and entropy generation of CuO-water nanofluid in a microchannel considering slip velocity and temperature jump. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 775–790. [Google Scholar] [CrossRef]
- Uddin, J.; Bég, O.A.; Ismail, A.I. Radiative Convective Nanofluid Flow Past a Stretching/Shrinking Sheet with Slip Effects. J. Thermophys. Heat Transf. 2015, 29, 513–523. [Google Scholar] [CrossRef]
- Shaw, S.; Kameswaran, P.K.; Sibanda, P. Effects of slip on nonlinear convection in nanofluid flow on stretching surfaces. Bound. Value Probl. 2016, 2016, 2. [Google Scholar] [CrossRef] [Green Version]
- López, A.; Ibáñez, G.; Pantoja, J.; Moreira, J.; Lastres, O. Entropy generation analysis of MHD nanofluid flow in a porous vertical microchannel with nonlinear thermal radiation, slip flow and convective-radiative boundary conditions. Int. J. Heat Mass Transf. 2017, 107, 982–994. [Google Scholar] [CrossRef]
- Khan, M.I.; Waqas, M.; Hayat, T.; Alsaedi, A. Colloidal study of Casson fluid with homogeneous-heterogeneous reactions. J. Colloid Interface Sci. 2017, 498, 85–90. [Google Scholar] [CrossRef] [PubMed]
- Vyas, P.; Soni, S. Entropy analysis for MHD Casson fluid flow in a channel subjected toweakly temperature dependent convection and hydrodynamic slip. J. Rajasthan Acad. Phys. Sci. 2016, 15, 1–18. [Google Scholar]
- Ng, C.-O. Combined pressure-driven and electroosmotic flow of Casson fluid through a slit microchannel. J. Non-Newton. Fluid Mech. 2013, 198, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Eegunjobi, A.S.; Makinde, O.D. MHD mixed convection slip flow of radiation Cassonfluid with entropy generation in a channel filled with porous media. Defect Diffus. Forum 2017, 374, 47–66. [Google Scholar] [CrossRef]
- Passos, A.D.; Chatzieleftheriou, V.A.; Mouza, A.A.; Paras, S.V. Cassonfluid flow in a microchannel containing a flow disturbing rib. Chem. Eng. Sci. 2016, 148, 229–237. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Mujtaba, M.; Quadri, A.; Issa, C. Thermal radiation effect son MHD forced convection flow adjacent to a non-isothermal wedge in the presence of a heat source or sink. Heat Mass Transf. 2003, 39, 305–312. [Google Scholar] [CrossRef]
- Hayat, T.; Qasim, M. Influence of thermal radiation and Joule heating on MHD flow of a Maxwell fluid in the presence of thermophoresis. Int. J. Heat Mass Transf. 2010, 53, 4780–4788. [Google Scholar] [CrossRef]
- Ahmed, N.; Khan, U.; Zaidi, Z.A.; Jan, S.U.; Waheed, A.; Mohyud-Din, S.T. MHD Flow of an incompressible fluid through porous medium between dilating and squeezing permeable walls. J. Porous Media 2014, 17, 861–867. [Google Scholar] [CrossRef]
- Kandasamy, R.; Muhaimin, I.; Rosmila, A. The performance evaluation of unsteady MHD non-Darcy nanofluid flow over a porous wedge due to renewable (solar) energy. Renew. Energy 2014, 64, 1–9. [Google Scholar] [CrossRef] [Green Version]
- Mohyud-Din, S.T.; Zaidi, Z.A.; Khan, U.; Ahmed, N. On heat and mass transfer analysis for the flow of a nanofluid between rotating parallel plates. Aerosp. Sci. Technol. 2015, 46, 514–522. [Google Scholar] [CrossRef]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T.; Bin-Mohsin, B. Nonlinear radiation effects on MHD flow of nanofluid over a nonlinearly stretching/shrinking wedge. Neural Comput. Appl. 2016, 28, 2041–2050. [Google Scholar] [CrossRef]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T. Influence of viscous dissipation and Joule heating on MHD bio-convection flow over a porous wedge in the presence of nanoparticles and gyrotacticmicro organisms. Springerplus 2016, 5, 2043. [Google Scholar] [CrossRef] [Green Version]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T.; Bin-Mohsin, B. Abio-convection model for MHD flow and heat transfer over a porous wedge containing nanoparticles and gyrotactic microorganisms. J. Biol. Syst. 2016, 24, 409–429. [Google Scholar] [CrossRef]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T. Analysis of magnetohydrodynamic flow and heat transfer of Cu–water nanofluid between parallel plates for different shapes of nanoparticles. Neural Comput. Appl. 2018, 29, 695–703. [Google Scholar] [CrossRef]
- Ahmed, N.; Khan, U.; Mohyud-Din, S.T. Influence of nonlinear thermal radiation on the viscous flow through a deformable asymmetric porous channel: A numerical study. J. Mol. Liq. 2017, 225, 167–173. [Google Scholar] [CrossRef]
- Ahmed, N.; Khan, U.; Mohyud-Din, S.T. MHD nanofluid flow through a deformable asymmetric porous channel. Eng. Comput. 2017, 34, 852–868. [Google Scholar] [CrossRef]
- Kumari, M.; Takhar, H.S.; Nath, G. Mixed convection flow over a vertical wedge embedded in a highly porous medium. Heat Mass Transf. 2001, 37, 139–146. [Google Scholar] [CrossRef] [Green Version]
- Gorla, R.S.R.; Chamkha, A.J.; Rashad, A.M. Mixed convective boundary layer flow over a vertical wedge embedded in a porous medium saturated with a nanofluid: Natural Convection Dominated Regime. Nanoscale Res. Lett. 2011, 6, 207. [Google Scholar] [CrossRef] [Green Version]
- Chamkha, A.J.; Abbasbandy, S.; Rashad, A.M.; Vajravelu, K. Radiation effects on mixed convection over a wedge embedded in a porou smedium filled with nanofluid. Transp. Porous Media 2012, 91, 261–279. [Google Scholar] [CrossRef]
- Chamkha, A.J.; Rashad, A.M.; Gorla RS, R. Non-similar solutions for mixed convection along a wedge embedded in a porous medium saturated by a non-Newtonian nanofluid: Natural convection dominated regime. Int. J. Numer. Methods Heat Fluid Flow 2014, 24, 1471–1486. [Google Scholar] [CrossRef]
- Magyari, E.; Chamkha, A.J. Combined effect of heat generation or absorption and first-order chemical reaction on micropolar fluid flows over a uniformly stretched permeable surface: The full analytical solution. Int. J. Therm. Sci. 2010, 49, 1821–1828. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Bhattacharyya, K. Unsteady flow of a Maxwell fluid over a stretching surface in presence of chemical reaction. J. Egypt. Math. Soc. 2012, 20, 229–234. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharyya, K.; Mukhopadhyay, S.; Layek, G.C. Unsteady MHD boundary layer flow with diffusion and first-order chemical reaction over a permeable stretching sheet with suction or blowing. Chem. Eng. Commun. 2013, 200, 379–397. [Google Scholar] [CrossRef]
- Ganapathirao, M.; Ravindran, R.; Momoniat, E. Effects of chemical reaction, heat and mass transfer on an unsteady mixed convection boundary layer flow over a wedge with heat generation/absorption in the presence of suction or injection. Heat Mass Transf. 2015, 51, 289–300. [Google Scholar] [CrossRef]
- Khan, U.; Adnan; Ahmed, N.; Mohyud-Din, S.T. Influence of the shape factor on the flow and heat transfer of a water-based nanofluid in a rotating system. Eur. Phys. J. Plus 2017, 132, 166. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Ahmed, N.; Khan, U.; Rashidi, M.M. A numerical study of thermo-diffusion, diffusion-thermo and chemical reaction effects on flow of a micropolar fluid in an asymmetric channel with dilating and contracting permeable walls. Eng. Comput. 2017, 34, 587–602. [Google Scholar] [CrossRef]
- Mohyud-Din, S.T.; Ahmed, N.; Khan, U.; Waheed, A.; Hussain, S.; Darus, M. On Combined Effects of Heat Transfer and Chemical Reaction for the Flow through an Asymmetric Channel with Orthogonally Deformable Porous Walls. Math. Probl. Eng. 2016, 2016, 2568785. [Google Scholar] [CrossRef]
- Mukhopadhyay, S.; Mandal, I.C. Boundary layer flow and heat transfer of a Casson fluid past a symmetric porous wedge with surface heat flux. Chin. Phys. B 2014, 23, 044702. [Google Scholar] [CrossRef]
- El-Dabe, N.T.; Ghaly, A.Y.; Rizkallah, R.R.; Ewis, K.M.; Al-Bareda, A.S. Solution of mhd boundary layer flow of non-newtonian Casson fluid on a moving wedge with heat and mass transfer and induced magnetic field. J. Appl. Math. Phys. 2015, 3, 649–663. [Google Scholar] [CrossRef] [Green Version]
- Ullah, I.; Khan, I.; Shafie, S. Hydromagnetic Falkner-Skan flow of Casson fluid past a moving wedge with heat transfer. Alex. Eng. J. 2016, 55, 2139–2148. [Google Scholar] [CrossRef] [Green Version]
- Adnan; Khan, U.; Ahmed, N.; Mohyud-Din, S.T. Thermo-diffusion and diffusion-thermo effects on flow of second grade fluid between two inclined plane walls. J. Mol. Liq. 2016, 224, 1074–1082. [Google Scholar] [CrossRef]
- Khan, U.; Ahmed, N.; Mohyud-Din, S.T.; Sikander, W. Flow of carbon nanotubes suspended nanofluid in stretchable non-parallel walls. Neural Comput. Appl. 2018, 30, 2859–2871. [Google Scholar] [CrossRef]
- Ahmed, N.; Adnan; Khan, U.; Mohyud-Din, S.T. Influence of thermal radiation and viscous dissipation on squeezed flow of water between Riga plates saturated with carbon nanotubes. Colloids Surf. A Physicochem. Eng. Asp. 2017, 522, 389–398. [Google Scholar] [CrossRef]
- Ahmed, N.; Adnan, I.; Khan, U.; Mohyud-Din, S.T.; Manzoor, R. Influence of viscous dissipation on a copper oxide nanofluid in an oblique channel: Implementation of the KKL model. Eur. Phys. J. Plus 2017, 132, 237. [Google Scholar] [CrossRef]
- Nikan, O.; Avazzadeh, Z. A localisation technique based on radial basis function partition of unity for solving Sobolev equation arising in fluid dynamics. Appl. Math. Comput. 2021, 401, 126063. [Google Scholar] [CrossRef]



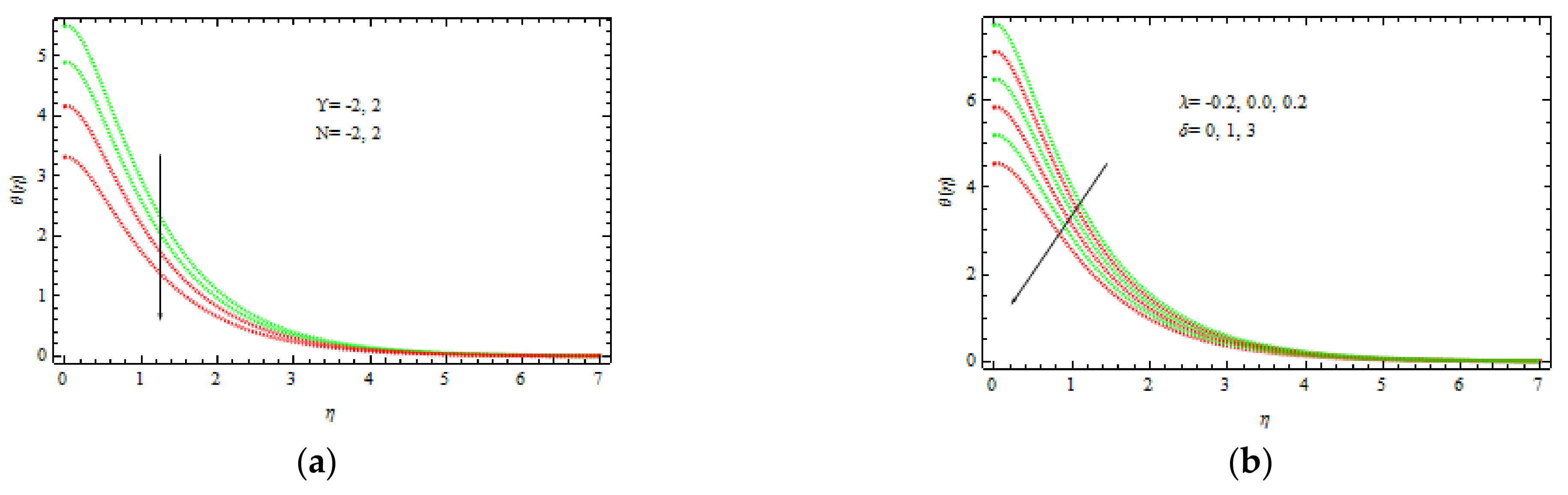
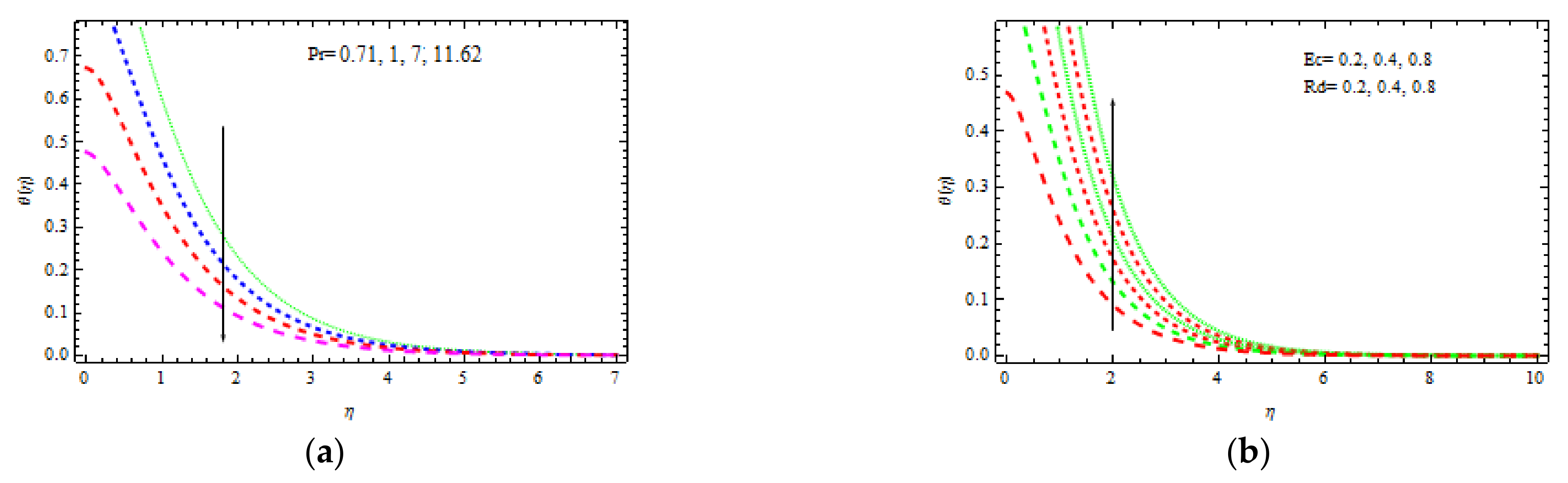
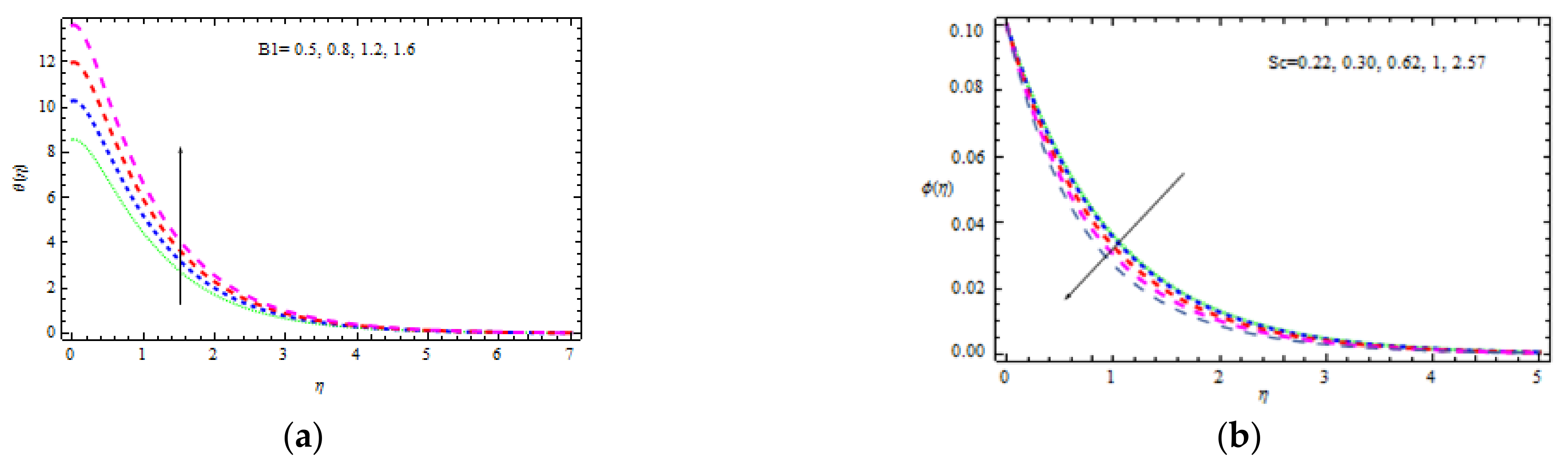
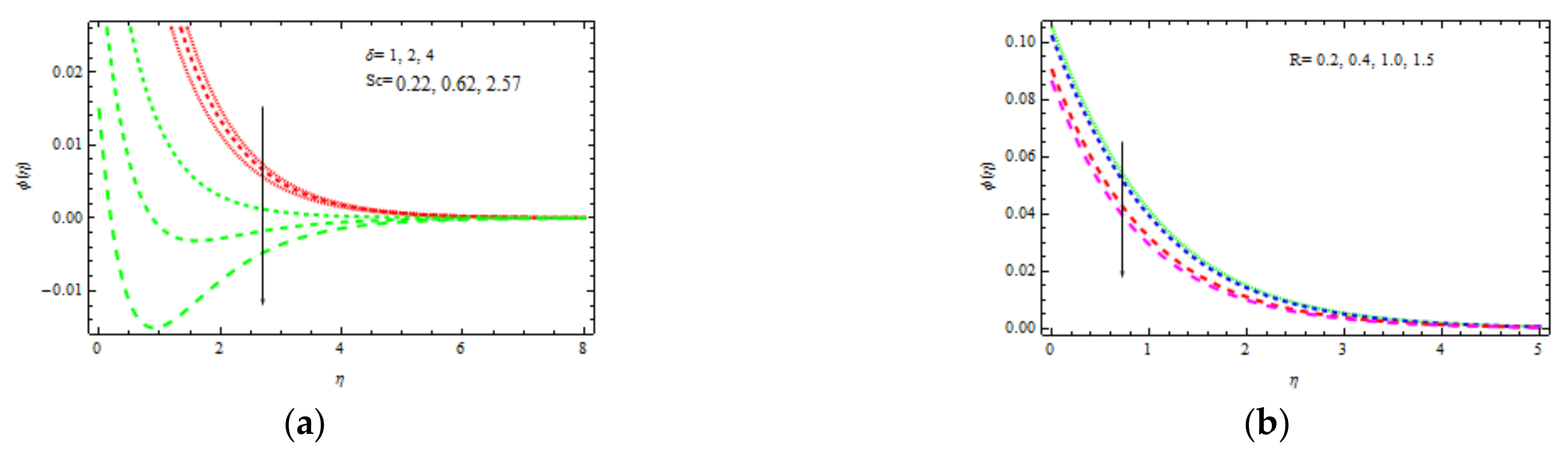
| Gorla et al. [32] | Kumari et al. [31] | Present | |
|---|---|---|---|
| 0.0 | 0.45851 | 0.458506 | 0.45845 |
| 0.2 | 0.51361 | 0.513605 | 0.51356 |
| 0.34 | 0.55787 | 0.557868 | 0.55779 |
| 0.46 | 0.64387 | 0.643869 | 0.64380 |
| 1.0 | 0.72112 | 0.721117 | 0.72116 |
| 1.5 | 0.81124 | 0.811240 | 0.81116 |
| 2.0 | 0.91654 | 0.916538 | 0.916535 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, Z.; Rasheed, H.U.; Khan, I.; Abu-Zinadah, H.; Aldahlan, M.A. Mathematical Simulation of Casson MHD Flow through a Permeable Moving Wedge with Nonlinear Chemical Reaction and Nonlinear Thermal Radiation. Materials 2022, 15, 747. https://doi.org/10.3390/ma15030747
Khan Z, Rasheed HU, Khan I, Abu-Zinadah H, Aldahlan MA. Mathematical Simulation of Casson MHD Flow through a Permeable Moving Wedge with Nonlinear Chemical Reaction and Nonlinear Thermal Radiation. Materials. 2022; 15(3):747. https://doi.org/10.3390/ma15030747
Chicago/Turabian StyleKhan, Zeeshan, Haroon Ur Rasheed, Ilyas Khan, Hanaa Abu-Zinadah, and Maha A. Aldahlan. 2022. "Mathematical Simulation of Casson MHD Flow through a Permeable Moving Wedge with Nonlinear Chemical Reaction and Nonlinear Thermal Radiation" Materials 15, no. 3: 747. https://doi.org/10.3390/ma15030747
APA StyleKhan, Z., Rasheed, H. U., Khan, I., Abu-Zinadah, H., & Aldahlan, M. A. (2022). Mathematical Simulation of Casson MHD Flow through a Permeable Moving Wedge with Nonlinear Chemical Reaction and Nonlinear Thermal Radiation. Materials, 15(3), 747. https://doi.org/10.3390/ma15030747







