Simulation of Continuous Dynamic Recrystallization Using a Level-Set Method
Abstract
1. Introduction
- Discontinuous DRX (DDRX), if recrystallized grains nucleate at some specific locations, generally close to grain boundaries (GB), and then grow and consume the deformed grains surrounding them. DDRX is therefore characterized by spatial and temporal discontinuity at the polycrystal scale.
- Continuous DRX (CDRX), when recrystallized grains form slowly and continuously during deformation. In that case, grain formation is induced by the progressive reorganization of dislocations into cells or subgrains and the gradual increase in misorientation angle between those subgrains.
- Geometric DRX (GDRX), at large strains when grains become serrated and some GBs start to meet and enclose new grains.
2. Numerical Framework
2.1. Grain Boundary Description
2.2. Generation and Evolution of Microstructures
2.2.1. Generation of A Two-Level Microstructure
2.2.2. Progressive Formation of Subgrains
- Rearrangement into LAGB that bound new subgrains. Subgrain formation is described through following Equation [14]:where is the surface of LAGB created. is a coefficient describing the fraction of dislocations recovered to form new subgrains. D is the grain diameter, is a grain reference diameter, and m is a fixed coefficient. is the number of sets of dislocations and the disorientation of newly formed subgrains.
- Stacking into pre-existing LAGBs, which is modeled according to the following Equation [14]:
- Absorption during HAGB migration. This is naturally captured by affecting the areas swept by moving boundaries with a low dislocation density as described earlier.
2.3. Material Parameters
3. Results and Discussion
3.1. Modeling of GG of a Fully Substructured Microstructure
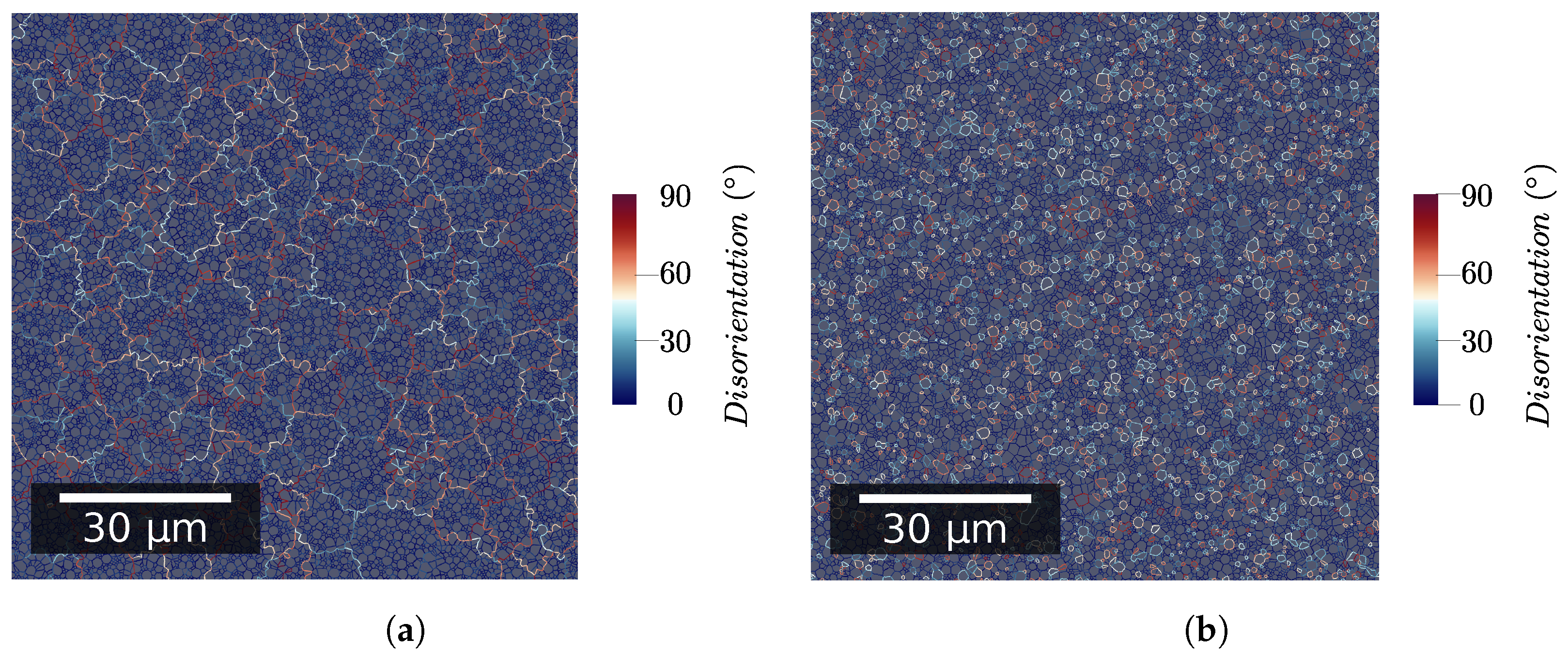
3.1.1. Influence of Microstructure Topology
- With subgrains located inside grains using the generation method described in Section 2.2.1 (Figure 2a);
- With LAGB and HAGB evenly distributed throughout the whole representative volume element (RVE) (see Figure 2b).
3.1.2. Influence of Subgrain Parameters
- With grain and subgrain size distributions taken from experimental data;
- With grain distribution taken from experimental data and a unique subgrain size.
- GB energy is taken heterogeneous and GB mobility is considered constant;
- GB energy and mobility are both considered heterogeneous.
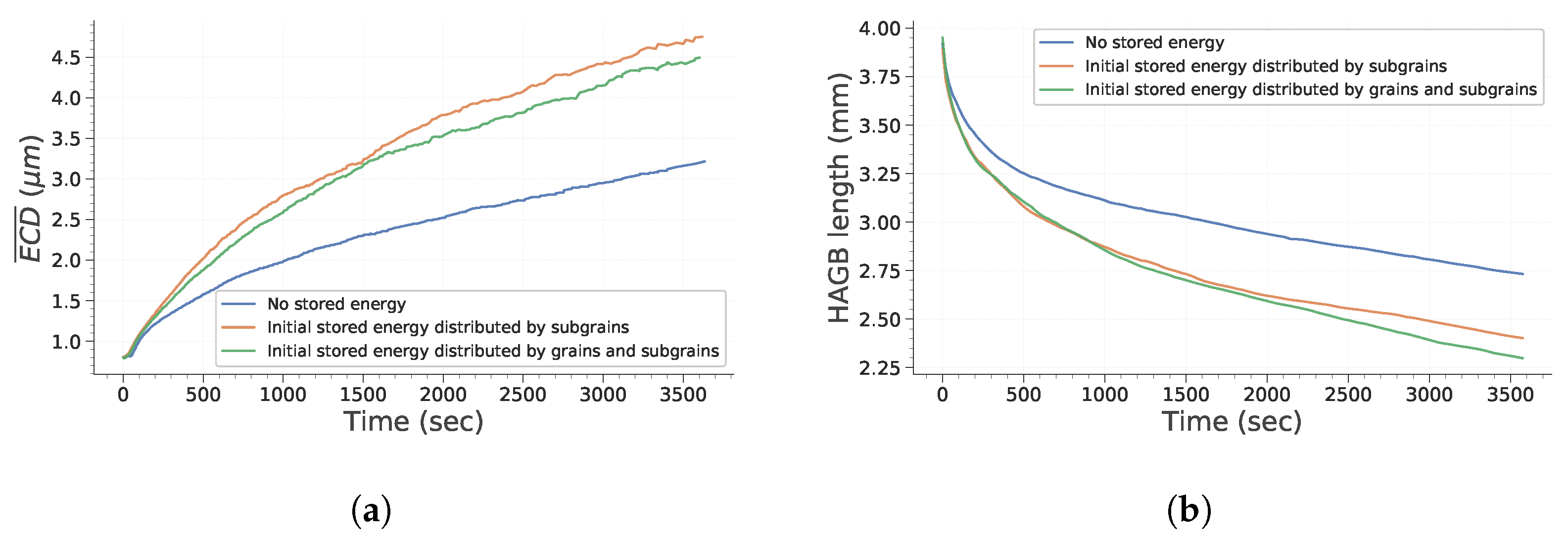
3.1.3. Influence of Stored Energy
- Stored energy is initialized per subgrain by considering a dislocation density distribution taken from estimation of geometrically necessary dislocations (GND) by EBSD measurements.
- Stored energy is initialized per grain using the same distribution. Then, subgrain energy is initialized weighing the parent grain using coefficients (named ) respecting normal distribution. The parameters of this normal distribution are defined as follows: ; ; . The distribution parameters have been set to ensure that subgrains inside grains do not have the exact same energy and that it is still a driving pressure.
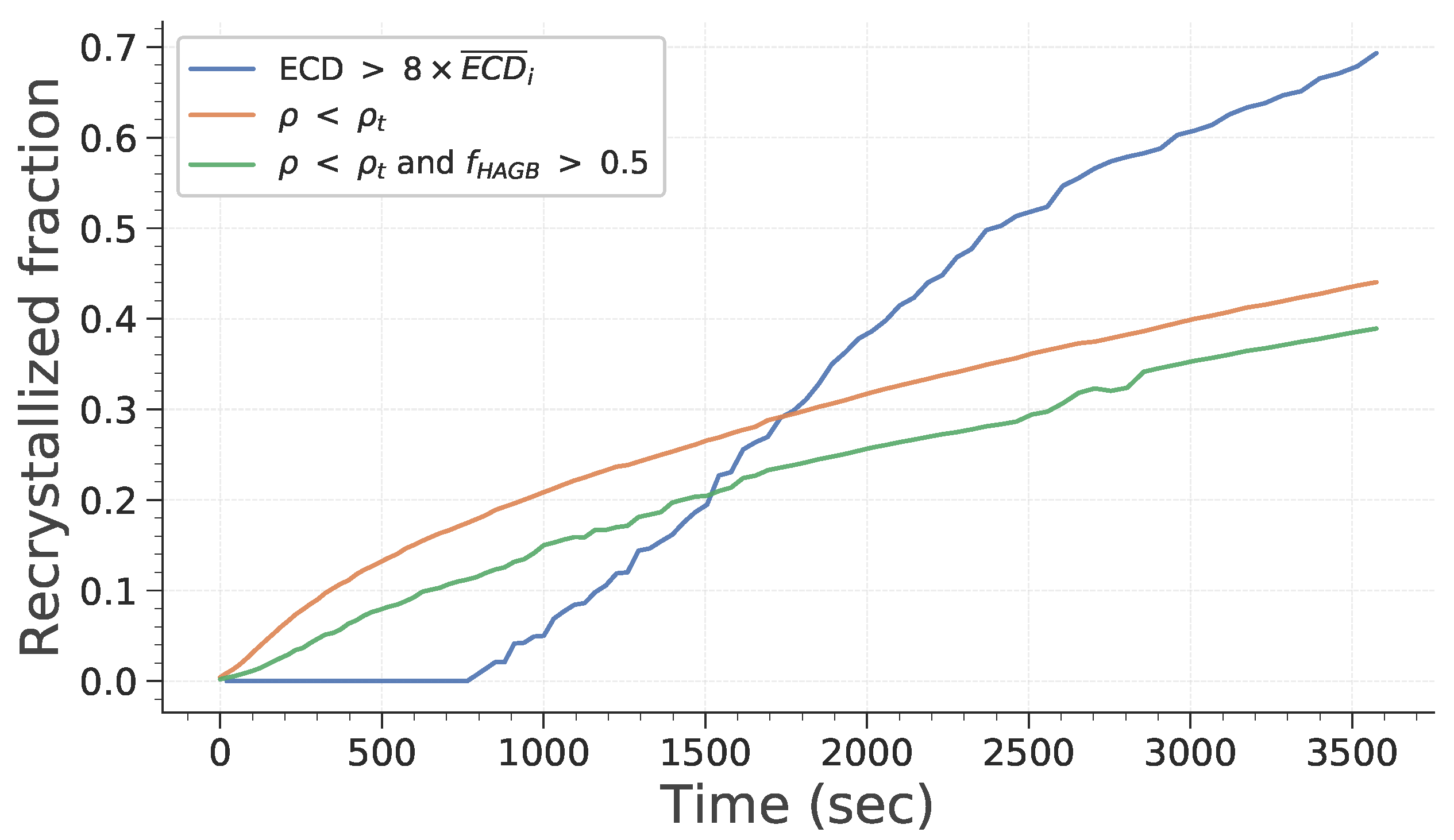
3.1.4. Discussion of The Numerical Criterion for Identification of Recrystallized Grains
- They are eight times bigger than the initial mean subgrain size;
- Their internal dislocation density is lower than a given threshold;
- Their internal dislocation density is lower than a given threshold and at least half of the boundaries surrounding it are HAGBs.
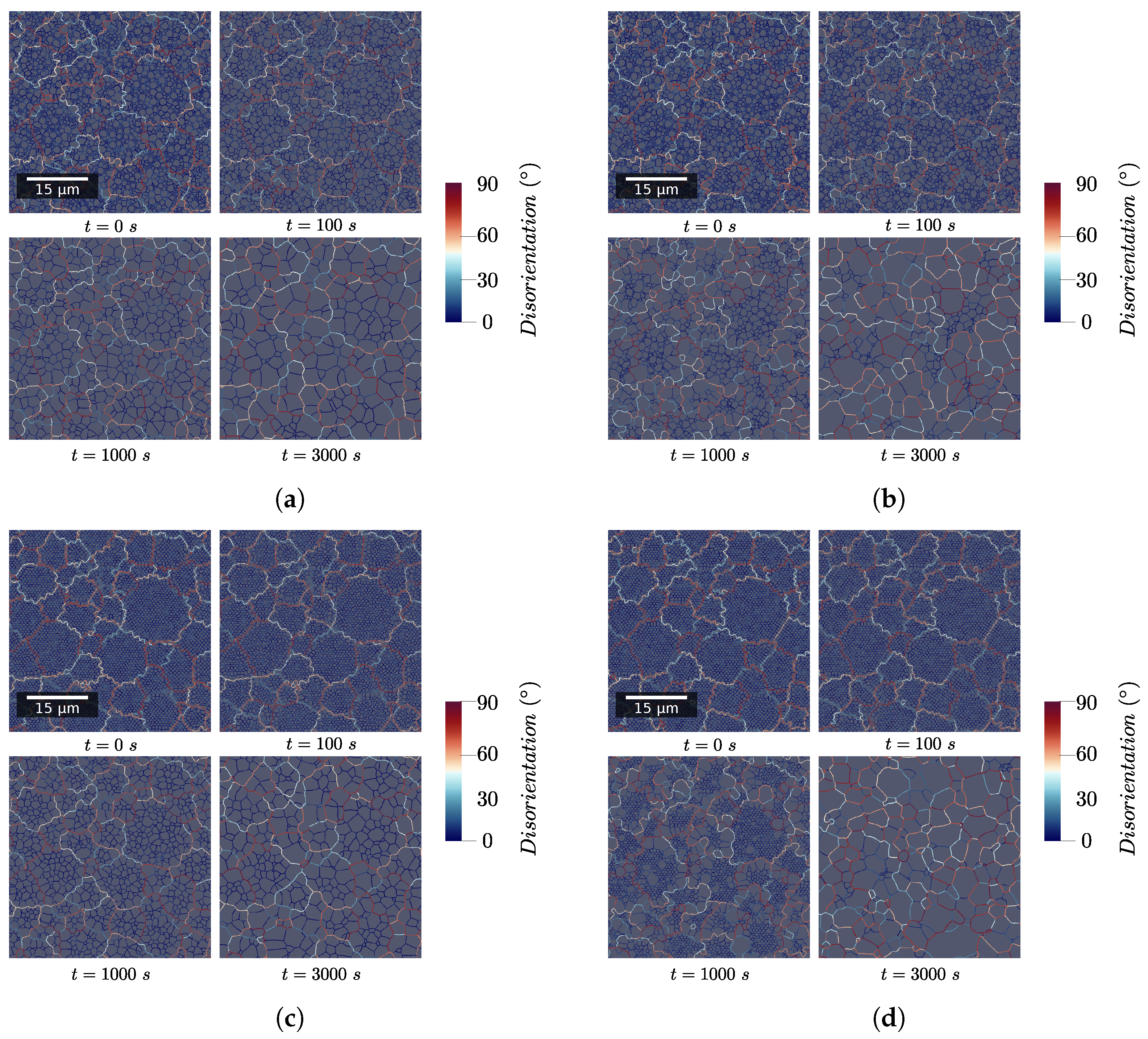
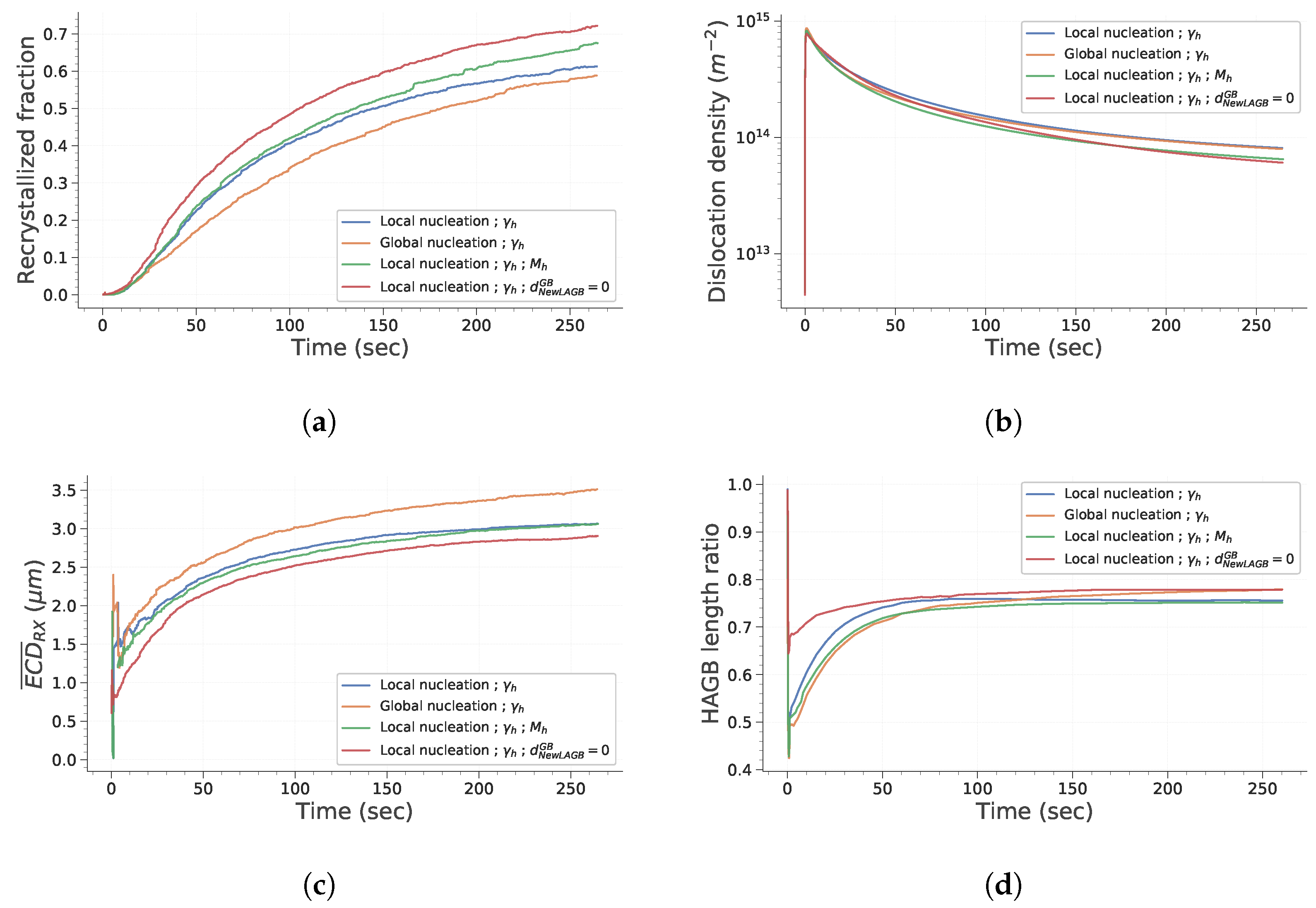
3.2. Modeling of CDRX and PDRX
- (a)
- The number of subgrains that are formed at each deformation increment is computed individually per grain/subgrain. GB energy is described by the RS equation and GB mobility is isotropic.
- (b)
- The number of subgrains that are formed at each deformation increment is computed for the whole domain. Then, new subgrains are positioned randomly within the RVE. GB energy is described by RS Equation (Equation (15)) and GB mobility is isotropic.
- (c)
- (d)
- This last case respects the same rules than the first one, except that new subgrains can be placed on pre-existing grain boundaries.
- m,
- only grains, i.e., entities bounded by at least of HAGB, are included in the measure of recrystallized grains.
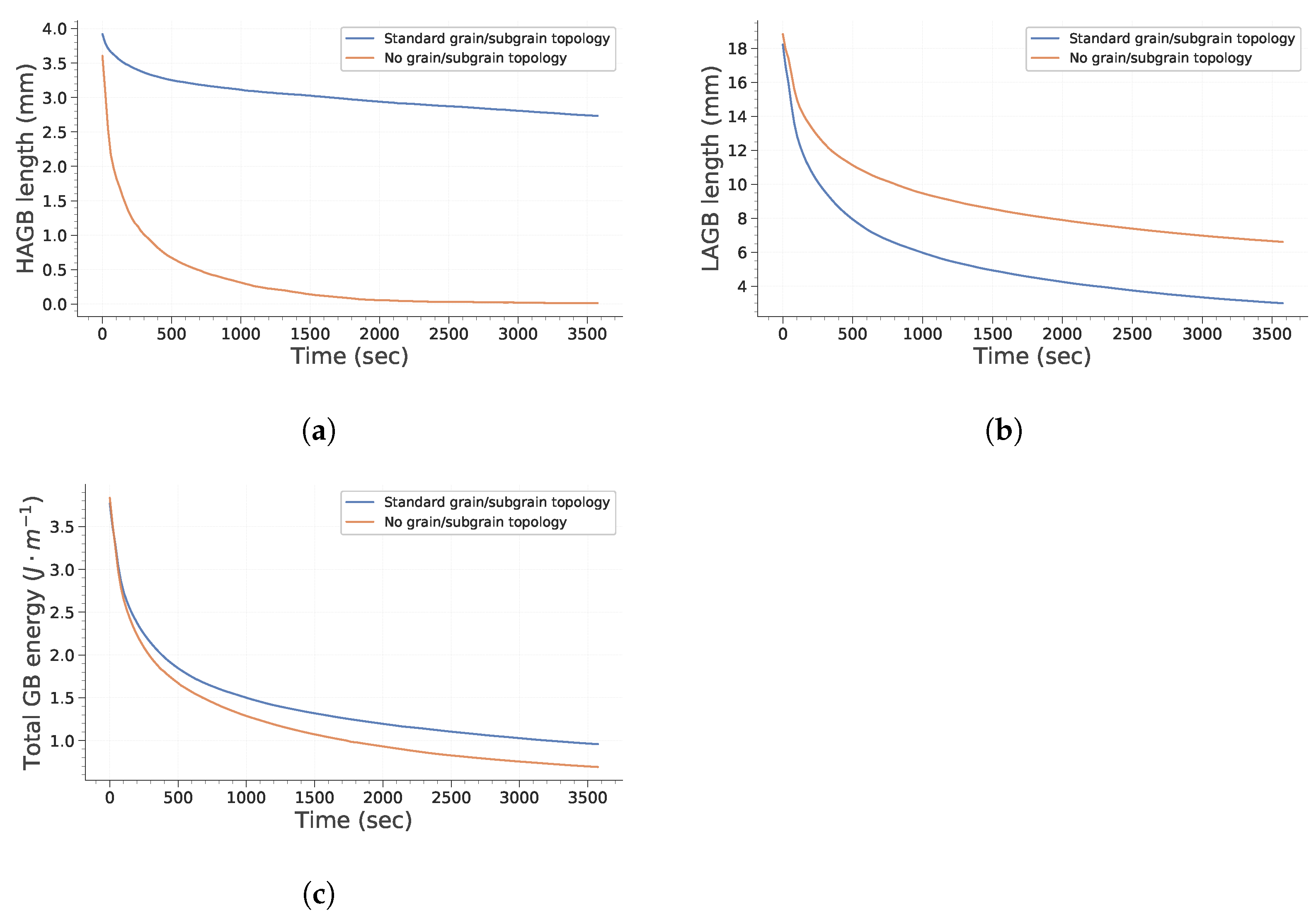
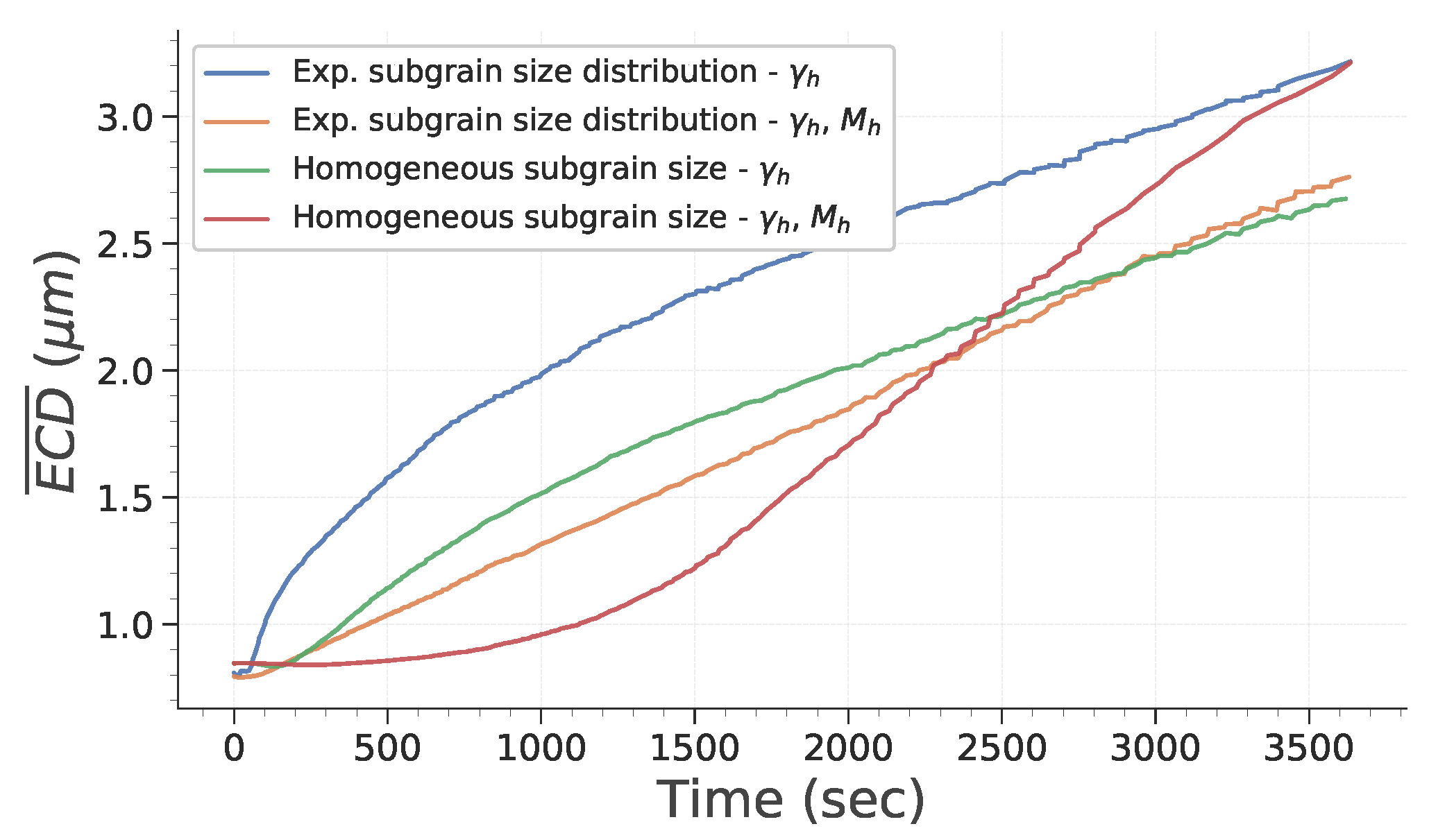
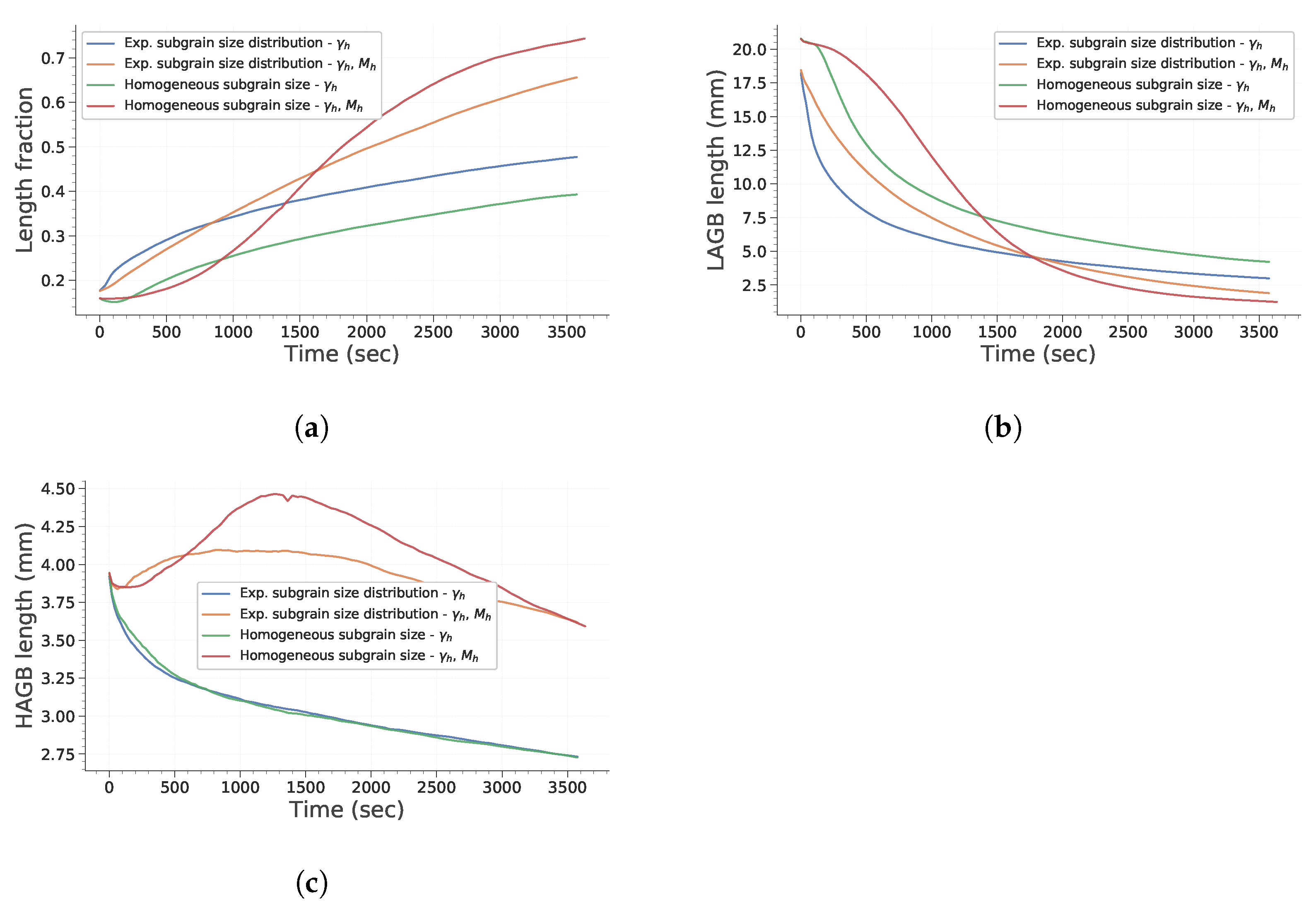
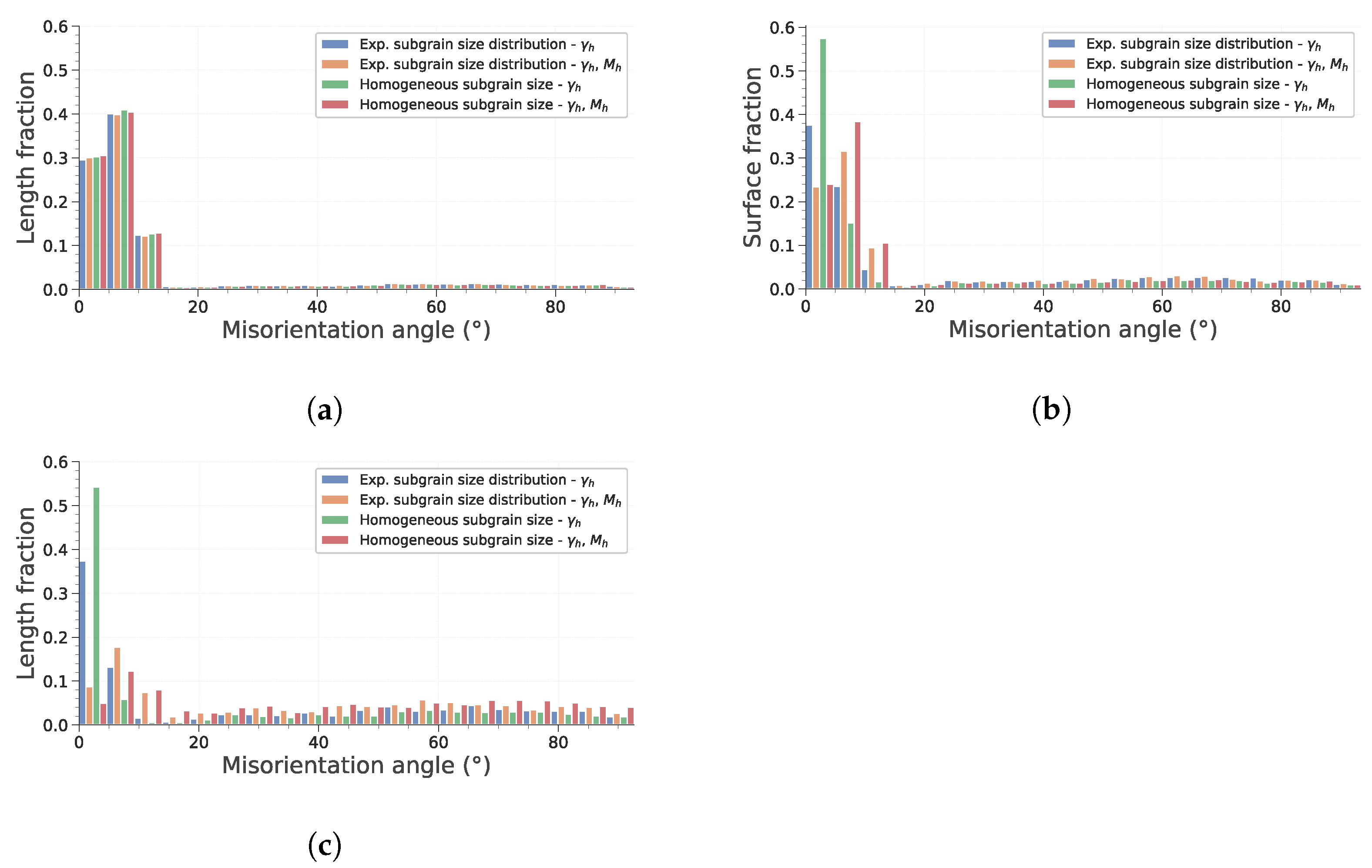
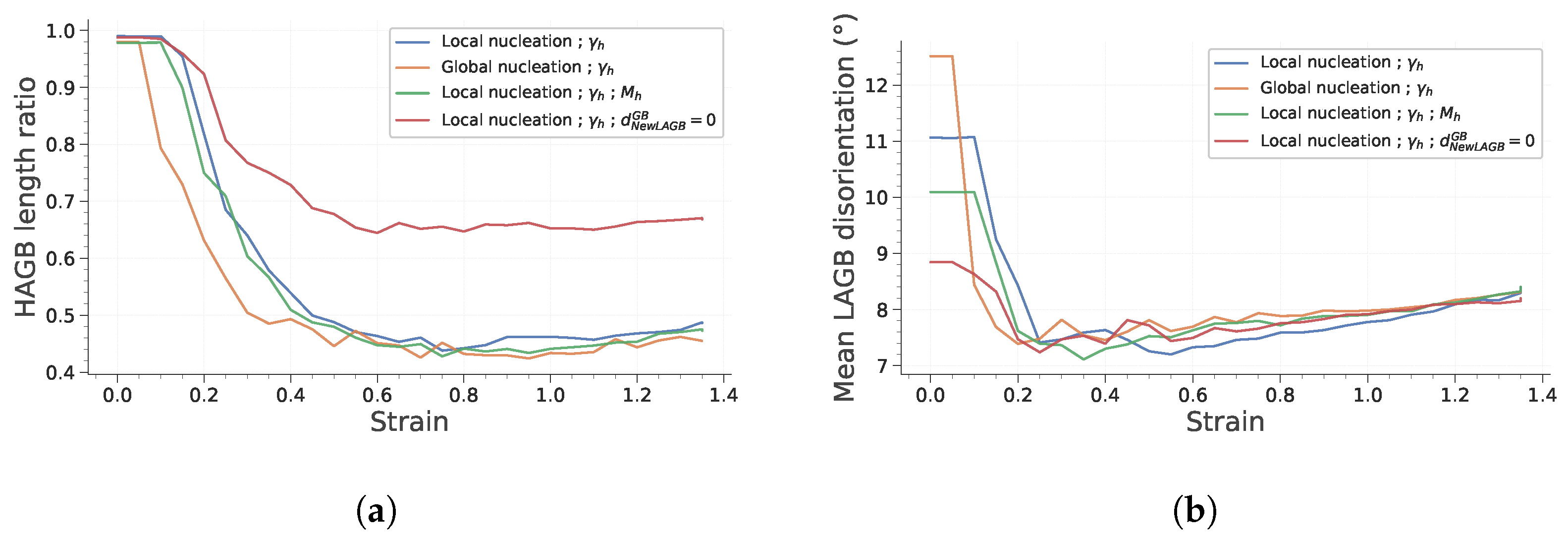
3.2.1. Evolution of Main Microstructure Descriptors during CDRX and PDRX
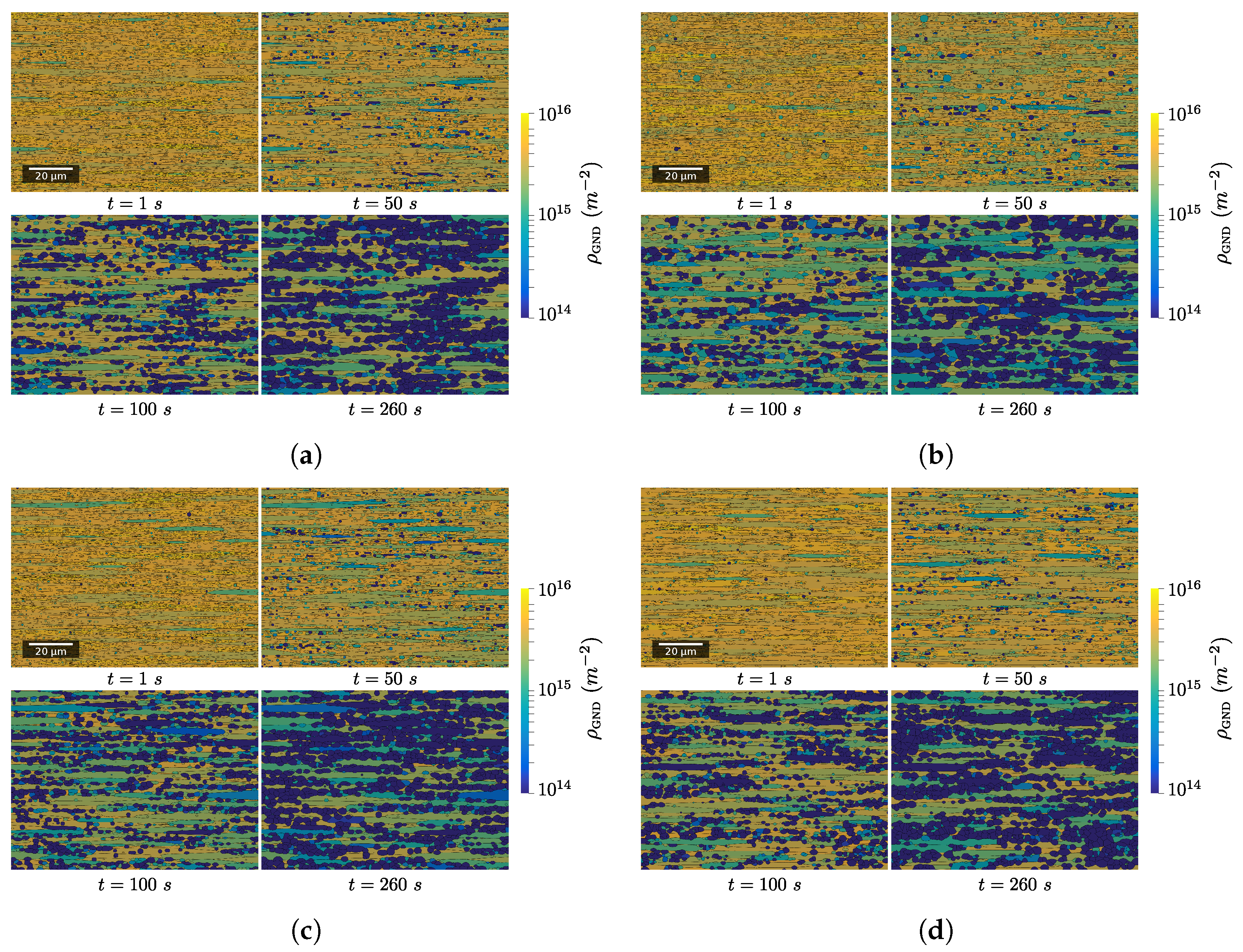
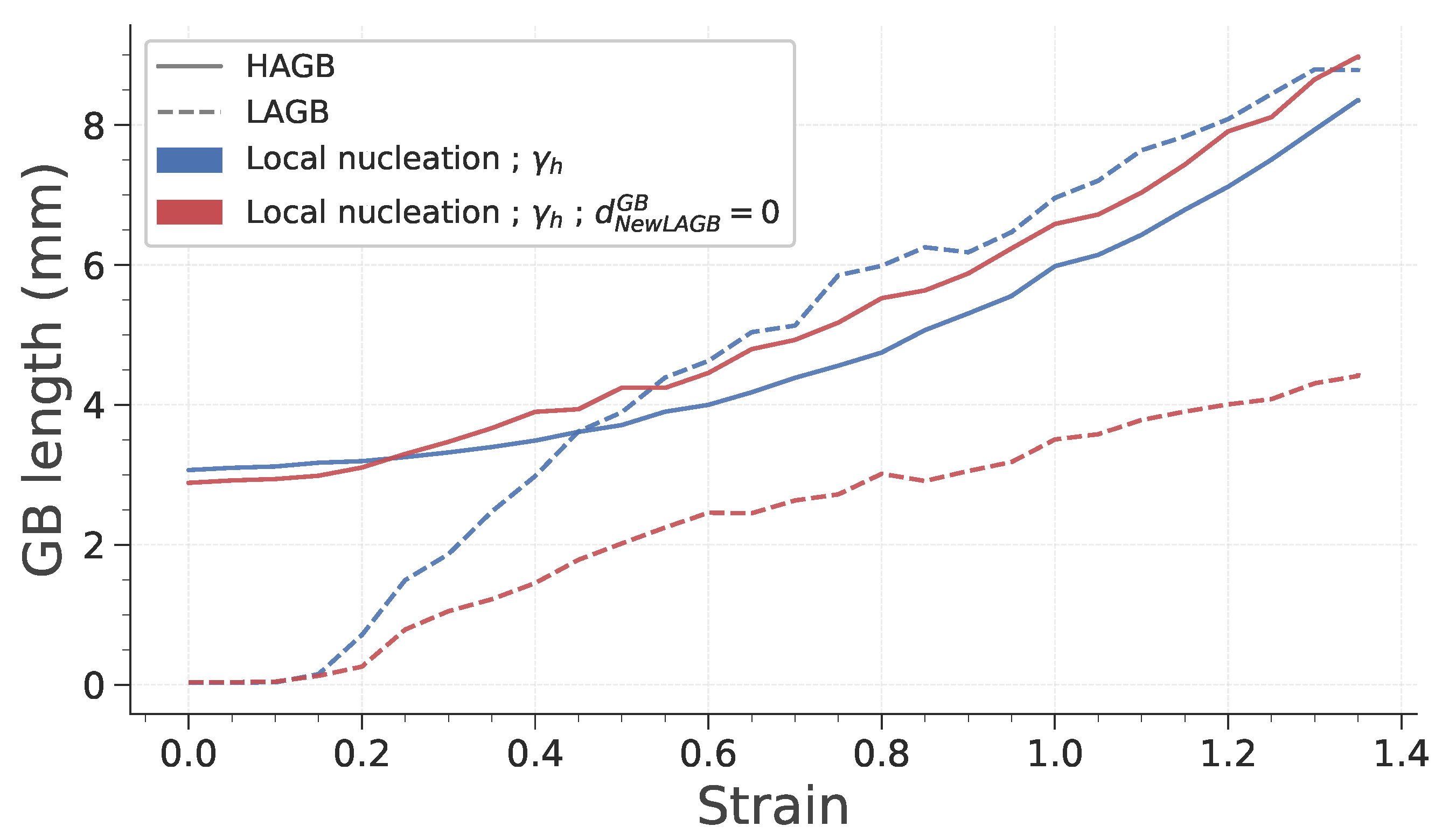
3.2.2. Evolution of the Subgrain Network during Deformation
4. Conclusions
- The influence of the microstructure topology;
- The significance of the definition of LAGB and subgrain properties. Indeed, it appeared that the strategy adopted by the microstructure to reduce the total system energy is different depending on those parameters. The observations have led to the deduction that heterogeneous mobility encourages a temporary increase in HAGB length to accelerate the general energy decrease. They also illustrated that preferential subgrain growth is much more significant if subgrains all have initially the same size;
- The fact that internal dislocation density has a non-negligible impact.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
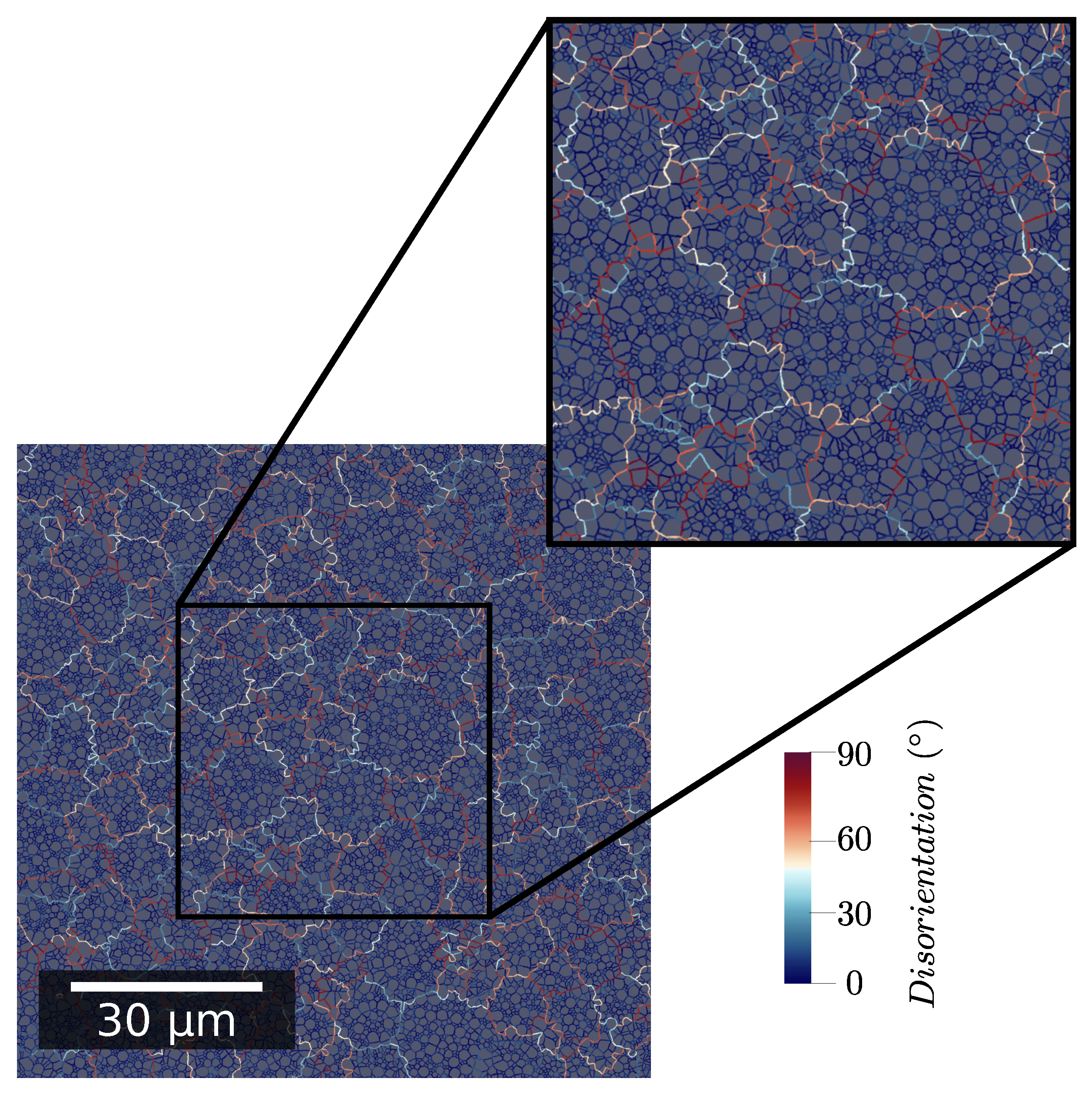
Appendix A. Evolution of the GB Network in the Whole Simulated RVE

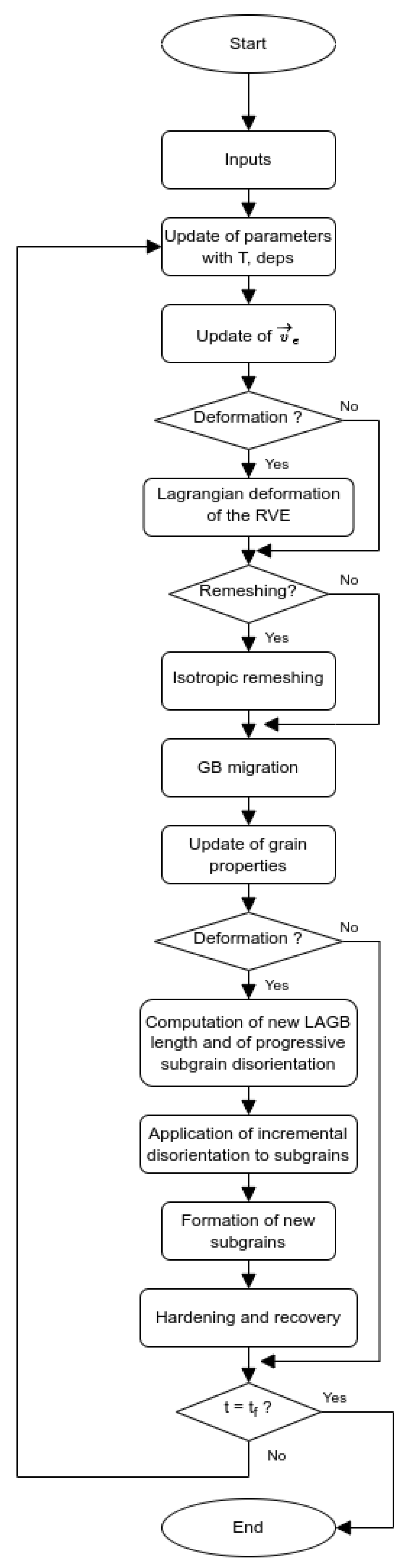
Appendix B. Description of the Algorithm Underlying the Numerical Framework
References
- Rollett, A.; Rohrer, G.S.; Humphreys, J. Recrystallization and Related Annealing Phenomena; Elsevier: Amsterdam, The Netherland, 2017. [Google Scholar]
- Doherty, R.; Hughes, D.; Humphreys, F.; Jonas, J.; Jensen, D.; Kassner, M.; King, W.; McNelley, T.; McQueen, H.; Rollett, A. Current issues in recrystallization: A review. Mater. Sci. Eng. 1997, 238, 219–274. [Google Scholar] [CrossRef]
- Huang, K.; Logé, R. A review of dynamic recrystallization phenomena in metallic materials. Mater. Des. 2016, 111, 548–574. [Google Scholar] [CrossRef]
- Sakai, T.; Belyakov, A.; Kaibyshev, R.; Miura, H.; Jonas, J.J. Dynamic and post-dynamic recrystallization under hot, cold and severe plastic deformation conditions. Prog. Mater. Sci. 2014, 60, 130–207. [Google Scholar] [CrossRef]
- Kim, S.I.; Ko, B.C.; Lee, C.M.; Hwang, S.K.; Yoo, Y.C. Evolution of dynamic recrystallisation in AISI 304 stainless steel. Mater. Sci. Technol. 2003, 19, 1648–1652. [Google Scholar] [CrossRef]
- Wang, M.; Sun, C.; Fu, M.; Liu, Z.; Wang, C. Experimental investigations and constitutive modeling of the dynamic recrystallization behavior of Inconel 740 superalloy. Mater. Sci. Eng. 2020, 793, 139939. [Google Scholar] [CrossRef]
- Hallberg, H. Approaches to Modeling of Recrystallization. Metals 2011, 1, 16–48. [Google Scholar] [CrossRef]
- Hallberg, H.; Wallin, M.; Ristinmaa, M. Simulation of discontinuous dynamic recrystallization in pure Cu using a probabilistic cellular automaton. Comput. Mater. Sci. 2010, 49, 25–34. [Google Scholar] [CrossRef]
- Beltran, O.; Huang, K.; Logé, R. A mean field model of dynamic and post-dynamic recrystallization predicting kinetics, grain size and flow stress. Comput. Mater. Sci. 2015, 102, 293–303. [Google Scholar] [CrossRef]
- Maire, L.; Scholtes, B.; Moussa, C.; Bozzolo, N.; Muñoz, D.P.; Settefrati, A.; Bernacki, M. Modeling of dynamic and post-dynamic recrystallization by coupling a full field approach to phenomenological laws. Mater. Des. 2017, 133, 498–519. [Google Scholar] [CrossRef]
- Souza, P.M.; Hodgson, P.D.; Rolfe, B.; Singh, R.P.; Beladi, H. Effect of initial microstructure and beta phase evolution on dynamic recrystallization behaviour of Ti6Al4V alloy—An EBSD based investigation. J. Alloy. Compd. 2019, 793, 467–479. [Google Scholar] [CrossRef]
- Liu, S.; Kent, D.; Zhan, H.; Doan, N.; Wang, C.; Yu, S.; Dargusch, M.; Wang, G. Influence of strain rate and crystallographic orientation on dynamic recrystallization of pure Zn during room-temperature compression. J. Mater. Sci. Technol. 2021, 86, 237–250. [Google Scholar] [CrossRef]
- Qiang, F.; Bouzy, E.; Kou, H.; Zhang, Y.; Wang, L.; Li, J. Grain fragmentation associated continuous dynamic recrystallization (CDRX) of hexagonal structure during uniaxial isothermal compression: High-temperature α phase in TiAl alloys. Intermetallics 2021, 129, 107028. [Google Scholar] [CrossRef]
- Gourdet, S.; Montheillet, F. A model of continuous dynamic recrystallization. Acta Mater. 2003, 51, 2685–2699. [Google Scholar] [CrossRef]
- Buzolin, R.; Canelo-Yubero, D.; Warchomicka, F.; Lasnik, M.; Krumphals, A.; Poletti, M. Refinement of the Ti-17 microstructure after hot deformation: Coupled mesoscale model. Mater. Sci. Eng. 2021, 800, 140268. [Google Scholar] [CrossRef]
- Maizza, G.; Pero, R.; Richetta, M.; Montanari, R. Continuous dynamic recrystallization (CDRX) model for aluminum alloys. J. Mater. Sci. 2018, 53, 4563–4573. [Google Scholar] [CrossRef]
- Li, Y.; Gu, B.; Jiang, S.; Liu, Y.; Shi, Z.; Lin, J. A CDRX-based material model for hot deformation of aluminium alloys. Int. J. Plast. 2020, 134, 102844. [Google Scholar] [CrossRef]
- Bernacki, M.; Logé, R.; Coupez, T. Level set framework for the finite-element modelling of recrystallization and grain growth in polycrystalline materials. Scr. Mater. 2011, 64, 525–528. [Google Scholar] [CrossRef]
- Fausty, J.; Bozzolo, N.; Pino Muñoz, D.; Bernacki, M. A novel level-set finite element formulation for grain growth with heterogeneous grain boundary energies. Mater. Des. 2018, 160, 578–590. [Google Scholar] [CrossRef]
- Murgas, B.; Florez, S.; Bozzolo, N.; Fausty, J.; Bernacki, M. Comparative Study and Limits of Different Level-Set Formulations for the Modeling of Anisotropic Grain Growth. Materials 2021, 14, 3883. [Google Scholar] [CrossRef]
- Scholtes, B.; Shakoor, M.; Settefrati, A.; Bouchard, P.O.; Bozzolo, N.; Bernacki, M. New finite element developments for the full field modeling of microstructural evolutions using the level-set method. Comput. Mater. Sci. 2015, 109, 388–398. [Google Scholar] [CrossRef]
- Bernacki, M.; Chastel, Y.; Coupez, T.; Logé, R. Level set framework for the numerical modelling of primary recrystallization in polycrystalline materials. Scr. Mater. 2008, 58, 1129–1132. [Google Scholar] [CrossRef]
- Dieter, G.E.; Bacon, D.J. Mechanical Metallurgy; McGraw-hill: New York, NY, USA, 1976; Volume 3. [Google Scholar]
- Fausty, J.; Bozzolo, N.; Bernacki, M. A 2D level set finite element grain coarsening study with heterogeneous grain boundary energies. Appl. Math. Model. 2020, 78, 505–518. [Google Scholar] [CrossRef]
- Florez, S.; Alvarado, K.; Murgas, B.; Bozzolo, N.; Chatain, D.; Krill, C.E.; Wang, M.; Rohrer, G.S.; Bernacki, M. Statistical behaviour of interfaces subjected to curvature flow and torque effects applied to microstructural evolutions. Acta Mater. 2022, 222, 117459. [Google Scholar] [CrossRef]
- Hitti, K.; Laure, P.; Coupez, T.; Silva, L.; Bernacki, M. Precise generation of complex statistical Representative Volume Elements (RVEs) in a finite element context. Comput. Mater. Sci. 2012, 61, 224–238. [Google Scholar] [CrossRef]
- Hitti, K.; Bernacki, M. Optimized Dropping and Rolling (ODR) method for packing of poly-disperse spheres. Appl. Math. Model. 2013, 37, 5715–5722. [Google Scholar] [CrossRef]
- Després, A.; Greenwood, M.; Sinclair, C. A mean-field model of static recrystallization considering orientation spreads and their time-evolution. Acta Mater. 2020, 199, 116–128. [Google Scholar] [CrossRef]
- Holm, E.; Miodownik, M.; Rollett, A. On abnormal subgrain growth and the origin of recrystallization nuclei. Acta Mater. 2003, 51, 2701–2716. [Google Scholar] [CrossRef]
- Suwa, Y.; Saito, Y.; Onodera, H. Phase-field simulation of recrystallization based on the unified subgrain growth theory. Comput. Mater. Sci. 2008, 44, 286–295. [Google Scholar] [CrossRef]
- Laasraoui, A.; Jonas, J. Prediction of steel flow stresses at high temperatures and strain rates. Metall. Trans. 1991, 22, 1545–1558. [Google Scholar] [CrossRef]
- Bernacki, M.; Resk, H.; Coupez, T.; Logé, R. Finite element model of primary recrystallization in polycrystalline aggregates using a level set framework. Model. Simul. Mater. Sci. Eng. 2009, 17, 064006. [Google Scholar] [CrossRef]
- Roux, E.; Bernacki, M.; Bouchard, P. A level-set and anisotropic adaptive remeshing strategy for the modeling of void growth under large plastic strain. Comput. Mater. Sci. 2013, 68, 32–46. [Google Scholar] [CrossRef]
- Read, W.T.; Shockley, W. Dislocation Models of Crystal Grain Boundaries. Phys. Rev. 1950, 78, 275–289. [Google Scholar] [CrossRef]
- Huang, Y.; Humphreys, F. Subgrain growth and low angle boundary mobility in aluminium crystals of orientation 110 <001>. Acta Mater. 2000, 48, 2017–2030. [Google Scholar] [CrossRef]
- Carneiro, I.; Simões, S. Recent Advances in EBSD Characterization of Metals. Metals 2020, 10, 1097. [Google Scholar] [CrossRef]
- Gaillac, A.; Barberis, P. Numerical modeling of zirconium alloys hot extrusion. In Proceedings of the 18th International Symposium on Zirconium in the Nuclear Industry, ASTM STP1597, Hilton Head, South Carolina, 15–19 May 2016; pp. 127–150. [Google Scholar]
- Chauvy, C.; Barberis, P.; Montheillet, F. Microstructure transformation during warm working of β-treated lamellar Zircaloy-4 within the upper α-range. Mater. Sci. Eng. 2006, 431, 59–67. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grand, V.; Flipon, B.; Gaillac, A.; Bernacki, M. Simulation of Continuous Dynamic Recrystallization Using a Level-Set Method. Materials 2022, 15, 8547. https://doi.org/10.3390/ma15238547
Grand V, Flipon B, Gaillac A, Bernacki M. Simulation of Continuous Dynamic Recrystallization Using a Level-Set Method. Materials. 2022; 15(23):8547. https://doi.org/10.3390/ma15238547
Chicago/Turabian StyleGrand, Victor, Baptiste Flipon, Alexis Gaillac, and Marc Bernacki. 2022. "Simulation of Continuous Dynamic Recrystallization Using a Level-Set Method" Materials 15, no. 23: 8547. https://doi.org/10.3390/ma15238547
APA StyleGrand, V., Flipon, B., Gaillac, A., & Bernacki, M. (2022). Simulation of Continuous Dynamic Recrystallization Using a Level-Set Method. Materials, 15(23), 8547. https://doi.org/10.3390/ma15238547





