Influences of Separator Thickness and Surface Coating on Lithium Dendrite Growth: A Phase-Field Study
Abstract
1. Introduction
2. Methods
3. Results and Discussion
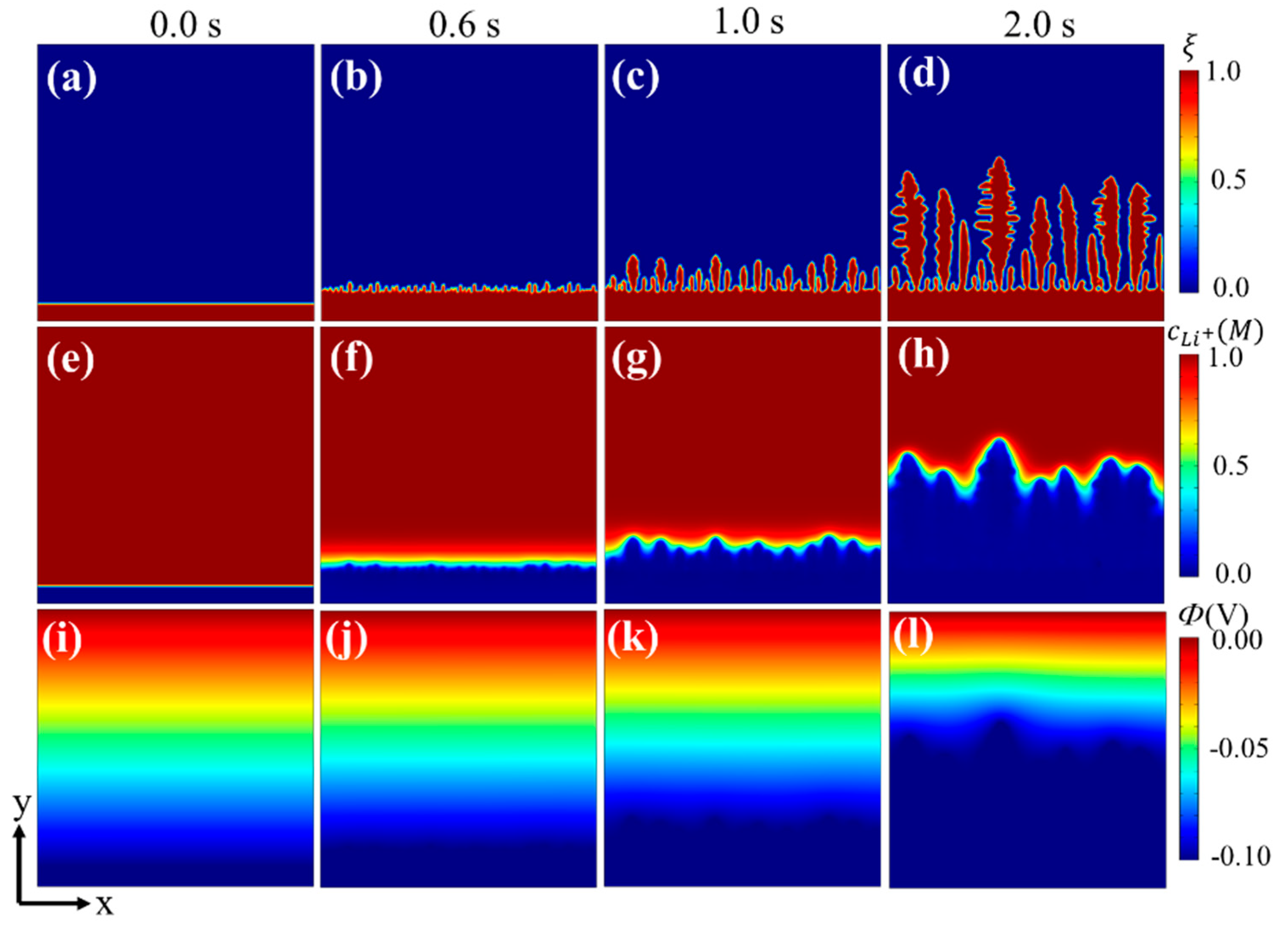
3.1. Li Dendrite Growth in Batteries without Separators
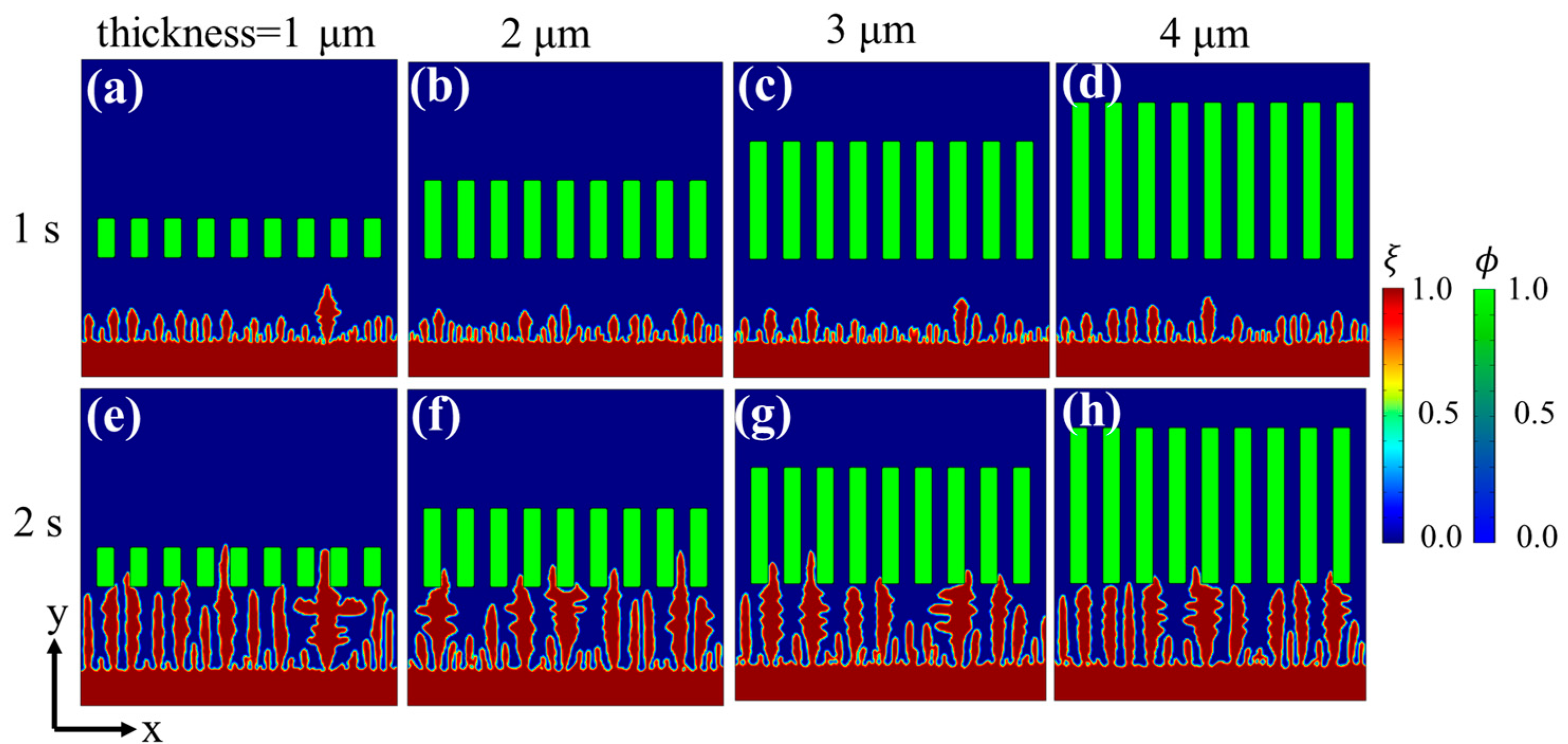
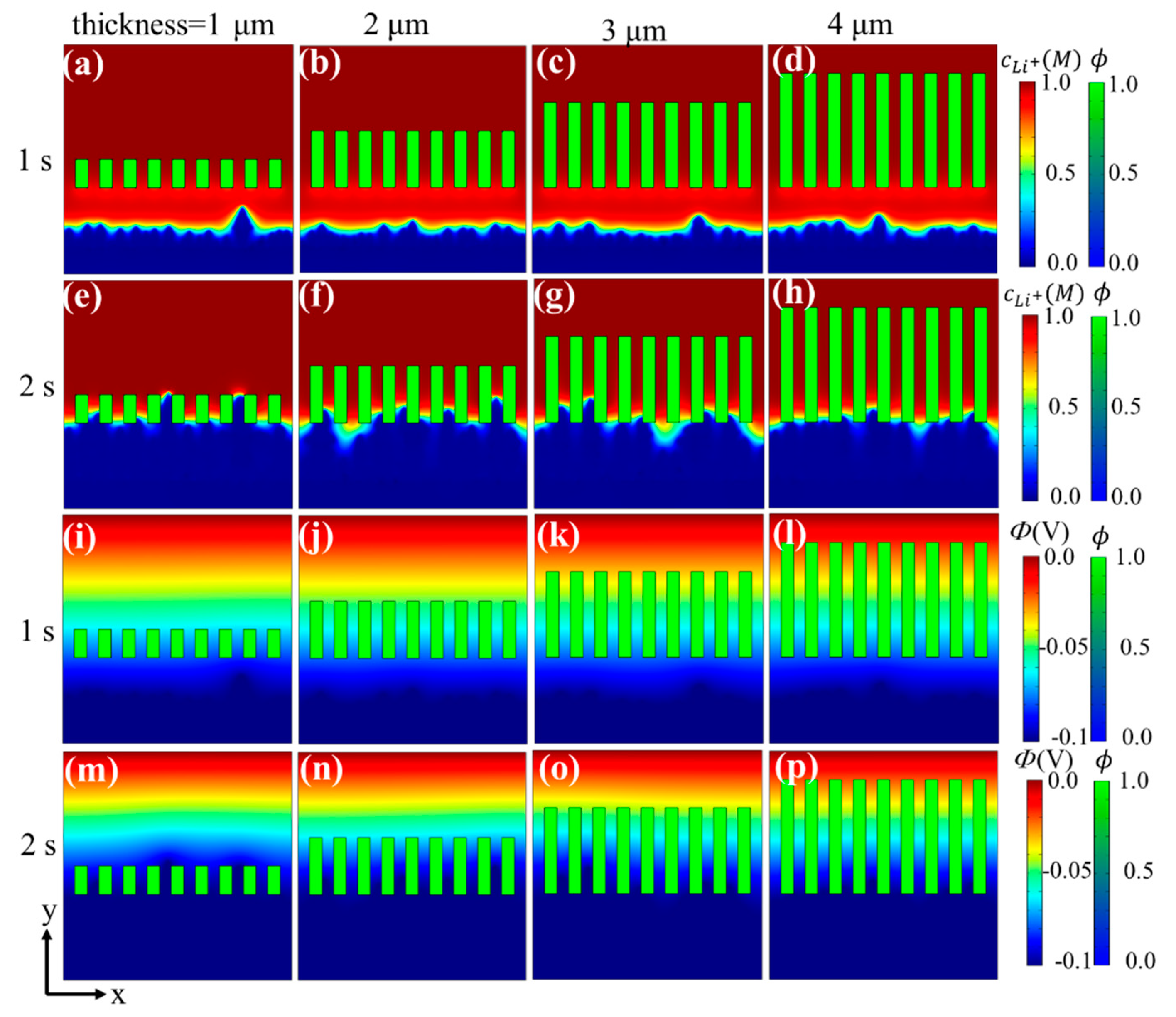
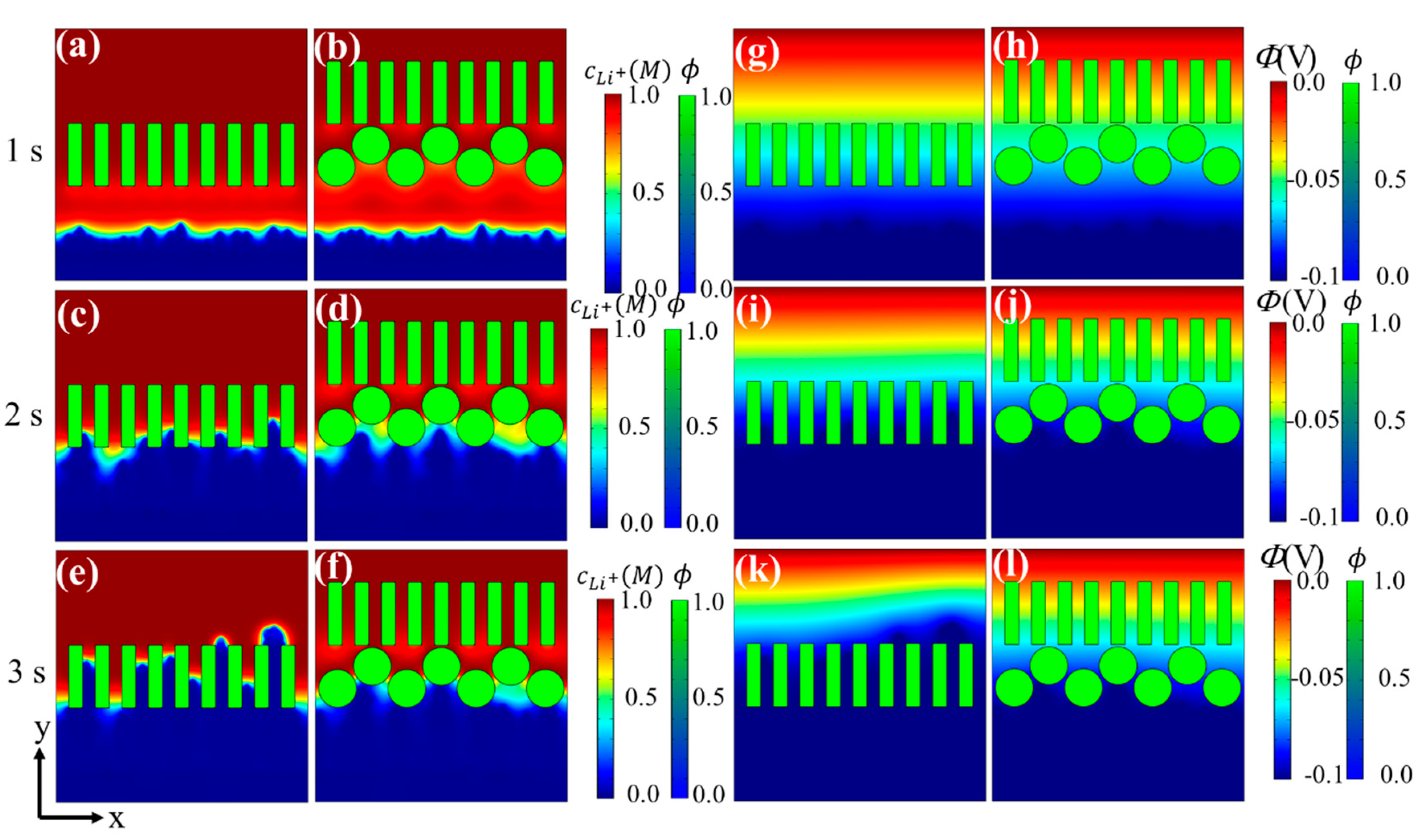
3.2. Effects of Separator Thickness on Li Dendrite Growth
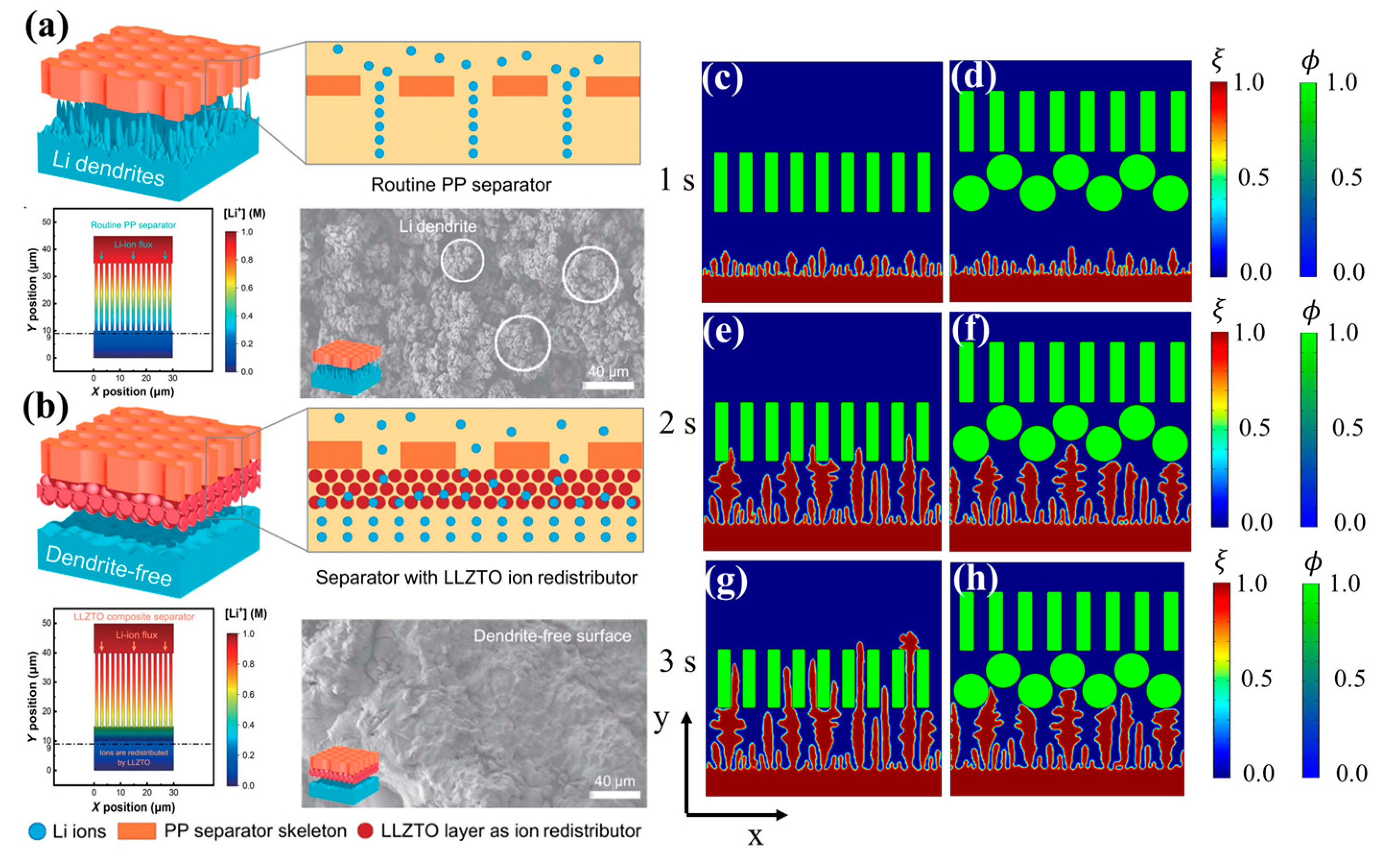
3.3. Effects of Surface Coating on Li Dendrite Growth
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, F.; Yuan, Y.-X.; Cheng, X.-B.; Bai, Y.; Li, Y.; Wu, C.; Zhang, Q. Perspectives for restraining harsh lithium dendrite growth: Towards robust lithium metal anodes. Energy Storage Mater. 2018, 15, 148–170. [Google Scholar] [CrossRef]
- Pang, Q.; Liang, X.; Shyamsunder, A.; Nazar, L.F. An in vivo formed solid electrolyte surface layer enables stable plating of Li metal. Joule 2017, 1, 871–886. [Google Scholar] [CrossRef]
- Mendizabal, A.O.; Gomez, N.; Aguesse, F.; López-Aranguren, P. Designing Spinel Li4Ti5O12 Electrode as Anode Material for Poly(ethylene) oxide-Based Solid-State Batteries. Materials 2021, 14, 1213. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Wang, C.; Pastel, G.; Kuang, Y.; Xie, H.; Li, Y.; Liu, B.; Luo, W.; Chen, C.; Hu, L. 3D Wettable Framework for Dendrite-Free Alkali Metal Anodes. Adv. Energy Mater. 2018, 8, 1800635. [Google Scholar] [CrossRef]
- Zheng, Z.-J.; Su, Q.; Zhang, Q.; Hu, X.-C.; Yin, Y.-X.; Wen, R.; Ye, H.; Wang, Z.-B.; Guo, Y.-G. Low volume change composite lithium metal anodes. Nano Energy 2019, 64, 103910. [Google Scholar] [CrossRef]
- Wang, X.; Zhai, H.; Qie, B.; Cheng, Q.; Li, A.; Borovilas, J.; Xu, B.; Shi, C.; Jin, T.; Liao, X. Rechargeable solid-state lithium metal batteries with vertically aligned ceramic nanoparticle/polymer composite electrolyte. Nano Energy 2019, 60, 205–212. [Google Scholar] [CrossRef]
- Akkinepally, B.; Reddy, I.N.; Ko, T.J.; Yoo, K.; Shim, J. Dopant effect on Li+ ion transport in NASICON-type solid electrolyte: Insights from molecular dynamics simulations and experiments. Ceram. Int. 2022, 48, 12142–12151. [Google Scholar] [CrossRef]
- Lin, D.; Zhuo, D.; Liu, Y.; Cui, Y. All-integrated bifunctional separator for Li dendrite detection via novel solution synthesis of a thermostable polyimide separator. J. Am. Chem. Soc. 2016, 138, 11044–11050. [Google Scholar] [CrossRef]
- Chen, M.; Shao, M.; Jin, J.; Cui, L.; Tu, H.; Fu, X. Configurational and structural design of separators toward shuttling-free and dendrite-free lithium-sulfur batteries: A review. Energy Storage Mater. 2022, 47, 629–648. [Google Scholar] [CrossRef]
- Shi, F.; Pei, A.; Vailionis, A.; Xie, J.; Liu, B.; Zhao, J.; Gong, Y.; Cui, Y. Strong texturing of lithium metal in batteries. Proc. Natl. Acad. Sci. USA 2017, 114, 12138–12143. [Google Scholar] [CrossRef]
- Sreedeep, S.; Natarajan, S.; Aravindan, V. Recent advancements in LiCoPO4 cathodes using electrolyte additives. Curr. Opin. Electrochem. 2022, 31, 100868. [Google Scholar] [CrossRef]
- Ren, W.; Zheng, Y.; Cui, Z.; Tao, Y.; Li, B.; Wang, W. Recent progress of functional separators in dendrite inhibition for lithium metal batteries. Energy Storage Mater. 2021, 35, 157–168. [Google Scholar] [CrossRef]
- Ding, F.; Xu, W.; Graff, G.L.; Zhang, J.; Sushko, M.L.; Chen, X.; Shao, Y.; Engelhard, M.H.; Nie, Z.; Xiao, J. Dendrite-free lithium deposition via self-healing electrostatic shield mechanism. J. Am. Chem. Soc. 2013, 135, 4450–4456. [Google Scholar] [CrossRef] [PubMed]
- Zhang, S.S.; Fan, X.; Wang, C. Preventing lithium dendrite-related electrical shorting in rechargeable batteries by coating separator with a Li-killing additive. J. Mater. Chem. A 2018, 6, 10755–10760. [Google Scholar] [CrossRef]
- Kim, P.J.H.; Pol, V.G. Surface functionalization of a conventional polypropylene separator with an aluminum nitride layer toward ultrastable and high-rate lithium metal anodes. ACS Appl. Mater. Interfaces 2019, 11, 3917–3924. [Google Scholar] [CrossRef] [PubMed]
- Ma, L.; Chen, R.; Hu, Y.; Zhang, W.; Zhu, G.; Zhao, P.; Chen, T.; Wang, C.; Yan, W.; Wang, Y. Nanoporous and lyophilic battery separator from regenerated eggshell membrane with effective suppression of dendritic lithium growth. Energy Storage Mater. 2018, 14, 258–266. [Google Scholar] [CrossRef]
- Shin, W.-K.; Kannan, A.G.; Kim, D.-W. Effective suppression of dendritic lithium growth using an ultrathin coating of nitrogen and sulfur codoped graphene nanosheets on polymer separator for lithium metal batteries. ACS Appl. Mater. Interfaces 2015, 7, 23700–23707. [Google Scholar] [CrossRef]
- Wang, Q.; Zhang, G.; Li, Y.; Hong, Z.; Wang, D.; Shi, S. Application of phase-field method in rechargeable batteries. NPJ Comput. Mater. 2020, 6, 1–8. [Google Scholar] [CrossRef]
- Chen, L.-Q. Phase-field models for microstructure evolution. Annu. Rev. Mater. Res. 2002, 32, 113–140. [Google Scholar] [CrossRef]
- Jana, A.; Ely, D.R.; García, R.E. Dendrite-separator interactions in lithium-based batteries. J. Power Sources 2015, 275, 912–921. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, G.; Chen, B.; Zhao, W.; Sha, L.; Wang, D.; Yu, J.; Shi, S. Understanding the separator pore size inhibition effect on lithium dendrite via phase-field simulations. Chin. Chem. Lett. 2022, 33, 3287–3290. [Google Scholar] [CrossRef]
- Chen, L.; Zhang, H.W.; Liang, L.Y.; Liu, Z.; Qi, Y.; Lu, P.; Chen, J.; Chen, L.-Q. Modulation of dendritic patterns during electrodeposition: A nonlinear phase-field model. J. Power Sources 2015, 300, 376–385. [Google Scholar] [CrossRef]
- Hong, Z.; Viswanathan, V. Phase-field simulations of lithium dendrite growth with open-source software. ACS Energy Lett. 2018, 3, 1737–1743. [Google Scholar] [CrossRef]
- Yu, B.-C.; Park, K.; Jang, J.-H.; Goodenough, J.B. Cellulose-based porous membrane for suppressing Li dendrite formation in lithium–sulfur battery. ACS Energy Lett. 2016, 1, 633–637. [Google Scholar] [CrossRef]
- Zhao, C.-Z.; Chen, P.-Y.; Zhang, R.; Chen, X.; Li, B.-Q.; Zhang, X.-Q.; Cheng, X.-B.; Zhang, Q. An ion redistributor for dendrite-free lithium metal anodes. Sci. Adv. 2018, 4, eaat3446. [Google Scholar] [CrossRef] [PubMed]
| Parameters | Symbol | Value |
|---|---|---|
| Interfacial mobility 1 | ||
| Interfacial mobility 2 | ||
| Reaction constant | ||
| Gradient energy coefficient 1 | ||
| Gradient energy coefficient 2 | ||
| Anisotropic strength | ||
| Anisotropic mode number | ||
| Barrier height 1 | ||
| Barrier height 2 | ||
| Barrier height 3 | ||
| Diffusion coefficient of Li+ | ||
| Li+ concentration of electrolyte | ||
| Charge transfer coefficient | ||
| Electric conductivity of electrode | ||
| Electric conductivity of electrolyte | ||
| Accumulation constant | ||
| Temperature |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Sha, L.; Lv, P.; Qiu, N.; Zhao, W.; Chen, B.; Hu, P.; Zhang, G. Influences of Separator Thickness and Surface Coating on Lithium Dendrite Growth: A Phase-Field Study. Materials 2022, 15, 7912. https://doi.org/10.3390/ma15227912
Li Y, Sha L, Lv P, Qiu N, Zhao W, Chen B, Hu P, Zhang G. Influences of Separator Thickness and Surface Coating on Lithium Dendrite Growth: A Phase-Field Study. Materials. 2022; 15(22):7912. https://doi.org/10.3390/ma15227912
Chicago/Turabian StyleLi, Yajie, Liting Sha, Peili Lv, Na Qiu, Wei Zhao, Bin Chen, Pu Hu, and Geng Zhang. 2022. "Influences of Separator Thickness and Surface Coating on Lithium Dendrite Growth: A Phase-Field Study" Materials 15, no. 22: 7912. https://doi.org/10.3390/ma15227912
APA StyleLi, Y., Sha, L., Lv, P., Qiu, N., Zhao, W., Chen, B., Hu, P., & Zhang, G. (2022). Influences of Separator Thickness and Surface Coating on Lithium Dendrite Growth: A Phase-Field Study. Materials, 15(22), 7912. https://doi.org/10.3390/ma15227912






