Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core
Abstract
1. Introduction
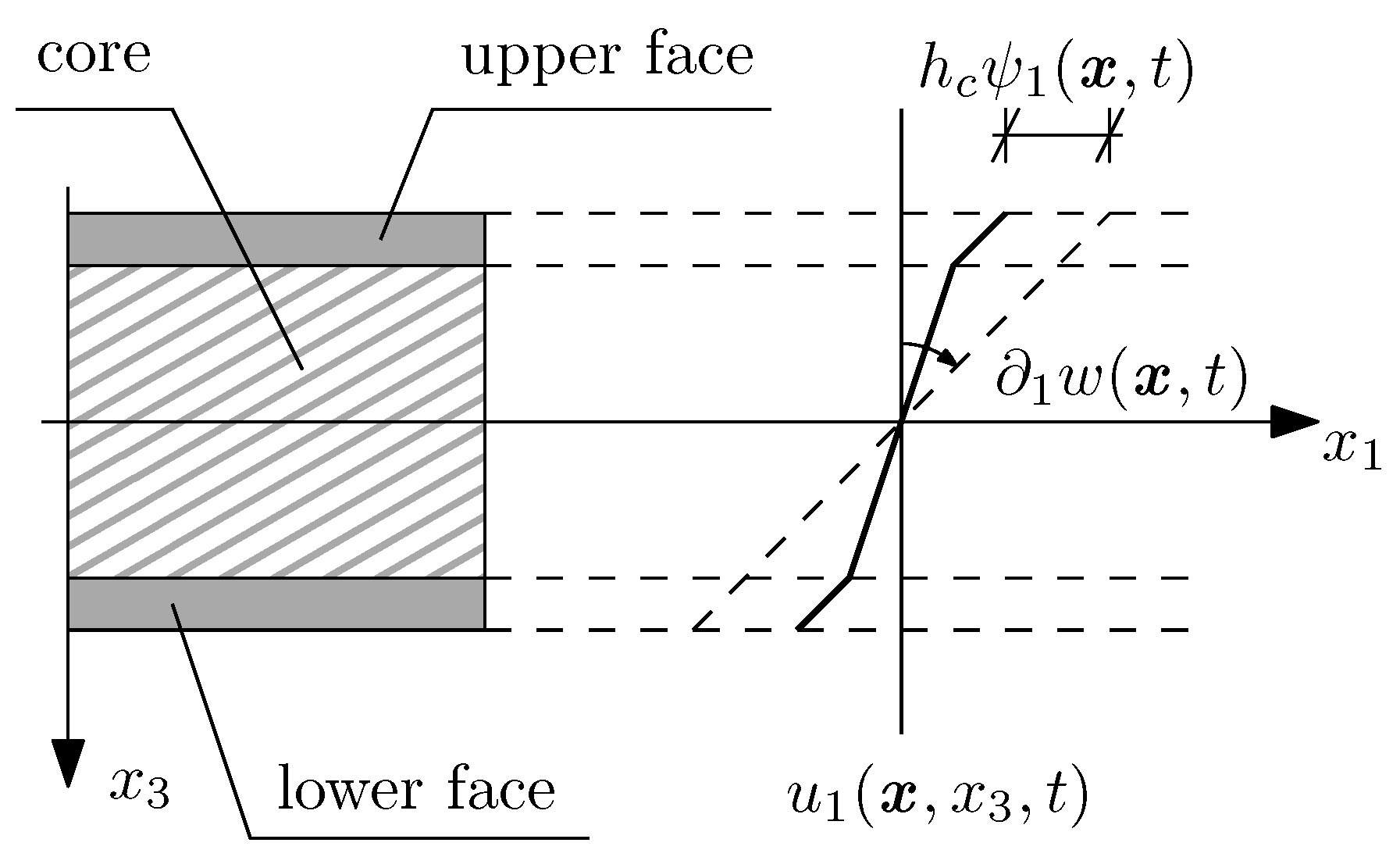
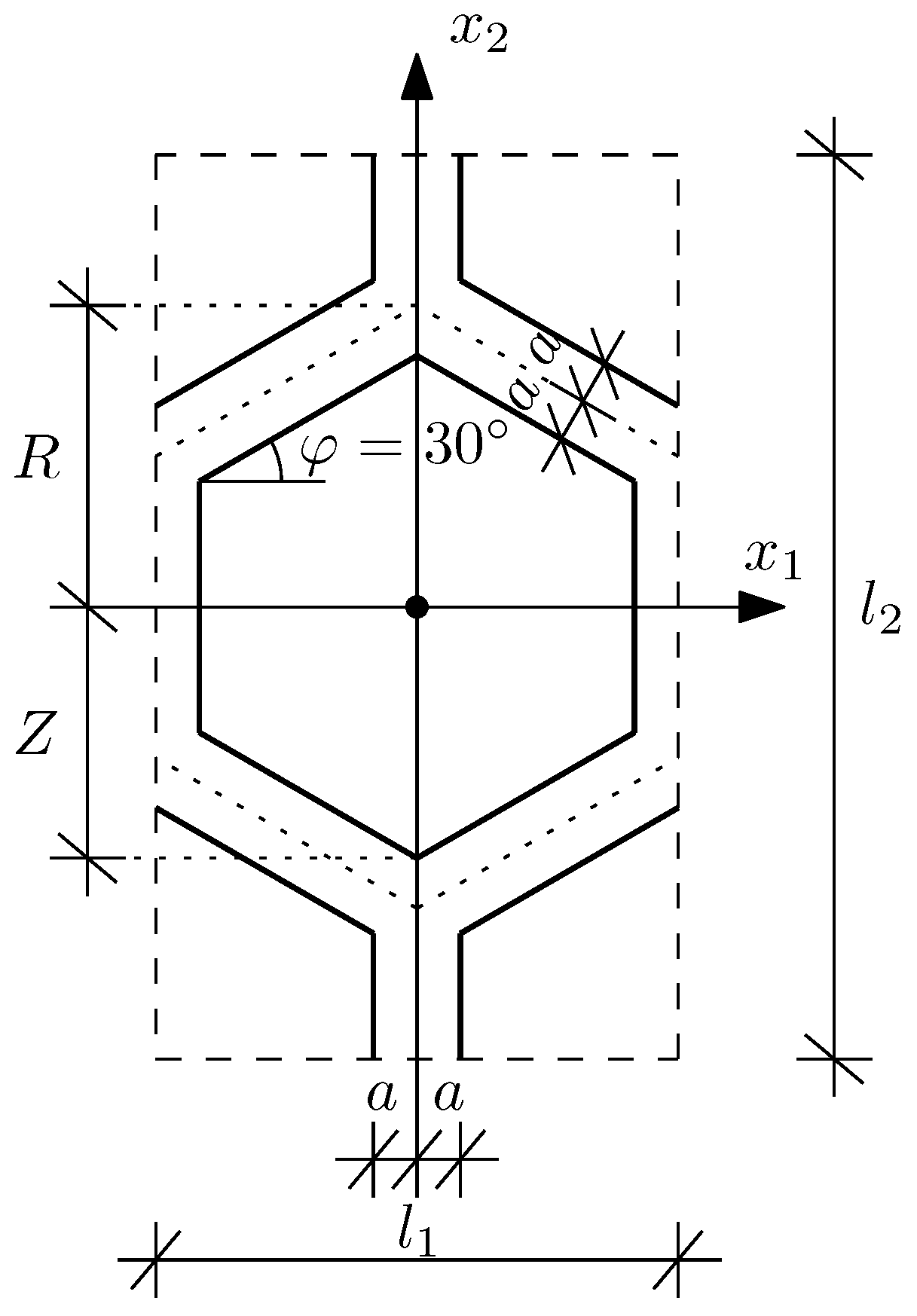
2. Modelling Foundations
3. Derivation of Initial Governing Equations
4. Basics of Tolerance Averaging Technique
- ,
- ,
- ,
- ,
5. Governing Equations of the Tolerance Model
6. Free Vibration Analysis of Sandwich Plate with Honeycomb Core
- Size I ()—
- Size II ()—
- Size III ()—
- Mode I—
- Mode II—
- Mode III—
- Mode IV—
- Mode V—
- Mode VI—
- The relative errors between the results tend to decrease as the parameter a (representing the thickness of the walls of honeycomb in the periodicity cell) raises. It should be noticed that the initially assumed in-plane displacement field, cf. (1), is dedicated to sandwich structures with cores filling the whole available space between faces. In the case of ’thick’ honeycomb core, characterised by high values of parameter a, this assumption is still applicable, which results in generally satisfactory convergence of results. In the case of ’thin’ honeycomb, this assumption is slowly corrupting. As a consequence, the relative errors between averaged solution and FEM can reach up to 25% (cf. ).
- There are significant differences in the results of the averaged models in Case I and Case II. The only difference between those cases is a set of fluctuation shape functions. It can be noticed that for structures with lower thickness of the core , the assumption of negligibly small fluctuations of vertical displacements (Case II) is applicable. Hence, it produces results, which in general are convergent with the FEM analysis. Meanwhile, for higher values of the thickness , it seems that the fluctuations of vertical displacements are more influential, hence they cannot be neglected (Case I).
- In order to obtain precise results within the averaged model, the considered structure should be made of a sufficiently large quantity of periodicity cells. It can be noticed that for relatively small periodic structures (), the convergence of results in Case II is usually worse than in the case of larger structures (). It can be caused by boundary conditions, which produce disturbances in displacement fields on a considerable span of the plate. A similar remark can be made for Case I, excluding structures with a low thickness of the core , for which such a set of fluctuation shape functions is not applicable.
- Another reason for lower accuracy of results in the case of small periodic structures () can be the issue of slowly varying functions. Based on the definitions, a slowly varying function is a function, which is ’almost’ constant on any periodicity cell, with respect to a certain tolerance parameter . In the case of small periodic structures, this assumption can be satisfied only for higher values of parameter , which results in lower accuracy of the proposed solution.
- It can be noticed that in several cases the discrepancies in the results between the averaged model and FEM analysis tend to raise higher modes of vibrations. The reason for this phenomenon can be also connected with difficulties in satisfying the condition of nearly constant values of slowly varying functions on a basic periodicity cell. In the case of higher modes of vibrations, this condition must yield higher values of tolerance parameter and, consequently, lower accuracy of the obtained results. Nevertheless, with a properly adjusted fluctuation shape functions, the averaged model can be used to estimate several basic free vibration frequencies of any of the analysed sandwich structures.
7. Final Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Tewari, K.; Pandit, M.; Budarapu, P.; Natarajan, S. Analysis of sandwich structures with corrugated and spiderweb-inspired cores for aerospace applications. Thin-Walled Struct. 2022, 180, 109812. [Google Scholar] [CrossRef]
- Kheyabani, A.; Massarwa, E.; Kefal, A. Multiscale structural analysis of thick sandwich structures using parametric HFGMC micromechanics and isogeometric plate formulation based on refined zigzag theory. Compos. Struct. 2022, 297, 115988. [Google Scholar] [CrossRef]
- Chen, Y.; Ma, H.; Li, A.; Fang, H.; Liu, Y.; Li, H. Hydroelastic analysis of double-segment floating sandwich structures under wave action. Ocean Eng. 2022, 260, 111993. [Google Scholar] [CrossRef]
- Deng, Y.; Zhou, N.; Li, X.; Wang, X.; Wei, G.; Jia, H. Dynamic response and failure mechanism of S-shaped CFRP foldcore sandwich structure under low-velocity impact. Thin-Walled Struct. 2022, 173, 109007. [Google Scholar] [CrossRef]
- Lee, H.M.; Kim, D.H.; Kim, D.Y.; Kim, M.S.; Park, J.; Yoon, G.H. Enhancement of vibration attenuation and shock absorption in composite sandwich structures with porous foams and surface patterns. Compos. Struct. 2022, 295, 115755. [Google Scholar] [CrossRef]
- Pang, Y.; Yan, X.; Qu, J.; Wu, L. Dynamic response of polyurethane foam and fiber orthogonal corrugated sandwich structure subjected to low-velocity impact. Compos. Struct. 2022, 282, 114994. [Google Scholar] [CrossRef]
- Alanbay, B.; Batra, R. Optimization of blast mitigating sandwich structures with fiber-reinforced face sheets and PVC foam layers as core. Thin-Walled Struct. 2022, 179, 109721. [Google Scholar] [CrossRef]
- Hedayati, R.; Yousefi, A.; Bodaghi, M. Sandwich structures with repairable cores based on truncated cube cells. Compos. Part B Eng. 2022, 243, 110124. [Google Scholar] [CrossRef]
- Hu, H.; Belouettar, S.; Potier-Ferry, M.; Daya, E.M. Review and assessment of various theories for modeling sandwich composites. Compos. Struct. 2008, 84, 282–292. [Google Scholar] [CrossRef]
- Hu, H.; Belouettar, S.; Potier-Ferry, M.; Makradi, A.; Koutsawa, Y. Assessment of various kinematic models for instability analysis of sandwich beams. Eng. Struct. 2011, 33, 572–579. [Google Scholar] [CrossRef]
- Carrera, E.; Brischetto, S. A survey with numerical assessment of classical and refined theories for the analysis of sandwich plates. Appl. Mech. Rev. 2009, 62, 1–17. [Google Scholar] [CrossRef]
- Iurlaro, L.; Gherlone, M.; Di Sciuva, M.; Tessler, A. Assessment of the Refined Zigzag Theory for bending, vibration, and buckling of sandwich plates: A comparative study of different theories. Compos. Struct. 2013, 106, 777–792. [Google Scholar] [CrossRef]
- Al-Furjan, M.; Shan, L.; Shen, X.; Kolahchi, R.; Rajak, D.K. Combination of FEM-DQM for nonlinear mechanics of porous GPL-reinforced sandwich nanoplates based on various theories. Thin-Walled Struct. 2022, 178, 109495. [Google Scholar] [CrossRef]
- Magnucki, K.; Lewinski, J.; Far, M.; Michalak, P. Three-point bending of an expanded-tapered sandwich beam - Analytical and numerical FEM study. Mech. Res. Commun. 2020, 103, 103471. [Google Scholar] [CrossRef]
- Lu, Q.; Liu, C.; Wang, P. Band gap enhancement and vibration reduction of functionally graded sandwich metastructure beam. Compos. Struct. 2022, 292, 115650. [Google Scholar] [CrossRef]
- Xue, B.; Peng, Y.X.; Ren, S.F.; Liu, N.N.; Zhang, Q. Investigation of impact resistance performance of pyramid lattice sandwich structure based on SPH-FEM. Compos. Struct. 2021, 261, 113561. [Google Scholar] [CrossRef]
- Park, H. Investigation on low velocity impact behavior of sandwich composite and monolithic laminate plates using FEM analysis. Compos. Struct. 2019, 220, 842–846. [Google Scholar] [CrossRef]
- Ge, L.; Zheng, H.; Li, H.; Liu, B.; Su, H.; Fang, D. Compression behavior of a novel sandwich structure with bi-directional corrugated core. Thin-Walled Struct. 2021, 161, 107413. [Google Scholar] [CrossRef]
- Meng, L.; Lan, X.; Zhao, J.; Li, H.; Wang, Z.; Gao, L. Failure analysis of bio-inspired corrugated sandwich structures fabricated by laser powder bed fusion under three-point bending. Compos. Struct. 2021, 263, 113724. [Google Scholar] [CrossRef]
- Li, C.H.; Yan, J.B.; Guan, H.N. Finite element analysis on enhanced C-channel connectors in SCS sandwich composite structures. Structures 2021, 30, 818–837. [Google Scholar] [CrossRef]
- Ye, X.; Zhao, C.; He, K.; Zhou, L.; Li, X.; Wang, J. Blast behaviors of precast concrete sandwich EPS panels: FEM and theoretical analysis. Eng. Struct. 2021, 226, 111345. [Google Scholar] [CrossRef]
- Gay, D.; Hoa, S.V. Composite Materials. Design and Applications; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Allen, H.G. Analysis and Design of Structural Sandwich Panels; Pergamon Press Ltd.: London, UK, 1969. [Google Scholar]
- Malek, S.; Gibson, L. Effective elastic properties of periodic hexagonal honeycombs. Mech. Mater. 2015, 91, 226–240. [Google Scholar] [CrossRef]
- Woźniak, C. Mathematical Modelling and Analysis in Continuum Mechanics of Microstructured Media; Publishing House of Silesian University of Technology: Gliwice, Poland, 2010. [Google Scholar]
- Jędrysiak, J.; Kaźmierczak-Sobińska, M. Theoretical Analysis of Buckling for Functionally Graded Thin Plates with Microstructure Resting on an Elastic Foundation. Materials 2020, 13, 4031. [Google Scholar] [CrossRef] [PubMed]
- Marczak, J.; Jędrysiak, J. The Stability Analysis of Periodic Beams Interacting with Periodic Elastic Foundation with the Use of the Tolerance Averaging Technique. Materials 2021, 14, 5923. [Google Scholar] [CrossRef] [PubMed]
- Jędrysiak, J. Tolerance Modelling of Vibrations and Stability for Periodic Slender Visco-Elastic Beams on a Foundation with Damping. Revisiting. Materials 2020, 13, 3939. [Google Scholar] [CrossRef]
- Tomczyk, B.; Bagdasaryan, V.; Gołąbczak, M.; Litawska, A. On the modelling of stability problems for thin cylindrical shells with two-directional micro-periodic structure. Compos. Struct. 2021, 275, 114495. [Google Scholar] [CrossRef]
- Jędrysiak, J. Non-asymptotic modelling of dynamics and stability for visco-elastic periodic beams on a periodic damping foundation. Compos. Struct. 2021, 259, 113442. [Google Scholar] [CrossRef]
- Domagalski, Ł.; Świątek, M.; Jędrysiak, J. An analytical-numerical approach to vibration analysis of periodic Timoshenko beams. Compos. Struct. 2019, 211, 490–501. [Google Scholar] [CrossRef]
- Domagalski, Ł. Free and forced large amplitude vibrations of periodically inhomogeneous slender beams. Arch. Civ. Mech. Eng. 2018, 18, 1506–1519. [Google Scholar] [CrossRef]
- Domagalski, Ł. Comparison of the Natural Vibration Frequencies of Timoshenko and Bernoulli Periodic Beams. Materials 2021, 14, 7628. [Google Scholar] [CrossRef]
- Ostrowski, P.; Jędrysiak, J. Dependence of temperature fluctuations on randomized material properties in two-component periodic laminate. Compos. Struct. 2021, 257, 113171. [Google Scholar] [CrossRef]
- Wyczółkowski, R.; Bagdasaryan, V.; Tomczyk, B. Modelling of effective thermal conductivity of a packed bed of steel bars with the use of chosen literature models. Compos. Struct. 2022, 282, 115025. [Google Scholar] [CrossRef]
- Kubacka, E.; Ostrowski, P. A Finite Difference Algorithm Applied to the Averaged Equations of the Heat Conduction Issue in Biperiodic Composites—Robin Boundary Conditions. Materials 2021, 14, 6329. [Google Scholar] [CrossRef] [PubMed]
- Kubacka, E.; Ostrowski, P. Heat conduction issue in biperiodic composite using Finite Difference Method. Compos. Struct. 2021, 261, 113310. [Google Scholar] [CrossRef]
- Marczak, J. On the correctness of results of averaged models of periodic sandwich plates depending on the set of fluctuation shape functions. Compos. Struct. 2020, 244, 112269. [Google Scholar] [CrossRef]



| Mode | |||||||
|---|---|---|---|---|---|---|---|
| 100 | I | 10.7% | 16.9% | 2.0% | 8.8% | −1.7% | 5.4% |
| II | 14.1% | 20.0% | 5.6% | 12.1% | 3.5% | 10.2% | |
| III | 16.9% | 22.7% | 9.3% | 15.6% | 8.3% | 14.7% | |
| IV | 16.1% | 22.0% | 9.1% | 15.4% | 2.0% | 8.8% | |
| V | 16.8% | 22.6% | 10.5% | 16.7% | 5.7% | 12.2% | |
| VI | 18.8% | 24.5% | 14.6% | 20.5% | 10.7% | 16.9% | |
| 50 | I | 4.6% | 11.8% | −3.1% | 4.7% | −5.6% | 2.4% |
| II | 8.1% | 15.1% | −0.5% | 7.2% | −1.9% | 5.8% | |
| III | 11.7% | 18.4% | 2.9% | 10.3% | 2.2% | 9.6% | |
| IV | 11.5% | 18.2% | 3.2% | 10.6% | −3.1% | 4.8% | |
| V | 11.9% | 18.6% | 4.4% | 11.7% | −0.4% | 7.3% | |
| VI | 14.1% | 20.7% | 9.2% | 16.1% | 4.4% | 11.7% | |
| 25 | I | −1.5% | 6.6% | −6.8% | 1.7% | −8.4% | 0.2% |
| II | 1.6% | 9.4% | −5.4% | 3.0% | −5.6% | 2.8% | |
| III | 5.6% | 13.1% | −2.6% | 5.6% | −2.4% | 5.8% | |
| IV | 5.4% | 12.9% | −1.9% | 6.2% | −6.7% | 1.7% | |
| V | 5.2% | 12.7% | −1.6% | 6.5% | −5.2% | 3.1% | |
| VI | 7.3% | 14.7% | 2.6% | 10.4% | −1.8% | 6.3% | |
| Mode | |||||||
|---|---|---|---|---|---|---|---|
| 100 | I | 4.7% | 10.2% | 0.2% | 6.0% | −1.3% | 4.5% |
| II | 7.0% | 12.3% | 1.9% | 7.6% | 0.9% | 6.7% | |
| III | 9.0% | 14.3% | 3.9% | 9.4% | 4.4% | 9.9% | |
| IV | 9.5% | 14.7% | 4.1% | 9.7% | 0.1% | 5.9% | |
| V | 10.0% | 15.3% | 4.8% | 10.3% | 1.8% | 7.5% | |
| VI | 12.6% | 17.8% | 8.0% | 13.4% | 4.9% | 10.4% | |
| 50 | I | −0.3% | 6.0% | −3.7% | 2.8% | −4.7% | 1.8% |
| II | 1.7% | 7.8% | −2.6% | 3.8% | −3.1% | 3.3% | |
| III | 4.0% | 10.0% | −1.1% | 5.2% | −1.0% | 5.3% | |
| IV | 4.9% | 10.9% | −0.6% | 5.7% | −3.7% | 2.7% | |
| V | 5.3% | 11.2% | −0.2% | 6.0% | −2.7% | 3.7% | |
| VI | 8.2% | 14.0% | 3.0% | 9.1% | −0.2% | 6.1% | |
| 25 | I | −4.6% | 2.1% | −6.4% | 0.5% | −7.1% | −0.2% |
| II | −3.7% | 3.0% | −6.1% | 0.7% | −5.8% | 1.1% | |
| III | −1.7% | 4.9% | −5.0% | 1.8% | −4.3% | 2.4% | |
| IV | −0.2% | 6.2% | −4.2% | 2.6% | −6.4% | 0.5% | |
| V | −0.7% | 5.8% | −4.6% | 2.2% | −6.2% | 0.7% | |
| VI | 1.7% | 8.1% | −2.5% | 4.1% | −4.8% | 2.0% | |
| Mode | |||||||
|---|---|---|---|---|---|---|---|
| 100 | I | 1.4% | 6.7% | −1.4% | 4.0% | −2.1% | 3.4% |
| II | 3.2% | 8.3% | −0.4% | 5.0% | −0.9% | 4.5% | |
| III | 4.9% | 10.0% | 0.9% | 6.2% | 0.6% | 5.9% | |
| IV | 4.6% | 9.7% | 0.7% | 6.0% | −1.4% | 4.0% | |
| V | 5.2% | 10.3% | 1.3% | 6.5% | −0.4% | 5.0% | |
| VI | 7.1% | 12.1% | 3.5% | 8.6% | 1.5% | 6.7% | |
| 50 | I | −2.5% | 3.3% | −4.1% | 1.8% | −4.5% | 1.4% |
| II | −1.2% | 4.5% | −3.7% | 2.2% | −3.8% | 2.1% | |
| III | 0.4% | 6.1% | −2.8% | 3.0% | −2.8% | 3.0% | |
| IV | 0.0% | 5.7% | −2.9% | 2.9% | −4.2% | 1.7% | |
| V | 0.5% | 6.1% | −2.7% | 3.1% | −3.7% | 2.2% | |
| VI | 2.0% | 7.5% | −1.1% | 4.6% | −2.5% | 3.2% | |
| 25 | I | −5.6% | 0.5% | −6.2% | 0.0% | −6.6% | −0.4% |
| II | −5.2% | 0.9% | −6.2% | −0.1% | −5.7% | 0.5% | |
| III | −3.9% | 2.1% | −5.5% | 0.6% | −4.9% | 1.2% | |
| IV | −4.0% | 2.0% | −5.2% | 0.9% | −6.2% | 0.0% | |
| V | −4.4% | 1.7% | −5.7% | 0.5% | −6.3% | −0.1% | |
| VI | −4.1% | 1.9% | −5.1% | 1.0% | −5.8% | 0.3% | |
| Mode | |||||||
|---|---|---|---|---|---|---|---|
| 100 | I | 1.2% | 3.7% | −0.5% | 2.0% | −1.1% | 1.4% |
| II | 2.1% | 4.5% | -0.2% | 2.4% | −0.6% | 1.9% | |
| III | 3.0% | 5.5% | 0.5% | 3.0% | 0.2% | 2.7% | |
| IV | 4.0% | 6.4% | 1.1% | 3.5% | −0.5% | 2.0% | |
| V | 4.2% | 6.6% | 1.3% | 3.7% | −-0.2% | 2.3% | |
| VI | 6.1% | 8.5% | 2.9% | 5.4% | 1.0% | 3.5% | |
| 50 | I | −1.6% | 1.3% | −2.4% | 0.5% | −2.7% | 0.3% |
| II | −1.1% | 1.8% | −2.3% | 0.7% | −2.3% | 0.7% | |
| III | −0.3% | 2.6% | −1.9% | 1.1% | −1.9% | 1.1% | |
| IV | 0.3% | 3.1% | −1.5% | 1.4% | −2.4% | 0.5% | |
| V | 0.3% | 3.2% | −1.6% | 1.4% | −2.3% | 0.7% | |
| VI | 1.7% | 4.5% | −0.6% | 2.3% | −1.7% | 1.2% | |
| 25 | I | −3.9% | −0.7% | −4.0% | −0.8% | −4.4% | −1.1% |
| II | −4.1% | −0.9% | −4.3% | −1.0% | −2.7% | 0.5% | |
| III | −3.5% | −0.3% | −3.9% | −0.7% | −3.2% | 0.0% | |
| IV | −2.7% | 0.5% | −3.2% | 0.0% | −4.0% | −0.8% | |
| V | −3.5% | −0.3% | −3.8% | −0.6% | −4.3% | −1.1% | |
| VI | −3.2% | 0.0% | −3.6% | −0.4% | −4.2% | −1.0% | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Marczak, J. Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core. Materials 2022, 15, 7611. https://doi.org/10.3390/ma15217611
Marczak J. Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core. Materials. 2022; 15(21):7611. https://doi.org/10.3390/ma15217611
Chicago/Turabian StyleMarczak, Jakub. 2022. "Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core" Materials 15, no. 21: 7611. https://doi.org/10.3390/ma15217611
APA StyleMarczak, J. (2022). Tolerance Modelling of Vibrations of a Sandwich Plate with Honeycomb Core. Materials, 15(21), 7611. https://doi.org/10.3390/ma15217611






