A Multiscale Overview of Modelling Rolling Cyclic Fatigue in Bearing Elements
Abstract
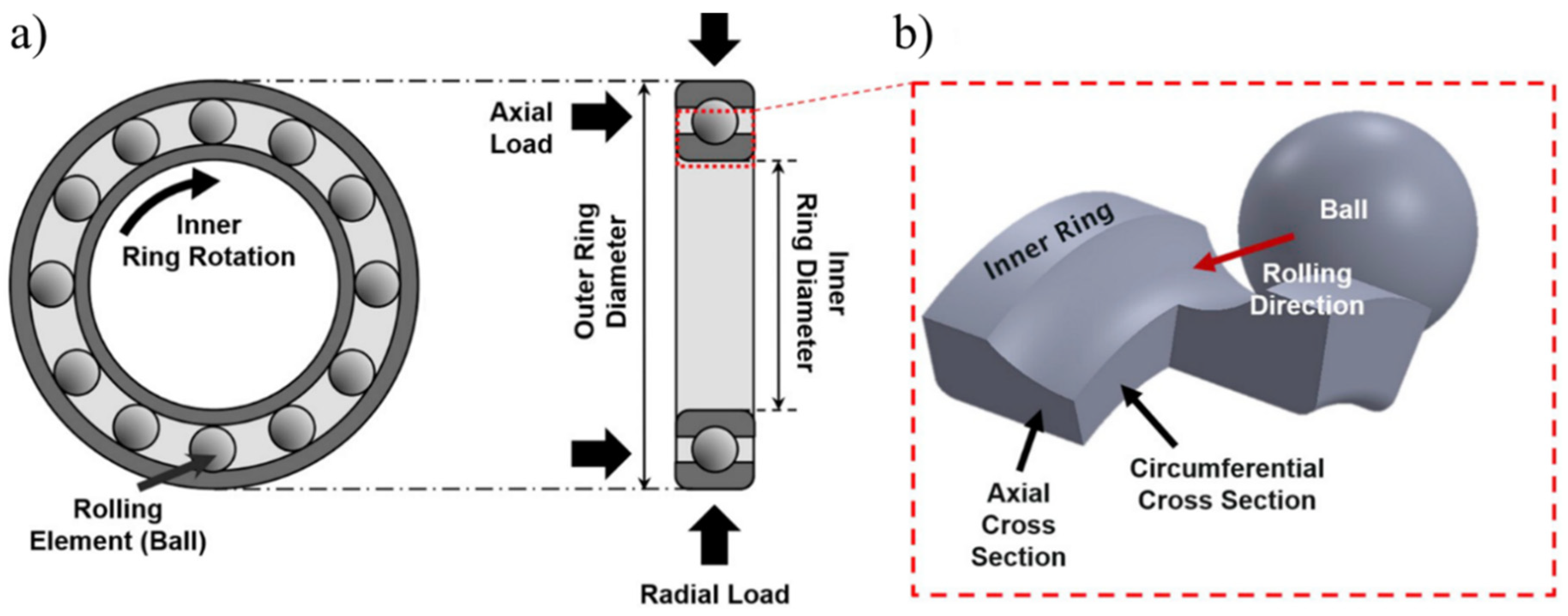
:1. Background
2. Bearing Steel
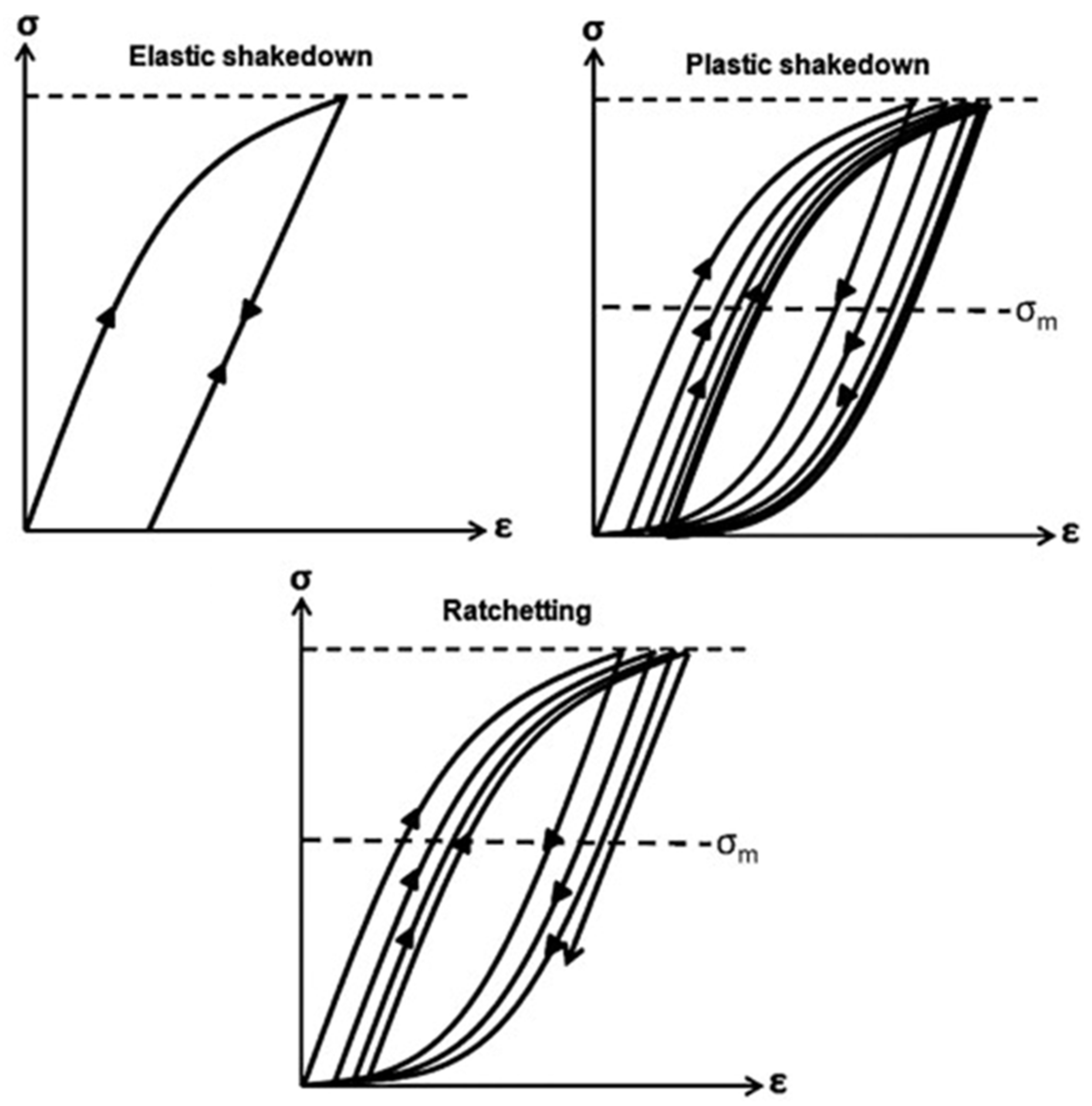
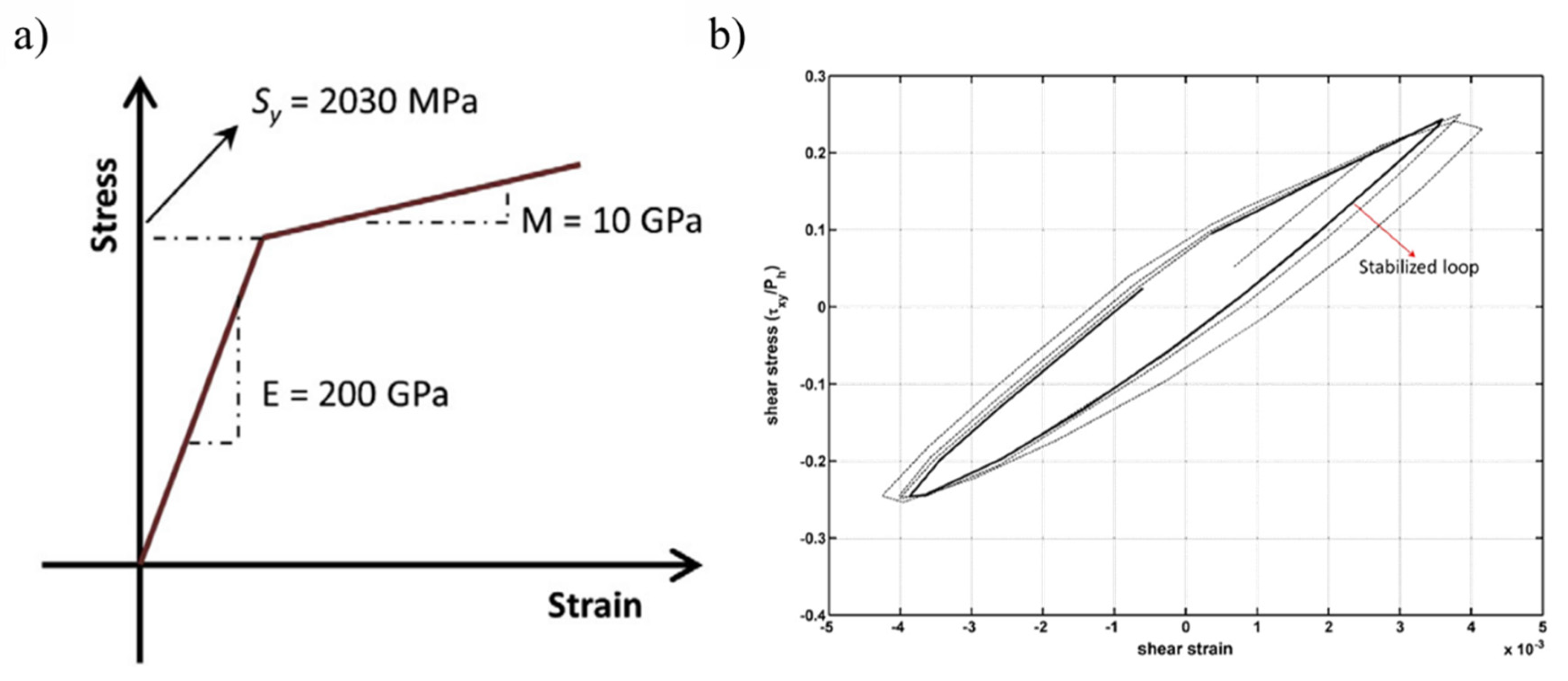
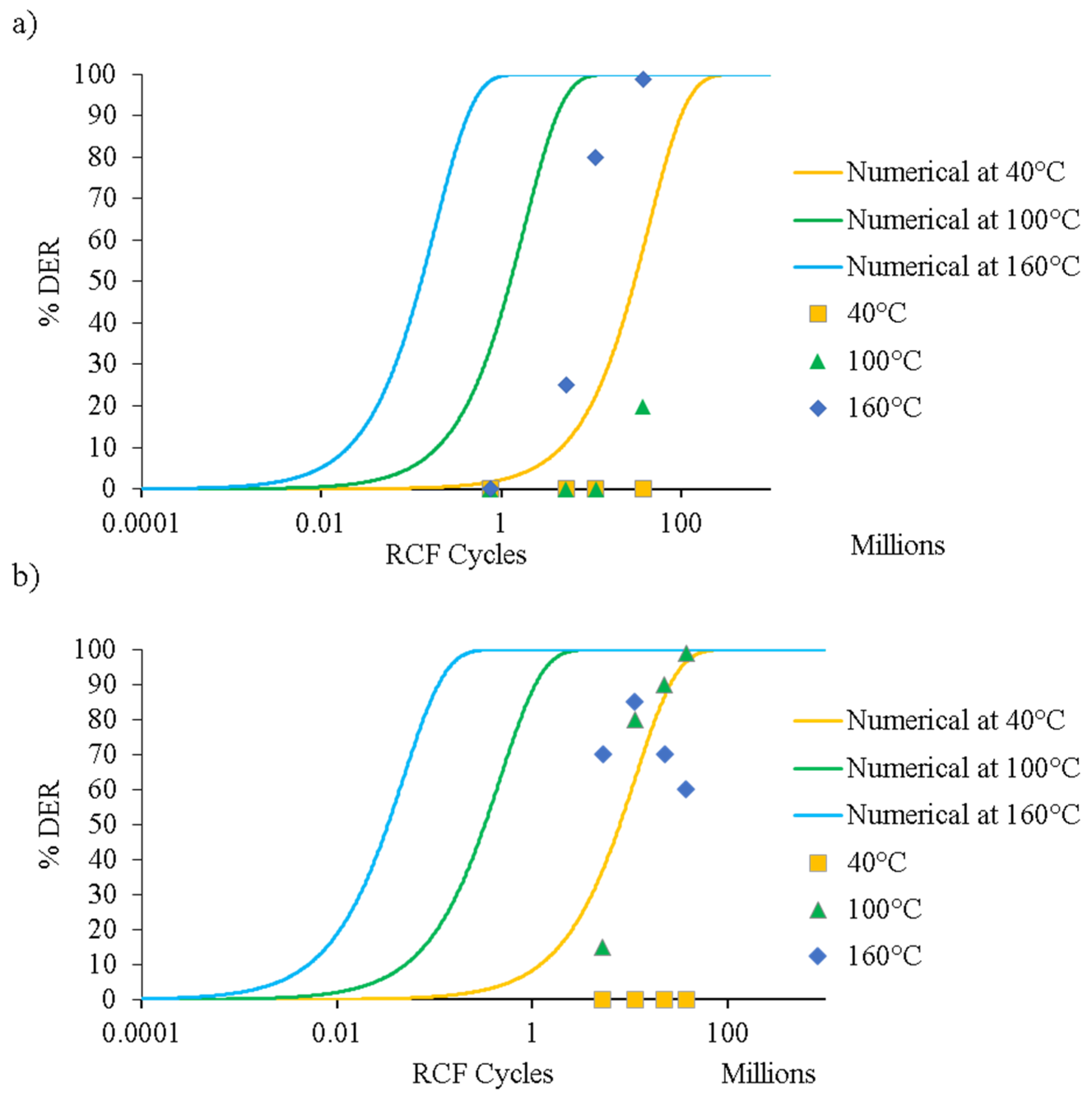
3. Material Modelling
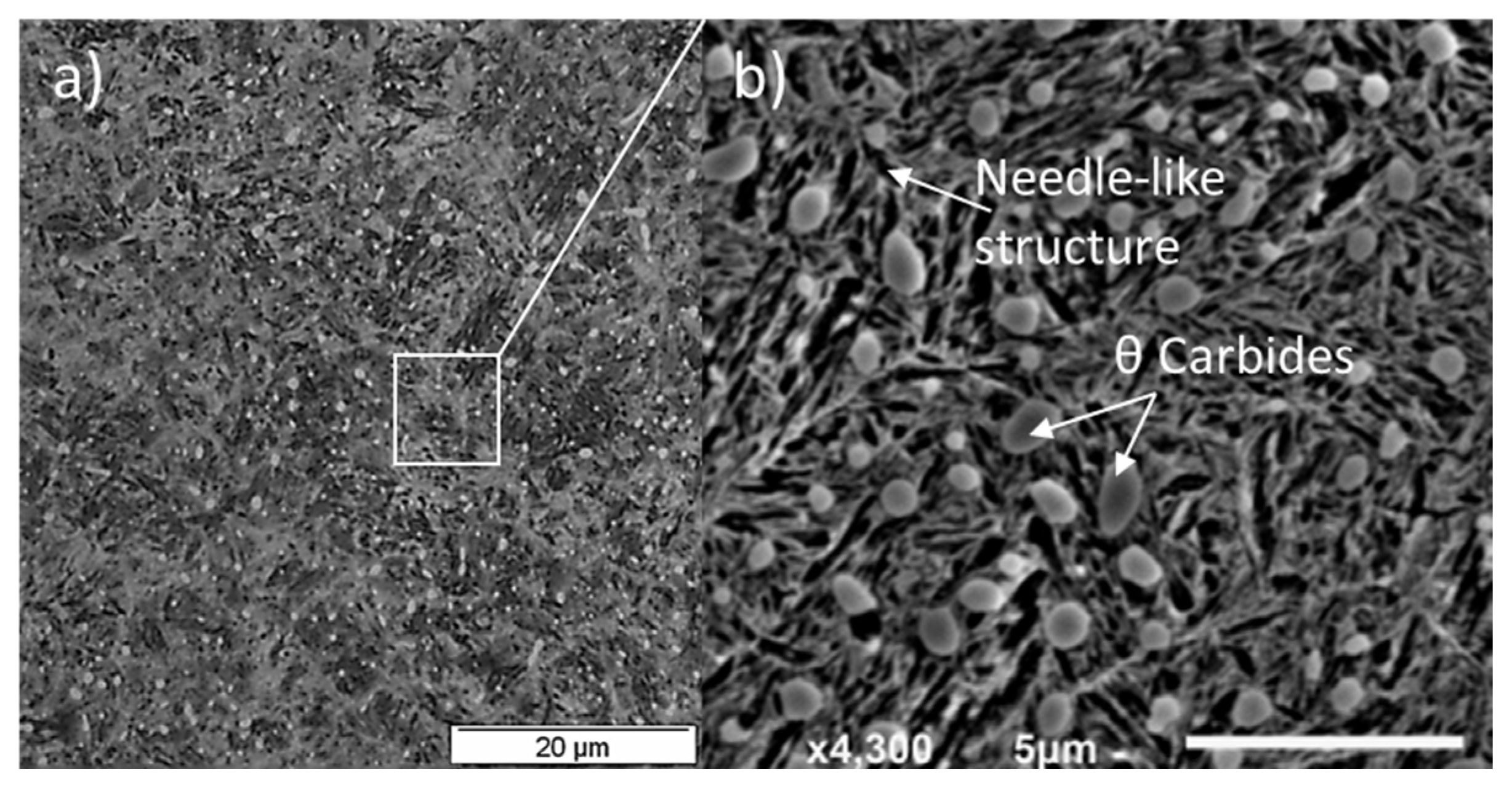
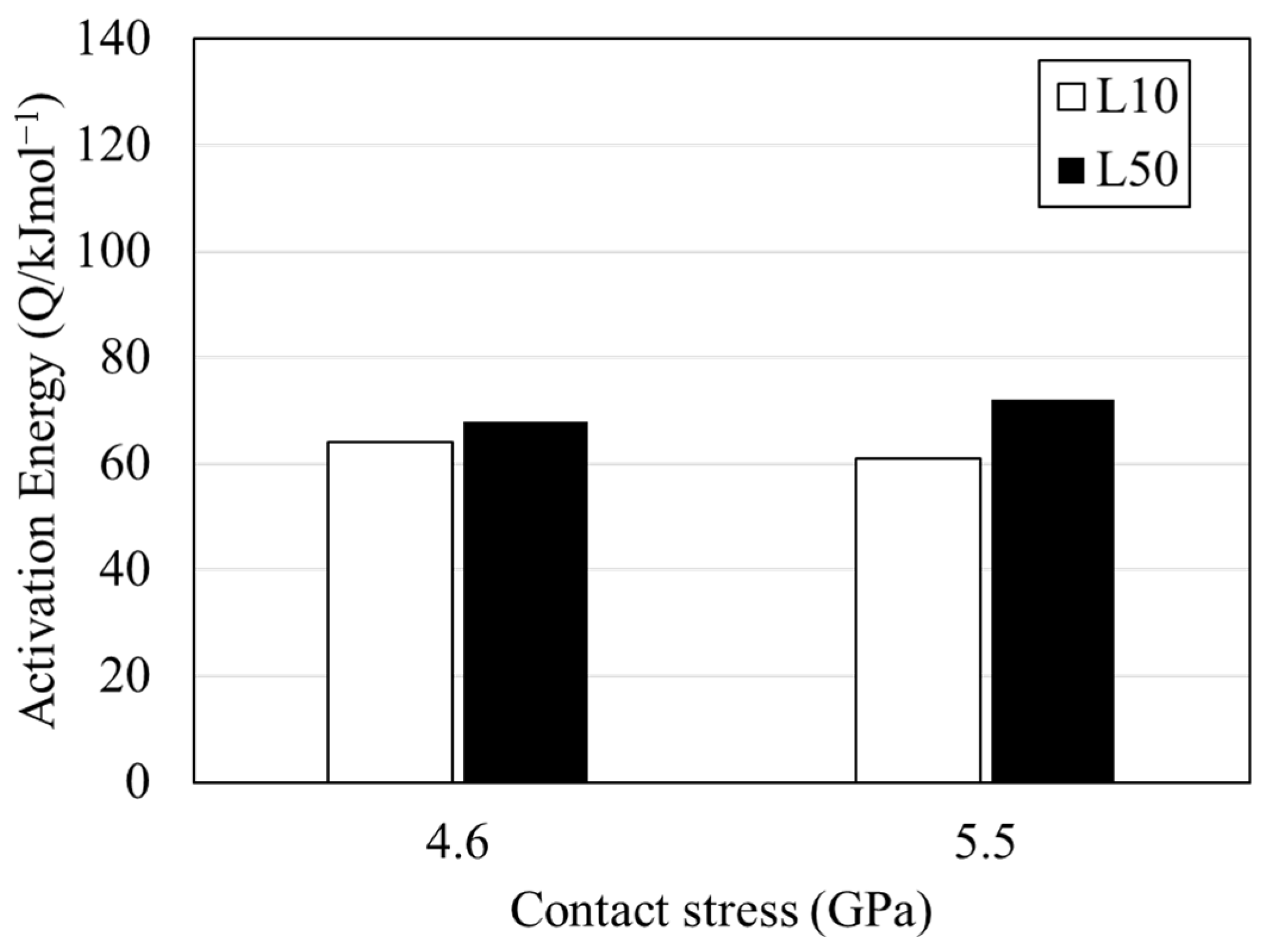
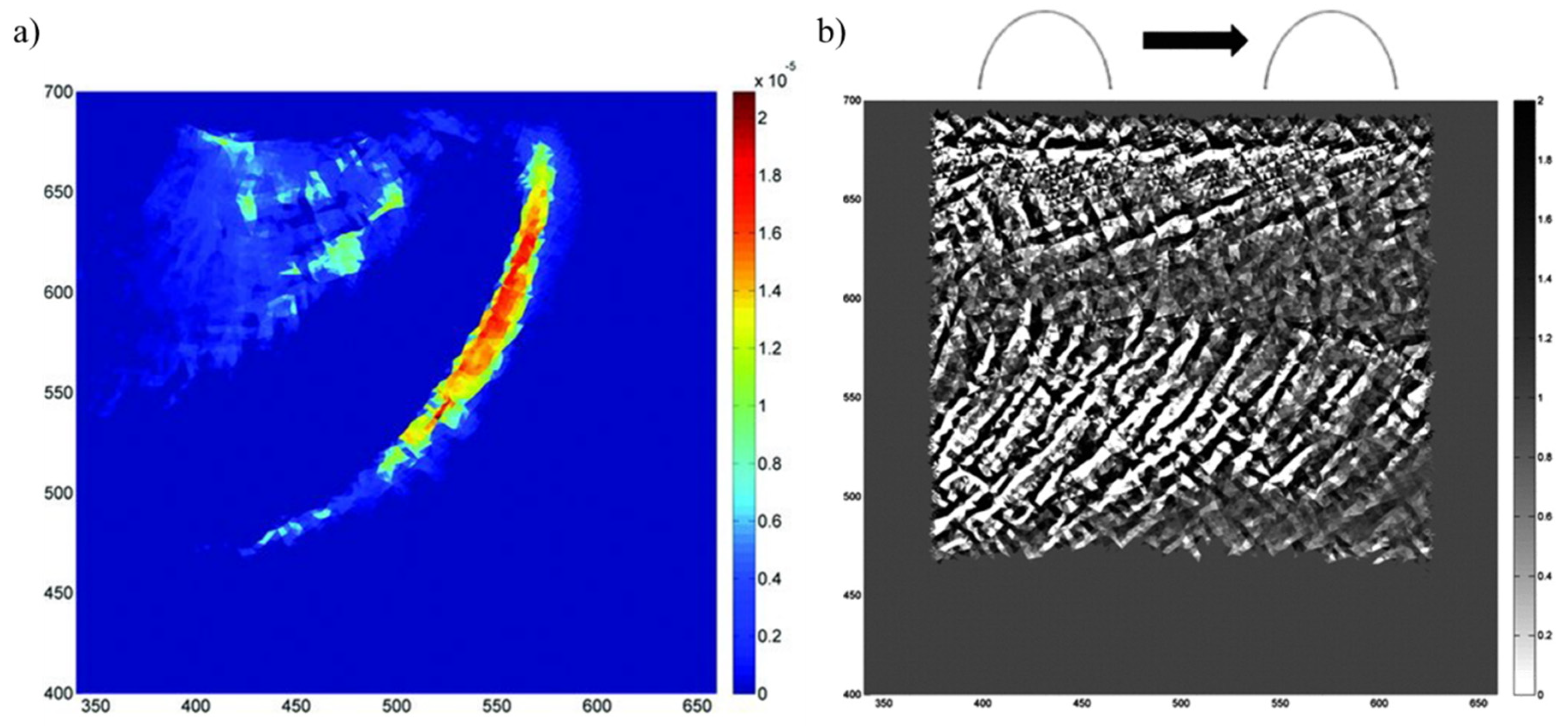
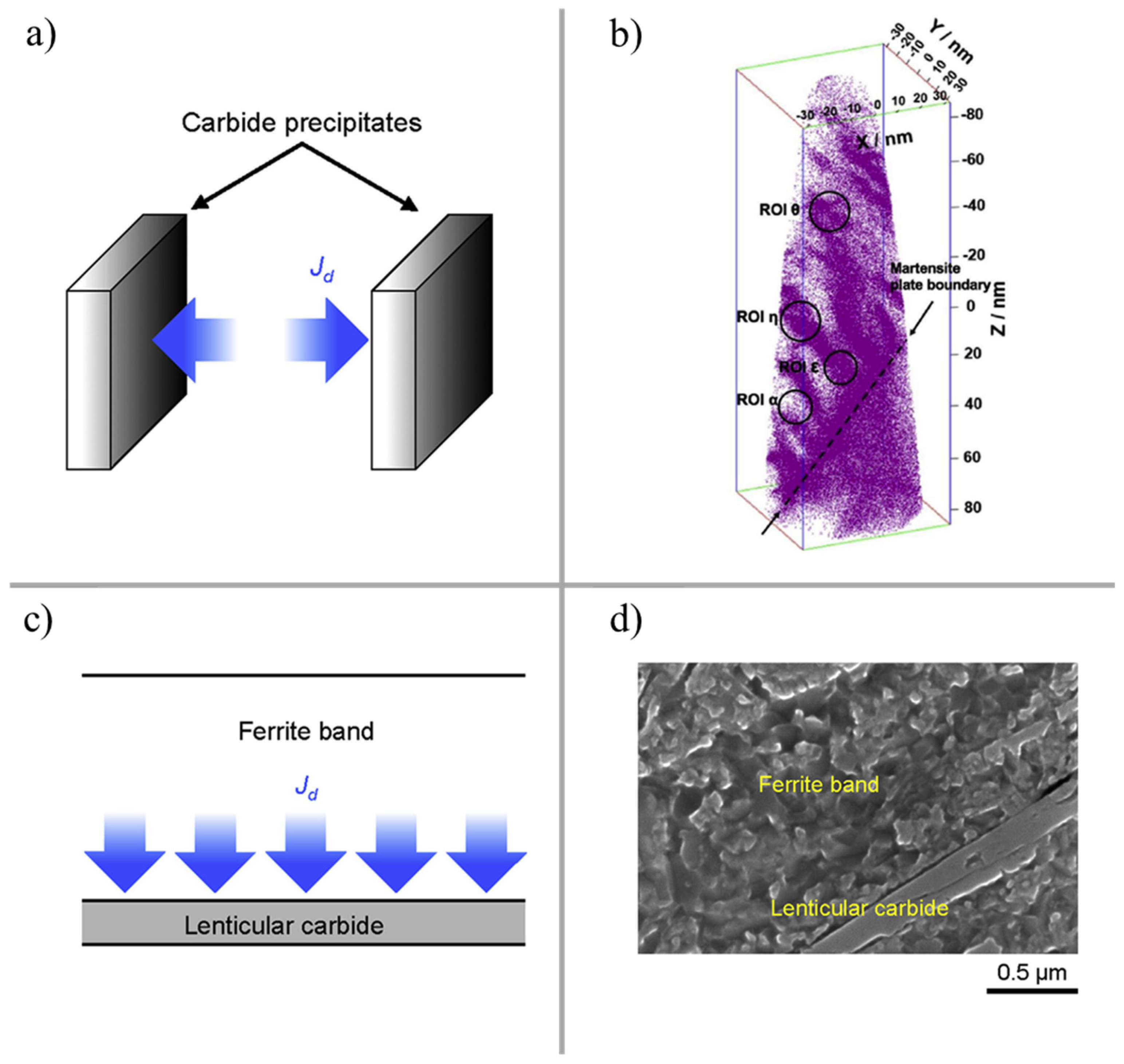
4. Microstructural Modelling
5. Overview and Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kang, J.-H.; Hosseinkhani, B.; Rivera-Díaz-del-Castillo, P.E. Rolling contact fatigue in bearings: Multiscale overview. Mater. Sci. Technol. 2012, 28, 44–49. [Google Scholar] [CrossRef]
- El Laithy, M.; Wang, L.; Harvey, T.J.; Vierneusel, B.; Correns, M.; Blass, T.J.T.I. Further understanding of rolling contact fatigue in rolling element bearings—A review. Tribol. Int. 2019, 140, 105849. [Google Scholar] [CrossRef]
- Voskamp, A.; Österlund, R.; Becker, P.; Vingsbo, O. Gradual changes in residual stress and microstructure during contact fatigue in ball bearings. Met. Technol. 1980, 7, 14–21. [Google Scholar] [CrossRef]
- Lundberg, G.; Palmgren, A. Dynamic Capacity of Rolling Bearings. J. Appl. Mech. 1949, 16, 165–172. [Google Scholar] [CrossRef]
- Khurram, M.; Mufti, R.A.; Bhutta, M.U.; Afzal, N.; Abdullah, M.U.; Rahman, S.U.; Rehman, S.U.; Zahid, R.; Mahmood, K.; Ashfaq, M.; et al. Roller sliding in engine valve train: Effect of oil film thickness considering lubricant composition. Tribol. Int. 2020, 149, 105829. [Google Scholar] [CrossRef]
- Abdullah, M.U.; Shah, S.R.; Bhutta, M.U.; Mufti, R.A.; Khurram, M.; Najeeb, M.H.; Arshad, W.; Ogawa, K. Benefits of wonder process craft on engine valve train performance. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2018, 233, 1125–1135. [Google Scholar] [CrossRef]
- Klecka, M.A.; Subhash, G.; Arakere, N.K. Microstructure–property relationships in M50-NiL and P675 case-hardened bearing steels. Tribol. Trans. 2013, 56, 1046–1059. [Google Scholar] [CrossRef]
- Merwin, J.; Johnson, K. An analysis of plastic deformation in rolling contact. Proc. Inst. Mech. Eng. 1963, 177, 676–690. [Google Scholar]
- Ruud, C. A review of selected non-destructive methods for residual stress measurement. NDT Int. 1982, 15, 15–23. [Google Scholar] [CrossRef]
- Warhadpande, A.; Sadeghi, F.; Evans, R.D.; Kotzalas, M.N. Influence of plasticity-induced residual stresses on rolling contact fatigue. Tribol. Trans. 2012, 55, 422–437. [Google Scholar] [CrossRef]
- Khan, Z.A.; Hadfield, M.; Tobe, S.; Wang, Y. Residual stress variations during rolling contact fatigue of refrigerant lubricated silicon nitride bearing elements. Ceram. Int. 2006, 32, 751–754. [Google Scholar] [CrossRef]
- Shen, Y.; Moghadam, S.M.; Sadeghi, F.; Paulson, K.; Trice, R.W. Effect of retained austenite—Compressive residual stresses on rolling contact fatigue life of carburized AISI 8620 steel. Int. J. Fatigue 2015, 75, 135–144. [Google Scholar] [CrossRef]
- Nazir, M.H.; Khan, Z.A.; Saeed, A. Experimental analysis and modelling of c-crack propagation in silicon nitride ball bearing element under rolling contact fatigue. Tribol. Int. 2018, 126, 386–401. [Google Scholar] [CrossRef]
- Bush, J.; Grube, W.; Robinson, G. Microstructural and residual stress changes in hardened steel due to rolling contact. Trans. ASM 1961, 54, 390–412. [Google Scholar]
- Polonsky, I.; Keer, L. On white etching band formation in rolling bearings. J. Mech. Phys. Solids 1995, 43, 637–669. [Google Scholar] [CrossRef]
- Šmeļova, V.; Schwedt, A.; Wang, L.; Holweger, W.; Mayer, J. Electron microscopy investigations of microstructural alterations due to classical Rolling Contact Fatigue (RCF) in martensitic AISI 52100 bearing steel. Int. J. Fatigue 2017, 98, 142–154. [Google Scholar] [CrossRef]
- El Laithy, M.; Wang, L.; Harvey, T.J.; Vierneusel, B. Re-investigation of dark etching regions and white etching bands in SAE 52100 bearing steel due to rolling contact fatigue. Int. J. Fatigue 2020, 136, 105591. [Google Scholar] [CrossRef]
- Kanetani, K.; Ushioda, K. Mechanism of White Band (WB) Formation due to Rolling Contact Fatigue in Carburized SAE4320 Steel. Mater. Transations 2020, 61, 1750–1759. [Google Scholar] [CrossRef]
- Su, Y.-S.; Li, S.-X.; Yu, F.; Lu, S.-Y.; Wang, Y.-G.J.I.J.o.F. Revealing the shear band origin of white etching area in rolling contact fatigue of bearing steel. Int. J. Fatigue 2021, 142, 105929. [Google Scholar] [CrossRef]
- Arakere, N.K. Gigacycle rolling contact fatigue of bearing steels: A review. Int. J. Fatigue 2016, 93, 238–249. [Google Scholar] [CrossRef]
- Yin, H.; Wu, Y.; Liu, D.; Zhang, P.; Zhang, G.; Fu, H.J.M. Rolling Contact Fatigue-Related Microstructural Alterations in Bearing Steels: A Brief Review. Metals 2022, 12, 910. [Google Scholar] [CrossRef]
- Voskamp, A. Material response to rolling contact loading. ASME Trans. J. Tribol. 1985, 107, 359–364. [Google Scholar] [CrossRef]
- Morris, D.; Sadeghi, F.; Chen, Y.-C.; Wang, C.; Wang, B. Effect of Residual Stresses on Microstructural Evolution Due to Rolling Contact Fatigue. J. Tribol. 2018, 140, 061402. [Google Scholar] [CrossRef]
- Cherolis, N.E.; Benac, D.J.; Pridemore, W.D. Fatigue in Rotating Equipment: Is it HCF or LCF? J. Fail. Anal. Prev. 2016, 16, 828–841. [Google Scholar] [CrossRef]
- Beswick, J.M. Bearing Steel Technology: Advances and State of the Art in Bearing Steel Quality Assurance: 7th Volume; ASTM International: West Conshohocken, PA, USA, 2007. [Google Scholar]
- Bhadeshia, H.; Honeycombe, R. Steels: Microstructure and Properties; Butterworth-Heinemann: Oxford, UK, 2017. [Google Scholar]
- Barrow, A.; Rivera-Díaz-del-Castillo, P. Nanoprecipitation in bearing steels. Acta Mater. 2011, 59, 7155–7167. [Google Scholar] [CrossRef]
- Song, W.; Choi, P.-P.; Inden, G.; Prahl, U.; Raabe, D.; Bleck, W. On the spheroidized carbide dissolution and elemental partitioning in high carbon bearing steel 100Cr6. Metall. Mater. Trans. A 2014, 45, 595–606. [Google Scholar] [CrossRef]
- Abdullah, M.U. Finite Element Modelling of Deep Zone Residual Stresses in Rolling Contact Bearing Elements; Bournemouth University: Poole, UK, 2022. [Google Scholar]
- Radhakrishnan, V.; Ramanathan, S. Plastic deformation in rolling contact. Wear 1975, 32, 211–221. [Google Scholar] [CrossRef]
- Lorösch, H.-K. Influence of Load on the Magnitude of the Life Exponent for Rolling Bearings, in Rolling Contact Fatigue Testing of Bearing Steels; ASTM International: West Conshohocken, PA, USA, 1982. [Google Scholar]
- Bhattacharyya, A.; Pandkar, A.; Subhash, G.; Arakere, N. Cyclic constitutive response and effective S–N diagram of M50 NiL case-hardened bearing steel subjected to Rolling Contact Fatigue. J. Tribol. 2015, 137, 041102. [Google Scholar] [CrossRef]
- Turteltaub, S.; Suiker, A. Transformation-induced plasticity in ferrous alloys. J. Mech. Phys. Solids 2005, 53, 1747–1788. [Google Scholar] [CrossRef]
- Johnson, K. The strength of surfaces in rolling contact. Proc. Inst. Mech. Eng. Part C Mech. Eng. Sci. 1989, 203, 151–163. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Subhash, G.; Arakere, N. Evolution of subsurface plastic zone due to rolling contact fatigue of M-50 NiL case hardened bearing steel. Int. J. Fatigue 2014, 59, 102–113. [Google Scholar] [CrossRef]
- Zaretsky, E.V. Rolling bearing steels—A technical and historical perspective. Mater. Sci. Technol. 2012, 28, 58–69. [Google Scholar] [CrossRef] [Green Version]
- Chaboche, J.L. A review of some plasticity and viscoplasticity constitutive theories. Int. J. Plast. 2008, 24, 1642–1693. [Google Scholar] [CrossRef]
- Jhansale, H.; Topper, T. Engineering Analysis of the Inelastic stress Response of a Structural Metal under Variable Cyclic Strains, in Cyclic Stress-Strain Behavior—Analysis, Experimentation, and Failure Prediction; ASTM International: West Conshohocken, PA, USA, 1971. [Google Scholar]
- Österlund, R.; Vingsbo, O. Phase changes in fatigued ball bearings. Metall. Mater. Trans. A 1980, 11, 701–707. [Google Scholar] [CrossRef]
- Sadeghi, F.; Jalalahmadi, B.; Slack, T.S.; Raje, N.; Arakere, N.K. A review of rolling contact fatigue. J. Tribol. 2009, 131, 041403. [Google Scholar] [CrossRef]
- Arakere, N.; Subhash, G. Work hardening response of M50-NiL case hardened bearing steel during shakedown in rolling contact fatigue. Mater. Sci. Technol. 2012, 28, 34–38. [Google Scholar] [CrossRef]
- Jhansale, H. A new parameter for the hysteretic stress-strain behavior of metals. ASME J. Eng. Mater. Technol. 1975, 97, 33–38. [Google Scholar] [CrossRef]
- Hahn, G.; Bhargava, V.; Rubin, C.; Chen, Q.; Kim, K. Analysis of the rolling contact residual stresses and cyclic plastic deformation of SAE 52100 steel ball bearings. J. Tribol. 1987, 109, 618–626. [Google Scholar] [CrossRef]
- Swahn, H.; Becker, P.; Vingsbo, O. Martensite decay during rolling contact fatigue in ball bearings. Metall. Mater. Trans. A 1976, 7, 1099–1110. [Google Scholar] [CrossRef]
- Hahn, G.T.; Bhargava, V.; Chen, Q. The cyclic stress-strain properties, hysteresis loop shape, and kinematic hardening of two high-strength bearing steels. Metall. Trans. A 1990, 21, 653. [Google Scholar] [CrossRef]
- Christ, H.J.; Sommer, C.; Mughrabi, H.; Voskamp, A.; Beswick, J.; Hengerer, F. Fatigue behaviour of three variants of the roller bearing steel SAE 52100. Fatigue Fract. Eng. Mater. Struct. 1992, 15, 855–870. [Google Scholar] [CrossRef]
- Kang, J.-H.; Hosseinkhani, B.; Vegter, R.H.; Rivera-Díaz-del-Castillo, P.E. Modelling dislocation assisted tempering during rolling contact fatigue in bearing steels. Int. J. Fatigue 2015, 75, 115–125. [Google Scholar] [CrossRef]
- Fu, H.; Galindo-Nava, E.; Rivera-Díaz-del-Castillo, P. Modelling and characterisation of stress-induced carbide precipitation in bearing steels under rolling contact fatigue. Acta Mater. 2017, 128, 176–187. [Google Scholar] [CrossRef]
- Warhadpande, A.; Sadeghi, F.; Evans, R.D. Microstructural alterations in bearing steels under rolling contact fatigue part 1—Historical overview. Tribol. Trans. 2013, 56, 349–358. [Google Scholar] [CrossRef]
- Hamilton, G. Plastic flow in rollers loaded above the yield point. Proc. Inst. Mech. Eng. 1963, 177, 667–675. [Google Scholar]
- Bhargava, V.; Hahn, G.; Rubin, C. An elastic-plastic finite element model of rolling contact, Part 1: Analysis of single contacts. J. Appl. Mech. 1985, 52, 67–74. [Google Scholar] [CrossRef]
- Crook, A. Simulated gear-tooth contacts: Some experiments upon their lubrication and subsurface deformations. Proc. Inst. Mech. Eng. 1957, 171, 187–214. [Google Scholar] [CrossRef]
- Nazir, M.H.; Khan, Z.A.; Saeed, A.; Siddaiah, A.; Menezes, P.L. Synergistic wear-corrosion analysis and modelling of nanocomposite coatings. Tribol. Int. 2018, 121, 30–44. [Google Scholar] [CrossRef]
- Howell, M.; Hahn, G.; Rubin, C.; McDowell, D. Finite element analysis of rolling contact for nonlinear kinematic hardening bearing steel. ASME J. Tribol. 1995, 117, 729–736. [Google Scholar] [CrossRef]
- Bower, A.; Johnson, K. The influence of strain hardening on cumulative plastic deformation in rolling and sliding line contact. J. Mech. Phys. Solids 1989, 37, 471–493. [Google Scholar] [CrossRef]
- Jacq, C.; Nélias, D.; Lormand, G.; Girodin, D. Development of a three-dimensional semi-analytical elastic-plastic contact code. J. Trib. 2002, 124, 653–667. [Google Scholar] [CrossRef]
- Chaise, T.; Nélias, D. Contact pressure and residual strain in 3D elasto-plastic rolling contact for a circular or elliptical point contact. J. Tribol. 2011, 133, 041402. [Google Scholar] [CrossRef]
- Chen, W.W.; Wang, Q.J.; Wang, F.; Keer, L.M.; Cao, J. Three-dimensional repeated elasto-plastic point contacts, rolling, and sliding. J. Appl. Mech. 2008, 75, 021021. [Google Scholar] [CrossRef]
- Walvekar, A.A.; Sadeghi, F. Rolling contact fatigue of case carburized steels. Int. J. Fatigue 2017, 95, 264–281. [Google Scholar] [CrossRef]
- Golmohammadi, Z.; Walvekar, A.; Sadeghi, F. A 3D efficient finite element model to simulate rolling contact fatigue under high loading conditions. Tribol. Int. 2018, 126, 258–269. [Google Scholar] [CrossRef]
- Bomidi, J.A.R.; Sadeghi, F. Three-Dimensional Finite Element Elastic–Plastic Model for Subsurface Initiated Spalling in Rolling Contacts. J. Tribol. 2013, 136, 011402. [Google Scholar] [CrossRef]
- Warhadpande, A.; Sadeghi, F.; Kotzalas, M.N.; Doll, G. Effects of plasticity on subsurface initiated spalling in rolling contact fatigue. Int. J. Fatigue 2012, 36, 80–95. [Google Scholar] [CrossRef]
- Popescu, G.; Morales-Espejel, G.E.; Wemekamp, B.; Gabelli, A. An engineering model for three-dimensional elastic-plastic rolling contact analyses. Tribol. Trans. 2006, 49, 387–399. [Google Scholar] [CrossRef]
- Hill, R. The Mathematical Theory of Plasticity; Oxford University Press: Oxford, UK, 1998; Volume 11. [Google Scholar]
- Prager, W. A new methods of analyzing stresses and strains in work hardening plastic solids. J. Appl. Mech. (ASME) 1956, 23, 493–496. [Google Scholar] [CrossRef]
- McDowell, D. A two surface model for transient nonproportional cyclic plasticity, Part 1: Development of appropriate equations. J. Appl. Mech. (ASME) 1985, 52, 298–302. [Google Scholar] [CrossRef]
- Mroz, Z. On the Theory of Steady Plastic Cycles in Structures; Institute of Fundamental Problems of Technology: Warsaw, Poland, 1971. [Google Scholar]
- Pandkar, A.S.; Arakere, N.; Subhash, G. Microstructure-sensitive accumulation of plastic strain due to ratcheting in bearing steels subject to Rolling Contact Fatigue. Int. J. Fatigue 2014, 63, 191–202. [Google Scholar] [CrossRef]
- Chaboche, J.-L. Time-independent constitutive theories for cyclic plasticity. Int. J. Plast. 1986, 2, 149–188. [Google Scholar] [CrossRef]
- Kabo, E.; Ekberg, A. Fatigue initiation in railway wheels—A numerical study of the influence of defects. Wear 2002, 253, 26–34. [Google Scholar] [CrossRef]
- Taraf, M.; Zahaf, E.; Oussouaddi, O.; Zeghloul, A. Numerical analysis for predicting the rolling contact fatigue crack initiation in a railway wheel steel. Tribol. Int. 2010, 43, 585–593. [Google Scholar] [CrossRef]
- Portier, L.; Calloch, S.; Marquis, D.; Geyer, P. Ratchetting under tension–torsion loadings: Experiments and modelling. Int. J. Plast. 2000, 16, 303–335. [Google Scholar] [CrossRef]
- Pandkar, A.S.; Arakere, N.; Subhash, G. Ratcheting-based microstructure-sensitive modeling of the cyclic hardening response of case-hardened bearing steels subject to Rolling Contact Fatigue. Int. J. Fatigue 2015, 73, 119–131. [Google Scholar] [CrossRef]
- Bomidi, J.A.R.; Weinzapfel, N.; Slack, T.; Mobasher Moghaddam, S.; Sadeghi, F.; Liebel, A.; Weber, J.; Kreis, T. Experimental and Numerical Investigation of Torsion Fatigue of Bearing Steel. J. Tribol. 2013, 135, 031103. [Google Scholar] [CrossRef]
- Abdullah, M.U.; Khan, Z.A.; Kruhoeffer, W.; Blass, T. A 3D Finite Element Model of Rolling Contact Fatigue for Evolved Material Response and Residual Stress Estimation. Tribol. Lett. 2020, 68, 122. [Google Scholar] [CrossRef]
- Tabor, D. Indentation Hardness and Its Measurement: Some Cautionary Comments, in Microindentation Techniques in Materials Science and Engineering; ASTM International: West Conshohocken, PA, USA, 1985. [Google Scholar]
- Kanetani, K.; Mikami, T.; Ushioda, K. Effect of Retained Austenite on Sub-surface Initiated Spalling during Rolling Contact Fatigue in Carburized SAE4320 Steel. ISIJ Int. 2020, 60, 1774–1783. [Google Scholar] [CrossRef]
- Jiang, Y.; Sehitoglu, H. Modeling of cyclic ratchetting plasticity, part I: Development of constitutive relations. J. Appl. Mech. 1996, 63, 720–725. [Google Scholar] [CrossRef]
- Walvekar, A.A.; Morris, D.; Golmohammadi, Z.; Sadeghi, F.; Correns, M. A Novel Modeling Approach to Simulate Rolling Contact Fatigue and Three-Dimensional Spalls. J. Tribol. 2018, 140, 031101. [Google Scholar] [CrossRef]
- Preparata, F.P.; Shamos, M.I. Computational Geometry: An Introduction; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Jalalahmadi, B.; Sadeghi, F. A Voronoi Finite Element Study of Fatigue Life Scatter in Rolling Contacts. J. Tribol. 2009, 131, 022203. [Google Scholar] [CrossRef]
- Raje, N.; Sadeghi, F.; Rateick, J.R.G.; Hoeprich, M.R. A Numerical Model for Life Scatter in Rolling Element Bearings. J. Tribol. 2007, 130, 011011. [Google Scholar] [CrossRef]
- Weinzapfel, N.; Sadeghi, F.; Bakolas, V. An approach for modeling material grain structure in investigations of Hertzian subsurface stresses and rolling contact fatigue. J. Tribol. 2010, 132, 041404. [Google Scholar] [CrossRef]
- Weinzapfel, N.; Sadeghi, F.; Bakolas, V.; Liebel, A. A 3D Finite Element Study of Fatigue Life Dispersion in Rolling Line Contacts. J. Tribol. 2011, 133, 042202. [Google Scholar] [CrossRef]
- Shen, F.; Zhou, K. An elasto-plastic-damage model for initiation and propagation of spalling in rolling bearings. Int. J. Mech. Sci. 2019, 161, 105058. [Google Scholar] [CrossRef]
- Martin, J.; Borgese, S.; Eberhardt, A. Microstructural alterations of rolling—Bearing steel undergoing cyclic stressing. ASME J. Basic Eng. 1966, 88, 555–565. [Google Scholar] [CrossRef]
- Lund, T. Structural alterations in fatigue-tested ball- bearing steel. Jernkontorets Ann. 1969, 153, 337–343. [Google Scholar]
- Mitamura, N.; Hidaka, H.; Takaki, S. Microstructural development in bearing steel during rolling contact fatigue. Mater. Sci. Forum 2007, 539–543, 4255–4260. [Google Scholar] [CrossRef]
- Warhadpande, A.; Sadeghi, F.; Evans, R.D. Microstructural Alterations in Bearing Steels under Rolling Contact Fatigue: Part 2—Diffusion-Based Modeling Approach. Tribol. Trans. 2014, 57, 66–76. [Google Scholar] [CrossRef]
- Johnson, K. Formation of Shear Bands in Ball-Bearing Races; University of Cambridge, Department of Engineering: Cambridge, UK, 1988. [Google Scholar]
- Bhargava, V.; Hahn, G.; Rubin, C. Rolling contact deformation, etching effects, and failure of high-strength bearing steel. Met. Mater Trans A 1990, 21, 1921–1931. [Google Scholar] [CrossRef]
- Zwirlein, O.; Schlicht, H. Werkstoffanstrengung bei Wälzbeanspruchung–Einfluß von Reibung und Eigenspannungen. Mater. Und Werkst. 1980, 11, 1–14. [Google Scholar] [CrossRef]
- Zwirlein, O.; Schlicht, H. Rolling Contact Fatigue Mechanisms—Accelerated Testing versus Field Performance, in Rolling Contact Fatigue Testing of Bearing Steels; ASTM International: West Conshohocken, PA, USA, 1982. [Google Scholar]
- Abdullah, M.U.; Khan, Z.A.; Kruhoeffer, W.; Blass, T.; Vierneusel, B. Development of white etching bands under accelerated rolling contact fatigue. Tribol. Int. 2021, 164, 107240. [Google Scholar] [CrossRef]
- Buchwald, J.; Heckel, R. An analysis of microstructural changes in 52100 steel bearings during cyclic stressing(Microstructural changes in 52100 steel bearing inner rings during cyclic stressing, obtaining thickening rate data on white-etching regions and lenticular carbides). ASM Trans. Q. 1968, 61, 750–756. [Google Scholar]
- Mirza, M.; Sellars, C.; Karhausen, K.; Evans, P. Multipass rolling of aluminium alloys: Finite element simulations and microstructural evolution. Mater. Sci. Technol. 2001, 17, 874–879. [Google Scholar] [CrossRef]
- Lindahl, E.; Österlund, R. 212 transmission electron microscopy applied to phase transformations in ball bearings. Ultramicroscopy 1982, 9, 355–364. [Google Scholar] [CrossRef]
- Pernach, M.; Pietrzyk, M. Numerical solution of the diffusion equation with moving boundary applied to modelling of the austenite–ferrite phase transformation. Comput. Mater. Sci. 2008, 44, 783–791. [Google Scholar] [CrossRef]
- Zhao, L. Modeling of oxygen diffusion along grain boundaries in a nickel-based superalloy. J. Eng. Mater. Technol. 2011, 133, 031002. [Google Scholar] [CrossRef]
- Fu, H.; Rivera-Díaz-del-Castillo, P. Evolution of White Etching Bands in 100Cr6 Bearing Steel under Rolling Contact-Fatigue. Metals 2019, 9, 491. [Google Scholar] [CrossRef]
- Cottrell, A.H.; Bilby, B. Dislocation theory of yielding and strain ageing of iron. Proc. Phys. Society. Sect. A 1949, 62, 49. [Google Scholar] [CrossRef]
- Fu, H.; Song, W.; Galindo-Nava, E.I.; Rivera-Díaz-del-Castillo, P.E. Strain-induced martensite decay in bearing steels under rolling contact fatigue: Modelling and atomic-scale characterisation. Acta Mater. 2017, 139, 163–173. [Google Scholar] [CrossRef]
- Fu, H.; Rivera-Díaz-del-Castillo, P.E. A unified theory for microstructural alterations in bearing steels under rolling contact fatigue. Acta Mater. 2018, 155, 43–55. [Google Scholar] [CrossRef]
- Abdullah, M.U.; Khan, Z.A.; Kruhoeffer, W. Evaluation of Dark Etching Regions for Standard Bearing Steel under Accelerated Rolling Contact Fatigue. Tribol. Int. 2020, 152, 106579. [Google Scholar] [CrossRef]
- Fu, H. Microstructural Alterations in Bearing Steels under Rolling Contact Fatigue; University of Cambridge: Cambridge, UK, 2017. [Google Scholar]
- El Laithy, M.; Wang, L.; Harvey, T.J.; Vierneusel, B. Semi-empirical model for predicting LAB and HAB formation in bearing steels. Int. J. Fatigue 2021, 148, 106230. [Google Scholar] [CrossRef]
- El Laithy, M.; Wang, L.; Harvey, T.J.; Schwedt, A.; Vierneusel, B.; Mayer, J. White etching bands formation mechanisms due to rolling contact fatigue. Acta Mater. 2022, 232, 117932. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abdullah, M.U.; Khan, Z.A. A Multiscale Overview of Modelling Rolling Cyclic Fatigue in Bearing Elements. Materials 2022, 15, 5885. https://doi.org/10.3390/ma15175885
Abdullah MU, Khan ZA. A Multiscale Overview of Modelling Rolling Cyclic Fatigue in Bearing Elements. Materials. 2022; 15(17):5885. https://doi.org/10.3390/ma15175885
Chicago/Turabian StyleAbdullah, Muhammad U., and Zulfiqar A. Khan. 2022. "A Multiscale Overview of Modelling Rolling Cyclic Fatigue in Bearing Elements" Materials 15, no. 17: 5885. https://doi.org/10.3390/ma15175885
APA StyleAbdullah, M. U., & Khan, Z. A. (2022). A Multiscale Overview of Modelling Rolling Cyclic Fatigue in Bearing Elements. Materials, 15(17), 5885. https://doi.org/10.3390/ma15175885






