Study on the Dynamic Characteristics of a SiC-Based Capacitive Micro-Accelerometer in Rarefied Air
Abstract
:1. Introduction
2. Effective Viscosity in the Rarefied Air
2.1. Effective Viscosity Coefficient
2.2. Analysis of Effective Viscosity
3. Squeeze-Film Air Damping in the Rarefied Air
3.1. Squeeze-Film Effect
3.2. Damping Coefficient and Relative Damping Ratio
3.3. Simulation of Squeeze-Film Air Damping
4. Dynamic Characteristic Analysis
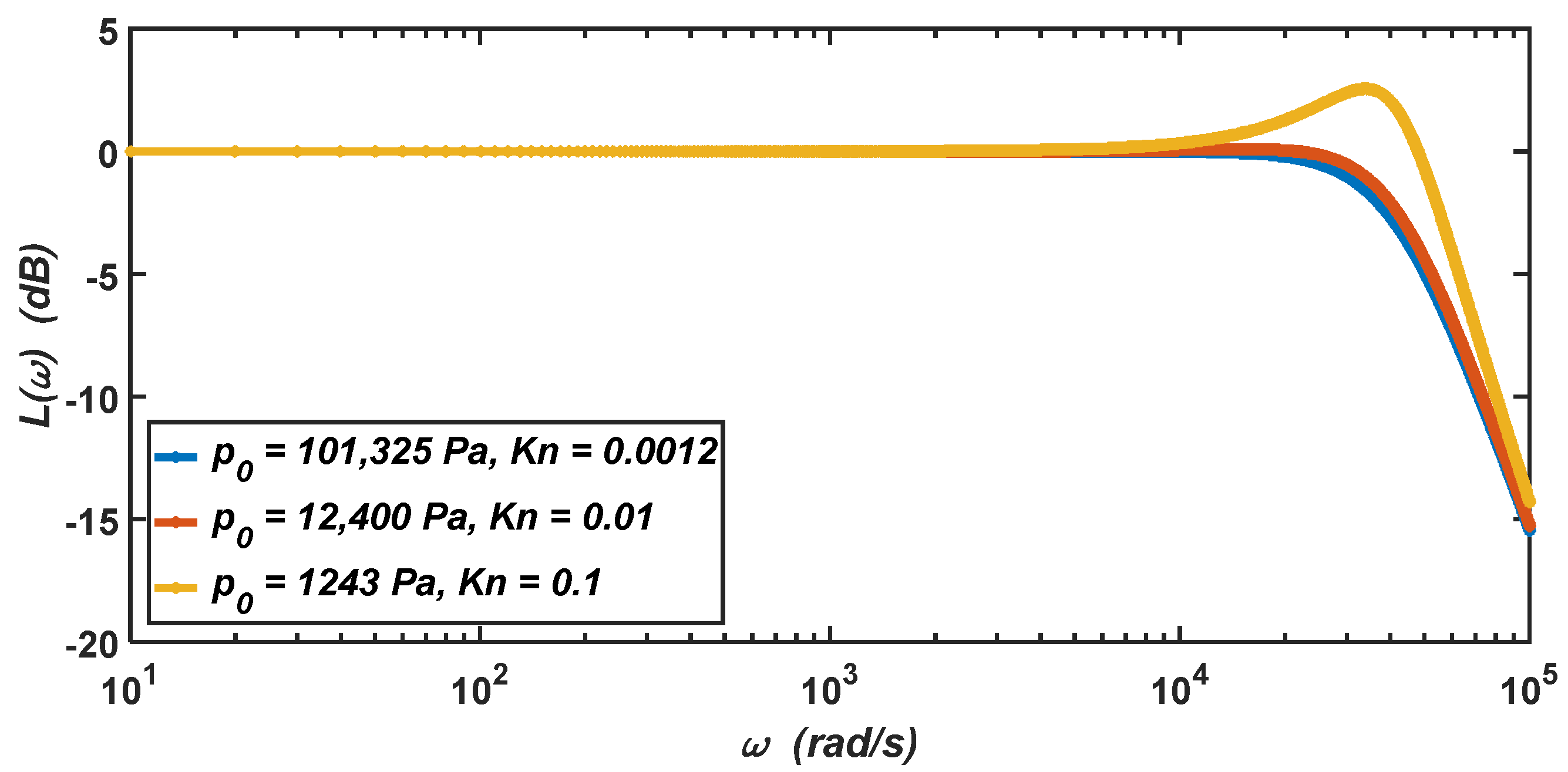
4.1. Amplitude–Frequency Characteristics
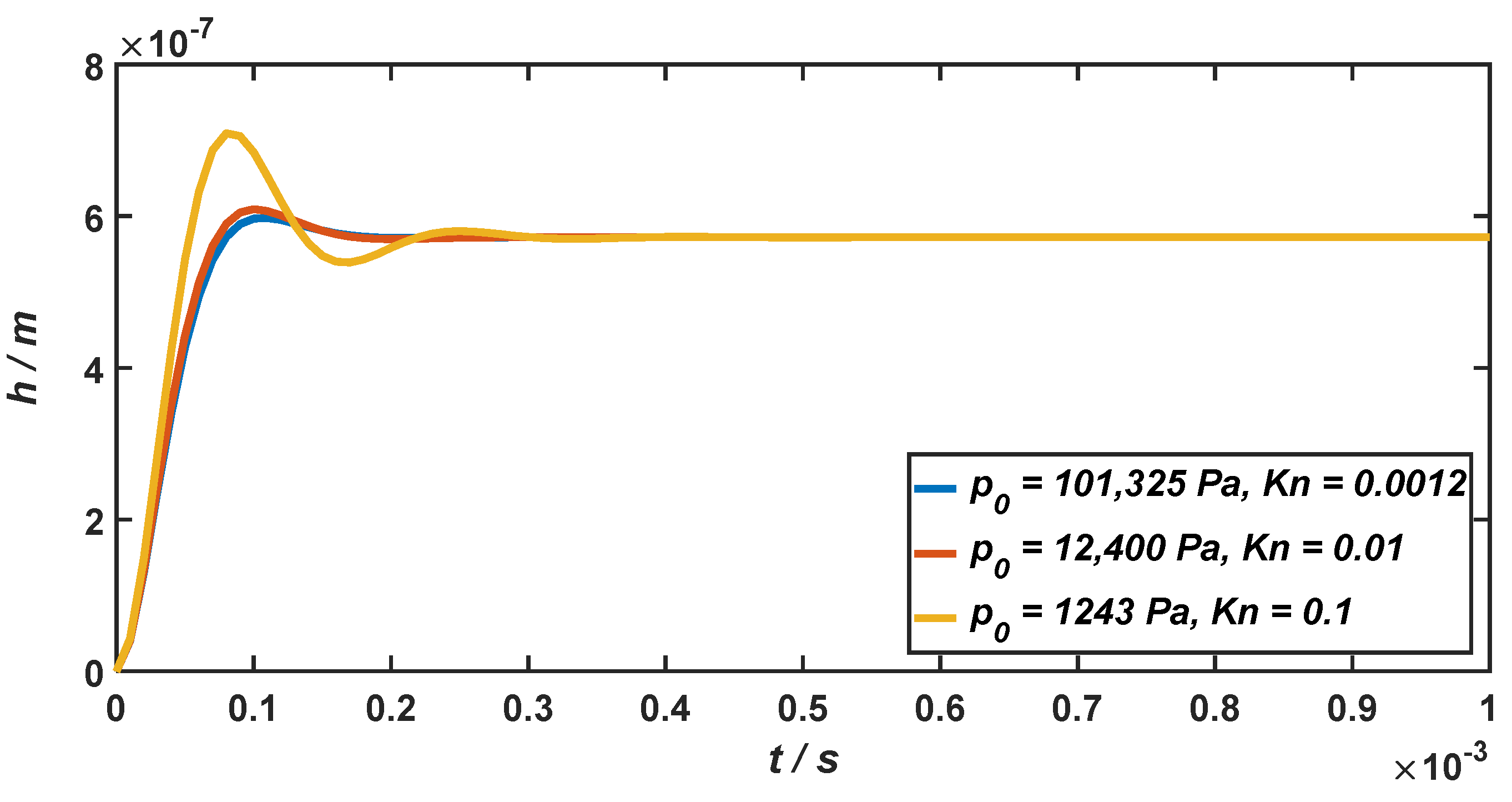
4.2. Step Responses
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Wu, Q.F.; Chen, W.F. Introduction. In DSMC Method for Thermochemical Nonequilibrium Flow of High-Temperature Rarefied Gas; National University of Defense Technology Press: Changsha, China, 1999; pp. 1–4. [Google Scholar]
- Myong, R.S.; Xu, K. Special issue on recent hot topics in rarefied gas dynamics. Int. J. Comput. Fluid Dyn. 2022, 35, 563–565. [Google Scholar] [CrossRef]
- Xu, S.S.; Wu, Z.N.; Li, Q.; Hong, Y.J. Hybrid continuum/DSMC computation of rocket mode lightcraft flow in near space with high temperature and rarefaction effect. Comput. Fluids 2009, 38, 1394–1404. [Google Scholar] [CrossRef]
- Yu, Z.Q.; Chen, S.P.; Mou, Y.; Hu, F. Electrostatic-Fluid-Structure 3D numerical simulation of a MEMS electrostatic comb resonator. Sensors 2022, 22, 1056. [Google Scholar] [CrossRef] [PubMed]
- Mehdipoor, M.; Ghavifekr, H.B. Design and analysis of a new MEMS biosensor based on coupled mechanical resonators for microfluidics applications. Analog. Integr. Circuits Signal Process. 2022, 111, 277–286. [Google Scholar] [CrossRef]
- Bao, M.H.; Yang, H. Squeeze film air damping in MEMS. Sens. Actuators A Phys. 2007, 136, 3–27. [Google Scholar] [CrossRef]
- Lu, C.H.; Li, P.; Fang, Y.M. Efficient molecular model for squeeze-film damping in rarefied air. Chin. Phys. B. 2019, 28, 098501. [Google Scholar] [CrossRef]
- Burgdorfer, A. The influence of the molecular mean free path on the performance of hydrodynamic gas lubricated bearings. J. Basic Eng. 1959, 81, 94–98. [Google Scholar] [CrossRef]
- Hsia, Y.T.; Domoto, G.A. An experimental investigation of molecular rarefaction effects in gas lubricated bearings at ultra-low clearances. J. Lubr. Technol. 1983, 105, 120–129. [Google Scholar] [CrossRef]
- Guo, X.H.; Alexeenko, A. Compact model of squeeze-film damping based on rarefied flow simulations. J. Micromech. Microeng. 2009, 19, 045026. [Google Scholar] [CrossRef]
- Guo, X.H.; Alexeenko, A.; Li, J.; Xiu, D. Uncertainty quantification models for micro-scale squeeze-film damping. Int. J. Numer. Methods Eng. 2010, 84, 1257–1272. [Google Scholar] [CrossRef]
- Cercignani, C.; Daneri, A. Flow of a rarefied gas between two parallel plates. J. Appl. Phys. 1963, 34, 3509–3513. [Google Scholar] [CrossRef]
- Cercignani, C.; Pagani, C.D. Variational approach to boundary-value problems in kinetic theory. Phys. Fluids 1966, 9, 1167–1173. [Google Scholar] [CrossRef]
- Fukui, S.; Kaneko, R. Analysis of ultra-thin gas film lubrication based on the linearized Boltzmann-equation (influence of accommodation coefficient). JSME Int. J. 1987, 30, 1660–1666. [Google Scholar] [CrossRef]
- Fukui, S.; Kaneko, R. Analysis of ultra-thin gas film lubrication based on linearized Boltzmann equation: First report-derivation of a generalized lubrication equation including thermal creep flow. ASME J. Tribol. 1988, 110, 253–262. [Google Scholar] [CrossRef]
- Fukui, S.; Kaneko, R. A database for interpolation of Poiseuille flow rates for high Knudsen number lubrication problems. ASME J. Tribol. 1990, 112, 78–83. [Google Scholar] [CrossRef]
- Veijola, T.; Kuisma, H.; Lahdenperä, J.; Ryhänen, T. Equivalent-circuit model of the squeezed gas film in a silicon accelerometer. Sens. Actuators A Phys. 1995, 48, 239–248. [Google Scholar] [CrossRef]
- Mol, L.; Rocha, L.A.; Cretu, E.; Wolffenbuttel, R.F. Squeezed film damping measurements on a parallel-plate MEMS in the free molecule regime. J. Micromech. Microeng. 2009, 19, 074021. [Google Scholar] [CrossRef]
- Leung, R.; Cheung, H.; Gang, H.; Ye, W. A Monte Carlo simulation approach for the modeling of free-molecule squeeze-film damping of flexible microresonators. Microfluid. Nanofluid. 2010, 9, 809–818. [Google Scholar] [CrossRef]
- Kaczynski, J.; Ranacher, C.; Fleury, C. Computationally efficient model for viscous damping in perforated MEMS structures. Sens. Actuators A Phys. 2020, 314, 112201. [Google Scholar] [CrossRef]
- Tian, X.; Sheng, W.; Guo, Z.; Xing, W.; Tang, R. Modeling and analysis of a SiC microstructure-based capacitive micro-accelerometer. Materials 2021, 14, 6222. [Google Scholar] [CrossRef]
- Seidel, H.; Riedel, H.; Kolbeck, R.; Mück, G.; Kupke, W.; Königer, M. Capacitive silicon accelerometer with highly symmetrical design. Sens. Actuators A Phys. 1990, 21, 312–315. [Google Scholar] [CrossRef]
- Veijola, T.; Turowski, M. Compact damping models for laterally moving microstructures with gas-rarefaction effects. J. Microelectromech. Syst. 2001, 10, 263–273. [Google Scholar] [CrossRef]
- Bao, M.H.; Yang, H.; Yin, H.; Sun, Y. Energy transfer model for squeeze-film air damping in low vacuum. J. Micromech. Microeng. 2002, 12, 341–346. [Google Scholar] [CrossRef]
- Michalis, V.K.; Kalarakis, A.N.; Skouras, E.D.; Burganos, V.N. Rarefaction effects on gas viscosity in the Knudsen transition regime. Microfluid. Nanofluid. 2010, 9, 847–853. [Google Scholar] [CrossRef]
- Kim, S.H.; Pitsch, H.; Boyd, I.D. Slip velocity and Knudsen layer in the lattice Boltzmann method for microscale flows. Phys. Rev. E 2008, 77, 026704. [Google Scholar] [CrossRef] [PubMed]
- Benmessaoud, M.; Nasreddine, M.M. Optimization of MEMS capacitive accelerometer. Microsyst. Technol. 2013, 19, 713–720. [Google Scholar] [CrossRef] [Green Version]
- Dong, Y.; Lal, A. The squeeze film damping effect of perforated microscanners: Modeling and characterization. Smart Mater. Struct. 2006, 15, 480–484. [Google Scholar] [CrossRef]
- Ishfaque, A.; Kim, B. Analytical solution for squeeze film damping of MEMS perforated circular plates using Green’s function. Nonlinear Dyn. 2017, 87, 1603–1616. [Google Scholar] [CrossRef]
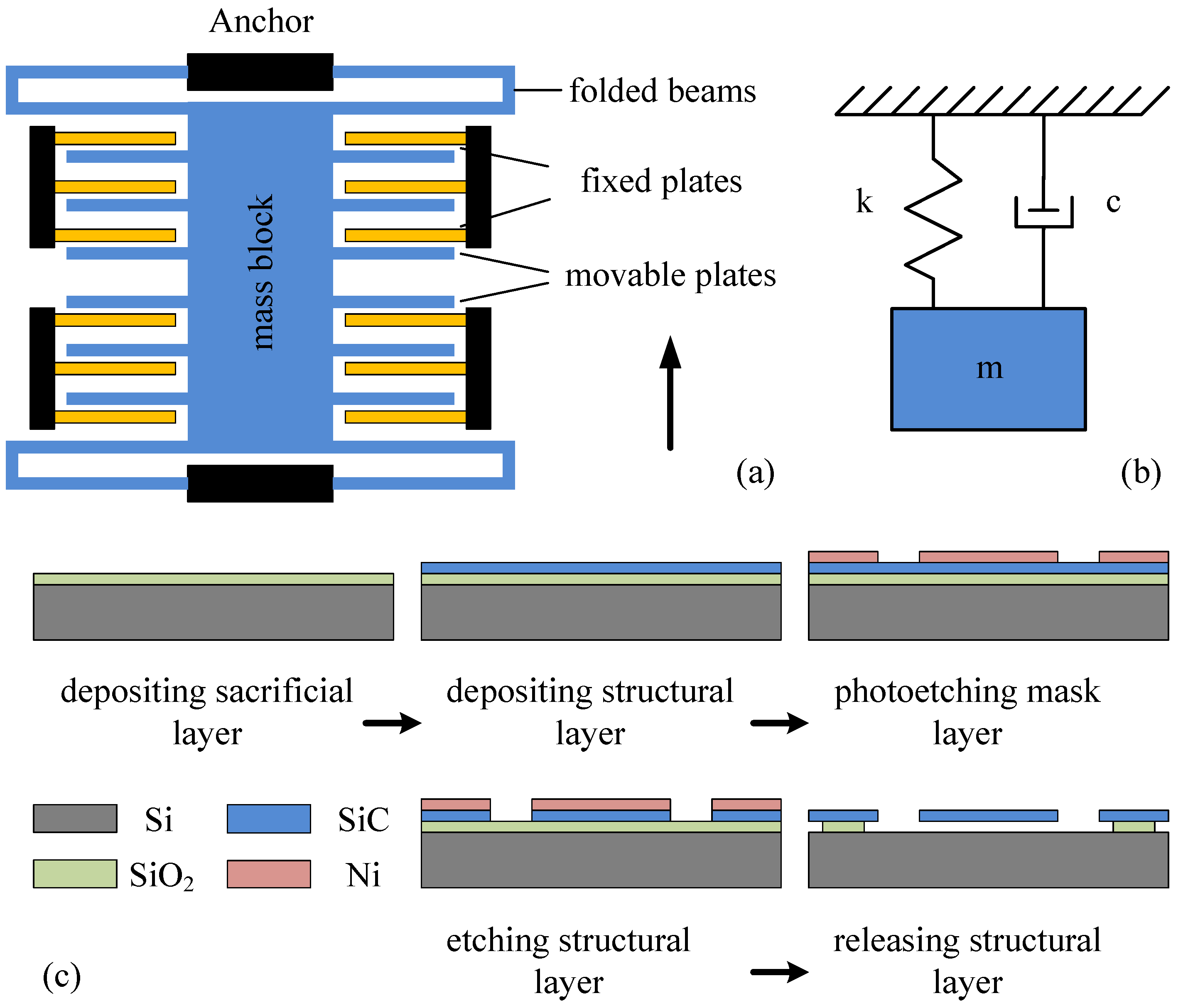

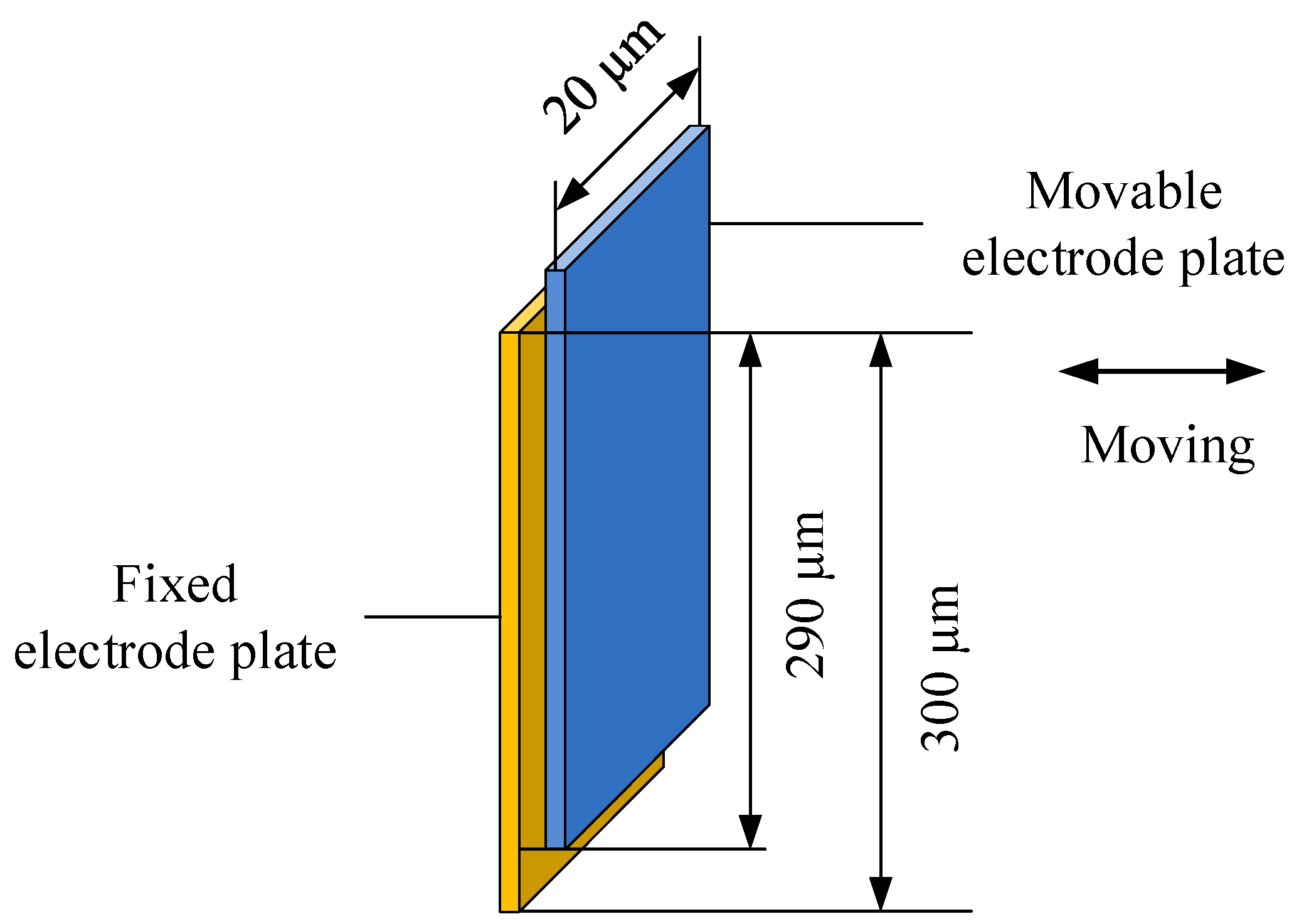


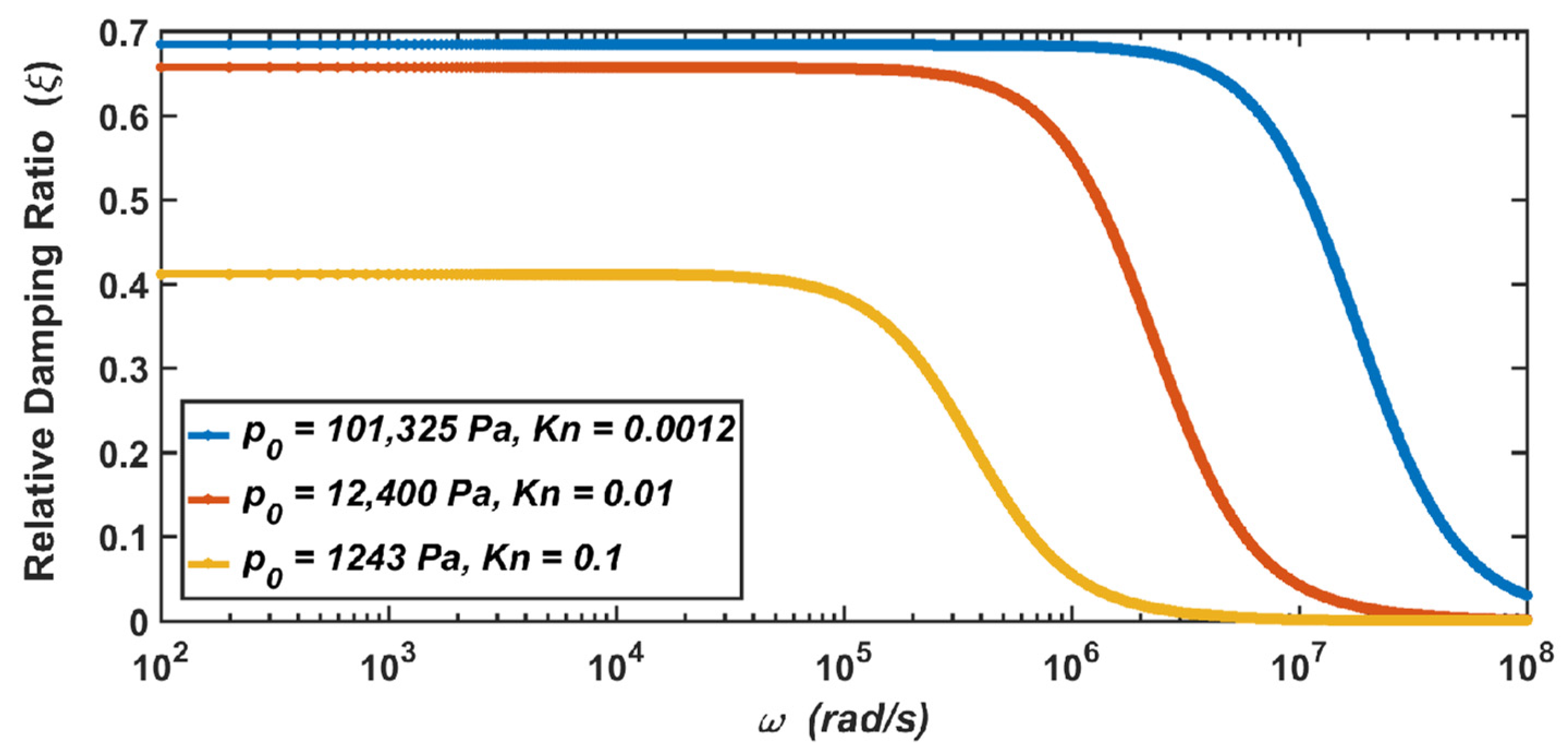
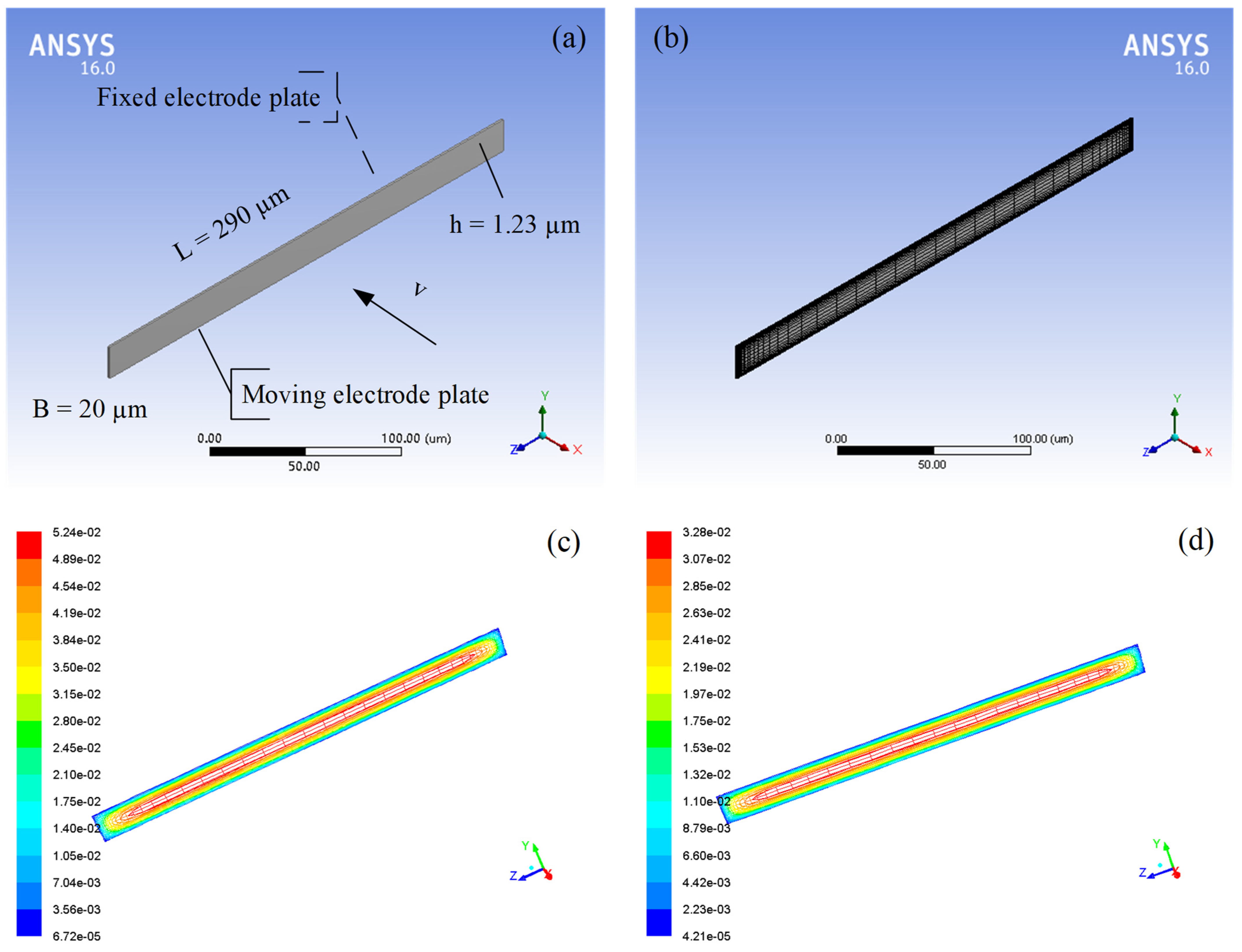
| Accelerometer | Length (µm) | Width (µm) | Thickness (µm) |
|---|---|---|---|
| Mass block | 500 | 350 | 20 |
| Movable electrode plates | 300 | 4 | 20 |
| Fixed electrode plates | 300 | 4 | 20 |
| Folded support beams | 350 | 4 | 20 |
| Air Pressure | 101,325 Pa (1 atm) | 12,400 Pa | 1243 Pa |
|---|---|---|---|
| Mean free path (m) | 2.4537 × 10−8 | 2.005 × 10−7 | 2.0001 × 10−6 |
| Knudsen number | 0.0012 | 0.01 | 0.1 |
| Effective viscosity coefficient (N·s/m2) | 1.7821 × 10−5 | 1.7098 × 10−5 | 1.0716 × 10−5 |
| Decline ratio (%) | - | 4.1% | 39.9% |
| Parameters | 12,400 Pa | 1243 Pa |
|---|---|---|
| Acceleration of gravity (m/s2) | 9.8 | 9.8 |
| Air density (kg/m3) | 0.15 | 0.015 |
| Effective viscosity coefficient (N·s/m) | 1.7098 × 10−5 | 1.0716 × 10−5 |
| Environment temperature (°C) | 15 | 15 |
| Speed of the moving plate (µm/s) | 10 | 10 |
| Structure | Parameter | 12,400 Pa | 1243 Pa | ||
|---|---|---|---|---|---|
| Theoretical Value | Simulated Value | Theoretical Value | Simulated Value | ||
| Comb structure | Air damping force (N) | 2.04 × 10−10 | 1.96 × 10−10 | 1.28 × 10−10 | 1.23 × 10−10 |
| Damping coefficient | 2.04 × 10−5 | 1.96 × 10−5 | 1.28 × 10−5 | 1.23 × 10−5 | |
| Accelerometer | Air damping force (N) | 8.16 × 10−9 | 7.84 × 10−9 | 5.12 × 10−9 | 4.92 × 10−9 |
| Damping coefficient | 8.16 × 10−4 | 7.84 × 10−4 | 5.12 × 10−4 | 4.92 × 10−4 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, X.; Sheng, W. Study on the Dynamic Characteristics of a SiC-Based Capacitive Micro-Accelerometer in Rarefied Air. Materials 2022, 15, 4692. https://doi.org/10.3390/ma15134692
Tian X, Sheng W. Study on the Dynamic Characteristics of a SiC-Based Capacitive Micro-Accelerometer in Rarefied Air. Materials. 2022; 15(13):4692. https://doi.org/10.3390/ma15134692
Chicago/Turabian StyleTian, Xiang, and Wei Sheng. 2022. "Study on the Dynamic Characteristics of a SiC-Based Capacitive Micro-Accelerometer in Rarefied Air" Materials 15, no. 13: 4692. https://doi.org/10.3390/ma15134692
APA StyleTian, X., & Sheng, W. (2022). Study on the Dynamic Characteristics of a SiC-Based Capacitive Micro-Accelerometer in Rarefied Air. Materials, 15(13), 4692. https://doi.org/10.3390/ma15134692






