Determination of Transverse Shear Stiffness of Sandwich Panels with a Corrugated Core by Numerical Homogenization
Abstract
1. Introduction
2. Materials and Methods
3. Results
3.1. Stiffnesses Variation Due to Different Approach for Modelling Cross-Direction Section
3.2. Stiffnesses Variation Due to Different Finite Element Type
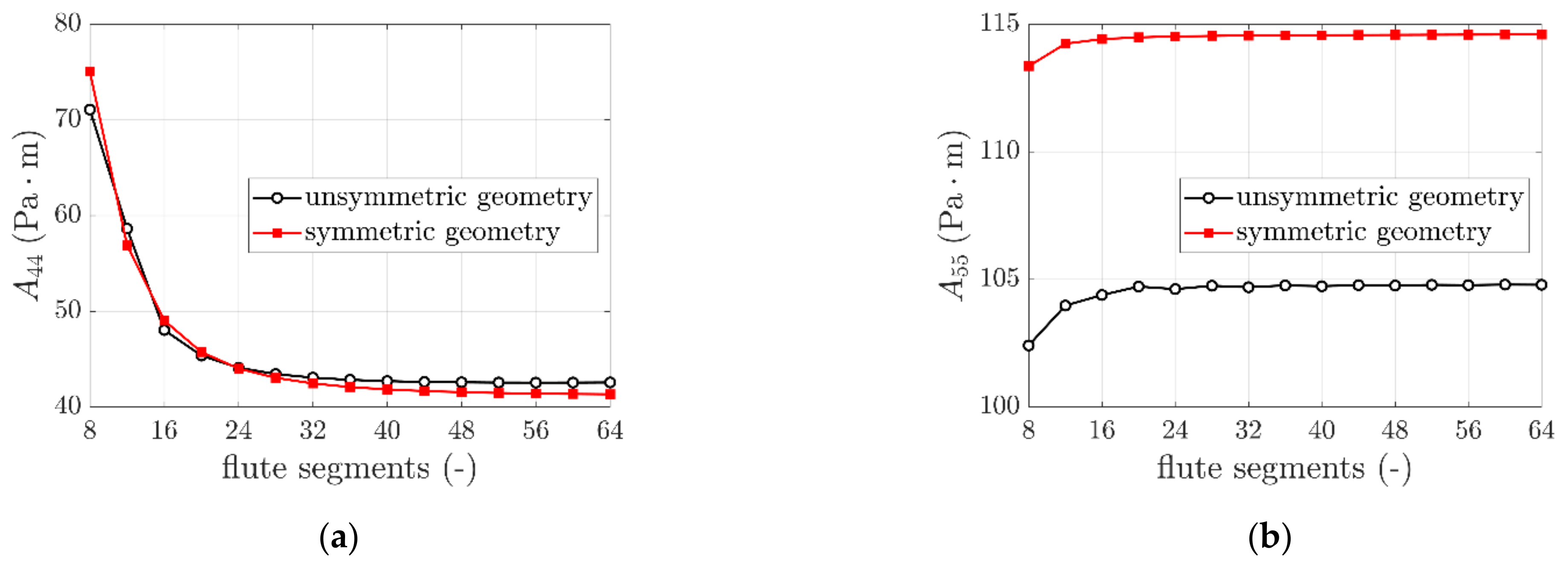
3.3. Stiffnesses Variation Due to Different Fluting Discretization
3.4. Stiffnesses Variation Due to Different Numbers of Periods
4. Discussion
4.1. Different Approach of Modelling Cross-Direction Section
4.2. Different Finite Element Type
4.3. Different Fluting Discretization
4.4. Different Numbers of Periods
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- McKee, R.C.; Gander, J.W.; Wachuta, J.R. Compression strength formula for corrugated boxes. Paperboard Packag. 1963, 48, 149–159. [Google Scholar]
- Allerby, I.M.; Laing, G.N.; Cardwell, R.D. Compressive strength—From components to corrugated containers. Appita Conf. Notes 1985, 1–11. [Google Scholar]
- Batelka, J.J.; Smith, C.N. Package Compression Model; Institute of Paper Science and Technology: Atlanta, GA, USA, 1993. [Google Scholar]
- Urbanik, T.J.; Frank, B. Box compression analysis of world-wide data spanning 46 years. Wood Fiber Sci. 2006, 38, 399–416. [Google Scholar]
- Ristinmaa, M.; Ottosen, N.S.; Korin, C. Analytical Prediction of Package Collapse Loads-Basic considerations. Nord. Pulp Pap. Res. J. 2012, 27, 806–813. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Estimation of the compressive strength of corrugated cardboard boxes with various openings. Energies 2021, 14, 155. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Estimation of the compressive strength of corrugated cardboard boxes with various perforations. Energies 2021, 14, 1095. [Google Scholar] [CrossRef]
- Schrampfer, K.E.; Whitsitt, W.J.; Baum, G.A. Combined Board Edge Crush (ECT) Technology; Institute of Paper Chemistry: Appleton, WI, USA, 1987. [Google Scholar]
- Norstrand, T. On buckling loads for edge-loaded orthotropic plates including transverse shear. Comp. Struct. 2004, 65, 1–6. [Google Scholar] [CrossRef]
- Urbanik, T.J.; Saliklis, E.P. Finite element corroboration of buckling phenomena observed in corrugated boxes. Wood Fiber Sci. 2003, 35, 322–333. [Google Scholar]
- Garbowski, T.; Borysiewicz, A. The Stability of Corrugated Board Packages. Pol. Pap. Rev. 2014, 70, 452–458. (In Polish) [Google Scholar]
- Garbowski, T.; Przybyszewski, G. The Sensitivity Analysis of Critical Force in Box Compression Test. Pol. Pap. Rev. 2015, 71, 275–280. (In Polish) [Google Scholar]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Torsional and transversal stiffness of orthotropic sandwich panels. Materials 2020, 13, 5016. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. The role of buckling in the estimation of compressive strength of corrugated cardboard boxes. Materials 2020, 13, 4578. [Google Scholar] [CrossRef]
- Fadiji, T.; Coetzee, C.J.; Opara, U.L. Compression strength of ventilated corrugated paperboard packages: Numerical modelling, experimental validation and effects of vent geometric design. Biosyst. Eng. 2016, 151, 231–247. [Google Scholar] [CrossRef]
- Garbowski, T.; Jarmuszczak, M. Numerical strength estimate of corrugated board packages. Part 1. Theoretical assumptions in numerical modeling of paperboard packages. Pol. Pap. Rev. 2014, 70, 219–222. (In Polish) [Google Scholar]
- Garbowski, T.; Jarmuszczak, M. Numerical Strength Estimate of Corrugated Board Packages. Part 2. Experimental tests and numerical analysis of paperboard packages. Pol. Pap. Rev. 2014, 70, 277–281. (In Polish) [Google Scholar]
- Fadiji, T.; Ambaw, A.; Coetzee, C.J.; Berry, T.M.; Opara, U.L. Application of finite element analysis to predict the mechanical strength of ventilated corrugated paperboard packaging for handling fresh produce. Biosyst. Eng. 2018, 174, 260–281. [Google Scholar] [CrossRef]
- Suarez, B.; Muneta, M.L.M.; Sanz-Bobi, J.D.; Romero, G. Application of homogenization approaches to the numerical analysis of seating made of multi-wall corrugated cardboard. Compos. Struct. 2021, 262, 113642. [Google Scholar] [CrossRef]
- Simon, J.W. A Review of Recent Trends and Challenges in Computational Modeling of Paper and Paperboard at Different Scales. Arch. Comput. Methods Eng. 2020. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Taylor, R.L. The Finite Element Method for Solid and Structural Mechanics, 6th ed.; Butterworth-Heinemann: Oxford, UK, 2005. [Google Scholar]
- Bathe, K.J.; Dvorkin, E.N. A four node plate bending element based on Mindlin-Reissner plate theory and mixed interpolation. Int. J. Numer. Meth. Eng. 1985, 21, 367–383. [Google Scholar] [CrossRef]
- Dvorkin, E.N.; Bathe, K.J. A continuum mechanics based four node shell element for general non-linear analysis. Eng. Comput. 1984, 1, 77–88. [Google Scholar] [CrossRef]
- MacNeal, R.H. A simple quadrilateral shell element. Comput. Struct. 1978, 8, 175–183. [Google Scholar] [CrossRef]
- MacNeal, R.H. Finite Elements: Their Design and Performance; Marcel Dekker: New York, NY, USA, 1994. [Google Scholar]
- Donea, J.; Lamain, L.G. A modified representation of transverse shear in C0 quadrilateral plate elements. Comput. Methods Appl. Mech. Eng. 1987, 63, 183–207. [Google Scholar] [CrossRef]
- Onate, E.; Castro, J. Derivation of plate elements based on assumed shear strain fields. In Recent Advances on Computational Structural Mechanics; Ladeveze, P., Zienkiewicz, O.C., Eds.; Elsevier Pub: Amsterdam, The Netherlands, 1991. [Google Scholar]
- Hohe, J. A direct homogenization approach for determination of the stiffness matrix for microheterogeneous plates with application to sandwich panels. Compos. Part B 2003, 34, 615–626. [Google Scholar] [CrossRef]
- Buannic, N.; Cartraud, P.; Quesnel, T. Homogenization of corrugated core sandwich panels. Comp. Struct. 2003, 59, 299–312. [Google Scholar] [CrossRef]
- Biancolini, M.E. Evaluation of equivalent stiffness properties of corrugated board. Comp. Struct. 2005, 69, 322–328. [Google Scholar] [CrossRef]
- Garbowski, T.; Jarmuszczak, M. Homogenization of corrugated paperboard. Part 1. Analytical homogenization. Pol. Pap. Rev. 2014, 70, 345–349. (In Polish) [Google Scholar]
- Garbowski, T.; Jarmuszczak, M. Homogenization of corrugated paperboard. Part 2. Numerical homogenization. Pol. Pap. Rev. 2014, 70, 390–394. (In Polish) [Google Scholar]
- Marek, A.; Garbowski, T. Homogenization of sandwich panels. Comput. Assist. Methods Eng. Sci. 2015, 22, 39–50. [Google Scholar]
- Garbowski, T.; Marek, A. Homogenization of corrugated boards through inverse analysis. In Proceedings of the 1st International Conference on Engineering and Applied Sciences Optimization, Kos Island, Greece, 4–6 June 2014; pp. 1751–1766. [Google Scholar]
- Nordstrand, T.; Carlsson, L. Evaluation of transverse shear stiffness of structural core sandwich plates. Comp. Struct. 1997, 37, 145–153. [Google Scholar] [CrossRef]
- Nordstrand, T. Basic Testing and Strength Design of Corrugated Board and Containers. Ph.D. Thesis, Lund University, Lund, Sweden, 2003. [Google Scholar]
- Avilés, F.; Carlsson, L.A.; May-Pat, A. A shear-corrected formulation of the sandwich twist specimen. Exp. Mech. 2012, 52, 17–23. [Google Scholar] [CrossRef]
- Garbowski, T.; Gajewski, T.; Grabski, J.K. Role of transverse shear modulus in the performance of corrugated materials. Materials 2020, 13, 3791. [Google Scholar] [CrossRef] [PubMed]
- Richardson, M.O.W.; Wisheart, M.J. Review of low-velocity impact properties of composite materials. Compos. Part A Appl. Sci. Manuf. 1996, 27, 1123–1131. [Google Scholar] [CrossRef]
- Zangana, S.; Epaarachchi, J.; Ferdous, W.; Leng, J. A novel hybridised composite sandwich core with Glass, Kevlar and Zylon fibres—Investigation under low-velocity impact. Int. J. Impact Eng. 2020, 137, 103430. [Google Scholar] [CrossRef]




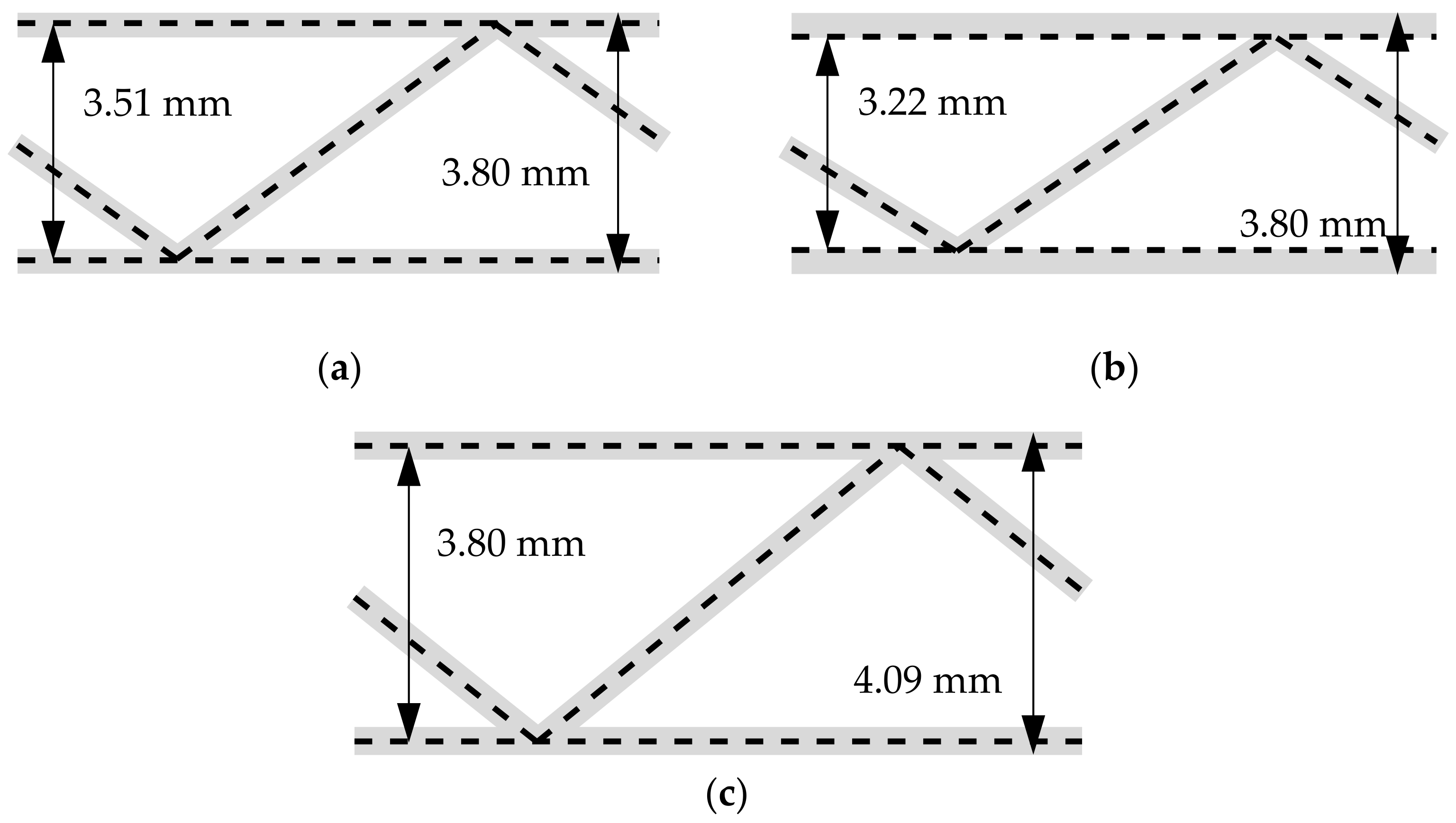



| Layers | (mm) | (MPa) | (MPa) | (-) | (MPa) | (MPa) | (MPa) |
|---|---|---|---|---|---|---|---|
| liners | 0.29 | 3326 | 1694 | 0.34 | 859 | 429.5 | 429.5 |
| fluting | 0.30 | 2614 | 1532 | 0.32 | 724 | 362 | 362 |
| Stiffness | Ref. [30] | Axial Geometry | Inner Geometry | Outer Geometry |
|---|---|---|---|---|
| 2158 | 2140 | 2154 | 2131 | |
| 1660 | 1665 | 1643 | 1687 | |
| 379.9 | 382.9 | 385.4 | 381.9 | |
| 677.6 | 662.5 | 668.4 | 656.8 | |
| 6.370 | 6.392 | 6.389 | 7.482 | |
| 3.824 | 3.859 | 3.740 | 4.549 | |
| 1.092 | 1.115 | 1.113 | 1.305 | |
| 1.655 | 1.656 | 1.639 | 1.937 | |
| , | - | 202.4 | 179.4 | 218.5 |
| , | - | 99.0 | 89.0 | 112.4 |
| Stiffness | Quadrilateral Element (S4) | Reduced Quadrilateral Element (S4R) | Triangular Element (S3) | QLLL Element |
|---|---|---|---|---|
| 2219 | 2218 | 2225 | 2128 | |
| 1694 | 1694 | 1694 | 1677 | |
| 411.8 | 411.5 | 413.4 | 378.9 | |
| 659.3 | 659.3 | 659.6 | 659.7 | |
| 6.521 | 6.517 | 6.535 | 6.443 | |
| 4.071 | 4.066 | 4.091 | 4.035 | |
| 1.149 | 1.148 | 1.152 | 1.135 | |
| 1.729 | 1.728 | 1.731 | 1.716 | |
| , | 140.5 | 139.8 | 143.8 | 71.1 |
| , | 132.6 | 132.4 | 135.6 | 102.4 |
| nodes/element | 969/896 | 969/896 | 969/1792 | 969/896 |
| Stiffness | Unsymmetric 8 Segments | Unsymmetric 16 Segments | Unsymmetric 32 Segments | Symmetric 8 Segments | Symmetric 16 Segments | Symmetric 32 Segments |
|---|---|---|---|---|---|---|
| 2128 | 2108 | 2106 | 2126 | 2114 | 2107 | |
| 1677 | 1681 | 1682 | 1678 | 1681 | 1682 | |
| 378.9 | 373.7 | 373.4 | 380.4 | 375.9 | 373.7 | |
| 659.7 | 658.7 | 658.3 | 659.6 | 658.4 | 658.1 | |
| 6.443 | 6.433 | 6.432 | 6.445 | 6.435 | 6.429 | |
| 4.035 | 4.087 | 4.101 | 4.033 | 4.086 | 4.099 | |
| 1.135 | 1.130 | 1.130 | 1.137 | 1.131 | 1.129 | |
| 1.715 | 1.728 | 1.732 | 1.682 | 1.694 | 1.698 | |
| , | 71.1 | 48.0 | 43.1 | 75.0 | 49.0 | 42.5 |
| , | 102.4 | 104.4 | 104.7 | 113.4 | 114.4 | 114.6 |
| Stiffness | Unsymmetric 1 Period | Unsymmetric 2 Periods | Unsymmetric 3 Periods | Symmetric 1 Period | Symmetric 2 Periods | Symmetric 3 Periods |
|---|---|---|---|---|---|---|
| 2108 | 2106 | 2106 | 2114 | 2110 | 2108 | |
| 1681 | 1680 | 1680 | 1681 | 1681 | 1681 | |
| 373.7 | 373.4 | 373.3 | 375.9 | 374.5 | 374.0 | |
| 658.7 | 658.5 | 658.4 | 658.4 | 658.4 | 658.4 | |
| 6.433 | 6.445 | 6.458 | 6.435 | 6.428 | 6.426 | |
| 4.087 | 4.085 | 4.085 | 4.086 | 4.085 | 4.084 | |
| 1.130 | 1.129 | 1.129 | 1.131 | 1.129 | 1.128 | |
| 1.728 | 1.713 | 1.710 | 1.694 | 1.694 | 1.694 | |
| , | 48.0 | 45.9 | 45.1 | 49.0 | 46.4 | 45.4 |
| , | 104.4 | 102.8 | 102.3 | 114.4 | 107.8 | 105.6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Garbowski, T.; Gajewski, T. Determination of Transverse Shear Stiffness of Sandwich Panels with a Corrugated Core by Numerical Homogenization. Materials 2021, 14, 1976. https://doi.org/10.3390/ma14081976
Garbowski T, Gajewski T. Determination of Transverse Shear Stiffness of Sandwich Panels with a Corrugated Core by Numerical Homogenization. Materials. 2021; 14(8):1976. https://doi.org/10.3390/ma14081976
Chicago/Turabian StyleGarbowski, Tomasz, and Tomasz Gajewski. 2021. "Determination of Transverse Shear Stiffness of Sandwich Panels with a Corrugated Core by Numerical Homogenization" Materials 14, no. 8: 1976. https://doi.org/10.3390/ma14081976
APA StyleGarbowski, T., & Gajewski, T. (2021). Determination of Transverse Shear Stiffness of Sandwich Panels with a Corrugated Core by Numerical Homogenization. Materials, 14(8), 1976. https://doi.org/10.3390/ma14081976







