Stochastic Model for Energy Propagation in Disordered Granular Chains
Abstract
1. Introduction
2. Granular Chain Model
2.1. Linearized Equation of Motion
2.2. Neglect of Contact Damping
2.3. Implication of Mass-Disorder in a Monodisperse Chain
2.4. Linear Model Solution
2.5. Impulse Propagation Condition
2.6. Standing Wave Condition
2.7. Mass Disorder/Disorder Parameter () and Ensembles
3. Energy Evolution and the Master Equation Model
3.1. Energy Conservation
3.2. Total Energy in the Wavenumber Domain
3.3. Binning Energy
3.4. Stochastic Master Equation
3.5. Computing the Elements of Matrix
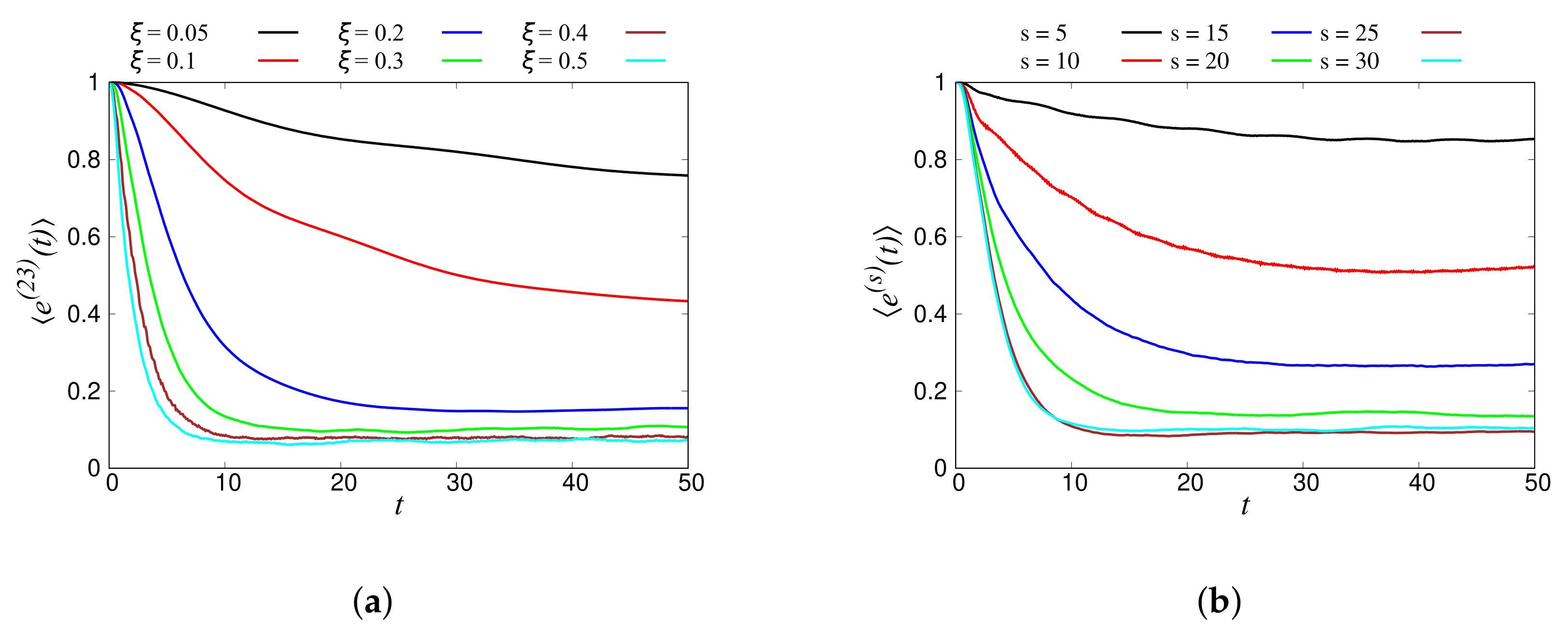
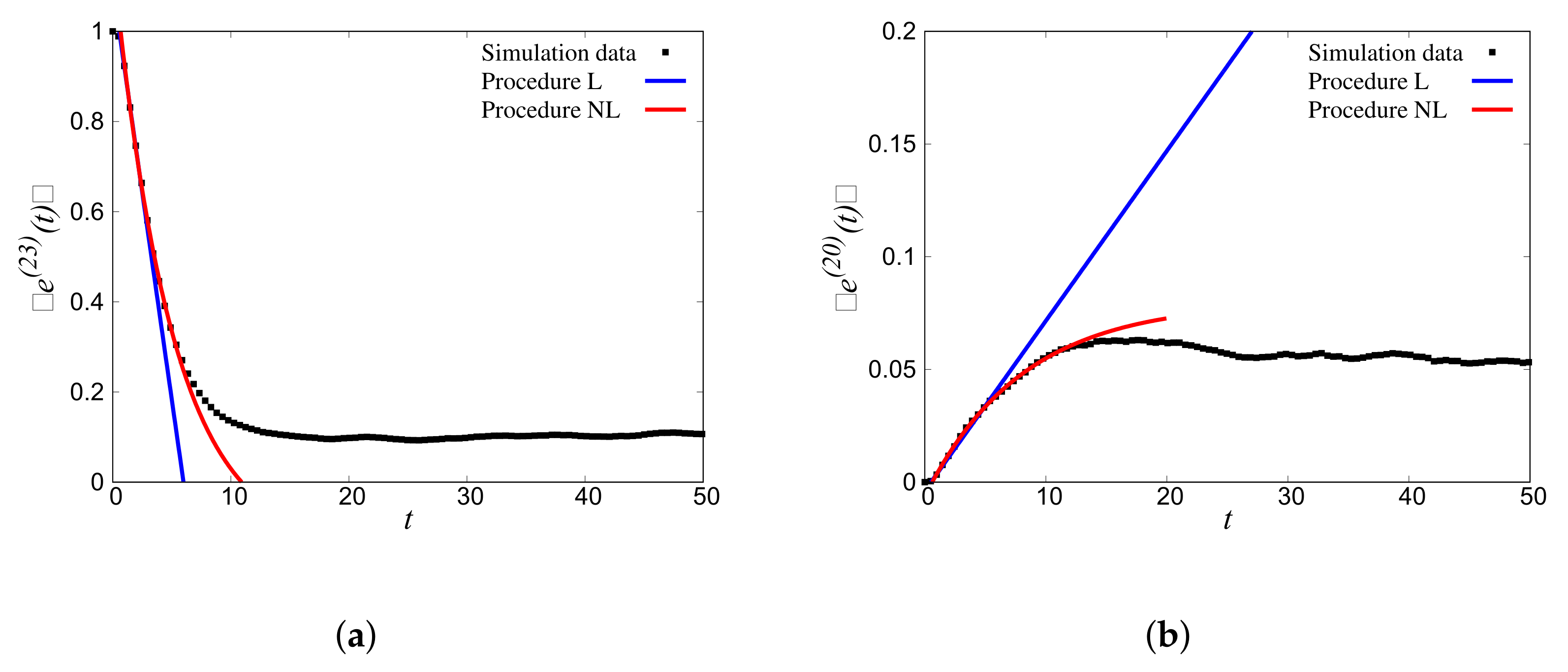
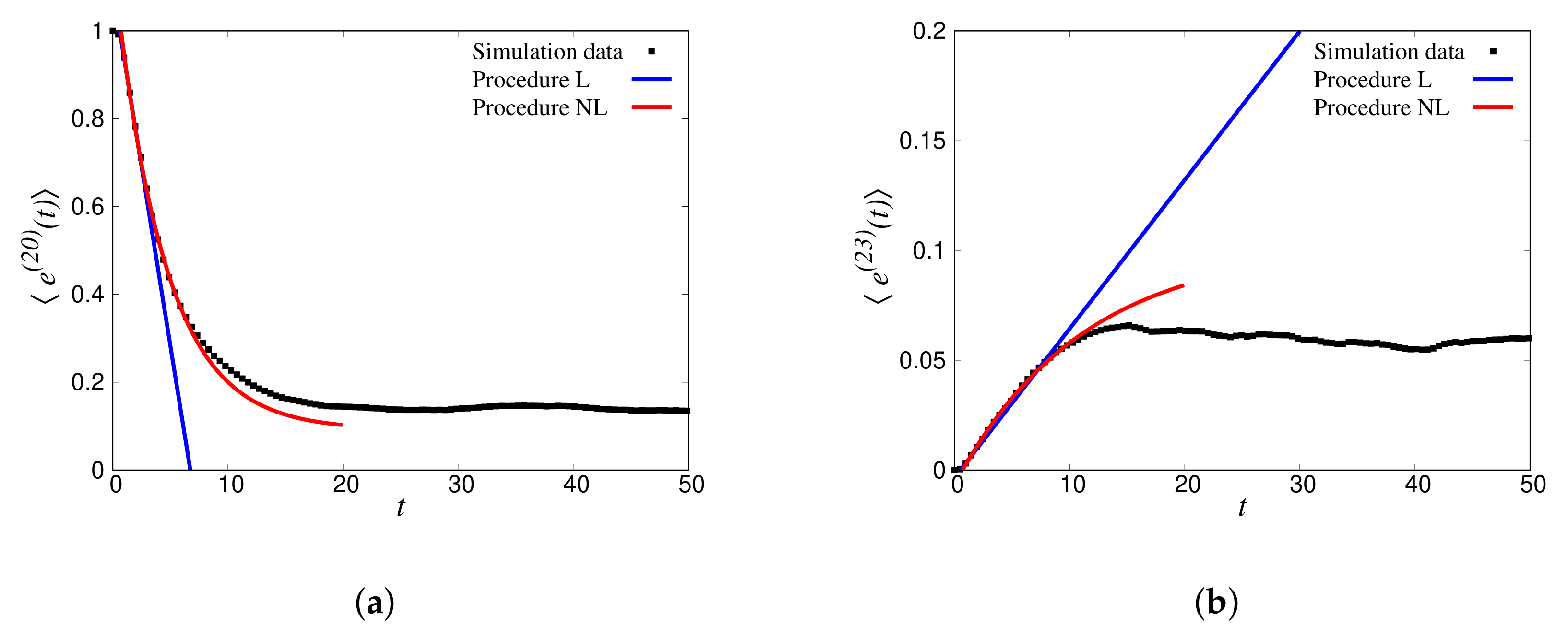
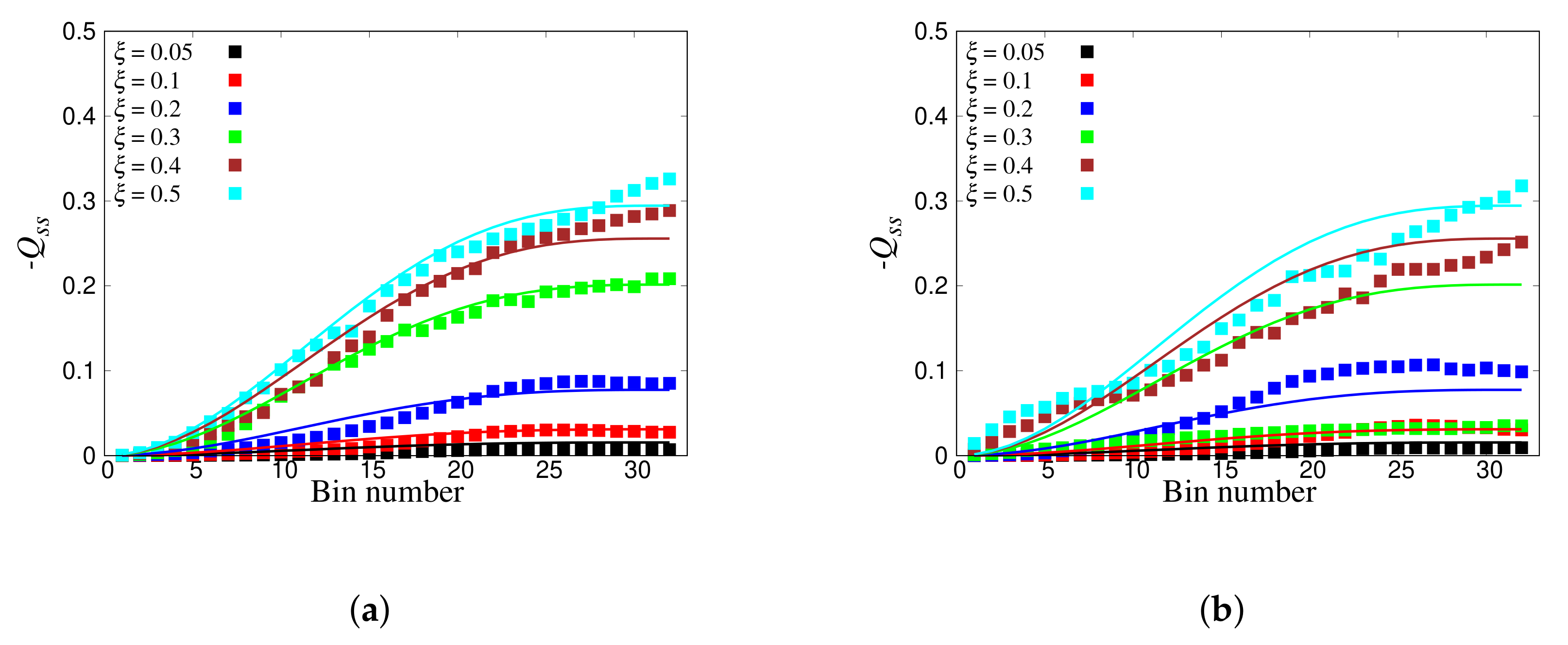
4. Results and Discussion
4.1. Energy Propagation with Distance
4.1.1. First Particle Excitation
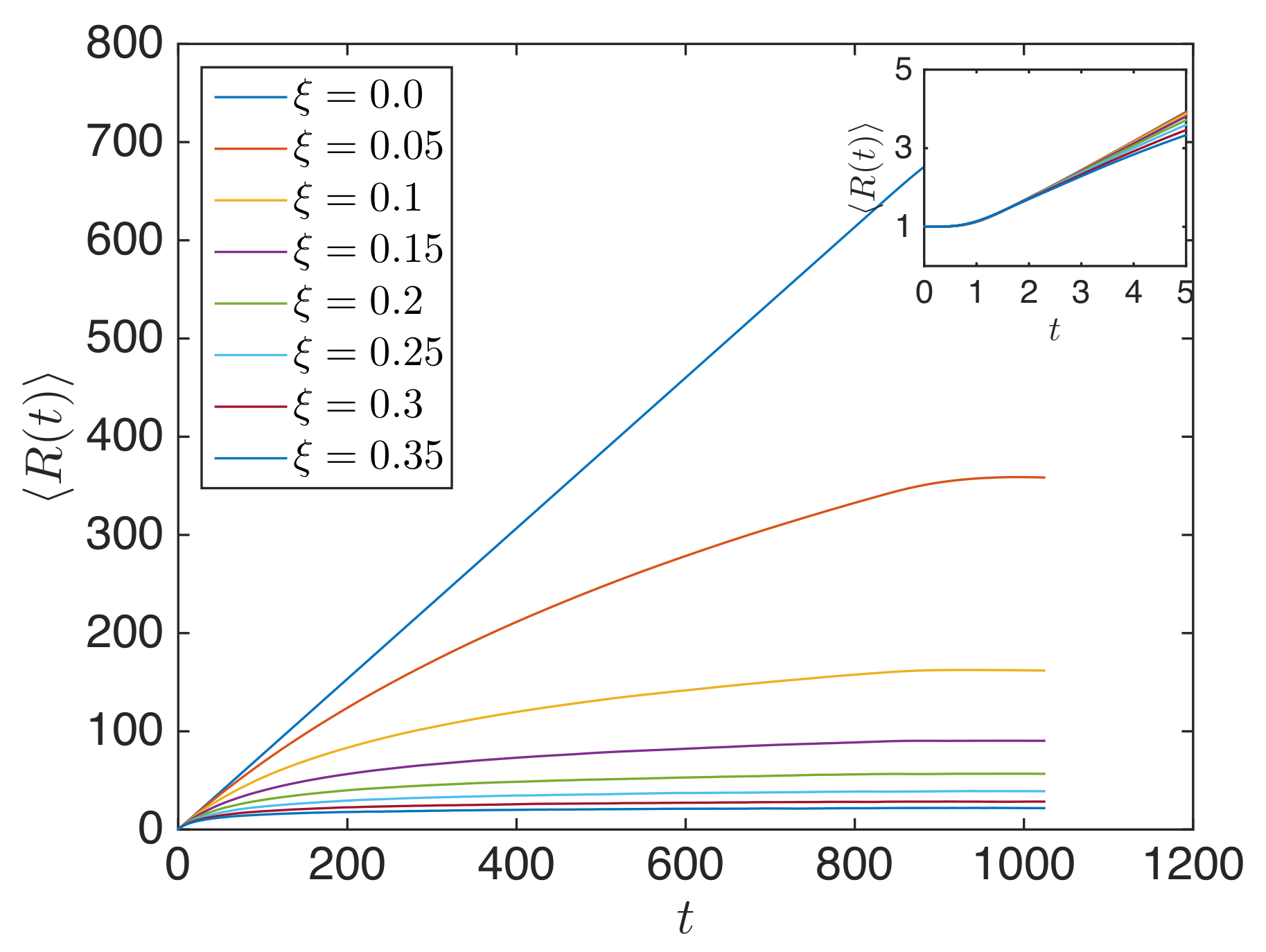
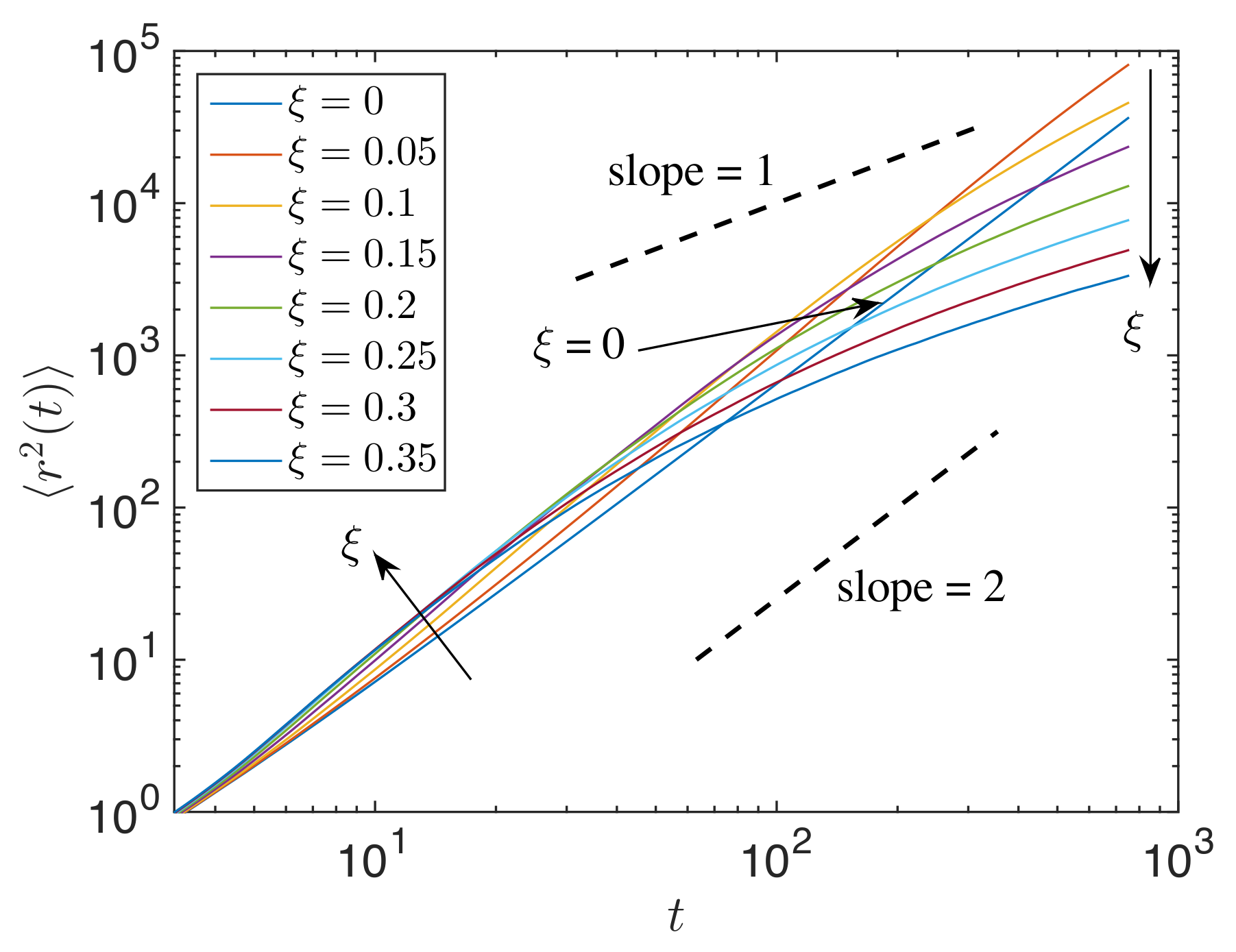
4.1.2. Diffusion
4.1.3. Center Particle Excitation
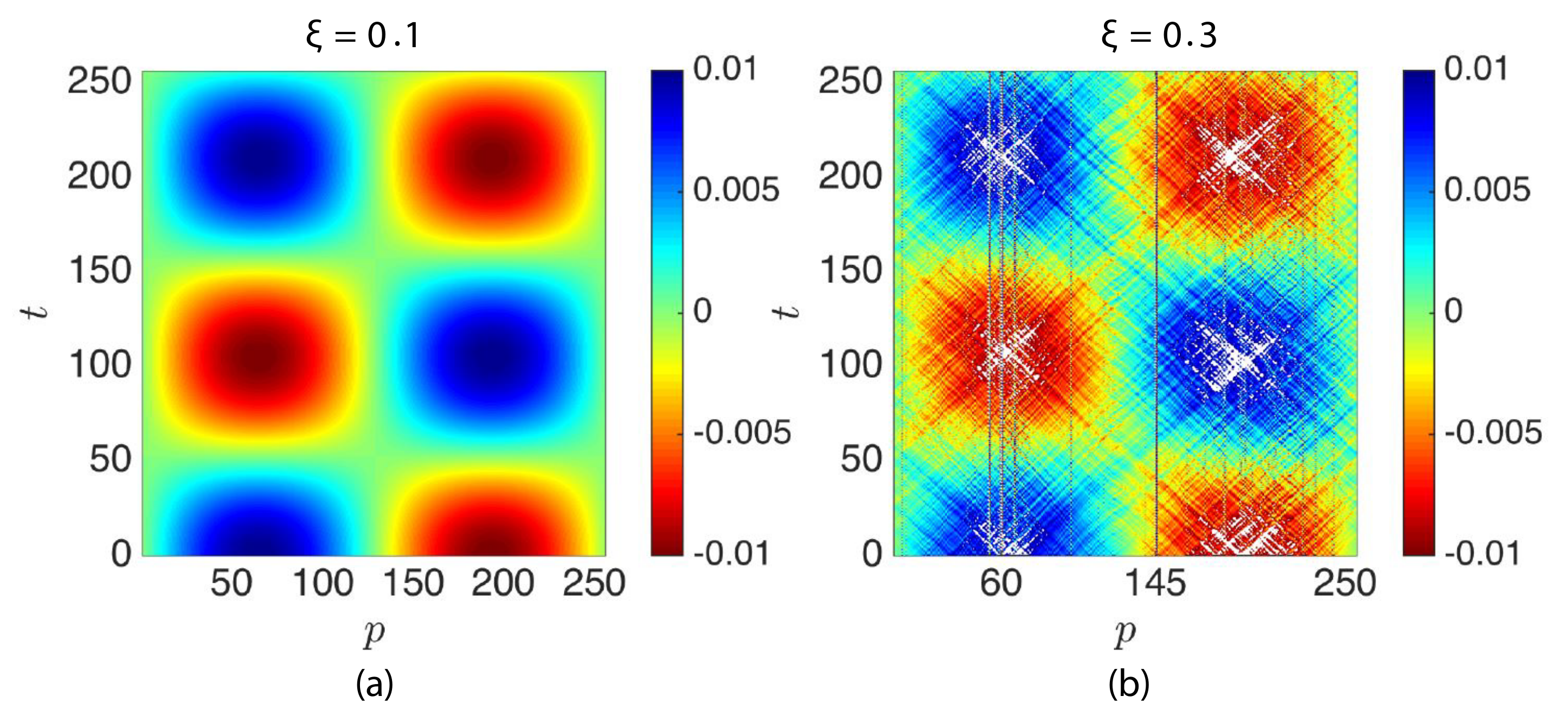
4.2. Energy Propagation in Space and Time
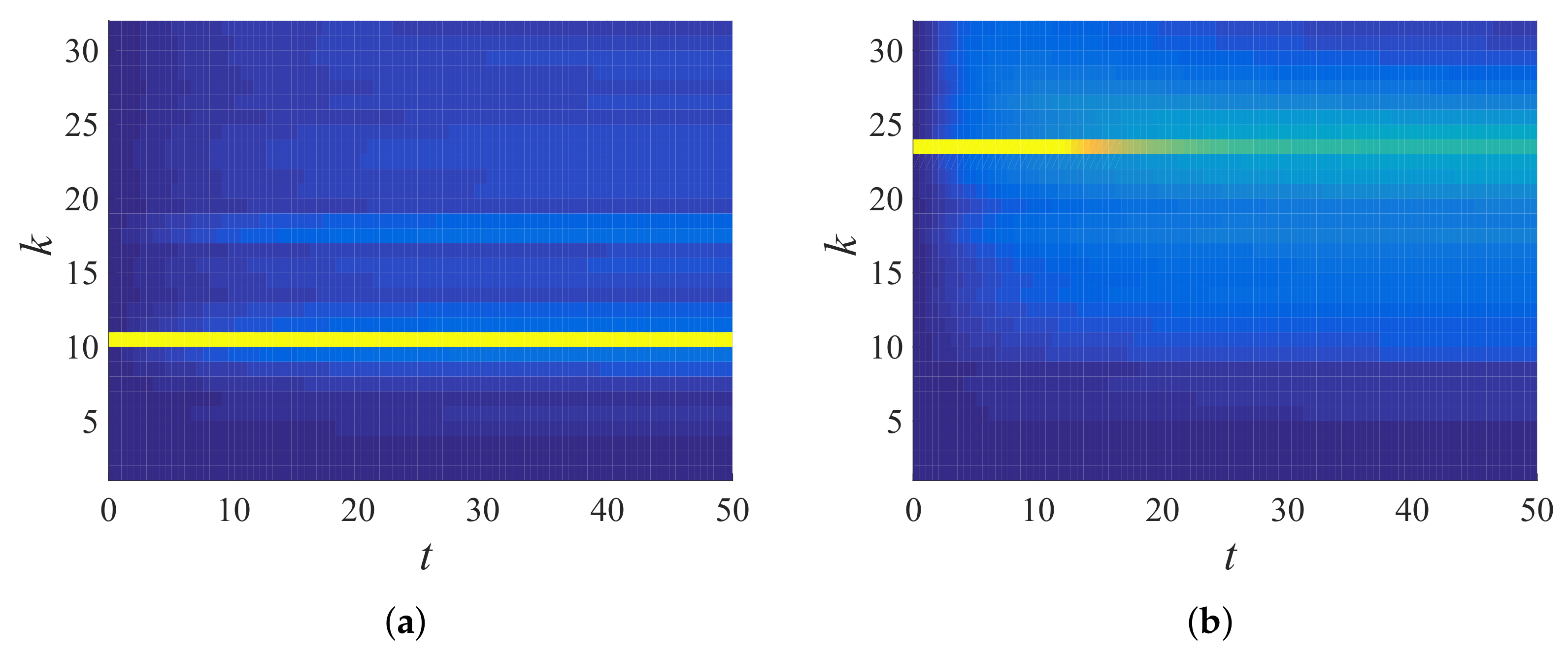
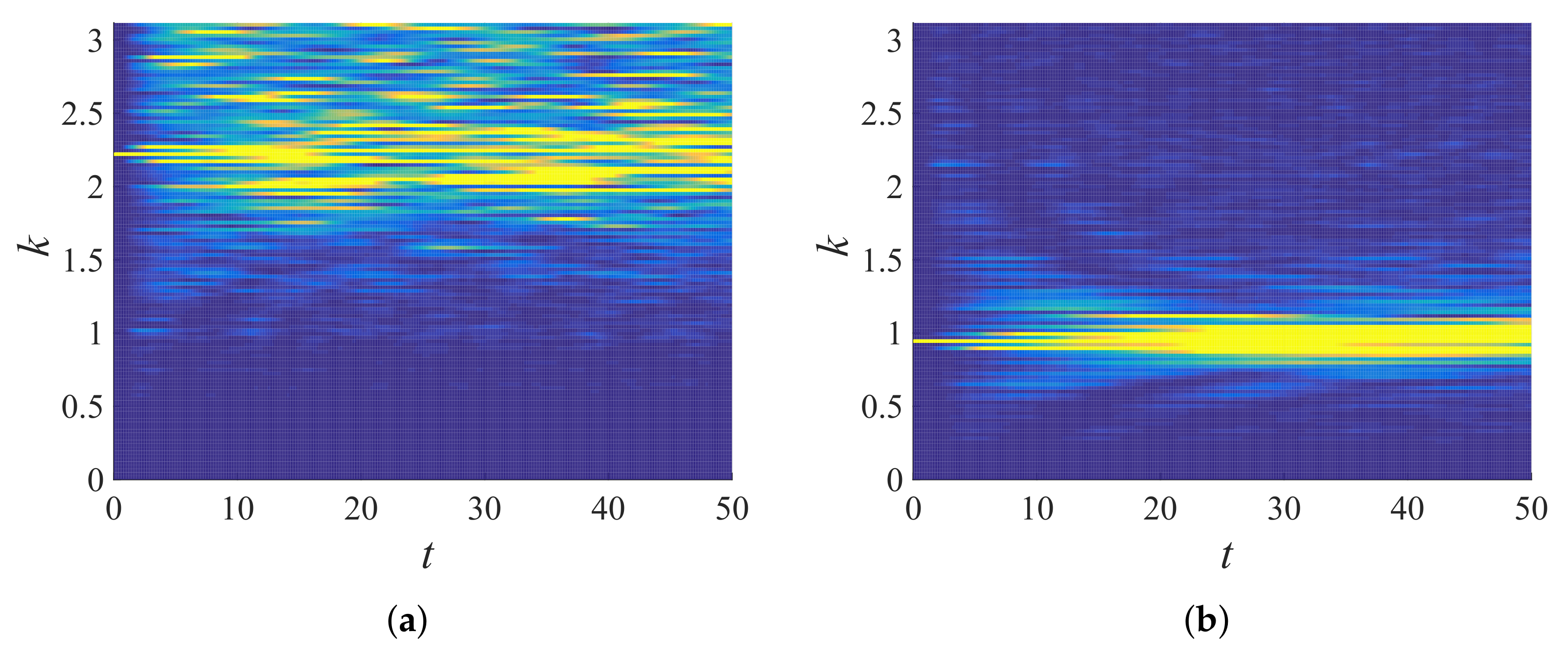
4.3. Energy Propagation Across Wave Numbers
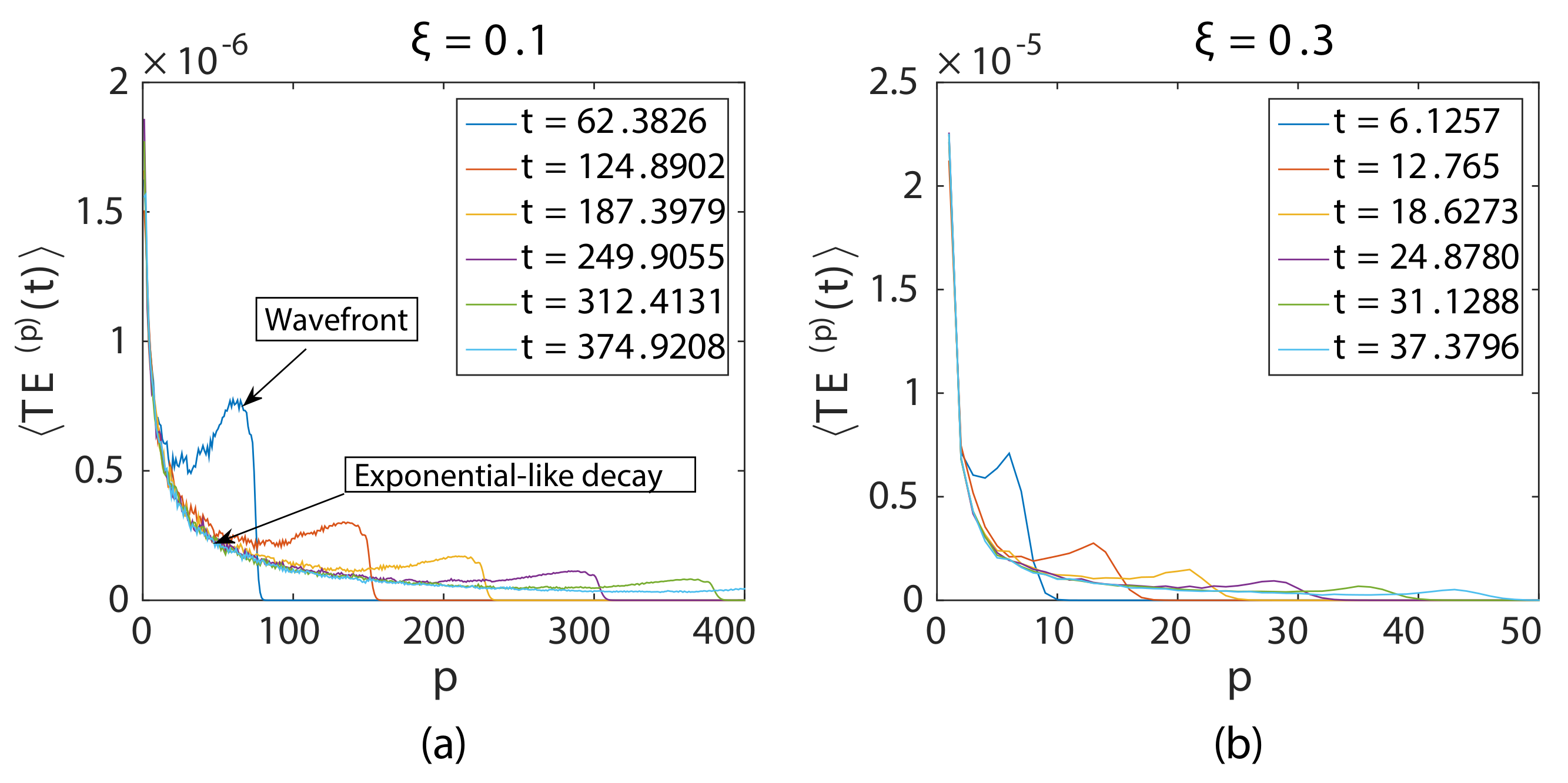
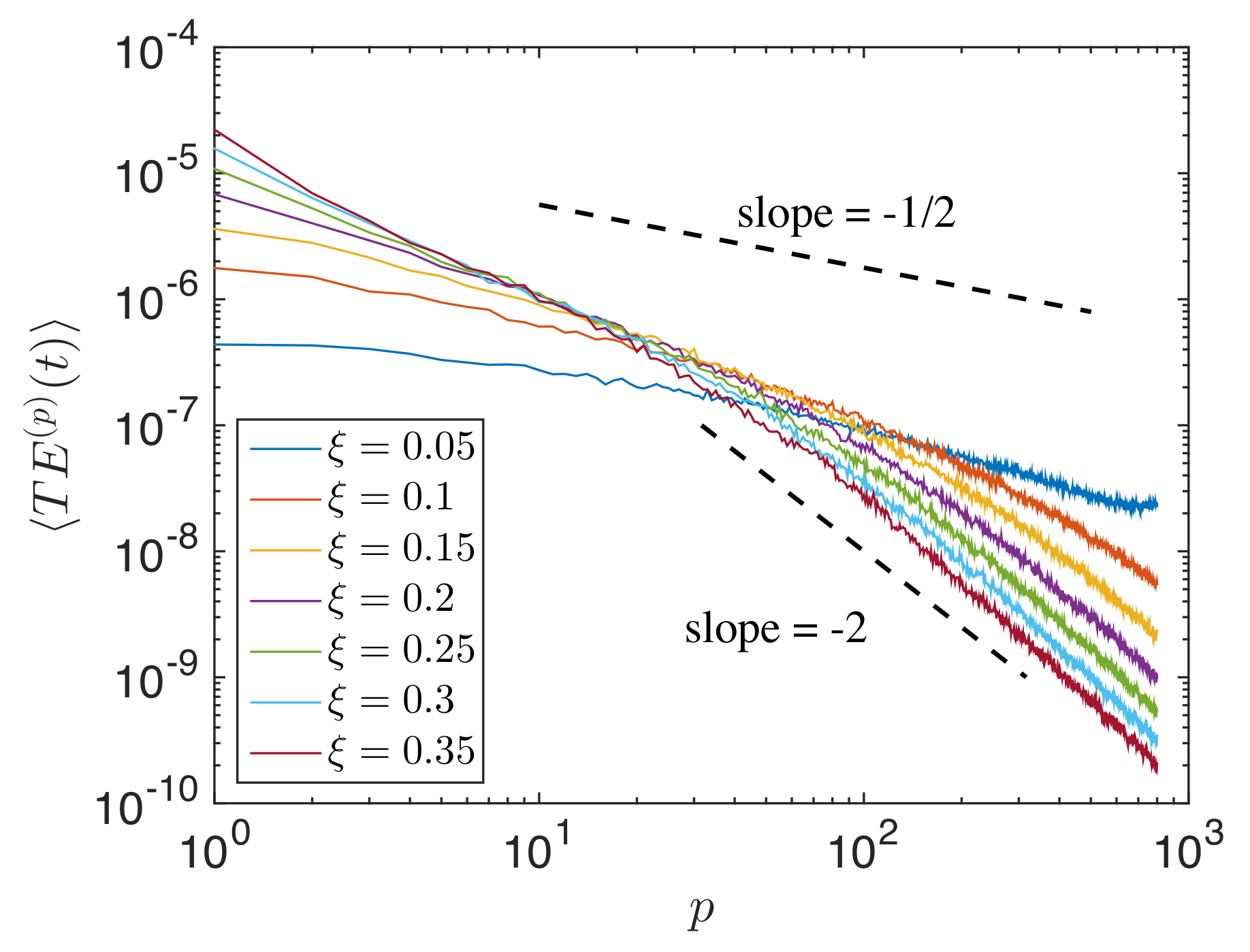
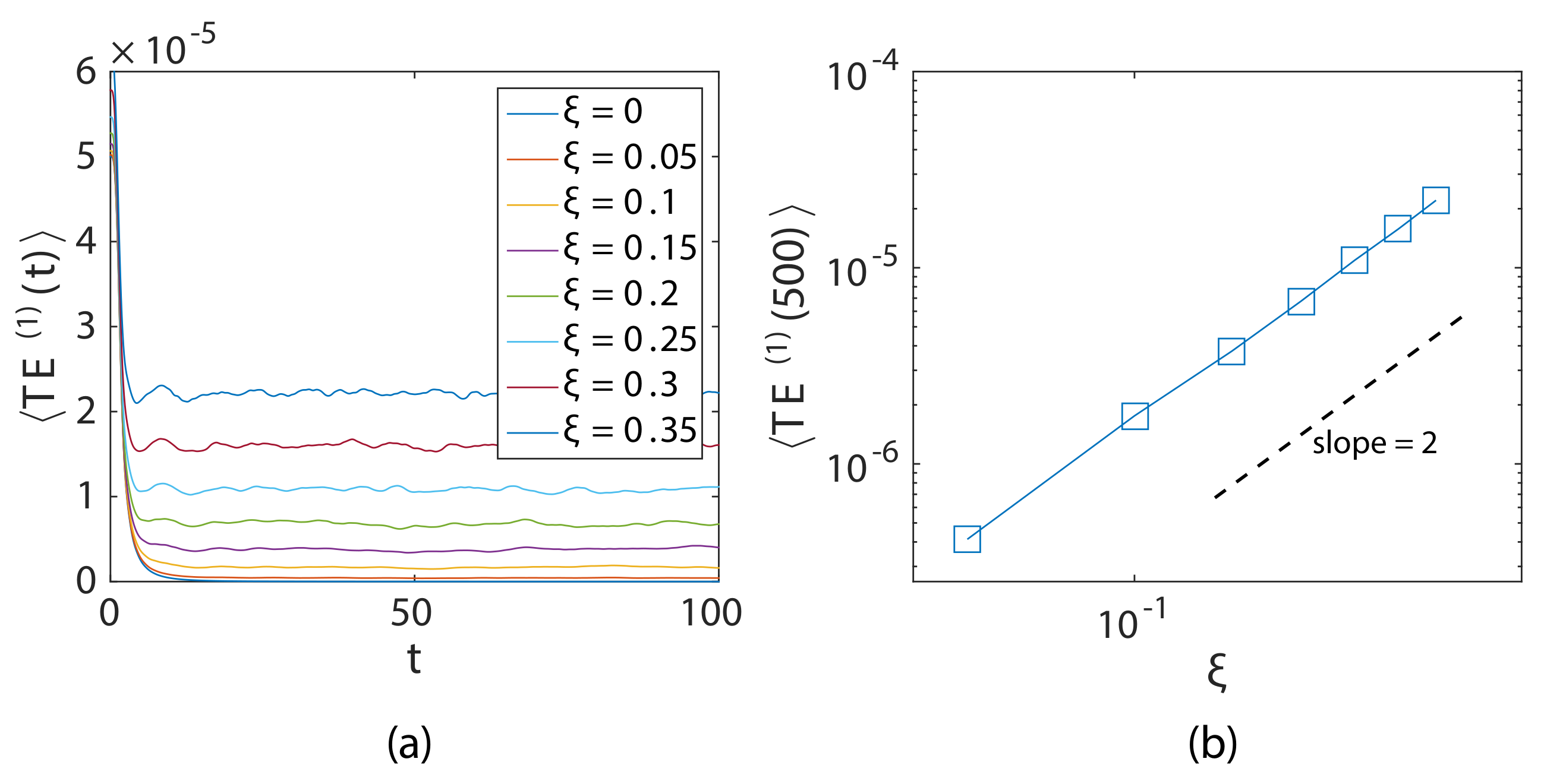
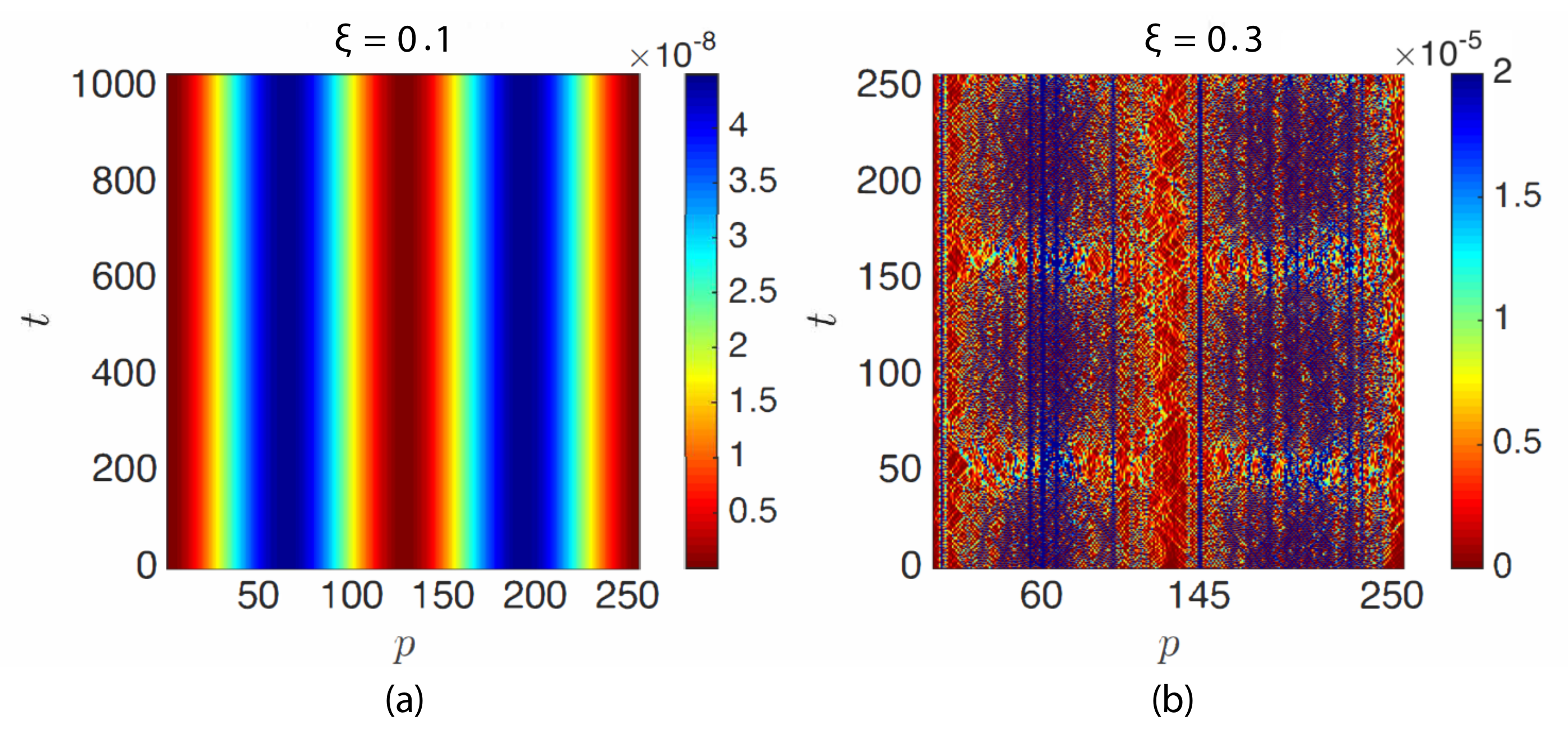
4.4. Attenuation and Energy Transfer
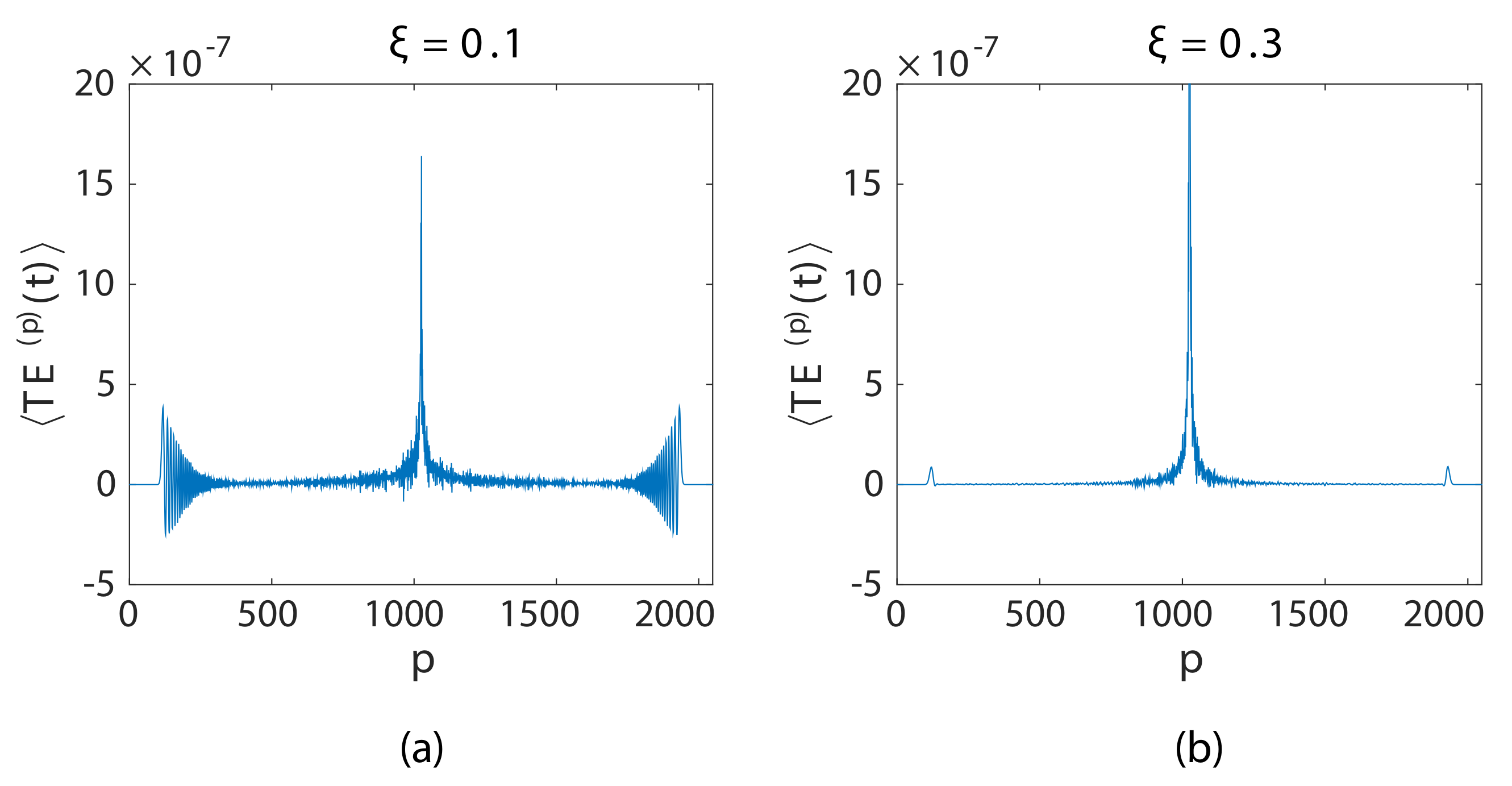
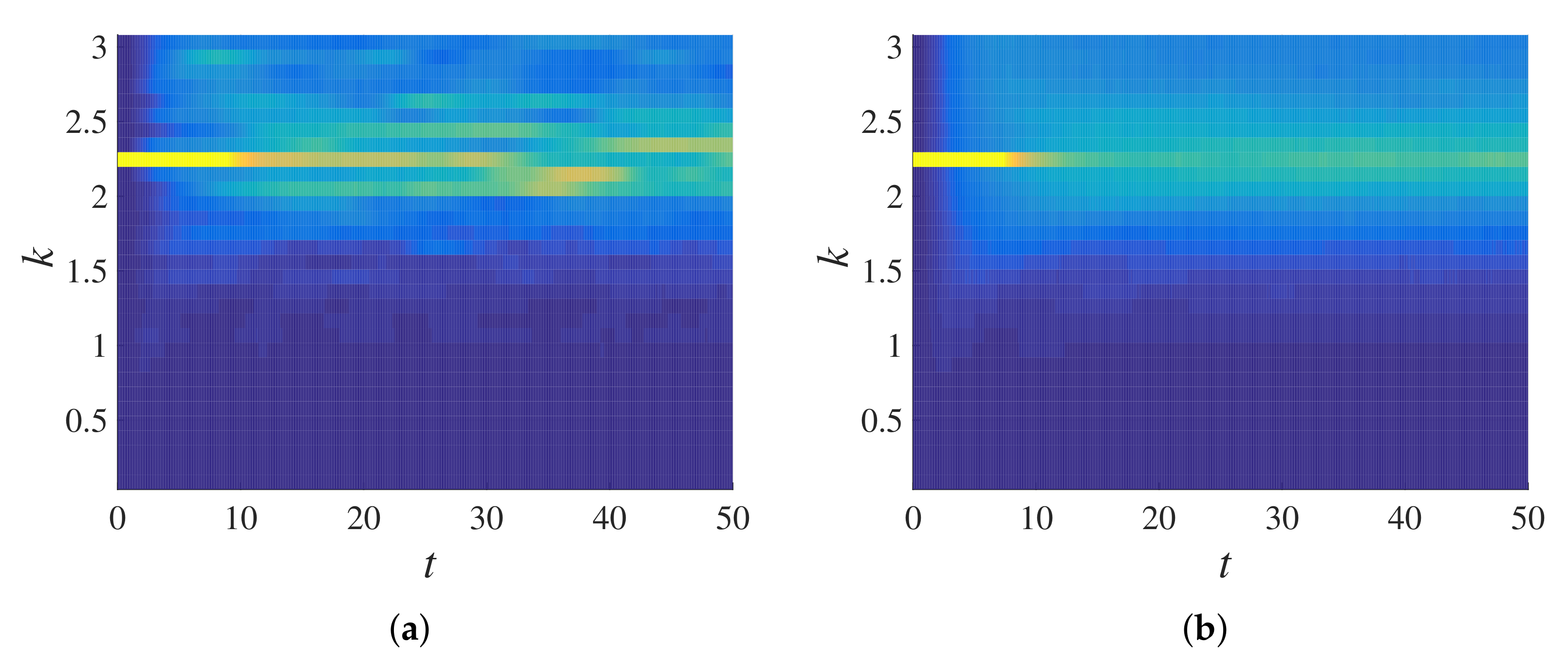
4.5. Stochastic Modeling—Energy Propagation in the Wavenumber
5. Conclusions
- Impulse initial conditions for studying energy propagation with distance (Section 2.5 and Section 4.1).
- Standing wave initial conditions for studying energy evolution in time by transfer across wavenumbers (Section 2.6, Section 4.2, Section 4.3, Section 4.4).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
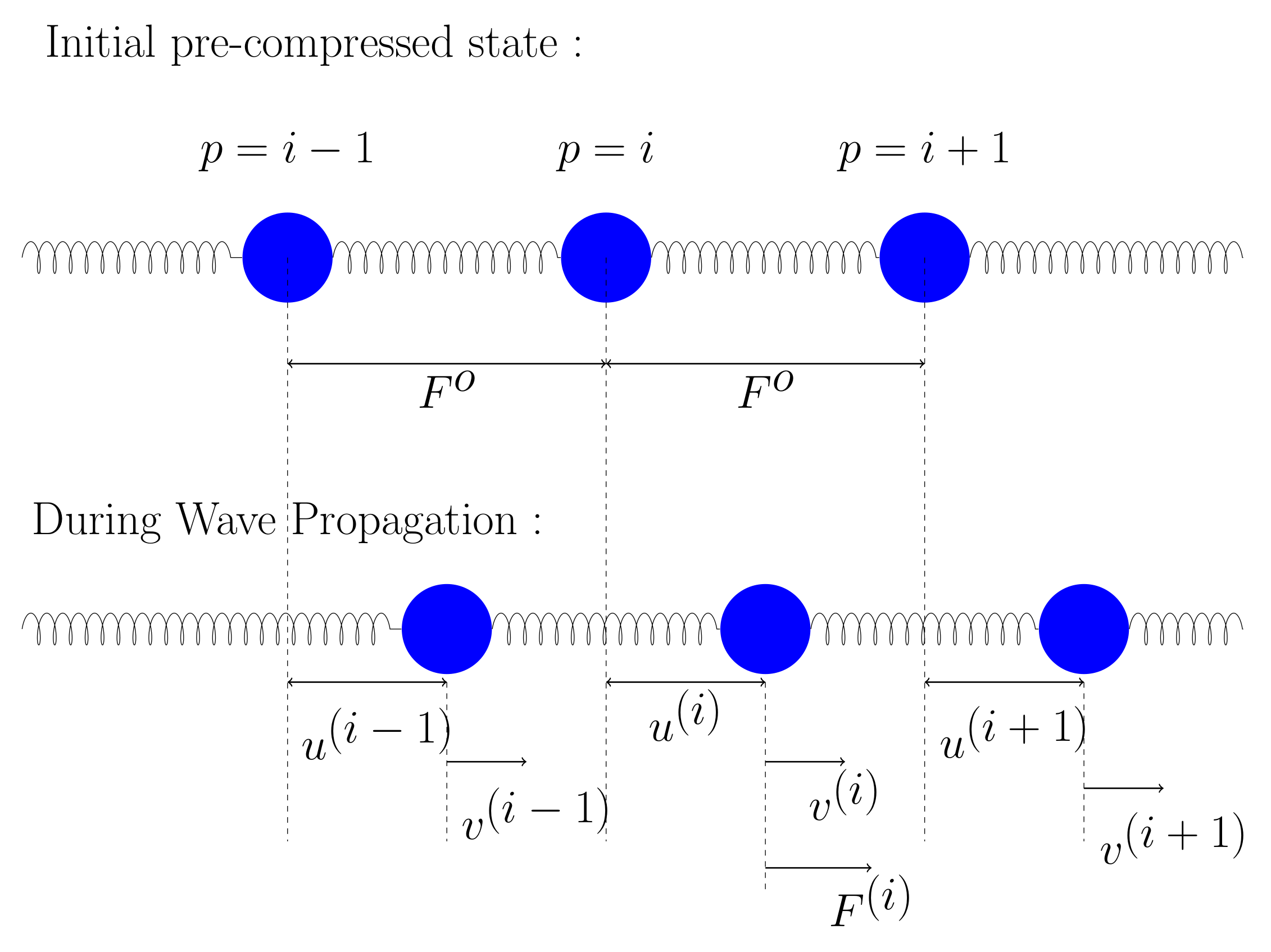
Appendix A.1. Kinetic Energy
Appendix A.2. Potential Energy
References
- Mouraille, O.; Luding, S. Sound wave propagation in weakly polydisperse granular materials. Ultrasonics 2008, 48, 498–505. [Google Scholar] [CrossRef]
- Coste, C.; Gilles, B. Sound propagation in a constrained lattice of beads: High-frequency behavior and dispersion relation. Phys. Rev. E 2008, 77, 021302. [Google Scholar] [CrossRef]
- Lawney, B.P.; Luding, S. Mass–Disorder Effects on the Frequency Filtering in One–Dimensional Discrete Particle Systems. In AIP Conference Proceedings; AIP: New York, NY, USA, 2013; Volume 1542, pp. 535–538. [Google Scholar]
- Lawney, B.P.; Luding, S. Frequency filtering in disordered granular chains. Acta Mech. 2014, 225, 2385–2407. [Google Scholar] [CrossRef][Green Version]
- Mouraille, O.; Luding, S. Mechanic Waves in Sand: Effect of Polydispersity. In Partec; University of Erlangen-Nuremberg, Institute of Particle Technology: Nuremberg, Germany, 2007; Volume 2007. [Google Scholar]
- Scales, J.A.; Van Vleck, E.S. Lyapunov exponents and localization in randomly layered media. J. Comput. Phys. 1997, 133, 27–42. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, W.; Lambros, J.; Bergman, L.A.; Vakakis, A.F. Pulse transmission and acoustic non-reciprocity in a granular channel with symmetry-breaking clearances. Granul. Matter 2020, 22, 1–16. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P.G. Quantitative Seismology; University Science Books: New York, NY, USA, 2002. [Google Scholar]
- Sato, H.; Fehler, M.C.; Maeda, T. Seismic Wave Propagation and Scattering in the Heterogeneous Earth; Springer: Berlin, Germany, 2012; Volume 496. [Google Scholar]
- Sheng, P. Introduction to Wave Scattering, Localization and Mesoscopic Phenomena; Springer Science & Business Media: Berlin, Germany, 2006; Volume 88. [Google Scholar]
- Tournat, V.; Gusev, V. Acoustics of unconsolidated “model” granular media: An overview of recent results and several open problems. Acta Acust. United Acust. 2010, 96, 208–224. [Google Scholar] [CrossRef]
- Aki, K.; Chouet, B. Origin of coda waves: Source, attenuation, and scattering effects. J. Geophys. Res. 1975, 80, 3322–3342. [Google Scholar] [CrossRef]
- Weaver, R.L.; Sachse, W. Diffusion of ultrasound in a glass bead slurry. J. Acoust. Soc. Am. 1995, 97, 2094–2102. [Google Scholar] [CrossRef]
- Güven, I.; Luding, S.; Steeb, H. Incoherent waves in fluid-saturated sintered granular systems: Scattering phenomena. J. Vib. Acoust. 2018, 140, 011018. [Google Scholar] [CrossRef]
- Page, J. Ultrasonic wave transport in strongly scattering media. Nano Opt. At. 2011, 173, 75–93. [Google Scholar]
- Trujillo, L.; Peniche, F.; Jia, X. Multiple Scattering of Elastic Waves in Granular Media: Theory and Experiments. In Waves in Fluids and Solids; IntechOpen: Rijeka, Croatia, 2011; Chapter 5. [Google Scholar]
- Daraio, C.; Nesterenko, V.; Herbold, E.; Jin, S. Energy trapping and shock disintegration in a composite granular medium. Phys. Rev. Lett. 2006, 96, 058002. [Google Scholar] [CrossRef] [PubMed]
- Shearer, P.M. Introduction to Seismology; Cambridge University Press: Cambridge, UK, 2019. [Google Scholar]
- Wu, R.S.; Aki, K. Scattering and Attenuation of Seismic Waves, Part II; Birkhäuser: Basel, Switzerland, 1989. [Google Scholar]
- Bacigalupo, A.; Gambarotta, L. Dispersive wave propagation in two-dimensional rigid periodic blocky materials with elastic interfaces. J. Mech. Phys. Solids 2017, 102, 165–186. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, J. Tunable traveling wave properties in one-dimensional chains composed from hollow cylinders: From compression to rarefaction waves. Int. J. Mech. Sci. 2020, 191, 106073. [Google Scholar] [CrossRef]
- Kawahara, J. Scattering attenuation of elastic waves due to low-contrast inclusions. Wave Motion 2011, 48, 290–300. [Google Scholar] [CrossRef]
- Yang, J.; Gonzalez, M.; Kim, E.; Agbasi, C.; Sutton, M. Attenuation of solitary waves and localization of breathers in 1D granular crystals visualized via high speed photography. Exp. Mech. 2014, 54, 1043–1057. [Google Scholar] [CrossRef]
- Misra, A.; Nejadsadeghi, N. Longitudinal and transverse elastic waves in 1D granular materials modeled as micromorphic continua. Wave Motion 2019, 90, 175–195. [Google Scholar] [CrossRef]
- Achilleos, V.; Theocharis, G.; Skokos, C. Energy transport in one-dimensional disordered granular solids. Phys. Rev. E 2016, 93, 022903. [Google Scholar] [CrossRef] [PubMed]
- Van Der Baan, M. Acoustic wave propagation in one dimensional random media: The wave localization approach. Geophys. J. Int. 2001, 145, 631–646. [Google Scholar] [CrossRef]
- Allen, P.B.; Kelner, J. Evolution of a vibrational wave packet on a disordered chain. Am. J. Phys. 1998, 66, 497–506. [Google Scholar] [CrossRef]
- Ostojic, S.; Somfai, E.; Nienhuis, B. Scale invariance and universality of force networks in static granular matter. Nature 2006, 439, 828. [Google Scholar] [CrossRef]
- Weaver, R.L. Information from Seismic Noise. Science 2005, 307, 1568–1569. [Google Scholar] [CrossRef] [PubMed]
- Merkel, A.; Tournat, V.; Gusev, V. Dispersion of elastic waves in three-dimensional noncohesive granular phononic crystals: Properties of rotational modes. Phys. Rev. E 2010, 82, 031305. [Google Scholar] [CrossRef]
- Van den Wildenberg, S.; van Hecke, M.; Jia, X. Evolution of granular packings by nonlinear acoustic waves. EPL Europhys. Lett. 2013, 101, 14004. [Google Scholar] [CrossRef]
- Gilles, B.; Coste, C. Low-frequency behavior of beads constrained on a lattice. Phys. Rev. Lett. 2003, 90, 174302. [Google Scholar] [CrossRef]
- Brillouin, L. Wave Propagation in Periodic Structures: Electric Filters and Crystal Lattices; Dover: New York, NY, USA, 1953; Volume 2. [Google Scholar]
- Kondic, L. Energy propagation through dense granular systems. Granul. Matter 2019, 21, 85. [Google Scholar] [CrossRef]
- Agnolin, I.; Roux, J.N. Internal states of model isotropic granular packings. I. Assembling process, geometry, and contact networks. Phys. Rev. E 2007, 76, 61302. [Google Scholar] [CrossRef]
- Makse, H.; Gland, N.; Johnson, D.; Schwartz, L. Why effective medium theory fails in granular materials. Phys. Rev. Lett. 1999, 83, 5070. [Google Scholar] [CrossRef]
- Cundall, P.A.; Strack, O.D. A discrete numerical model for granular assemblies. Geotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Luding, S. Introduction to discrete element methods: Basic of contact force models and how to perform the micro-macro transition to continuum theory. Eur. J. Environ. Civ. Eng. 2008, 12, 785–826. [Google Scholar] [CrossRef]
- Marketos, G.; O’Sullivan, C. A micromechanics-based analytical method for wave propagation through a granular material. Soil Dyn. Earthq. Eng. 2013, 45, 25–34. [Google Scholar] [CrossRef]
- Zhang, Y.; McFarland, D.M.; Vakakis, A.F. Propagating discrete breathers in forced one-dimensional granular networks: Theory and experiment. Granul. Matter 2017, 19, 59. [Google Scholar] [CrossRef]
- Göncü, F.; Luding, S. Effect of particle friction and polydispersity on the macroscopic stress–strain relations of granular materials. Acta Geotech. 2013, 8, 629–643. [Google Scholar] [CrossRef]
- Taghizadeh, K. Elasticity and Wave Propagation in Granular Materials. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2019. [Google Scholar] [CrossRef]
- Hiraiwa, M.; Wallen, S.; Boechler, N. Acoustic wave propagation in disordered microscale granular media under compression. Granul. Matter 2017, 19, 62. [Google Scholar] [CrossRef]
- Potekin, R.; McFarland, D.M.; Vakakis, A.F. Nonlinear wave scattering at the flexible interface of a granular dimer chain. Granul. Matter 2016, 18, 68. [Google Scholar] [CrossRef]
- Sears, F.M.; Bonner, B.P. Ultrasonic attenuation measurement by spectral ratios utilizing signal processing techniques. IEEE Trans. Geosci. Remote Sens. 1981, 19, 95–99. [Google Scholar] [CrossRef]
- Van Kampen, N.G. Stochastic Processes in Physics and Chemistry; Elsevier: Amsterdam, The Netherlands, 1992; Volume 1. [Google Scholar]
- Manjunath, M.; Awasthi, A.P.; Geubelle, P.H. Wave propagation in random granular chains. Phys. Rev. E 2012, 85, 031308. [Google Scholar] [CrossRef]
- Sen, S.; Manciu, M.; Sinkovits, R.S.; Hurd, A.J. Nonlinear acoustics in granular assemblies. Granul. Matter 2001, 3, 33–39. [Google Scholar]
- Nesterenko, V. Propagation of nonlinear compression pulses in granular media. J. Appl. Mech. Techn. Phys. 1983, 24, 733–743. [Google Scholar] [CrossRef]
- Shrivastava, K.; Luding, S. Effect of disorder on bulk sound wave speed: A multiscale spectral analysis. Nonlinear Process. Geophys. 2017, 24, 435. [Google Scholar] [CrossRef]
- Zhang, W.; Xu, J. Universal design law of equivalent systems for Nesterenko solitary waves transmission. Granul. Matter 2020, 22, 1–12. [Google Scholar] [CrossRef]
- Géradin, M.; Rixen, D.J. Mechanical Vibrations: Theory and Application to Structural Dynamics; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar]
- Landau, L.D.; Lifshitz, E.M. Theory of Elasticity; Pergamon Press: Oxford, UK, 1970; Volume 7. [Google Scholar]
- Schreck, C.F.; O’Hern, C.S.; Shattuck, M.D. Vibrations of jammed disk packings with Hertzian interactions. Granul. Matter 2014, 16, 209–216. [Google Scholar] [CrossRef]
- Zeravcic, Z.; Lohse, D.; Van Saarloos, W. Collective oscillations in bubble clouds. J. Fluid Mech. 2011, 680, 114. [Google Scholar] [CrossRef][Green Version]
- Kruyt, N. Micromechanical study of dispersion and damping characteristics of granular materials. J. Mech. Mater. Struct. 2012, 7, 347–361. [Google Scholar] [CrossRef]
- Shrivastava, R.K.; Luding, S. Wave Propagation of Spectral Energy Content in a Granular Chain. In EPJ Web of Conferences; Powders & Grains 2017: Montpellier, France, 2017; Volume 140, p. 02023. [Google Scholar]
- Pillage, L.T.; Rohrer, R.A. Asymptotic waveform evaluation for timing analysis. IEEE Trans. Comput. Aided Des. Integr. Circuits Syst. 1990, 9, 352–366. [Google Scholar] [CrossRef]
- Liang, Y.; Lee, H.; Lim, S.; Lin, W.; Lee, K.; Wu, C. Proper orthogonal decomposition and its applications—Part I: Theory. J. Sound Vib. 2002, 252, 527–544. [Google Scholar] [CrossRef]
- Liang, Y.; Lin, W.; Lee, H.; Lim, S.; Lee, K.; Sun, H. Proper orthogonal decomposition and its applications—Part II: Model reduction for MEMS dynamical analysis. J. Sound Vib. 2002, 256, 515–532. [Google Scholar] [CrossRef]
- Rayleigh, J.W.S.B. The Theory of Sound; Macmillan: New York, NY, USA, 1896; Volume 2. [Google Scholar]
- Kerschen, G.; Golinval, J.C.; Vakakis, A.F.; Bergman, L.A. The method of proper orthogonal decomposition for dynamical characterization and order reduction of mechanical systems: An overview. Nonlinear Dyn. 2005, 41, 147–169. [Google Scholar] [CrossRef]
- Tournat, V.; Gusev, V.E.; Castagnède, B. Self-demodulation of elastic waves in a one-dimensional granular chain. Phys. Rev. E 2004, 70, 056603. [Google Scholar] [CrossRef]
- Mouraille, O. Sound Propagation in Dry Granular Materials: Discrete Element Simulations, Theory, and Experiments. Ph.D. Thesis, University of Twente, Enschede, The Netherlands, 2009. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taghizadeh, K.; Shrivastava, R.K.; Luding, S. Stochastic Model for Energy Propagation in Disordered Granular Chains. Materials 2021, 14, 1815. https://doi.org/10.3390/ma14071815
Taghizadeh K, Shrivastava RK, Luding S. Stochastic Model for Energy Propagation in Disordered Granular Chains. Materials. 2021; 14(7):1815. https://doi.org/10.3390/ma14071815
Chicago/Turabian StyleTaghizadeh, Kianoosh, Rohit Kumar Shrivastava, and Stefan Luding. 2021. "Stochastic Model for Energy Propagation in Disordered Granular Chains" Materials 14, no. 7: 1815. https://doi.org/10.3390/ma14071815
APA StyleTaghizadeh, K., Shrivastava, R. K., & Luding, S. (2021). Stochastic Model for Energy Propagation in Disordered Granular Chains. Materials, 14(7), 1815. https://doi.org/10.3390/ma14071815