Numerically Exploring the Potential of Abating the Energy Flow Peaks through Tough, Single Network Hydrogel Vibration Isolators with Chemical and Physical Cross-Links †
Abstract
1. Introduction
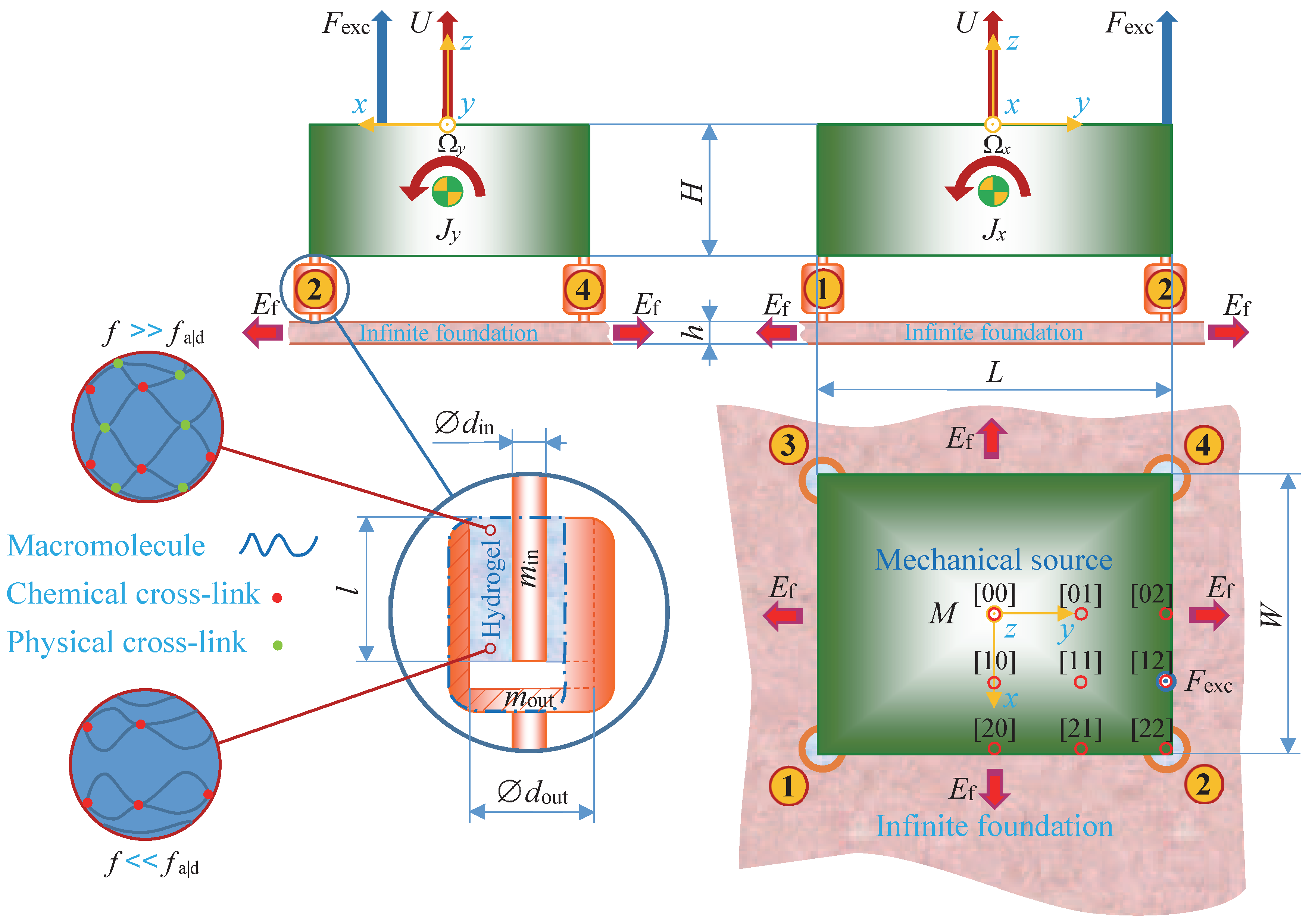
2. Materials and Methods
3. Results and Discussion
3.1. Source, Vibration Isolator Bushing, Foundation and Material Parameters
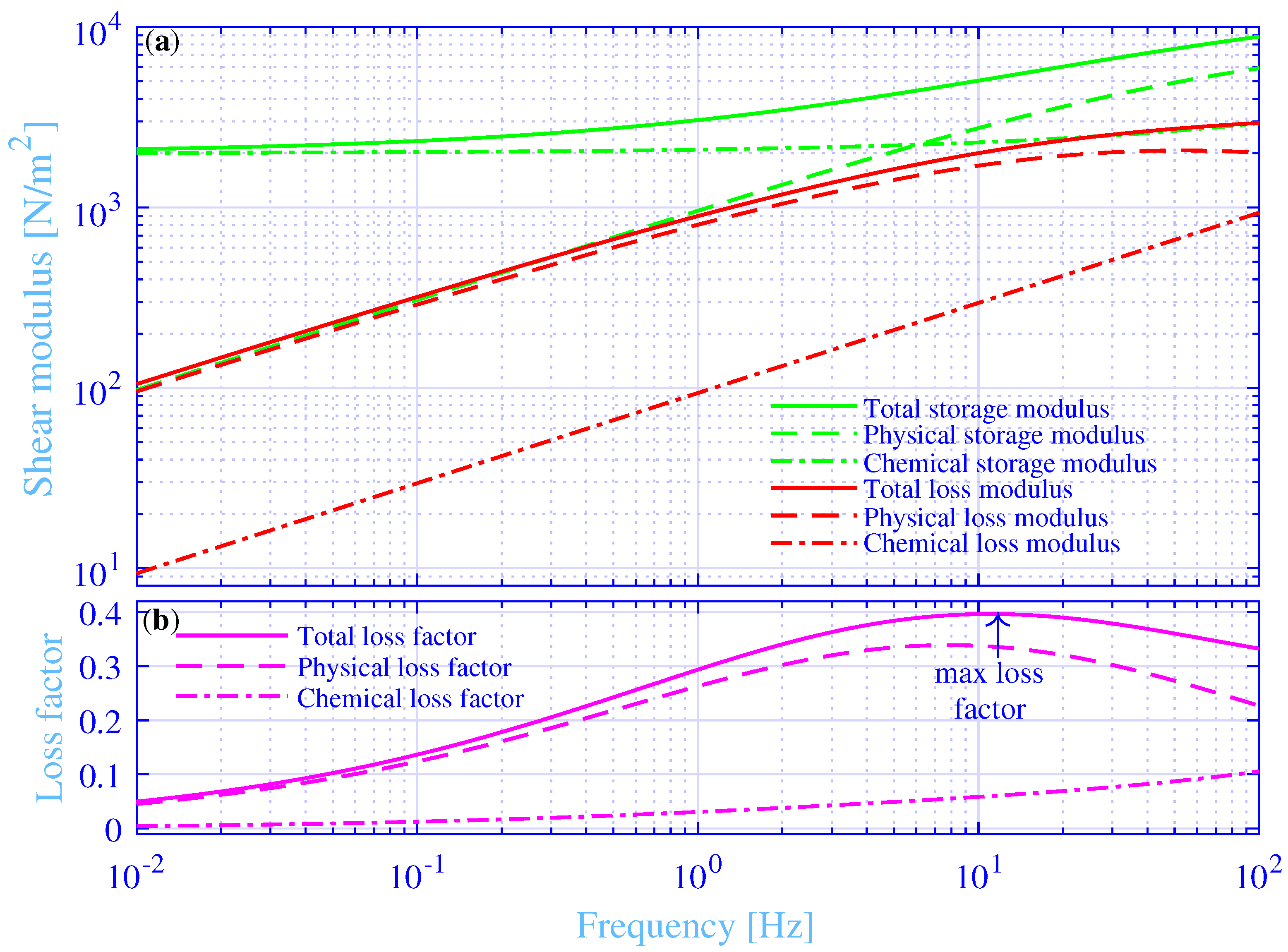
3.2. Hydrogel Shear Modulus
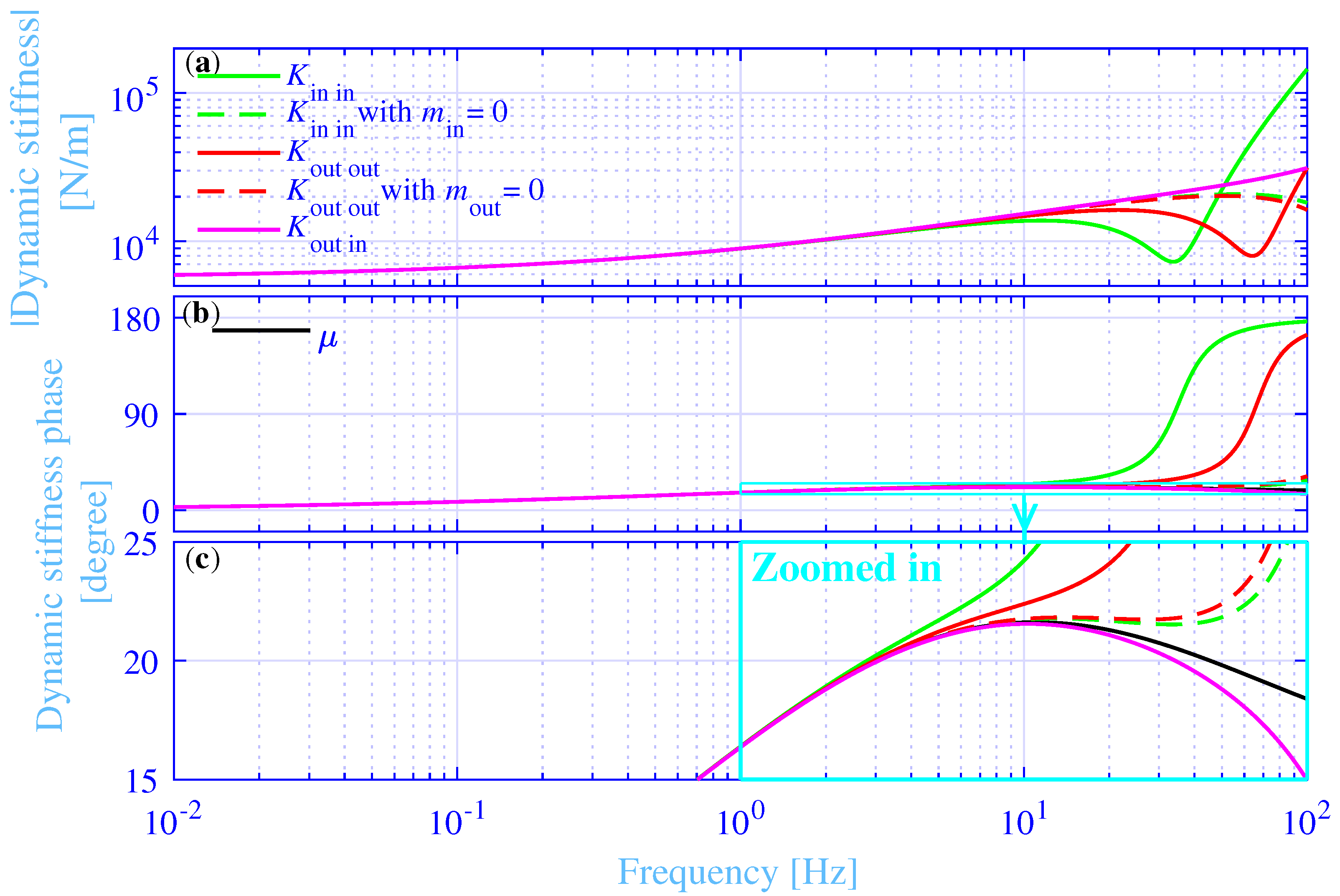
3.3. Dynamic Stiffness
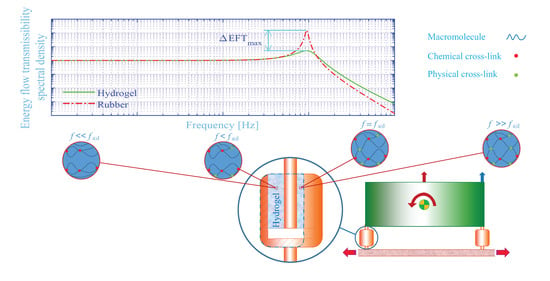
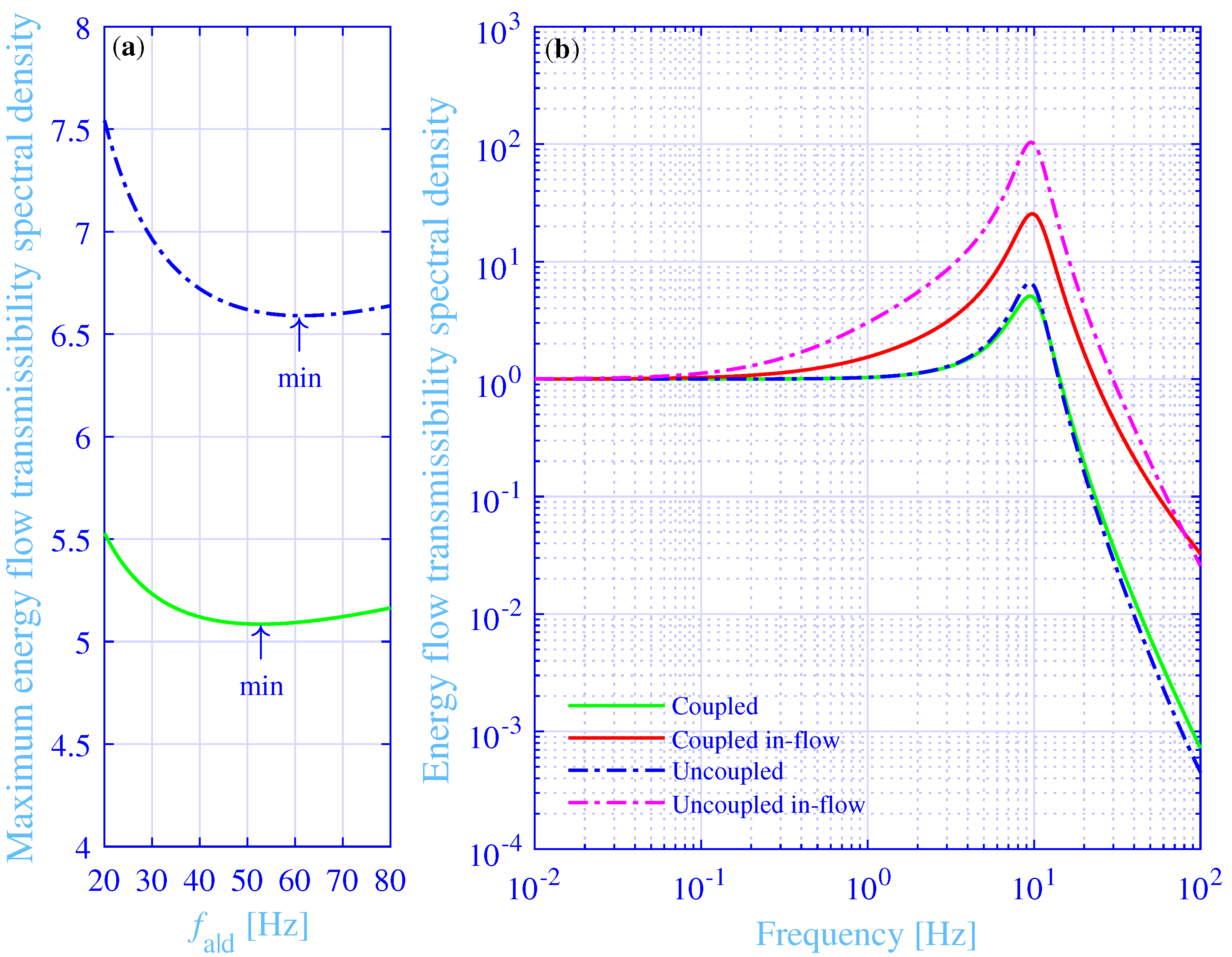
3.4. Energy Flow Transmissibility
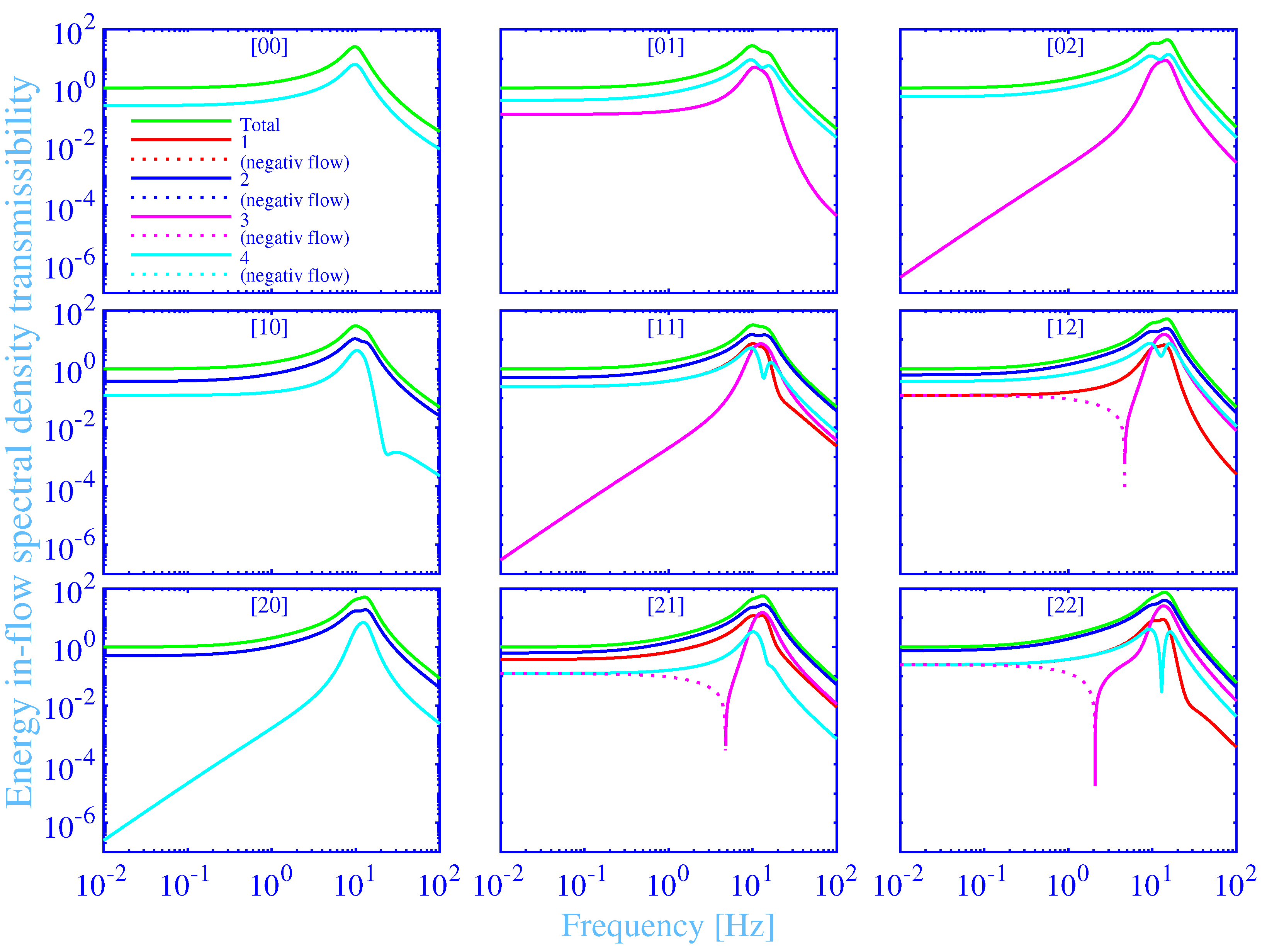
3.5. Energy In-Flow Transmissibility
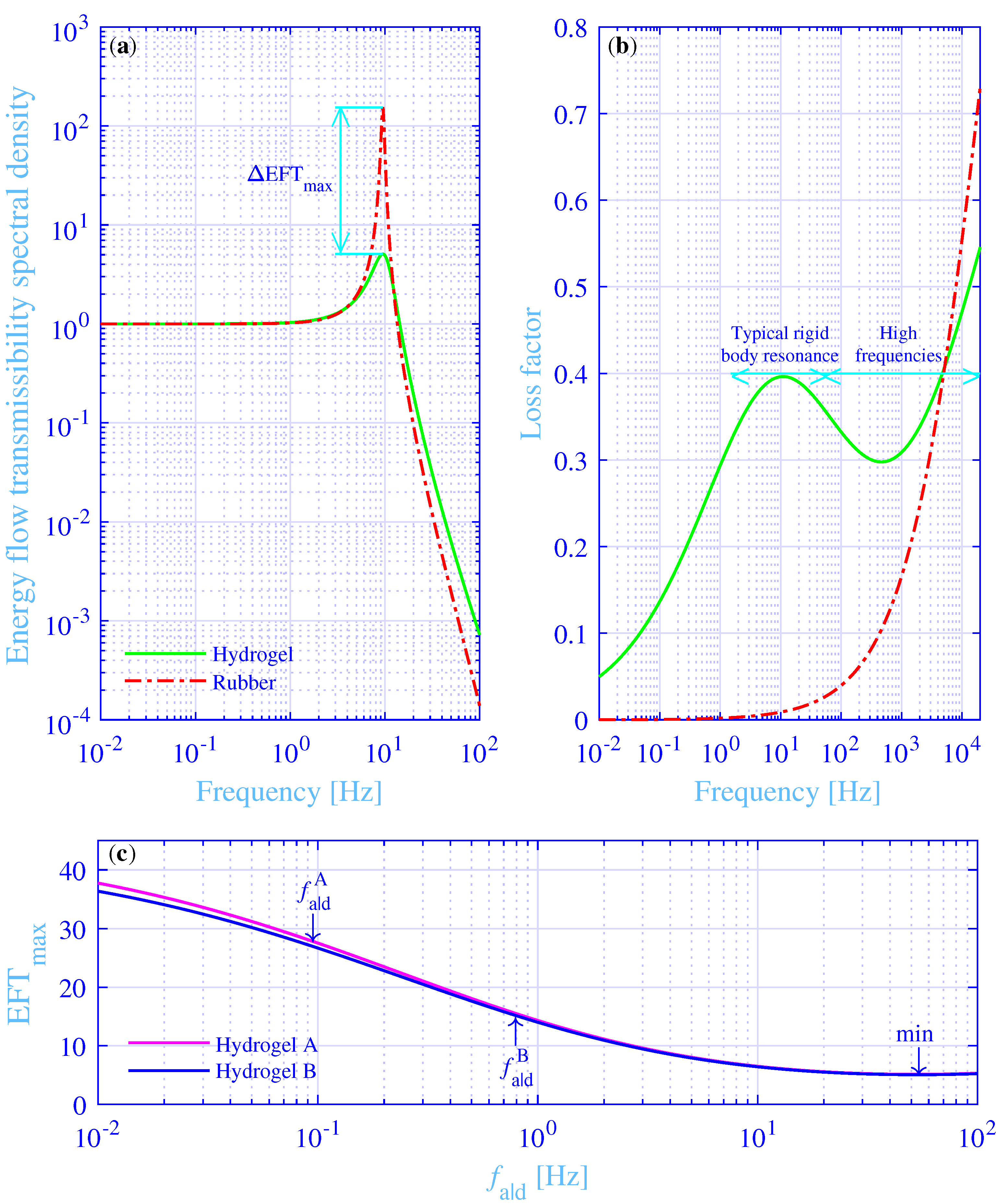
3.6. Minimum Analysis
- [N/mN/m]
- [NmNm]
- [N/mN/m]
- [N/mN/m]
- []
3.7. Comparison with a Natural Rubber Vibration Isolation System
4. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Snowdon, J.C. Vibration and Shock in Damped Mechanical Systems; John Wiley and Sons Ltd.: New York, NY, USA, 1968. [Google Scholar]
- Mead, D.J. Passive Vibration Control; John Wiley and Sons Ltd.: Chichester, UK, 1998. [Google Scholar]
- Ungar, E.E. Use of Vibration Isolators. In Handbook of Noise and Vibration Control; Crocker, M.J., Ed.; Wiley InterScience, John Wiley and Sons Ltd.: Hooboken, NJ, USA, 2007; Chapter 61; pp. 725–733. [Google Scholar] [CrossRef]
- Yang, C.H.; Wang, M.X.; Haider, H.; Yang, J.H.; Sun, J.Y.; Chen, Y.M.; Zhou, J.; Suo, Z. Strengthening alginate/polyacrylamide hydrogels using various multivalent cations. ACS Appl. Mater. Interfaces 2013, 5, 10418–10422. [Google Scholar] [CrossRef] [PubMed]
- Kari, L. Torsional energy flow trough a tough hydrogel vibration isolator. In Proceedings of the MEDYNA2020, 3rd Euro-Mediterranean Conference on Structural Dynamics and Vibroacoustics, Napoli, Italy, 17–19 February 2020; pp. 237–240. [Google Scholar]
- Feng, Q.; Fan, L.; Huo, L.; Song, G. Vibration reduction of an existing glass window through a viscoelastic material-based retrofit. Appl. Sci. 2018, 8, 1061. [Google Scholar] [CrossRef]
- Zhang, X.; Yang, D. Mechanical properties of auxetic cellular material consisting of re-entrant hexagonal honeycombs. Materials 2016, 9, 900. [Google Scholar] [CrossRef] [PubMed]
- Kuznetsova, T.A.; Zubar, T.I.; Lapitskaya, V.A.; Sudzilouskaya, K.A.; Chizhik, S.A.; Didenko, A.L.; Svetlichnyi, V.M.; Vylegzhanina, M.E.; Kudryavtsev, V.V.; Sukhanova, T.E. Tribological properties investigation of the thermoplastic elastomers surface with the AFM lateral forces mode. IOP Conf. Ser. Mater. Sci. Eng. 2017, 256, 012022. [Google Scholar] [CrossRef]
- Lin, W.C.; Fan, W.; Marcellan, A.; Hourdet, D.; Creton, C. Large strain and fracture properties of poly(dimethylacrylamide)/silica hybrid hydrogels. Macromolecules 2010, 43, 2554–2563. [Google Scholar] [CrossRef]
- Carlsson, L.; Rose, S.; Hourdet, D.; Marcellan, A. Nano-hybrid self-crosslinked PDMA/silica hydrogels. Soft Matter 2010, 6, 3619–3631. [Google Scholar] [CrossRef]
- Peak, C.W.; Wilker, J.J.; Schmidt, G. A review on tough and sticky hydrogels. Colloid Polym. Sci. 2013, 291, 2031–2047. [Google Scholar] [CrossRef]
- Mayumi, K.; Marcellan, A.; Ducouret, G.; Creton, C.; Narita, T. Stress–strain relationship of highly stretchable dual cross-link gels: Separability of strain and time effect. ACS Macro Lett. 2013, 2, 1065–1068. [Google Scholar] [CrossRef]
- Hao, J.; Weiss, R.A. Mechanical behavior of hybrid hydrogels composed of a physical and a chemical network. Polymer 2013, 54, 2174–2182. [Google Scholar] [CrossRef]
- Rose, S.; Dizeux, A.; Narita, T.; Hourdet, D.; Marcellan, A. Time dependence of dissipative and recovery processes in nanohybrid hydrogels. Macromolecules 2013, 46, 4095–4104. [Google Scholar] [CrossRef]
- Narita, T.; Mayumi, K.; Ducouret, G.; Hébraud, P. Viscoelastic properties of poly(vinyl alcohol) hydrogels having permanent and transient cross-links studied by microrheology, classical rheometry, and dynamic light scattering. Macromolecules 2013, 46, 4174–4183. [Google Scholar] [CrossRef]
- Long, R.; Mayumi, K.; Creton, C.; Narita, T.; Hui, C.Y. Time dependent behavior of a dual cross-link self-healing gel: Theory and experiments. Macromolecules 2014, 47, 7243–7250. [Google Scholar] [CrossRef]
- Zhao, X. Multi-scale multi-mechanism design of tough hydrogels: Building dissipation into stretchy networks. Soft Matter 2014, 10, 672–687. [Google Scholar] [CrossRef]
- Long, R.; Mayumi, K.; Creton, C.; Narita, T.; Hui, C.Y. Rheology of a dual crosslink self-healing gel: Theory and measurement using parallel-plate torsional rheometry. J. Rheol. 2015, 59, 643–665. [Google Scholar] [CrossRef]
- Branca, C.; Crupi, C.; D’Angelo, G.; Khouzami, K.; Rifici, S.; Visco, A.; Wanderlingh, U. Effect of montmorillonite on the rheological properties of dually crosslinked guar gum-based hydrogels. J. Appl. Polym. Sci. 2015, 132, 41373. [Google Scholar] [CrossRef]
- Lin, P.; Ma, S.; Wang, X.; Zhou, F. Molecularly engineered dual-crosslinked hydrogel with ultrahigh mechanical strength, toughness, and good self-recovery. Adv. Mater. 2015, 27, 2054–2059. [Google Scholar] [CrossRef]
- Zhang, H.; Peng, H.; Li, Y.; Xu, Y.; Weng, W. Compositional- and time-dependent dissipation, recovery and fracture toughness in hydrophobically reinforced hybrid hydrogels. Polymer 2015, 80, 130–137. [Google Scholar] [CrossRef]
- Haraguchi, K.; Li, H.J.; Xu, Y.; Li, G. Copolymer nanocomposite hydrogels: Unique tensile mechanical properties and network structures. Polymer 2016, 96, 94–103. [Google Scholar] [CrossRef]
- Karobi, S.N.; Sun, T.L.; Kurokawa, T.; Luo, F.; Nakajima, T.; Nonoyama, T.; Gong, J.P. Creep behavior and delayed fracture of tough polyampholyte hydrogels by tensile test. Macromolecules 2016, 49, 5630–5636. [Google Scholar] [CrossRef]
- Czarnecki, S.; Rossow, T.; Seiffert, S. Hybrid polymer-network hydrogels with tunable mechanical response. Polymers 2016, 8, 82. [Google Scholar] [CrossRef] [PubMed]
- Mayumi, K.; Guo, J.; Narita, T.; Hui, C.Y.; Creton, C. Fracture of dual crosslink gels with permanent and transient crosslinks. Extrem. Mech. Lett. 2016, 6, 52–59. [Google Scholar] [CrossRef]
- Zhong, M.; Liu, Y.T.; Liu, X.Y.; Shi, F.K.; Zhang, L.Q.; Zhu, M.F.; Xie, X.M. Dually cross-linked single network poly(acrylic acid) hydrogels with superior mechanical properties and water absorbency. Soft Matter 2016, 12, 5420–5428. [Google Scholar] [CrossRef] [PubMed]
- Creton, C. 50th Anniversary perspective: Networks and gels: Soft but dynamic and tough. Macromolecules 2017, 50, 8297–8316. [Google Scholar] [CrossRef]
- Guo, J.; Long, R.; Mayumi, K.; Hui, C.Y. Mechanics of a dual cross-link gel with dynamic bonds: Steady state kinetics and large deformation effects. Macromolecules 2016, 49, 3497–3507. [Google Scholar] [CrossRef]
- Zhao, J.; Mayumi, K.; Creton, C.; Narita, T. Rheological properties of tough hydrogels based on an associating polymer with permanent and transient crosslinks: Effects of crosslinking density. J. Rheol. 2017, 61, 1371–1383. [Google Scholar] [CrossRef]
- Zou, X.; Kui, X.; Zhang, R.; Zhang, Y.; Wang, X.; Wu, Q.; Chen, T.; Sun, P. Viscoelasticity and structures in chemically and physically dual-cross-linked hydrogels: Insights from rheology and proton multiple-quantum NMR spectroscopy. Macromolecules 2017, 50, 9340–9352. [Google Scholar] [CrossRef]
- Liu, M.; Guo, J.; Hui, C.Y.; Creton, C.; Narita, T.; Zehnder, A. Time–temperature equivalence in a PVA dual cross-link self-healing hydrogel. J. Rheol. 2018, 62, 991–1000. [Google Scholar] [CrossRef]
- Nicol, E.; Nicolai, T.; Zhao, J.; Narita, T. Photo-cross-linked self-assembled poly(ethylene oxide)-based hydrogels containing hybrid junctions with dynamic and permanent cross-links. ACS Macro Lett. 2018, 7, 683–687. [Google Scholar] [CrossRef]
- Kari, L. Effective visco-elastic models of tough, doubly cross-linked, single-network polyvinyl alcohol (PVA) hydrogels. Additively separable fractional-derivative based models for chemical and physical cross-links. Contin. Mech. Thermodyn. 2020, in press. [Google Scholar] [CrossRef]
- Kari, L. Are single polymer network hydrogels with chemical and physical cross-links a promising dynamic vibration absorber material? A aimulation model inquiry. Materials 2020, 13, 5127. [Google Scholar] [CrossRef] [PubMed]
- Wang, W.; Zhang, Y.; Liu, W. Bioinspired fabrication of high strength hydrogels from non-covalent interactions. Prog. Polym. Sci. 2017, 71, 1–25. [Google Scholar] [CrossRef]
- Zhou, X.; Guo, B.; Zhang, L.; Hu, G.H. Progress in bio-inspired sacrificial bonds in artificial polymeric materials. Chem. Soc. Rev. 2017, 46, 6301–6329. [Google Scholar] [CrossRef]
- Ahmed, E.M. Hydrogel: Preparation, characterization, and applications: A review. J. Adv. Res. 2015, 6, 105–121. [Google Scholar] [CrossRef] [PubMed]
- Luo, F.; Sun, T.L.; Nakajima, T.; Kurokawa, T.; Zhao, Y.; Ihsan, A.B.; Guo, H.L.; Li, X.F.; Gong, J.P. Crack blunting and advancing behaviors of tough and self-healing polyampholyte hydrogel. Macromolecules 2014, 47, 6037–6046. [Google Scholar] [CrossRef]
- Rouse, P.E., Jr. A theory of the linear viscoelastic properties of dilute solutions of coiling polymers. J. Chem. Phys. 1953, 2, 1272–1280. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus—A different approach to the analysis of viscoelastically damped structures. AIAA J. 1983, 21, 741–748. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. A theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 1983, 27, 201–210. [Google Scholar] [CrossRef]
- Koeller, R.C. Applications of fractional calculus to the theory of viscoelasticity. J. Appl. Mech. 1984, 51, 299–307. [Google Scholar] [CrossRef]
- Torvik, P.J.; Bagley, R.L. On the appearance of the fractional derivative in the behavior of real materials. J. Appl. Mech. 1984, 51, 294–298. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. On the fractional calculus model of viscoelastic behavior. J. Rheol. 1986, 30, 133–155. [Google Scholar] [CrossRef]
- Pritz, T. Analysis of four-parameter fractional derivative model of real solid materials. J. Sound Vib. 1996, 195, 103–115. [Google Scholar] [CrossRef]
- Enelund, M.; Olssson, P. Damping described by fading memory—analysis andapplication to fractional derivative models. Int. J. Solids Struct. 1999, 36, 939–970. [Google Scholar] [CrossRef]
- Pritz, T. Verification of local Kramers–Kronig relations for complex modulus by means of fractional derivative model. J. Sound Vib. 1999, 228, 1145–1165. [Google Scholar] [CrossRef]
- Kari, L. On the waveguide modelling of dynamic stiffness of cylindrical vibration isolators. Part I: The model, solution and experimental comparison. J. Sound Vib. 2001, 244, 211–233. [Google Scholar] [CrossRef]
- Kari, L.; Eriksson, P.; Stenberg, B. Dynamic stiffness of natural rubber cylinders in the audible frequency range using wave guides. Kaut. Gummi Kunstst. 2001, 54, 106–111. [Google Scholar]
- Surguladze, T.A. On certain applications of fractional calculus to viscoelasticity. J. Math. Sci. 2002, 112, 4517–4557. [Google Scholar] [CrossRef]
- Sjöberg, M.; Kari, L. Non-linear behavior of a rubber isolator system using fractional derivatives. Veh. Syst. Dyn. 2002, 37, 217–236. [Google Scholar] [CrossRef]
- Kari, L. Dynamic stiffness matrix of a long rubber bush mounting. Rubber Chem. Technol. 2002, 75, 747–770. [Google Scholar] [CrossRef]
- Adolfsson, K.; Enelund, M. Fractional derivative viscoelasticity at large deformations. Nonlinear Dyn. 2003, 33, 301–321. [Google Scholar] [CrossRef]
- Sjöberg, M.; Kari, L. Nonlinear isolator dynamics at finite deformations: An effective hyperelastic, fractional derivative, generalized friction model. Nonlinear Dyn. 2003, 33, 323–336. [Google Scholar] [CrossRef]
- Kari, L. Audible-frequency stiffness of a primary suspension isolator on a high speed tilting bogie. Proc. Inst. Mech. Eng. F J. Rail Rapid Transit 2003, 217, 47–62. [Google Scholar] [CrossRef]
- Kari, L. On the dynamic stiffness of preloaded vibration isolators in the audible frequency range: Modeling and experiments. J. Acoust. Soc. Am. 2003, 113, 1909–1921. [Google Scholar] [CrossRef]
- Pritz, T. Five-parameter fractional derivative model for polymeric damping materials. J. Sound Vib. 2003, 265, 935–952. [Google Scholar] [CrossRef]
- Adolfsson, K.; Enelund, M.; Olsson, P. On the fractional order model of viscoelasticity. Mech. Time-Depend. Mater. 2005, 9, 15–34. [Google Scholar] [CrossRef]
- Coja, M.; Kari, L. Axial audio-frequency stiffness of a bush mounting—The waveguide solution. Appl. Math. Modell. 2007, 31, 38–53. [Google Scholar] [CrossRef]
- García Tárrago, M.J.; Kari, L.; Vinolas, J.; Gil-Negrete, N. Frequency and amplitude dependence of the axial and radial stiffness of carbon-black filled rubber bushings. Polym. Test. 2007, 26, 629–638. [Google Scholar] [CrossRef]
- Hanyga, A. Fractional-order relaxation laws in non-linear viscoelasticity. Contin. Mech. Thermodyn. 2007, 19, 25–36. [Google Scholar] [CrossRef]
- García Tárrago, M.J.; Vinolas, J.; Kari, L. Axial stiffness of carbon black filled rubber bushings. Kaut. Gummi Kunsts. 2007, 60, 43–48. [Google Scholar]
- García Tárrago, M.J.; Kari, L.; Viñolas, J.; Gil-Negrete, N. Torsion stiffness of a rubber bushing: A simple engineering design formula including the amplitude dependence. J. Strain Anal. Eng. 2007, 42, 13–21. [Google Scholar] [CrossRef]
- Gil-Negrete, N.; Vinolas, J.; Kari, L. A nonlinear rubber material model combining fractional order viscoelasticity and amplitude dependent effects. J. Appl. Mech. 2009, 76, 011009. [Google Scholar] [CrossRef]
- Lewandowski, R.; Pawlak, Z. Dynamic analysis of frames with viscoelastic dampers modelled by rheological models with fractional derivatives. J. Sound Vib. 2011, 330, 923–936. [Google Scholar] [CrossRef]
- Blom, P.; Kari, L. A nonlinear constitutive audio frequency magneto-sensitive rubber model including amplitude, frequency and magnetic field dependence. J. Sound Vib. 2011, 330, 947–954. [Google Scholar] [CrossRef]
- Östberg, M.; Kari, L. Transverse, tilting and cross-coupling stiffness of cylindrical rubber isolators in the audible frequency range—The wave-guide solution. J. Sound Vib. 2011, 330, 3222–3244. [Google Scholar] [CrossRef]
- Müller, S.; Kästner, M.; Brummund, J.; Ulbricht, V. A nonlinear fractional viscoelastic material model for polymers. Comput. Mater. Sci. 2011, 50, 2938–2949. [Google Scholar] [CrossRef]
- Blom, P.; Kari, L. The frequency, amplitude and magnetic field dependent torsional stiffness of a magneto-sensitive rubber bushing. Int. J. Mech. Sci. 2012, 60, 54–58. [Google Scholar] [CrossRef]
- Wharmby, A.W.; Bagley, R.L. Generalization of a theoretical basis for the application of fractional calculus to viscoelasticity. J. Rheol. 2013, 57, 1429–1440. [Google Scholar] [CrossRef]
- Alberdi-Muniain, A.; Gil-Negrete, N.; Kari, L. Modelling energy flow through magneto-sensitive vibration isolators. Int. J. Eng. Sci. 2013, 65, 22–39. [Google Scholar] [CrossRef]
- Müller, S.; Kästner, M.; Brummund, J.; Ulbricht, V. On the numerical handling of fractional viscoelastic material models in a FE analysis. Comput. Mech. 2013, 51, 999–1012. [Google Scholar] [CrossRef]
- Östberg, M.; Coja, M.; Kari, L. Dynamic stiffness of hollowed cylindrical rubber vibration isolators—the wave-guide solution. Int. J. Solids Struct. 2013, 50, 1791–1811. [Google Scholar] [CrossRef]
- Wollscheid, D.; Lion, A. The benefit of fractional derivatives in modelling the dynamics of filler-reinforced rubber under large strains: A comparison with the Maxwell-element approach. Comput. Mech. 2014, 53, 1015–1031. [Google Scholar] [CrossRef]
- Rouleau, L.; Pirk, R.; Pluymers, B.; Desmet, W. Characterization and modeling of the viscoelastic behavior of a self-adhesive rubber using dynamic mechanical analysis tests. J. Aerosp. Technol. Manag. 2015, 7, 200–208. [Google Scholar] [CrossRef]
- Pirk, R.; Rouleau, L.; Desmet, W.; Pluymers, B. Validating the modeling of sandwich structures with constrained layer damping using fractional derivative models. J. Braz. Soc. Mech. Sci. Eng. 2016, 38, 1959–1972. [Google Scholar] [CrossRef]
- Fredette, L.; Singh, R. Estimation of the transient response of a tuned, fractionally damped elastomeric isolator. J. Sound Vib. 2016, 382, 1–12. [Google Scholar] [CrossRef]
- Guo, X.; Yan, G.; Benyahia, L.; Sahraoui, S. Fitting stress relaxation experiments with fractional Zener model to predict high frequency moduli of polymeric acoustic foams. Mech. Time-Depend. Mater. 2016, 20, 523–533. [Google Scholar] [CrossRef]
- Rouleau, L.; Deü, J.F.; Legay, A. A comparison of model reduction techniques based on modal projection for structures with frequency-dependent damping. Mech. Syst. Sig. Process. 2017, 90, 110–125. [Google Scholar] [CrossRef]
- Fredette, L.; Singh, R. High frequency, multi-axis dynamic stiffness analysis of a fractionally damped elastomeric isolator using continuous system theory. J. Sound Vib. 2017, 389, 468–483. [Google Scholar] [CrossRef]
- Kim, S.; Singh, R. A comparison between fractional-order and integer-order differential finite deformation viscoelastic models: Effects of filler content and loading rate on material parameters. Int. J. Appl. Mech. 2018, 10, 1850099. [Google Scholar] [CrossRef]
- Rouleau, L.; Legay, A.; Deü, J.F. Interface finite elements for the modelling of constrained viscoelastic layers. Compos. Struct. 2018, 204, 847–854. [Google Scholar] [CrossRef]
- Wang, B.; Kari, L. A nonlinear constitutive model by spring, fractional derivative and modified bounding surface model to represent the amplitude, frequency and the magnetic dependency for magneto-sensitive rubber. J. Sound Vib. 2019, 438, 344–352. [Google Scholar] [CrossRef]
- Sahraoui, S.; Zekri, N. On fractional modeling of viscoelastic foams. Mech. Res. Commun. 2019, 96, 62–66. [Google Scholar] [CrossRef]
- Wang, B.; Kari, L. Modeling and vibration control of a smart vibration isolation system based on magneto-sensitive rubber. Smart Mater. Struct. 2019, 28, 065026. [Google Scholar] [CrossRef]
- Freundlich, J. Transient vibrations of a fractional Kelvin–Voigt viscoelastic cantilever beam with a tip mass and subjected to a base excitation. J. Sound Vib. 2019, 438, 99–115. [Google Scholar] [CrossRef]
- Wang, B.; Kari, L. One dimensional constitutive model of isotropic magneto-sensitive rubber under shear deformation with amplitude, frequency and magnetic dependency. IOP Conf. Ser. Mater. Sci. Eng. 2020, 855, 012002. [Google Scholar] [CrossRef]
- Wang, B.; Kari, L. A visco-elastic-plastic constitutive model of isotropic magneto-sensitive rubber with amplitude, frequency and magnetic dependency. Int. J. Plast. 2020, 132, 102756. [Google Scholar] [CrossRef]
- Henriques, I.R.; Rouleau, L.; Castello, D.A.; Borges, L.A.; Deü, J.F. Viscoelastic behavior of polymeric foams: Experiments and modeling. Mech. Mater. 2020, 148, 103506. [Google Scholar] [CrossRef]
- Wang, B.; Kari, L. Constitutive model of isotropic magneto-sensitive rubber with amplitude, frequency, magnetic and temperature dependence under a continuum mechanics basis. Polymers 2021, 13, 472. [Google Scholar] [CrossRef] [PubMed]
- Kari, L. Dynamic stiffness of chemically and physically ageing rubber vibration isolators in the audible frequency range. Part 1: Constitutive equations. Contin. Mech. Thermodyn. 2017, 29, 1027–1046. [Google Scholar] [CrossRef]
- Kari, L. Dynamic stiffness of chemically and physically ageing rubber vibration isolators in the audible frequency range. Part 2: Waveguide solution. Contin. Mech. Thermodyn. 2017, 29, 1047–1059. [Google Scholar] [CrossRef]
- Machado, J.T.; Kiryakova, V.; Mainardi, F. Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef]
- Morman, K.N.; Pan, T.Y. Application of finite-elementanalysis in the design of automotive elastomeric components. Rubber Chem. Technol. 1988, 61, 503–533. [Google Scholar] [CrossRef]
- Chen, J.S.; Wu, C.T. On computational issues in large deformation analysis of rubber bushings. J. Struct. Mech. 1997, 25, 287–309. [Google Scholar] [CrossRef]
- Busfield, J.J.C.; Davies, C.K.L. Stiffness of simple bonded elastomer bushes. Part 1—Initial behaviour. Plast. Rubber Compos. 2001, 30, 243–257. [Google Scholar] [CrossRef]
- Kadlowec, J.; Wineman, A.; Hulbert, G. Elastomer bushing response: Experiments and finite element modeling. Acta Mech. 2003, 163, 25–38. [Google Scholar] [CrossRef]
- Kadlowec, J.; Gerrard, D.; Pearlman, H. Coupled axial–torsional behavior of cylindrical elastomer bushings. Polym. Test. 2009, 28, 139–144. [Google Scholar] [CrossRef]
- Adkins, J.E.; Gent, A.N. Load-deflexion relations of rubber bush mountings. Br. J. Appl. Phys. 1954, 5, 354–358. [Google Scholar] [CrossRef]
- Hill, J.M. Radical deflections of rubber bush mountings of finite lengths. Int. J. Eng. Sci. 1975, 13, 407–422. [Google Scholar] [CrossRef]
- Hill, J.M. The effect of precompression on the load–deflection relations of long rubber bush mountings. J. Appl. Polym. Sci. 1975, 19, 747–755. [Google Scholar] [CrossRef]
- Rivas-Torres, J.; Tudon-Martinez, J.C.; Lozoya-Santos, J.J.; Ramirez-Mendoza, R.A.; Spaggiari, A. Analytical design and optimization of an automotive rubber bushing. Shock Vib. 2019, 2019, 1873958. [Google Scholar] [CrossRef]
- Gil-Negrete, N.; Viñolas, J.; Kari, L. A simplified methodology to predict the dynamic stiffness of carbon-black filled rubber isolators using a finite element code. J. Sound Vib. 2006, 296, 757–776. [Google Scholar] [CrossRef]
- Östberg, M.; Jerrelind, J.; Kari, L. A study of the influence of rubber bushings on the audible frequency behaviour of a truck damper. Int. J. Heavy Veh. Syst. 2014, 21, 281–294. [Google Scholar] [CrossRef]
- Lee, H.S.; Shin, J.K.; Msolli, S.; Kim, H.S. Prediction of the dynamic equivalent stiffness for a rubber bushing using the finite element method and empirical modeling. Int. J. Mech. Mater. Des. 2019, 15, 77–91. [Google Scholar] [CrossRef]
- Lyon, R.H.; Maidanik, G. Power flow between linearly coupled oscillators. J. Acoust. Soc. Am. 1962, 34, 623–639. [Google Scholar] [CrossRef]
- Goyder, H.G.D.; White, R.G. Vibrational power flow from machines into built-up structures, part I: Introduction and approximate analyses of beam and plate-like foundations. J. Sound Vib. 1980, 68, 59–75. [Google Scholar] [CrossRef]
- Goyder, H.G.D.; White, R.G. Vibrational power flow from machines into built-up structures, part II: Wave propagation and power flow in beam-stiffened plates. J. Sound Vib. 1980, 68, 77–96. [Google Scholar] [CrossRef]
- Goyder, H.G.D.; White, R.G. Vibrational power flow from machines into built-up structures, part III: Power flow through isolation systems. J. Sound Vib. 1980, 68, 97–117. [Google Scholar] [CrossRef]
- Pinnington, R.J.; White, R.G. Power flow through machine isolators to resonant and non-resonant beams. J. Sound Vib. 1981, 75, 179–197. [Google Scholar] [CrossRef]
- Pinnington, R.J. Vibrational power transmission to a seating of a vibration isolated motor. J. Sound Vib. 1987, 118, 515–530. [Google Scholar] [CrossRef]
- Pinnington, R.J.; Pearce, D.C.R. Multipole expansion of the vibration transmission between a source and a receiver. J. Sound Vib. 1990, 142, 461–479. [Google Scholar] [CrossRef]
- Li, W.L.; Lavrich, P. Prediction of power flows through machine vibration isolators. J. Sound Vib. 1999, 224, 757–774. [Google Scholar] [CrossRef]
- Li, W.L.; Daniels, M.; Zhou, W. Vibrational power transmission from a machine to its supporting cylindrical shell. J. Sound Vib. 2002, 257, 283–299. [Google Scholar] [CrossRef]
- Singh, R.; Kim, S. Examination of multi-dimensional vibration isolation measures and their correlation to sound radiation over a broad frequency range. J. Sound Vib. 2003, 262, 365–377. [Google Scholar] [CrossRef]
- Falsone, G.; Ferro, G. Best performing parameters of linear and non-linear seismic base-isolator systems obtained by the power flow analysis. Comput. Struct. 2006, 84, 2291–2305. [Google Scholar] [CrossRef]
- Xie, S.; Or, S.W.; Chana, H.L.W.; Choy, P.K.; Liu, P.C.K. Analysis of vibration power flow from a vibrating machinery to a floating elastic panel. Mech. Syst. Sig. Process. 2007, 21, 389–404. [Google Scholar] [CrossRef]
- Blom, P.; Kari, L. Smart audio frequency energy flow control by magneto-sensitive rubber isolators. Smart Mater. Struct. 2008, 17, 015043. [Google Scholar] [CrossRef]
- Alberdi-Muniain, A.; Gil-Negrete, N.; Kari, L. Direct energy flow measurement in magneto-sensitive vibration isolator systems. J. Sound Vib. 2012, 331, 1994–2006. [Google Scholar] [CrossRef]
- Alberdi-Muniain, A.; Gil-Negrete, N.; Kari, L. Indirect energy flow measurement in magneto-sensitive vibration isolator systems. Appl. Acoust. 2013, 74, 575–584. [Google Scholar] [CrossRef]
- Huo, R.; Yu, H.; Guan, Y.F. Dynamic analysis of multi-supported nonlinear vibration isolation system mounted on flexible foundation. Appl. Mech. Mater. 2013, 419, 223–227. [Google Scholar] [CrossRef]
- Yang, J.; Xiong, Y.P.; Xing, J.T. Vibration power flow and force transmission behaviour of a nonlinear isolator mounted on a nonlinear base. Int. J. Mech. Sci. 2016, 115–116, 238–252. [Google Scholar] [CrossRef]
- Sheng, M.; Wang, T.; Wang, M.; Wang, X.; Zhao, X. Effect of distributive mass of spring on power flow in engineering test. J. Sound Vib. 2018, 424, 365–377. [Google Scholar] [CrossRef]
- Kim, Y.S.; Kim, E.Y.; Lee, S.K. Strategy for vibration reduction of a centrifugal turbo blower in a fuel cell electric vehicle based on vibrational power flow analysis. Proc. Inst. Mech. Eng. D J. Automob. Eng. 2010, 224, 985–995. [Google Scholar] [CrossRef]
- Lu, Z.Q.; Shao, D.; Ding, H.; Chen, L.Q. Power flow in a two-stage nonlinear vibration isolation system with high-static-low-dynamic stiffness. Shock Vib. 2018, 2018, 1697639. [Google Scholar] [CrossRef]
- Yang, J.; Jiang, J.Z.; Zhu, X.; Chen, H. Performance of a dual-stage inerter-based vibration isolator. Procedia Eng. 2017, 199, 1822–1827. [Google Scholar] [CrossRef]
- Moorhouse, A.T.; Gibbs, B.M. Measurement of structure-borne sound emission from resiliently mounted machines Situ. J. Sound Vib. 1995, 180, 143–161. [Google Scholar] [CrossRef]
- Moorhouse, A.T.; Gibbs, B.M. Structure-borne sound power emission from resiliently mounted fans: Case studies and diagnosis. J. Sound Vib. 1995, 186, 781–803. [Google Scholar] [CrossRef]
- Rejab, M.N.A.; Rahman, R.A.; Hamzah, R.I.R.; Hussain, J.I.I.; Ahmad, N.; Ismail, A. Measurement of vibration power flow through elastomeric powertrain mounts in passenger car. Appl. Mech. Mater. 2013, 471, 30–34. [Google Scholar] [CrossRef]
- Sun, H.L.; Chen, H.B.; Zhang, K.; Zhang, P.Q. Research on performance indices of vibration isolation system. Appl. Acoust. 2008, 69, 789–795. [Google Scholar] [CrossRef]
- Acri, A. A literature review on energy flow analysis of vibrating structures. Part I: Interface energy flow. ASRO J. Appl. Mech. 2016, 1, 12–15. [Google Scholar]
- Cremer, L.; Heckl, M.; Petersson, B.A.T. Structure-Borne Sound; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar] [CrossRef]
- Bendat, J.S.; Piersol, A.G. Engineering Applications of Correlation and Spectral Analysis; John Wiley and Sons Ltd.: New York, NY, USA, 1993. [Google Scholar]
- MATLAB. 9.9.0.1467703 (R2020b); The MathWorks Inc.: Natick, MA, USA, 2020. [Google Scholar]
- Fornberg, B. Generation of finite difference formulas on arbitrarily spaced grids. Math. Comput. 1988, 51, 699–706. [Google Scholar] [CrossRef]


| Mechanical Source | Vibration Isolators | Foundation | ||||||
|---|---|---|---|---|---|---|---|---|
| Quantity | Value | Quantity | Value | Quantity | Value | |||
| Width | W | 0.200 m | Length | l | 0.100 m | Young’s | ||
| Length | L | 0.500 m | Inner diameter | 0.040 m | modulus | 210 GN/m | ||
| Hight | H | 0.050 m | Outer diameter | 0.050 m | Poisson’s | |||
| Density | 2700 kg/m | Inner sleeve mass | 0.407 kg | ratio: | 0.300 | |||
| Mass | M | 13.50 kg | Outer sleeve mass | 0.111 kg | Thickness | h | 0.010 m | |
| Moment | Density | 1000 kg/m | Density | 780 kg/m | ||||
| of inertia | 0.284 kg m | Static modulus | 2000 N/m | |||||
| Moment | Relaxation intensity | Δ | 5.00 | |||||
| of inertia | 0.478 kg m | Chemical Rouse stress intensity factor | 0.0662 | |||||
| Max physical loss modulus frequency | 50 to 53 Hz | |||||||
| [Hz] | [Hz] | [Hz] | [Hz] | [Hz] | |||
|---|---|---|---|---|---|---|---|
| 52.7 | 5.09 | 9.49 | 25.5 | 9.75 | 11.0 | 7.81 (8.78) | |
| 52.2 | 5.02 | 9.50 | 27.7 | 9.88 | 10.9 | 7.75 (8.70) | |
| 50.9 | 4.83 | 9.55 | 44.5 | 15.1 | 10.6 | 7.57 (8.49) | |
| 52.7 | 5.08 | 9.49 | 29.6 | 9.99 | 11.0 | 7.81 (8.78) | |
| 52.2 | 5.01 | 9.51 | 31.8 | 10.2 | 10.9 | 7.74 (8.70) | |
| 50.9 | 4.83 | 9.55 | 51.0 | 14.7 | 10.6 | 7.57 (8.49) | |
| 52.6 | 5.06 | 9.50 | 50.7 | 12.8 | 11.0 | 7.80 (8.77) | |
| 52.1 | 5.00 | 9.51 | 56.4 | 13.1 | 10.9 | 7.74 (8.69) | |
| 50.9 | 4.82 | 9.56 | 73.7 | 13.9 | 10.6 | 7.56 (8.48) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kari, L. Numerically Exploring the Potential of Abating the Energy Flow Peaks through Tough, Single Network Hydrogel Vibration Isolators with Chemical and Physical Cross-Links. Materials 2021, 14, 886. https://doi.org/10.3390/ma14040886
Kari L. Numerically Exploring the Potential of Abating the Energy Flow Peaks through Tough, Single Network Hydrogel Vibration Isolators with Chemical and Physical Cross-Links. Materials. 2021; 14(4):886. https://doi.org/10.3390/ma14040886
Chicago/Turabian StyleKari, Leif. 2021. "Numerically Exploring the Potential of Abating the Energy Flow Peaks through Tough, Single Network Hydrogel Vibration Isolators with Chemical and Physical Cross-Links" Materials 14, no. 4: 886. https://doi.org/10.3390/ma14040886
APA StyleKari, L. (2021). Numerically Exploring the Potential of Abating the Energy Flow Peaks through Tough, Single Network Hydrogel Vibration Isolators with Chemical and Physical Cross-Links. Materials, 14(4), 886. https://doi.org/10.3390/ma14040886