Numerical Modeling and Evaluation of PEM Used for Fuel Cell Vehicles
Abstract
:1. Introduction
2. Materials and Methods
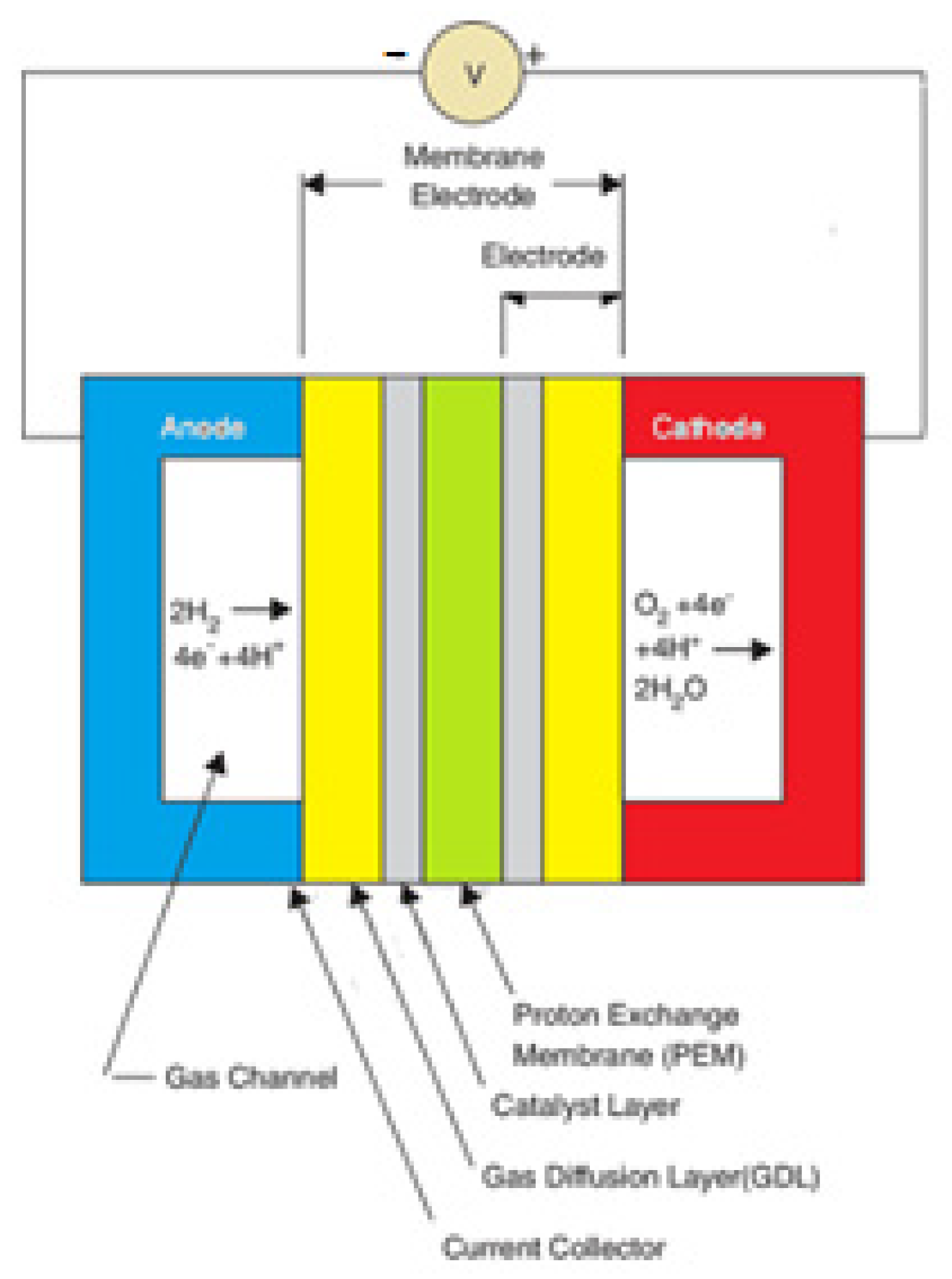
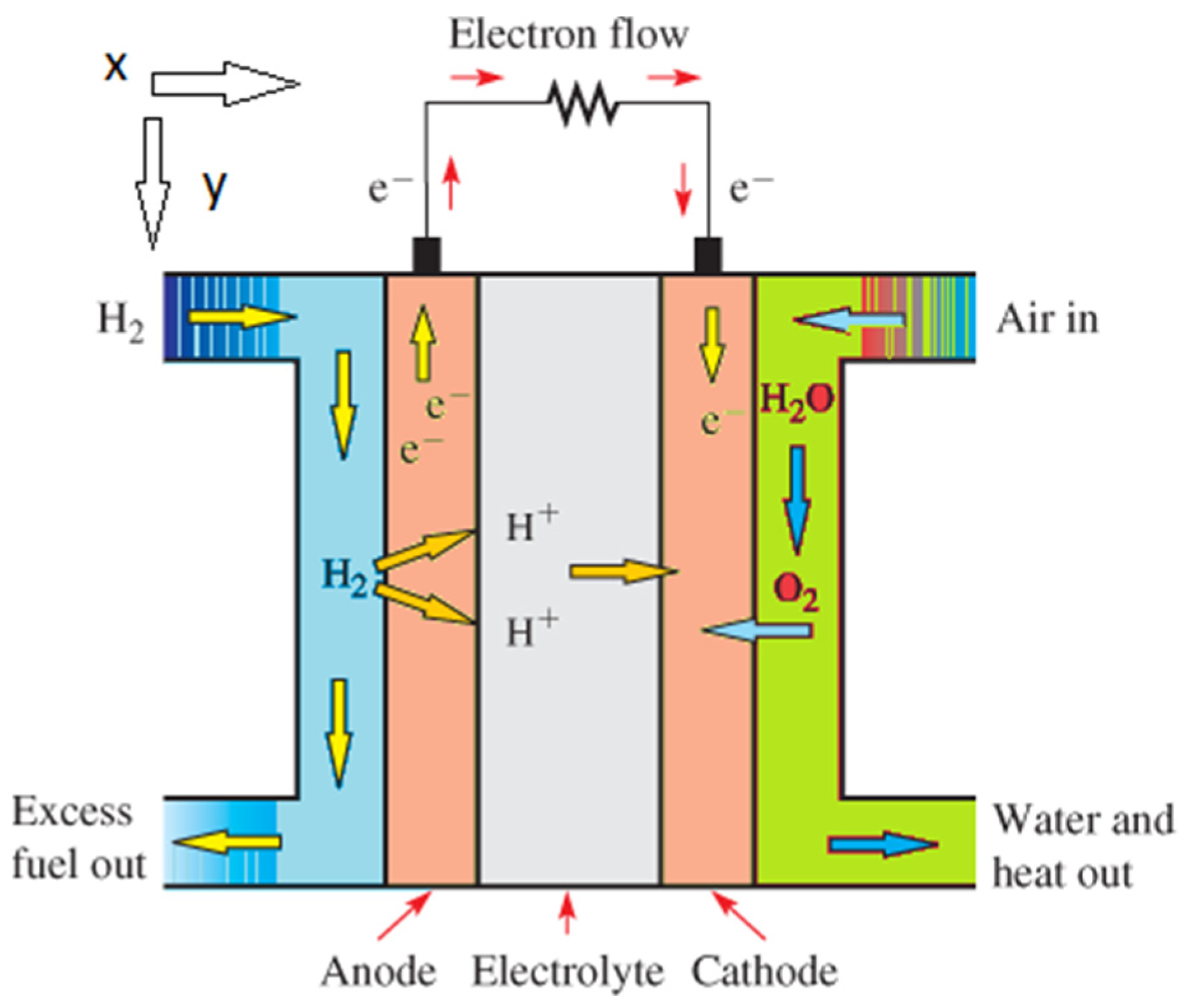
2.1. Polymer Membrane Fuel Cell
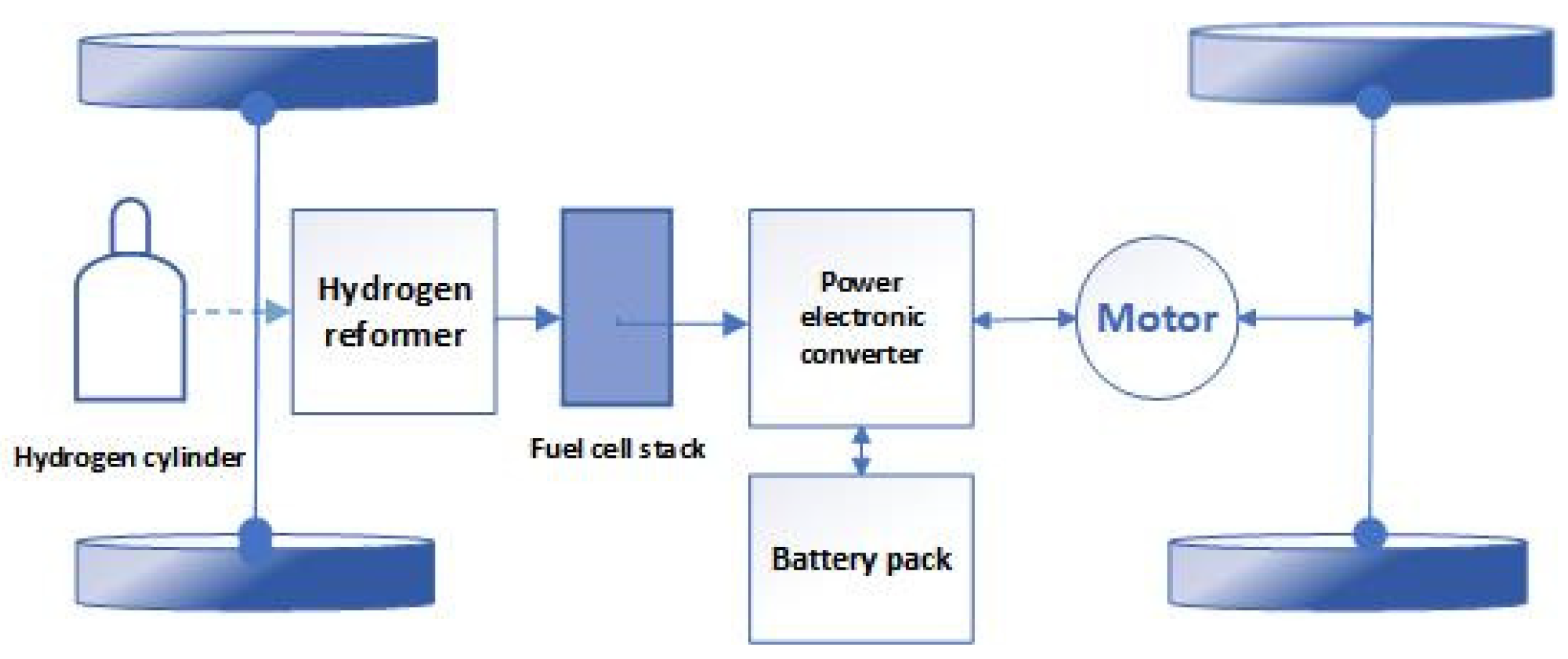
2.2. Fuel Cell Vehicle System
2.3. Mathematical Equations
2.3.1. Equations Governing the Transport of Various Species
2.3.2. Flood State Equations
2.3.3. Equations Governing Cell Electrochemistry
2.3.4. Equations Governing Thermal Energy
2.3.5. Other Equations
2.4. Solution
- Water absorption/desorption at the GDL–membrane interfaces.
- Heat released/absorbed due to phase change of water in the GDL.
- Heat due to half-reaction entropy.
- Heat generated by cathode electrochemical activation.
- Convective heat transfer between gas flow within the channel and on its surface.
- The ideal gas mixture is considered in both anode and cathode gas flow channels.
- Homogeneous porosity and permeability of the GDP and their corresponding effective porosity and permeability parameters are defined.
- The thickness of the catalytic layer and GDL is considered very small in the micrometer range.
- Negligible voltage drop due to the catalytic layers and bipolar plates.
- The flow in the channels is assumed to be laminar. The simulation is performed one-dimensionally, due to the millimeter dimensions of the channels.
- The governing equations are assumed in a steady state.
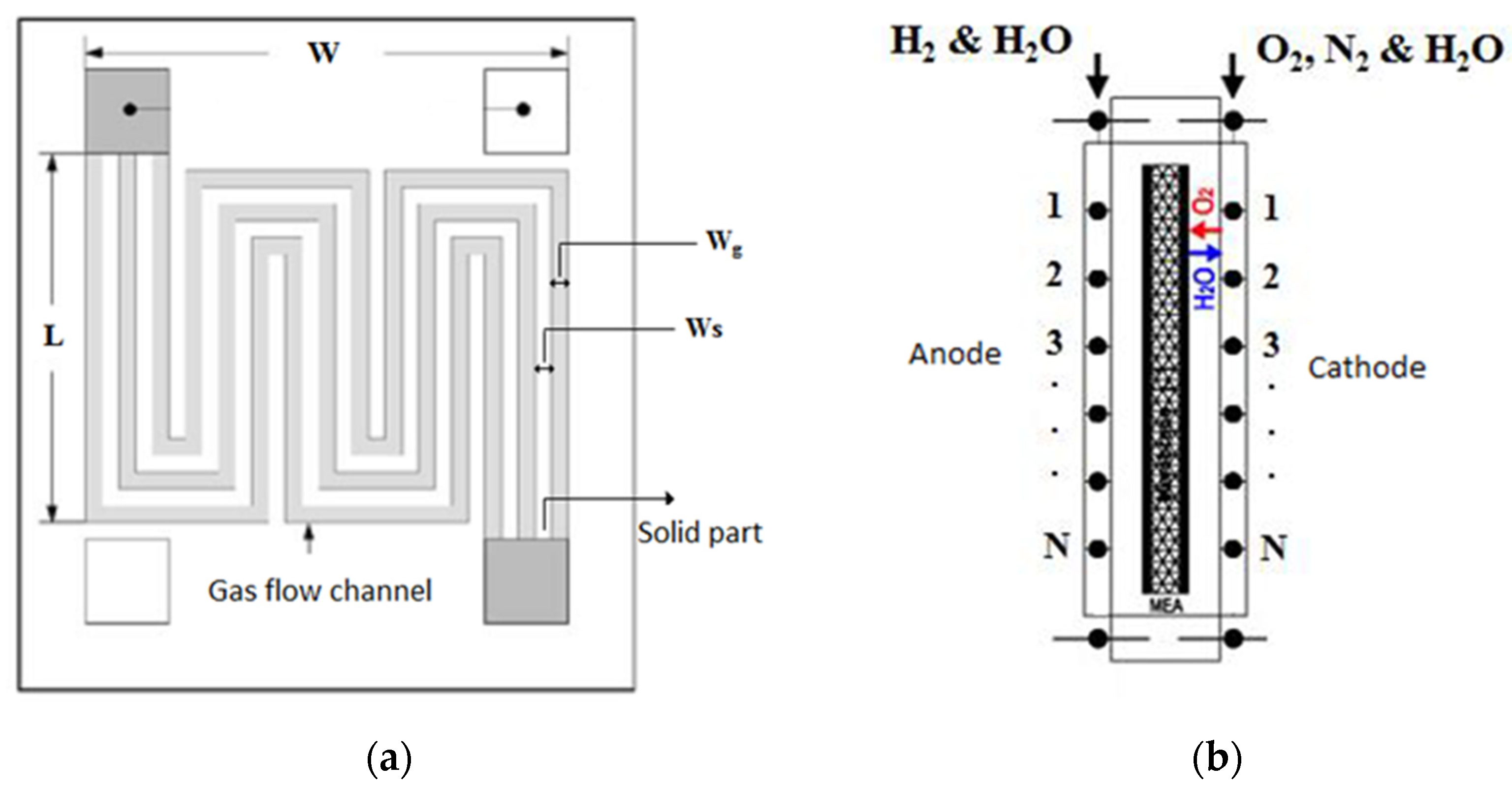
2.5. Parameters Used in This Modeling
2.6. Laboratory Test and Polarization Curve
3. Results and Discussion
3.1. Numerical Pattern Validation
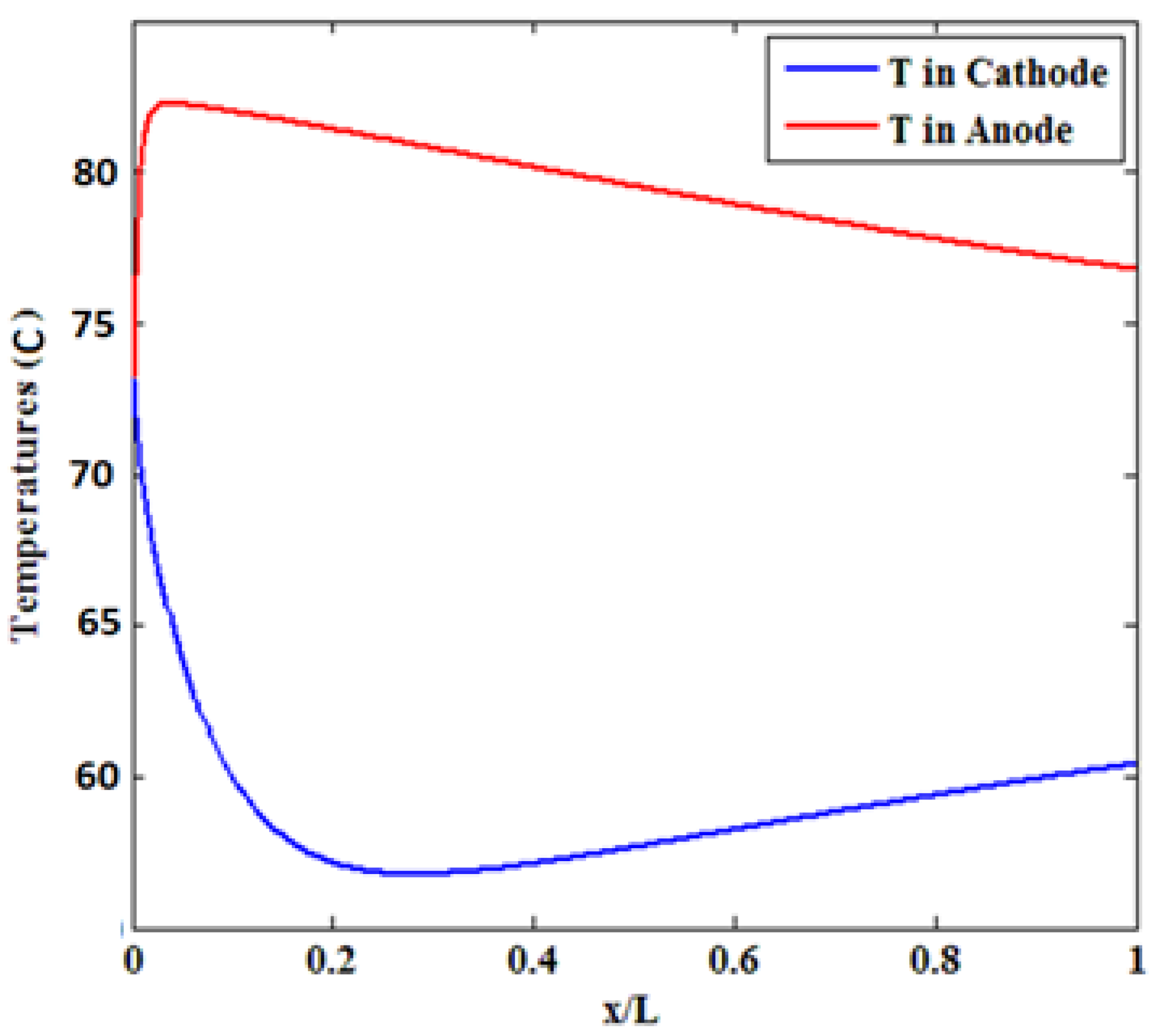
3.2. Effect of Inlet Temperature
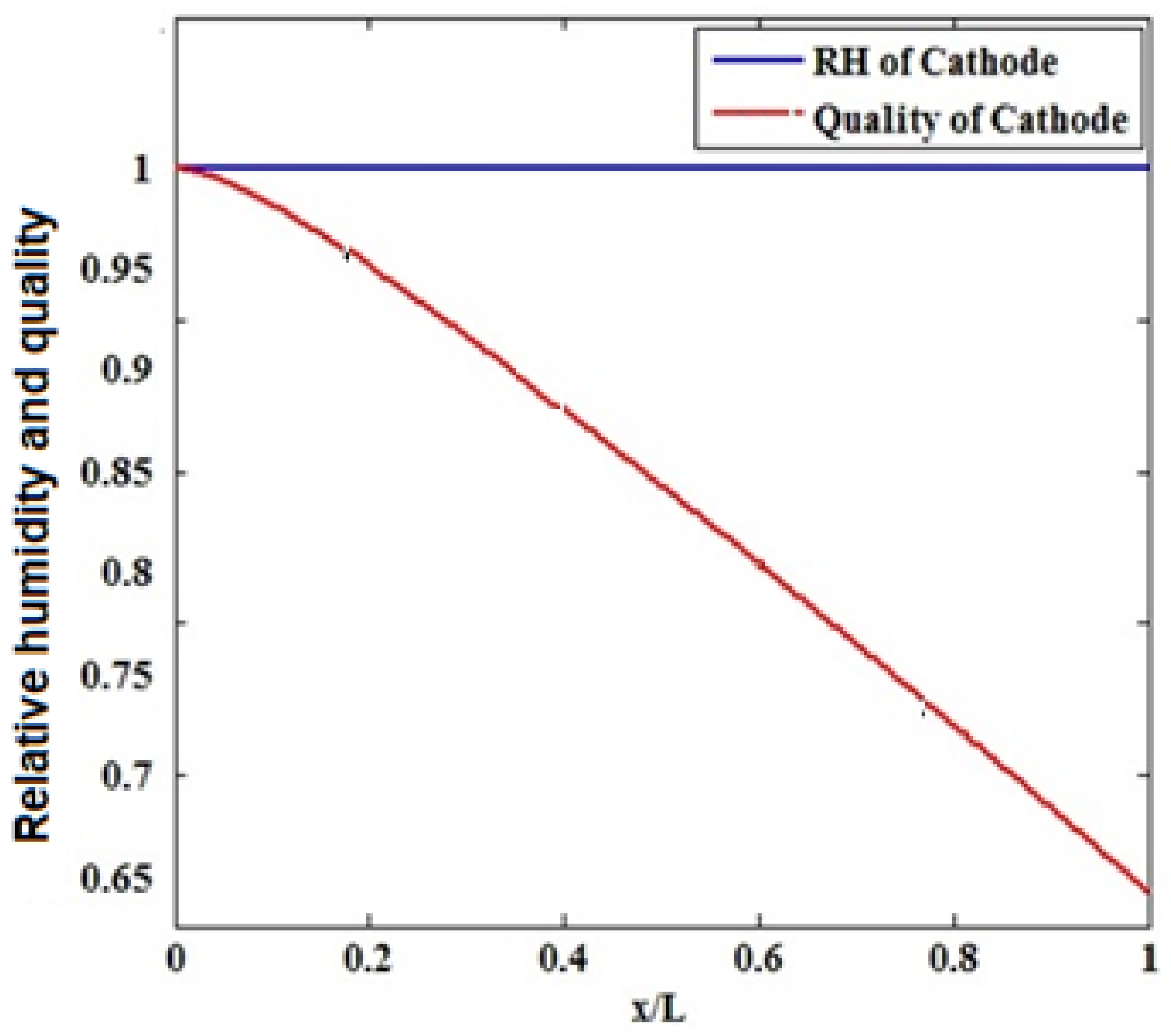
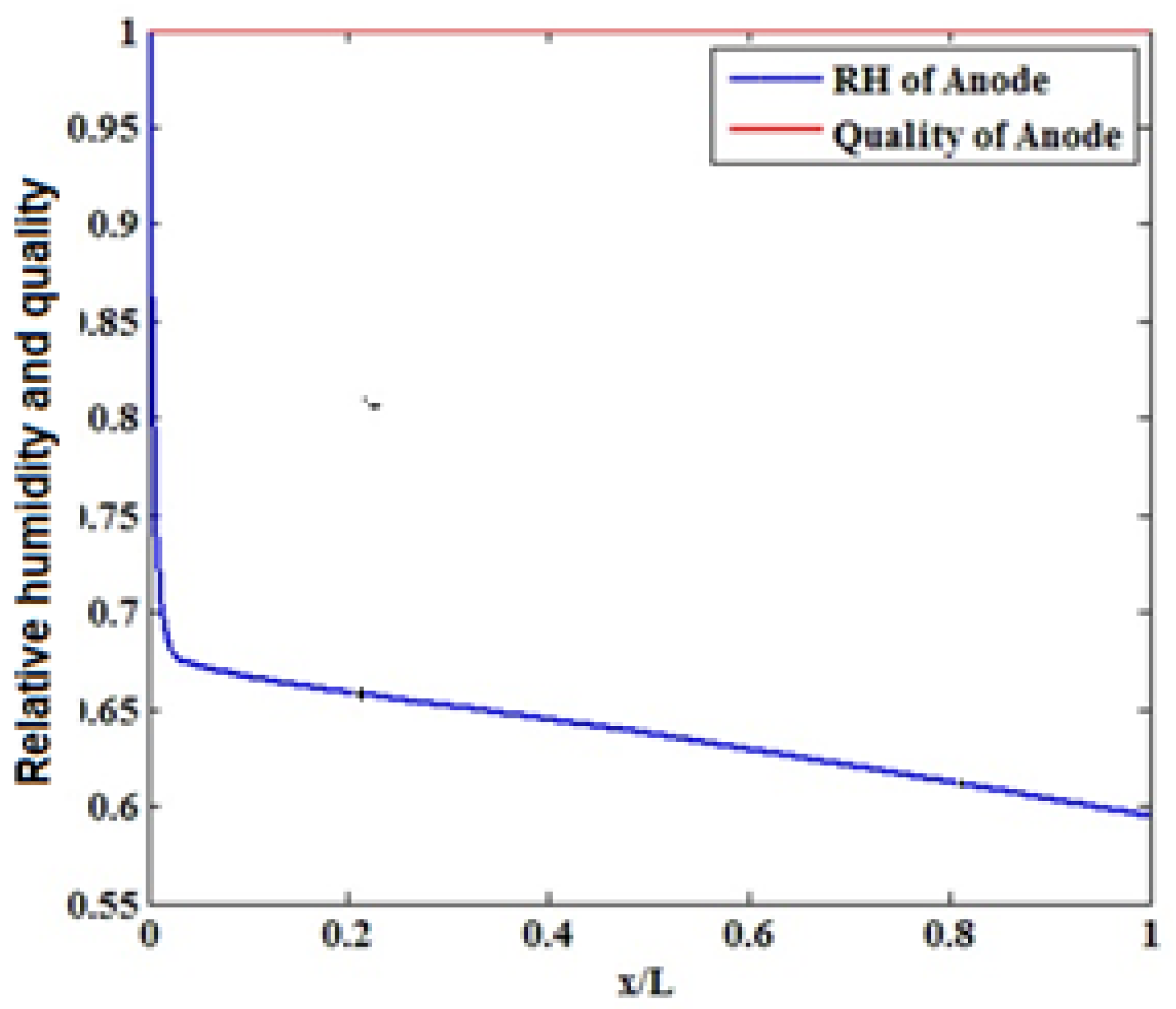
3.3. The Effect of Humidifying the Inlet Gases
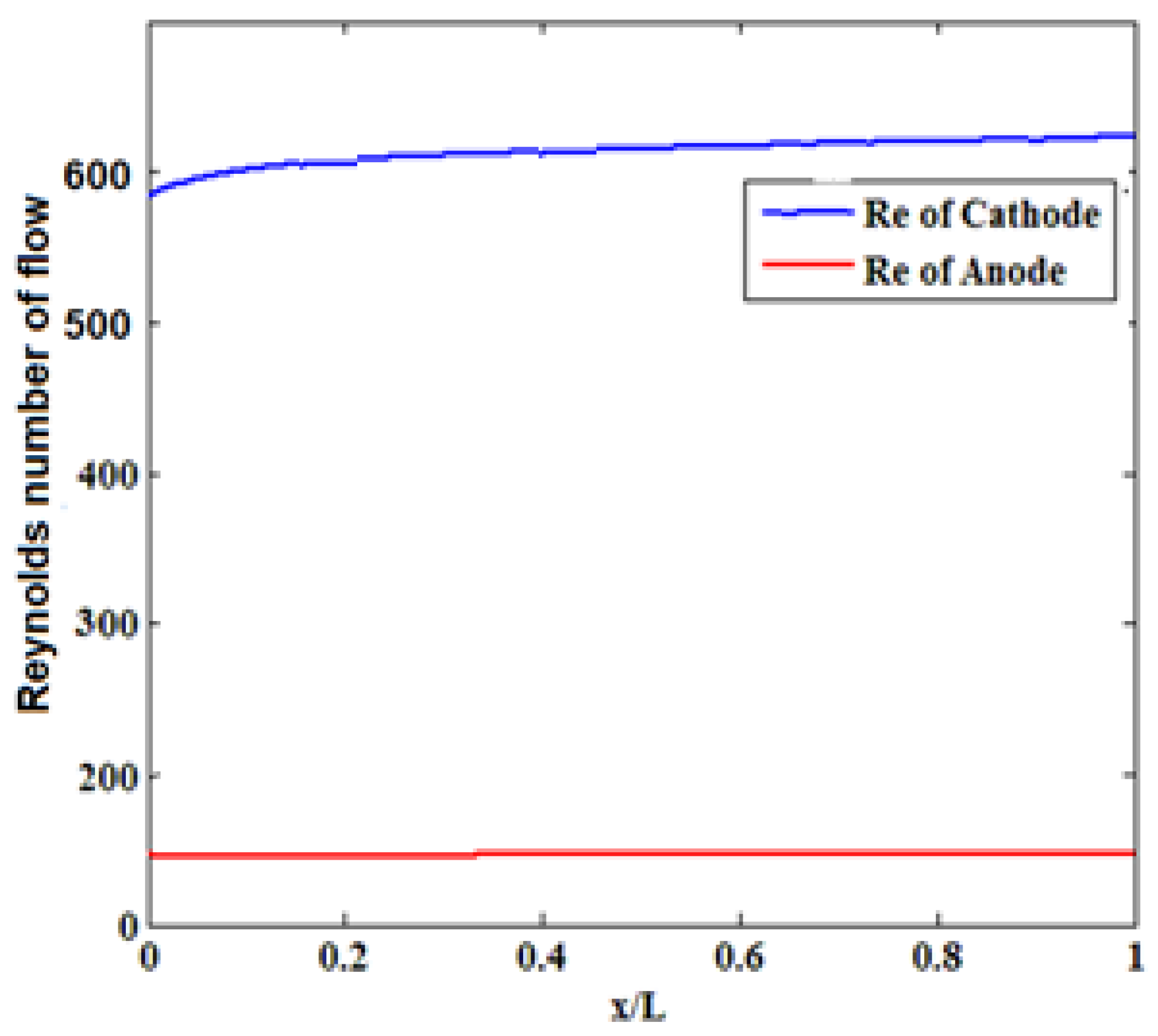
3.4. Reynolds Number
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| L | Bipolar plate height |
| W | Bipolar plane width |
| W_g | Groove width |
| H_g | Groove depth |
| W_s | The width of the solid part |
| K_c | Constant condensate/water evaporation rate |
| I_ave | Average current density |
| S | Stoichiometric coefficient |
| T | Temperature |
| RH | relative humidity |
| P | Pressure |
| E | Cell voltage |
| GDL | Gas penetration layer |
| D | Infiltration coefficient |
| Ecell | Cell voltage |
| K | Hydraulic permeability |
| Cathode inlet temperature | |
| Anode inlet temperature | |
| Anode inlet pressure | |
| Cathode inlet pressure | |
| Ρ | Density |
| σ | Surface tension |
| ν | Cinematic viscosity |
| Cathode surface temperature | |
| Anode surface temperature | |
| Tmem | Membrane temperature |
| tm | Membrane thickness |
| The thickness of the gas penetration layer | |
| anode stoichiometric coefficient | |
| cathode stoichiometric coefficient | |
| cross-section | |
| Faraday constant | |
| input molar flow rate to flow channels for hydrogen and oxygen, respectively | |
| the vapor phase index | |
| R | demonstrates the universal gas constant |
| permeability of liquid water in the GDL | |
| the liquid water density | |
| the water molecular weight | |
| output voltage of a cell | |
| the activation overpotential (v), ohmic overpotential (v) | |
| and | partial pressures related to hydrogen and oxygen (Pa) |
| the oxygen concentration at the catalyst level | |
| the oxygen concentration in the flow channel | |
| the oxygen-effective diffusion coefficient in the gaseous mixture | |
| the gaseous mixture heat capacity | |
| the gas mixture thermal conductivity | |
| the friction coefficient | |
| the fluid viscosity | |
| , , and | the total value of the molar rate, mass rate, and volumetric flow rate of the gas entering the flow channels |
| and | partial pressure and saturation pressure of water vapor |
References
- Wang, Z.; Xu, C.; Wang, X.; Liao, Z.; Du, X. Numerical investigation of water and temperature distributions in a proton exchange membrane electrolysis cell. Sci. China Technol. Sci. 2021, 64, 1555–1566. [Google Scholar] [CrossRef]
- Upadhyay, M.; Lee, S.; Jung, S.; Choi, Y.; Moon, S.; Lim, H. Systematic assessment of the anode flow field hydrodynamics in a new circular PEM wa-ter electrolyser. Int. J. Hydrogen Energy 2020, 45, 20765–20775. [Google Scholar] [CrossRef]
- Zinser, A.; Papakonstantinou, G.; Sundmacher, K. Analysis of mass transport processes in the anodic porous transport layer in PEM water electrolyzes. Int. J. Hydrogen Energy 2019, 44, 28077–28087. [Google Scholar] [CrossRef]
- Nafchi, F.M.; Afshari, E.; Baniasadi, E.; Javani, N. A parametric study of polymer membrane electrolyzes performance, energy and exergy analyses. Int. J. Hydrogen Energy 2019, 44, 18662–18670. [Google Scholar] [CrossRef]
- Baykara, S.Z. Hydrogen: A brief overview on its sources, production and environmental impact. Int. J. Hydrogen Energy 2018, 43, 10605–10614. [Google Scholar] [CrossRef]
- Schmidt, O.; Gambhir, A.; Staffell, I.; Hawkes, A.; Nelson, J.; Few, S. Future cost and performance of water electrolysis: An expert elicitation study. Int. J. Hydrogen Energy 2017, 42, 30470–30492. [Google Scholar] [CrossRef]
- Dincer, I.; Acar, C. Review and evaluation of hydrogen production methods for better sustainability. Int. J. Hydrogen Energy 2015, 40, 11094–11111. [Google Scholar] [CrossRef]
- Meng, H.; Ruan, B. Numerical studies of cold-start phenomena in PEM fuel cells: A review. Int. J. Energy Res. 2010, 35, 2–14. [Google Scholar] [CrossRef]
- Perry, M.; Fuller, T.F. A Historical Perspective of Fuel Cell Technology in the 20th Century. J. Electrochem. Soc. 2002, 149, S59–S67. [Google Scholar] [CrossRef]
- Wang, C.-Y. Fundamental Models for Fuel Cell Engineering. Chem. Rev. 2004, 104, 4727–4766. [Google Scholar] [CrossRef]
- Bernardi, D.M.; Verbrugge, M.W. A mathematical model of the solid-polymer-electrolyte fuel cell. J. Electrochem. Soc. 1992, 139, 2451–2477. [Google Scholar] [CrossRef]
- Motupally, S.; Becker, A.J.; Weidner, J. Diffusion of Water in Nafion 115 Membranes. J. Electrochem. Soc. 2000, 147, 3171–3177. [Google Scholar] [CrossRef]
- Costamagna, P. Transport phenomena in polymeric membrane fuel cells. Chem. Eng. Sci. 2001, 56, 323–332. [Google Scholar] [CrossRef]
- Meng, H.; Wang, C.-Y. Large-scale simulation of polymer electrolyte fuel cells by parallel computing. Chem. Eng. Sci. 2004, 59, 3331–3343. [Google Scholar] [CrossRef]
- Ju, H.; Meng, H.; Wang, C.-Y. A single-phase, non-isothermal model for PEM fuel cells. Int. J. Heat Mass Transf. 2005, 48, 1303–1315. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, C.Y. Modeling polymer electrolyte fuel cells with large density and velocity changes. J. Electrochem. Soc. 2005, 152, A441–A452. [Google Scholar] [CrossRef]
- Meng, H. A three-dimensional PEM fuel cell model with consistent treatment of water transport in MEA. J. Power Sources 2006, 162, 426–435. [Google Scholar] [CrossRef]
- Ju, H.; Wang, C.-Y. Experimental Validation of a PEM Fuel Cell Model by Current Distribution Data. J. Electrochem. Soc. 2004, 151, A1954–A1960. [Google Scholar] [CrossRef]
- Tsushima, S.; Teranishi, K.; Hirai, S. Magnetic Resonance Imaging of the Water Distribution within a Polymer Electrolyte Membrane in Fuel Cells. Electrochem. Solid-State Lett. 2004, 7, A269–A272. [Google Scholar] [CrossRef]
- Spernjak, D.; Prasad, A.K.; Advani, S.G. Experimental investigation of liquid water formation and transport in a transparent single-serpentine PEM fuel cell. J. Power Sources 2007, 170, 334–344. [Google Scholar] [CrossRef]
- Hooshyari, K.H.; Moradi, M.; Salarizadeh, P. Novel nanocomposite membranes based on PBI and doped-perovskite nanoparticles as a strategy for improving PEMFC performance at high temperatures. Int. J. Energy Res. 2020, 10, 2709–2721. [Google Scholar] [CrossRef]
- Hussey, D.; Jacobson, D.; Arif, M.; Owejan, J.; Gagliardo, J.; Trabold, T. Neutron images of the through-plane water distribution of an operating PEM fuel cell. J. Power Sources 2007, 172, 225–228. [Google Scholar] [CrossRef]
- Gurau, V.; Zawodzinski, T.A.; Mann, J.A. Two-Phase Transport in PEM Fuel Cell Cathodes. J. Fuel Cell Sci. Technol. 2008, 5, 021009. [Google Scholar] [CrossRef]
- Le, A.D.; Zhou, B.; Shiu, H.-R.; Lee, C.-I.; Chang, W.-C. Numerical simulation and experimental validation of liquid water behaviors in a proton exchange membrane fuel cell cathode with serpentine channels. J. Power Sources 2010, 195, 7302–7315. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, C.-Y. Transient analysis of polymer electrolyte fuel cells. Electrochim. Acta 2005, 50, 1307–1315. [Google Scholar] [CrossRef]
- Shimpalee, S.; Lee, W.K.; Van Zee, J.W.; Naseri-Neshat, H. Predicting the transient response of a serpentine flow-field PEMFC I. Excess to normal fuel and air. J. Power Sources 2006, 156, 355–368. [Google Scholar] [CrossRef]
- Wu, H.; Berg, P.; Li, X. Non-isothermal transient modeling of water transport in PEM fuel cells. J. Power Sources 2007, 165, 232–243. [Google Scholar] [CrossRef]
- Meng, H. Numerical investigation of transient responses of a PEM fuel cell using a two-phase non-isothermal mixed-domain model. J. Power Sources 2007, 171, 738–746. [Google Scholar] [CrossRef]
- Sun, H.; Zhang, G.; Guo, L.; Liu, H. A Study of dynamic characteristics of PEM fuel cells by measuring local currents. Int. J. Hydrogen Energy 2009, 34, 5529–5536. [Google Scholar] [CrossRef]
- Ge, S.; Wang, C.-Y. Characteristics of subzero startup and water/ice formation on the catalyst layer in a polymer electrolyte fuel cell. Electrochim. Acta 2007, 52, 4825–4835. [Google Scholar] [CrossRef]
- Um, S.; Wang, C.-Y. Computational study of water transport in proton exchange membrane fuel cells. J. Power Sources 2006, 156, 211–223. [Google Scholar] [CrossRef]
- Sundaresan, M.; Moore, R. Polymer electrolyte fuel cell stack thermal model to evaluate sub-freezing startup. J. Power Sources 2005, 145, 534–545. [Google Scholar] [CrossRef]
- Ahluwalia, R.K.; Wang, X. Rapid self-start of polymer electrolyte fuel cell stacks from subfreezing temperatures. J. Power Sources 2006, 162, 502–512. [Google Scholar] [CrossRef]
- Zhang, X.; Guo, J.; Chen, J. The parametric optimum analysis of a proton exchange membrane (PEM) fuel cell and its load matching. Energy 2010, 35, 5294–5299. [Google Scholar] [CrossRef]
- Watari, T.; Wang, H.; Kuwahara, K.; Tanaka, K.; Kita, H.; Okamoto, K.-I. Water vapor sorption and diffusion properties of sulfonated polyimide membranes. J. Membr. Sci. 2003, 219, 137–147. [Google Scholar] [CrossRef]
- Burnett, D.J.; Garcia, A.R.; Thielmann, F. Measuring moisture sorption and diffusion kinetics on proton exchange membranes using a gravimetric vapor sorption apparatus. J. Power Sources 2006, 160, 426–430. [Google Scholar] [CrossRef]
- Mizoguchi, K.; Terada, K.; Naito, Y.; Kamiya, Y. Gas Transport in Nafion Membrane. J. Power Sources. 2009, 49, 3517–3518. [Google Scholar]
- Dreyfus, B.; Gebel, G.; Aldebert, P.; Pineri, M.; Escoubes, M.; Thomas, M. Distribution of the « micelles » in hydrated perfluorinated ionomer membranes from SANS experiments. J. De Phys. 1990, 51, 1341–1354. [Google Scholar] [CrossRef]
- Ramousse, J.; Lottin, O.; Didierjean, S.; Maillet, D. Heat sources in proton exchange membrane (PEM) fuel cells. J. Power Sources 2009, 192, 435–441. [Google Scholar] [CrossRef]
- Conway, B.; Wilkinson, D. Non-isothermal cell potentials and evaluation of entropies of ions and of activation for single electrode processes in non-aqueous media. Electrochim. Acta 1993, 38, 997–1013. [Google Scholar] [CrossRef]
- Pehlivan, K.; Hassan, I.; Vaillancourt, M. Experimental study on two-phase flow and pressure drop in millimeter-size chan-nels. Therm. Eng. 2006, 26, 1506–1514. [Google Scholar] [CrossRef]
- Lin, G.; He, W.; Van Nguyen, T. Modeling Liquid Water Effects in the Gas Diffusion and Catalyst Layers of the Cathode of a PEM Fuel Cell. J. Electrochem. Soc. 2004, 151, A1999–A2006. [Google Scholar] [CrossRef]
- Jain, S.; Kumar, L. Fundamentals of Power Electronics Controlled Electric Propulsion. In Power Electronics Handbook; Elsevier BV: Amsterdam, The Netherlands, 2018; pp. 1023–1065. [Google Scholar]
- Berning, T.; Djilali, N. A 3D, multiphase, multi-component model of the cathode and anode of a PEM fuel cell. J. ElectroChem. Soc. 2003, 150, A1589–A1598. [Google Scholar] [CrossRef]
- Springer, T.E.; Zawodzinski, T.A.; Gottesfeld, S. Polymer Electrolyte Fuel Cell Model. J. Electrochem. Soc. 1991, 138, 2334–2342. [Google Scholar] [CrossRef]
- Nguyen, T.V.; White, R.E. A water and heat management model for proton-exchange-membrane fuel cells. J. ElectroChem. Soc. 1993, 140, 2178–2186. [Google Scholar] [CrossRef]
- Pritchard, P.J. Fox and McDonald’s Introduction to Fluid Mechanics, 8th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2011; p. 800. [Google Scholar]
- Ravikanth, S.V.; Dustin, K.D. Development of new correlations for the Nusselt number and the friction factor under turbulent flow of nanofluids in flat tubes. Int. J. Heat Mass Transf. 2014, 80, 353–367. [Google Scholar]
- Frano, B. PEM Fuel Cells: Theory and Practice; Elsevier Academic Press: Burlington, MA, USA, 2005; p. 456. ISBN 9780123877109. [Google Scholar]
- Luo, Y.; Guo, Q.; Du, Q.; Yin, Y.; Jiao, K. Analysis of cold start processes in proton exchange membrane fuel cell stacks. J. Power Sources 2013, 224, 99–114. [Google Scholar] [CrossRef]
- Han, B.; Meng, H. Numerical studies of interfacial phenomena in liquid water transport in polymer electrolyte membrane fuel cells using the lattice Boltzmann method. Int. J. Hydrogen Energy 2013, 38, 5053–5059. [Google Scholar] [CrossRef]
- Manahan, M.P.; Mench, M.M. Laser perforated fuel cell diffusion media: Engineering interfaces for improved ionic and oxy-gen. J. Electrochem. Soc. 2012, 159, F322. [Google Scholar] [CrossRef]
- Gerteisen, D.; Heilmann, T.; Ziegler, C. Enhancing liquid water transport by laser perforation of a GDL in a PEM fuel cell. J. Power Sources 2008, 177, 348–354. [Google Scholar] [CrossRef]
- He, G.L.; Yamazaki, Y.; Abudula, A. A three-dimensional analysis of the effect of anisotropic gas diffusion layer (GDL) thermal conductivity on the heat transfer and two-phase behavior in a proton exchange membrane fuel cell (PEMFC). J. Power Sources 2010, 195, 1551–1560. [Google Scholar] [CrossRef]
- Bapat, C.J.; Thynell, S.T. Effect of anisotropic thermal conductivity of the GDL and current collector rib width on two-phase transport in a PEM fuel cell. J. Power Sources 2008, 179, 240–251. [Google Scholar] [CrossRef]
- Bajpai, H.; Khandelwal, M.; Kumbur, E.; Mench, M. A computational model for assessing impact of interfacial morphology on polymer electrolyte fuel cell performance. J. Power Sources 2010, 195, 4196–4205. [Google Scholar] [CrossRef]
- Hizir, F.E.; Ural, S.O.; Kumbur, E.C.; Mench, M.M. Characterization of interfacial morphology in polymer electrolyte fuel cells: Mi-croporous layer and catalyst layer surfaces. J. Power Sources 2010, 195, 3463–3471. [Google Scholar] [CrossRef]
- Mondal, B.; Jiao, K.; Li, X. Three-dimensional simulation of water droplet movement in PEM fuel cell flow channels with hy-drophilic surfaces. Int. J. Energy Res. 2011, 35, 1200–1212. [Google Scholar] [CrossRef]
- Chen, L.; Luan, H.; He, Y.-L.; Tao, W.-Q. Effects of Roughness of Gas Diffusion Layer Surface on Liquid Water Transport in Micro Gas Channels of a Proton Exchange Membrane Fuel Cell. Numer. Heat Transf. Part A Appl. 2012, 62, 295–318. [Google Scholar] [CrossRef]
- Jiao, K.; Zhou, B. Effects of electrode wettability on liquid water behaviors in PEM fuel cell cathode. J. Power Sources 2008, 175, 106–119. [Google Scholar] [CrossRef]
- Ji, Y.; Luo, G.; Wang, C.Y. Pore-Level liquid water transportthrough composite diffusion media of PEMFC. J. Electrochem. Soc. 2010, 157, B1753–B1761. [Google Scholar] [CrossRef]
- Gracz, W.; Marcinkowski, D.; Golimowski, W.; Szwajca, F.; Strzelczyk, M.; Wasilewski, J.; Krzaczek, P. Multifaceted Com-parison Efficiency and Emission Characteristics of Multi-Fuel Power Generator Fueled by Different Fuels and Biofuels. Energies 2021, 14, 3388. [Google Scholar] [CrossRef]
- Dzieniszewski, G.; Kuboń, M.; Pristavka, M.; Findura, P. Operating Parameters and Environmental Indicators of Diesel En-gines Fed with Crop-Based Fuels. Agric. Eng. 2021, 25, 13–28. [Google Scholar]
- Hiramitsu, Y.; Mitsuzawa, N.; Okada, K.; Hori, M. Effects of ionomer content and oxygen permeation of the catalyst layer on proton exchange membrane fuel cell cold start-up. J. Power Sources 2010, 195, 1038–1045. [Google Scholar] [CrossRef]


| Number | Variable Name | Parameter Type | Variable Symbols 1 | Title 2 | Title 3 |
|---|---|---|---|---|---|
| 1 | Bipolar plate dimensions and groove dimensions | Constant | L_BP & W_BP | data | data |
| 2 | Number of nodes | Variable | N_s | data | data |
| 3 | The temperature of anode and cathode bipolar plate surface, membrane, and electrode surface | Variable | t_c_In & t_a_In | ||
| 4 | Dry membrane weight, thickness, and porosity | Constant | M_m_dry & t_m & Phi | ||
| 5 | The diffusion coefficient, anode, and cathode heat transfer coefficient | Constant | D_std_O2_H2O & D_std_O2_N2 & h_h_a & h_h_c | ||
| 6 | Oxygen and nitrogen molar fraction in the inlet air, the total inlet pressure of the cathode and anode | Constant | Yo2_c_In & Yn2_c_In & P_c_In & P_a_In | ||
| 7 | Average current density, relative humidity of cathode and anode inputs | Variable | I_ave & RH_c_In & RH_a_In |
| Title 1 | Parameter | Amount |
|---|---|---|
| cell | ∘I | 100 (Am−2) |
| 2 | 2.0 | |
| Ium | 2.1 × 104 (Am−2) | |
| cell | Ecell | 0.01–1.04 (v) |
| w | 1 (mm) | |
| Flow channel | h | 1 (mm) |
| ws | 1 (mm) | |
| ng | 5 | |
| Sc | 1.7 | |
| Flow channel | Sa | 1.1 |
| 70 (°C) | ||
| Flow channel | 70 (°C) | |
| Flow channel | 1.0 | |
| Flow channel | 1.0 | |
| Flow channel | 1.5 (atm) | |
| Flow channel | 1.5 (atm) | |
| Gas flow distributor layer | 0.21 | |
| Gas flow distributor layer | 0.3 (mm) | |
| Gas flow distributor layer | Φ | 60% |
| Gas flow distributor layer | 1 × 10−10 (m2) | |
| Gas flow distributor layer | dPc/dS | −28.42 (Pa m−1) |
| Gas flow distributor layer | kc | 1 (s−1) |
| Gas flow distributor layer | 0 | |
| Membrane | tm | 0.1275 (mm) |
| Membrane | Tmem | 70 (°C) |
| Membrane | m,dry | 2000 (kg m−3) |
| Membrane | Mm,dry | 1.1 (kg mole−1) |
| Bipolar plates | W | 9.9 (cm) |
| Bipolar plates | L | 9.9 (cm) |
| ns | 400 | |
| Other parameters | Iave | 2000 (Am−2) |
| Other parameters | 70 (°C) | |
| Other parameters | 70 (°C) | |
| Other parameters | 70 (°C) | |
| Other parameters | 70 (°C) | |
| Other parameters | Do | 5.5 × 10−11 (m2s−1) |
| Other parameters | 0.36 × 10−4 (m2s−1) | |
| Other parameters | 0.18 × 10−4 (m2s−1) | |
| Other parameters | ha | 25 (Wm−2k−1) |
| Other parameters | hc | 25 (Wm−2k−1) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Darvishi, Y.; Hassan-Beygi, S.R.; Zarafshan, P.; Hooshyari, K.; Malaga-Toboła, U.; Gancarz, M. Numerical Modeling and Evaluation of PEM Used for Fuel Cell Vehicles. Materials 2021, 14, 7907. https://doi.org/10.3390/ma14247907
Darvishi Y, Hassan-Beygi SR, Zarafshan P, Hooshyari K, Malaga-Toboła U, Gancarz M. Numerical Modeling and Evaluation of PEM Used for Fuel Cell Vehicles. Materials. 2021; 14(24):7907. https://doi.org/10.3390/ma14247907
Chicago/Turabian StyleDarvishi, Yousef, Seyed Reza Hassan-Beygi, Payam Zarafshan, Khadijeh Hooshyari, Urszula Malaga-Toboła, and Marek Gancarz. 2021. "Numerical Modeling and Evaluation of PEM Used for Fuel Cell Vehicles" Materials 14, no. 24: 7907. https://doi.org/10.3390/ma14247907
APA StyleDarvishi, Y., Hassan-Beygi, S. R., Zarafshan, P., Hooshyari, K., Malaga-Toboła, U., & Gancarz, M. (2021). Numerical Modeling and Evaluation of PEM Used for Fuel Cell Vehicles. Materials, 14(24), 7907. https://doi.org/10.3390/ma14247907







