Throughput Improvement in Femtosecond Laser Ablation of Nickel by Double Pulses
Abstract
:1. Introduction
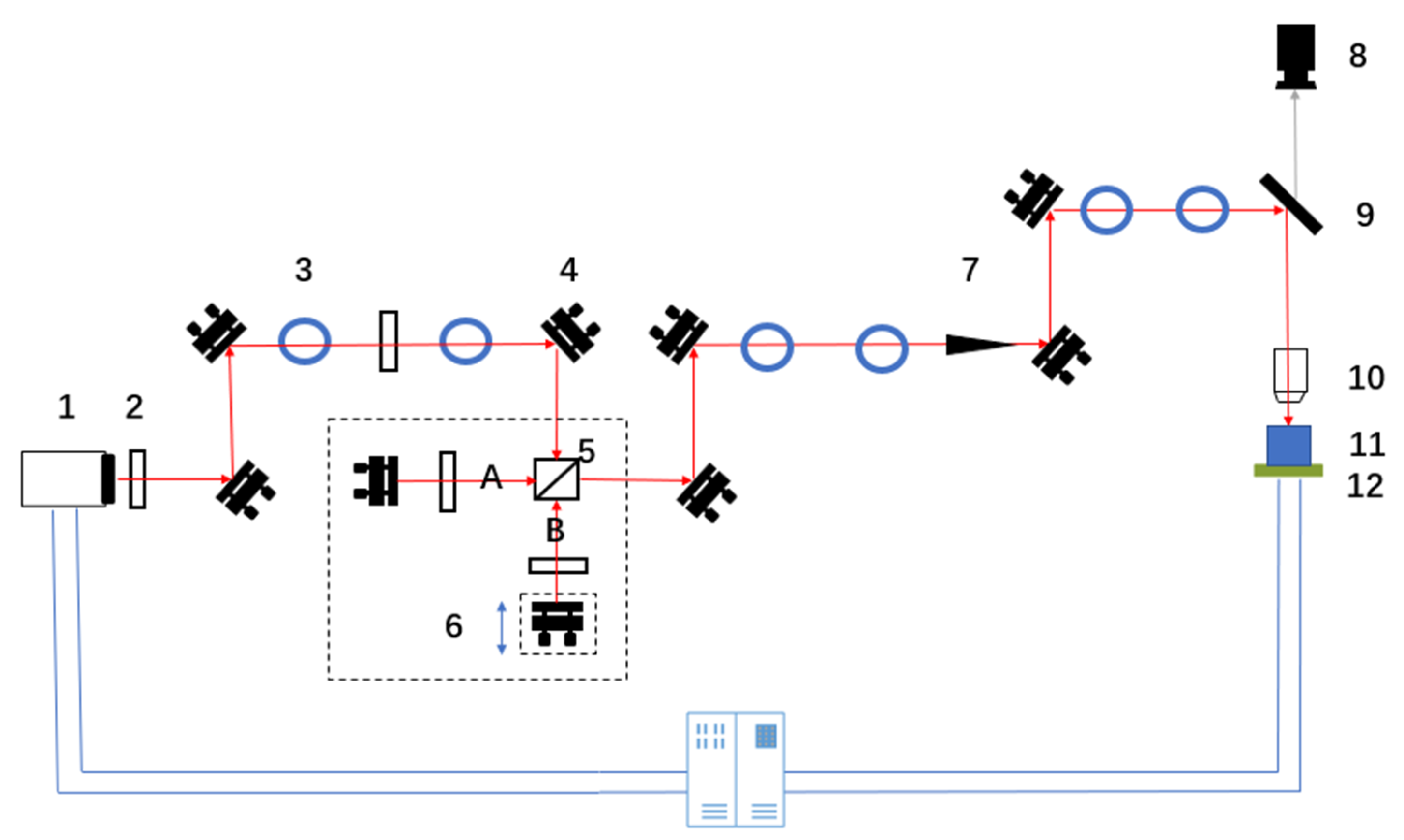
2. Materials and Methods
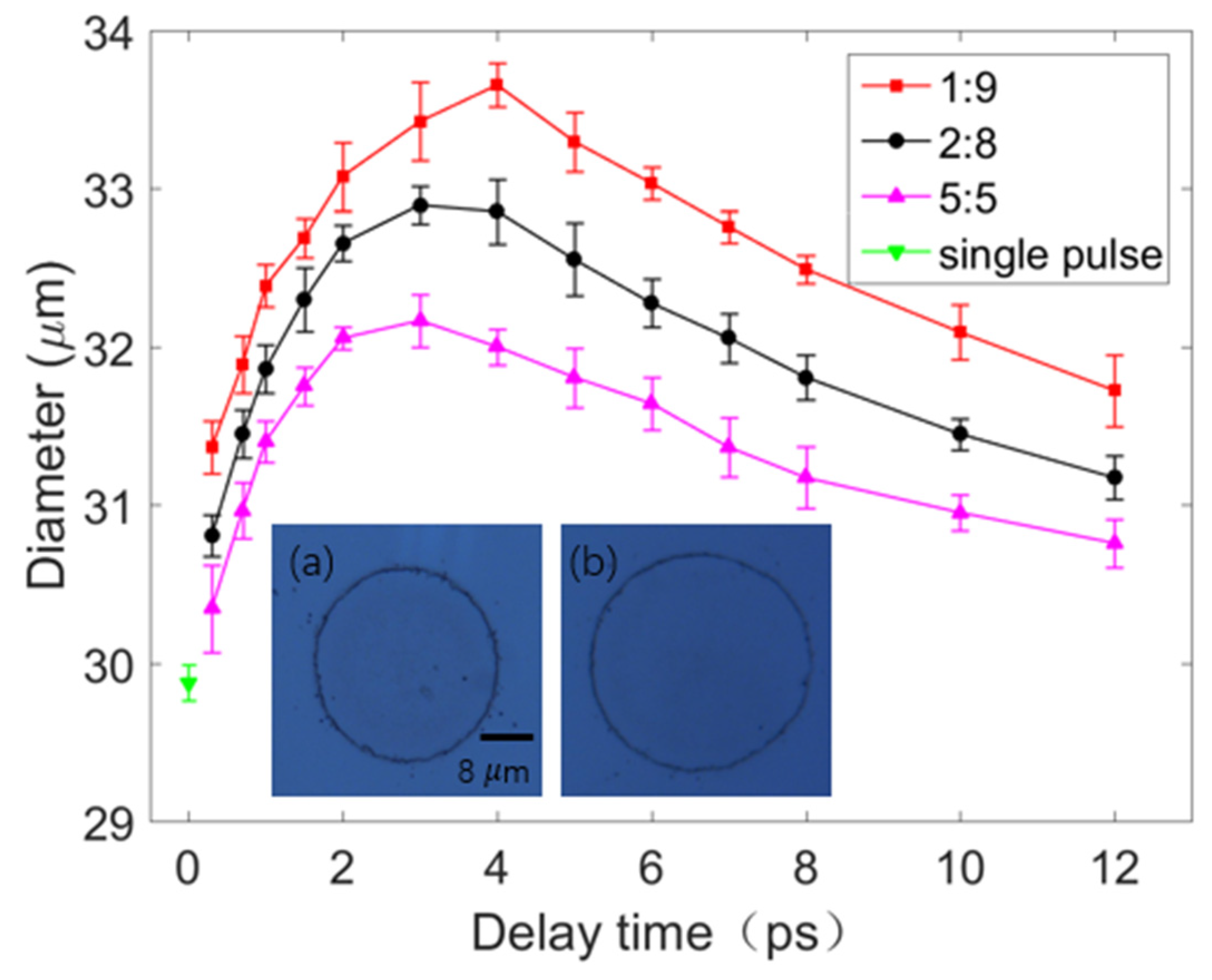
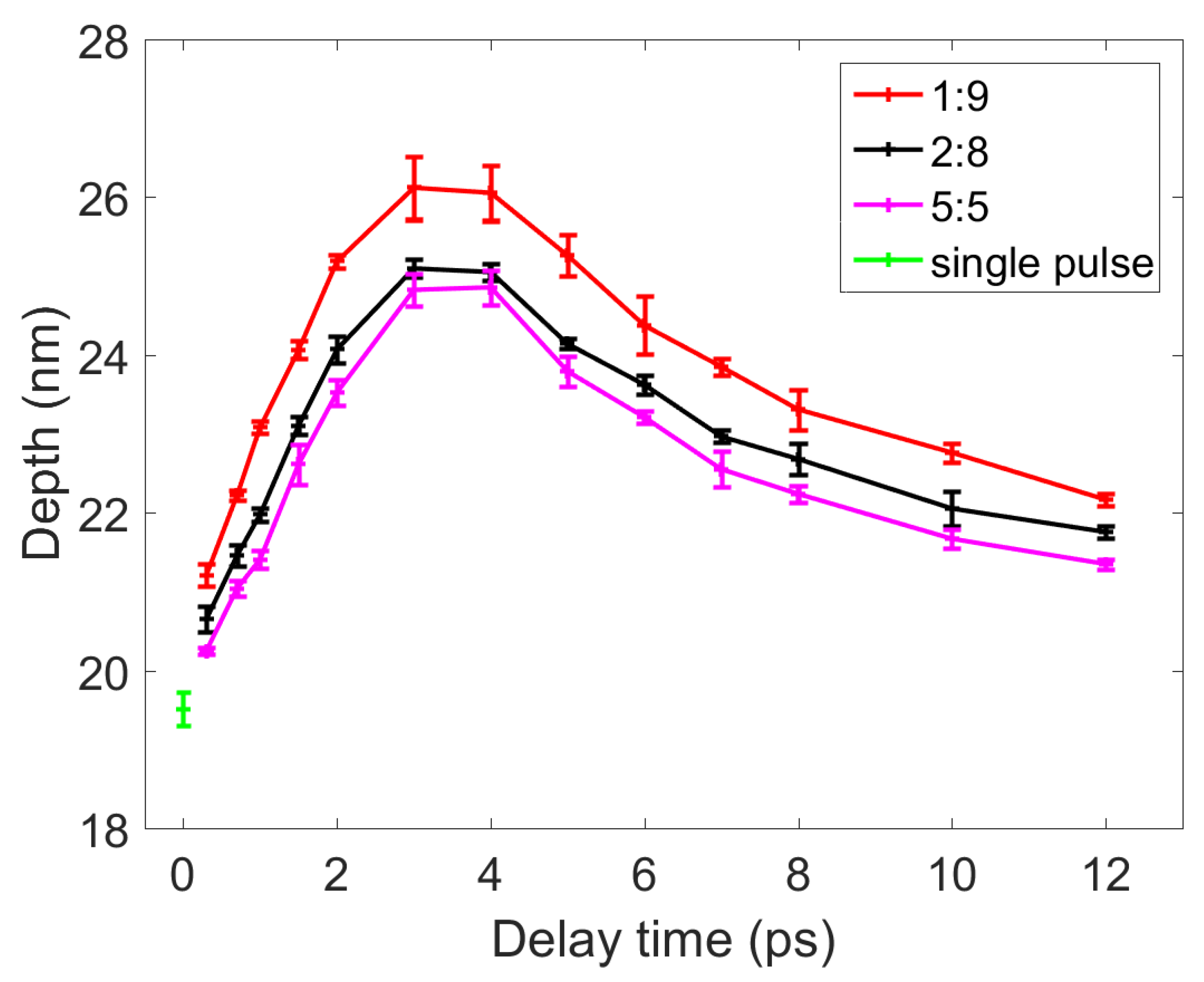
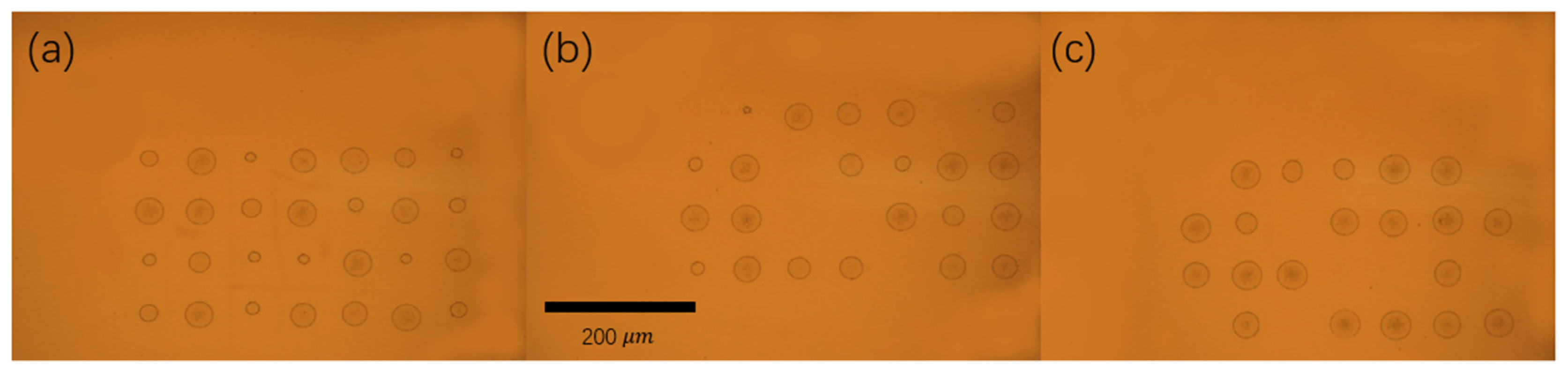
3. Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhao, Y.; Han, Q.; Cheng, Z.; Jiang, L.; Qu, L. Integrated graphene systems by laser irradiation for advanced devices. Nano Today 2017, 12, 14–30. [Google Scholar] [CrossRef]
- Yuan, Y.; Jiang, L.; Li, X.; Zuo, P.; Xu, C.; Tian, M.; Zhang, X.; Wang, S.; Lu, B.; Shao, C.; et al. Laser photonic-reduction stamping for graphene-based micro-supercapacitors ultrafast fabrication. Nat. Commun. 2020, 11, 6185. [Google Scholar] [CrossRef]
- Wang, Z.; Wu, Q.; Yang, C.; Zhang, X.; Chen, Z.; Rupp, R.A.; Xu, J. Nonlinear spectrum broadening of femtosecond laser pulses in photorefractive waveguide arrays. Opt. Express 2010, 18, 10112–10119. [Google Scholar] [CrossRef]
- Wang, C.; Luo, Z.; Duan, J.; Jiang, L.; Sun, X.; Hu, Y.; Zhou, J.; Lu, Y. Adjustable annular rings of periodic surface structures induced by spatially shaped femtosecond laser. Laser Phys. Lett. 2015, 12, 056001. [Google Scholar] [CrossRef]
- Wang, A.; Jiang, L.; Li, X.; Li, Y.; Dong, X.; Qu, L.; Duan, X.; Lu, Y. Mask-free patterning of high-conductivity metal nanowires in open air by spatially modulated femtosecond laser pulses. Adv. Mater. 2015, 27, 6238–6243. [Google Scholar] [CrossRef]
- Fan, P.; Bai, B.; Long, J.; Jiang, D.; Jin, G.; Zhang, H.; Zhong, M. Broadband high-performance infrared antireflection nanowires facilely grown on ultrafast laser structured Cu surface. Nano Lett. 2015, 15, 5988–5994. [Google Scholar] [CrossRef]
- Cao, Y.Y.; Takeyasu, N.; Tanaka, T.; Duan, X.M.; Kawata, S. 3D metallic nanostructure fabrication by surfactant-assisted multiphoton-induced reduction. Small 2009, 5, 1144–1148. [Google Scholar] [CrossRef] [PubMed]
- Kerse, C.; Kalaycıoğlu, H.; Elahi, P.; Çetin, B.; Kesim, D.K.; Akçaalan, Ö.; Yavaş, S.; Aşık, M.D.; Öktem, B.; Hoogland, H.; et al. Ablation-cooled material removal with ultrafast bursts of pulses. Nature 2016, 537, 84–88. [Google Scholar] [CrossRef] [PubMed]
- Jiang, L.; Tsai, H.L. Repeatable nanostructures in dielectrics by femtosecond laser pulse trains. Appl. Phys. Lett. 2005, 87, 151104. [Google Scholar] [CrossRef]
- Wang, M.; Yang, L.; Zhang, S.; Wang, Y. Experimental investigation on the spiral trepanning of K24 superalloy with femtosecond laser. Opt. Laser Technol. 2018, 101, 284–290. [Google Scholar] [CrossRef]
- Lundgren, E.H.; Forsman, A.C.; Hoppe, M.L.; Moreno, K.A.; Nikroo, A. Fabrication of pressurized 2 mm beryllium targets for ICF experiments. Fusion Sci. Technol. 2007, 51, 576–580. [Google Scholar]
- Lundgren, E.H.; Forsman, A.C. Laser forming of shaped fill holes in beryllium targets for inertial confinement fusion experiments. Fusion Sci. Technol. 2009, 55, 325–330. [Google Scholar]
- Shen, J.; Yang, Z.; Liu, X.; Shi, Y.; Zhao, P.; Sun, S.; Hu, B. Analysis of Enhanced Laser-Induced Breakdown Spectroscopy with Double Femtosecond Laser Pulses. Plasma Sci. Technol. 2015, 17, 147–152. [Google Scholar] [CrossRef]
- Chandu, B.; Bharati, M.S.S.; Albrycht, P.; Rao, S.V. Fabrication of nanocages on nickel using femtosecond laser ablation and trace level detection of malachite green and Nile blue dyes using surface enhanced Raman spectroscopic technique. Opt. Laser Technol. 2020, 131, 106454. [Google Scholar]
- Cheng, Y.; Yang, Q.; Lu, Y.; Yong, J.; Fang, Y.; Hou, X.; Chen, F. A femtosecond Bessel laser for preparing a nontoxic slippery liquid-infused porous surface (SLIPS) for improving the hemocompatibility of NiTi alloys. Biomater. Sci. 2020, 8, 6505–6514. [Google Scholar] [CrossRef] [PubMed]
- Suematsu, Y.; Saito, K.; Koyama, M.; Enokida, Y.; Okura, Y.; Nakayasu, T.; Sukegawa, T. Development of micro-mirror slicer integral field unit for space-borne solar spectrographs. CEAS Space J. 2017, 9, 421–431. [Google Scholar]
- Liu, Y.Z. Coaxial waterjet-assisted laser drilling of film cooling holes in turbine blades. Int. J. Mach. Tools Manuf. 2020, 150, 103510. [Google Scholar] [CrossRef]
- Hang, Y.S.; Xu, Z.Y.; Wang, F.; Zhang, C.X. Electrochemical Discharge Drilling of Inclined Micro Holes with Step Feeding Method. Int. J. Electrochem. Sci. 2020, 15, 2713–2726. [Google Scholar]
- Zhang, Z.; Wang, W.; Jiang, R.; Zhang, X.; Xiong, Y.; Mao, Z. Investigation on geometric precision and surface quality of microholes machined by ultrafast laser. Opt. Laser Technol. 2020, 121, 105834. [Google Scholar]
- Zhang, K.; Zhang, J.; Jiang, L.; Li, X.; Liu, Y.; Li, B.; Lu, L. Ablation enhancement of metal in ultrashort double-pulse experiments. Appl. Phys. Lett. 2018, 112, 261906. [Google Scholar] [CrossRef]
- Donnelly, T.; Lunney, J.G.; Amoruso, S.; Bruzzese, R.; Wang, X.; Ni, X. Double pulse ultrafast laser ablation of nickel in vacuum. J. Appl. Phys. 2009, 106, 013304. [Google Scholar] [CrossRef] [Green Version]
- Gaudiuso, C.; Giannuzzi, G.; Volpe, A.; Lugarà, P.M.; Choquet, I.; Ancona, A. Incubation during laser ablation with bursts of femtosecond pulses with picosecond delays. Opt. Express 2018, 26, 3801–3813. [Google Scholar] [CrossRef]
- Mur, J. Near-THz bursts of pulses-Governing surface ablation mechanisms for laser material processing. Appl. Surf. Sci. 2019, 478, 355–360. [Google Scholar] [CrossRef]
- Liu, Z.; Wu, B.; Xu, R.; Zhao, K.; Shin, Y.C. Microhole drilling by double laser pulses with different pulse energies. J. Manuf. Sci. Eng. 2018, 140, 091015. [Google Scholar] [CrossRef] [Green Version]
- Du, G.; Chen, F.; Yang, Q.; Si, J.; Hou, X. Ultrafast temperature relaxation evolution in Au film under femtosecond laser pulses irradiation. Opt. Commun. 2010, 283, 1869–1872. [Google Scholar] [CrossRef]
- Du, G.; Yang, Q.; Chen, F.; Ou, Y.; Wu, Y.; Hou, X. Ultrafast dynamics of laser thermal excitation in gold film triggered by temporally shaped double pulses. Int. J. Therm. Sci. 2015, 90, 197–202. [Google Scholar] [CrossRef]
- Du, G.; Yang, Q.; Chen, F.; Si, J.; Hou, X. Insight into the thermionic emission regimes under gold film thermal relaxation excited by a femtosecond pulse. Appl. Surf. Sci. 2011, 257, 9177–9182. [Google Scholar] [CrossRef]
- Ren, Y.; Chen, J.; Zhang, Y.; Huang, J. Ultrashort laser pulse energy deposition in metal films with phase changes. Appl. Phys. Lett. 2011, 98, 191105. [Google Scholar] [CrossRef]
- Chen, J.; Latham, W.; Beraun, J.E. The role of electron–phonon coupling in ultrafast laser heating. J. Laser Appl. 2005, 17, 63–68. [Google Scholar] [CrossRef]
- Wellershoff, S.S.; Hohlfeld, J.; Güdde, J.; Matthias, E. The role of electron–phonon coupling in femtosecond laser damage of metals. Appl. Phys. A 1999, 69, S99–S107. [Google Scholar]
- Chen, A.; Xu, H.; Jiang, Y.; Sui, L.; Ding, D.; Liu, H.; Jin, M.X. Modeling of femtosecond laser damage threshold on the two-layer metal films. Appl. Surf. Sci. 2010, 257, 1678–1683. [Google Scholar]
- Lin, Z.; Zhigilei, L.V.; Celli, V. Electron-phonon coupling and electron heat capacity of metals under conditions of strong electron-phonon nonequilibrium. Phys. Rev. B 2008, 77, 075133. [Google Scholar] [CrossRef] [Green Version]
- Zhigilei, L.V.; Lin, Z.; Ivanov, D.S. Atomistic modeling of short pulse laser ablation of metals: Connections between melting, spallation, and phase explosion. J. Phys. Chem. C 2019, 113, 11892–11906. [Google Scholar]
- Mueller, B.; Rethfeld, B. Relaxation dynamics in laser-excited metals under nonequilibrium conditions. Phys. Rev. B 2013, 87, 196. [Google Scholar] [CrossRef]
- Jiang, L.; Tsai, H.L. A plasma model combined with an improved two-temperature equation for ultrafast laser ablation of dielectrics. J. Appl. Phys. 2008, 104, 151104. [Google Scholar]
- Jia, X.; Zhao, X. Numerical investigation of ultrashort laser interaction with dielectric materials based on a plasma temperature combined model. J. Manuf. Process. 2017, 28, 508–514. [Google Scholar] [CrossRef]
- Zhang, J.; Chen, Y.; Hu, M.; Chen, X. An improved three-dimensional two-temperature model for multi-pulse femtosecond laser ablation of aluminum. J. Appl. Phys. 2015, 117, 063104. [Google Scholar] [CrossRef] [Green Version]

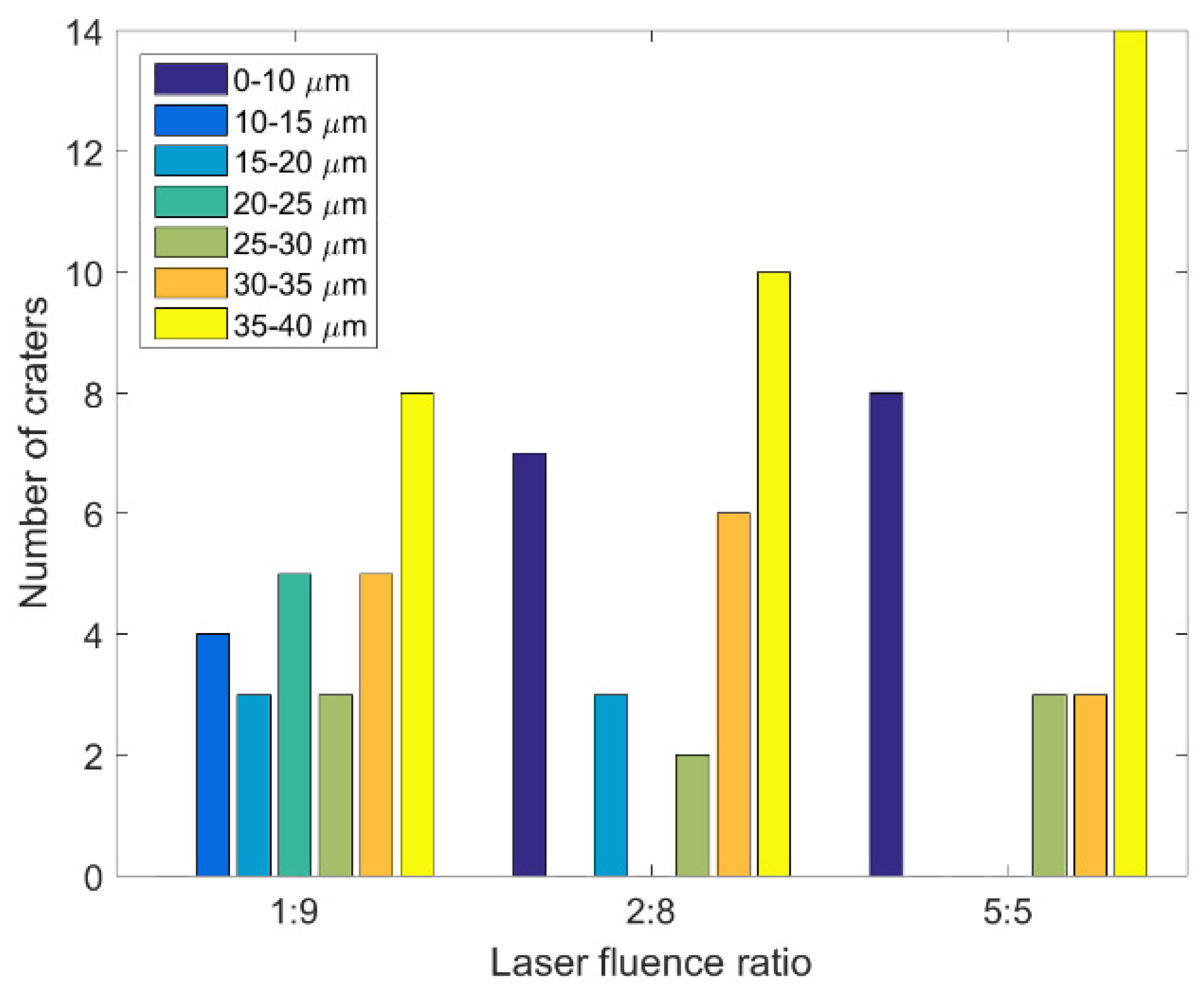
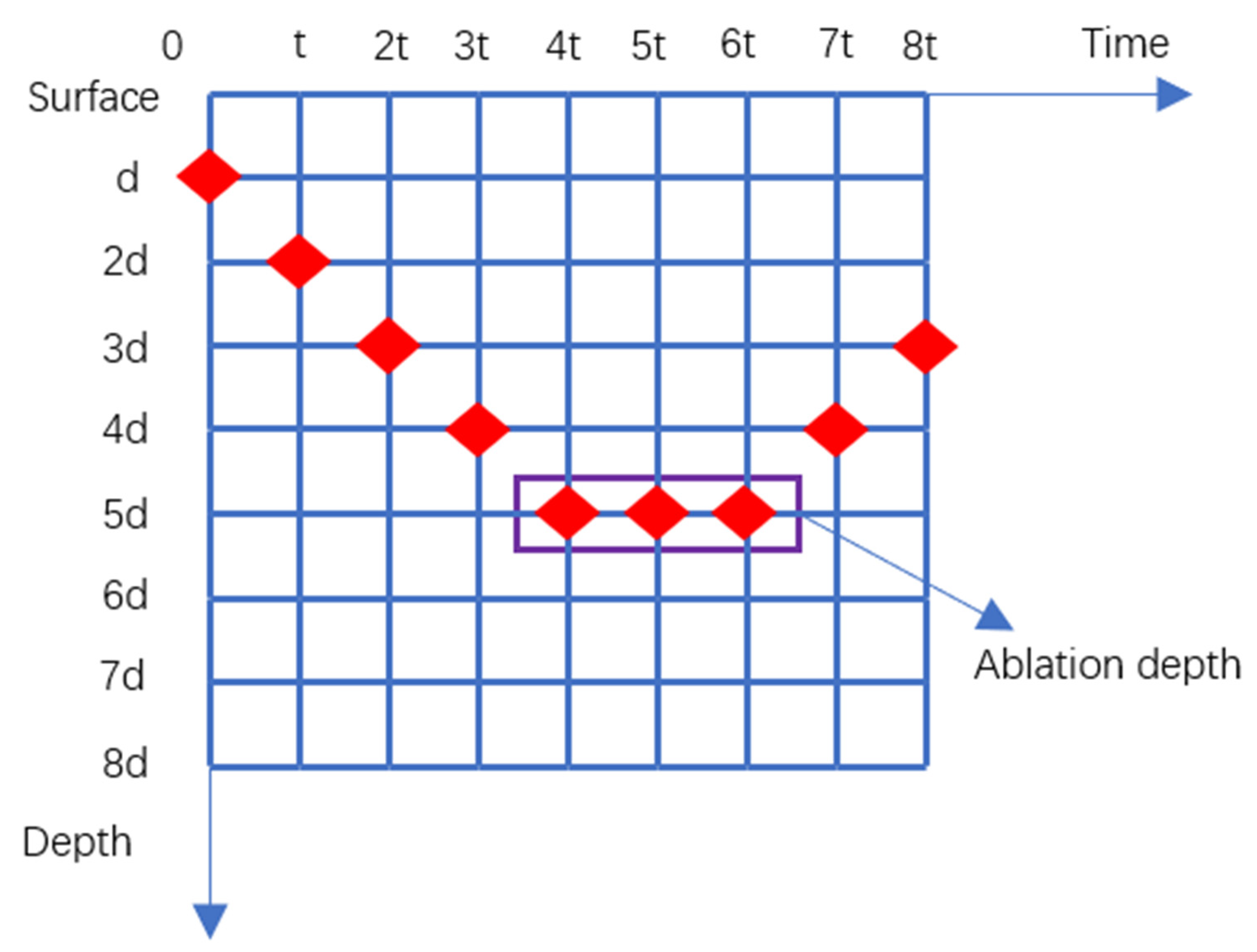
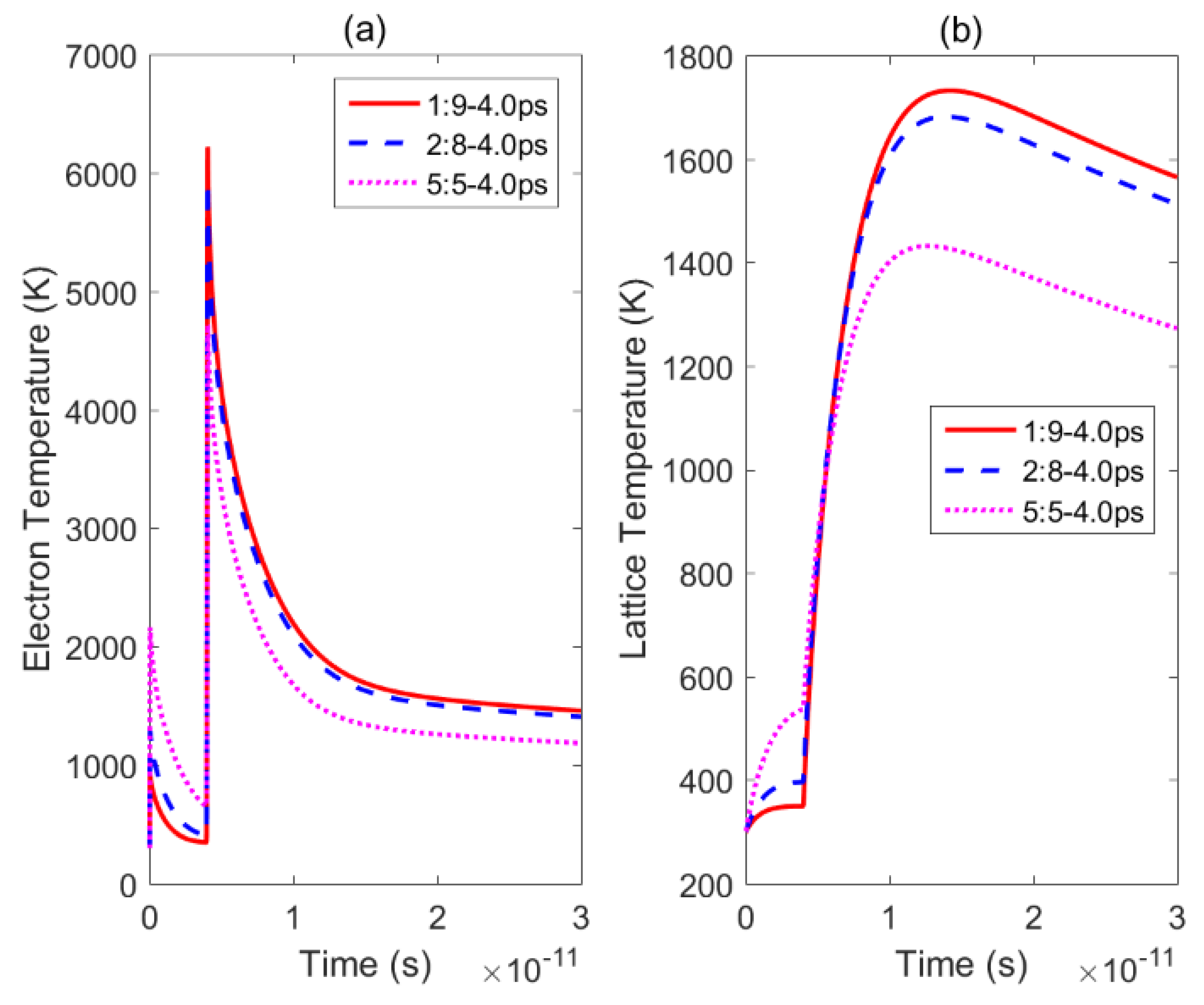
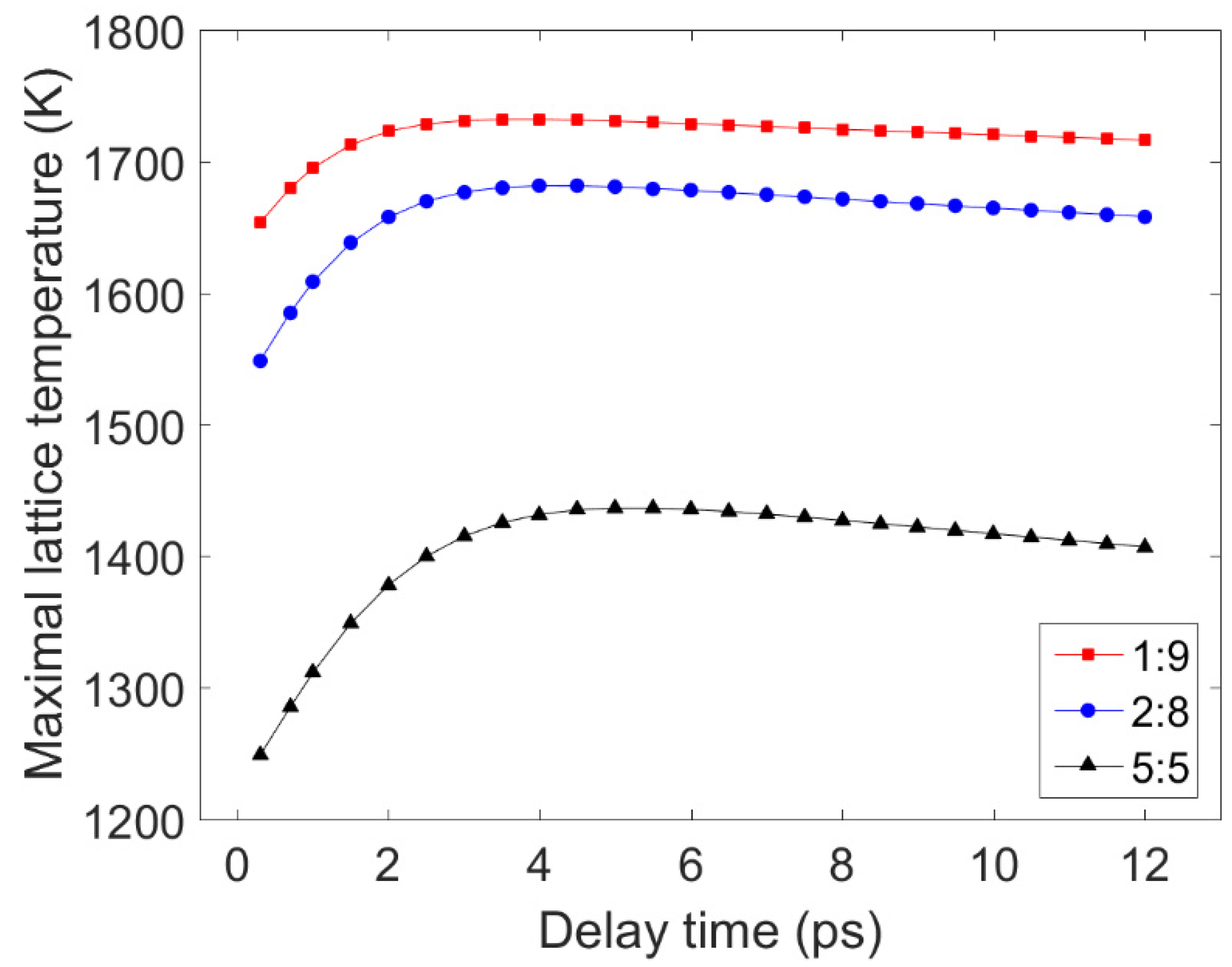
| Diameter | 1:9 | 2:8 | 5:5 |
|---|---|---|---|
| 0~10 | 0 | 25.0% | 28.6% |
| 10~15 | 14.2% | 0 | 0 |
| 15~20 | 10.7% | 10.7% | 0 |
| 20~25 | 17.9% | 0 | 0 |
| 25~30 | 10.7% | 7.2% | 10.7% |
| 30~35 | 17.9% | 21.4% | 10.7% |
| 35~40 | 28.6% | 35.7% | 50.0% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chu, K.; Guo, B.; Jiang, L.; Hua, Y.; Gao, S.; Jia, J.; Zhan, N. Throughput Improvement in Femtosecond Laser Ablation of Nickel by Double Pulses. Materials 2021, 14, 6355. https://doi.org/10.3390/ma14216355
Chu K, Guo B, Jiang L, Hua Y, Gao S, Jia J, Zhan N. Throughput Improvement in Femtosecond Laser Ablation of Nickel by Double Pulses. Materials. 2021; 14(21):6355. https://doi.org/10.3390/ma14216355
Chicago/Turabian StyleChu, Kunpeng, Baoshan Guo, Lan Jiang, Yanhong Hua, Shuai Gao, Jingang Jia, and Ningwei Zhan. 2021. "Throughput Improvement in Femtosecond Laser Ablation of Nickel by Double Pulses" Materials 14, no. 21: 6355. https://doi.org/10.3390/ma14216355
APA StyleChu, K., Guo, B., Jiang, L., Hua, Y., Gao, S., Jia, J., & Zhan, N. (2021). Throughput Improvement in Femtosecond Laser Ablation of Nickel by Double Pulses. Materials, 14(21), 6355. https://doi.org/10.3390/ma14216355






