Estimation of the Plastic Zone in Fatigue via Micro-Indentation
Abstract
:1. Introduction
2. Materials and Methods
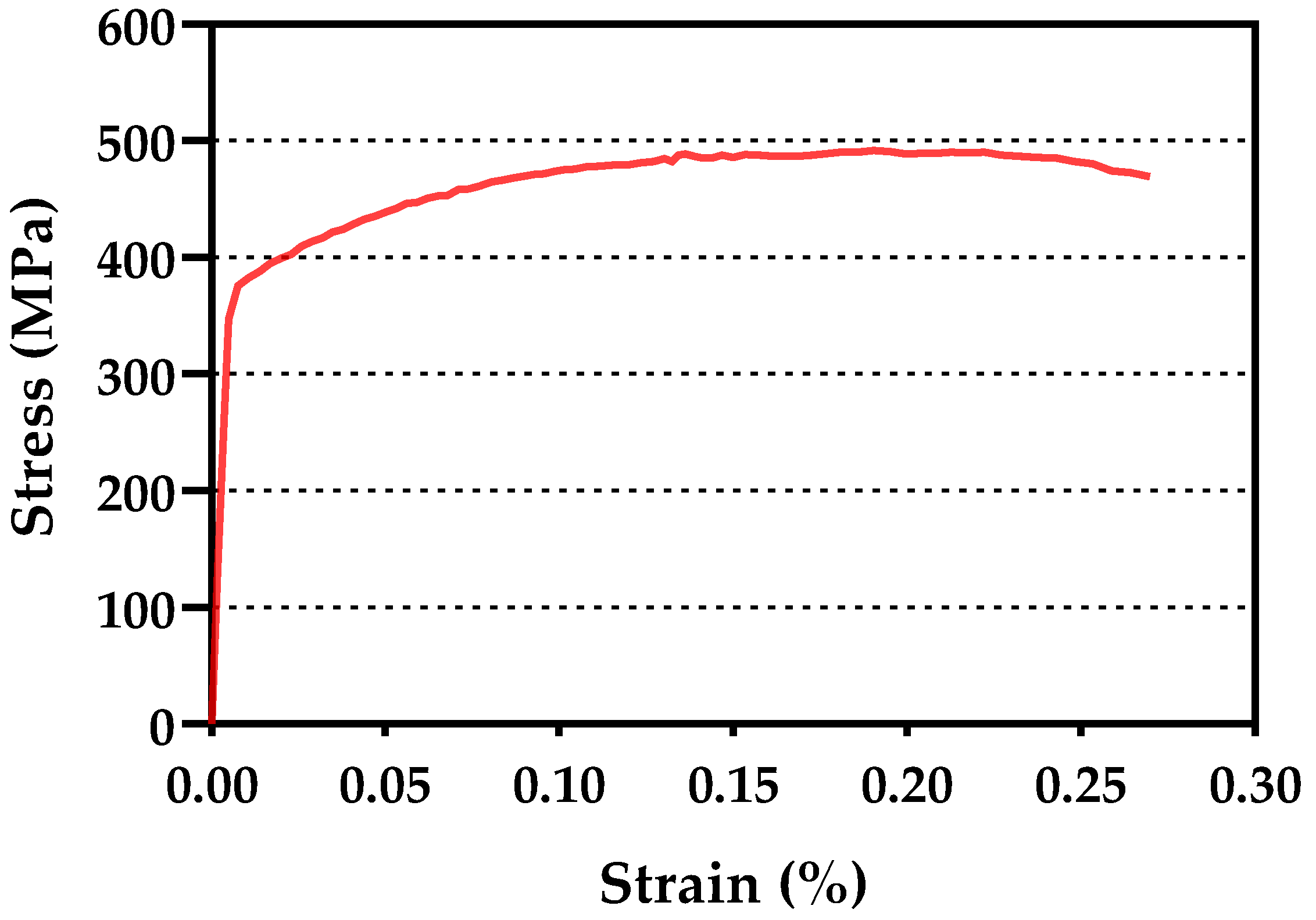
2.1. Aluminium Alloy 2024-T351
2.2. Fatigue Experiment
3. Micro-Indentation Technique
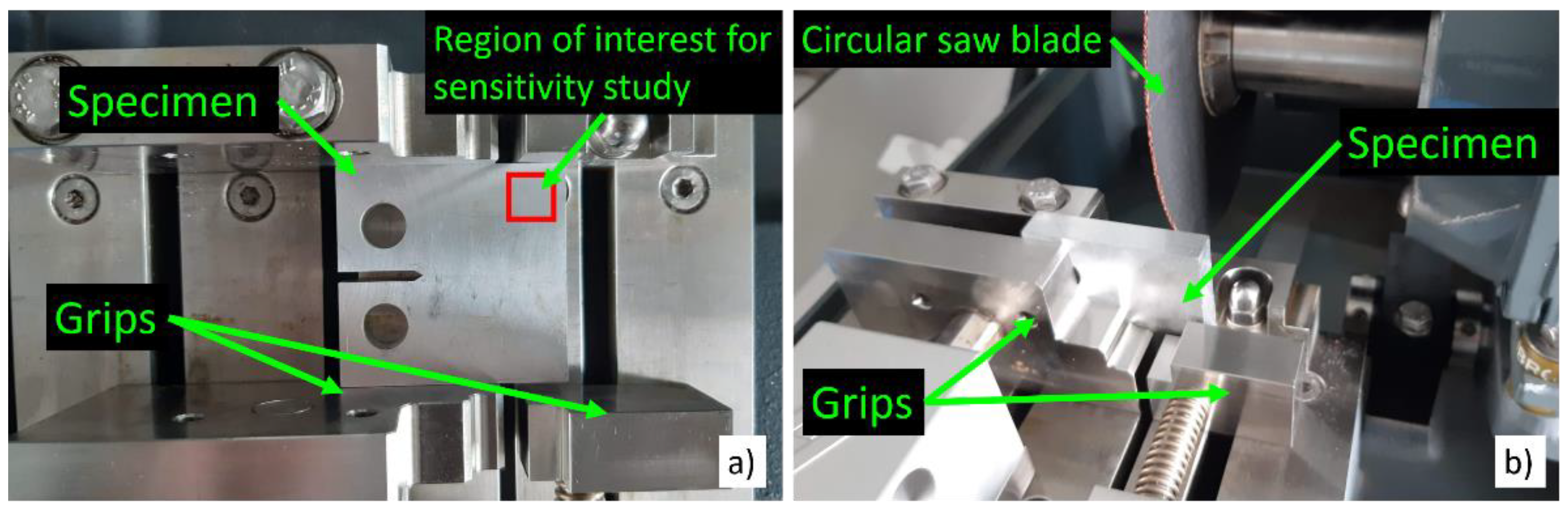
3.1. Description of the Micro-Indentation Experimental Procedure
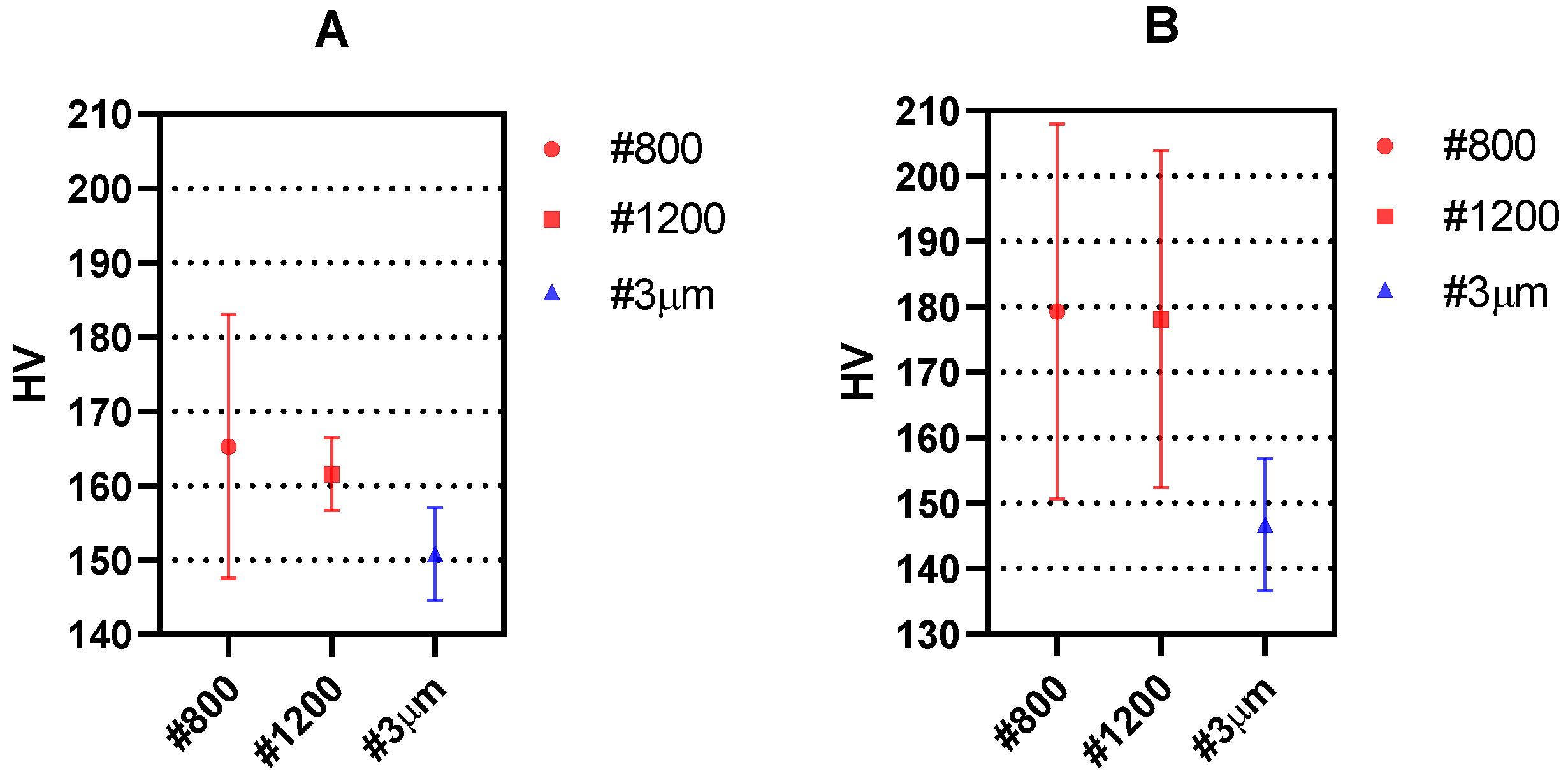
3.2. Sensitivity Analysis
4. Results and Discussion
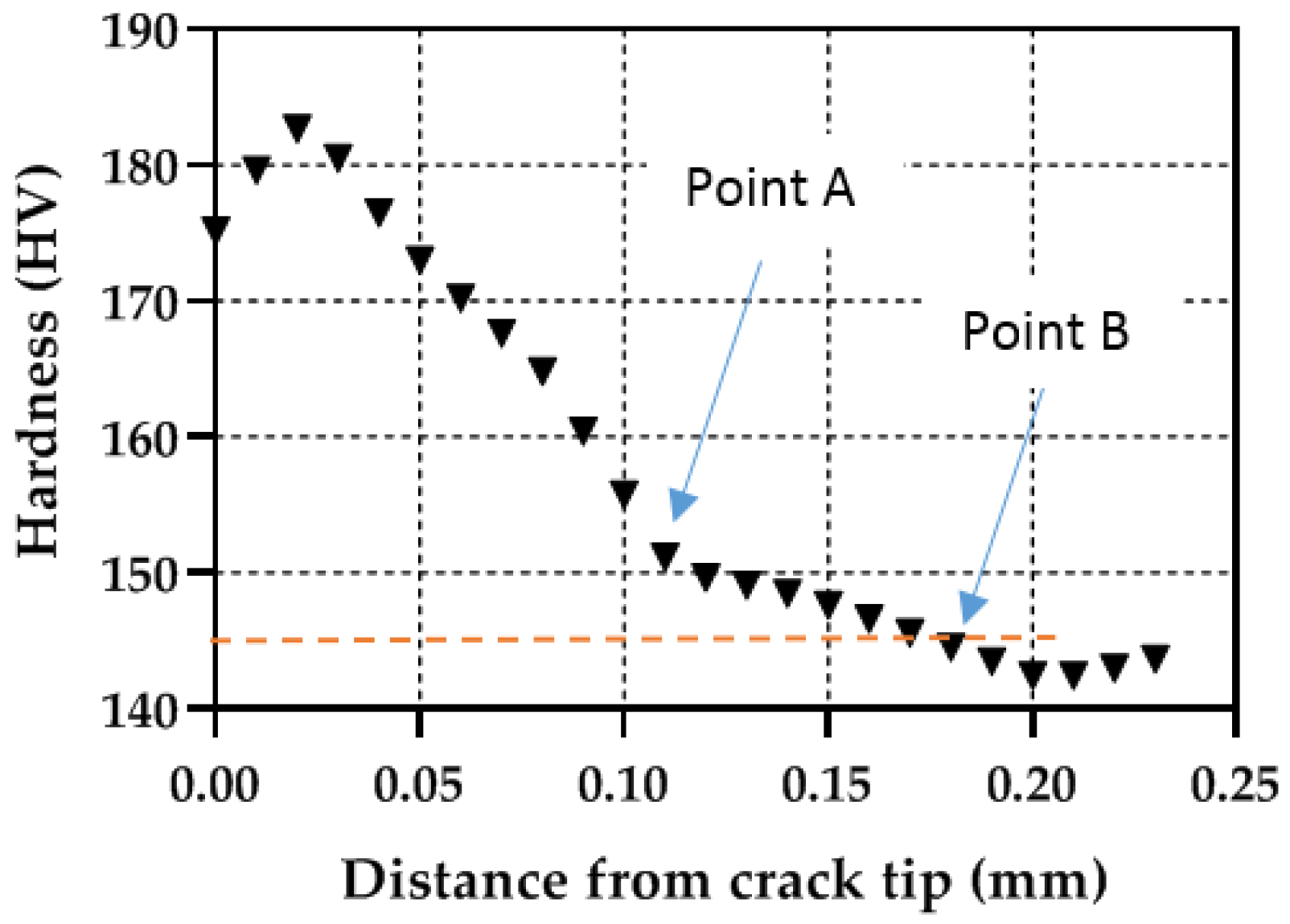
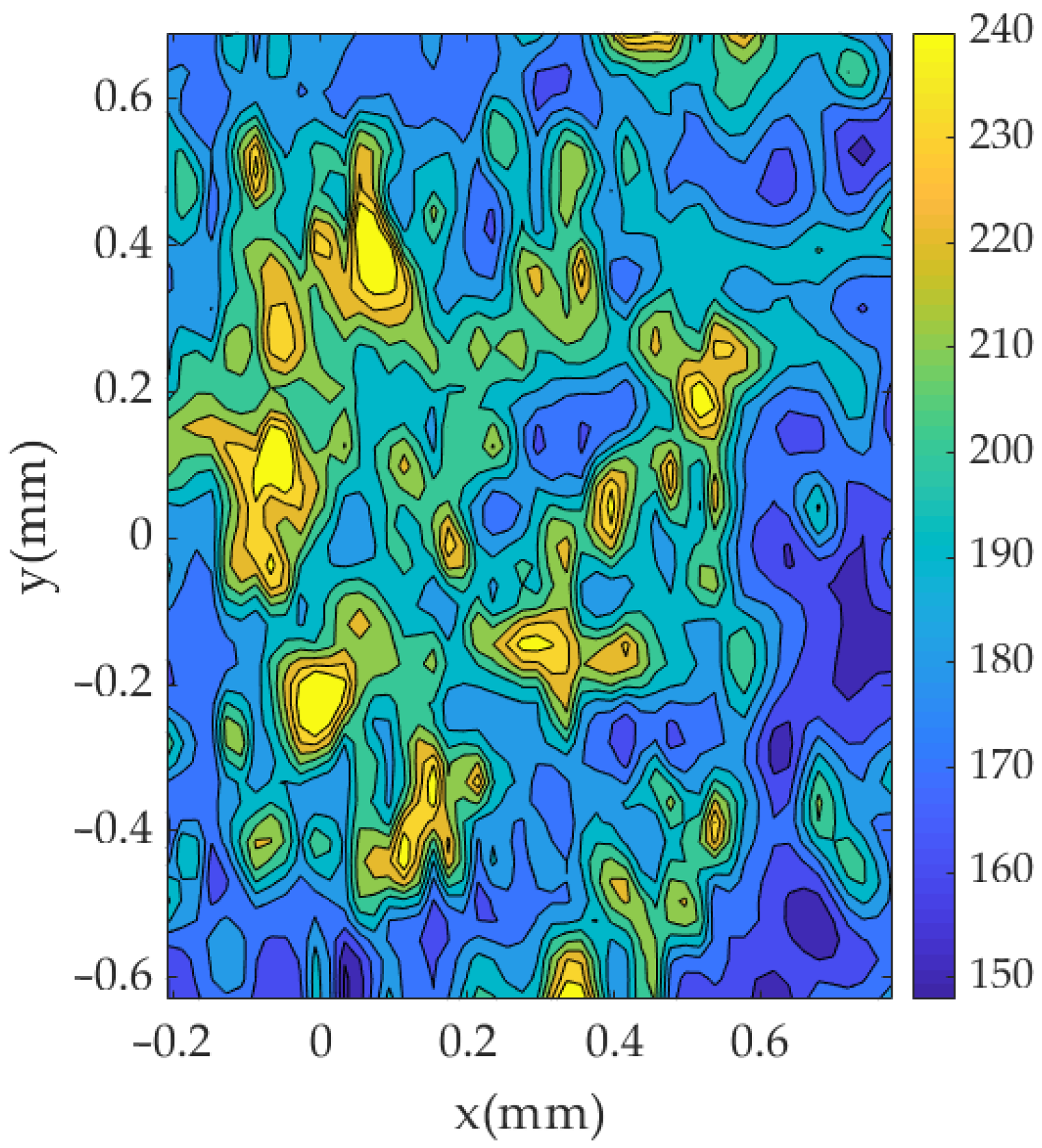
4.1. Plastic Zone Estimation
4.2. Preliminary Analysis on S355 Steel
5. Conclusions and Future Research
- The effect of the microstructure has been identified as the main cause of noise for the results. The fact that the size of the indentations is very similar to the hard precipitates of this alloy most likely decreases the quality of the results.
- The results and the sensitivity analysis indicate that for the current alloy, the best results are obtained by achieving a #3 μm surface finish, using 25 gf load for 15 s. Those parameters provide a good trade-off in terms of resolution and quality of each micro-indentation to produce a good enough map that can be used for quantitative analysis of plastic zones.
- The results have also shown that the shape and size can be approximately estimated from the 2D contour maps, as compared to Westergaard’s estimation of the plastic zone. The hardness profiles along the crack growing direction have allowed acceptable estimations of the plastic zone to be made, both for the monotonic and the cyclic plastic zones. For the cases studied, the estimations of the monotonic plastic zones have overall been better than those of the cyclic plastic zone.
- The results indicate that 0.2 mm appears to be the approximate size of the plastic zone that this methodology captures reliably. Plastic zones well below this figure can be spotted but visualisation of size and shape can be difficult.
- Based on previous works, a new criterion is proposed to separate the elastic from the plastic contribution from the micro-hardness measurements which enables us to retrieve the monotonic and the cyclic plastic zone. The current procedure for inferring the plastic zone around a fatigue crack tip is based on the hardening of the material being tested. Accordingly, applying this procedure to materials experiencing low strain hardening will yield less accurate results.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Banerjee, S. Influence of specimen size and configuration on the plastic zone size, toughness and crack growth. Engineering Fract. Mech. 1981, 15, 343–390. [Google Scholar] [CrossRef]
- Davidson, D.L.; Lankford, J. Fatigue crack tip plastic tip strain in high-strength aluminum alloys. Fatigue Fract. Eng. Mater. Struct. 1980, 3, 289–303. [Google Scholar] [CrossRef]
- ASTM International. Fracture Toughness Testing and its Applications; ASTM International: West Conshohocken, PA, USA, 1965. [Google Scholar]
- Lu, L.; Wang, S. Relationship between crack growth resistance curves and critical CTOA. Eng. Fract. Mech. 2017, 173, 146–156. [Google Scholar] [CrossRef]
- Dougherty, J.D.; Srivatsan, T.S.; Padovan, J. Fatigue crack propagation and closure behavior of modified 1070 steel: Experimental results. Eng. Fract. Mech. 1997, 56, 189–212. [Google Scholar] [CrossRef]
- Branco, R.; Antunes, F.V.; Costa, J.D. A review on 3D-FE adaptive remeshing techniques for crack growth modelling. Eng. Fract. Mech. 2015, 141, 170–195. [Google Scholar] [CrossRef]
- Lopez-Crespo, P.; Camas, D.; Antunes, F.V.; Yates, J.R. A study of the evolution of crack tip plasticity along a crack front. Theor. Appl. Fract. Mech. 2018, 98, 59–66. [Google Scholar] [CrossRef]
- Sukumar, N.; Moës, N.; Moran, B.; Belytschko, T. Extended finite element method for three-dimensional crack modelling. Int. J. Numer. Methods Eng. 2000, 48, 1549–1570. [Google Scholar] [CrossRef]
- Bergara, A.; Dorado, J.I.; Martin-Meizoso, A.; Martínez-Esnaola, J.M. Fatigue crack propagation in complex stress fields: Experiments and numerical simulations using the Extended Finite Element Method (XFEM). Int. J. Fatigue 2017, 103, 112–121. [Google Scholar] [CrossRef]
- Cheong, K.-S.; Smillie, M.J.; Knowles, D.M. Predicting fatigue crack initiation through image-based micromechanical modeling. Acta Mater. 2007, 55, 1757–1768. [Google Scholar] [CrossRef]
- McDowell, D.L.; Dunne, F.P.E. Microstructure-sensitive computational modeling of fatigue crack formation. Int. J. Fatigue 2010, 32, 1521–1542. [Google Scholar] [CrossRef]
- Paris, P.C.; Gomez, C.; Anderson, W.P. A rational analytic theory of fatigue. Trend Eng. 1961, 13, 9–14. [Google Scholar]
- Walker, K. The effect of stress ratio during crack propagation and fatigue for 2024-T3 and 7075-T6 aluminum. In Effects of Environment and Complex Load History for Fatigue Life, STP 462; American Society for Testing and Materials: Philadelphia, PA, USA, 1970; pp. 1–14. [Google Scholar]
- Forman, R.G.; Kearney, V.E.; Engle, R.M. Numerical analysis of crack propagation in cyclic-loaded structures. J. Basic Eng. 1967, 89, 459–464. [Google Scholar] [CrossRef]
- Rice, J.R. A Path Independent Integral and the Approximate Analysis of Strain Concentration by Notches and Cracks. J. Appl. Mech. 1968, 35, 379. [Google Scholar] [CrossRef] [Green Version]
- Dowd, O.N.; Shih, C.F. Two-Parameter Fracture Mechanics: Theory and Applications. In Fracture Mechanics. ASTM STP 1207, 24; American Society for Testing and Materials: West Conshohocken, PA, USA, 1994; pp. 21–47. [Google Scholar]
- Chao, Y.J.; Yang, S.; Sutton, M.A. On the fracture of solids characterized by one or two parameters: Theory and practice. J. Mech. Phys. Solids 1994, 42, 629–647. [Google Scholar] [CrossRef]
- Zhu, X.-K.; Leis, B.N. Bending modified J–Q theory and crack-tip constraint quantification. Int. J. Fract. 2006, 141, 115–134. [Google Scholar] [CrossRef]
- Nyström, M.; Soderlund, E.; Karlsson, B. Plastic zones around fatigue cracks studied by ultra-low-load indentation technique. Int. J. Fatigue 1995, 17, 141–147. [Google Scholar] [CrossRef]
- Ghodrat, S.; Riemslag, A.C.; Kestens, L.A.I. Measuring Plasticity with Orientation Contrast Microscopy in Aluminium 6061-T4. Metals 2017, 7, 108. [Google Scholar] [CrossRef] [Green Version]
- Pineau, A.G.; Pelloux, R.M. Influence of Strain-Induced Martensitic Transformations on Fatigue Crack Growth Rates in Stainless Steels. Metall. Trans. 1974, 5, 1103–1112. [Google Scholar] [CrossRef]
- Kwun, S.I.; Park, S.H. Plastic zone size measurement by critical grain growth method. Scr. Metall. 1987, 21, 797–800. [Google Scholar] [CrossRef]
- Maeng, M.-Y.; Kim, M.-H. Comparative study on the fatigue crack growth behavior of 316L and 316LN stainless steels: Effect of microstructure of cyclic plastic strain zone at crack tip. J. Nucl. Mater. 2000, 282, 32–39. [Google Scholar] [CrossRef]
- Kudari, S.K.; Maiti, B.; Ray, K.K. Experimental investigation on possible dependence of plastic zone size on specimen geometry. Frattura ed Integrita Strutturale2 2009, 7, 57–64. [Google Scholar] [CrossRef] [Green Version]
- Do, T.-D.; Chalon, F.; Duong, P.-T.-M. Determination of the Plastic Zone Size by Using Nanotest for Aluminum Alloy 2024T351: Proceedings of the International Conference, ICERA 2018. In Advances in Engineering Research and Application; Lecture Notes in Networks and Systems Series; Springer: Berlin/Heidelberg, Germany, 2018; pp. 236–245. [Google Scholar]
- Gwider, A.; Ranganathan, N. Plastic Zones at a Fatigue Crack Tip. J. Fail. Anal. Prev. 2019, 19, 673–681. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Subhash, G.; Arakere, N. Evolution of subsurface plastic zone due to rolling contact fatigue of M-50 NiL case hardened bearing steel. Int. J. Fatigue 2014, 59, 102–113. [Google Scholar] [CrossRef]
- ASTM International. ASTM E112-10, Standard Test Methods for Determining Average Grain Size; ASTM International: West Conshohocken, PA, USA, 2010; pp. 1–27. [Google Scholar]
- ASTM International. ASTM E647, Standard Test Method for Measurement of Fatigue Crack Growth Rates; ASTM International: West Conshohocken, PA, USA, 2001. [Google Scholar]
- ASTM International. ASTM E384-08, Microindentation Hardness of Materials; ASTM International: West Conshohocken, PA, USA, 2008; pp. 1–24. [Google Scholar]
- Image J. Available online: https://imagej.nih.gov/ij/ (accessed on 2 May 2020).
- Gonzalez, R.C.; Woods, R.E. Digital Image Processing; Pearson Prentice Hall: Hoboken, NJ, USA, 2008. [Google Scholar]
- Vasco-Olmo, J.M.; James, M.N.; Christopher, C.J.; Patterson, E.A.; Díaz, F.A. Assessment of crack tip plastic zone size and shape and its influence on crack tip shielding. Fatigue Fract. Eng. Mater. Struct. 2016, 39, 969–989. [Google Scholar] [CrossRef] [Green Version]
- Westergaard, H.M. Bearing pressures and cracks. J. Appl. Mech. 1939, 61, A49–A53. [Google Scholar] [CrossRef]
- Broek, D. Elementary Engineering Fracture Mechanics, 4th ed.; Kluwer Academic Publishers: Boston, MA, USA, 1986. [Google Scholar]
- Janssen, M.; Zuidema, J.; Wanhill, R.J.H. Fracture Mechanics, 2nd ed.; Spon Press: Abingdon, UK, 2006. [Google Scholar]
- Suresh, S. Fatigue of Materials, 2nd ed.; Cambridge University Press: Cambridge, UK, 1998. [Google Scholar]
- Purcell, A.H.; Weertman, J. Crack Tip Area in Fatigued Copper Single Crystals. Metall. Trans. 1974, 5, 1805–1809. [Google Scholar] [CrossRef]
- Suresh, S.; Ritchie, R.O. Propagation of short fatigue cracks. Int. Mater. Rev. 1984, 29, 445–475. [Google Scholar] [CrossRef]
- Miller, K.J. Materials science perspective of metal fatigue resistance. Mater. Sci. Technol. 1993, 9, 453–462. [Google Scholar] [CrossRef]
- Dowling, N.E. Mechanical Behavior of Materials. Engineering Methods for Deformation, Fracture, and Fatigue, 4th ed.; Pearson Education Limited: London, UK, 2012. [Google Scholar]
- Shenoy, M.; Zhang, J.; McDowell, D.L. Estimating fatigue sensitivity to polycrystalline Ni-base superalloy microstructures using a computational approach. Fatigue Fract. Eng. Mater. Struct. 2007, 30, 889–904. [Google Scholar] [CrossRef]







| Mn | Si | Cr | Cu | Zn | Pb | Fe | Ti | Mg |
|---|---|---|---|---|---|---|---|---|
| 0.650 | 0.070 | 0.010 | 4.570 | 0.060 | 0.004 | 0.120 | 0.039 | 1.500 |
| Yield Stress, σy | 375 MPa |
| Ultimate Tensile Stress, σu | 492 MPa |
| Young’s Modulus, E | 73 GPa |
| Specimen ID | a, mm | ΔKI, MPa√m |
|---|---|---|
| P1 | 30.40 | 9.91 |
| P2 | 37.61 | 20.61 |
| P3 | 40.25 | 30.23 |
| Sample | Type of PZ | PZexp (mm) | PZtheo (mm) | Error (%) | Difference (mm) |
|---|---|---|---|---|---|
| P1 | Cyclic | 0.11 | 0.04 | 175 | 0.07 |
| Mono | 0.18 | 0.20 | −10.0 | −0.02 | |
| P2 | Cyclic | 0.21 | 0.15 | 40.0 | 0.06 |
| Mono | 0.69 | 0.75 | −8.00 | −0.06 | |
| P3 | Cyclic | 0.35 | 0.34 | 2.94 | 0.01 |
| Mono | 1.78 | 1.64 | 8.54 | 0.14 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lopez-Crespo, C.; Cruces, A.S.; Seitl, S.; Moreno, B.; Lopez-Crespo, P. Estimation of the Plastic Zone in Fatigue via Micro-Indentation. Materials 2021, 14, 5885. https://doi.org/10.3390/ma14195885
Lopez-Crespo C, Cruces AS, Seitl S, Moreno B, Lopez-Crespo P. Estimation of the Plastic Zone in Fatigue via Micro-Indentation. Materials. 2021; 14(19):5885. https://doi.org/10.3390/ma14195885
Chicago/Turabian StyleLopez-Crespo, Cristina, Alejandro S. Cruces, Stanislav Seitl, Belen Moreno, and Pablo Lopez-Crespo. 2021. "Estimation of the Plastic Zone in Fatigue via Micro-Indentation" Materials 14, no. 19: 5885. https://doi.org/10.3390/ma14195885
APA StyleLopez-Crespo, C., Cruces, A. S., Seitl, S., Moreno, B., & Lopez-Crespo, P. (2021). Estimation of the Plastic Zone in Fatigue via Micro-Indentation. Materials, 14(19), 5885. https://doi.org/10.3390/ma14195885






