Analytical and Numerical Modelling of Creep Deformation of Viscoelastic Thick-Walled Cylinder with Fractional Maxwell Model
Abstract
:1. Introduction
2. Materials
2.1. Constitutive Equations
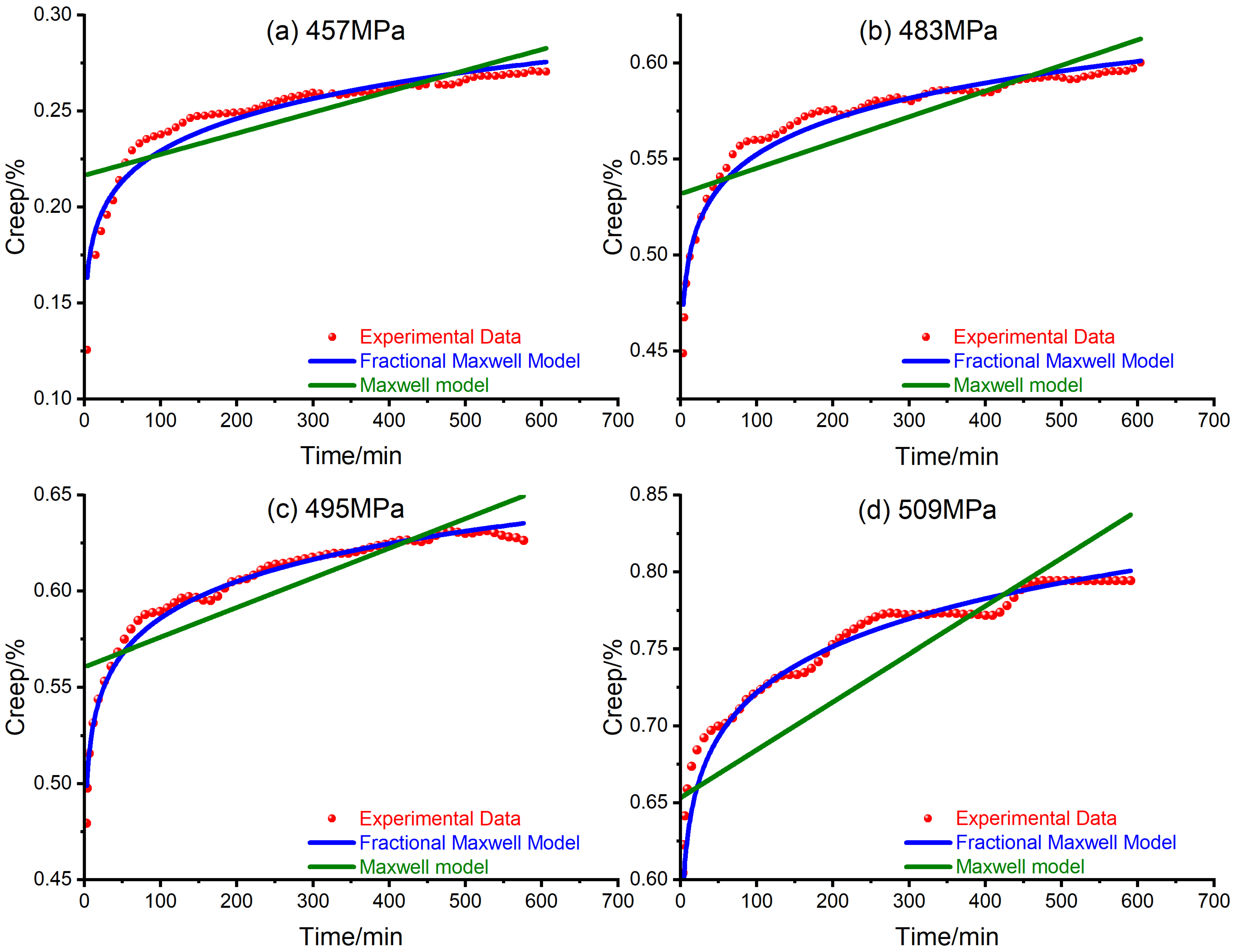
2.2. Fitting with Fractional Maxwell Model
3. Methods
4. Results
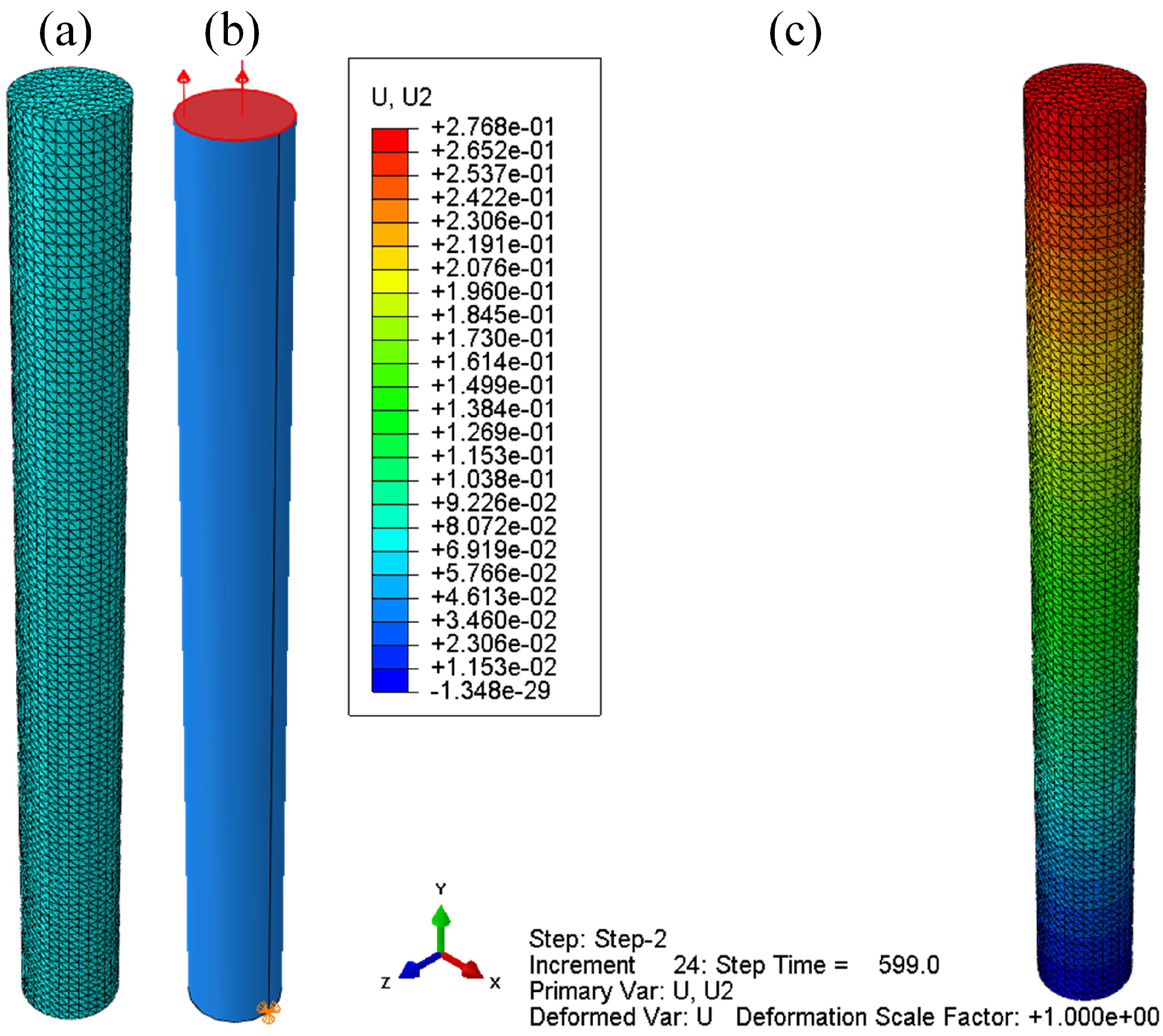
4.1. Numerical Implementation of Fractional Maxwell Model
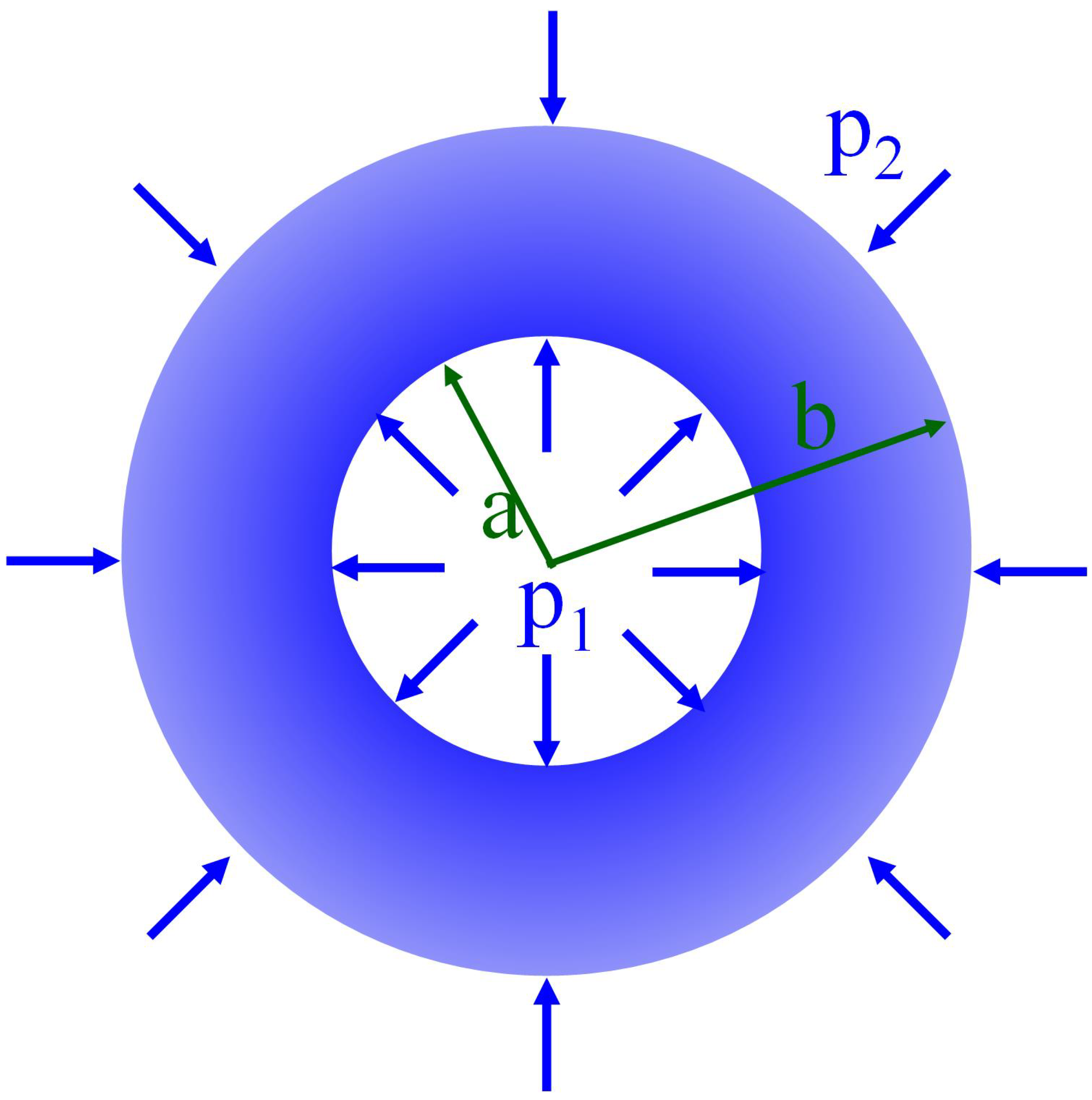
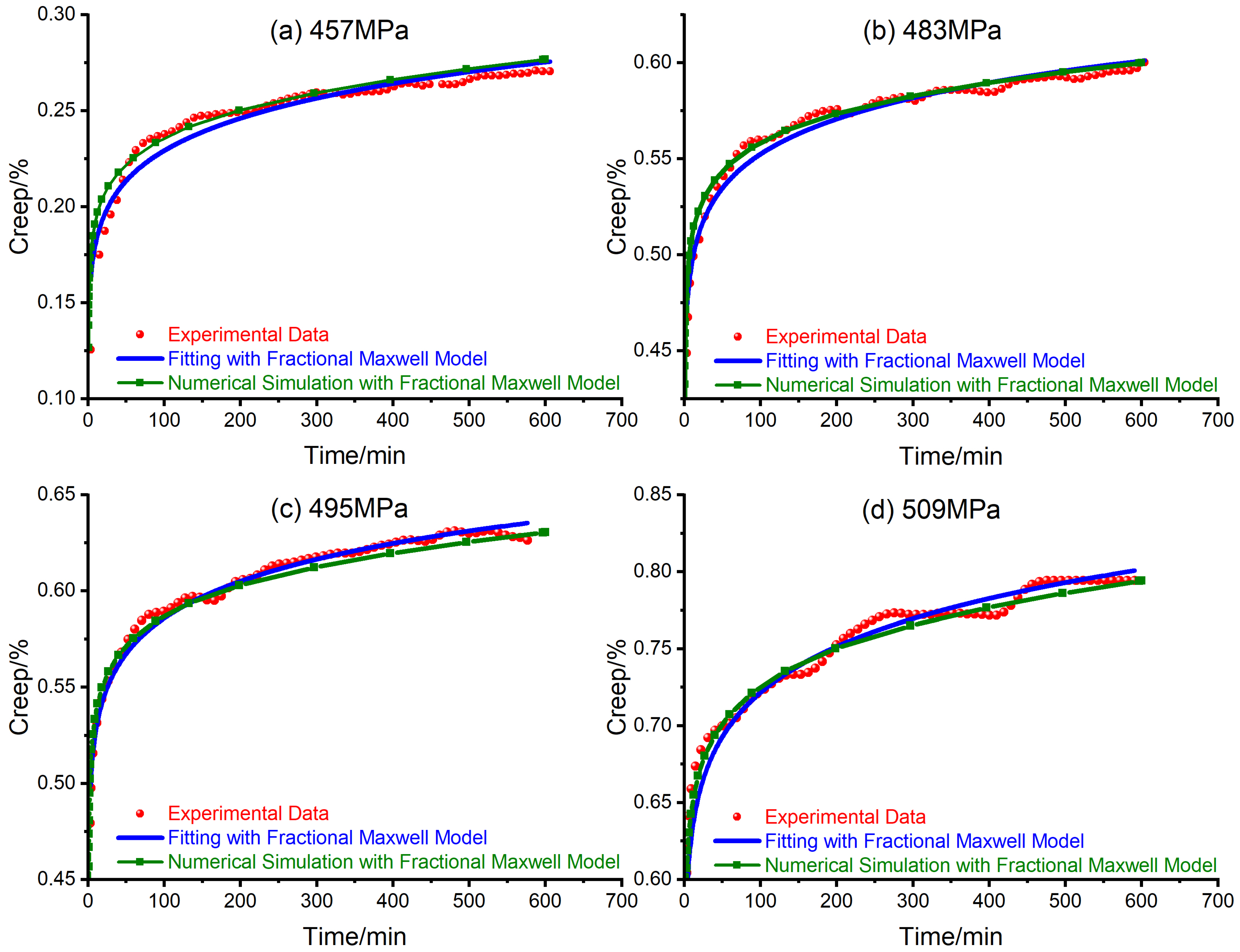
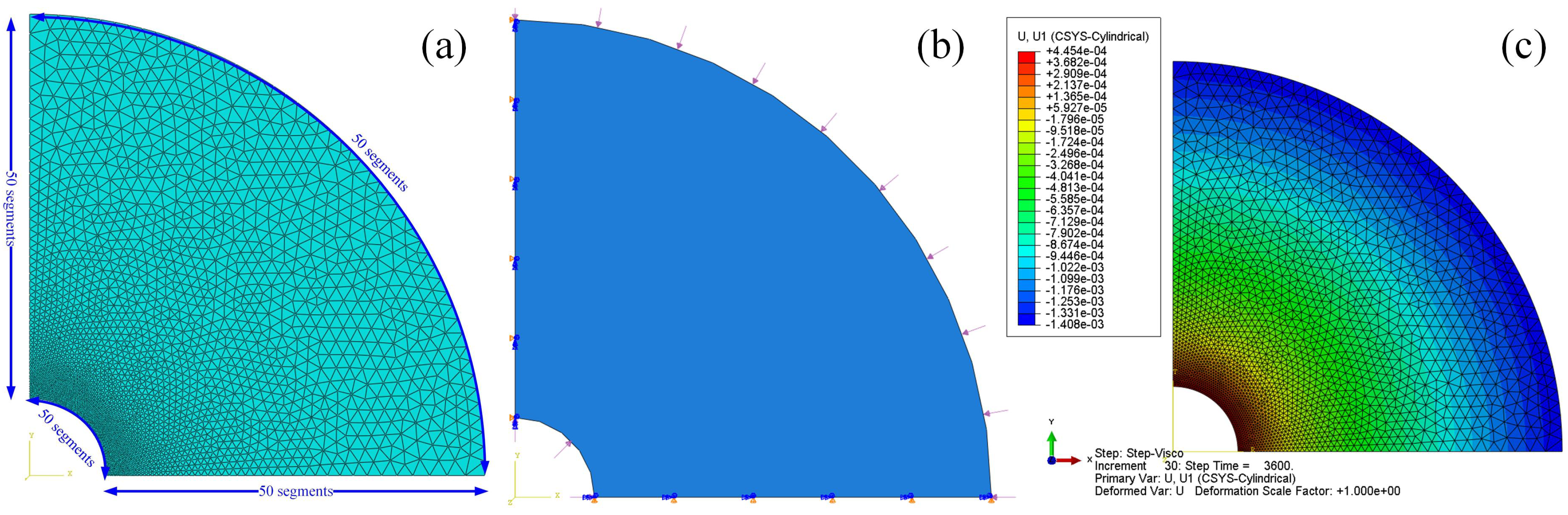
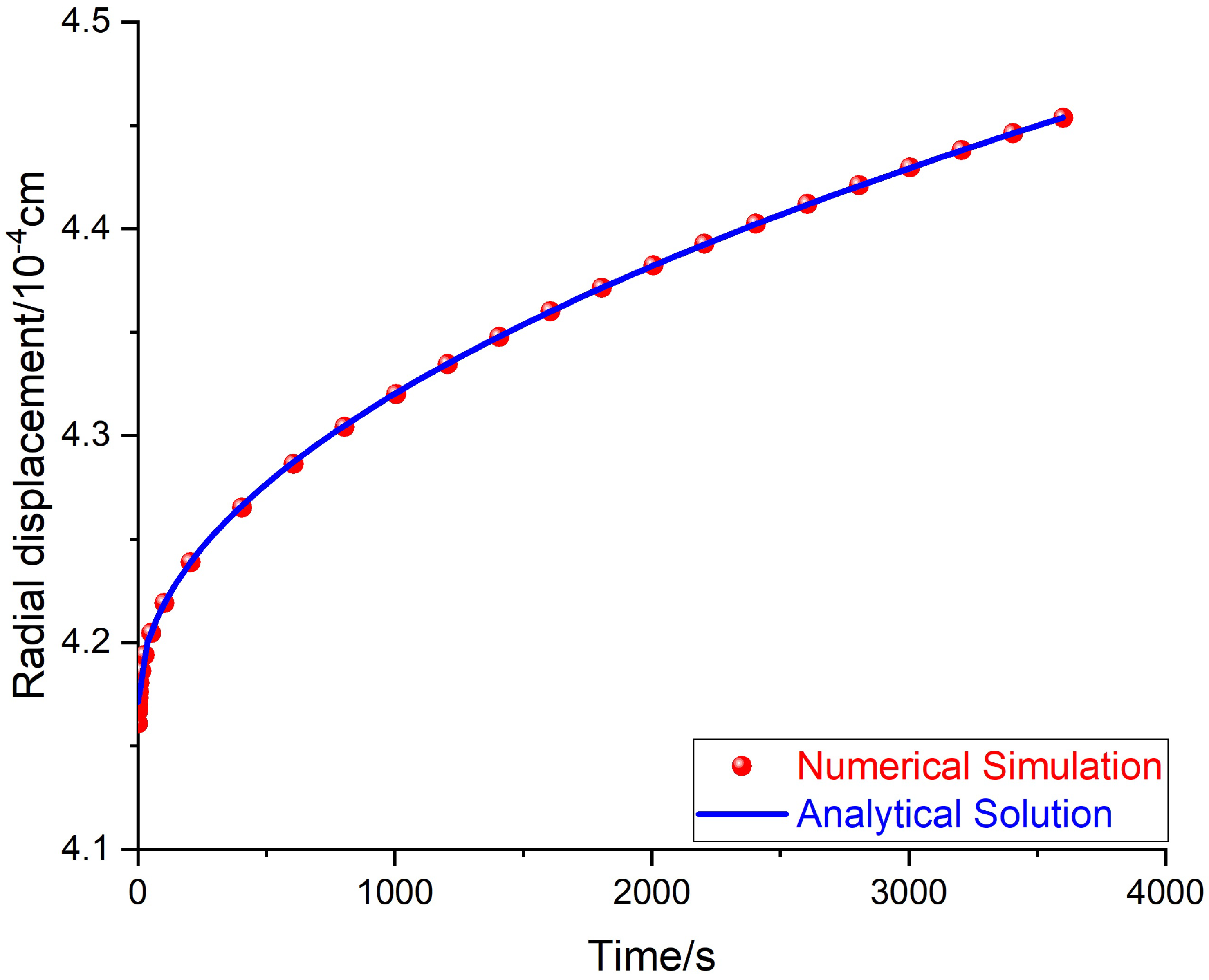
4.2. Numerical Simulation of Creep Deformation of Thick-Walled Cylinder under Internal Pressure
5. Discussion
6. Conclusions
- Compared to the traditional integer Maxwell model, the fractional Maxwell model is an efficient nonlinear model to capture the essence of creep deformation of steels both in the transient and the steady-state stages.
- In analogy with the analytical models based on traditional integer creep models, the analytical solutions based on fractional models can also be established with the help of the elastic–viscoelastic correspondence principle.
- The implementation of the fractional Maxwell model with the help of the second development function enriches the material libraries of the general-purpose finite element software and enables the capability of finite element software to conduct more realistic numerical simulations.
- The numerical simulation results validate the creep displacement of a thick-walled cylinder under constant internal pressure predicted by the analytical solution based on the fractional Maxwell model. Hence, the analytical solution of creep displacement of a thick-walled cylinder under constant internal pressure based on the fractional Maxwell model can be used with confidence to predict the time-dependent deformations.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Abdalla, H.M.A.; Casagrande, D.; Bona, F.D. A Dynamic Programming Setting for Functionally Graded Thick-Walled Cylinders. Materials 2020, 13, 3988. [Google Scholar] [CrossRef] [PubMed]
- Rouse, J.; Hyde, C. A Comparison of Simple Methods to Incorporate Material Temperature Dependency in the Green’s Function Method for Estimating Transient Thermal Stresses in Thick-Walled Power Plant Components. Materials 2016, 9, 26. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shinozuka, M. Stresses in an incompressible viscoelastic- plastic thick-walled cylinder. AIAA J. 1964, 2, 1800–1804. [Google Scholar] [CrossRef]
- Attia, Y.; Fitzgeorge, D.; Pope, J. An experimental investigation of residual stresses in hollow cylinders due to the creep produced by thermal stresses. J. Mech. Phys. Solids 1954, 2, 238–258. [Google Scholar] [CrossRef]
- Schwiebert, P. Elastic, plastic and creep deformations in long, thick-walled cylinders of workhardening material subjected to transient thermal and mechanical loading. Int. J. Mech. Sci. 1965, 7, 115–125. [Google Scholar] [CrossRef]
- Pai, D. Steady-state creep analysis of thick-walled orthotropic cylinders. Int. J. Mech. Sci. 1967, 9, 335–348. [Google Scholar] [CrossRef]
- Sim, R.; Penny, R. Plane strain creep behaviour of thick-walled cylinders. Int. J. Mech. Sci. 1971, 13, 987–1009. [Google Scholar] [CrossRef]
- Bhatnagar, N.; Arya, V. Large strain creep analysis of thick-walled cylinders. Int. J. Non-Linear Mech. 1974, 9, 127–140. [Google Scholar] [CrossRef]
- Bhatnagar, N.; Kulkarni, P.; Arya, V. Creep analysis of an internally pressurised orthotropic rotating cylinder. Nucl. Eng. Des. 1984, 83, 379–388. [Google Scholar] [CrossRef]
- Bhatnagar, N.; Kulkarni, P.; Arya, V. Creep analysis of orthotropic rotating cylinders considering finite strains. Int. J. Non-Linear Mech. 1986, 21, 61–71. [Google Scholar] [CrossRef]
- Bhatnagar, N.; Kulkarni, P.; Arya, V. Analysis of an orthotropic thick-walled cylinder under primary creep conditions. Int. J. Press. Vessel. Pip. 1986, 23, 165–185. [Google Scholar] [CrossRef]
- Simonian, A. Calculation of thermal stresses in thick-walled cylinders taking account of non-linear creep. Int. J. Eng. Sci. 1979, 17, 513–519. [Google Scholar] [CrossRef]
- Brust, F.; Leis, B. A model for predicting primary creep damage in axial cracked cylinders—I. Theory. Eng. Fract. Mech. 1992, 43, 615–627. [Google Scholar] [CrossRef]
- Combescure, A. Simplified prediction of creep buckling of cylinders under external pressure. Part 1: Finite element validation. Eur. J. Mech. A/Solids 1998, 17, 1021–1036. [Google Scholar] [CrossRef]
- Gonçalves, J.O.F. A lower bound to the creep rupture time of pressurised thick cylinders. Int. J. Mech. Sci. 2004, 46, 527–539. [Google Scholar] [CrossRef]
- Debnath, L. A brief historical introduction to fractional calculus. Int. J. Math. Educ. Sci. Technol. 2004, 35, 487–501. [Google Scholar] [CrossRef]
- Machado, J.T.; Kiryakova, V.; Mainardi, F. Recent history of fractional calculus. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 1140–1153. [Google Scholar] [CrossRef] [Green Version]
- Mainardi, F. An historical perspective on fractional calculus in linear viscoelasticity. Fract. Calc. Appl. Anal. 2012, 15. [Google Scholar] [CrossRef] [Green Version]
- Müller, S.; Kästner, M.; Brummund, J.; Ulbricht, V. A nonlinear fractional viscoelastic material model for polymers. Comput. Mater. Sci. 2011, 50, 2938–2949. [Google Scholar] [CrossRef]
- Xu, Z.; Chen, W. A fractional-order model on new experiments of linear viscoelastic creep of Hami Melon. Comput. Math. Appl. 2013, 66, 677–681. [Google Scholar] [CrossRef]
- Qi, H.; Xu, M. Unsteady flow of viscoelastic fluid with fractional Maxwell model in a channel. Mech. Res. Commun. 2007, 34, 210–212. [Google Scholar] [CrossRef]
- Ding, X.; Zhang, G.; Zhao, B.; Wang, Y. Unexpected viscoelastic deformation of tight sandstone: Insights and predictions from the fractional Maxwell model. Sci. Rep. 2017, 7, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Ding, X.; Zhang, F.; Zhang, G.; Yang, L.; Shao, J. Modeling of hydraulic fracturing in viscoelastic formations with the fractional Maxwell model. Comput. Geotech. 2020, 126, 103723. [Google Scholar] [CrossRef]
- Ding, X.; Zhang, F.; Zhang, G. Modelling of time-dependent proppant embedment and its influence on tight gas production. J. Nat. Gas Sci. Eng. 2020, 82, 103519. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Calculus and Waves in Linear Viscoelasticity: An Introduction to Mathematical Models; World Scientific: Singapore, 2010. [Google Scholar]
- Diethelm, K. The Analysis of Fractional Differential Equations; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Pipkin, A.C. Lectures on Viscoelasticity Theory; Springer: New York, NY, USA, 1986. [Google Scholar]
- Mainardi, F.; Spada, G. Creep, relaxation and viscosity properties for basic fractional models in rheology. Eur. Phys. J. Spec. Top. 2011, 193, 133–160. [Google Scholar] [CrossRef] [Green Version]
- Carpinteri, A.; Mainardi, F. Fractals and Fractional Calculus in Continuum Mechanics; Springer: Vienna, Austria, 1997. [Google Scholar]
- Wang, W.; Yan, S.; Liu, J. Studies on temperature induced creep in high strength Q460 steel. Mater. Struct. 2016, 50. [Google Scholar] [CrossRef]
- Jaeger, J.C.; Cook, N.G.W.; Zimmerman, R. Fundamentals of Rock Mechanics, 4th ed.; Wiley-Blackwell: Oxford, UK, 2007. [Google Scholar]
- Abaqus. Abaqus User Subroutines Reference Manual, 6.14 ed.; Dassault Systèmes Simulia Corp: Providence, RI, USA, 2014. [Google Scholar]
- Christensen, R.M. Theory of Viscoelasticity: An Introduction, 2nd ed.; Dover Publications Inc.: New York, NY, USA, 1982. [Google Scholar]

| Stress/MPa | Creep Model | ||||
|---|---|---|---|---|---|
| Fractional Maxwell Model | Maxwell Model | ||||
| Pa | Pa | ||||
| 457 | 7.01834 | 3.41866 | 0.10518 | 2.12553 | 4.03905 |
| 483 | 1.10758 | 1.11339 | 0.0469802 | 0.908136 | 3.60626 |
| 495 | 0.704099 | 1.07122 | 0.0461791 | 0.882804 | 3.2086 |
| 509 | 1.40625 | 0.952868 | 0.0585737 | 0.779106 | 1.63443 |
| Loading Step | Time/s | Amplitude | Initial Step/s | Minimum Step/s | Maximum Step/s |
|---|---|---|---|---|---|
| Initial_Loading | 0 | 0 | / | / | / |
| Elastic_Loading | 1 | 1 | 10 | 10 | 0.1 |
| Viscous_Loading | 599 | 1 | 1 | 10 | 100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, X.; Chen, N.; Zhang, Y.; Zhang, F. Analytical and Numerical Modelling of Creep Deformation of Viscoelastic Thick-Walled Cylinder with Fractional Maxwell Model. Materials 2021, 14, 4849. https://doi.org/10.3390/ma14174849
Ding X, Chen N, Zhang Y, Zhang F. Analytical and Numerical Modelling of Creep Deformation of Viscoelastic Thick-Walled Cylinder with Fractional Maxwell Model. Materials. 2021; 14(17):4849. https://doi.org/10.3390/ma14174849
Chicago/Turabian StyleDing, Xiang, Na Chen, Yan Zhang, and Fan Zhang. 2021. "Analytical and Numerical Modelling of Creep Deformation of Viscoelastic Thick-Walled Cylinder with Fractional Maxwell Model" Materials 14, no. 17: 4849. https://doi.org/10.3390/ma14174849
APA StyleDing, X., Chen, N., Zhang, Y., & Zhang, F. (2021). Analytical and Numerical Modelling of Creep Deformation of Viscoelastic Thick-Walled Cylinder with Fractional Maxwell Model. Materials, 14(17), 4849. https://doi.org/10.3390/ma14174849








