Time-Resolved PIV Measurements and Turbulence Characteristics of Flow Inside an Open-Cell Metal Foam
Abstract
:1. Introduction
2. Materials and Methods
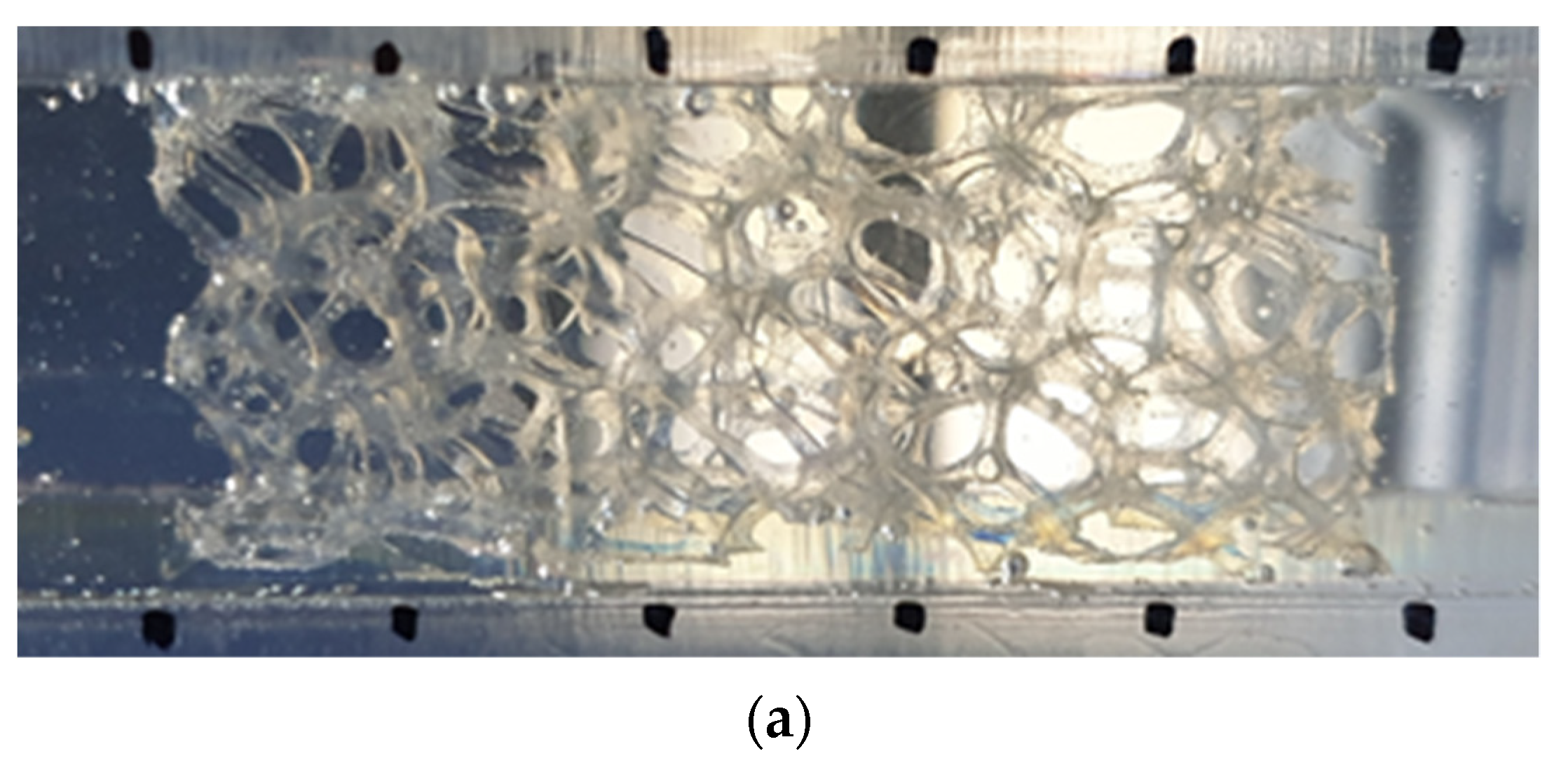
2.1. Fabrication and Refractive Index Matching of Transparent Metal Foam Replica
- The support material must be removed completely since it is not transparent. This was done using a water jet after soaking the model for half a day.
- Wet sanding must be done to obtain the best transparency. The sanding was started from a coarse sand to finer one. All the surfaces were sanded, and each time, the model was inspected under a light source to recognize the sandpaper size change where surface defects were no longer visible after last sanding pass. Each sanding step was done perpendicular to the previous one.
- The printed model was then placed in a water channel with water circulating at ambient temperature for 48 h.
- For the final step, polishing was done by a polishing substantial to achieve a glossy high-quality surface.
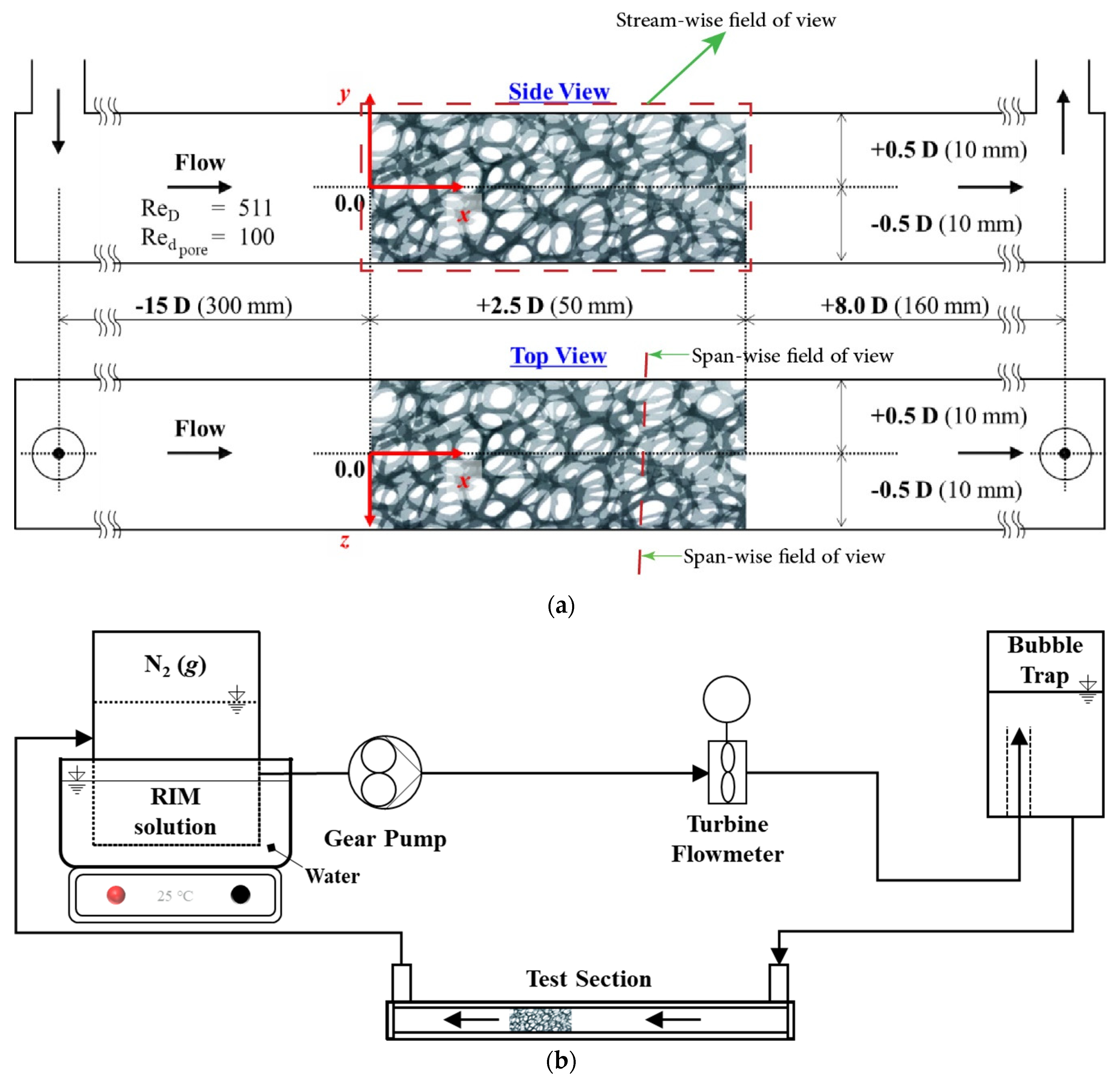
2.2. Experiment Test Section
2.3. PIV Measurement Setup
2.4. Preliminary Experiments
3. Results and Discussion
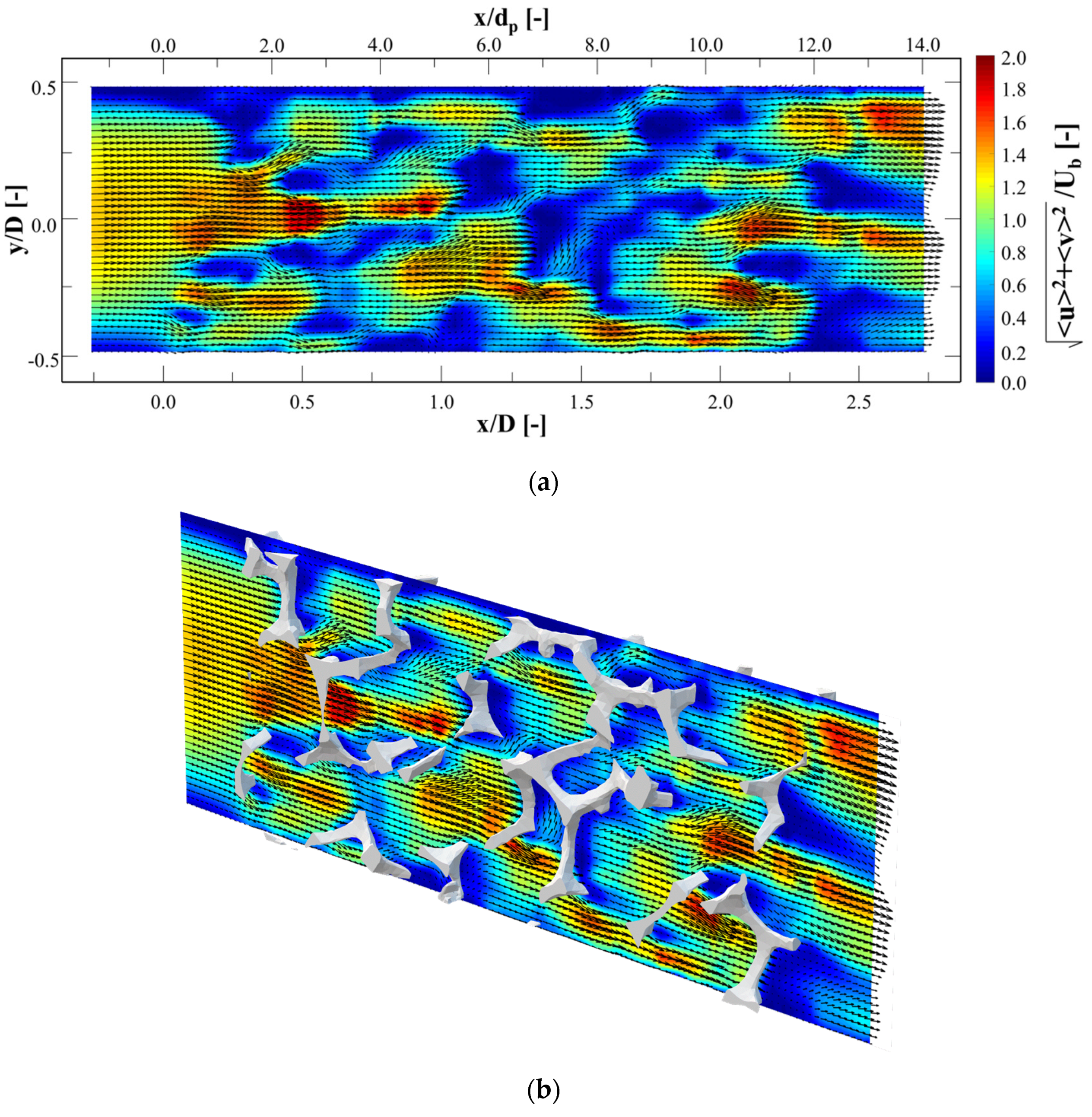
3.1. Velocity Magnitude
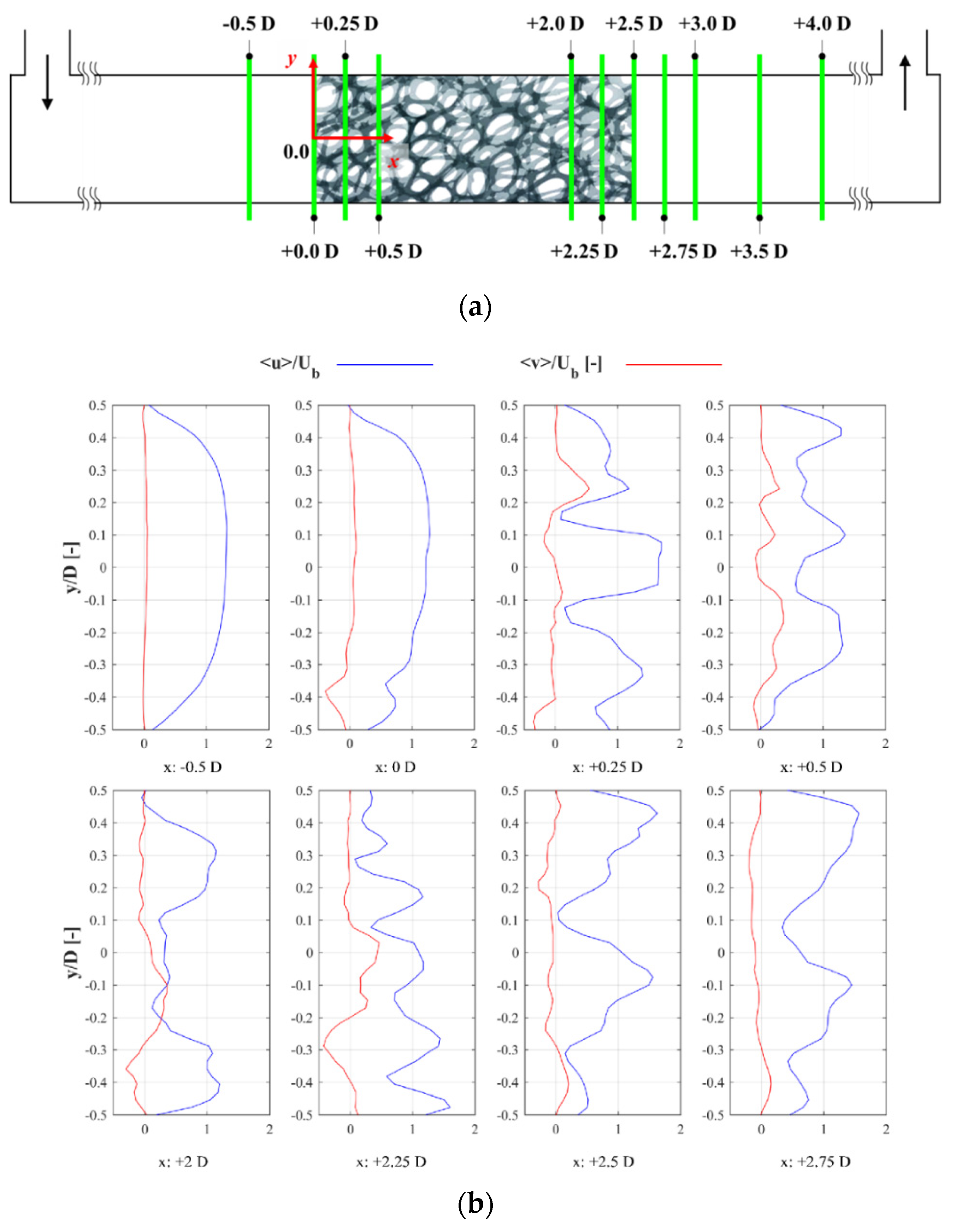
3.2. Mean Velocity Profile
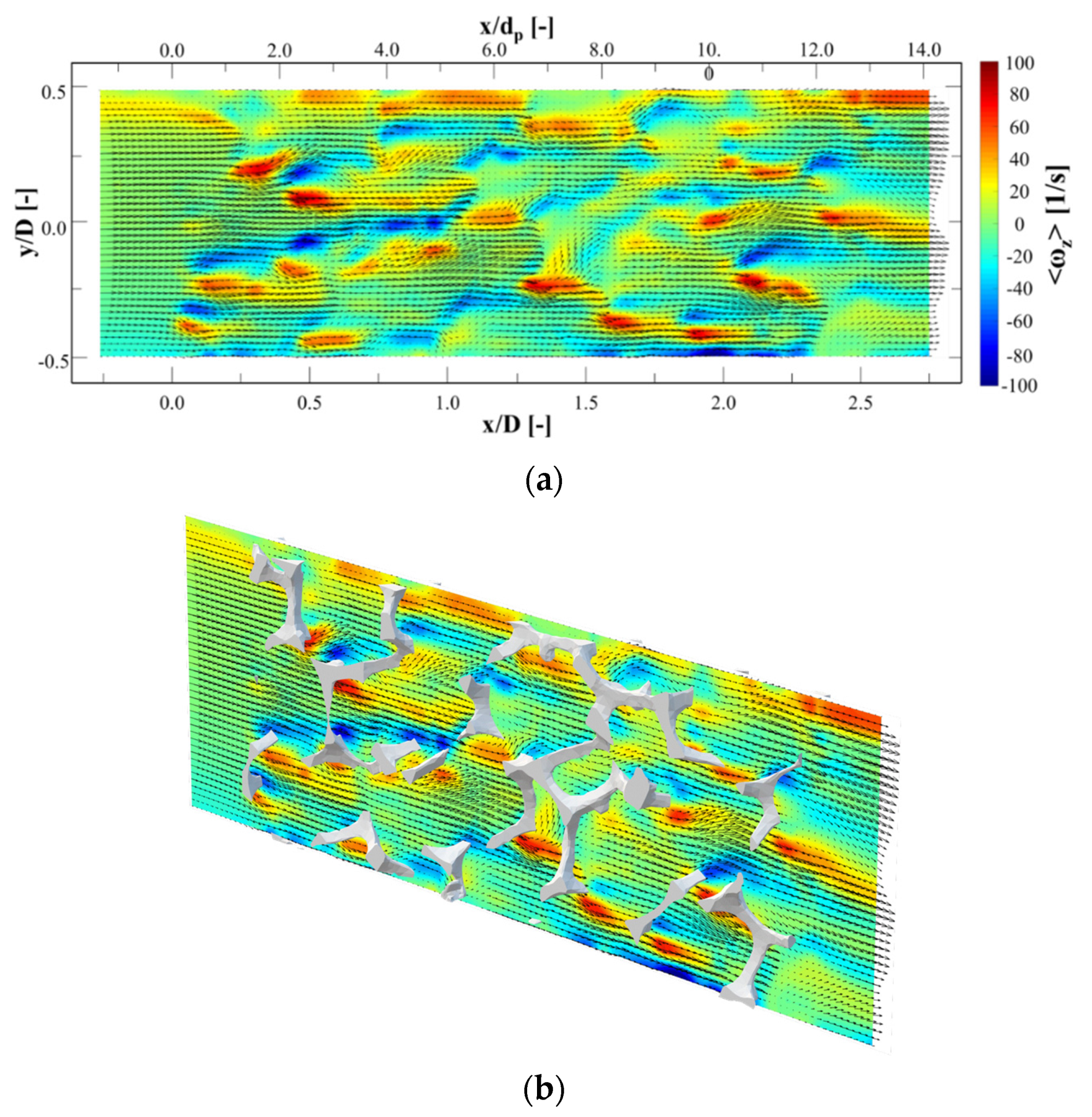
3.3. Mean Vorticity
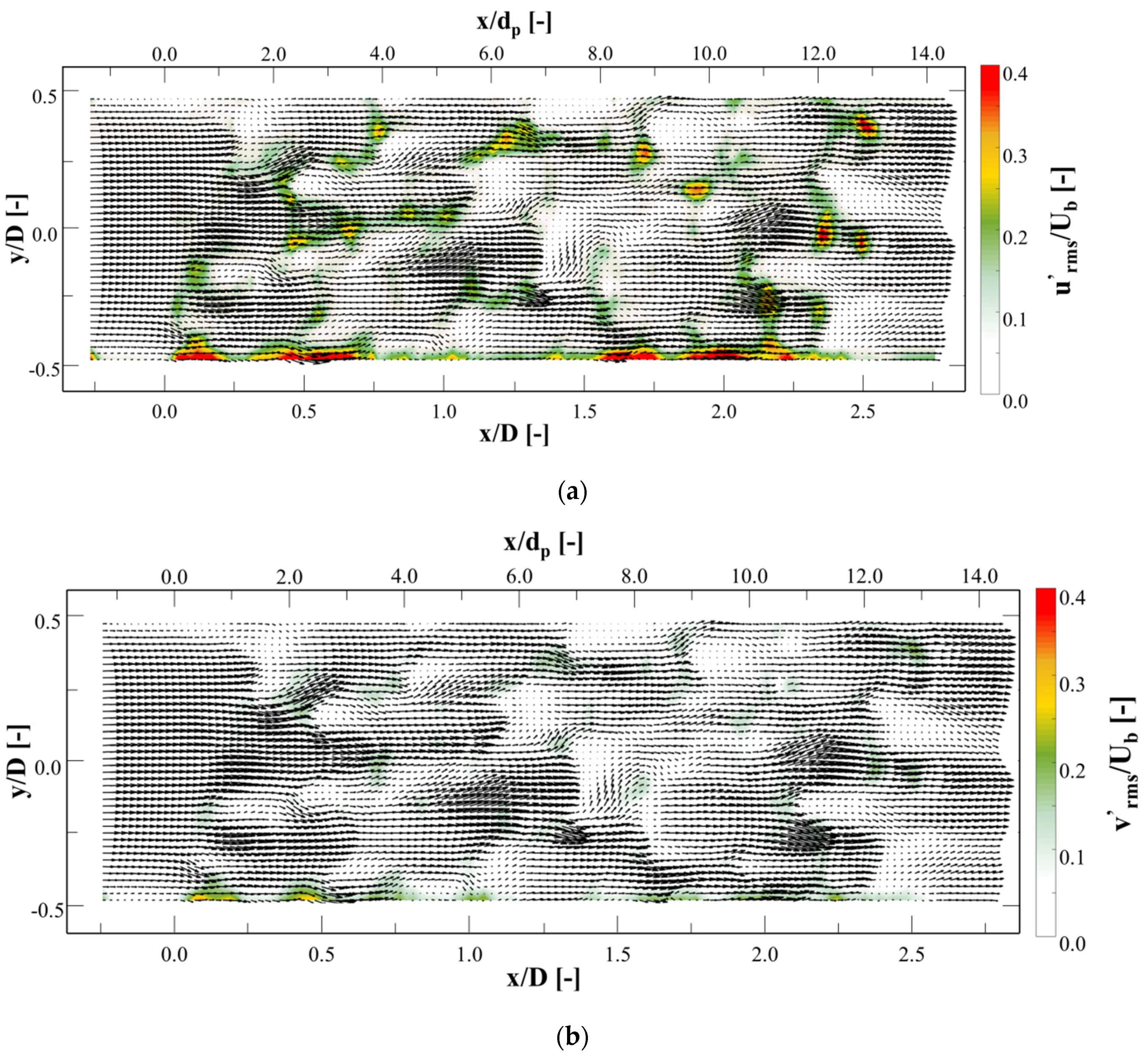
3.4. Integral Time Scale and Length Scale
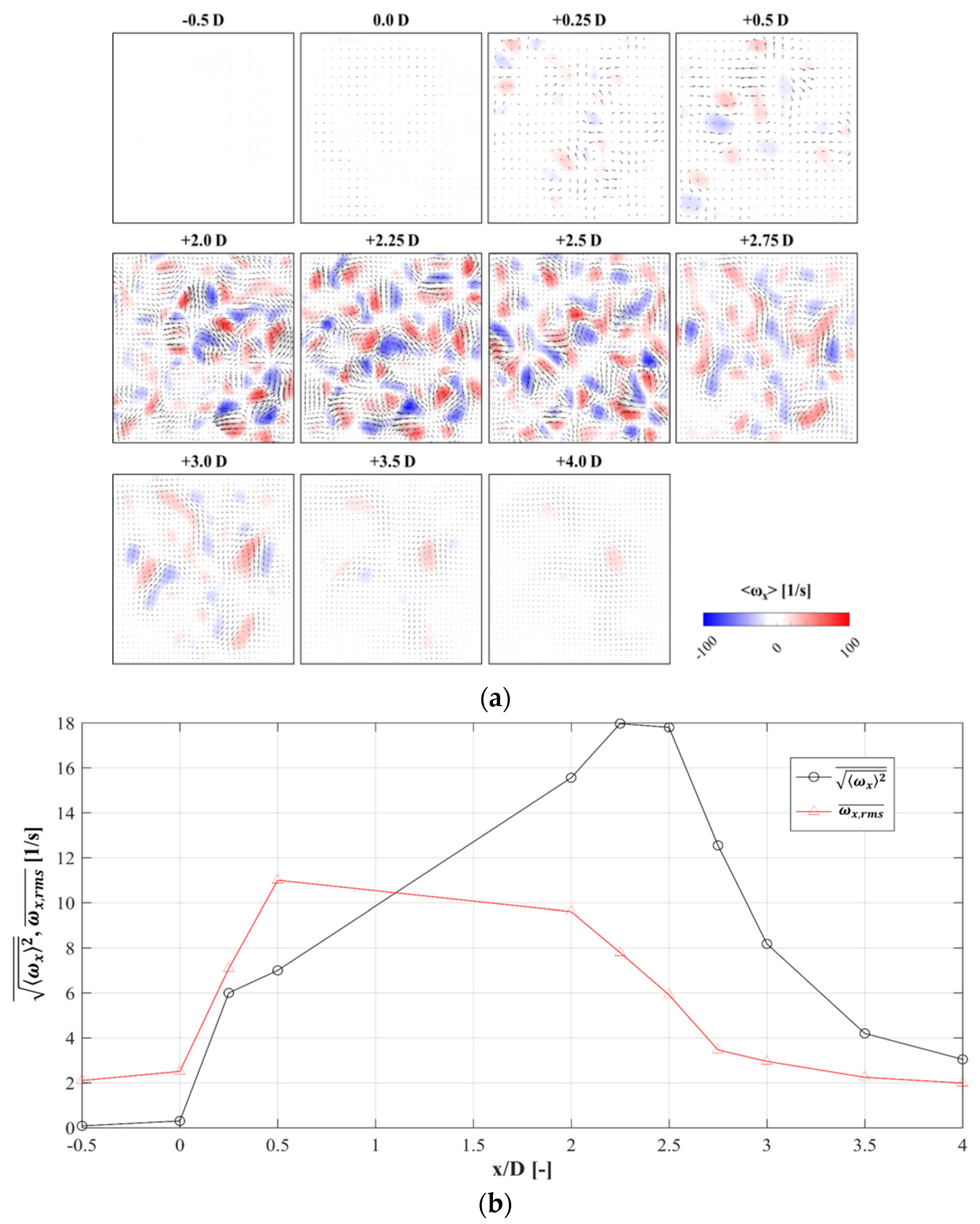
3.5. Span-Wise Vorticity
3.6. Finite-Time Lyapunov Exponent (FTLE)
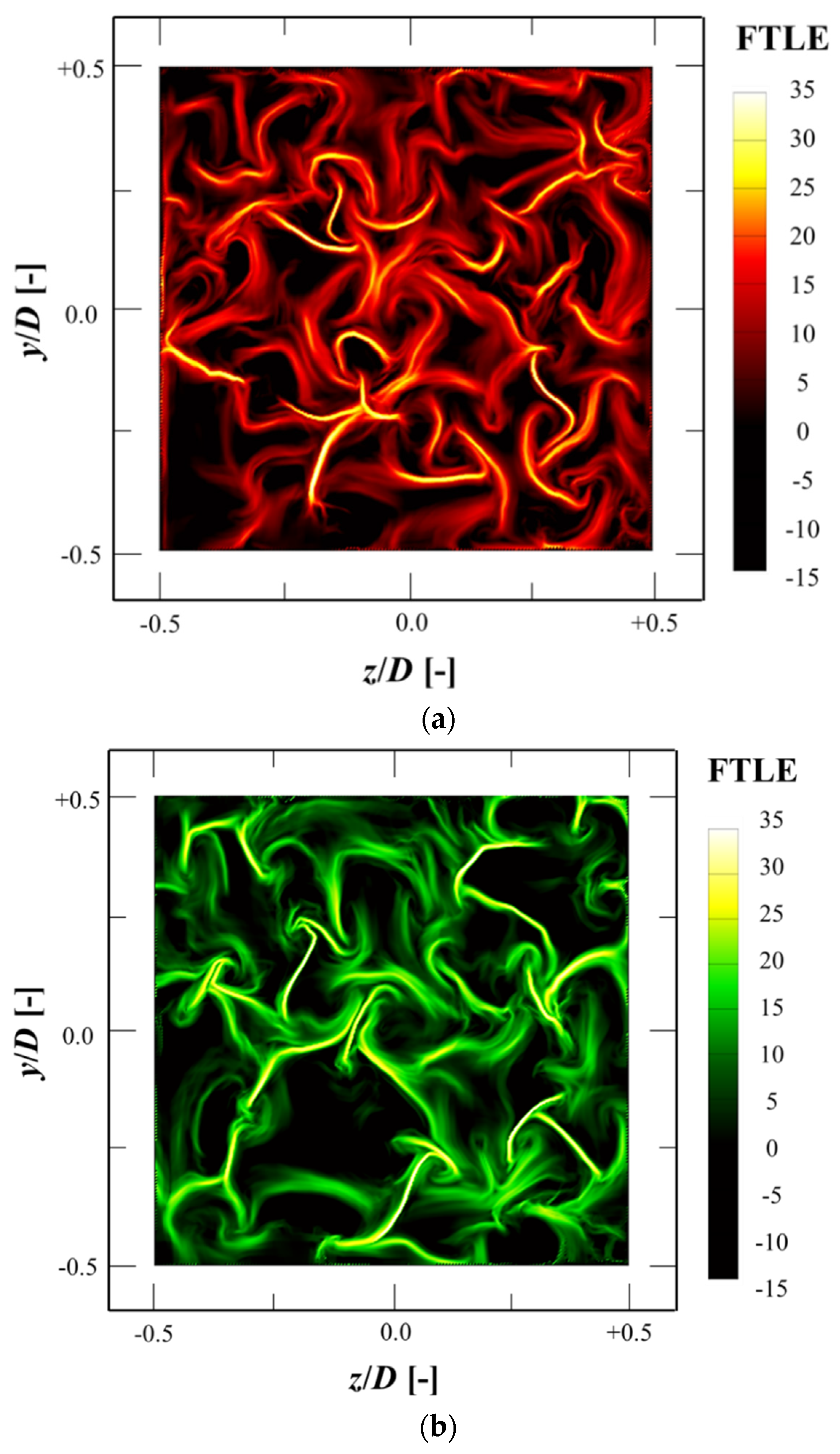
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hlushkou, D.; Piatrusha, S.; Tallarek, U. Impact of diffusion on transverse dispersion in two-dimensional ordered and random porous media. Phys. Rev. E 2017, 95, 063108. [Google Scholar] [CrossRef] [Green Version]
- Zhao, C.; Hobbs, B.; Ord, A. Chemical dissolution-front instability associated with water-rock reactions in groundwater hydrology: Analyses of porosity-permeability relationship effects. J. Hydrol. 2016, 540, 1078–1087. [Google Scholar] [CrossRef]
- Nematollahi, O.; Abadi, G.B.; Kim, D.Y.; Kim, K.C. Experimental study of the effect of brazed compact metal-foam evaporator in an organic Rankine cycle performance: Toward a compact ORC. Energy Convers. Manag. 2018, 173, 37–45. [Google Scholar] [CrossRef]
- Nguyen, T.; Muyshondt, R.; Hassan, Y.A.; Anand, N.K. Experimental investigation of cross flow mixing in a randomly packed bed and stream-wise vortex characteristics using particle image velocimetry and proper orthogonal decomposition analysis. Phys. Fluids 2019, 31, 025101. [Google Scholar] [CrossRef]
- Hidalgo, J.J.; Dentz, M. Mixing across fluid interfaces compressed by convective flow in porous media. J. Fluid Mech. 2018, 838, 105–128. [Google Scholar] [CrossRef] [Green Version]
- Niu, S.P.; Park, K.; Yu, J.H.; Kim, Y. Operation and performance evaluation of high-speed filter using porous non-woven filamentous fibre for the treatment of turbid water. Environ. Technol. 2016, 37, 577–589. [Google Scholar] [CrossRef]
- Hutter, C.; Zenklusen, A.; Lang, R.; von Rohr, P.R. Axial dispersion in metal foams and stream-wise -periodic porous media. Chem. Eng. Sci. 2011, 66, 1132–1141. [Google Scholar] [CrossRef]
- Hutter, C.; Büchi, D.; Zuber, V.; von Rohr, P.R. Heat transfer in metal foams and designed porous media. Chem. Eng. Sci. 2011, 66, 3806–3814. [Google Scholar] [CrossRef]
- Calmidi, V.V.; Mahajan, R.L. Forced Convection in High Porosity Metal Foams. J. Heat Transf. 2000, 122, 557–565. [Google Scholar] [CrossRef]
- Kraynik, A.M. The Structure of Random Foam. Adv. Eng. Mater. 2006, 8, 900–906. [Google Scholar] [CrossRef]
- Mercer, C.; He, M.; Wang, R.; Evans, A. Mechanisms governing the inelastic deformation of cortical bone and application to trabecular bone. Acta Biomater. 2006, 2, 59–68. [Google Scholar] [CrossRef] [PubMed]
- Moon, C.; Kim, D.; Abadi, G.B.; Yoon, S.Y.; Kim, K.C. Effect of ligament hollowness on heat transfer characteristics of open-cell metal foam. Int. J. Heat Mass Transf. 2016, 102, 911–918. [Google Scholar] [CrossRef]
- Kuruneru, S.; Saha, S.; Sauret, E.; Gu, Y. Transient heat transfer and non-isothermal particle-laden gas flows through porous metal foams of differing structure. Appl. Therm. Eng. 2019, 150, 888–903. [Google Scholar] [CrossRef]
- Jin, L.W.; Leong, K.C. Pressure drop and friction factor of steady and oscillating flows in open-cell porous media. Transp. Porous Media 2007, 72, 37–52. [Google Scholar] [CrossRef]
- Linul, E.; Marsavina, L.; Kováčik, J. Collapse mechanisms of metal foam matrix composites under static and dynamic loading conditions. Mater. Sci. Eng. A 2017, 690, 214–224. [Google Scholar] [CrossRef]
- Hassan, Y.A.; Dominguez-Ontiveros, E. Flow visualization in a pebble bed reactor experiment using PIV and refractive index matching techniques. Nucl. Eng. Des. 2008, 238, 3080–3085. [Google Scholar] [CrossRef]
- Hwang, J.-J.; Yeh, R.-H.; Chao, C.-H. Measurement of Interstitial Convective Heat Transfer and Frictional Drag for Flow Across Metal Foams. J. Heat Transf. 2002, 124, 120–129. [Google Scholar] [CrossRef]
- Eggenschwiler, P.D.; Tsinoglou, D.N.; Seyfert, J.; Bach, C.; Vogt, U.; Gorbar, M. Ceramic foam substrates for automotive catalyst applications: Fluid mechanic analysis. Exp. Fluids 2009, 47, 209–222. [Google Scholar] [CrossRef]
- Hutter, C.; Allemann, C.; Kuhn, S.; Von Rohr, P.R. Scalar transport in a milli-scale metal foam reactor. Chem. Eng. Sci. 2010, 65, 3169–3178. [Google Scholar] [CrossRef]
- Butscher, D.; Hutter, C.; Kuhn, S.; Von Rohr, P.R. Particle image velocimetry in a foam-like porous structure using refractive index matching: A method to characterize the hydrodynamic performance of porous structures. Exp. Fluids 2012, 53, 1123–1132. [Google Scholar] [CrossRef] [Green Version]
- Onstad, A.J.; Elkins, C.J.; Medina, F.; Wicker, R.B.; Eaton, J.K. Full-field measurements of flow through a scaled metal foam replica. Exp. Fluids 2011, 50, 1571–1585. [Google Scholar] [CrossRef]
- Aycock, K.I.; Hariharan, P.; Craven, B.A. Particle image velocimetry measurements in an anatomical vascular model fabricated using inkjet 3D printing. Exp. Fluids 2017, 58, 154. [Google Scholar] [CrossRef]
- Gallagher, M.B.; Aycock, K.I.; Craven, B.A.; Manning, K.B. Steady Flow in a Patient-Averaged Inferior Vena Cava—Part I: Particle Image Velocimetry Measurements at Rest and Exercise Conditions. Cardiovasc. Eng. Technol. 2018, 9, 641–653. [Google Scholar] [CrossRef] [PubMed]
- Narrow, T.L.; Yoda, M.; Abdel-Khalik, S.I. A simple model for the refractive index of sodium iodide aqueous solutions. Exp. Fluids 2000, 28, 282–283. [Google Scholar] [CrossRef]
- Mendez, M.; Raiola, M.; Masullo, A.; Discetti, S.; Ianiro, A.; Theunissen, R.; Buchlin, J.-M. POD-based background removal for particle image velocimetry. Exp. Therm. Fluid Sci. 2017, 80, 181–192. [Google Scholar] [CrossRef] [Green Version]
- Thielicke, W.; Stamhuis, E.J. PIVlab—Towards User-friendly, Affordable and Accurate Digital Particle Image Velocimetry in MATLAB. J. Open Res. Softw. 2014, 2, 30. [Google Scholar] [CrossRef] [Green Version]
- Keane, R.D.; Adrian, R.J. Optimization of particle image velocimeters. I. Double pulsed systems. Meas. Sci. Technol. 1990, 1, 1202–1215. [Google Scholar] [CrossRef]
- Nobach, H.; Honkanen, M. Two-dimensional Gaussian regression for sub-pixel displacement estimation in particle image velocimetry or particle position estimation in particle tracking velocimetry. Exp. Fluids 2005, 38, 511–515. [Google Scholar] [CrossRef]
- Westerweel, J.; Scarano, F. Universal outlier detection for PIV data. Exp. Fluids 2005, 39, 1096–1100. [Google Scholar] [CrossRef]
- Ziazi, R.M.; Liburdy, J.A. Vortical Structure Characteristics of Transitional Flow Through Porous Media. In Proceedings of the ASME-JSME-KSME 2019 8th Joint Fluids Engineering Conference, San Francisco, CA, USA, 28 July–1 August 2019. [Google Scholar]
- Khayamyan, S.; Lundström, T.S.; Hellström, J.G.I.; Gren, P.; Lycksam, H. Measurements of Transitional and Turbulent Flow in a Randomly Packed Bed of Spheres with Particle Image Velocimetry. Transp. Porous Media 2017, 116, 413–431. [Google Scholar] [CrossRef] [Green Version]
- Hlushkou, D.; Tallarek, U. Transition from creeping via viscous-inertial to turbulent flow in fixed beds. J. Chromatogr. A 2006, 1126, 70–85. [Google Scholar] [CrossRef] [PubMed]
- Moon, C.; Kim, H.D.; Kim, K.C. Kelvin-cell-based metal foam heat exchanger with elliptical struts for low energy consumption. Appl. Therm. Eng. 2018, 144, 540–550. [Google Scholar] [CrossRef]
- Swamy, N.V.C.; Gowda, B.H.L.; Lakshminath, V.R. Auto-correlation measurements and integral time scales in three-dimensional turbulent boundary layers. Flow Turbul. Combust. 1979, 35, 237–249. [Google Scholar] [CrossRef]
- Liu, H.; Hayat, I.; Jin, Y.; Chamorro, L.P. On the Evolution of the Integral Time Scale within Wind Farms. Energies 2018, 11, 93. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Wang, J.-J.; Feng, L.-H.; He, G.-S.; Wang, Z.-Y. Laminar vortex rings impinging onto porous walls with a constant porosity. J. Fluid Mech. 2018, 837, 729–764. [Google Scholar] [CrossRef]





| Porosity (ε) | |||
|---|---|---|---|
| 0.92 | 0.8 ± 0.06 mm | 3.9 ± 0.33 mm | 9.02 ± 1.06 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, Y.; Moon, C.; Nematollahi, O.; Kim, H.D.; Kim, K.C. Time-Resolved PIV Measurements and Turbulence Characteristics of Flow Inside an Open-Cell Metal Foam. Materials 2021, 14, 3566. https://doi.org/10.3390/ma14133566
Kim Y, Moon C, Nematollahi O, Kim HD, Kim KC. Time-Resolved PIV Measurements and Turbulence Characteristics of Flow Inside an Open-Cell Metal Foam. Materials. 2021; 14(13):3566. https://doi.org/10.3390/ma14133566
Chicago/Turabian StyleKim, Youngwoo, Chanhee Moon, Omid Nematollahi, Hyun Dong Kim, and Kyung Chun Kim. 2021. "Time-Resolved PIV Measurements and Turbulence Characteristics of Flow Inside an Open-Cell Metal Foam" Materials 14, no. 13: 3566. https://doi.org/10.3390/ma14133566
APA StyleKim, Y., Moon, C., Nematollahi, O., Kim, H. D., & Kim, K. C. (2021). Time-Resolved PIV Measurements and Turbulence Characteristics of Flow Inside an Open-Cell Metal Foam. Materials, 14(13), 3566. https://doi.org/10.3390/ma14133566







