Heating and Compression at Elevated Temperature of Thin-Walled Titanium Channel Section Columns
Abstract
:1. Introduction
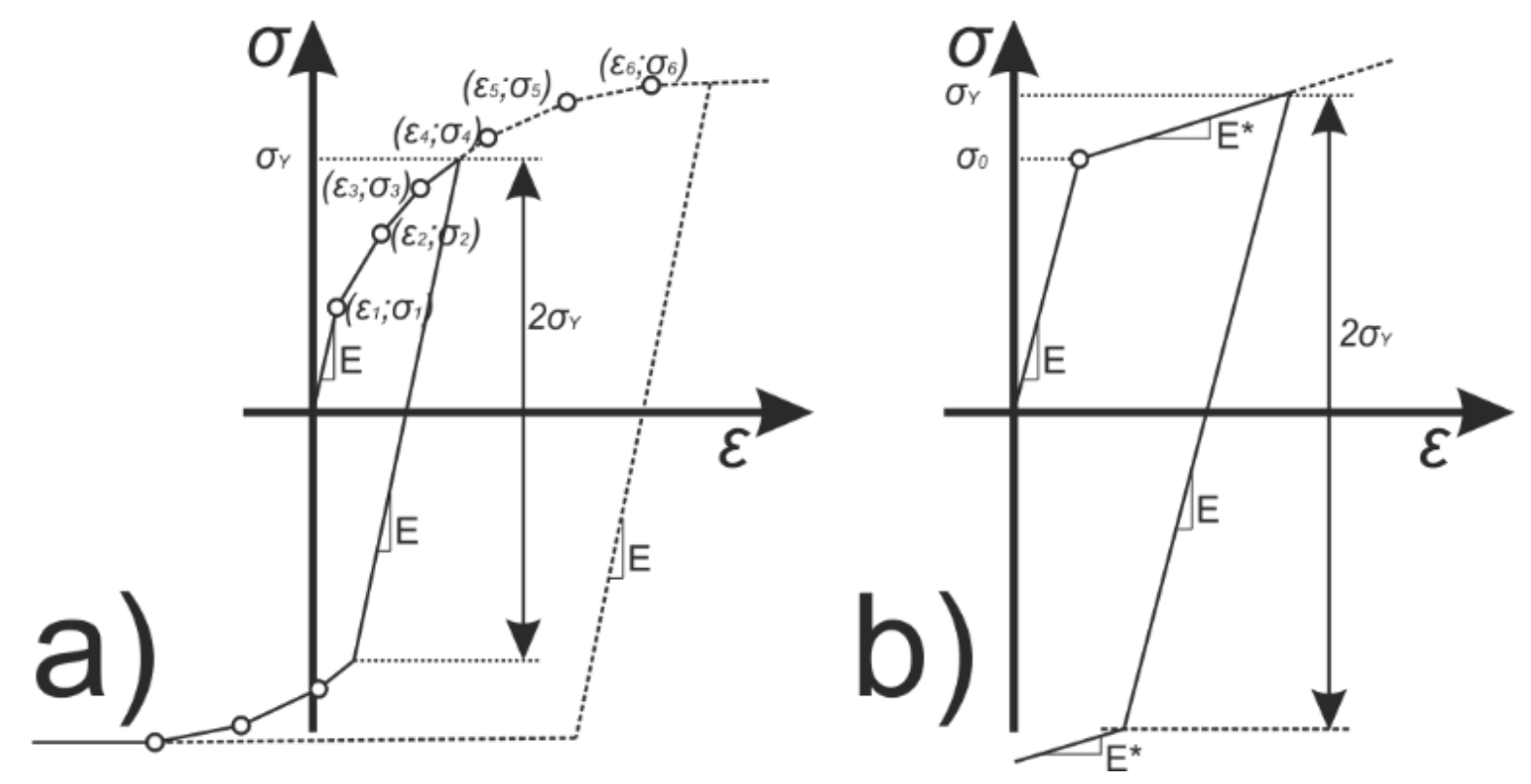
2. Considered Plasticity Models
- the yield criterion that defines the material state at the transition from elastic to elastic-plastic behavior;
- the flow rule that determines the increment in plastic strain from the increment in load;
- the hardening rule that gives the evolution in the yield criterion during plastic deformation.
3. Experimental Procedure
- initial compression of the column,
- heating the preloaded column,
- compression of the column at elevated temperature.
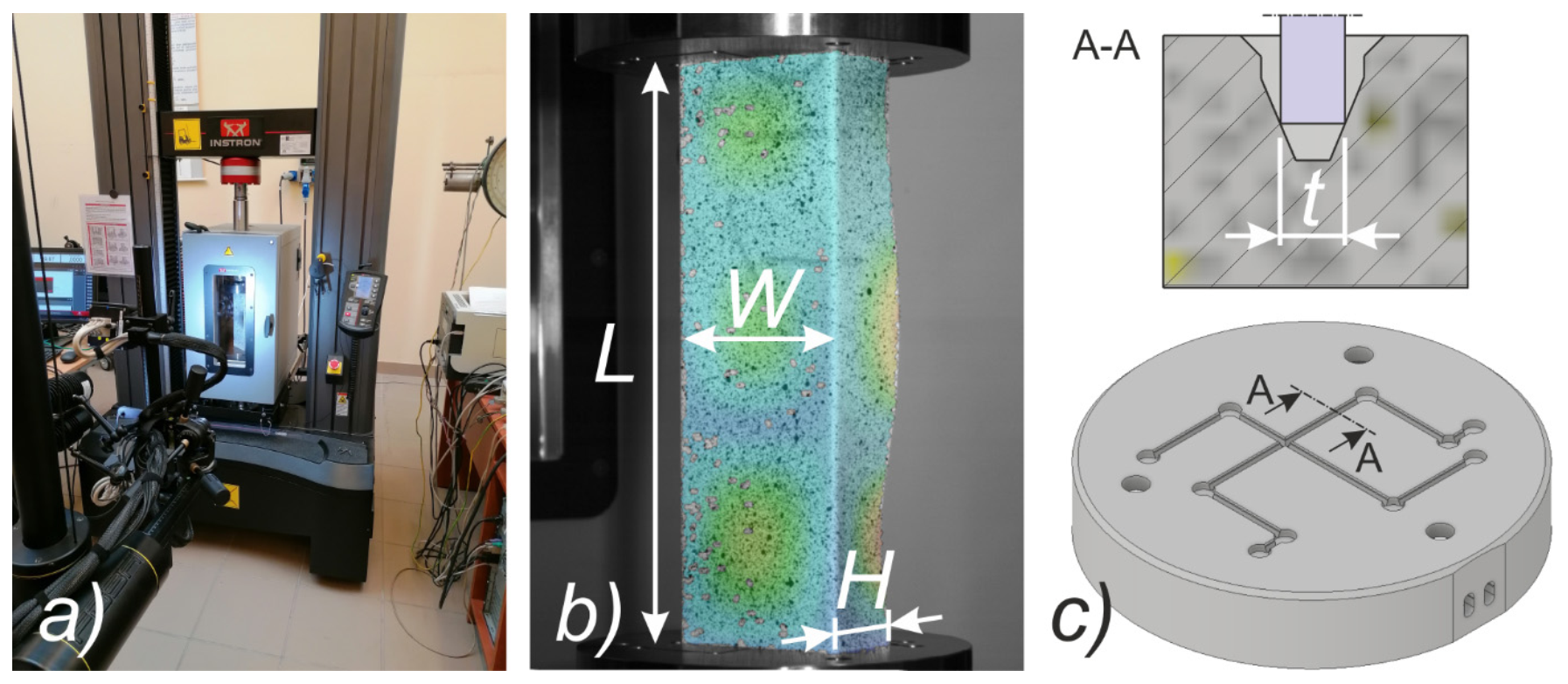
3.1. Test Stand
3.2. Material Properties
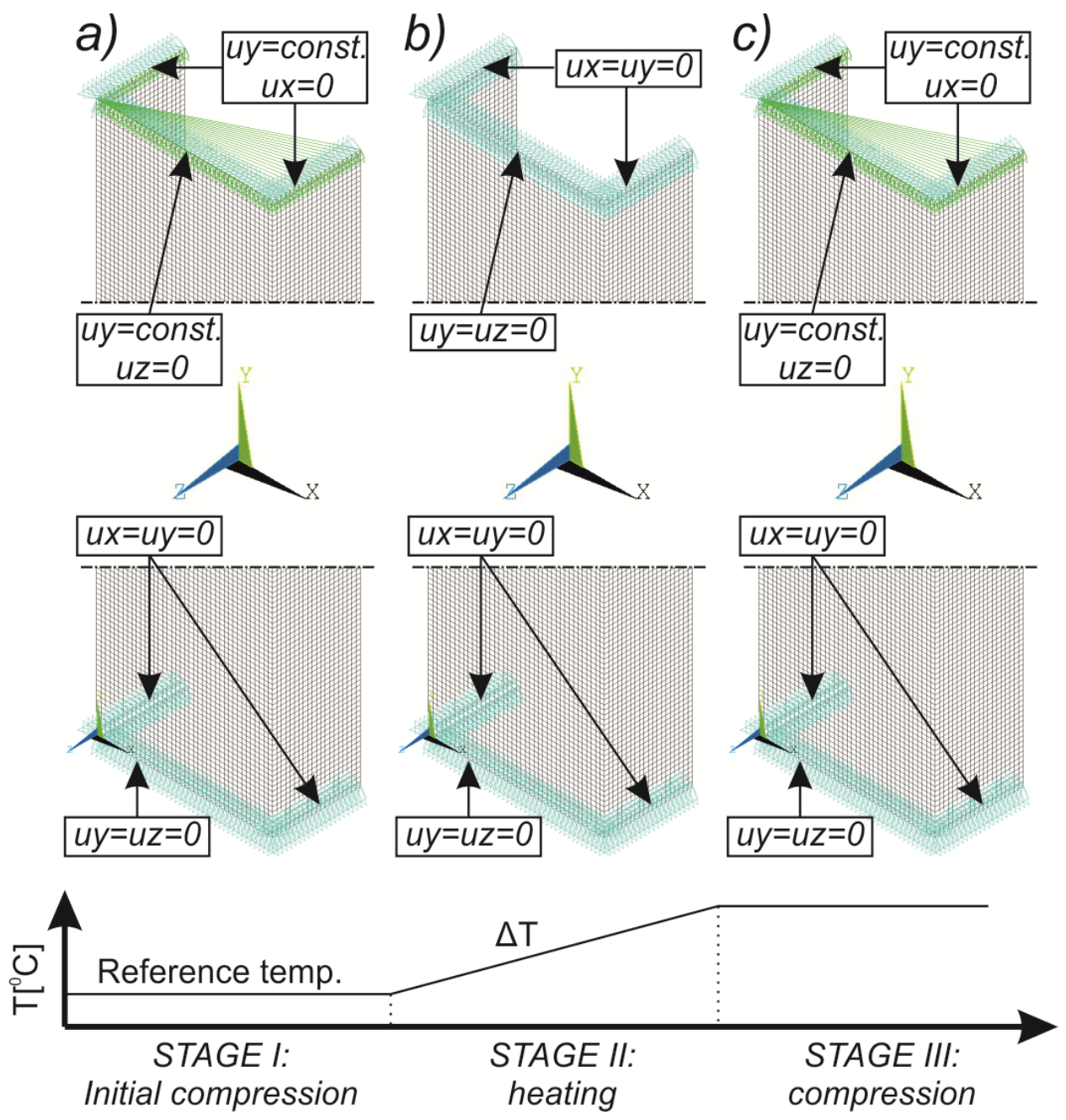
4. FE Model with Assumed Boundary Conditions
5. Discussion
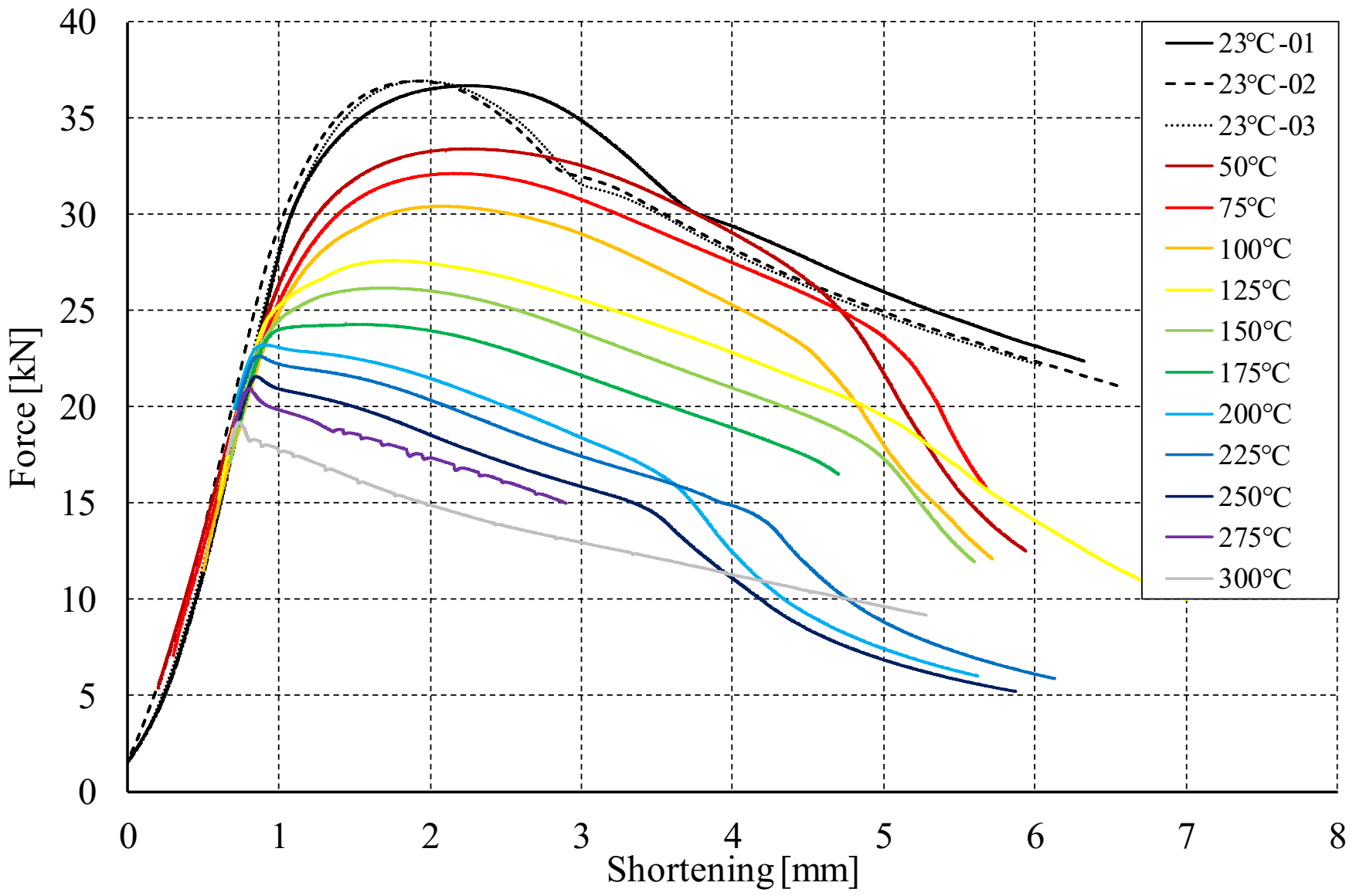
5.1. Compression of Channel Section Profiles at Ambient Temperature
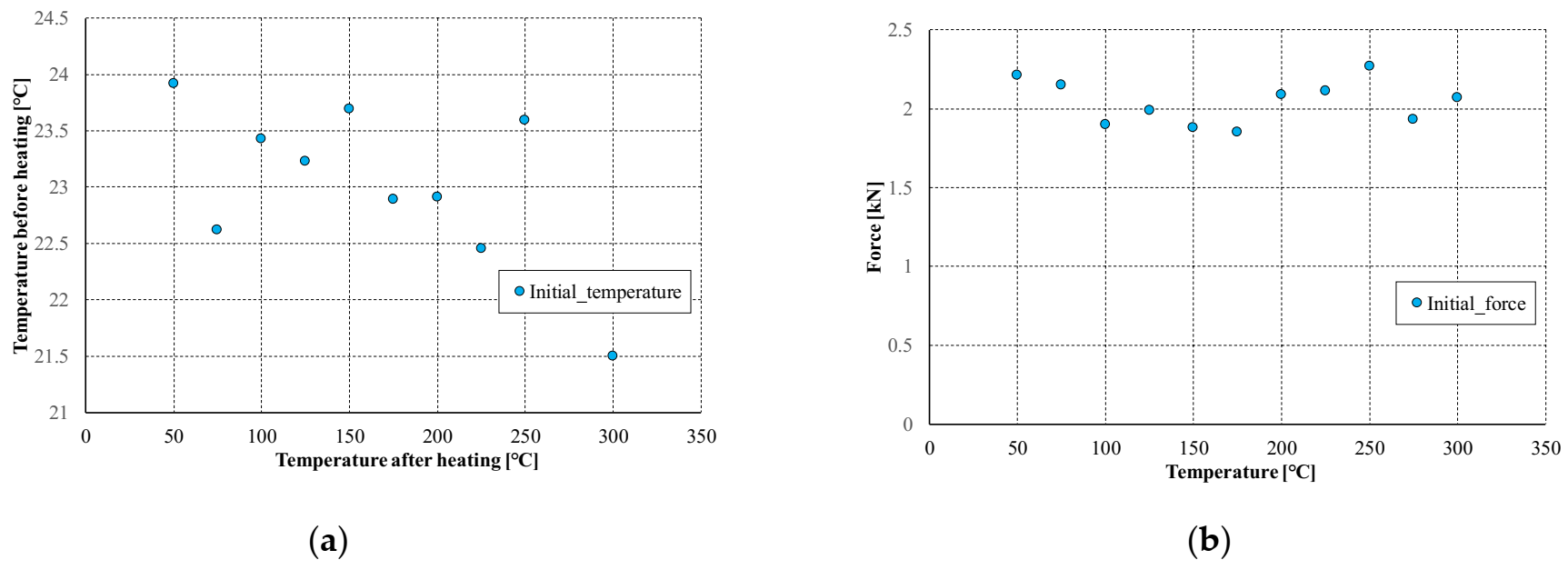
5.2. Initial Compression
5.3. Heating
5.4. Compression after Heating
6. Conclusions
- the proximity of the first buckling loads may cause the phenomenon of the coexistence of different buckling modes under the same test conditions and lead to a state in which the final buckling mode is determined by slight differences in boundary conditions and more specifically the arrangement of the loading and supporting edges in the test stand grooves;
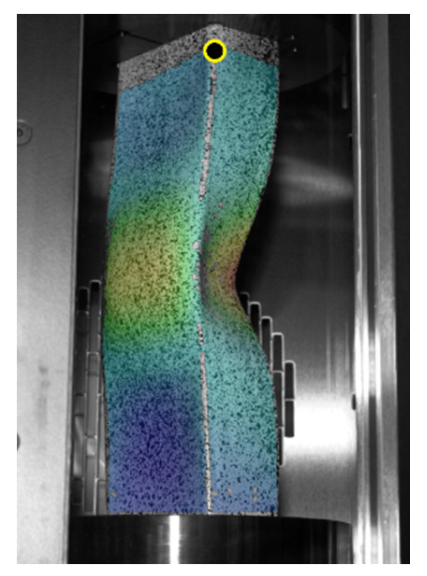
- the presence of three buckling half-waves in the case of tests with the use of elevated temperatures resulted in the formation of a plastic mechanism in the middle of the span of the analyzed profiles. This is an advantageous phenomenon because the plastic mechanism is located at a considerable distance from the loading and supporting edges and allows to assume that the assumed boundary conditions are maintained in the full test range. In the case of two buckling half-waves, the plastic mechanism is always formed close to the loading or supporting edges, which means that the boundary conditions, especially in the range after reaching the maximum compressive force, may deviate from the theoretical assumptions;
- the temperature increase in a statically indeterminate system causes a reduction in the load capacity of the profile, the greater the higher the temperature increase. Already an increase in temperature at the level of 27 °C induces a reduction of the load capacity by 10%, while compression at a temperature of 300 °C reduces the nominal load capacity of the profile by half;
- for the considered profile, thermal buckling occurs at the temperature of 175 °C. Initially, the flanges buckle, followed by the web of the channel section columns;
- compression after thermal buckling allows to achieve compressive forces not much higher than those determined in the heating process. In general, the tested columns lose their stability almost immediately after thermal buckling.
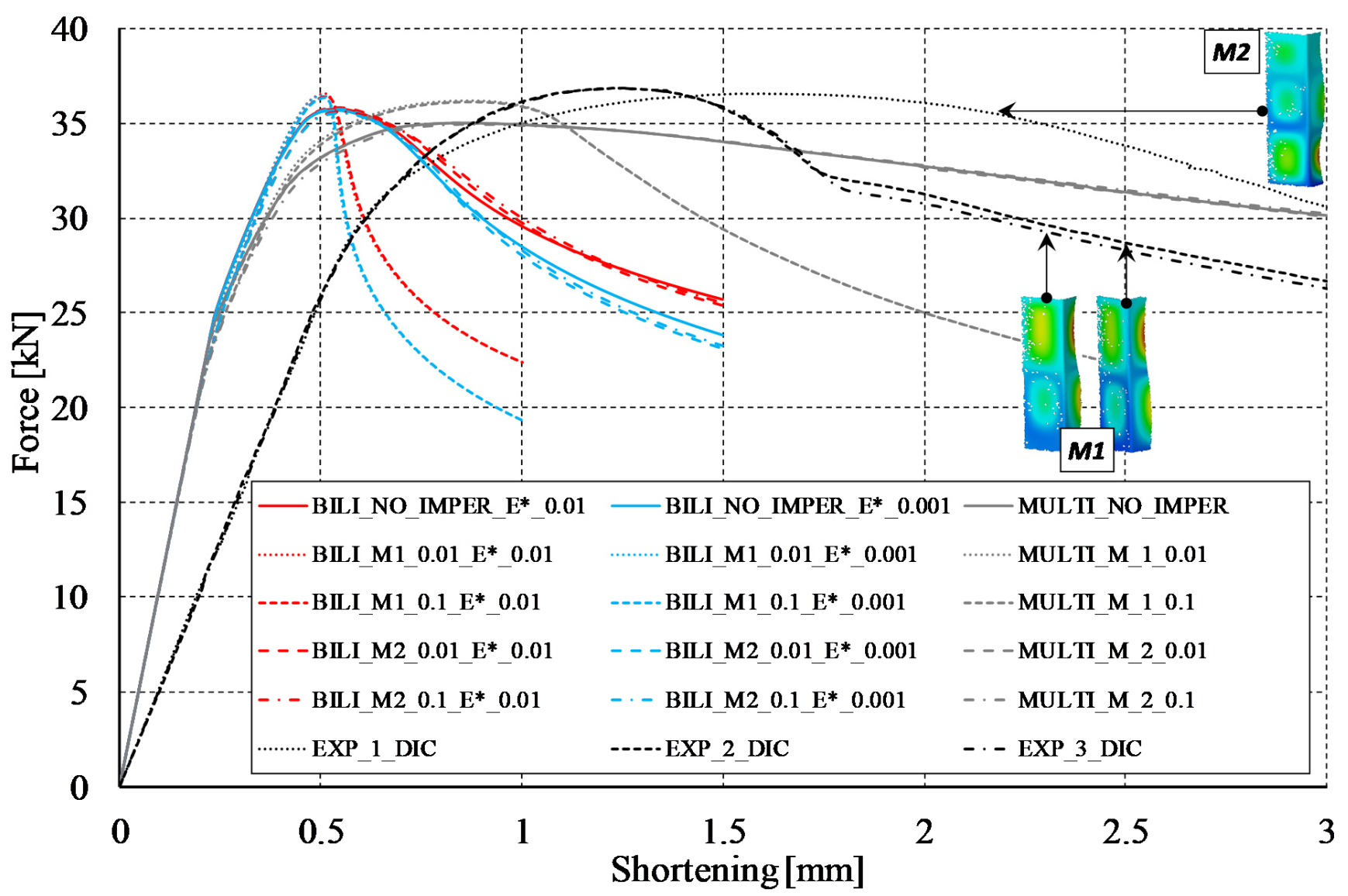
- in the experimental tests, no change in the initial buckling mode was observed, while in the numerical tests the key parameter is the initial imperfection amplitude. Taking this parameter at the level of one-tenth of the wall thickness of the considered profile allows to maintain the nominally assumed buckling mode in the full range of the considered loads. In numerical terms, the change of buckling mode concerned only the change from two to three buckling half-waves along the length of the profiles and it occurred only during heating—stage II;
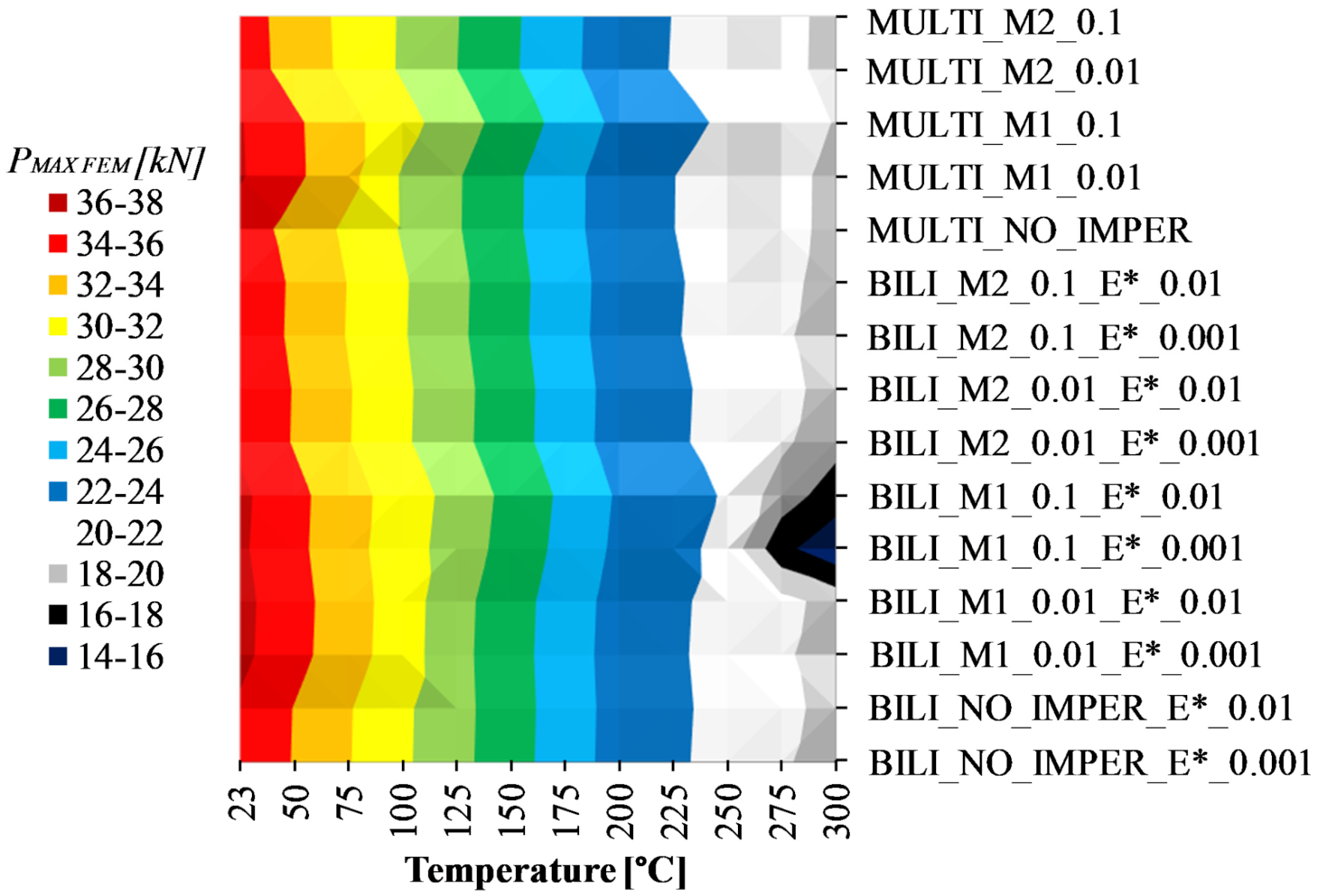
- almost all proposed numerical models allow for accurate estimation of both the reaction forces during heating and the maximum compressive forces recorded during compression at elevated temperatures. It also proves the correctness of the determined material characteristics as well as the suitability of shell models for estimating the response of a thin-walled structure subjected to thermomechanical loading;
- from the perspective of all the results achieved, the best qualitative and quantitative results seem to be achieved with the implementation of the multilinear model. Despite the similar values of the characteristic parameters describing the tests carried out, such as: the reaction force at the end of heating or the maximum force during compression at elevated temperature, the use of the multilinear model also allows for the achievement of force vs. shortening curves in the full load range similar to the experimental ones. In numerical terms, it was also easier to maintain the convergence of the solution using the multilinear model, which in turn also translated into a shorter computational time.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Bodunrin, M.O.; Chown, L.H.; Omotoyinbo, J.A. Development of low-cost titanium alloys: A chronicle of challenges and opportunities. Mater. Today Proc. 2021, 38, 564–569. [Google Scholar] [CrossRef]
- Guo, R.; Liu, B.; Xu, R.; Cao, Y.; Qiu, J.; Chen, F.; Yan, Z.; Liu, Y. Microstructure and mechanical properties of powder metallurgy high temperature titanium alloy with high Si content. Mater. Sci. Eng. A 2020, 777, 138993. [Google Scholar] [CrossRef]
- Alabort, E.; Barba, D.; Shagiev, M.R.; Murzinova, M.A.; Galeyev, R.M.; Valiakhmetov, O.R.; Aletdinov, A.F.; Reed, R.C. Alloys-by-design: Application to titanium alloys for optimal superplasticity. Acta Mater. 2019, 178, 275–287. [Google Scholar] [CrossRef]
- Koizumi, H.; Takeuchi, Y.; Imai, H.; Kawai, T.; Yoneyama, T. Application of titanium and titanium alloys to fixed dental prostheses. J. Prosthodont. Res. 2019, 63, 266–270. [Google Scholar] [CrossRef] [PubMed]
- Fan, S.; Jia, L.; Lyu, X.; Sun, W.; Chen, M.; Zheng, J. Experimental investigation of austenitic stainless steel material at elevated temperatures. Constr. Build. Mater. 2017, 155, 267–285. [Google Scholar] [CrossRef]
- Li, H.-T.; Young, B. Material properties of cold-formed high strength steel at elevated temperatures. Thin Walled Struct. 2017, 115, 289–299. [Google Scholar] [CrossRef]
- Fang, H.; Chan, T.-M. Axial compressive strength of welded S460 steel columns at elevated temperatures. Thin Walled Struct. 2018, 129, 213–224. [Google Scholar] [CrossRef]
- Su, M.-N.; Zhang, Y.; Young, B. Design of aluminium alloy beams at elevated temperatures. Thin Walled Struct. 2019, 140, 506–515. [Google Scholar] [CrossRef]
- Li, H.-T.; Young, B. Cold-formed high strength steel SHS and RHS beams at elevated temperatures. J. Constr. Steel Res. 2019, 158, 475–485. [Google Scholar] [CrossRef]
- Jin, T.; Ha, N.S.; Le, V.T.; Goo, N.S.; Jeon, H.C. Thermal buckling measurement of a laminated composite plate under a uniform temperature distribution using the digital image correlation method. Compos. Struct. 2015, 123, 420–429. [Google Scholar] [CrossRef]
- Jin, T.L.; Ha, N.S.; Goo, N.S. A study of the thermal buckling behavior of a circular aluminum plate using the digital image correlation technique and finite element analysis. Thin Walled Struct. 2014, 77, 187–197. [Google Scholar] [CrossRef]
- Nguyena, P.L.; Vua, X.H.; Ferriera, E. Thermo-mechanical performance of Carbon Fiber Reinforced Polymer (CFRP), with and without fire protection material, under combined elevated temperature and mechanical loading conditions. Compos. Part B Eng. 2019, 169, 164–173. [Google Scholar] [CrossRef]
- Zhou, F.; Zhang, J.; Song, S.; Yang, D.; Wang, C. Effect of Temperature on Material Properties of Carbon Fiber Reinforced Polymer (CFRP) Tendons: Experiments and Model Assessment. Materials 2019, 12, 1025. [Google Scholar] [CrossRef] [Green Version]
- Glassman, J.D.; Gomez, A.; Moreyra Garlock, M.E.; Ricles, J. Mechanical properties of weathering steels at elevated temperatures. J. Constr. Steel Res. 2020, 168, 105996. [Google Scholar] [CrossRef]
- Khaneghahi, M.H.; Najafabadi, E.P.; Bazli, M.; Oskouei, A.V.; Zhao, X.-L. The effect of elevated temperatures on the compressive section capacity of pultruded GFRP profiles. Constr. Build. Mater. 2020, 249, 118725. [Google Scholar] [CrossRef]
- Jo, B.; Sagawa, W.; Okamoto, K. Measurement of buckling load for metallic plate columns in severe accident conditions. Nucl. Eng. Des. 2014, 274, 118–128. [Google Scholar] [CrossRef]
- Zhang, Y.; Yang, L.; Lu, W.; Wei, D.; Meng, T.; Gao, S. Microstructure and elevated temperature mechanical properties of IN718 alloy fabricated by laser metal deposition. Mater. Sci. Eng. A 2020, 771, 138580. [Google Scholar] [CrossRef]
- Su, M.-N.; Young, B. Material properties of normal and high strength aluminium alloys at elevated temperatures. Thin Walled Struct. 2019, 137, 463–471. [Google Scholar] [CrossRef]
- Rokilan, M.; Mahendran, M. Elevated temperature mechanical properties of cold-rolled steel sheets and cold-formed steel sections. J. Constr. Steel Res. 2020, 167, 105851. [Google Scholar] [CrossRef]
- Liang, Y.; Manninen, T.; Zhao, O.; Walport, F.; Gardner, L. Elevated temperature material properties of a new high-chromium austenitic stainless steel. J. Constr. Steel Res. 2019, 152, 261–273. [Google Scholar] [CrossRef] [Green Version]
- Xiong, M.-X.; Liew, J.R. Experimental study to differentiate mechanical behaviours of TMCP and QT high strength steel at elevated temperatures. Constr. Build. Mater. 2020, 242, 118105. [Google Scholar] [CrossRef]
- Wang, W.; Zhang, L.; Ge, Y.; Xu, L. Behaviour of restrained high strength steel columns at elevated temperature. J. Constr. Steel Res. 2018, 148, 251–264. [Google Scholar] [CrossRef]
- Wang, W.; Zhou, H.; Xu, L. Creep buckling of high strength Q460 steel columns at elevated temperatures. J. Constr. Steel Res. 2019, 157, 414–425. [Google Scholar] [CrossRef]
- Trabelsi, S.; Frikha, A.; Zghal, S.; Dammak, F. Thermal post-buckling analysis of functionally graded material structures using a modified FSDT. Int. J. Mech. Sci. 2018, 144, 74–89. [Google Scholar] [CrossRef]
- Wattanasakulpong, N.; Prusty, G.B.; Kelly, D.W. Thermal buckling and elastic vibration of third-order shear deformable functionally graded beams. Int. J. Mech. Sci. 2011, 53, 734–743. [Google Scholar] [CrossRef]
- Bui, T.Q.; Do, T.V.; Ton, L.H.T.; Doan, D.H.; Tanaka, S.; Pham, D.T.; Nguyen-Van, T.-A.; Yu, T.; Hirose, S. On the high temperature mechanical behaviors analysis of heated functionally graded plates using FEM and a new third-order shear deformation plate theory. Compos. Part B Eng. 2016, 92, 218–224. [Google Scholar] [CrossRef]
- Javani, M.; Kiani, Y.; Eslami, M. Thermal buckling of FG graphene platelet reinforced composite annular sector plates. Thin Walled Struct. 2020, 148, 106589. [Google Scholar] [CrossRef]
- Kołakowski, Z.; Teter, A. Load carrying capacity of functionally graded columns with open cross-sections under static compression. Compos. Struct. 2015, 129, 1–7. [Google Scholar] [CrossRef]
- Debski, H.; Jonak, J. Failure analysis of thin-walled composite channel section columns. Compos. Struct. 2015, 132, 567–574. [Google Scholar] [CrossRef]
- Zaczynska, M.; Kolakowski, Z. The influence of the internal forces of the buckling modes on the load-carrying capacity of composite medium-length beams under bending. Materials 2020, 13, 455. [Google Scholar] [CrossRef] [Green Version]
- Urbaniak, M.; Teter, A.; Kubiak, T. Influence of boundary conditions on the critical and failure load in the GFPR channel cross-section columns subjected to compression. Compos. Struct. 2015, 134, 199–208. [Google Scholar] [CrossRef]
- Czechowski, L.; Kędziora, S.; Kołakowski, Z. The buckling and post-buckling of steel c-columns in elevated temperature. Materials 2019, 13, 74. [Google Scholar] [CrossRef] [Green Version]
- Czechowski, L.; Gliszczyński, A.; Wiącek, N. The collapse of titanium c-column due to thermal compression. Materials 2020, 13, 4193. [Google Scholar] [CrossRef]
- Aramis 4M. GOM Aramis 2020; GOM: Braunschweig, Germany, 2012. [Google Scholar]
- Hill, R. The Mathematical Theory of Plasticity; Oxford University Press: New York, NY, USA, 1983. [Google Scholar]
- Chen, W.F.; Han, D.J. Plasticity for Structural Engineers; Springer: New York, NY, USA, 1988. [Google Scholar]
- Von Mises, R. Mechanik der festen Körper im plastisch deformablen Zustand. Göttin. Nach. Math. Phys. 1913, 1, 582–592. [Google Scholar]
- Janik, M.; Dyja, H.; Berski, S.; Banaszek, G. Two-dimensional thermomechanical analysis of continuous casting process. J. Mater. Process. Technol. 2004, 153, 578–582. [Google Scholar] [CrossRef]
- Basquin, O. Tangent Modulus and the Strength of Steel Columns in Tests; U.S. Government Printing Office: Washington, DC, USA, 1924.
- Kamocka, M.; Mania, R. Post-buckling response of FML column with delamination. Compos. Struct. 2019, 230, 111511. [Google Scholar] [CrossRef]
- Wang, T.; Chiesa, L.; Takayasu, M. Fundamental simulations of transverse load effects on Nb3Sn strands using finite element analysis. AIP Conf. Proc. 2012, 243, 243–251. [Google Scholar] [CrossRef]
- ISO. ISO 6892-1:2019 Metallic Materials—Tensile Testing—Part 1: Method of Test at Room Temperature; ISO: Geneva, Switzerland, 2019. [Google Scholar]
- ISO. ISO 6892-2:2018 Metallic Materials—Tensile Testing—Part 2: Method of Test at Elevated Temperature; ISO: Geneva, Switzerland, 2018. [Google Scholar]
- Kaufman, J.G. Properties of Aluminum Alloys: Tensile, Creep, and Fatigue Data at High and Low Temperatures; ASM International: Novelty, OH, USA, 1999. [Google Scholar]
- Anys. User’s Guide ANSYS 18.2; Ansys Inc.: Houston, TX, USA, 2017. [Google Scholar]
- Gliszczyński, A.; Kubiak, T. Load-carrying capacity of thin-walled composite beams subjected to pure bending. Thin Walled Struct. 2017, 115, 76–85. [Google Scholar] [CrossRef]
- Kubiak, T.; Kolakowski, Z.; Swiniarski, J.; Urbaniak, J.; Gliszczynski, A. Local buckling and post-buckling of composite channel-section beams—Numerical and experimental investigations. Compos. Part B Eng. 2016, 91, 176–188. [Google Scholar] [CrossRef]
- Gliszczyński, A.; Czechowski, L. Collapse of channel section composite profile subjected to bending. Part I: Numerical investigations. Compos. Struct. 2017, 178, 383–394. [Google Scholar] [CrossRef]
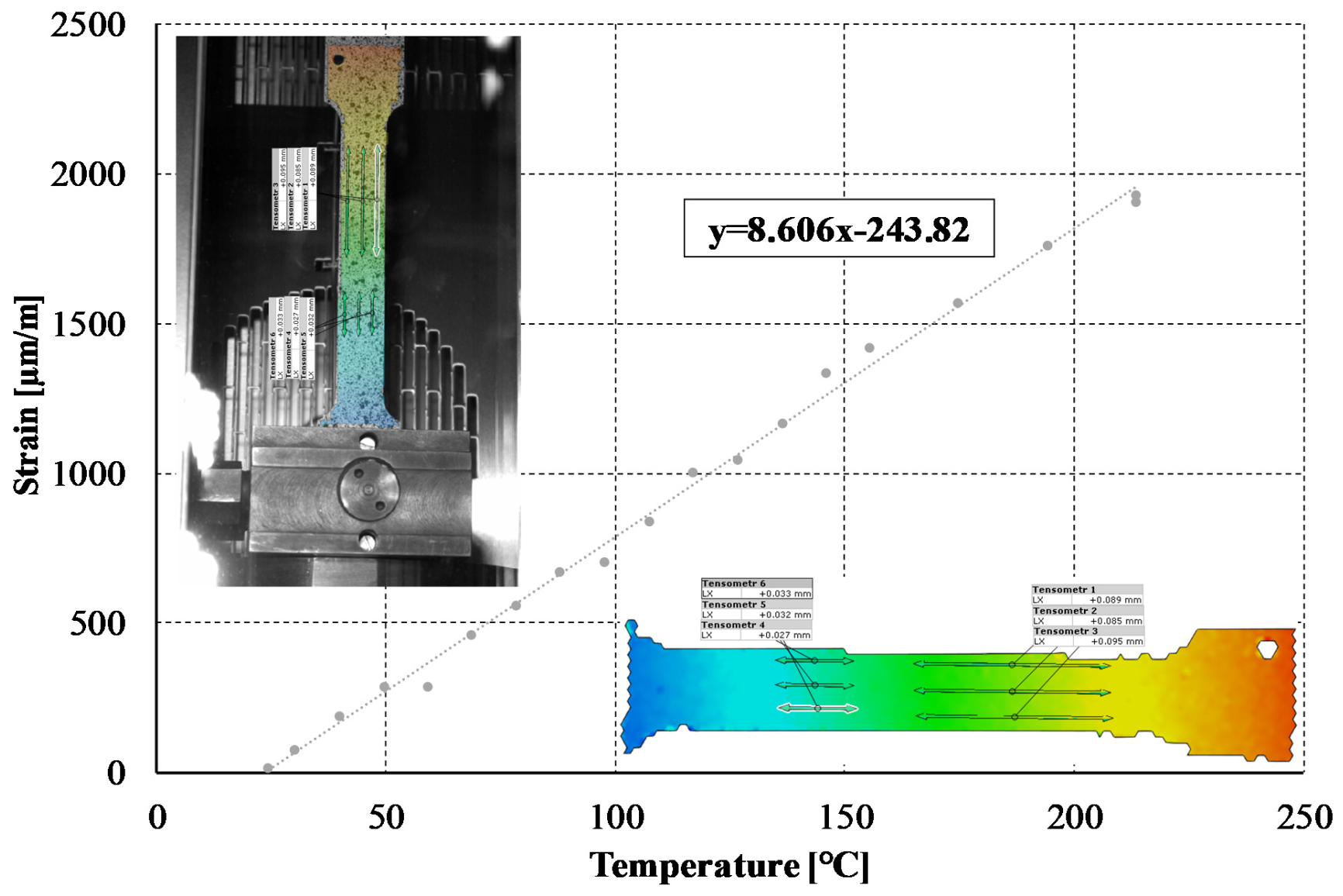
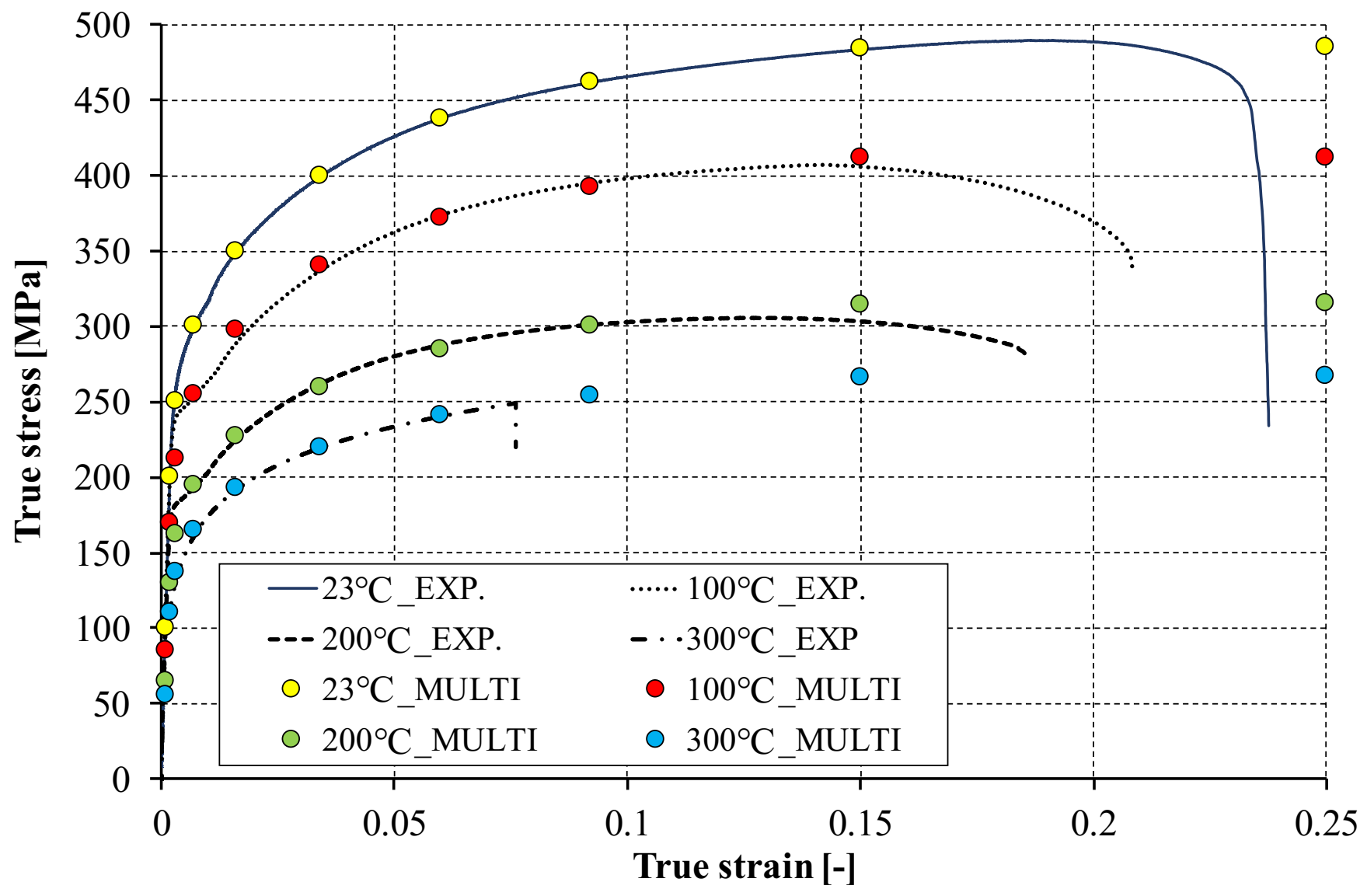






| E [MPa] | ν [-] | α [1/K × 10−6] | E* [MPa] |
|---|---|---|---|
| 109,300 | 0.34 | 9.2 | 1093 and 109.3 |
| 23 °C | 50 °C | 75 °C | 100 °C | 125 °C | 150 °C | 175 °C | 200 °C | 225 °C | 250 °C | 275 °C | 300 °C | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| σ0 | 240 | 228 | 216 | 204 | 192 | 180 | 168 | 156 | 150 | 144 | 141 | 132 |
| E | 109.3 | 108.4 | 107.2 | 105.7 | 103.7 | 101.4 | 98.7 | 95.6 | 92.1 | 88.3 | 84.1 | 79.5 |
| 23 °C | 50 °C | 75 °C | 100 °C | 125 °C | 150 °C | 175 °C | 200 °C | 225 °C | 250 °C | 275 °C | 300 °C | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| True strain [×10−2] | True stress [MPa] | |||||||||||
| 0.091 | 100 | 95 | 90 | 85 | 80 | 75 | 70 | 65 | 63 | 60 | 59 | 55 |
| 0.177 | 200 | 190 | 180 | 170 | 160 | 150 | 140 | 130 | 125 | 120 | 118 | 110 |
| 0.227 | 250 | 238 | 225 | 213 | 200 | 188 | 175 | 163 | 156 | 150 | 147 | 138 |
| 0.7 | 300 | 285 | 270 | 255 | 240 | 225 | 210 | 195 | 188 | 180 | 177 | 165 |
| 1.6 | 350 | 333 | 315 | 298 | 280 | 263 | 245 | 228 | 219 | 210 | 206 | 193 |
| 3.4 | 400 | 380 | 360 | 340 | 320 | 300 | 280 | 260 | 250 | 240 | 236 | 220 |
| 6 | 438 | 416 | 394 | 372 | 350 | 329 | 307 | 285 | 274 | 263 | 258 | 241 |
| 9.2 | 462 | 439 | 416 | 393 | 370 | 347 | 323 | 300 | 289 | 277 | 272 | 254 |
| 15 | 484 | 460 | 436 | 411 | 387 | 363 | 339 | 315 | 303 | 290 | 285 | 266 |
| 25 | 485 | 461 | 437 | 412 | 388 | 364 | 340 | 315 | 303 | 291 | 286 | 267 |
| 1st–M1 | 2nd–M2 | 3rd | |
|---|---|---|---|
| Pcr [kN] | 24.416 | 25.091 | 29.806 |
| m [-] | 2 | 3 | 4 |
| Isotropic Hardening | Bilinear–BILI | Multilinear–MULTI | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Mode: | - | 1st [m = 2]–M1 | 2nd [m = 3]–M2 | - | 1st [m = 2]–M1 | 2nd [m = 3]–M2 | ||||
| Imperfection: | NO IMPER | 0.01 × t | 0.1 × t | 0.01 × t | 0.1 × t | NO IMPER | 0.01 × t | 0.1 × t | 0.01 × t | 0.1 × t |
| Tangent modulus–E* | 0.001 × E and 0.01 × E | 0.001 × E and 0.01 × E | 0.001 × E and 0.01 × E | 0.001 × E and 0.01 × E | 0.001 × E and 0.01 × E | N/A | N/A | N/A | N/A | N/A |
| FEM | DIC Aramis® | UTM | |
|---|---|---|---|
| K [kN/mm] | ~105 | ~52 | ~33 |
| 50 °C | 75 °C | 100 °C | 125 °C | 150 °C | 175 °C | 200 °C | 225 °C | 250 °C | 275 °C | 300 °C |
|---|---|---|---|---|---|---|---|---|---|---|
 |  |  |  |  |  |  |  |  |  |  |
| 23 °C | 50 °C | 75 °C | 100 °C | 125 °C | 150 °C | 175 °C | 200 °C | 225 °C | 250 °C | 275 °C | 300 °C |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 36.9 | 33.3 | 32.1 | 30.3 | 27.5 | 26.1 | 24.2 | 23.2 | 22.6 | 21.5 | 20.7 | 19.1 |
| Isotropic Hardening: | Bilinear | Multilinear | Exp. | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mode: | - | 1st [m = 2] | 2nd [m = 3] | - | 1st [m = 2] | 2nd [m = 3] | N/A | ||||||
| Imperfection: | - | 0.01 × t | 0.1 × t | 0.01 × t | 0.1 × t | - | 0.01 × t | 0.1 × t | 0.01 × t | 0.1 × t | N/A | ||
| 23 °C | 3 | 2 | 2 | 3 | 3 | 3 | 2 | 2 | 3 | 3 | 3 | 2 | 2 |
| 50 °C | 3 | 2 | 2 | 3 | 3 | 3 | 2 | 2 | 3 | 3 | 3 | ||
| 75 °C | 3 | 2 | 2 | 3 | 3 | 3 | 2 | 2 | 3 | 3 | 3 | ||
| 100 °C | 3 | 2 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 2 | ||
| 125 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 2 | ||
| 150 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 3 | ||
| 175 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 3 | ||
| 200 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 3 | ||
| 225 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 3 | ||
| 250 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 2 | ||
| 275 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 2 | ||
| 300 °C | 3 | 3 | 2 | 3 | 3 | 3 | 3 | 2 | 3 | 3 | 2 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gliszczyński, A.; Czechowski, L.; Wiącek, N. Heating and Compression at Elevated Temperature of Thin-Walled Titanium Channel Section Columns. Materials 2021, 14, 2928. https://doi.org/10.3390/ma14112928
Gliszczyński A, Czechowski L, Wiącek N. Heating and Compression at Elevated Temperature of Thin-Walled Titanium Channel Section Columns. Materials. 2021; 14(11):2928. https://doi.org/10.3390/ma14112928
Chicago/Turabian StyleGliszczyński, Adrian, Leszek Czechowski, and Nina Wiącek. 2021. "Heating and Compression at Elevated Temperature of Thin-Walled Titanium Channel Section Columns" Materials 14, no. 11: 2928. https://doi.org/10.3390/ma14112928
APA StyleGliszczyński, A., Czechowski, L., & Wiącek, N. (2021). Heating and Compression at Elevated Temperature of Thin-Walled Titanium Channel Section Columns. Materials, 14(11), 2928. https://doi.org/10.3390/ma14112928






