Modelling of High Velocity Impact on Concrete Structures Using a Rate-Dependent Plastic-Damage Microplane Approach at Finite Strains
Abstract
1. Introduction
2. Material Constitutive Laws at Finite Strains
2.1. Microplane Approach with V-D Split
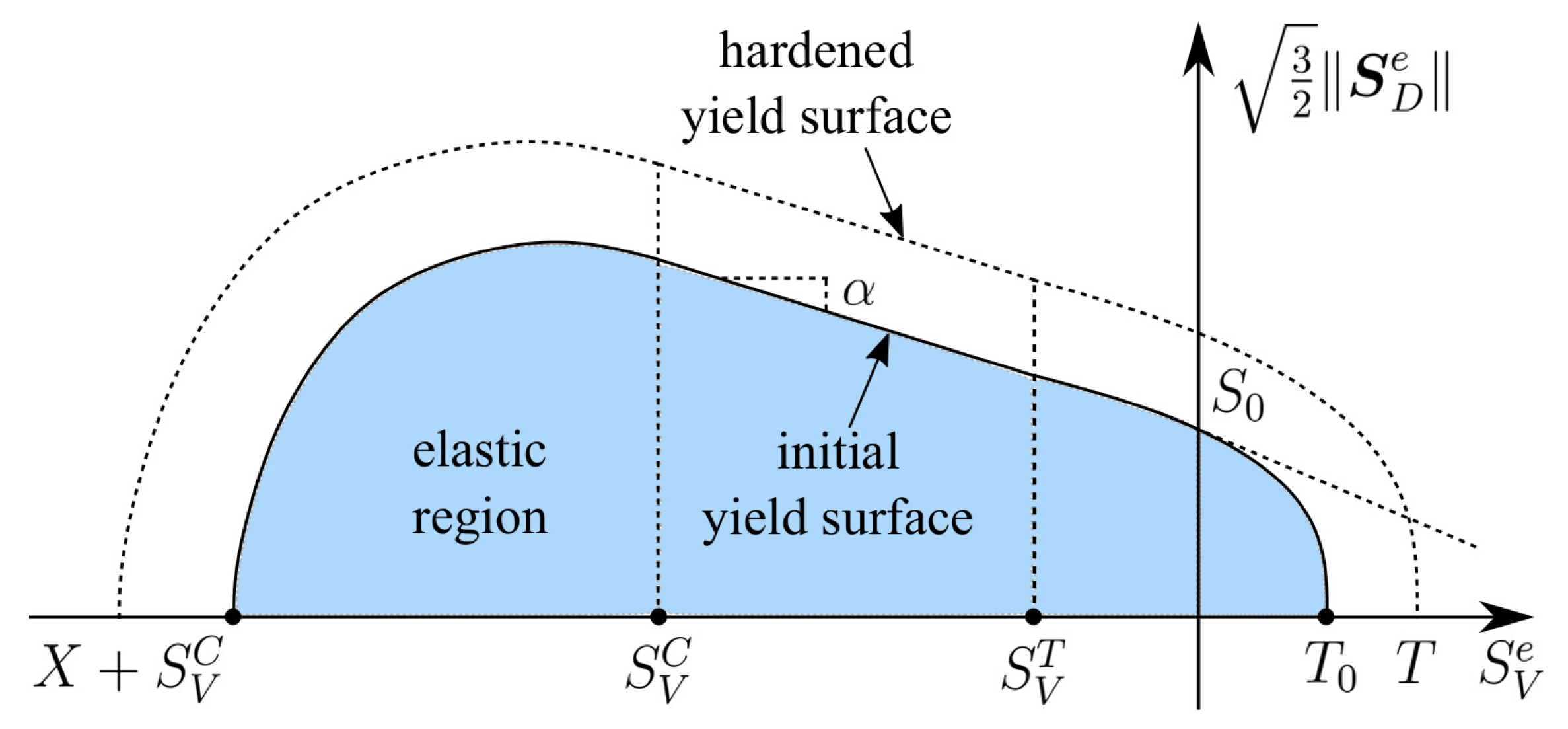
2.2. Modified Smooth Three Surface Drucker–Prager Yield Criterion
2.3. Damage Evolution Law
2.4. Rate Dependency
2.5. Implicit Gradient Enhancement
3. Algorithmic Aspects
3.1. Finite Element Formulation
3.2. Stress Return Algorithm
4. Penetration Model
4.1. Contact Mechanism
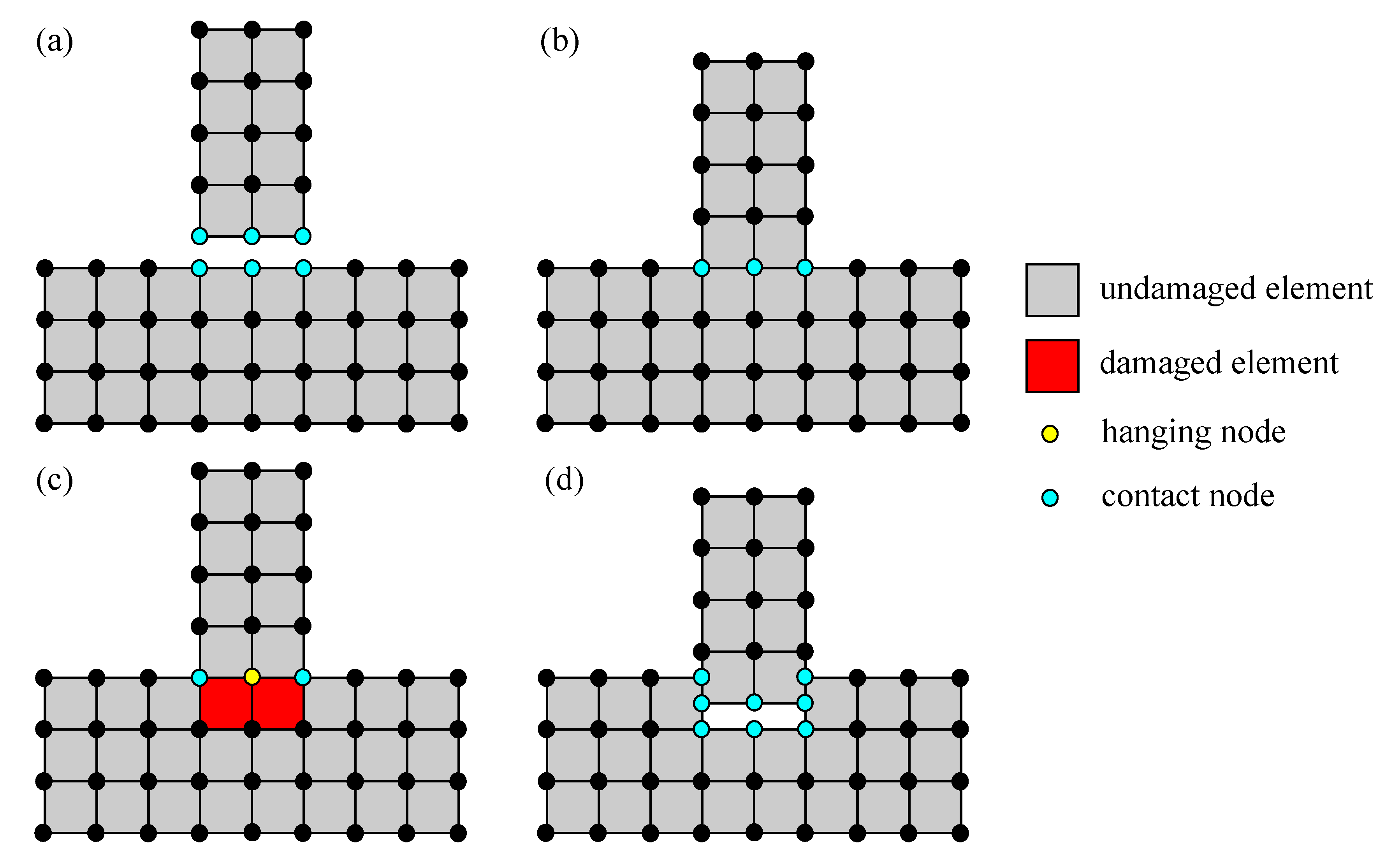
4.2. Adaptive Element Erosion
4.3. Coupled Contact and Adaptive Element Erosion
5. Model Parameters Adjustment
5.1. Four-Point Bending Test at Cyclic Loading
5.2. Homogeneous Test
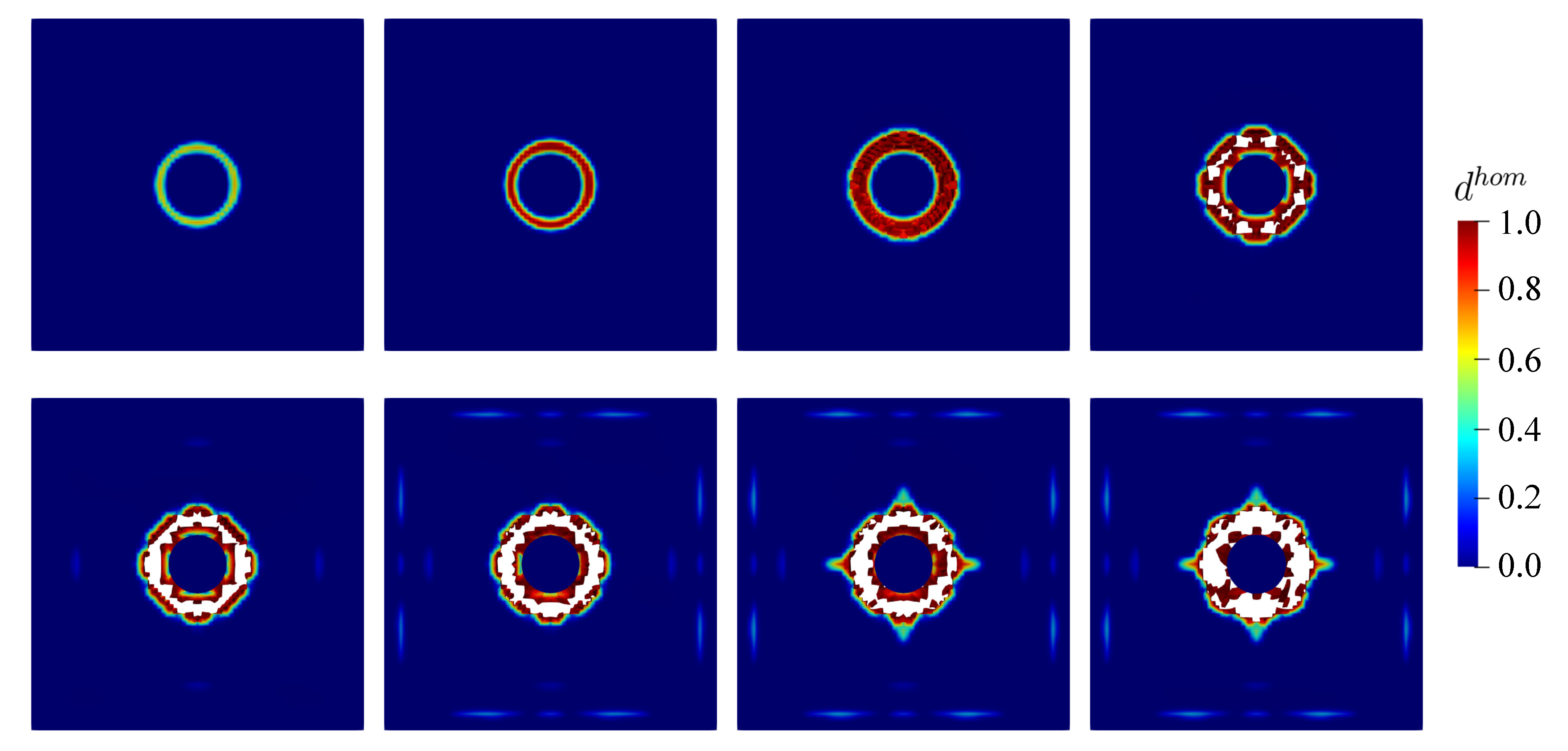
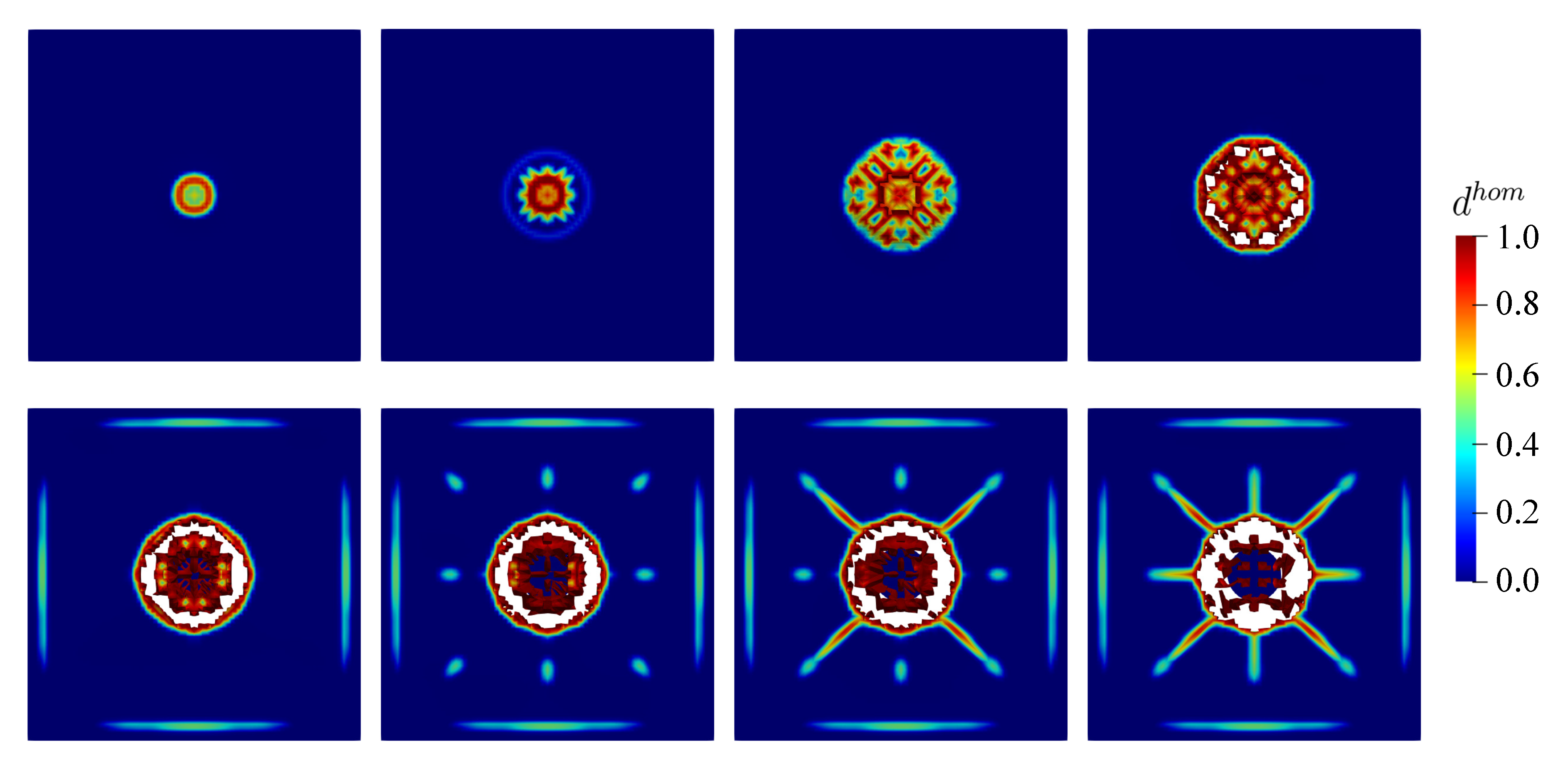
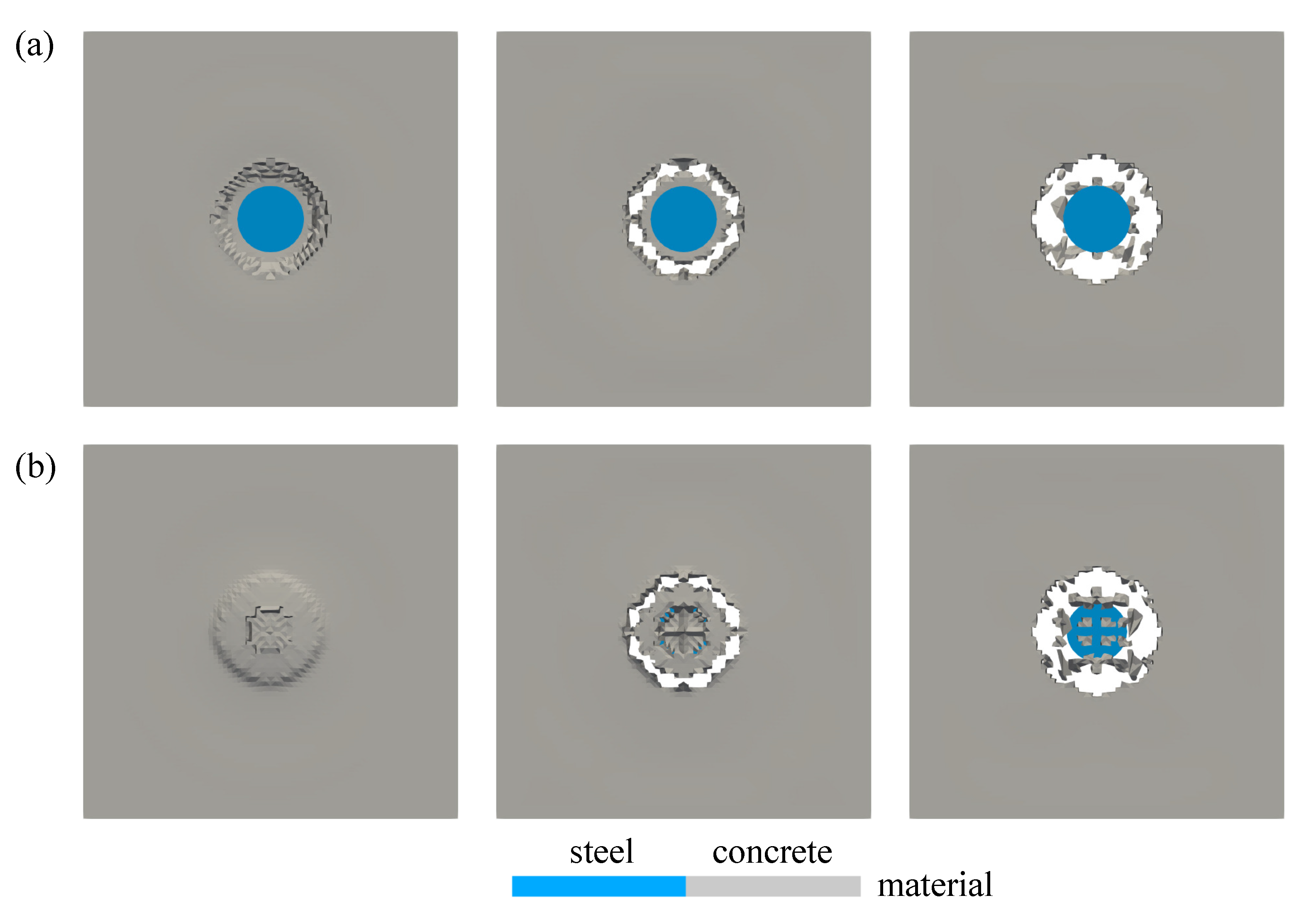
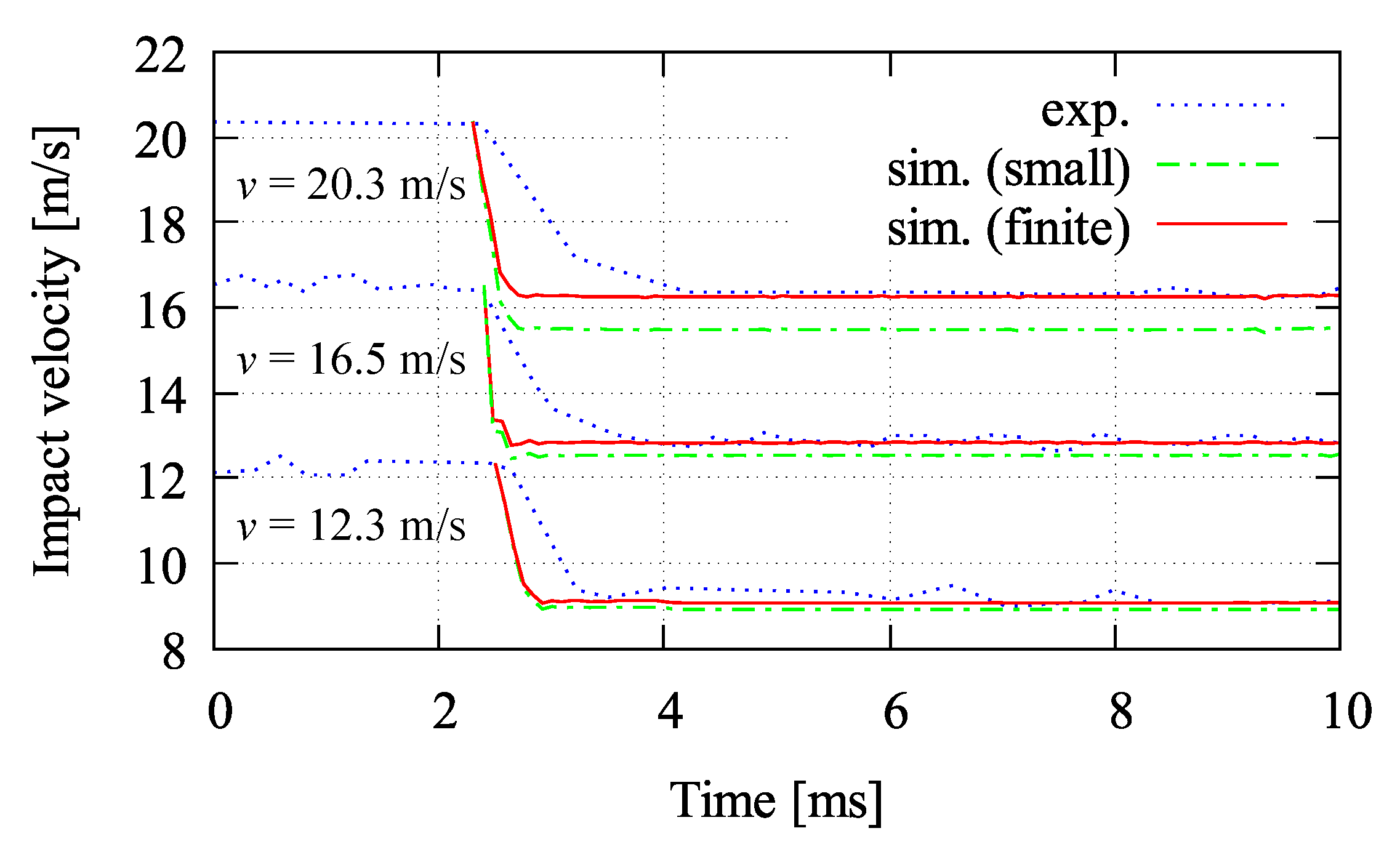
6. Numerical Examples
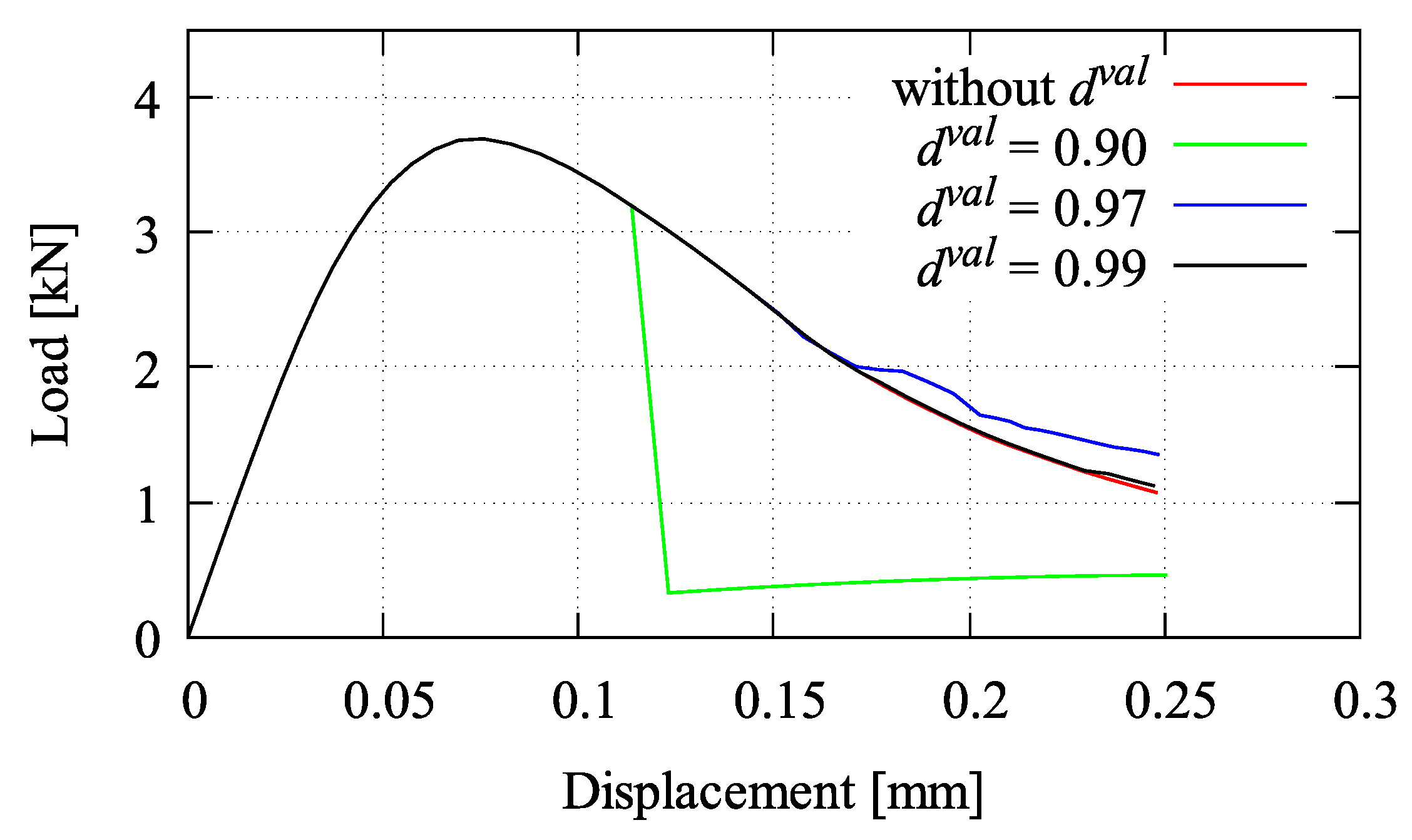
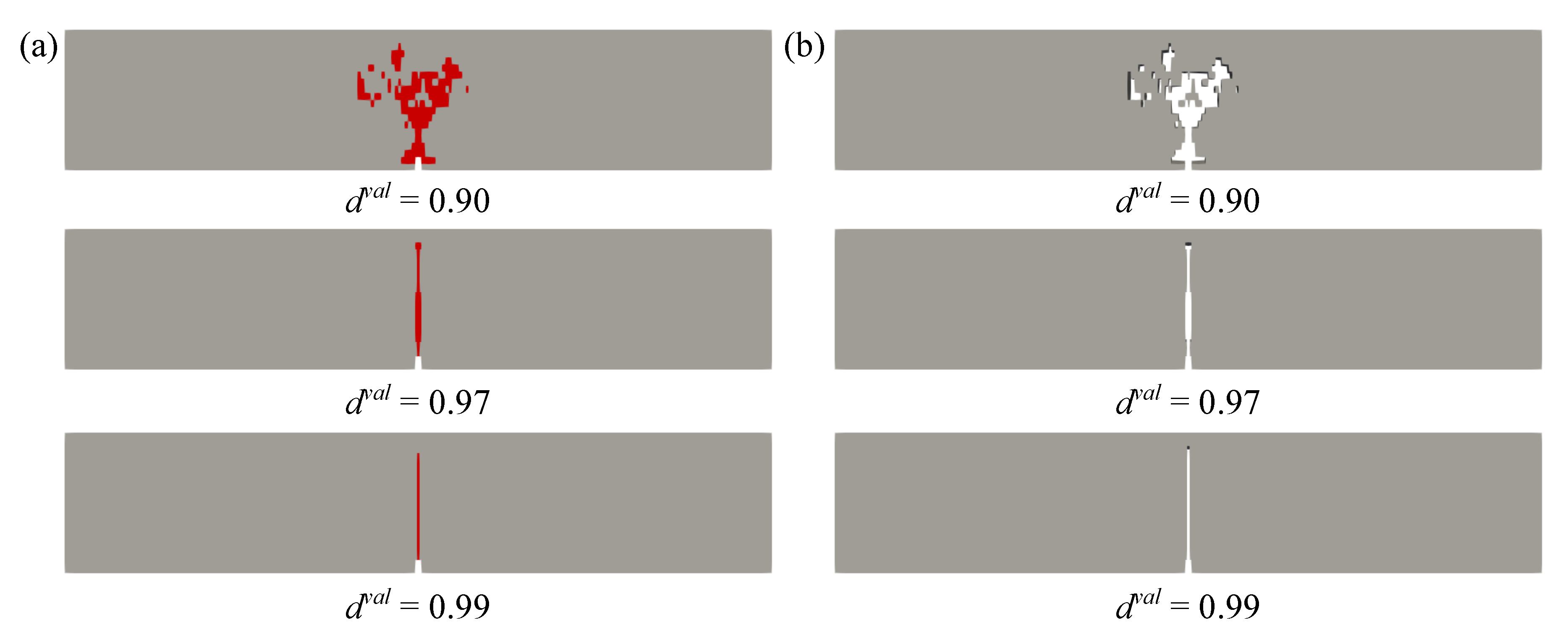
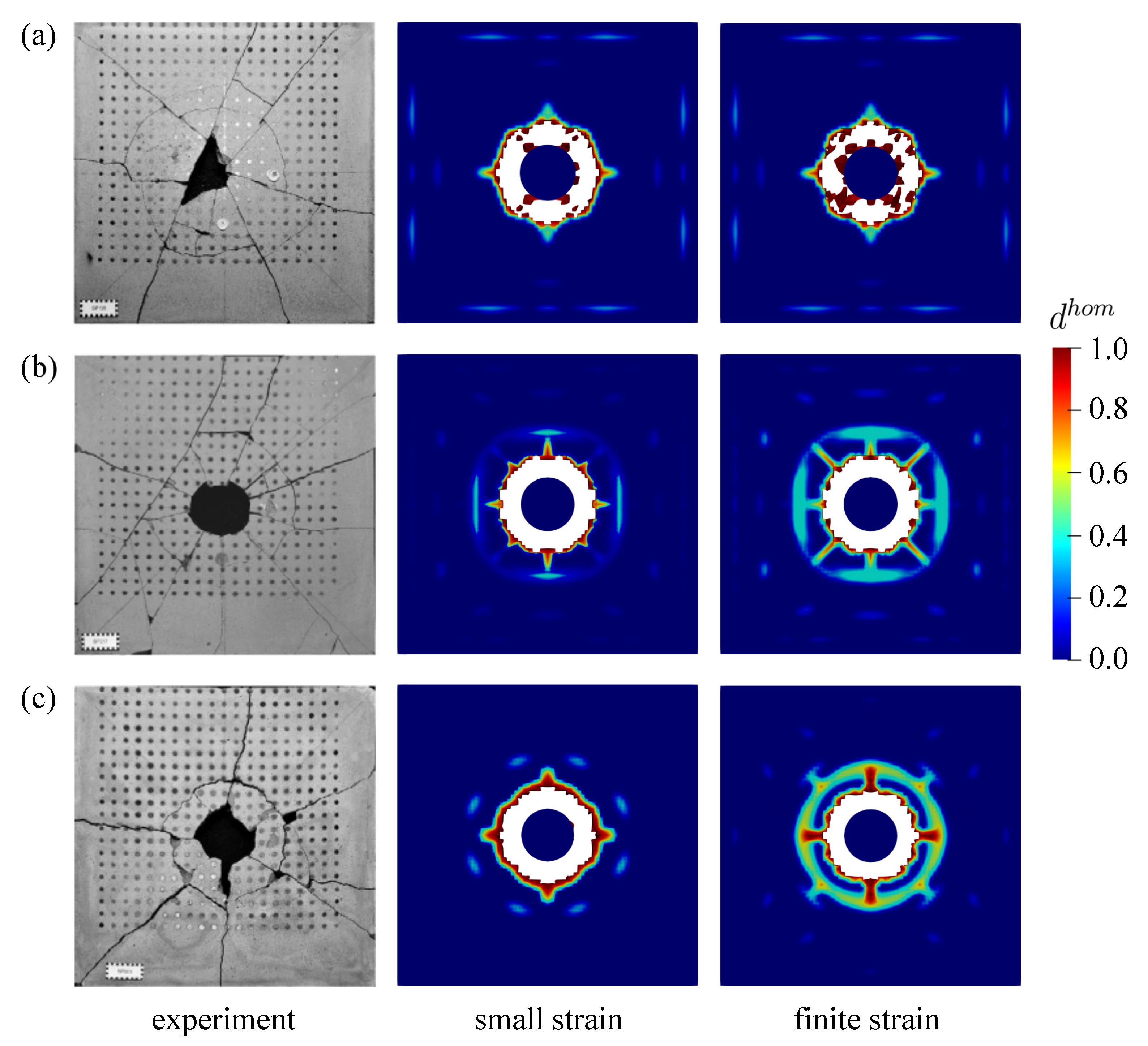
6.1. Thick Plate Simulation
6.2. Small Thin Plate Simulation
7. Conclusions and Outlook
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Algorithmic Tangent
Appendix A.1. Effective Elastoplastic Tangent
Appendix A.2. Total Tangent
References
- Cicekli, U.; Voyiadjis, G.Z.; Al-Rub, R.K.A. A plasticity and anisotropic damage model for plain concrete. Int. J. Plast. 2007, 23, 1874–1900. [Google Scholar] [CrossRef]
- Grassl, P.; Jirásek, M. Damage-plastic model for concrete failure. Int. J. Solids Struct. 2006, 43, 7166–7196. [Google Scholar] [CrossRef]
- Grassl, P.; Xenos, D.; Nyström, U.; Rempling, R.; Gylltoft, K. CDPM2: A damage-plasticity approach to modelling the failure of concrete. Int. J. Solids Struct. 2013, 50, 3805–3816. [Google Scholar] [CrossRef]
- Jason, L.; Huerta, A.; Pijaudier-Cabot, G.; Ghavamian, S. An elastic plastic damage formulation for concrete: Application to elementary tests and comparison with an isotropic damage model. Comput. Methods Appl. Mech. Eng. 2006, 195, 7077–7092. [Google Scholar] [CrossRef]
- Lee, J.; Fenves, G.L. Plastic-damage model for cyclic loading of concrete structures. J. Eng. Mech. 1998, 124, 892–900. [Google Scholar] [CrossRef]
- Meschke, G.; Lackner, R.; Mang, H.A. An anisotropic elastoplastic-damage model for plain concrete. Int. J. Numer. Methods Eng. 1998, 42, 703–727. [Google Scholar] [CrossRef]
- Nguyen, G.D.; Houlsby, G.T. A coupled damage-plasticity model for concrete based on thermodynamic principles: Part I: Model formulation and parameter identification. Int. J. Numer. Anal. Methods Geomech. 2008, 32, 353–389. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Taqieddin, Z.N.; Kattan, P.I. Anisotropic damage-plasticity model for concrete. Int. J. Plast. 2008, 24, 1946–1965. [Google Scholar] [CrossRef]
- Zhang, J.; Li, J.; Ju, J.W. 3D elastoplastic damage model for concrete based on novel decomposition of stress. Int. J. Solids Struct. 2016, 94, 125–137. [Google Scholar] [CrossRef]
- Ahad, F.R.; Enakoutsa, K.; Solanki, K.N.; Bammann, D.J. Nonlocal modelling in high-velocity impact failure of 6061-T6 aluminum. Int. J. Plast. 2014, 55, 108–132. [Google Scholar] [CrossRef]
- Majorana, C.E.; Salomoni, V.A.; Mazzucco, G.; Khoury, G.A. An approach for modelling concrete spalling in finite strains. Math. Comput. Simul. 2010, 80, 1694–1712. [Google Scholar] [CrossRef]
- Nguyen, G.D.; Korsunsky, A.M.; Belnoue, J.P.H. A nonlocal coupled damage-plasticity model for the analysis of ductile failure. Int. J. Plast. 2015, 64, 56–75. [Google Scholar] [CrossRef]
- Zhu, Q.Z.; Shao, J.F.; Mainguy, M. A micromechanics-based elastoplastic damage model for granular materials at low confining pressure. Int. J. Plast. 2010, 26, 586–602. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Oh, B. Microplane model for concrete for fracture analysis of concrete structures. In Proceedings of the Symposium on the Interaction of Non-nuclear Munitions with Structures, U.S. Air Force Academy, Colorado Springs, Air Force Academy, CO, USA, 10–13 May 1983; pp. 49–53. [Google Scholar]
- Bažant, Z.P.; Gambarova, P.G. Crack shear in concrete: Crack band microplane model. J. Struct. Eng. 1984, 110, 2015–2035. [Google Scholar] [CrossRef]
- Caner, F.; Bažant, Z.P. Microplane model M7 for plain concrete. I: Formulation. J. Eng. Mech. 2013, 139, 1714–1723. [Google Scholar] [CrossRef]
- Kuhl, E.; Ramm, E. Microplane modelling of cohesive frictional materials. Eur. J. Mech. A Solids 2000, 19, S121–S143. [Google Scholar]
- Kuhl, E. Numerische Modelle Für Kohäsive Reibungsmaterialien. Ph.D. Thesis, Institut für Baustatik, Universität Stuttgart, Stuttgart, Germany, 2000. [Google Scholar]
- Leukart, M. Kombinierte Anisotrope Schädigung und Plastizität bei Kohäsiven Reibungsmaterialien. Ph.D. Thesis, Institut für Baustatik, Universität Stuttgart, Stuttgart, Germany, 2005. [Google Scholar]
- Peerlings, R.H.J.; de Borst, R.; Brekelmans, W.A.M.; de Vree, J.H.P. Gradient enhanced damage for quasi-brittle materials. Int. J. Numer. Methods Eng. 1996, 39, 3391–3403. [Google Scholar] [CrossRef]
- Peerlings, R.H.J.; Geers, M.G.D.; de Borst, R.; Brekelmans, W.A.M. A critical comparison of nonlocal and gradient-enhanced softening continua. Int. J. Solids Struct. 2001, 38, 7723–7746. [Google Scholar] [CrossRef]
- Zreid, I.; Kaliske, M. Regularization of microplane damage models using an implicit gradient enhancement. Int. J. Solids Struct. 2014, 51, 3480–3489. [Google Scholar] [CrossRef]
- Zreid, I.; Kaliske, M. An implicit gradient formulation for microplane Drucker-Prager plasticity. Int. J. Plast. 2016, 83, 252–272. [Google Scholar] [CrossRef]
- Zreid, I.; Kaliske, M. A gradient-enhanced plasticity-damage microplane model for concrete. Comput. Mech. 2018, 62, 1239–1257. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Kim, J.J.H.; Brocca, M. Finite strain tube-squash test of concrete at high pressures and shear angles up to 70 degrees. ACI Mater. J. 1999, 96, 580–592. [Google Scholar]
- Indriyantho, B.R.; Zreid, I.; Kaliske, M. Finite strain extension of a gradient enhanced microplane damage model for concrete at static and dynamic loading. Eng. Fract. Mech. 2019, 216, 106501. [Google Scholar] [CrossRef]
- Indriyantho, B.R.; Kaliske, M. A finite strain microplane-plasticity model for concrete. In Proceedings of the 8th GACM Colloquium on Computational Mechanics for Young Scientists from Academia and Industry, German Association for Computational Mechanics (GACM), Kassel, Germany, 28–30 August 2019; Kassel University Press: Kassel, Germany, 2019; pp. 39–42. [Google Scholar]
- Indriyantho, B.R.; Zreid, I.; Kaliske, M. A nonlocal softening plasticity based on microplane theory for concrete at finite strains. Comput. Struct. 2020, 241, 106333. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Adley, M.D.; Carol, I.; Jirásek, M.; Akers, S.A.; Rohani, B.; Cargile, J.D.; Caner, F.C. Large-strain generalization of microplane model for concrete and application. J. Eng. Mech. 2000, 126, 971–980. [Google Scholar] [CrossRef]
- Huang, F.; Wu, H.; Jin, Q.; Zhang, Q. A numerical simulation on the perforation of concrete targets. Int. J. Impact Eng. 2005, 32, 173–187. [Google Scholar] [CrossRef]
- Bažant, Z.P. Finite strain generalization of small-strain constitutive relations for any finite strain tensor and additive volumetic-deviatoric split. Int. J. Solids Struct. 1996, 33, 2887–2897. [Google Scholar] [CrossRef]
- Lee, E.H. Elastic-plastic deformation at finite strains. J. Appl. Mech. 1969, 36, 1–6. [Google Scholar] [CrossRef]
- Dolarevic, S.; Ibrahimbegovic, A. A modified three-surface elasto-plastic cap model and its numerical implementation. Comput. Struct. 2007, 85, 419–430. [Google Scholar] [CrossRef]
- Schwer, L.E.; Murray, Y.D. A three-invariant smooth cap model with mixed hardening. Int. J. Numer. Anal. Methods Geomech. 1994, 18, 657–688. [Google Scholar] [CrossRef]
- Jiang, H.; Zhao, J. Calibration of the continuous surface cap model for concrete. Finite Elem. Anal. Des. 2015, 97, 1–19. [Google Scholar] [CrossRef]
- Wang, W.M.; Sluys, L.J.; de Borst, R. Viscoplasticity for instabilities due to strain softening and strain-rate softening. Int. J. Numer. Methods Eng. 1997, 40, 3839–3864. [Google Scholar] [CrossRef]
- Fuchs, A.; Kaliske, M. A gradient enhanced viscoplasticity-damage microplane model for concrete at static and transient loading. In Proceedings of the 10th International Conference on Fracture Mechanics of Concrete and Concrete Structures, Bayonne, France, 23–26 June 2019; Pijaudier-Cabot, G., Grassl, P., La Borderie, C., Eds.; FraMCoS-X: Bayonne, France, 2019; pp. 1–8. [Google Scholar]
- Qinami, A.; Pandolfi, A.; Kaliske, M. Variational eigenerosion for rate-dependent plasticity in concrete modelling at small strain. Int. J. Numer. Methods Eng. 2020, 121, 1388–1409. [Google Scholar] [CrossRef]
- Grassl, P.; Jirásek, M. Plastic model with non-local damage applied to concrete. Int. J. Numer. Anal. Methods Geomech. 2006, 43, 71–90. [Google Scholar] [CrossRef]
- Poh, L.H.; Swaddiwudhipong, S. Over-nonlocal gradient enhanced plastic-damage model for concrete. Int. J. Solids Struct. 2009, 46, 4369–4378. [Google Scholar]
- Ožbolt, J.; Bošnjak, J.; Sola, E. Dynamic fracture of concrete compact tension specimen: Experimental and numerical study. Int. J. Solids Struct. 2013, 50, 4270–4278. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Pijaudier-Cabot, G. Measurement of characteristic length of nonlocal continuum. J. Eng. Mech. 1989, 115, 755–767. [Google Scholar] [CrossRef]
- Le Bellégo, C.; Dubé, J.F.; Pijaudier-Cabot, G.; Gérard, B. Calibration of nonlocal damage model from size effect tests. Eur. J. Mech. A Solids 2003, 22, 33–46. [Google Scholar] [CrossRef]
- Xenos, D.; Grégoire, D.; Morel, S.; Grassl, P. Calibration of nonlocal models for tensile fracture in quasi-brittle heterogeneous materials. J. Mech. Phys. Solids 2015, 82, 48–60. [Google Scholar] [CrossRef]
- Di Luzio, G. A symmetric over-nonlocal microplane model M4 for fracture in concrete. Int. J. Solids Struct. 2007, 44, 4418–4441. [Google Scholar] [CrossRef]
- Hordijk, D.A. Local Approach to Fatigue of Concrete. Ph.D. Thesis, Delft University of Technology, Delft, The Netherlands, 1991. [Google Scholar]
- Qinami, A.; Zreid, I.; Fleischhauer, R.; Kaliske, M. Modeling of impact on concrete plates by use of the microplane approach. Int. J. Non-Linear Mech. 2016, 80, 107–121. [Google Scholar] [CrossRef]
- Hering, M.; Kühn, T.; Curbach, M. Small-scale plate tests with fine concrete in experiment and first simplified simulation. Struct. Concr. 2020, 1–13. [Google Scholar] [CrossRef]



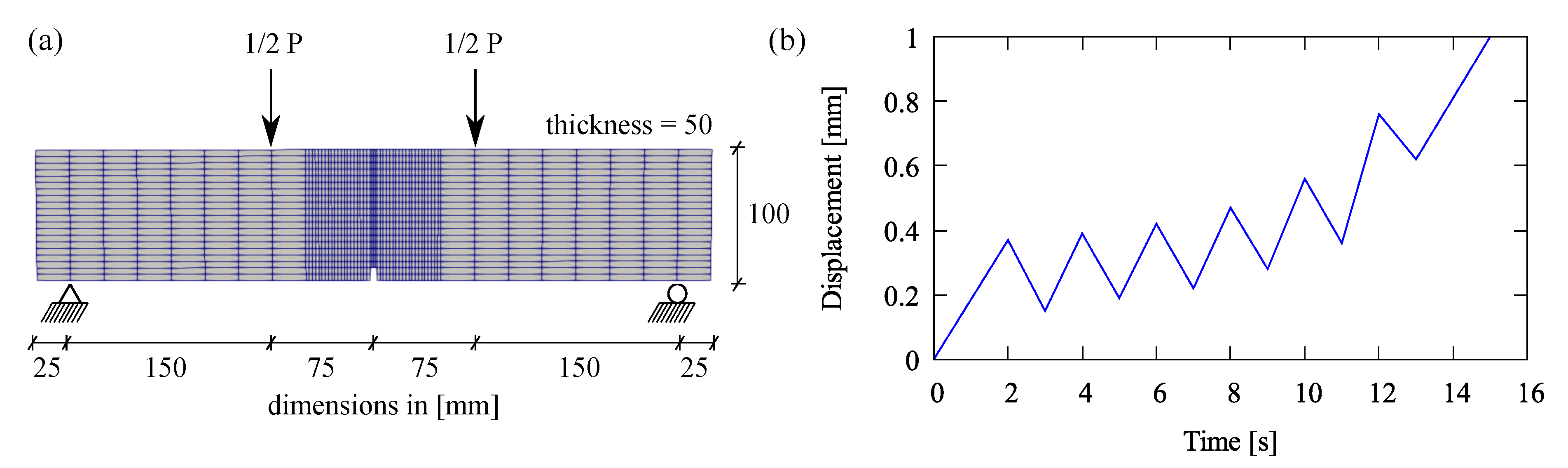
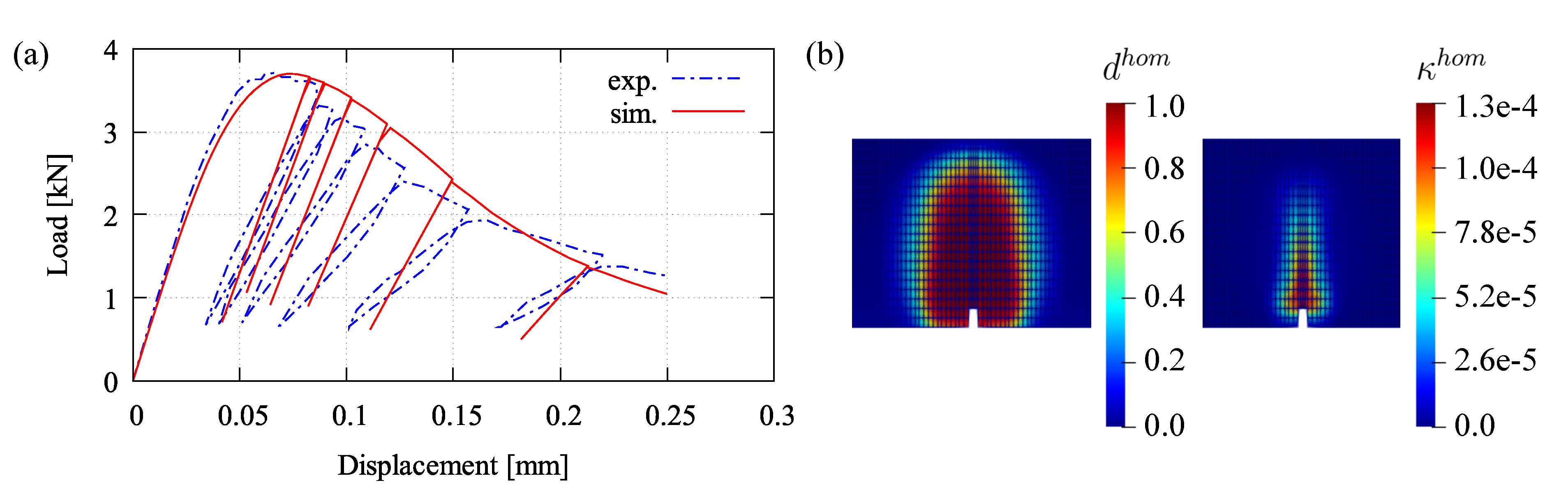


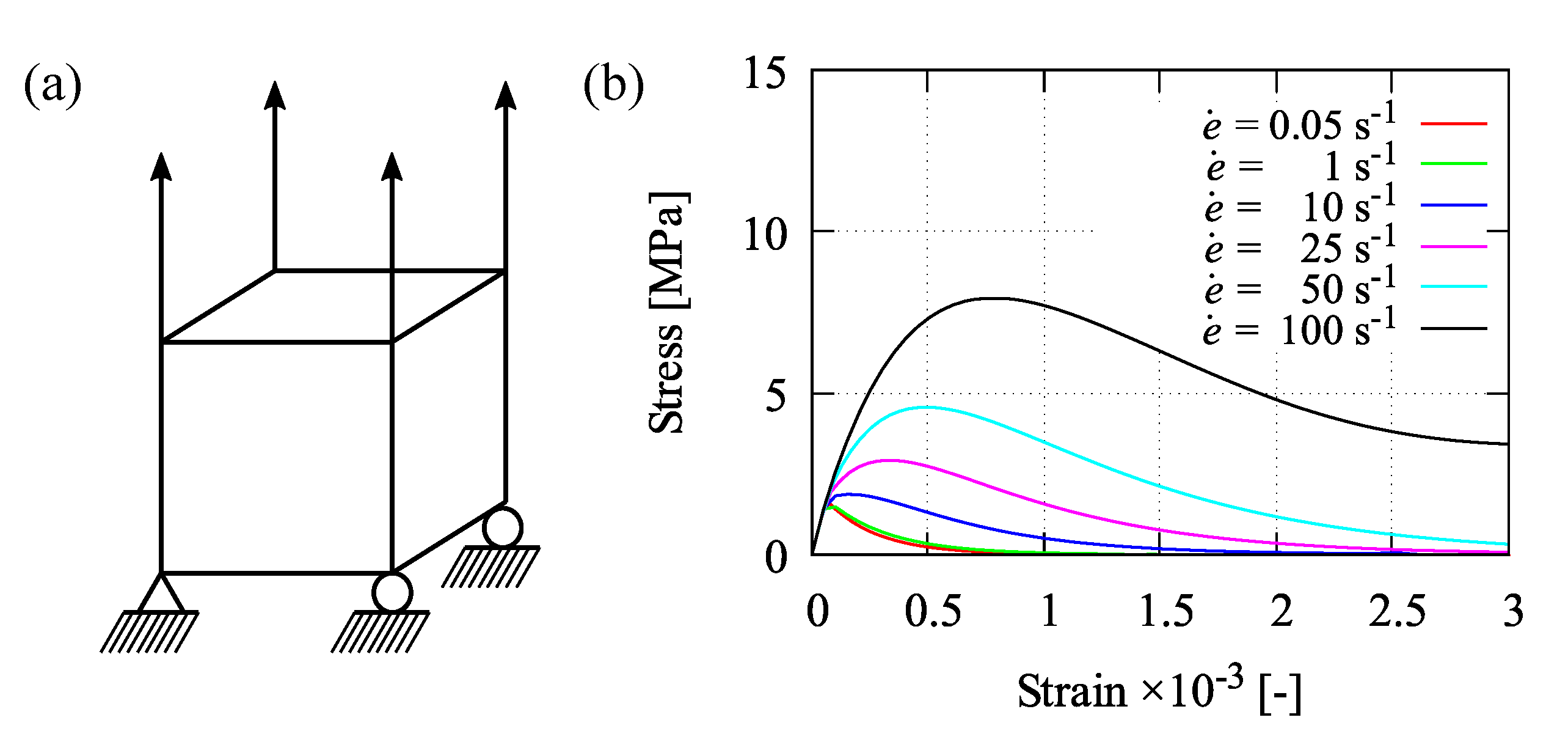

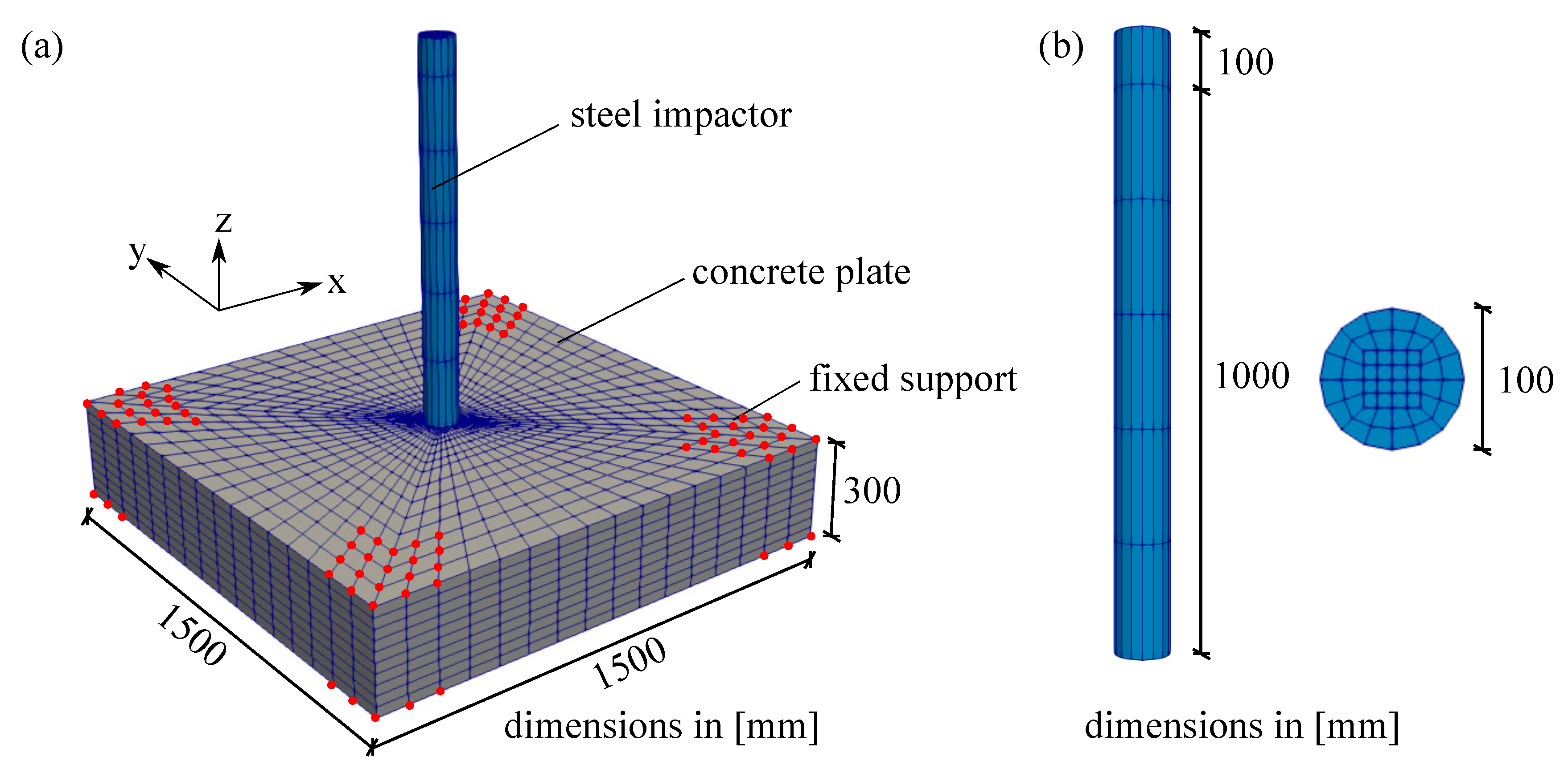


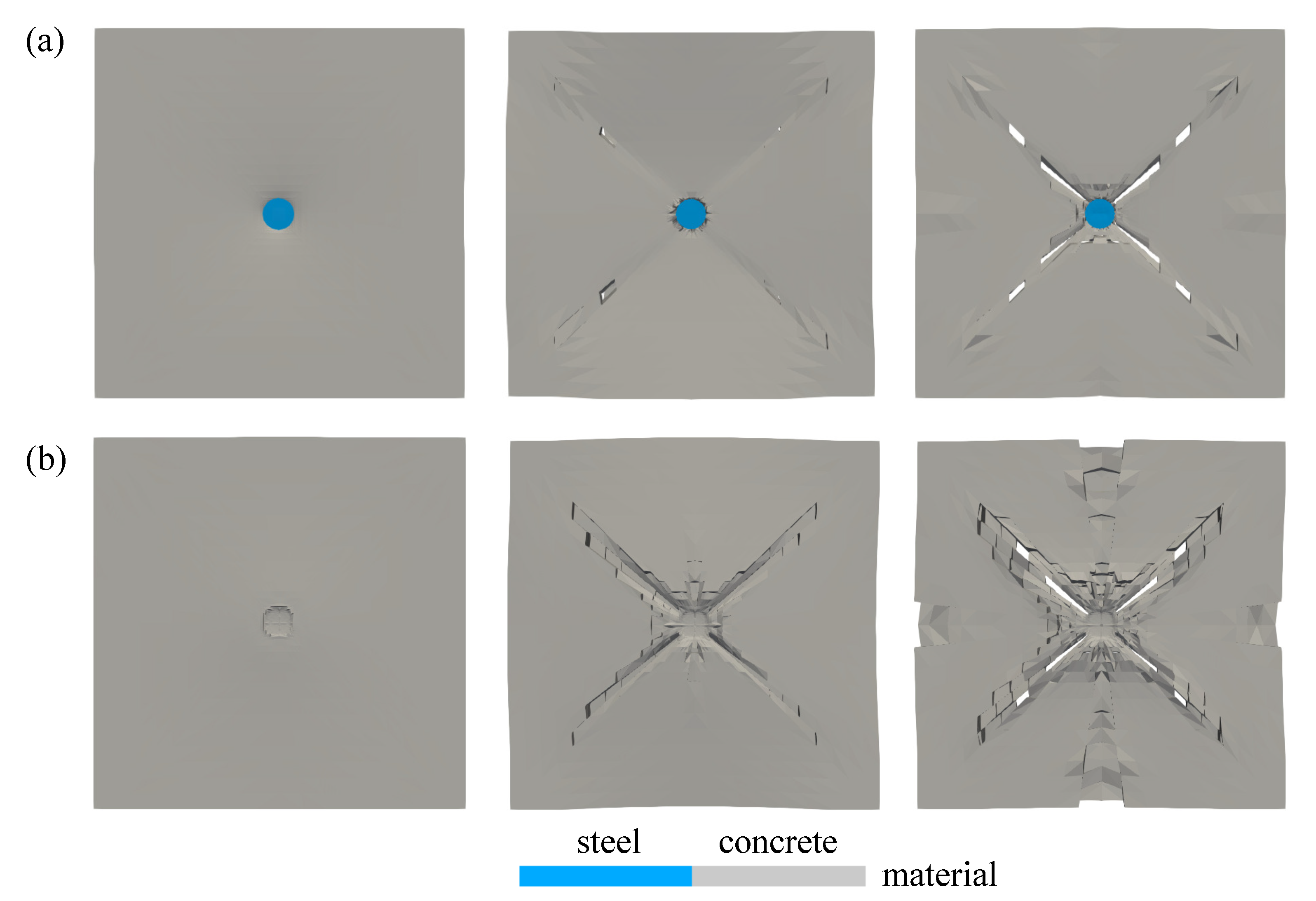
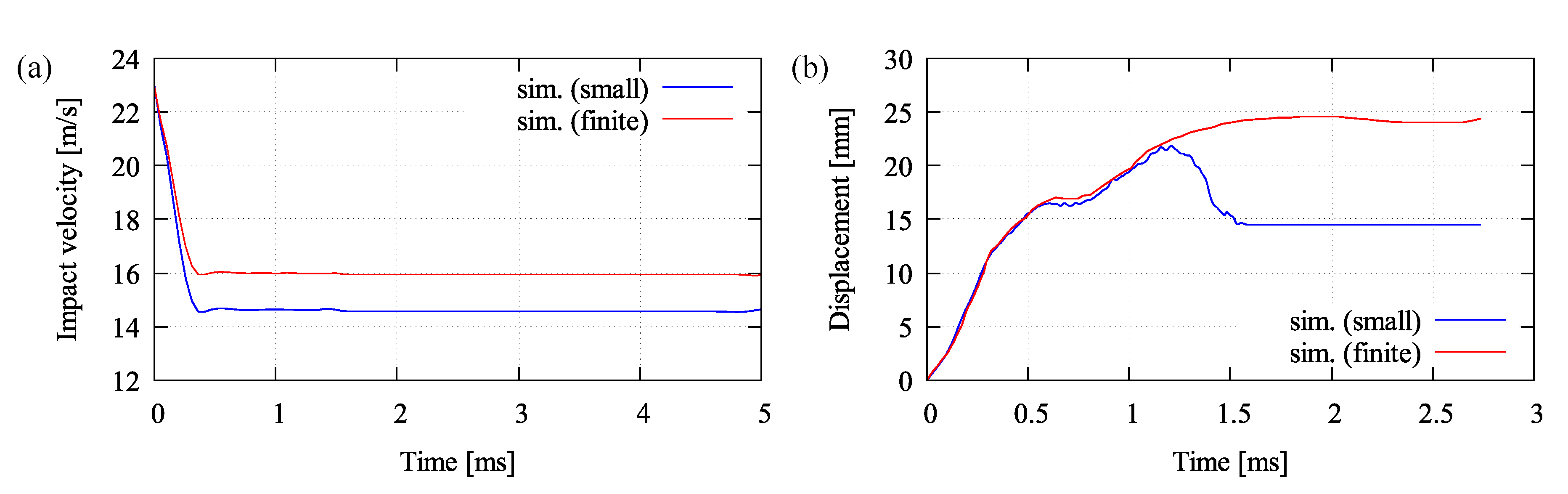

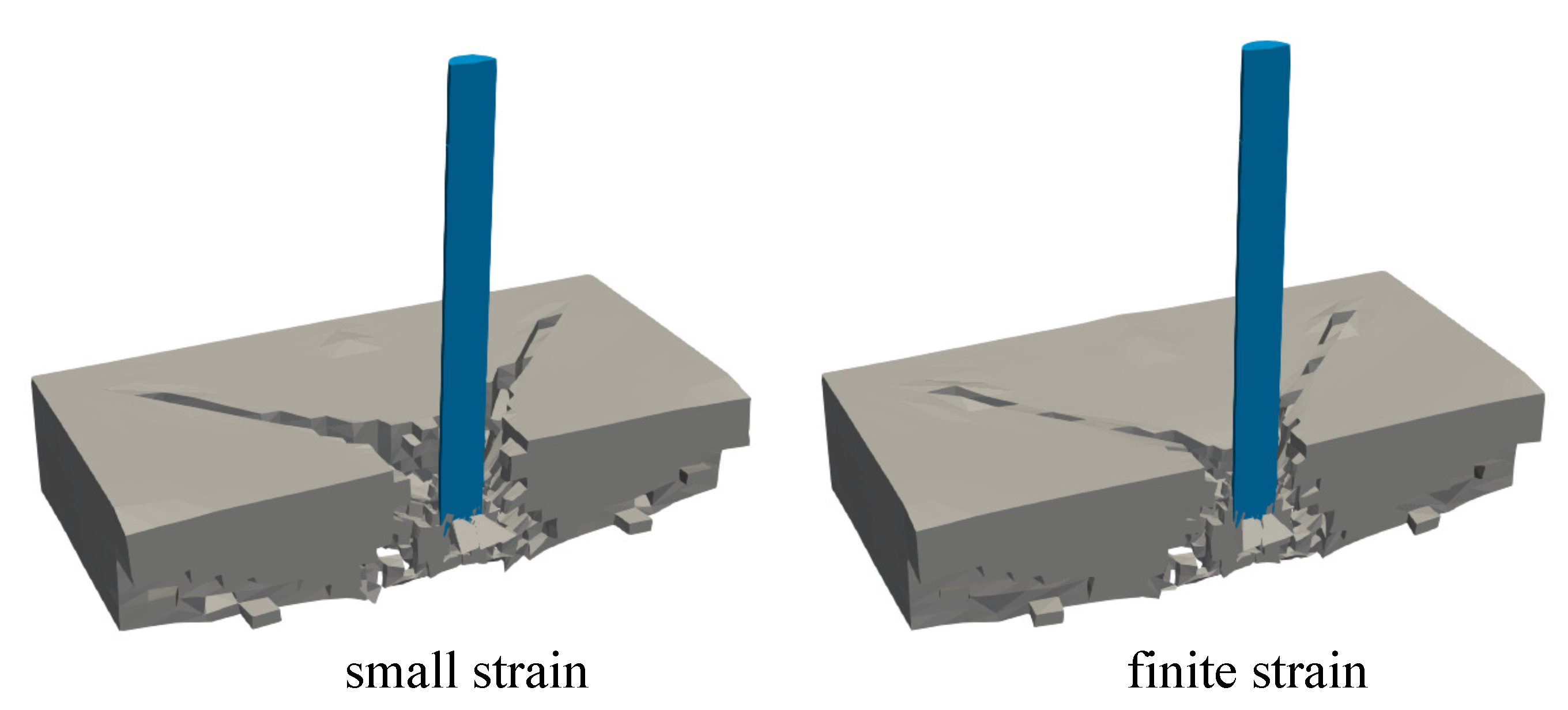
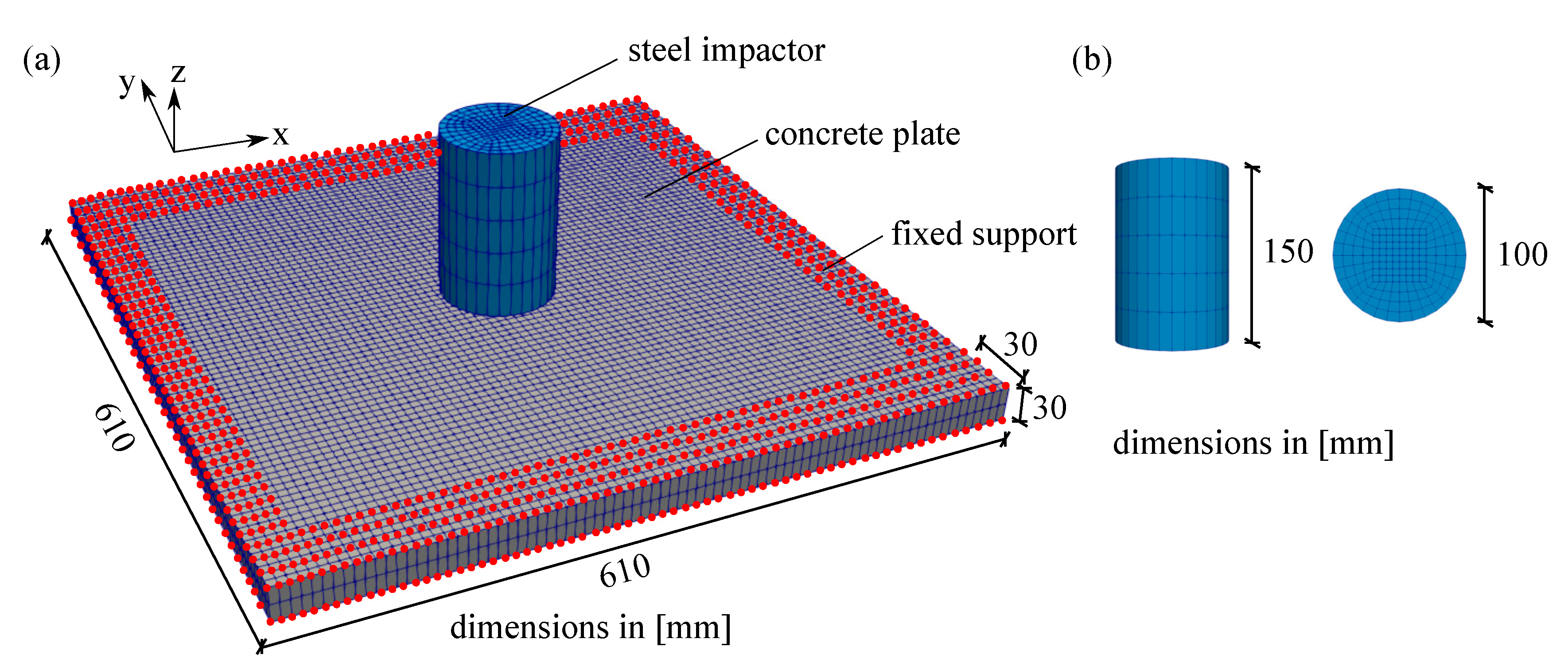


| Parameter | Concrete | |
|---|---|---|
| E | [MPa] | 38,000 |
| [-] | 0.2 | |
| [MPa] | 20 | |
| [-] | 1 | |
| D | [MPa] | |
| [MPa] | ||
| R | [-] | 2 |
| [-] | 0 | |
| [-] | ||
| [-] | ||
| [-] | ||
| c | [] | 20 |
| m | [-] | 2.5 |
| Parameter | Concrete Plate | Steel Impactor | |
|---|---|---|---|
| E | [MPa] | 40,000 | 210,000 |
| [-] | 0.2 | 0.3 | |
| [] | 2400 | 7850 | |
| [MPa] | 70 | - | |
| [-] | 1 | - | |
| D | [MPa] | - | |
| [MPa] | - | ||
| R | [-] | 2 | - |
| [-] | 0 | - | |
| [-] | - | ||
| [-] | - | ||
| [-] | - | ||
| c | [] | 50 | - |
| m | [-] | 2.5 | - |
| [s/MPa] | 1 | - | |
| Parameter | Concrete Plate | Steel Impactor | |
|---|---|---|---|
| E | [MPa] | 33,100 | 210,000 |
| [-] | 0.2 | 0.3 | |
| [] | 2150 | 8000 | |
| [MPa] | 95 | - | |
| [-] | 1 | - | |
| D | [MPa] | - | |
| [MPa] | - | ||
| R | [-] | 2 | - |
| [-] | 0 | - | |
| [-] | - | ||
| [-] | - | ||
| [-] | - | ||
| c | [] | 20 | - |
| m | [-] | 2.5 | - |
| [s/MPa] | 1 | - | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Indriyantho, B.R.; Zreid, I.; Fleischhauer, R.; Kaliske, M. Modelling of High Velocity Impact on Concrete Structures Using a Rate-Dependent Plastic-Damage Microplane Approach at Finite Strains. Materials 2020, 13, 5165. https://doi.org/10.3390/ma13225165
Indriyantho BR, Zreid I, Fleischhauer R, Kaliske M. Modelling of High Velocity Impact on Concrete Structures Using a Rate-Dependent Plastic-Damage Microplane Approach at Finite Strains. Materials. 2020; 13(22):5165. https://doi.org/10.3390/ma13225165
Chicago/Turabian StyleIndriyantho, Bobby Rio, Imadeddin Zreid, Robert Fleischhauer, and Michael Kaliske. 2020. "Modelling of High Velocity Impact on Concrete Structures Using a Rate-Dependent Plastic-Damage Microplane Approach at Finite Strains" Materials 13, no. 22: 5165. https://doi.org/10.3390/ma13225165
APA StyleIndriyantho, B. R., Zreid, I., Fleischhauer, R., & Kaliske, M. (2020). Modelling of High Velocity Impact on Concrete Structures Using a Rate-Dependent Plastic-Damage Microplane Approach at Finite Strains. Materials, 13(22), 5165. https://doi.org/10.3390/ma13225165





