Effect of the Free Volume on the Electronic Structure of Cu70Zr30 Metallic Glasses
Abstract
1. Introduction
2. Methods
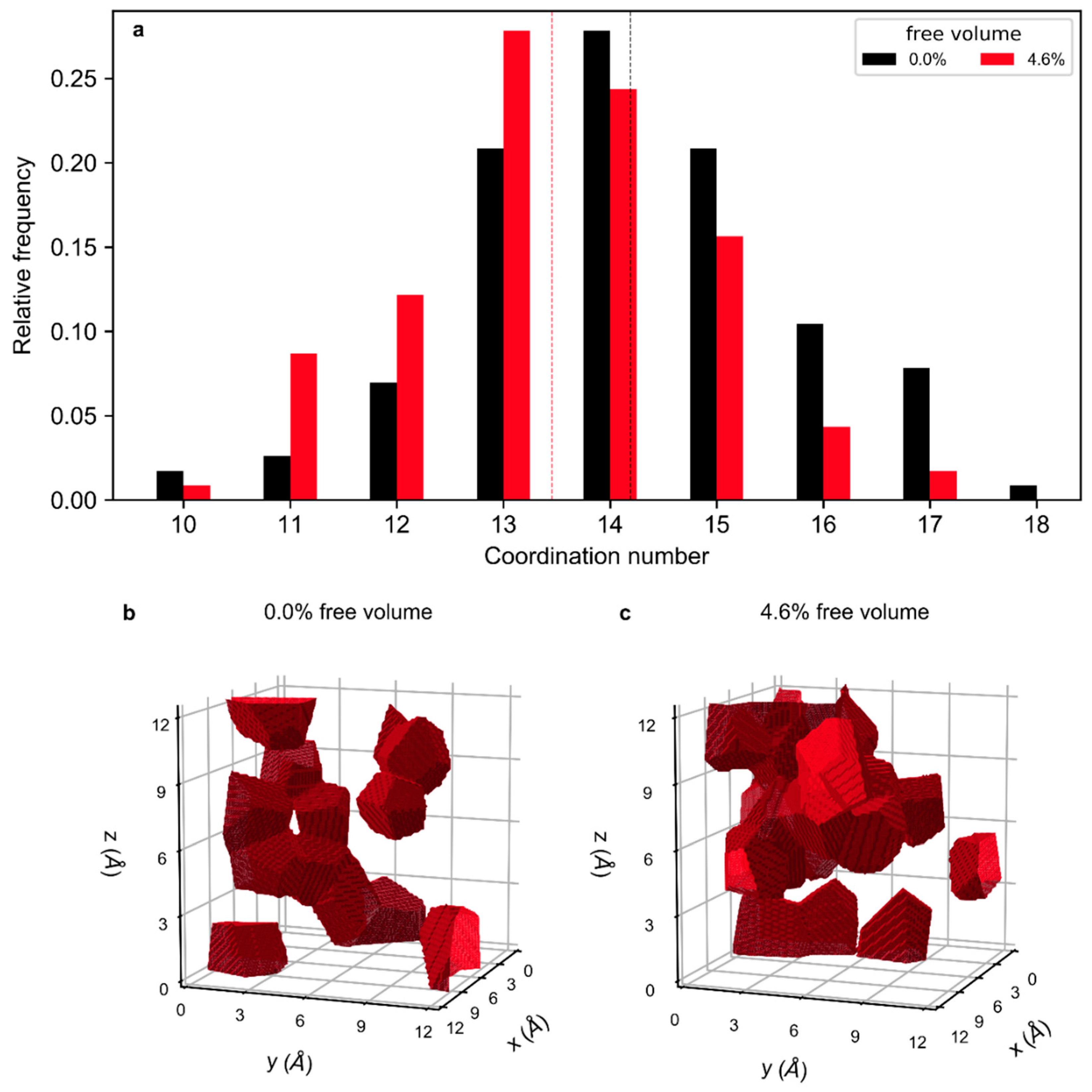
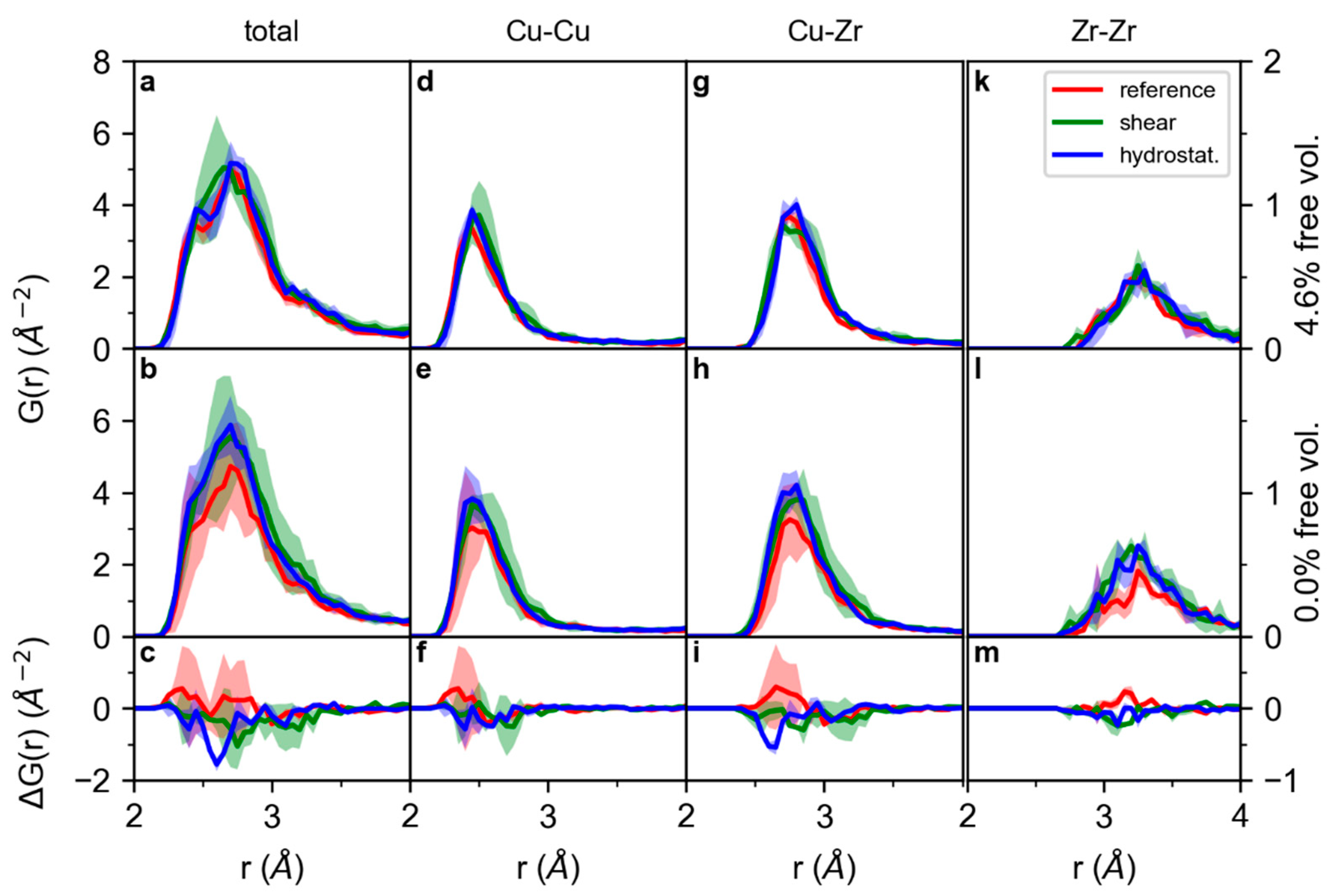
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Ashby, M.; Greer, A.L. Metallic glasses as structural materials. Scr. Mater. 2006, 54, 321–326. [Google Scholar] [CrossRef]
- Sun, Y.; Concustell, A.; Greer, A.L. Thermomechanical processing of metallic glasses: Extending the range of the glassy state. Nat. Rev. Mater. 2016, 1, 16039. [Google Scholar] [CrossRef]
- Pan, J.; Ivanov, Y.P.; Zhou, W.H.; Li, Y.; Greer, A.L. Strain-hardening and suppression of shear-banding in rejuvenated bulk metallic glass. Nature 2020, 578, 559–562. [Google Scholar] [CrossRef] [PubMed]
- Argon, A.S. Plastic deformation in metallic glasses. Acta Metall. 1979, 27, 47–58. [Google Scholar] [CrossRef]
- Spaepen, F. A microscopic mechanism for steady state inhomogeneous flow in metallic glasses. Acta Metall. 1977, 25, 407–415. [Google Scholar] [CrossRef]
- Egami, T.; Iwashita, T.; Dmowski, W. Mechanical Properties of Metallic Glasses. Metals 2013, 3, 77–113. [Google Scholar] [CrossRef]
- Li, N.; Liu, L.; Chen, Q.; Pan, J.; Chan, K.C. The effect of free volume on the deformation behaviour of a Zr-based metallic glass under nanoindentation. J. Phys. D Appl. Phys. 2007, 40, 6055–6059. [Google Scholar] [CrossRef]
- Slipenyuk, A.; Eckert, J. Correlation between enthalpy change and free volume reduction during structural relaxation of Zr55Cu30Al10Ni5 metallic glass. Scr. Mater. 2004, 50, 39–44. [Google Scholar] [CrossRef]
- Haruyama, O.; Nakayama, Y.; Wada, R.; Tokunaga, H.; Okada, J.; Ishikawa, T.; Yokoyama, Y. Volume and enthalpy relaxation in Zr55Cu30Ni5Al10 bulk metallic glass. Acta Mater. 2010, 58, 1829–1836. [Google Scholar] [CrossRef]
- Evenson, Z.; Busch, R. Equilibrium viscosity, enthalpy recovery and free volume relaxation in a Zr44Ti11Ni10Cu10Be25 bulk metallic glass. Acta Mater. 2011, 59, 4404–4415. [Google Scholar] [CrossRef]
- Yavari, A.R.; Le Moulec, A.; Inoue, A.; Nishiyama, N.; Lupu, N.; Matsubara, E.; Botta, W.J.; Vaughan, G.; Di Michiel, M.; Kvick, Å. Excess free volume in metallic glasses measured by X-ray diffraction. Acta Mater. 2005, 53, 1611–1619. [Google Scholar] [CrossRef]
- Fan, C.; Liu, C.T.; Chen, G.; Liaw, P.K. Quantitatively defining free-volume, interconnecting-zone and cluster in metallic glasses. Intermetallics 2015, 57, 98–100. [Google Scholar] [CrossRef]
- Ye, J.C.; Lu, J.; Liu, C.T.; Wang, Q.; Yang, Y. Atomistic free-volume zones and inelastic deformation of metallic glasses. Nat. Mater. 2010, 9, 619–623. [Google Scholar] [CrossRef]
- Schnabel, V.; Jaya, B.N.; Köhler, M.; Music, D.; Kirchlechner, C.; Dehm, G.; Raabe, D.; Schneider, J.M. Electronic hybridisation implications for the damage-tolerance of thin film metallic glasses. Sci. Rep. 2016, 6, 36556. [Google Scholar] [CrossRef] [PubMed]
- Evertz, S.; Kirchlechner, I.; Soler, R.; Kirchlechner, C.; Kontis, P.; Bednarcik, J.; Gault, B.; Dehm, G.; Raabe, D.; Schneider, J.M. Electronic structure based design of thin film metallic glasses with superior fracture toughness. Mater. Design 2020, 186, 108327. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Hostert, C.; Music, D.; Bednarcik, J.; Keckes, J.; Kapaklis, V.; Hjorvarsson, B.; Schneider, J.M. Ab initio molecular dynamics model for density, elastic properties and short range order of Co-Fe-Ta-B metallic glass thin films. J. Phys. Condens. Mat. 2011, 23, 475401. [Google Scholar] [CrossRef][Green Version]
- Ozaki, T.; Kino, H. Numerical atomic basis orbitals from H to Kr. Phys. Rev. B 2004, 69, 195113. [Google Scholar] [CrossRef]
- Ozaki, T.; Kino, H. Efficient projector expansion for the ab initio LCAO method. Phys. Rev. B 2005, 72, 45121. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169–11186. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758–1775. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953–17979. [Google Scholar] [CrossRef] [PubMed]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Birch, F. Finite strain isotherm and velocities for single-crystal and polycrystalline NaCl at high pressures and 300° K. J. Geophys. Res. 1978, 83, 1257. [Google Scholar] [CrossRef]
- Music, D.; Takahashi, T.; Vitos, L.; Asker, C.; Abrikosov, I.A.; Schneider, J.M. Elastic properties of Fe–Mn random alloys studied by ab initio calculations. Appl. Phys. Lett. 2007, 91, 191904. [Google Scholar] [CrossRef]
- Dronskowski, R.; Bloechl, P.E. Crystal orbital Hamilton populations (COHP): Energy-resolved visualization of chemical bonding in solids based on density-functional calculations. J. Phys. Chem. 1993, 97, 8617–8624. [Google Scholar] [CrossRef]
- Deringer, V.L.; Tchougréeff, A.L.; Dronskowski, R. Crystal orbital Hamilton population (COHP) analysis as projected from plane-wave basis sets. J. Phys. Chem. A 2011, 115, 5461–5466. [Google Scholar] [CrossRef]
- Maintz, S.; Deringer, V.L.; Tchougréeff, A.L.; Dronskowski, R. Analytic projection from plane-wave and PAW wavefunctions and application to chemical-bonding analysis in solids. J. Comput. Chem. 2013, 34, 2557–2567. [Google Scholar] [CrossRef]
- Maintz, S.; Deringer, V.L.; Tchougréeff, A.L.; Dronskowski, R. LOBSTER: A tool to extract chemical bonding from plane-wave based DFT. J. Comput. Chem. 2016, 37, 1030–1035. [Google Scholar] [CrossRef]
- Spaepen, F. Homogeneous flow of metallic glasses: A free volume perspective. Scr. Mater. 2006, 54, 363–367. [Google Scholar] [CrossRef]
- Dyre, J.C.; Wang, W.H. The instantaneous shear modulus in the shoving model. J. Chem. Phys. 2012, 136, 224108. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.G.; Zhao, D.Q.; Pan, M.X.; Wang, W.H.; Song, S.X.; Nieh, T.G. Correlation between onset of yielding and free volume in metallic glasses. Scr. Mater. 2010, 62, 477–480. [Google Scholar] [CrossRef]
- Lejaeghere, K.; van Speybroeck, V.; van Oost, G.; Cottenier, S. Error Estimates for Solid-State Density-Functional Theory Predictions: An Overview by Means of the Ground-State Elemental Crystals. Crit. Rev. Solid State Mater. Sci. 2014, 39, 1–24. [Google Scholar] [CrossRef]
- Carter, F.L. On Deriving Density of States Information from Chemical Bond Considerations. In Electronic Density of States Based on Invited and Contributed Papers and Discussion, 3rd Materials Research Symposium; Bennet, L.H., Ed.; NBS Special Publication: Washington, DC, USA, 1971; pp. 385–405. [Google Scholar]
- Li, F.; Liu, X.J.; Lu, Z.P. Atomic structural evolution during glass formation of a Cu–Zr binary metallic glass. Comp. Mater. Sci. 2014, 85, 147–153. [Google Scholar] [CrossRef]
- Babanov, Y.A.; Schvetsov, V.R.; Sidorenko, A.F. Atomic structure of binary amorphous alloys by combined EXAFS and X-ray scattering. Phys. B Condens. Mat. 1995, 208–209, 375–376. [Google Scholar] [CrossRef]
- Evertz, S.; Music, D.; Schnabel, V.; Bednarcik, J.; Schneider, J.M. Thermal expansion of Pd-based metallic glasses by ab initio methods and high energy X-ray diffraction. Sci. Rep. 2017, 7, 15744. [Google Scholar] [CrossRef]




| Free Volume (%) | Total Energy (eV) | Energy per Atom (eV/Atom) | Supercell Volume (Å3) | Energy Density (eV/Å3) | Bulk Modulus (GPa) | Shear Modulus (GPa) |
|---|---|---|---|---|---|---|
| Structural model 1 | ||||||
| 0.0 | −590.6 | −5.12 | 1847.5 | −0.320 | 114.4 | 39.2 |
| 0.9 | −582.2 | −5.11 | 1847.5 | −0.315 | 111.3 | 37.9 |
| 1.8 | −576.3 | −5.10 | 1847.5 | −0.312 | 113.4 | 37.2 |
| 2.7 | −576.4 | −5.14 | 1847.5 | −0.312 | 116.1 | 38.7 |
| 3.6 | −566.4 | −5.10 | 1847.5 | −0.307 | 111.5 | 35.5 |
| 4.5 | −548.1 | −4.98 | 1847.5 | −0.297 | 106.1 | 35.9 |
| Structural model 2 | ||||||
| 0.0 | −589.9 | −5.13 | 1857.0 | −0.317 | 113.7 | 40.2 |
| 0.9 | −582.5 | −5.11 | 1857.0 | −0.314 | 111.3 | 38.1 |
| 1.8 | −574.2 | −5.08 | 1857.0 | −0.309 | 112.3 | 36.9 |
| 2.7 | −567.3 | −5.06 | 1857.0 | −0.305 | 112.0 | 34.4 |
| 3.6 | −570.9 | −5.14 | 1857.0 | −0.307 | 115.1 | 37.4 |
| 4.5 | −557.3 | −5.06 | 1857.0 | −0.300 | 112.1 | 34.2 |
| Structural model 3 | ||||||
| 0.0 | −592.9 | −5.16 | 1831.8 | −0.324 | 114.9 | 43.0 |
| 0.9 | −585.8 | −5.14 | 1831.8 | −0.320 | 111.1 | 41.5 |
| 1.8 | −577.1 | −5.11 | 1831.8 | −0.315 | 108.3 | 40.4 |
| 2.7 | −573.2 | −5.12 | 1831.8 | −0.313 | 103.7 | 38.5 |
| 3.6 | −569.8 | −5.13 | 1831.8 | −0.311 | 102.3 | 37.9 |
| 4.5 | −559.2 | −5.08 | 1831.8 | −0.305 | 96.5 | 35.6 |
| Structural model 4 | ||||||
| 0.0 | −588.7 | −5.12 | 1839.0 | −0.320 | 114.6 | 38.4 |
| 0.9 | −581.7 | −5.10 | 1839.0 | −0.316 | 111.2 | 40.3 |
| 1.8 | −572.4 | −5.07 | 1839.0 | −0.311 | 110.4 | 37.4 |
| 2.7 | −569.4 | −5.08 | 1839.0 | −0.309 | 110.7 | 38.8 |
| 3.6 | −564.0 | −5.08 | 1839.0 | −0.307 | 110.6 | 36.4 |
| 4.5 | −554.6 | −5.04 | 1839.0 | −0.302 | 110.1 | 37.2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Evertz, S.; Schneider, J.M. Effect of the Free Volume on the Electronic Structure of Cu70Zr30 Metallic Glasses. Materials 2020, 13, 4911. https://doi.org/10.3390/ma13214911
Evertz S, Schneider JM. Effect of the Free Volume on the Electronic Structure of Cu70Zr30 Metallic Glasses. Materials. 2020; 13(21):4911. https://doi.org/10.3390/ma13214911
Chicago/Turabian StyleEvertz, Simon, and Jochen M. Schneider. 2020. "Effect of the Free Volume on the Electronic Structure of Cu70Zr30 Metallic Glasses" Materials 13, no. 21: 4911. https://doi.org/10.3390/ma13214911
APA StyleEvertz, S., & Schneider, J. M. (2020). Effect of the Free Volume on the Electronic Structure of Cu70Zr30 Metallic Glasses. Materials, 13(21), 4911. https://doi.org/10.3390/ma13214911





