Influence of the Elastoplastic Strain on Fatigue Durability Determined with the Use of the Spectral Method
Abstract
1. Introduction
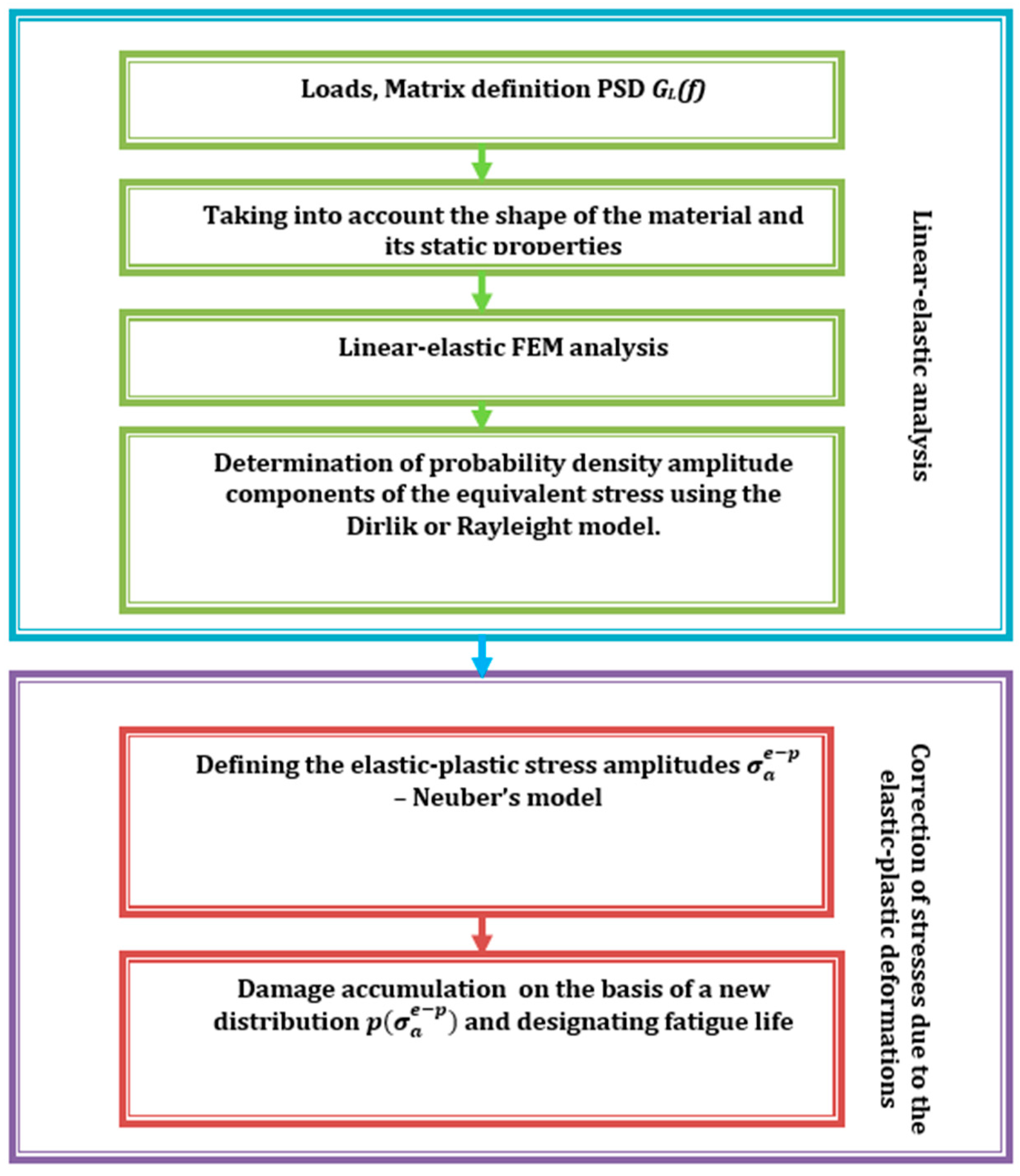
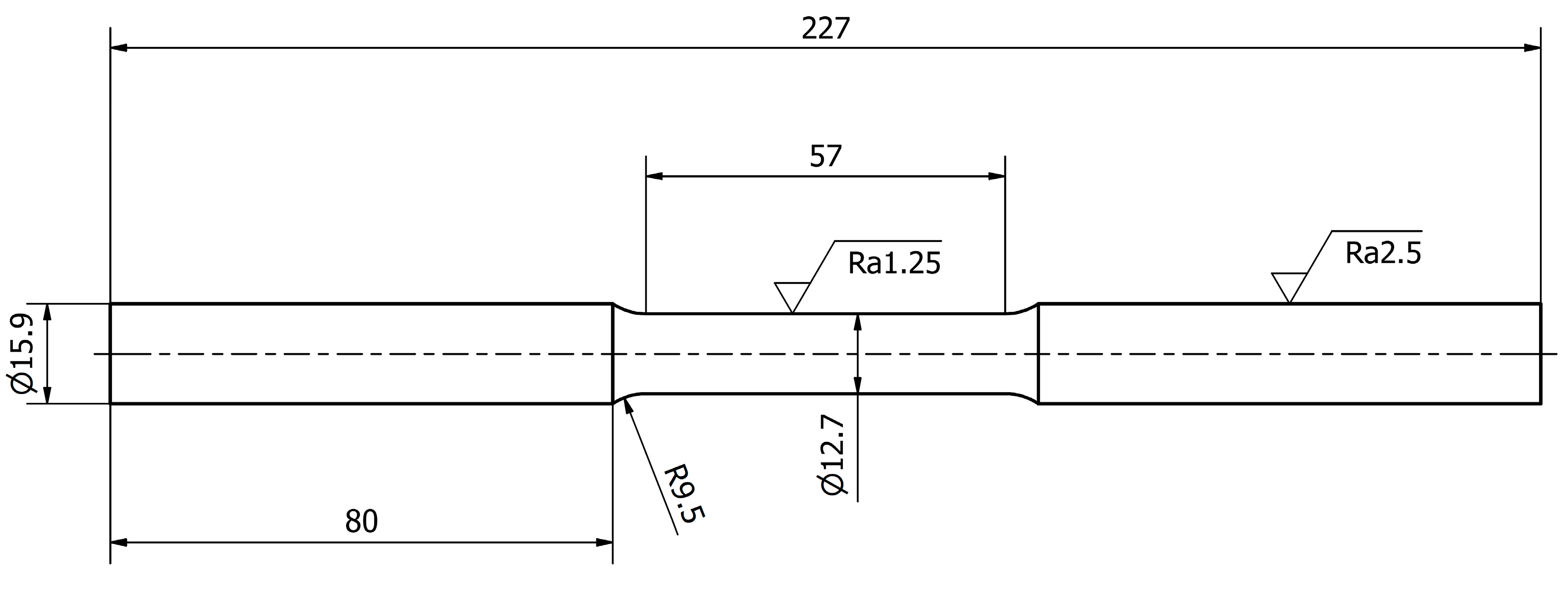
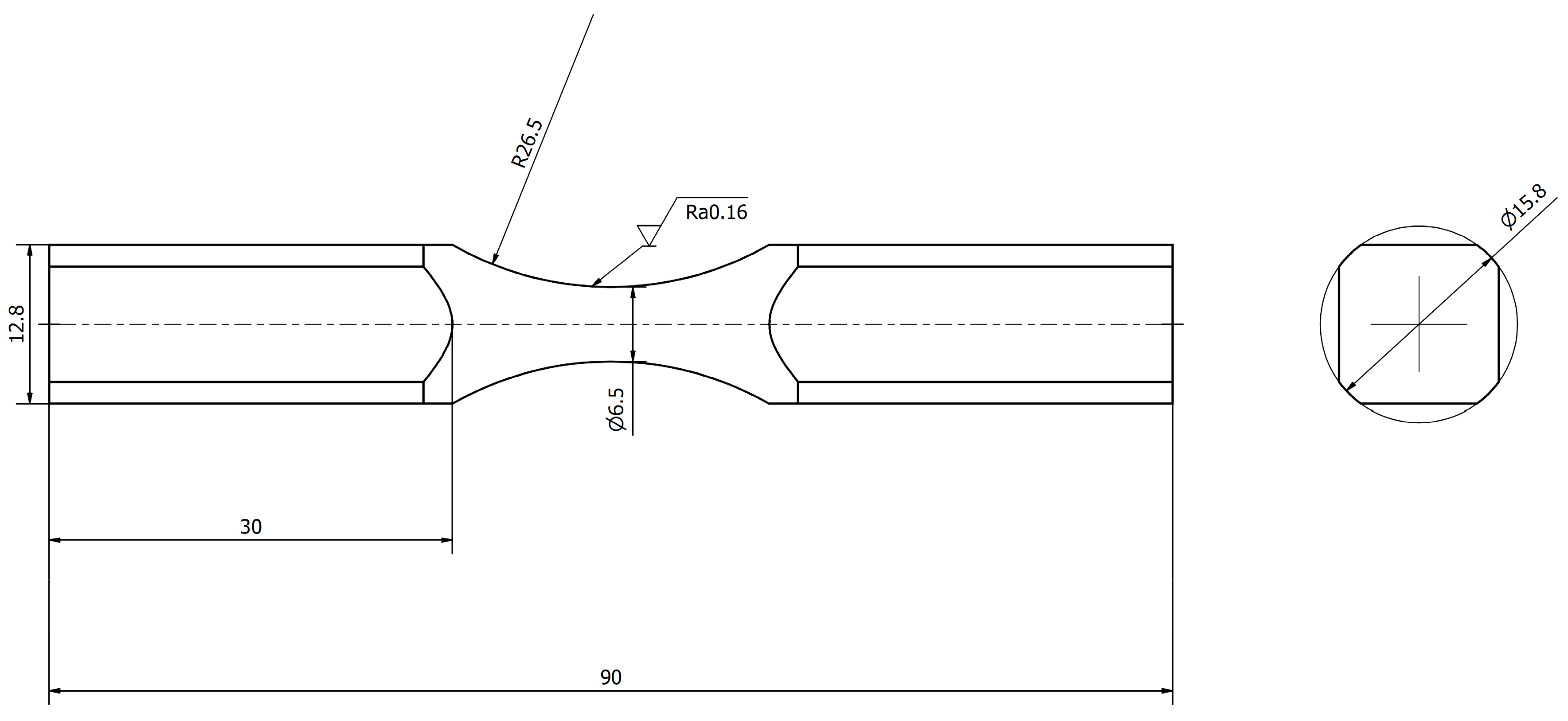
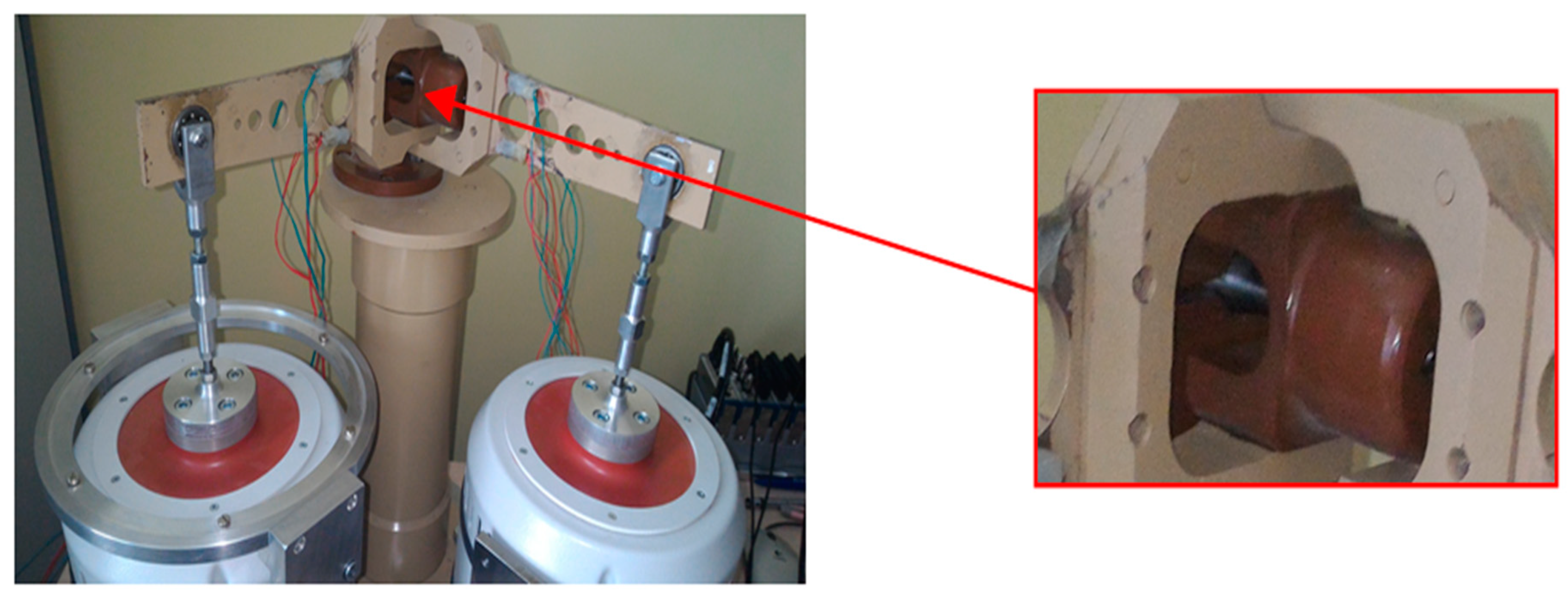
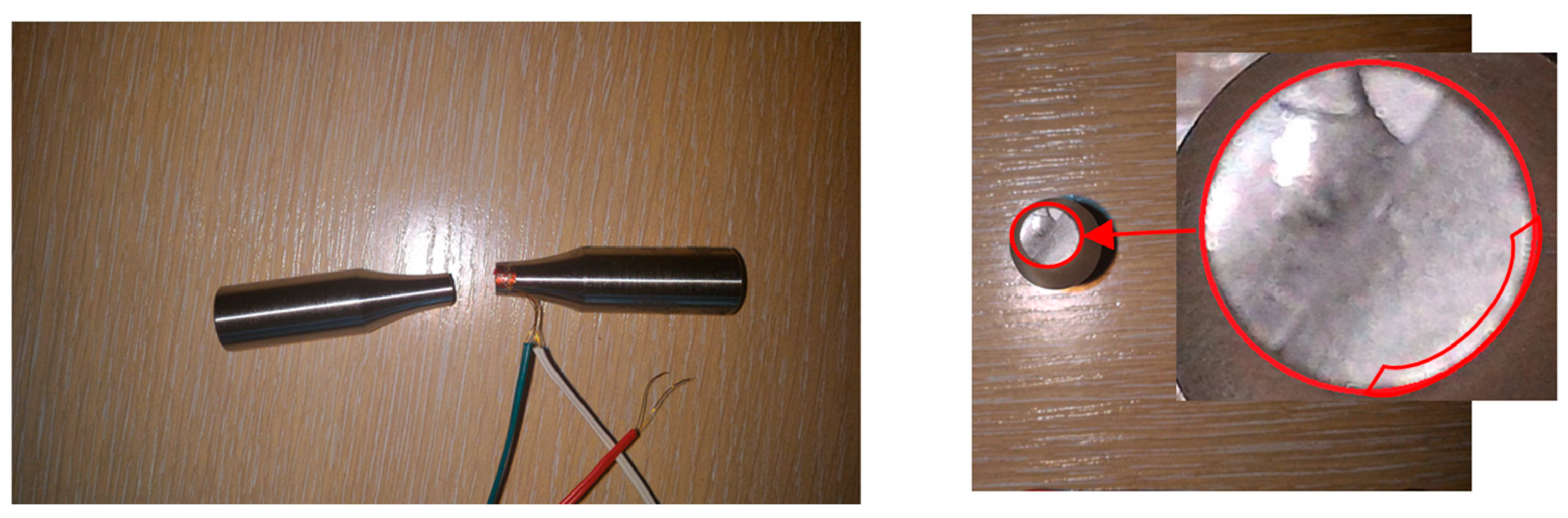
2. Materials and Methods
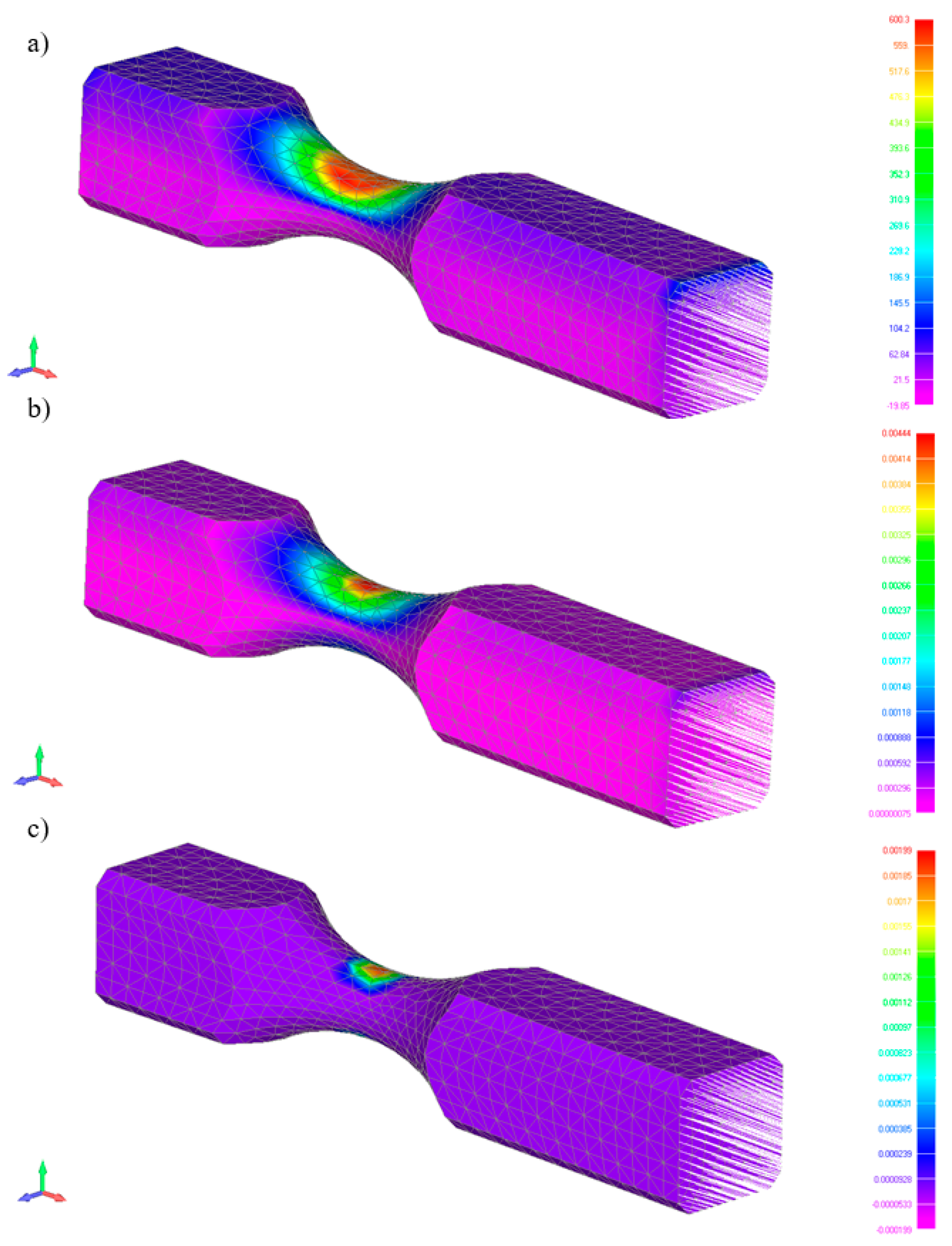
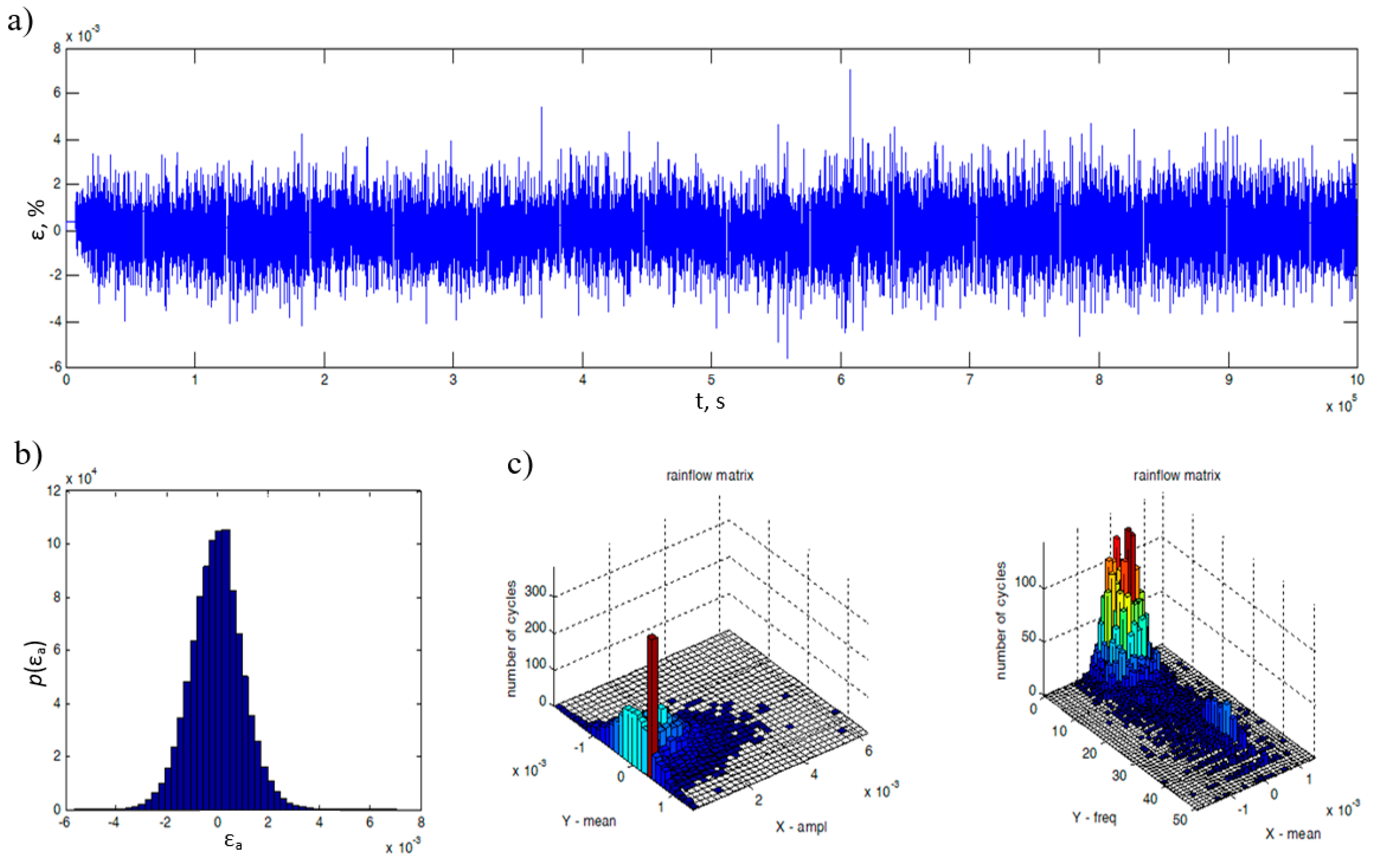
3. Results
4. Discussion
5. Conclusions and Observations
- An algorithm for taking into account the elastoplastic strain in the process of fatigue life assessment with the use of spectral method is presented.
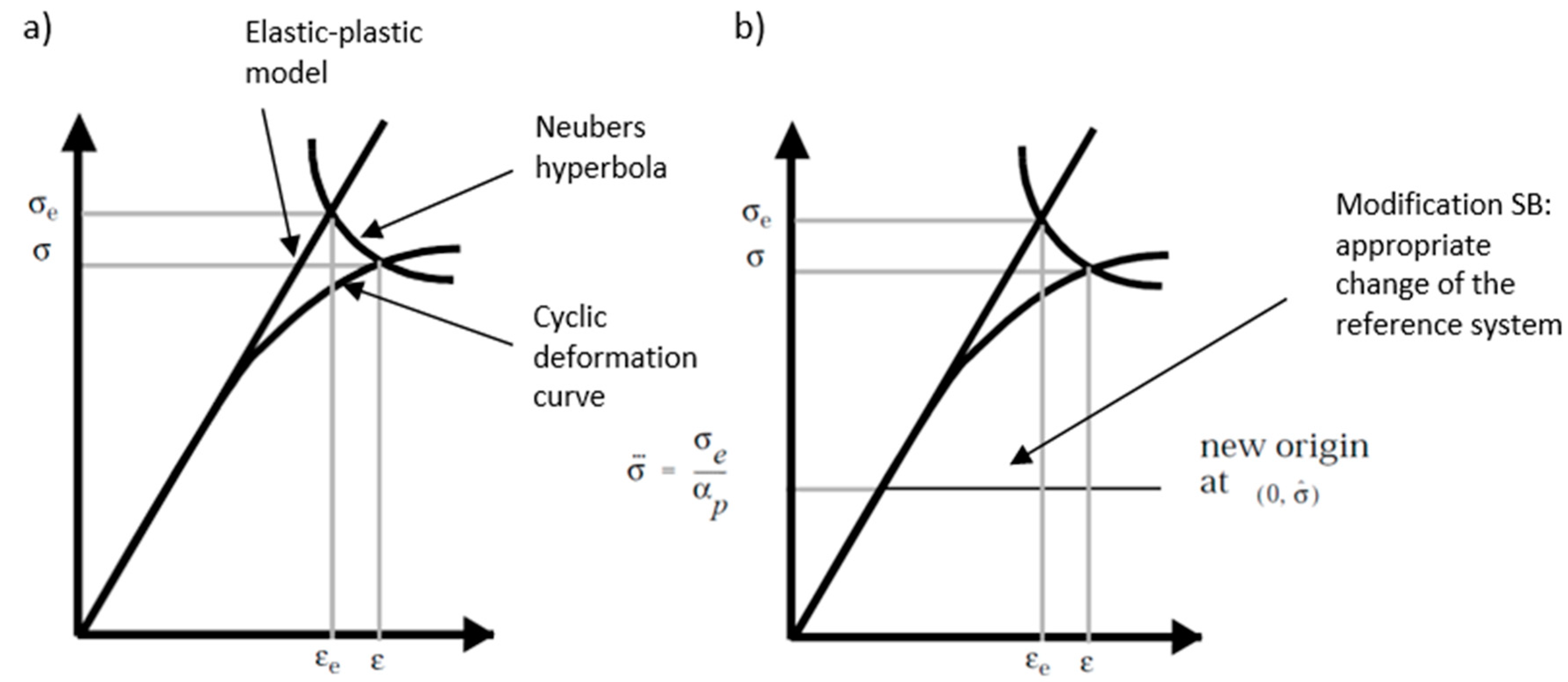
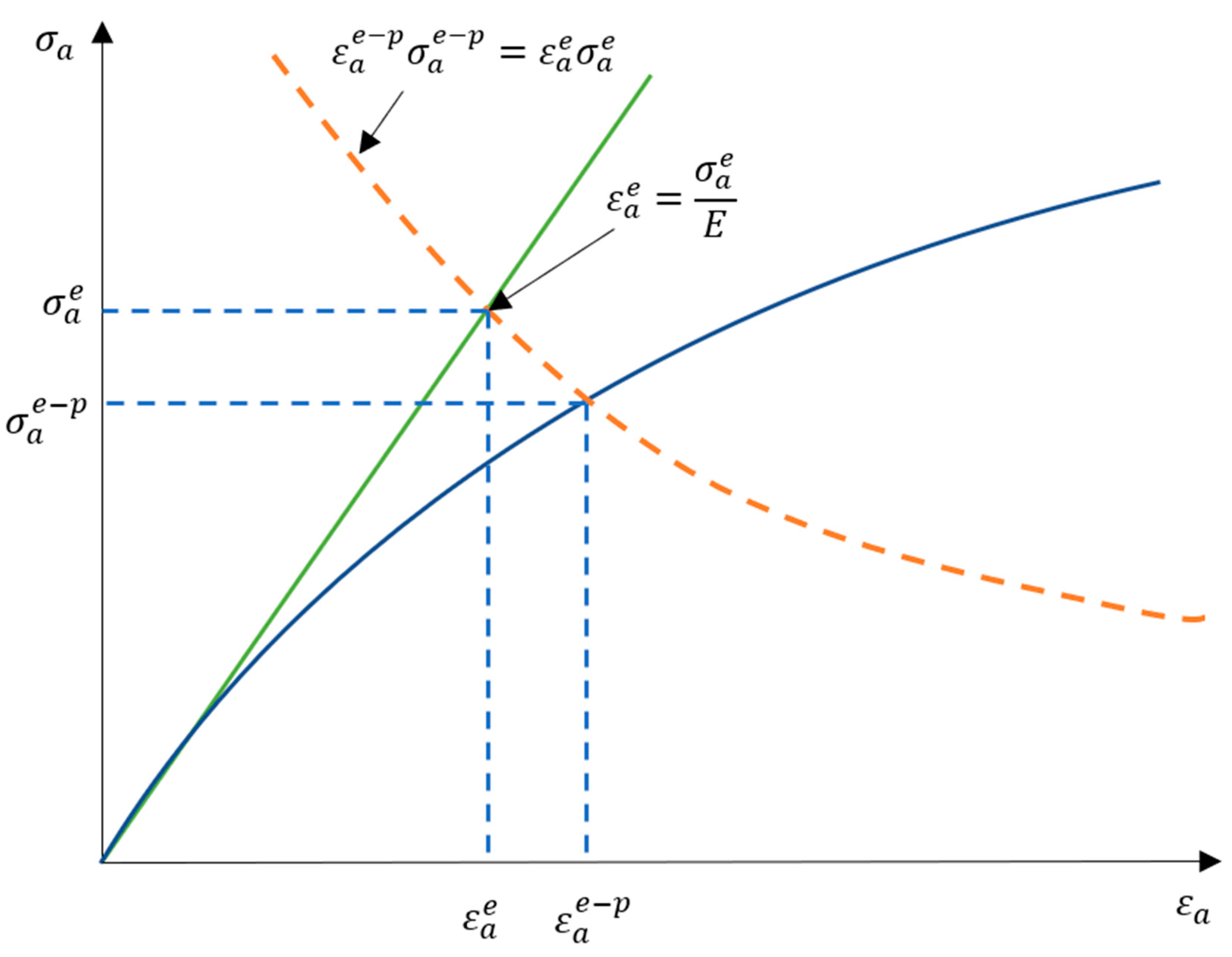
- In order to use the proposed correction, we need to use the Neuber hyperbola to obtain the values corresponding to the elastic stress amplitudes obtained during the probability density calculation and their corresponding elastoplastic stress amplitudes.
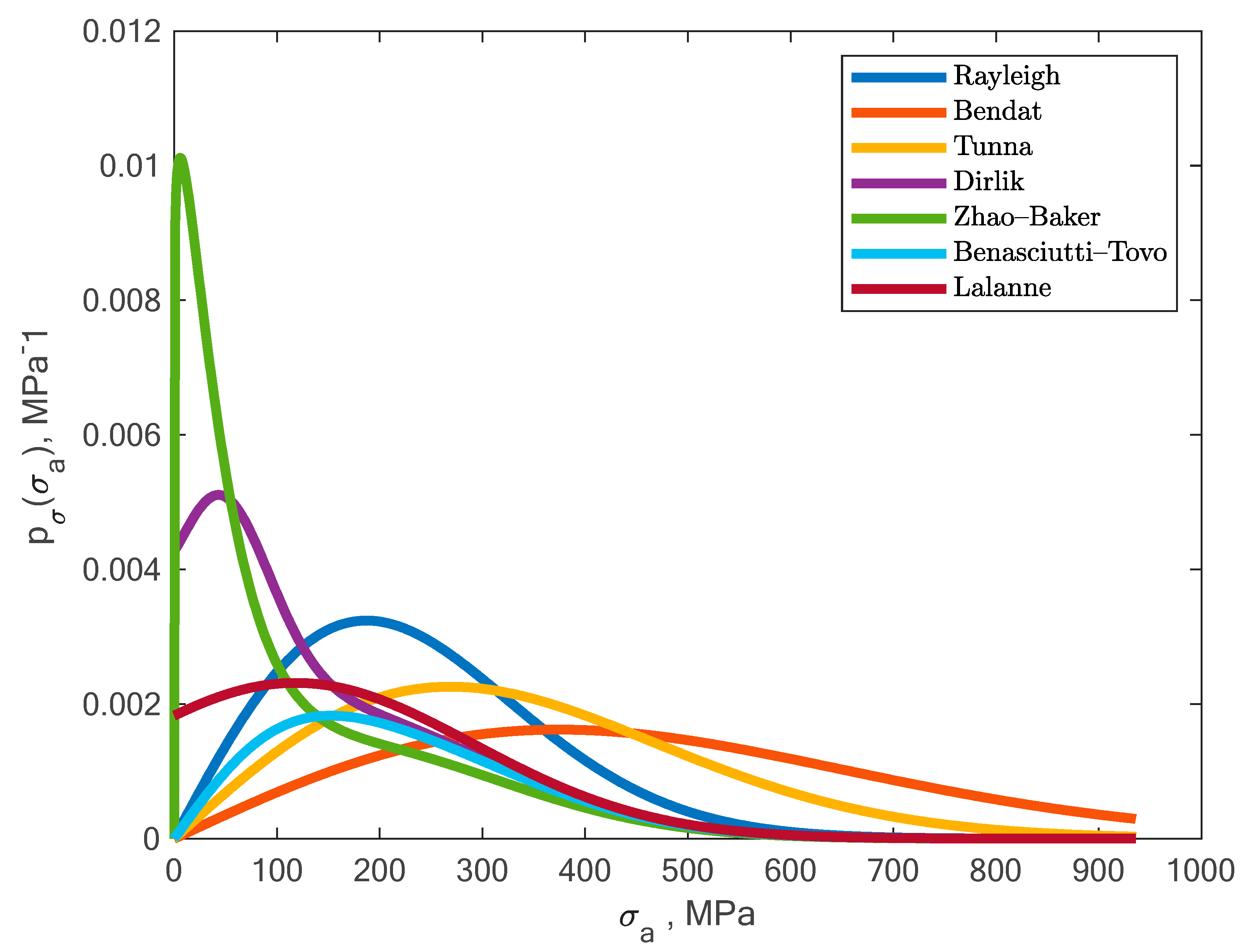
- Correction due to elastoplastic stress is successfully applied to determine fatigue life in combination with all four distribution models.
- In the case of no correction, in terms of elastoplastic strain, we obtain overestimated fatigue calculation results.
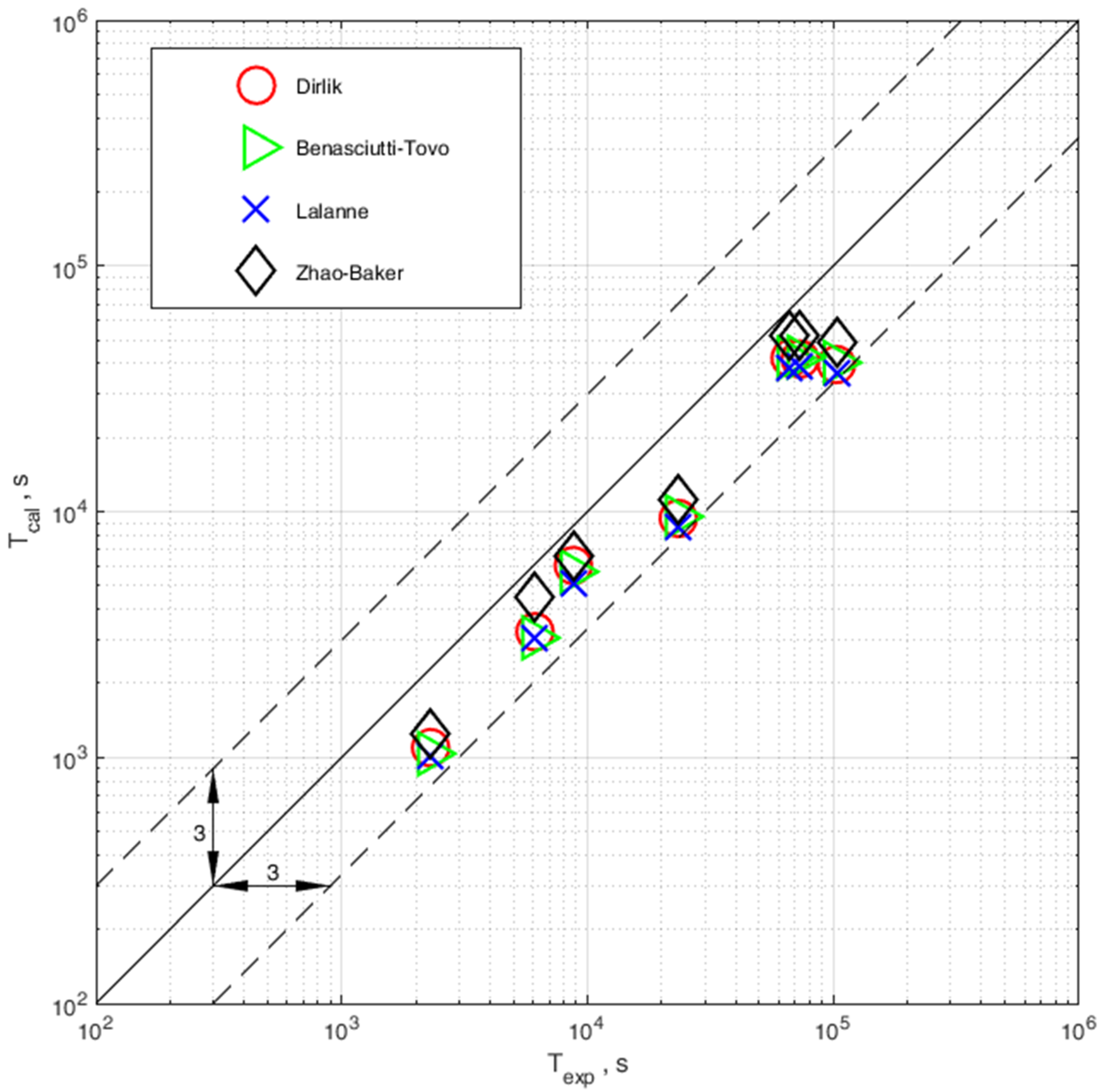
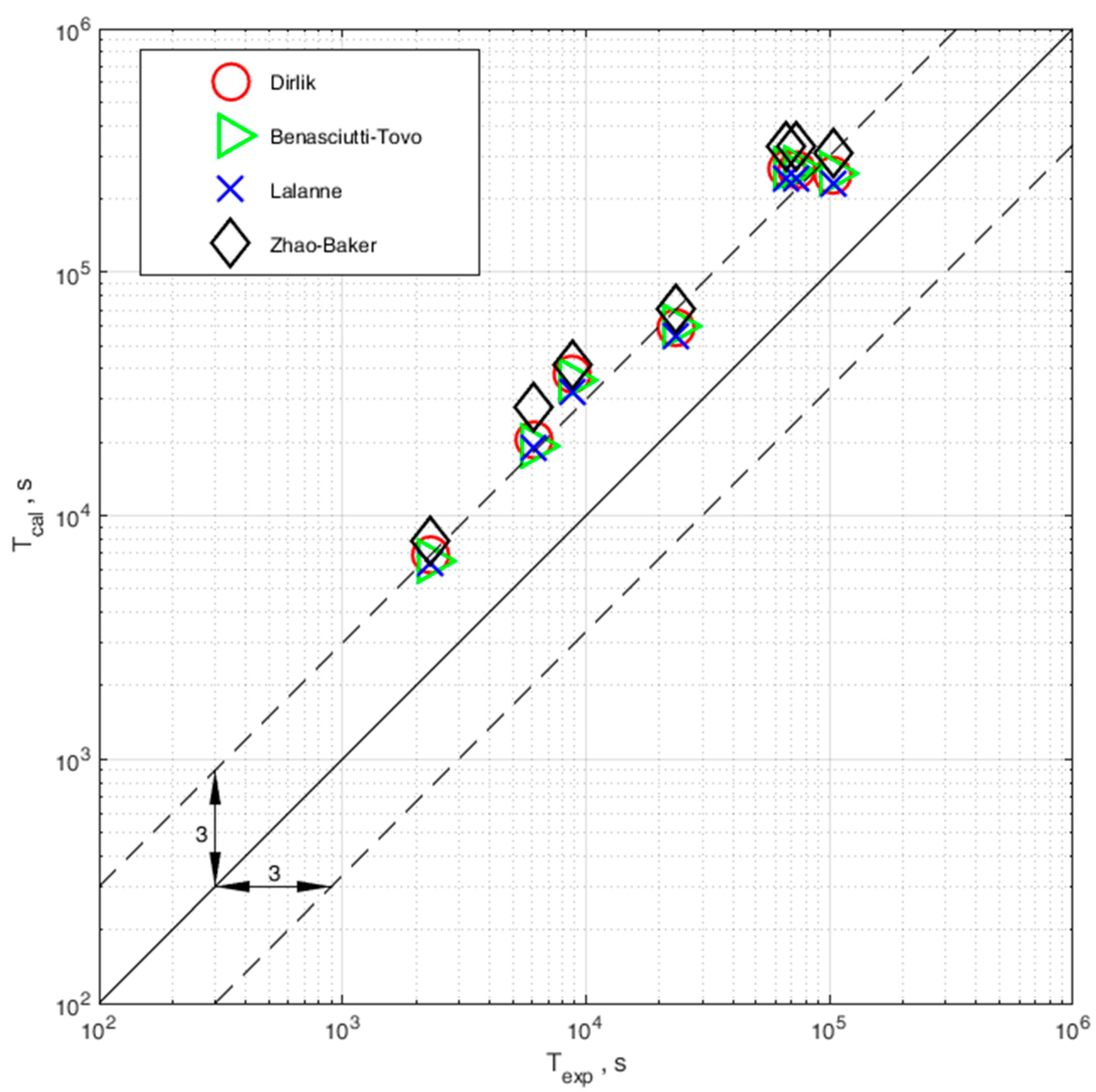
- Comparison of experimental and computational durability with correction shows that calculations are within a safe scatter band of 3.
- All models used to calculate the probability density function enable obtaining results in the desired scatter band, and the computation results are on the safe side, as they do not overestimate the experimental results.
Author Contributions
Funding
Conflicts of Interest
References
- Banvillet, A.; Łagoda, T.; Macha, E.; Niesłony, A.; Palin-Luc, T.; Vittori, J.-F. Fatigue life under non-Gaussian random loading from various models. Int. J. Fatigue 2004, 26, 349–363. [Google Scholar] [CrossRef]
- Benasciutti, D.; Tovo, R. Comparison of spectral methods for fatigue analysis of broad-band Gaussian random processes. Probabilistic Eng. Mech. 2006, 21, 287–299. [Google Scholar] [CrossRef]
- Benasciutti, D.; Tovo, R. Spectral methods for lifetime prediction under wide-band stationary random processes. Int. J. Fatigue 2005, 27, 867–877. [Google Scholar] [CrossRef]
- Böhm, M.; Nieslony, A. Strain-based multiaxial fatigue life evaluation using spectral method. In Proceedings of the 3rd International Conference on Material and Component Performance under Variable Amplitude Loading, VAL 2015, Prague, Czech Republic, 23–26 March 2015; Papuga, J., Ruzicka, M., Eds.; Elsevier Science BV: Amsterdam, The Netherlands, 2015; Volume 101, pp. 52–60. [Google Scholar]
- Łagoda, T.; Macha, E.; Niesłony, A. Fatigue life calculation by means of the cycle counting and spectral methods under multiaxial random loading. Fatigue Fract. Eng. Mater. Struct. 2005, 28, 409–420. [Google Scholar] [CrossRef]
- Nieslony, A.; Macha, E. Spectral Method in Multiaxial Random Fatigue. In Lecture Notes in Applied and Computational Mechanics; Springer: Berlin/Heidelberg, Germany, 2007; ISBN 978-3-540-73822-0. [Google Scholar]
- Benzing, J.T.; Liu, Y.; Zhang, X.; Luecke, W.E.; Ponge, D.; Dutta, A.; Oskay, C.; Raabe, D.; Wittig, J.E. Experimental and numerical study of mechanical properties of multi-phase medium-Mn TWIP-TRIP steel: Influences of strain rate and phase constituents. Acta Mater. 2019, 177, 250–265. [Google Scholar] [CrossRef]
- Das, A.; Tarafder, S.; Sivaprasad, S.; Chakrabarti, D. Influence of microstructure and strain rate on the strain partitioning behaviour of dual phase steels. Mater. Sci. Eng. A 2019, 754, 348–360. [Google Scholar] [CrossRef]
- Khosravani, M.R.; Anders, D.; Weinberg, K. Influence of strain rate on fracture behavior of sandwich composite T-joints. Eur. J. Mech.-A/Solids 2019, 78, 103821. [Google Scholar] [CrossRef]
- Rognon, H.; Da Silva Botelho, T.; Tawfiq, I.; Galtier, A.; Bennebach, M. Modeling of Plasticity in Spectral Methods for Fatigue Damage Estimation of Narrowband Random Vibrations. In Proceedings of the Volume 1: 23rd Biennial Conference on Mechanical Vibration and Noise, Parts A and B, Washington, DC, USA, 28–31 August 2011; ASMEDC: Washington, DC, USA, 2011; pp. 771–779. [Google Scholar]
- Pitoiset, X.; Preumont, A. Spectral methods for multiaxial random fatigue analysis of metallic structures. Int. J. Fatigue 2000, 22, 541–550. [Google Scholar] [CrossRef]
- Benasciutti, D. An analytical approach to measure the accuracy of various definitions of the “equivalent von Mises stress” in vibration multiaxial fatigue. In Proceedings of the International Conference on Engineering Vibration, Ljubljana, Slovenia, 7–10 September 2015; pp. 743–752. [Google Scholar]
- Neuber, H. Kerbspannungslehre: Theorie der Spannungskonzentration Genaue Berechnung der Festigkeit, 4th ed.; Klassiker der Technik; Springer: Berlin/Heidelberg, Germany, 2001; ISBN 978-3-540-67657-7. [Google Scholar]
- Palmieri, M.; Česnik, M.; Slavič, J.; Cianetti, F.; Boltežar, M. Non-Gaussianity and non-stationarity in vibration fatigue. Int. J. Fatigue 2017, 97, 9–19. [Google Scholar] [CrossRef]
- Braccesi, C.; Cianetti, F.; Lori, G.; Pioli, D. The frequency domain approach in virtual fatigue estimation of non-linear systems: The problem of non-Gaussian states of stress. Int. J. Fatigue 2009, 31, 766–775. [Google Scholar] [CrossRef]
- Chiang, D.-Y. The generalized Masing models for deteriorating hysteresis and cyclic plasticity. Appl. Math. Model. 1999, 23, 847–863. [Google Scholar] [CrossRef]
- Knop, M.; Jones, R.; Molent, L.; Wang, C. On the Glinka and Neuber methods for calculating notch tip strains under cyclic load spectra. Int. J. Fatigue 2000, 22, 743–755. [Google Scholar] [CrossRef]
- Böhm, M.; Kowalski, M.; Nieslony, A. Multiaxial Fatigue Test Stand Concept—Stand and Control Design. In Mechatronics: Ideas for Industrial Applications; Awrejcewicz, J., Szewczyk, R., Trojnacki, M., Kaliczynska, M., Eds.; Springer International Publishing AG: Cham, Switzerland, 2015; Volume 317, pp. 437–445. ISBN 978-3-319-10990-9. [Google Scholar]
- Nieslony, A.; Böhm, M. Determination of Fatigue Life on the Basis of Experimental Fatigue Diagrams Under Constant Amplitude Load with Mean Stress. In Fatigue Failure and Fracture Mechanics; Skibicki, D., Ed.; Trans Tech Publications Ltd.: Stafa-Zurich, Switzerland, 2012; Volume 726, pp. 33–38. [Google Scholar]
- Gadamchetty, G.; Pandey, A.; Gawture, M. On Practical Implementation of the Ramberg-Osgood Model for FE Simulation. SAE Int. J. Mater. Manf. 2016, 9, 200–205. [Google Scholar] [CrossRef]
- Kamaya, M. Ramberg–Osgood type stress–train curve estimation using yield and ultimate strengths for failure assessments. Int. J. Press. Vessel. Pip. 2016, 137, 1–12. [Google Scholar] [CrossRef]
- Dirlik, T. Application of Computers in Fatigue Analysis. Ph.D. Thesis, University of Warwick, Coventry, UK, 1985. [Google Scholar]
- Nieslony, A.; Böhm, M. Mean stress effect correction in frequency-domain methods for fatigue life assessment. In Proceedings of the 3rd International Conference on Material and Component Performance under Variable Amplitude Loading, VAL 2015, Prague, Czech Republic, 23–26 March 2015; Papuga, J., Ruzicka, M., Eds.; Elsevier Science BV: Amsterdam, The Netherlands, 2015; Volume 101, pp. 347–354. [Google Scholar]
- Niesłony, A.; Böhm, M.; Łagoda, T.; Cianetti, F. The use of spectral method for fatigue life assessment for non-gaussian random loads. Acta Mech. Autom. 2016, 10, 100–103. [Google Scholar] [CrossRef]
- Zhao, W.; Baker, M. On the probability density function of rainflow stress range for stationary Gaussian processes. Int. J. Fatigue 1992, 14, 121–135. [Google Scholar] [CrossRef]
- Lalanne, C. Mechanical Vibration and Shock Analysis, Fatigue Damage; John Wiley & Sons: Hoboken, NJ, USA, 2013; ISBN 978-1-118-61893-6. [Google Scholar]
- Niesłony, A.; Böhm, M. Universal Method for Applying the Mean-Stress Effect Correction in Stochastic Fatigue-Damage Accumulation. MPC 2016, 5, 352–363. [Google Scholar] [CrossRef]
- Łagoda, T.; Macha, E. Energy approach to fatigue under combined cyclic bending with torsion of smooth and notched specimens. Mater. Sci. 1998, 34, 630–639. [Google Scholar] [CrossRef]
- MSC. Fatigue User’s Guide. Available online: https://kupdf.net/download/msc-fatigue-user-39-s-guide_589dd9c76454a7865ab1e8d6_pdf (accessed on 18 December 2019).
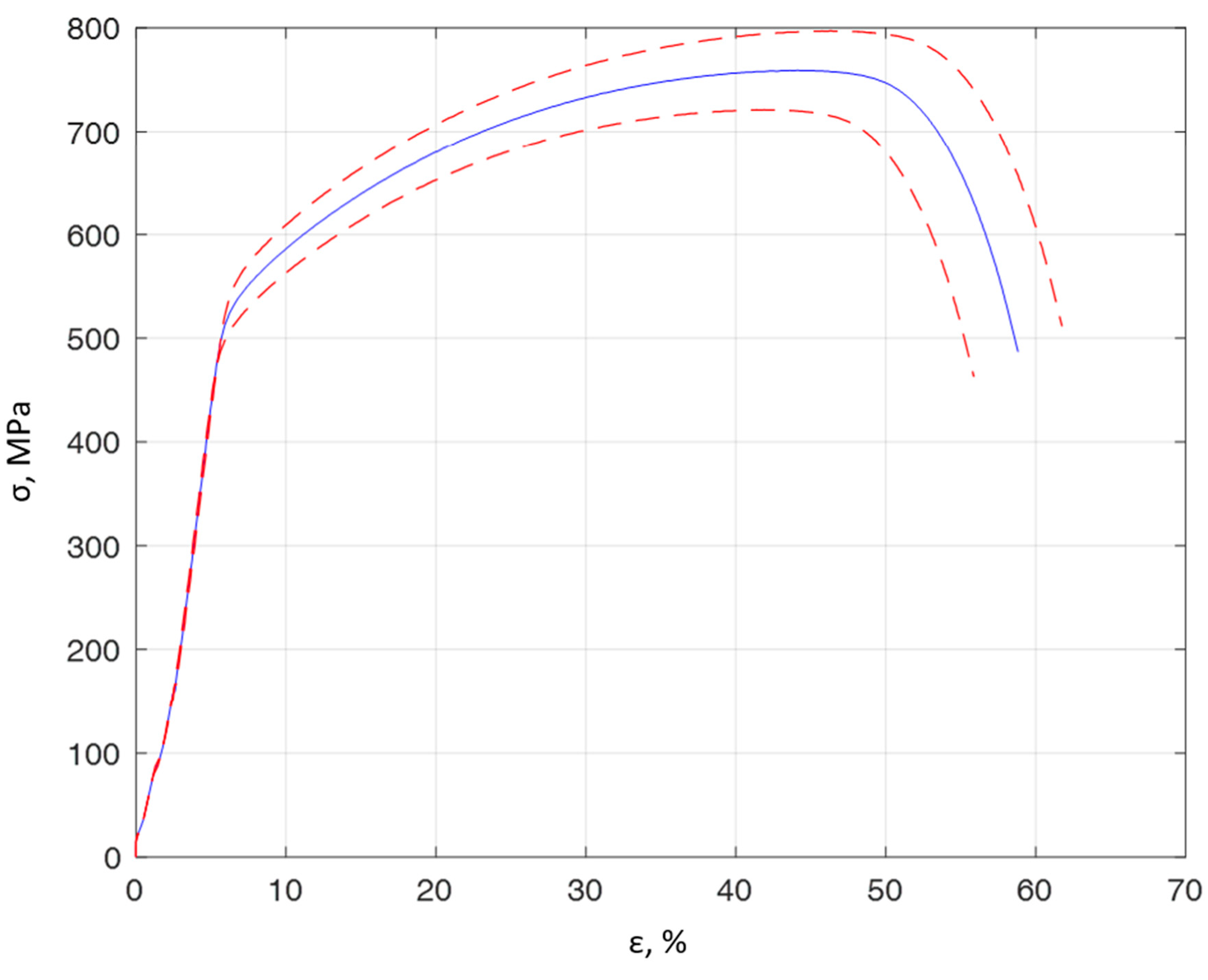
| Rm MPa | Re MPa | E GPa | υ |
|---|---|---|---|
| 750 | 515 | 200 | 0.29 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Böhm, M.; Kowalski, M.; Niesłony, A. Influence of the Elastoplastic Strain on Fatigue Durability Determined with the Use of the Spectral Method. Materials 2020, 13, 423. https://doi.org/10.3390/ma13020423
Böhm M, Kowalski M, Niesłony A. Influence of the Elastoplastic Strain on Fatigue Durability Determined with the Use of the Spectral Method. Materials. 2020; 13(2):423. https://doi.org/10.3390/ma13020423
Chicago/Turabian StyleBöhm, Michał, Mateusz Kowalski, and Adam Niesłony. 2020. "Influence of the Elastoplastic Strain on Fatigue Durability Determined with the Use of the Spectral Method" Materials 13, no. 2: 423. https://doi.org/10.3390/ma13020423
APA StyleBöhm, M., Kowalski, M., & Niesłony, A. (2020). Influence of the Elastoplastic Strain on Fatigue Durability Determined with the Use of the Spectral Method. Materials, 13(2), 423. https://doi.org/10.3390/ma13020423






