Structural and Micromechanical Properties of Ternary Granular Packings: Effect of Particle Size Ratio and Number Fraction of Particle Size Classes
Abstract
1. Introduction
2. Materials and Methods
3. Results
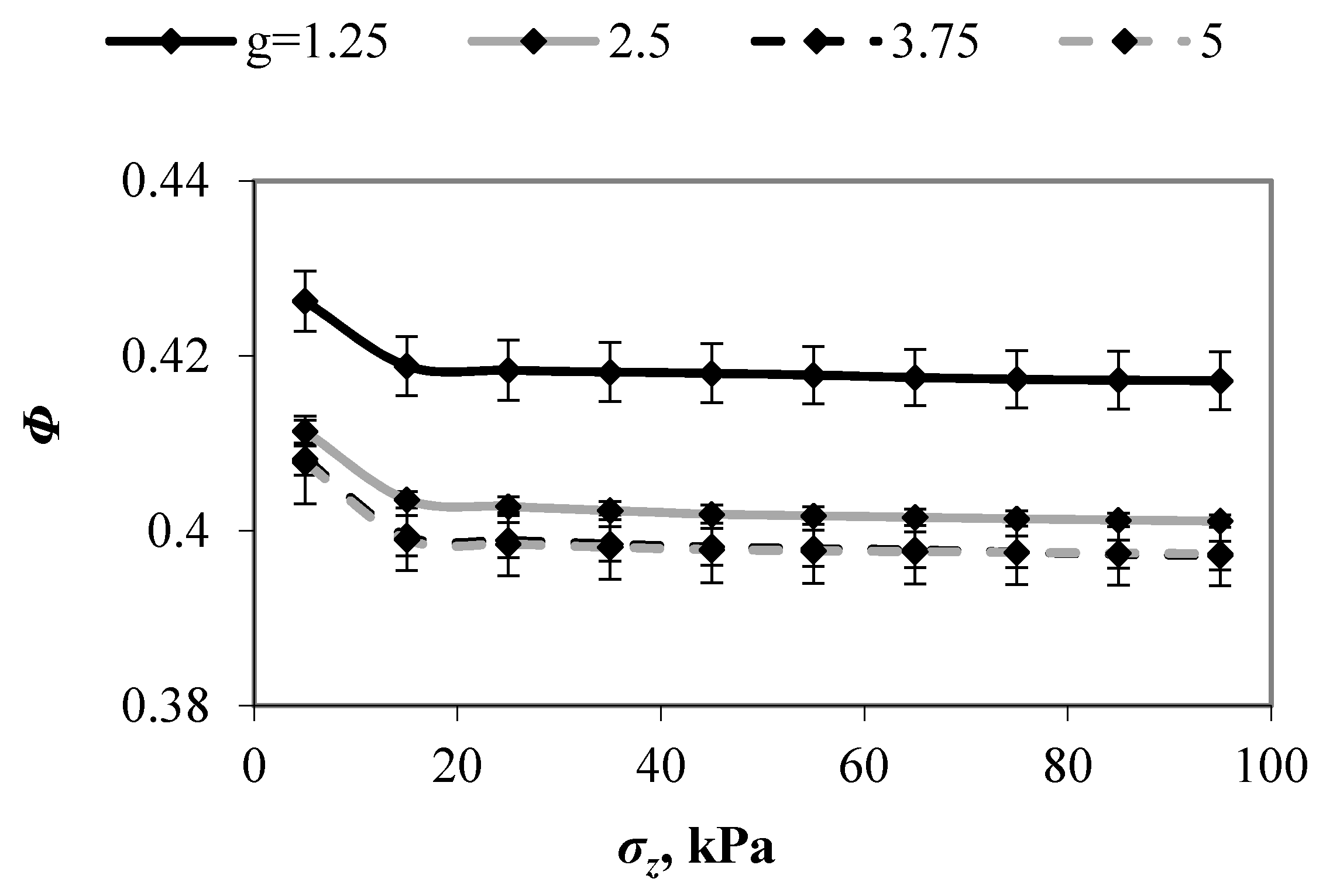
3.1. Effect of the Particle Size Ratio
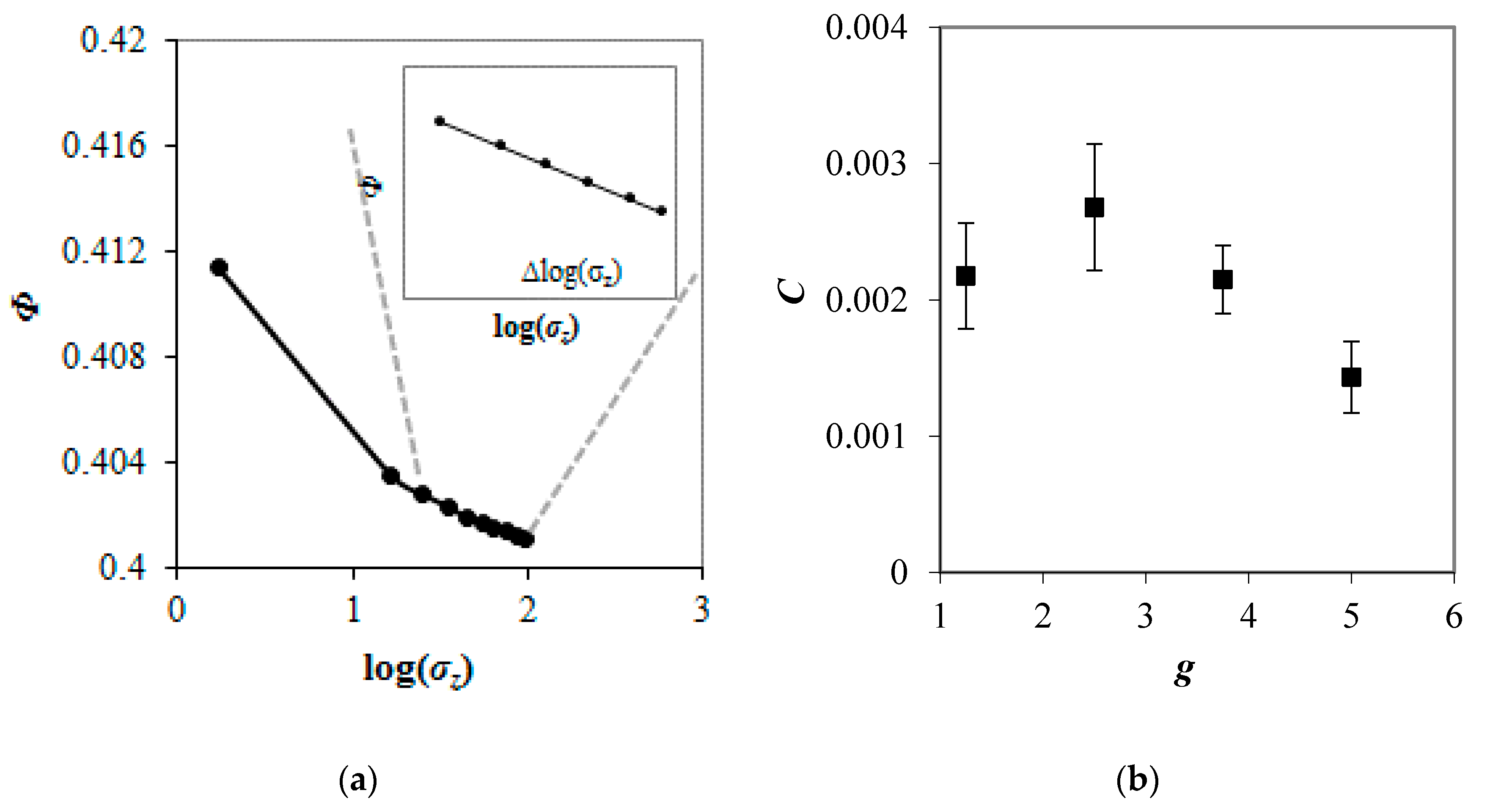
3.1.1. Porosity and Compression Index
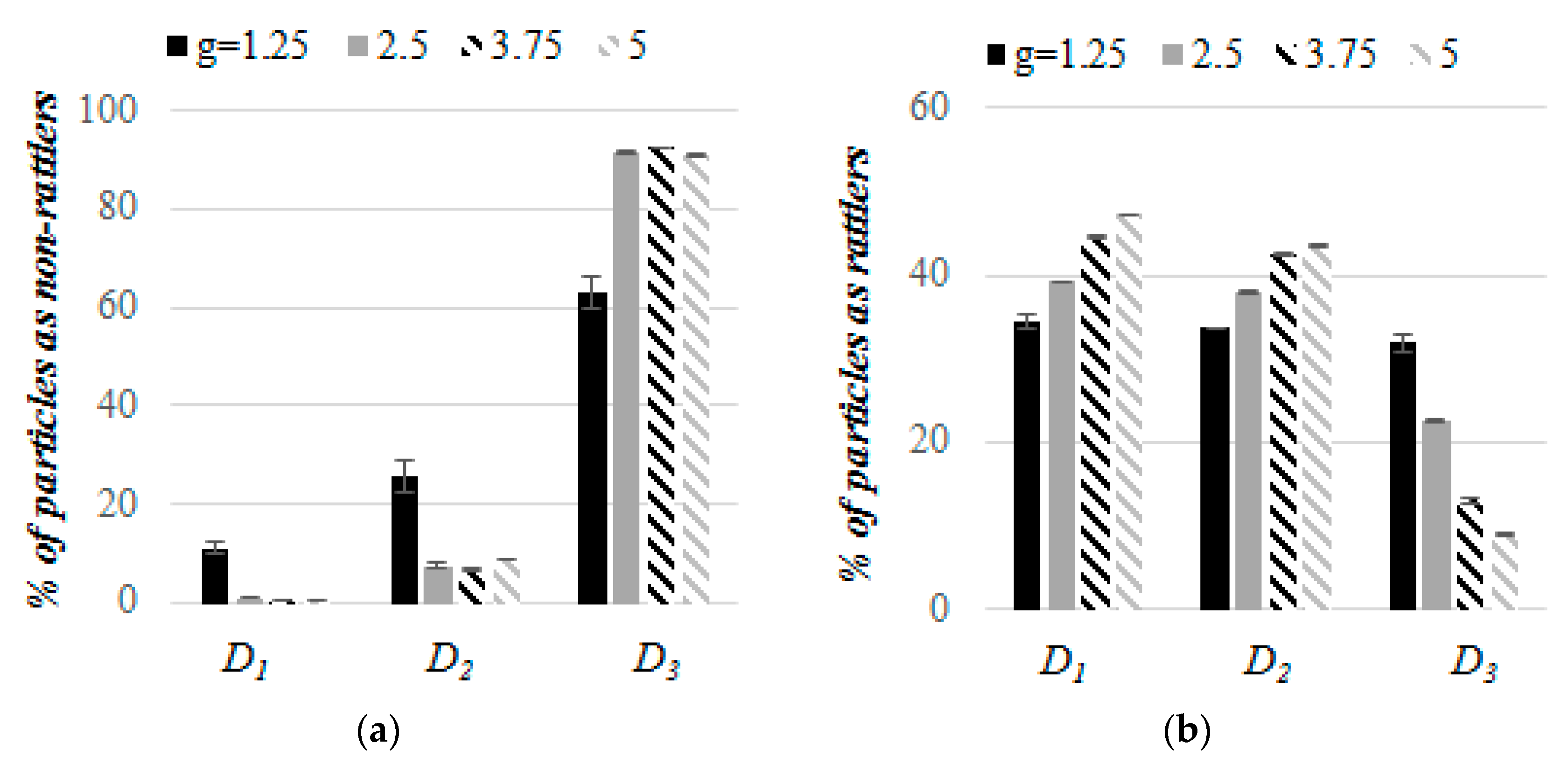
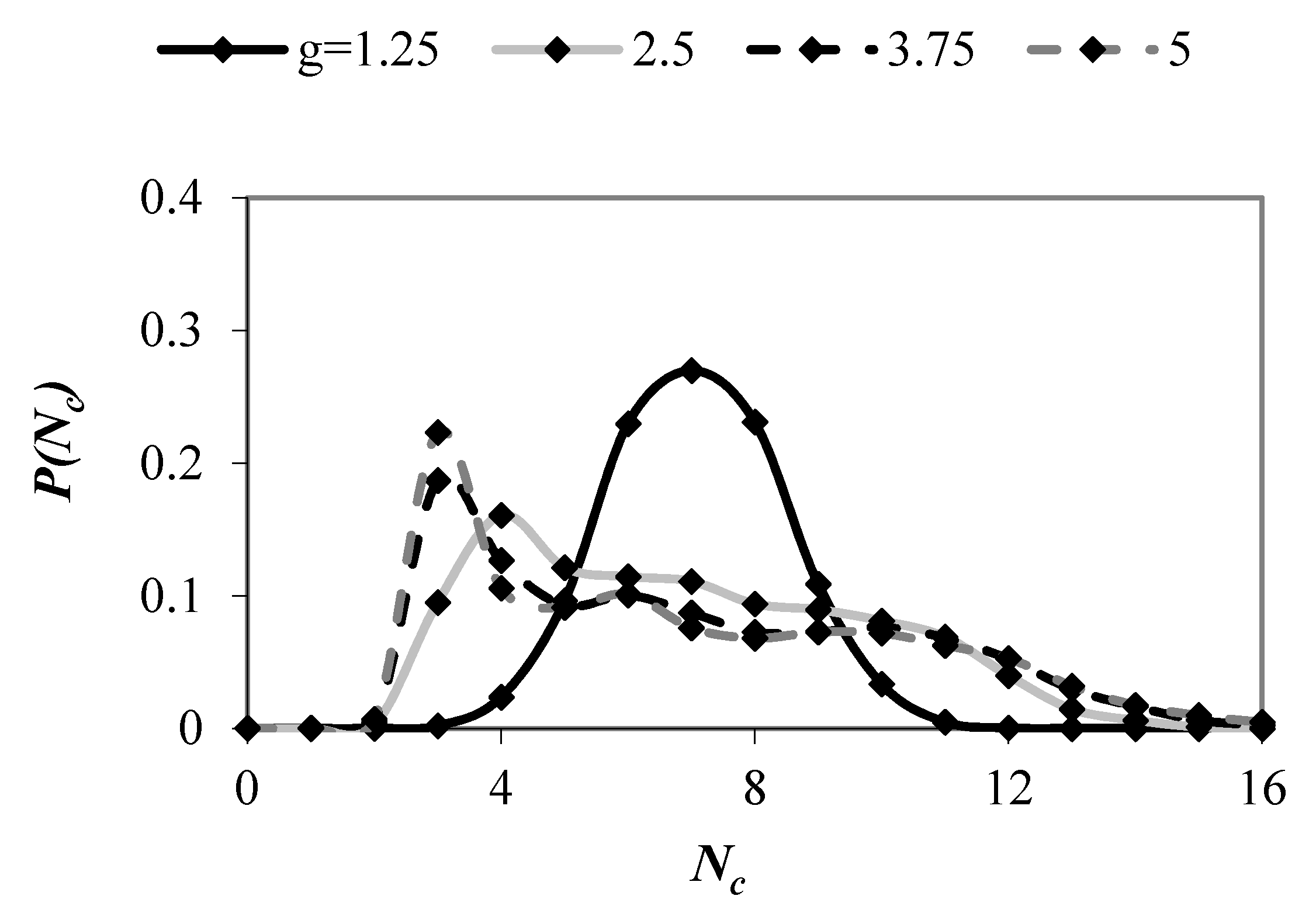
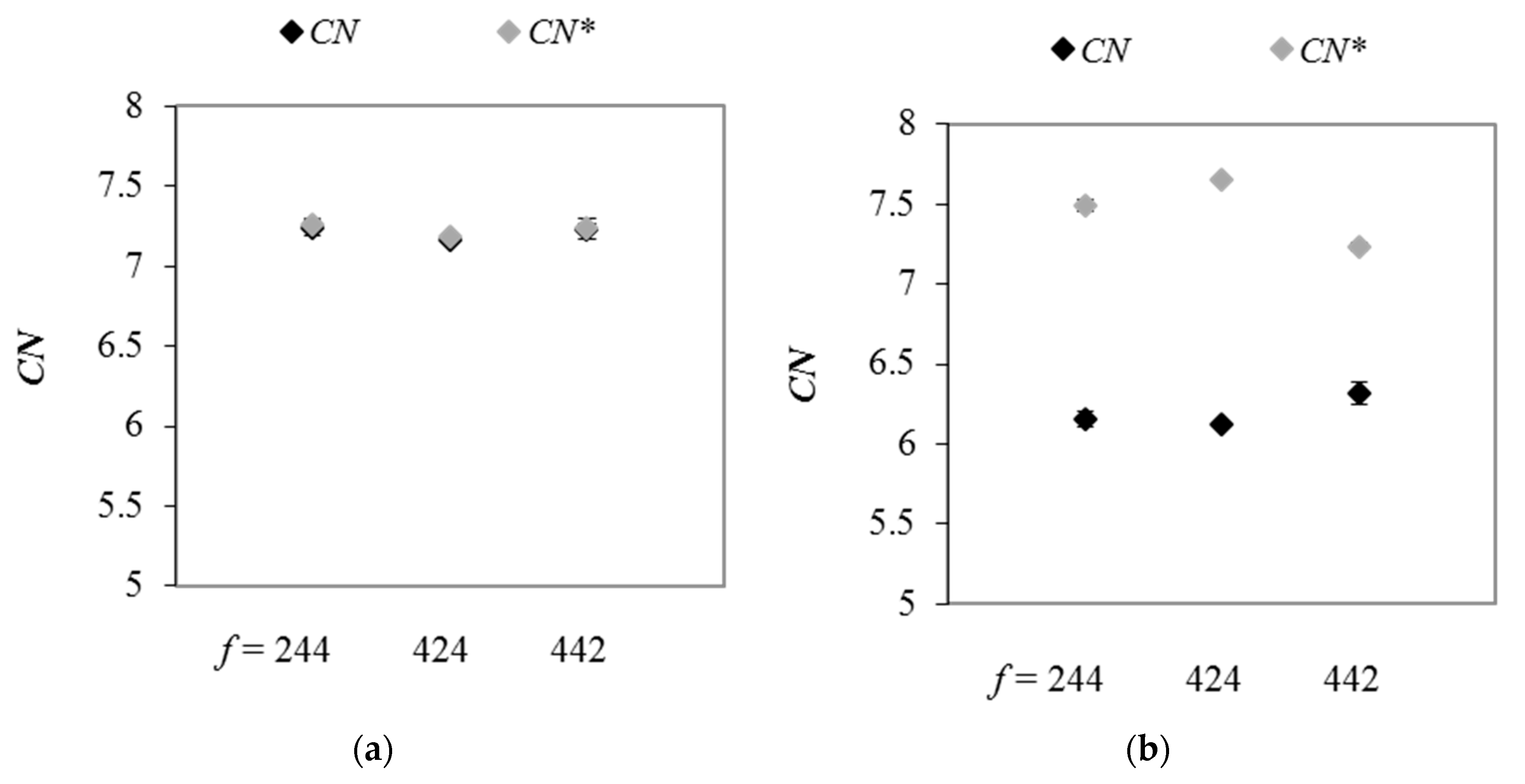
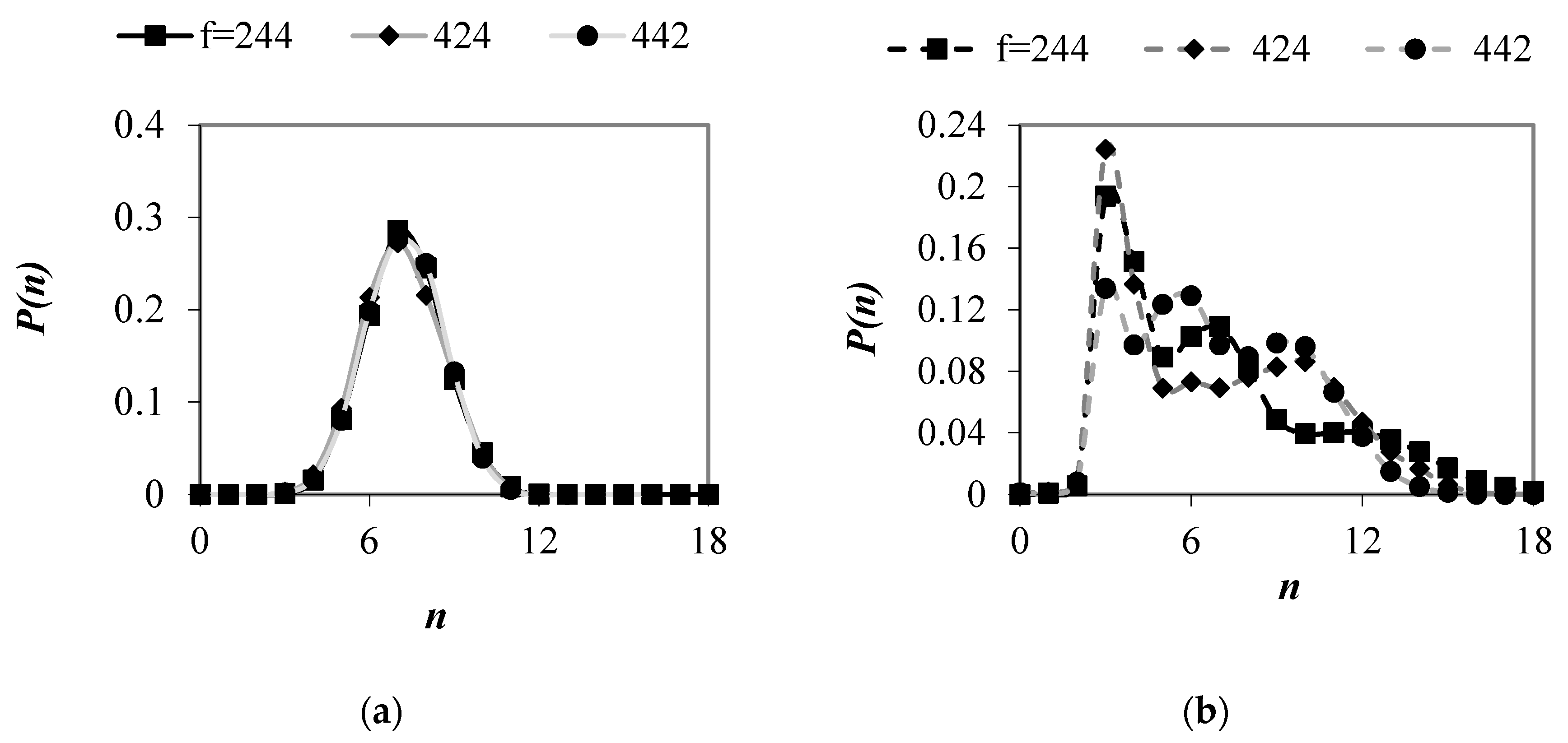
3.1.2. Coordination Numbers
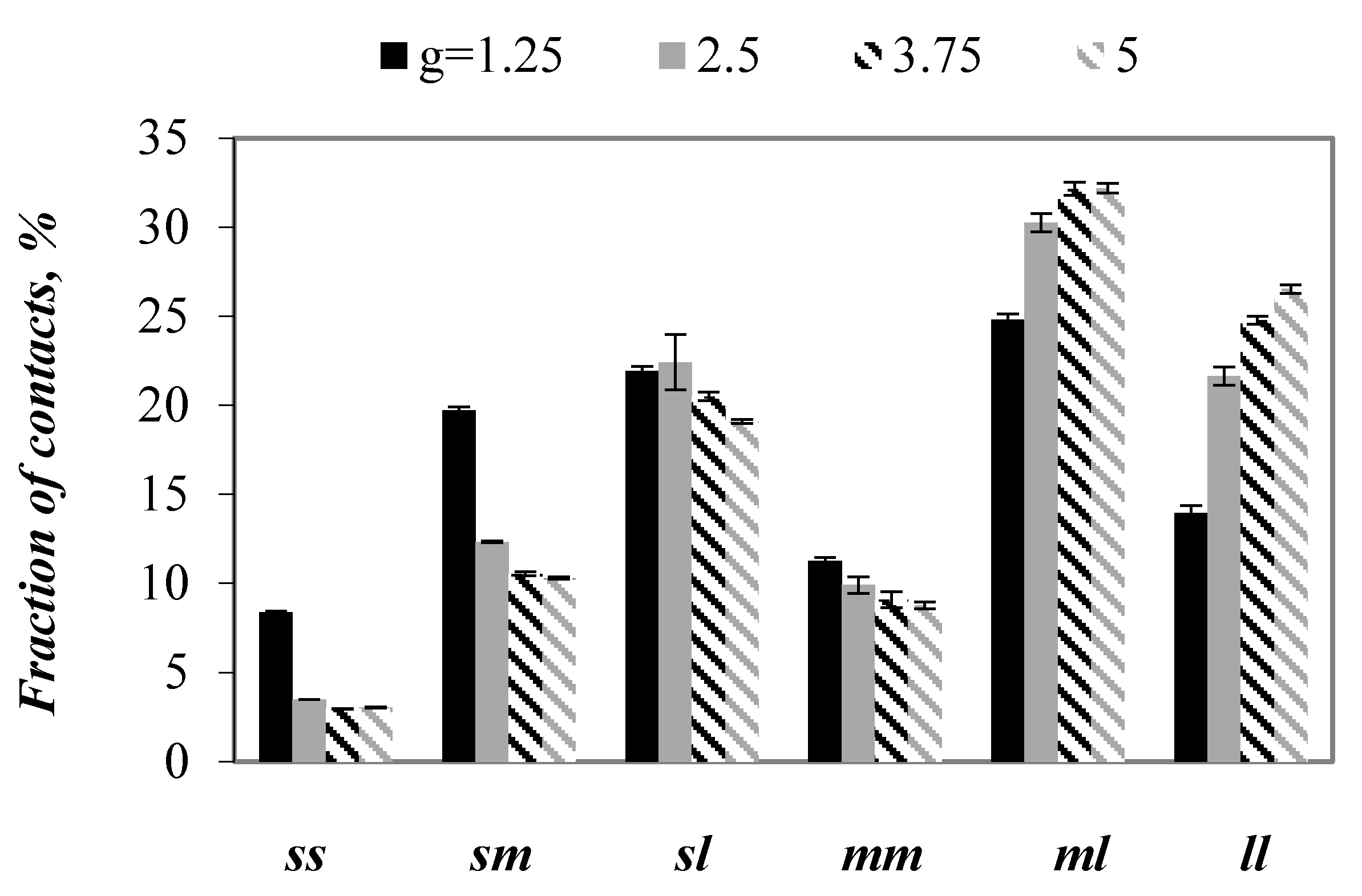
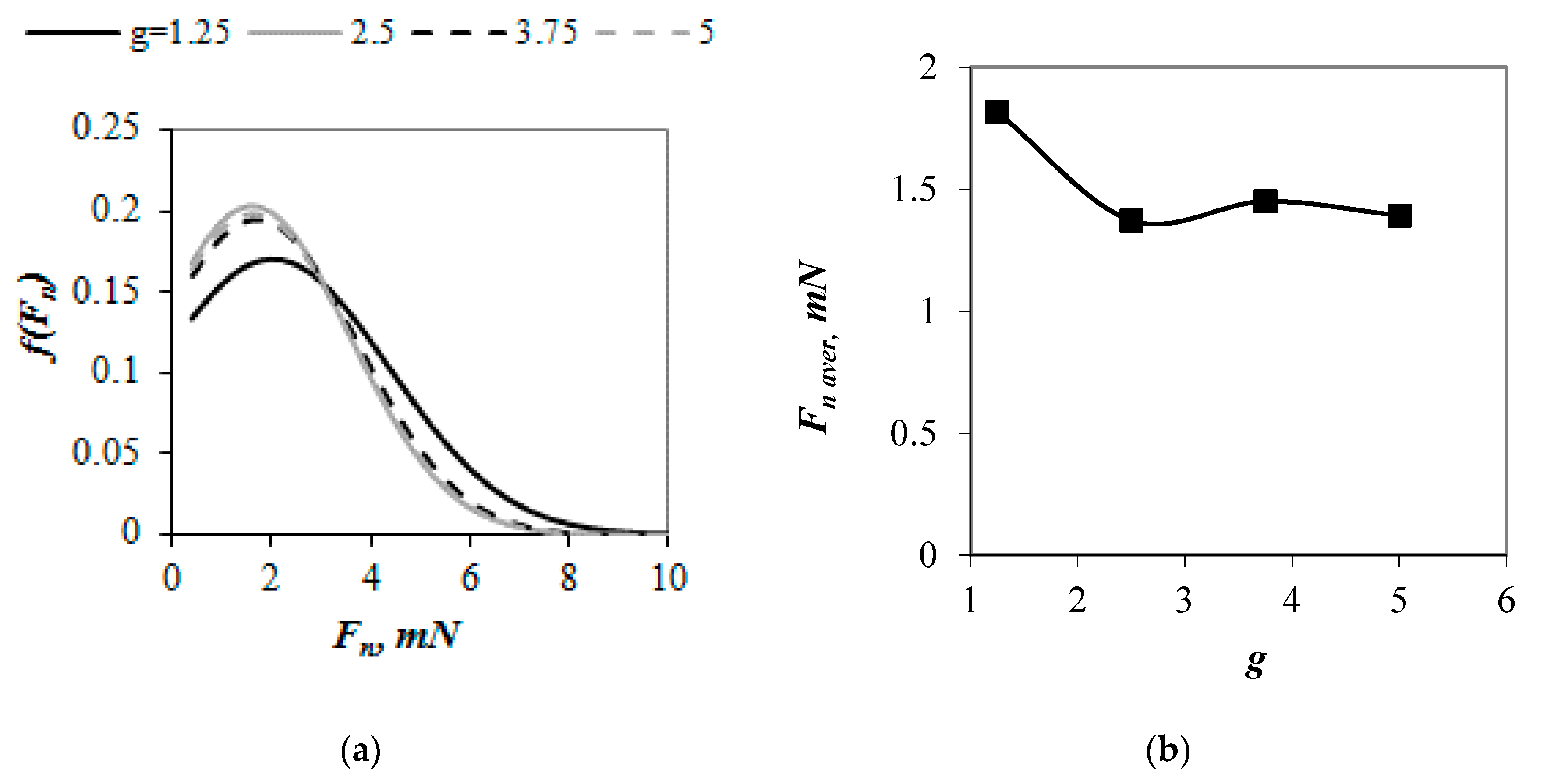
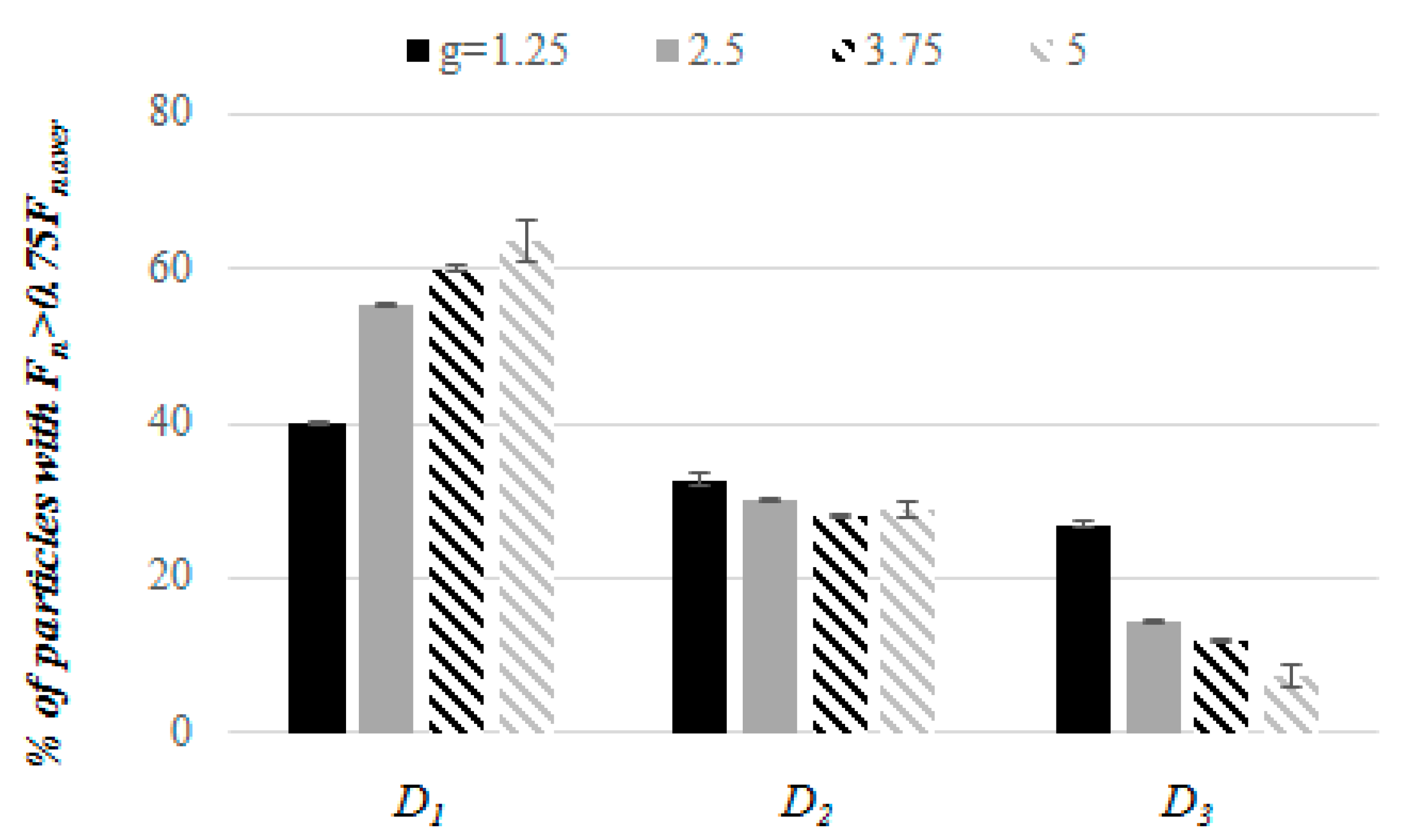
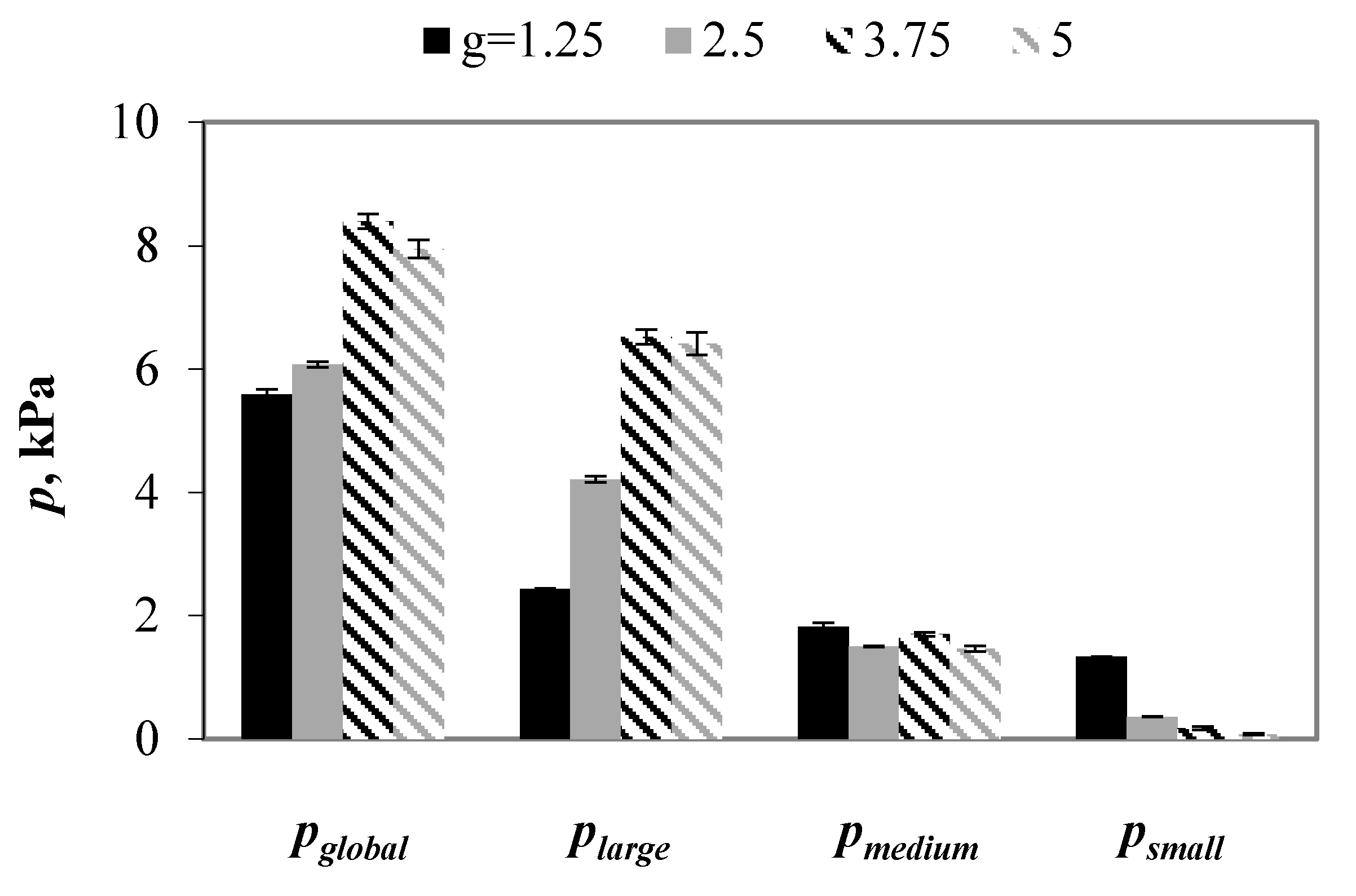
3.1.3. Contact Forces and Stress Transmission through the Contacts
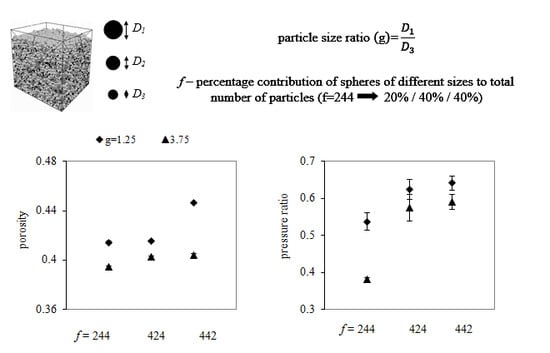
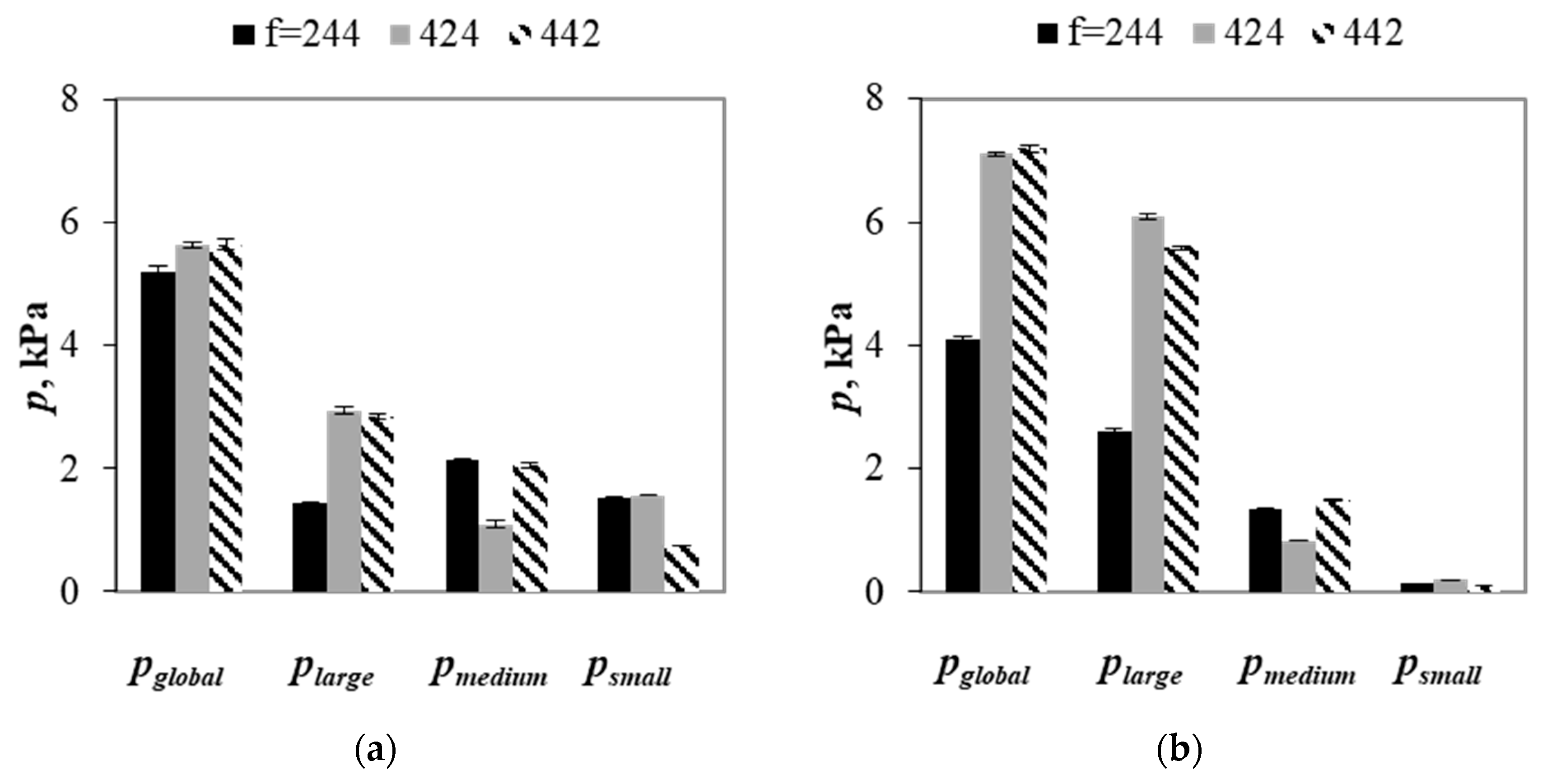
3.2. Effect of the Number Fraction of Particle Size Classes
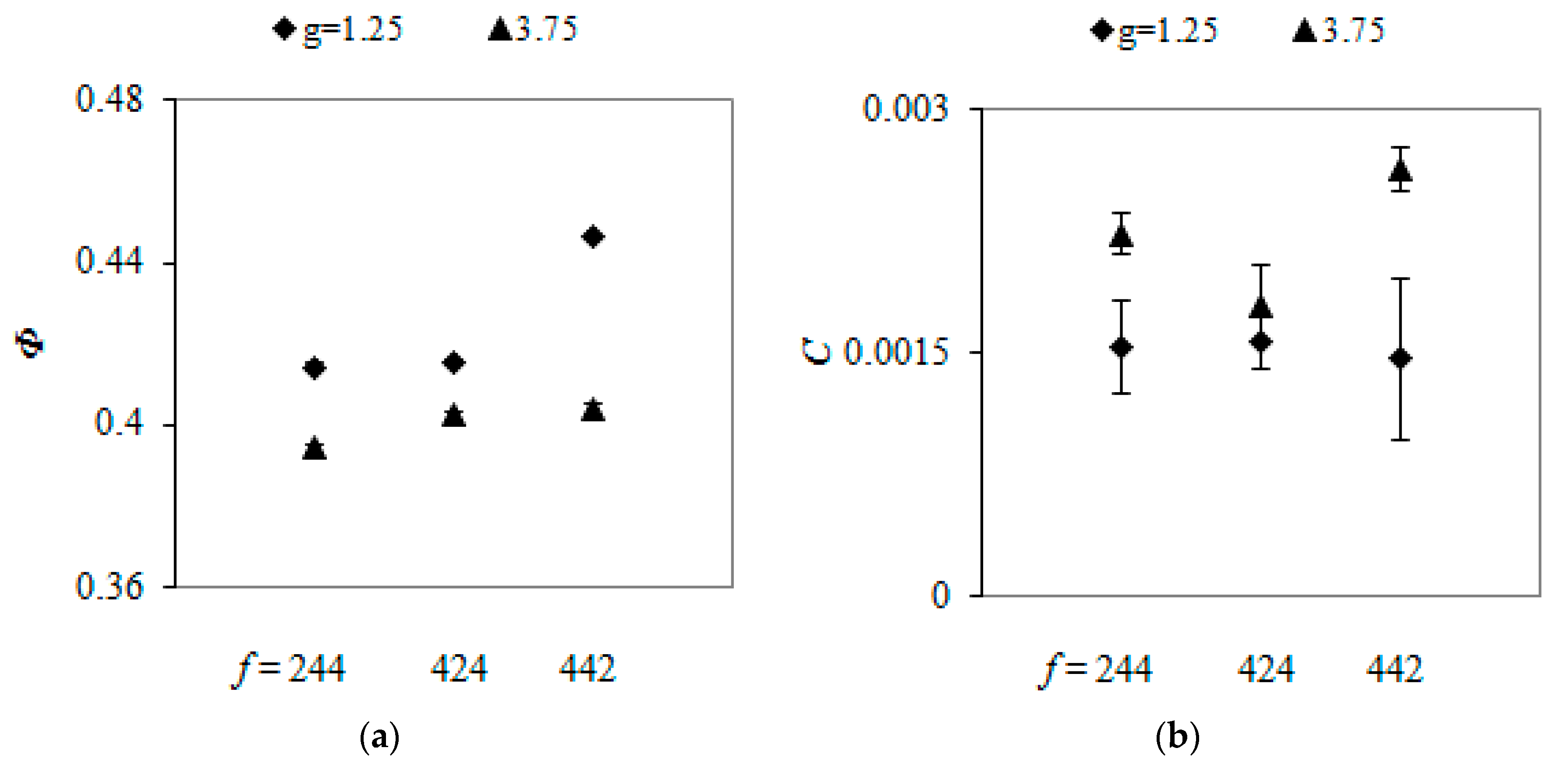
3.2.1. Porosity and Compression Index
3.2.2. Coordination Numbers
3.2.3. Stress Transmission through the Contacts
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- McGeary, R.K. Mechanical Packing of Spherical Particles. J. Am. Ceram. Soc. 1961, 44, 513–523. [Google Scholar] [CrossRef]
- Rassolusly, S.M.K. The packing density of ‘perfect’ binary mixtures. Powder Technol. 1999, 103, 145–150. [Google Scholar] [CrossRef]
- Zou, R.P.; Bian, X.; Pinson, D.; Yang, R.Y.; Yu, A.B.; Zulli, P. Coordination Number of Ternary Mixtures of Spheres. Part. Part. Syst. Char. 2003, 20, 335–341. [Google Scholar] [CrossRef]
- Wiącek, J. Geometrical parameters of binary granular mixtures with size ratio and volume fraction: Experiments and DEM simulations. Granul. Matter 2016, 18, 42. [Google Scholar] [CrossRef]
- Voivret, C.; Radjaï, F.; Delenne, J.Y.; El Youssoufi, M.S. Space-filling properties of polydisperse granular media. Phys. Rev. E 2007, 76, 021301. [Google Scholar] [CrossRef] [PubMed]
- Wiącek, J.; Molenda, M. Microstructure and micromechanics of polydisperse granular materials: Effect of the shape of particle size distribution. Powder Technol. 2014, 268, 237–343. [Google Scholar] [CrossRef]
- Sánchez, J.; Auvient, G.; Cambou, B.Ł. Coordination number and geometric anisotropy in binary sphere mixtures. In Geomechanics from Micro to Macro, Proceedings of IS-Cambridge; CRC Press: Boca Raton, FL, USA, 2014; Volume 1. [Google Scholar]
- Taha, H.; Nguyen, N.-S.; Marot, D.; Hijazi, A.; Abou-Saleh, K. Micro-scale investigation of the role of finer grains in the behavior of bidisperse granular materials. Granul. Matter 2019, 21, 28. [Google Scholar] [CrossRef]
- Westman, A.E.R.; Hugill, H.R. The packing of particles. J. Am. Ceram. Soc. 1930, 13, 767–779. [Google Scholar] [CrossRef]
- Wong, V.; Kwan, A.K.H. A 3-parameter model for packing density prediction of ternary mixes of spherical particles. Powder Technol. 2014, 268, 357–367. [Google Scholar] [CrossRef]
- Stovall, T.; De Larrard, F.; Buil, M. Linear Packing Density Model of Grain Mixtures. Powder Technol. 1986, 48, 1–12. [Google Scholar] [CrossRef]
- Yan, Y.; Zhang, L.; Luo, X.; Li, C.; Hu, F. A new method for calculating the primary porosity of unconsolidated sands based on packing texture: Application to modern beach sand. Mar. Petrol. Geol. 2018, 98, 384–396. [Google Scholar] [CrossRef]
- Kwan, A.K.H.; Chan, K.W.; Wong, V. A 3-parameter particle packing model incorporating the wedging effect. Powder Technol. 2013, 237, 172–179. [Google Scholar] [CrossRef]
- Mota, M.; Teixeira, J.A.; Yleshin, A. Image modeling of mixed granular porous media. Fluid Part. Sep. J. 1999, 12, 1–9. [Google Scholar]
- Wiącek, J.; Molenda, M.; Stasiak, M. Effect of number of granulometric fractions on structure and micromechanics of compressed granular packings. Particuology 2018, 39, 88–95. [Google Scholar] [CrossRef]
- Yi, L.Y.; Dong, K.J.; Zou, R.P.; You, A.B. Coordination Number of the Packing of Ternary Mixtures of Spheres: DEM Simulations versus Measurements. Ind. Eng. Chem. Res. 2011, 50, 8773–8785. [Google Scholar] [CrossRef]
- Martin, C.L.; Bouvard, D. Isostatic compaction of bimodal powder mixtures and composities. Int. J. Mech. Sci. 2004, 46, 907–927. [Google Scholar] [CrossRef]
- Iddir, H.; Arastoopour, H.; Hrenya, C.M. Analysis of binary and ternary granular mixtures behavior using the kinetic theory approach. Powder Technol. 2005, 151, 117–125. [Google Scholar] [CrossRef]
- Göncü, F.; Durán, O.; Luding, S. Constitutive relations for the isotropic deformation of frictionless packings of polydisperse spheres. C. R. Mecanique 2010, 338, 570–586. [Google Scholar] [CrossRef]
- Gong, J.; Nie, Z.; Zhu, Y.; Liang, Z.; Wang, X. Exploring the effects of particle shape and content of fines on the shear behavior of sand-fines mixtures via the DEM. Comput. Geotech. 2019, 106, 161–176. [Google Scholar] [CrossRef]
- Wiącek, J.; Parafiniuk, P.; Stasiak, M. Effect of particle size ratio and contribution of particle size fractions on micromechanics of uniaxially compressed binary sphere mixtures. Granul. Matter 2017, 19, 34. [Google Scholar] [CrossRef][Green Version]
- Gu, X.Q.; Yang, J. A discrete element analysis of elastic properties of granular materials. Granul. Matter 2013, 15, 139–147. [Google Scholar] [CrossRef]
- Wiącek, J.; Molenda, M. Effect of particle size distribution on micro- and macromechanical response of granular packings under compression. Int. J. Solids Struct. 2014, 51, 4189–4195. [Google Scholar] [CrossRef]
- Available online: www.dem-solutions.com/software/edem-software (accessed on 1 November 2019).
- Cundall, P.A.; Strack, O.D. A discrete element model for granular assemblies. Géotechnique 1979, 29, 47–65. [Google Scholar] [CrossRef]
- Thornton, C.; Cummins, S.J.; Cleary, P.W. An investigation of the comparative behavior of alternative contact force models during inelastic collisions. Powder Technol. 2013, 233, 30–46. [Google Scholar] [CrossRef]
- EN 1991–4. Actions on Structures. Part 4. Silos and Tanks; CEN: Brussels, Belgium, 2006. [Google Scholar]
- Wiącek, J.; Molenda, M. Representative elementary volume analysis of polydisperse granular packings using discrete element method. Particuology 2016, 27, 88–94. [Google Scholar] [CrossRef]
- Furnas, C.C. Grading Aggregates I-Mathematical Relations for Beds of Broken Solids of Maximum Density. Ind. Eng. Chem. Res. 1931, 23, 1052–1058. [Google Scholar] [CrossRef]
- Shire, T.; O’Sullivan, C.; Hanley, K. The influence of finer fraction and size-ratio on the micro-scale properties of dense bimodal materials. Geomech. Micro Macro 2014, 1, 231–236. [Google Scholar]
- Fei, K. Experimental study of the mechanical behavior of clay-aggregate mixtures. Eng. Geol. 2016, 210, 1–9. [Google Scholar] [CrossRef]
- Bodman, G.B.; Constantin, G.K. Influence of Particle Size Distribution in Soil Compaction. Hilgardia 1965, 36, 567–591. [Google Scholar] [CrossRef]
- Lade, P.V.; Yamamuro, J.A., Jr.; Liggio, C.D. Effects of fines content on void ratio, compressibility, and static liquefaction of silty sand. Geomech. Eng. 2009, 1, 1–15. [Google Scholar] [CrossRef]
- Sun, Y.; Xiao, Y.; Hanif, K.F. Compressibility dependence on grain size distribution and relative density in sands. Sci. China Technol. Sci. 2015, 58, 443–448. [Google Scholar] [CrossRef]
- Wiącek, J.; Molenda, M. Effect of particle polydispersity on micromechanical properties and energy dissipation in granular mixtures. Particuology 2014, 16, 91–99. [Google Scholar] [CrossRef]
- Taiebat, M. Mautabaruka, P.; Pellenq, R.; Radjai, F. Effect of particle size polydispersity on 3D packings of spherical particles. EPJ Web Conf. 2017, 140, 02030. [Google Scholar] [CrossRef]
- Minh, N.H.; Cheng, Y.P. On the contact force distributions of granular mixtures under 1D-compression. Granul. Matter 2016, 18, 18. [Google Scholar] [CrossRef]
- Andrade, J.E.; Avila, C.F.; Hall, S.A.; Lenoir, N.; Viggiani, G. Multiscale modeling and characterization of granular matter: From grain kinematics to continuum mechanics. J. Mech. Phys. Solids 2011, 59, 237–250. [Google Scholar] [CrossRef]


| Parameter | Poisson’s Ratio ν | Shear Modulus G | Density ρ | Coefficient of Restitution e | Coefficient of Static Friction µs | Coefficient of Rolling Friction µr | |||
|---|---|---|---|---|---|---|---|---|---|
| Value | 0.3 | 77 GPa | 7804 kg/m3 | Particle-particle | 0.4 | Particle-particle | 0.321 | Particle-particle | 0.01 |
| Particle-wall | 0.4 | Particle-wall | 0.216 | Particle-wall | 0.01 | ||||
| g | Dk, mm | N | ||
|---|---|---|---|---|
| D1 | D2 | D3 | ||
| 1.25 | 6 | 5.4 | 4.8 | 9450 |
| 2.5 | 6 | 4.2 | 2.4 | 13,800 |
| 3.75 | 6 | 3.8 | 1.6 | 18,000 |
| 5 | 6 | 3.6 | 1.2 | 18,300 |
| g | fk, % | f | Nk | N | ||||
|---|---|---|---|---|---|---|---|---|
| f1 | f2 | f3 | N1 | N2 | N3 | |||
| 1.25 | 20 | 40 | 40 | 244 | 1890 | 3780 | 3780 | 9450 |
| 40 | 20 | 40 | 424 | 3780 | 1890 | 3780 | 9450 | |
| 40 | 40 | 20 | 442 | 3780 | 3780 | 1890 | 9450 | |
| 3.75 | 20 | 40 | 40 | 244 | 3000 | 6000 | 6000 | 15,000 |
| 40 | 20 | 40 | 424 | 6000 | 3000 | 6000 | 15,000 | |
| 40 | 40 | 20 | 442 | 6000 | 6000 | 3000 | 15,000 | |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wiącek, J.; Stasiak, M.; Kafashan, J. Structural and Micromechanical Properties of Ternary Granular Packings: Effect of Particle Size Ratio and Number Fraction of Particle Size Classes. Materials 2020, 13, 339. https://doi.org/10.3390/ma13020339
Wiącek J, Stasiak M, Kafashan J. Structural and Micromechanical Properties of Ternary Granular Packings: Effect of Particle Size Ratio and Number Fraction of Particle Size Classes. Materials. 2020; 13(2):339. https://doi.org/10.3390/ma13020339
Chicago/Turabian StyleWiącek, Joanna, Mateusz Stasiak, and Jalal Kafashan. 2020. "Structural and Micromechanical Properties of Ternary Granular Packings: Effect of Particle Size Ratio and Number Fraction of Particle Size Classes" Materials 13, no. 2: 339. https://doi.org/10.3390/ma13020339
APA StyleWiącek, J., Stasiak, M., & Kafashan, J. (2020). Structural and Micromechanical Properties of Ternary Granular Packings: Effect of Particle Size Ratio and Number Fraction of Particle Size Classes. Materials, 13(2), 339. https://doi.org/10.3390/ma13020339