Estimation of the Dissipative Heat Sources Related to the Total Energy Input of a CFRP Composite by Using the Second Amplitude Harmonic of the Thermal Signal
Abstract
1. Introduction
2. Theory: Fatigue Energy Assessments in Composites
2.1. Total Energy Input
2.2. Second Amplitude Harmonic of the Thermal Signal
3. Material and Experimental Campaign
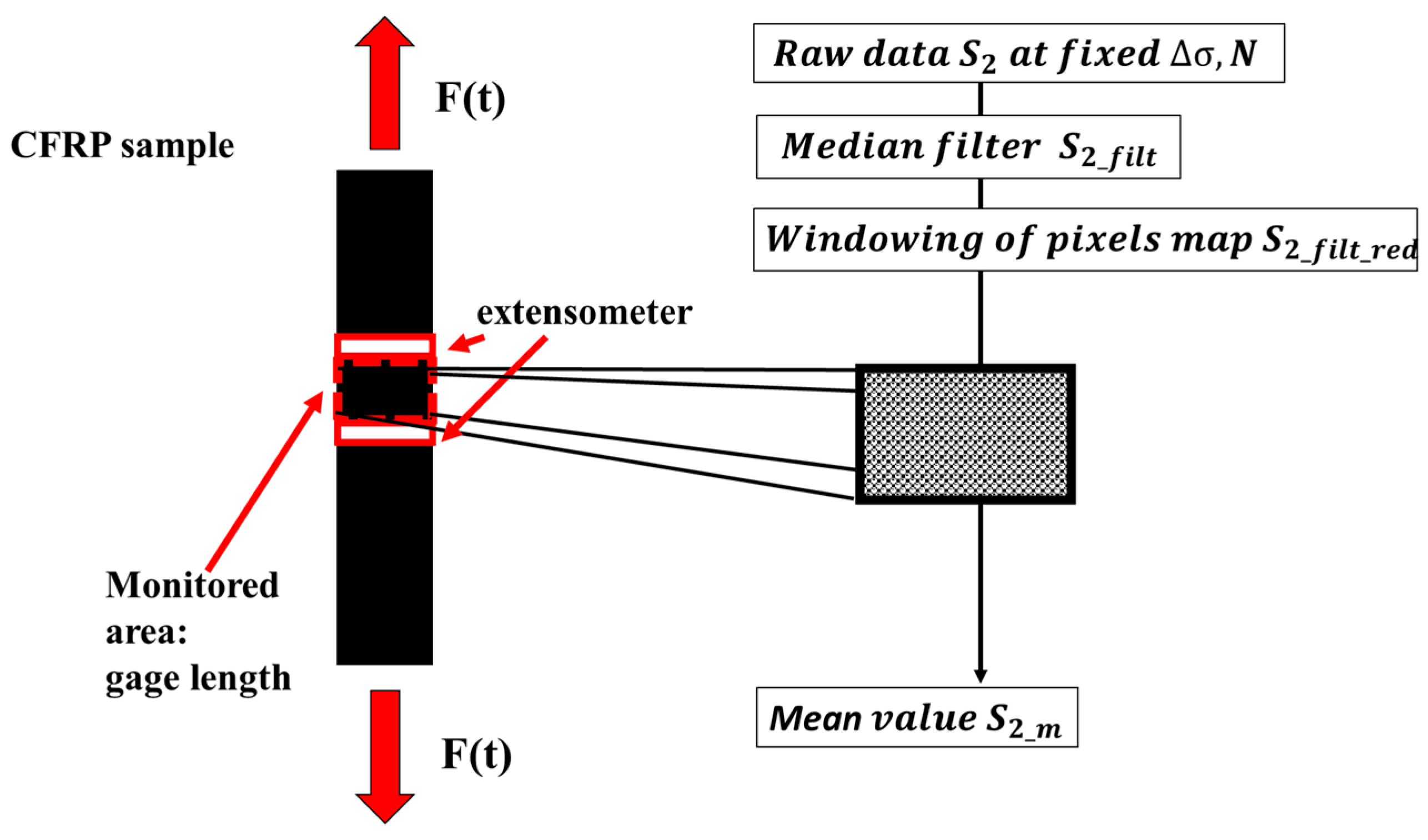
4. Data Processing
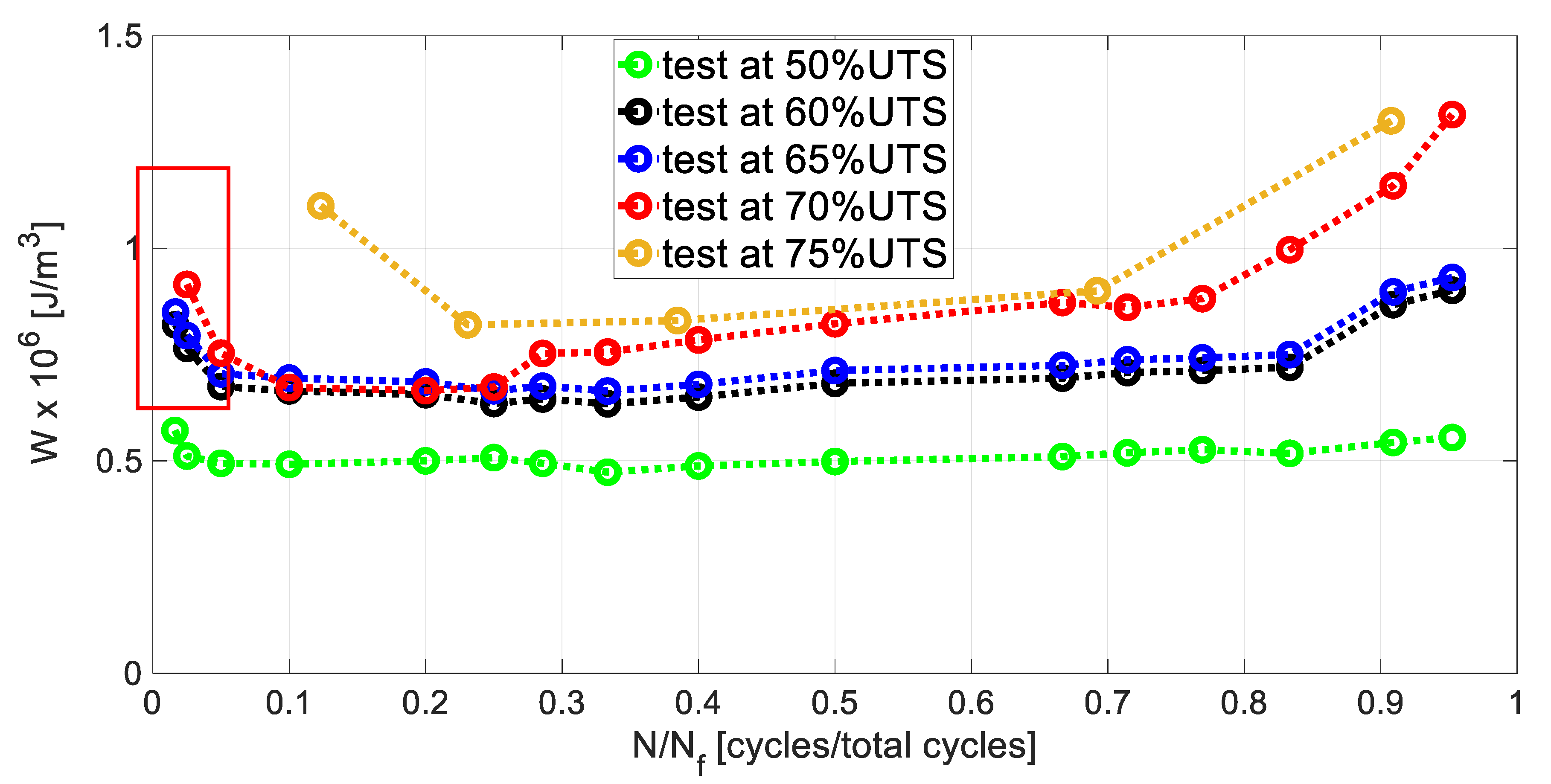
4.1. Hysteresis Loop Measurements (W)
- assessment of the strain-stress couples (εi, σi) of the N-th cycle. One cycle is composed of 16 (εi, σi) data couples;
- finding the maximum (minimum) stresses (σmax) imax and (σmin) imin where the indexes imax (min) represent the values of the index i in correspondence of the maximum (minimum) stresses, respectively, σmax and σmin;
- finding the extremities of the hysteresis loop in term of the couples:
- ○
- ε(σmax)imax, (σmax)imax;
- ○
- ε(σmin)imin, (σmin)imin;
- integration of the stress-strain data (εi, σi), i = imax:imax + 8, via the trapezoidal method to assess Wlower;
- integration of the stress-strain data (εi, σi), i = imin:imin + 8, via the trapezoidal method to assess Wupper; and,
- assessment of energy input per cycle W = Wupper − Wlower.
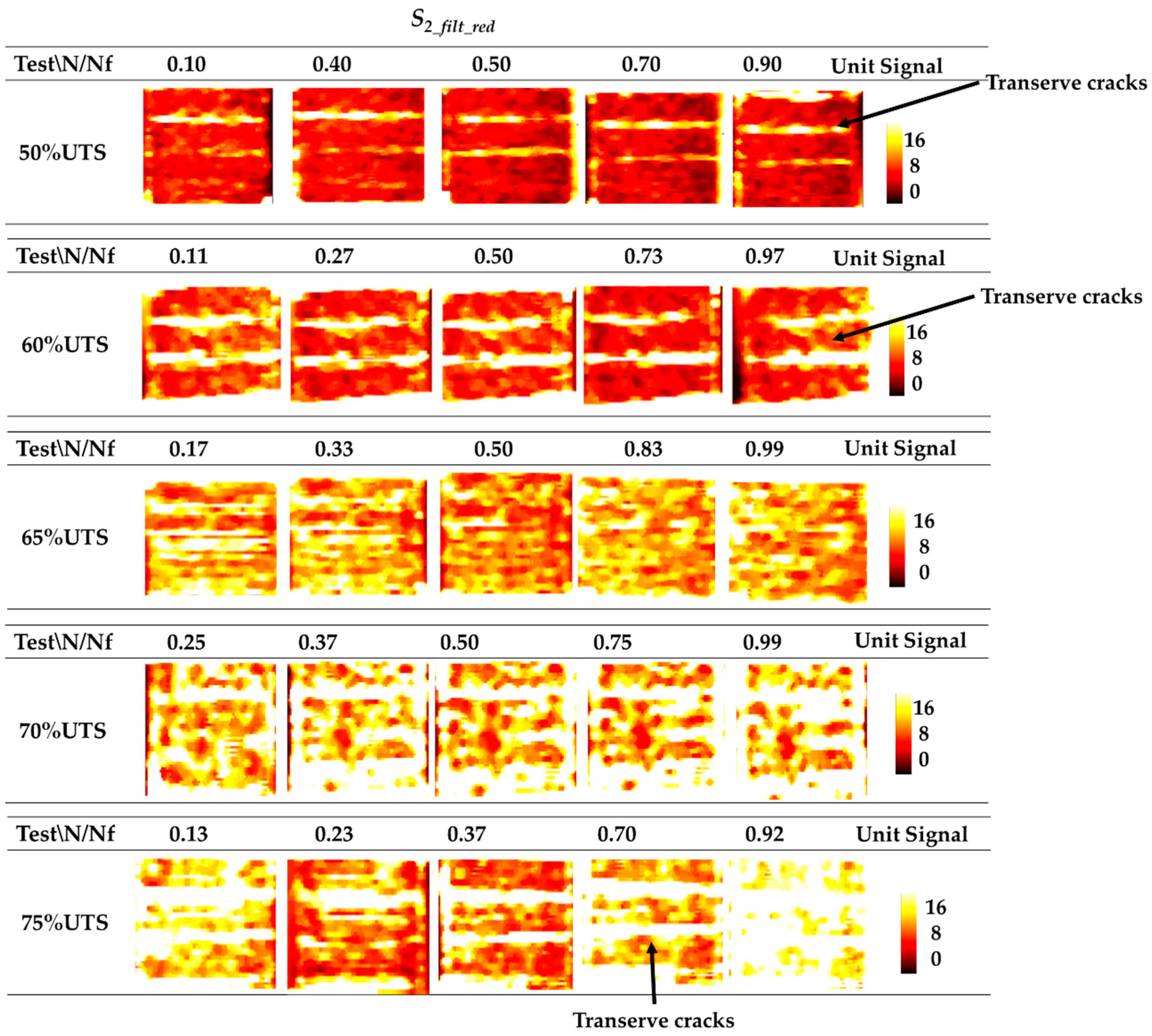
4.2. Thermal Signal Analysis
- application of the two-dimension spatial median filter (Medfilt2) to obtain S2_filt. Each output pixel contains the median value in a 3-by-3 neighbourhood around the corresponding pixel in the input image;
- reduction of the thermal scene to the gage length area to obtain S2_filt_red, to make the signal value in the same area considered for extensometer analysis; and,
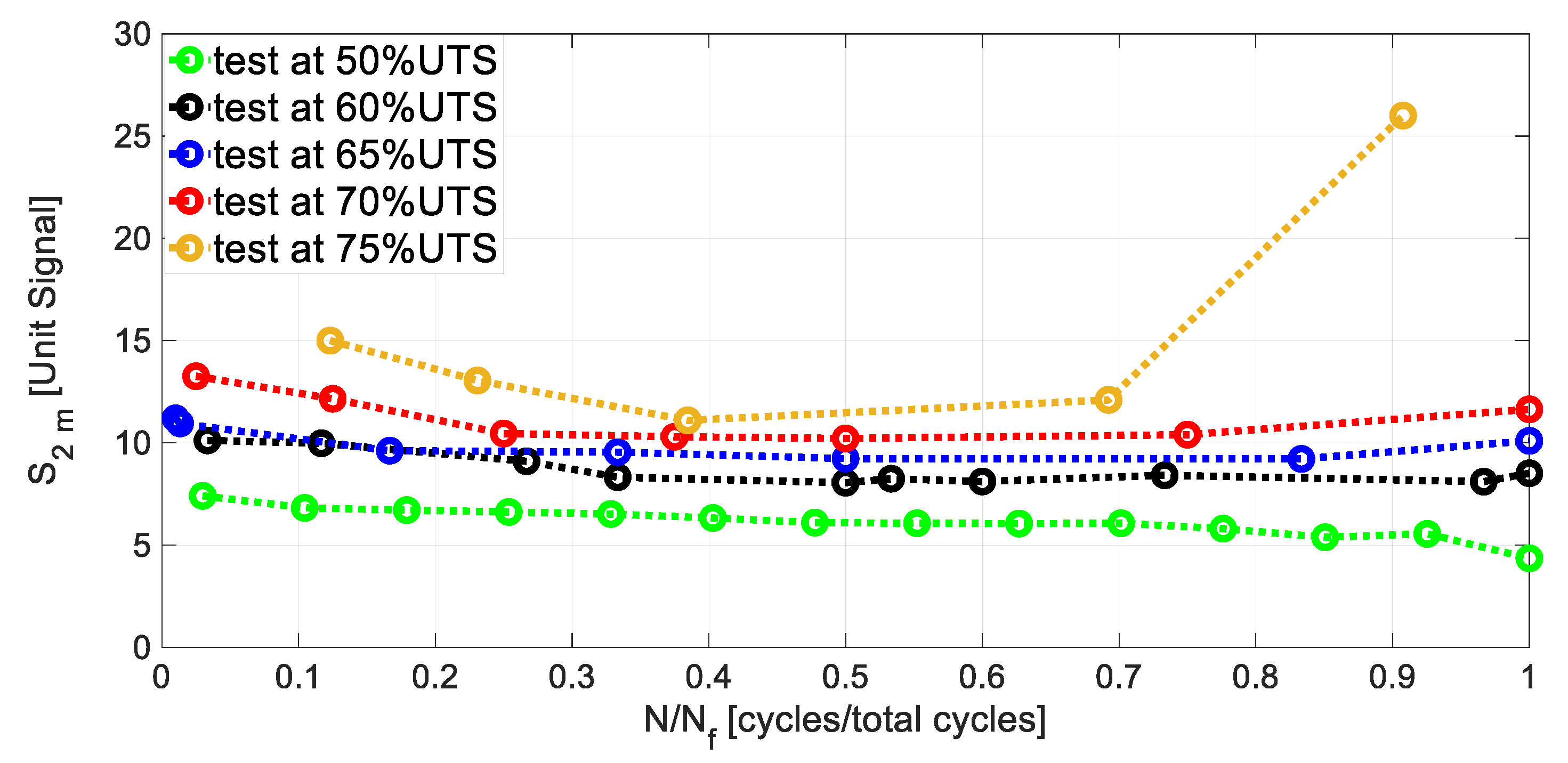
- evaluation of the mean value of the signal S2_m.
5. Results
5.1. Area under Hysteresis Loop (W) and Evolution through Constant Amplitude Loadings
5.2. Second Harmonic Amplitude of the Temperature Signal Related to the Energy Dissipated
6. Discussion
6.1. Procedure to Assess a Damage State Parameter for the Specific Stress Level
6.2. Assessment of the Fatigue Curve by Means of Total Energy Input Estimations
7. Conclusions
- the realization of a real-time damage monitoring,
- the assessment of the fatigue behaviour of real components where it is difficult to measure the area under the hysteresis loop by means of an extensometer, and
- the assessment of parameters capable of validating the theoretical and numerical models.
- the validation of the present approach on real components; and,
- the determination of S/N curve by using just thermal data.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Es | energy stored in the material a cycle [J/m3] |
| Ed | energy dissipated as heat in the material a cycle [J/m3] |
| N | actual number of cycles |
| N/Nf | ratio between actual cycles and cycles at failure |
| Q | energy exchanged between material and environment in a cycle [J/m3] |
| UTS | ultimate tensile strength of the material [MPa] |
| R | loading ratio |
| thermal signal | |
| S2_m | processed value of the second harmonic amplitude of the thermal signal: mean value in the gage length |
| S0 | mean temperature signal |
| S1 | amplitude of the first harmonic amplitude of the thermal signal |
| S2 | the second harmonic amplitude of the thermal signal |
| S2_M | mean value in the steady state condition of the S2_m data series [Unit Signal] |
| W | total energy input per unit volume in a cycle [J/m3] |
| Wmech | mean value in the steady state condition of the W data series [J/m3] |
| Wtherm | total energy input obtained by means of the calibration between Wmech and S2_M [J/m3] |
| ΔU | internal energy changes of the material a cycle [J/m3] |
| Δσ | is the stress amplitude |
| ε | actual strain of the hysteresis loop |
| σ | actual stress of the hysteresis loop |
| σWmech | stresses obtained by using Ellyn’s model and W data series [MPa] |
| σWtherm | stresses obtained by using Ellyn’s model and Wtherm data series [MPa] |
| φi | phase shifts of the harmonics (i = 1,2). |
References
- Carswell, W.S. Damage Mechanics and Composite Behaviour. Compos. Struct. 1988, 10, 335–342. [Google Scholar] [CrossRef]
- Ellyin, E.; El-Kadi, H. A Fatigue Failure Criterion for Fiber Reinforced Composite Laminae. Compos. Struct. 1990, 15, 61–74. [Google Scholar] [CrossRef]
- Degrieck, J.; Van Paepegem, W. Fatigue damage modeling of fibre-reinforced composite materials: Review. Appl. Mech. Rev. 2001, 54, 279–300. [Google Scholar] [CrossRef]
- Huanga, J.; Pastor, M.L.; Garnier, C.; Gong, X.J. A new model for fatigue life prediction based on infrared thermography and degradation process for CFRP composite laminates. Int. J. Fatigue 2019, 120, 87–95. [Google Scholar] [CrossRef]
- Ogin, S.L.; Smith, P.A.; Beaumont, P.W.R. Matrix cracking and stiffness reduction during the fatigue of a ~0/90s GFRP laminate. Compos. Sci. Technol. 1985, 22, 23–31. [Google Scholar] [CrossRef]
- Beaumont, P.W.R. The fatigue damage mechanics of composite laminates. In Wang ASD and Haritos GKeds, Damage Mechanics in Composites, Proceedings of the Winter Annual Meeting of the ASME, Boston, MA, USA, 13–18 December 1987; ASME: New York, NY, USA, 1987; pp. 53–63. [Google Scholar]
- Spearing, S.M.; Beaumont, P.W.R. Fatigue damage mechanics of composite materials. I: Experimental measurement of damage and post-fatigue properties. Compos. Sci. Technol. 1992, 44, 159–168. [Google Scholar] [CrossRef]
- Sendeckyj, G.P. Life prediction for resin-matrix composite materials. In Fatigue of Composite Materials; Composite Material Series 4; Elsevier: Amsterdam, The Netherlands, 1991; pp. 431–483. [Google Scholar]
- Highsmith, A.L.; Reifsnider, K.L. Stiffness-Reduction Mechanisms in Composite Laminates. Damage in Composite Materials; ASTM STP 775; American Society for Testing and Materials: West Conshohocken, PA, USA, 1982; pp. 103–117. [Google Scholar]
- Naderi, M.; Khonsari, M.M. On the role of damage energy in the fatigue degradation characterisatin of a composite laminate. Compos. Part B 2013, 45, 528–537. [Google Scholar] [CrossRef]
- Talreja, R.; Yalvac, S.; Yats, L.D.; Wetters, D.G. Transverse cracking and stiffness reduction in cross-ply laminates of different matrix toughness. J. Compos. Mater. 1992, 26, 1644–1663. [Google Scholar] [CrossRef]
- Carraro, P.A.; Maragoni, L.; Quaresimin, M. Prediction of the crack density evolution in multidirectional laminates under fatigue loading. Comp. Sci. Tech. 2017, 145, 24–39. [Google Scholar] [CrossRef]
- Johnson, P.; Chang, F. Characterization of matrix crack-induced laminate failure—Part I: Experiments. J. Compos. Mater. 2001, 35, 2009–2035. [Google Scholar] [CrossRef]
- Takeda, N.; Ogihara, S. Initiation and growth of delamination from the tips of transverse cracks in cfrp cross-ply laminates. Compos. Sci. Technol. 1994, 52, 309–318. [Google Scholar] [CrossRef]
- Golos, K.; Ellyin, F. A Total Strain Energy Density Theory for Cumulative Fatigue Damage. J. Press. Vessel. Technol. 1988, 110, 36–41. [Google Scholar] [CrossRef]
- Pakdel, H.; Mohammadi, B. Stiffness degradation of composite lamintates due to matrix cracking and induced delamintion during tension-tension fatigue. Eng. Fract. Mech. 2019, 216, 1–11. [Google Scholar] [CrossRef]
- Dharan, C.K.H.; Tan, T.F. A hysteresis-based damage parameter for notched composite laminates subjected to cyclic loading. J. Mater. Sci. 2007, 42, 2204–2207. [Google Scholar] [CrossRef]
- Natarajan, V.; GangaRao, H.V.S.; Shekar, V. Fatigue response of fabric-reinforced polymeric composites. J. Compos. Mater. 2005, 39, 1541–1559. [Google Scholar] [CrossRef]
- Varvani-Farahani, A.; Haftchenari, H.; Panbechi, M. An energy-based fatigue damage parameter for off-axis unidirectional FRP composites. Compos. Struct. 2007, 79, 381–389. [Google Scholar] [CrossRef]
- Plumtree, A.; Cheng, G.X. A fatigue damage parameter for off-axisunidirectional fiber-reinforced composites. Int. J. Fatigue 1999, 21, 849–856. [Google Scholar] [CrossRef]
- Behera, A.; Dupare, P.; Thawre, M.M.; Ballal, A.R. Effect of fatigue loading on stiffness degradation, energy dissipation, and matrix cracking damage of CFRP [+/−45](3S) composite laminate. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 2302–2314. [Google Scholar] [CrossRef]
- Liu, H.; Cui, H.; Wen, W.; Kang, H. Fatigue characterization of T300/924 polymer composites with voids under tension-tension and compression-compression cyclic loading. Fatigue Fract. Eng. Mater. Struct. 2018, 41, 597–610. [Google Scholar] [CrossRef]
- Tsamtsakis, D.; Wevers, M.; De Meester, P. Acoustic emission of CFRP laminates during fatigue loading. J. Reinf. Plast. Compos. 1998, 17, 1185–1201. [Google Scholar] [CrossRef]
- Unnthorsson, R.; Runarsson, T.P.; Jonsson, M.T. AE entropy for the conditionmonitoring of CFRP subjected to cyclic fatigue. J. Acoust. Emiss. 2008, 26, 262–269. [Google Scholar]
- Bourchaka, M.; Farrowa, I.R.; Bonda, I.P.; Rowland, C.W.; Menana, F. Acoustic emission energy as a fatigue damage parameter for CFRP composites. Int. J. Fatigue 2007, 29, 457–470. [Google Scholar] [CrossRef]
- Shukla, A.; Agarwal, B.D.; Bhushan, B. Determination of stress intensity factor in orthotropic composite materials using strain gages. Author links open overlay panel. Eng. Fract. Mech. 1989, 32, 469–477. [Google Scholar] [CrossRef]
- Périé, J.N.; Calloch, S.; Cluzel, C.; Hild, F. Analysis of a multiaxial test on a C/C composite by using digital image correlation and a damage model. Exp. Mech. 2002, 42, 318–328. [Google Scholar] [CrossRef]
- Goidescu, C.; Welemane, H.; Garnier, C.; Fazzini, M.; Brault, R.; Peronnet, E.; Mistou, S. Damage investigation in CFRP composites using fullfield measurement techchniques: Combination of digital image stereo-correlation, infrared thermography and X-ray tomography. Compos. Part B Eng. 2013, 48, 95–105. [Google Scholar] [CrossRef]
- Toubal, L.; Karama, M.; Lorrain, B. Damage evolution and infrared thermography in woven composite laminates under fatigue loading. Int. J. Fatigue 2006, 28, 1867–1872. [Google Scholar] [CrossRef]
- Montesano, F.; Fawaz, Z.; Bougherara, H. Use of infrared thermography to investigate the fatigue behavior of a carbon fiber reinforced polymer composite b. Compos. Struct. 2013, 97, 76–83. [Google Scholar] [CrossRef]
- De Finis, R.; Palumbo, D.; Ancona, F.; Galietti, U. Fatigue Limit Evaluation of Various Martensitic Stainless Steels with New Robust Thermographic Data Analysis. Int. J Fatigue 2015, 74, 88–96. [Google Scholar] [CrossRef]
- Sarker, M.; Ali Hadigheh, S.; Dias-da-Costaa, D. A performance-based characterization of CFRP composite deterioration using active infrared thermography. Compos. Struct. 2020, 241, 112134. [Google Scholar] [CrossRef]
- Emery, T.R.; Dulieu-Barton, J.M. Thermoelastic Stress Analysis of damage mechanisms in composite materials. Compos. Part A 2010, 41, 1729–1742. [Google Scholar] [CrossRef]
- Shiozawa, D.; Sakagami, T.; Nakamura, Y.; Nonaka, S.; Hamada, K. Fatigue Damage Evaluation of Short Carbon Fiber Reinforced Plastics Based on Phase Information of Thermoelastic Temperature Change. Sensors 2017, 17, 2824. [Google Scholar] [CrossRef] [PubMed]
- De Finis, R.; Palumbo, D.; Galietti, U. A multianalysis thermography-based approach for fatigue and damage investigations of ASTM A182 F6NM steel at two stress ratios. Fatigue Fract. Eng. Mater. Struct. 2019, 42, 267–283. [Google Scholar] [CrossRef]
- Palumbo, D.; De Finis, R.; Demelio, G.P.; Galietti, U. A new rapid thermographic method to assess the fatigue limit in GFRP composites. Compos. Part B Eng. 2016, 103, 60–67. [Google Scholar] [CrossRef]
- De Finis, R.; Palumbo, D.; Galietti, U. Fatigue damage analysis of composite materials using thermography-based techniques. Procedia Struct. Integr. 2019, 18, 781–791. [Google Scholar] [CrossRef]
- Lisle, T.; Bouvet, C.; Pastor, M.L.; Margueres, P.; Corral, R.P. Damage analysis and fracture toughness evaluation in a thin woven composite laminate under static tension using infrared thermography. Compos. Part A 2013, 53, 75–87. [Google Scholar] [CrossRef][Green Version]
- Longbiao, L. A hysteresis dissipated energy-based damage parameter for lifeprediction of carbon fiber-reinforced ceramic-matrix composites under fatigue loading. Compos. Part B 2015, 82, 108–128. [Google Scholar] [CrossRef]
- Sakagami, T.; Kubo, S.; Tamura, E.; Nishimura, T. Identification of plastic-zone based on double frequency lock-in thermographic temperature measurement. In Proceedings of the International Conference of Fracture (ICF11 2015), Catania, Italy, 20–25 March 2015. [Google Scholar]
- Krapez, J.K.; Pacou, D.; Gardette, G. Lock-In Thermography and Fatigue Limit of Metals. In Proceedings of the Quantitative Infrared Thermography (QIRT), Reims, France, 18–21 July 2000. [Google Scholar]
- Enke, N. An enhanced theory for Thermographic stress analysis of isotropic materials. In Proceedings of Stress and Vibration: Recent Developments in Industrial Measurement and Analysis, Proceedings of the Sira/Stress and Vibration: Recent Developments in Measurement and Analysis, London, UK, 31 July 1989. [Google Scholar]
- Wang, W.J.; Dulieu-Barton, J.M.; Li, Q. Assessment of Non-Adiabatic Behaviour in Thermoelastic Stress Analysis of Small Scale Components. Exp. Mech. 2010, 50, 449–461. [Google Scholar] [CrossRef]
- Belnoue, J.P.H.; Mesogitis, T. Understanding the buckling behaviour of steered tows in Automated Dry Fibre Placement (ADFP) placement pre-preg laminates. Compos. Part A 2017, 102, 196–206. [Google Scholar] [CrossRef]
- Belhaj, M.; Deleglise, M. Dry fiber automated placement of carbon fibrous preforms. Compos. Part. B 2013, 50, 107–111. [Google Scholar] [CrossRef]
- IRTA- Manual. Diagnostic Engineering Solutions (DES srl); Des srl: Bari, Italy, 2015. [Google Scholar]
- De Finis, R.; Palumbo, D.; Galietti, U. Mechanical behaviour of stainless steels under dynamic loading: An investigation with thermal methods. J. Imaging 2016, 2, 32. [Google Scholar] [CrossRef]
- Ospina Cadavid, M.; Al-Khudairi, O.; Hadavini, H.; Goodwin, D.; Liaghat, G.H. Experimental Studies of Stiffness Degradation and Dissipated Energy in Glass Fibre Reinforced Polymer Composite under Fatigue Loading. Polym. Polym. Compos. 2017, 25, 435–446. [Google Scholar]



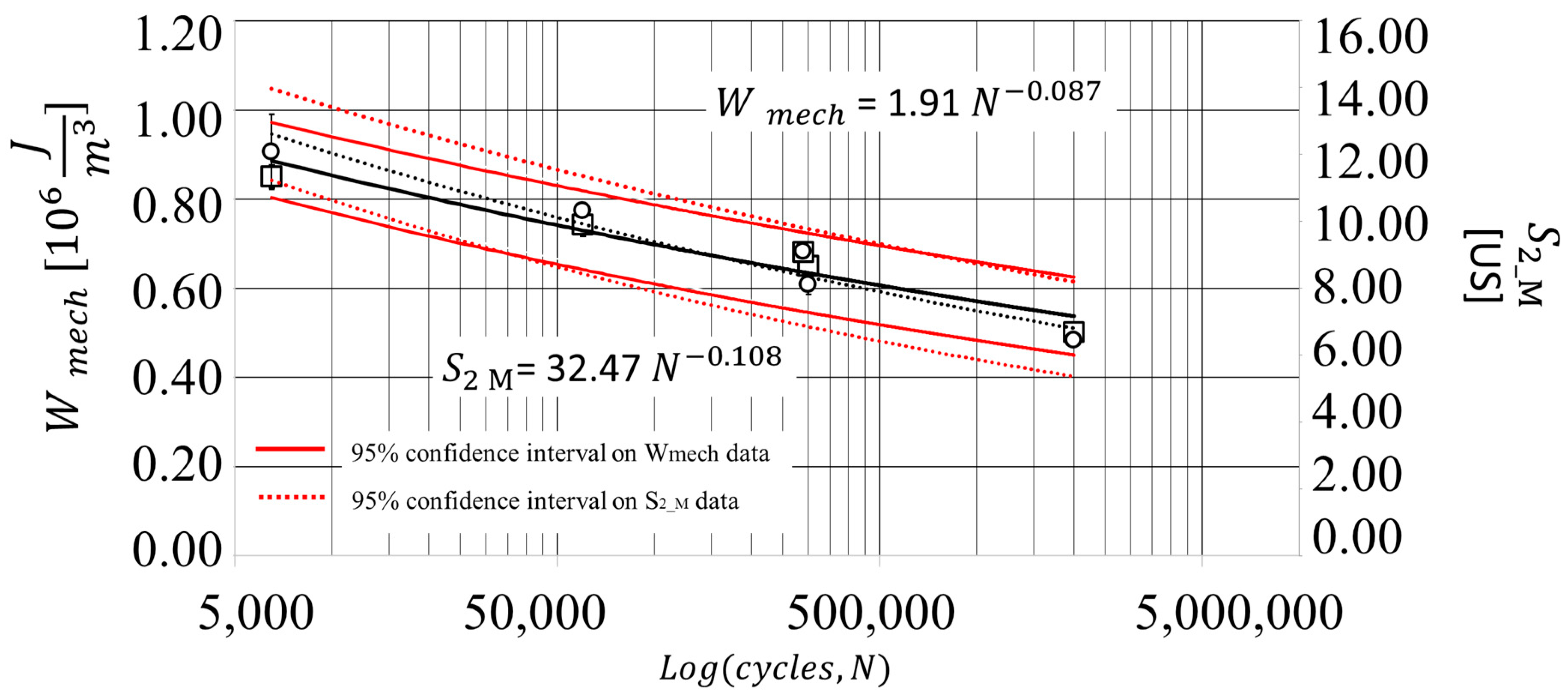
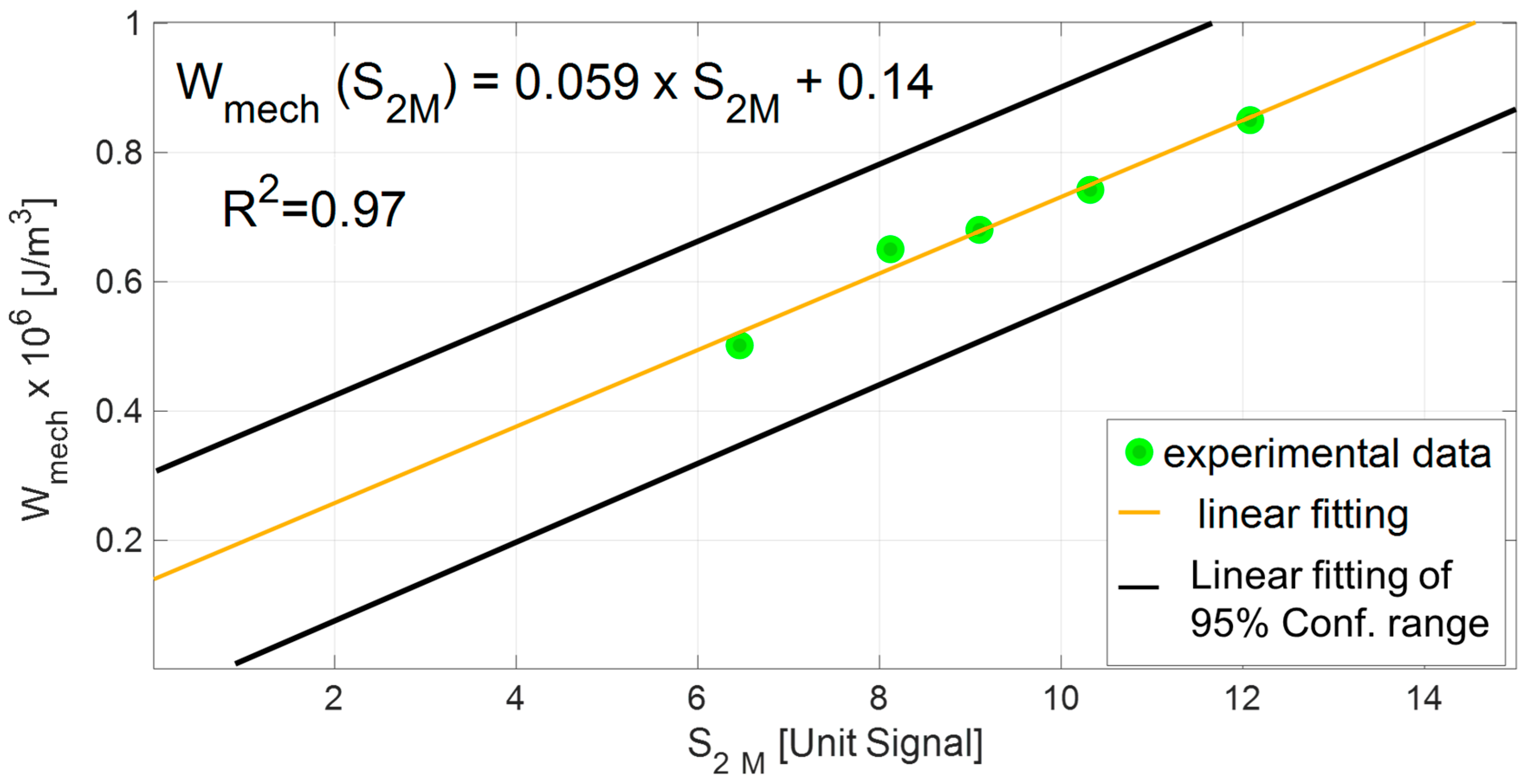

| Property | Mean Value * [MPa] | Standard Deviation * [MPa] |
|---|---|---|
| Young’s modulus | 55,800 | 1600 |
| Ultimate Tensile Strength | 825 | 85 |
| Cycles | Maximum Stress [MPa] | %UTS |
|---|---|---|
| 1765 | 660.75 | 80 |
| 2875 | 660.75 | 80 |
| 6500 | 619.25 | 75 * |
| 12,323 | 619.49 | 75 |
| 60,041 | 578.16 | 70 * |
| 75,461 | 578.16 | 70 |
| 291,610 | 536.86 | 65 * |
| 300,334 | 495.56 | 60 * |
| 2,000,000 | 412.97 | 50 * |
| 2,000,000 | 412.97 | 50 |
| Stress [MPa] | Wmech [106 × J/m3] | S2_M [Unit Signal] |
|---|---|---|
| 412.50 | 0.50 | 6.46 |
| 495.75 | 0.65 | 8.12 |
| 577.50 | 0.74 | 10.32 |
| 536.25 | 0.68 | 9.10 |
| 618.25 | 0.85 | 12.08 |
| Cycles of the S/N Curve | ||
|---|---|---|
| 2,000,000.00 | 447.97 | 481.17 |
| 300,334.00 | 504.92 | 526.28 |
| 60,000.00 | 596.91 | 580.58 |
| 290,000.00 | 547.14 | 551.13 |
| 6500.00 | 611.56 | 620.61 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
De Finis, R.; Palumbo, D. Estimation of the Dissipative Heat Sources Related to the Total Energy Input of a CFRP Composite by Using the Second Amplitude Harmonic of the Thermal Signal. Materials 2020, 13, 2820. https://doi.org/10.3390/ma13122820
De Finis R, Palumbo D. Estimation of the Dissipative Heat Sources Related to the Total Energy Input of a CFRP Composite by Using the Second Amplitude Harmonic of the Thermal Signal. Materials. 2020; 13(12):2820. https://doi.org/10.3390/ma13122820
Chicago/Turabian StyleDe Finis, Rosa, and Davide Palumbo. 2020. "Estimation of the Dissipative Heat Sources Related to the Total Energy Input of a CFRP Composite by Using the Second Amplitude Harmonic of the Thermal Signal" Materials 13, no. 12: 2820. https://doi.org/10.3390/ma13122820
APA StyleDe Finis, R., & Palumbo, D. (2020). Estimation of the Dissipative Heat Sources Related to the Total Energy Input of a CFRP Composite by Using the Second Amplitude Harmonic of the Thermal Signal. Materials, 13(12), 2820. https://doi.org/10.3390/ma13122820






