A Simplified Sablik’s Approach to Model the Effect of Compaction Pressure on the Shape of Hysteresis Loops in Soft Magnetic Composite Cores
Abstract
:1. Introduction
2. The JAS Model Equations
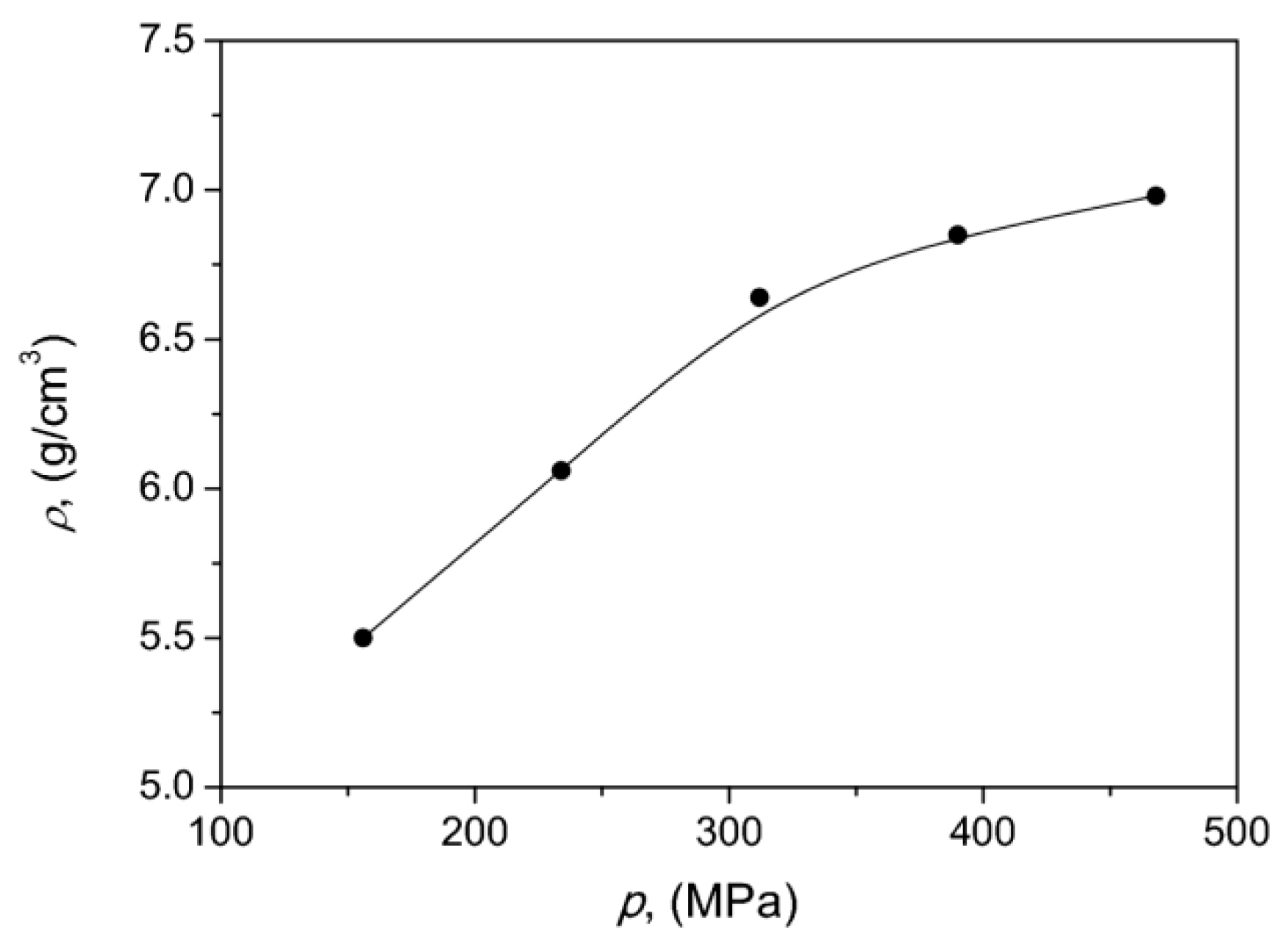
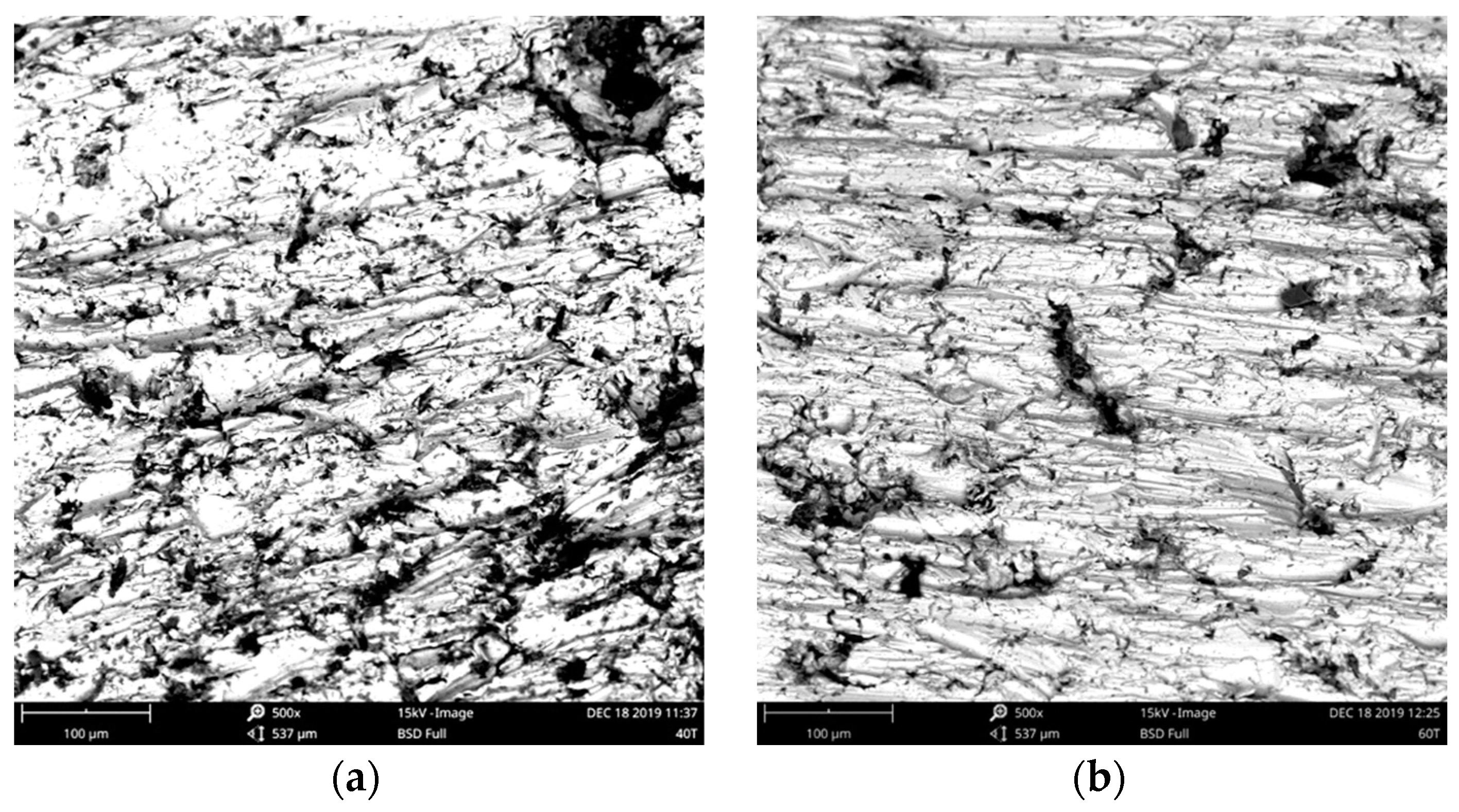
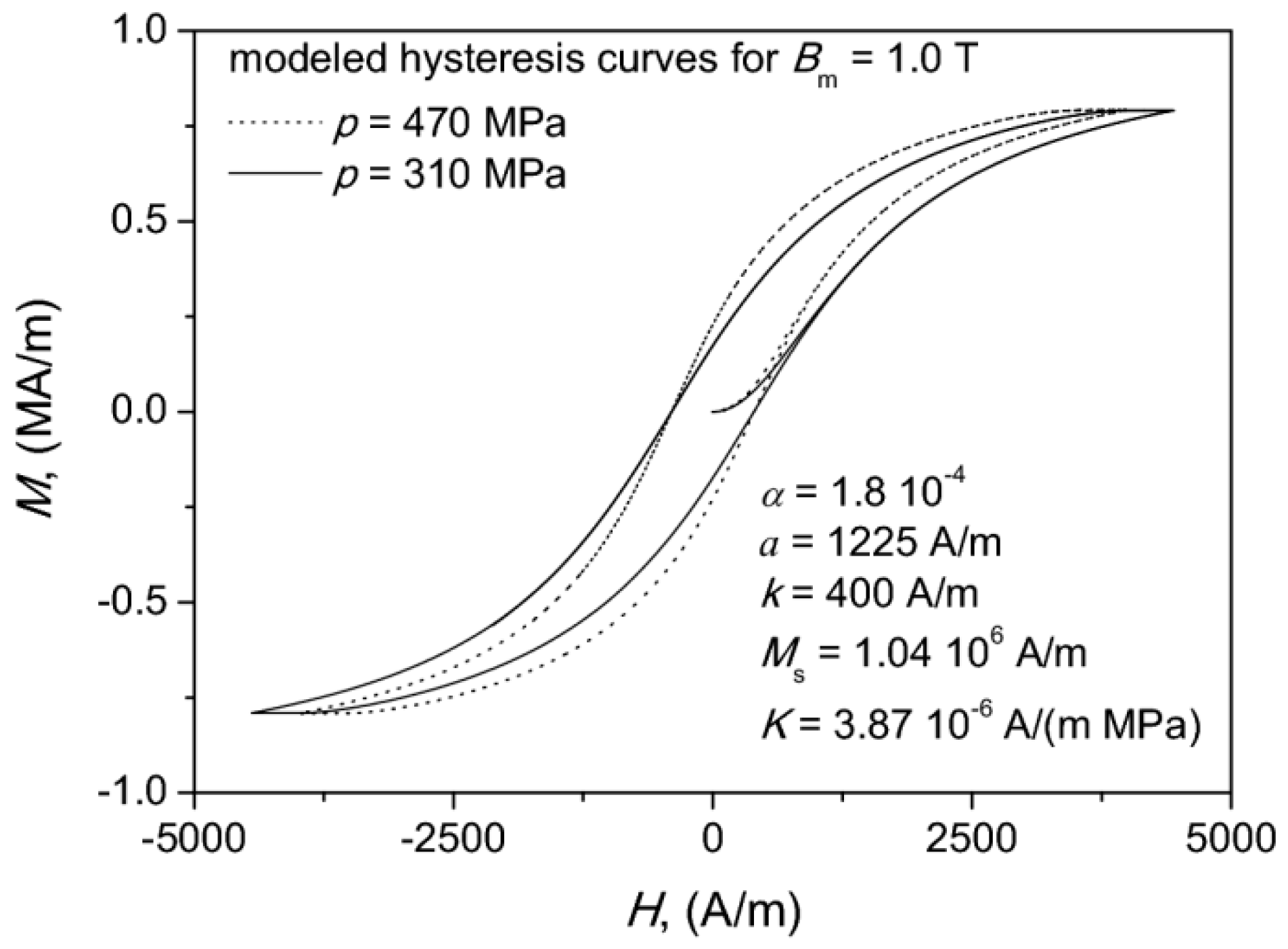
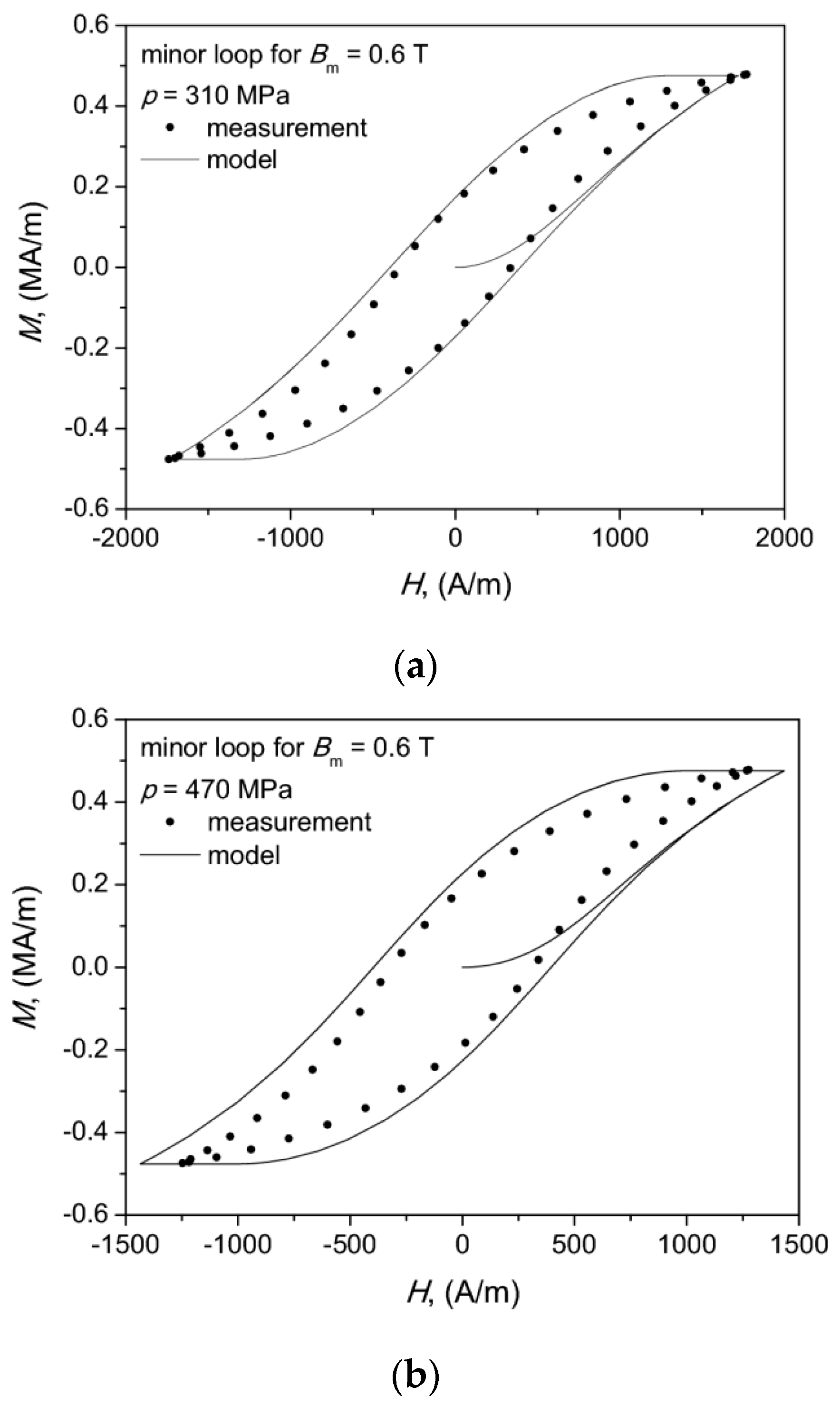
3. Measurements, Modeling
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Fuller Brown, W., Jr. Theory of magnetoelastic effects in ferromagnetism. J. Appl. Phys. 1965, 36, 994–1000. [Google Scholar] [CrossRef]
- du Trémolet de Lacheisserie, E. Magnetostriction—Theory and Applications of Magnetoelasticity; CRC Press: Boca Raton, FL, USA, 1993. [Google Scholar]
- Boll, R.; Overshott, K. Magnetic sensors. In Sensors, a Comprehensive Survey; Göpel, W.J., Zemel, N., Eds.; Wiley-VCH: Weinheim, Germany, 1989. [Google Scholar]
- Bieńkowski, A.; Szewczyk, R. The possibility of utilizing the high permeability magnetic materials in construction of magnetoelastic stress and force sensors. Sens. Actuators A 2004, 113, 270–276. [Google Scholar] [CrossRef]
- Belahcen, A. Magnetoelastic coupling in rotating electrical machines. IEEE Trans. Magn. 2005, 41, 1624–1627. [Google Scholar] [CrossRef]
- Joule, J.P. On a new class of magnetic forces. Ann. Electr. Magn. Chem. 1842, 8, 219–224. [Google Scholar]
- Villari, E. Ueber die Aenderungen des magnetischen Moments, welche der Zug und das Hindurchleiten eines galvanischen Stroms in einem Stabe von Stahl oder Eisen hervorbringt. Ann. Phys. Chem. 1865, 126, 87–122. [Google Scholar] [CrossRef] [Green Version]
- Hirsinger, L.; Billardon, R. Magneto-elastic finite element analysis including magnetic forces and magnetostriction effects. IEEE Trans. Magn. 1995, 31, 1877–1880. [Google Scholar] [CrossRef]
- Buiron, N.; Hirsinger, L.; Billardon, N. A multi-scale model for magneto-elastic couplings. Le Journal de Physique IV 1999, 9, Pr9-187–Pr9-196. [Google Scholar]
- Schneider, C.S. Effect of stress on the shape of ferromagnetic hysteresis loops. J. Appl. Phys. 2005, 97, 10E503. [Google Scholar] [CrossRef]
- Daniel, L.; Rekik, M.; Hubert, O. A multiscale model for magneto-elastic behaviour including hysteresis effects. Arch. Appl. Mech. 2014, 84, 1307–1323. [Google Scholar] [CrossRef] [Green Version]
- Sai Ram, B.; Baghel, A.P.S.; Kulkarni, S.V.; Chwastek, K.; Daniel, L. A hybrid product-multi-scale (MS) model for magneto-elastic behavior of soft-magnetic materials. Physica B 2019, 571, 301–306. [Google Scholar] [CrossRef]
- Jiles, D.C.; Atherton, D.L. Ferromagnetic hysteresis. IEEE Trans. Magn. 1983, 19, 2183–2185. [Google Scholar] [CrossRef]
- Jiles, D.C.; Atherton, D.L. Theory of the magnetisation process in ferromagnets and its application to the magnetomechanical effect. J. Phys. D Appl. Phys. 1984, 17, 1265–1281. [Google Scholar] [CrossRef]
- Sablik, M.J.; Kwun, H.; Burkhardt, G.L.; Jiles, D.C. Model for tensile and compressive stress on ferromagnetic hysteresis. J. Appl. Phys. 1987, 61, 3799–3801. [Google Scholar] [CrossRef]
- Sablik, M.J.; Jiles, D.C. Coupled magnetoelastic theory of magnetic and magnetostrictive hysteresis. IEEE Trans. Magn. 1993, 29, 2113–2123. [Google Scholar] [CrossRef] [Green Version]
- Sablik, M.J. A model for asymmetry in magnetic property behavior under tensile and compressive stress in steel. IEEE Trans. Magn. 1997, 33, 3958–3960. [Google Scholar] [CrossRef]
- Schneider, C.S.; Winchell, S.D. Hysteresis in conducting ferromagnets. Physica B 2006, 372, 269–272. [Google Scholar] [CrossRef]
- Weiss, P. L’hypothèse du champ moléculaire et la propriété ferromagnétique. J. Phys. 1907, 6, 665–690. [Google Scholar] [CrossRef]
- Visintin, A. A Weiss-type model of ferromagnetism. Physica B 2000, 275, 87–91. [Google Scholar] [CrossRef]
- Albert, C.; Ferrari, L.; Fröhlich, J.; Schlein, B. Magnetism and the Weiss exchange field—A theoretical analysis inspired by recent experiments. J. Stat. Phys. B 2006, 125, 77–124. [Google Scholar] [CrossRef] [Green Version]
- Bertotti, G. Hysteresis in Magnetism; Academic Press: San Diego, CA, USA, 1998. [Google Scholar]
- Apicella, V.; Clemente, C.S.; Davino, D.; Leone, D.; Visone, C. Review of modeling and control of magnetostrictive actuators. Actuators 2019, 8, 45. [Google Scholar] [CrossRef] [Green Version]
- Li, J.; Xu, M. Modified Jiles-Atherton-Sablik model for asymmetry in magnetomechanical effect under tensile and compressive stress. J. Appl. Phys. 2011, 110, 063918. [Google Scholar] [CrossRef]
- Lo, C.C.; Kinser, E.; Jiles, D.C. Modeling the interrelating effects of plastic deformation and stress on magnetic properties of materials. J. Appl. Phys. 2003, 93, 6626–6628. [Google Scholar] [CrossRef] [Green Version]
- Sablik, M.J.; Rios, S.; Landgraf, F.J.G.; Yonamine, T.; de Campos, M.F. Modeling of sharp change in magnetic hysteresis behaviour of electrical steel at small plastic deformation. J. Appl. Phys. 2005, 97, 10E518. [Google Scholar] [CrossRef]
- Li, J.; Xu, M.; Leng, J.; Xiu, M. Modeling plastic deformation effect on magnetization in ferromagnetic materials. J. Appl. Phys. 2012, 111, 063909. [Google Scholar] [CrossRef]
- Leng, J.; Liu, Y.; Zhou, G.; Gao, Y. Metal magnetic memory signal response to plastic deformation of low carbon steel. NDT E Int. 2013, 55, 42–46. [Google Scholar] [CrossRef]
- Singh, D.; Martin, F.; Raasilo, P.; Belahcen, A. Magneto-mechanical model for hysteresis in electrical steel sheet. IEEE Trans. Magn. 2016, 52, 7301109. [Google Scholar] [CrossRef] [Green Version]
- Hergli, K.; Marouani, H.; Zidi, M. Numerical determination of Jiles-Atherton hysteresis parameters: Magnetic behavior under mechanical deformation. Physica B 2018, 549, 74–81. [Google Scholar] [CrossRef]
- Bieńkowski, A.; Szewczyk, R.; Kolano, R. Magnetoelastic Villari effect in nanocrystalline Fe73.5Nb3Cu1Si13.5B9 alloy. Phys. Stat. Solidi A 2002, 189, 821–824. [Google Scholar] [CrossRef]
- Szewczyk, R.; Salach, J.; Bieńkowski, A. Modeling of magnetoelastic materials for force and torque sensors. Solid State Phenom. 2009, 144, 124–129. [Google Scholar] [CrossRef]
- Szewczyk, R.; Bieńkowski, A. Magnetoelastic Villari effect in high permeability Mn-Zn ferrites and modeling of this effect. J. Magn. Magn. Mater. 2003, 254–255, 284–286. [Google Scholar] [CrossRef]
- Schneider, C.S.; Cannell, P.Y.; Watts, K.T. Magnetoelasticity for large stresses. IEEE Trans. Magn. 1992, 28, 2626–2631. [Google Scholar] [CrossRef]
- Suliga, M.; Borowik, L.; Chwastek, K.; Pawlik, P. A non-destructive method to determine residual stress in drawn wires based on magnetic measurements. Przegląd Elektrotechniczny 2014, 12, 161–164. [Google Scholar]
- Suliga, M.; Kruzel, R.; Chwastek, K.; Jakubas, A.; Pawlik, P. The effect of residual stresses on the coercive field strength of drawn wires. Acta Phys. Pol. A 2017, 131, 1114–1116. [Google Scholar] [CrossRef]
- Benabou, A.; Clénet, S.; Piriou, F. Comparison of Preisach and Jiles-Atherton models to take into account hysteresis phenomenon for finite element analysis. J. Magn. Magn. Mater. 2003, 261, 139–160. [Google Scholar] [CrossRef]
- Miljavec, D.; Zidarič, B. Introducing a domain flexing function in the Jiles–Atherton hysteresis model. J. Magn. Magn. Mater. 2008, 320, 763–768. [Google Scholar] [CrossRef]
- Ślusarek, B.; Chwastek, K.; Szczygłowski, J.; Jankowski, B. Modelling hysteresis loops of SMC cores. Solid State Phenom. 2015, 220, 652–660. [Google Scholar] [CrossRef]
- Chwastek, K. Modelling offset minor hysteresis loops with the modified Jiles–Atherton description. J. Phys. D Appl. Phys. 2009, 42, 165002. [Google Scholar] [CrossRef]
- Zirka, S.E.; Moroz, Y.I.; Harrison, R.G.; Chwastek, K. On physical aspects of the Jiles-Atherton hysteresis models. J. Appl. Phys. 2012, 112, 043916. [Google Scholar] [CrossRef]
- Toms, H.L.; Colclaser, R.G., Jr.; Krefta, M.P. Two-dimensional finite element magnetic modeling for scalar hysteresis effects. IEEE Trans. Magn. 2001, 37, 982–988. [Google Scholar] [CrossRef]
- Jastrzębski, R.; Chwastek, K. A comparison of macroscopic descriptions of magnetization curves. ITM Web Conf. 2017, 15, 03003. [Google Scholar] [CrossRef] [Green Version]
- Jiles, D.C.; Atherton, D.L. Theory of ferromagnetic hysteresis. J. Magn. Magn. Mater. 1986, 61, 48–60. [Google Scholar] [CrossRef]
- Chwastek, K. Problems in descriptions of hysteresis. Przegląd Elektrotechniczny 2010, 4, 24–27. [Google Scholar]
- Jakubas, A.; Jastrzębski, R.; Chwastek, K. Modeling the effect of compaction pressure on hysteresis curves of self-developed SMC cores. COMPEL 2019, 38, 1154–1163. [Google Scholar] [CrossRef]
- Stevens, K.J. Stress dependence of ferromagnetic hysteresis loops for two grades of steel. NDT E Int. 2000, 33, 111–121. [Google Scholar] [CrossRef]
- Szewczyk, R.; Bieńkowski, A. Application of the energy-based model for the magnetoelastic properties of amorphous alloys. J. Magn. Magn. Mater. 2004, 272–276, 728–730. [Google Scholar] [CrossRef]
- Bhadeshia, H.K. Mathematical models in materials science. Mater. Sci. Technol. 2008, 24, 128–136. [Google Scholar] [CrossRef]
- Sadowski, N.; Batistela, N.J.; Bastos, J.P.A.; Lajoie-Mazenc, M. An inverse Jiles-Atherton model to take into account hysteresis in time-stepping finite-element computations. IEEE Trans. Magn. 2002, 38, 797–800. [Google Scholar] [CrossRef]
- Chwastek, K. Modelling of dynamic hysteresis loops using the Jiles-Atherton approach. Math. Comp. Model. Dyn. Syst. 2009, 15, 95–105. [Google Scholar] [CrossRef]
- Anhalt, M.; Weidenfeller, B.; Mattei, J.-L. Inner demagnetization factor in polymer-bonded soft magnetic composites. J. Magn. Magn. Mater. 2008, 320, e844–e848. [Google Scholar] [CrossRef] [Green Version]
- Jakubas, A.; Gębara, P.; Seme, S.; Gnatowski, A.; Chwastek, K. Magnetic properties of SMC cores produced at a low compating temperature. Acta Phys. Pol. A 2017, 131, 1289–1293. [Google Scholar] [CrossRef]
- Tumański, S. Handbook of Magnetic Measurements; CRC Press: Boca Raton, FL, USA, 2011. [Google Scholar]
- Ślusarek, B.; Szczygłowski, J.; Chwastek, K.; Jankowski, B. A correlation of magnetic properties with material density for soft magnetic composite cores. COMPEL 2015, 34, 637–646. [Google Scholar] [CrossRef]
- Shokrollahi, H.; Janghorban, K. The effect of compaction parameters and particle size on magnetic properties of iron-based alloys used in soft magnetic composites. Mater. Sci. Eng. B 2006, 134, 41–43. [Google Scholar] [CrossRef]
- Chwastek, K.; Szczygłowski, J. An alternative method to estimate the parameters of Jiles-Atherton model. J. Magn. Magn. Mater. 2007, 314, 47–51. [Google Scholar] [CrossRef]
- Lederer, D.; Igarashi, H.; Kost, A.; Honma, T. On the parameter identification and application of the Jiles–Atherton hysteresis model for numerical modeling of measured characteristics. IEEE Trans. Magn. 1999, 35, 1211–1214. [Google Scholar] [CrossRef]
- Włodarski, Z. The Jiles-Atherton model with variable pinning parameter. IEEE Trans. Magn. 2003, 39, 1990–1992. [Google Scholar] [CrossRef]
- Gmyrek, Z. Numerical modeling of static hysteresis loop using variable parameters. Int. J. Numer. Model. 2014, 27, 199–212. [Google Scholar] [CrossRef]




© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jakubas, A.; Chwastek, K. A Simplified Sablik’s Approach to Model the Effect of Compaction Pressure on the Shape of Hysteresis Loops in Soft Magnetic Composite Cores. Materials 2020, 13, 170. https://doi.org/10.3390/ma13010170
Jakubas A, Chwastek K. A Simplified Sablik’s Approach to Model the Effect of Compaction Pressure on the Shape of Hysteresis Loops in Soft Magnetic Composite Cores. Materials. 2020; 13(1):170. https://doi.org/10.3390/ma13010170
Chicago/Turabian StyleJakubas, Adam, and Krzysztof Chwastek. 2020. "A Simplified Sablik’s Approach to Model the Effect of Compaction Pressure on the Shape of Hysteresis Loops in Soft Magnetic Composite Cores" Materials 13, no. 1: 170. https://doi.org/10.3390/ma13010170
APA StyleJakubas, A., & Chwastek, K. (2020). A Simplified Sablik’s Approach to Model the Effect of Compaction Pressure on the Shape of Hysteresis Loops in Soft Magnetic Composite Cores. Materials, 13(1), 170. https://doi.org/10.3390/ma13010170





