Design of Reinforcement in Nano- and Microcomposites
Abstract
1. Introduction
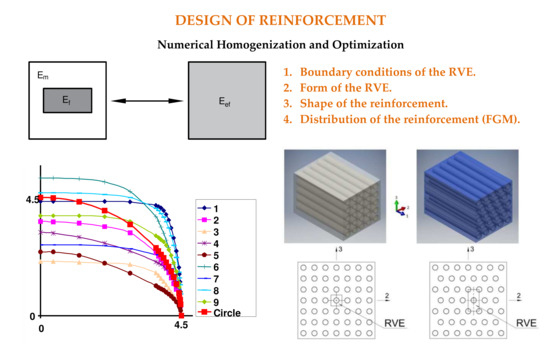
- Boundary conditions of the representative volume element,
- Form of the representative volume element,
- Shape of the reinforcement,
- Distribution of the reinforcement.
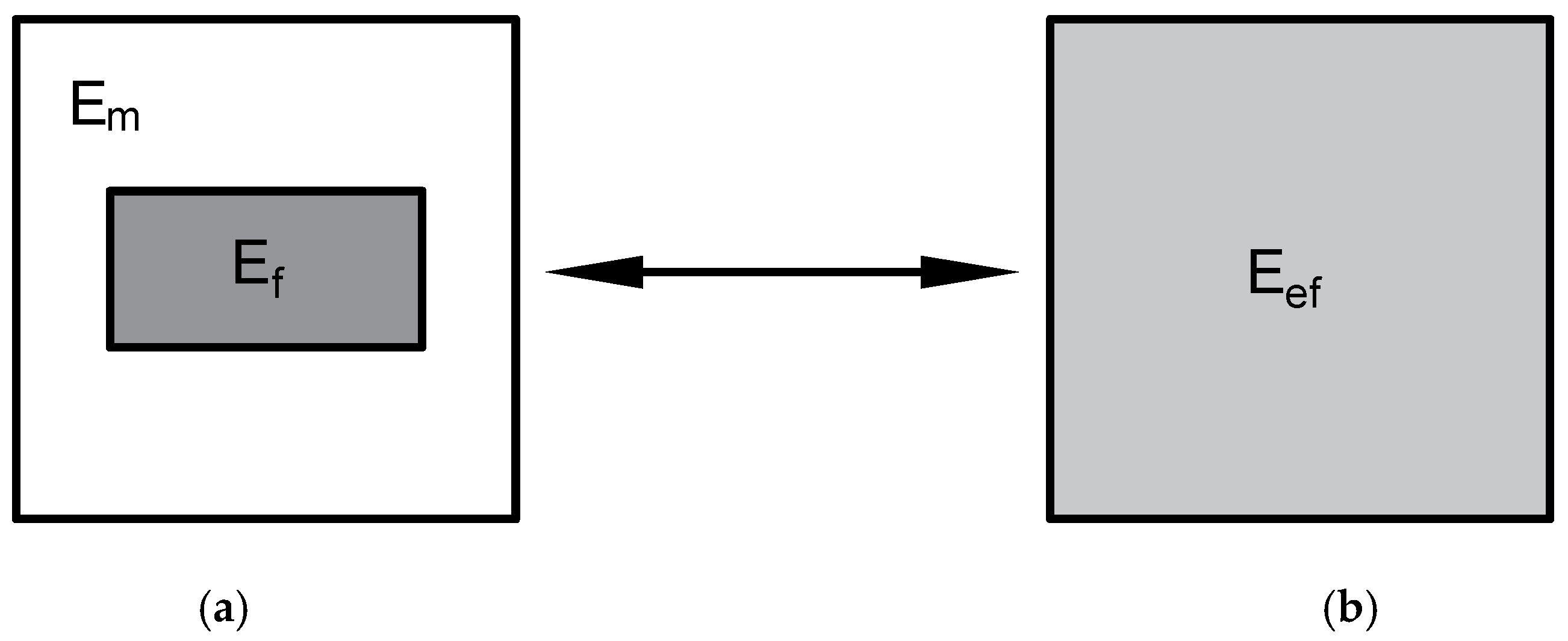
2. Preliminary Remarks
3. Boundary Conditions
- Linear displacement boundary condition (Dirichlet condition),
- Constant traction boundary condition (Neumann condition),
- Periodic boundary condition.
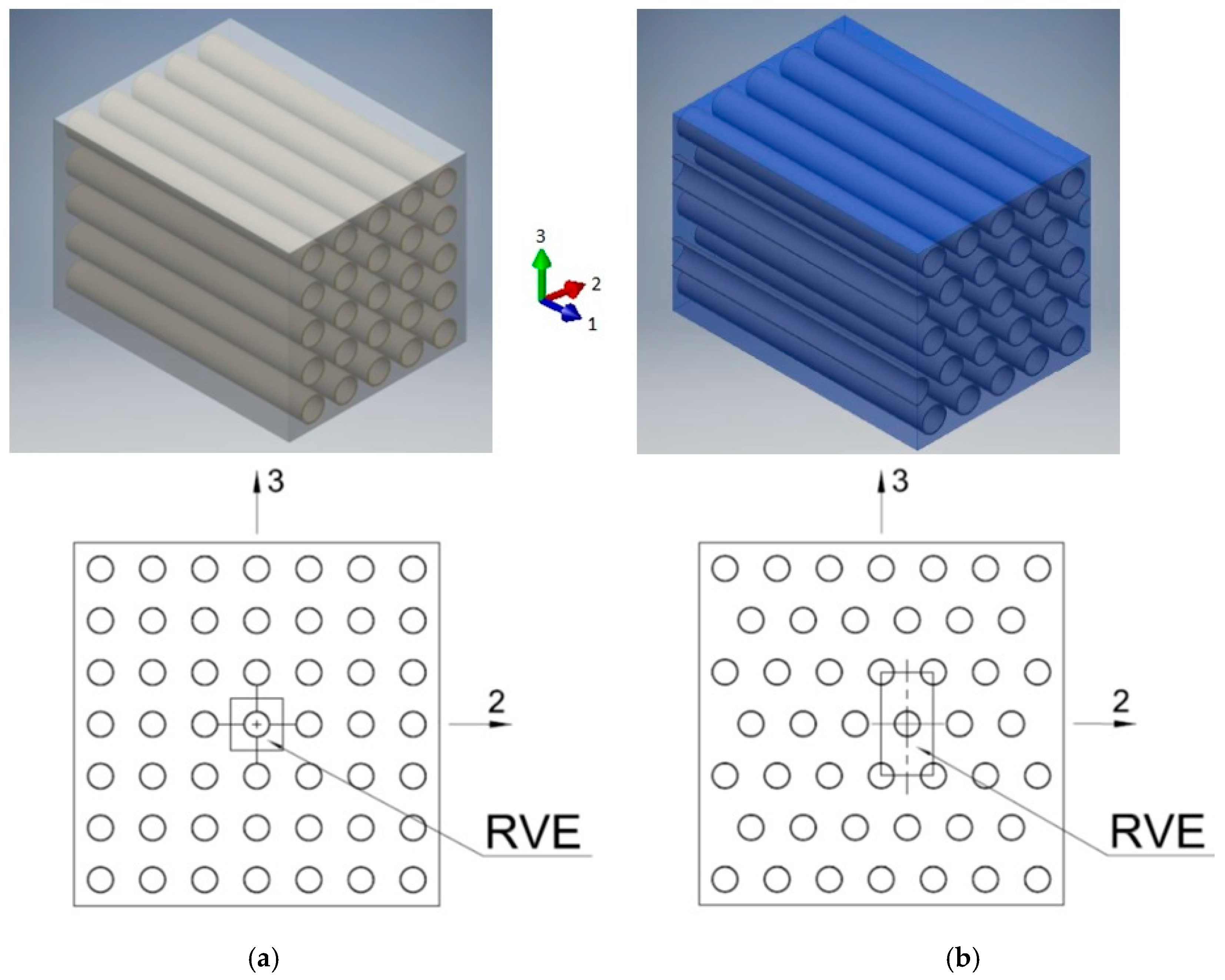
4. Form of the Representative Volume Element
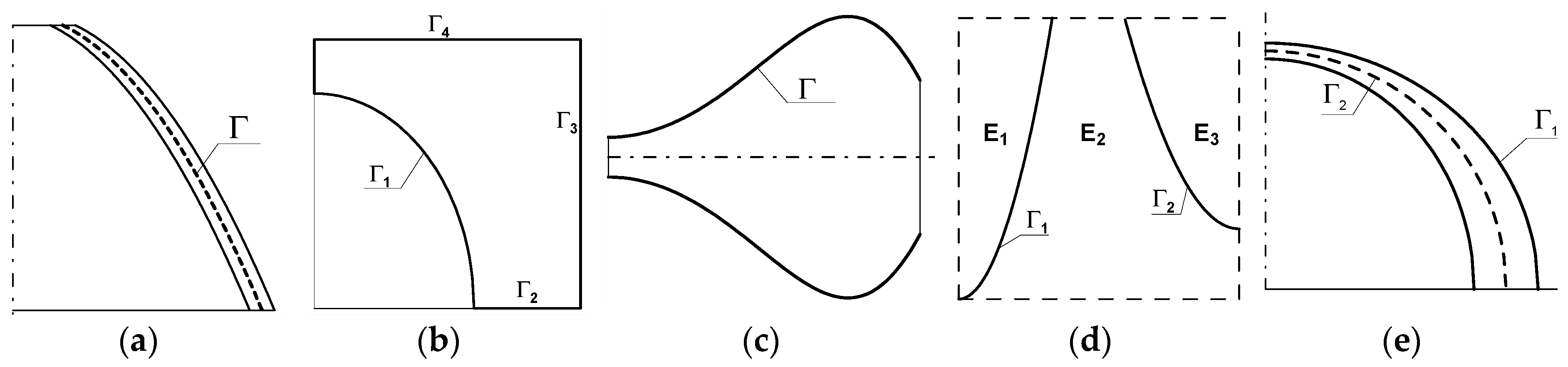
5. Optimization Problems with the Use of 2D Curves
- Set of nodes’ approximates curve Γ (see, e.g., Lee et al. [64]) created by discretization of finite elements, then the number of design variables is large and equal to the number of nodes on the edge Γ, and additionally increases in cases of description concentration of stresses; this method is used very rarely.
- Curve Γ is described by elementary analytic functions, in which case it is unambiguously defined by giving a finite fixed number (see Pedersen [65,66]), much smaller than in the previous case; however, there is always a problem with the introduction of the function and assessment, whether it is sufficient to solve a specific optimization problem; this method has unfortunately too little generality.
- Application of spline functions to the definition of curve Γ, where the spline functions are uniquely determined by specifying the coordinates of a finite number of key points Pi (i = 0,1,2, ..., I); the number of basis points is equivalent to the number of design variables.
5.1. Representation of the Curve Γ through Elementary Functions
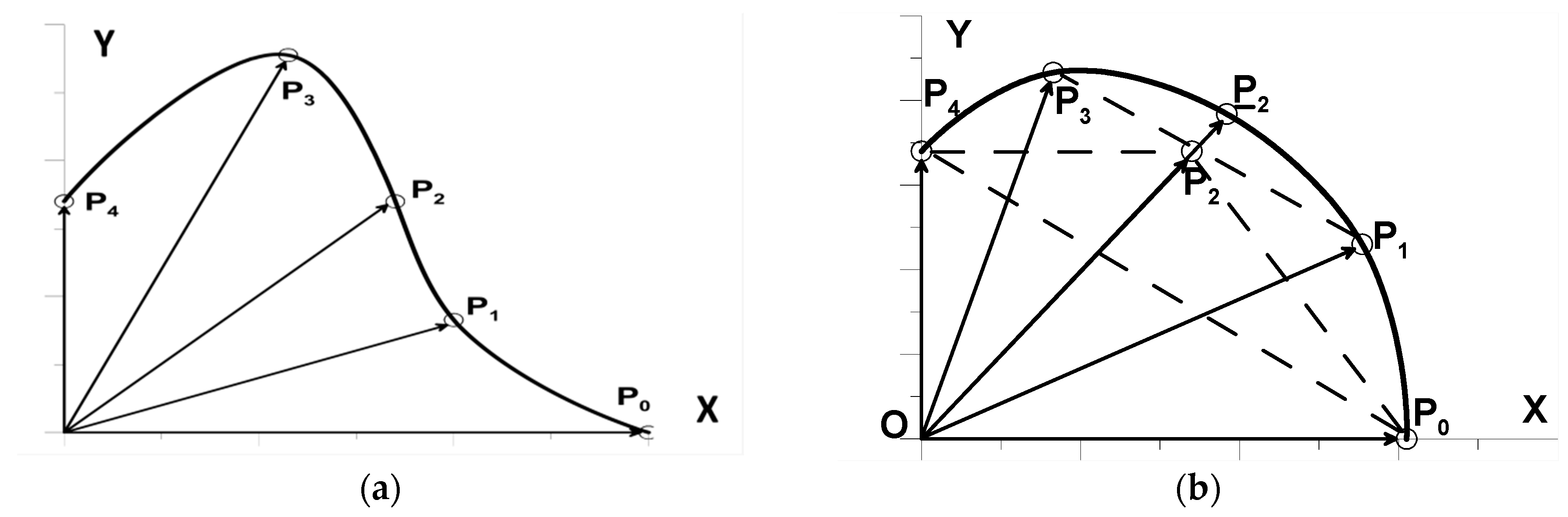
5.2. Generation of Key Point Population
5.2.1. No Constraints—Equidistant Key Points
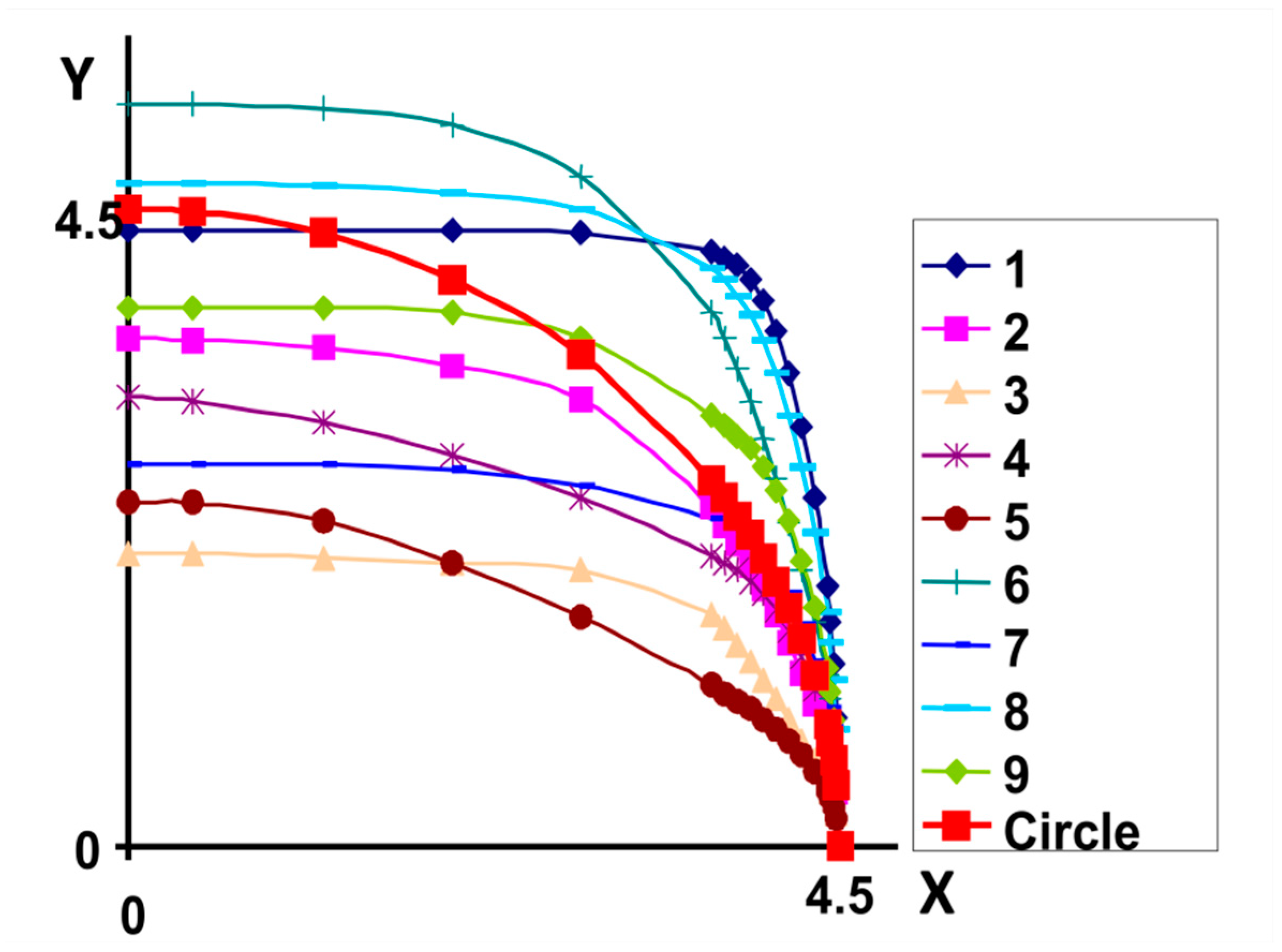
5.2.2. Generation of Convex Curves
5.2.3. Generation of a convex Curve with Constraints in the Form of a Constant Area Bounded by a Curve Γ
- (a)
- one of the angles on the edge of the curve is 0° and the other 90°,
- (b)
- one of the angles on the edge of the curve is not equal to 0° or 90°.
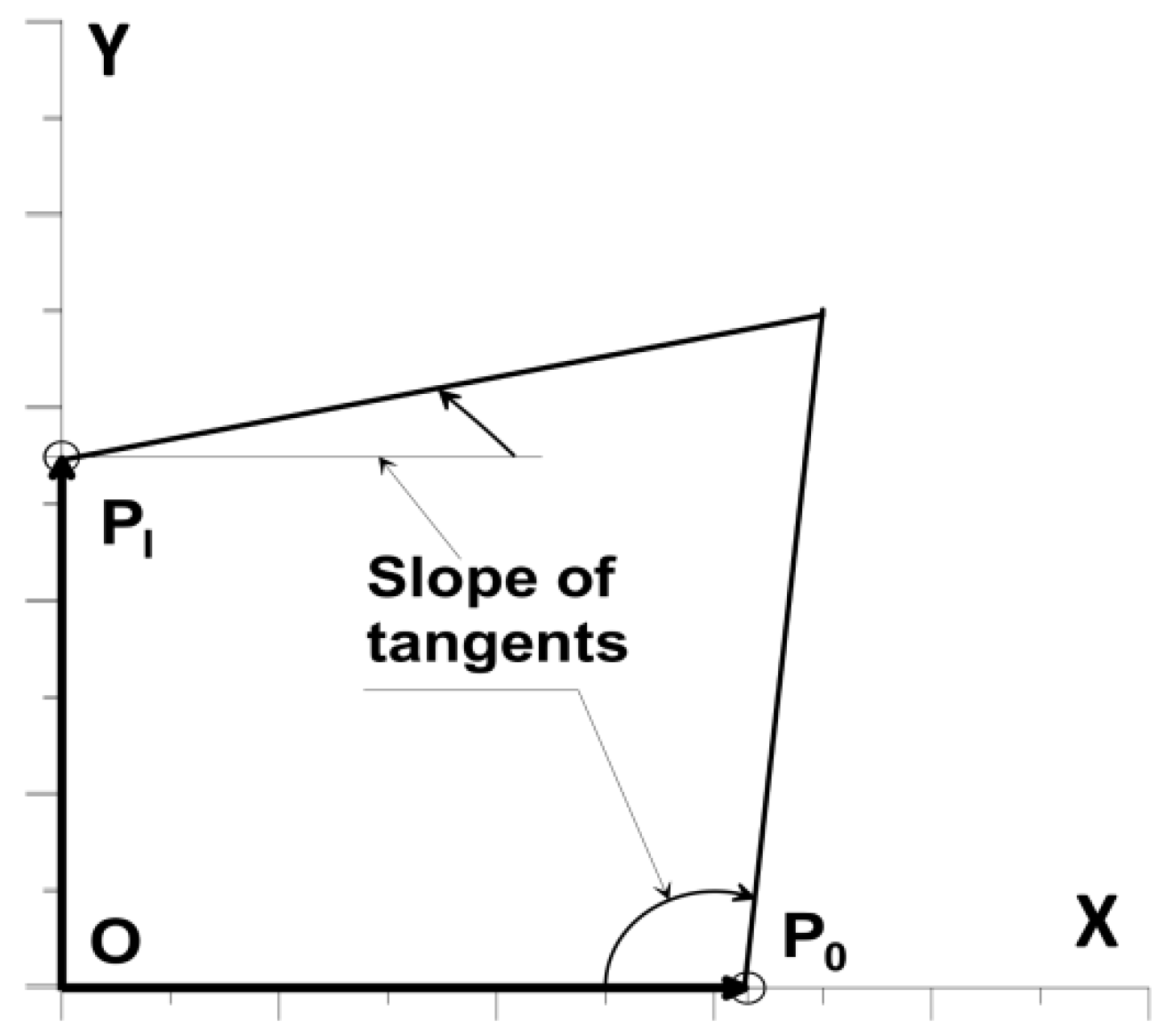
- Convexity of a polygon stretched on basis vectors,
- Boundary conditions at the ends of the curve.
6. Shape of the Reinforcement
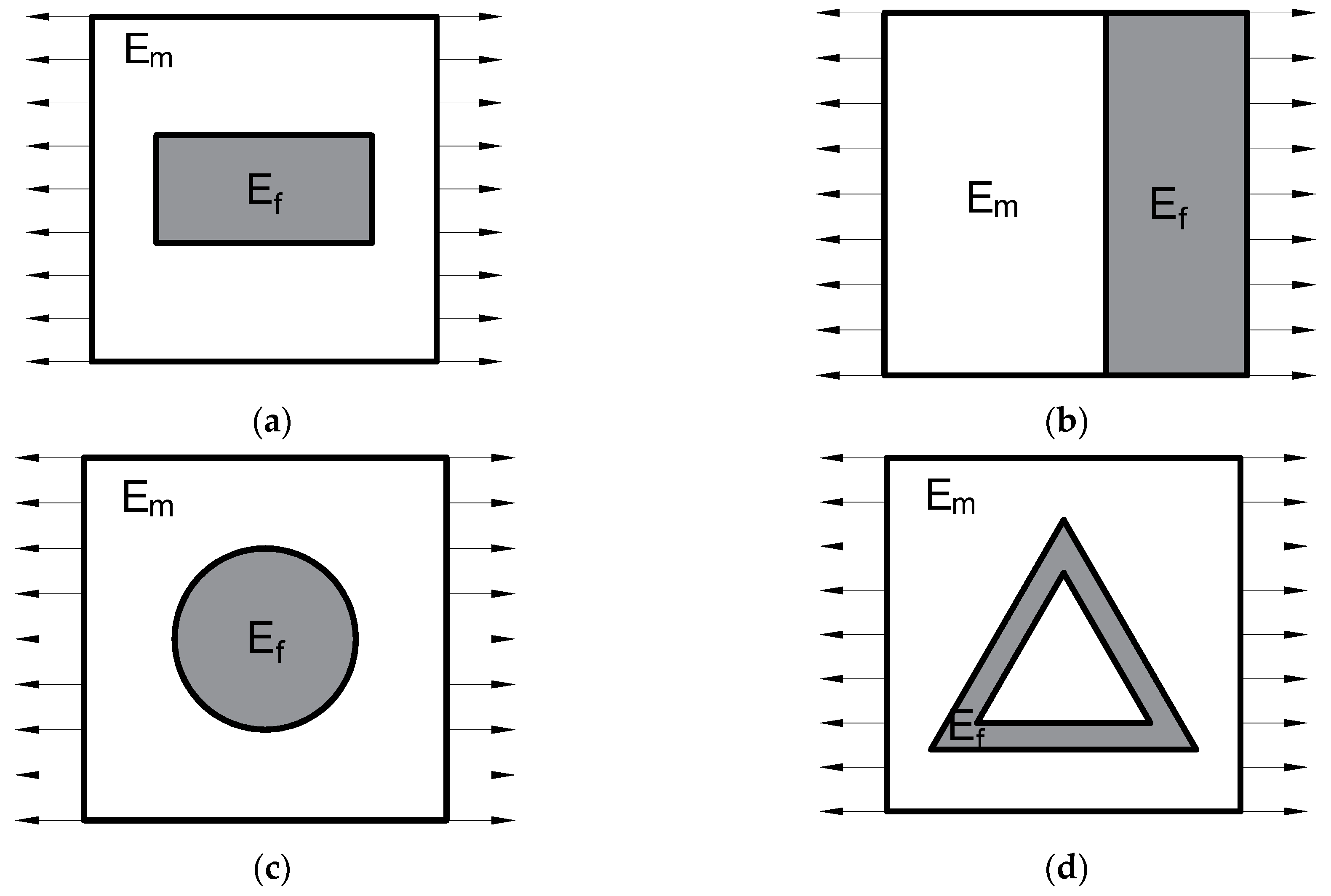
6.1. 2D Homogenization Problem
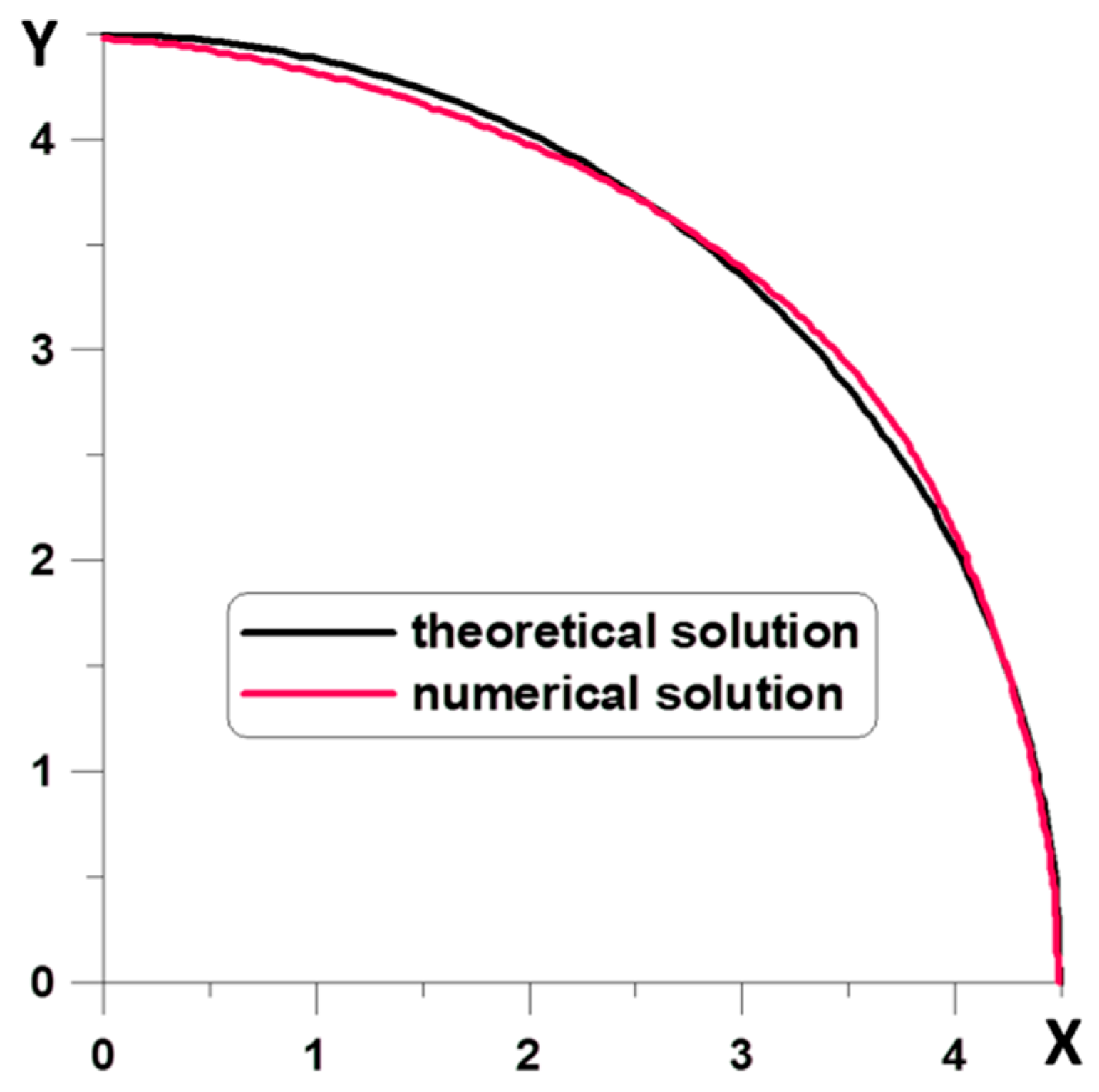
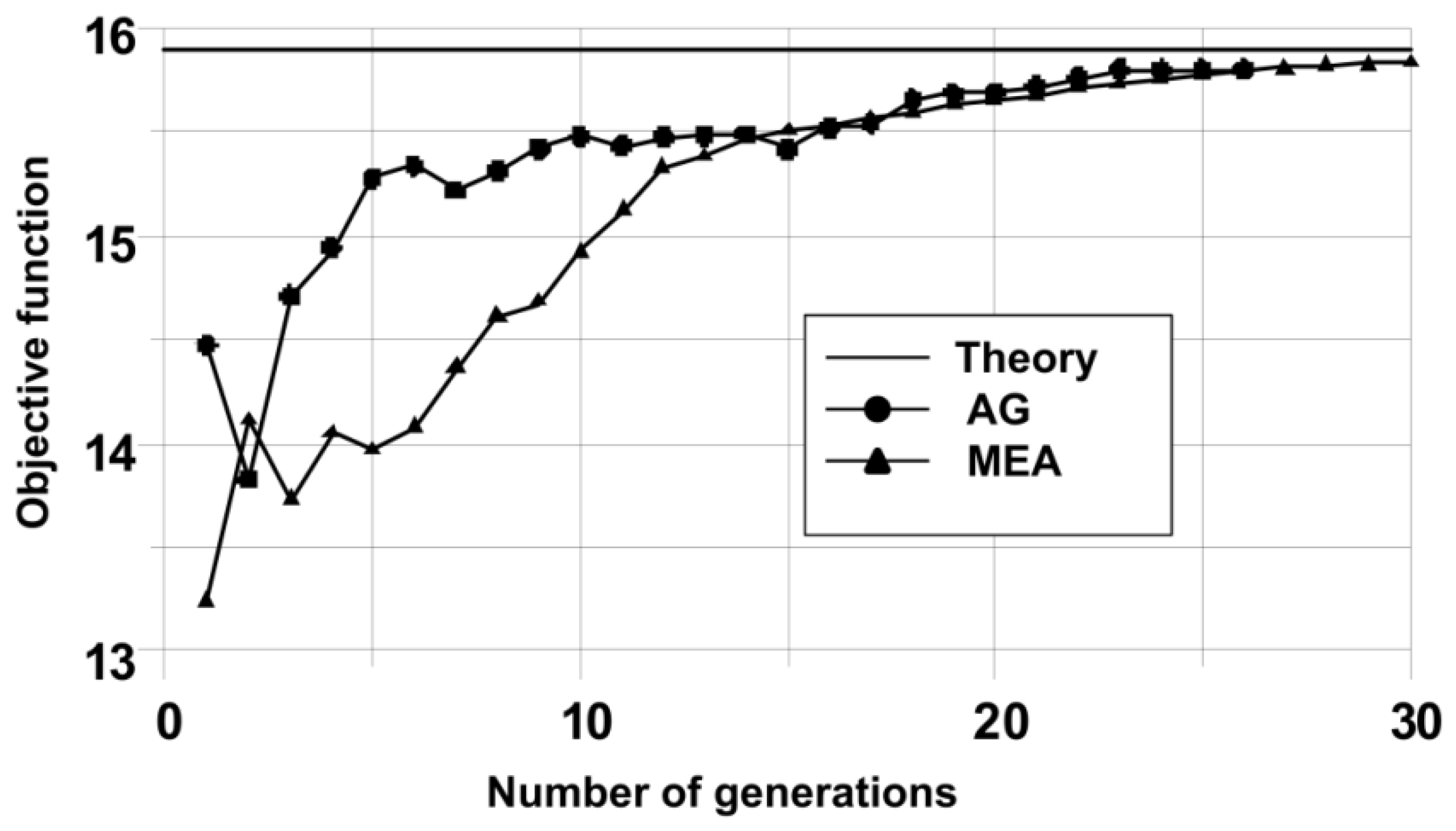
6.2. Isoperimetric Problem—Verification of the Accuracy of Numerical Solutions
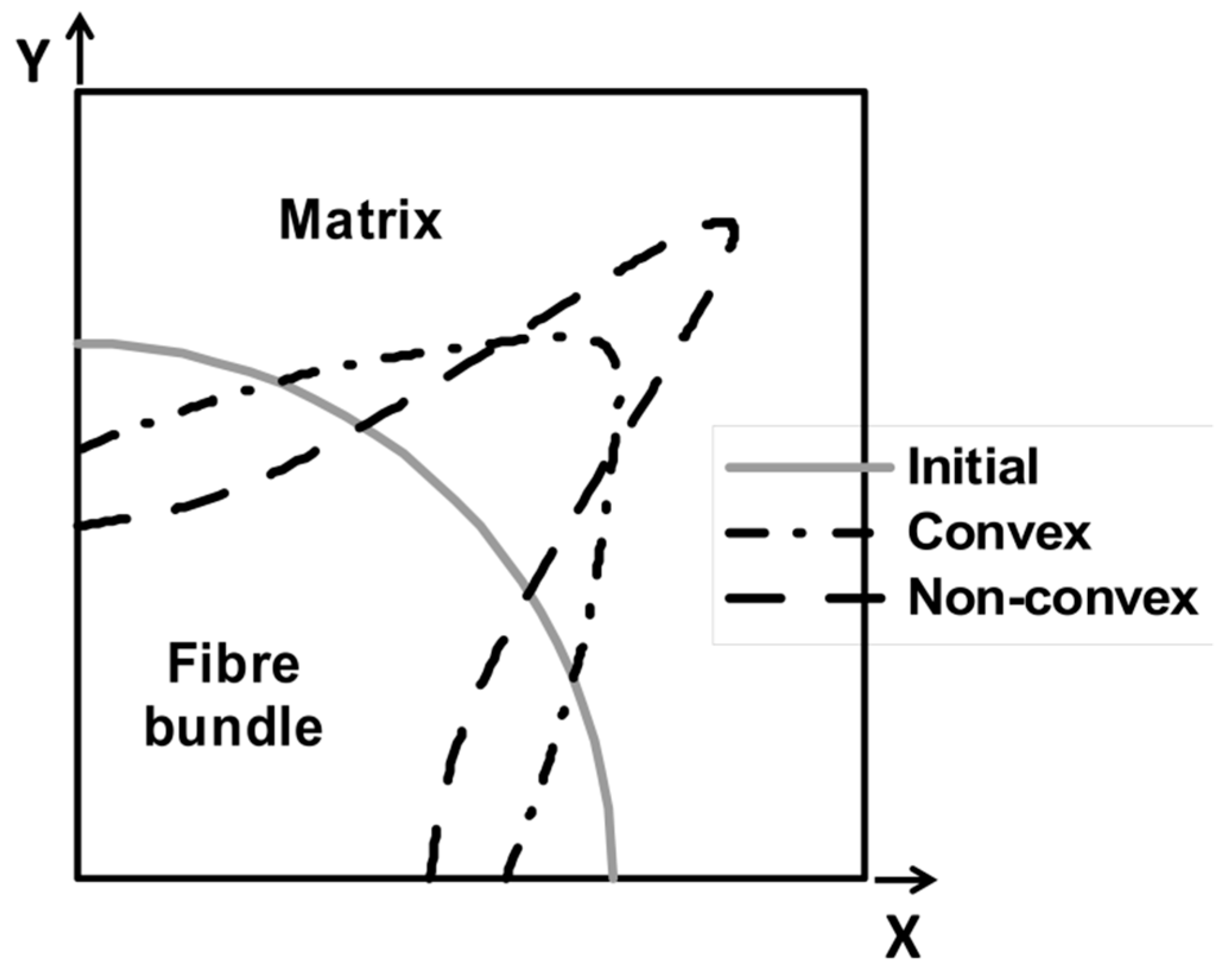
6.3. Shape of Fibre Bundles
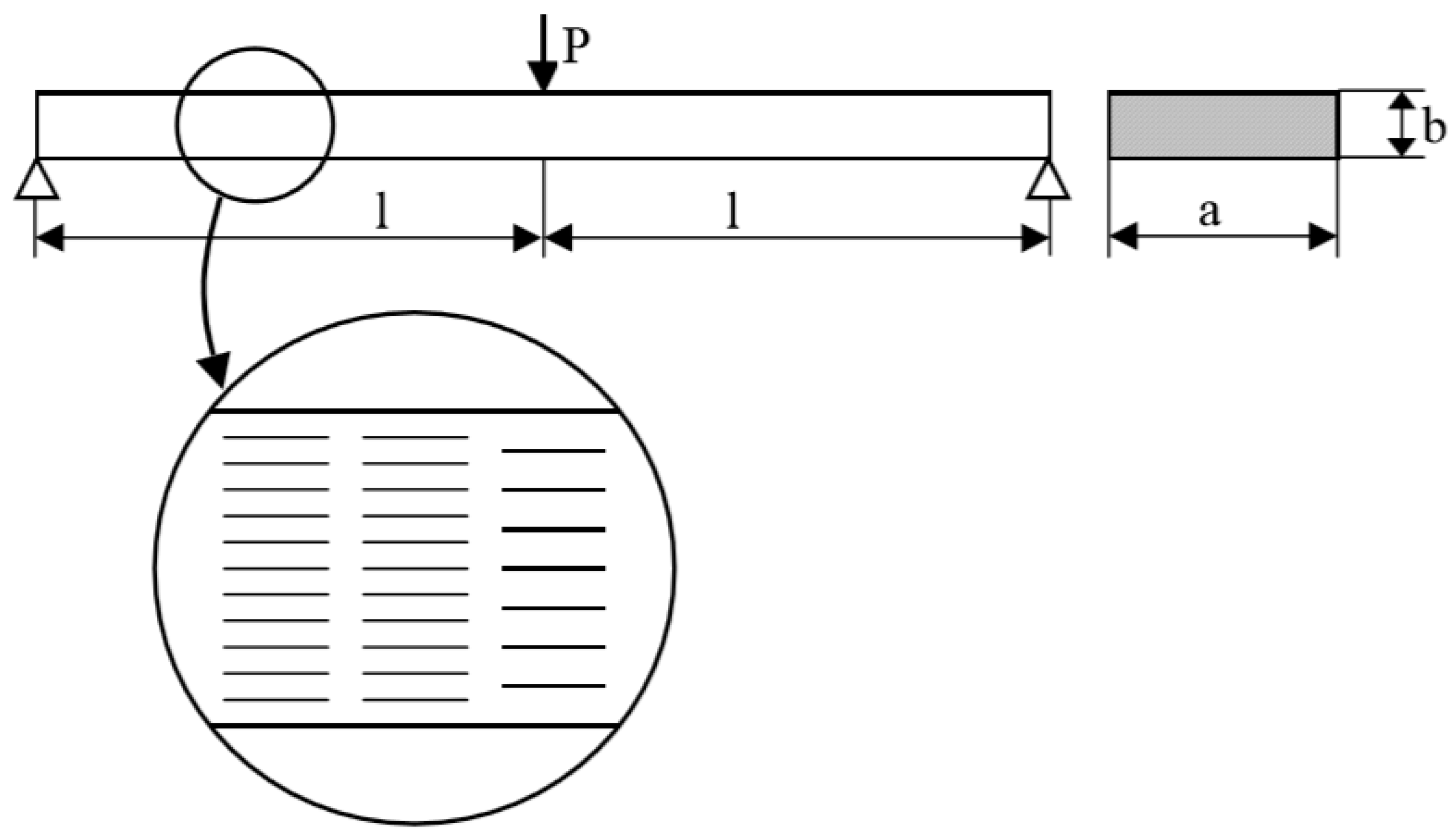
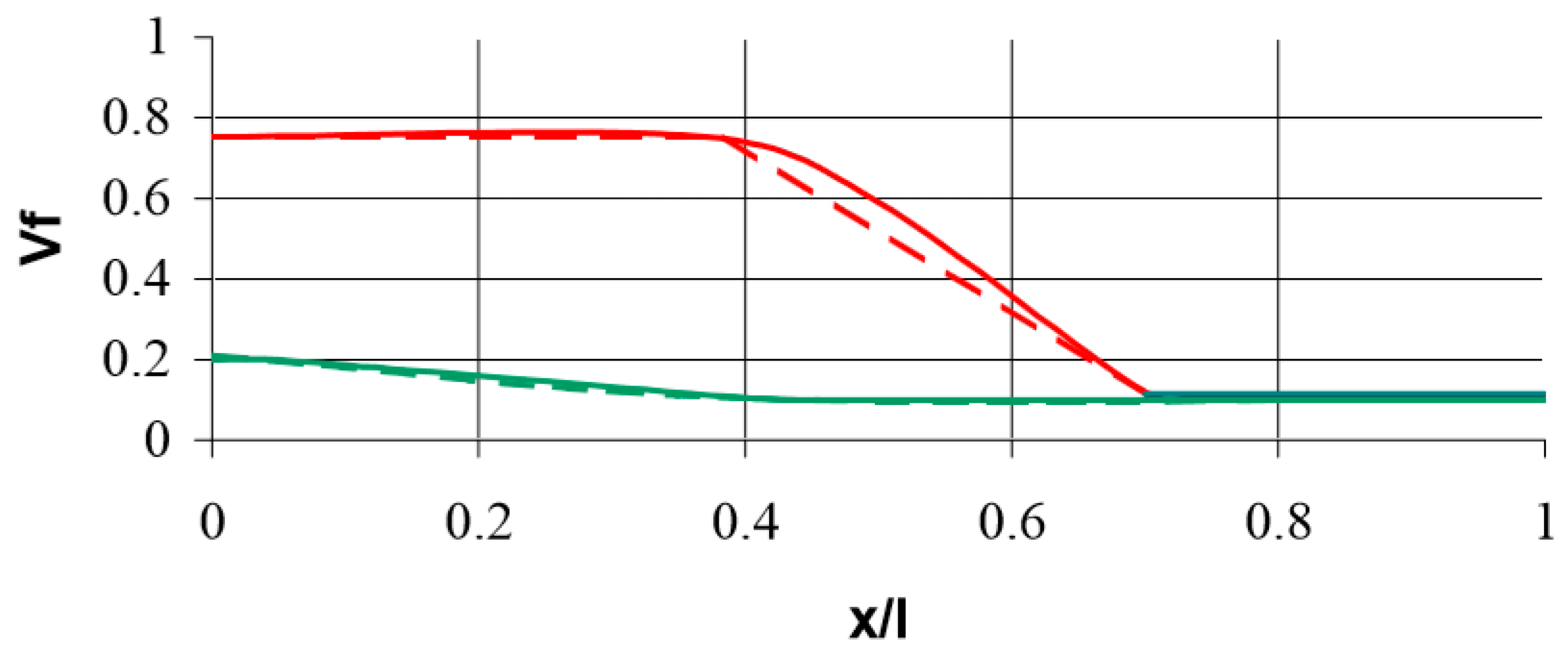
7. Distribution of the Reinforcement
8. Conclusions
- The method of description (representation) of the 2D curve related to the number of design variables included in the optimization process was presented.
- There was a good agreement of theoretical and numerical solutions, both in the case of using genetic algorithms (GA) as well as a modified evolutionary strategy (MEA) for the shape optimization of the single fibre.
- In shape optimization of fibre bundles, a circle was assumed as the original shape. In the numerical computations, the algorithm of modified evolutionary strategy (MEA) was used. The shape of the fibre bundle depends on the conditions limiting the form of the curve Γ understood in the sense of its convexity or not.
- The composite beam reinforced by short fibres, loaded at the centre and having a variable density of fibres along the length, was considered, being an example of FGM. The optimization problem deals with topology optimization since we were looking for the optimal material distribution. The results showed a good correlation between numerical and analytical studies.
- Some examples of numerical homogenization of various 2D and 3D RVE have been studied to present the influence of boundary conditions, form of RVE, shape and distribution of the reinforcement on the effective material properties.
- The comparison of numerical homogenization and micromechanical models showed that micromechanical models are appropriate in the case of the simple shapes of reinforcement.
- However, for complicated shapes of the reinforcement, the application of the numerical homogenization is crucial because the determination of characteristic relations such as d/l ratio is problematic.
Author Contributions
Funding
Conflicts of Interest
Appendix A
References
- Tsai, S.W. Theory of Composites Design; Think Composites: Dayton, OH, USA, 1992. [Google Scholar]
- Aboudi, J.; Arnold, S.M.; Bednarcyk, B.A. Micromechanics of Composite Materials. A Generalized Multiscale Analysis Approach; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Nemat-Nasser, S.; Hori, M. Micromechanics: Overall Properties of Heterogeneous Materials; Elsevier: Amsterdam, The Netherlands, 1993. [Google Scholar]
- Voigt, W. On the relation between the elasticity constants of isotropic bodies. Ann. Phys. Chem. 1889, 274, 573–587. [Google Scholar] [CrossRef]
- Reuss, A. Determination of the yield point of the polycrystals based on the yield conditions of single crystals. Z. Angew. Math. Mech. 1929, 9, 49–58. (In German) [Google Scholar] [CrossRef]
- Qin, Q.H.; Yang, Q.S. Macro-Micro Theory on Multifield Coupling Behavior of Heterogeneous Materials; Springer: Berlin, Germany, 2008. [Google Scholar]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. Lond. 1957, A241, 376–396. [Google Scholar]
- Hill, R. A self-consistent mechanics of composite materials. J. Mech. Phys. Solids 1965, 13, 213–222. [Google Scholar] [CrossRef]
- Christensen, R.M. Mechanics of Composite Materials; John Wiley & Sons Inc.: Hoboken, NJ, USA, 1979. [Google Scholar]
- Hashin, Z.; Shtrikman, S. A variational approach to the theory of the elastic behaviour of multiphase materials. J. Mech. Phys. Solids 1963, 11, 127–140. [Google Scholar] [CrossRef]
- Halpin, J.C. Stiffness and expansion estimates for oriented short fiber composites. J. Compos. Mater. 1969, 3, 732–734. [Google Scholar] [CrossRef]
- Halpin, J.C.; Kardos, J.L. The Halpin-Tsai equations: A review. Polym. Eng. Sci. 1976, 16, 344–352. [Google Scholar]
- Mori, T.; Tanaka, K. Average stress in matrix and average elastic energy of materials with misfitting inclusions. Acta Metal. 1973, 21, 571–574. [Google Scholar] [CrossRef]
- Benveniste, Y. A new approach to the application of Mori-Tanaka’s theory in composite materials. Mech. Mater. 1987, 6, 147–157. [Google Scholar] [CrossRef]
- Mura, T. Micromechanics of Defects in Solids; MN Publishers: London, UK, 1982. [Google Scholar]
- Bensoussan, A.; Lions, J.L.; Papanicolau, G. Asymptotic Analysis for Periodic Structure; Elsevier: Amsterdam, The Netherlands, 1978. [Google Scholar]
- Suquet, P.M. Elements of homogenization for inelastic solid mechanics. In Homogenization Techniques for Composite Media; Sanchez-Palencia, E., Zaoui, A., Eds.; Springer: Berlin, Germany, 1987; pp. 194–275. [Google Scholar]
- Aboudi, J. Mechanics of Composite Materials. A Unified Micromechanical Approach; Elsevier: New York, NY, USA, 1991. [Google Scholar]
- Chen, X.L.; Liu, Y.J. Square representative volume elements for evaluating the effective material properties of carbon nanotube-based composites. Comput. Mater. Sci. 2004, 29, 1–11. [Google Scholar] [CrossRef]
- Muc, A.; Jamróz, M. Homogenization models for carbon nanotubes. Mech. Compos. Mater. 2004, 40, 101–106. [Google Scholar] [CrossRef]
- Georgantzinos, S.K.; Giannopoulos, G.I.; Anifantis, N.K. Investigation of stress–strain behavior of single walled carbon nanotube/rubber composites by a multi-scale finite element method. Theor. Appl. Fract. Mech. 2009, 52, 158–164. [Google Scholar] [CrossRef]
- Shokrieh, M.M.; Rafiee, R. A review of the mechanical properties of isolated carbon nanotubes and carbon nanotube composites. Mech. Compos. Mater. 2010, 46, 155–172. [Google Scholar] [CrossRef]
- Muc, A. Design and identification methods of effective mechanical properties for carbon nanotubes. Mater. Des. 2010, 31, 1671–1675. [Google Scholar] [CrossRef]
- Chwał, M.; Kędziora, P.; Barski, M. Carbon nanotube/polymer nanocomposites: A brief modeling overview. Key Eng. Mater. 2013, 542, 29–42. [Google Scholar] [CrossRef]
- Chwał, M. Numerical evaluation of effective material constants for CNT-based polymeric nanocomposites. Adv. Mater. Res. 2014, 849, 88–93. [Google Scholar] [CrossRef]
- Arash, B.; Wang, Q.; Varadan, V.K. Mechanical properties of carbon nanotube/polymer composites. Sci. Rep. 2014, 4, 6479. [Google Scholar] [CrossRef]
- Malagu, M.; Goudarzi, M.; Lyulin, A.; Benvenuti, E.; Simone, A. Diameter-dependent elastic properties of carbon nanotube-polymer composites. Compos. B Eng. 2017, 131, 260–281. [Google Scholar] [CrossRef]
- Tserpes, K.I.; Silvestre, N. (Eds.) Modeling of Carbon Nanotubes, Graphene and Their Composites; Springer: Berlin, Germany, 2014. [Google Scholar]
- Alian, A.R.; Kundalwal, S.I.; Meguid, S.A. Multiscale modeling of carbon nanotube epoxy composites. Polymer 2015, 70, 149–160. [Google Scholar] [CrossRef]
- Gupta, A.K.; Harsha, S.P. Analysis of mechanical properties of carbon nanotube reinforced polymer composites using multi-scale finite element modeling approach. Compos. B Eng. 2016, 95, 172–178. [Google Scholar] [CrossRef]
- Chwał, M.; Muc, A. Transversely isotropic properties of carbon nanotube/polymer composites. Compos. B Eng. 2016, 88, 295–300. [Google Scholar] [CrossRef]
- Chwał, M. Deformations and tensile fracture of carbon nanotubes based on the numerical homogenization. Acta Phys. Pol. A 2017, 131, 440–442. [Google Scholar] [CrossRef]
- Radue, M.S.; Odegard, G.M. Multiscale modeling of carbon fiber/carbon nanotube/epoxy hybrid composites: Comparison of epoxy matrices. Compos. Sci. Technol. 2018, 166, 20–26. [Google Scholar] [CrossRef]
- Savvas, D.; Stefanou, G.; Papadopoulos, V.; Papadrakakis, M. Effect of waviness and orientation of carbon nanotubes on random apparent material properties and RVE size of CNT reinforced composites. Compos. Struct. 2016, 152, 870–882. [Google Scholar] [CrossRef]
- Muc, A. Choice of design variables in the stacking sequence optimization for laminated structures. Mech. Compos. Mater. 2016, 52, 211–224. [Google Scholar] [CrossRef]
- Muc, A.; Chwał, M. Analytical discrete stacking sequence optimization of rectangular composite plates subjected to buckling and FPF constraints. J. Theor. Appl. Mech. 2016, 54, 423–436. [Google Scholar] [CrossRef]
- Muc, A.; Romanowicz, P.; Chwał, M. Description of the resin curing process—formulation and optimization. Polymers 2019, 11, 127. [Google Scholar] [CrossRef]
- Chwał, M.; Muc, A. Simplified optimal modeling of resin injection molding process. e-Polymers 2019. accepted. [Google Scholar]
- Qian, L.F.; Batra, R.C.; Chen, L.M. Static and dynamic deformations of thick functionally graded elastic plate by using higher-ordershear and normal deformable plate theory and meshless local Petrov–Galerkin method. Compos. B Eng. 2004, 35, 685–697. [Google Scholar] [CrossRef]
- Jin, P.; Zhong, X. Flutter characteristic study of composite sandwich panel with functionally graded foam core. Int. J. Aerosp. Eng. 2016, 7971435. [Google Scholar] [CrossRef]
- Xu, X.J.; Meng, J.M. A model for functionally graded materials. Compos. B Eng. 2018, 145, 70–80. [Google Scholar] [CrossRef]
- Bendsoe, M.P.; Kikuchi, N. Generating optimal topologies in structural design using a homogenization method. Comput. Methods Appl. Mech. Eng. 1988, 71, 197–224. [Google Scholar] [CrossRef]
- Hassani, B.; Hinton, E. A review of homogenization and topology optimization: I—Homogenization theory for media with periodic structure. Comput. Struct. 1998, 69, 707–717. [Google Scholar] [CrossRef]
- Hassani, B.; Hinton, E. A review of homogenization and topology optimization: II—Analytical and numerical solution of homogenization equations. Comput. Struct. 1998, 69, 719–738. [Google Scholar] [CrossRef]
- Chen, Y.; Zhou, S.; Li, Q. Multiobjective topology optimization for finite periodic structures. Comput. Struct. 2010, 88, 806–811. [Google Scholar] [CrossRef]
- Groen, J.; Sigmund, O. Homogenization-based topology optimization for high-resolution manufacturable microstructures. Int. J. Numer. Meth. Eng. 2018, 113, 1148–1163. [Google Scholar] [CrossRef]
- Gao, J.; Luo, Z.; Li, H.; Gao, L. Topology optimization for multiscale design of porous composites with multi-domain microstructures. Comput. Methods Appl. Mech. Eng. 2019, 344, 451–476. [Google Scholar] [CrossRef]
- Sanchez-Palencia, E. Non-Homogenous Media and Vibration Theory; Lecture Notes in Physics; Springer: Berlin, Germany, 1980; p. 127. [Google Scholar]
- Xia, Z.; Zhou, C.; Yong, Q.; Wang, X. On selection of repeated unit cell model and application of unified periodic boundary conditions in micro-mechanical analysis of composites. Int. J. Sol. Struct. 2006, 43, 266–278. [Google Scholar] [CrossRef]
- Nguyen, V.D.; Bechet, E.; Geuzaine, C.; Noels, L. Imposing periodic boundary condition on arbitrary meshes by polynomial interpolation. Comput. Mater. Sci. 2012, 55, 390–406. [Google Scholar] [CrossRef]
- Sun, C.T.; Vaidya, R.S. Prediction of composite properties from a representative volume element. Compos. Sci. Technol. 1996, 56, 171–179. [Google Scholar] [CrossRef]
- Hashin, Z.; Rosen, B.W. The elastic moduli of fiber-reinforced materials. J. Appl. Mech. 1964, 31, 223–232. [Google Scholar] [CrossRef]
- Hill, R. Elastic properties of reinforced solids: Some theoretical principles. J. Mech. Phys. Solids 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Jones, R.M. Mechanics of Composite Materials; Taylor & Francis: Hoboken, NJ, USA, 1999. [Google Scholar]
- Vasiliev, V.V.; Morozov, E.V. Mechanics and Analysis of Composite Materials; Elsevier: Amsterdam, The Netherlands, 2001. [Google Scholar]
- Muc, A. Modeling of CNTs/nanocomposites deformations and tensile fracture. In Proceedings of the 17th International Conference on Composite Materials, ICCM-17, Edinburgh, Scotland, 27–31 July 2009. Code 85394. [Google Scholar]
- Muc, A.; Chwał, M. Vibration control of defects in carbon nanotubes. In Proceedings of the Symposium on Dynamics Modeling and Interaction Control in Virtual and Real Environments, Solid Mechanics and Its Applications, Budapest, Hungary, 7–11 June 2011; Volume 30, pp. 239–246. [Google Scholar]
- Muc, A.; Banaś, A.; Chwał, M. Free vibrations of carbon nanotubes with defects. Mech. Mech. Eng. 2013, 17, 157–166. [Google Scholar]
- Chwał, M. Nonlocal analysis of natural vibrations of carbon nanotubes. J. Mater. Eng. Perform. 2018, 27, 6087–6096. [Google Scholar] [CrossRef]
- Chwał, M.; Muc, A. Buckling and free vibrations of nanoplates—Comparison of nonlocal strain and stress approaches. Appl. Sci. 2019, 9, 1409. [Google Scholar] [CrossRef]
- Vanin, G.A. Micro-Mechanics of Composite Materials; Nauka Dumka: Kiev, Ukraine, 1985. [Google Scholar]
- Ramakrishnam, C.V.; Francavilla, A. Structural shape optimization using penalty functions. J. Struct. Mech. 1974, 3, 403–422. [Google Scholar] [CrossRef]
- Barthelemy, B.; Haftka, R.T. Accuracy analysis of the semi-analytical method for shape sensitivity calculation. Mech. Struct. Mach. 1993, 21, 407–432. [Google Scholar]
- Lee, M.S.; Kikuchi, N.; Scott, R.A. Shape optimization in laminated composite plates. Comput. Methods Appl. Mech. Eng. 1989, 72, 29–55. [Google Scholar] [CrossRef]
- Pedersen, P. Design for minimum stress concentration—Some practical aspect. In Structural Optimization; Rozvany, G.I.N., Karihaloo, B.L., Eds.; Kluwer: Dordrecht, The Netherlands, 1988; pp. 225–232. [Google Scholar]
- Pedersen, P.; Tobiesen, L.; Jensen, S.H. Shapes of orthotropic plates for minimum energy concentration. Mech. Struct. Mach. 1992, 20, 499–514. [Google Scholar] [CrossRef]
- Muc, A.; Gurba, W. Genetic algorithms and finite element analysis in optimization of composite structures. Compos. Struct. 2001, 54, 275–281. [Google Scholar] [CrossRef]
- Muc, A.; Muc-Wierzgoń, M. An evolution strategy in structural optimization problems for plates and shells. Compos. Struct. 2012, 94, 1461–1470. [Google Scholar] [CrossRef]
- Muc, A. Evolutionary design of engineering constructions. Lat. Am. J. Solids Struct. 2018, 15, e87. [Google Scholar] [CrossRef]
- Kristiansen, E.S.; Madsen, N.F. On the optimum shape of fillets in plates subjected to multiple in-plane loading cases. Int. J. Numer. Methods Eng. 1976, 10, 1007–1019. [Google Scholar] [CrossRef]
- Savvas, D.; Stefanou, G.; Papadrakakis, M.; Deodatis, G. Homogenization of random heterogeneous media with inclusions of arbitrary shape modeled by XFEM. Comput. Mech. 2014, 54, 1221–1235. [Google Scholar] [CrossRef]
- Fujii, T.; Zako, M. Damage Mechanics of Composite Materials; Mir: Moscow, Russia, 1982. (In Russian) [Google Scholar]
- Cox, H.L. The elasticity and strength of paper and other fibrous materials. Br. J. Appl. Phys. 1952, 3, 73–79. [Google Scholar] [CrossRef]
- Strzelecki, T. (Ed.) Mechanics of Nonhomogeneous Media. Theory of Homogenization; DWE: Wroclaw, Poland, 1996. (In Polish) [Google Scholar]
- Tatarkiewicz, K. Variational Calculus. Part 1; WNT: Warszawa, Poland, 1969. (In Polish) [Google Scholar]
- Muc, A.; Stawiarski, A.; Romanowicz, P. Experimental investigations of compressed sandwich composite/honeycomb cylindrical shells. Appl. Comp. Mater. 2018, 25, 177–189. [Google Scholar] [CrossRef]
- Muc, A.; Janczy, S.; Nogowczyk, R. Failure modes of sandwich structures with FRP faces—Theory vs experiments. In Proceedings of the 16th International Conference on Composite Materials, Kyoto, Japan, 8–13 July 2007. [Google Scholar]
- Muc, A.; Zuchara, P. Optimization of sandwich structures having FRP faces. Arch. Civ. Eng. 1999, 45, 289–304. [Google Scholar]
- Muc, A.; Zuchara, P. Buckling and failure analysis of FRP faced sandwich plates. Compos. Struct. 2000, 48, 145–150. [Google Scholar] [CrossRef]
- Muc, A.; Zuchara, P. Sandwich plates—Free vibrations and damping analysis. Mech. Compos. Mater. 1998, 34, 203–211. [Google Scholar] [CrossRef]
- Baniczuk, N.W.; Iwanowa, S.J.; Szaranjuk, A.W. Dynamics of Structures. Analysis and Optimisation; Nauka: Moscow, Russia, 1989. (In Russian) [Google Scholar]
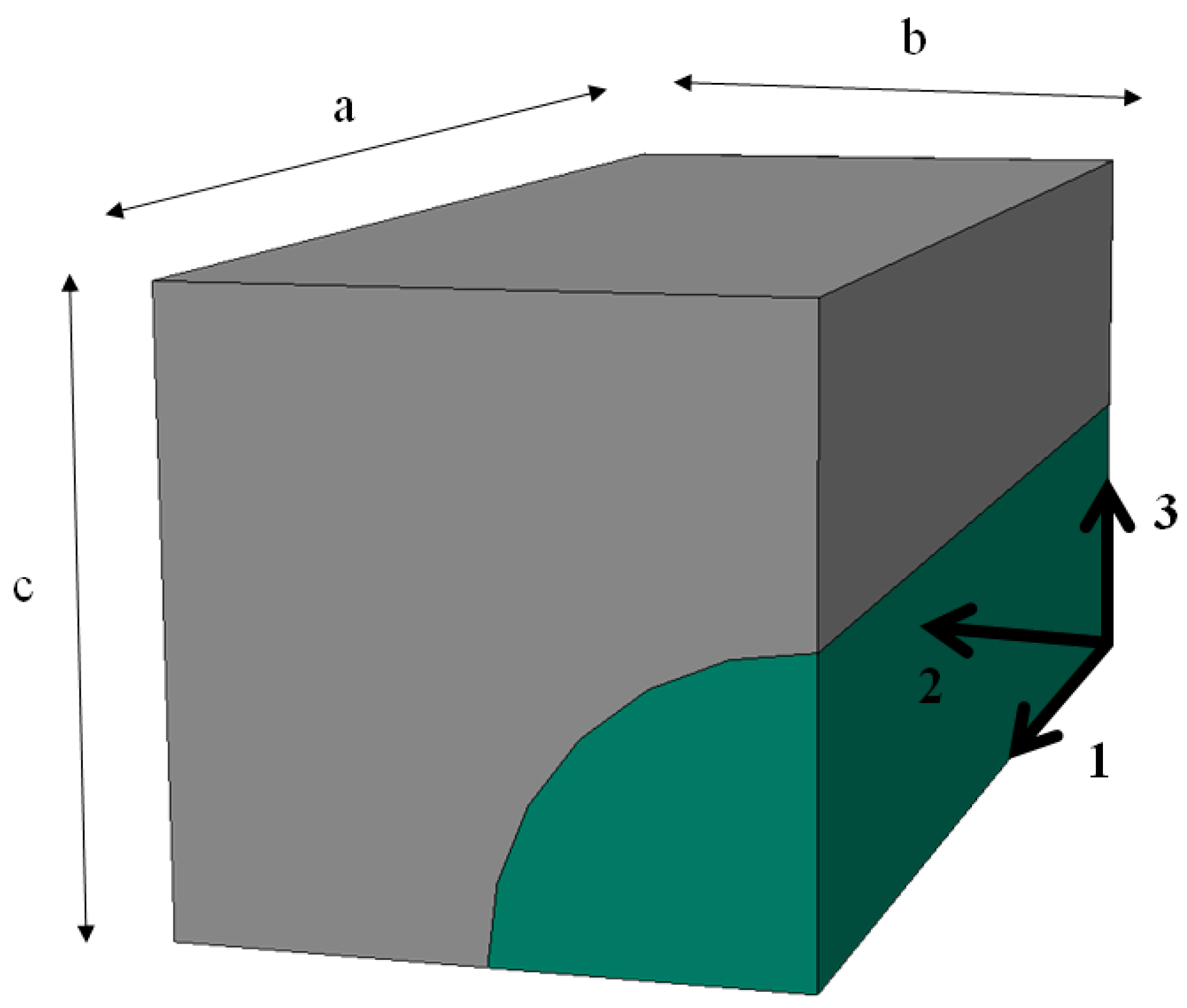
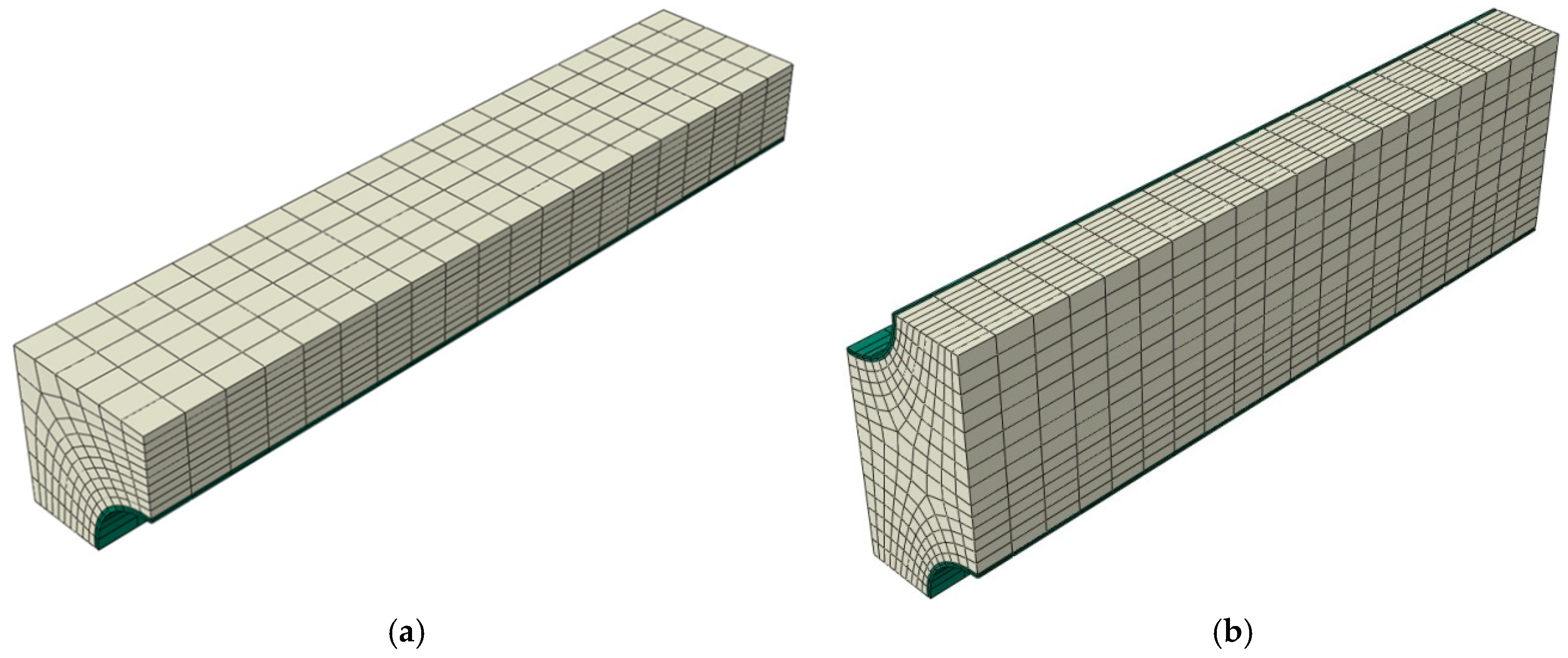

| Loading | Constants | Boundary Displacements | |||||
|---|---|---|---|---|---|---|---|
| x1 Direction | x2 Direction | x3 Direction | |||||
| x1 = 0 | x1 = a | x2 = 0 | x2 = b | x3 = 0 | x3 = c | ||
| Axial normal | and | u1 = 0 | u1 = e | u2 = 0 | u3 = 0 | ||
| Transverse normal | and | u1 = 0 | u2 = 0 | u2 = e | u3 = 0 | ||
| Axial shear | u2 = 0 u3 = 0 | u2 = e u3 = 0 | u1 = 0 u3 = 0 | u1 = 0 u3 = 0 | u3 = 0 | u3 = 0 | |
| Material Constant | Carbon Nanotube | Matrix |
|---|---|---|
| Young’s modulus (GPa) | 1000 | 3.2 |
| Poisson’s ratio | 0.3 | 0.3 |
| Parameter | Value | |
| Outer diameter of CNT (nm) | 10 | |
| Thickness of CNT (nm) | 0.3 | |
| Length of CNT (nm) | 100 | |
| Volume fraction of CNTs | 2.75% | |
| Parameter | Square Array | Hexagonal Array |
| Dimensions of RVE: 2a × 2b × 2c (nm) | 100 × 20 × 20 | 100 × 20 × 40 |
| Total number of FE for the whole RVE | 30,880 | 36,160 |
| Element type | C3D8R—8-node linear hexahedrons with reduced integration | |
| Model | (GPa) | (GPa) | (GPa) | (GPa) | ||
|---|---|---|---|---|---|---|
| FEM–square array | 30.62 | 3.90 | 0.3 | 0.6 | 4.29 | 1.22 |
| FEM–hexagonal array | 30.60 | 4.15 | 0.3 | 0.56 | 4.16 | 1.32 |
| Vanin model | 30.61 | 3.62 | 0.3 | 0.41 | 1.3 | 1.28 |
| Material Constant | Reinforcement | Matrix |
|---|---|---|
| Young’s modulus (GPa) | 70.4 | 3.2 |
| Poisson’s ratio | 0.22 | 0.35 |
| 2D RVEs | Effective Young’s Modulus (GPa) | ||
|---|---|---|---|
| FEM | Micromechanical Models | Rule of Mixture (Equation (A2)) | |
| bilayered structure | 9.301 | 9.119—(Equation (A3)) | 24.704 |
| align short fibre | 8.26 | 3.798—Cox (Equation (A5)) 8.777—H-T (Equation (A4)) | 24.704 |
| triangular shape of the reinforcement | 4.588 | 6.987—Cox (Equation (A5)) 7.280—H-T (Equation (A4)) | 9.92 |
| circular inclusion | 5.635 | - | 24.704 |
| Notation | Theoretical Solution | The Type of Algorithm | |
|---|---|---|---|
| Genetic Algorithm | Modified Evolutionary Strategy | ||
| The length of the arc— | 7.06858 | 7.06113 Error: 0.11% | 7.06344 Error: 0.073% |
| Area—Area | 15.90431 | 15.7987 Error: 0.66% | 15.83521 Error: 0.43% |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chwał, M.; Muc, A. Design of Reinforcement in Nano- and Microcomposites. Materials 2019, 12, 1474. https://doi.org/10.3390/ma12091474
Chwał M, Muc A. Design of Reinforcement in Nano- and Microcomposites. Materials. 2019; 12(9):1474. https://doi.org/10.3390/ma12091474
Chicago/Turabian StyleChwał, Małgorzata, and Aleksander Muc. 2019. "Design of Reinforcement in Nano- and Microcomposites" Materials 12, no. 9: 1474. https://doi.org/10.3390/ma12091474
APA StyleChwał, M., & Muc, A. (2019). Design of Reinforcement in Nano- and Microcomposites. Materials, 12(9), 1474. https://doi.org/10.3390/ma12091474