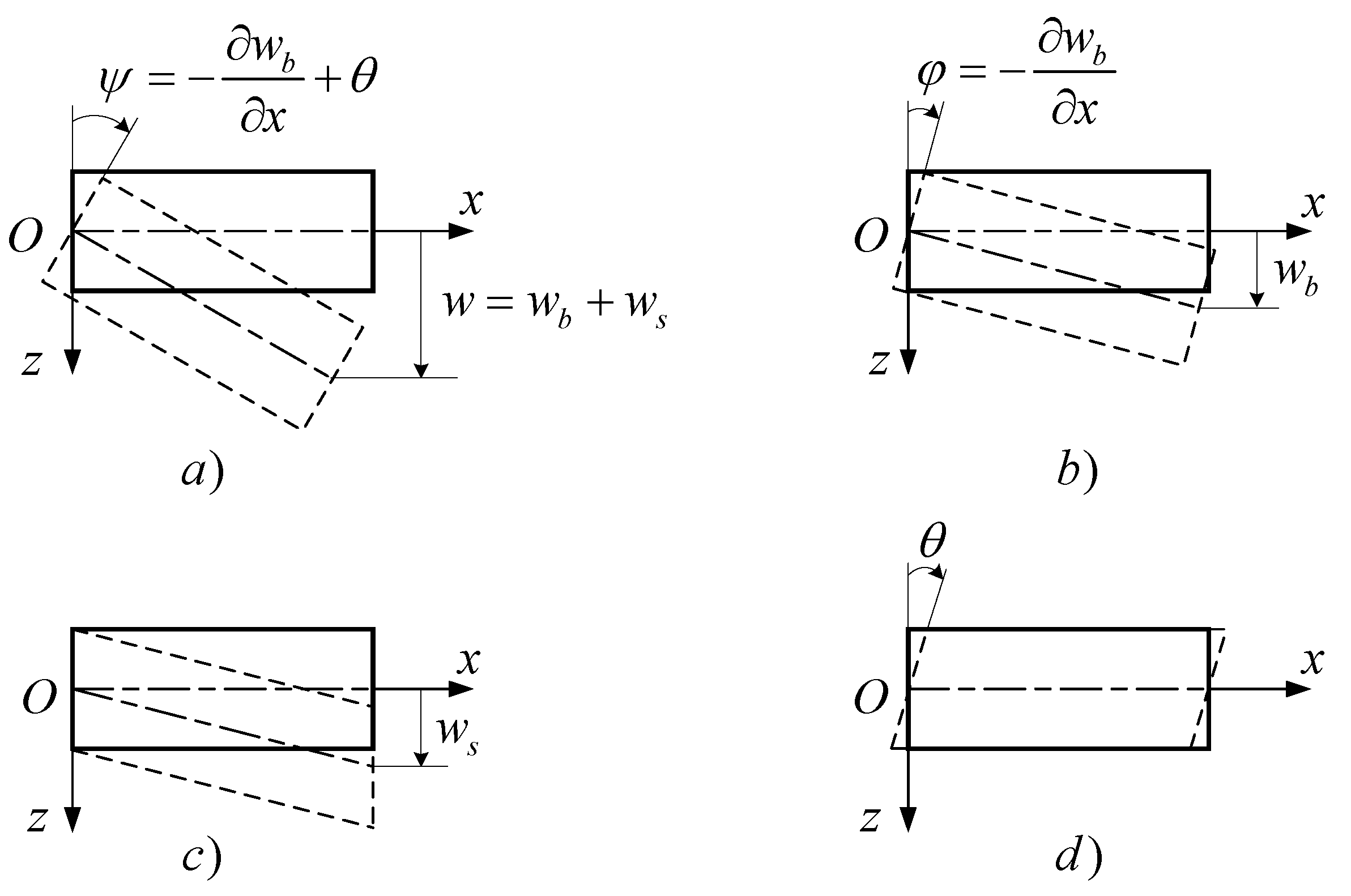
Figure 1.
The beam model with bending and shear deflection. (a) Total deflection and rotation, (b) pure bending and rotation, (c) transverse shear deflection, (d) shear angle rotation.
Figure 1.
The beam model with bending and shear deflection. (a) Total deflection and rotation, (b) pure bending and rotation, (c) transverse shear deflection, (d) shear angle rotation.
Figure 2.
Modeling of variable thickness FGM beam.
Figure 2.
Modeling of variable thickness FGM beam.
Figure 3.
The buckled form of the beam.
Figure 3.
The buckled form of the beam.
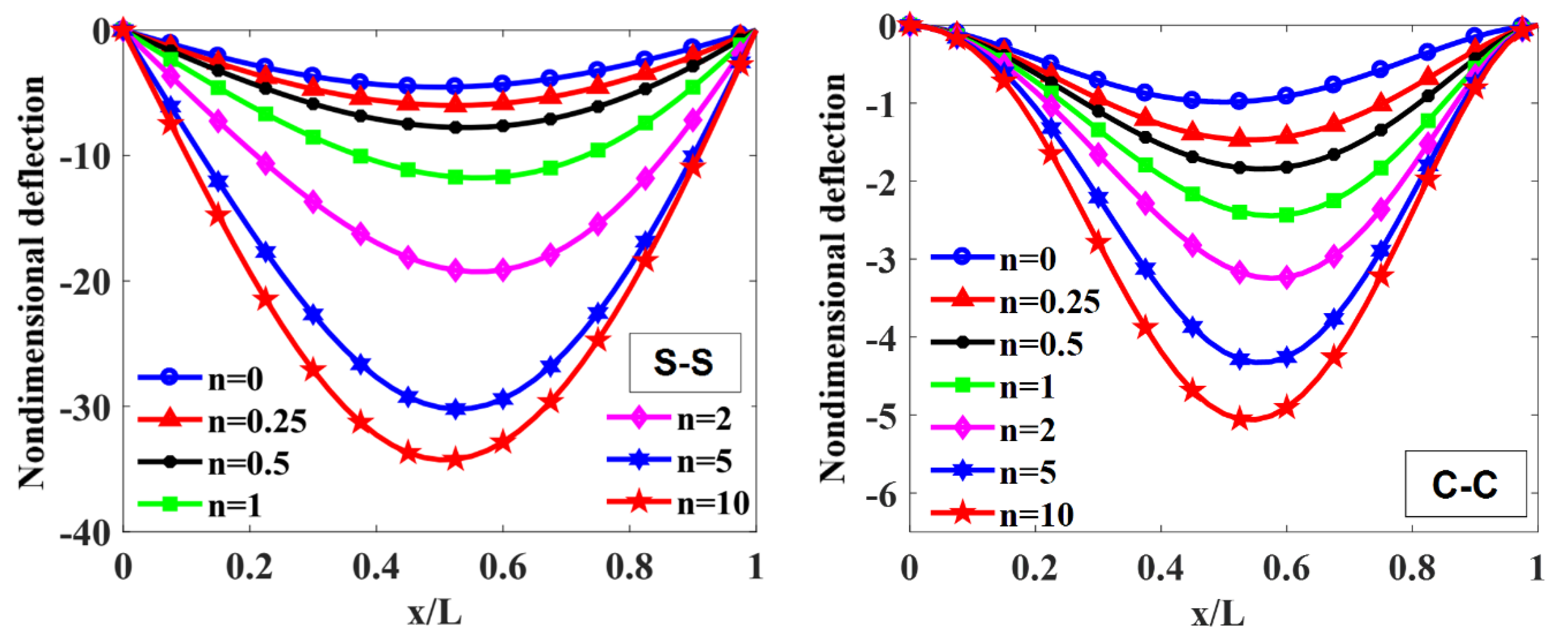
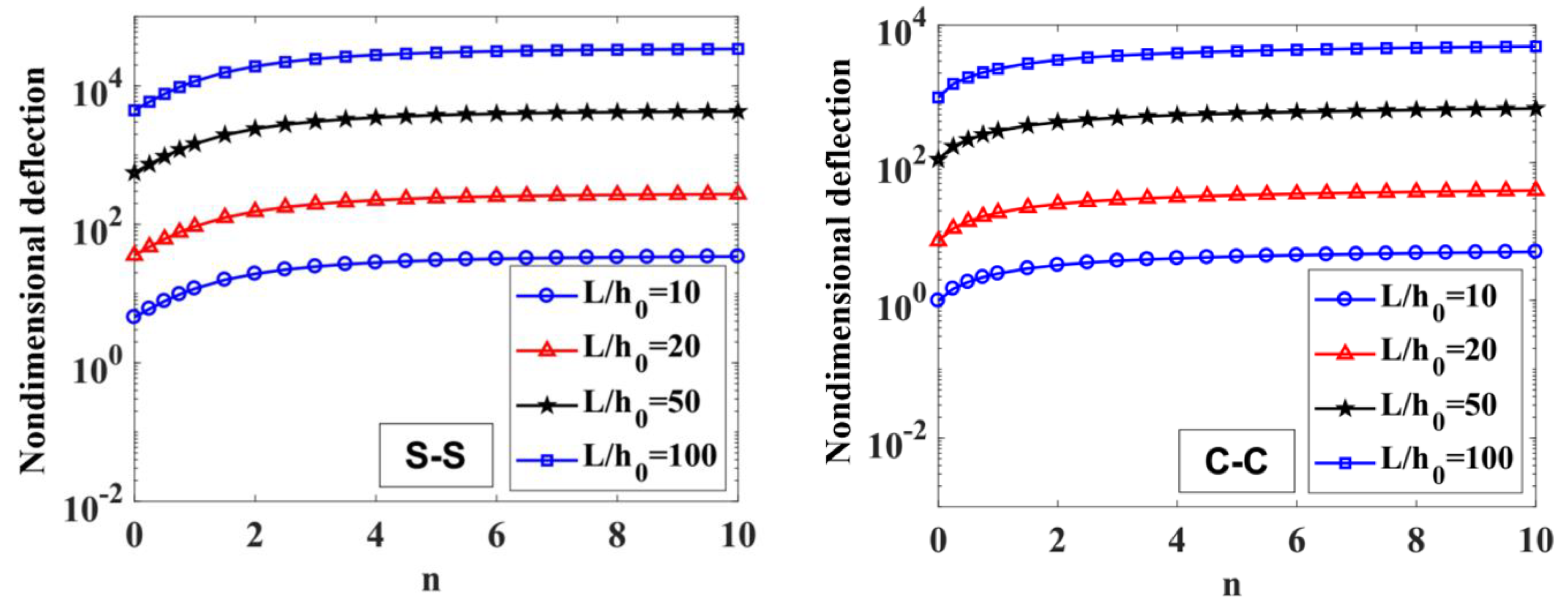
Figure 4.
The non-dimensional transverse deflection of S-S and C-C supported FGM beam with different values of index when and .
Figure 4.
The non-dimensional transverse deflection of S-S and C-C supported FGM beam with different values of index when and .
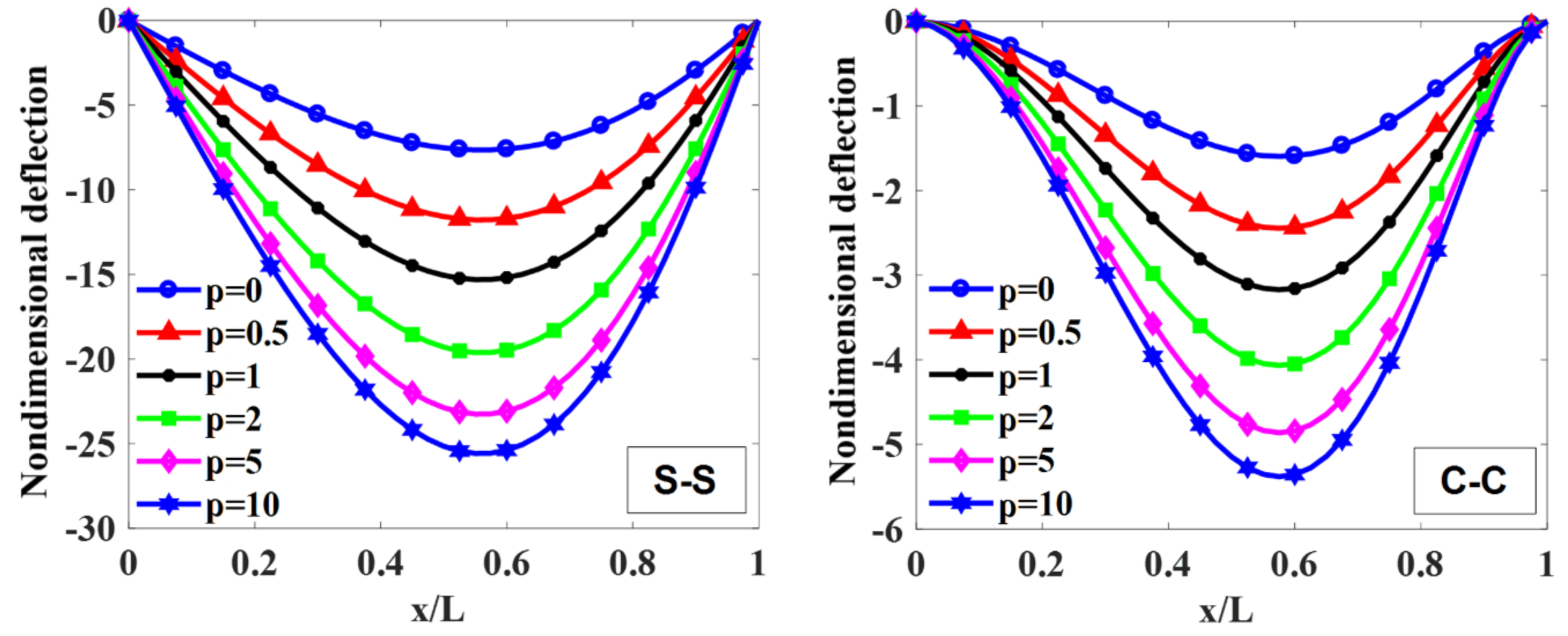
Figure 5.
The non-dimensional transverse deflection of S-S and C-C supported FGM beam with different values of index with and .
Figure 5.
The non-dimensional transverse deflection of S-S and C-C supported FGM beam with different values of index with and .
Figure 6.
The maximum non-dimensional transverse deflection, , of S-S and C-C supported FGM beam depend on index with and .
Figure 6.
The maximum non-dimensional transverse deflection, , of S-S and C-C supported FGM beam depend on index with and .
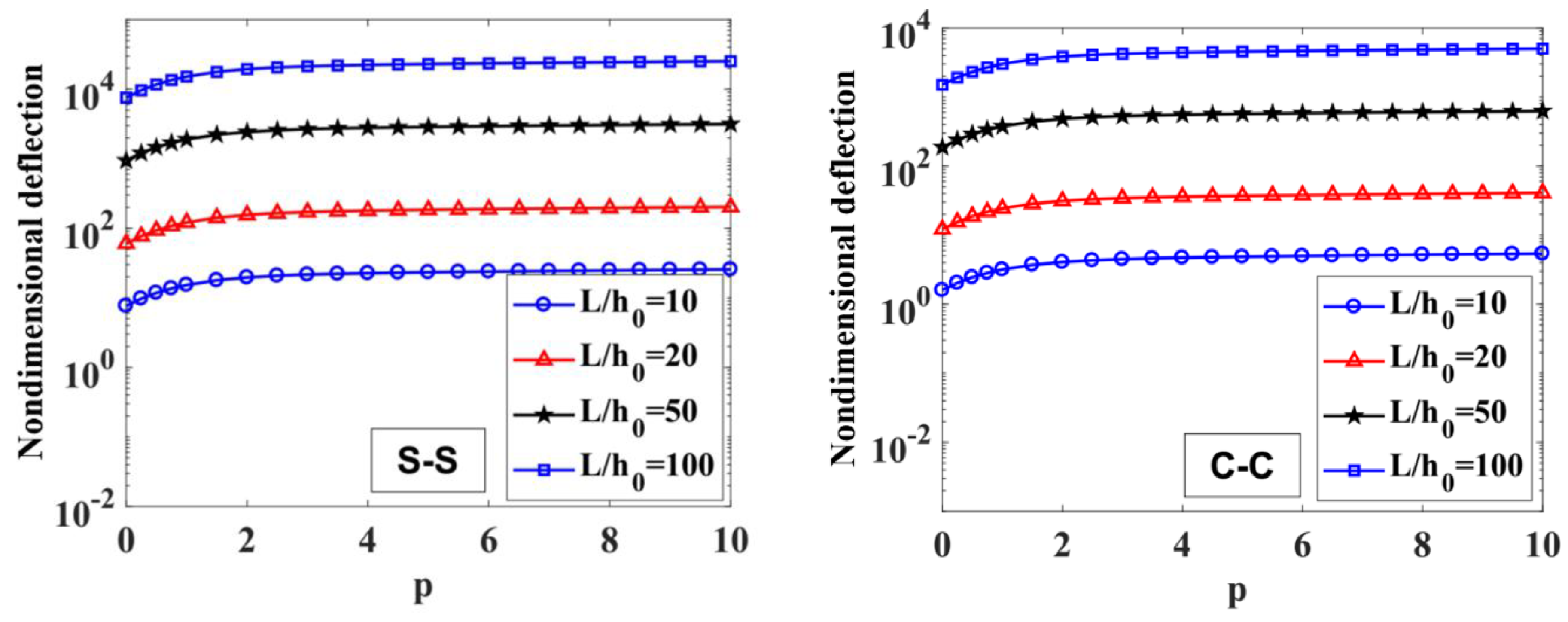
Figure 7.
The maximum non-dimensional transverse deflection, , of S-S and C-C supported FGM beam depending on index with and .
Figure 7.
The maximum non-dimensional transverse deflection, , of S-S and C-C supported FGM beam depending on index with and .
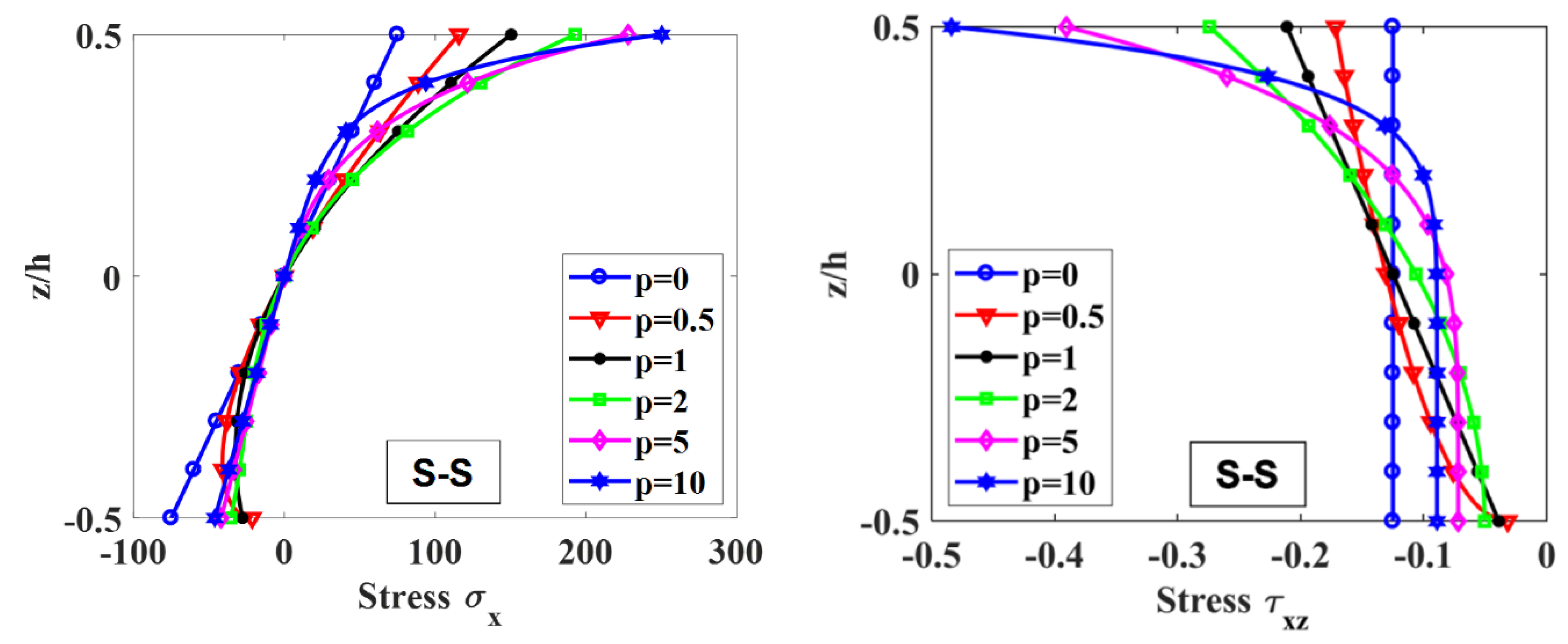
Figure 8.
Distribution of axial normal stress, , and shear stress, , at the mid-span of the beam across the height of S-S supported FGM beam under uniform load with .
Figure 8.
Distribution of axial normal stress, , and shear stress, , at the mid-span of the beam across the height of S-S supported FGM beam under uniform load with .
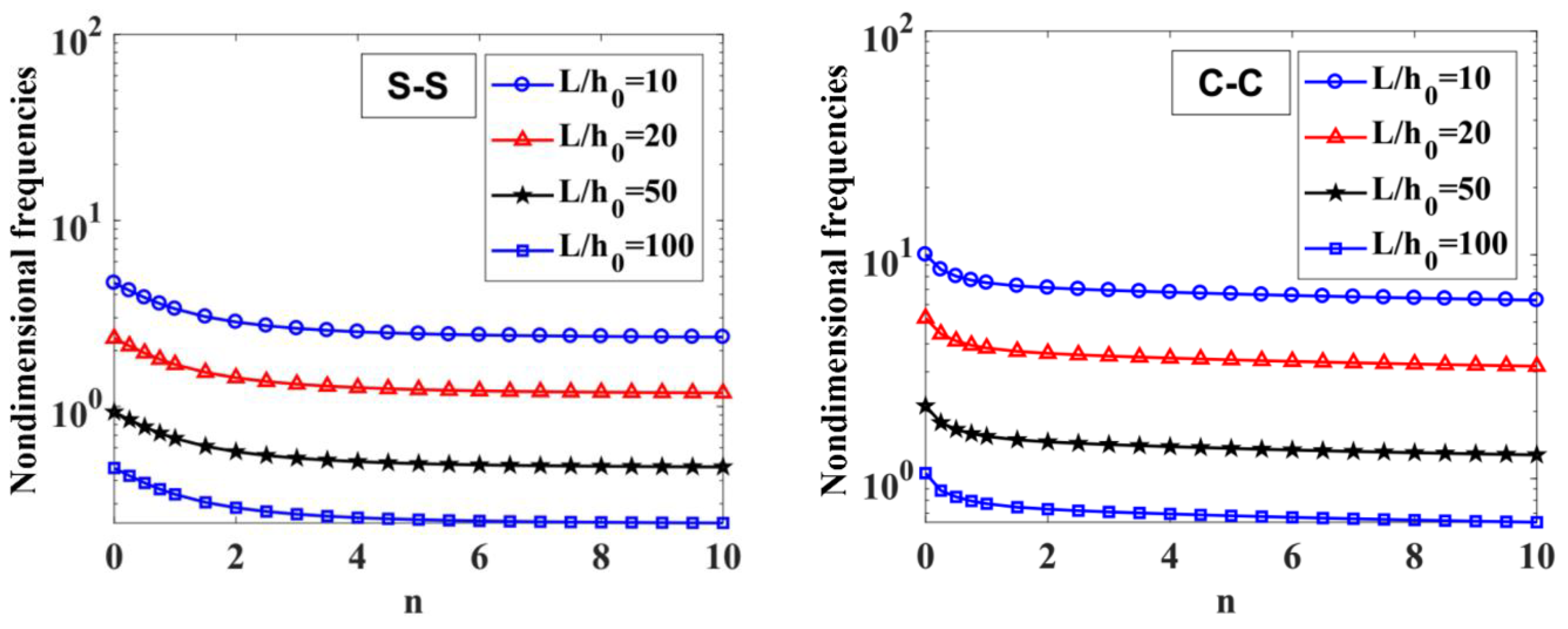
Figure 9.
The influence of index on the fundamental non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with and .
Figure 9.
The influence of index on the fundamental non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with and .
Figure 10.
The influence of on the fundamental non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with and .
Figure 10.
The influence of on the fundamental non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with and .
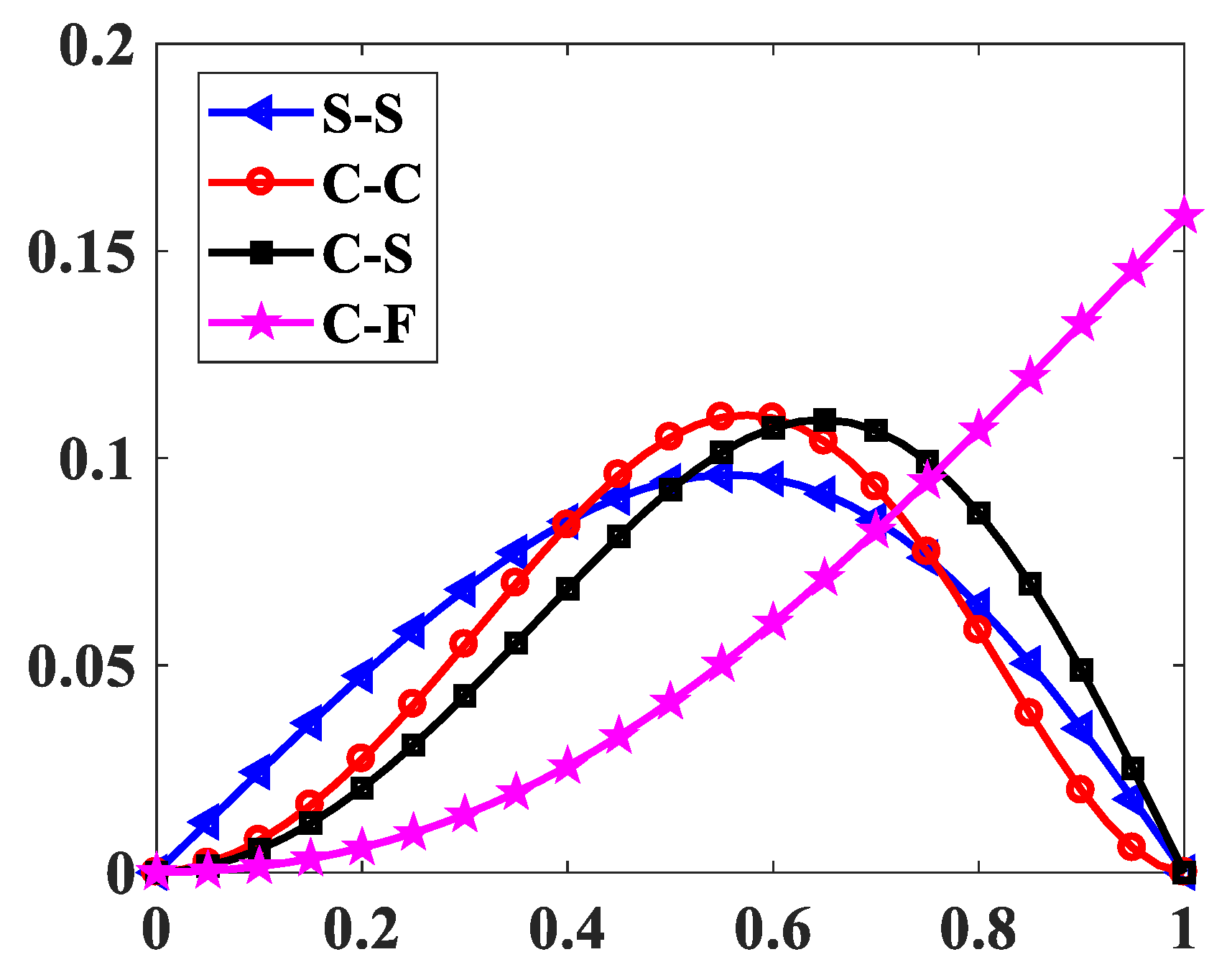
Figure 11.
The first four mode shapes of the FGM beam with .
Figure 11.
The first four mode shapes of the FGM beam with .
Figure 12.
The first mode shapes of the FGM beam with .
Figure 12.
The first mode shapes of the FGM beam with .
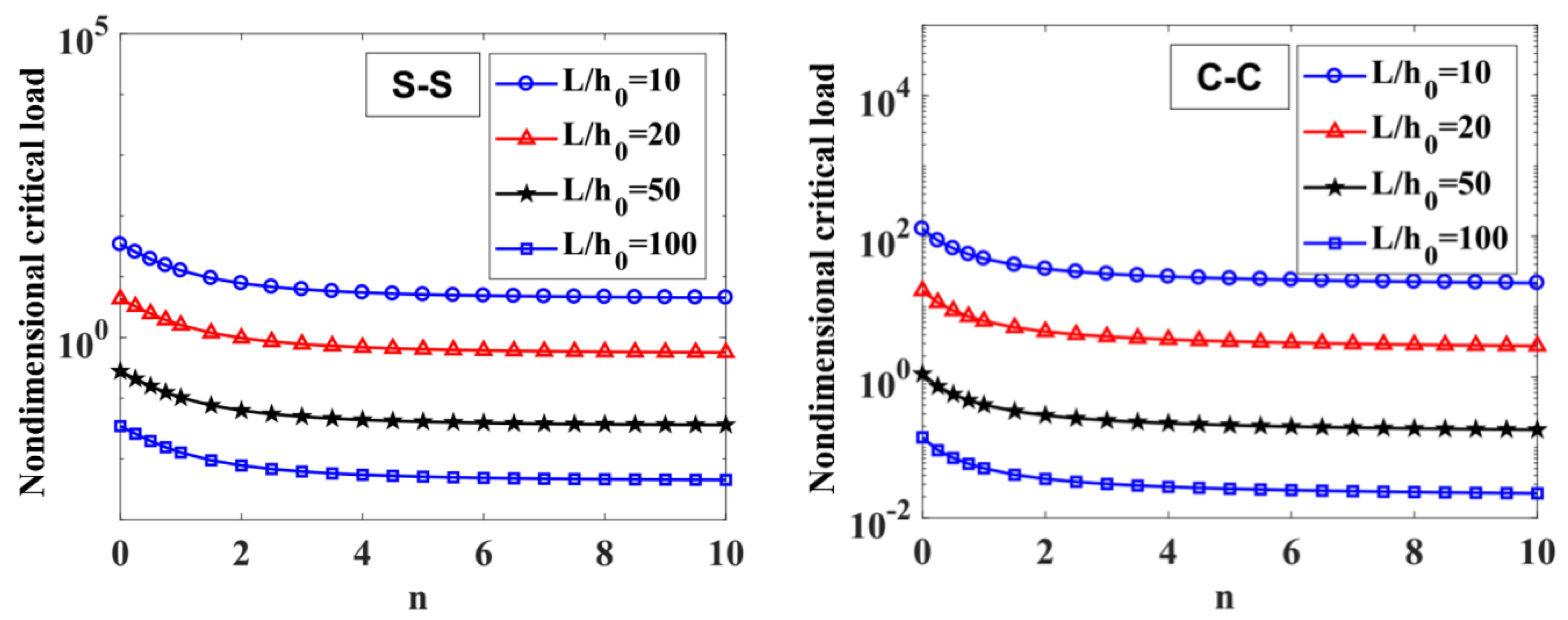
Figure 13.
The influence of index on the non-dimensional critical load, , of an S-S and C-C supported FGM beam with and .
Figure 13.
The influence of index on the non-dimensional critical load, , of an S-S and C-C supported FGM beam with and .
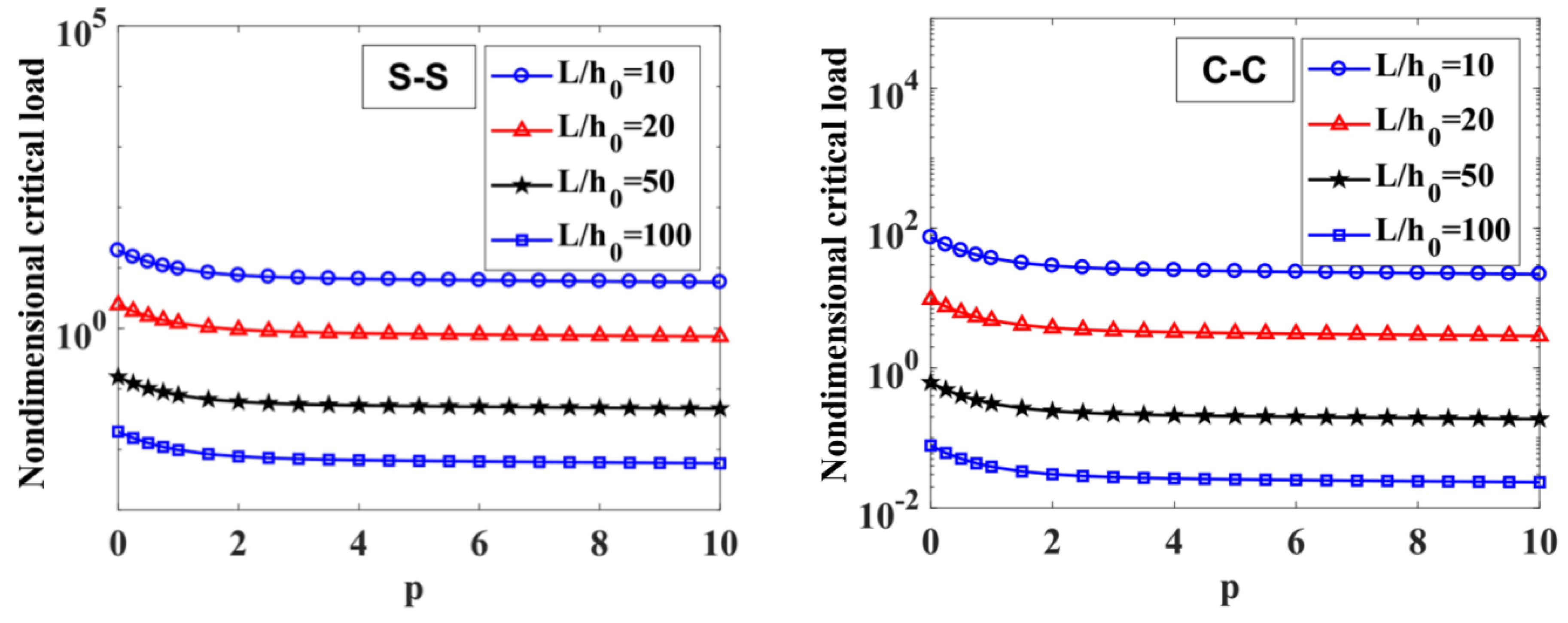
Figure 14.
The influence of index on the non-dimensional critical load, , of S-S and C-C supported FGM beam with and .
Figure 14.
The influence of index on the non-dimensional critical load, , of S-S and C-C supported FGM beam with and .
Figure 15.
The first buckling mode shapes of the FGM beam with .
Figure 15.
The first buckling mode shapes of the FGM beam with .
Figure 16.
The first buckling mode shapes of the FGM beam with .
Figure 16.
The first buckling mode shapes of the FGM beam with .
Table 1.
Comparison of non-dimensional mid-span deflection of an S-S supported isotropic beam for different values of the thickness parameter, with .
Table 1.
Comparison of non-dimensional mid-span deflection of an S-S supported isotropic beam for different values of the thickness parameter, with .
| n = 1 | n = 2 | n = 3 |
|---|
| [35] | Abaqus | Present | [35] | Abaqus | Present | [35] | Abaqus | Present |
|---|
| 0.05 | 1.3370 | 1.3500 | 1.3405 | 1.3111 | 1.3237 | 1.3144 | 1.3349 | 1.3356 | 1.3385 |
| 0.10 | 1.3441 | 1.3584 | 1.3493 | 1.2889 | 1.3021 | 1.2920 | 1.3359 | 1.3368 | 1.3395 |
| 0.20 | 1.3727 | 1.3967 | 1.3849 | 1.2479 | 1.2626 | 1.2508 | 1.3400 | 1.3416 | 1.3437 |
| 0.30 | 1.4204 | 1.4625 | 1.4497 | 1.2103 | 1.2279 | 1.2138 | 1.3467 | 1.3500 | 1.3510 |
| 0.40 | 1.4872 | 1.5534 | 1.5502 | 1.1747 | 1.1956 | 1.1801 | 1.3561 | 1.3428 | 1.3632 |
Table 2.
Comparison of non-dimensional deflection at the central point of the S-S supported FGM beam with constant thickness and different values of the ratio of , .
Table 2.
Comparison of non-dimensional deflection at the central point of the S-S supported FGM beam with constant thickness and different values of the ratio of , .
| p | L/h = 5 | L/h = 20 |
|---|
| [20] | SBT [35] | HBT [35] | Present | [20] | SBT [35] | HBT [35] | Present |
|---|
| 0 | 3.1657 | 3.1649 | 3.1654 | 3.1657 | 2.8962 | 2.8962 | 2.8962 | 2.8962 |
| 0.5 | 4.8292 | 4.8278 | 4.8285 | 4.8348 | 4.4645 | 4.4644 | 4.4644 | 4.4648 |
| 1 | 6.2599 | 6.2586 | 6.2594 | 6.2599 | 5.8049 | 5.8049 | 5.8049 | 5.8049 |
| 2 | 8.0602 | 8.0683 | 8.0675 | 8.0303 | 7.4415 | 7.4421 | 7.4420 | 7.4397 |
| 5 | 9.7802 | 9.8367 | 9.8271 | 9.6483 | 8.8151 | 8.8188 | 8.8181 | 8.8069 |
| 10 | 10.8979 | 10.9420 | 10.9375 | 10.7194 | 9.6879 | 9.6908 | 9.6905 | 9.6767 |
Table 3.
Maximum non-dimensional transverse deflection, , of a variable thickness beam.
Table 3.
Maximum non-dimensional transverse deflection, , of a variable thickness beam.
| | S-S | C-C |
|---|
| n = 0 | n = 0.5 | n = 1 | n = 2 | n = 0 | n = 0.5 | n = 1 | n = 2 |
|---|
| 10 | 0 | 2.9501 | 5.0428 | 7.6450 | 12.4967 | 0.6475 | 1.2046 | 1.5965 | 2.1175 |
| 0.5 | 4.5388 | 7.7639 | 11.7761 | 19.2590 | 0.9867 | 1.8432 | 2.4462 | 3.2483 |
| 1 | 5.8959 | 10.0885 | 15.3053 | 25.0363 | 1.2763 | 2.3884 | 3.1717 | 4.2139 |
| 2 | 7.5578 | 12.9313 | 19.6172 | 32.0881 | 1.6376 | 3.0634 | 4.0674 | 5.4034 |
| 5 | 8.9752 | 15.3402 | 23.2520 | 38.0049 | 1.9745 | 3.6699 | 4.8622 | 6.4472 |
| 10 | 9.8853 | 16.8831 | 25.5737 | 41.7764 | 2.1995 | 4.0688 | 5.3818 | 7.1264 |
| 20 | 0 | 2.8962 | 4.9748 | 7.5688 | 12.4132 | 0.5936 | 1.1383 | 1.5228 | 2.0363 |
| 0.5 | 4.4648 | 7.6707 | 11.6719 | 19.1450 | 0.9127 | 1.7523 | 2.3451 | 3.1368 |
| 1 | 5.8049 | 9.9740 | 15.1774 | 24.8963 | 1.1853 | 2.2770 | 3.0475 | 4.0769 |
| 2 | 7.4397 | 12.7829 | 19.4513 | 31.9066 | 1.5194 | 2.9186 | 3.9062 | 5.2256 |
| 5 | 8.8069 | 15.1288 | 23.0158 | 37.7459 | 1.8063 | 3.4628 | 4.6323 | 6.1939 |
| 10 | 9.6767 | 16.6219 | 25.2822 | 41.4567 | 1.9910 | 3.8118 | 5.0969 | 6.8128 |
| 50 | 0 | 2.8812 | 4.9578 | 7.5456 | 12.4021 | 0.5785 | 1.1233 | 1.5022 | 2.0150 |
| 0.5 | 4.4441 | 7.6483 | 11.6397 | 19.1344 | 0.8920 | 1.7328 | 2.3168 | 3.1082 |
| 1 | 5.7794 | 9.9470 | 15.1372 | 24.8863 | 1.1598 | 2.2536 | 3.0127 | 4.0421 |
| 2 | 7.4066 | 12.7482 | 19.3989 | 31.8952 | 1.4864 | 2.8883 | 3.8610 | 5.1806 |
| 5 | 8.7597 | 15.0791 | 22.9408 | 37.7255 | 1.7591 | 3.4164 | 4.5675 | 6.1296 |
| 10 | 9.6183 | 16.5627 | 25.1875 | 41.4396 | 1.9326 | 3.7526 | 5.0161 | 6.7344 |
| 100 | 0 | 2.8790 | 4.9590 | 7.5518 | 12.4096 | 0.5764 | 1.1247 | 1.5013 | 2.0129 |
| 0.5 | 4.4413 | 7.6516 | 11.6519 | 19.1490 | 0.8890 | 1.7356 | 2.3162 | 3.1058 |
| 1 | 5.7758 | 9.9518 | 15.1541 | 24.9056 | 1.1561 | 2.2575 | 3.0123 | 4.0393 |
| 2 | 7.4018 | 12.7551 | 19.4218 | 31.9211 | 1.4816 | 2.8933 | 3.8607 | 5.1771 |
| 5 | 8.7529 | 15.0879 | 22.9710 | 37.7591 | 1.7524 | 3.4219 | 4.5666 | 6.1242 |
| 10 | 9.6098 | 16.5766 | 25.2311 | 41.4862 | 1.9242 | 3.7590 | 5.0162 | 6.7286 |
Table 4.
Comparison of the first three non-dimensional natural frequencies for a cantilever isotropic bean with different values of the taper ratio.
Table 4.
Comparison of the first three non-dimensional natural frequencies for a cantilever isotropic bean with different values of the taper ratio.
| c | | | |
|---|
| [34] | Abaqus | Present | [34] | Abaqus | Present | [34] | Abaqus | Present |
|---|
| 0.1 | 3.559 | 3.562 | 3.553 | 21.338 | 21.140 | 21.132 | 58.980 | 57.510 | 57.663 |
| 0.2 | 3.608 | 3.612 | 3.603 | 20.621 | 20.453 | 20.439 | 56.192 | 54.939 | 55.045 |
| 0.3 | 3.667 | 3.669 | 3.662 | 19.881 | 19.739 | 19.720 | 53.322 | 52.269 | 52.331 |
| 0.4 | 3.737 | 3.739 | 3.732 | 19.114 | 18.996 | 18.975 | 50.354 | 49.487 | 49.513 |
| 0.5 | 3.824 | 3.826 | 3.819 | 18.317 | 18.222 | 18.198 | 47.265 | 46.568 | 46.565 |
| 0.6 | 3.934 | 3.936 | 3.930 | 17.488 | 17.413 | 17.391 | 44.025 | 43.482 | 43.464 |
| 0.7 | 4.082 | 4.083 | 4.078 | 16.625 | 16.568 | 16.548 | 40.588 | 40.186 | 40.155 |
| 0.8 | 4.292 | 4.293 | 4.290 | 15.743 | 15.701 | 15.691 | 36.885 | 36.608 | 36.583 |
| 0.9 | 4.631 | 4.631 | 4.630 | 14.931 | 14.902 | 14.911 | 32.833 | 32.671 | 32.688 |
Table 5.
Comparison of the first three non-dimensional natural frequencies of beam with and .
Table 5.
Comparison of the first three non-dimensional natural frequencies of beam with and .
| L/h | p | | | |
|---|
| SBT [14] | HBT [14] | Present | SBT [14] | HBT [14] | Present | SBT [14] | HBT [14] | Present |
|---|
| 5 | 0 | 5.1531 | 5.1527 | 5.2220 | 17.8868 | 17.8810 | 18.4730 | 34.2344 | 34.2085 | 35.6198 |
| 0.5 | 4.4110 | 4.4107 | 4.4693 | 15.4631 | 15.4587 | 15.9861 | 29.8569 | 29.8373 | 31.1588 |
| 1 | 3.9907 | 3.9904 | 4.0497 | 14.0138 | 14.0098 | 14.5588 | 27.1152 | 27.0971 | 28.5214 |
| 2 | 3.6263 | 3.6265 | 3.6936 | 12.6411 | 12.6407 | 13.2636 | 24.3237 | 24.3151 | 25.9539 |
| 5 | 3.3998 | 3.4014 | 3.4882 | 11.5324 | 11.5444 | 12.3067 | 21.6943 | 21.7187 | 23.6695 |
| 10 | 3.2811 | 3.2817 | 3.3644 | 11.0216 | 11.0246 | 11.7210 | 20.5581 | 20.5569 | 22.2828 |
| 20 | 0 | 5.4603 | 5.4603 | 5.4658 | 21.5736 | 21.5732 | 21.6578 | 47.5950 | 47.5930 | 47.9905 |
| 0.5 | 4.6511 | 4.6511 | 4.6556 | 18.3965 | 18.3962 | 18.4665 | 40.6542 | 40.6526 | 40.9852 |
| 1 | 4.2051 | 4.2051 | 4.2096 | 16.6347 | 16.6344 | 16.7048 | 36.7692 | 36.7679 | 37.1020 |
| 2 | 3.8361 | 3.8361 | 3.8413 | 15.1617 | 15.1619 | 15.2418 | 33.4681 | 33.4691 | 33.8471 |
| 5 | 3.6484 | 3.6485 | 3.6554 | 14.3728 | 14.3748 | 14.4806 | 31.5699 | 31.5789 | 32.0740 |
| 10 | 3.5389 | 3.5390 | 3.5457 | 13.9255 | 13.9264 | 14.0289 | 30.5337 | 30.5373 | 31.0136 |
Table 6.
First three non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with depending on index and index .
Table 6.
First three non-dimensional natural frequencies, , of S-S and C-C supported FGM beam with depending on index and index .
| Mode | p | S-S | C-C |
|---|
| n = 0 | n = 0.5 | n = 1 | n = 2 | n = 0 | n = 0.5 | n = 1 | n = 2 |
|---|
| 1 | 0 | 5.4144 | 4.5186 | 3.9296 | 3.3404 | 11.6989 | 9.3900 | 8.7793 | 8.3438 |
| 0.5 | 4.6165 | 3.8514 | 3.3485 | 2.8457 | 10.0244 | 8.0292 | 7.5020 | 7.1257 |
| 1 | 4.1761 | 3.4834 | 3.0282 | 2.5733 | 9.0882 | 7.2725 | 6.7930 | 6.4505 |
| 2 | 3.8104 | 3.1785 | 2.7632 | 2.3481 | 8.2881 | 6.6336 | 6.1967 | 5.8846 |
| 5 | 3.6201 | 3.0214 | 2.6277 | 2.2339 | 7.8125 | 6.2736 | 5.8667 | 5.5765 |
| 10 | 3.5072 | 2.9282 | 2.5476 | 2.1664 | 7.5246 | 6.0571 | 5.6688 | 5.3921 |
| 2 | 0 | 20.8896 | 17.5473 | 15.6625 | 13.8003 | 30.2375 | 25.1511 | 23.2809 | 21.5200 |
| 0.5 | 17.8784 | 14.9960 | 13.3746 | 11.7763 | 26.0655 | 21.6021 | 19.9692 | 18.4359 |
| 1 | 16.1999 | 13.5793 | 12.1067 | 10.6567 | 23.6957 | 19.6054 | 18.1126 | 16.7125 |
| 2 | 14.7756 | 12.3871 | 11.0448 | 9.7226 | 21.5964 | 17.8751 | 16.5163 | 15.2415 |
| 5 | 13.9541 | 11.7253 | 10.4682 | 9.2250 | 20.1629 | 16.7856 | 15.5430 | 14.3714 |
| 10 | 13.4588 | 11.3281 | 10.1235 | 8.9283 | 19.2868 | 16.1225 | 14.9524 | 13.8450 |
| 3 | 0 | 44.4998 | 37.8226 | 34.0651 | 30.3772 | 55.1307 | 47.1283 | 43.6107 | 40.0379 |
| 0.5 | 38.2849 | 32.4491 | 29.1820 | 25.9891 | 47.8038 | 40.6706 | 37.5628 | 34.4232 |
| 1 | 34.7730 | 29.4349 | 26.4536 | 23.5455 | 43.5761 | 36.9917 | 34.1349 | 31.2560 |
| 2 | 31.6987 | 26.8401 | 24.1253 | 21.4760 | 39.6909 | 33.7104 | 31.1133 | 28.4944 |
| 5 | 29.6875 | 25.2494 | 22.7497 | 20.2930 | 36.7108 | 31.4169 | 29.0861 | 26.7146 |
| 10 | 28.4609 | 24.2835 | 21.9179 | 19.5804 | 34.8885 | 30.0141 | 27.8481 | 25.6299 |
Table 7.
Fundamental non-dimensional natural frequencies, of (S-S), and (C-C) FGM beams depend on ratio, , and index .
Table 7.
Fundamental non-dimensional natural frequencies, of (S-S), and (C-C) FGM beams depend on ratio, , and index .
| p | S-S | C-C |
|---|
| n = 0 | n = 0.5 | n = 1 | n = 2 | n = 0 | n = 0.5 | n = 1 | n = 2 |
|---|
| 10 | 0 | 5.4144 | 4.5186 | 3.9296 | 3.3404 | 11.6989 | 9.3900 | 8.7793 | 8.3438 |
| 0.5 | 4.6165 | 3.8514 | 3.3485 | 2.8457 | 10.0244 | 8.0292 | 7.5020 | 7.1257 |
| 1 | 4.1761 | 3.4834 | 3.0282 | 2.5733 | 9.0882 | 7.2725 | 6.7930 | 6.4505 |
| 2 | 3.8104 | 3.1785 | 2.7632 | 2.3481 | 8.2881 | 6.6336 | 6.1967 | 5.8846 |
| 5 | 3.6201 | 3.0214 | 2.6277 | 2.2339 | 7.8125 | 6.2736 | 5.8667 | 5.5765 |
| 10 | 3.5072 | 2.9282 | 2.5476 | 2.1664 | 7.5246 | 6.0571 | 5.6688 | 5.3921 |
| 20 | 0 | 2.7329 | 2.2752 | 1.9750 | 1.6760 | 6.1175 | 4.8350 | 4.4997 | 4.2589 |
| 0.5 | 2.3278 | 1.9377 | 1.6820 | 1.4273 | 5.2178 | 4.1212 | 3.8349 | 3.6291 |
| 1 | 2.1048 | 1.7520 | 1.5207 | 1.2904 | 4.7208 | 3.7276 | 3.4683 | 3.2820 |
| 2 | 1.9207 | 1.5987 | 1.3877 | 1.1775 | 4.3072 | 3.4012 | 3.1647 | 2.9947 |
| 5 | 1.8277 | 1.5215 | 1.3208 | 1.1209 | 4.0899 | 3.2328 | 3.0088 | 2.8478 |
| 10 | 1.7729 | 1.4759 | 1.2814 | 1.0875 | 3.9606 | 3.1328 | 2.9163 | 2.7608 |
| 50 | 0 | 1.0961 | 0.9118 | 0.7912 | 0.6708 | 2.4797 | 1.9477 | 1.8127 | 1.7133 |
| 0.5 | 0.9334 | 0.7763 | 0.6737 | 0.5712 | 2.1120 | 1.6585 | 1.5437 | 1.4589 |
| 1 | 0.8438 | 0.7019 | 0.6091 | 0.5164 | 1.9096 | 1.4993 | 1.3957 | 1.3190 |
| 2 | 0.7700 | 0.6405 | 0.5558 | 0.4712 | 1.7425 | 1.3682 | 1.2736 | 1.2036 |
| 5 | 0.7331 | 0.6097 | 0.5292 | 0.4486 | 1.6584 | 1.3025 | 1.2124 | 1.1457 |
| 10 | 0.7114 | 0.5915 | 0.5135 | 0.4352 | 1.6087 | 1.2636 | 1.1763 | 1.1114 |
| 100 | 0 | 0.5483 | 0.4559 | 0.3955 | 0.3353 | 1.2422 | 0.9734 | 0.9069 | 0.8572 |
| 0.5 | 0.4668 | 0.3881 | 0.3368 | 0.2855 | 1.0578 | 0.8287 | 0.7721 | 0.7299 |
| 1 | 0.4221 | 0.3509 | 0.3044 | 0.2581 | 0.9563 | 0.7492 | 0.6981 | 0.6598 |
| 2 | 0.3851 | 0.3202 | 0.2778 | 0.2355 | 0.8727 | 0.6837 | 0.6370 | 0.6021 |
| 5 | 0.3667 | 0.3048 | 0.2645 | 0.2242 | 0.8308 | 0.6509 | 0.6064 | 0.5732 |
| 10 | 0.3558 | 0.2957 | 0.2566 | 0.2175 | 0.8062 | 0.6314 | 0.5883 | 0.5560 |
Table 8.
Comparison of the buckling load of the constant thickness beam with different values of the ratio of for S-S and C-C support conditions.
Table 8.
Comparison of the buckling load of the constant thickness beam with different values of the ratio of for S-S and C-C support conditions.
| L/h | S-S | C-C |
|---|
| Analytical Solution [8] | [52] | [8] | Abaqus | Present | Analytical Solution [8] | [52] | [8] | Abaqus | Present |
|---|
| 10 | 8013.8 | 8021.8 | 8013.86 | 8020.9 | 8013.83 | 29766 | 29877 | 29770 | 29864 | 29767.2 |
| 100 | 8.223 | 8.231 | 8.2225 | 8.2258 | 8.2225 | 32.864 | 32.999 | 32.864 | 32.917 | 32.864 |
| 1000 | 0.0082 | 0.0082 | 0.00822 | 0.00823 | 0.00822 | 0.0329 | 0.0330 | 0.0329 | 0.03295 | 0.0329 |
Table 9.
Comparison of the nondimensional buckling load of the constant thickness FGM beam with the ratio of and for the S-S and C-C support condition.
Table 9.
Comparison of the nondimensional buckling load of the constant thickness FGM beam with the ratio of and for the S-S and C-C support condition.
| p | L/h = 5, C-C | L/h = 5, S-S | L/h = 10, C-C | L/h = 10, S-S |
|---|
| [9] | Present | [9] | Present | [9] | Present | [9] | Present |
|---|
| 0 | 154.35 | 154.37 | 48.835 | 48.836 | 195.34 | 195.35 | 52.309 | 52.308 |
| 0.5 | 103.22 | 103.23 | 31.967 | 31.968 | 127.87 | 127.87 | 33.996 | 33.997 |
| 1 | 80.498 | 80.505 | 24.687 | 24.687 | 98.749 | 98.752 | 26.171 | 26.171 |
| 2 | 62.614 | 62.620 | 19.245 | 19.245 | 76.980 | 76.983 | 20.416 | 20.416 |
| 5 | 50.384 | 50.389 | 16.024 | 16.024 | 64.096 | 64.099 | 17.192 | 17.194 |
| 10 | 44.267 | 44.272 | 14.427 | 14.427 | 57.708 | 57.711 | 15.612 | 15.612 |
Table 10.
Non-dimensional critical load, , of an S-S and C-C variable thickness FGM beam depending on the ratio of and index .
Table 10.
Non-dimensional critical load, , of an S-S and C-C variable thickness FGM beam depending on the ratio of and index .
| p | S-S | C-C |
|---|
| n = 0 | n = 0.5 | n = 1 | n = 2 | n = 0 | n = 0.5 | n = 1 | n = 2 |
|---|
| 10 | 0 | 52.2381 | 29.9212 | 19.4184 | 11.9650 | 194.3954 | 103.3309 | 73.8638 | 52.8577 |
| 0.5 | 33.9560 | 19.4365 | 12.6075 | 7.7642 | 127.3125 | 67.4450 | 48.1566 | 34.4249 |
| 1 | 26.1410 | 14.9587 | 9.7007 | 5.9727 | 98.3404 | 52.0179 | 37.1226 | 26.5247 |
| 2 | 20.3927 | 11.6700 | 7.5685 | 4.6601 | 76.6582 | 40.5622 | 28.9508 | 20.6879 |
| 5 | 17.1703 | 9.8358 | 6.3844 | 3.9343 | 63.7783 | 33.9271 | 24.2601 | 17.3648 |
| 10 | 15.5882 | 8.9358 | 5.8042 | 3.5789 | 57.3891 | 30.6474 | 21.9459 | 15.7276 |
| 20 | 0 | 6.6546 | 3.7940 | 2.4532 | 1.5062 | 26.1201 | 13.5473 | 9.6064 | 6.8244 |
| 0.5 | 4.3168 | 2.4607 | 1.5908 | 0.9766 | 16.9785 | 8.7970 | 6.2363 | 4.4291 |
| 1 | 3.3203 | 1.8925 | 1.2234 | 0.7510 | 13.0709 | 6.7692 | 4.7982 | 3.4074 |
| 2 | 2.5907 | 1.4766 | 0.9546 | 0.5860 | 10.1967 | 5.2811 | 3.7435 | 2.6585 |
| 5 | 2.1884 | 1.2476 | 0.8067 | 0.4953 | 8.5855 | 4.4534 | 3.1582 | 2.2438 |
| 10 | 1.9917 | 1.1355 | 0.7344 | 0.4510 | 7.7944 | 4.0473 | 2.8713 | 2.0406 |
| 50 | 0 | 0.4282 | 0.2437 | 0.1575 | 0.0965 | 1.7075 | 0.8770 | 0.6219 | 0.4404 |
| 0.5 | 0.2776 | 0.1580 | 0.1021 | 0.0625 | 1.1074 | 0.5685 | 0.4032 | 0.2855 |
| 1 | 0.2135 | 0.1215 | 0.0785 | 0.0481 | 0.8516 | 0.4371 | 0.3101 | 0.2195 |
| 2 | 0.1666 | 0.0948 | 0.0613 | 0.0375 | 0.6645 | 0.3411 | 0.2419 | 0.1713 |
| 5 | 0.1408 | 0.0801 | 0.0518 | 0.0317 | 0.5616 | 0.2883 | 0.2045 | 0.1448 |
| 10 | 0.1283 | 0.0730 | 0.0472 | 0.0289 | 0.5112 | 0.2625 | 0.1863 | 0.1318 |
| 100 | 0 | 0.0536 | 0.0305 | 0.0197 | 0.0121 | 0.2141 | 0.1095 | 0.0778 | 0.0551 |
| 0.5 | 0.0347 | 0.0197 | 0.0128 | 0.0078 | 0.1388 | 0.0710 | 0.0504 | 0.0357 |
| 1 | 0.0267 | 0.0152 | 0.0098 | 0.0060 | 0.1067 | 0.0546 | 0.0387 | 0.0274 |
| 2 | 0.0208 | 0.0118 | 0.0076 | 0.0047 | 0.0833 | 0.0426 | 0.0302 | 0.0214 |
| 5 | 0.0176 | 0.0100 | 0.0065 | 0.0040 | 0.0704 | 0.0360 | 0.0256 | 0.0181 |
| 10 | 0.0160 | 0.0091 | 0.0059 | 0.0036 | 0.0641 | 0.0328 | 0.0233 | 0.0165 |