Probabilistic Modeling of Chloride Penetration with Respect to Concrete Heterogeneity and Epoxy-Coating on the Reinforcement
Abstract
1. Introduction
2. Probabilistic 2D Model for Chloride Ingress Heterogeneous RC Structures
- C(x,t) = the chloride ion concentration (%) at a distance x from the surface of concrete in time t;
- Dc = effective diffusion coefficient (m2/s), which characterizes the concrete ability to withstand the penetration of chlorides.
- Dc,28 = chloride diffusion coefficient (m2/s) measured at selected concrete age;
- t28 = age of concrete measured at period of 28 days (years);
- t = concrete age (years);
- m = aging factor.
- C(h) = covariance function of single variable h;
- lc = correlation length;
- h = single variable ;
- xi, xj = location vectors of point i and point j;
- = distribution variance.
- = the cth realization of the random field ;
- K = number of eigenmodes summed up;
- λ = eigenvalues of covariance matrix of the grid nodes;
- ψ = eigenvectors of covariance matrix of the grid nodes;
- Cxg = covariance matrix between the grid nodes and the center of the facet located at x.
3. Numerical Examples
4. Results
4.1. Deterministic Approach—Distribution of the Diffusion Coefficient
4.2. Deterministic Approach—Distribution of Chloride Concentration
4.3. Deterministic Approach—Chloride Concentration over the Time
4.4. Probabilistic Solution
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hooton, R.D.; Thomas, M.D.A.; Standish, K. Prediction of Chloride Penetration in Concrete; The National Academies of Sciences, Engineering, and Medicine: Washington, DC, USA, 2001; p. 412. [Google Scholar]
- Marsavina, L.; Audenaert, K.; De Schutter, G.; Faur, N.; Marsavina, D. Experimental and numerical determination of the chloride penetration in cracked concrete. Constr. Build. Mater. 2009, 23, 264–274. [Google Scholar] [CrossRef]
- Vořechovská, D.; Podroužek, J.; Chromá, M.; Rovnaníková, P.; Teplý, B. Modeling of chloride concentration effect on reinforcement corrosion. Comput. Civ. Infrastruct. Eng. 2009, 24, 446–458. [Google Scholar] [CrossRef]
- Konečný, P.; Brožovský, J.; Ghosh, P. Evaluation of Chloride Influence on the Cracking in Reinforced Concrete Using Korozeeneck Software. Trans. VŠB Tech. Univ. Ostrava Civ. Eng. Ser. 2011, 11, 1–7. [Google Scholar] [CrossRef]
- Szweda, Z.; Zybura, A. Theoretical model and experimental tests on chloride diffusion and migration processes in concrete. Procedia Eng. 2013, 57, 1121–1130. [Google Scholar]
- Stewart, M.G.; Rosowsky, D.V. Time-dependent reliability of deteriorating reinforced concrete bridge decks. Struct. Saf. 1998, 20, 91–109. [Google Scholar] [CrossRef]
- Tikalsky, P.J.; Pustka, D.; Marek, P. Statistical variations in chloride diffusion in concrete bridges. ACI Struct. J. 2005, 102, 481–486. [Google Scholar]
- Teplý, B.; Vořechovská, D. Reinforcement corrosion: Limit states, reliability and modelling. J. Adv. Concr. Technol. 2012, 10, 353–362. [Google Scholar]
- Ghosh, P.; Konečný, P.; Lehner, P.; Tikalsky, P.J.P.J. Probabilistic time-dependent sensitivity analysis of HPC bridge deck exposed to chlorides. Comput. Concr. 2017, 19, 305–313. [Google Scholar] [CrossRef]
- Lehner, P.; Konečný, P.; Ghosh, P.; Tran, Q. Numerical analysis of chloride diffusion considering time-dependent diffusion coefficient. Int. J. Math. Comput. Simul. 2014, 8, 103–106. [Google Scholar]
- Konečný, P.; Lehner, P. Durability assessment of concrete bridge deck considering waterproof membrane and epoxy-coated reinforcement. Perspect. Sci. 2016, 7, 222–227. [Google Scholar] [CrossRef][Green Version]
- Herrmann, H.J.; Hansen, A.; Roux, S. Fracture of disordered, elastic lattices in two dimensions. Phys. Rev. B 1989, 39, 637–648. [Google Scholar] [CrossRef] [PubMed]
- Cusatis, G.; Bažant, Z.; Cedolin, L. Confinement-Shear Lattice Model for Concrete Damage in Tension and Compression: I. Theory. J. Eng. Mech. 2003, 129, 1449–1458. [Google Scholar] [CrossRef]
- Cusatis, G.; Cedolin, L. Two-scale study of concrete fracturing behavior. Eng. Fract. Mech. 2007, 74, 3–17. [Google Scholar] [CrossRef]
- Cusatis, G.; Pelessone, D.; Mencarelli, A. Lattice Discrete Particle Model (LDPM) for failure behavior of concrete. I: Theory. Cem. Concr. Compos. 2011, 33, 881–890. [Google Scholar] [CrossRef]
- Grassl, P.; Bažant, Z.P. Random Lattice-Particle Simulation of Statistical Size Effect in Quasi-Brittle Structures Failing at Crack Initiation. J. Eng. Mech. 2009, 135, 85–92. [Google Scholar] [CrossRef][Green Version]
- Eliáš, J.; Vořechovský, M.; Skoček, J.; Bažant, Z.P. Stochastic discrete meso-scale simulations of concrete fracture: Comparison to experimental data. Eng. Fract. Mech. 2015, 135, 1–16. [Google Scholar] [CrossRef]
- Stanish, K.D.; Hooton, R.D.; Thomas, M.D.A. AASHTO T259 Standard method of test for resistance of concrete to chloride ion penetration. In Testing the Chloride Penetration Resistance of Concrete: A Literature Review; American Association of State Highway and Transportation Officials: Washington, DC, USA, 2012; Volume 2. [Google Scholar]
- Beckhoff, B.; Kanngießer, B.; Langhoff, N.; Wedell, R.; Wolff, H. Handbook of Practical X-Ray Fluorescence Analysis; Springer: Berlin/Heidelberg, Germany, 2006; ISBN 978-3-540-28603-5. [Google Scholar]
- Khanzadeh Moradllo, M.; Sudbrink, B.; Hu, Q.; Aboustait, M.; Tabb, B.; Ley, M.T.; Davis, J.M. Using micro X-ray fluorescence to image chloride profiles in concrete. Cem. Concr. Res. 2017, 92, 128–141. [Google Scholar] [CrossRef]
- Gottlieb, C.; Millar, S.; Günther, T.; Wilsch, G. Revealing hidden spectral information of chlorine and sulfur in data of a mobile Laser-induced Breakdown Spectroscopy system using chemometrics. Spectrochim. Acta Part B At. Spectrosc. 2017, 132, 43–49. [Google Scholar] [CrossRef]
- Millar, S.; Wilsch, G.; Eichler, T.; Gottlieb, C.; Wiggenhauser, H. Laser Induced Breakdown Spectroscopy (LIBS) in civil engineering — Innovative analysis of building materials. Beton Stahlbetonbau 2015, 110, 501–510. [Google Scholar] [CrossRef]
- Jiang, W.; Shen, X.; Xia, J.; Mao, L.; Yang, J.; Liu, Q. A numerical study on chloride diffusion in freeze-thaw affected concrete. Constr. Build. Mater. 2018, 179, 553–565. [Google Scholar] [CrossRef]
- Li, C.; Der Kiureghian, A. Optimal Discretization of Random Fields. J. Eng. Mech. 1993, 119, 1136–1154. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Vořechovský, M.; Novák, D. Asymptotic Prediction of Energetic-Statistical Size Effect from Deterministic Finite-Element Solutions. J. Eng. Mech. 2007, 133, 153–162. [Google Scholar] [CrossRef]
- Roubin, E.; Colliat, J.B.; Benkemoun, N. Meso-scale modeling of concrete: A morphological description based on excursion sets of Random Fields. Comput. Mater. Sci. 2015, 102, 183–195. [Google Scholar] [CrossRef]
- Vořechovský, M.; Sadílek, V. Computational modeling of size effects in concrete specimens under uniaxial tension. Int. J. Fract. 2008, 154, 27–49. [Google Scholar] [CrossRef]
- Grassl, P.; Grégoire, D.; Rojas Solano, L.; Pijaudier-Cabot, G. Meso-scale modelling of the size effect on the fracture process zone of concrete. Int. J. Solids Struct. 2012, 49, 1818–1827. [Google Scholar] [CrossRef]
- Syroka-Korol, E.; Tejchman, J.; Mróz, Z. FE investigations of the effect of fluctuating local tensile strength on coupled energetic-statistical size effect in concrete beams. Eng. Struct. 2015, 103, 239–259. [Google Scholar] [CrossRef]
- Vořechovský, M. Interplay of size effects in concrete specimens under tension studied via computational stochastic fracture mechanics. Int. J. Solids Struct. 2007, 44, 2715–2731. [Google Scholar] [CrossRef]
- Weyers, R.E.; Pyc, W.; Sprinkel, M.M. Estimating the service life of epoxy-coated reinforcing steel. ACI Mater. J. 1998, 95, 546–557. [Google Scholar]
- Konecny, P.; Lehner, P. Effect of cracking and randomness of inputs on corrosion initiation of reinforced concrete bridge decks exposed to chlorides. Frat. Integrita Strutt. 2017, 39, 29–37. [Google Scholar] [CrossRef]
- Le, T.D.; Lehner, P.; Konečný, P. Advanced Model of Chloride Penetration Considering Concrete Heterogeneity. Procedia Struct. Integr. 2018, 13, 1702–1707. [Google Scholar] [CrossRef]
- Pack, S.W.; Jung, M.S.; Song, H.W.; Kim, S.H.; Ann, K.Y. Prediction of time dependent chloride transport in concrete structures exposed to a marine environment. Cem. Concr. Res. 2010, 40, 302–312. [Google Scholar] [CrossRef]
- Thomas, M.D.A.; Bamforth, P.B. Modelling chloride diffusion in concrete effect of fly ash and slag. Cem. Concr. Res. 1999, 29, 487–495. [Google Scholar] [CrossRef]
- Roubin, E. Meso-Scale {FE} and Morphological Modeling of Heterogeneous Media: Application to Cementitious Materials. Ph.D. Thesis, École Normale Supérieure Paris-Saclay, Cachan, France, October 2013. [Google Scholar]
- Ghosh, P.; Tran, Q. Correlation Between Bulk and Surface Resistivity of Concrete. Int. J. Concr. Struct. Mater. 2015, 9, 119–132. [Google Scholar] [CrossRef]
- Kaděrová, J. Probabilistic Discrete Model of Concrete Fracturing. Ph.D. Thesis, Brno University of Technology, Brno, Czech Republic, 2018. [Google Scholar]
- Scannell, W.T.; Sohanghpurwala, A.A. Verification of Effectiveness of Epoxy-Coated Rebars; Concorr Inc.: Sterling, VA, USA, 1998; pp. 5–96. [Google Scholar]
- Darwin, D.; Browning, J.; O’Reilly, M.; Xing, L.; Ji, J. Critical chloride corrosion threshold of galvanized reinforcing bars. ACI Mater. J. 2009, 106, 176–183. [Google Scholar]
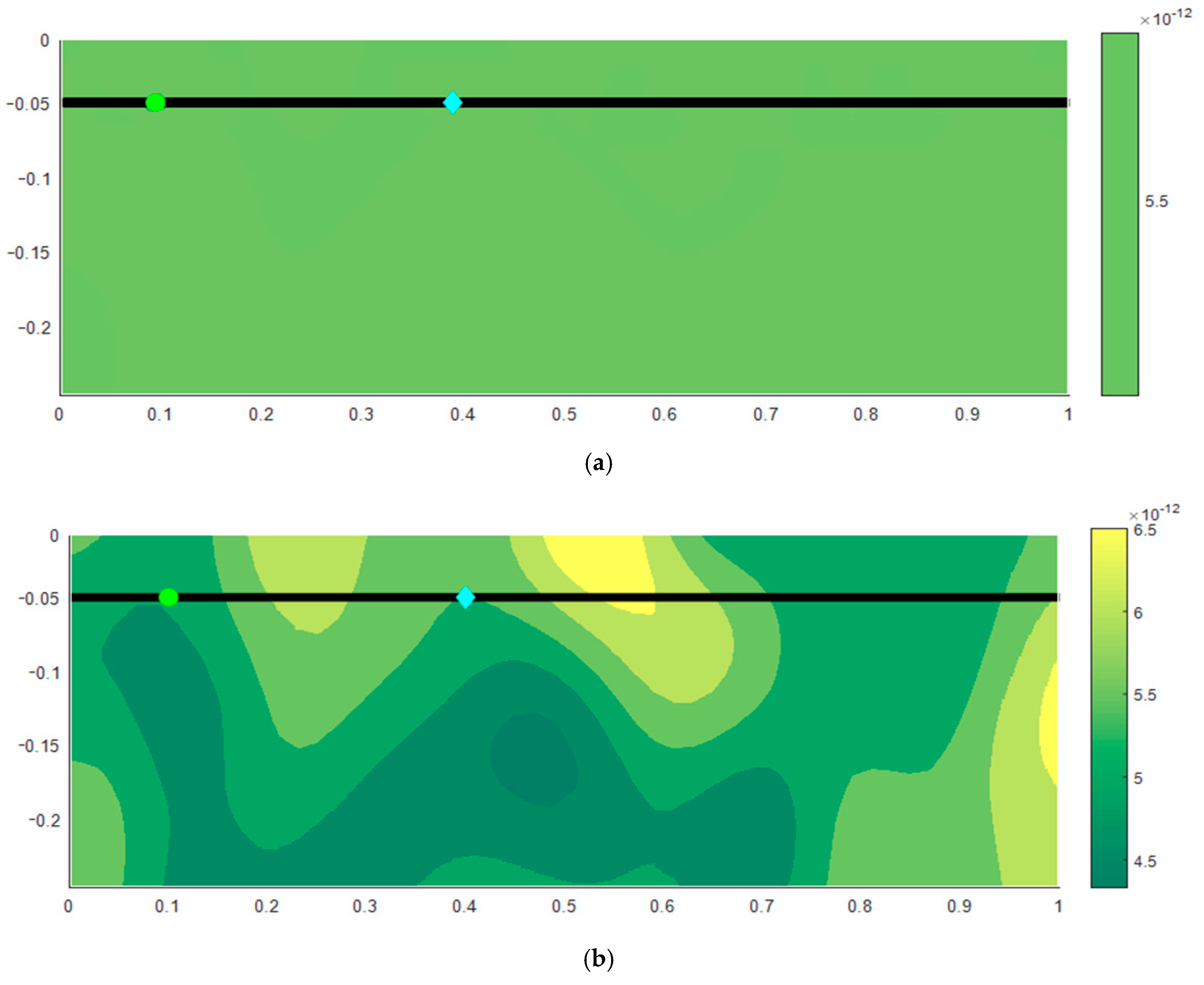


| Parameter | Unit | Deterministic Approach | Probabilistic Approach | |
|---|---|---|---|---|
| Range/Value | Probability Density Function | |||
| Diffusion coefficient, Dc,28 | × 10−12 m²/s | 5.585 | 4.49 ÷ 6.67 | Constant [37] |
| Aging factor, m | – | 0.284 | 0.284 | Constant [37] |
| Variation coefficient, cvRV | – | 0.14 | 0.14 | Constant [38] |
| Width of investigated cross-section, b | m | 1.0 | 1.0 | Constant [32] |
| Height of investigated cross-section surface, h | m | 0.23 | 0.23 | Constant [32] |
| Size of finite element | m | 0.02 × 0.01 | 0.02 × 0.01 | Constant |
| Depth of reinforcement, x | m | 0.05 | 0.04 ÷ 0.11 | Histogram [39] |
| Chloride threshold for corrosion initiation, Cth | % weight of cement | 0.2 | 0.09 ÷ 0.51 | Histogram [40] |
| Concentration of chloride at the surface, C0 | % weight of cement | 0.6 | 0.21 ÷ 1.63 | Histogram [32] |
| Initial concentration of chloride in the cross section, Cb | % weight of cement | 0 | 0 | Constant |
| Frequency of defects in the reinforcement coating, Mashn | m-1 | 1 | 0 ÷ 10 | Histogram [32] |
| Relative spacing of the first defect in the reinforcement coating, Mashi | m | 0.2 | 0 ÷ 1.0 | Uniform distribution |
| Correlation length, lc | m | 0.1 | 0.1 | Constant [28,38] |
| Monitored life span, t | years | 100 | 100 | Constant |
| Number of simulations | – | 1 | 10,000 | Constant |
| Model | Type | 10 Years | 100 Years |
|---|---|---|---|
| Homogeneous | Black bar as well as epoxy-coated bar | 0.075 | 0.316 |
| Heterogeneous | Maximum (black bar) | 0.100 | 0.340 |
| Minimum (black bar) | 0.064 | 0.312 | |
| Mean (black bar) | 0.081 | 0.326 | |
| Epo1 | 0.079 | 0.323 | |
| Epo2 | 0.099 | 0.339 |
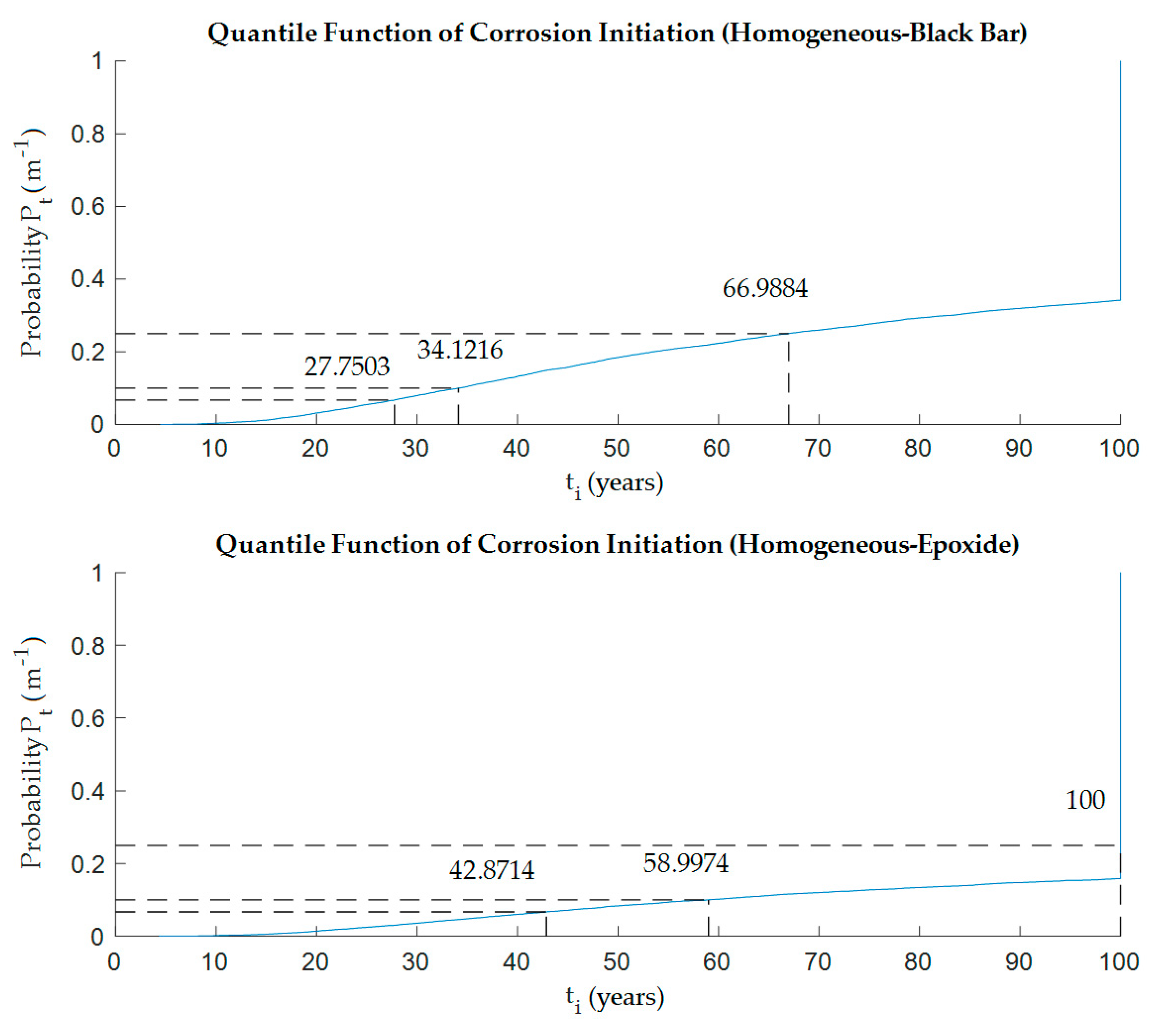
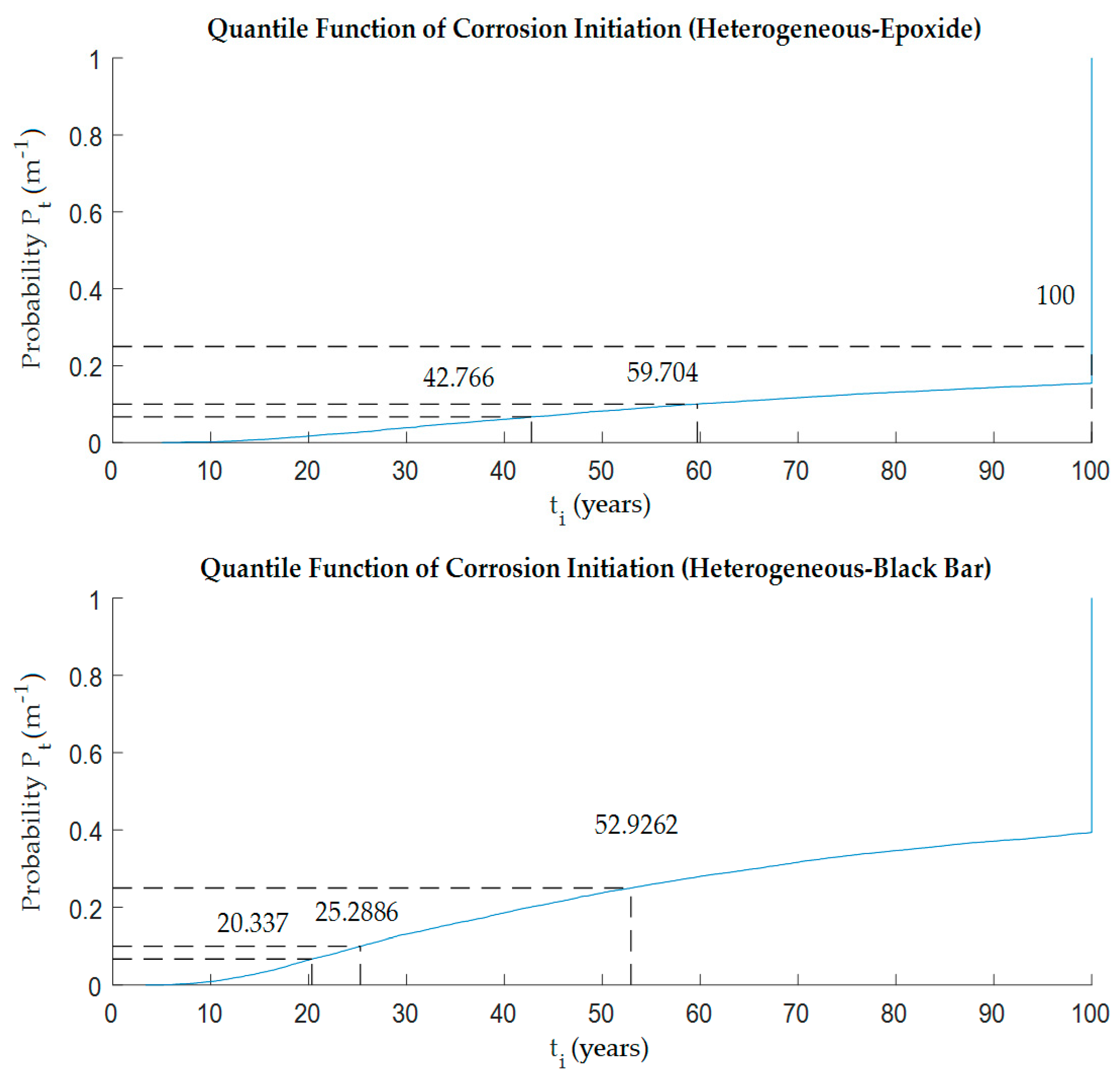
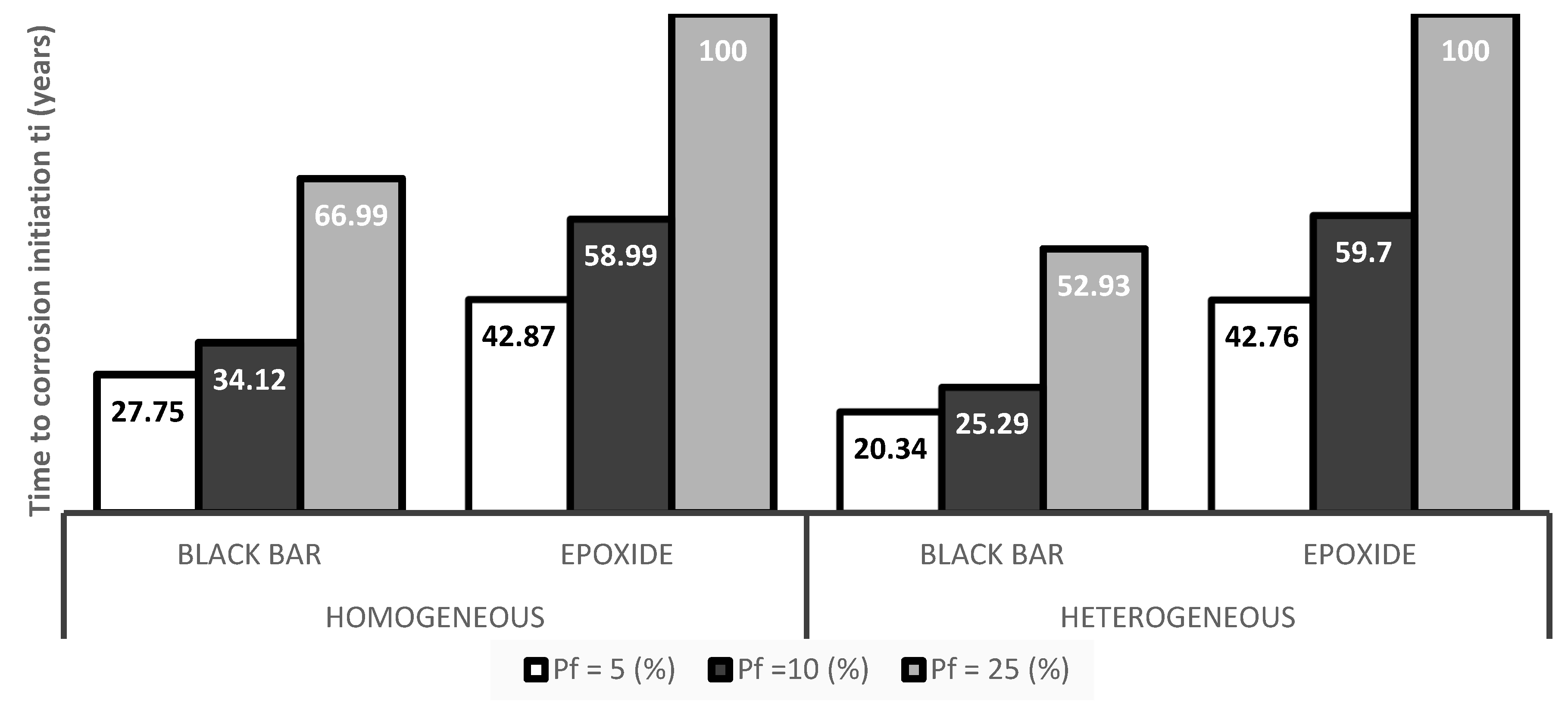
| Model | Type | Probability of Corrosion Initiation Pf (%) | ||
|---|---|---|---|---|
| 5 | 10 | 25 | ||
| Homogeneous | Black bar | 27.75 | 34.12 | 66.99 |
| Epoxide | 42.87 | 58.99 | 100.00 | |
| Heterogeneous | Black bar | 20.34 | 25.29 | 52.93 |
| Epoxide | 42.76 | 59.70 | 100.00 | |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Le, T.D.; Lehner, P.; Konečný, P. Probabilistic Modeling of Chloride Penetration with Respect to Concrete Heterogeneity and Epoxy-Coating on the Reinforcement. Materials 2019, 12, 4068. https://doi.org/10.3390/ma12244068
Le TD, Lehner P, Konečný P. Probabilistic Modeling of Chloride Penetration with Respect to Concrete Heterogeneity and Epoxy-Coating on the Reinforcement. Materials. 2019; 12(24):4068. https://doi.org/10.3390/ma12244068
Chicago/Turabian StyleLe, Tuan Duc, Petr Lehner, and Petr Konečný. 2019. "Probabilistic Modeling of Chloride Penetration with Respect to Concrete Heterogeneity and Epoxy-Coating on the Reinforcement" Materials 12, no. 24: 4068. https://doi.org/10.3390/ma12244068
APA StyleLe, T. D., Lehner, P., & Konečný, P. (2019). Probabilistic Modeling of Chloride Penetration with Respect to Concrete Heterogeneity and Epoxy-Coating on the Reinforcement. Materials, 12(24), 4068. https://doi.org/10.3390/ma12244068






