A Review of the Characteristics, Synthesis, and Thermodynamics of Type-II Weyl Semimetal WTe2
Abstract
1. Introduction
2. Structure and Characteristics of WTe2
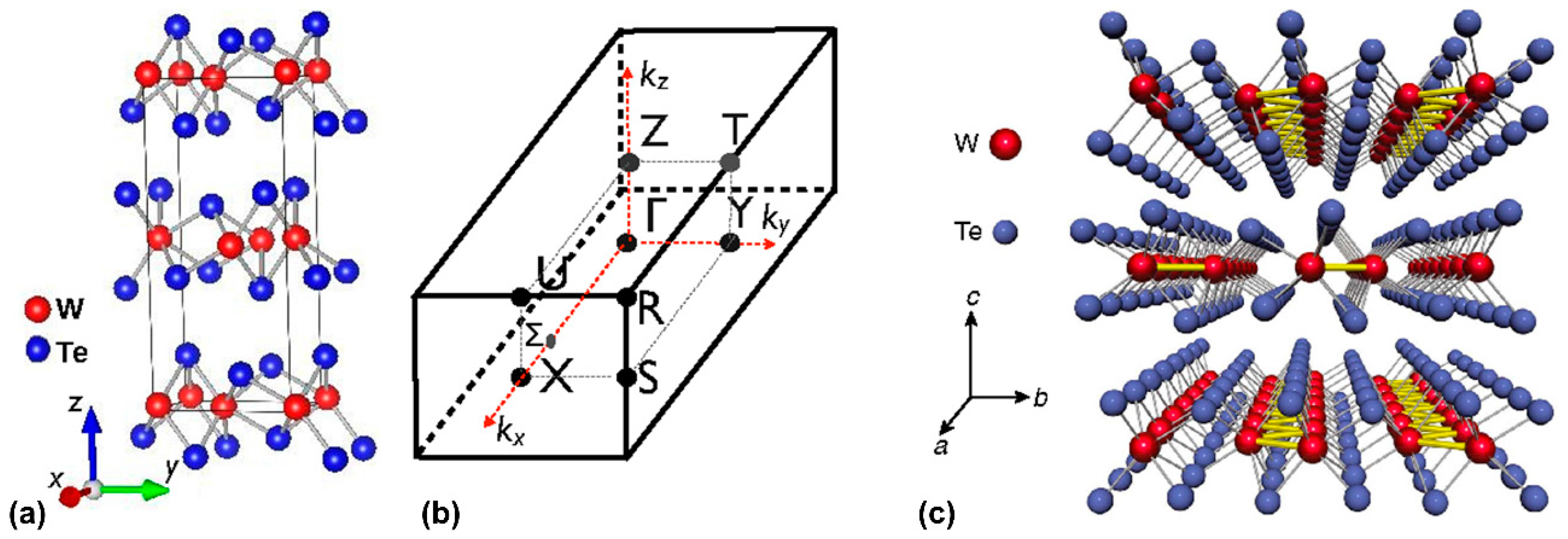
2.1. Crystal Structure and Surface Structure
2.2. Classification
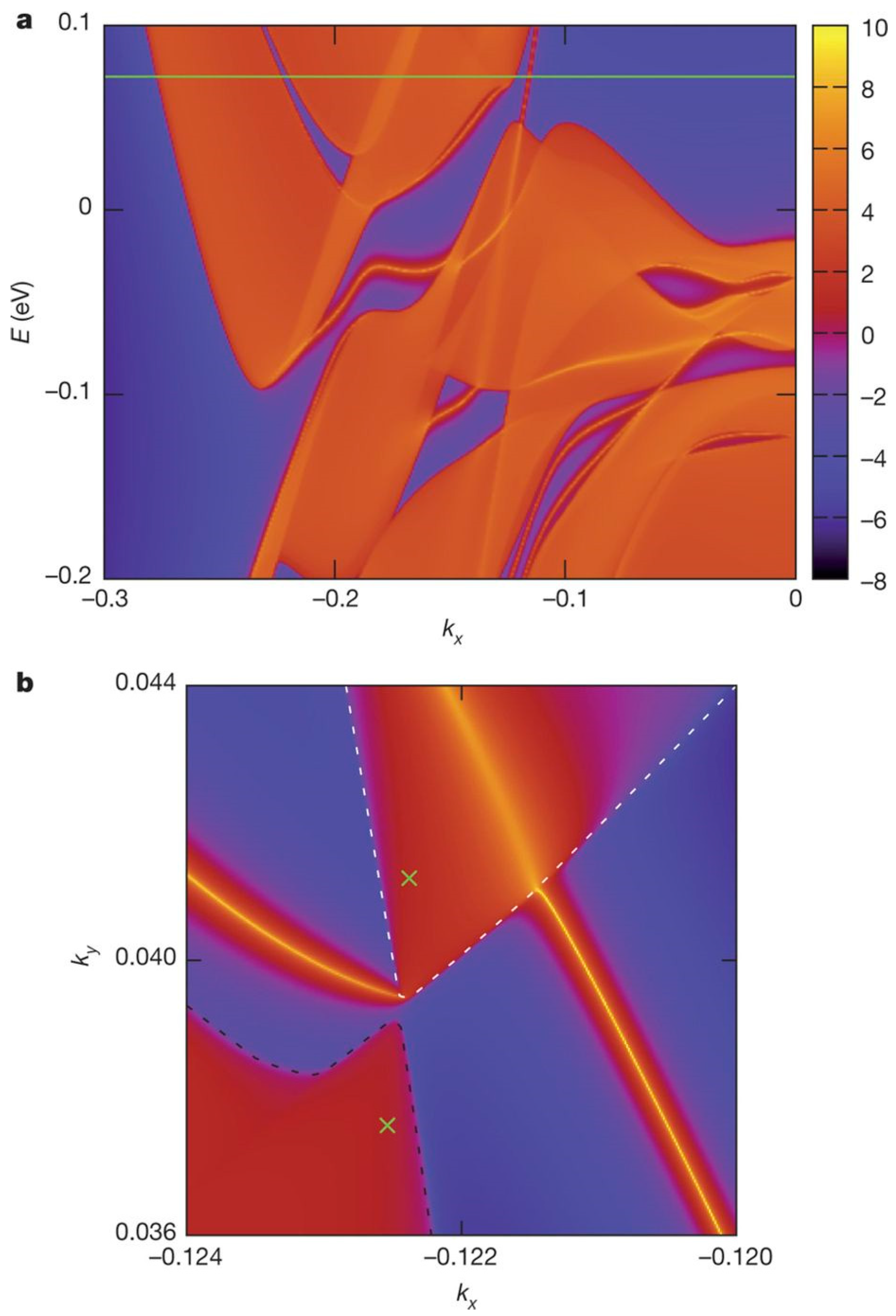
2.3. Novel Weyl Fermions, Fermi Arcs, and Surface State
2.4. Chiral Anomaly and Non-Saturable Large MR
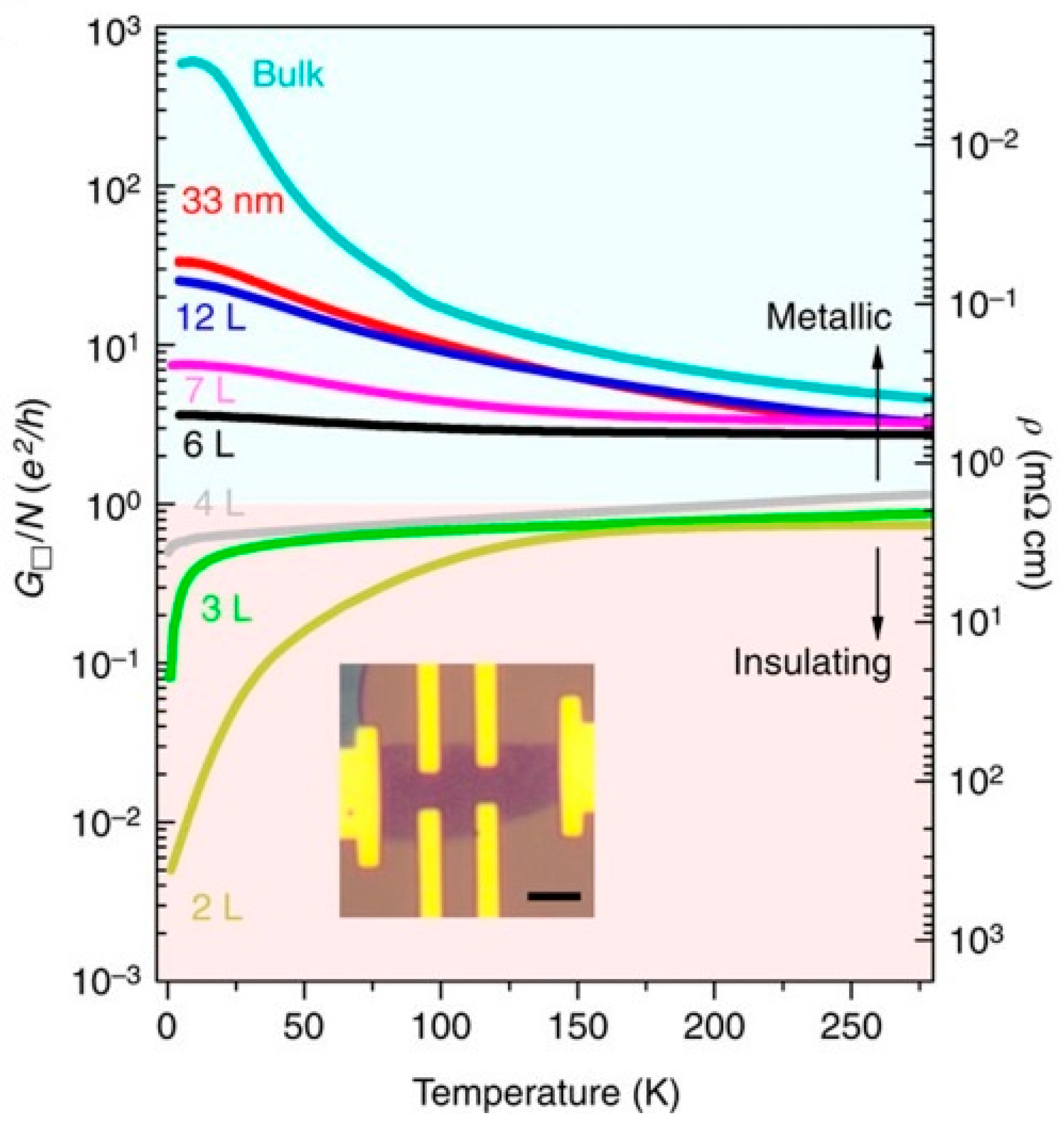
2.5. Metal–Insulator Transitions
2.6. Superconductivity
3. Preparation of WTe2
3.1. Solid-State Reaction
3.2. Atmospheric Chemical Vapor Reaction
3.3. Chemical Vapor Deposition (CVD)
3.4. Solution Synthesis
3.5. Other Synthesis Methods
4. Studies of Thermodynamics
4.1. Numerical Works
4.1.1. Anisotropic Ultra-Low Thermal Conductivity
4.1.2. Temperature-Dependent Thermal Conductivity
4.1.3. Temperature-Dependent Thermal Expansion and Mechanical Properties
4.1.4. Scale-Dependent Thermal Conductivity
4.1.5. Specific Heat and Debye Temperature
4.2. Experimental Works
5. Summary and Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Xu, S.Y.; Belopolski, I.; Alidoust, N.; Neupane, M.; Bian, G.; Zhang, C.L.; Sankar, R.; Chang, G.Q.; Yuan, Z.J.; Lee, C.C.; et al. Discovery of a Weyl fermion semimetal and topological Fermi arcs. Science 2015, 349, 613–617. [Google Scholar] [CrossRef] [PubMed]
- Deng, K.; Wan, G.L.; Deng, P.; Zhang, K.N.; Ding, S.J.; Wang, E.Y.; Yan, M.Z.; Huang, H.Q.; Zhang, H.Y.; Xu, Z.L.; et al. Experimental observation of topological Fermi arcs in type-II Weyl semimetal MoTe2. Nat. Phys. 2016, 12, 1105–1111. [Google Scholar] [CrossRef]
- Wu, Y.; Mou, D.X.; Jo, N.H.; Sun, K.W.; Huang, L.N.; Bud’ko, S.L.; Canfield, P.C.; Kaminski, A. Observation of Fermi arcs in the type-II Weyl semimetal candidate WTe2. Phys. Rev. B 2016, 94, 121113. [Google Scholar] [CrossRef]
- Huang, L.; McCormick, T.M.; Ochi, M.; Zhao, Z.Y.; Suzuki, M.T.; Arita, R.; Wu, Y.; Mou, D.X.; Cao, H.B.; Yan, J.Q.; et al. Spectroscopic evidence for a type-II Weyl semimetallic state in MoTe2. Nat. Mater. 2016, 15, 1155–1160. [Google Scholar] [CrossRef] [PubMed]
- Peng, L.; Yuan, Y.; Li, G.; Yang, X.; Xian, J.; Yi, C.; Shi, Y.; Fu, Y. Observation of topological states residing at step edges of WTe2. Nat. Commun. 2017, 8, 659. [Google Scholar] [CrossRef] [PubMed]
- Weng, H.M.; Fang, C.; Fang, Z.; Bernevig, B.A.; Dai, X. Weyl semimetal phase in non-centrosymmetric transition-metal monophosphides. Phys. Rev. X 2015, 5, 011029. [Google Scholar]
- Mleczko, M.J.; Xu, R.L.; Okabe, K.; Kuo, H.-H.; Fisher, I.R.; Wong, H.S.P.; Nishi, Y.; Pop, E. High current density and low thermal conductivity of atomically thin semimetallic WTe2. ACS Nano 2016, 10, 7507–7514. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.L.; Chen, Y.N.; Han, Z.; Li, W. Strong anisotropic thermal conductivity of monolayer WTe2. 2D Mater. 2016, 3, 045010. [Google Scholar] [CrossRef]
- MacNeill, D.; Stiehl, G.M.; Guimarães, M.H.D.; Reynolds, N.D.; Buhrman, R.A.; Ralph, D.C. Thickness dependence of spin-orbit torques generated by WTe2. Phys. Rev. B 2017, 96, 054450. [Google Scholar] [CrossRef]
- Wang, Y.J.; Liu, E.F.; Liu, H.M.; Pan, Y.M.; Zhang, L.Q.; Zeng, J.W.; Fu, Y.J.; Wang, M.; Xu, K.; Huang, Z.; et al. Gate-tunable negative longitudinal magnetoresistance in the predicted type-II Weyl semimetal WTe2. Nat. Commun. 2016, 7, 13142. [Google Scholar] [CrossRef] [PubMed]
- Lu, N.; Zhang, C.; Lee, C.; Oviedo, J.P.; Nguyen, M.A.T.; Peng, X.; Wallace, R.M.; Mallouk, T.E.; Robinson, J.A.; Wang, J.; et al. Atomic and electronic structures of WTe2 probed by high resolution electron microscopy and ab initio calculations. J. Phys. Chem. C 2016, 120, 8364–8369. [Google Scholar] [CrossRef]
- Soluyanov, A.A.; Gresch, D.; Wang, Z.J.; Wu, Q.S.; Troyer, M.; Dai, X.; Bernevig, B.A. Type-II Weyl semimetals. Nature 2015, 527, 495–498. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Jhon, Y.I.; Park, J.; Kim, J.H.; Lee, S.; Jhon, Y.M. Anomalous lattice dynamics of mono-, bi-, and tri-layer WTe2. arXiv 2015, arXiv:1508.03244. [Google Scholar]
- Dawson, W.G.; Bullett, D.W. Electronic structure and crystallography of MoTe2 and WTe2. J. Phys. C Solid State Phys. 1987, 20, 6159–6174. [Google Scholar] [CrossRef]
- Augustin, J.; Eyert, V.; Böker, T.; Frentrup, W.; Dwelk, H.; Janowitz, C.; Manzke, R. Electronic band structure of the layered compound Td-WTe2. Phys. Rev. B 2000, 62, 10812–10823. [Google Scholar] [CrossRef]
- Crossley, A.; Myhra, S.; Sofield, C.J. STM analysis of WTe2 surfaces—Correlation with crystal and electronic structures. Surf. Sci. 1994, 318, 39–45. [Google Scholar] [CrossRef]
- Hla, S.W.; Marinković, V.; Prodan, A.; Muševič, I. STM/AFM investigations of β-MoTe2, α-MoTe2 and WTe2. Surf. Sci. 1996, 352–354, 105–111. [Google Scholar] [CrossRef]
- Kawahara, K.; Ni, Z.; Arafune, R.; Shirasawa, T.; Lin, C.; Minamitani, E.; Watanabe, S.; Kawai, M.; Takagi, N. Surface structure of novel semimetal WTe2. Appl. Phys. Express 2017, 10, 045702. [Google Scholar] [CrossRef]
- Song, Y.; Wang, X.; Mi, W. Spin splitting and reemergence of charge compensation in monolayer WTe2 by 3D transition-metal adsorption. Phys. Chem. Chem. Phys. 2017, 19, 7721–7727. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Jo, N.H.; Mou, D.; Huang, L.; Bud’ko, S.L.; Canfield, P.C.; Kaminski, A. Three-dimensionality of the bulk electronic structure in WTe2. Phys. Rev. B 2017, 95, 195138. [Google Scholar] [CrossRef]
- Di Sante, D.; Das, P.K.; Bigi, C.; Ergönenc, Z.; Gürtler, N.; Krieger, J.A.; Schmitt, T.; Ali, M.N.; Rossi, G.; Thomale, R.; et al. Three-dimensional electronic structure of the type-II weyl semimetal WTe2. Phys. Rev. Lett. 2017, 119, 026403. [Google Scholar] [CrossRef] [PubMed]
- Bruno, F.Y.; Tamai, A.; Wu, Q.S.; Cucchi, I.; Barreteau, C.; de la Torre, A.; McKeown Walker, S.; Riccò, S.; Wang, Z.; Kim, T.K.; et al. Observation of large topologically trivial Fermi arcs in the candidate type-II Weyl semimetal WTe2. Phys. Rev. B 2016, 94, 121112. [Google Scholar] [CrossRef]
- Sánchez-Barriga, J.; Vergniory, M.G.; Evtushinsky, D.; Aguilera, I.; Varykhalov, A.; Blügel, S.; Rader, O. Surface Fermi arc connectivity in the type-II Weyl semimetal candidate WTe2. Phys. Rev. B 2016, 94, 161401. [Google Scholar] [CrossRef]
- Feng, B.; Chan, Y.-H.; Feng, Y.; Liu, R.-Y.; Chou, M.-Y.; Kuroda, K.; Yaji, K.; Harasawa, A.; Moras, P.; Barinov, A.; et al. Spin texture in type-II Weyl semimetal WTe2. Phys. Rev. B 2016, 94, 195134. [Google Scholar] [CrossRef]
- Lin, C.-L.; Arafune, R.; Liu, R.-Y.; Yoshimura, M.; Feng, B.; Kawahara, K.; Ni, Z.; Minamitani, E.; Watanabe, S.; Shi, Y.; et al. Visualizing type-II Weyl points in tungsten ditelluride by quasiparticle interference. ACS Nano 2017, 11, 11459–11465. [Google Scholar] [CrossRef] [PubMed]
- Zhang, W.; Wu, Q.; Zhang, L.; Cheong, S.-W.; Soluyanov, A.A.; Wu, W. Quasiparticle interference of surface states in the type-II Weyl semimetal WTe2. Phys. Rev. B 2017, 96, 165125. [Google Scholar] [CrossRef]
- Zhang, E.; Chen, R.; Huang, C.; Yu, J.; Zhang, K.; Wang, W.; Liu, S.; Ling, J.; Wan, X.; Lu, H.-Z.; et al. Tunable positive to negative magnetoresistance in atomically thin WTe2. Nano Lett. 2017, 17, 878–885. [Google Scholar] [CrossRef] [PubMed]
- Kang, D.; Zhou, Y.; Yi, W.; Yang, C.; Guo, J.; Shi, Y.; Zhang, S.; Wang, Z.; Zhang, C.; Jiang, S.; et al. Superconductivity emerging from a suppressed large magnetoresistant state in tungsten ditelluride. Nat. Commun. 2015, 6, 7804. [Google Scholar] [CrossRef] [PubMed]
- Jyoti, K.; Maitra, T. Electronic structure and Fermi surface topology of WTe2 in a magnetic field. AIP Conf. Proc. 2018, 1953, 110013. [Google Scholar]
- Woods, J.M.; Shen, J.; Kumaravadivel, P.; Pang, Y.; Xie, Y.; Pan, G.A.; Li, M.; Altman, E.I.; Lu, L.; Cha, J.J. Suppression of magnetoresistance in thin WTe2 flakes by surface oxidation. ACS Appl. Mater. Interfaces 2017, 9, 23175–23180. [Google Scholar] [CrossRef] [PubMed]
- Fu, D.; Zhang, B.; Pan, X.; Fei, F.; Chen, Y.; Gao, M.; Wu, S.; He, J.; Bai, Z.; Pan, Y.; et al. Tuning the electrical transport of type-II Weyl semimetal WTe2 nanodevices by Ga+ ion implantation. Sci. Rep. 2017, 7, 12688. [Google Scholar] [CrossRef] [PubMed]
- Pan, X.; Pan, Y.; Jiang, J.; Zuo, H.; Liu, H.; Chen, X.; Wei, Z.; Zhang, S.; Wang, Z.; Wan, X.; et al. Carrier balance and linear magnetoresistance in type-II Weyl semimetal WTe2. Front. Phys. 2017, 12, 127203. [Google Scholar] [CrossRef]
- Fatemi, V.; Gibson, Q.D.; Watanabe, K.; Taniguchi, T.; Cava, R.J.; Jarillo-Herrero, P. Magnetoresistance and quantum oscillations of an electrostatically tuned semimetal-to-metal transition in ultrathin WTe2. Phys. Rev. B 2017, 95, 041410. [Google Scholar] [CrossRef]
- Ali, M.N.; Xiong, J.; Flynn, S.; Tao, J.; Gibson, Q.D.; Schoop, L.M.; Liang, T.; Haldolaarachchige, N.; Hirschberger, M.; Ong, N.P.; et al. Large, non-saturating magnetoresistance in WTe2. Nature 2014, 514, 205–208. [Google Scholar] [CrossRef] [PubMed]
- Caputo, M.; Khalil, L.; Papalazarou, E.; Nilforoushan, N.; Perfetti, L.; Taleb-Ibrahimi, A.; Gibson, Q.D.; Cava, R.J.; Marsi, M. Dynamics of out-of-equilibrium electron and hole pockets in the type-II Weyl semimetal candidate WTe2. Phys. Rev. B 2018, 97, 115115. [Google Scholar] [CrossRef]
- Koichi, N. First-principles simulation on thermoelectric propertiesof transition metal dichalcogenide monolayers. Jpn. J. Appl. Phys. 2018, 57, 06HE04. [Google Scholar]
- Li, J.; Cheng, S.; Liu, Z.; Zhang, W.; Chang, H. Centimeter-scale, large-area, few-Layer 1T’-WTe2 films by chemical vapor deposition and its long-term stability in ambient condition. J. Phys. Chem. C 2018, 122, 7005–7012. [Google Scholar] [CrossRef]
- Wang, L.; Gutiérrez-Lezama, I.; Barreteau, C.; Ubrig, N.; Giannini, E.; Morpurgo, A.F. Tuning magnetotransport in a compensated semimetal at the atomic scale. Nat. Commun. 2015, 6, 8892. [Google Scholar] [CrossRef] [PubMed]
- Chan, Y.T.; Alireza, P.L.; Yip, K.Y.; Niu, Q.; Lai, K.T.; Goh, S.K. Nearly isotropic superconductivity in the layered Weyl semimetal WTe2 at 98.5 kbar. Phys. Rev. B 2017, 96, 180504. [Google Scholar] [CrossRef]
- Asaba, T.; Wang, Y.; Li, G.; Xiang, Z.; Tinsman, C.; Chen, L.; Zhou, S.; Zhao, S.; Laleyan, D.; Li, Y.; et al. Magnetic field enhanced superconductivity in epitaxial thin film WTe2. Sci. Rep.-UK 2018, 8, 6520. [Google Scholar] [CrossRef] [PubMed]
- Xia, J.; Li, D.; Zhou, J.; Yu, P.; Lin, J.; Kuo, J.; Li, H.; Liu, Z.; Yan, J.; Shen, Z. Pressure-induced phase transition in Weyl semimetallic WTe2. Small 2017, 13, 1701887. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.; Jang, H.; Woods, J.M.; Xie, Y.; Kumaravadivel, P.; Pan, G.A.; Liu, J.; Liu, Y.; Cahill, D.G.; Cha, J.J. Direct synthesis of large-scale WTe2 thin films with low thermal conductivity. Adv. Funct. Mater. 2017, 27, 1605928. [Google Scholar] [CrossRef]
- Chen, K.; Chen, Z.; Wan, X.; Zheng, Z.; Xie, F.; Chen, W.; Gui, X.; Chen, H.; Xie, W.; Xu, J. A simple method for synthesis of high-quality millimeter-scale 1T’ transition-metal telluride and near-field nanooptical properties. Adv. Mater. 2017, 29, 1700704. [Google Scholar] [CrossRef] [PubMed]
- Carl, H.N.; William, M.P.; Zhaoli, G.; Hojin, K.; Mehmet, N.; Robert, B.W.; Liang, Z.T.; Youngkuk, K.; Christopher, E.K.; Frank, S.; et al. Large-area synthesis of high-quality monolayer 1T’-WTe2 flakes. 2D Mater. 2017, 4, 021008. [Google Scholar]
- Zhou, J.; Liu, F.; Lin, J.; Huang, X.; Xia, J.; Zhang, B.; Zeng, Q.; Wang, H.; Zhu, C.; Niu, L.; et al. Large-area and high-quality 2D transition metal telluride. Adv. Mater. 2017, 29, 1603471. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Fujisawa, K.; Terrones, M.; Schaak, R.E. Solution synthesis of few-layer WTe2 and MoxW1−xTe2 nanostructures. J. Mater. Chem. C 2017, 5, 11317–11323. [Google Scholar] [CrossRef]
- Giri, A.; Yang, H.; Jang, W.; Kwak, J.; Thiyagarajan, K.; Pal, M.; Lee, D.; Singh, R.; Kim, C.; Cho, K.; et al. Synthesis of atomically thin transition metal ditelluride films by rapid chemical transformation in solution phase. Chem. Mater. 2018, 30, 2463–2473. [Google Scholar] [CrossRef]
- Lee, J.H. Synthesis of Single-Crystalline Tungsten Ditelluride (WTe2) Nanostructures Using Eutectic Metal Alloys. Master’s Thesis, Ulsan National Institute of Science and Technology, Ulsan, Korea, 2017. [Google Scholar]
- Vijay, D.; Chirag, V.; Pathak, V.M.; Soalanki, G.K.; Patel, K.D. Structural and optical properties of WTe2 single crystals synthesized by DVT technique. AIP Conf. Proc. 2018, 1953, 070020. [Google Scholar]
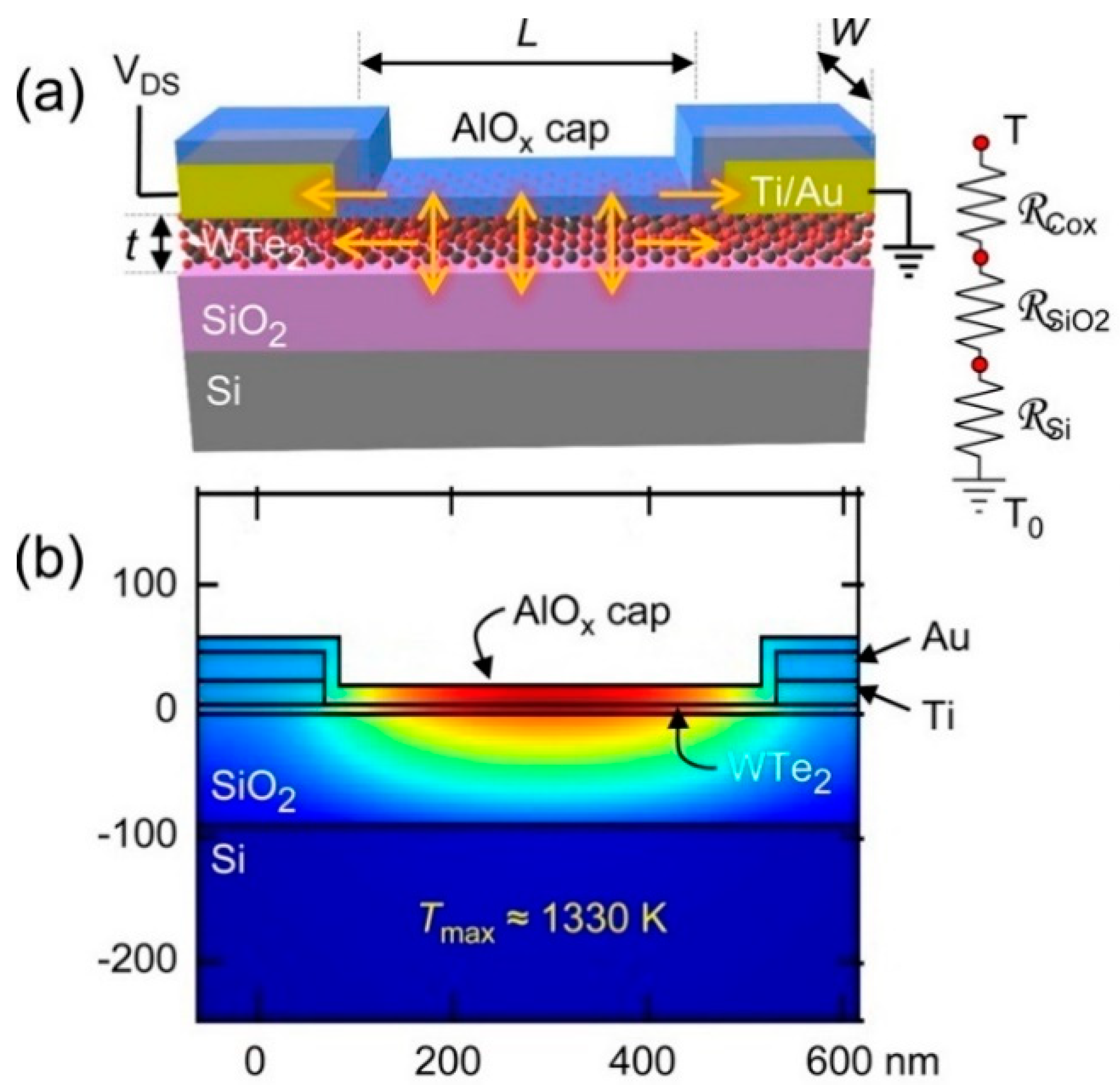
- Xu, R.; Mleczko, M.J.; Bohaichuk, S.; Nishi, Y.; Pop, E. Thermal limitations of two-dimensional semi-metallic WTe2 devices. In Proceedings of the Silicon Nanoelectronics Workshop, Honolulu, HI, USA, 12–13 June 2016; IEEE: Honolulu, HI, USA, 2016; pp. 22–23. [Google Scholar]
- Liu, G.; Sun, H.Y.; Jian, Z.; Li, Q.F.; Wan, X.-G. First-principles study of lattice thermal conductivity of Td-WTe2. New J. Phys. 2016, 18, 033017. [Google Scholar] [CrossRef]
- Sun, H.; Li, Q. Anisotropic phonon transport, thermal expansion and thermomechanics in monolayer Td-WTe2. arXiv 2016, arXiv:1607.03639. [Google Scholar]
- Manoj, K.J.; Anjali, S.; Dattatray, J.L.; Catherine, R.R.; Kanishka, B.; Claudia, F.; Umesh, V.W.; Rao, C.N.R. A combined experimental and theoretical study of the structural, electronic and vibrational properties of bulk and few-layer Td-WTe2. J. Phys. Condens. Matter 2015, 27, 285401. [Google Scholar]
- Chiritescu, C.; Cahill, D.G.; Nguyen, N.; Johnson, D.; Bodapati, A.; Keblinski, P.; Zschack, P. Ultralow thermal conductivity in disordered, layered WSe2 crystals. Science 2007, 315, 351–353. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.F.; Gong, P.L.; Zeng, Z. Correlation between structure, phonon spectra, thermal expansion, and thermomechanics of single-layer MoS2. Phys. Rev. B 2014, 90, 045409. [Google Scholar] [CrossRef]
- Huang, L.F.; Zeng, Z. Lattice dynamics and disorder-induced contraction in functionalized graphene. J. Appl. Phys. 2013, 113, 083524. [Google Scholar] [CrossRef]
- Lv, H.Y.; Lu, W.J.; Shao, D.F.; Liu, Y.; Tan, S.G.; Sun, Y.P. Perfect charge compensation in WTe2 for the extraordinary magnetoresistance: From bulk to monolayer. EPL 2015, 110, 37004. [Google Scholar] [CrossRef]
- Qian, X.; Liu, J.; Fu, L.; Li, J. Quantum spin Hall effect in two-dimensional transition metal dichalcogenides. Science 2014, 346, 1344. [Google Scholar] [CrossRef] [PubMed]
- Mar, A.; Jobic, S.; Ibers, J.A. Metal-metal vs. tellurium-tellurium bonding in WTe2 and its ternary variants TaIrTe4 and NbIrTe4. J. Am. Chem. Soc. 1992, 114, 8963–8971. [Google Scholar] [CrossRef]
- Cao, Y.; Sheremetyeva, N.; Liang, L.; Yuan, H.; Zhong, T.; Meunier, V.; Pan, M. Anomalous vibrational modes in few layer WTe2 revealed by polarized Raman scattering and first-principles calculations. 2D Mater. 2017, 4, 035024. [Google Scholar] [CrossRef]
- Wang, Z.-Y.; Zhou, Y.; Wang, X.; Wang, F.; Sun, Q.; Guo, Z.; Jia, Y. Effects of in-plane stiffness and charge transfer on thermal expansion of monolayer transition metal dichalcogenide. Chin. Phys. B 2015, 24, 026501. [Google Scholar] [CrossRef]
- Callanan, J.E.; Hope, G.A.; Weir, R.D.; Westrum, E.F. Thermodynamic properties of tungsten ditelluride (WTe2) I. The preparation and lowtemperature heat capacity at temperatures from 6 K to 326 K. J. Chem. Thermodyn. 1992, 24, 627–638. [Google Scholar] [CrossRef]
- Kumar, S.; Schwingenschlögl, U. Thermoelectric response of bulk and monolayer MoSe2 and WSe2. Chem. Mater. 2015, 27, 1278–1284. [Google Scholar] [CrossRef]
- Mavrokefalos, A.; Nguyen, N.T.; Pettes, M.T.; Johnson, D.C.; Shi, L. In-plane thermal conductivity of disordered layered WSe2 and (W)x(WSe2)y superlattice films. Appl. Phys. Lett. 2007, 91, 171912. [Google Scholar] [CrossRef]
- Jang, H.; Ryder, C.R.; Wood, J.D.; Hersam, M.C.; Cahill, D.G. 3D anisotropic thermal conductivity of exfoliated rhenium disulfide. Adv. Mater. 2017, 29, 1700650. [Google Scholar] [CrossRef] [PubMed]
- Yalon, E.; McClellan, C.J.; Smithe, K.K.H.; Muñoz Rojo, M.; Xu, R.L.; Suryavanshi, S.V.; Gabourie, A.J.; Neumann, C.M.; Xiong, F.; Farimani, A.B.; et al. Energy dissipation in monolayer MoS2 electronics. Nano Lett. 2017, 17, 3429–3433. [Google Scholar] [CrossRef] [PubMed]
- Lindsay, L.; Broido, D.A. Enhanced thermal conductivity and isotope effect in single-layer hexagonal boron nitride. Phys. Rev. B 2011, 84, 155421. [Google Scholar] [CrossRef]
- Balandin, A.A.; Ghosh, S.; Bao, W.; Calizo, I.; Teweldebrhan, D.; Miao, F.; Lau, C.N. Superior thermal conductivity of single-layer graphene. Nano Lett. 2008, 8, 902–907. [Google Scholar] [CrossRef] [PubMed]



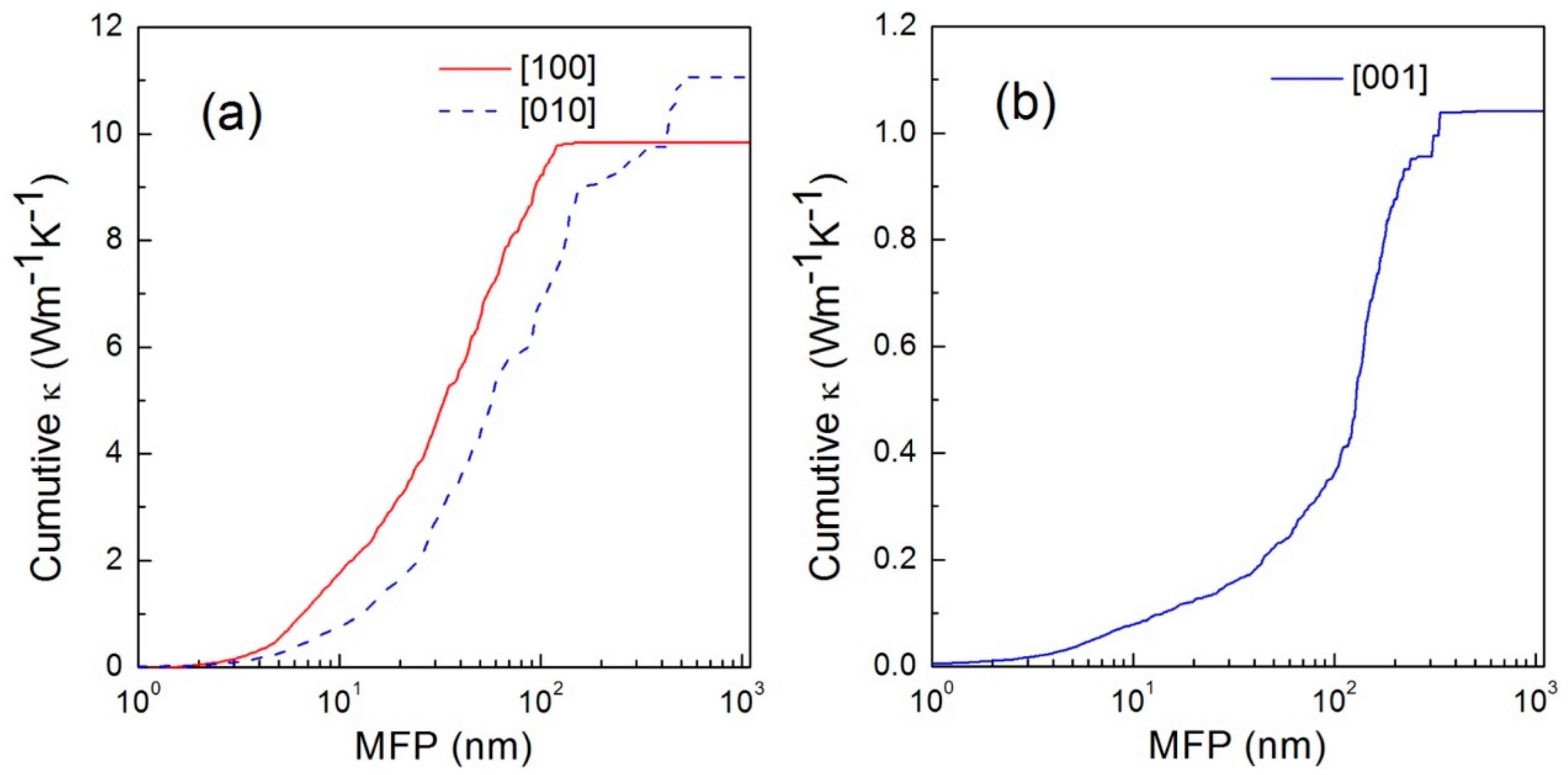
| Material | [100] (W·m−1·K−1) | [010] (W·m−1·K−1) | [001] (W·m−1·K−1) | T (K) | Methods | Ref. |
|---|---|---|---|---|---|---|
| Monolayer WTe2 | 9 | 20 | - | 300 | First-principle | [8] |
| Td-WTe2 | 9.03 | 7.69 | 0.46 | 300 | First-principle | [51] |
| Monolayer Td-WTe2 | 10 | 20 | - | 300 | First-principle | [52] |
| Atomically thin WTe2 | 2.5–3.5 to 9–11 in the [100] direction | 80–300 | Experiment | [7] | ||
| 4–20 layers Td-WTe2 flakes | 3–10 | 80–300 | Experiment and theory | [50] | ||
| Few-layer Td-WTe2 | 0.96–1.06 (total κ), 0.8 (mean κ) | 300–623 | Experiment | [53] | ||
| Polycrystalline WTe2 film | Less than 2 | 0.8 | 300 | Experiment | [42] | |
| Single-crystal WTe2 flake | 15 ± 3 | |||||
| WSe2 (62 nm thick) | 0.048 ([001], the lowest value in solid) | 300 | Experiment and simulation | [54] | ||
| Monolayer WSe2 | 50 | 50 | 6 | 300 | First-principle and theory | [63] |
| Single-crystal WSe2 platelets | 9.7 | 9.7 | 2.09 | 300 | Experiment | [64] |
| ReS2 flakes (60–450 nm) | 70 ± 18 | 50 ± 13 | 0.55 ± 0.07 | 300 | Experiment | [65] |
| MoS2 transistors | 14 ± 4 | 300 | Experiment | [66] | ||
| Single-layer h-BN | 600 | 300 | Numerical solution | [67] | ||
| Single-layer graphene | About (4840 ± 440) to (5300 ± 480) | 300 | Non-contact measurement | [68] | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, W.; Yu, W.; Liu, X.; Wang, Y.; Shi, J. A Review of the Characteristics, Synthesis, and Thermodynamics of Type-II Weyl Semimetal WTe2. Materials 2018, 11, 1185. https://doi.org/10.3390/ma11071185
Tian W, Yu W, Liu X, Wang Y, Shi J. A Review of the Characteristics, Synthesis, and Thermodynamics of Type-II Weyl Semimetal WTe2. Materials. 2018; 11(7):1185. https://doi.org/10.3390/ma11071185
Chicago/Turabian StyleTian, Wenchao, Wenbo Yu, Xiaohan Liu, Yongkun Wang, and Jing Shi. 2018. "A Review of the Characteristics, Synthesis, and Thermodynamics of Type-II Weyl Semimetal WTe2" Materials 11, no. 7: 1185. https://doi.org/10.3390/ma11071185
APA StyleTian, W., Yu, W., Liu, X., Wang, Y., & Shi, J. (2018). A Review of the Characteristics, Synthesis, and Thermodynamics of Type-II Weyl Semimetal WTe2. Materials, 11(7), 1185. https://doi.org/10.3390/ma11071185





