Directed Thermal Diffusions through Metamaterial Source Illusion with Homogeneous Natural Media
Abstract
1. Introduction
2. Theoretical Derivations of Conductivities Tensors and Geometrical Models
2.1. Theoretical Derivation of Conductivities Tensor
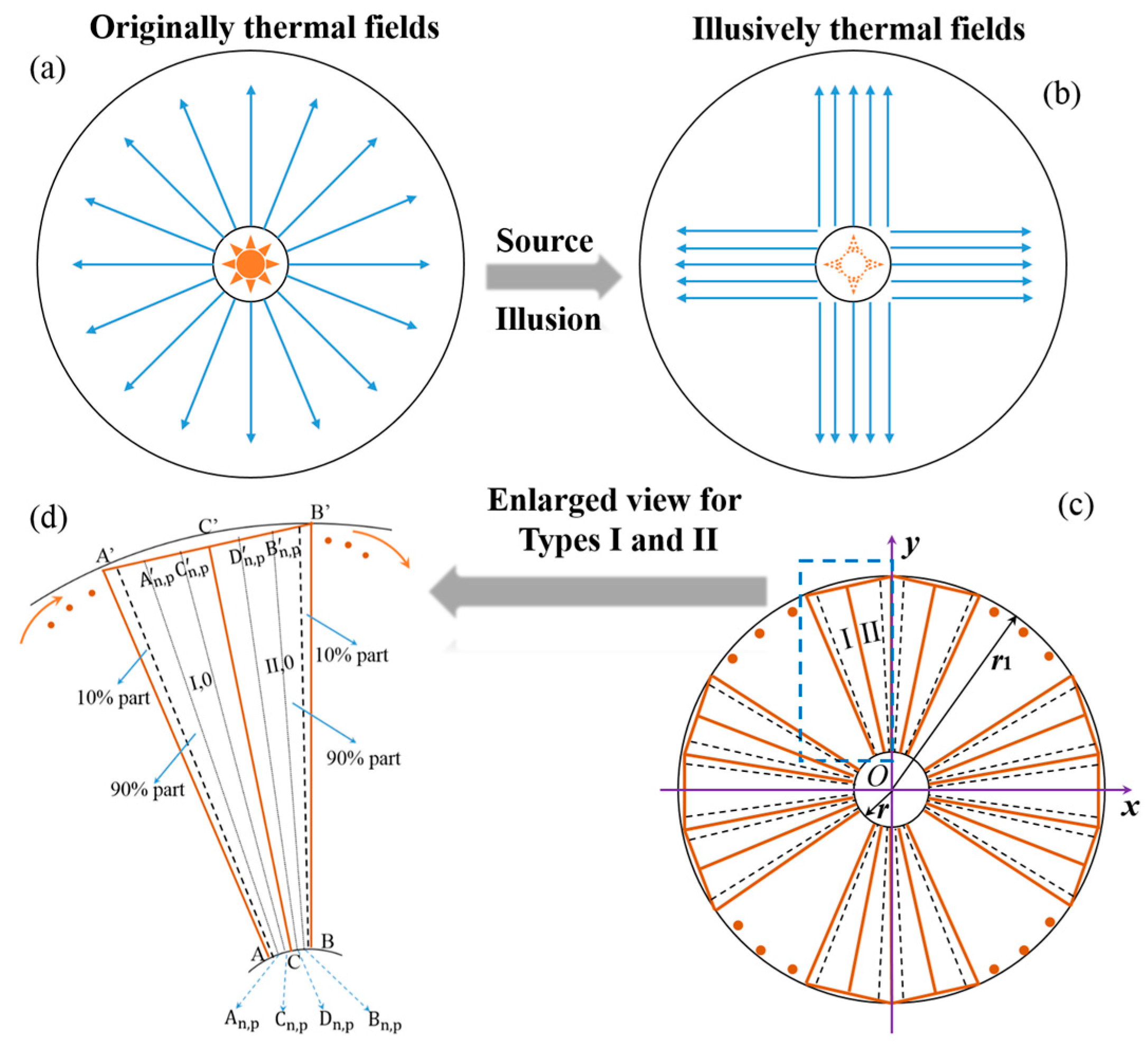
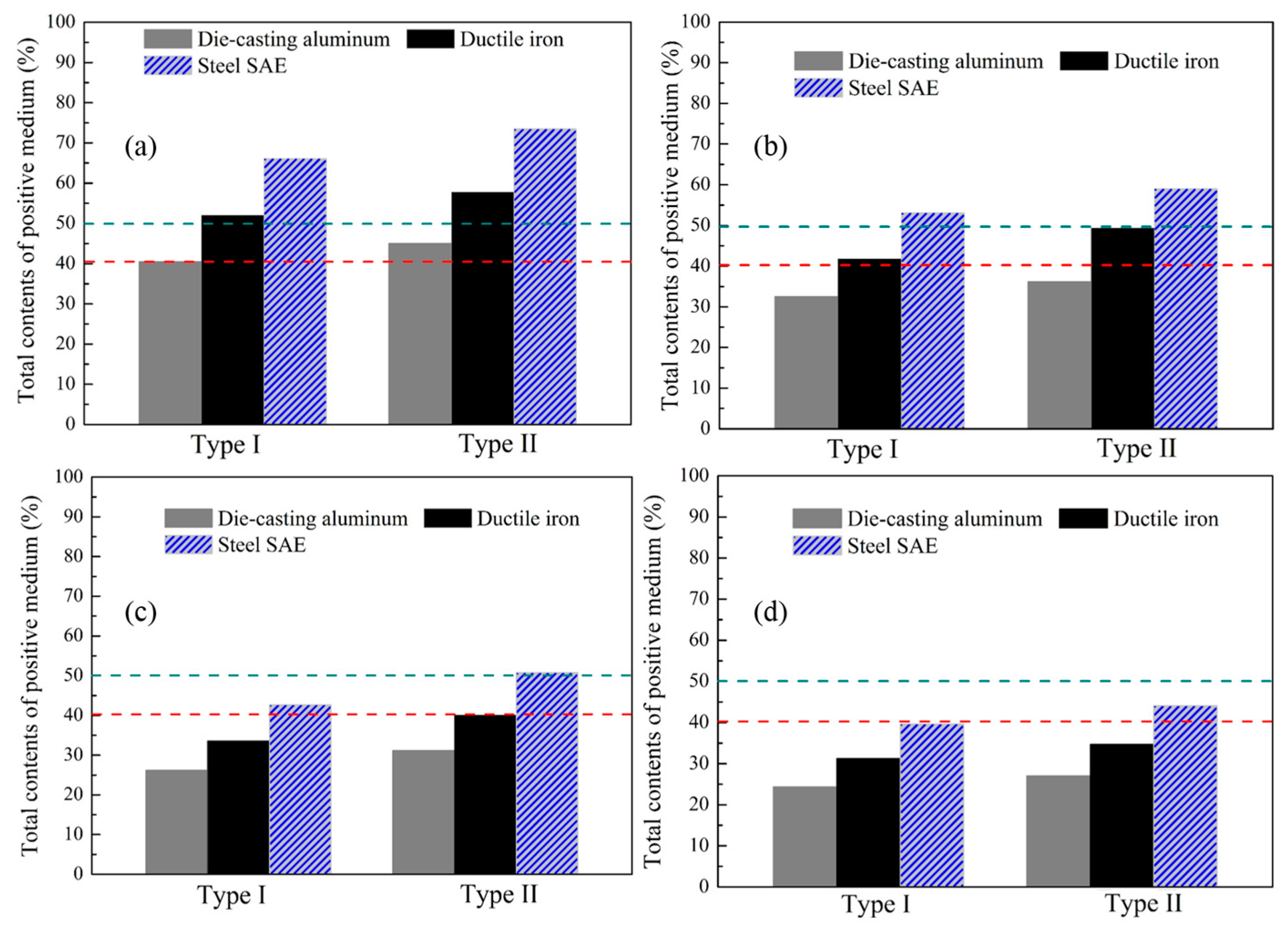
2.2. Selections of Materials and Geometric Profiles of Thermal Source Illusions
3. Demonstration of the Proposed Schemes and Discussions
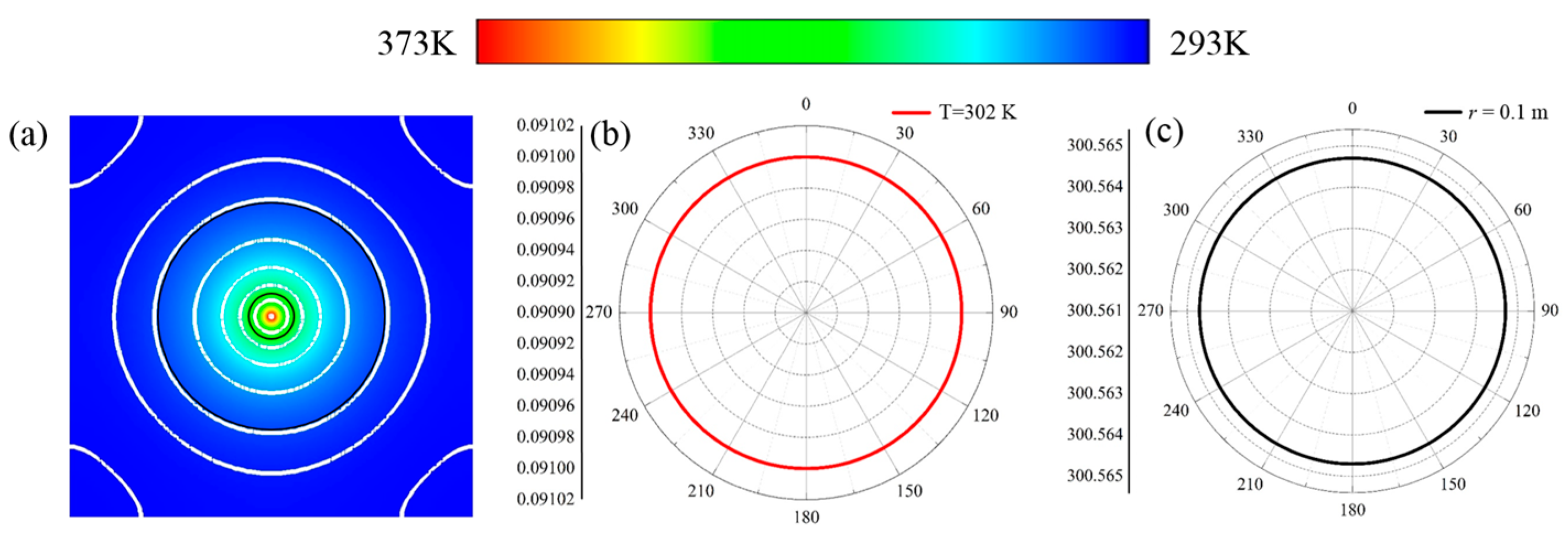
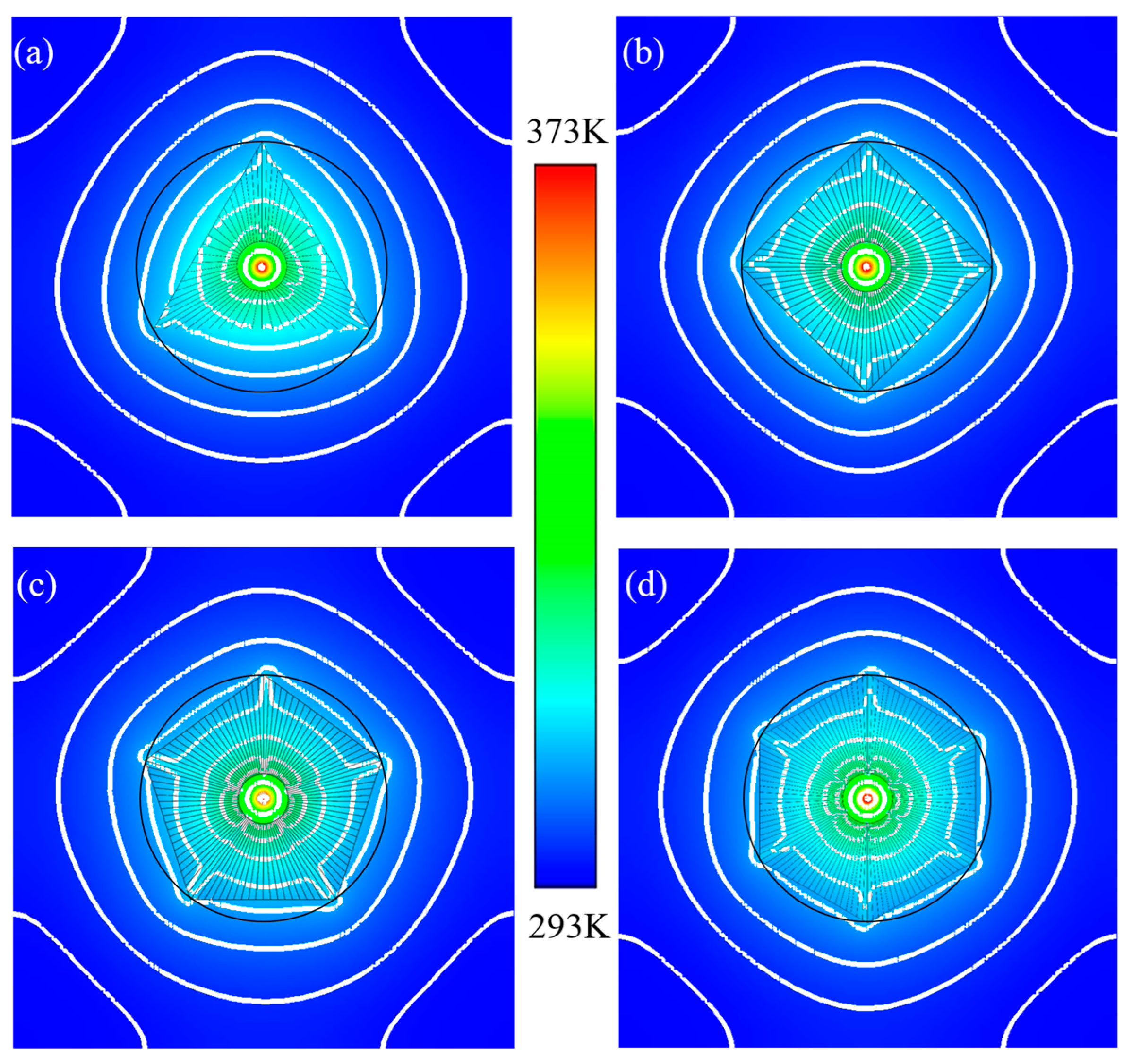
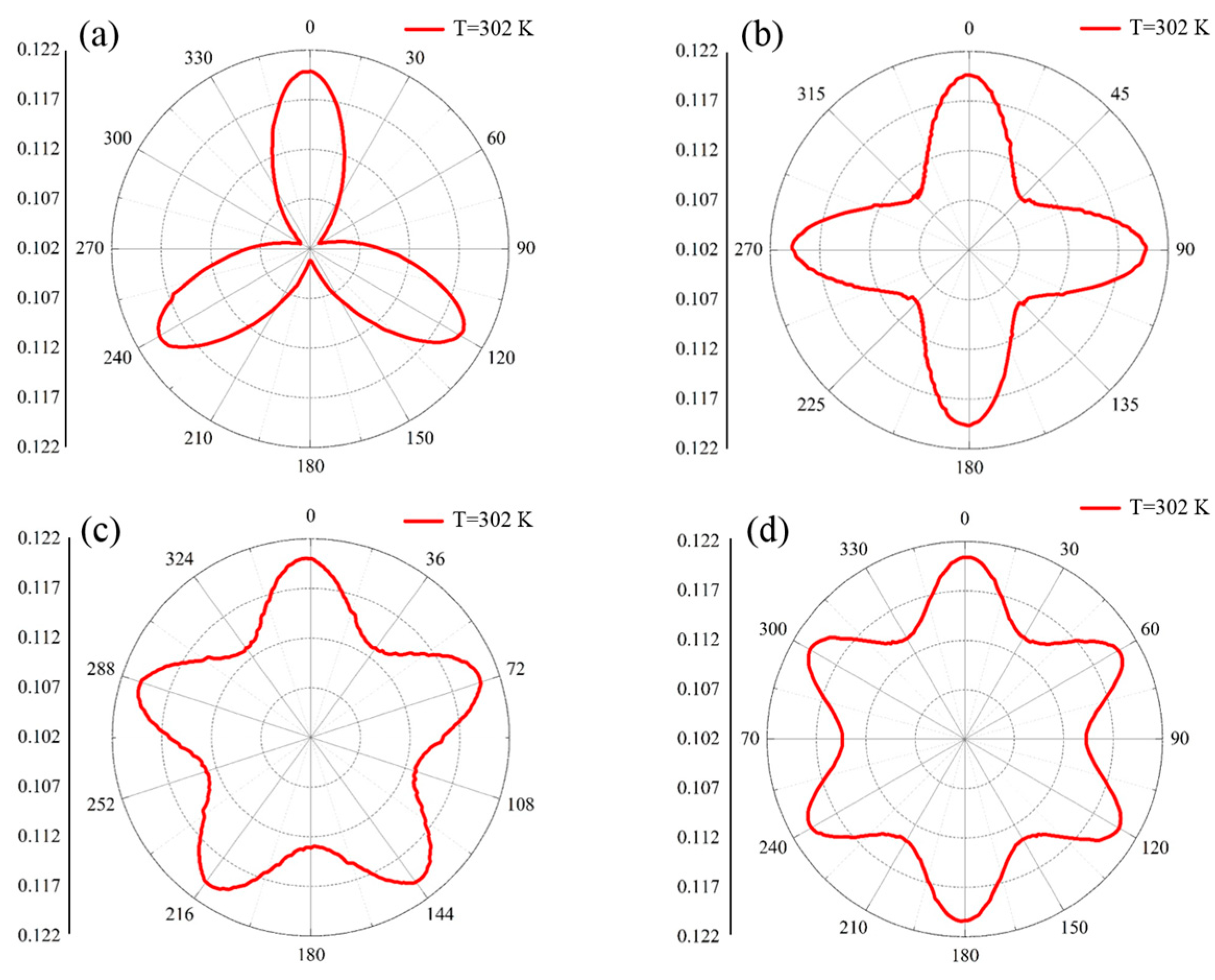
3.1. Temperature Distributions of the Proposed Thermal Source Illusions
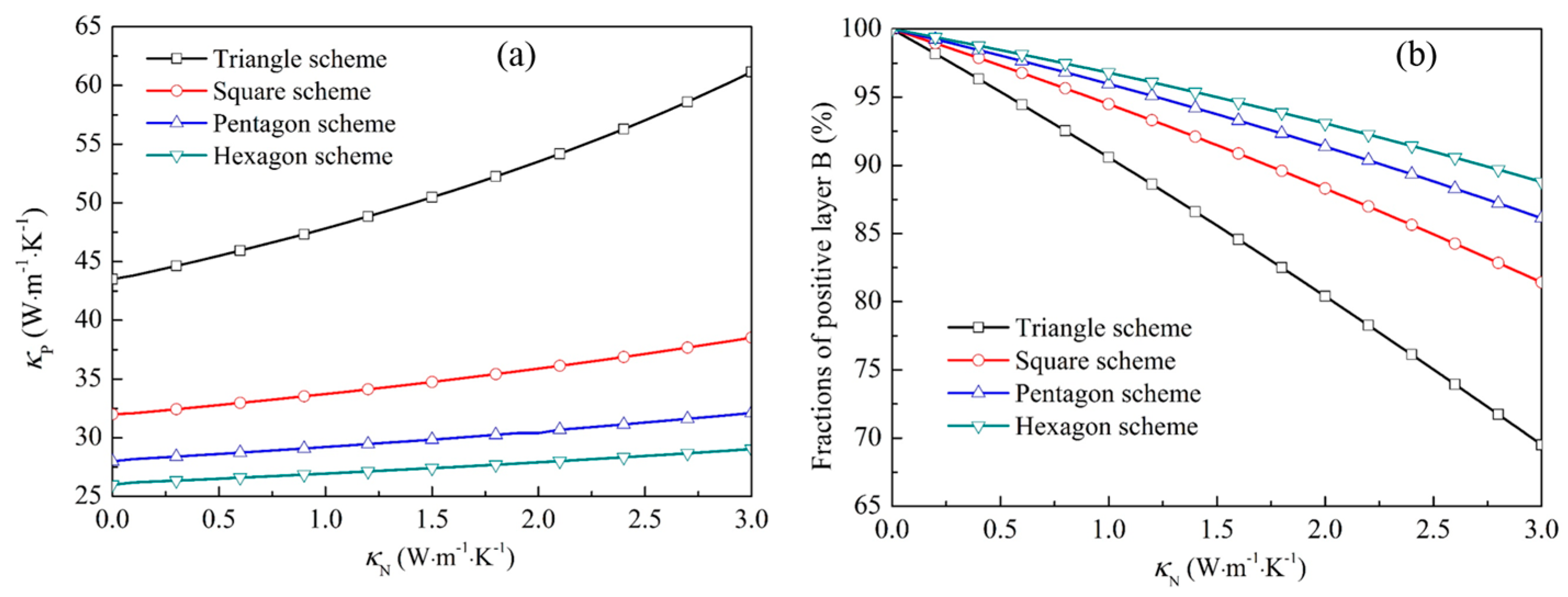
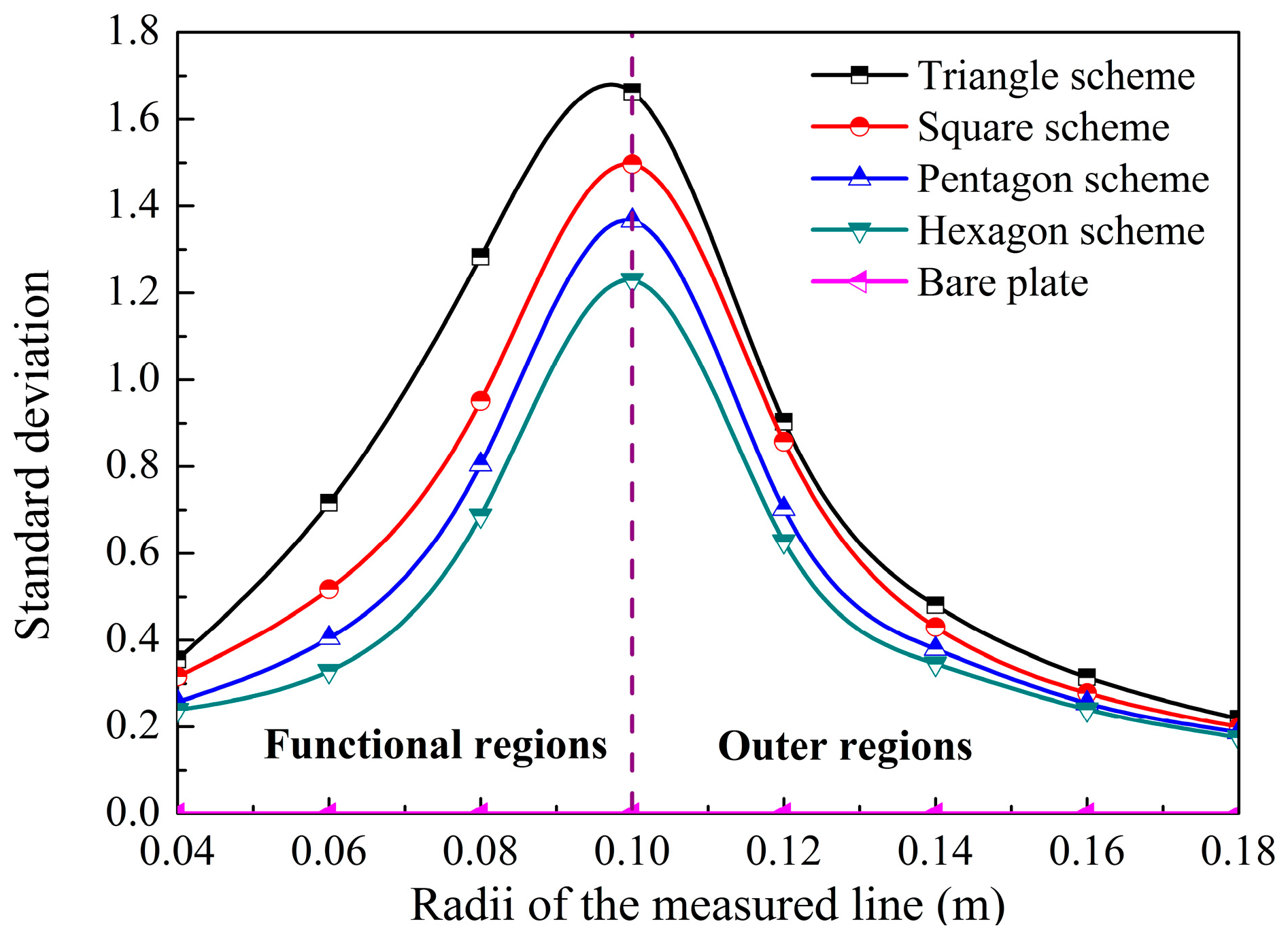
3.2. Characteristics of Temperature Distribution
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| Structural Parameters (m) | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Scheme | Triangle | Square | Pentagon | Hexagon | Bare plate | ||||||||
| Outer radii | 0.1 | 0.1 | 0.1 | 0.1 | / | ||||||||
| Inner radii | 0.02 | 0.02 | 0.02 | 0.02 | / | ||||||||
| Background | 0.4 | 0.4 | 0.4 | 0.4 | 0.4 | ||||||||
| Employed mediums for each proposed scheme | |||||||||||||
| Type | I | II | Center and background | ||||||||||
| Triangle | 10% copper, 40.54% die-casting aluminum A380, 49.46% PDMS | 45.05% die-casting aluminum A380, 54.95% PDMS | Nickel Steel (50% Ni) | ||||||||||
| Square | 10% copper, 41.78% ductile iron, 48.22% PDMS | 49.42% ductile iron, 50.58% PDMS | Nickel Steel (50% Ni) | ||||||||||
| Pentagon | 10% copper, 42.70% steel SAE, 47.30% PDMS | 50.83% steel SAE, 49.17% PDMS | Nickel Steel (50% Ni) | ||||||||||
| Hexagon | 10% aluminum alloy (6063), 39.71% steel SAE, 50.29% PDMS | 44.12% steel SAE, 55.88% PDMS | Nickel Steel (50% Ni) | ||||||||||
| Bare plate | Nickel Steel (50% Ni) | ||||||||||||
| Material parameters | |||||||||||||
| Material | Copper | Aluminum alloy (6063) | PDMS | Nickel steel (50% Ni) | Aluminum A380 | Ductile iron | Steel SAE | ||||||
| Conductivity (W∙m−1∙K−1) | 398 | 201 | 0.15 | 19.6 | 96.2 | 75 | 59 | ||||||
| Density (kg∙m−3) | 8930 | 2690 | 1000 | 8260 | 2710 | 7100 | 7830 | ||||||
| Specific heat (J∙kg−1∙K−1) | 386 | 900 | 1450 | 460 | 963 | 450 | 460 | ||||||
| Boundary conditions for all the schemes | |||||||||||||
| Left wall | Right wall | Top wall | Bottom wall | Ambient | Central point source | ||||||||
| Thermal isolation | Thermal isolation | Thermal isolation | Thermal isolation | 293 K | 373 K | ||||||||
Appendix B
Appendix B.1. Comparison between the Proposed Methodology and Well-Established Methods
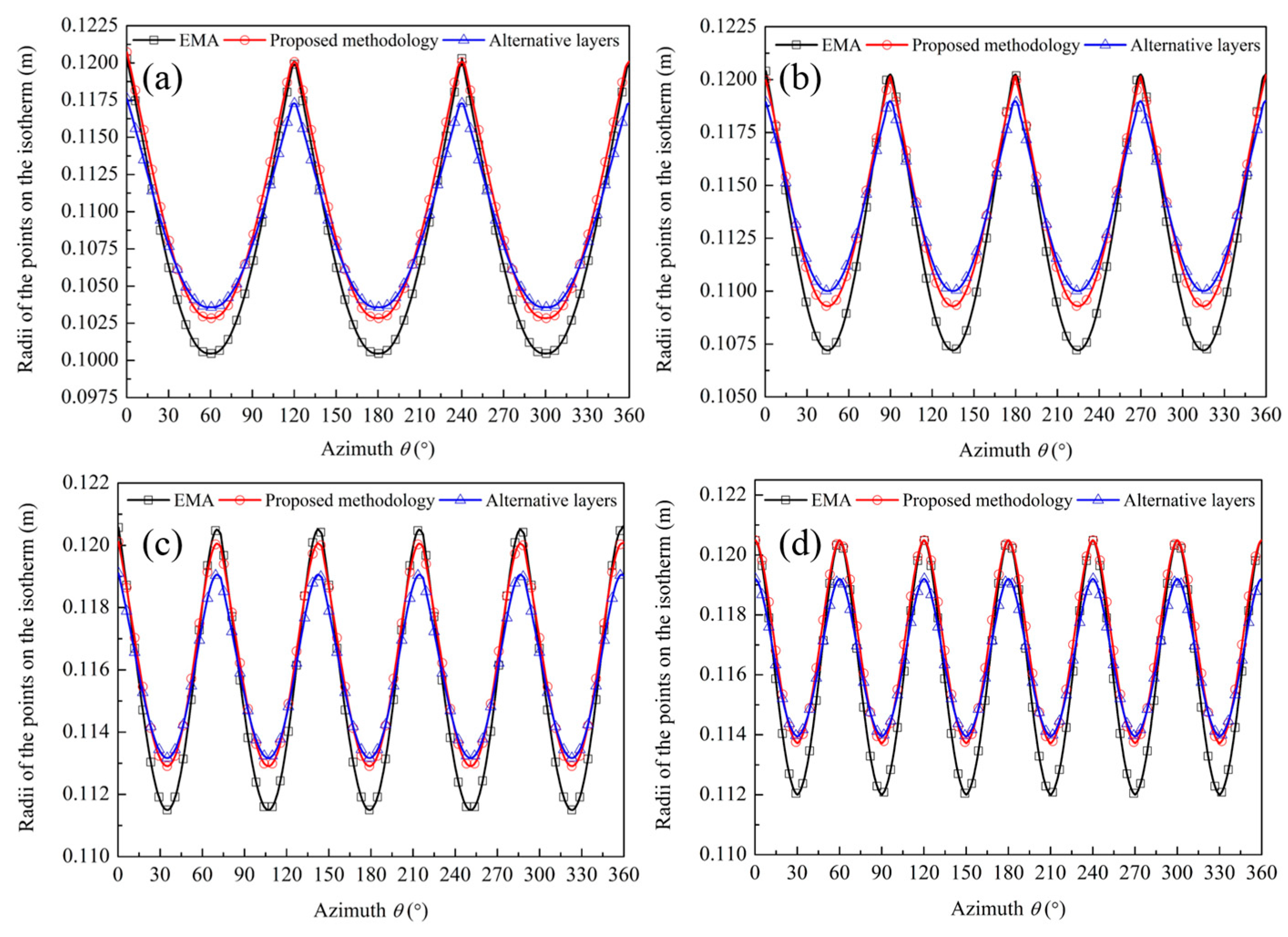
Appendix B.2. Independence Analysis of the Presented Schemes
| Illusive Schemes (Figure 6) | Grid Number | ||||
|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | |
| Triangle | 39,214 | 48,961 | 59,865 | 69,023 | 78,165 |
| Square | 46,650 | 57,732 | 67,836 | 76,962 | 86,142 |
| Pentagon | 54,685 | 65,985 | 74,986 | 86,316 | 97,645 |
| Hexagon | 62,947 | 73,546 | 82,643 | 91,687 | 102,567 |

References
- Pendry, J.B.; Shurig, D.; Smith, D.R. Controlling electromagnetic fields. Science 2006, 312, 1780–1782. [Google Scholar] [CrossRef] [PubMed]
- Leonhardt, U. Optical conformal mapping. Science 2006, 312, 1777–1780. [Google Scholar] [CrossRef] [PubMed]
- Shurig, D.; Mock, J.J.; Justice, B.J. Metamaterial electromagnetic cloak at microwave frequencies. Science 2006, 314, 977–980. [Google Scholar] [CrossRef] [PubMed]
- Ni, X.; Wong, Z.J.; Mrejen, M.; Wang, Y.; Zhang, X. An ultrathin invisibility skin cloak for visible light. Science 2015, 349, 1310–1314. [Google Scholar] [CrossRef] [PubMed]
- Bückmann, T.; Stenger, N.; Kadic, M.; Kaschke, J.; Andreas Frölich, A.; Kennerknecht, T.; Eberl, C.; Thiel, M.; Wegener, M. Tailored 3D mechanical metamaterials made by dip-in direct-laser-writing optical lithography. Adv. Mater. 2012, 24, 2710–2714. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Statharas, E.C.; Yao, K.; Hong, M. A broadband acoustic metamaterial with impedance matching layer of gradient index. Appl. Phys. Lett. 2017, 110, 241903. [Google Scholar] [CrossRef]
- Fan, C.Z.; Gao, Y.; Huang, J.P. Shaped graded materials with an apparent negative thermal conductivity. Appl. Phys. Lett. 2008, 92, 251907. [Google Scholar] [CrossRef]
- Liberal, I.; Engheta, N. Near-zero refractive index photonics. Nat. Photonics 2017, 11, 149. [Google Scholar] [CrossRef]
- Sihvola, A.H. Electromagnetic Mixing Formulas and Applications; IET: Stevenage, UK, 1999. [Google Scholar]
- Rahm, M.; Cummer, S.A.; Schurig, D.; Pendry, J.B.; Smith, D.R. Optical design of reflectionless complex media by finite embedded coordinate transformations. Phys. Rev. Lett. 2008, 100, 063903. [Google Scholar] [CrossRef] [PubMed]
- Jiang, W.X.; Cui, T.J.; Ma, H.F.; Zhou, X.Y.; Cheng, Q. Cylindrical-to-plane-wave conversion via embedded optical transformation. Appl. Phys. Lett. 2008, 92, 261903. [Google Scholar] [CrossRef]
- Jiang, W.X.; Cui, T.J.; Ma, H.F.; Yang, X.M.; Cheng, Q. Layered high-gain lens antennas via discrete optical transformation. Appl. Phys. Lett. 2008, 92, 261906. [Google Scholar] [CrossRef]
- Cheng, Q.; Jiang, W.X.; Cui, T.J. Multi-beam generations at pre-designed directions based on anisotropic zero-index metamaterials. Appl. Phys. Lett. 2011, 99, 131913. [Google Scholar] [CrossRef]
- Zhang, K.; Ding, X.; Wo, D.; Meng, F.; Wu, Q. Experimental validation of ultra-thin metalenses for N-beam emissions based on transformation optics. Appl. Phys. Lett. 2016, 108, 053508. [Google Scholar]
- La Spada, L.; Haq, S.; Hao, Y. Modeling and design for electromagnetic surface wave devices. Radio Sci. 2017, 52, 1049–1057. [Google Scholar] [CrossRef]
- Song, G.Y.; Jiang, W.X.; Cheng, Q.; Wu, L.T.; Dong, H.Y.; Cui, T.J. Acoustic magnifying lens for far-field high resolution imaging based on transformation acoustics. Adv. Mater. Technol. 2017, 2, 1700089. [Google Scholar] [CrossRef]
- Liu, Y.; Liang, Z.; Liu, F.; Diba, O.; Lamb, A.; Li, J. Source illusion devices for flexural lamb waves using elastic metasurfaces. Phys. Rev. Lett. 2017, 119, 034301. [Google Scholar] [CrossRef] [PubMed]
- Han, T.; Bai, X.; Thong, J.T.L.; Li, B.; Qiu, C.W. Full control and manipulation of heat signatures: Cloaking, camouflage and thermal metamaterials. Adv. Mater. 2014, 26, 1731–1734. [Google Scholar] [CrossRef] [PubMed]
- Han, T.; Qiu, C.W. Transformation Laplacian metamaterials: Recent advances in manipulating thermal and dc fields. J. Opt. 2016, 18, 044003. [Google Scholar] [CrossRef]
- Yang, T.Z.; Su, Y.; Xu, W.; Yang, X.D. Transient thermal camouflage and heat signature control. Appl. Phys. Lett. 2016, 109, 121905. [Google Scholar] [CrossRef]
- He, X.; Wu, L. Illusion thermodynamics: A camouflage technique changing an object into another one with arbitrary cross section. Appl. Phys. Lett. 2014, 105, 221904. [Google Scholar] [CrossRef]
- Chen, T.H.; Yang, F.; Mei, Z.L. A simple and flexible thermal illusion device and its experimental verification. Phys. Status Solidi A 2015, 212, 1746–1750. [Google Scholar] [CrossRef]
- Zhu, N.Q.; Shen, X.Y.; Huang, J.P. Converting the patterns of local heat flux via thermal illusion device. AIP Adv. 2015, 5, 053401. [Google Scholar] [CrossRef]
- Hou, Q.; Zhao, X.; Meng, T.; Liu, C. Illusion thermal device based on material with constant anisotropic thermal conductivity for location camouflage. Appl. Phys. Lett. 2016, 109, 103506. [Google Scholar] [CrossRef]
- Wang, J.; Bi, Y.; Hou, Q. Three-dimensional illusion thermal device for location camouflage. Sci. Rep. 2017, 7, 7541. [Google Scholar] [CrossRef] [PubMed]
- Vemuri, K.P.; Bandaru, P.R. Geometrical considerations in the control and manipulation of conductive heat flux in multilayered thermal metamaterials. Appl. Phys. Lett. 2013, 103, 133111. [Google Scholar] [CrossRef]
- Yang, T.; Vemuri, K.P.; Bandaru, P.R. Experimental evidence for the bending of heat flux in a thermal metamaterial. Appl. Phys. Lett. 2014, 105, 083908. [Google Scholar] [CrossRef]
- Xu, G.Q.; Zhang, H.C.; Jin, Y.; Li, S.; Li, Y. Control and design heat flux bending in thermal devices with transformation optics. Opt. Express 2017, 25, A419–A431. [Google Scholar] [CrossRef] [PubMed]
- Schittny, R.; Kadic, M.; Guenneau, S.; Wegener, M. Experiments on transformation thermodynamics: Molding the flow of heat. Phys. Rev. Lett. 2013, 110, 195901. [Google Scholar] [CrossRef] [PubMed]
- Han, T.; Bai, X.; Gao, D.; Thong, J.T.L.; Li, B.; Qiu, C.W. Experimental demonstration of a bilayer thermal cloak. Phys. Rev. Lett. 2014, 112, 054302. [Google Scholar] [CrossRef] [PubMed]
- Xu, G.Q.; Zhang, H.C.; Zou, Q.; Jin, Y. Predicting and analyzing interaction of the thermal cloaking performance through response surface method. Int. J. Heat Mass Trans. 2017, 109, 746–754. [Google Scholar] [CrossRef]
- Han, T.; Bai, X.; Liu, D.; Gao, D.; Li, B.; Thong, J.T.L.; Qiu, C.W. Manipulating steady heat conduction by sensu-shaped thermal metamaterials. Sci. Rep. 2015, 5, 11552. [Google Scholar] [CrossRef] [PubMed]
- Park, G.; Kang, S.; Lee, H.; Choi, W. Tunable multifunctional thermal metamaterials: Manipulation of local heat flux via assembly of unit-cell thermal shifters. Sci. Rep. 2017, 7, 41000. [Google Scholar] [CrossRef] [PubMed]
- Xu, G.Q.; Zhang, H.C.; Zhang, X.; Jin, Y. Investigating the thermodynamic performances of to-based metamaterial tunable cells with an entropy generation approach. Entropy 2017, 19, 538. [Google Scholar] [CrossRef]
- Iovine, R.; La Spada, L.; Vegni, L. Nanoplasmonic sensor for chemical measurements. In Optical Sensors 2013; International Society for Optics and Photonics: Cardiff, UK, 2013; Volume 8774, p. 877411. [Google Scholar]
- Novoselov, K.S.; Fal’ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar] [CrossRef] [PubMed]
- McManus, T.M.; La Spada, L.; Hao, Y. Isotropic and anisotropic surface wave cloaking techniques. J. Opt. 2016, 18, 044005. [Google Scholar] [CrossRef]
- La Spada, L.; McManus, T.M.; Dyke, A.; Haq, S.; Zhang, L.; Cheng, Q.; Hao, Y. Surface wave cloak from graded refractive index nanocomposites. Sci. Rep. 2016, 6, 29363. [Google Scholar] [CrossRef] [PubMed]
- Iovine, R.; La Spada, L.; Vegni, L. Optical properties of modified nanorod particles for biomedical sensing. IEEE Trans. Magn. 2014, 50, 169–172. [Google Scholar] [CrossRef]
- Liu, Y.; Hao, Y.; Li, K.; Gong, S. Radar cross section reduction of a microstrip antenna based on polarization conversion metamaterial. IEEE Antennas Wirel. Propag. Lett. 2016, 15, 80–83. [Google Scholar] [CrossRef]
- Cai, W.; Chettiar, U.K.; Kildishev, A.V.; Shalaev, V.M. Optical cloaking with metamaterials. Nat. Photonics 2007, 1, 224. [Google Scholar] [CrossRef]
- Zheludev, N.I.; Kivshar, Y.S. From metamaterials to metadevices. Nat. Mater. 2012, 11, 917. [Google Scholar] [CrossRef] [PubMed]
- La Spada, L.; Iovine, R.; Vegni, L. Electromagnetic modeling of dielectric mixtures. J. Res. Updates Polym. Sci. 2013, 2, 194–200. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, G.; Zhang, H.; Jin, L.; Jin, Y. Directed Thermal Diffusions through Metamaterial Source Illusion with Homogeneous Natural Media. Materials 2018, 11, 629. https://doi.org/10.3390/ma11040629
Xu G, Zhang H, Jin L, Jin Y. Directed Thermal Diffusions through Metamaterial Source Illusion with Homogeneous Natural Media. Materials. 2018; 11(4):629. https://doi.org/10.3390/ma11040629
Chicago/Turabian StyleXu, Guoqiang, Haochun Zhang, Liang Jin, and Yan Jin. 2018. "Directed Thermal Diffusions through Metamaterial Source Illusion with Homogeneous Natural Media" Materials 11, no. 4: 629. https://doi.org/10.3390/ma11040629
APA StyleXu, G., Zhang, H., Jin, L., & Jin, Y. (2018). Directed Thermal Diffusions through Metamaterial Source Illusion with Homogeneous Natural Media. Materials, 11(4), 629. https://doi.org/10.3390/ma11040629







