Subsurface Damage in Polishing Process of Silicon Carbide Ceramic
Abstract
:1. Introduction
2. Theoretical Analysis
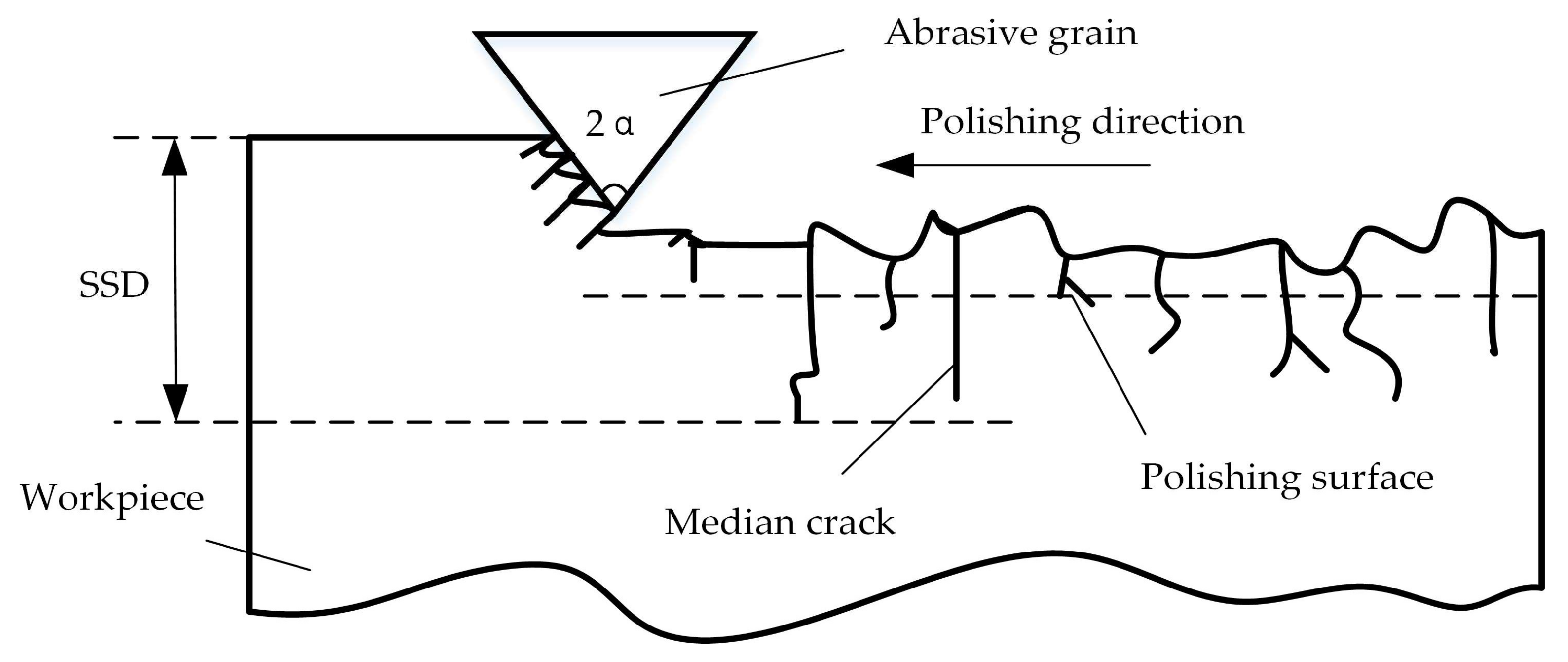
2.1. SSD Models
2.2. Models of Single-Grit Penetration Depth
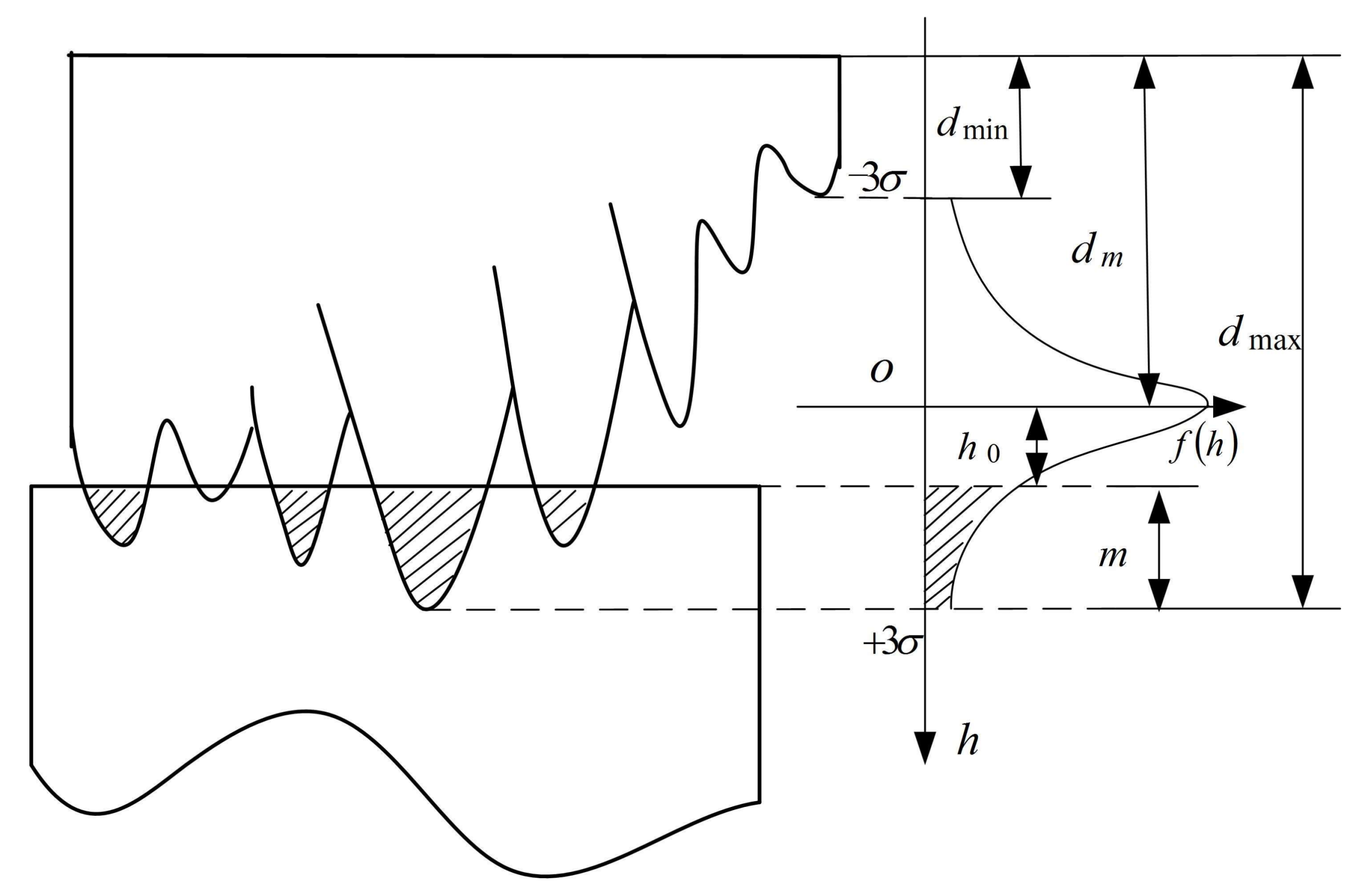
2.2.1. The Height Distribution of ABRASIVE Protrusion
2.2.2. The Number of Effective Grains
2.2.3. The Depth of a Single Abrasive Grain
2.3. Analysis for Dynamic Parameters
2.4. Analysis for SSD Depth Models
3. Numerical Simulation
3.1. Constitutive Models
3.2. Simulation Methods
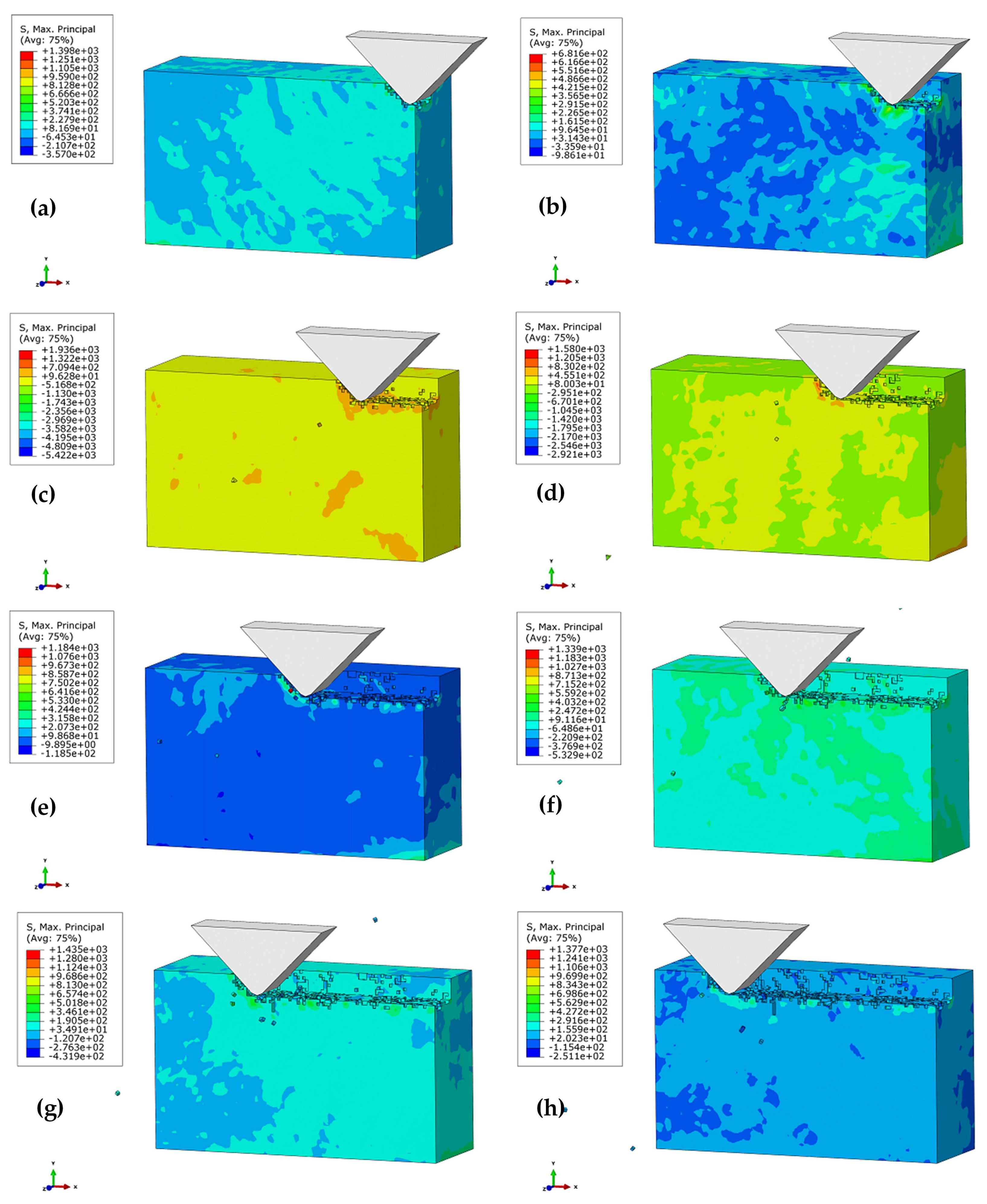
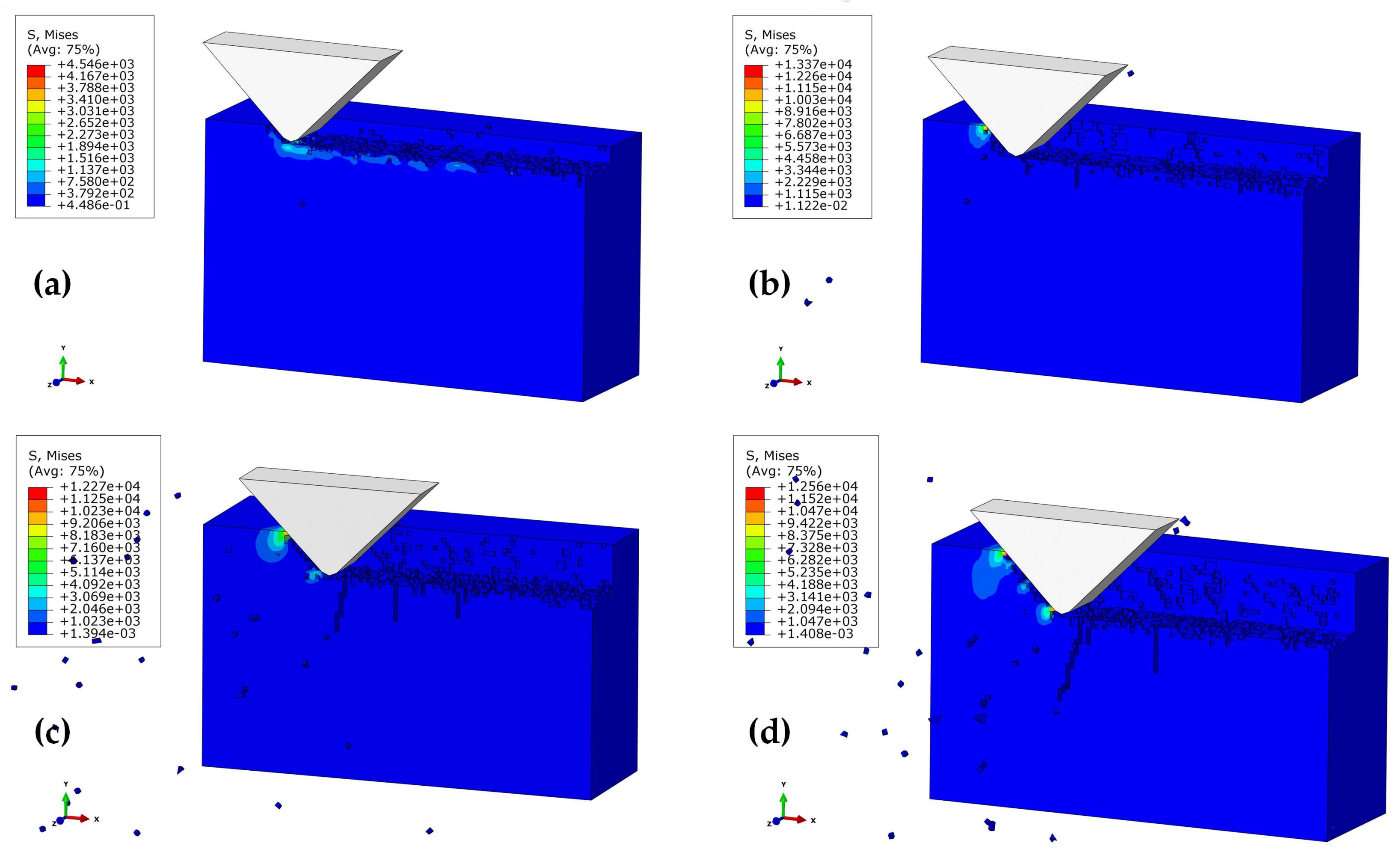
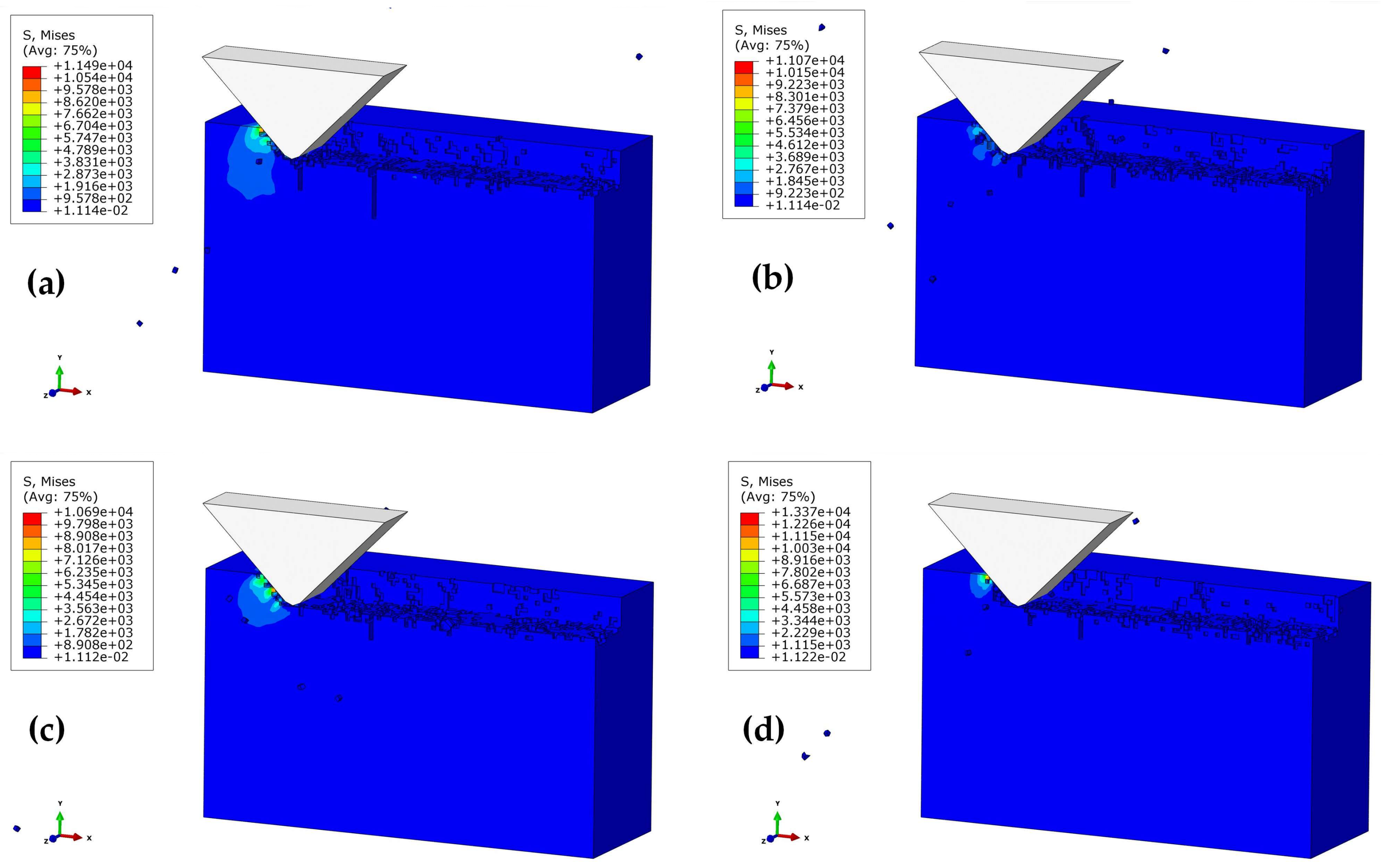
3.3. Simulation Results and Discussion
3.3.1. Analysis for Single-Grit Polishing Process
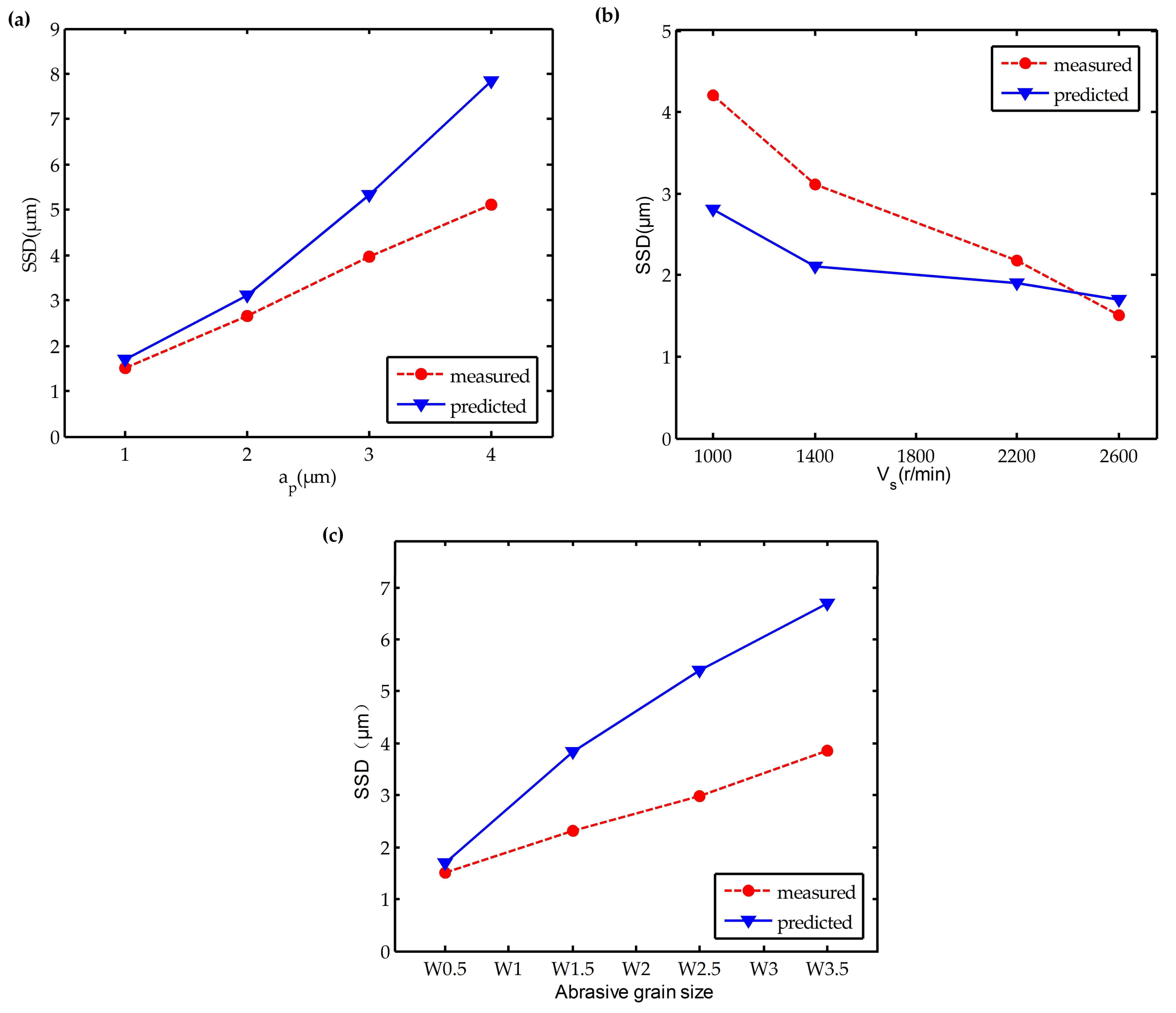
3.3.2. Effect of Polishing Depth on SSD Depth
3.3.3. Effect of Polishing Speed on SSD Depth
3.3.4. Effect of Abrasive Grain Size on SSD Depth
4. Validation of Experimental Results
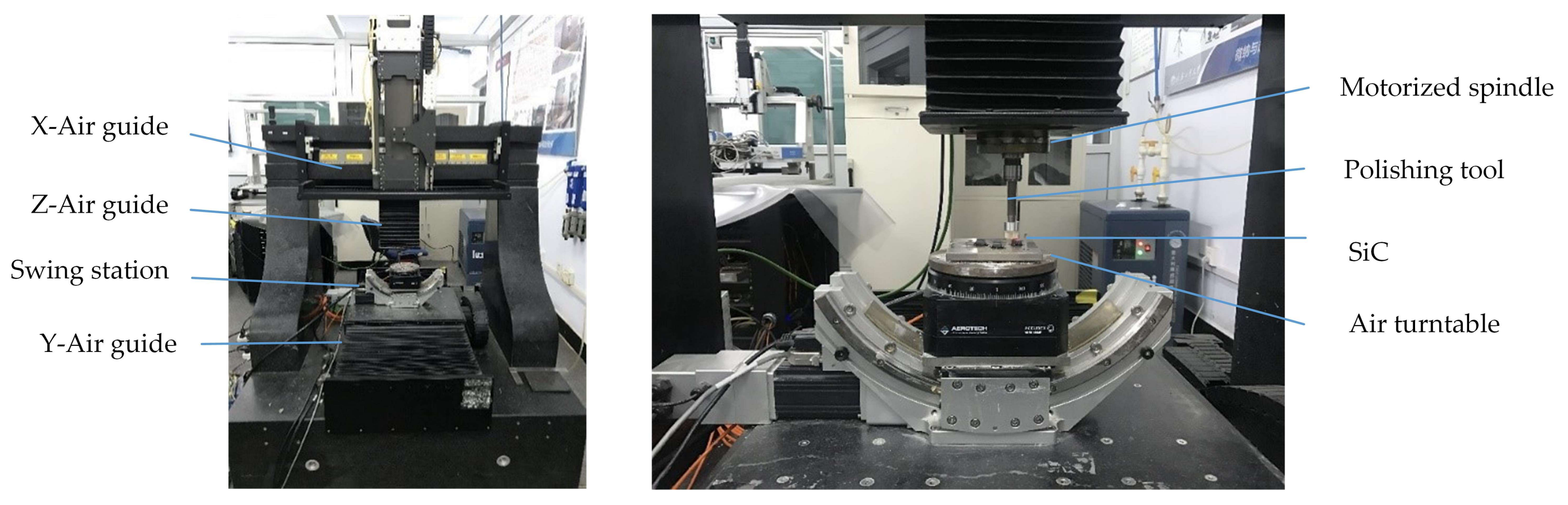
4.1. Experimental Preparation
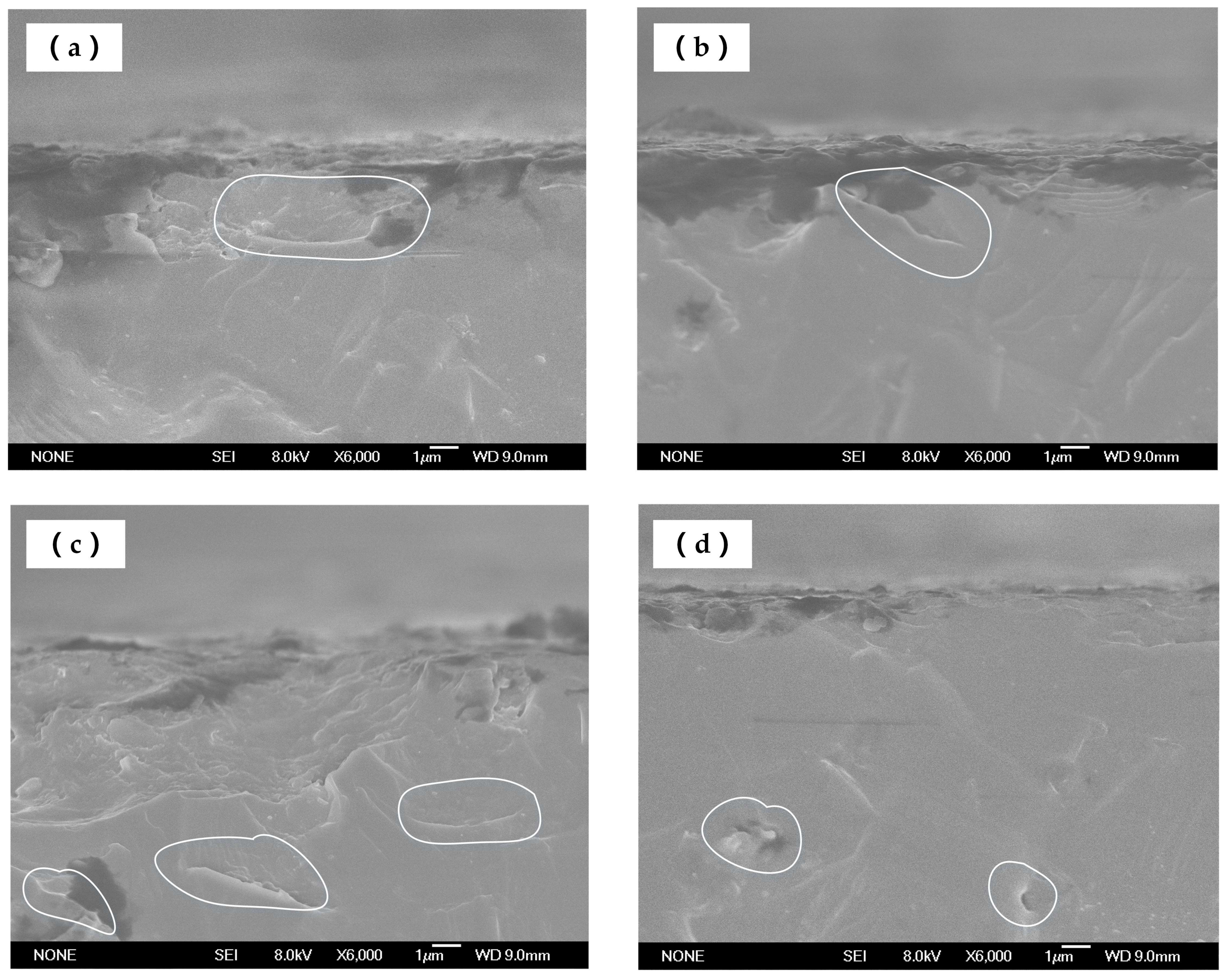
4.2. Experimental Results and Discussion
5. Conclusions
- (1)
- On the basis of the dynamic relationship between the abrasive particles and the workpiece in the theoretical analysis, when , the material removal is mainly brittle fracture. The SSD depth increases as the abrasive grain angle and load (proportional to the polishing depth) increases, and decreases as the speed increases.
- (2)
- In the simulation, the formation and propagation of cracks are presented. The simulation results indicate that the polishing depth, abrasive grain size, and polishing speed have the most significant effect on SSD, respectively. By increasing polishing depth and abrasive grain size, the SSD depth increases, and an increasing polishing speed results in a decrease in SSD depth.
- (3)
- The polishing experiments under different processing parameters are carried out. The experimental results validate the theoretical and simulation results.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Zhang, Y.; Zhang, J.; Han, J.; He, X.; Yao, W. Large-scale fabrication of lightweight Si/SiC ceramic composite optical mirror. Mater. Lett. 2004, 58, 1204–1208. [Google Scholar] [CrossRef]
- Ealey, M.A.; Weaver, G.Q. Developmental history and trends for reaction bonded silicon carbide mirrors. In Proceedings of the SPIE’s 1996 International Symposium on Optical Science, Engineering, and Instrumentation, Denver, CO, USA, 4–9 August 1996; pp. 66–72. [Google Scholar]
- Zhang, Z.; Yang, X.; Zheng, L.; Xue, D. High-performance grinding of a 2-m scale silicon carbide mirror blank for the space-based telescope. Int. J. Adv. Manuf. Technol. 2017, 89, 463–473. [Google Scholar] [CrossRef]
- Agarwal, S.; Rao, P.V. Grinding characteristics, material removal and damage formation mechanisms in high removal rate grinding of silicon carbide. Int. J. Mach. Tools Manuf. 2010, 50, 1077–1087. [Google Scholar] [CrossRef]
- Yang, M.Y.; Lee, H.C. Local material removal mechanism considering curvature effect in the polishing process of the small aspherical lens die. J. Mater. Process. Technol. 2001, 116, 298–304. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Riemer, O.; Gessenharter, A. Finishing of structured surfaces by abrasive polishing. Precis. Eng. 2006, 30, 325–336. [Google Scholar] [CrossRef]
- Wang, G.; Wang, Y.; Xu, Z. Modeling and analysis of the material removal depth for stone polishing. J. Mater. Process. Technol. 2009, 209, 2453–2463. [Google Scholar] [CrossRef]
- Qi, J.; Zhang, D.; Li, S.; Chen, B. A micro-model of the material removal depth for the polishing process. Int. J. Adv. Manuf. Technol. 2016, 86, 2759–2770. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, C.; Feng, P.; Zhang, J. A model for prediction of subsurface damage in rotary ultrasonic face milling of optical K9 glass. Int. J. Adv. Manuf. Technol. 2016, 83, 347–355. [Google Scholar] [CrossRef]
- Liu, T.; Ge, P.; Bi, W.; Gao, Y. Subsurface crack damage in silicon wafers induced by resin bonded diamond wire sawing. Mater. Sci. Semicond. Process. 2017, 57, 147–156. [Google Scholar] [CrossRef]
- Esmaeilzare, A.; Rahimi, A.; Rezaei, S.M. Investigation of subsurface damages and surface roughness in grinding process of Zerodur® glass–ceramic. Appl. Surf. Sci. 2014, 313, 67–75. [Google Scholar] [CrossRef]
- Chen, J.; Fang, Q.; Li, P. Effect of grinding wheel spindle vibration on surface roughness and subsurface damage in brittle material grinding. Int. J. Mach. Tools Manuf. 2015, 91, 12–23. [Google Scholar] [CrossRef]
- Zhu, D.; Yan, S.; Li, B. Single-grit modeling and simulation of crack initiation and propagation in SiC grinding using maximum undeformed chip thickness. Comput. Mater. Sci. 2014, 92, 13–21. [Google Scholar] [CrossRef]
- Liu, Y.; Li, B.; Wu, C.; Zheng, Y. Simulation-based evaluation of surface micro-cracks and fracture toughness in high-speed grinding of silicon carbide ceramics. Int. J. Adv. Manuf. Technol. 2016, 86, 799–808. [Google Scholar] [CrossRef]
- Komanduri, R.; Chandrasekaran, N.; Raff, L.M. Effect of tool geometry in nanometric cutting: A molecular dynamics simulation approach. Wear 1998, 219, 84–97. [Google Scholar] [CrossRef]
- Dai, H.; Chen, G.; Zhou, C.; Fang, Q.; Fei, X. A numerical study of ultraprecision machining of monocrystalline silicon with laser nano-structured diamond tools by atomistic simulation. Appl. Surf. Sci. 2017, 393, 405–416. [Google Scholar] [CrossRef]
- Li, J.; Fang, Q.; Zhang, L.; Liu, Y. Subsurface damage mechanism of high speed grinding process in single crystal silicon revealed by atomistic simulations. Appl. Surf. Sci. 2015, 324, 464–474. [Google Scholar] [CrossRef]
- Lucca, D.A.; Brinksmeier, E.; Goch, G. Progress in assessing surface and subsurface integrity. CIRP Ann. 1998, 47, 669–693. [Google Scholar] [CrossRef]
- Agarwal, S.; Rao, P.V. Experimental investigation of surface/subsurface damage formation and material removal mechanisms in SiC grinding. Int. J. Mach. Tools Manuf. 2008, 48, 698–710. [Google Scholar] [CrossRef]
- Jiang, C.; Xu, J.; Wang, C. Experimental investigation of subsurface damage of optical glass in precision grinding using a brittle material removal fraction. Int. J. Adv. Manuf. Technol. 2017, 90, 725–730. [Google Scholar] [CrossRef]
- Blaineau, P.; André, D.; Laheurte, R.; Darnis, P.; Darbois, N.; Cahuc, O.; Neauport, J. Subsurface mechanical damage during bound abrasive grinding of fused silica glass. Appl. Surf. Sci. 2015, 353, 764–773. [Google Scholar] [CrossRef]
- Wang, C.; Fang, Q.; Chen, J.; Liu, Y.; Jin, T. Subsurface damage in high-speed grinding of brittle materials considering kinematic characteristics of the grinding process. Int. J. Adv. Manuf. Technol. 2016, 83, 937–948. [Google Scholar] [CrossRef]
- Jing, X.; Maiti, S.; Subhash, G. A New Analytical Model for Estimation of Scratch-Induced Damage in Brittle Solids. J. Am. Ceram. Soc. 2007, 90, 885–892. [Google Scholar] [CrossRef]
- Lambropoulos, J.C.; Jacobs, S.D.; Ruckman, J. Material removal mechanisms from grinding to polishing. Ceram. Trans. 1999, 102, 113–128. [Google Scholar]
- Gu, W.; Yao, Z.; Li, K. Evaluation of subsurface crack depth during scratch test for optical glass BK7. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2011, 225, 2767–2774. [Google Scholar] [CrossRef]
- Xi, F.; Zhou, D. Modeling surface roughness in the stone polishing process. Int. J. Mach. Tools Manuf. 2005, 45, 365–372. [Google Scholar] [CrossRef]
- Doman, D.A.; Warkentin, A.; Bauer, R. A survey of recent grinding wheel topography models. Int. J. Mach. Tools Manuf. 2006, 46, 343–352. [Google Scholar] [CrossRef]
- Zhao, Y.; Chang, L. A micro-contact and wear model for chemical–mechanical polishing of silicon wafers. Wear 2002, 252, 220–226. [Google Scholar] [CrossRef]
- Bifano, T.G.; Dow, T.A.; Scattergood, R.O. Ductile-regime grinding: A new technology for machining brittle materials. J. Eng. Ind. 1991, 113, 184–189. [Google Scholar] [CrossRef]
- Holmquist, T.J.; Johnson, G.R. Characterization and evaluation of silicon carbide for high-velocity impact. J. Appl. Phys. 2005, 97, 093502. [Google Scholar] [CrossRef]
- Johnson, G.R.; Holmquist, T.J. An improved computational constitutive model for brittle materials. In Proceedings of the AIP Conference, Colorado Springs, CO, USA, 28 June–2 July 1993; pp. 981–984. [Google Scholar]




| M (Mesh Size) | (μm) | (μm) | σ (μm) |
|---|---|---|---|
| 180 | 84.4 | 47.3 | 12.4 |
| 320 | 47.5 | 21.1 | 8.8 |
| 400 | 38.0 | 15.5 | 7.5 |
| 600 | 25.3 | 8.8 | 5.5 |
| 800 | 19.0 | 5.9 | 4.4 |
| 1000 | 15.2 | 4.3 | 3.6 |
| 1500 | 10.1 | 2.4 | 2.6 |
| 2000 | 7.6 | 1.6 | 2.0 |
| 4000 | 3.8 | 0.6 | 1.1 |
| 6000 | 2.5 | 0.35 | 0.7 |
| 10,000 | 1.5 | 0.17 | 0.4 |
| Material Properties Parameters | Value |
|---|---|
| Density ρ (kg/m3) | 3215 |
| Elastic modulus E (GPa) | 454 |
| Poisson’s ratio | 0.25 |
| Yield strength σ (MPa) | 620 |
| Specific heat [J/(kg·K)] | 526.3 |
| Conductivity [W/(m·K)] | 180 |
| Hardness (GPa) | 29.4 |
| Constitutive Model | A | N | B | M | C | ||
| 3215 | 193 | 0.96 | 0.65 | 0.35 | 1.0 | 0.009 | |
| β | |||||||
| 12.2 | 1.3 | 11.7 | 5.13 | 1.0 | 1.0 | 0.75 | |
| β | |||||||
| 1.0 | 220 | 361 | 0 | ||||
| Failure Model | FS | Damage | |||||
| 0.48 | 0.48 | 1.2 | 0.0 | 0.2 | 0 |
| Polishing Parameters | Value |
|---|---|
| Polishing depth ap (μm) | 1, 2, 3, 4 |
| Polishing speed Vs (mm/s) | 523, 733, 1151, 1364 |
| Abrasive grain size h (μm) | 5, 6, 7, 8 |
| Test | Polishing Depth ap (μm) | Polishing Speed Vs (mm/s) | Abrasive Grain Size | Predicted SSD (μm) | Measured SSD (μm) |
|---|---|---|---|---|---|
| 1 | 1 | 2600 | W 0.5 | 1.697 | 1.515 |
| 2 | 2 | 2600 | W 0.5 | 3.110 | 2.231 |
| 3 | 3 | 2600 | W 0.5 | 5.340 | 3.961 |
| 4 | 4 | 2600 | W 0.5 | 7.834 | 5.113 |
| 5 | 1 | 1000 | W 0.5 | 2.804 | 4.212 |
| 6 | 1 | 1400 | W 0.5 | 2.102 | 3.116 |
| 7 | 1 | 2200 | W 0.5 | 1.898 | 2.176 |
| 9 | 1 | 2600 | W 1.5 | 3.844 | 2.325 |
| 10 | 1 | 2600 | W 2.5 | 5.398 | 2.987 |
| 11 | 1 | 2600 | W 3.5 | 6.697 | 3.857 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, Y.; Zhu, W.; Lin, J.; Lu, M.; Kang, M. Subsurface Damage in Polishing Process of Silicon Carbide Ceramic. Materials 2018, 11, 506. https://doi.org/10.3390/ma11040506
Gu Y, Zhu W, Lin J, Lu M, Kang M. Subsurface Damage in Polishing Process of Silicon Carbide Ceramic. Materials. 2018; 11(4):506. https://doi.org/10.3390/ma11040506
Chicago/Turabian StyleGu, Yan, Wenhui Zhu, Jieqiong Lin, Mingming Lu, and Mingshuo Kang. 2018. "Subsurface Damage in Polishing Process of Silicon Carbide Ceramic" Materials 11, no. 4: 506. https://doi.org/10.3390/ma11040506
APA StyleGu, Y., Zhu, W., Lin, J., Lu, M., & Kang, M. (2018). Subsurface Damage in Polishing Process of Silicon Carbide Ceramic. Materials, 11(4), 506. https://doi.org/10.3390/ma11040506






