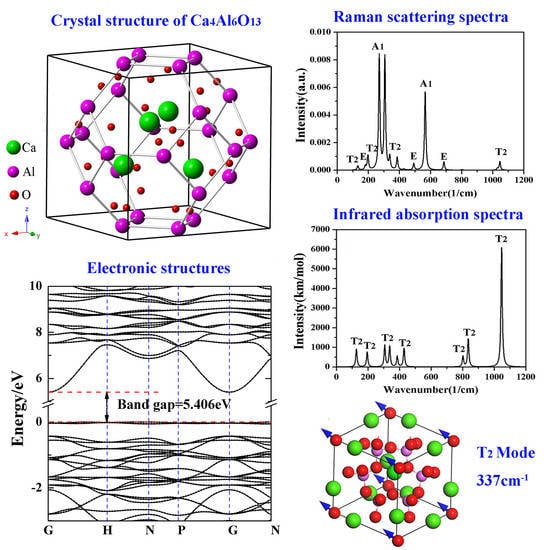
Electronic, Optical, and Lattice Dynamical Properties of Tetracalcium Trialuminate (Ca4Al6O13)
Abstract
1. Introduction
2. Crystal Structure and Computational Details
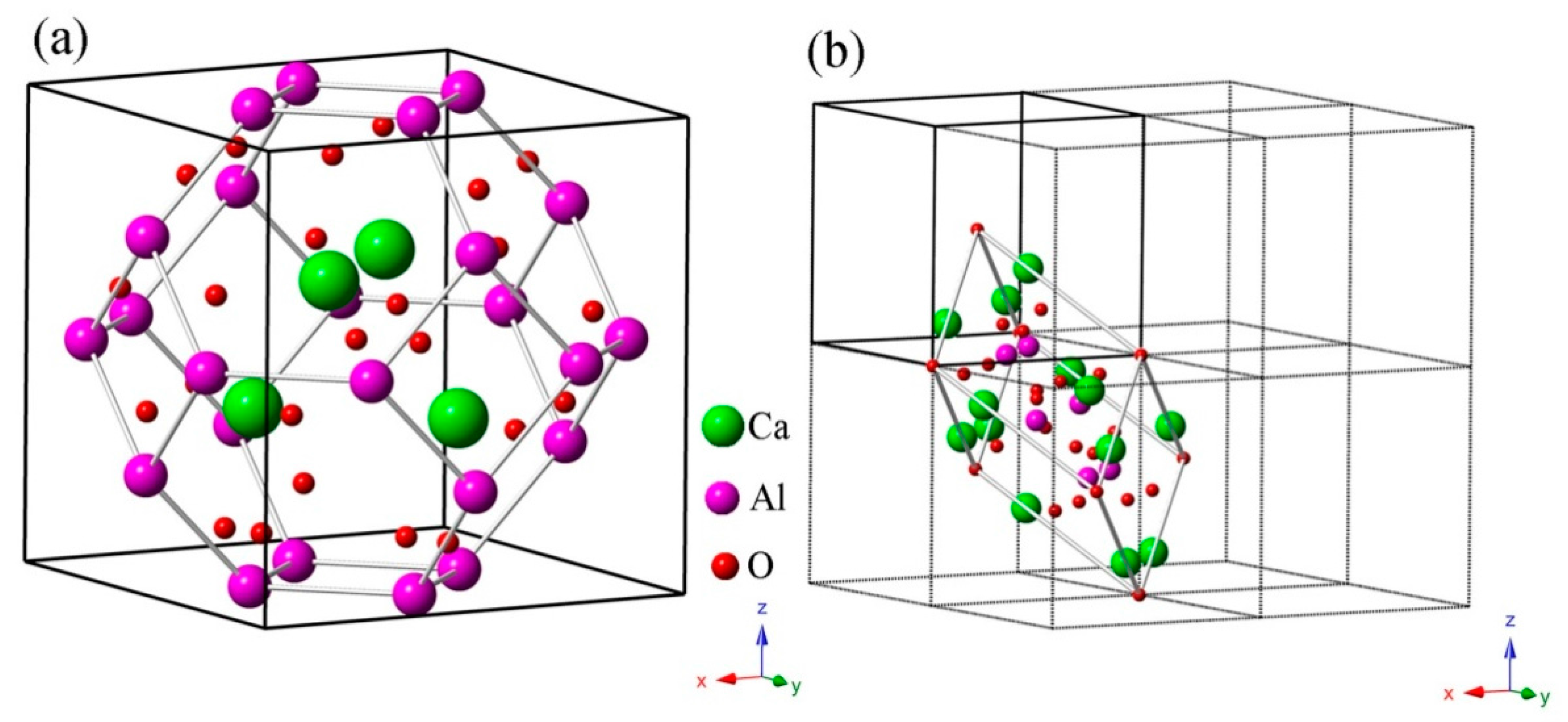
2.1. Crystal Structure
2.2. Computational Details
3. Results and Discussion
3.1. Structural Parameters
3.2. Mechanical Properties
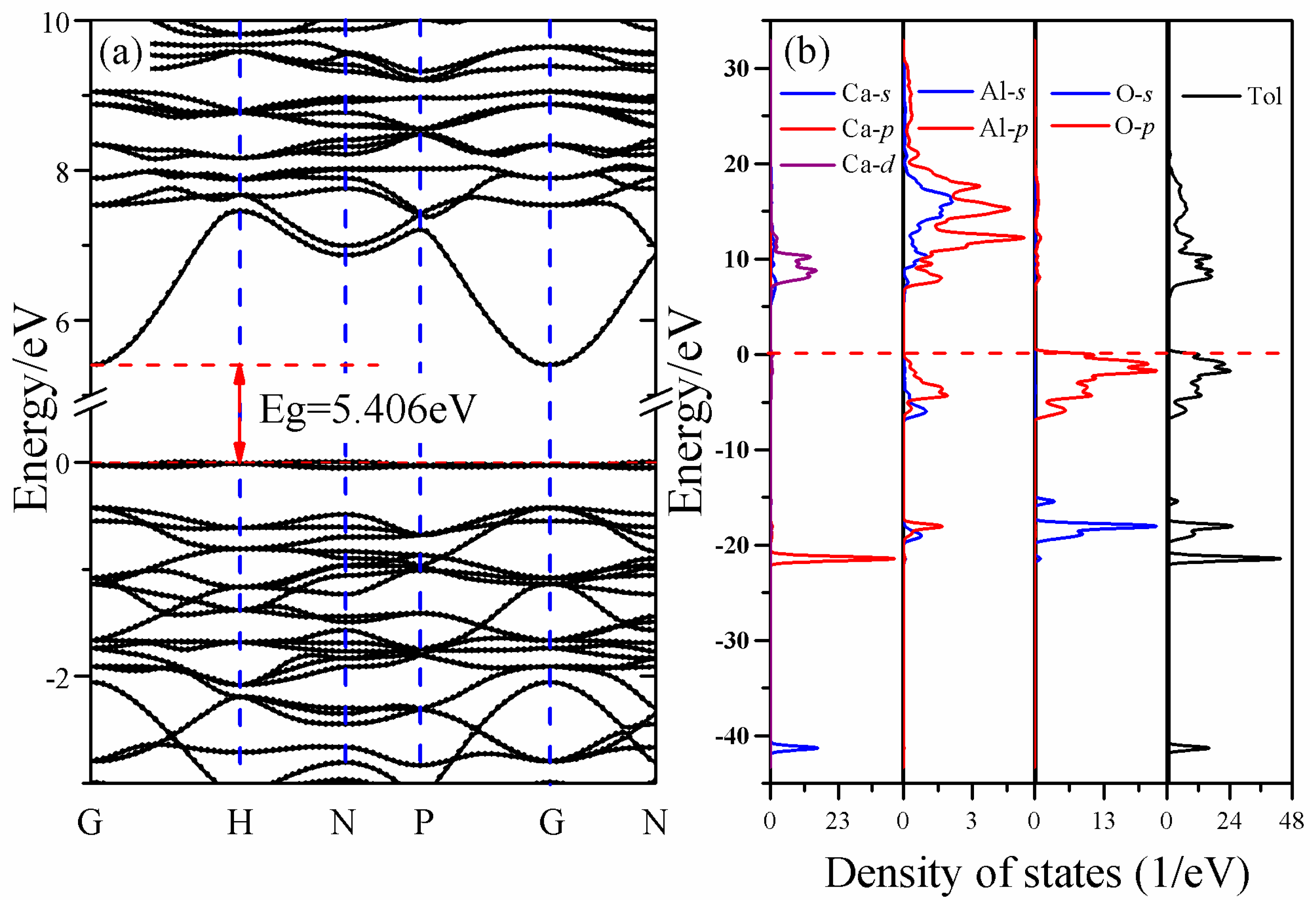
3.3. Electronic Structures
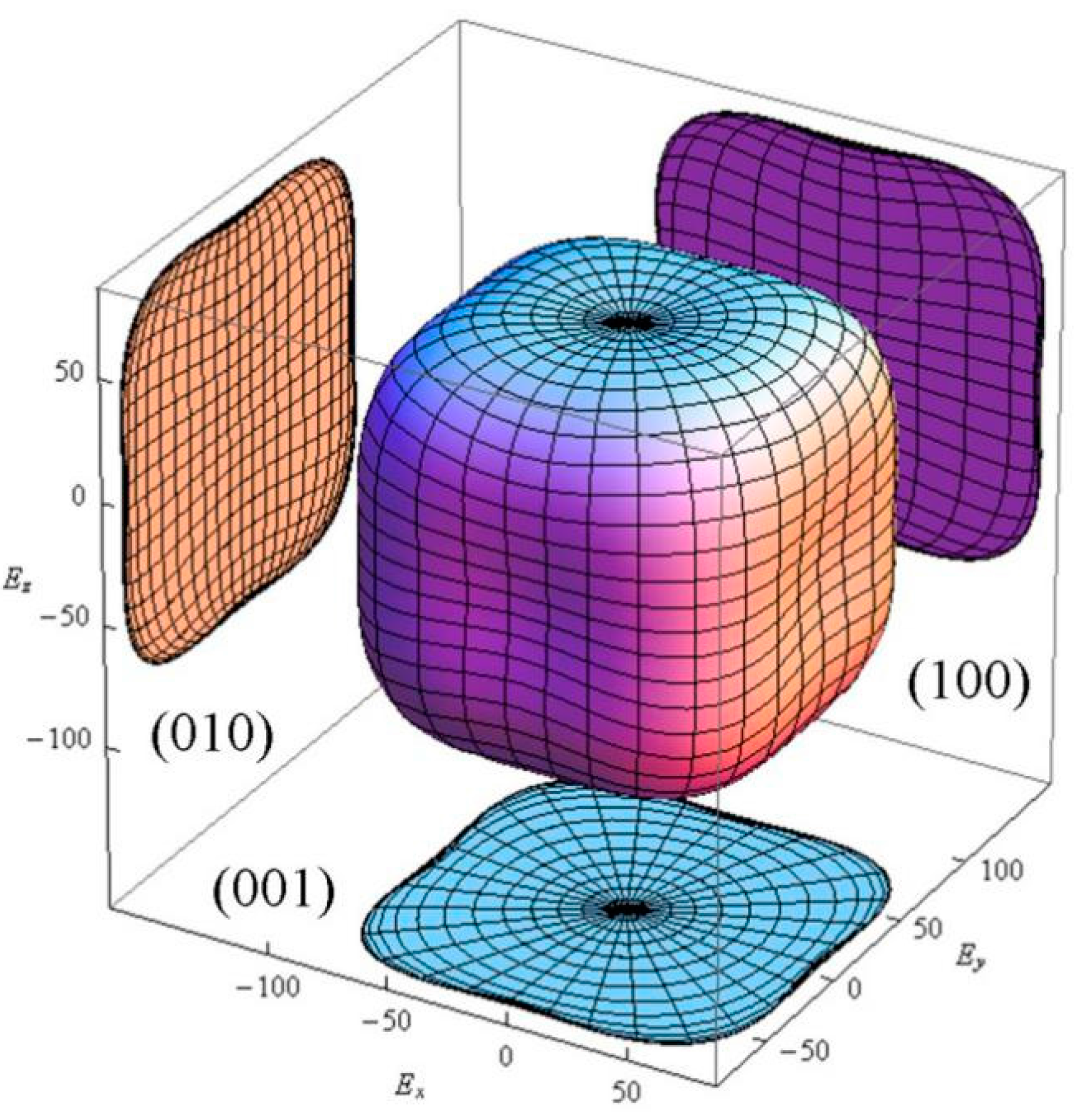
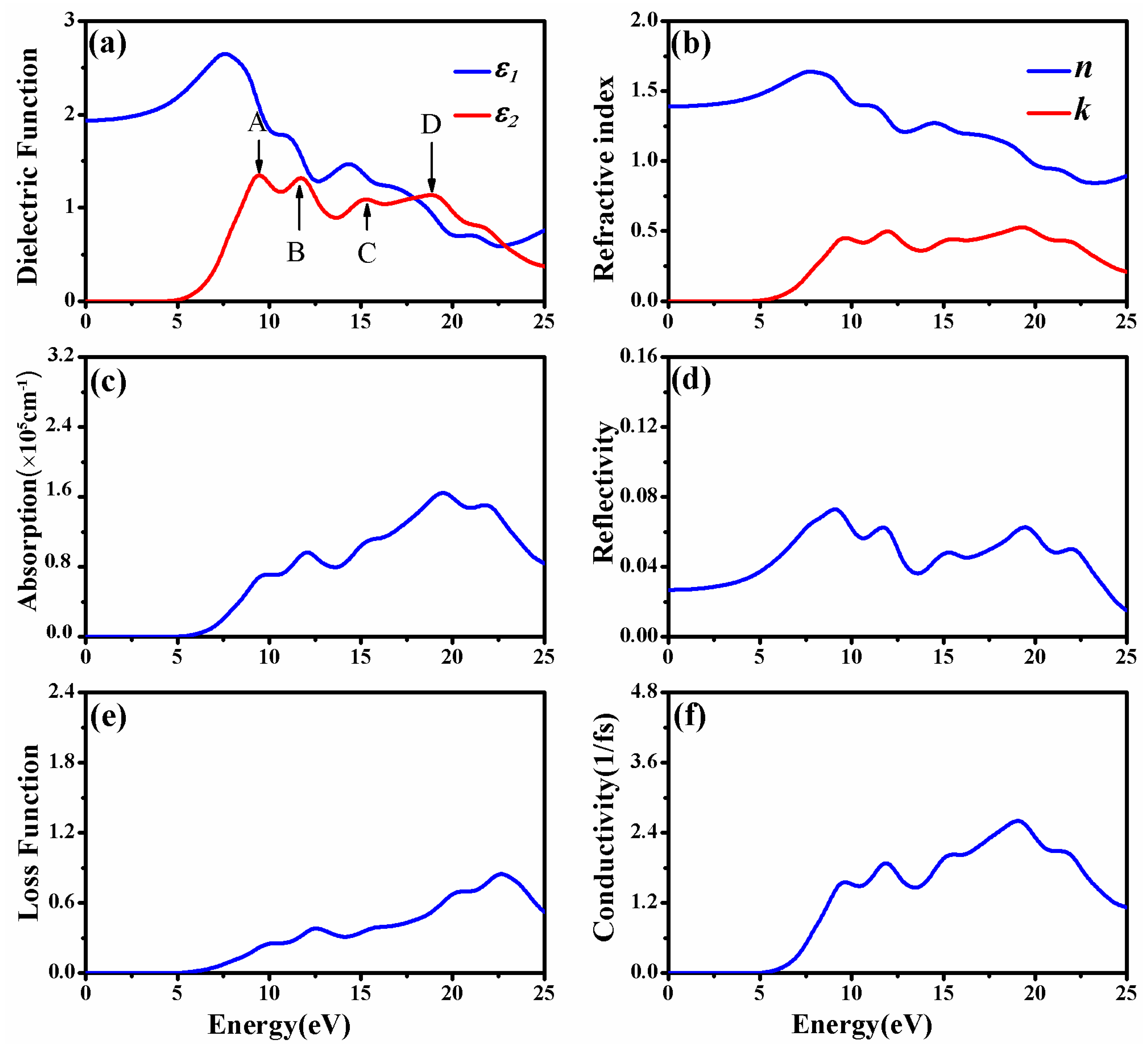
3.4. Optical Properties
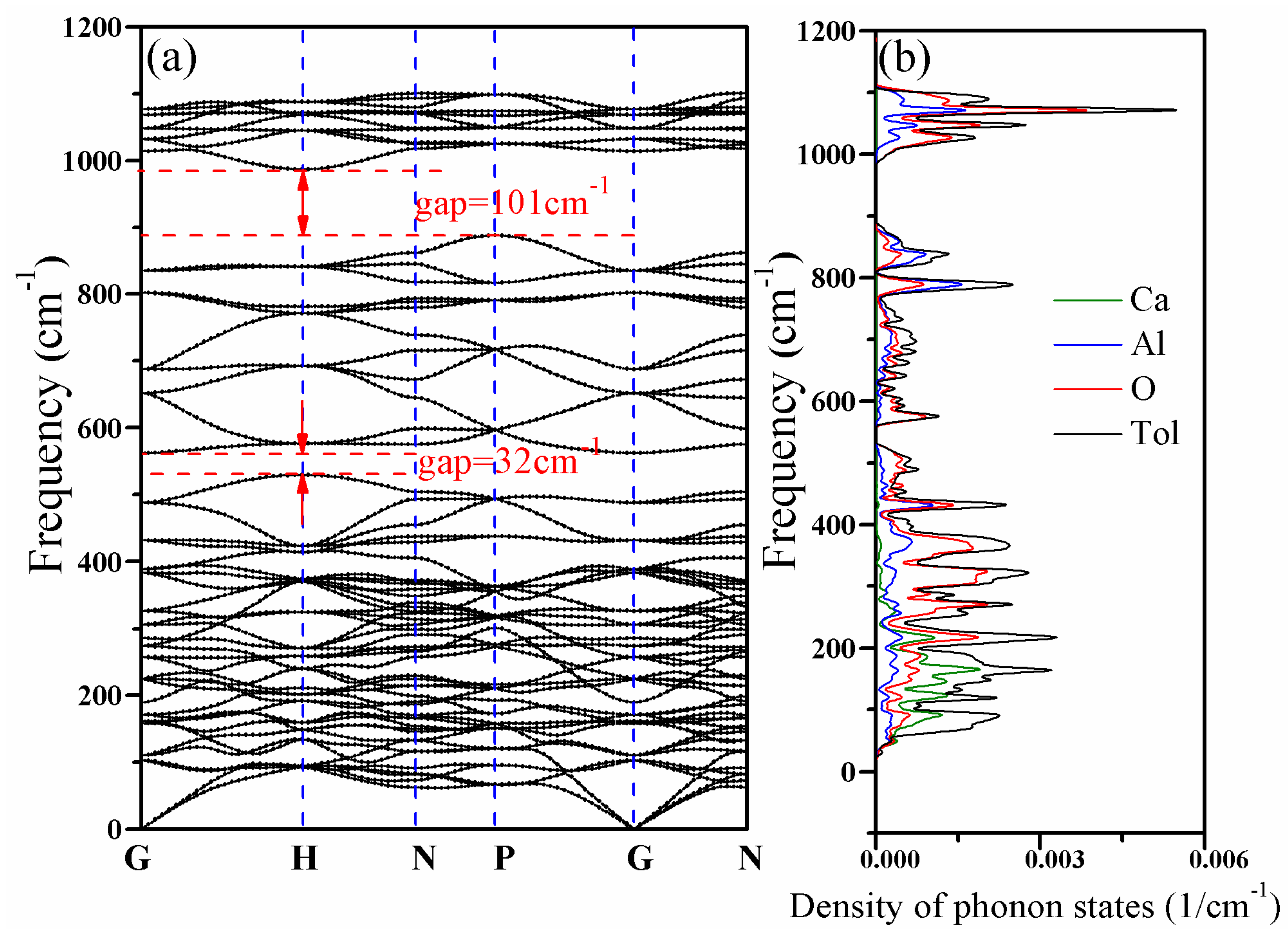
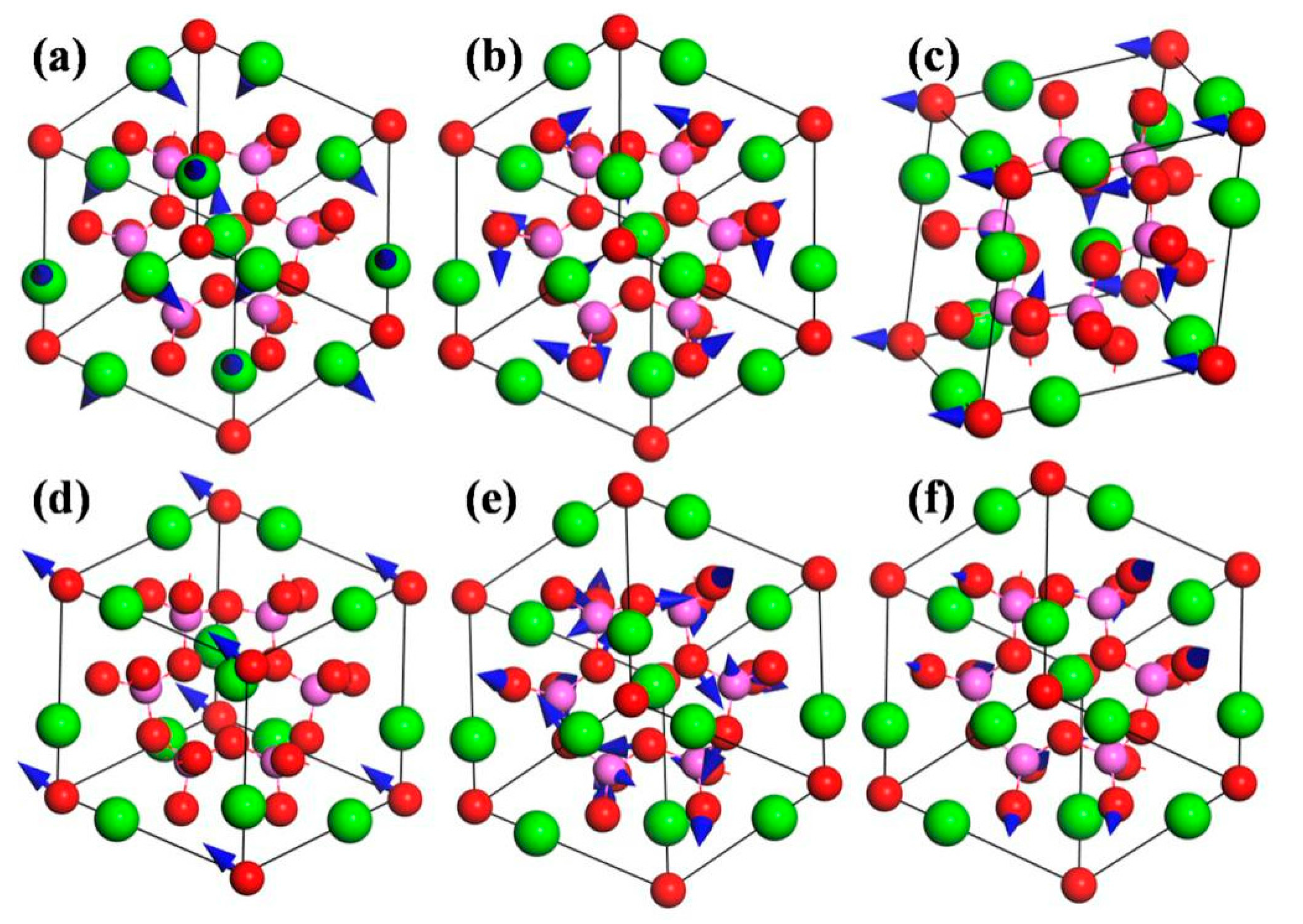
3.5. Phonon Spectra
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Torréns-Martín, D.; Fernández-Carrasco, L.; Martínez-Ramírez, S.; Ibáñez, J.; Artús, L.; Matschei, T. Raman Spectroscopy of Anhydrous and Hydrated Calcium Aluminates and Sulfoaluminates. J. Am. Ceram. Soc. 2013, 96, 3589–3595. [Google Scholar] [CrossRef]
- Migal, V.P.; Skurikhin, V.V.; Gershkovich, S.I.; Fedorova, O.S.; Rusakova, G.V.; Alekseev, P.E. High-alumina cembor cements for low-cement refractory concretes. Refract. Ind. Ceram. 2012, 53, 4–8. [Google Scholar] [CrossRef]
- Aitasallo, T.; Holsa, J.; Jungner, H.; Lastusaari, M.; Niittykoski, J. Thermoluminescence study of persistent luminescence materials: Eu2+- and R3+-doped calcium aluminates, CaAl2O4: Eu2+,R3+. J. Phys. Chem. B 2006, 110, 4589–4598. [Google Scholar] [CrossRef] [PubMed]
- Suzuki, Y.; Kondo, N.; Ohji, T. In situ synthesis and microstructure of porous CaAl4O7 monolith and CaAl4O7/CaZrO3 composite. J. Ceram. Soc. Jpn. 2001, 109, 205–209. [Google Scholar] [CrossRef]
- Yuan, X.; Xu, Y.B.; He, Y.Y. Synthesis of CaAl4O7 via citric acid precursor. J. Alloys Compd. 2007, 441, 251–254. [Google Scholar] [CrossRef]
- Kumar, A.S.; Kumar, R.A.; Bhattacharjee, R.R. Synthesis and optical characterization of Tm3+ doped CaAl4O7 for near-UV LED-based white light. J. Lumines. 2017, 182, 130–136. [Google Scholar] [CrossRef]
- Chen, J.H.; Chen, H.Y.; Mi, W.J.; Cao, Z.; Li, B.; Liang, C.J. Substitution of Ba for Ca in the structure of CaAl12O19. J. Am. Ceram. Soc. 2017, 100, 413–418. [Google Scholar] [CrossRef]
- Liu, X.Y.; Yang, D.X.; Huang, Z.H.; Yi, S.; Ding, H.; Fang, M.H.; Zhang, S.W.; Liu, Y.G. Novel Synthesis Method and Characterization of Porous Calcium Hexa-Aluminate Ceramics. J. Am. Ceram. Soc. 2014, 97, 2702–2704. [Google Scholar] [CrossRef]
- Liu, W.N.; Chang, J.A. Novel synthesis method of Ca3Al2O6 using an ethanol solution technique. J. Ceram. Soc. Jpn. 2010, 118, 617–619. [Google Scholar] [CrossRef][Green Version]
- Sakakura, T.; Tanaka, K.; Takenaka, Y.; Matsuishi, S.; Hosono, H.; Kishimoto, S. Determination of the local structure of a cage with an oxygen ion in Ca12Al14O33. Acta Crystallogr. Sect. B-Struct. Sci. 2011, 67, 193–204. [Google Scholar] [CrossRef] [PubMed]
- Kajihara, K.; Matsuishi, S.; Hayashi, K.; Hirano, M.; Hosono, H. Vibrational dynamics and oxygen diffusion in a nanoporous oxide ion conductor 12CaO center dot 7Al2O3 studied by 18O labeling and micro-Raman spectroscopy. J. Phys. Chem. C 2007, 111, 14855–14861. [Google Scholar] [CrossRef]
- Kahlenberg, V.; Fischer, R.X.; Shaw, C.S.J. Rietveld analysis of dicalcium aluminate (Ca2Al2O5)—A new high pressure phase with the brownmillerite-type structure. Am. Miner. 2000, 85, 1061–1065. [Google Scholar] [CrossRef]
- Misra, S.K.; Andronenko, S.I. Single-crystal EPR of the Eu2+ ion in pentacalcium-oxide trialuminate, 5CaO·3Al2O3. J. Phys. Chem. Solids 2000, 61, 1913–1917. [Google Scholar] [CrossRef]
- Kahlenberg, V.; Fischer, R.X.; Shaw, C.S.J. High-pressure Ca4Al6O13: An example of a calcium aluminate with three different types of coordination polyhedra for aluminum. Am. Miner. 2000, 85, 1492–1496. [Google Scholar] [CrossRef]
- Peters, L.; Knorr, K.; Evans, J.S.O.; Senyshyn, A.; Rahmoun, N.S.; Depmeier, W. Proton positions in and thermal behaviour of the phase 4CaO·3Al2O3·3H2O and its thermal decomposition to |(OCa4)2|[Al12O24]-SOD, determined by neutron/X-ray powder diffraction and IR spectroscopic investigations. Z. Krist. 2007, 222, 365–375. [Google Scholar] [CrossRef]
- Yuan, X.; Xu, Y.B.; He, Y.Y. Synthesis of Ca3Al2O6 via citric acid precursor. Mater. Sci. Eng. A-Struct. Mater. Prop. Microstruct. Process. 2007, 447, 142–145. [Google Scholar] [CrossRef]
- Jiang, X.X.; Molokeev, M.S.; Gong, P.F.; Yang, Y.; Wang, W.; Wang, S.H.; Wu, S.F.; Wang, Y.X.; Huang, R.J.; Li, L.F.; et al. Near-Zero Thermal Expansion and High Ultraviolet Transparency in a Borate Crystal of Zn4B6O13. Adv. Mater. 2016, 28, 7936–7940. [Google Scholar] [CrossRef] [PubMed]
- Segall, M.D.; Lindan, P.J.D.; Probert, M.J.; Pickard, C.J.; Hasnip, P.J.; Clark, S.J.; Payne, M.C. First-principles simulation: Ideas, illustrations and the CASTEP code. J. Phys.-Condes. Matter 2002, 14, 2717–2744. [Google Scholar] [CrossRef]
- Lavrentyev, A.A.; Gabrelian, B.V.; Vu, V.T.; Ananchenko, L.N.; Isaenko, L.I.; Yelisseyev, A.P.; Khyzhun, O.Y. Electronic structure and optical properties of noncentrosymmetric LiGaSe2: Experimental measurements and DFT band structure calculations. Opt. Mater. 2017, 66, 149–159. [Google Scholar] [CrossRef]
- Garza, A.J.; Scuseria, G.E. Predicting Band Gaps with Hybrid Density Functionals. J. Phys. Chem. Lett. 2016, 20, 4165–4170. [Google Scholar] [CrossRef] [PubMed]
- Piasecki, M.; Brik, M.G.; Barchiy, I.E.; Ozga, K.; Kityk, I.V.; El-Naggar, A.M.; Albassam, A.A.; Malakhovskaya, T.A.; Lakshminarayana, G. Band structure, electronic and optical features of Tl4SnX3 (X = S, Te) ternary compounds for optoelectronic applications. J. Alloys Compd. 2017, 710, 600–607. [Google Scholar] [CrossRef]
- Wu, Z.-J.; Zhao, E.-J.; Xiang, H.-P.; Hao, X.-F.; Liu, X.-J.; Meng, J. Crystal structures and elastic properties of superhard IrN2 and IrN3 from first principles. Phys. Rev. B 2007, 76, 054115. [Google Scholar] [CrossRef]
- Zahedi, E.; Xiao, B. DFT study of structural, elastic properties and thermodynamic parameters of Bi2S3 under hydrostatic pressures. Comput. Mater. Sci. 2015, 101, 301–312. [Google Scholar] [CrossRef]
- Vaitheeswaran, G.; Kanchana, V.; Svane, A.; Delin, A. Elastic properties of MgCNi3—A superconducting perovskite. J. Phys. Condens. Matter 2007, 19, 326214. [Google Scholar] [CrossRef]
- Manzano, H.; Dolado, J.S.; Ayuela, A. Structural, mechanical, and reactivity properties of tricalcium Aluminate using first-principles calculations. J. Am. Ceram. Soc. 2009, 92, 897–902. [Google Scholar] [CrossRef]
- Chen, W.-H.; Cheng, H.-C.; Yu, C.-F. The mechanical, thermodynamic, and electronic properties of cubic Au4Al crystal via first-principles calculations. J. Alloy. Compd. 2016, 689, 857–864. [Google Scholar] [CrossRef]
- Hussain, A.; Mehmood, S.; Rasool, M.N.; Aryal, S.; Rulis, P.; Ching, W.Y. Electronic structure, mechanical, and optical properties of CaO·Al2O3 system: A first principles approach. Indian J. Phys. 2016, 90, 917–929. [Google Scholar] [CrossRef]
- Qu, B.Y.; Zhang, B.; Wang, L.; Zhou, R.L.; Zeng, X.C. Mechanistic Study of the Persistent Luminescence of CaAl2O4:Eu,Nd. Chem. Mater. 2015, 27, 2195–2202. [Google Scholar] [CrossRef]
- Chao, L.M.; Bao, L.H.; Wei, W.; Tegus, O. Optical properties of Yb-doped LaB6 front first-principles calculation. Mod. Phys. Lett. B 2016, 30, 7. [Google Scholar] [CrossRef]
- Tian, J.H.; Song, T.; Sun, X.W.; Wang, T.; Jiang, G. First-Principles Study on the Half-Metallic Ferromagnetism and Optical Properties of Fe-Doped CdSe and Co-Doped CdSe. J. Supercond. Nov. Magn. 2017, 30, 521–528. [Google Scholar] [CrossRef]
- Duan, Y.; Sun, Y. First-principles calculations of optical properties of Mg2Pb. Sci. China Phys. Mech. Astron. 2014, 57, 233–238. [Google Scholar] [CrossRef]
- Deligoz, E.; Colakoglu, K.; Ozisik, H.; Cifti, Y.O. The first principles investigation of lattice dynamical and thermodynamical properties of Al2Ca and Al2Mg compounds in the cubic Laves structure. Comput. Mater. Sci. 2013, 68, 27–31. [Google Scholar] [CrossRef]
- Shi, L.; Hu, J.; Qin, Y.; Duan, Y.; Wu, L.; Yang, X.; Tang, G. First-principles study of structural, elastic and lattice dynamical properties of chalcopyrite BeSiV2 and MgSiV2 (V=P, As, Sb). J. Alloys Compd. 2014, 611 (Suppl. C), 210–218. [Google Scholar] [CrossRef]

| 93.18 | 93.18 | 93.18 | 36.25 | 35.05 | 35.65 | 77.53 | 0.33 |
| Modes | Frequencies and Atoms Involved | ||||
|---|---|---|---|---|---|
| T2(R+IR) | 132 (Ca, Al, O) | 197 (Ca, Al, O) | 246 (Ca, O) | 306 (O) | 337 (O) |
| 385 (Al, O) | 428 (Al, O) | 801 (Al, O) | 835 (Al, O) | 1046 (Al, O) | |
| T1(S) | 108 (Ca, Al, O) | 177 (Ca, Al, O) | 263 (Al, O) | 385 (Al, O) | 651(Al, O) |
| 1089 (Al, O) | 1075 (Al, O) | - | - | - | |
| E(R) | 180 (Ca) | 262 (Al, O) | 492 (Al, O) | 686 (Al, O) | 1030 (Al, O) |
| A2(S) | 183 (Al, O) | 1012 (Al, O) | - | - | - |
| A1(R) | 270 (Ca, O) | 284 (Ca, O) | 565 (O) | - | - |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mei, H.; Zhong, Y.; Wang, P.; Jia, Z.; Li, C.; Cheng, N. Electronic, Optical, and Lattice Dynamical Properties of Tetracalcium Trialuminate (Ca4Al6O13). Materials 2018, 11, 449. https://doi.org/10.3390/ma11030449
Mei H, Zhong Y, Wang P, Jia Z, Li C, Cheng N. Electronic, Optical, and Lattice Dynamical Properties of Tetracalcium Trialuminate (Ca4Al6O13). Materials. 2018; 11(3):449. https://doi.org/10.3390/ma11030449
Chicago/Turabian StyleMei, Huayue, Yuhan Zhong, Peida Wang, Zhenyuan Jia, Chunmei Li, and Nanpu Cheng. 2018. "Electronic, Optical, and Lattice Dynamical Properties of Tetracalcium Trialuminate (Ca4Al6O13)" Materials 11, no. 3: 449. https://doi.org/10.3390/ma11030449
APA StyleMei, H., Zhong, Y., Wang, P., Jia, Z., Li, C., & Cheng, N. (2018). Electronic, Optical, and Lattice Dynamical Properties of Tetracalcium Trialuminate (Ca4Al6O13). Materials, 11(3), 449. https://doi.org/10.3390/ma11030449