Fabrication of Stretchable Circuits on Polydimethylsiloxane (PDMS) Pre-Stretched Substrates by Inkjet Printing Silver Nanoparticles
Abstract
1. Introduction
2. Materials and Equipment
2.1. Elastomer Substrates
2.2. Inkjet Printing
3. Methodology
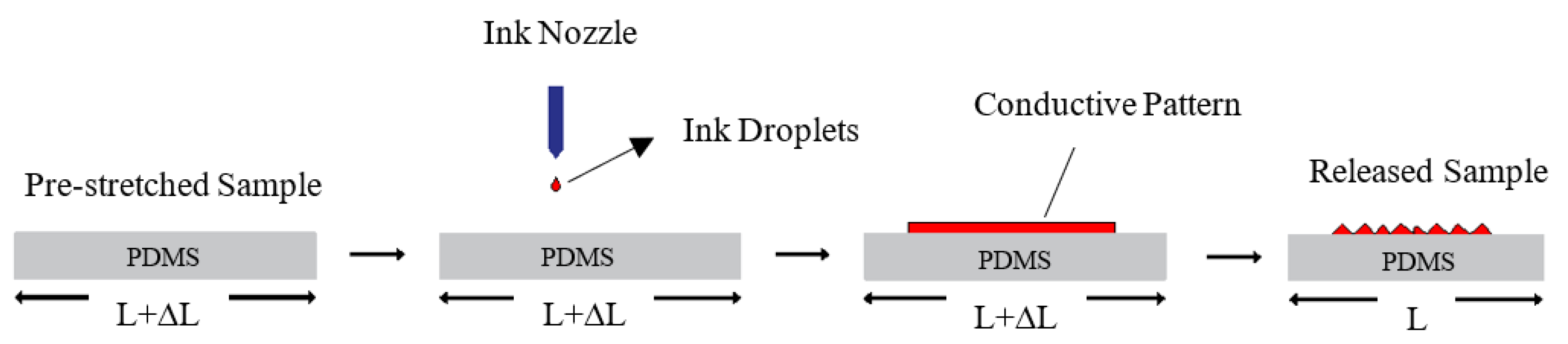
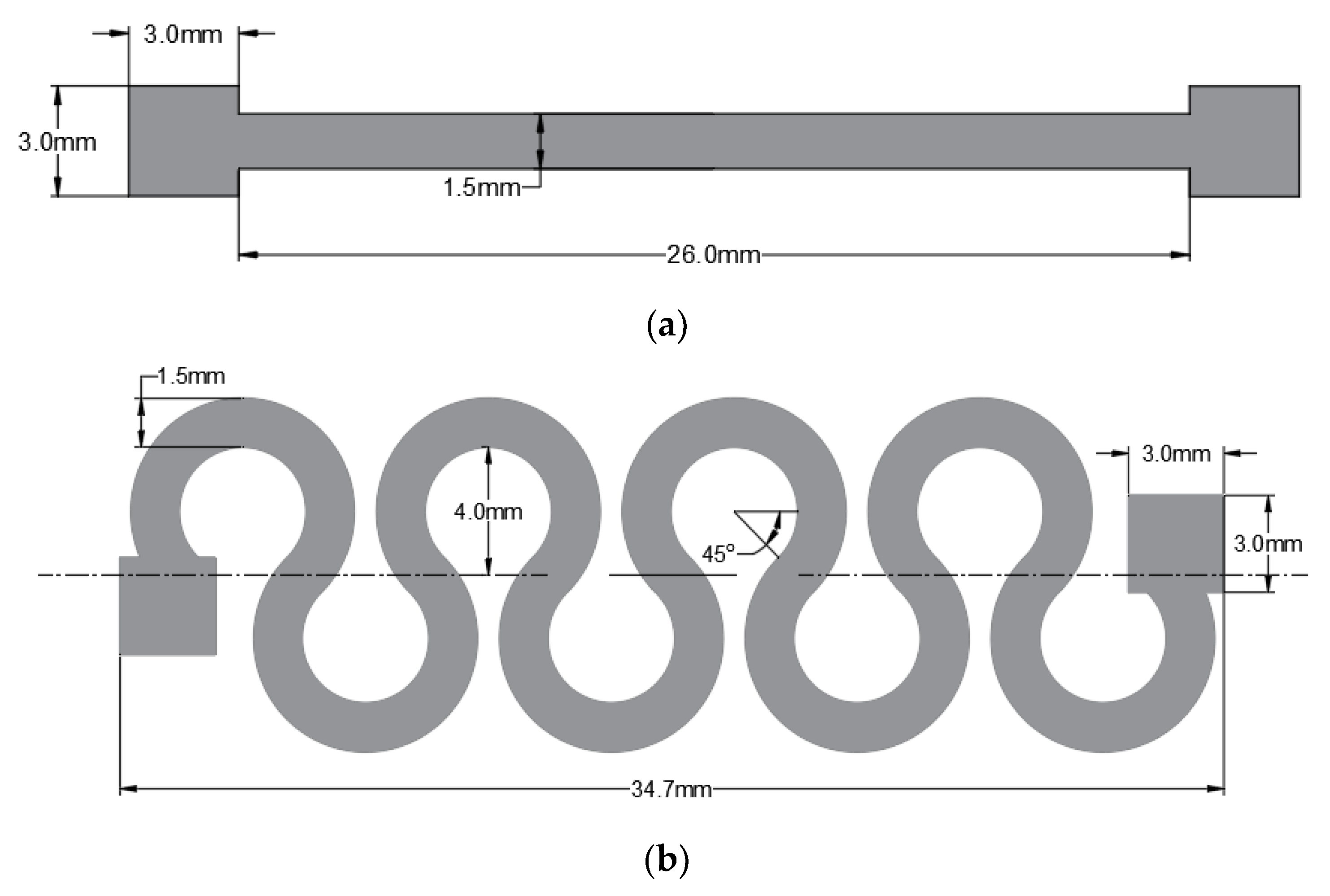
3.1. Conductive Patterns
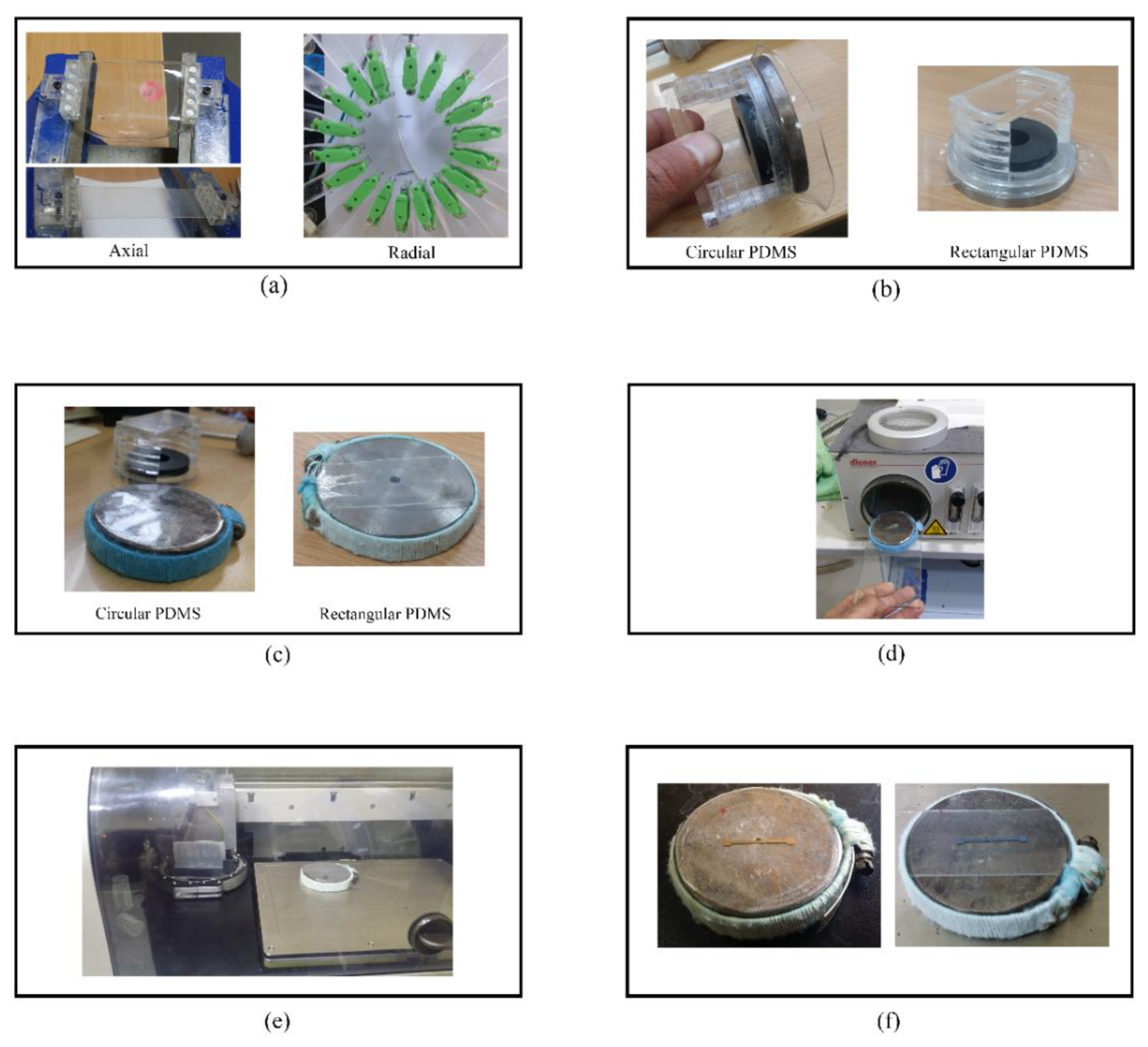
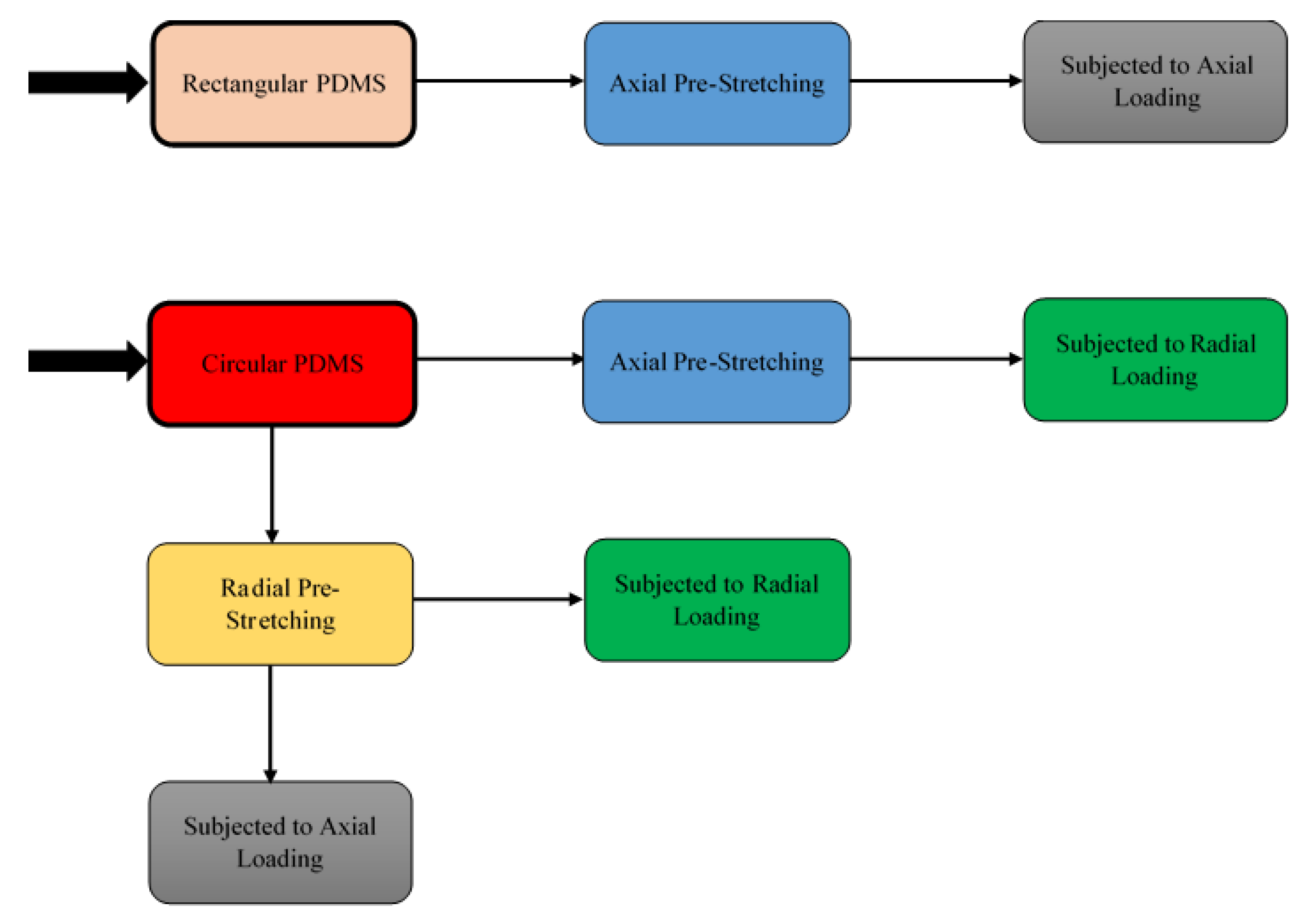
3.2. Pre-Stretching of PDMS Samples
3.3. Tests Performed
4. Results and Discussion
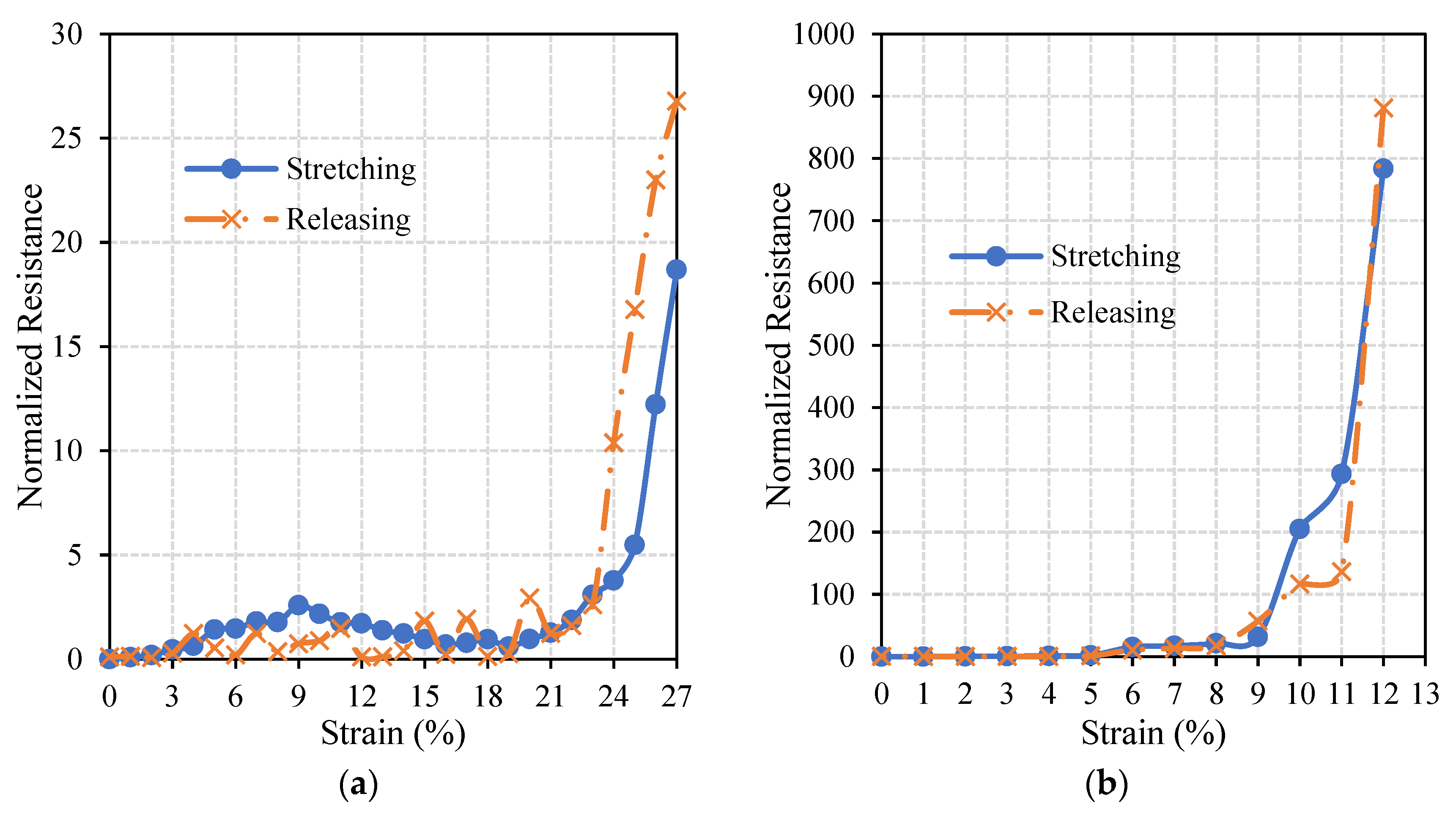
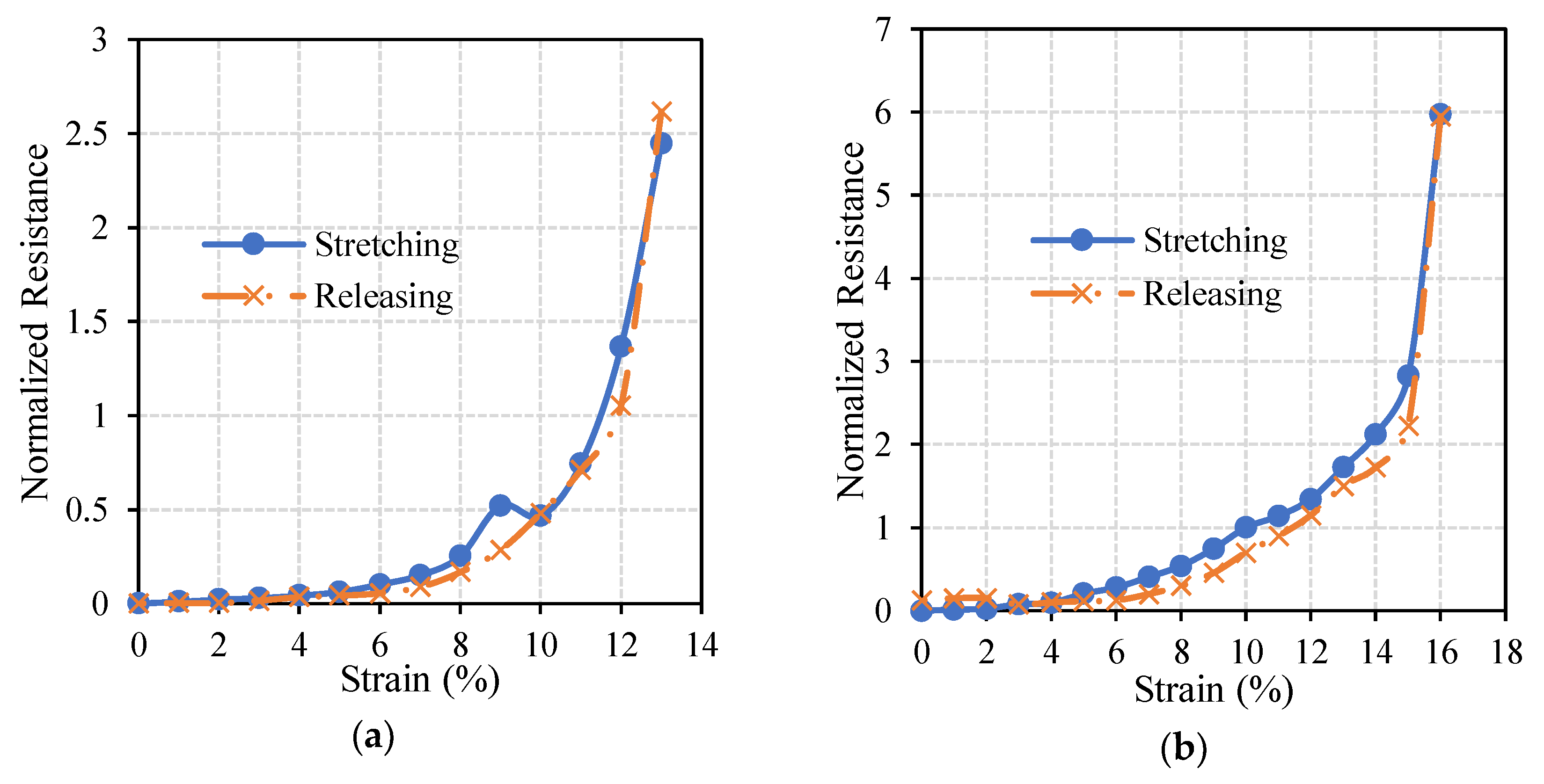
4.1. Straight Conductive Lines
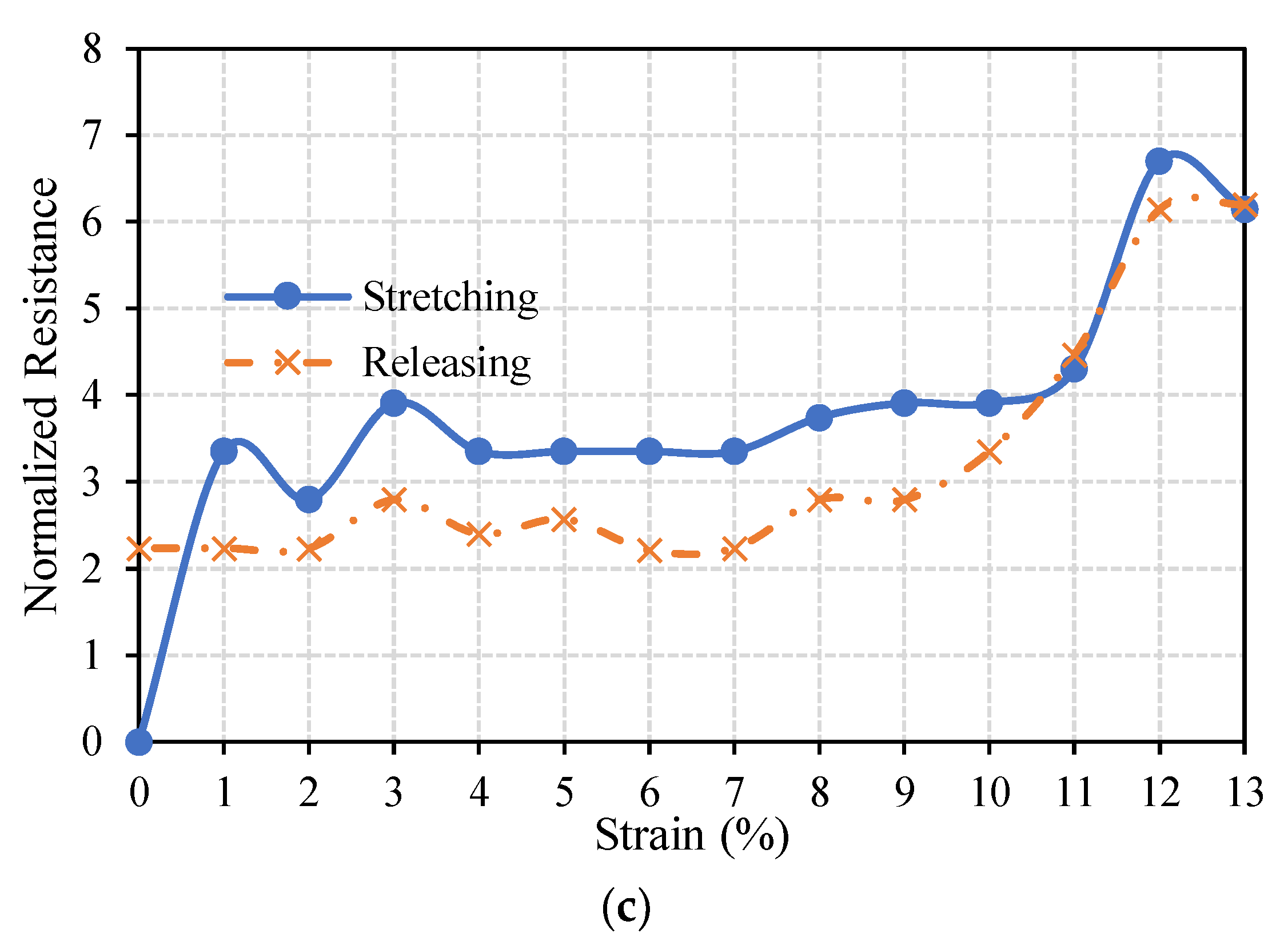
4.2. Conductive Horseshoe Patterns
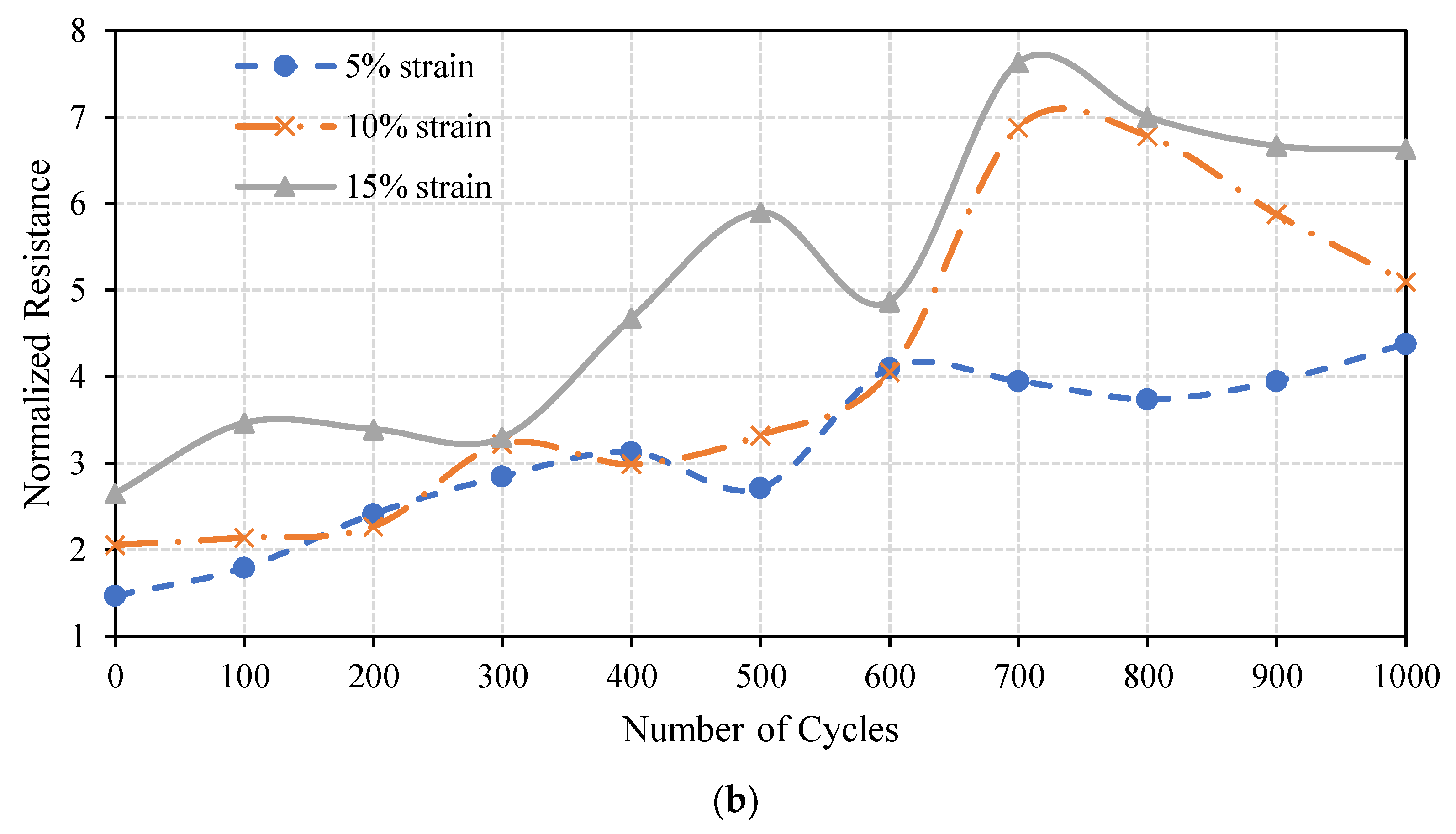
4.3. Radial Cyclic Test
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Robinson, A.; Aziz, A.; Liu, Q.; Suo, Z.; Lacour, S.P. Hybrid stretchable circuits on silicone substrate. J. Appl. Phys. 2014, 115, 15–20. [Google Scholar] [CrossRef]
- Sun, J.; Jiang, J.; Bao, B.; Wang, S.; He, M.; Zhang, X.; Song, Y. Fabrication of bendable circuits on a polydimethylsiloxane (PDMS) surface by inkjet printing semi-wrapped structures. Materials 2016, 9, 253. [Google Scholar] [CrossRef] [PubMed]
- Dickey, M.D. Stretchable and soft electronics using liquid metals. Adv. Mater. 2017, 29, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Rogers, J.A.; Someya, T.; Huang, Y. Materials and mechanics for stretchable electronics. Science 2010, 327, 1603–1607. [Google Scholar] [CrossRef] [PubMed]
- Larmagnac, A.; Eggenberger, S.; Janossy, H.; Vörös, J. Stretchable electronics based on Ag-PDMS composites. Sci. Rep. 2014, 4, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Adrega, T.; Lacour, S.P. Stretchable gold conductors embedded in PDMS and patterned by photolithography: Fabrication and electromechanical characterization. J. Micromech. Microeng. 2010, 20. [Google Scholar] [CrossRef]
- Liimatta, T.; Halonen, E.; Sillanpaa, H.; Niittynen, J.; Mantysalo, M. Inkjet printing in manufacturing of stretchable interconnects. Proc. Electron. Compon. Technol. Conf. 2014, 151–156. [Google Scholar] [CrossRef]
- Abu-Khalaf, J.M.; Park, J.W.; Mascaro, D.J.; Mascaro, S.A. Stretchable fingernail sensors for measurement of fingertip force. In Proceedings of the World Haptics 2009—Third Joint EuroHaptics conference and Symposium on Haptic Interfaces for Virtual Environment and Teleoperator Systems, Salt Lake City, UT, USA, 18–20 March 2009; pp. 625–626. [Google Scholar] [CrossRef]
- Jiang, J.; Bao, B.; Li, M.; Sun, J.; Zhang, C.; Li, Y.; Li, F.; Yao, X.; Song, Y. Fabrication of transparent multilayer circuits by inkjet printing. Adv. Mater. 2016, 28, 1420–1426. [Google Scholar] [CrossRef] [PubMed]
- Huang, Q.; Al-Milaji, K.N.; Zhao, H. Inkjet printing of silver nanowires for stretchable heaters. ACS Appl. Nano Mater. 2018, 1, 4528–4536. [Google Scholar] [CrossRef]
- Kim, K.S.; Jung, K.H.; Jung, S.B. Design and fabrication of screen-printed silver circuits for stretchable electronics. Microelectron. Eng. 2014, 120, 216–220. [Google Scholar] [CrossRef]
- Liang, J.; Tong, K.; Pei, Q. A water-based silver-nanowire screen-print ink for the fabrication of stretchable conductors and wearable thin-film transistors. Adv. Mater. 2016, 28, 5986–5996. [Google Scholar] [CrossRef] [PubMed]
- Wu, J.; Wang, R.; Yu, H.; Li, G.; Xu, K.; Tien, N.C.; Roberts, R.C.; Li, D. Inkjet-printed microelectrodes on PDMS as biosensors for functionalized microfluidic systems. Lab Chip 2015, 15, 690–695. [Google Scholar] [CrossRef] [PubMed]
- Al-Halhouli, A.; Qitouqa, H.; Alashqar, A.; Abu-Khalaf, J. Inkjet printing for the fabrication of flexible/stretchable wearable electronic devices and sensors. Sens. Rev. 2018, 38, 438–452. [Google Scholar] [CrossRef]
- Yokus, M.A.; Foote, R.; Jur, J.S. Printed stretchable interconnects for smart garments: design, fabrication, and characterization. IEEE Sens. J. 2016, 16, 7967–7976. [Google Scholar] [CrossRef]
- Abu-Khalaf, J.; Saraireh, R.; Eisa, S.; Al-Halhouli, A. Experimental characterization of inkjet-printed stretchable circuits for wearable sensor applications. Sensors 2018, 18. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.H.; Rogers, J.A. Stretchable electronics: Materials strategies and devices. Adv. Mater. 2008, 20, 4887–4892. [Google Scholar] [CrossRef]
- Lacour, S.P.; Jones, J.; Suo, Z.; Wagner, S. Design and performance of thin metal film interconnects for skin-like electronic circuits. IEEE Electron Device Lett. 2004, 25, 179–181. [Google Scholar] [CrossRef]
- Hong, Y.; Lee, B.; Byun, J.; Oh, E.; Kim, H.; Kim, S.; Lee, S.; Kim, D.; Yoon, J. Key enabling technology for stretchable led display and electronic system. SID Symp. Dig. Tech. Pap. 2017, 48, 253–256. [Google Scholar] [CrossRef]
- Lee, J.; Chung, S.; Song, H.; Kim, S.; Hong, Y. Lateral-crack-free, buckled, inkjet-printed silver electrodes on highly pre-stretched elastomeric substrates. J. Phys. Appl. Phys. 2013, 46. [Google Scholar] [CrossRef]
- Gong, S.; Cheng, W. One-dimensional nanomaterials for soft electronics. Adv. Electron. Mater. 2017, 3. [Google Scholar] [CrossRef]
- Kim, K.; Kim, J.; Hyun, B.G.; Ji, S.; Kim, S.-Y.; Kim, S.; An, B.W.; Park, J.-U. Stretchable and transparent electrodes based on in-plane structures. Nanoscale 2015, 7, 14577–14594. [Google Scholar] [CrossRef] [PubMed]
- Gray, D.S.; Tien, J.; Chen, C.S. High-conductivity elastomeric electronics. Adv. Mater. 2004, 16, 393–397. [Google Scholar] [CrossRef]
- Xiao, J.; Carlson, A.; Liu, Z.J.; Huang, Y.; Jiang, H.; Rogers, J.A. Stretchable and compressible thin films of stiff materials on compliant wavy substrates. Appl. Phys. Lett. 2008, 93. [Google Scholar] [CrossRef]
- Cho, K.; Koh, J.; Kim, S.; Chu, W.; Hong, Y.; Ahn, S. Review of manufacturing processes for soft biomimetic robots. Int. J. Precis. Eng. Manuf. 2009. [Google Scholar] [CrossRef]
- Kim, Y.; Ren, X.; Kim, J.W.; Noh, H. Direct inkjet printing of micro-scale silver electrodes on polydimethylsiloxane (PDMS) microchip. J. Micromech. Microeng. 2014, 24. [Google Scholar] [CrossRef]
- Cummins, G.; Desmulliez, M.P.Y.; Cummins, G.; Marc, P.; Desmulliez, Y. Inkjet printing of conductive materials: A review. Circ. World 2013. [Google Scholar] [CrossRef]
- Vaithilingam, J.; Saleh, E.; Tuck, C.; Wildman, R.; Ashcroft, I.; Hague, R.; Dickens, P. 3D-inkjet Printing of Flexible and Stretchable Electronics. In Proceedings of the 26th Solid Freeform Fabrication Symposium, Austin, TX, USA, 10–12 August 2015; pp. 1513–1526. [Google Scholar]
- RADIAL STRETCHING SYSTEM|Scientific Open Source/Hardware Test Equipment. Available online: http://www.somap.jku.at/rss/ (accessed on 27 February 2018).
- Suikkola, J.; Björninen, T.; Mosallaei, M.; Kankkunen, T.; Iso-Ketola, P.; Ukkonen, L.; Vanhala, J.; Mäntysalo, M. Screen-printing fabrication and characterization of stretchable electronics. Sci. Rep. 2016, 6. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.; Tao, X.M. Three-dimensionally deformable, highly stretchable, permeable, durable and washable fabric circuit boards. Proc. R. Soc. Math. Phys. Eng. Sci. 2014, 470, 20140472. [Google Scholar] [CrossRef] [PubMed]


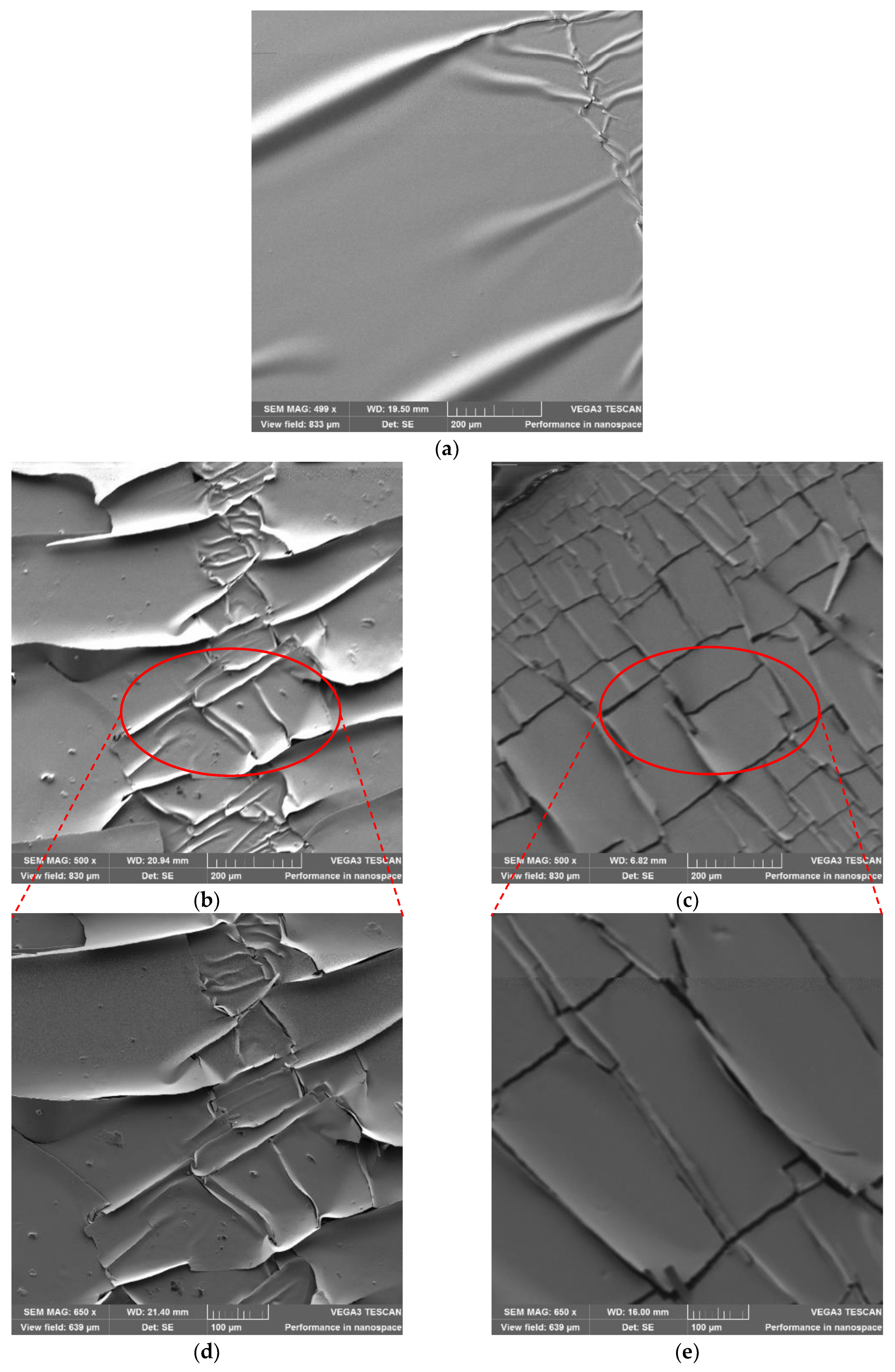


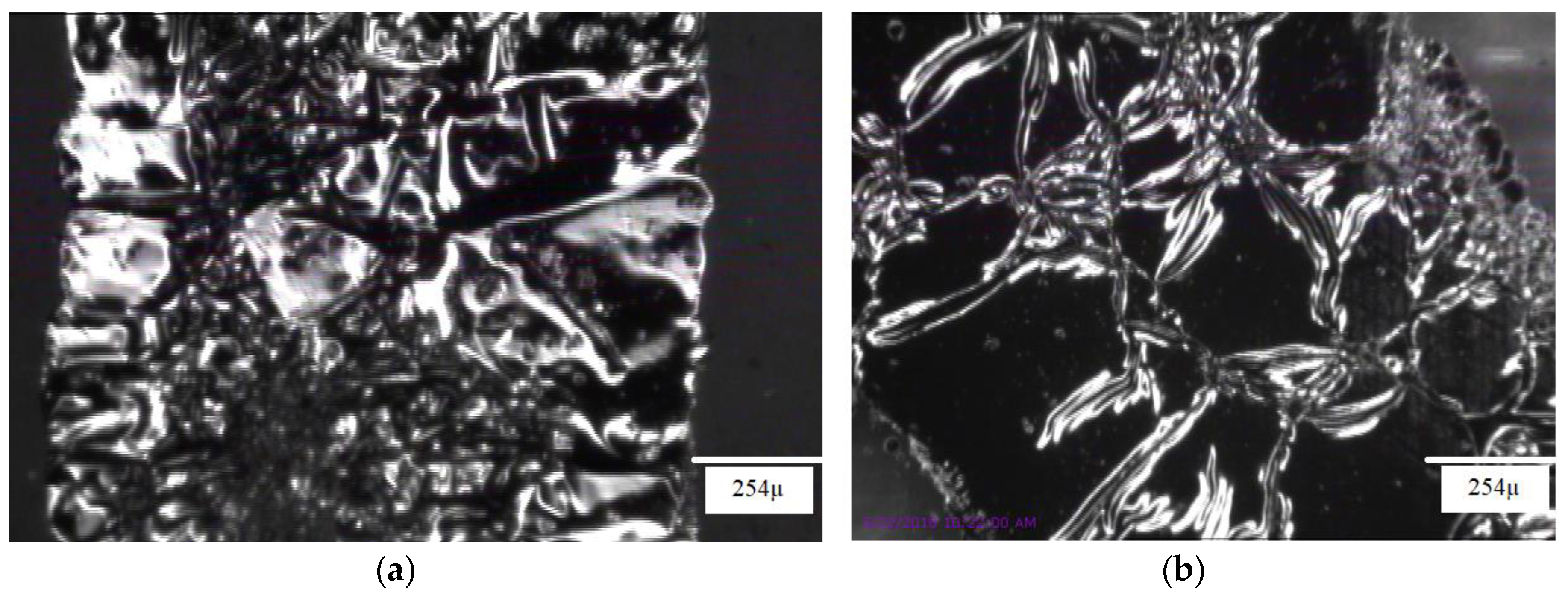

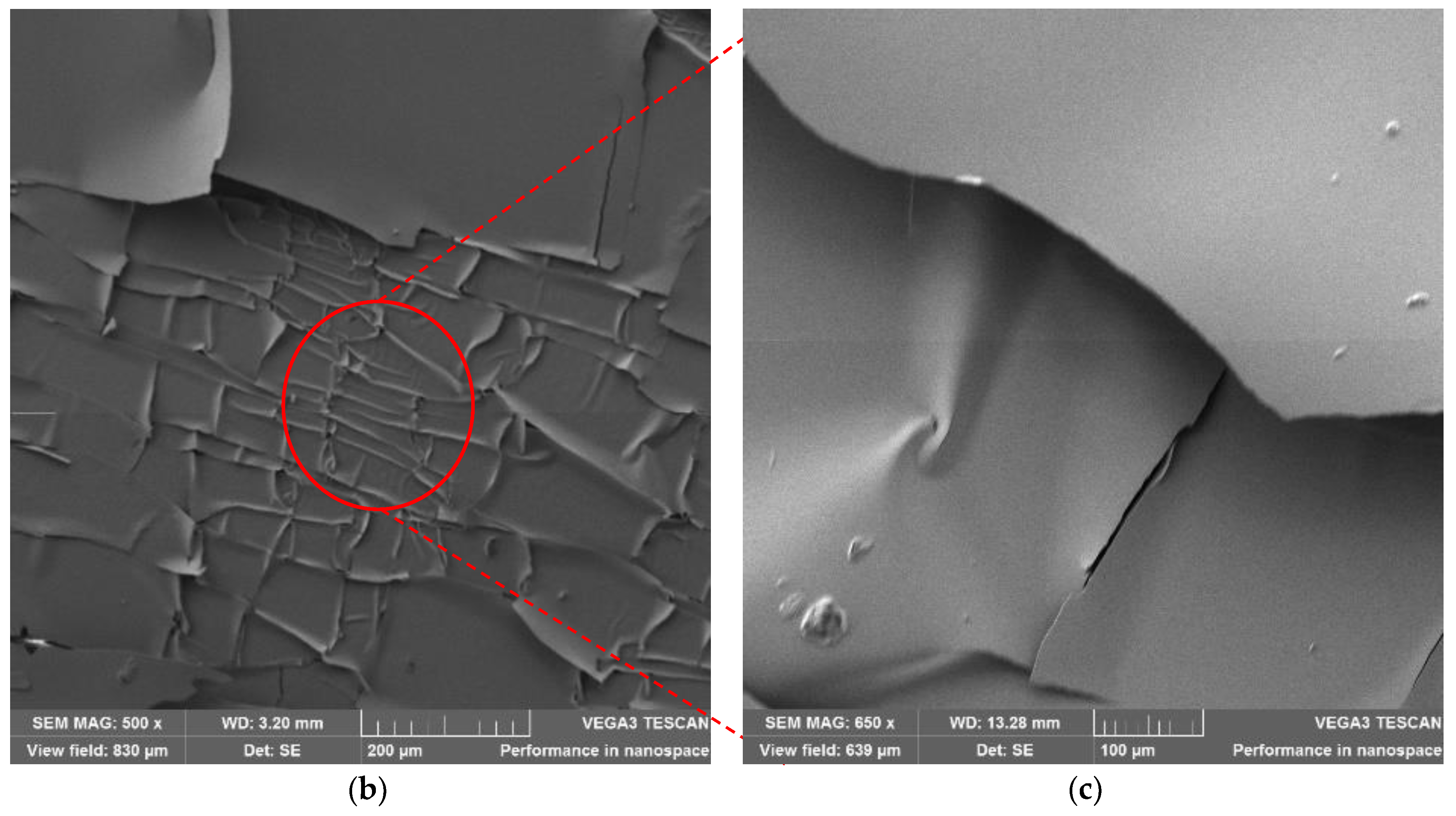

| Pre-Stretching Strain | 0% | 27% | ||||
|---|---|---|---|---|---|---|
| Number of Layers | 1 | 2 | 1 | 2 | ||
| Line Width | 1.5 mm | 2 mm | 1.5 mm | 1.5 mm | 2 mm | 1.5 mm |
| (Ohm) | 20 | 22 | 9.3 | 69.1 | 40 | 37 |
| (Ohm) | 98 | 380 | 14.5 | 698 | 279 | 84 |
| Average Breakdown Strain (%) | 4.5 | 9 | 4.5 | 13 | 16 | 13.5 |
| Pattern | Straight-Line | Horseshoe | ||||
|---|---|---|---|---|---|---|
| Cyclic Strain | 5% | 10% | 15% | 5% | 10% | 15% |
| (Ohm) | 21.96 | 29.13 | 38.54 | 50.26 | 67 | 68.9 |
| (Ohm) | 90.52 | 286.43 | 565.75 | 270.47 | 528.36 | 595.02 |
| (Ohm) | 43.83 | 219.05 | 119.13 | 101 | 141 | 200.47 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abu-Khalaf, J.M.; Al-Ghussain, L.; Al-Halhouli, A. Fabrication of Stretchable Circuits on Polydimethylsiloxane (PDMS) Pre-Stretched Substrates by Inkjet Printing Silver Nanoparticles. Materials 2018, 11, 2377. https://doi.org/10.3390/ma11122377
Abu-Khalaf JM, Al-Ghussain L, Al-Halhouli A. Fabrication of Stretchable Circuits on Polydimethylsiloxane (PDMS) Pre-Stretched Substrates by Inkjet Printing Silver Nanoparticles. Materials. 2018; 11(12):2377. https://doi.org/10.3390/ma11122377
Chicago/Turabian StyleAbu-Khalaf, Jumana M., Loiy Al-Ghussain, and Ala’aldeen Al-Halhouli. 2018. "Fabrication of Stretchable Circuits on Polydimethylsiloxane (PDMS) Pre-Stretched Substrates by Inkjet Printing Silver Nanoparticles" Materials 11, no. 12: 2377. https://doi.org/10.3390/ma11122377
APA StyleAbu-Khalaf, J. M., Al-Ghussain, L., & Al-Halhouli, A. (2018). Fabrication of Stretchable Circuits on Polydimethylsiloxane (PDMS) Pre-Stretched Substrates by Inkjet Printing Silver Nanoparticles. Materials, 11(12), 2377. https://doi.org/10.3390/ma11122377






