Combined Damage Index to Detect Plastic Deformation in Metals Using Acoustic Emission and Nonlinear Ultrasonics
Abstract
:1. Introduction
2. Materials and Test Procedures
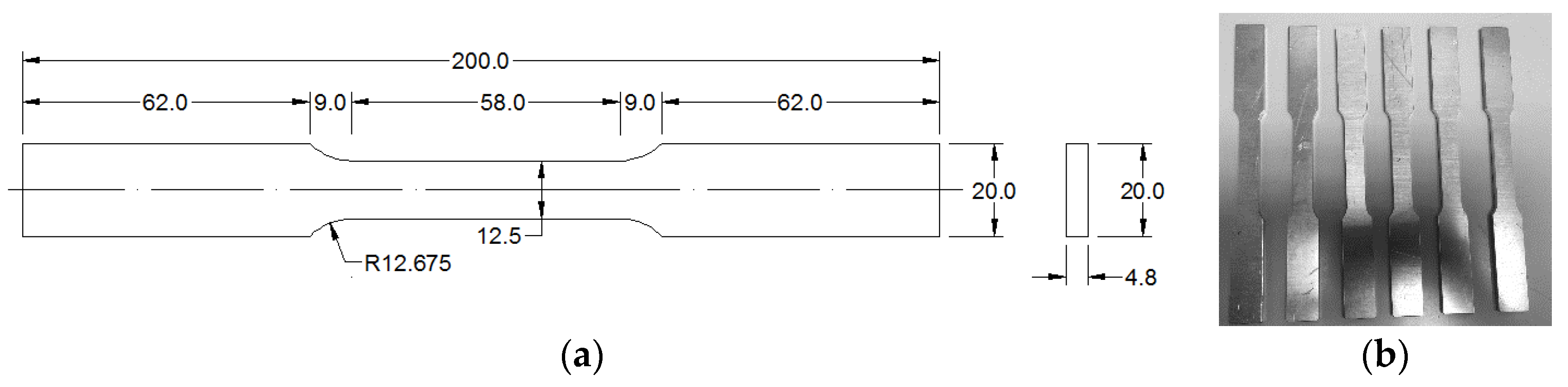
2.1. Description of Material and Samples
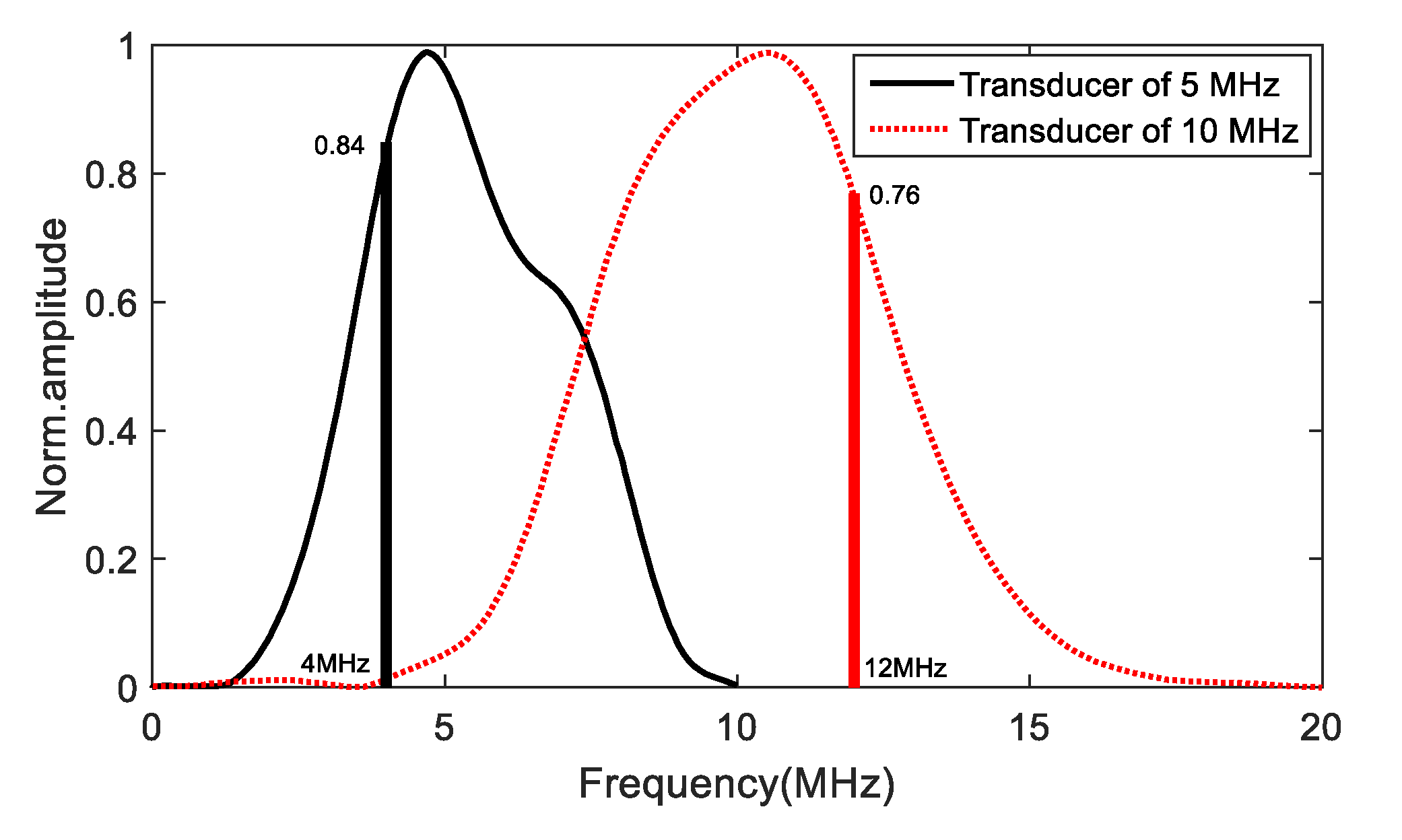
2.2. Experimental Setup
3. Results and Discussion
3.1. Characteristics of Mechanical Behaviors
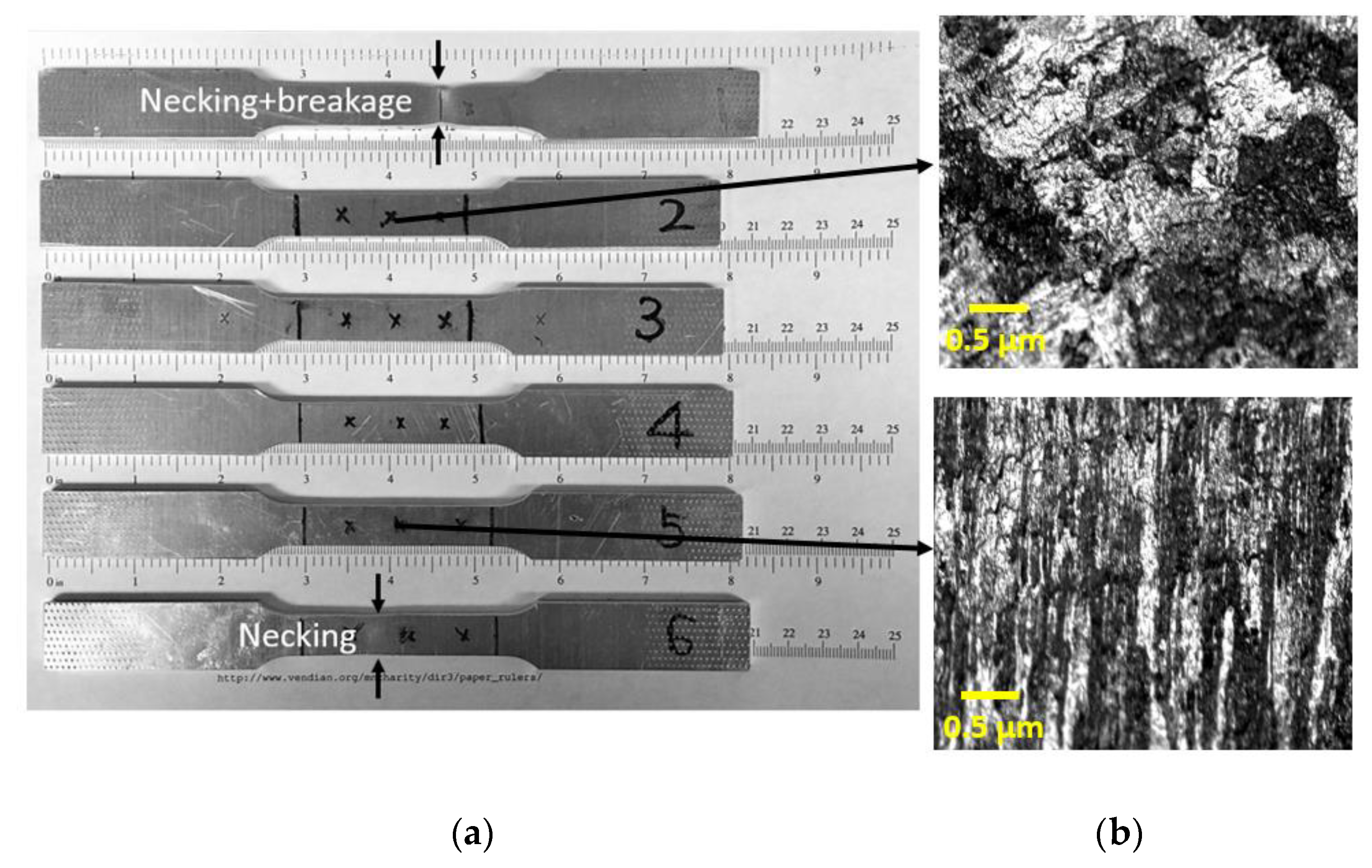
3.1.1. Tensile Testing
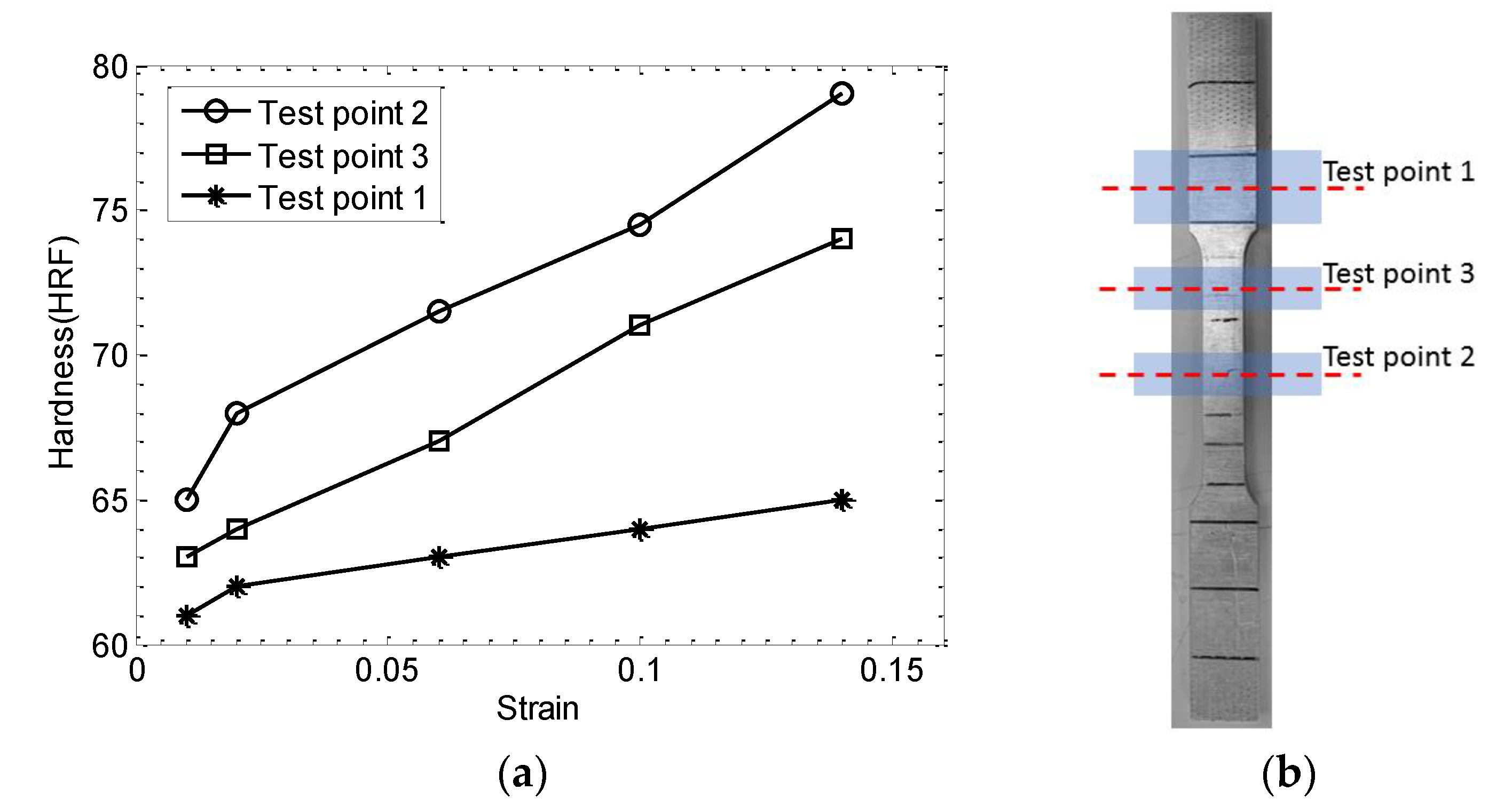
3.1.2. Hardness Testing
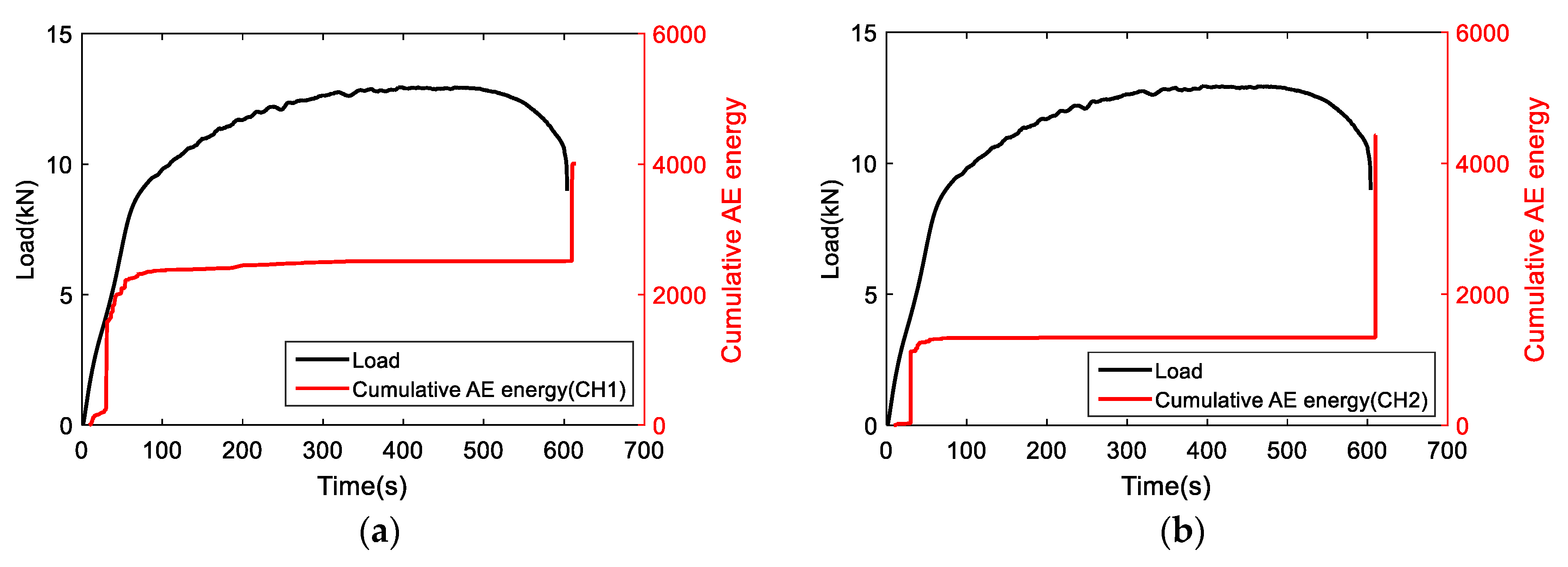
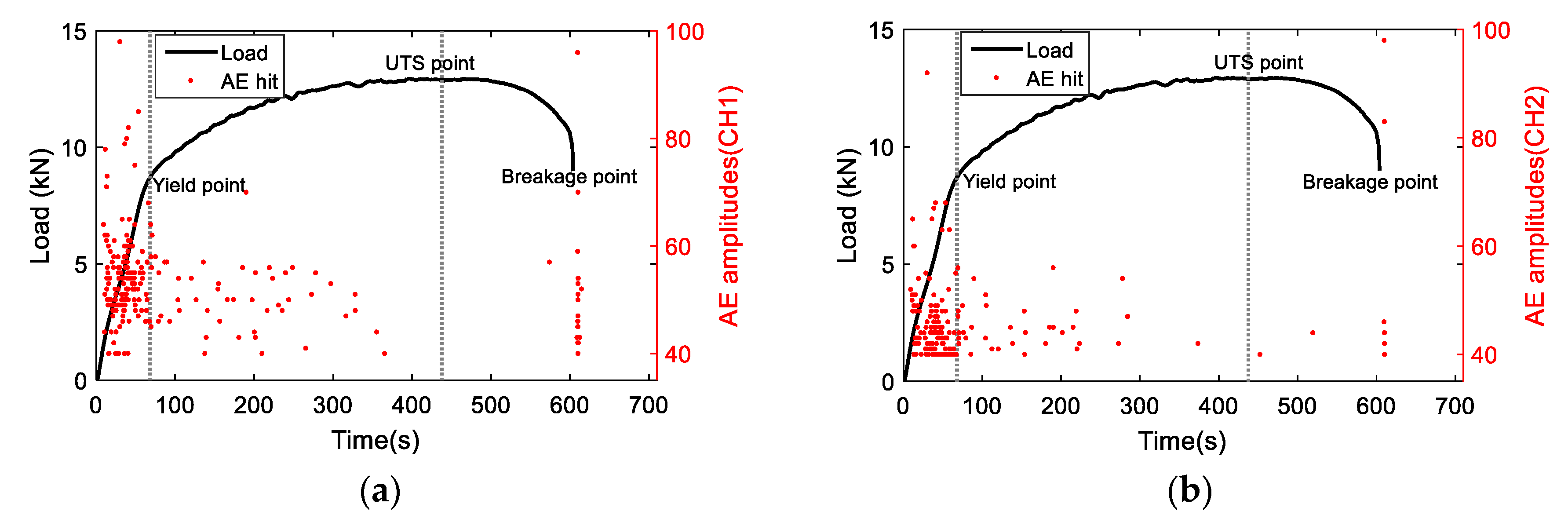
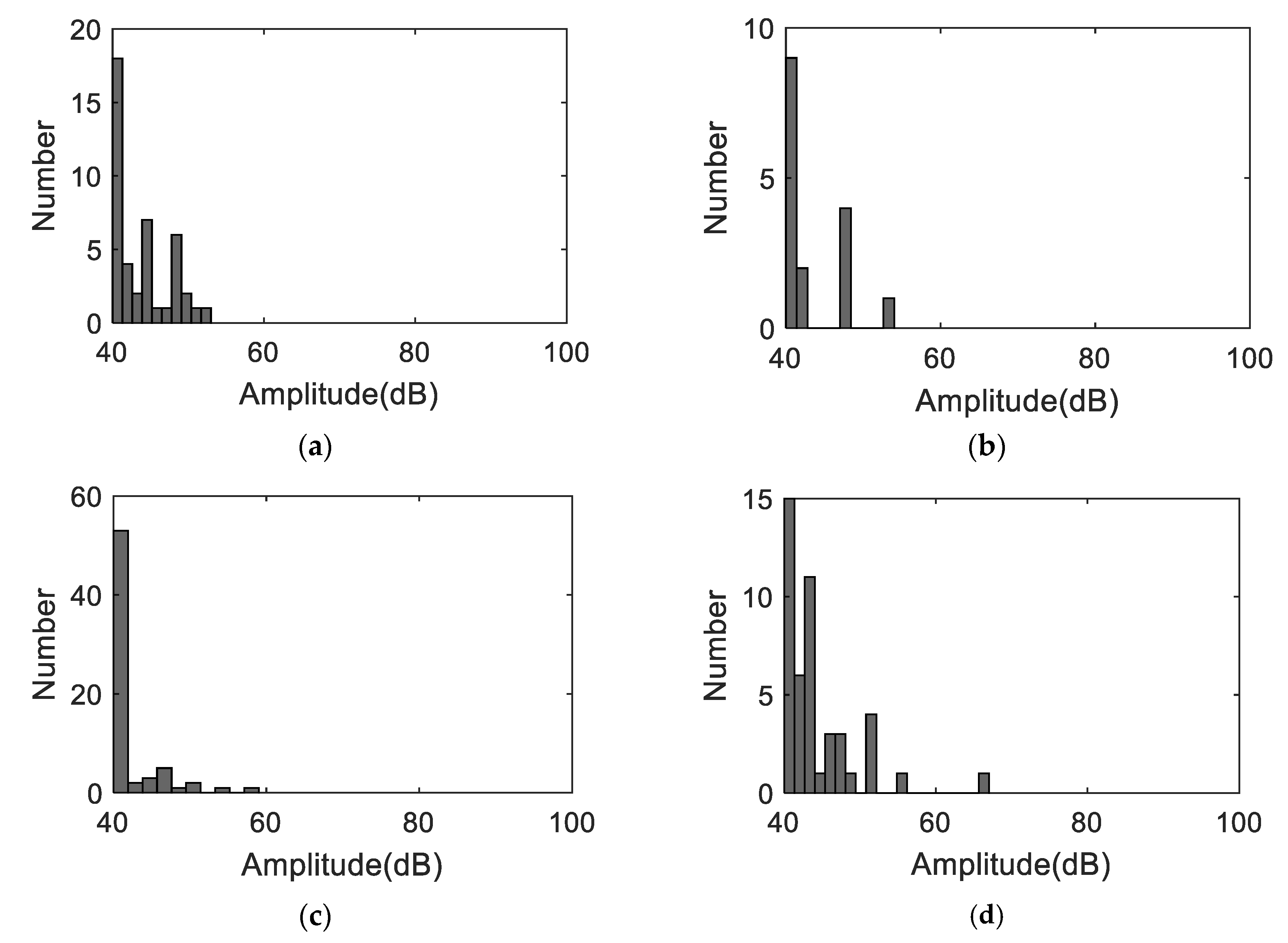
3.2. Real-Time Monitoring of Damage Evolution: Acoustic Emission (AE)
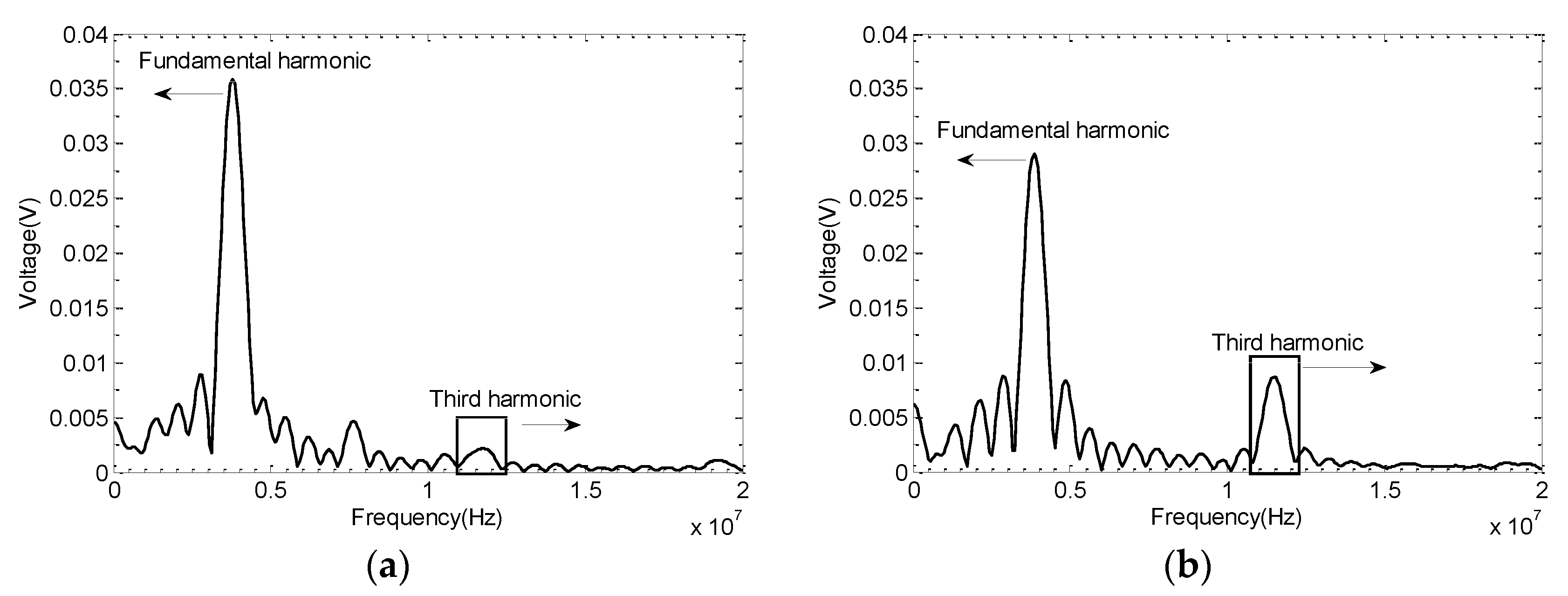
3.3. Post-Test Evaluation of Damage Evolution: Ultrasonics
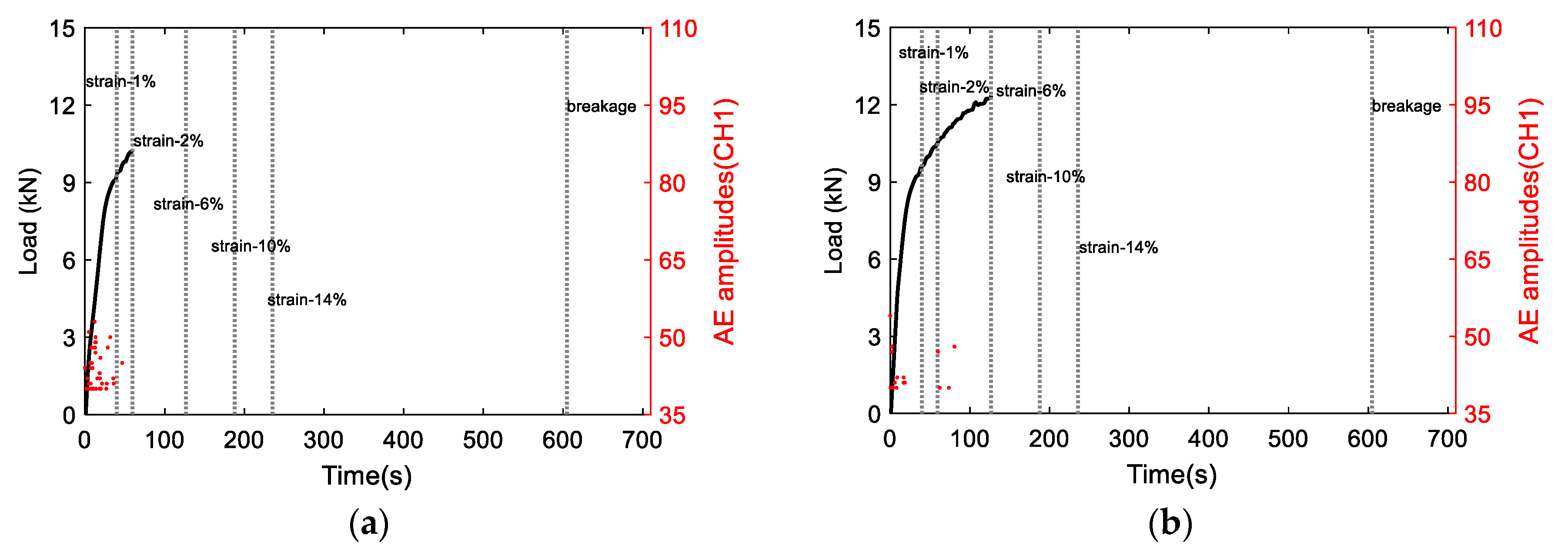
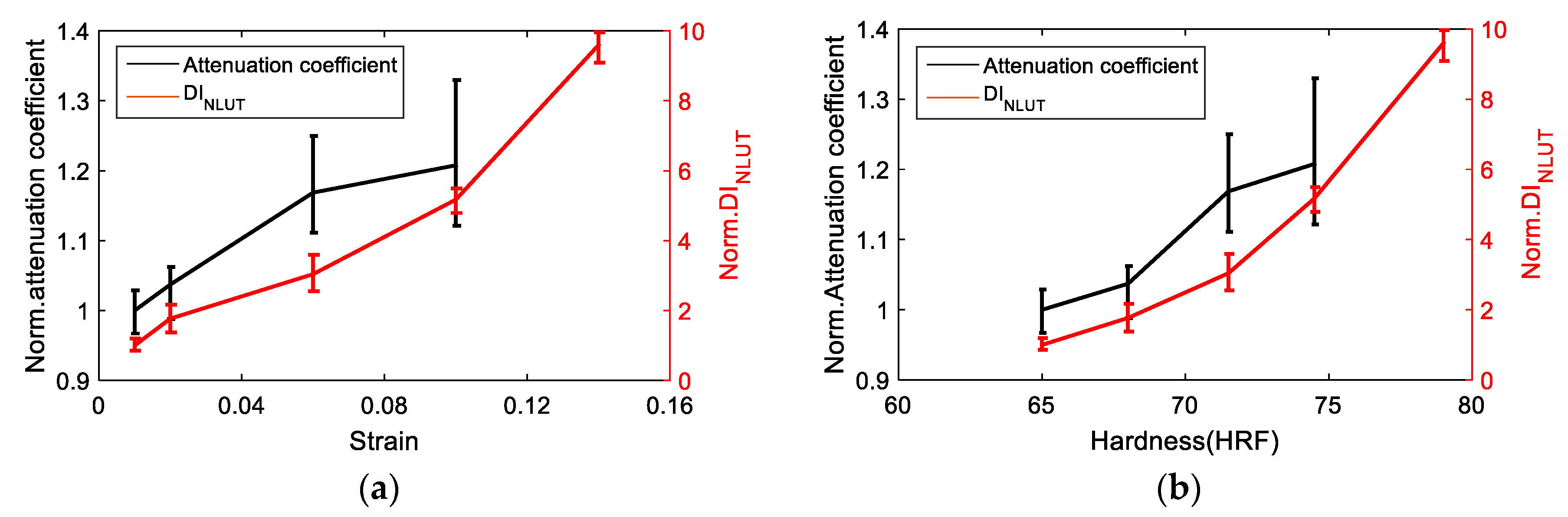
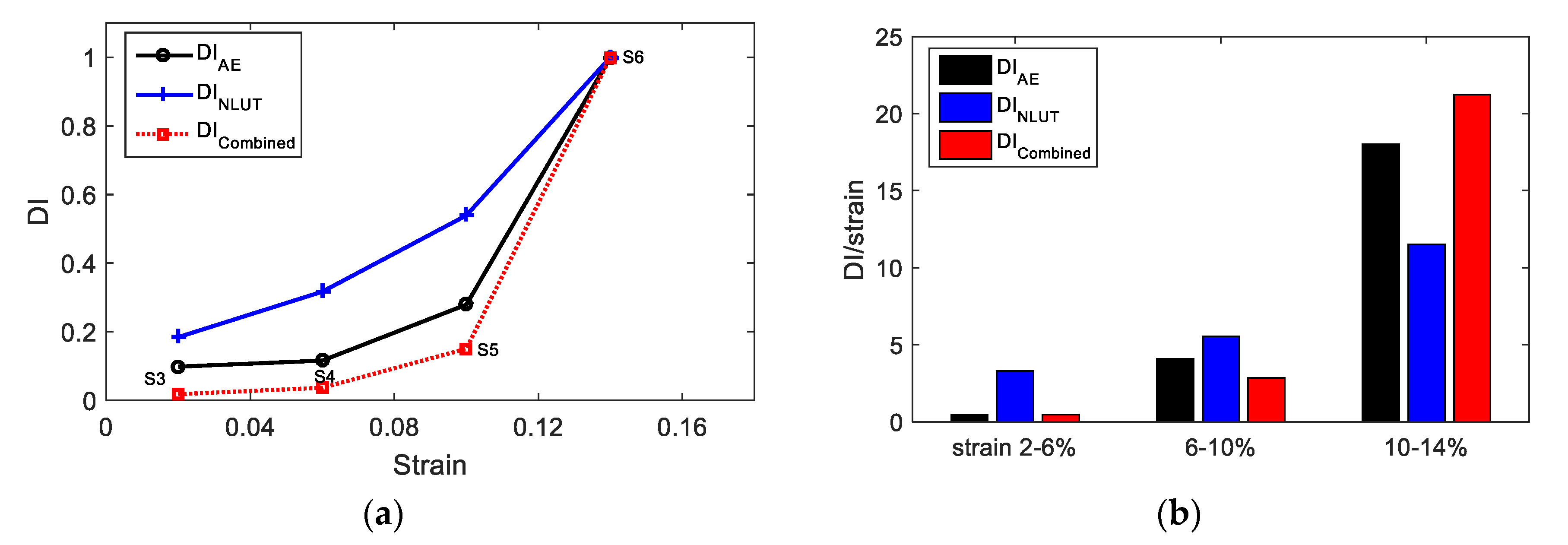
3.4. The Results of Combined AE, UT, and Strains Level
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Nomenclature
| NDE | Nondestructive evaluation |
| AE | Acoustic emission |
| LUT | Linear ultrasonics |
| NLUT | Nonlinear ultrasonics |
| UT | Ultrasonic testing |
| SEM | Scanning electron microscopy |
| UTS | Ultimate tensile strength |
| ANP(γ) | Acoustic nonlinearity parameters |
| DI | Damage index |
| µs | Microsecond |
| E | Young’s modulus |
| ν | Poisson ratio |
| ρ | Density |
| Abs Eng. | AE absolute energy |
References
- Davis, J.R. Aluminum and Aluminum Alloys; ASM international: Materials Park, OH, USA, 1993; ISBN 087170496X. [Google Scholar]
- Park, S.H.; Bae, J.H.; Kim, S.-H.; Yoon, J.; You, B.S. Effect of Initial Grain Size on Microstructure and Mechanical Properties of Extruded Mg-9Al-0.6Zn Alloy. Metall. Mater. Trans. A 2015, 46, 5482–5488. [Google Scholar] [CrossRef]
- Iannitti, G.; Bonora, N.; Bourne, N.; Ruggiero, A.; Testa, G. Damage development in rod-on-rod impact test on 1100 pure aluminum. AIP Conf. Proc. 2017, 1793, 100017. [Google Scholar]
- Wisner, B.; Kontsos, A. In situ monitoring of particle fracture in aluminium alloys. Fatigue Fract. Eng. Mater. Struct. 2018, 41, 581–596. [Google Scholar] [CrossRef]
- Kiesewetter, N.; Schiller, P. The acoustic emission from moving dislocations in aluminium. Phys. Status Solidi 1976, 38, 569–576. [Google Scholar] [CrossRef]
- Hazeli, K.; Askari, H.; Cuadra, J.; Streller, F.; Carpick, R.W.; Zbib, H.M.; Kontsos, A. Microstructure-sensitive investigation of magnesium alloy fatigue. Int. J. Plast. 2015, 68, 55–76. [Google Scholar] [CrossRef]
- Han, Z.; Luo, H.; Zhang, Y.; Cao, J. Effects of micro-structure on fatigue crack propagation and acoustic emission behaviors in a micro-alloyed steel. Mater. Sci. Eng. A 2013, 559, 534–542. [Google Scholar] [CrossRef]
- Shaira, M.; Godin, N.; Guy, P.; Vanel, L.; Courbon, J. Evaluation of the strain-induced martensitic transformation by acoustic emission monitoring in 304 L austenitic stainless steel: Identification of the AE signature of the martensitic transformation and power-law statistics. Mater. Sci. Eng. A 2008, 492, 392–399. [Google Scholar] [CrossRef]
- Barile, C.; Casavola, C.; Pappalettera, G.; Pappalettere, C. Hybrid thermography and acoustic emission testing of fatigue crack propagation in Aluminum Samples. In Fracture, Fatigue, Failure, and Damage Evolution; Springer: New York, NY, USA, 2015; Volume 5, pp. 247–252. [Google Scholar]
- Barile, C.; Casavola, C.; Pappalettera, G.; Pappalettere, C. Acoustic emission analysis of aluminum specimen subjected to laser annealing. In Residual Stress, Thermomechanics & Infrared Imaging, Hybrid Techniques and Inverse Problems; Springer: New York, NY, USA, 2014; Volume 8, pp. 309–315. [Google Scholar]
- Krautkramer, J.; Krautkramer, H. Ultrasonic Testing of Materials; Springer: Berlin/Heidelberg, Germany, 1983; ISBN 9783662023570. [Google Scholar]
- Mazal, P.; Vlasic, F.; Koula, V. Use of Acoustic Emission Method for Identification of Fatigue Micro-cracks Creation. Procedia Eng. 2015, 133, 379–388. [Google Scholar] [CrossRef]
- Mostavi, A.; Kamali, N.; Tehrani, N.; Chi, S.W.; Ozevin, D.; Indacochea, J.E. Wavelet based harmonics decomposition of ultrasonic signal in assessment of plastic strain in aluminum. Meas. J. Int. Meas. Confed. 2017, 106, 66–78. [Google Scholar] [CrossRef]
- Aggelis, D.G.; Barkoula, N.M.; Matikas, T.E.; Paipetis, A.S. Acoustic structural health monitoring of composite materials: Damage identification and evaluation in cross ply laminates using acoustic emission and ultrasonics. Compos. Sci. Technol. 2012, 72, 1127–1133. [Google Scholar] [CrossRef]
- Shah, A.A.; Ribakov, Y. Effectiveness of nonlinear ultrasonic and acoustic emission evaluation of concrete with distributed damages. Mater. Des. 2010, 31, 3777–3784. [Google Scholar] [CrossRef]
- Ohtsu, M.; Shigeishi, M.; Sakata, Y. Nondestructive evaluation of defects in concrete by quantitative acoustic emission and ultrasonics. Ultrasonics 1998, 36, 187–195. [Google Scholar] [CrossRef]
- Turner, S.L.; Rabani, A.; Axinte, D.A.; King, C.W. Dynamic ultrasonic contact detection using acoustic emissions. Ultrasonics 2014, 54, 749–753. [Google Scholar] [CrossRef] [PubMed]
- Fin, N.; Dodiuk, H.; Yaniv, A.E.; Drori, L. Oxide treatments of Al 2024 for adhesive bonding—Surface characterization. Appl. Surf. Sci. 1987, 28, 11–33. [Google Scholar] [CrossRef]
- ASTM E8, ASTM E8/E8M-16a, Standard Test Methods for Tension Testing of Metallic Materials; ASTM International: West Conshohocken, PA, USA, 2016.
- ASTM E3-11, Preparation of Metallographic Specimens; ASTM International: West Conshohocken, PA, USA, 2017.
- Davis, J.R. Surface Hardening of Steels: Understanding the Basics; ASM international: West Conshohocken, PA, USA, 2002; ISBN 1615032509. [Google Scholar]
- Pavlina, E.J.; Van Tyne, C.J. Correlation of Yield Strength and Tensile Strength with Hardness for Steels. J. Mater. Eng. Perform. 2008, 17, 888–893. [Google Scholar] [CrossRef]
- Wadley, H.N.G.; Scruby, C.B.; Speake, J.H. Acoustic emission for physical examination of metals. Met. Rev. 1980, 25, 41–64. [Google Scholar] [CrossRef] [Green Version]
- Lockner, D. The role of acoustic emission in the study of rock fracture. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1993, 30, 883–899. [Google Scholar] [CrossRef]
- Hase, A.; Mishina, H.; Wada, M. Correlation between features of acoustic emission signals and mechanical wear mechanisms. Wear 2012, 292–293, 144–150. [Google Scholar] [CrossRef]
- Cyra, G.; Tanaka, C. Effects of helical angle of router bit on acoustic emission. J. Wood Sci. 1998, 44, 169–176. [Google Scholar] [CrossRef]
- Vinogradov, A.Y.; Merson, D.L. The nature of acoustic emission during deformation processes in metallic materials. Low Temp. Phys. 2018, 44, 930–937. [Google Scholar] [CrossRef]
- Ferrer, C.; Salas, F.; Pascual, M.; Orozco, J. Discrete acoustic emission waves during stick-slip friction between steel samples. Tribol. Int. 2010, 43, 1–6. [Google Scholar] [CrossRef]
- Kim, J.-Y.; Jacobs, L.J.; Qu, J.; Littles, J.W. Experimental characterization of fatigue damage in a nickel-base superalloy using nonlinear ultrasonic waves. J. Acoust. Soc. Am. 2006, 120, 1266. [Google Scholar] [CrossRef]
- Shui, G.; Wang, Y.S.; Gong, F. Evaluation of plastic damage for metallic materials under tensile load using nonlinear longitudinal waves. NDT E Int. 2013, 55, 1–8. [Google Scholar] [CrossRef]
- Shah, A.A.; Ribakov, Y. Non-linear ultrasonic evaluation of damaged concrete based on higher order harmonic generation. Mater. Des. 2009, 30, 4095–4102. [Google Scholar] [CrossRef]

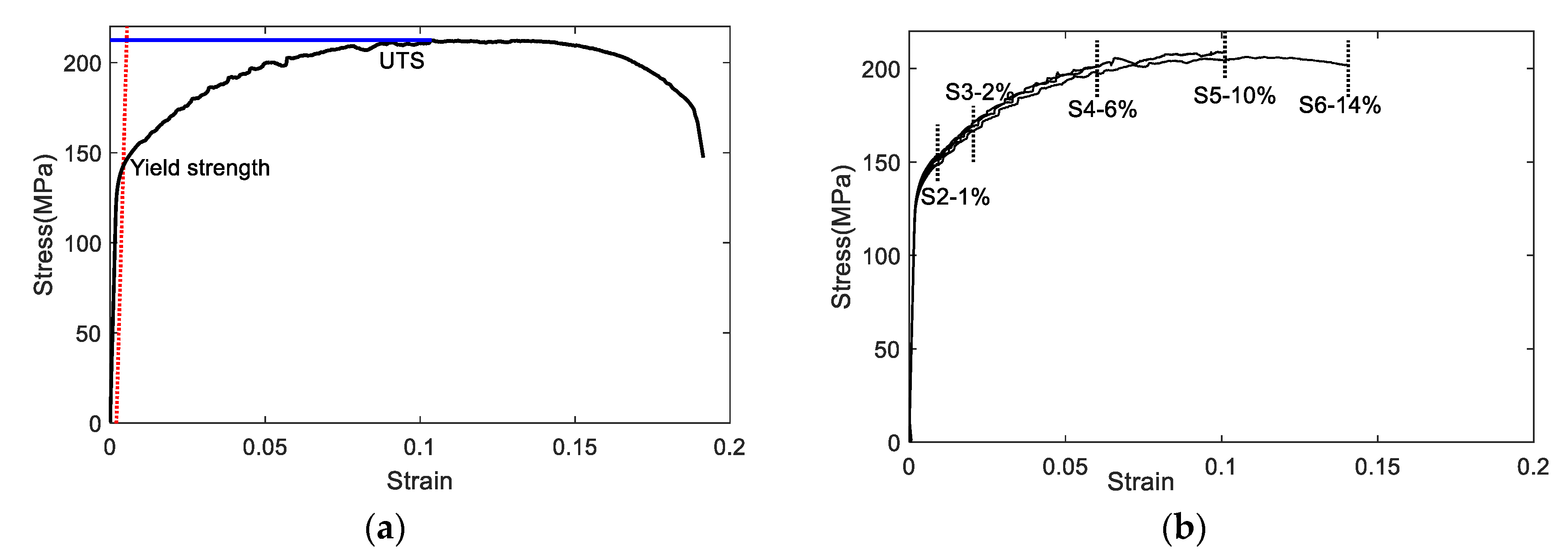

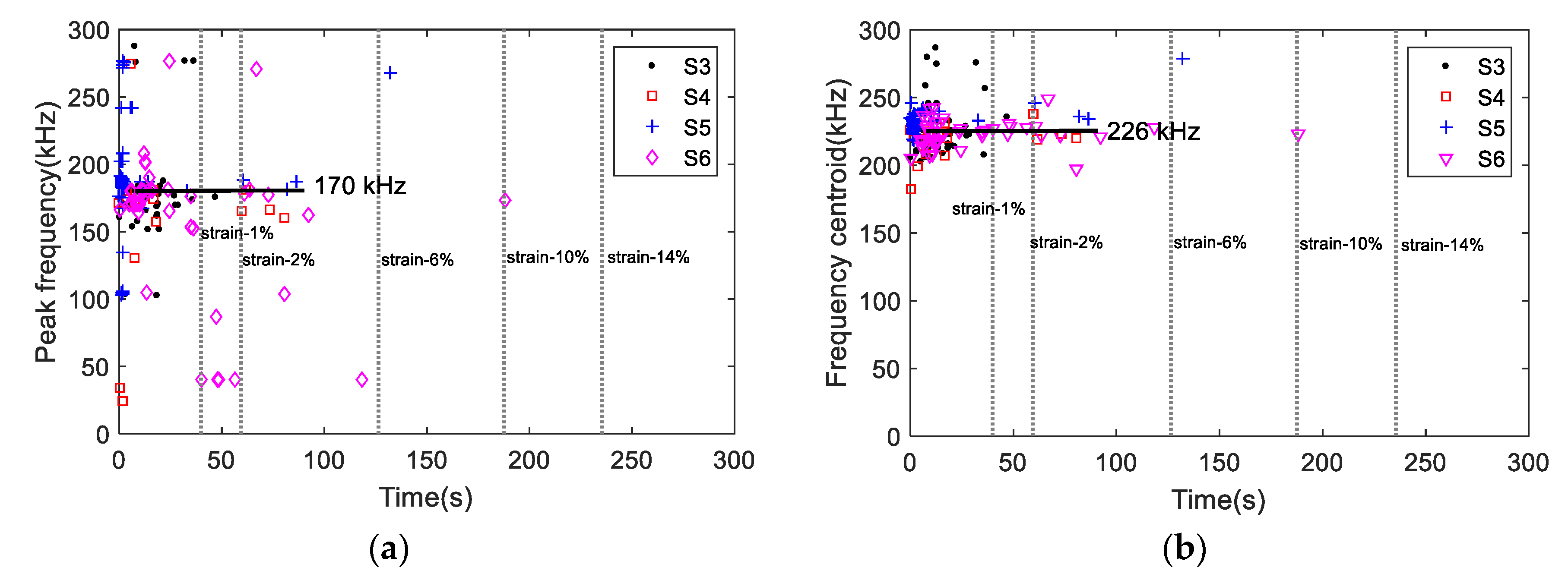
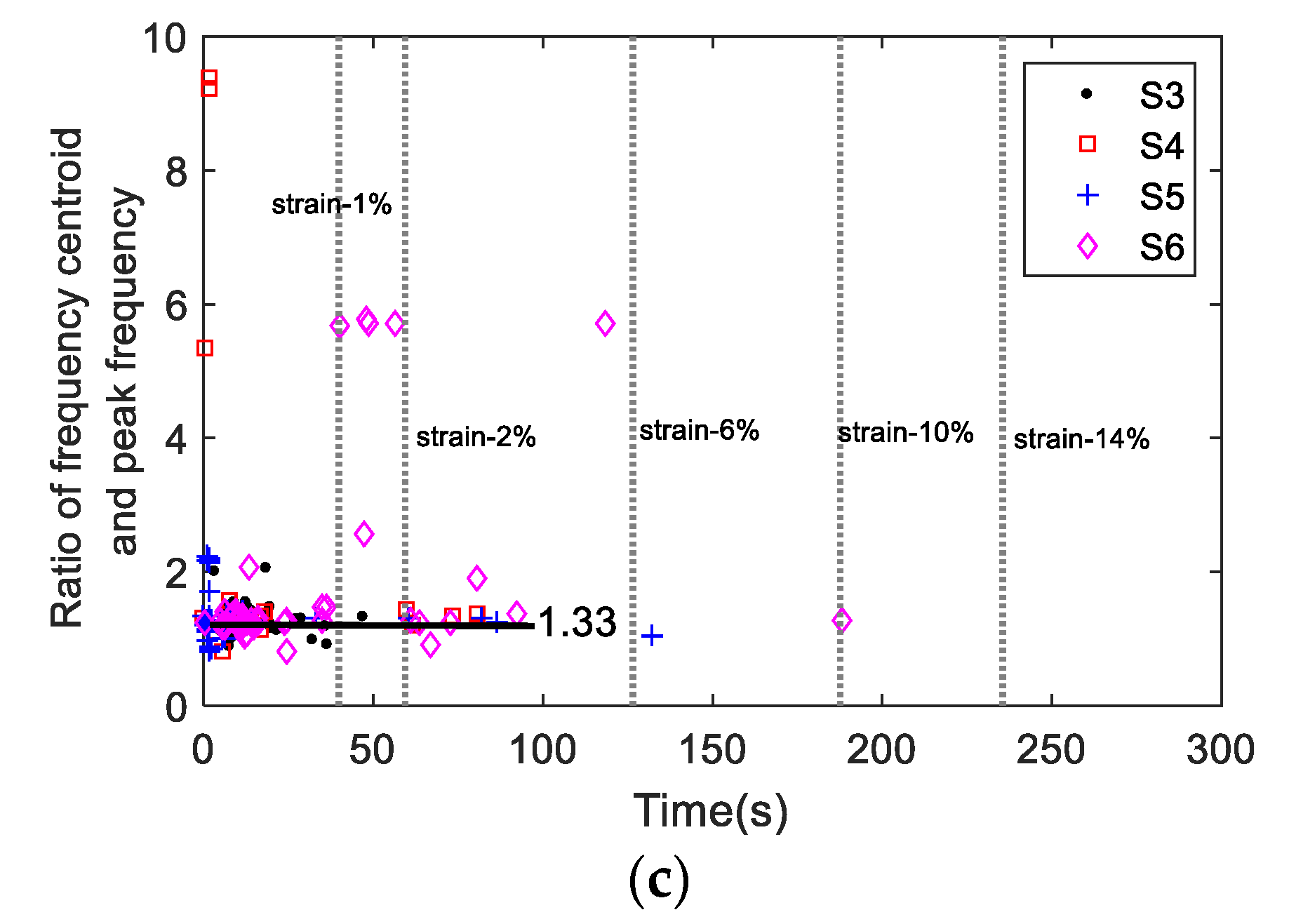
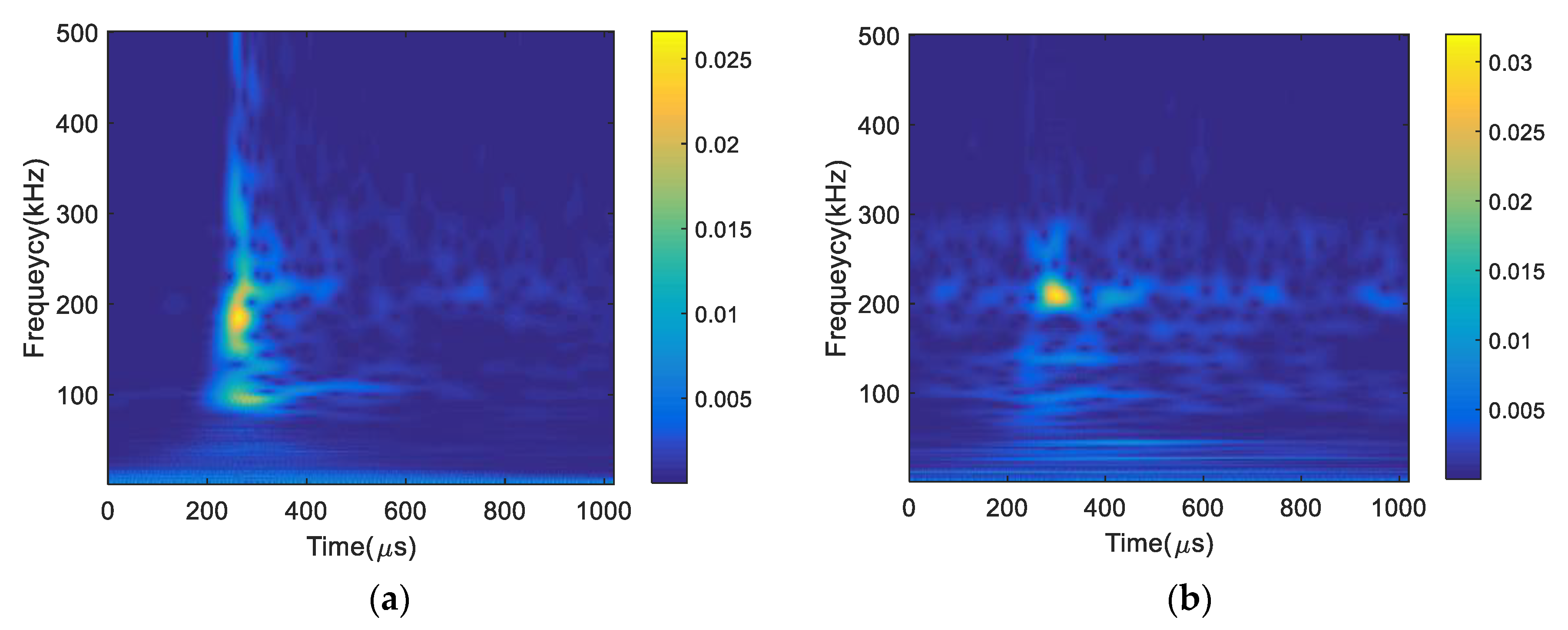
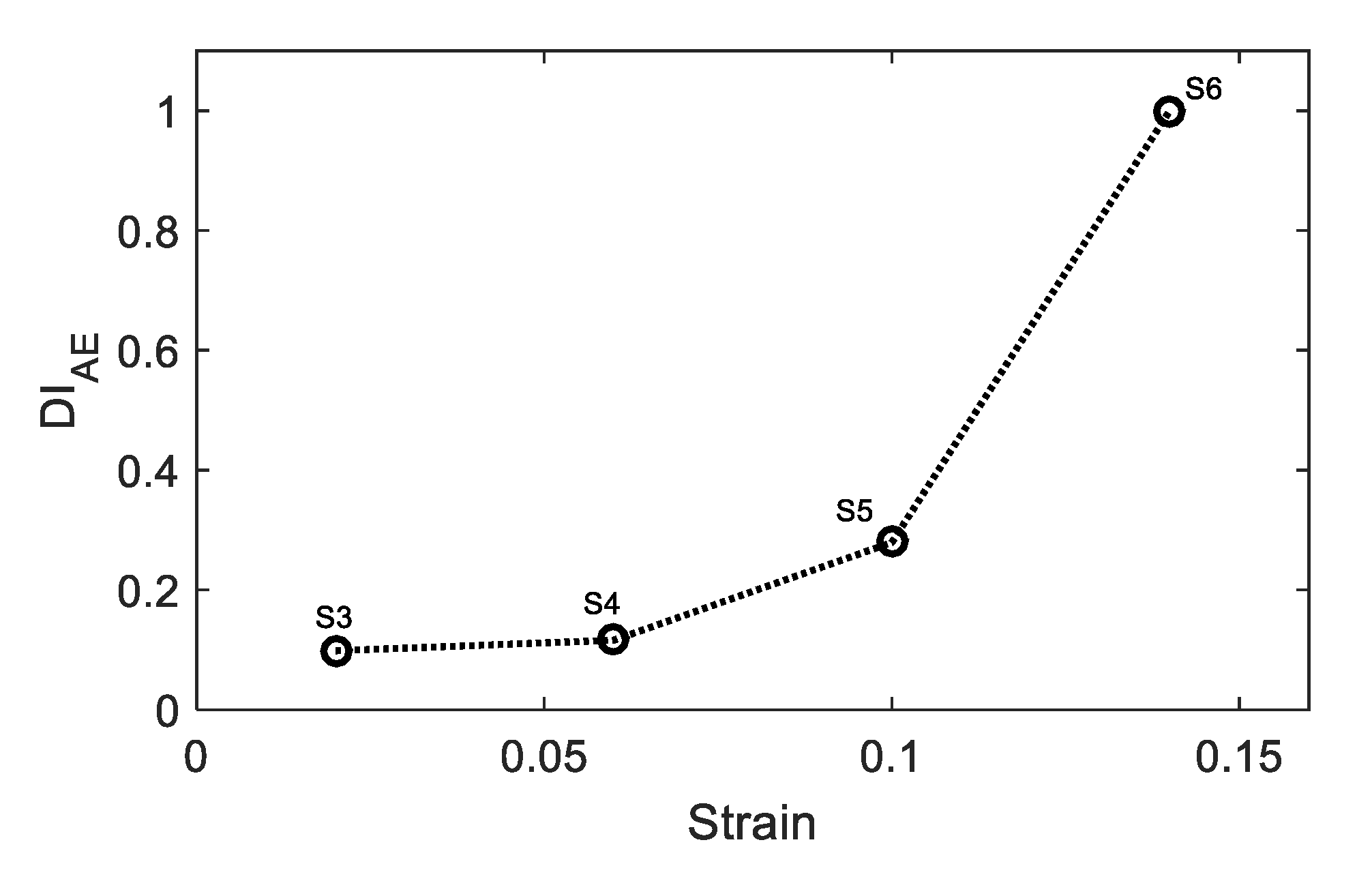
| Properties | Young’s Modulus E (GPa) | Poisson Ratio ν | ρ (kg/m3) | σys (MPa) | Strain (Yield) | UTS (MPa) | UTS Strain | Elongation (%) |
|---|---|---|---|---|---|---|---|---|
| Value | 64 | 0.33 | 2710 | 142.7 | 0.0042 | 212.5 | 0.12 | 19 |
| Sample | Test Point 1 | Test Point 2 | Test Point 3 |
|---|---|---|---|
| S2 (1%) * | 61.0 | 65.0 | 63.0 |
| S3 (2%) * | 62.0 | 68.0 | 64.0 |
| S4 (6%) * | 63.0 | 71.5 | 67.0 |
| S5 (10%) * | 64.0 | 74.5 | 71.0 |
| S6 (14%) * | 65.0 | 79.0 | 74.0 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, L.; Oskoe, S.K.; Li, H.; Ozevin, D. Combined Damage Index to Detect Plastic Deformation in Metals Using Acoustic Emission and Nonlinear Ultrasonics. Materials 2018, 11, 2151. https://doi.org/10.3390/ma11112151
Zhang L, Oskoe SK, Li H, Ozevin D. Combined Damage Index to Detect Plastic Deformation in Metals Using Acoustic Emission and Nonlinear Ultrasonics. Materials. 2018; 11(11):2151. https://doi.org/10.3390/ma11112151
Chicago/Turabian StyleZhang, Lu, Sepideh Karkouti Oskoe, Hongyu Li, and Didem Ozevin. 2018. "Combined Damage Index to Detect Plastic Deformation in Metals Using Acoustic Emission and Nonlinear Ultrasonics" Materials 11, no. 11: 2151. https://doi.org/10.3390/ma11112151
APA StyleZhang, L., Oskoe, S. K., Li, H., & Ozevin, D. (2018). Combined Damage Index to Detect Plastic Deformation in Metals Using Acoustic Emission and Nonlinear Ultrasonics. Materials, 11(11), 2151. https://doi.org/10.3390/ma11112151





