Reconfigurable Multifunctional Metasurface Hybridized with Vanadium Dioxide at Terahertz Frequencies
Abstract
1. Introduction
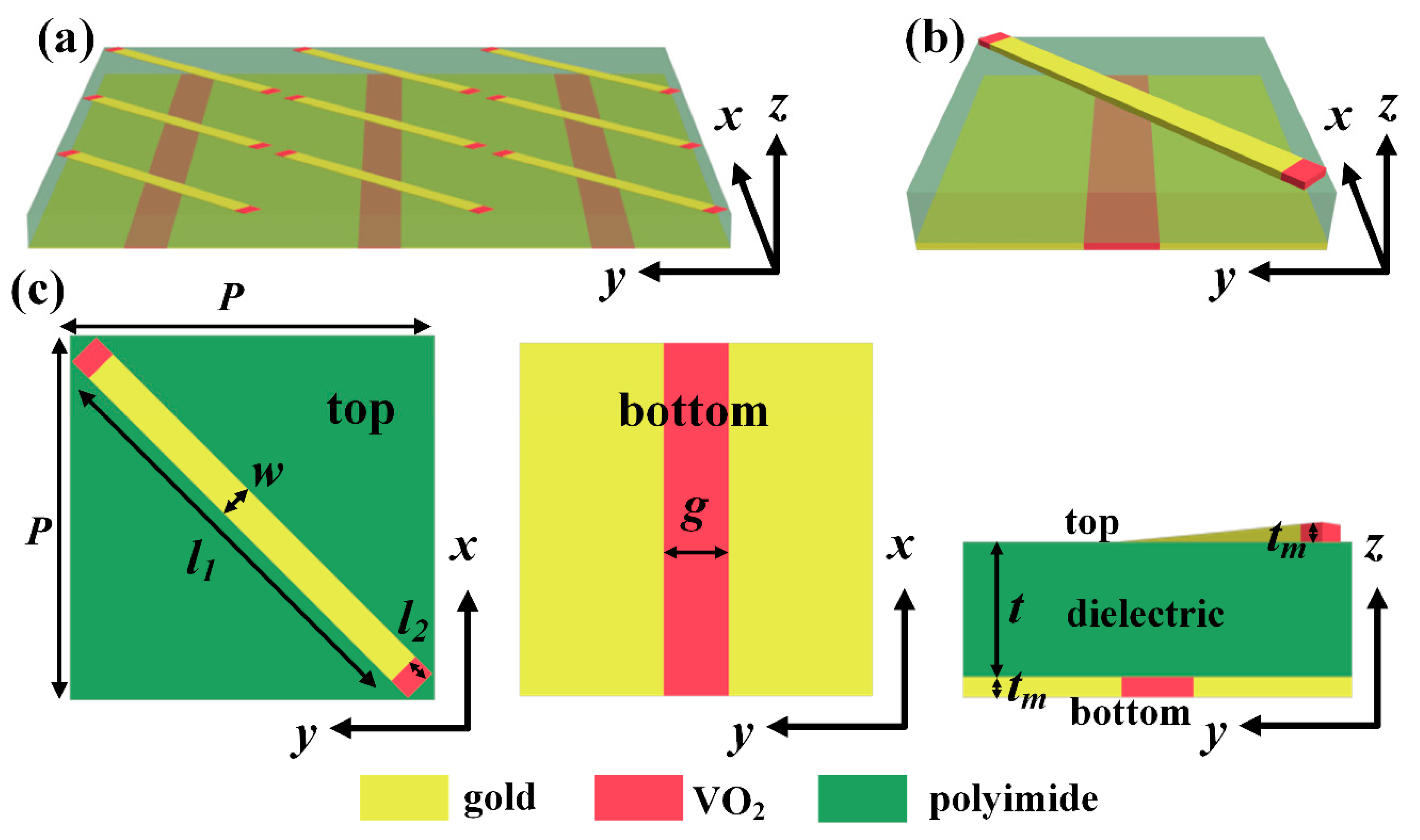
2. The Design of Reconfigurable Multifunctional Metasurface
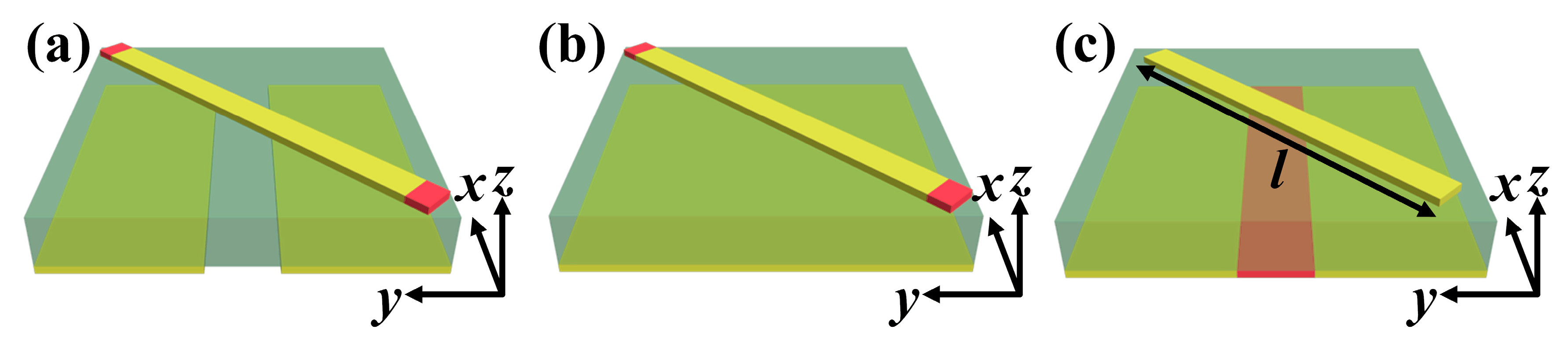
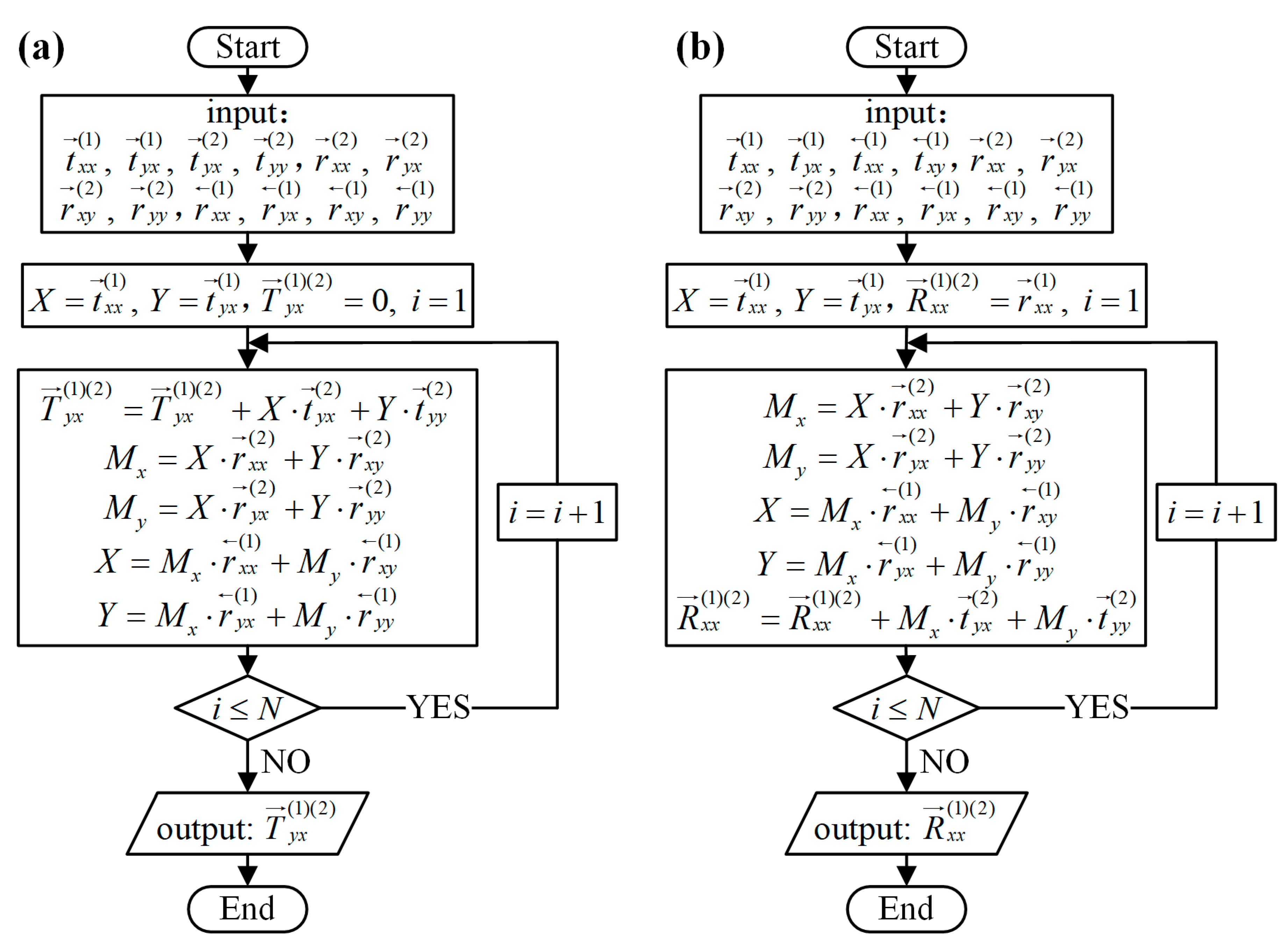
3. Mechanism of the Reconfigurable Multifunctional Metasurface
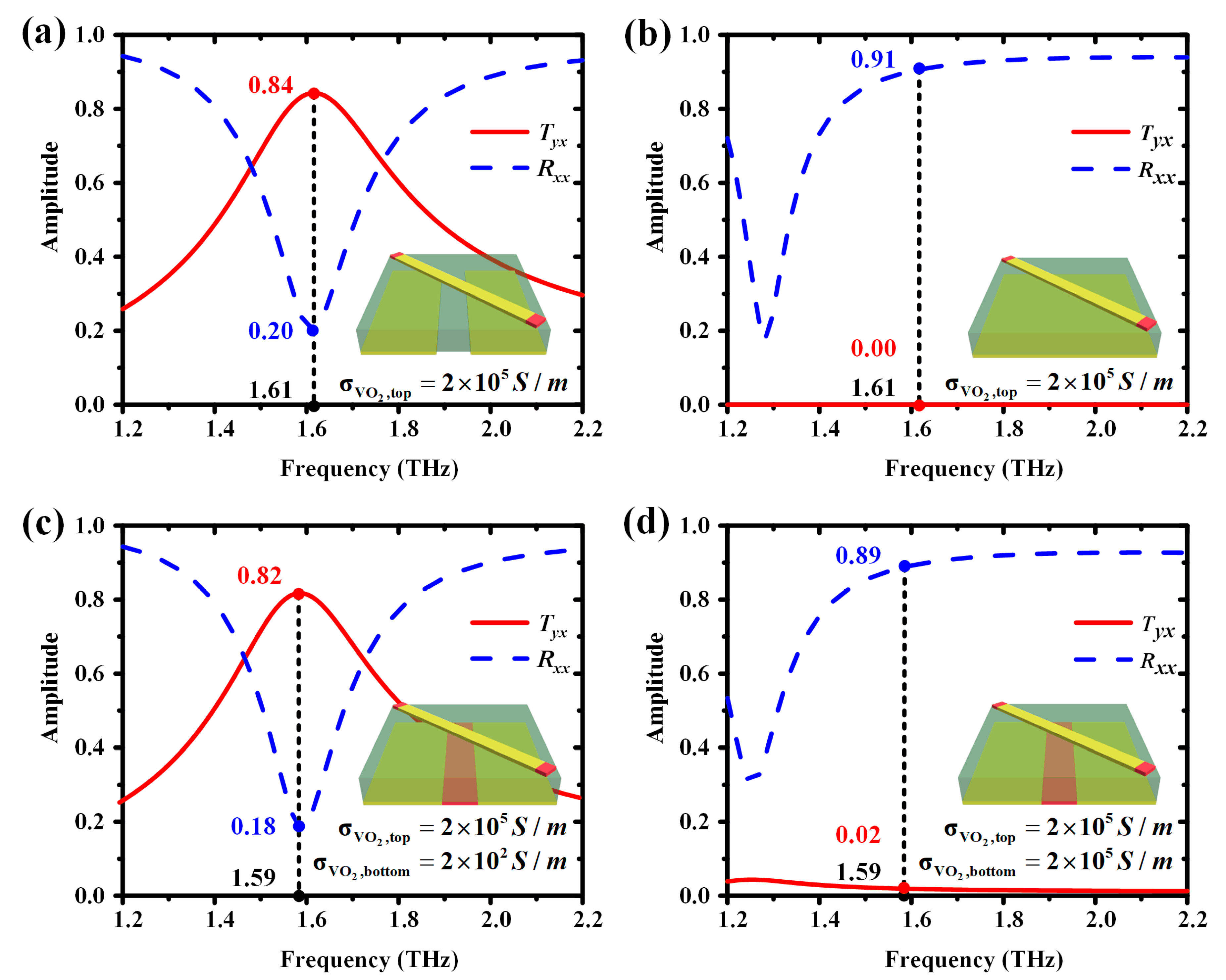
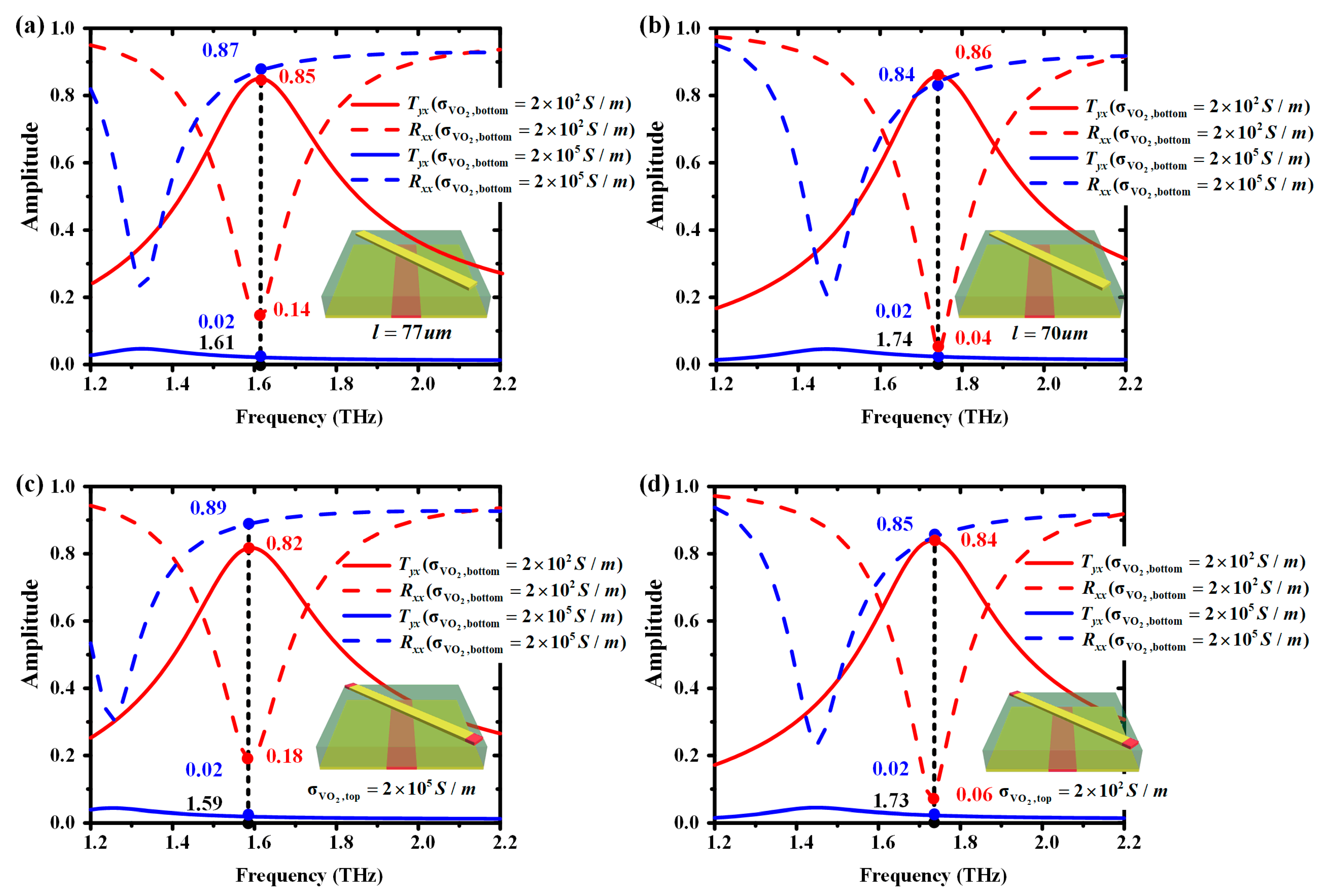
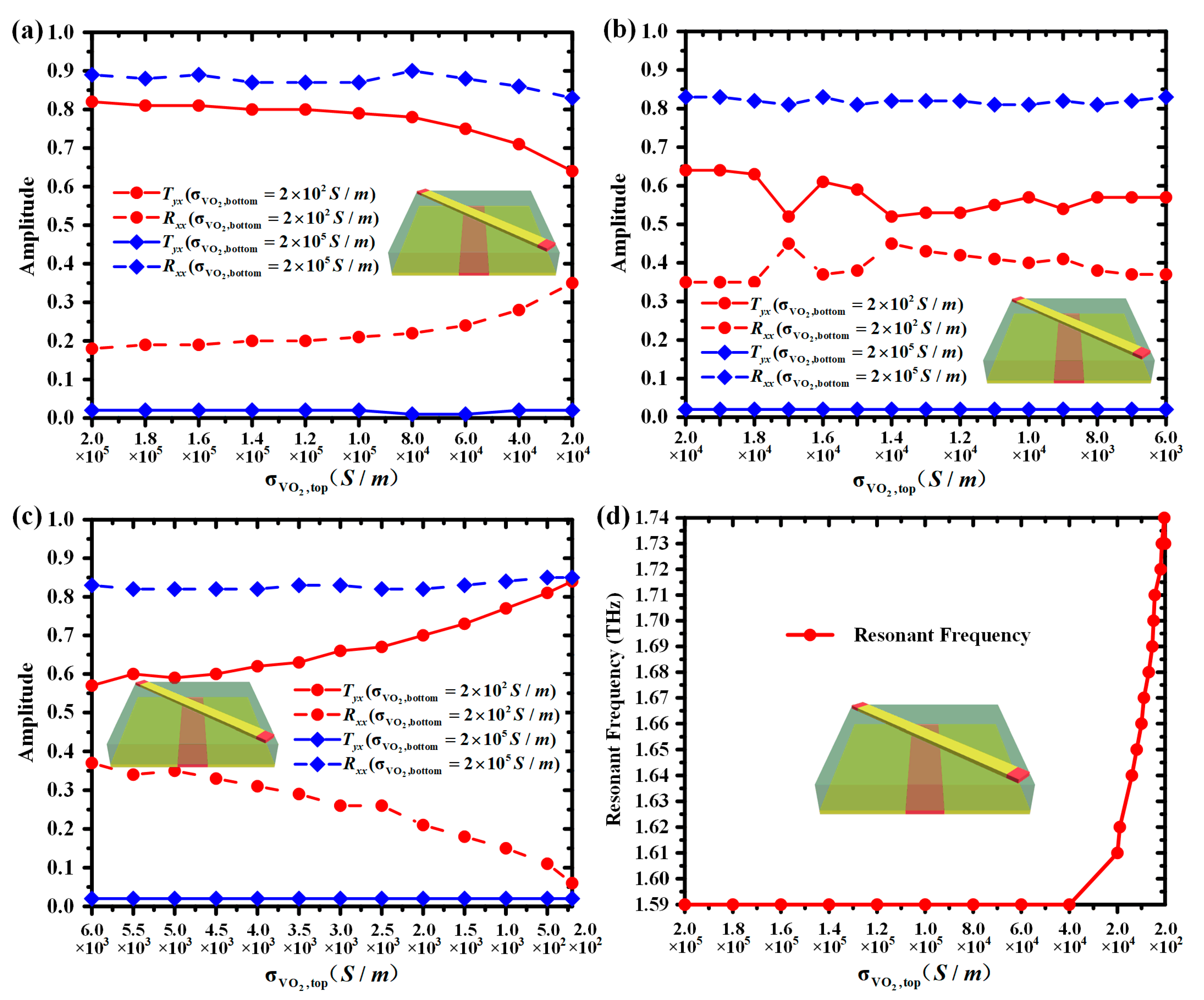
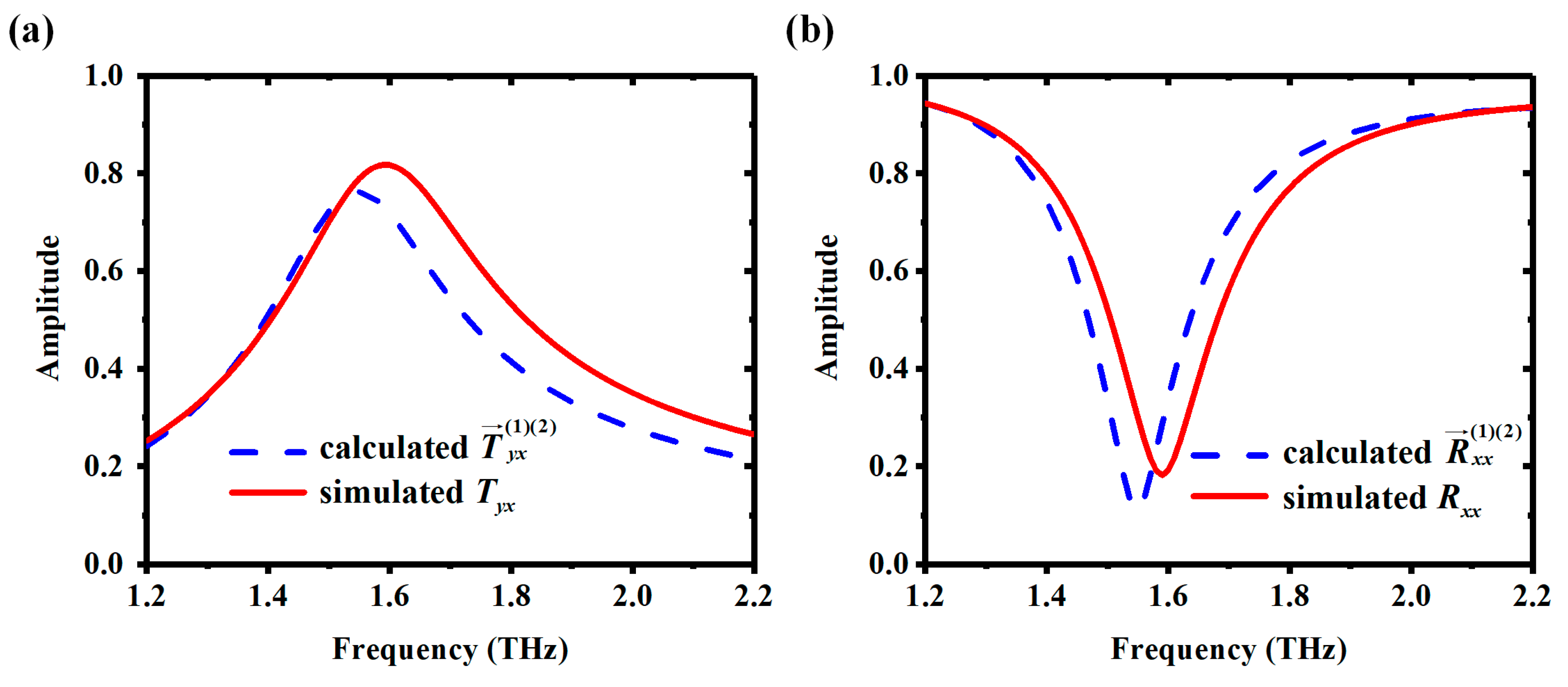
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Shelby, R.A.; Smith, D.R.; Schultz, S. Experimental verification of a negative index of refraction. Science 2001, 292, 77–79. [Google Scholar] [CrossRef] [PubMed]
- Schurig, D.; Mock, J.J.; Justice, B.J.; Cummer, S.A.; Pendry, J.B.; Starr, A.F.; Smith, D.R. Metamaterial electromagnetic cloak at microwave frequencies. Science 2006, 314, 977–980. [Google Scholar] [CrossRef] [PubMed]
- Landy, N.I.; Sajuyigbe, S.; Mock, J.J.; Smith, D.R.; Padilla, W.J. Perfect metamaterial absorber. Phys. Rev. Lett. 2008, 100, 207402. [Google Scholar] [CrossRef] [PubMed]
- Monticone, F.; Alu, A. Metamaterials and plasmonics: From nanoparticles to nanoantenna arrays, metasurfaces, and metamaterials. Chin. Phys. B 2014, 23, 047809. [Google Scholar] [CrossRef]
- La Spada, L.; Vegni, L. Near-zero-index wires. Opt. Express 2017, 25, 23699–23708. [Google Scholar] [CrossRef] [PubMed]
- Huang, L.L.; Chen, X.Z.; Muhlenbernd, H.; Zhang, H.; Chen, S.M.; Bai, B.F.; Tan, Q.F.; Jin, G.F.; Cheah, K.W.; Qiu, C.W.; et al. Three-dimensional optical holography using a plasmonic metasurface. Nat. Commun. 2013, 4, 2808. [Google Scholar] [CrossRef]
- High, A.A.; Devlin, R.C.; Dibos, A.; Polking, M.; Wild, D.S.; Perczel, J.; de Leon, N.P.; Lukin, M.D.; Park, H. Visible-frequency hyperbolic metasurface. Nature 2015, 522, 192–196. [Google Scholar] [CrossRef] [PubMed]
- Ding, X.M.; Monticone, F.; Zhang, K.; Zhang, L.; Gao, D.L.; Burokur, S.N.; de Lustrac, A.; Wu, Q.; Qiu, C.W.; Alu, A. Ultrathin pancharatnam-berry metasurface with maximal cross-polarization efficiency. Adv. Mater. 2015, 27, 1195–1200. [Google Scholar] [CrossRef] [PubMed]
- Lee, Y.; Kim, S.J.; Park, H.; Lee, B. Metamaterials and metasurfaces for sensor applications. Sensors 2017, 17, 1726. [Google Scholar] [CrossRef] [PubMed]
- Lu, X.Y.; Zhang, T.Y.; Wan, R.G.; Xu, Y.T.; Zhao, C.H.; Guo, S. Numerical investigation of narrowband infrared absorber and sensor based on dielectric-metal metasurface. Opt. Express 2018, 26, 10179–10187. [Google Scholar] [CrossRef] [PubMed]
- Shadrivov, I.V.; Fan, K.; Padilla, W.J.; Liu, X. Experimental realization of a terahertz all-dielectric metasurface absorber. Opt. Express 2017, 25, 191–201. [Google Scholar]
- Zhu, J.F.; Li, S.F.; Deng, L.; Zhang, C.; Yang, Y.; Zhu, H.B. Broadband tunable terahertz polarization converter based on a sinusoidally-slotted graphene metamaterial. Opt. Mater. Express 2018, 8, 1164–1173. [Google Scholar] [CrossRef]
- Zhu, J.F.; Yang, Y.; Li, S.F. A photo-excited broadband to dual-band tunable terahertz prefect metamaterial polarization converter. Opt. Commun. 2018, 413, 336–340. [Google Scholar] [CrossRef]
- Liu, W.W.; Li, Z.C.; Cheng, H.; Tang, C.C.; Li, J.J.; Zhang, S.; Chen, S.Q.; Tian, J.G. Metasurface enabled wide-angle fourier lens. Adv. Mater. 2018, 30, 1706368. [Google Scholar] [CrossRef] [PubMed]
- Azad, A.K.; Efimov, A.V.; Ghosh, S.; Singleton, J.; Taylor, A.J.; Chen, H.T. Ultra-thin metasurface microwave flat lens for broadband applications. Appl. Phys. Lett. 2017, 110, 224101. [Google Scholar] [CrossRef] [PubMed]
- Caira, N.W.; Smith, D.R. Multispectral metasurface hologram at millimeter wavelengths. Appl. Optics 2018, 57, A19–A25. [Google Scholar] [CrossRef] [PubMed]
- Li, L.L.; Cui, T.J.; Ji, W.; Liu, S.; Ding, J.; Wan, X.; Li, Y.B.; Jiang, M.H.; Qiu, C.W.; Zhang, S. Electromagnetic reprogrammable coding-metasurface holograms. Nat. Commun 2017, 8, 197. [Google Scholar] [CrossRef] [PubMed]
- Lee, W.S.L.; Nirantar, S.; Headland, D.; Bhaskaran, M.; Sriram, S.; Fumeaux, C.; Withayachumnankul, W. Metasurface beam splitter: Broadband terahertz circular-polarization beam splitter. Adv. Opt. Mater. 2018, 6, 1700852. [Google Scholar] [CrossRef]
- Guo, J.Y.; Wang, X.K.; He, J.W.; Zhao, H.; Feng, S.F.; Han, P.; Ye, J.S.; Sun, W.F.; Situ, G.H.; Zhang, Y. Generation of radial polarized lorentz beam with single layer metasurface. Adv. Opt. Mater. 2018, 6, 1700925. [Google Scholar] [CrossRef]
- Zhuang, Y.Q.; Wang, G.M.; Cai, T.; Zhang, Q.F. Design of bifunctional metasurface based on independent control of transmission and reflection. Opt. Express 2018, 26, 3594–3603. [Google Scholar] [CrossRef] [PubMed]
- Deng, Z.L.; Cao, Y.Y.; Li, X.P.; Wang, G.P. A multifunctional metasurface: From extraordinary optical transmission to extraordinary optical diffraction in a single structure. Photonics Res. 2018, 6, 443–450. [Google Scholar] [CrossRef]
- Cheng, J.R.; Inampudi, S.; Mosallaei, H. Optimization-based dielectric metasurfaces for angle-selective multifunctional beam deflection. Sci. Rep. 2017, 7, 12228. [Google Scholar] [CrossRef] [PubMed]
- Cheng, H.; Wei, X.Y.; Yu, P.; Li, Z.C.; Liu, Z.; Li, J.J.; Chen, S.Q.; Tian, J.G. Integrating polarization conversion and nearly perfect absorption with multifunctional metasurfaces. Appl. Phys. Lett. 2017, 110, 171903. [Google Scholar] [CrossRef]
- Li, Y.B.; Cai, B.G.; Cheng, Q.; Cui, T.J. Isotropic holographic metasurfaces for dual-functional radiations without mutual interferences. Adv. Funct. Mater. 2016, 26, 29–35. [Google Scholar] [CrossRef]
- Cai, T.; Tang, S.W.; Wang, G.M.; Xu, H.X.; Sun, S.L.; He, Q.; Zhou, L. High-Performance Bifunctional Metasurfaces in Transmission and Reflection Geometries. Adv. Opt. Mater. 2016, 5, 1600506. [Google Scholar] [CrossRef]
- Ding, F.; Zhong, S.M.; Bozhevolnyi, S.I. Vanadium dioxide integrated metasurfaces with switchable functionalities at terahertz frequencies. Adv. Opt. Mater. 2018, 6, 1701204. [Google Scholar] [CrossRef]
- Deng, L.; Zhang, Y.Y.; Zhu, J.F.; Qu, M.J.; Wang, L.; Zhang, C. Independent manipulating of orthogonal-polarization terahertz waves using a reconfigurable graphene-based metasurface. Materials 2018, 11, 1817. [Google Scholar] [CrossRef] [PubMed]
- Deng, L.; Zhang, Y.Y.; Zhu, J.F.; Zhang, C. Wide-band circularly polarized reflectarray using graphene-based pancharatnam-berry phase unit-cells for terahertz communication. Materials 2018, 11, 956. [Google Scholar] [CrossRef] [PubMed]
- Zhang, C.; Deng, L.; Zhu, J.F.; Hong, W.J.; Wang, L.; Yang, W.J.; Li, S.F. Control of the spin angular momentum and orbital angular momentum of a reflected wave by multifunctional graphene metasurfaces. Materials 2018, 11, 1054. [Google Scholar] [CrossRef] [PubMed]
- Tarparelli, R.; Iovine, R.; Spada, L.L.; Vegni, L. Surface plasmon resonance of nanoshell particles with pmma-graphene core. Int. J. Comp. Math. Electr. Electron. Eng. 2014, 33, 2016–2029. [Google Scholar] [CrossRef]
- Zhao, Y.T.; Wu, B.A.; Huang, B.J.; Cheng, Q.A. Switchable broadband terahertz absorber/reflector enabled by hybrid graphene-gold metasurface. Opt. Express 2017, 25, 7161–7169. [Google Scholar] [CrossRef] [PubMed]
- Forouzmand, A.; Mosallaei, H. Real-time controllable and multifunctional metasurfaces utilizing indium tin oxide materials: A phased array perspective. IEEE Trans. Nanotechnol. 2017, 16, 296–306. [Google Scholar] [CrossRef]
- Chen, W.; Gao, J.; Zhang, G.; Cao, X.Y.; Yang, H.H.; Zheng, Y.J. A wideband coding reflective metasurface with multiple functionalities. Acta Phys. Sin. 2017, 66, 064203. [Google Scholar]
- Kim, S.; Wakatsuchi, H.; Rushton, J.J.; Sievenpiper, D.F. Switchable nonlinear metasurfaces for absorbing high power surface waves. Appl. Phys. Lett. 2016, 108, 041903. [Google Scholar] [CrossRef]
- Huang, C.; Zhang, C.L.; Yang, J.N.; Sun, B.; Zhao, B.; Luo, X.G. Reconfigurable metasurface for multifunctional control of electromagnetic waves. Adv. Opt. Mater. 2017, 5, 1700485. [Google Scholar] [CrossRef]
- You, J.W.; Panoiu, N.C. Polarization control using passive and active crossed graphene gratings. Opt. Express 2018, 26, 1882–1894. [Google Scholar] [CrossRef] [PubMed]
- Owiti, E.O.; Yang, H.N.; Liu, P.; Ominde, C.F.; Sun, X.D. Polarization converter with controllable birefringence based on hybrid all-dielectric-graphene metasurface. Nanoscale Res. Lett. 2018, 13, 38. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.C.; Cheng, Y.Z.; Cheng, Z.Z. Design of a photo-excited switchable broadband reflective linear polarization conversion metasurface for terahertz waves. IEEE Photonics J. 2018, 10, 4600210. [Google Scholar] [CrossRef]
- Kim, T.T.; Oh, S.S.; Kim, H.D.; Park, H.S.; Hess, O.; Min, B.; Zhang, S. Electrical access to critical coupling of circularly polarized waves in graphene chiral metamaterials. Sci. Adv. 2017, 3, e1701377. [Google Scholar] [CrossRef] [PubMed]
- Gao, X.; Yang, W.L.; Cao, W.P.; Chen, M.; Jiang, Y.N.; Yu, X.H.; Li, H.O. Bandwidth broadening of a graphene-based circular polarization converter by phase compensation. Opt. Express 2017, 25, 23945–23954. [Google Scholar] [CrossRef] [PubMed]
- Chen, M.; Chang, L.Z.; Gao, X.; Chen, H.; Wang, C.Y.; Xiao, X.F.; Zhao, D.P. Wideband tunable cross polarization converter based on a graphene metasurface with a hollow-carved “H” array. IEEE Photonics J. 2017, 9, 4601011. [Google Scholar] [CrossRef]
- Nakata, Y.; Urade, Y.; Okimura, K.; Nakanishi, T.; Miyamaru, F.; Takeda, M.W.; Kitano, M. Anisotropic Babinet-invertible metasurfaces to realize transmission-reflection switching for orthogonal polarizations of light. Phys. Rev. Appl. 2016, 6, 044022. [Google Scholar] [CrossRef]
- Yang, L.; Fan, F.; Chen, M.; Zhang, X.Z.; Chang, S.J. Multifunctional metasurfaces for terahertz polarization controller. Acta Phys. Sin. 2016, 65, 080702. [Google Scholar]
- Bian, Y.L.; Wu, C.; Li, H.Q.; Zhai, J.W. A tunable metamaterial dependent on electric field at terahertz with barium strontium titanate thin film. Appl. Phys. Lett. 2014, 104, 042906. [Google Scholar] [CrossRef]
- Benz, A.; Montano, I.; Klem, J.F.; Brener, I. Tunable metamaterials based on voltage controlled strong coupling. Appl. Phys. Lett. 2013, 103, 263116. [Google Scholar] [CrossRef]
- Tao, Z.; Wan, X.; Pan, B.C.; Cui, T.J. Reconfigurable conversions of reflection, transmission, and polarization states using active metasurface. Appl. Phys. Lett. 2017, 110, 121901. [Google Scholar] [CrossRef]
- Mak, K.F.; Sfeir, M.Y.; Wu, Y.; Lui, C.H.; Misewich, J.A.; Heinz, T.F. Measurement of the optical conductivity of graphene. Phys. Rev. Lett. 2008, 101, 196405. [Google Scholar] [CrossRef] [PubMed]
- Stauber, T.; Peres, N.M.R.; Geim, A.K. The optical conductivity of graphene in the visible region of the spectrum. Phys. Rev. B 2008, 78, 085432. [Google Scholar] [CrossRef]
- Decker, M.; Kremers, C.; Minovich, A.; Staude, I.; Miroshnichenko, A.E.; Chigrin, D.; Neshev, D.N.; Jagadish, C.; Kivshar, Y.S. Electro-optical switching by liquid-crystal controlled metasurfaces. Opt. Express 2013, 21, 8879–8885. [Google Scholar] [CrossRef] [PubMed]
- Kowerdziej, R.; Olifierczuk, M.; Parka, J.; Wrobel, J. Terahertz characterization of tunable metamaterial based on electrically controlled nematic liquid crystal. Appl. Phys. Lett. 2014, 105, 022908. [Google Scholar] [CrossRef]
- Lv, T.T.; Li, Y.X.; Ma, H.F.; Zhu, Z.; Li, Z.P.; Guan, C.Y.; Shi, J.H.; Zhang, H.; Cui, T.J. Hybrid metamaterial switching for manipulating chirality based on VO2 phase transition. Sci. Rep. 2016, 6, 23186. [Google Scholar] [CrossRef] [PubMed]
- Wu, S.R.; Lai, K.L.; Wang, C.M. Passive temperature control based on a phase change metasurface. Sci. Rep. 2018, 8, 7684. [Google Scholar] [CrossRef] [PubMed]
- He, J.W.; Xie, Z.W.; Sun, W.F.; Wang, X.K.; Ji, Y.D.; Wang, S.; Lin, Y.; Zhang, Y. Terahertz tunable metasurface lens based on vanadium dioxide phase transition. Plasmonics 2016, 11, 1285–1290. [Google Scholar] [CrossRef]
- Wang, D.C.; Zhang, L.C.; Gong, Y.D.; Jian, L.K.; Venkatesan, T.; Qiu, C.W.; Hong, M.H. Multiband switchable terahertz quarter-wave plates via phase-change metasurfaces. IEEE Photonics J. 2016, 8, 1–8. [Google Scholar] [CrossRef]
- Goldflam, M.D.; Liu, M.K.; Chapler, B.C.; Stinson, H.T.; Sternbach, A.J.; McLeod, A.S.; Zhang, J.D.; Geng, K.; Royal, M.; Kim, B.J.; et al. Voltage switching of a VO2, memory metasurface using ionic gel. Appl. Phys. Lett. 2014, 105, 041117. [Google Scholar] [CrossRef]
- Driscoll, T.; Kim, H.T.; Chae, B.G.; Kim, B.J.; Lee, Y.W.; Jokerst, N.M.; Palit, S.; Smith, D.R.; Di Ventra, M.; Basov, D.N. Memory metamaterials. Science 2009, 325, 1518–1521. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.X.; Qiao, S.; Sun, L.L.; Shi, Q.W.; Huang, W.X.; Li, L.; Yang, Z.Q. Photoinduced active terahertz metamaterials with nanostructured vanadium dioxide film deposited by sol-gel method. Opt. Express. 2014, 22, 11070–11078. [Google Scholar] [CrossRef] [PubMed]
- Grady, N.K.; Heyes, J.E.; Chowdhury, D.R.; Zeng, Y.; Reiten, M.T.; Azad, A.K.; Taylor, A.J.; Dalvit, D.A.R.; Chen, H.T. Terahertz metamaterials for linear polarization conversion and anomalous refraction. Science 2013, 340, 1304–1307. [Google Scholar] [CrossRef] [PubMed]
- Chiang, Y.J.; Yen, T.J. A composite-metamaterial-based terahertz-wave polarization rotator with an ultrathin thickness, an excellent conversion ratio, and enhanced transmission. Appl. Phys. Lett. 2013, 102, 011129. [Google Scholar] [CrossRef]


© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Hong, W.; Deng, L.; Li, S.; Zhang, C.; Zhu, J.; Wang, H. Reconfigurable Multifunctional Metasurface Hybridized with Vanadium Dioxide at Terahertz Frequencies. Materials 2018, 11, 2040. https://doi.org/10.3390/ma11102040
Wang L, Hong W, Deng L, Li S, Zhang C, Zhu J, Wang H. Reconfigurable Multifunctional Metasurface Hybridized with Vanadium Dioxide at Terahertz Frequencies. Materials. 2018; 11(10):2040. https://doi.org/10.3390/ma11102040
Chicago/Turabian StyleWang, Ling, Weijun Hong, Li Deng, Shufang Li, Chen Zhang, Jianfeng Zhu, and Hongjun Wang. 2018. "Reconfigurable Multifunctional Metasurface Hybridized with Vanadium Dioxide at Terahertz Frequencies" Materials 11, no. 10: 2040. https://doi.org/10.3390/ma11102040
APA StyleWang, L., Hong, W., Deng, L., Li, S., Zhang, C., Zhu, J., & Wang, H. (2018). Reconfigurable Multifunctional Metasurface Hybridized with Vanadium Dioxide at Terahertz Frequencies. Materials, 11(10), 2040. https://doi.org/10.3390/ma11102040




