A New Method for Predicting Erosion Damage of Suddenly Contracted Pipe Impacted by Particle Cluster via CFD-DEM
Abstract
1. Introduction
2. Solution Methodology
2.1. Particle-Particle Interaction
2.2. Momentum Exchange and Energy Dissipation
2.3. Particle-Wall Interaction
2.4. Governing Equations of Fluid Flow
2.5. Erosion Model
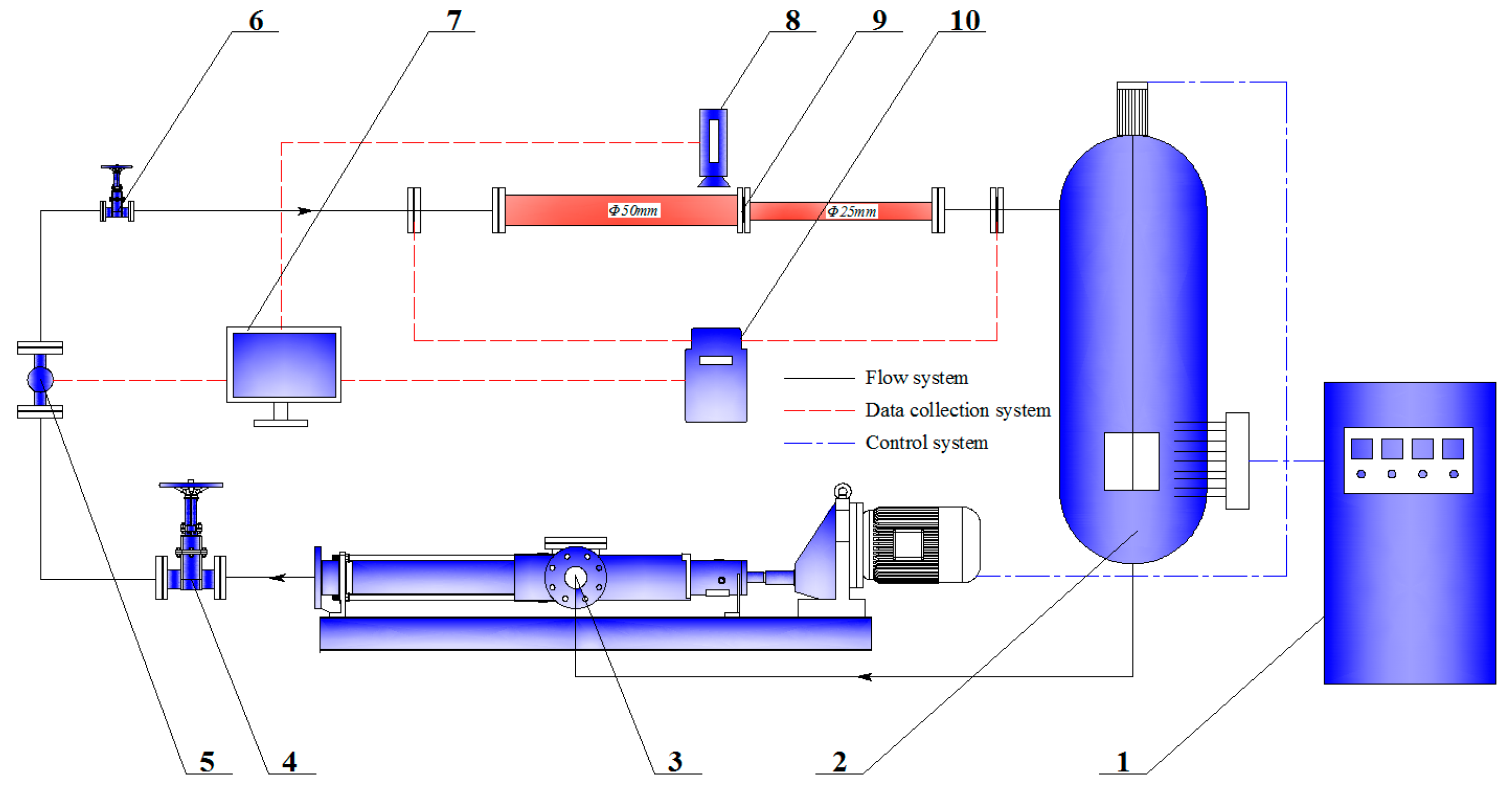
3. Implementation and Verification
3.1. Numerical Solving Step
3.2. Boundary Conditions
3.3. Full-Scale Experiment
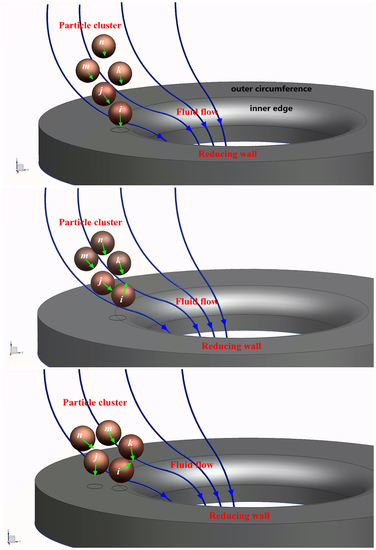
4. Results
4.1. Particle-Wall Impingement
4.2. Particle Cluster Erosion
4.3. Discussion
5. Conclusions
- (1)
- Formation of particle clusters results in interparticle collisions when certain particles bounce back from the wall and the succeeding particle erosion rate decreases with the change in particle impact velocity.
- (2)
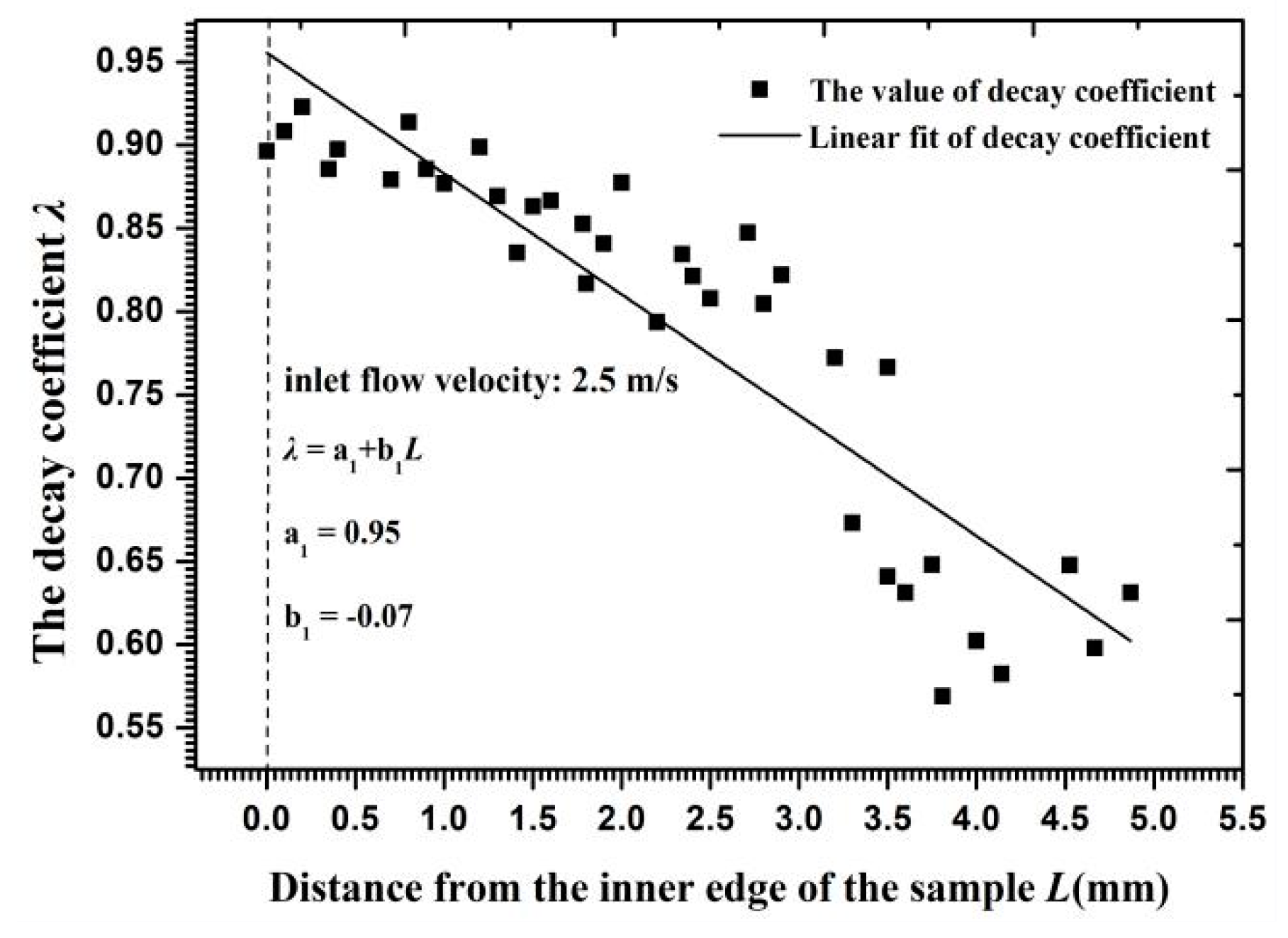
- During particle impact and rebound, the contact time of the target surface is less than that of the particle rebound time before the particle collides with another particle. The decay rate of a normal impact velocity changes from 60 to 90% along the radial surface and the decay rate of the tangential velocity changes in the range of 30 to 40%.
- (3)
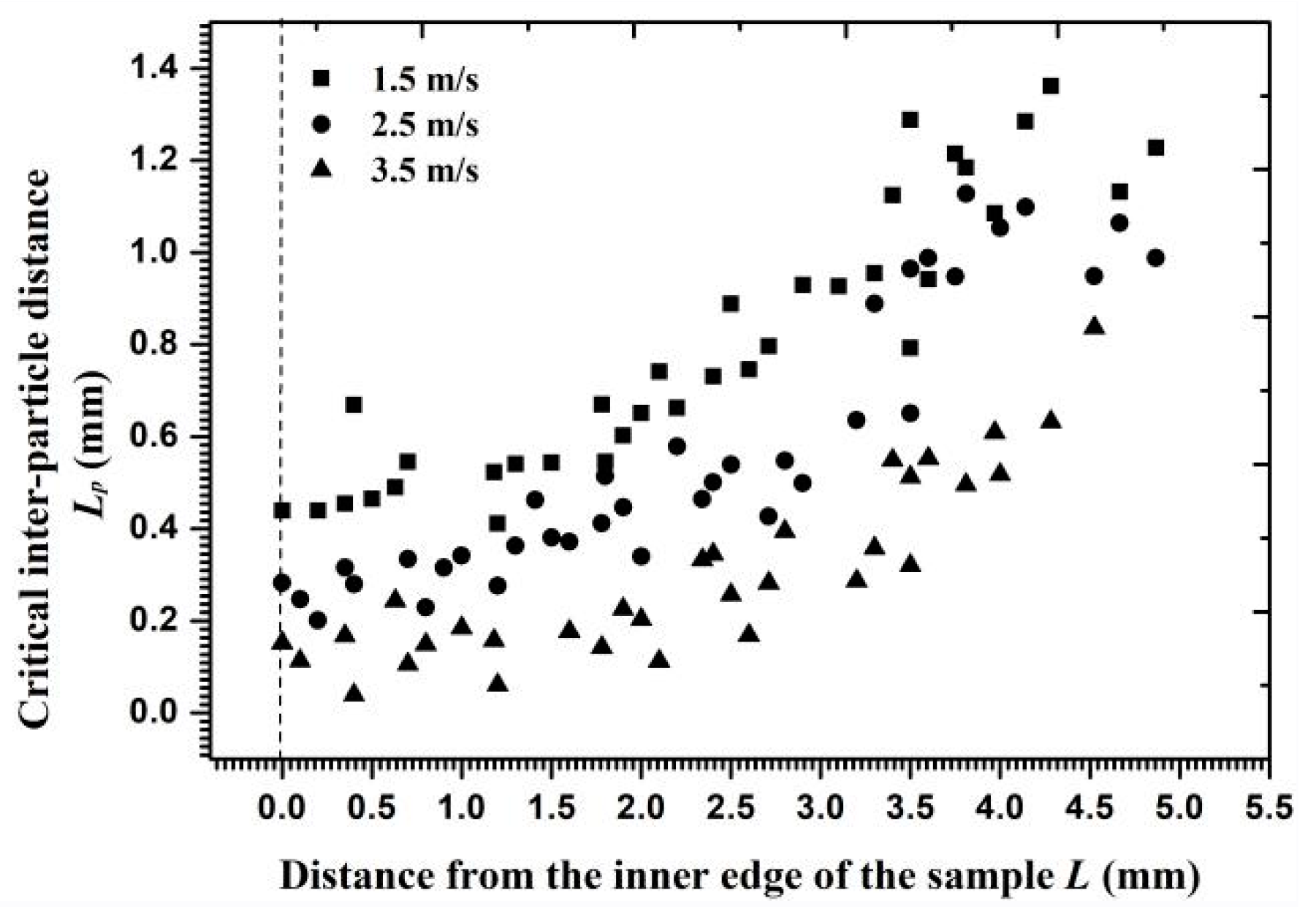
- A smaller critical interparticle distance, which indicates that particles must be closer to each other for a collision to exist, results in a lower clustered particle percentage of the total impact particles. Therefore, the probability and frequency of a particle cluster impact on the outer circumference are larger than those on the inner edge. Meanwhile, by updating the particle impact velocity and angle, the relative error between the calculation results and the experimental results was reduced by almost 11% compared with that of the complete independent impact setting.
- (4)
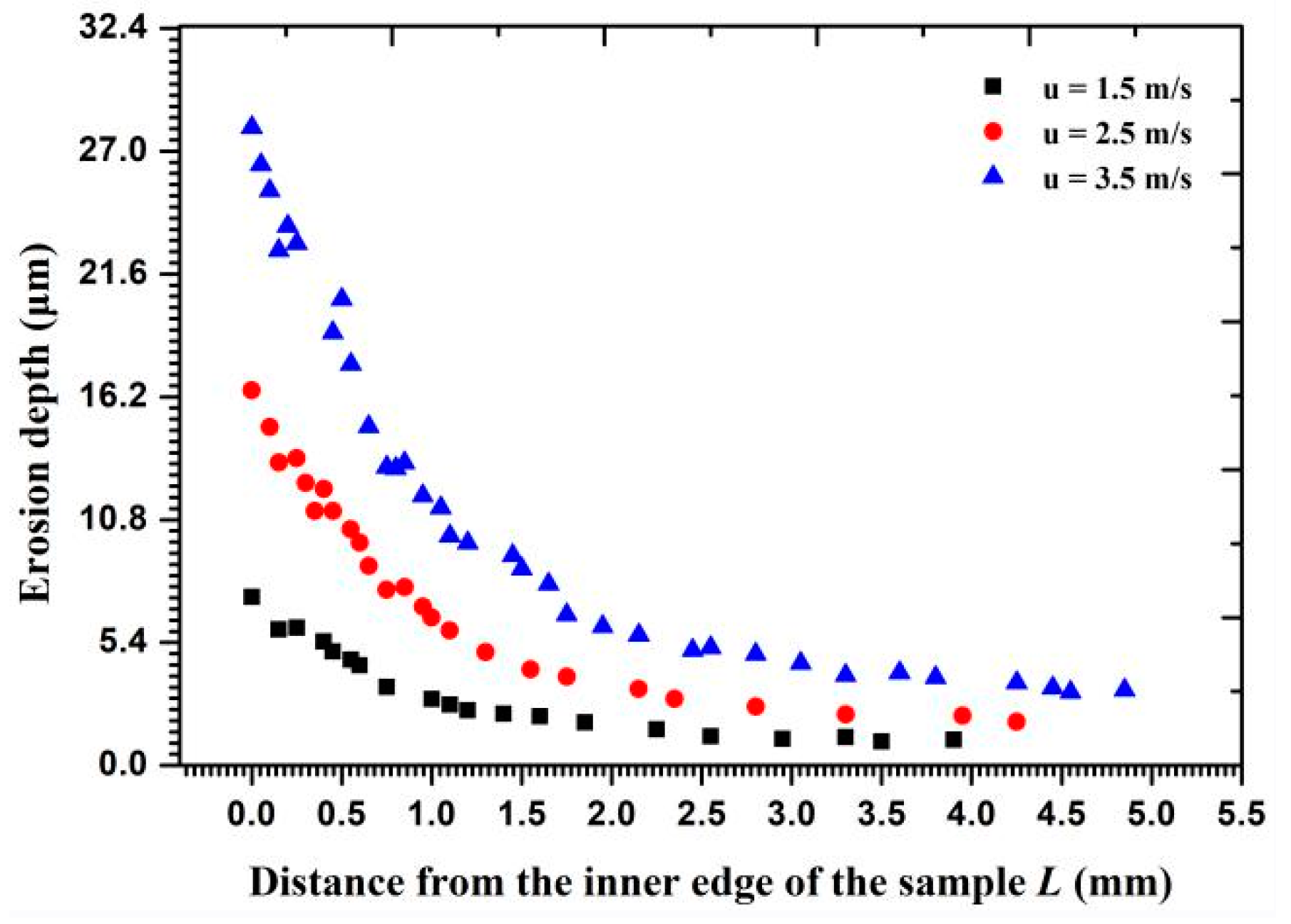
- The second impact velocity of the particle in IPE is almost three times the velocity in SPE on the inner edge and almost 10 times on the outer circumference because the particle velocity decays once in IPE and twice in SPE. The growth of flow velocity not only decreases the critical interparticle distance but also the decay coefficient in particle collision, indicating that the effect of a cluster impact on erosion can be weakened by an increasing flow velocity.
Author Contributions
Acknowledgments
Conflicts of Interest
Nomenclature
| Ap | particle cross-sectional area, m2 | te | contact time, s |
| Cd | drag coefficient, dimensionless | tr | release time, s |
| Cn | normal damping coefficient, kg·s−1 | t1 | rebound time, s |
| Ct | tangential damping coefficient, kg·s−1 | t2 | moving time for particle j, s |
| D | inside diameter of circular pipe, m | ∆tD | DEM time step, s |
| dp | diameter of particle, m | ∆tRa | Rayleigh time step, s |
| en | restitution coefficient in the normal direction, dimensionless | Tr | rolling friction torque, N·m |
| et | restitution coefficient in the tangential direction, dimensionless | Tt | torque generated by tangential forces, N·m |
| E | elastic modulus, Pa | u | fluid flow velocity, m |
| ER | total erosion rate, kg/kg | ∆v | the relative velocities of the centers of the spheres before and after a collision, m·s−1 |
| ERA | erosion rate caused by independent particle impact, kg/kg | vn | particle normal velocity, m·s−1 |
| ERI | erosion rate caused by grouped particle impact, kg/kg | vt | particle tangential velocity, m·s−1 |
| E* | equivalent elastic modulus, Pa | vref | the relative velocity of the contact points, m·s−1 |
| ∆En | normal energy loss of a particle caused by particle-particle collision, J | Vp | volume of particle, m3 |
| ∆Et | tangential energy loss of a particle caused by particle-particle collision, J | Vl | volume of liquid cell, m3 |
| Fb | buoyancy, N | ∆x | space step, m |
| Fd | drag force, N | Y | Young’s modulus, Pa |
| Fg | gravitational force, N | a | empirical constants in angle functions, dimensionless |
| Fl,i | the fluid force acting on a particle, N | b | |
| Fn | inter-particle contact force in the normal direction, N | w | |
| Fp,i | the particle force acting on the liquid, N | x | |
| Fij | contact force between particles, N | y | |
| Ft | inter-particle contact force in the tangential direction, N | z | |
| Fs | particle shape coefficient, dimensionless | Greek symbols | |
| Fnd | inter-particle damping force in the normal direction, N | ωi | unit angular velocity vector of the object, rad·s−1 |
| Ftd | inter-particle damping force in the tangential direction, N | λ | decay coefficient in the collision, dimensionless |
| G | shear modulus, Pa | κ | particle velocity loss rate in process of particle-wall impact, % |
| G* | equivalent shear modulus, Pa | ν | poisson ratio, dimensionless |
| H | maximum depth of indentation, m | δ | tangential displacement, m |
| Δh | depth of the extruding lips, m | μ | dynamic viscosity, Pa·s |
| I | moment of inertia, m4 | β | The angle between the particle velocity and the centerline of the two particles, ° |
| J | particle momentum, kg·m·s−1 | δk | identity tensor |
| K | dimensionless coefficient, dimensionless | δn | normal overlap, m |
| L | distance from the inner edge of the sample, m | δt | tangential overlap, m |
| Lp | critical inter-particle distance of particle, m | ρl | liquid density, kg·m−3 |
| Lp,e | the moving distance of the particle j along the centerline, m | μs | friction coefficient, dimensionless |
| Lp,r | the critical inter-particle distance in the release process, m | μr | rolling friction coefficient, dimensionless |
| Ly | particle spacing in the Y direction, m | φ | scattering angle for particle j, ° |
| Lz | particle spacing in the Z direction, m | ψ | scattering angle for particle i, ° |
| mp | single particle mass, kg | α | particle impact angle, ° |
| m* | equivalent mass, kg | α1 | new particle impact angle, ° |
| N | total particle number, dimensionless | αl | fluid volume fraction, % |
| Np | the number of sample points contained within the mesh cell of the particle, dimensionless | θ | critical angle of particle impact, ° |
| N1 | the number of particles in the particle group, dimensionless | θ* | critical angle between particles, ° |
| Nt | total number of sample points of the particle, dimensionless | ε | strain caused by the normal stress of indentation, m |
| Pn | function of properties of the material, Pa | Superscripts | |
| r | normal unit vector, dimensionless | n | flow behavior index |
| rp | radius of the erosion crater, m | m | impact velocity power law coefficient |
| R | radius of particle, m | (1) | pre-collision |
| Ri | maximum radius of deformation, m | (2) | post-collision |
| R* | equivalent radius, m | Subscripts | |
| Rl | inside radius of a circular pipe, m | i | particle i |
| Sn | normal stiffness, N·m−1 | j | particle j |
| St | tangential stiffness, N·m−1 | z | normal direction |
| t | tangential unit vector, dimensionless | y | tangential direction |
References
- Parsi, M.; Najmi, K.; Najafifard, F.; Hassani, S.; McLaury, B.S.; Shirazi, S.A. A comprehensive review of solid particle erosion modeling for oil and gas wells and pipelines applications. J. Nat. Gas. Sci. Eng. 2014, 21, 850–873. [Google Scholar] [CrossRef]
- Wood, R.J.K.; Jones, T.F.; Ganeshalingam, J.; Miles, N.J. Comparison of predicted and experimental erosion estimates in slurry ducts. Wear 2004, 256, 937–947. [Google Scholar] [CrossRef]
- Zhu, H.; Han, Q.; Wang, J.; He, S.; Wang, D. Numerical investigation of the process and flow erosion of flushing oil tank with nitrogen. Powder Technol. 2015, 275, 12–24. [Google Scholar] [CrossRef]
- Njobuenwu, D.O.; Fairweather, M. Modelling of pipe bend erosion by dilute particle suspensions. Comput. Chem. Eng. 2012, 42, 235–247. [Google Scholar] [CrossRef]
- Zhu, H.P.; Zhou, Z.Y.; Yang, R.Y.; Yu, A.B. Discrete particle simulation of particulate systems: Theoretical developments. Chem. Eng. Sci. 2007, 62, 3378–3396. [Google Scholar] [CrossRef]
- Zhang, Y.; McLaury, B.S.; Shirazi, S.A. Improvements of particle near-wall velocity and erosion predictions using a commercial CFD code. J. Fluids Eng. 2009, 131, 031303. [Google Scholar] [CrossRef]
- Bitter, J.G.A. Study of erosion phenomenon–1,2. Wear 1963, 5–21, 169–190. [Google Scholar] [CrossRef]
- Finnie, I. Erosion of surfaces by solid particles. Wear 1960, 3, 87–103. [Google Scholar] [CrossRef]
- Chen, J.K.; Wang, Y.S.; Li, X.F.; He, R.Y.; Han, S.; Chen, Y.L. Erosion prediction of liquid-particle two-phase flow in pipeline elbows via CFD–DEM coupling method. Powder Technol. 2015, 275, 182–187. [Google Scholar] [CrossRef]
- Zhao, Y.; Xu, L.; Zheng, J. CFD-DEM simulation of tube erosion in a fluidized bed. AiChE J. 2016, 63, 418–437. [Google Scholar] [CrossRef]
- Chen, X.; McLaury, B.S.; Shirazi, S.A. Application and experimental validation of a computational fluid dynamics (CFD)-based erosion prediction model in elbows and plugged tees. Comput. Fluids 2004, 33, 1251–1272. [Google Scholar] [CrossRef]
- McLaury, B.S. Predicting Solid Particle Erosion Resulting from Turbulent Fluctuations in Oilfield Geometries. Ph.D. Thesis, The University of Tulsa, Tulsa, OK, USA, 1996. [Google Scholar]
- Ahlert, K. Effects of Particle Impingement Angle and Surface Wetting on Solid Particle Erosion of AISI 1018 Steel. Master’s Thesis, The University of Tulsa, Tulsa, OK USA, 1994. [Google Scholar]
- Lv, Z.; Huang, C.Z.; Zhu, H.T.; Wang, P.Y.; Liu, Z.W. FEM analysis on the abrasive erosion process in ultrasonic-assisted abrasive waterjet machining. Int. J. Adv. Manuf. Technol. 2015, 78, 1641–1649. [Google Scholar] [CrossRef]
- Bedon, C.; Santarsiero, M. Laminated glass beams with thick embedded connections-Numerical analysis of full-scale specimens during cracking regime. Compos. Struct. 2018, 195, 308–324. [Google Scholar] [CrossRef]
- Larcher, M.; Arrigoni, M.; Bedon, C.; Van Doormaal, J.C.A.M.; Haberacker, C.; Husken, G.; Millon, O.; Saarenheimo, A.; Solomos, G.; Thamie, L.; et al. Design of blast-loaded glazing windows and façades: A review of essential requirements towards standardization. Adv. Civ. Eng. 2016, 2016, 2604232. [Google Scholar] [CrossRef]
- Malka, R.; Nešić, S.; Gulino, D.A. Erosion–corrosion and synergistic effects in disturbed liquid-particle flow. Wear 2007, 262, 791–799. [Google Scholar] [CrossRef]
- Blais, B.; Lassaigne, M.; Goniva, C.; Fradette, L.; Bertrand, F. Development of an unresolved CFD-DEM model for the flow of viscous suspensions and its application to solid-liquid mixing. J. Comput. Phys. 2016, 318, 201–221. [Google Scholar] [CrossRef]
- Iqbal, N.; Rauh, C. Coupling of discrete element model (DEM) with computational fluid mechanics (CFD): A validation study. Appl. Math. Comput. 2016, 277, 154–163. [Google Scholar] [CrossRef]
- Gupta, P.; Sun, J.; Ooi, Y.J. DEM-CFD simulation of a dense fluidized bed: Wall boundary and particle size effects. Powder Technol. 2016, 293, 37–47. [Google Scholar] [CrossRef]
- Mindlin, R.D.; Deresiewicz, H. Elastic spheres in contact under varying oblique forces. J. Appl. Mech. 1953, 20, 327–344. [Google Scholar]
- Barrios, G.K.P.; Carvalho, R.M.D.; Kwade, A.; Tavares, L.M. Contact parameter estimation for DEM simulation of iron ore pellet handling. Powder Technol. 2013, 248, 84–93. [Google Scholar] [CrossRef]
- Chung, Y.C.; Liao, H.H.; Hsiau, S.S. Convection behavior of non-spherical particles in a vibrating bed: Discrete element modeling and experimental validation. Powder Technol. 2013, 237, 53–66. [Google Scholar] [CrossRef]
- Tsuji, Y.; Tanaka, T.; Ishida, T. Lagrangian numerical-simulation of plug flow of cohesionless particles in a horizontal pipe. Powder Technol. 1992, 71, 239–250. [Google Scholar] [CrossRef]
- Chen, J.; Anandarajah, A. Van der Waals attraction between spherical particles. J. Colloid Interface Sci. 1996, 180, 519–523. [Google Scholar] [CrossRef]
- Zhang, Y. Application and Improvement of Computational Fluid Dynamics (CFD) in Solid Particle Erosion Modeling; ProQuest, UMI Dissertations Publishing: Ann Arbor, MI, USA, 2006. [Google Scholar]
- Crowe, C.T.; Schwarzkopf, J.D.; Sommerfeld, M.; Tsuji, Y. Particle-particle interaction. In Multiphase Flows with Droplets and Particles, 2nd ed.; CRC Publishing Inc.: New York, NY, USA, 2011; pp. 119–153. [Google Scholar]
- Walton, O.R. Numerical simulation of inelastic, frictional particle-particle interactions. In Particulate Two-Phase Flow; Roco, M.C., Ed.; Butterworth-Heinemann: Boston, MA, USA, 1993; pp. 1249–1253. [Google Scholar]
- Jenkins, J.T.; Zhang, C. Kinetic theory for identical, frictional, nearly elastic spheres. Phys. Fluids 2002, 14, 1228–1235. [Google Scholar] [CrossRef]
- Yang, L.; Padding, J.T.; Kuipers, J.A.M. Modification of kinetic theory for frictional spheres, Part I: Two-fluid model derivation and numerical implementation. Chem. Eng. Sci. 2016, 152, 767–782. [Google Scholar] [CrossRef]
- Huang, C.K.; Chiovelli, S.; Minev, P.; Luo, J.; Nandakumar, K. A comprehensive phenomenological model for erosion of materials in jet flow. Powder Technol. 2008, 187, 237–279. [Google Scholar] [CrossRef]
- Habib, M.A.; Ben-Mansour, R.; Badr, H.M.; Kabir, M.E. Erosion and penetration rates of a pipe protruded in a sudden contraction. Comput. Fluids 2008, 37, 146–160. [Google Scholar] [CrossRef]
- Habib, M.A.; Badr, H.M.; Ben-Mansour, R.; Kabir, M.E. Erosion rate correlations of a pipe protruded in an abrupt pipe contraction. Int. J. Impact Eng. 2007, 34, 1350–1369. [Google Scholar] [CrossRef]
- Grant, G.; Tabakoff, W. Erosion prediction in turbomachinery resulting from environmental solid particles. J. Aircraft 1975, 12, 471–478. [Google Scholar] [CrossRef]
- Gidaspow, D. Transport Equations. In Multiphase Flow and Fluidization; Academic Press: San Diego, CA, USA, 1994; pp. 36–42. [Google Scholar]
- Meng, H.C.; Ludema, K.C. Wear models and predictive Equations: their form and content. Wear 1995, 181–183, 443–457. [Google Scholar] [CrossRef]
- Zhao, Y.A.; Cai, W.B.; Cui, L.; Cheng, J.R.; Dou, Y.H. Erosion of premium connection cross-over joint in solid-liquid flow. In Proceedings of the International Conference on Engineering Technology and Application ICETA2015, Xia Men, China, November 2015. [Google Scholar]
- ANSYS FLUENT 15.0 User Guide; FLUENT Solutions; ANSYS Inc.: Canonsburg, PA, USA, 2014.
- EDEM 2.7 User Guide; DEM Solutions; DEM Solutions Ltd.: Edinburgh, UK, 2015.
- Ferziger, J.H.; Peric, M.; Leonard, A. Computational methods for fluid dynamics. Phys. Today 1997, 50, 80–84. [Google Scholar] [CrossRef]
- Kremmer, M.; Favier, J.F. A method for representing boundaries in discrete element modelling—Part II: Kinematics. Int. J. Numer. Meth. Eng. 2001, 51, 1423–1436. [Google Scholar] [CrossRef]
- Chen, X.; Zhong, W.; Zhou, X.; Jin, B.; Sun, B. CFD–DEM simulation of particle transport and deposition in pulmonary airway. Powder Technol. 2012, 228, 309–318. [Google Scholar] [CrossRef]
- Hobbs, A. Simulation of an aggregate dryer using coupled CFD and DEM methods. Int. J. Comput. Fluid D 2009, 23, 199–207. [Google Scholar] [CrossRef]
- Xu, Y.; Kafui, K.; Thornton, C.; Lian, G. Effects of material properties on granular flow in a silo using DEM simulation. Part. Sci. Technol. 2002, 20, 109–124. [Google Scholar] [CrossRef]
- Cheng, J.R.; Zhang, N.S.; Li, Z.; Dou, Y.H.; Cao, Y.P. Erosion failure of horizontal pipe reducing wall in power-law fluid containing particles via CFD–DEM coupling method. J. Fail. Anal. Prev. 2017, 17, 1067–1080. [Google Scholar] [CrossRef]
- Hutchings, I.M.; Macmillan, N.H.; Rickerby, D.G. Further studies of the oblique impact of a hard sphere against a ductile solid. Int. J. Mech. Sci. 1981, 23, 639–646. [Google Scholar] [CrossRef]
- Hutchings, I.M.; Winter, R.E. Solid particle erosion studies using single angular particles. Wear 1974, 27, 121–128. [Google Scholar] [CrossRef]
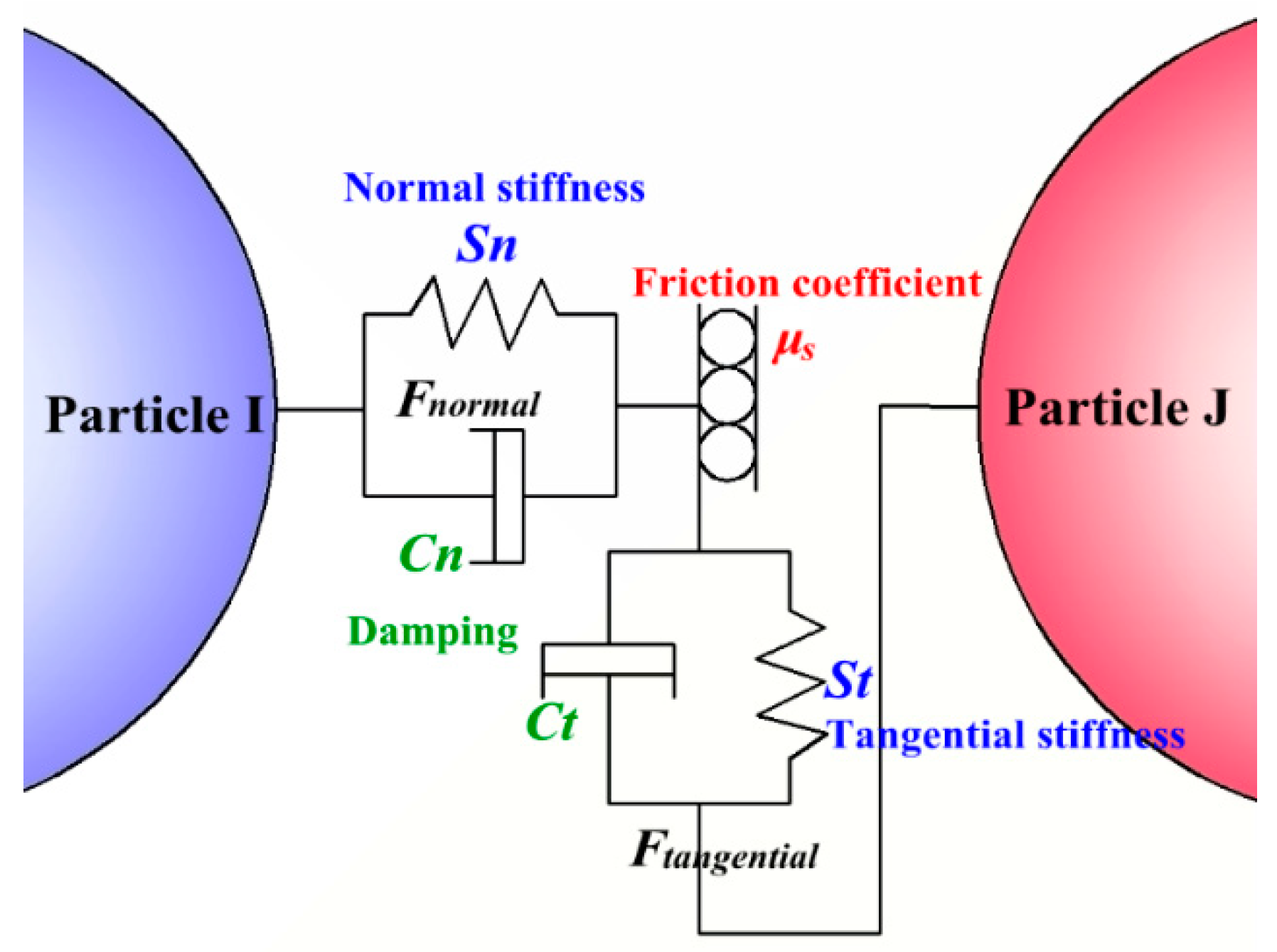
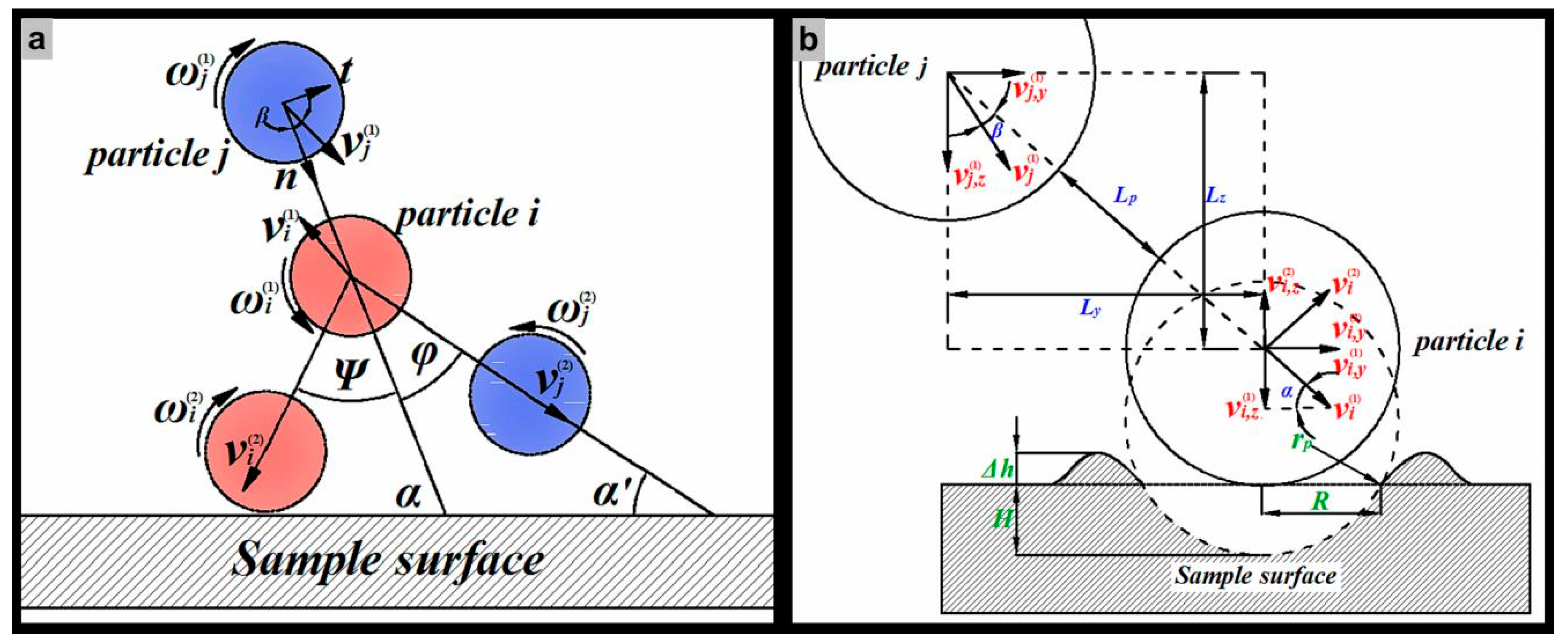



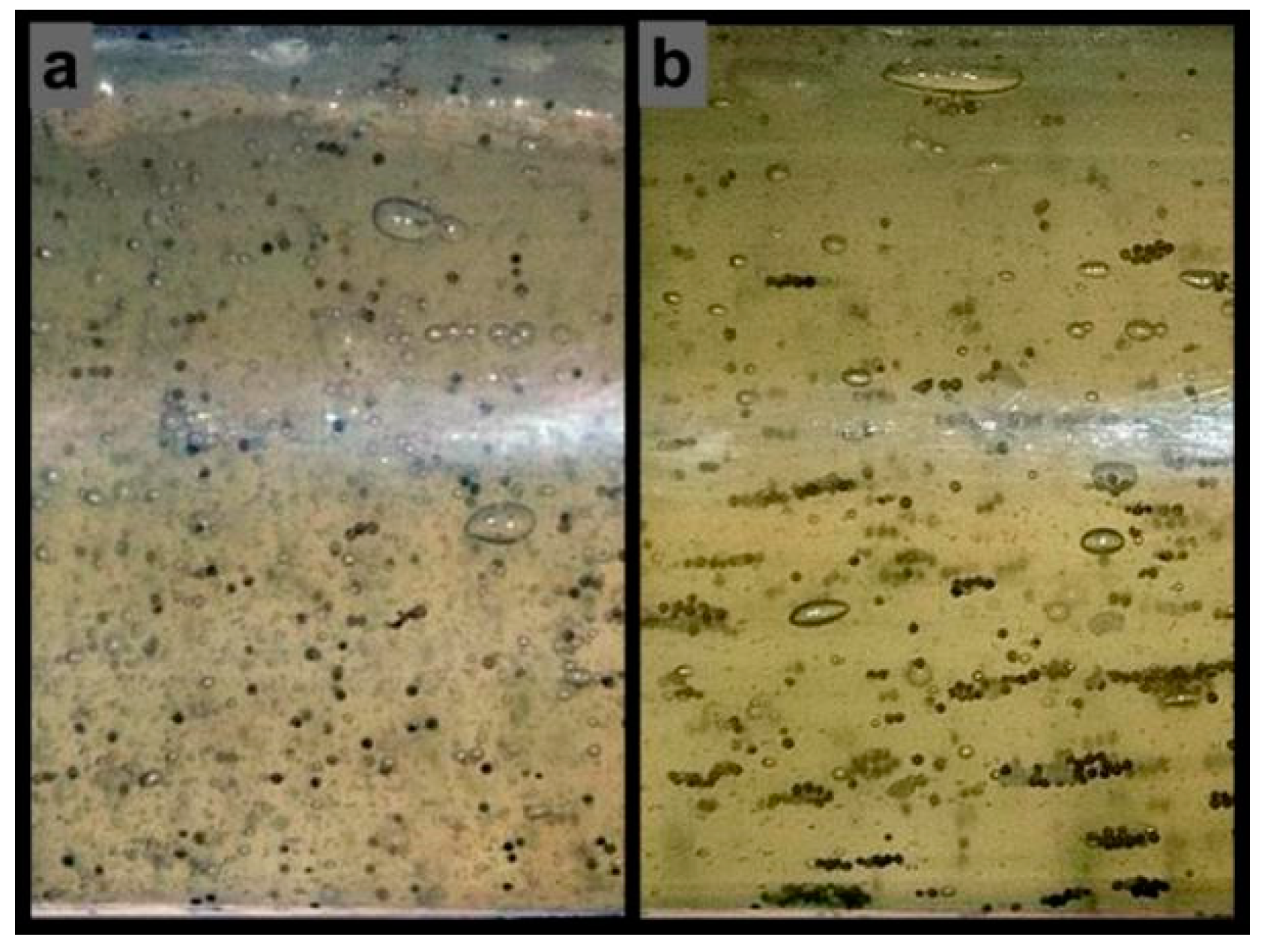



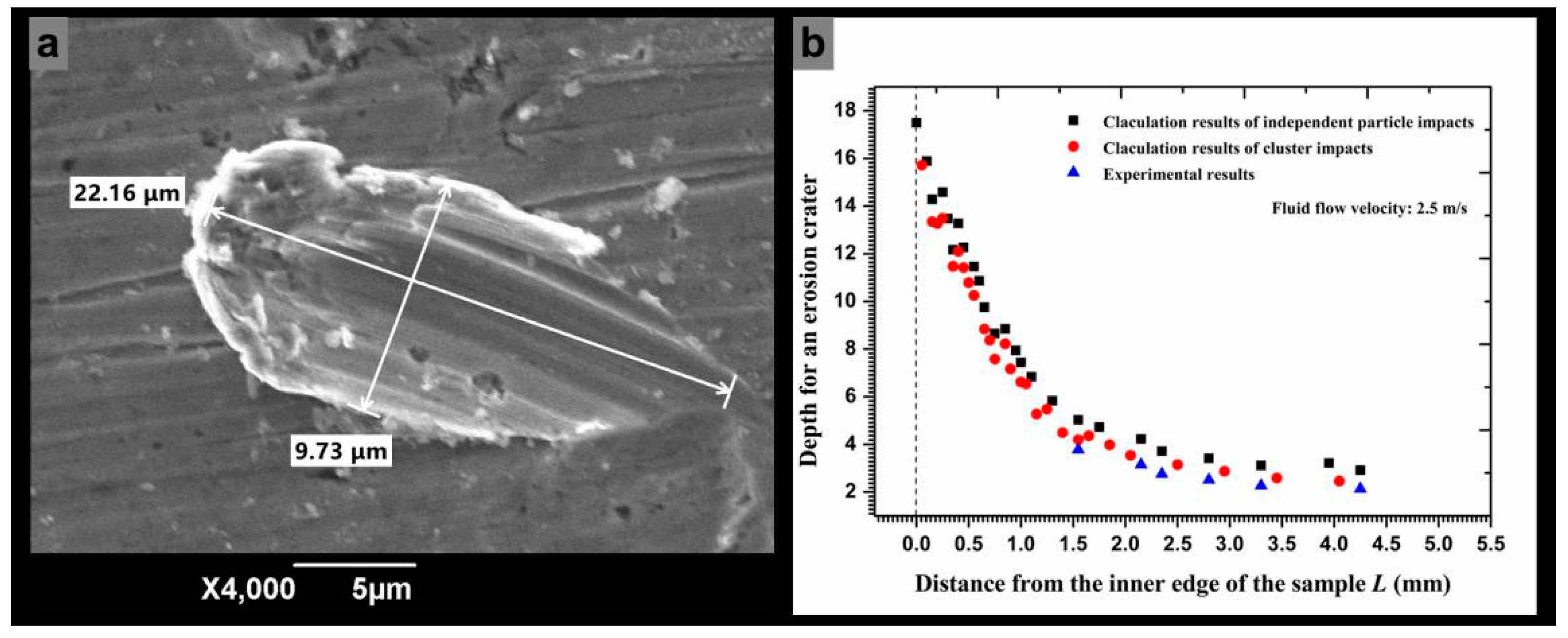
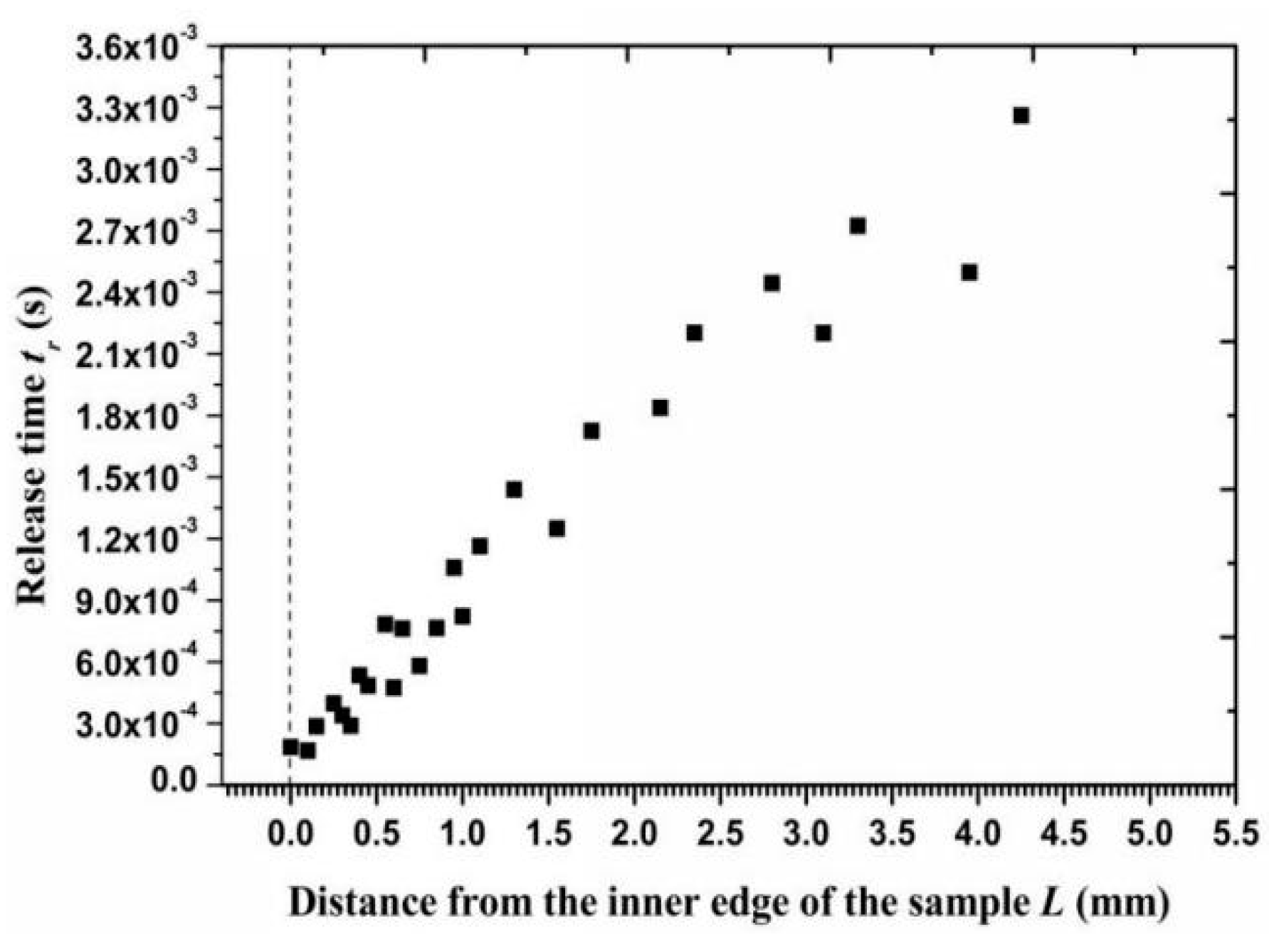

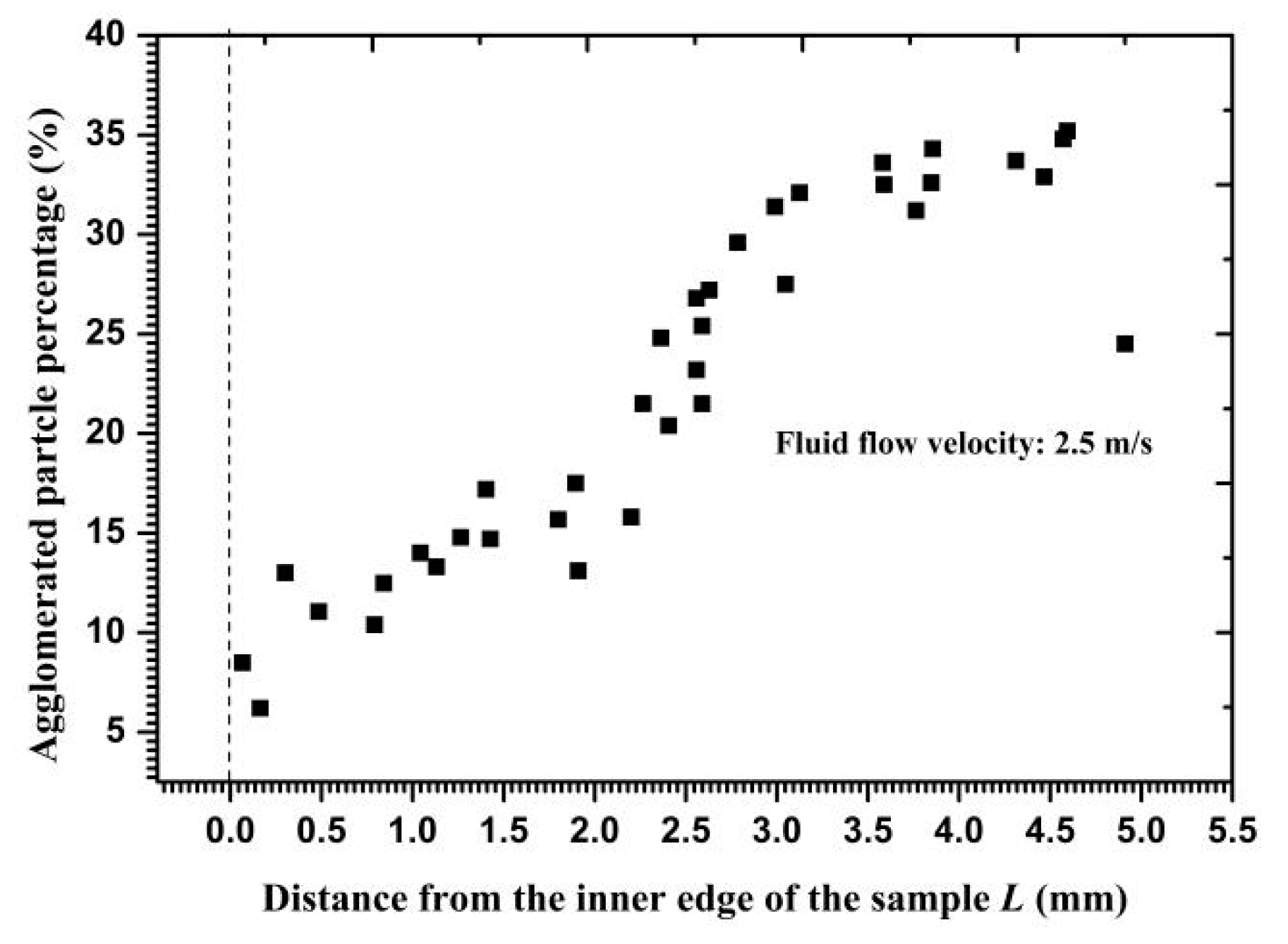



| Parameter | Expression |
|---|---|
| Normal stiffness constant (Sn) | |
| Tangential stiffness constant (St) | |
| Normal damping coefficient (Cn) | |
| Tangential damping coefficient (Ct) | |
| Torque by tangential forces (Tt,ij) | |
| Rolling friction torque (Tr,ij) | |
| Equivalent elastic modulus (E*) | |
| Equivalent shear modulus (G*) | |
| Equivalent radius (R*) | |
| Equivalent mass (m*) | |
| Dimensionless coefficient (γ) | |
| The relative velocities of the centers of the spheres before and after a collision (∆v) | |
| The relative velocity of the contact points | |
| The unit tangential vector (t) | |
| Dimensionless coefficient (K) |
| K | Fs | m | a | b | x | y | z | w | θ |
|---|---|---|---|---|---|---|---|---|---|
| 7.8 × 10−8 | 0.35 | 1.57 | 5.9 × 10−5 | −7.2 × 10−5 | 0.75 | −0.21 | 0.83 | −1.2 | 70 |
| DEM Parameter | Value |
|---|---|
| Liquid phase | |
| Liquid density (kg/m3) | 1020 |
| Liquid viscosity (mPa·s) | 375 |
| Solid phase | |
| Diameter (mm) | 0.65 |
| Mass (mg) | 0.26 |
| Sphericity | 0.85 |
| Particle density (kg/m3) | 1850 |
| Number of particles per calculation in each layer | 60 ± 5 |
| Dimensionless coefficient K | 0.4 |
| Coefficient of normal restitution en | 0.95 |
| Coefficient of normal restitution er | 0.36 |
| Coefficient of friction µ | 0.1 |
| Geometry | |
| Upstream pipe length in the axial direction (mm) | 400 |
| Downstream pipe length in the axial direction (mm) | 200 |
| Upstream pipe diameter (mm) | 50 |
| Downstream pipe diameter (mm) | 25 |
| Total grid cell number | 845, 732 |
| Length of virtual plane (mm) | 10 |
| Width of virtual plane (mm) | 3 |
| The distance from virtual planes to target wall (mm) | 300 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cheng, J.; Dou, Y.; Zhang, N.; Li, Z.; Wang, Z. A New Method for Predicting Erosion Damage of Suddenly Contracted Pipe Impacted by Particle Cluster via CFD-DEM. Materials 2018, 11, 1858. https://doi.org/10.3390/ma11101858
Cheng J, Dou Y, Zhang N, Li Z, Wang Z. A New Method for Predicting Erosion Damage of Suddenly Contracted Pipe Impacted by Particle Cluster via CFD-DEM. Materials. 2018; 11(10):1858. https://doi.org/10.3390/ma11101858
Chicago/Turabian StyleCheng, Jiarui, Yihua Dou, Ningsheng Zhang, Zhen Li, and Zhiguo Wang. 2018. "A New Method for Predicting Erosion Damage of Suddenly Contracted Pipe Impacted by Particle Cluster via CFD-DEM" Materials 11, no. 10: 1858. https://doi.org/10.3390/ma11101858
APA StyleCheng, J., Dou, Y., Zhang, N., Li, Z., & Wang, Z. (2018). A New Method for Predicting Erosion Damage of Suddenly Contracted Pipe Impacted by Particle Cluster via CFD-DEM. Materials, 11(10), 1858. https://doi.org/10.3390/ma11101858