Modeling of Dynamic Behavior of Carbon Fiber-Reinforced Polymer (CFRP) Composite under X-ray Radiation
Abstract
:1. Introduction
2. Mechanism of Stress-Wave Induced by X-ray Pulse
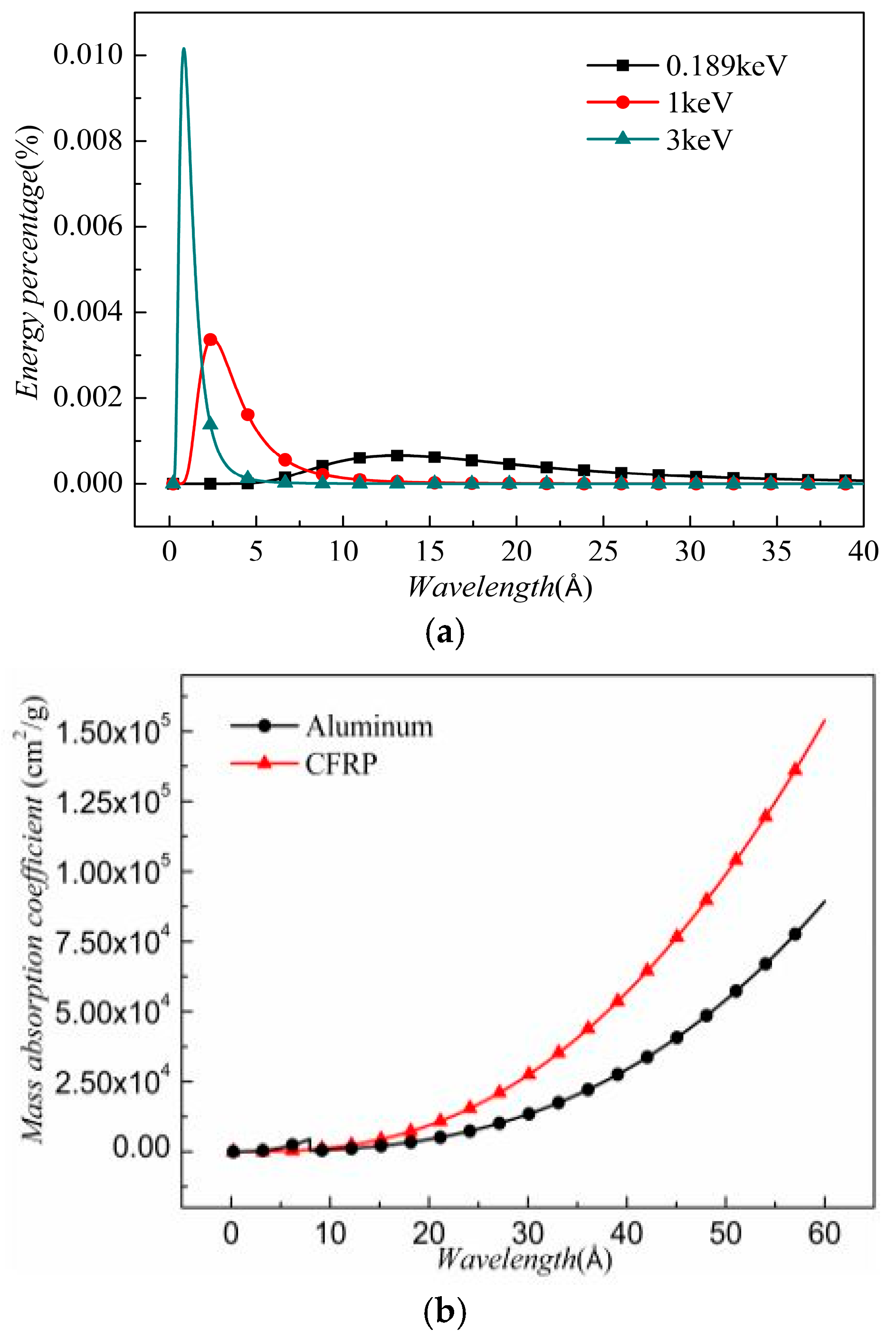
2.1. X-ray Radiation and Energy Deposition
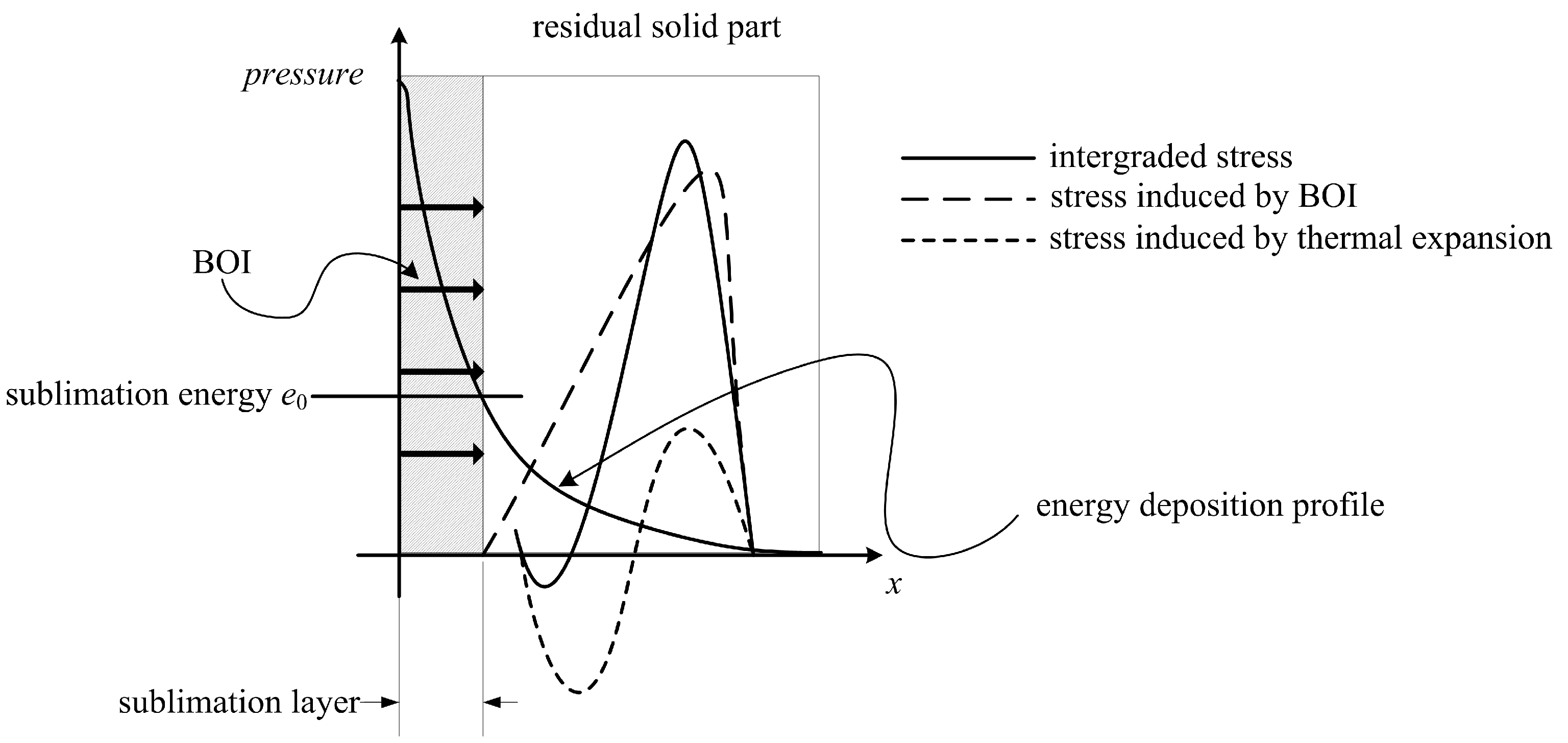
2.2. Stress Induced by Blow-Off Impulse (BOI) and Thermal Expansion
2.3. Elastic Constitutive Model
2.4. Plastic Constitutive Model
2.5. Modified Equation-of-State (EOS)
3. Finite Element Model (FEM)
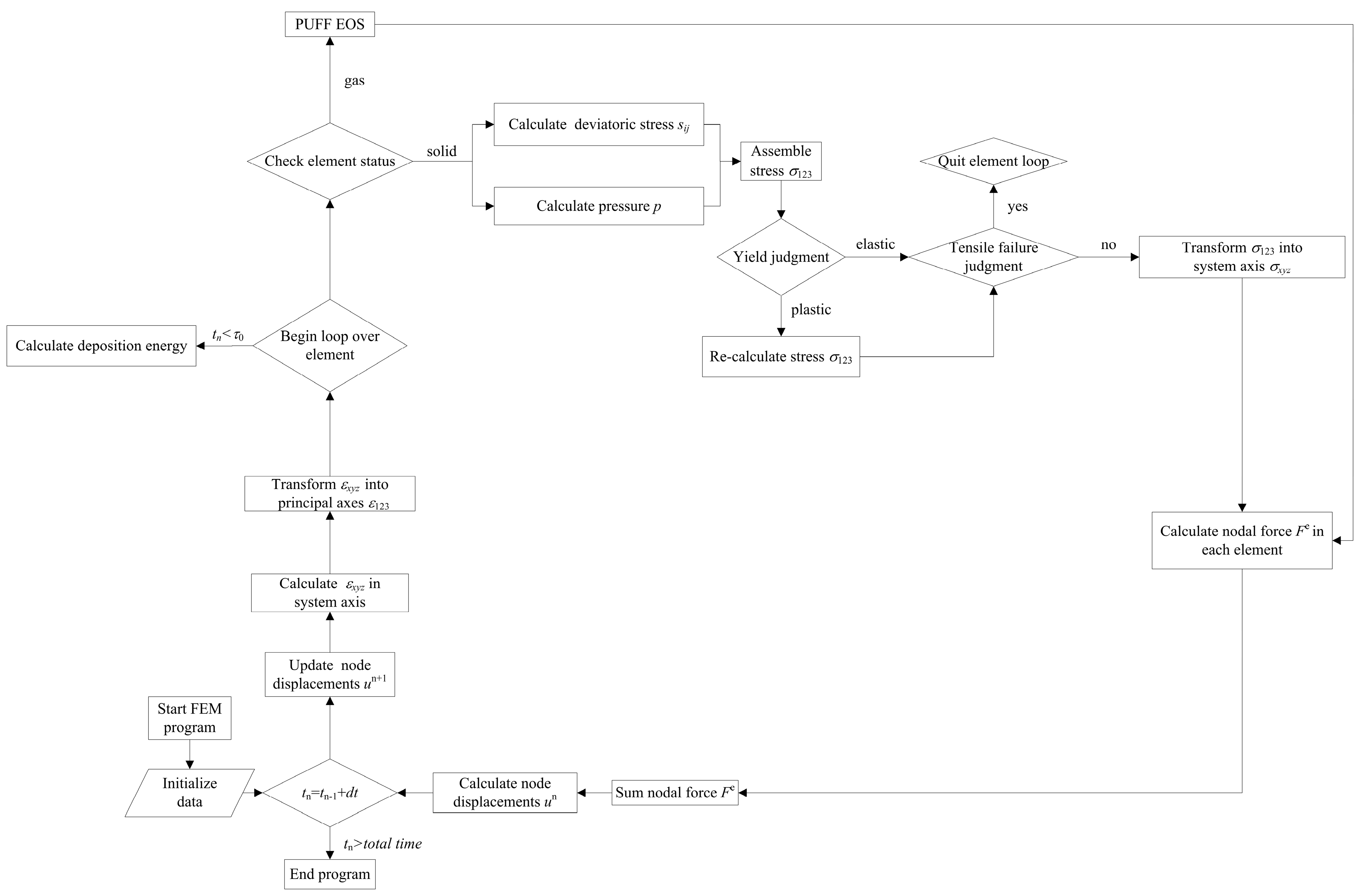
3.1. In-House FEM Code Implementation
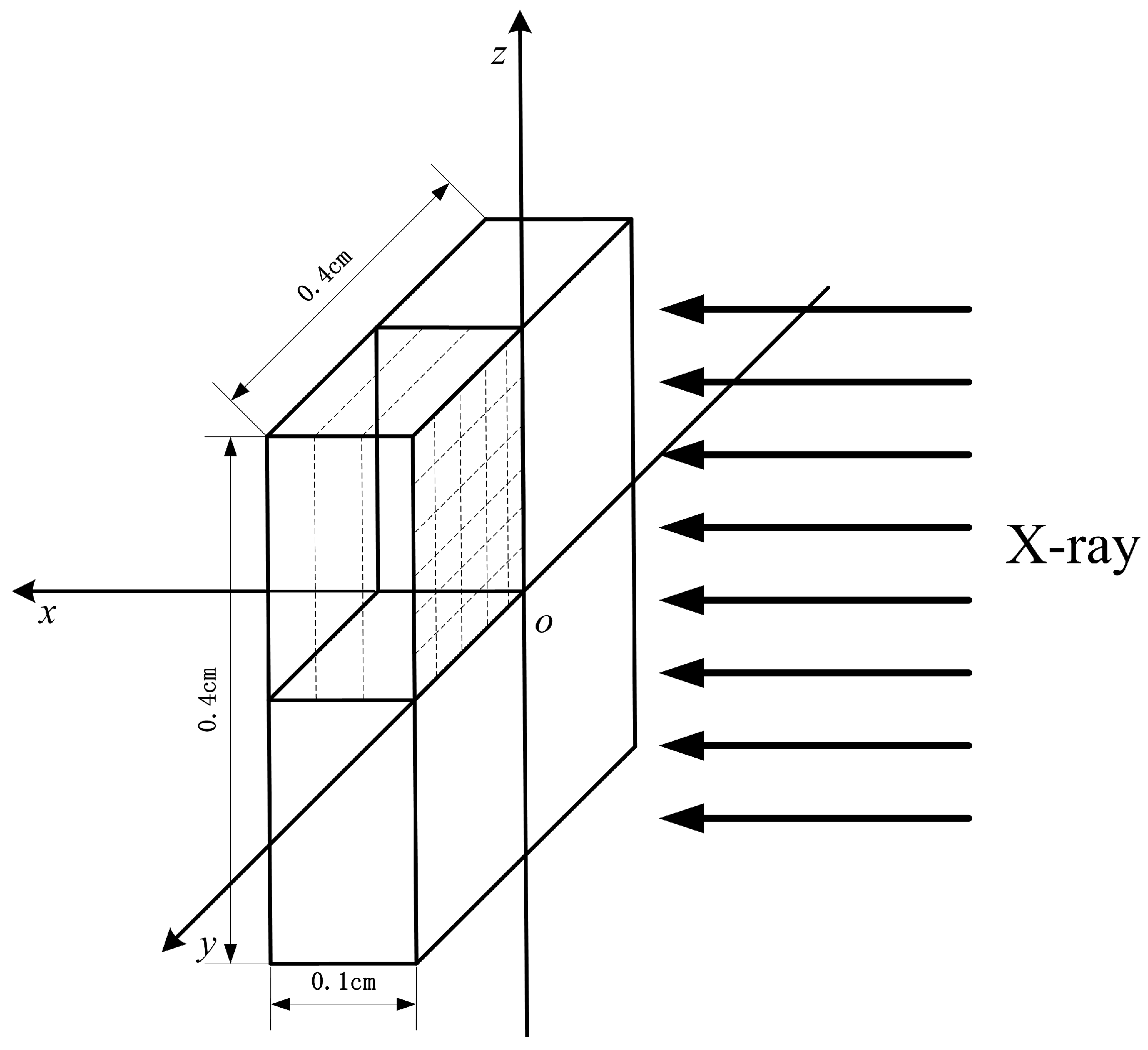
3.2. Geometry and Boundary Conditions
3.3. Material Properties
4. Results and Discussion
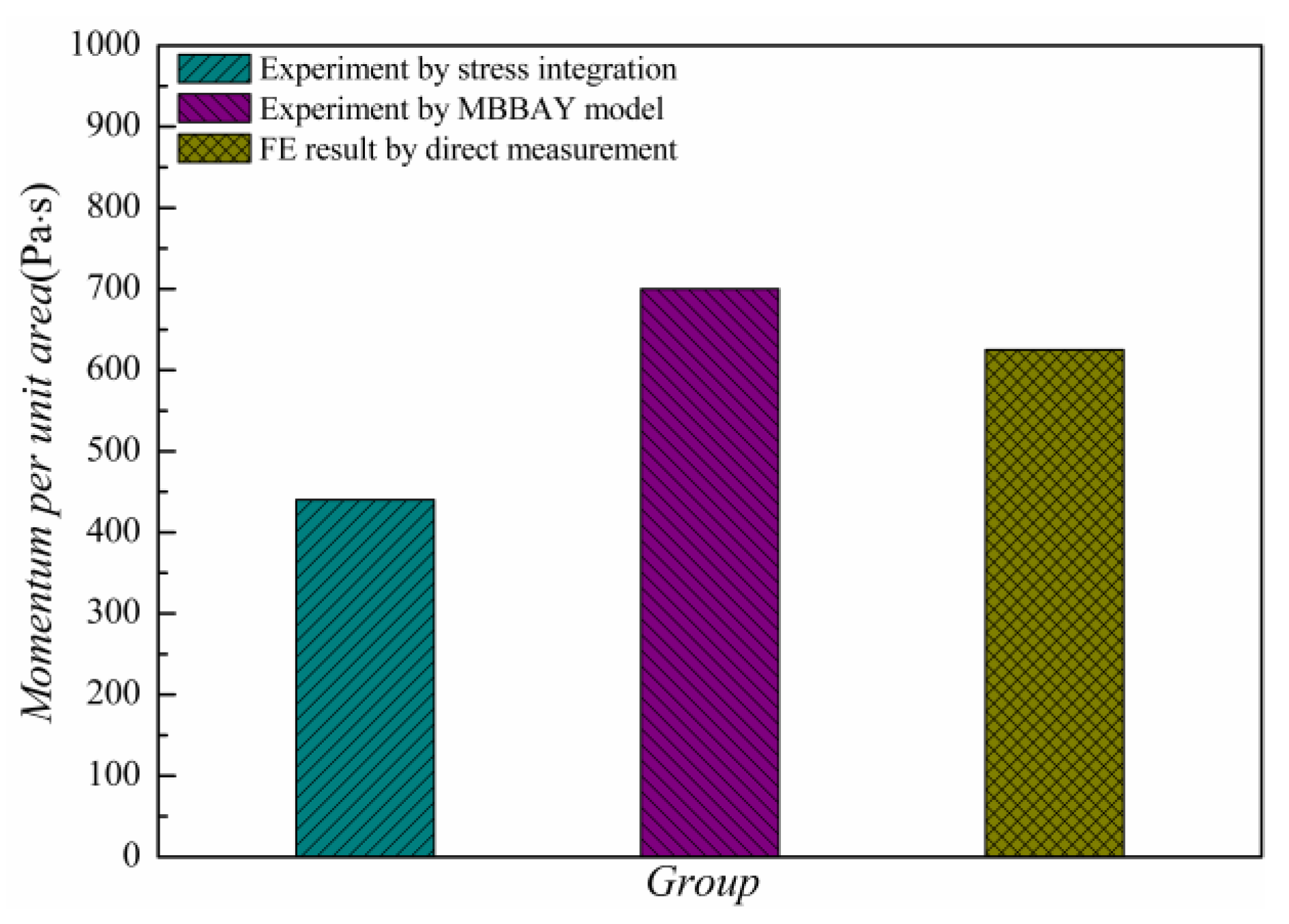
4.1. FEM Program Validation
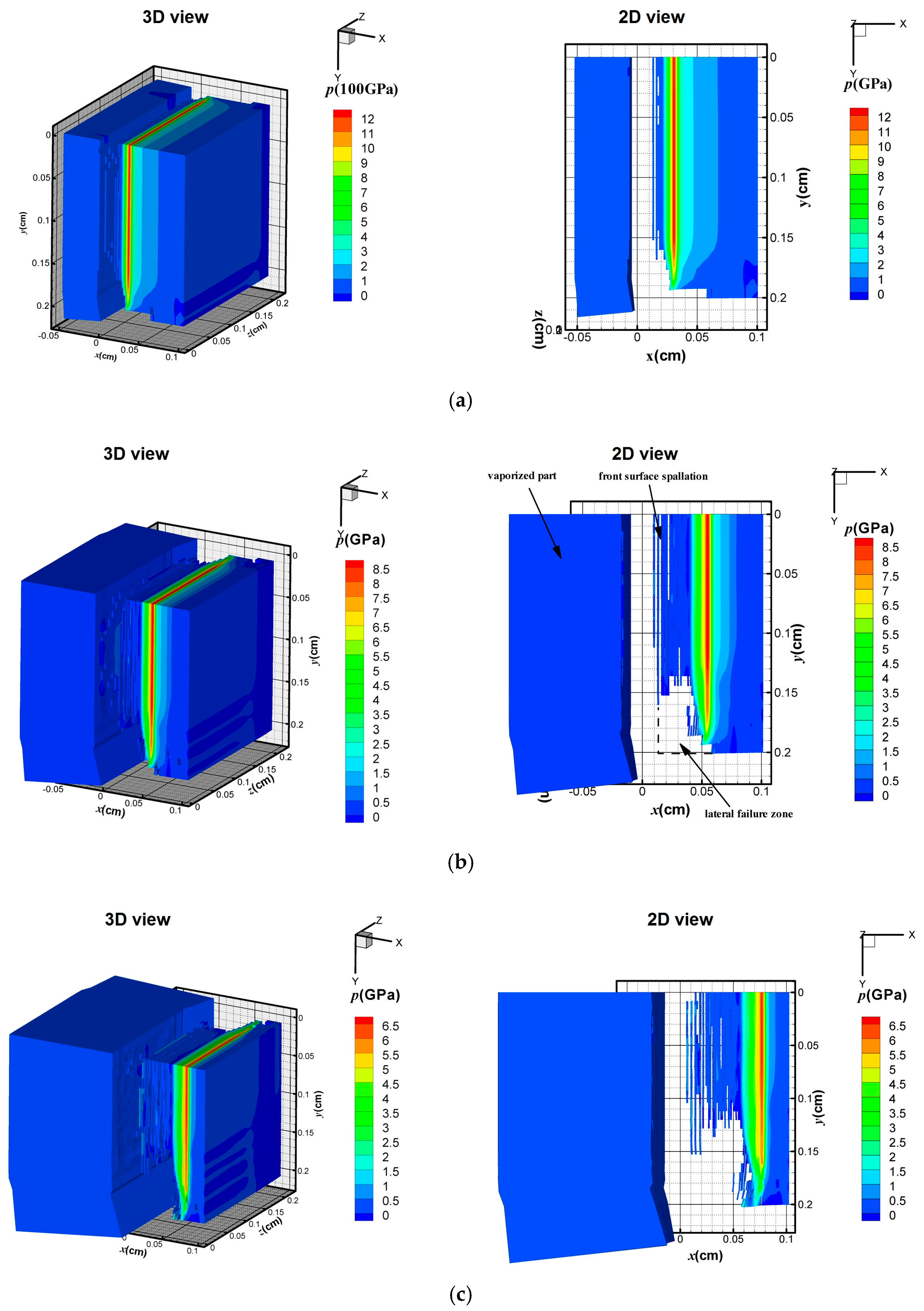
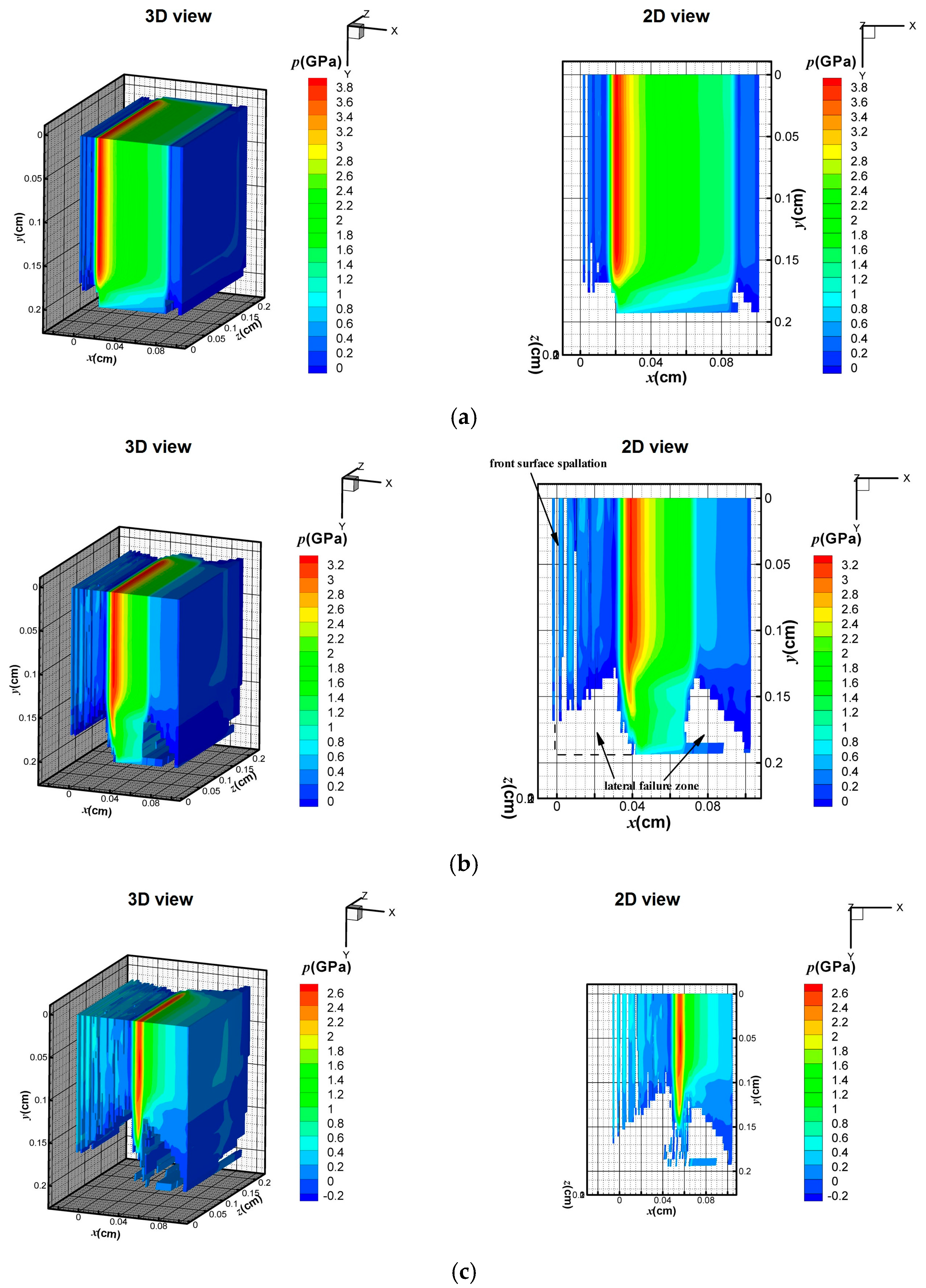
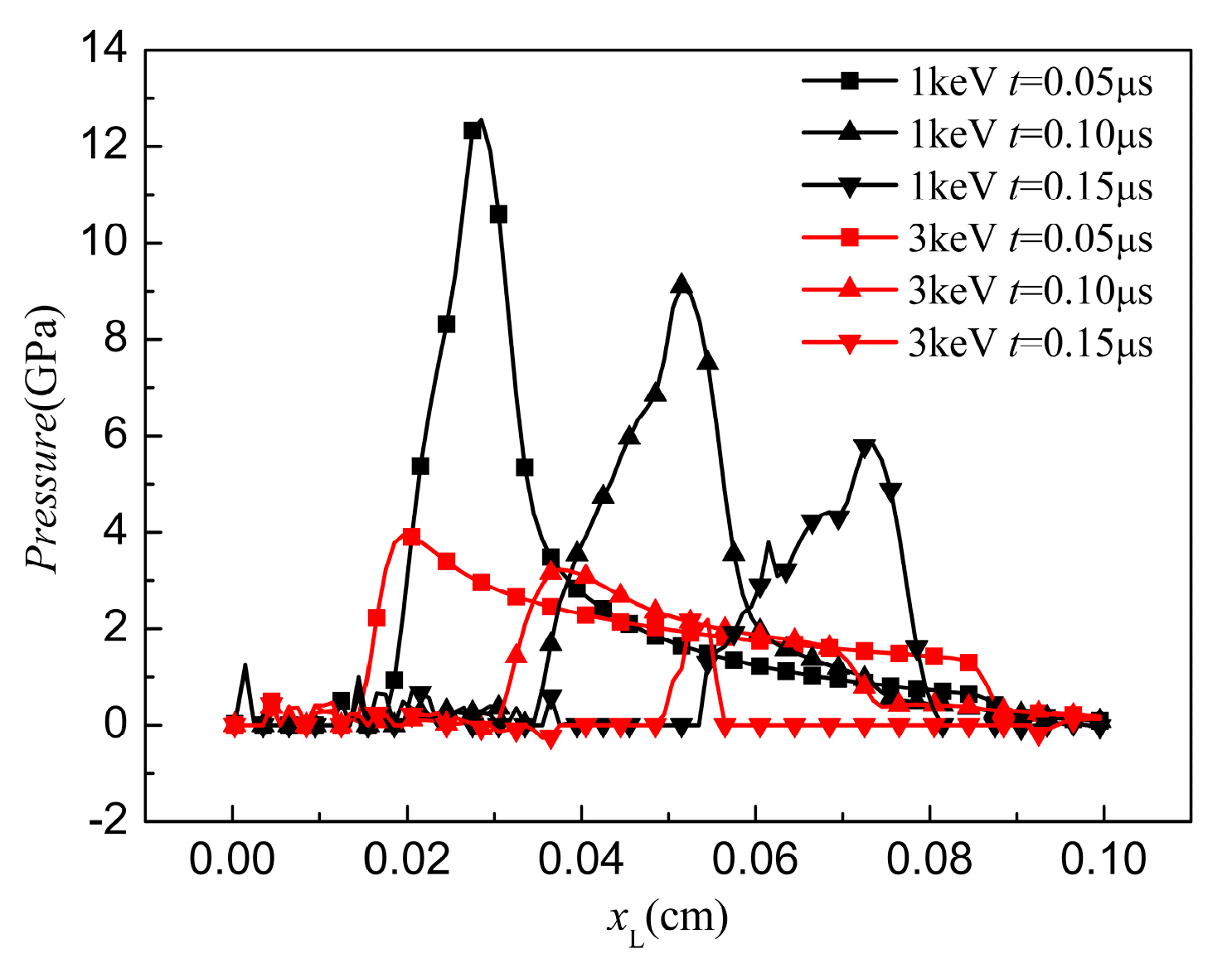
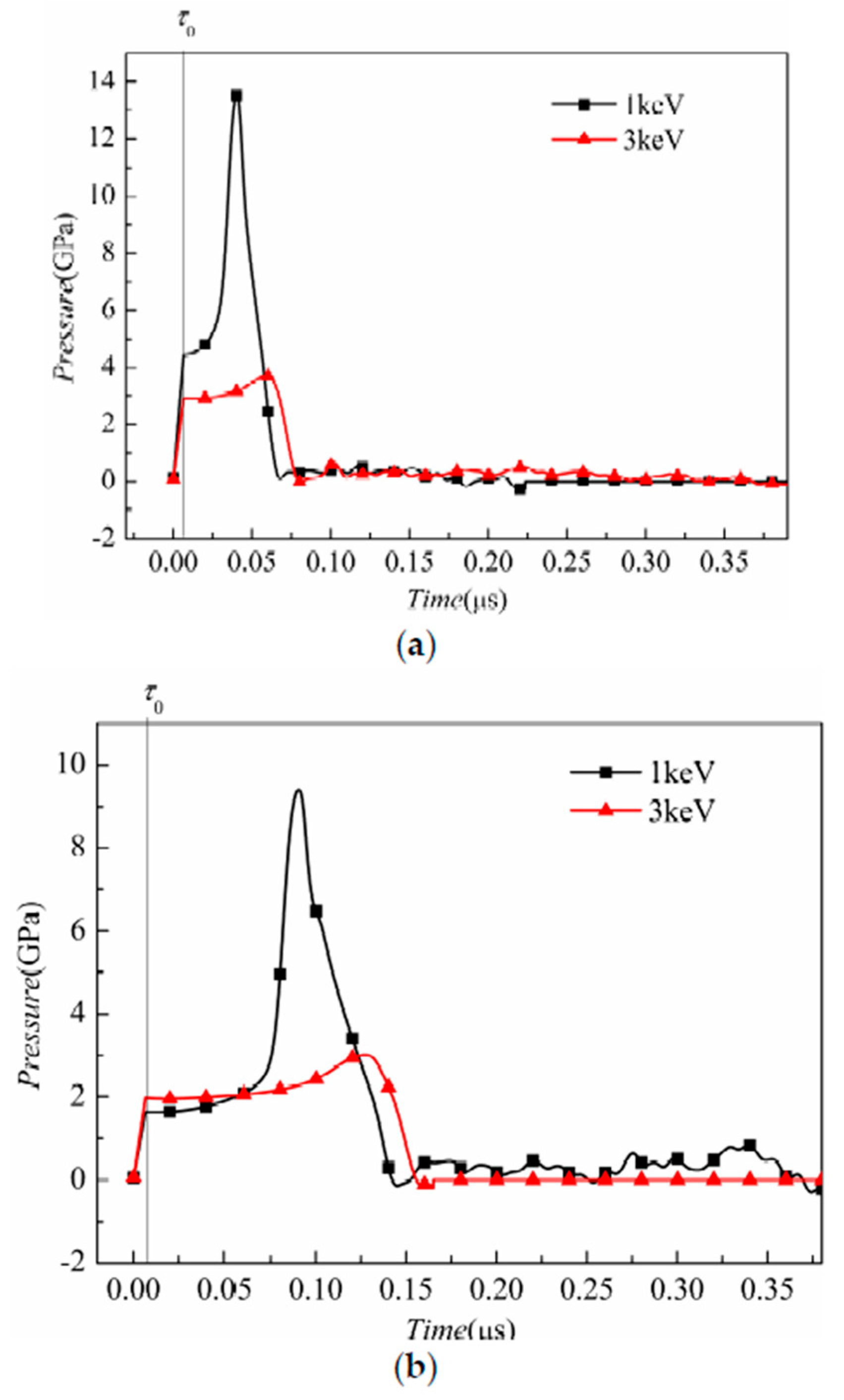
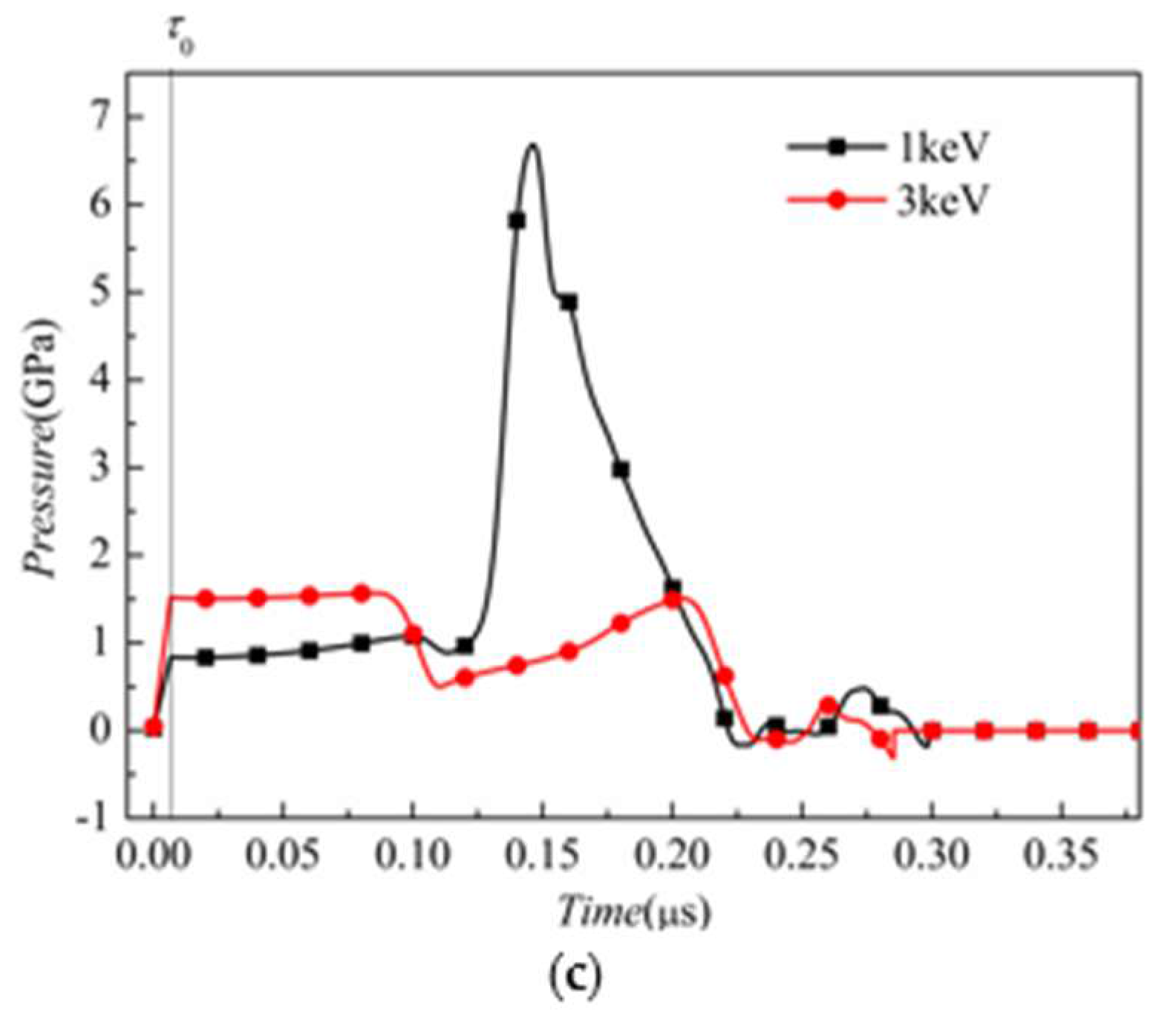
4.2. Dynamic Response ofCarbon Fiber-Reinforced Polymer(CFRP) Composite under Various X-ray Radiations
5. Conclusions
- (1)
- The FE prediction of BOI momentum in an aluminum panel was at the same level as the experimental result, indicating the effectiveness of the FE model.
- (2)
- Compared to the aluminum panel, the CFRP panel effectively reduced the BOI and peak stress under the same radiation condition.
- (3)
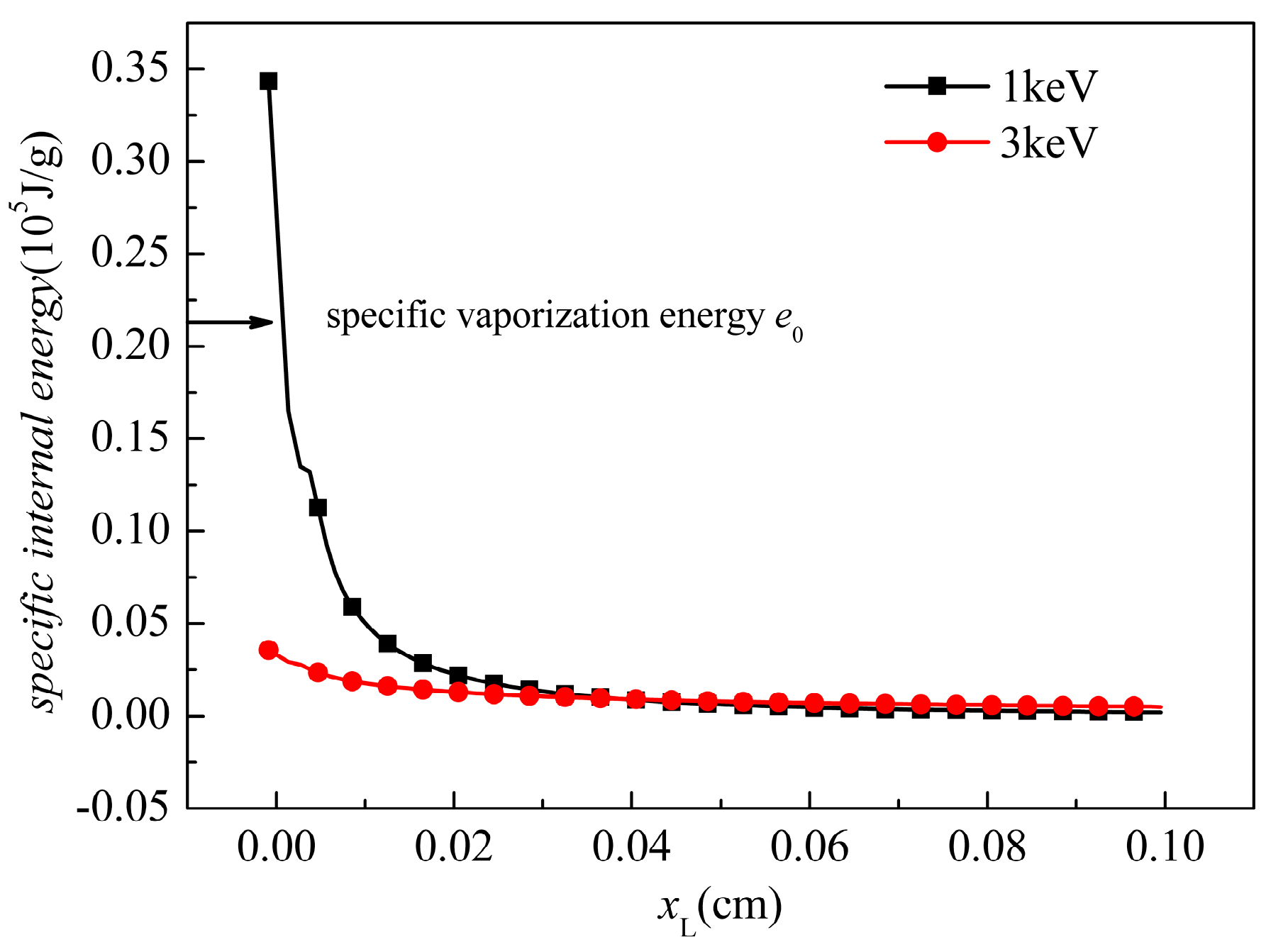
- The FE results revealed that 1 keV X-ray resulted in surface vaporization, whereas no surface vaporization was seen when 3 keV X-ray was used, although front-surface spallation was seen in both cases. Furthermore, the magnitude of the stress wave under 1 keV X-ray was higher than that under 3 keV X-ray.
6. Patents
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Liu, X.; Huang, L.; Xu, M.; Zhang, Z. Influence of different modeling strategies for CFRP on finite element simulation results. In Proceedings of the International Symposium on Materials Application and Engineering (SMAE 2016), MATEC Web of Conferences, Chiang Mai, Thailand, 20–21 August 2016. [Google Scholar]
- Moon, J.-B.; Jang, H.-K.; Kim, C.-G. High velocity impact characteristics of MWNT added CFRP at LEO space environment. Adv. Compos. Mater. 2017, 26, 391–406. [Google Scholar] [CrossRef]
- Ryan, S.; Schaefer, F.; Riedel, W. Numerical simulation of hypervelocity impact on CFRP/Al HC SP spacecraft structures causing penetration and fragment ejection. Int. J. Impact Eng. 2006, 33, 703–712. [Google Scholar] [CrossRef]
- Xu, J.; El Mansori, M. Cutting modeling of hybrid CFRP/Ti composite with induced damage analysis. Materials 2016, 9, 22. [Google Scholar] [CrossRef] [PubMed]
- Du, Z.; Zhu, M.; Wang, Z.; Yang, J. Design and application of composite platform with extreme low thermal deformation for satellite. Compos. Struct. 2016, 152, 693–703. [Google Scholar]
- Martin, A.; Boyd, I.D. Modeling of heat transfer attenuation by ablative gases during the stardust reentry. J. Thermophys. Heat Transf. 2015, 29, 450–466. [Google Scholar] [CrossRef]
- Tran, H.; Johnson, C.; Rasky, D.; Hui, F.; Hsu, M.; Chen, T.; Chen, Y.; Paragas, D.; Kobayashi, L. Phenolic Impregnated Carbon Ablators Pica as Thermal Protection System for Discovery Missions; NASA TM-110440; NASA: Washington, DC, USA, 1997.
- Jung, C.-H.; Lee, D.-H.; Hwang, I.-T.; Im, D.-S.; Shin, J.; Kang, P.-H.; Choi, J.-H. Fabrication and characterization of radiation-resistant ldpe/mwcnt nanocomposites. J. Nucl. Mater. 2013, 438, 41–45. [Google Scholar] [CrossRef]
- Nambiar, S.; Yeow, J.T. Polymer-composite materials for radiation protection. ACS Appl. Mater. Interfaces 2012, 4, 5717–5726. [Google Scholar] [CrossRef] [PubMed]
- Voicu, R. CFRP composite behaviour under extreme environment exposure. Mater. Plast. 2016, 53, 465–469. [Google Scholar]
- Nouri, N.; Panerai, F.; Tagavi, K.A.; Mansour, N.N.; Martin, A. Evaluation of the anisotropic radiative conductivity of a low-density carbon fiber material from realistic microscale imaging. Int. J. Heat Mass Transf. 2016, 95, 535–539. [Google Scholar] [CrossRef]
- Tong, T.; Tien, C. Radiative heat transfer in fibrous insulations—Part I: Analytical study. J. Heat Transf. 1983, 105, 70–75. [Google Scholar] [CrossRef]
- Tong, T.; Yang, Q.; Tien, C. Radiative heat transfer in fibrous insulations—Part II: Experimental study. J. Heat Transf. 1983, 105, 76–81. [Google Scholar] [CrossRef]
- Nouri, N.; Martin, A. Three dimensional radiative heat transfer model for the evaluation of the anisotropic effective conductivity of fibrous materials. Int. J. Heat Mass Transf. 2015, 83, 629–635. [Google Scholar] [CrossRef]
- Johnson, M.H.; Lippmann, B.A. Electromagnetic signals from nuclear explosions in outer space. Phys. Rev. 1960, 119, 827. [Google Scholar] [CrossRef]
- Karzas, W.; Latter, R. Electromagnetic radiation from a nuclear explosion in space. Phys. Rev. 1962, 126, 1919. [Google Scholar] [CrossRef]
- Smith, M.; Santoro, R. Effects of X-Radiation on the Lampshade Orbital Debris Satellite Shield—II; Oak Ridge National Lab.: Oak Ridge, TN, USA, 1990.
- Asay, J.; Hall, C.; Konrad, C.; Trott, W.; Chandler, G.; Fleming, K.; Holland, K.; Chhabildas, L.; Mehlhorn, T.; Vesey, R.; et al. Use of Z-pinch sources for high-pressure equation-of-state studies. Int. J. Impact Eng. 1999, 23, 27–38. [Google Scholar] [CrossRef]
- Lawrence, R.; Furnish, M.; Remo, J.L. Analytic Models for Pulsed X-ray Impulse Coupling. AIP Conf. Proc. 2012, 1426, 883–886. [Google Scholar]
- Remo, J.; Furnish, M. Analysis of Z-pinch shock wave experiments on meteorite and planetary materials. Int. J. Impact Eng. 2008, 35, 1516–1521. [Google Scholar] [CrossRef]
- Remo, J.; Lawrence, R.; Jacobsen, S.; Furnish, M. High energy density soft X-ray momentum coupling to comet analogs for neo mitigation. Acta Astronaut. 2016, 129, 384–388. [Google Scholar] [CrossRef]
- Remo, J.L.; Furnish, M.D.; Lawrence, R.J. Plasma-driven Z-pinch X-ray loading and momentum coupling in meteorite and planetary materials. J. Plasma Phys. 2013, 79, 121–141. [Google Scholar] [CrossRef]
- Langley, R. Analytical Relationships for Estimating the Effects of X-rays on Materials; McDonnell Douglas Astronautics Company-Huntington Beach: Huntington Beach, CA, USA, 1974. [Google Scholar]
- Lawrence, R. The Equivalence of Simple Models for Radiation-Induced Impulse; Sandia National Labs.: Albuquerque, NM, USA, 1991.
- Morland, L. Generation of thermoelastic stress waves by impulsive electromagnetic radiation. AIAA J. 1968, 6, 1063. [Google Scholar] [CrossRef]
- Gascoigne, H.; McIvor, I. Stress waves in layered thermoelastic media generated by impulsive energy deposition. AIAA J. 1971, 9, 937–943. [Google Scholar] [CrossRef]
- Cost, T.L. Dynamic Response of Missile Structures to Impulsive Loads Caused by Nuclear Effects Blowoff; Athena Engineering Company: Northport, AL, USA, 1976. [Google Scholar]
- Huang, X.; Tang, W.; Jiang, B. A modified anisotropic puff equation of state for composite materials. J. Compos. Mater. 2012, 46, 499–506. [Google Scholar] [CrossRef]
- Anderson, C.E.; Cox, P.; Johnson, G.; Maudlin, P. A constitutive formulation for anisotropic materials suitable for wave propagation computer programs—II. Comput. Mech. 1994, 15, 201–223. [Google Scholar] [CrossRef]
- O’Donoghue, P.E.; Anderson, C.E., Jr.; Friesenhahn, G.J.; Parr, C.H. A constitutive formulation for anisotropic materials suitable for wave propagation computer programs. J. Compos. Mater. 1992, 26, 1860–1884. [Google Scholar] [CrossRef]
- Clegg, R.; White, D.; Riedel, W.; Harwick, W. Hypervelocity impact damage prediction in composites: Part I—Material model and characterisation. Int. J. Impact Eng. 2006, 33, 190–200. [Google Scholar] [CrossRef]
- Riedel, W.; Nahme, H.; White, D.M.; Clegg, R.A. Hypervelocity impact damage prediction in composites: Part II—Experimental investigations and simulations. Int. J. Impact Eng. 2006, 33, 670–680. [Google Scholar] [CrossRef]
- Grujicic, M.; Bell, W.; Thompson, L.; Koudela, K.; Cheeseman, B. Ballistic-protection performance of carbon-nanotube-doped poly-vinyl-ester-epoxy matrix composite armor reinforced with e-glass fiber mats. Mater. Sci. Eng. A 2008, 479, 10–22. [Google Scholar] [CrossRef]
- Grujicic, M.; Pandurangan, B.; Koudela, K.; Cheeseman, B. A computational analysis of the ballistic performance of light-weight hybrid composite armors. Appl. Surf. Sci. 2006, 253, 730–745. [Google Scholar] [CrossRef]
- Haro, E.E.; Odeshi, A.G.; Szpunar, J.A. The energy absorption behavior of hybrid composite laminates containing nano-fillers under ballistic impact. Int. J. Impact Eng. 2016, 96, 11–22. [Google Scholar] [CrossRef]
- Min, S.; Chen, X.; Chai, Y.; Lowe, T. Effect of reinforcement continuity on the ballistic performance of composites reinforced with multiply plain weave fabric. Compos. Part B Eng. 2016, 90, 30–36. [Google Scholar] [CrossRef]
- Brode, H.L. Thermal Radiation from Nuclear Explosions; Rand Corp.: Santa Monica, CA, USA, 1963. [Google Scholar]
- Sasaki, S. X-ray Absorption Coefficients of the Elements (Li to Bi, U); National Laboratory for High Energy Physics: Tsukuba, Japan, 1990.
- Zhang, K.; Tang, W.; Ran, X. Study on X-ray energy deposition in hexahedron element and its application in cylindrical shell. At. Energy Sci. Technol. 2017, 51, 1084–1089. [Google Scholar]
- Chen, J.; Allahdadi, F.; Sun, C. A quadratic yield function for fiber-reinforced composites. J. Compos. Mater. 1997, 31, 788–811. [Google Scholar] [CrossRef]
- Chen, J.; Allahdadi, F.A.; Carney, T.C. High-velocity impact of graphite/epoxy composite laminates. Compos. Sci. Technol. 1997, 57, 1369–1379. [Google Scholar] [CrossRef]
- Liu, Z.; Yue, Z.; Wang, F.; Ji, Y. Combining analysis of coupled electrical-thermal and blow-off impulse effects on composite laminate induced by lightning strike. Appl. Compos. Mater. 2015, 22, 189–207. [Google Scholar] [CrossRef]
- Key, C.T.; Schumacher, S.C. CTH Reference Manual: Composite Capability and Technologies; Sandia National Laboratories: Albuquerque, NM, USA, 2009.
- Wicklein, M.; Ryan, S.; White, D.; Clegg, R. Hypervelocity impact on CFRP: Testing, material modelling, and numerical simulation. Int. J. Impact Eng. 2008, 35, 1861–1869. [Google Scholar] [CrossRef]
- Wu, H.-C. Continuum Mechanics and Plasticity; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]

| Elastic Parameters | Plastic Parameters | EOS Parameters | Failure Parameters | ||||
|---|---|---|---|---|---|---|---|
| E11 (GPa) | 9.7 | a11 | 0.669 | K′ (GPa) | 25.04 | σ11 (GPa) | 0.2457 |
| E22 (GPa) | 72.9 | a22 | 0.025 | 1.098 | σ22 (GPa) | 0.6190 | |
| E33 (GPa) | 22.89 | a33 | 1 | s | 1.049 | σ33 (GPa) | 0.1950 |
| v12 | 0.0187 | a12 | 0 | γ | 1.667 | σ12 (GPa) | 0.0475 |
| v13 | 0.218 | a13 | −0.471 | Estimated c0 (m/s) | 4003 | σ13 (GPa) | 0.0285 |
| v23 | 0.77 | a23 | −0.128 | ρ0 (g/cm3) | 1.563 | σ23 (GPa) | 0.0393 |
| G12 (GPa) | 0.873 | a44 | 0.061 | Estimated e0 (kJ/g) | 21.5 | ||
| G13 (GPa) | 0.558 | a55 | 3.157 | ||||
| G23 (GPa) | 48.35 | a66 | 2.128 | ||||
| Elastic Parameters | Plastic Parameters | EOS Parameters | Failure Parameters | ||||
|---|---|---|---|---|---|---|---|
| E (GPa) | 71.71 | k (GPa) | 0.5 | K (GPa) | 78.73 | σ (GPa) | 1.2 |
| v | 0.33 | 2.18 | |||||
| G (GPa) | 27.1 | s | 1.35 | ||||
| γ | 1.667 | ||||||
| c0 (m/s) | 5400 | ||||||
| ρ0 (g/cm3) | 2.7 | ||||||
| e0 (kJ/g) | 13.5 | ||||||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, K.; Tang, W.; Fu, K. Modeling of Dynamic Behavior of Carbon Fiber-Reinforced Polymer (CFRP) Composite under X-ray Radiation. Materials 2018, 11, 143. https://doi.org/10.3390/ma11010143
Zhang K, Tang W, Fu K. Modeling of Dynamic Behavior of Carbon Fiber-Reinforced Polymer (CFRP) Composite under X-ray Radiation. Materials. 2018; 11(1):143. https://doi.org/10.3390/ma11010143
Chicago/Turabian StyleZhang, Kun, Wenhui Tang, and Kunkun Fu. 2018. "Modeling of Dynamic Behavior of Carbon Fiber-Reinforced Polymer (CFRP) Composite under X-ray Radiation" Materials 11, no. 1: 143. https://doi.org/10.3390/ma11010143
APA StyleZhang, K., Tang, W., & Fu, K. (2018). Modeling of Dynamic Behavior of Carbon Fiber-Reinforced Polymer (CFRP) Composite under X-ray Radiation. Materials, 11(1), 143. https://doi.org/10.3390/ma11010143





