4.1. Results for Density and Tension
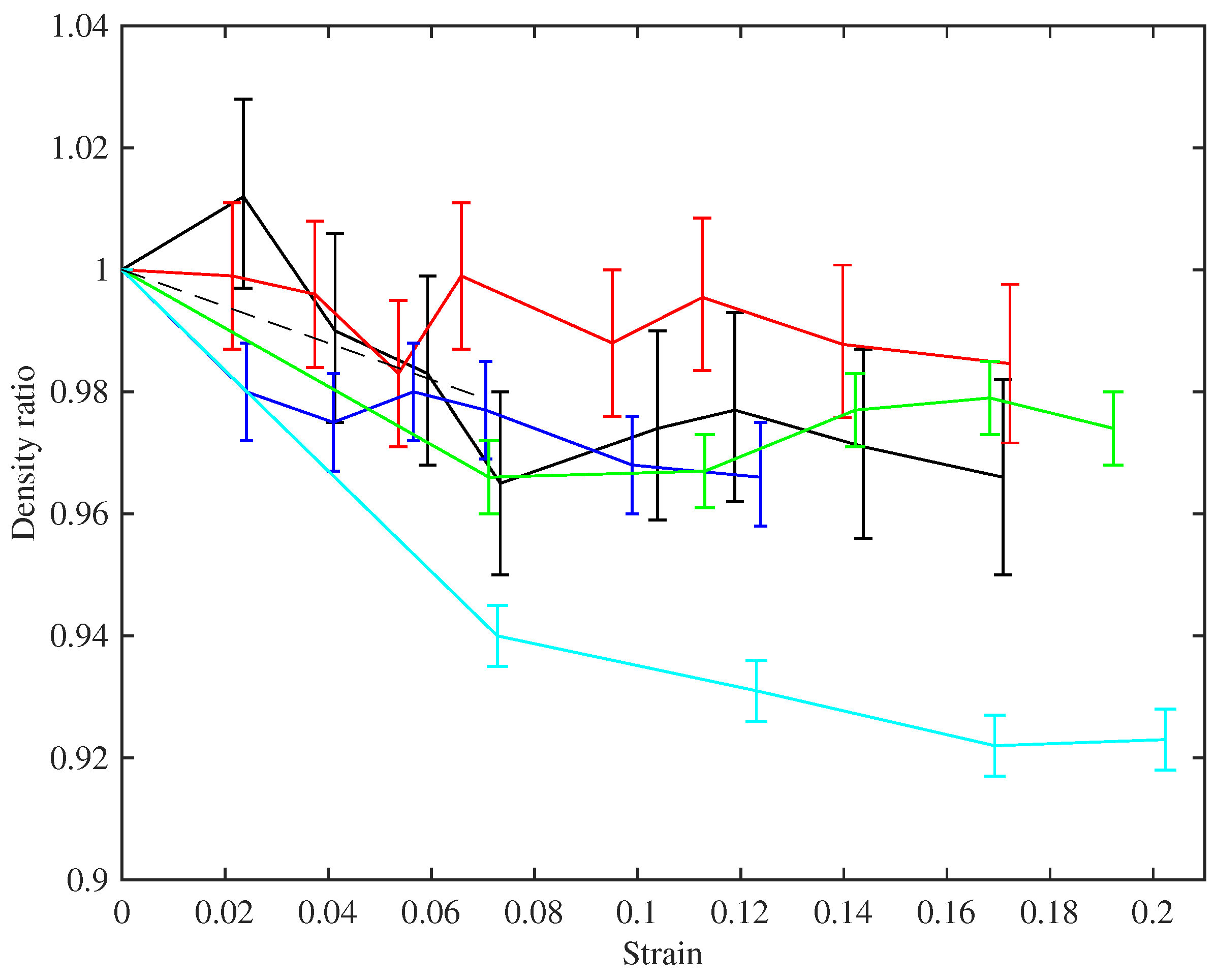
Figure 4 shows the ratio of the measured bulk density of the stretched strings to their unstretched bulk density, plotted against the strain. The stretched density values were calculated from the linear density at the base temperature, obtained from the constant length tests as described above, and the string diameter, which was measured using a manual micrometer with a resolution of 0.01 mm. The derivation of the strain values is described below in
Section 4.2. With the string mounted in the test rig, the diameter could only be measured in a few places and could therefore not be determined as accurately as when the string was first measured prior to testing (when multiple measurements were taken to reduce the measurement error and take better account of any variations along the string length). The error bars in
Figure 4 show the range in the density ratio values that could be expected given an error range of ±0.005 mm in the measured string diameter.
The measurements are quite noisy, but the trends follow the expectation described in the theoretical development in
Section 2. The string density falls on initial stretching, approximately following the prediction of Equation (
3), shown as the dashed line. Within the accuracy of these measurements, the density then remains essentially constant as the strings were stretched further: notice the very narrow range on the vertical axis in the plot. A useful corollary in terms of tuning adjustment is that maintaining a string at constant frequency should have the effect of maintaining it at constant stress, from Equation (
1). String 29 is an obvious outlier in this plot, showing a similar qualitative trend, but with density levelling out to around 92% of the original value, rather than something around 97% as seen for the other strings.
One important practical problem when designing strings is to predict the working tension once the string has settled.
Figure 5 shows the measured tension
of the different strings, at the base temperature of 20 °C, plotted against the notional tension
calculated from Equation (
1) using the unstretched linear density. Linear regression analysis has been used to fit a line through the operating points (black circles) for the nine Bowbrand strings (excluding String 29) tested at the usual humidity levels (around 55–65% RH at 20 °C). The line was constrained to go through the origin and gave a very good fit (
) with a gradient of 0.896. It is reassuring to see that the points for the Pirastro strings (blue circles) and the two Bowbrand strings (red circles) tested at lower humidity levels (around 20–26% RH at 20 °C) also lie close to the fitted line. This suggests that, as a rule of thumb, the working tension of a monofilament nylon musical string will be about 10% lower than the notional value obtained from its unstretched linear density. For the strings tested at multiple target frequencies, it can be seen that the actual tension fell further below the notional values as the tension increased, because the string linear density decreases, primarily as a result of a reduction in its diameter, as the string is stretched.
4.2. Results for Young’s Modulus
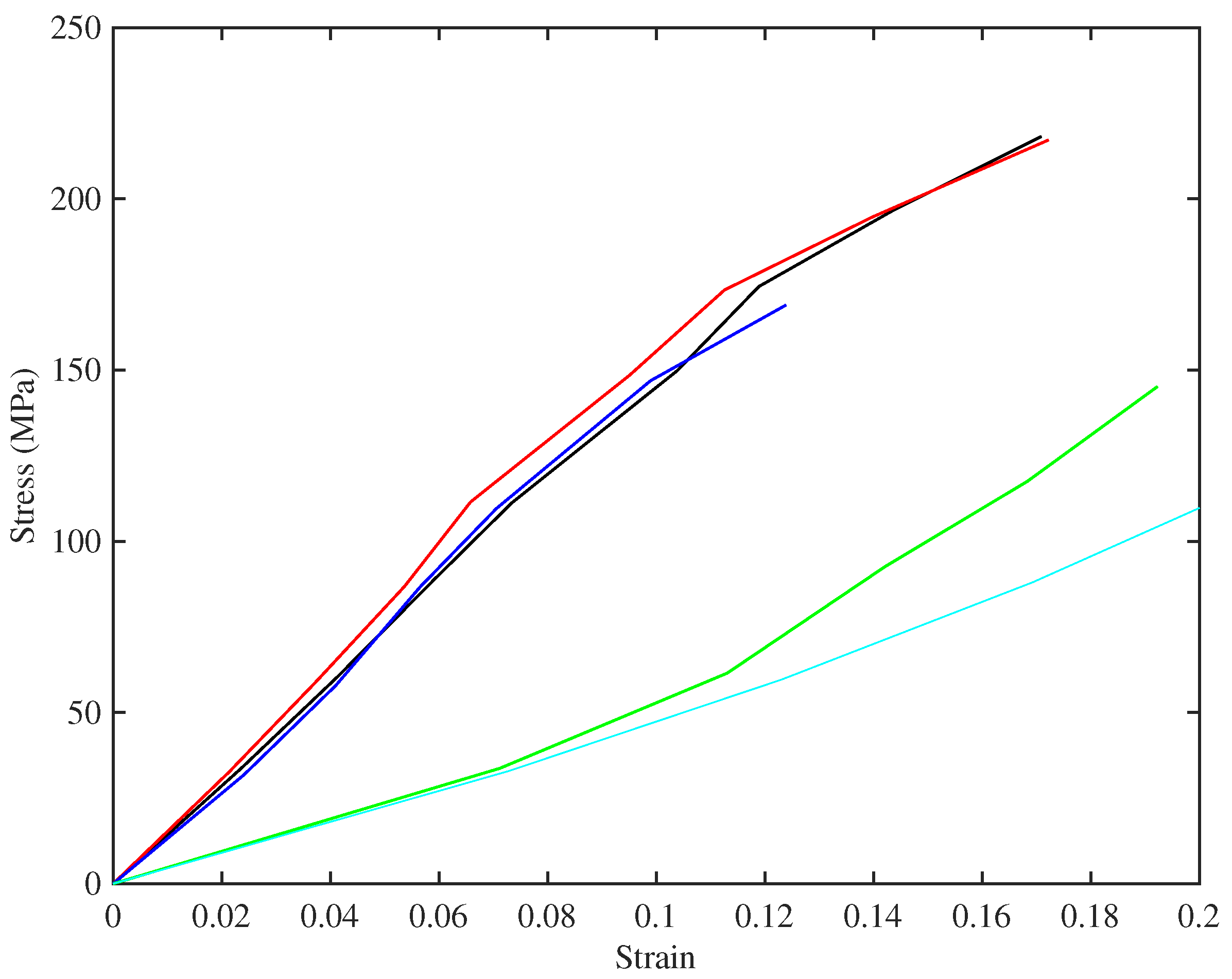
Figure 6 shows the long-term relation between stress and strain for the set of strings tested at multiple target frequencies. Each plotted point here represents the state of a string when it had settled at the chosen stress level (using the creep-monitoring approach described in
Section 3.4), at the base temperature of
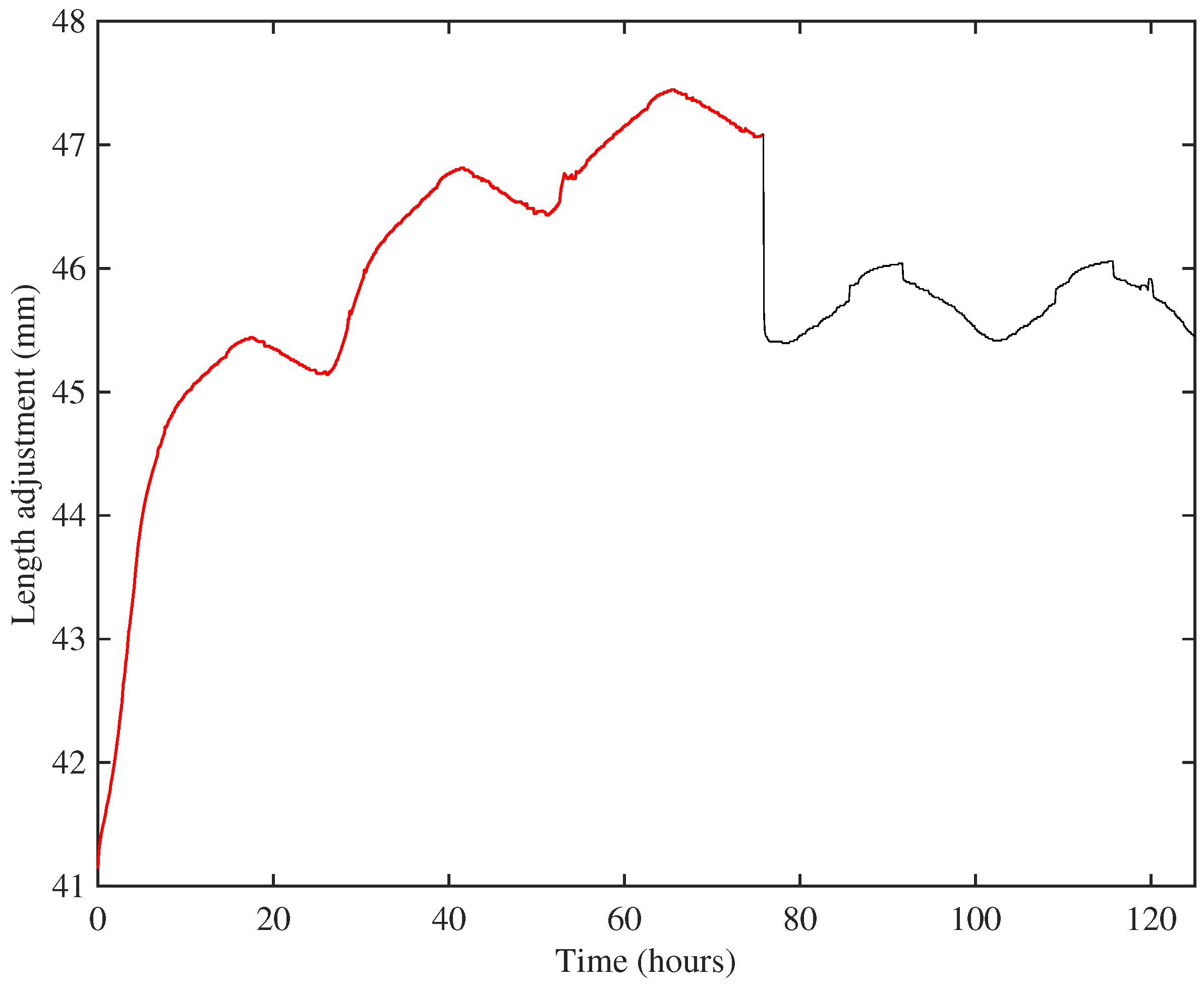
C; so the slopes of the curves give a direct measure of the “slow” Young’s modulus
. When a string was first mounted on the rig, it was hard to define accurately the zero point of length adjustment, because of the details of string take-up onto the winding shaft. As a result, the raw measurements of length adjustment
x had an unknown offset. However, it is obvious that the correct stress-strain relation should pass through the origin, so to produce this plot, the first three points with non-zero stress for each case have been used to define a quadratic, which was extrapolated back to zero to estimate the offset, which was then subtracted. The strain values used were then calculated from the corrected length adjustments using the exponential form of Equation (
7). The process seems to work reliably: the curves shown here all look reassuringly smooth and consistent at low stress levels, and within each group of strings the detailed agreement is excellent. An alternative approach would have been to deduce the strain from the measured linear density, assuming that the total mass remained constant, but that method would not have avoided the difficulties associated with initial take-up and straightening of the string.
It is immediately clear that the strings fall into two distinct groups, with very different behaviour. Referring to
Table 1, the same two groups can be identified from the bulk density values, although the disparity is less striking than in the stress-strain behaviour. The strings with lower density produce the upper cluster of lines in the plot: these strings appear generally to show a slight steepening of slope and, hence, increasing modulus, as strain increases up to about 0.07. At higher strain, the slope decreases, indicating reducing modulus. The higher-density strings have a low-strain modulus which is far lower, but on the whole they show increasing slope across the whole range of strain tested here. It seems likely that these groups have some fundamental difference in chemistry and perhaps manufacturing process. Interestingly, one of these strings (Number 29, E3) is the outlier in
Figure 4, whereas String 23a (A3), which looks similar here, showed behaviour in
Figure 4 that matched the lower-density strings.
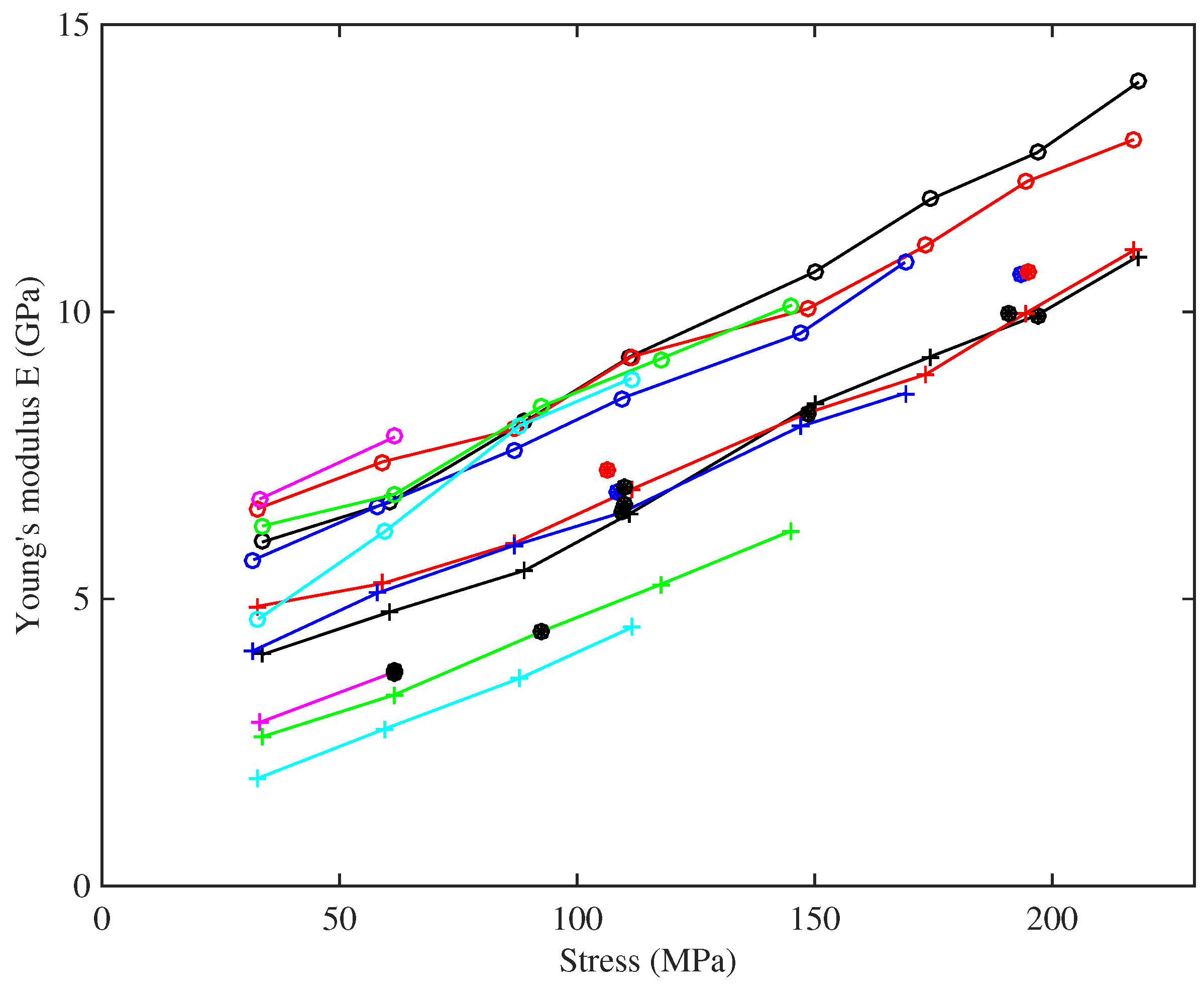
Figure 7 shows the base temperature Young’s modulus plotted against strain, for all three measurement methods. The slow modulus
has been calculated from the slopes of the lines in
Figure 6, while the measurements of
and
were described earlier. This plot reveals quite a complicated pattern of behaviour. For any given string (indicated by colour in the plot),
has the lowest value; the modulus
deduced from tension modulation comes next; and the modulus
deduced from bending stiffness is highest. This pattern is consistent with the intuitive expectation described in the Introduction: Young’s modulus increases as the time-scale of the measurement method reduces. It should be emphasised that all three moduli are relevant to the musical application. The long-term modulus
relates to the total length correction needed to settle a string to the desired pitch and, hence, determines the final tension shown in
Figure 5. The modulus
measured on a time-scale of minutes is the value needed to perform tuning adjustments, whether manual or automatic. The high-frequency modulus
influences the sound quality of the plucked string, because the inharmonicity of musical strings resulting from bending stiffness can be audible [
19]: it is one reason why different strings plucked in the same way can sound different.
It is clear from
Figure 7 that all three moduli also show strong variation with strain. The values of
and
both increase monotonically, over a considerable range:
for String 8 (A6), for example, ranges from around 4 GPa to over 10 GPa.
for the same string changes by a similar factor, with consistently higher values over the whole tested range.
for that string shows a different pattern, one already described qualitatively in the discussion of
Figure 6: the modulus increases with strain initially, but then declines somewhat for higher strain values. These values are significantly noisier than those of
and
, because they rely on estimating the slope of the stress-strain plot.
There is another very obvious feature of
Figure 7: a contrast in behaviour between the two groups of strings identified from the stress-strain plot. The strings with higher density show considerably lower values of Young’s modulus based on all three measurement methods. The pattern of
has already been discussed briefly; monotonically increasing with strain, unlike the results for the other group of strings. The results for
and
echo this trend. The ratio of
to
is bigger for these strings, as is directly visible in this log-scale plot. This may correlate with a more fundamental difference of material constitution, such as a different glass transition temperature. Many polymers show a relation between temperature response and frequency response known as “time-temperature superposition” (see for example, [
20]), so a different glass transition temperature might well translate into a different pattern of frequency dependence of elastic moduli.
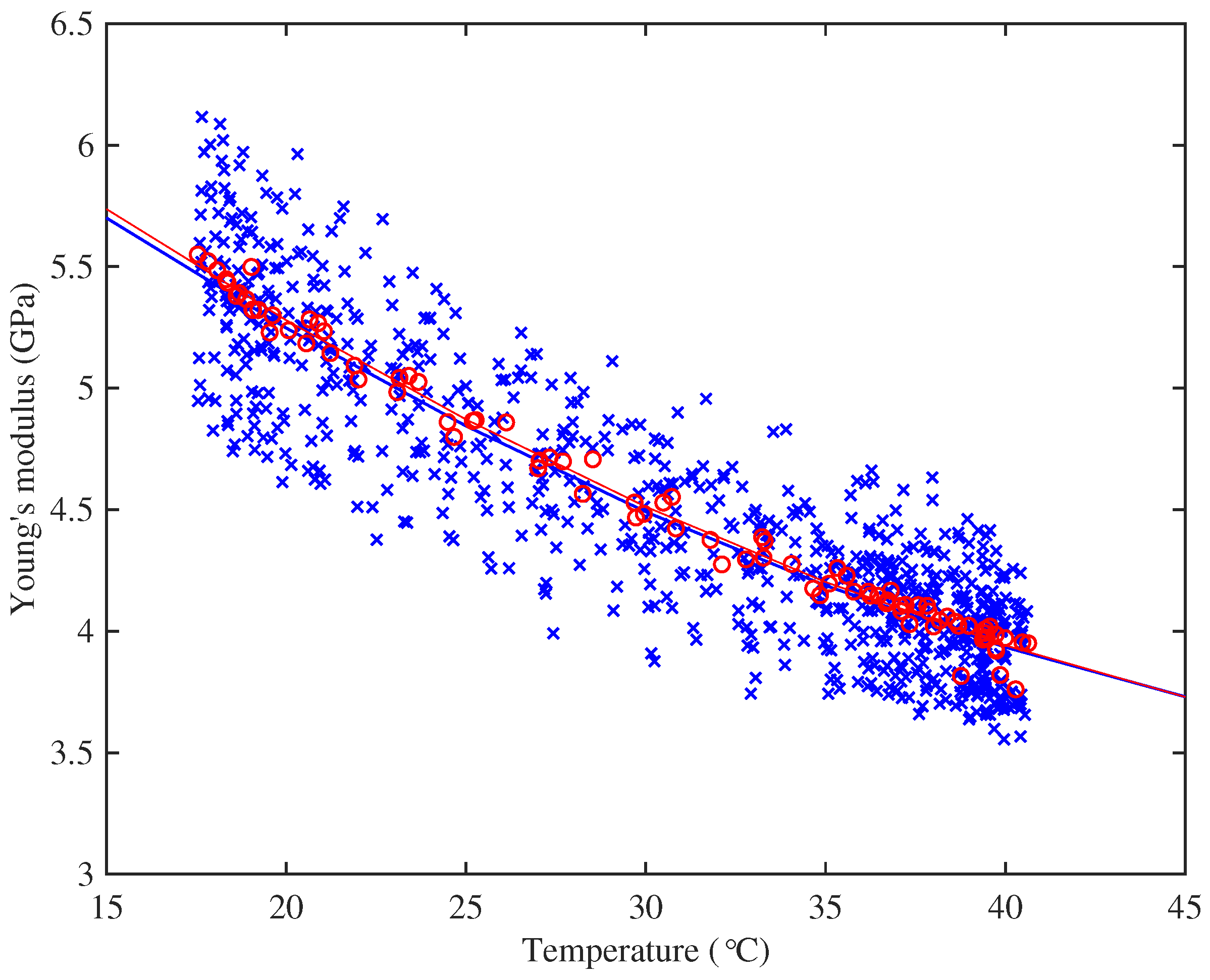
To explore this conjecture, specimens of strings representative of the two groups were subjected to DMA tests. The main results were very clear.
Figure 8 shows the variation of Young’s modulus with temperature for representative examples of strings from the two groups, measured at 1 Hz and 10 Hz: the string with higher density (String 29, E3) has its glass transition range at a significantly lower temperature than the string with lower density (String 5, A4). This figure also shows the expected qualitative effect of frequency: the 10-Hz results are consistently higher than the 1-Hz results for both strings. For the higher-density string, the glass transition straddles the nominal operating temperature of the tests reported here, so that the modulus will have varied significantly during the thermal cycles used. This may indicate the physical mechanism underlying the curvature reported earlier, in the discussion of
Section 3.5.
Figure 9 combines the DMA measurements for String 29 with the values from the other measurement methods already described, deduced by extrapolating the results in
Figure 7 to zero strain. Results are plotted on a logarithmic frequency scale. This plot illustrates the approach to correcting the calibration of the DMA tests: a best-fitted scale factor was found, to make the results consistent with the other tests. The plot seems very convincing: with this single scale factor, the results over the entire wide range of frequencies can be seen to fit into a single consistent trend. This suggests that the different measured values of Young’s modulus really do stem mainly from viscoelastic effects, rather than from some shortcoming in the experimental approach.
Returning to the main set of measurements, results from the previously mentioned study of string creep have indicated that the total creep, and hence, strain, depends not simply on the applied stress, but in a more complicated manner on the stretching history of the string and the maximum temperature to which it has been exposed. For the purposes of this study into the long-term behaviour of well-settled strings, it is far from clear a priori whether stress or strain would provide the more useful explanatory variable. In many cases, examining the responses as a function of the applied stress proved to be revealing, especially with regard to identifying the similarities and differences within and between the two groups of strings identified in the stress-strain plots of
Figure 6.
To illustrate this,
Figure 10 shows the results for
and
replotted (on a linear scale) against stress. All of the curves can be seen to increase fairly linearly with the stress, and with very similar gradients, for both measures of Young’s modulus and across all of the strings. This indicates a degree of strain hardening, more properly “strain stiffening”, presumably due to straightening of the nylon molecules. The fact that the modulus correlates more clearly with stress than with measured strain can be tentatively explained. Strain in a polymer like nylon results from two mechanisms: straightening of the molecular chains, which is mainly an elastic effect, and slip between adjacent chains as van der Waals bonds break and re-form, which is the mechanism of plastic creep. The results described here were obtained after the creep deformation had had time to equilibrate, and the total measured strain contains a large component of this creep. However, the stiffening effect is associated with the straightening mechanism, and perhaps, stress is a good surrogate measure of this elastic component of the strain. Note that the steady and fairly linear overall stress-strain relation seen in
Figure 6 suggests that the progressive reduction in the elastic stretching was being offset by an almost equivalent increase in stretching due to plastic creep.
Figure 10 shows that the observed range of variation in
also applies to the range of nominal operating points (black circles) for different string gauges: thicker strings operate at low stress, thinner strings at high stress (to the extent that the highest strings on a harp are very prone to spontaneous breakage). This variation would need to be taken into account when designing any automated electronic tuning system. Using the previously available values of around 3–5 GPa for the Young’s modulus of bulk nylon [
6] or nylon strings [
4,
5], when calculating the tuning adjustment scaling factor, would lead to unstable systems in many cases; with successive tuning adjustments overshooting the desired tuning until something broke.
For the results from tension modulation, it can be seen that the two tests run at lower humidity levels (red circles) gave slightly higher Young’s modulus values compared to strings of the same gauge, but the differences were not particularly dramatic given the very large difference in the humidity levels. The A4 Pirastro string, at a stress of about 110 MPa, had a Young’s modulus very similar to that of the Bowbrand A4 strings tested at the usual humidity levels, whereas the thinner A6 Pirastro string, at a stress level of nearly 200 MPa, behaved more like the Bowbrand A6 string tested at low humidity.
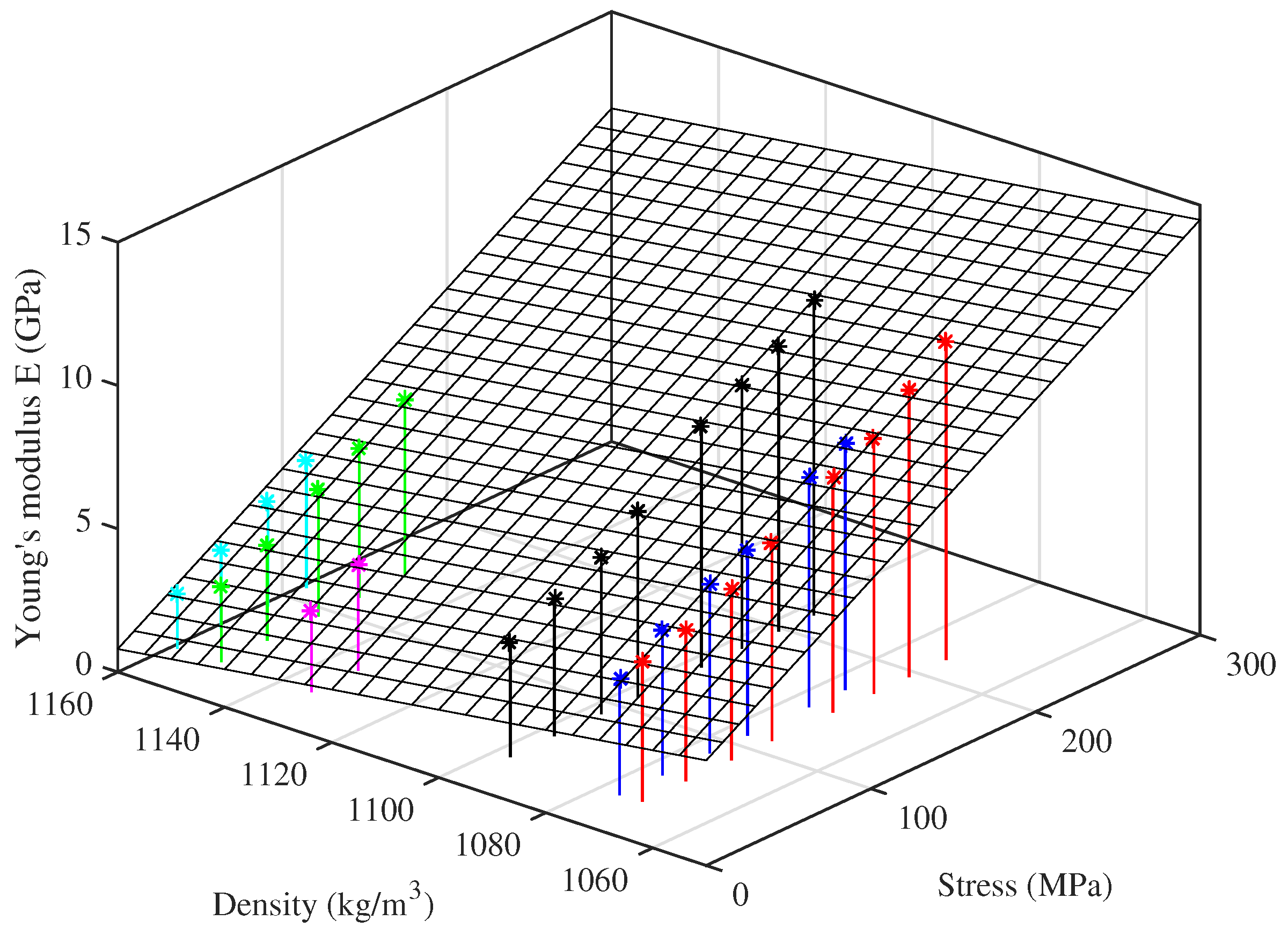
For the strings tested at multiple target frequencies, the Young’s modulus results derived from the string bending stiffness are quite tightly grouped together, while those obtained by tension modulation are more spaced out. This suggests that there is an additional factor influencing this measure of the Young’s modulus, not directly related to the string tension or stretching. Testing the strings at the same set of frequencies, and hence at approximately the same stress levels, allowed a pattern to be discerned in the Young’s modulus as a function of stress level and unstretched string density, shown in
Figure 11. This plot suggests that, for a given stress,
appears to fall fairly linearly as the string density increases. Presumably the density variations arise from chemical or microstructural differences, which also have an influence on the Young’s modulus.
Motivated by this observation, a curve-fit expression was derived using multiple regression analysis applied to the results from the ten Bowbrand strings tested at the usual humidity levels (around 55–65% RH at 20 °C). The tension-modulation Young’s modulus
at 20 °C can be estimated with reasonable accuracy from the notional stress
(expressed in MPa) and the unstretched bulk density
(expressed in kg/m
3) by the relation:
While the model is not perfect, it is certainly good enough to ensure a stable yet responsive string tuning control system for all of the tested strings. Indeed, an earlier version of this expression, based on fewer measurements and without the density term, was successfully used in the test rig control program for much of the testing.
Returning to
Figure 10, it can be seen that the values of
derived from bending stiffness exhibit a very similar linearly increasing trend with stress, while being consistently higher than those of
. Frequency dependence arising from viscoelasticity has been suggested as the main explanation of this (see
Figure 9 and the associated discussion), but it is not the only conceivable reason. Another possible contributory factor is that the stiffness of the strings might not be uniform across their cross-section, which would change the relationship between Young’s modulus and bending stiffness assumed in Equation (
18). Bending stiffness is preferentially influenced by the outer layers, whereas for axial stretching, the combined stiffness is a straightforward average over the whole cross-section.
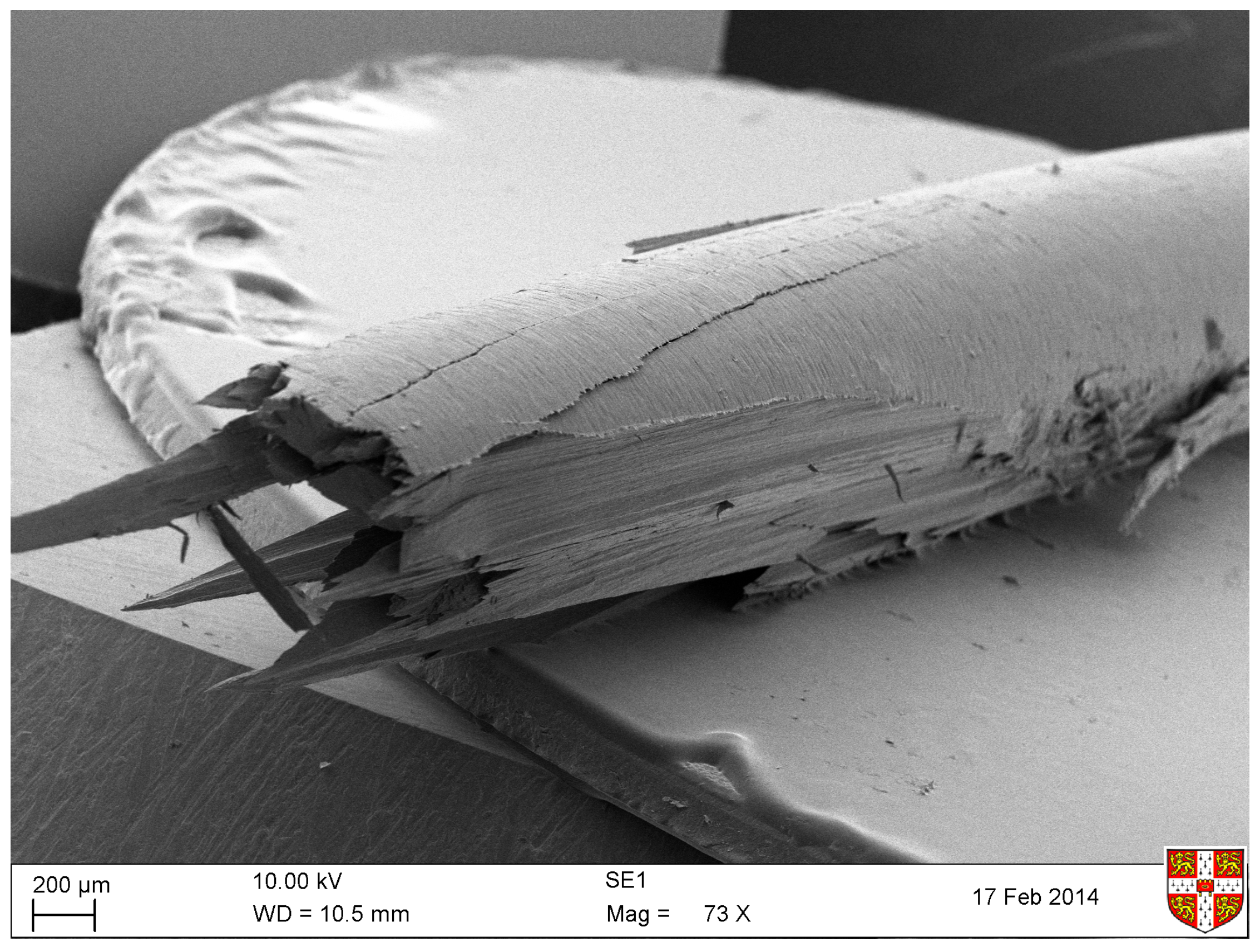
Figure 12 shows a scanning electron micrograph of the end of a section of the A4 (1.2 mm diameter) nylon harp string which has been dipped in liquid nitrogen and then snapped. The string appears to have a thin ‘skin’ wrapped transversely around the longitudinal ‘grain’, perhaps as a result of the grinding process used to reduce the string to the required diameter. Tests with thinner and thicker strings showed similar surface layers, and they all appeared to be just a few microns thick. This skin structure is interesting, but if anything, it would be expected to result in an opposite trend to the observation: if the outer layer of the string is less stiff in the axial direction than the core, the bending measurement would tend to give a lower modulus than the tension-modulation test.
4.3. Results for Thermal Tuning Sensitivity
Figure 13 shows a quantity of direct significance to the musician: the thermal tuning sensitivity, in
C, for the strings held at constant length with no tuning adjustments, plotted against the applied stress. The dashed lines show estimated curves, discussed below. In almost every case tested, the string went sharp as the temperature was increased, unless the temperature was taken high enough to cause the string to start creeping again. It is generally accepted that the minimum perceptible change in pitch of a musical note is around 3 ¢, though this varies with the pitch and complexity of the sound [
21]. Many of the strings tested varied at rates in excess of 1.5
C, so even a modest change in temperature could be expected to lead to noticeable changes in the string pitch. This phenomenon, familiar to harpists, was the original motivation for this study, in the hope of being able to design a tuning compensator to offset the problem. The two Pirastro strings behaved in a similar manner to the Bowbrand strings, and reducing the humidity level did not appear to affect the overall tuning sensitivity.
Figure 13 shows a striking difference between the lower- and higher-density groups of strings, with the higher-density strings showing significantly lower sensitivity to temperature changes. Within each group, however, the variation in thermal tuning sensitivity with stress was consistent. All of the results showed a very strong correlation between the observed thermal tuning sensitivity and the inverse of the applied stress. Referring to the right-hand side of Equation (
8), this rather suggests that the rate of change of stress with temperature, of an unadjusted nylon string, is more or less constant, both for a given string and a string of a given material or chemical make-up.
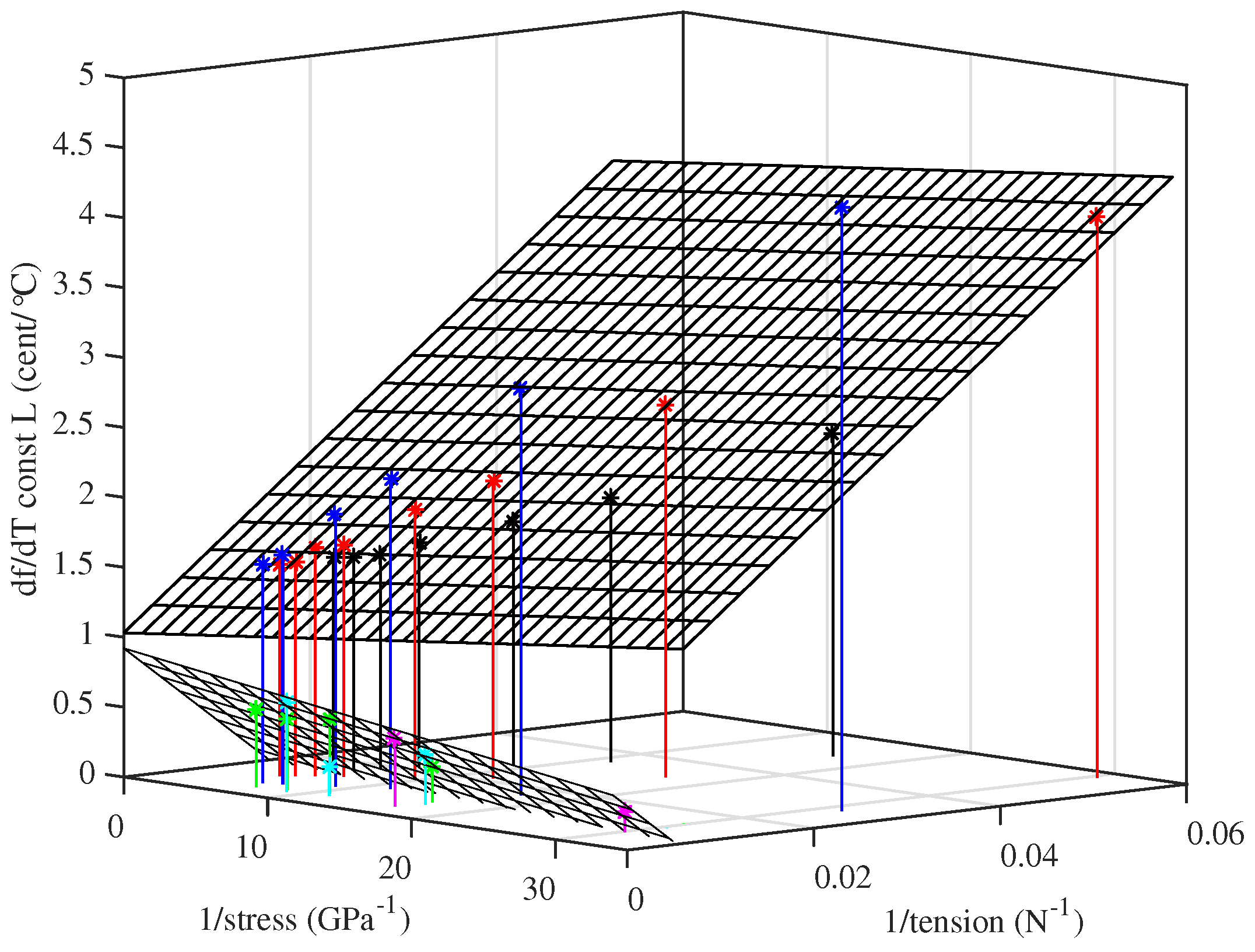
As for the Young’s modulus results, testing different strings at approximately the same set of stress levels enabled the behaviour in response to other variables to be explored. For any given stress, the tuning sensitivity was found to vary fairly linearly when plotted against either the inverse of the string tension, or the inverse of the cross-sectional area. However, while a single plane could be fitted to the Young’s modulus results across all of the strings tested (
Figure 11), separate planes with very different fitted coefficients were required for the thermal tuning sensitivity of the two groups of strings.
Figure 14 shows the results using the inverse of the tension as the second variable. It was found that the thermal tuning sensitivity results, for the strings held at constant length, could be described quite well (
= 0.99 and 0.82 for the lower- and higher-density groups, respectively) by an expression of the form:
where
and
c are constant coefficients. The dashed lines shown in
Figure 13 were obtained in this way: values of the fitted coefficients are given in the
Appendix A. The inverse-stress term is the most important. It may be noted that the notional stress
and tension
, calculated using the unstretched values for the string bulk and linear density, respectively, could be used in place of
and
without significantly affecting the goodness of fit.
Figure 15 shows the thermal tuning sensitivity when the strings were maintained at constant tension. The thermal tuning sensitivities of the strings were significantly reduced, with the sensitivities at the string operating points all smaller than 0.5
C and often less than 0.3
C; albeit with the thinner strings going slightly sharp as the temperature rose, while the thicker strings went slightly flat. The effects of thermal changes on the string tension were not entirely removed though. As the temperature rose and the string tightened up (see below), it had to be unwound from the winding shaft to compensate, adding extra mass and increasing the linear density. This usually worked against the thermal variation in the linear density, which was now the dominant effect.
A fairly linear relationship can be seen between the tuning sensitivity at the string operating points and the applied stress. The fitted line in
Figure 15 was matched to the data for the Bowbrand strings tested at the usual humidity levels (
). Noting that, except at the lowest stress levels, the tuning sensitivity of each string showed little variation with stress and recalling the string design scaling rules and the almost linear relationship between the tension and diameter at the operating points, it might be argued that a more fundamental explanatory parameter in this case is the inverse of the string diameter.
4.4. Thermal Variations in Tension and Linear Density
It is of some interest to dig a little deeper into the data for thermal sensitivity, to see the influence of different factors contributing to the tuning sensitivity.
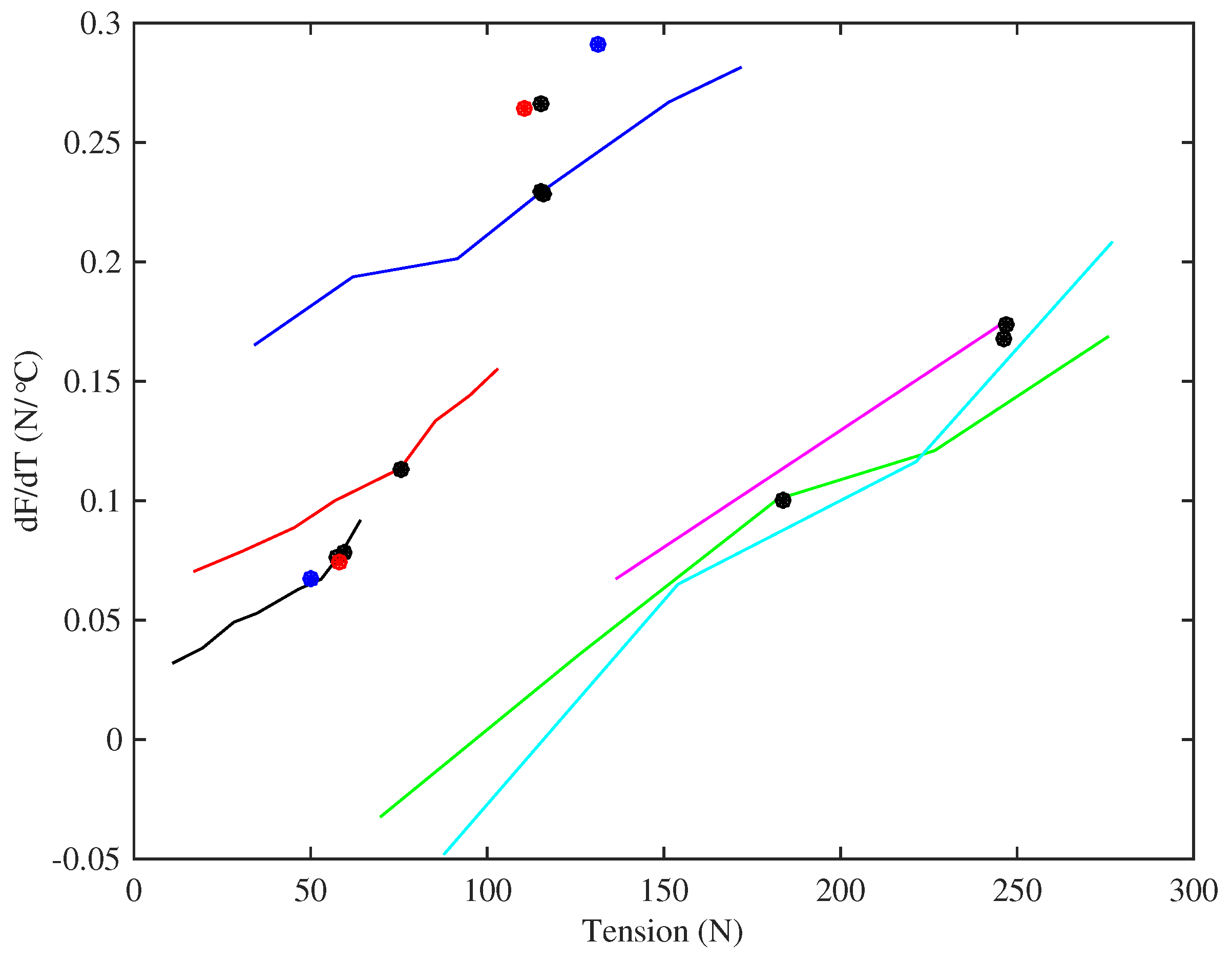
Figure 16 shows the gradients of the lines fitted to the tension versus temperature responses for the strings held at constant length with no tuning adjustments. In nearly every case tested the string tension increased as the temperature rose (provided the string was not heated far enough to cause it to start creeping again). There was a strong correlation between these thermal tension sensitivity values
and the string tension
at the base temperature, and
Figure 16 uses
(rather than stress) as the abscissa to illustrate this. The two Pirastro strings and the two Bowbrand strings tested at lower humidity levels show very similar behaviour to the rest of the Bowbrand strings. The responses for the strings tested at multiple target frequencies are fairly linear and nearly parallel, across both groups of strings, each having a gradient of about 0.001/°C.
Further analysis showed that the thermal tension sensitivity appeared to rise fairly linearly as the string cross-section increased, but with very different gradients for the two groups of strings (
Figure 17). Similar behaviour could be observed using the unstretched string diameter or linear density in place of the cross-section. A linear variation with the string cross-section would seem the more intuitively satisfying interpretation, however, as it would correspond directly to the observed correlation between the thermal tuning sensitivity and the inverse of the applied stress (see Equations (
10) and (
20)).
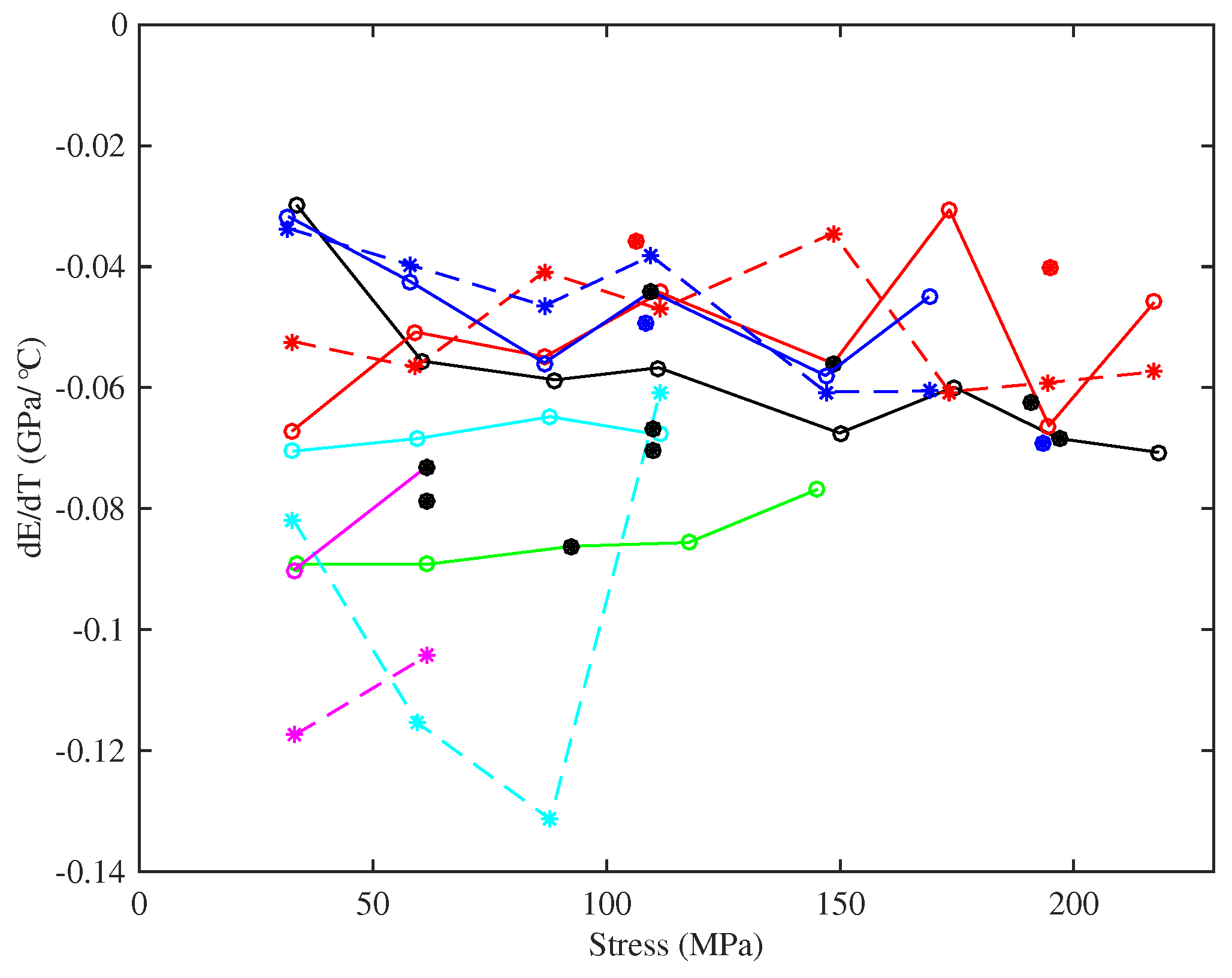
Figure 18 shows the gradients of lines fitted to Young’s modulus versus temperature responses. There is significant scatter in the responses, possibly due to the difficulty in extracting an accurate gradient measure in some cases, but the broad conclusion is that the rate of thermal variation in the Young’s modulus appeared to stay fairly constant as the applied stress increased; while, as seen earlier, the base temperature Young’s modulus increased steadily with stress. In all cases, the values were negative: the Young’s modulus falls as the temperature increases, which would make the string easier to stretch, hence tending to reduce the tension and make the string go flat. This negative gradient has already been seen in the DMA tests shown in
Figure 8 and is a familiar effect for all polymers [
20]. The thermal sensitivities of the strings tested at lower humidity levels were slightly lower than for other strings of the same gauges, but the differences were small compared to the range of variation seen in the strings tested at multiple target frequencies. The two Pirastro strings displayed rates of thermal variation in Young’s modulus that were broadly similar to the values obtained from the equivalent Bowbrand strings tested at the usual humidity levels.
Figure 18 includes the thermal variation gradients for the Young’s modulus values obtained from the string bending stiffness for the last four strings tested (these measurements were not taken for the earlier strings). The results for the thicker E3 and A2 strings show a greater degree of variation than those for the thinner A5 and A4 strings. This was due to poorer performance of the automated harmonic identification and curve fitting algorithm used to extract the bending stiffness values (
Section 3.6). For the thinner strings, at least, the thermal sensitivities of the two measures of Young’s modulus are notably similar given the consistent difference in the corresponding base-temperature Young’s modulus values. Recalling the earlier remarks that the quantities being measured were more strictly
and
rather than
per se, this suggests that any thermal variation in the string diameter was perhaps relatively small.
Figure 19 shows the CLTE values obtained for the various strings, plotted against the applied stress. The CLTE values were derived using Equation (
16), from the constant-length thermal tension sensitivity and the thermal variation in Young’s modulus. Again, there is significant scatter in the responses for the strings tested at multiple frequencies, but overall, the CLTE appeared to remain fairly constant as the stress was changed, at least for the lower-density set of strings.
In all cases, the CLTE was negative, indicating that the strings were trying to contract longitudinally as they were heated, which would tend to increase the tension and make the strings go sharp. This is a well-known effect, usually explained in terms of entropy [
22]. The nylon molecules can adopt more bent configurations than straightened configurations, so when heat is applied and energy added, the distribution of configurations changes with more molecules taking on bent configurations (or at least endeavouring to do so). As with the thermal variation in Young’s modulus, the CLTE values were slightly smaller for the strings tested at lower humidity levels, but again, the differences were not great; the Pirastro strings again showed values broadly similar to the equivalent Bowbrand strings.
It is worth noting that the CLTE values obtained for the strings were nearly two orders of magnitude larger than the expected range of values for the hardwood baseboard (
Section 3.1). Indeed, a steel base could probably have been used quite satisfactorily without requiring any compensation for its thermal expansion.
The two thermal effects, the reduction in the Young’s modulus and the thermal contraction of the string as the temperature rises, work against each other, with the balance between them determining the overall variation in the string tension. This balance between the two effects was, however, quite close, as is illustrated in
Figure 20: the two terms on the right side of Equation (
16) were almost equal (and opposite) and around an order of magnitude greater than the difference between them. Consequently, any measurement errors in the thermal variation of Young’s modulus were directly reflected into similar variations and errors in the CLTE values.
From Equation (
16) and the rather linear responses shown in
Figure 16, it might be expected that the
and
terms should both be constant for each string.
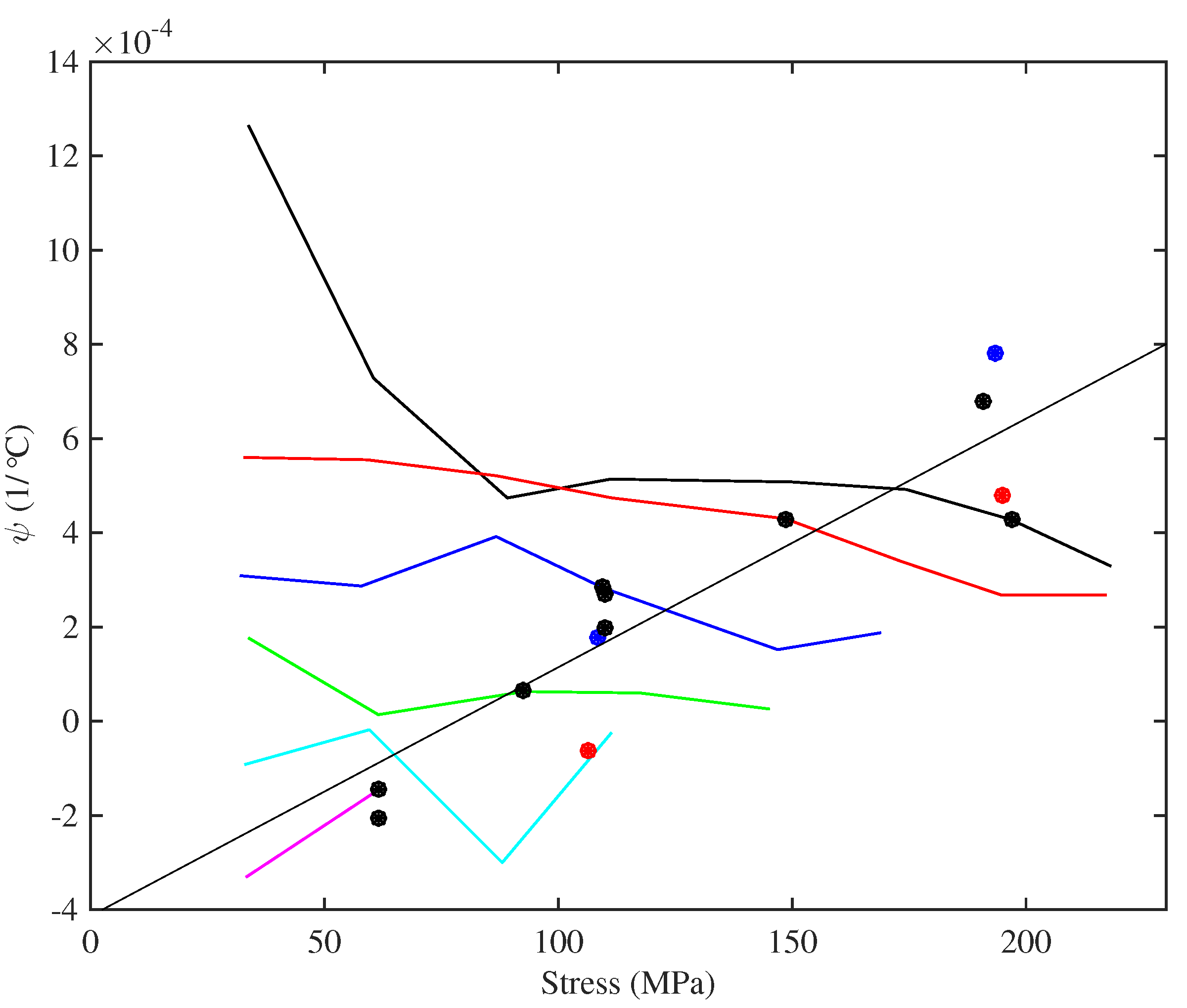
Figure 21, which shows
plotted against stress, demonstrates that this is not the case. The behaviour shown in
Figure 16 might therefore suggest some underlying connection between the thermal variation in Young’s modulus and the CLTE, common to both groups of strings.
The final measurement, shown in
Figure 22, is of the thermal variation coefficient
for the string linear density (see Equation (
11)). It is plotted against the applied stress. For the strings tested at multiple target frequencies, the thermal sensitivity of the string linear density appeared to be largely independent of the applied stress; at least at the higher stress levels. However, if only the string operating points are considered, a different pattern is seen: the results suggest a steady and almost linear increase in
with stress. The fitted line in
Figure 22 (
) was matched to the data for the Bowbrand strings tested at the usual humidity levels (around 55–65% RH at
C). As for the thermal tuning sensitivity of the strings held at constant tension, the best explanatory parameter might actually be the inverse of the string diameter. There are some striking similarities between
Figure 15 and
Figure 22, consistent with the thermal tuning sensitivity at constant tension being determined primarily by variations in the string linear density.
It is interesting to note that while most of the strings had positive values for , indicating that in those cases, the strings were losing mass (perhaps by drying out) as the temperature increased, the thickest strings had negative values, indicating they gained mass as the temperature rose. The two Pirastro strings appeared to follow the same trend as the Bowbrand strings, while the strings tested at lower humidity levels (around 20–26% RH at 20 °C) had lower values of than the comparable strings tested at the usual humidity levels. The latter result is, perhaps, unsurprising, since the thermal variation in both the absolute and relative humidity was much lower during the tests at the lower humidity levels.
In every case, the value of
was smaller, and often much smaller, than
, indicating that the tuning behaviour of the strings held at constant length (Equation (
10)) was dominated by the thermal variation in the string tension. In most cases, there was a reduction in the string linear density as the temperature rose, which contributed to the string sharpening, but this effect was small compared to the tension variation.