A Study on Maximum Wind Power Penetration Limit in Island Power System Considering High-Voltage Direct Current Interconnections
Abstract
:1. Introduction
2. Problem Formulation
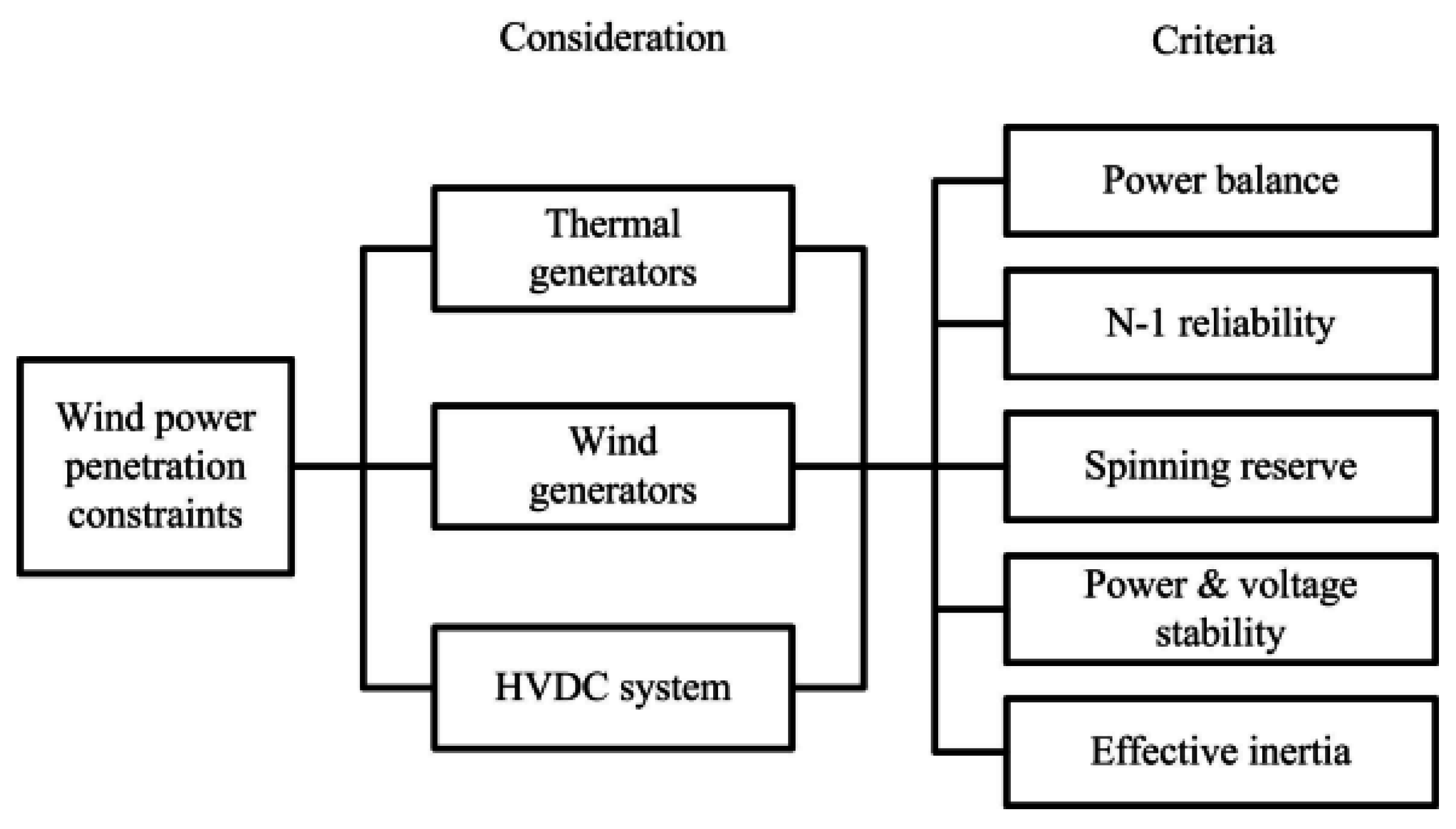
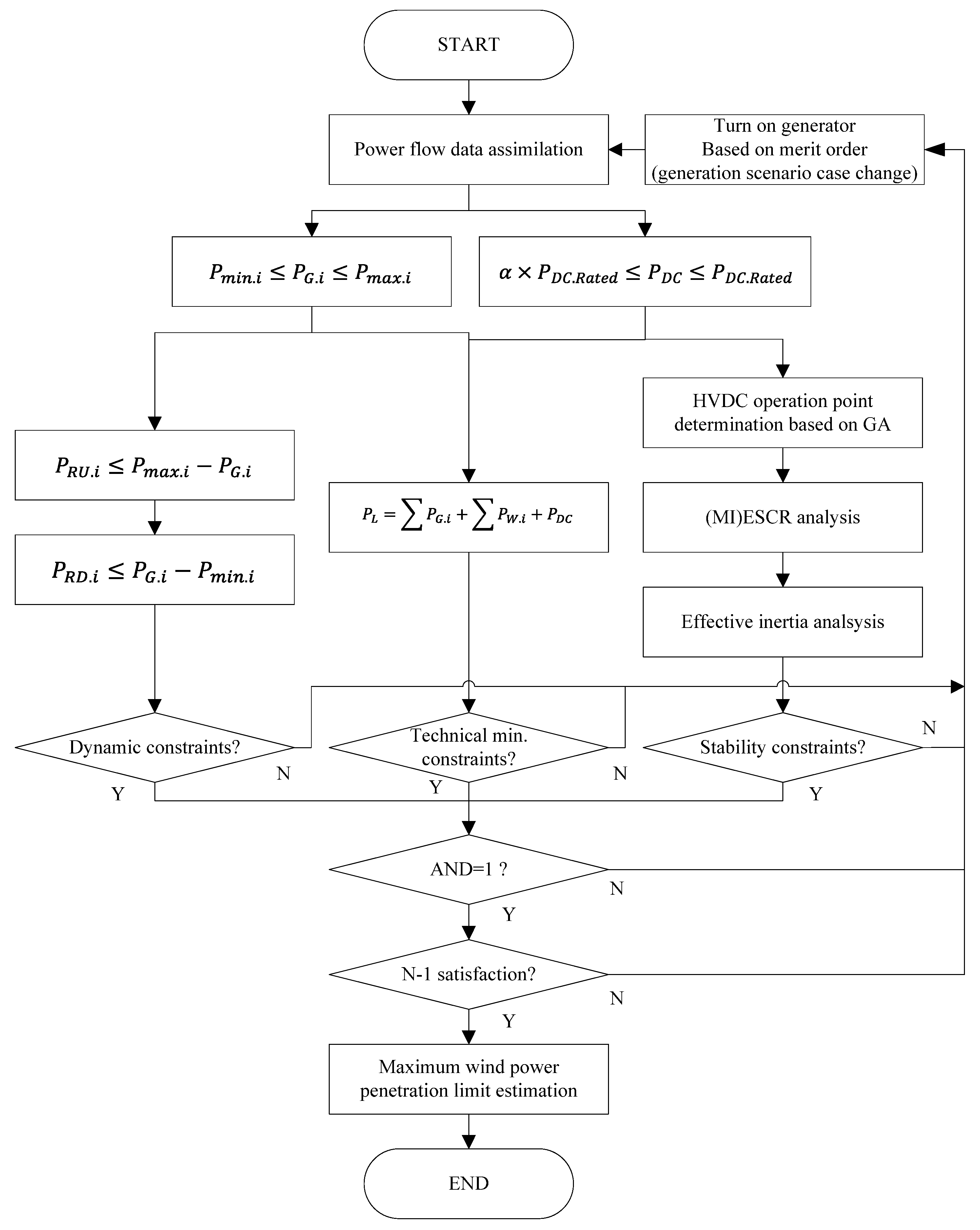
2.1. Thermal Generator Constraints
2.2. High-Voltage Direct Current Operation Range

2.3. Power Balance Constraints
2.3.1. Real Power Criteria
2.3.2. Reactive Power Criteria
Wind Power Side
High-Voltage Direct Current Side
2.4. N − 1 Reliability
2.5. Spinning Reserve
2.6. Power and Voltage Stability
2.7. Effective Inertia Constant Constraints
- -
- HVDC commutation failure
- -
- The faults in a HVDC system while sending and receiving to an end system
- -
- DC line fault
3. Case Study
3.1. Introduction of the Jeju Island Power System

| Generators | Pmax (MW) | Pmin (MW) | Inertia | Ramp Rate (MW/min) | Rating (MVA) |
|---|---|---|---|---|---|
| Jeju GT#1 (SC) | - | - | 2.56 | - | 50 |
| Jeju GT#2 (SC) | - | - | 2.56 | - | 50 |
| Jeju DP#1 | 40 | 26 | 6.71 | 2.0 | 44.96 |
| Jeju DP#2 | 40 | 26 | 6.71 | 2.0 | 44.96 |
| S-Jeju TP#3 | 100 | 50 | 5.93 | 5 | 130 |
| S-Jeju TP#4 | 100 | 50 | 5.93 | 5 | 130 |
| Jeju TP#2 | 75 | 42 | 5.4 | 1 | 97.06 |
| Jeju TP#3 | 75 | 42 | 5.4 | 1 | 97.06 |
| Hanlim CC | 105 | 41 | 6 | 8.7 | 150 |
| Specifications | HVDC Bipole 1 | Unit |
|---|---|---|
| Power rating | 150 × 2 (Bipoles) | MW |
| Voltage | 180 | kV |
| Current | 840 | A |
| Gamma firing angle (inverter) | 27 | Deg |
| Power reversal | Not available | - |
| % Impedance of transformer | 12 | % |
| Smoothing reactor | 60 | mH |
3.2. Power System Operation and Analysis Criteria

| Case | Jeju DP#1 | Jeju DP#2 | S-Jeju TP#3 | S-Jeju TP#4 | Jeju TP#2 | Jeju TP#3 | Pmax (MW) | Pmin (MW) | Ramp Rate (MW/min) |
|---|---|---|---|---|---|---|---|---|---|
| #1 | ON | - | - | - | - | - | 40 | 26 | 2 |
| #2 | ON | ON | - | - | - | - | 80 | 52 | 4 |
| #3 | ON | - | ON | - | - | 140 | 76 | 7 | |
| #4 | ON | - | - | - | ON | - | 115 | 68 | 3 |
| #5 | ON | ON | ON | - | - | 180 | 102 | 9 | |
| #6 | ON | ON | - | - | ON | - | 155 | 94 | 5 |
| #7 | ON | ON | ON | ON | - | - | 280 | 152 | 14 |
| #8 | ON | ON | ON | ON | - | 255 | 144 | 10 | |
| #9 | ON | ON | ON | ON | ON | - | 355 | 194 | 15 |
| #10 | ON | ON | ON | ON | ON | ON | 430 | 236 | 16 |
| Case | Spinning Reserve (MW) | SCC (MVA) | ESCR | Inertia | Hdc | |||
|---|---|---|---|---|---|---|---|---|
| w/o HVDC | With Unidirectional LCC | With Bidirectional LCC | With VSC | |||||
| #1 | 20 | 280 | 470 | 470 | 436.07 | 0.95 | 813.68 | 2.71 |
| #2 | 40 | 300 | 490 | 490 | 581.64 | 1.44 | 1115.36 | 3.72 |
| #3 | 70 | 330 | 520 | 520 | 918.91 | 2.56 | 1584.58 | 5.28 |
| #4 | 30 | 290 | 480 | 480 | 838.13 | 2.29 | 1337.81 | 4.46 |
| #5 | 90 | 350 | 540 | 540 | 1018.87 | 2.90 | 1886.26 | 6.29 |
| #6 | 50 | 310 | 500 | 500 | 939.12 | 2.63 | 1639.49 | 5.46 |
| #7 | 140 | 400 | 590 | 590 | 1335.39 | 3.95 | 2657.16 | 8.86 |
| #8 | 100 | 360 | 550 | 550 | 1376.38 | 4.09 | 2410.39 | 8.03 |
| #9 | 150 | 410 | 600 | 600 | 1692.71 | 5.14 | 3181.29 | 10.6 |
| #10 | 160 | 420 | 610 | 610 | 2055.5 | 6.35 | 3705.41 | 12.35 |
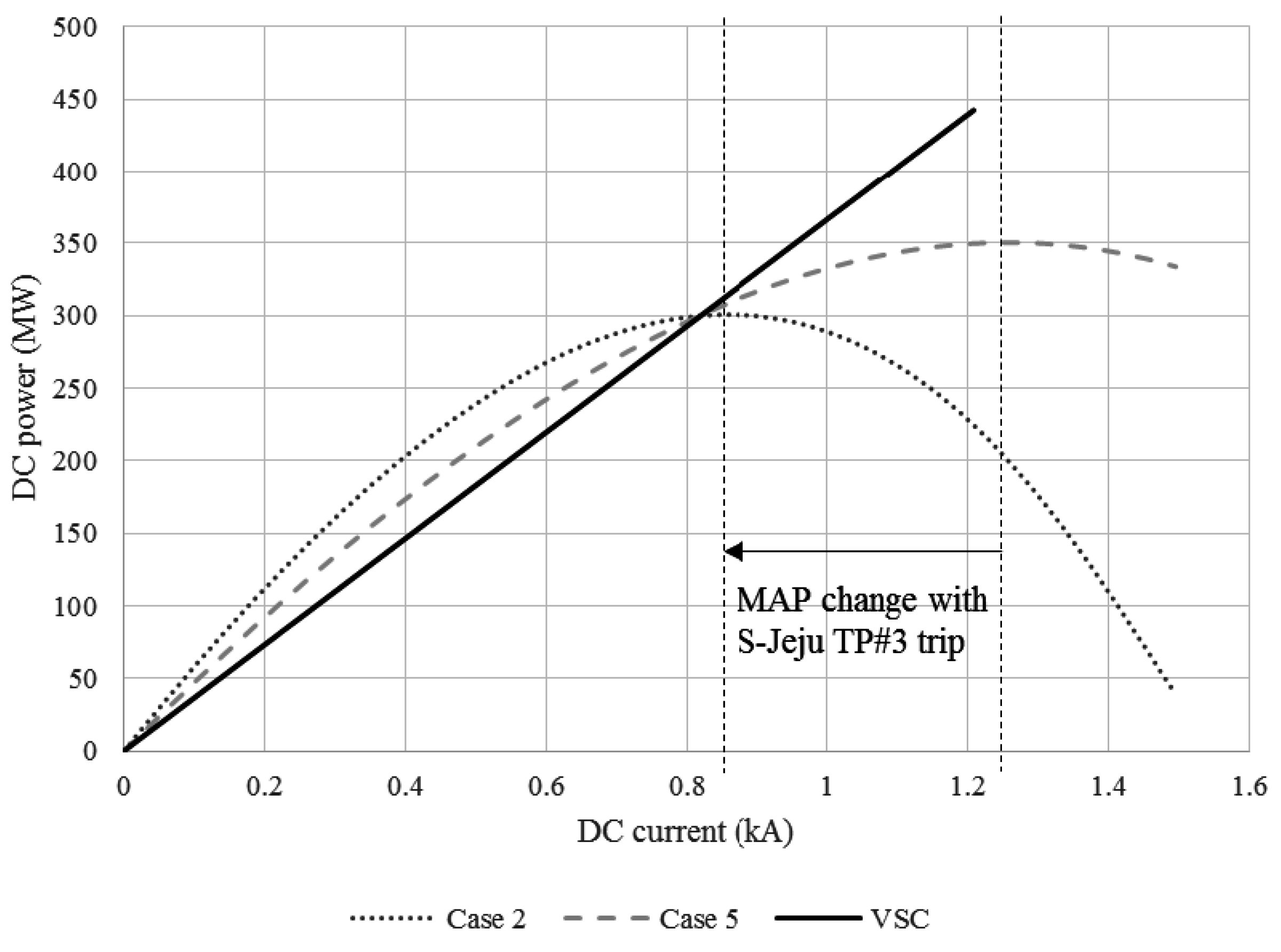
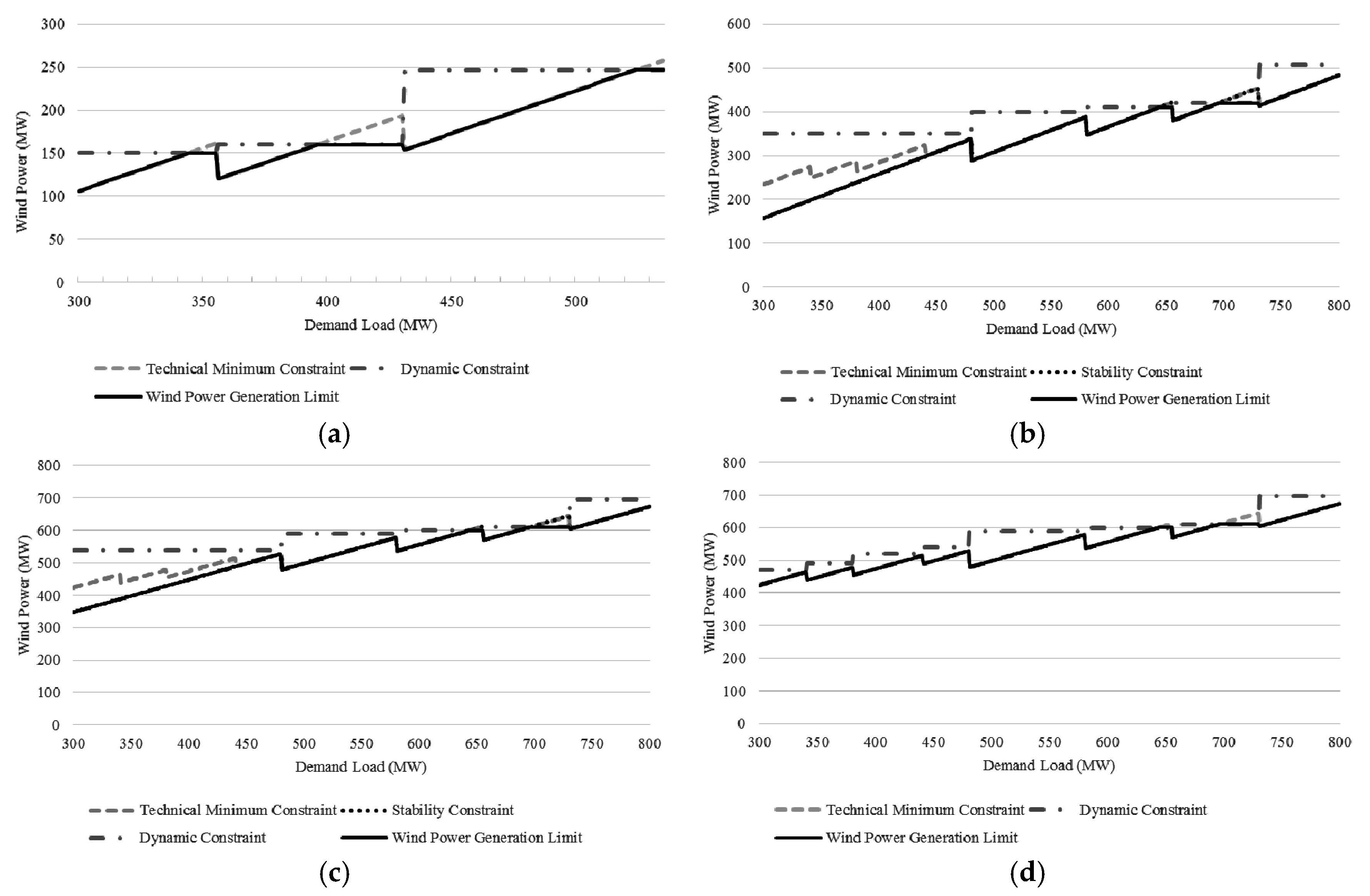
3.3. Maximum Wind Power Penetration Limit According to the Load Level
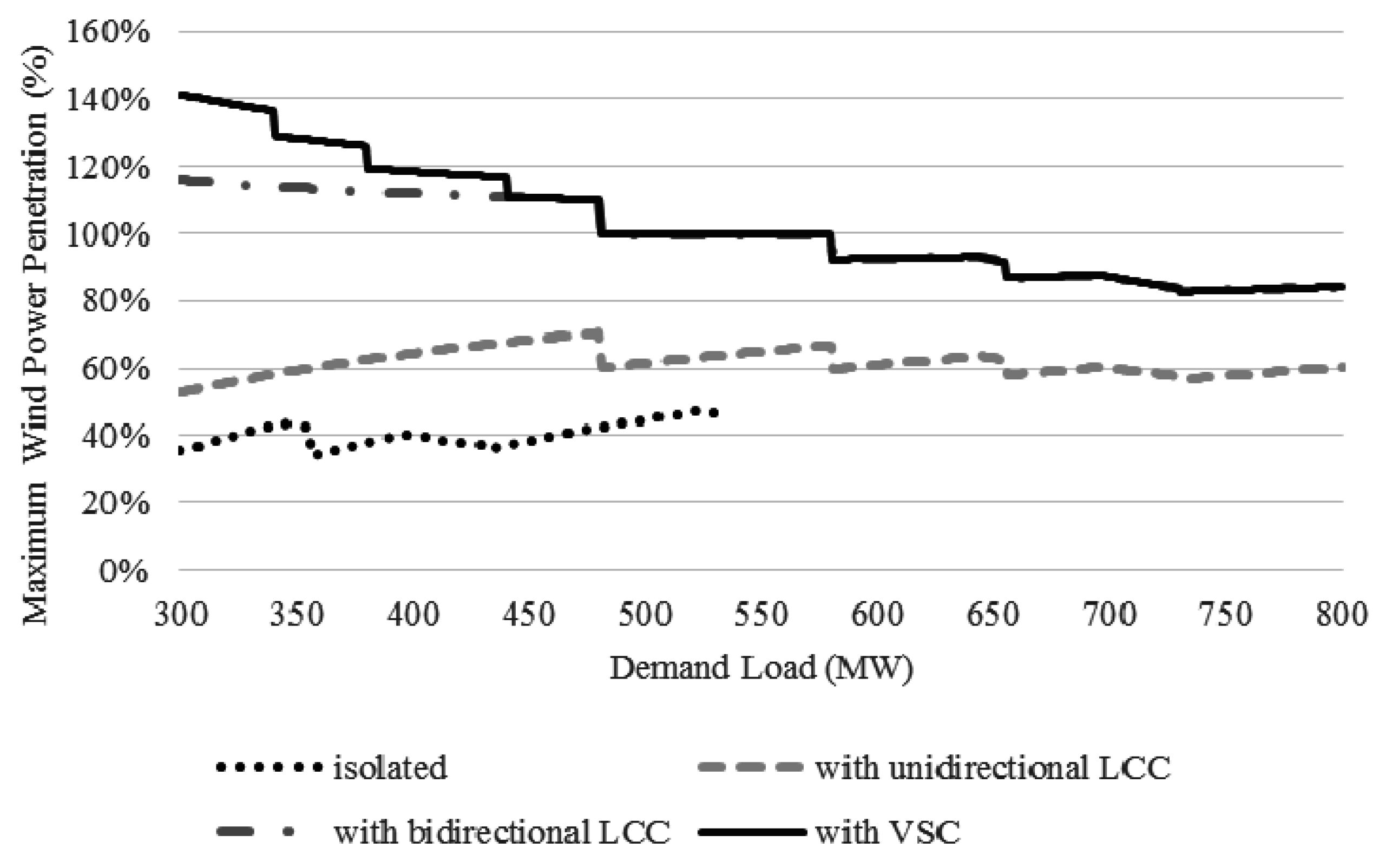
4. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| Total power generation of i-th generator | |
| Power generation of i-th generator | |
| Minimum active power generation limit of i-th generator | |
| Maximum active power generation limit of i-th generator | |
| Ramp-up rate of i-th generator | |
| Ramp-down rate of i-th generator | |
| Ramp up/down time | |
| Generation ramp-up capacity of i-th generator | |
| Generation ramp-down capacity of i-th generator | |
| HVDC power transfer | |
| Rated capacity of HVDC | |
| τ | Minimum HVDC operation point coefficient (p.u.) |
| Up spinning reserve capacity | |
| Down spinning reserve capacity | |
| Wind power fluctuation coefficient | |
| AC terminal voltage | |
| DC voltage | |
| Demand load in the system | |
| Total wind power generation in the system | |
| Wind power generation of i-th wind farm | |
| Reactive power consumption of HVDC system | |
| Reactive power compensation by shunt element | |
| No-load DC voltage | |
| DC current | |
| α | Firing(delay) angle |
| μ | Overlap angle |
| γ | Extinction angle |
| Short circuit capacity at converter bus | |
| Rotational inertia constant of i-th machine | |
| MVA rating of i-th machine | |
| Frequency deviation (p.u) | |
| Power mismatch (p.u) | |
| System nominal frequency | |
| Power loss period | |
| Fault duration time | |
| Power recovery time | |
| CC | Combined cycle gas turbine power-plant |
| GT | Gas turbine power-plant |
| DP | Diesel engine power-plant |
| TP | Thermal power-plant |
References
- Kennedy, S. Wind power planning: Assessing long-term costs and benefits. Energy Policy 2005, 33, 1661–1675. [Google Scholar] [CrossRef]
- Snyder, B.; Kaiser, M.J. Ecological and economic cost-benefit analysis of offshore wind energy. Renew. Energy 2008, 34, 1567–1578. [Google Scholar] [CrossRef]
- Wind Energy—The Facts: Costs & Prices; The European Wind Energy Association (EWEA): Brussels, Belgium, 2009; Volume 2, pp. 94–110.
- The European Wind Energy Association (EWEA). Wind in Power: 2013; European Statistics: Brussels, Belgium, 2014. [Google Scholar]
- 2012 Wind Technologies Market Report; U.S. Department of Energy: Washington, DC, USA, 2013.
- ERCOT Grid Operations Wind Integration Report; ERCOT: Austin TX, USA, 2014.
- Clausen, N.; Bindner, H.; Frandsen, S.; Hansen, J.C.; Hansen, L.H.; Lundsager, P. Isolated Systems with Wind Power an Implementation Guideline; Risø National Laboratory Technical Report Riso-R-1257 (EN); Risø National Laboratory: Roskilde, Denmark, 2001. [Google Scholar]
- Weisser, D.; Garcia, R.S. Instantaneous wind energy penetration in isolated electricity grids: Concepts and review. Renew. Energy 2005, 30, 1299–1308. [Google Scholar] [CrossRef]
- Milligan, M.; Kirby, B. Impact of Electric Industry Structure on High Wind Penetration Potential; National Renewable Energy Laboratory: Golden, CO, USA, 2009. [Google Scholar]
- Peter, B.E.; Thomas, A.; Hans, A.; Paul, S.; Wilhelm, W.; JuanMa, R.G. System operation with high wind penetration. IEEE Power Energy Mag. 2005, 3, 65–74. [Google Scholar]
- Integration of Large Scale Wind Generation Using HVDC and Power Electronics; Conseil International des Grands Réseaux Électriques Working Group B4.39: Paris, France, 2009.
- Papathanassiou, S.A.; Boulaxis, N.G. Power limitations and energy yield evaluation for wind farms operating in island systems. Renew. Energy 2006, 31, 457–479. [Google Scholar] [CrossRef]
- Luo, C.; Far, H.G.; Banakar, H.; Keung, P.; Ooi, B. Estimation of wind penetration as limited by frequency deviation. IEEE Trans. Energy Convers. 2007, 22, 783–791. [Google Scholar] [CrossRef]
- Chen, C.-L.; Lee, T.-Y.; Jan, R.-M. Optimal wind-thermal coordination dispatch in isolated power systems with large integration of wind capacity. Energy Convers. Manag. 2006, 47, 3456–3472. [Google Scholar] [CrossRef]
- Francisco, D.; Andreas, S.; Oriol, G.; Roberto, V. A review of energy storage technologies for wind power applications. Renew. Sustain. Energy Rev. 2012, 16, 2154–2171. [Google Scholar]
- Shan, J.; Botterud, A.; Ryan, S.M. Impact of Demand Response on Thermal Generation Investment with High Wind Penetration. IEEE Trans. Smart Grid 2013, 4, 2374–2383. [Google Scholar]
- Margaris, I.D.; Papathanassiou, S.A.; Hatziargyriou, N.D.; Hansen, A.-D.; Sorensen, P. Frequency Control in Autonomous Power Systems with High Wind Power Penetration. IEEE Trans. Sustain. Energy 2012, 3, 189–199. [Google Scholar] [CrossRef]
- Nasrolahpour, E.; Ghasemi, H. A stochastic security constrained unit commitment model for reconfigurable networks with high wind power penetration. Electr. Power Syst. Res. 2015, 121, 341–350. [Google Scholar] [CrossRef]
- Lyu, Y.; Liang, J.; Yan, J.; Yu, Z.; Sun, S.; Lu, G. On-line probabilistic dynamic security assessment considering large scale wind power penetration. In Proceedings of the International Conference on Power System Technology (POWERCON), Chengdu, China, 20–22 October 2014; pp. 2635–2641.
- Liu, W.; Liu, L.; Xu, G.; Liang, F.; Yang, Y.; Zhang, W.; Wu, Y. A Novel Hybrid-Fuel Storage System of Compressed Air Energy for China. Energies 2014, 7, 4988–5010. [Google Scholar] [CrossRef]
- Barberis Negra, N.; Todorovic, J.; Ackermann, T. Loss evaluation of HVAC and HVDC transmission solutions for large offshore wind farms. Electr. Power Syst. Res. 2006, 76, 916–927. [Google Scholar] [CrossRef]
- Bresesti, P.; Kling, W.L.; Hendriks, R.L.; Vailati, R. HVDC Connection of Offshore Wind Farms to the Transmission System. IEEE Trans. Energy Convers. 2007, 22, 37–43. [Google Scholar] [CrossRef]
- Wang, L.; Thi, M. Comparative Stability Analysis of Offshore Wind and Marine-Current Farms Feeding Into a Power Grid Using HVDC Links and HVAC Line. IEEE Trans. Power Deliv. 2013, 28, 2162–2171. [Google Scholar] [CrossRef]
- Bahrman, M. Offshore Wind Connections: HVDC for Offshore Grids; UWIG Technical Workshop: Maui, HI, USA, 2011. [Google Scholar]
- Chou, C.; Wu, Y.; Han, G.; Lee, C. Comparative Evaluation of the HVDC and HVAC Links Integrated in a Large Offshore Wind Farm—An Actual Case Study in Taiwan. IEEE Trans. Ind. Appl. 2012, 48, 1639–1648. [Google Scholar] [CrossRef]
- Kim, C.; Sood, V.K.; Jang, G. HVDC Transmission; Wiley: Hoboken, NJ, USA, 2009. [Google Scholar]
- Banakar, H.; Luo, C.; Ooi, B.T. Impacts of Wind Power Minute-to-Minute Variations on Power System Operation. Electr. Power Syst. Res. 2008, 23, 150–160. [Google Scholar] [CrossRef]
- Zhang, L.; Harnefors, L.; Nee, H.P. Modeling and control of VSC-HVDC links connected to island systems. IEEE Trans. Power Syst. 2011, 26, 783–793. [Google Scholar] [CrossRef]
- Kim, E.; Kim, J.; Kim, S.; Choi, J.; Lee, K.; Kim, H. Impact Analysis of Wind Farms in the Jeju Island Power System. IEEE Syst. J. 2012, 6, 134–139. [Google Scholar] [CrossRef]
- Park, J.; Park, Y.; Moon, S. Instantaneous Wind Power Penetration in Jeju Island. In Proceedings of the IEEE Power Engineering Society General Meeting, Pittsburgh, PA, USA, 20–24 July 2008.
- A Long-Term View of Power System Operation; Korea Power Exchange: Seoul, Korea, 2013; pp. 251–268.
- Ackermann, T. Wind Power in Power Systems; John Wiley & Sons, Ltd.: Chichester, UK, 2005. [Google Scholar]
- Kundur, P. Power System Stability and Control; McGraw-Hill Education: Columbus, OH, USA, 1994. [Google Scholar]
- Western Electricity Coordinating Council. Operating Reserves; WECC Standard BAL-STD-002-0; Western Electricity Coordinating Council: Salt Lake City, UT, USA, 2007. [Google Scholar]
- On Voltage and Power Stability in AC/DC Systems; Conseil International des Grands Réseaux Électriques Working Group 14.05: Paris, France, 2003.
- Franken, B.; Andersson, G. Analysis of HVDC converters connected to weak AC systems. IEEE Trans. Power Syst. 1990, 5, 235–242. [Google Scholar] [CrossRef]
- Denis, L.H.A.; Andersson, G. Voltage stability analysis of multi-infeed HVDC systems. IEEE Trans. Power Deliv. 1997, 12, 1309–1318. [Google Scholar]
- IEEE Guide for Planning DC Links Terminating at AC Locations Having Low Short-Circuit Capacities; IEEE Standard 1204-1997; IEEE: Piscataway, NJ, USA, 1997.
© 2015 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yoon, M.; Yoon, Y.-T.; Jang, G. A Study on Maximum Wind Power Penetration Limit in Island Power System Considering High-Voltage Direct Current Interconnections. Energies 2015, 8, 14244-14259. https://doi.org/10.3390/en81212425
Yoon M, Yoon Y-T, Jang G. A Study on Maximum Wind Power Penetration Limit in Island Power System Considering High-Voltage Direct Current Interconnections. Energies. 2015; 8(12):14244-14259. https://doi.org/10.3390/en81212425
Chicago/Turabian StyleYoon, Minhan, Yong-Tae Yoon, and Gilsoo Jang. 2015. "A Study on Maximum Wind Power Penetration Limit in Island Power System Considering High-Voltage Direct Current Interconnections" Energies 8, no. 12: 14244-14259. https://doi.org/10.3390/en81212425
APA StyleYoon, M., Yoon, Y.-T., & Jang, G. (2015). A Study on Maximum Wind Power Penetration Limit in Island Power System Considering High-Voltage Direct Current Interconnections. Energies, 8(12), 14244-14259. https://doi.org/10.3390/en81212425








