Influence of Layering and Fracture Angles on the Performance of Salt–Gypsum Composites: Implications for the Safety of Underground Energy Storage
Abstract
1. Introduction
2. Establishment and Simulation of Discrete Element Model
2.1. Numerical Modeling
2.2. Numerical Simulation Process
3. Macroscopic Mechanical Characteristics of Salt Paste Composite
3.1. Stress–Strain Curve
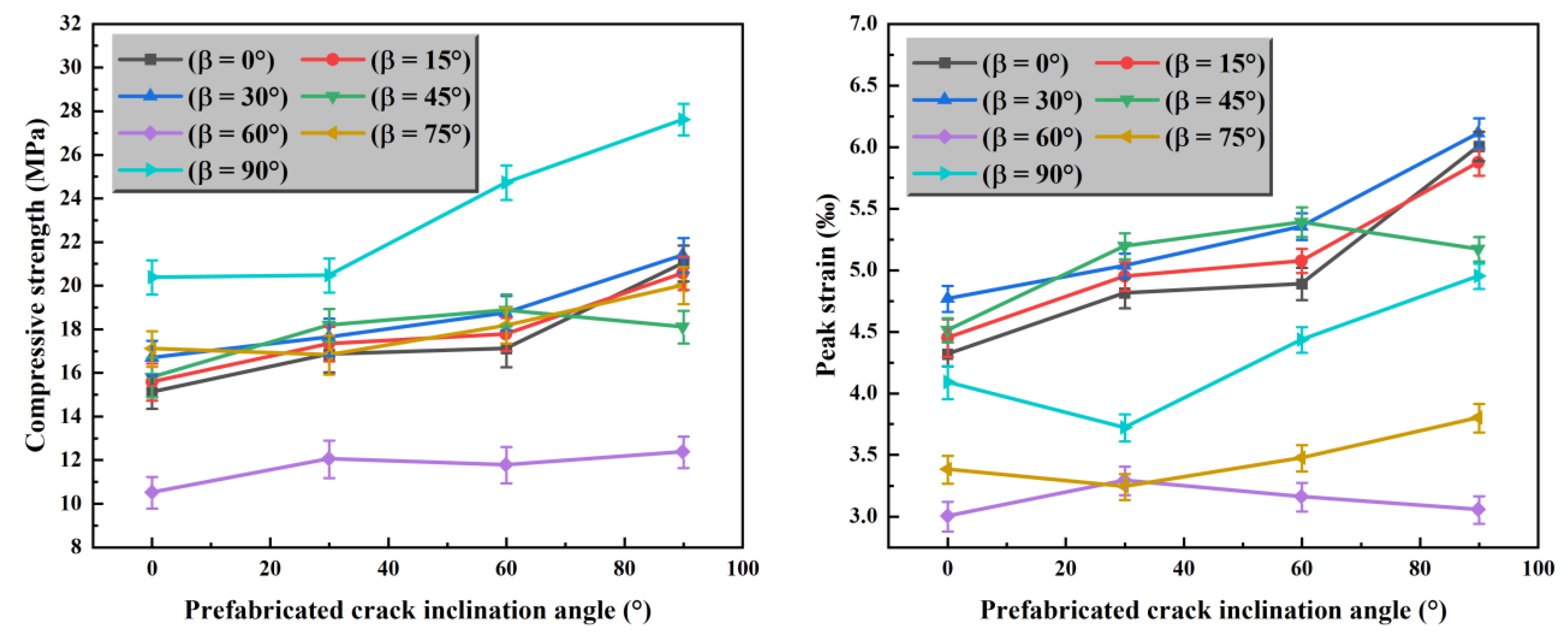
3.2. Compressive Strength
4. Damage and Fracture Characteristics of Salt Rock–Gypsum Composite
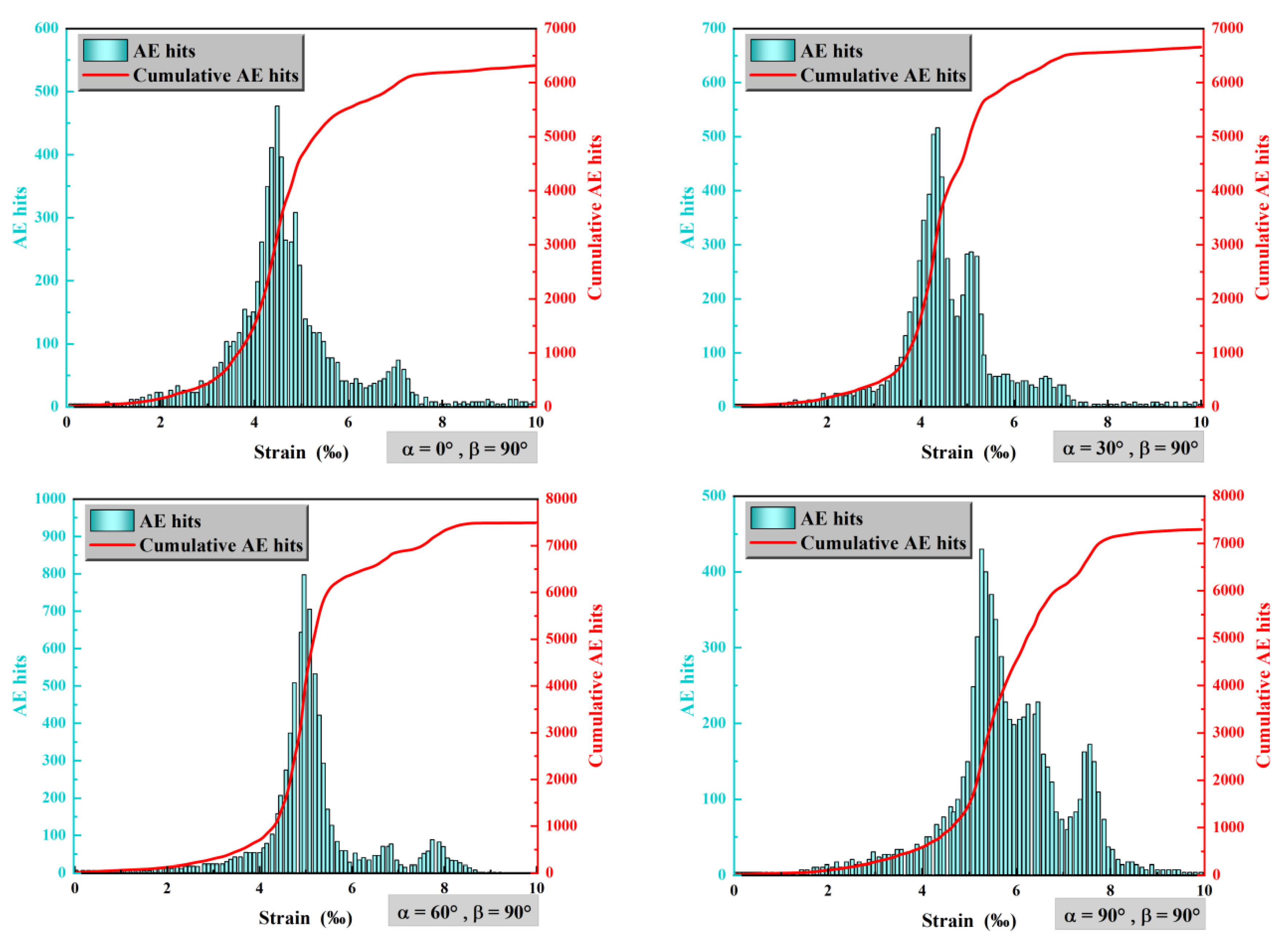
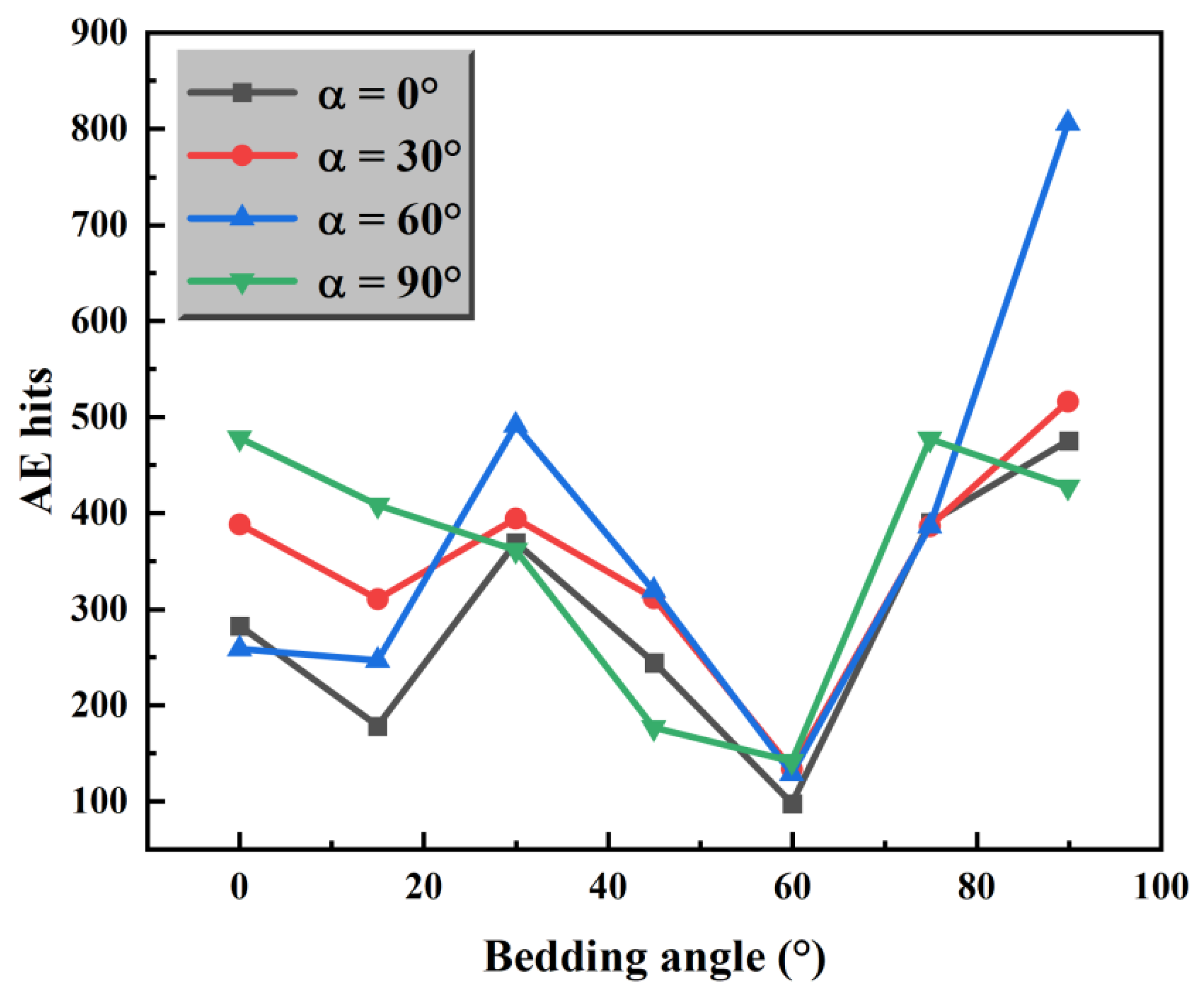
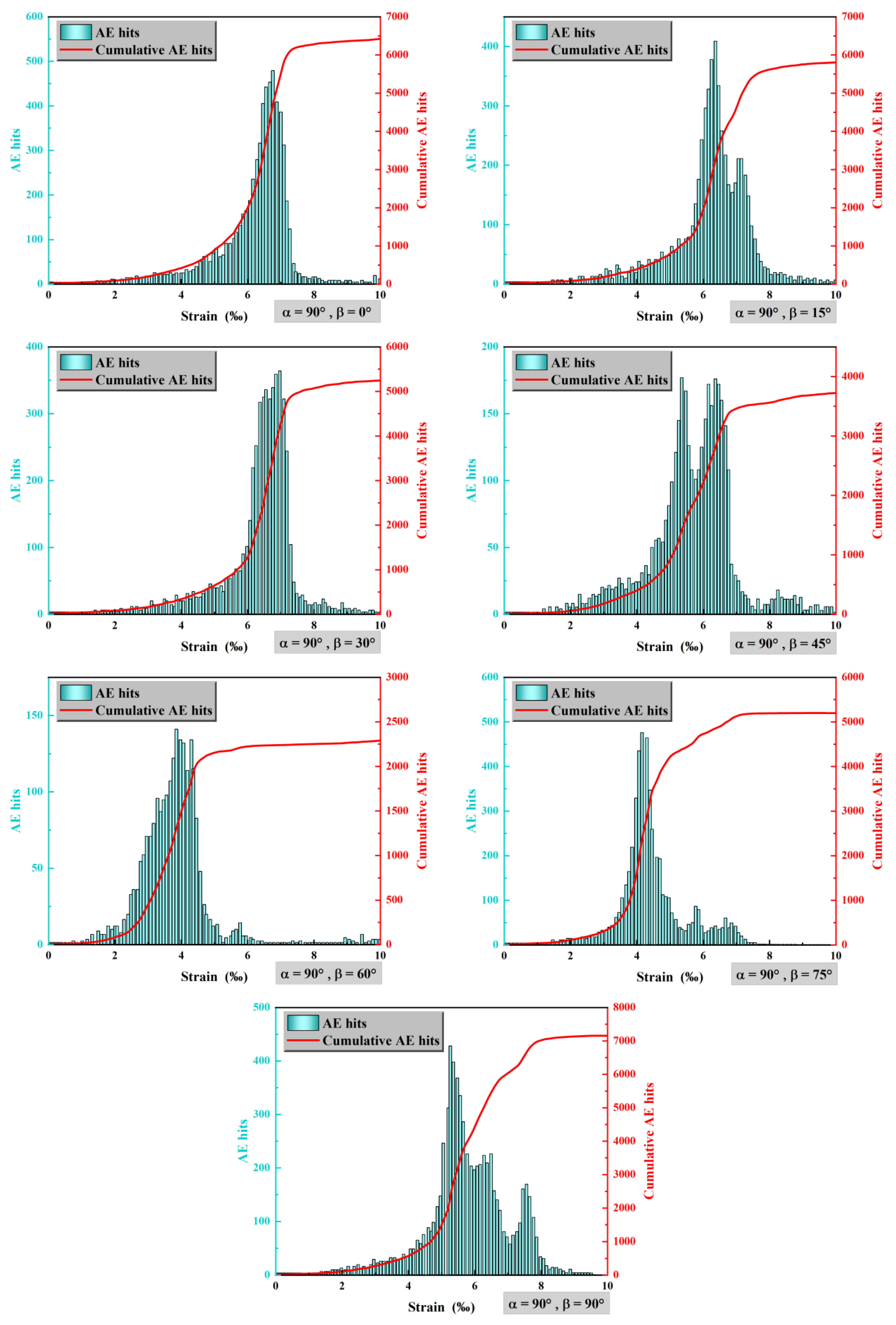
4.1. Acoustic Emission Characteristics of Salt Paste Composite
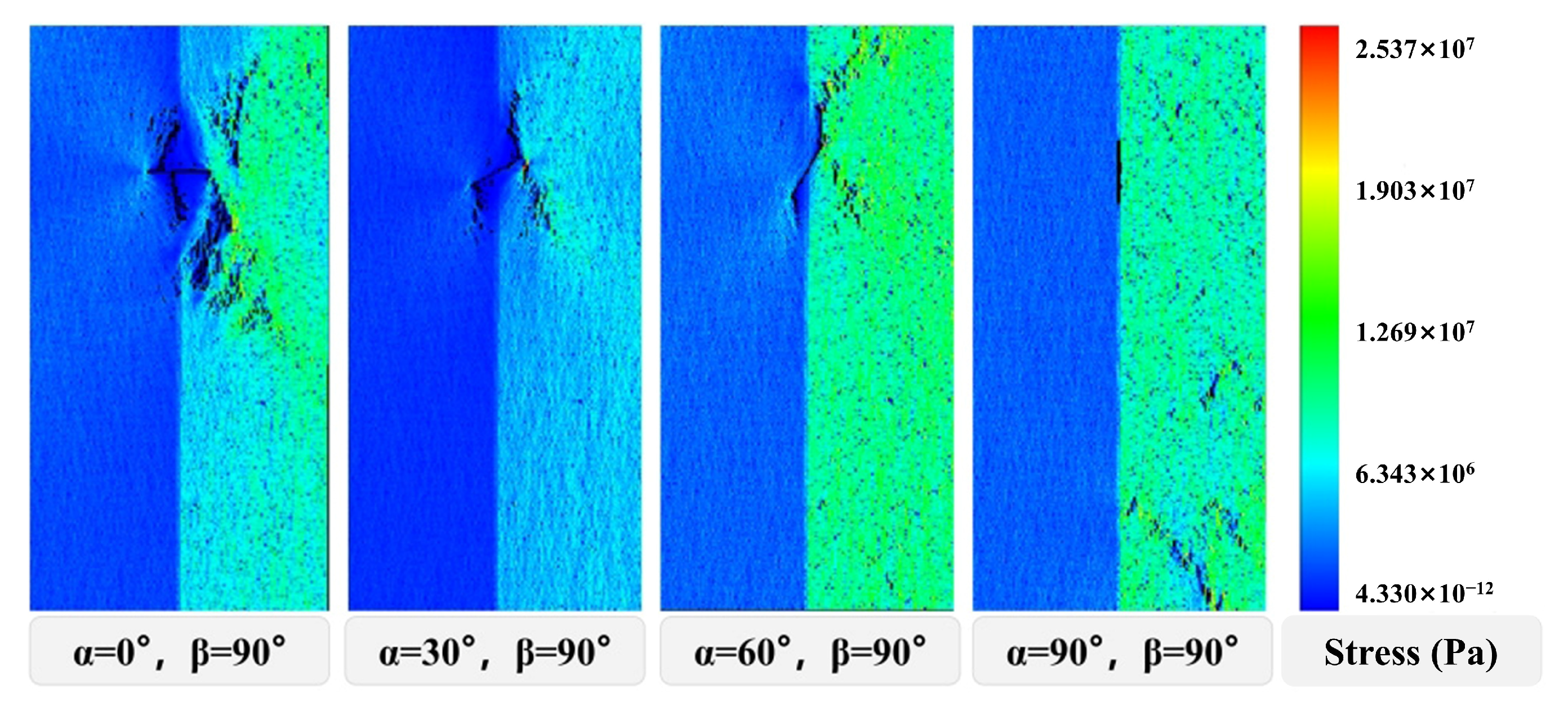
4.2. Fracture Characteristics of Salt Paste Composite
5. Discussion
5.1. Effect of Pre-Existing Fracture Angles on Compressive Strength and Failure Modes
5.2. Influence of Bedding Plane Dip Angles on Strength and Cracking Behavior
5.3. Acoustic Emission, Comparisons with Previous Studies, Engineering Implications, and Limitations
6. Conclusions
- (1)
- The orientation of the pre-existing fissure has a profound impact on the mechanical behavior of the composite. Specifically, when the fissure is oriented near 0°, the crack tends to coalesce at a lower stress level, leading to an early onset of extensive damage characterized by wide-spread microcrack connectivity. In contrast, when the fissure is oriented near 90°, the simulation results indicate a significant delay in the propagation of cracks, thereby increasing the composite’s capacity to sustain higher loads before failure occurs. The alignment of fissures relative to the loading direction plays a critical role in determining the overall structural integrity, as it influences the stress distribution and the subsequent energy release during fracture.
- (2)
- The bedding plane dip angle exerts a complex, non-monotonic effect on the compressive strength of the composite. As the bedding plane angle increases from 0° toward 60°, the peak compressive strength first rises, then reaches a pronounced minimum at around 60°. At this critical angle, the salt–gypsum interface is particularly susceptible to shear activation and delamination, which results in a rapid decrease in load-bearing capacity. Beyond 60°, as the bedding plane approaches a near-vertical orientation (90°), the strength increases again. This behavior underscores the importance of the bedding plane orientation in governing failure; the minimum strength at approximately 60° highlights a vulnerability that can be attributed to optimal conditions for interlayer sliding and stress concentration along the interface.
- (3)
- The AE activity is closely linked to the transition from gradual microcrack development to the rapid coalescence that characterizes macro-fracture. Sudden surges in AE events were observed to coincide with critical load levels, signaling a rapid release of strain energy and imminent structural failure. These AE patterns serve as a sensitive indicator of the evolving damage process and validate the notion that the interplay between fissure orientation and bedding plane dip not only governs the static strength but also the dynamic fracture propagation behavior.
- (4)
- This paper has direct implications for the design and operation of underground salt cavern gas storage facilities. In regions where geological surveys reveal a high prevalence of bedding planes with dip angles near 60°, extra caution must be exercised during site selection, or measures should be implemented to reinforce the interlayer stability. Moreover, in scenarios where low-angle or horizontal fissures are dominant, it becomes critical to adopt a phased approach to controlling injection and withdrawal pressures. Coupled with high-frequency micro-seismic monitoring, these measures can facilitate the early detection of rapid crack propagation or shear failure, thereby mitigating risks such as cavern collapse or gas leakage. The study thus provides a framework for tailoring design strategies and operational protocols based on the geometric characteristics of the rock mass.
- (5)
- This study provides valuable insights into the fracture behavior of salt–gypsum composites under static, isothermal conditions; several aspects remain for future exploration. It is essential to incorporate cyclic loading and temperature variations into the simulation framework, as these factors are inherent in real underground gas storage environments and significantly influence fatigue, creep, and thermal stress responses. Furthermore, given that salt rock exhibits pronounced time-dependent behavior, integrating viscoplastic or creep constitutive models would enhance the accuracy of long-term stability predictions. Extending the analysis to three-dimensional models would also provide a more realistic representation of complex geological conditions, including irregular interface geometries. Lastly, experimental validation using laboratory tests on specimens with controlled fissure and bedding configurations is critical for verifying and refining the numerical predictions. Coupling thermo-hydro-mechanical processes in future studies would further bridge the gap between simulation and field performance, ultimately contributing to safer and more reliable underground gas storage designs.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Farghali, M.; Osman, A.I.; Mohamed, I.M.; Chen, Z.; Chen, L.; Ihara, I.; Yap, P.-S.; Rooney, D.W. Strategies to save energy in the context of the energy crisis: A review. Environ. Chem. Lett. 2023, 21, 2003–2039. [Google Scholar] [CrossRef] [PubMed]
- Tarkowski, R. Underground hydrogen storage: Characteristics and prospects. Renew. Sustain. Energy Rev. 2019, 105, 86–94. [Google Scholar] [CrossRef]
- Lord, A.S.; Kobos, P.H.; Borns, D.J. Geologic storage of hydrogen: Scaling up to meet city transportation demands. Int. J. Hydrogen Energy 2014, 39, 15570–15582. [Google Scholar] [CrossRef]
- Matos, C.R.; Carneiro, J.F.; Silva, P.P. Overview of large-scale underground energy storage technologies for integration of renewable energies and criteria for reservoir identification. J. Energy Storage 2019, 21, 241–258. [Google Scholar] [CrossRef]
- Panfilov, M. Underground and pipeline hydrogen storage. In Compendium of Hydrogen Energy; Elsevier: Amsterdam, The Netherlands, 2016; Volume 2, pp. 91–115. [Google Scholar]
- Caglayan, D.G.; Weber, N.; Heinrichs, H.U.; Linßen, J.; Robinius, M.; Kukla, P.A.; Stolten, D. Technical potential of salt caverns for hydrogen storage in Europe. Int. J. Hydrogen Energy 2020, 45, 6793–6805. [Google Scholar] [CrossRef]
- Liu, W.; Li, Q.; Yang, C.; Shi, X.; Wan, J.; Jurado, M.J.; Li, Y.; Jiang, D.; Chen, J.; Qiao, W.; et al. The role of underground salt caverns for large-scale energy storage: A review and prospects. Energy Storage Mater. 2023, 63, 103045. [Google Scholar] [CrossRef]
- Gao, R.; Wu, F.; Chen, J.; Zhu, C.; He, Q. Accurate characterization of triaxial deformation and strength properties of salt rock based on logarithmic strain. J. Energy Storage 2022, 51, 104484. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, Q.; Liu, X.; Song, Z.; Feng, S. Creep properties and constitutive model for salt rock subjected to uniaxial trapezoidal cyclic loading. J. Energy Storage 2022, 52, 105023. [Google Scholar] [CrossRef]
- Gao, R.; Wu, F.; Chen, J.; Zhu, C.; Ji, C. Study on creep characteristics and constitutive model of typical argillaceous salt rock in energy storage caverns in China. J. Energy Storage 2022, 50, 104248. [Google Scholar] [CrossRef]
- Lin, H.; Liu, J.; Yang, J.; Ran, L.; Ding, G.; Wu, Z.; Lyu, C.; Bian, Y. Analysis of damage characteristics and energy evolution of salt rock under triaxial cyclic loading and unloading. J. Energy Storage 2022, 56, 106145. [Google Scholar] [CrossRef]
- Zhang, Q.; Song, Z.; Wang, J.; Zhang, Y.; Wang, T. Creep properties and constitutive model of salt rock. Adv. Civ. Eng. 2021, 2021, 8867673. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, Q.; Song, Z.; Zhang, Y. Creep properties and damage constitutive model of salt rock under uniaxial compression. Int. J. Damage Mech. 2020, 29, 902–922. [Google Scholar] [CrossRef]
- Liang, X.; Meng, T.; Feng, G.; Zhao, G.; Wang, Z.; Liu, P. Evolution of permeability and pore structure of salt rock and its self-healing mechanism under coupled thermo-hydro-mechanical environment. J. Energy Storage 2023, 66, 107476. [Google Scholar] [CrossRef]
- Tong, K.; He, J.; Chen, P.; Li, C.; Dai, W.; Sun, F.; Tong, Y.; Rao, S.; Wang, J. Key Technologies for the Efficient Development of Thick and Complex Carbonate Reservoirs in the Middle East. Energies 2024, 17, 4566. [Google Scholar] [CrossRef]
- Zhao, S.; Liu, H.; Zhu, Y.; Wang, S.; Yang, X. Quantitative Evaluation of Gypsum-Salt Caprock Sealing Capacity Based on Analytic Hierarchy Process—A Case Study from the Cambrian in the Tarim Basin, Western China. Energies 2022, 15, 7139. [Google Scholar] [CrossRef]
- Li, J.; Wan, J.; Liu, H.; Jurado, M.J.; He, Y.; Yuan, G.; Xia, Y. Stability analysis of a typical salt cavern gas storage in the Jintan area of China. Energies 2022, 15, 4167. [Google Scholar] [CrossRef]
- Shi, H.; Chen, W.; Zhang, H.; Song, L.; Li, M.; Wang, M.; Lu, P. Dynamic strength characteristics of fractured rock mass. Eng. Fract. Mech. 2023, 292, 109678. [Google Scholar] [CrossRef]
- Shi, H.; Zhang, H.; Song, L.; Yang, Z.; Yuan, G.; Xue, X.; Wang, Y. Failure characteristics of sandstone specimens with randomly distributed pre-cracks under uniaxial compression. Environ. Earth Sci. 2020, 79, 1–21. [Google Scholar] [CrossRef]
- Zhao, E.; Li, J.; Zhang, X.; Zhang, C.; Ren, Q.; Tan, T.; Wang, Y. Failure characteristics and the law of the energy evolution of granite with different pre-crack inclination angles under uniaxial compression loading. Front. Earth Sci. 2023, 11, 1218178. [Google Scholar] [CrossRef]
- Bi, X.; Wang, W.; Zhu, G.; Liu, X. Study of mechanical behavior of salt rock with prefabricated fissures under different confining pressures. Arab. J. Geosci. 2021, 14, 785. [Google Scholar] [CrossRef]
- Lyu, X.; Yun, L.; Xu, J.; Liu, H.; Yu, X.; Peng, P.; Ouyang, M.; Luo, Y. Sealing capacity evolution of gypsum salt caprocks under multi-cycle alternating stress during operations of underground gas storage. J. Pet. Sci. Eng. 2023, 220, 111244. [Google Scholar] [CrossRef]
- Zhao, Y.; Wang, X.; Tang, W.; Li, Y.; Lin, H.; Wang, Y.; Zhang, L. Creep behavior of layered salt rock under triaxial loading and unloading cycles. Appl. Rheol. 2023, 33, 20230103. [Google Scholar] [CrossRef]
- Wang, G.; Meng, T.; Feng, G.; Ma, L.; Yang, W.; He, Y.; Zhang, Z.; Liang, X. Influence of bedding strength and angle on fracture characteristics of sandstone under three-point bending conditions. Appl. Sci. 2023, 13, 8216. [Google Scholar] [CrossRef]
- Meng, T.; Hu, Y.; Fang, R.; Fu, Q.; Yu, W. Weakening mechanisms of gypsum interlayers from Yunying salt cavern subjected to a coupled thermo-hydro-chemical environment. J. Nat. Gas Sci. Eng. 2016, 30, 77–89. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, S.; Xie, Y.; Wang, T. Three-Dimensional Heterogeneity of the Pore and Fracture Development and Acoustic Emission Response Characteristics of Coal Rocks in the Yunnan Laochang Block. Energies 2024, 17, 1207. [Google Scholar] [CrossRef]
- Liu, X.; Liu, Z.; Li, X.; Gong, F.; Du, K. Experimental study on the effect of strain rate on rock acoustic emission characteristics. Int. J. Rock Mech. Min. Sci. 2020, 133, 104420. [Google Scholar] [CrossRef]
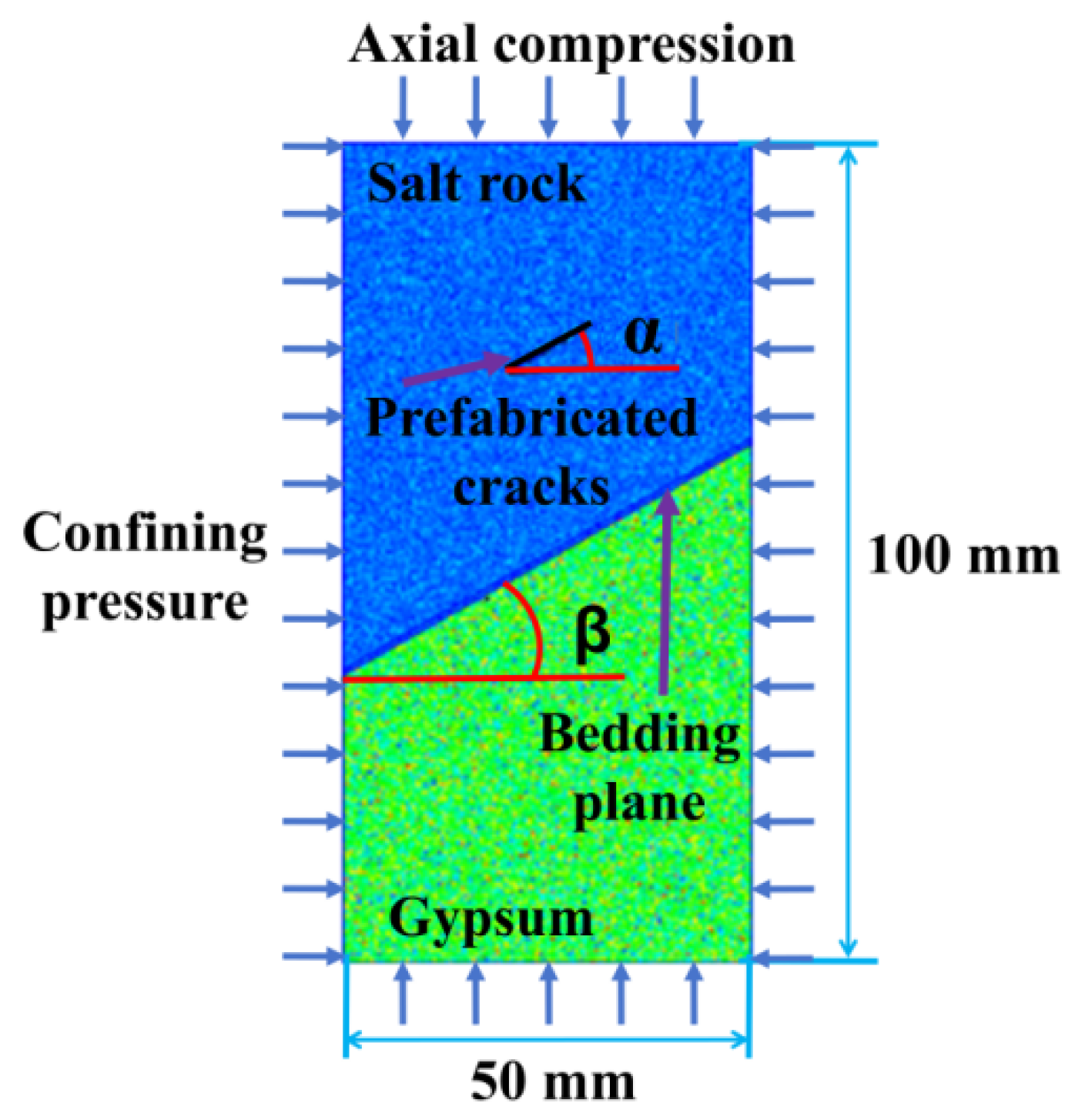
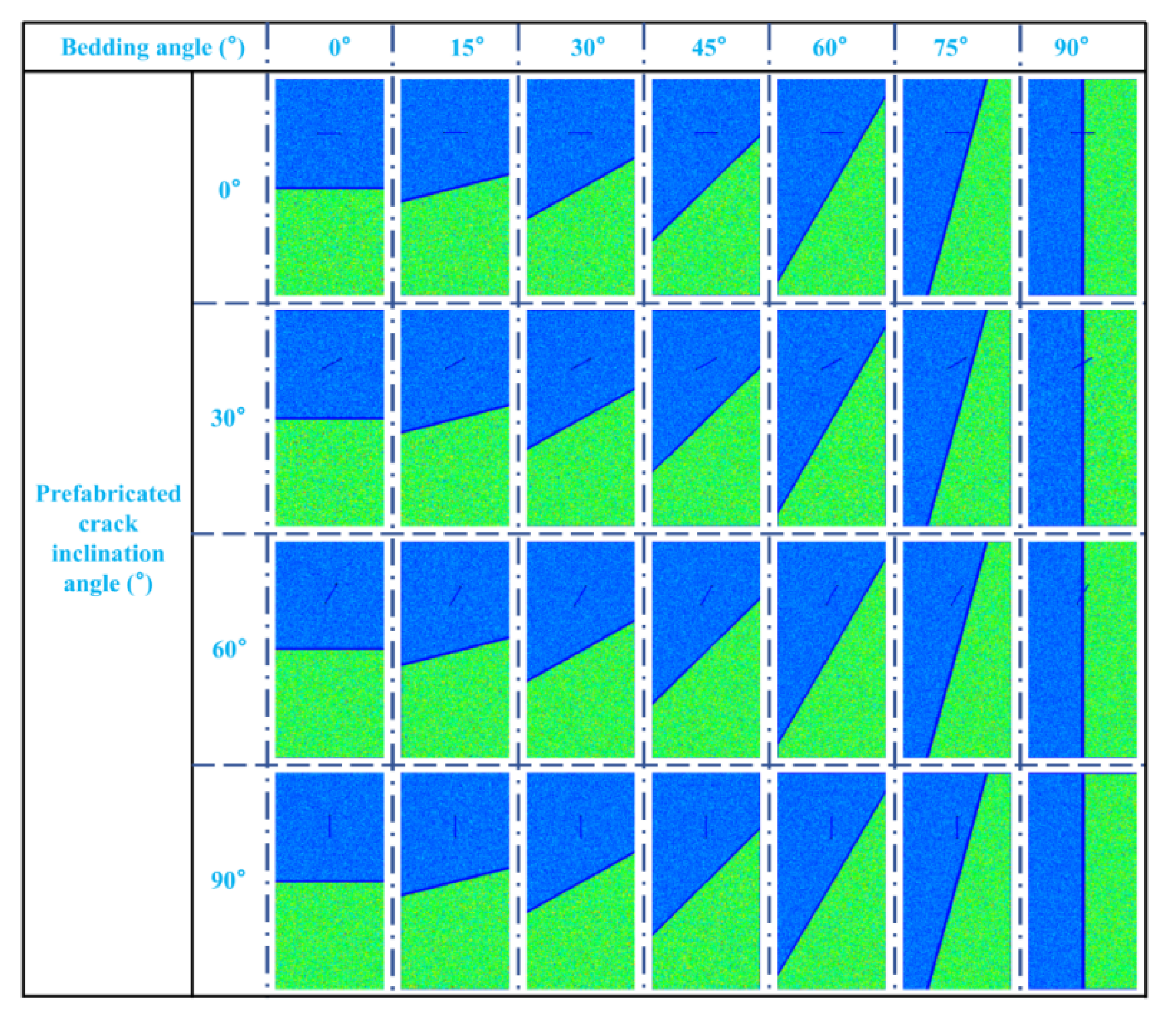


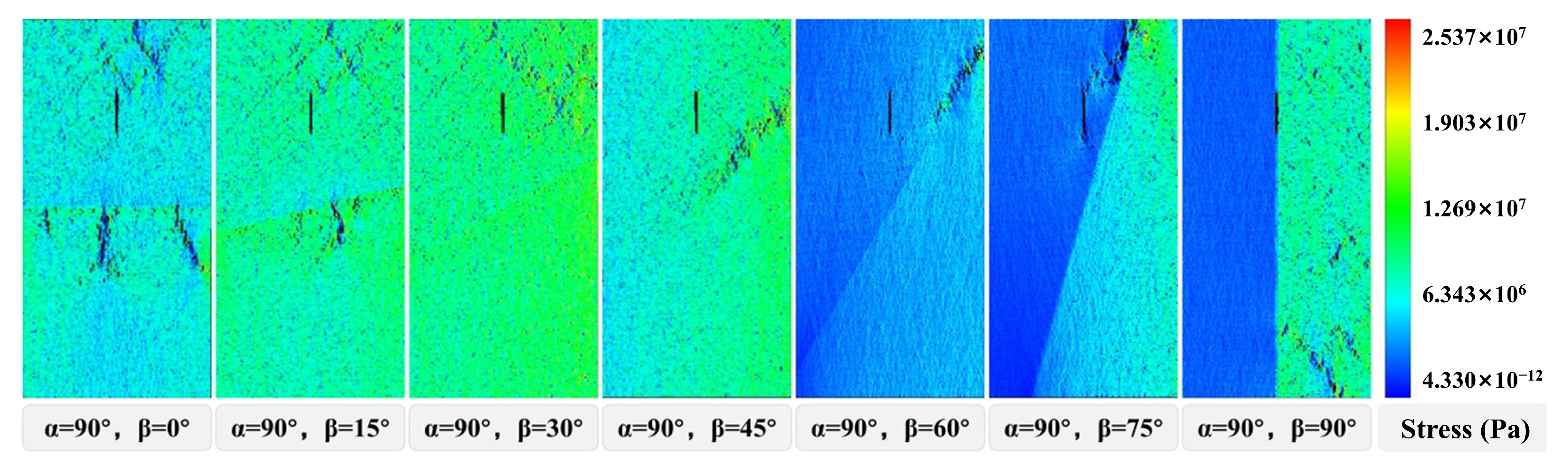



| Bedding Plane Dip Angle (°) | Left Vertex Coordinates | Right Vertex Coordinates |
|---|---|---|
| 0° | (0, 0.0497) | (0.05, 0.0503) |
| 15° | (0, 0.0430) | (0.05, 0.0558) |
| (0, 0.0436) | (0.05, 0.0564) | |
| 30° | (0, 0.0352) | (0.05, 0.0635) |
| (0, 0.0358) | (0.05, 0.0641) | |
| 45° | (0, 0.0246) | (0.05, 0.0740) |
| (0, 0.0252) | (0.05, 0.0746) | |
| 60° | (0, 0.0061) | (0.05, 0.0921) |
| (0, 0.0067) | (0.05, 0.0927) | |
| 75° | (0.011, 0) | (0.0384, 0.1) |
| (0.0116, 0) | (0.039, 0.1) | |
| 90° | (0.0247, 0) | (0.0253, 0.1) |
| Prefabricated Crack Inclination Angle (°) | Left Vertex Coordinates | Right Vertex Coordinates |
|---|---|---|
| 0° | (0.020, 0.075) | (0.030, 0.075) |
| 30° | (0.027, 0.0725) | (0.0293, 0.0775) |
| 60° | (0.0225, 0.0707) | (0.0275, 0.0793) |
| 90° | (0.025, 0.070) | (0.025, 0.080) |
| Material | Internal Friction Angle (°) | Tension and Compression Ratio | Compressive Strength (MPa) | Homogeneity Factor | Elastic Modulus (GPa) | Poisson Ratio |
|---|---|---|---|---|---|---|
| Salt rock | 36 | 10 | 22 | 3 | 7710 | 0.336 |
| Gypsum | 33 | 10 | 44 | 3 | 33,290 | 0.204 |
| Bedding plane | 30 | 10 | 30 | 3 | 15,000 | 0.3 |
| Bedding Plane Angle (β) | Fracture Angle (α) | Peak Compressive Strength (Relative) | Peak Strain | Cumulative AE Count (If Specified) | Fracture Behavior Description |
|---|---|---|---|---|---|
| 0° | 0° | 15.11166 | 4.31762 | 282 | Pre-existing fissure induces extensive crack propagation, with widespread damage in the gypsum layer. |
| 30° | 16.84864 | 4.8139 | 389 | Cracks extend mainly from the fissure ends; damage is more localized compared to 0°. | |
| 60° | 17.10918 | 4.88834 | 258 | Limited crack propagation with some shear-type features; damage is reduced compared to 0°. | |
| 90° | 21.01737 | 6.00496 | 479 | Minimal additional cracking; damage is confined near the fissure tips, delaying failure. | |
| 15° | 0° | 15.575 | 4.45 | 178 | Similar to 0° at β = 0° but slightly improved due to the bedding effect. |
| 30° | 17.325 | 4.95 | 311 | Localized damage with fissure extension; overall behavior remains similar. | |
| 60° | 17.7625 | 5.075 | 245 | Behavior similar to corresponding 0° case with minor bedding influence. | |
| 90° | 20.5625 | 5.875 | 410 | Limited cracking observed; overall stability improved. | |
| 30° | 0° | 16.68704 | 4.76773 | 369 | Cracks initiate from the fissure with moderate damage distribution. |
| 30° | 17.62836 | 5.03667 | 395 | Progressive crack extension along the fissure; damage develops gradually. | |
| 60° | 18.74083 | 5.35452 | 492 | Limited crack propagation with shear features evident. | |
| 90° | 21.39364 | 6.11247 | 363 | Minimal additional cracking; system shows stable behavior. | |
| 45° | 0° | 15.79268 | 4.5122 | 243 | Cracks propagate uniformly from the fissure and bedding interface. |
| 30° | 18.18293 | 5.19512 | 311 | Delayed failure with uniform crack extension; improved strength. | |
| 60° | 18.86585 | 5.39024 | 317 | Uniform propagation with combined fissure and bedding effects. | |
| 90° | 18.09756 | 5.17073 | 177 | Cracking is largely confined near the fissure tips, leading to optimal performance. | |
| 60° | 0° | 10.5 | 3 | 97.4 | Pronounced interlayer delamination and shear activation along the bedding plane cause rapid crack coalescence. |
| 30° | 12.03909 | 3.28947 | 133 | Mixed fracture mode with early bedding failure; overall strength is reduced. | |
| 60° | 11.76547 | 3.15789 | 129 | Rapid crack coalescence along the bedding plane; failure is predominantly controlled by interlayer weakness. | |
| 90° | 12.35831 | 3.05263 | 142 | Similar interlayer failure mode as other α values at β = 60°; overall strength remains low. | |
| 75° | 0° | 17.09797 | 3.37995 | 389 | Mixed fracture mode with localized crack concentration; strength improves relative to 60°. |
| 30° | 16.81419 | 3.24009 | 386 | Similar mixed behavior with improved load resistance. | |
| 60° | 18.16216 | 3.47319 | 385 | Uniform fracture with partial delamination; damage is more controlled. | |
| 90° | 20.00676 | 3.79953 | 478 | Limited cracking and improved stability are observed. | |
| 90° | 0° | 20.37241 | 4.08867 | 475 | Fracture initiates from the fissure with a uniform damage distribution across the composite. |
| 30° | 20.46897 | 3.71921 | 515 | Progressive crack extension with improved load resistance. | |
| 60° | 24.71724 | 4.4335 | 806 | Limited crack propagation, similar to behavior at lower bedding angles. | |
| 90° | 27.61379 | 4.95074 | 428 | Fracture is mainly confined within the gypsum layer; the fissure has minimal influence, resulting in optimal stability. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, F.; Meng, T.; Cao, K.; Zhang, P.; Zhang, Z.; Zhang, C.; Zhao, G.; Wang, Z.; Liu, P.; Wu, X. Influence of Layering and Fracture Angles on the Performance of Salt–Gypsum Composites: Implications for the Safety of Underground Energy Storage. Energies 2025, 18, 2287. https://doi.org/10.3390/en18092287
Wu F, Meng T, Cao K, Zhang P, Zhang Z, Zhang C, Zhao G, Wang Z, Liu P, Wu X. Influence of Layering and Fracture Angles on the Performance of Salt–Gypsum Composites: Implications for the Safety of Underground Energy Storage. Energies. 2025; 18(9):2287. https://doi.org/10.3390/en18092287
Chicago/Turabian StyleWu, Fengbiao, Tao Meng, Kehao Cao, Panpan Zhang, Ziying Zhang, Chuanda Zhang, Guanghui Zhao, Zhixia Wang, Pengtao Liu, and Xiaomeng Wu. 2025. "Influence of Layering and Fracture Angles on the Performance of Salt–Gypsum Composites: Implications for the Safety of Underground Energy Storage" Energies 18, no. 9: 2287. https://doi.org/10.3390/en18092287
APA StyleWu, F., Meng, T., Cao, K., Zhang, P., Zhang, Z., Zhang, C., Zhao, G., Wang, Z., Liu, P., & Wu, X. (2025). Influence of Layering and Fracture Angles on the Performance of Salt–Gypsum Composites: Implications for the Safety of Underground Energy Storage. Energies, 18(9), 2287. https://doi.org/10.3390/en18092287






